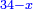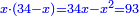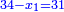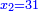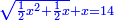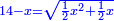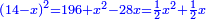Difference between revisions of "השאלות החרשות/האלמות"
(→Indeterminate Problems) |
(→Buy and Sell Problems) |
||
| (141 intermediate revisions by the same user not shown) | |||
| Line 89: | Line 89: | ||
| | | | ||
:Suppose its root is a thing plus a number. | :Suppose its root is a thing plus a number. | ||
| − | :<math>\scriptstyle n=\sqrt{x^2+5}=x+a</math> | + | :<math>\scriptstyle{\color{OliveGreen}{n=\sqrt{x^2+5}=x+a}}</math> |
|style="text-align:right;"|ותשים שרשו דבר ומספר | |style="text-align:right;"|ותשים שרשו דבר ומספר | ||
|- | |- | ||
| | | | ||
:When this number is multiplied by itself, its square is less than the additional five. | :When this number is multiplied by itself, its square is less than the additional five. | ||
| − | :<math>\scriptstyle a^2<5</math> | + | :<math>\scriptstyle{\color{OliveGreen}{a^2<5}}</math> |
|style="text-align:right;"|יהיה מאותו המספר אלגו כשיוכה בעצמו יהיה פחות מהחמשה הנוספים | |style="text-align:right;"|יהיה מאותו המספר אלגו כשיוכה בעצמו יהיה פחות מהחמשה הנוספים | ||
|- | |- | ||
| Line 187: | Line 187: | ||
| | | | ||
:The root of the square minus ten dirham is less than a thing. | :The root of the square minus ten dirham is less than a thing. | ||
| − | :<math>\scriptstyle n=\sqrt{x^2-10}>x</math> | + | :<math>\scriptstyle{\color{OliveGreen}{n=\sqrt{x^2-10}>x}}</math> |
|style="text-align:right;"|ושרש מאלגו פחות עשרה דרהמי הוא פחות מדבר | |style="text-align:right;"|ושרש מאלגו פחות עשרה דרהמי הוא פחות מדבר | ||
|- | |- | ||
| Line 220: | Line 220: | ||
:<math>\scriptstyle{\color{blue}{x^2+11-2x=x^2}}</math> | :<math>\scriptstyle{\color{blue}{x^2+11-2x=x^2}}</math> | ||
|style="text-align:right;"|ויהיה אלגו וי"א דרהמי פחות שני דברים ישוה אלגו | |style="text-align:right;"|ויהיה אלגו וי"א דרהמי פחות שני דברים ישוה אלגו | ||
| + | |- | ||
| + | | | ||
| + | :*<span style="color:Green>Restoration:</span> | ||
| + | | | ||
|- | |- | ||
| | | | ||
| Line 280: | Line 284: | ||
|- | |- | ||
| | | | ||
| − | :If you suppose the number that is subtracted from the thing is such that when multiplied by itself, its product is greater than the dirham that are subtracted from one square [<math>\scriptstyle a^2>b</math>]. | + | :If you suppose the number that is subtracted from the thing is such that when multiplied by itself, its product is greater than the dirham that are subtracted from one square [<math>\scriptstyle{\color{OliveGreen}{a^2>b}}</math>]. |
|style="text-align:right;"|ואם תניח המספר הנמשך לדבר כאשר תכה אותו בעצמו יתקבץ יותר מהדרהמי הנמשכים אל האלגו אחד | |style="text-align:right;"|ואם תניח המספר הנמשך לדבר כאשר תכה אותו בעצמו יתקבץ יותר מהדרהמי הנמשכים אל האלגו אחד | ||
|- | |- | ||
| | | | ||
:Know that the root of the square minus this number is then the same as the number minus the thing. | :Know that the root of the square minus this number is then the same as the number minus the thing. | ||
| − | :<math>\scriptstyle\sqrt{x^2-b}=a-x</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{x^2-b}=a-x}}</math> |
|style="text-align:right;"|תדע כי שרש האלגו ‫<ref>64v</ref>פחות אותו המספר יהיה אז אותו המספר מהדבר פחות אותו הדבר | |style="text-align:right;"|תדע כי שרש האלגו ‫<ref>64v</ref>פחות אותו המספר יהיה אז אותו המספר מהדבר פחות אותו הדבר | ||
|- | |- | ||
| | | | ||
:Since the root of the square minus ten is the same as the number minus the thing. | :Since the root of the square minus ten is the same as the number minus the thing. | ||
| − | :<math>\scriptstyle\sqrt{x^2-10}=a-x</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{x^2-10}=a-x}}</math> |
|style="text-align:right;"|בעבור כי שרש האלגו פחות עשרה יהיה אותו המספר הנמשך לדבר פחות דבר | |style="text-align:right;"|בעבור כי שרש האלגו פחות עשרה יהיה אותו המספר הנמשך לדבר פחות דבר | ||
|- | |- | ||
| | | | ||
:Also the product of the number minus the thing by itself is the same as the product of the thing minus the number by itself. | :Also the product of the number minus the thing by itself is the same as the product of the thing minus the number by itself. | ||
| − | :<math>\scriptstyle\left(a-x\right)^2=\left(x-a\right)^2</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\left(a-x\right)^2=\left(x-a\right)^2}}</math> |
|style="text-align:right;"|וכן הכאת <sup>ה</sup>מספר מהדבר פחות מהמספר בעצמו כמו הכאת הדבר פחות אותו המספר בעצמו | |style="text-align:right;"|וכן הכאת <sup>ה</sup>מספר מהדבר פחות מהמספר בעצמו כמו הכאת הדבר פחות אותו המספר בעצמו | ||
|- | |- | ||
| Line 304: | Line 308: | ||
|- | |- | ||
| | | | ||
| − | + | :This is an indeterminate problem. | |
|style="text-align:right;"|זאת השאלה אלמת | |style="text-align:right;"|זאת השאלה אלמת | ||
|- | |- | ||
| Line 312: | Line 316: | ||
|- | |- | ||
| | | | ||
| − | : | + | :Or, a thing plus half the dirham [<math>\scriptstyle{\color{blue}{\sqrt{x^2+3x}=x+\frac{1}{2}}}</math>]. |
|style="text-align:right;"|או דבר וחצי דרהם | |style="text-align:right;"|או דבר וחצי דרהם | ||
|- | |- | ||
| | | | ||
| − | : | + | :Or, a thing plus one dirham [<math>\scriptstyle{\color{blue}{\sqrt{x^2+3x}=x+1}}</math>] [?] |
|style="text-align:right;"|או דבר ואדרהם אחד על האלגו והוא בזאת השאלה שלשה דברים | |style="text-align:right;"|או דבר ואדרהם אחד על האלגו והוא בזאת השאלה שלשה דברים | ||
|- | |- | ||
| | | | ||
| − | :We suppose it is a thing plus one dirham [<math>\scriptstyle{\color{blue}{x+1=\sqrt{x^2+3x}}}</math>] | + | :We suppose it is a thing plus one dirham [<math>\scriptstyle{\color{blue}{x+1=\sqrt{x^2+3x}}}</math>]. |
|style="text-align:right;"|ונניחהו דבר ודרהם אחד | |style="text-align:right;"|ונניחהו דבר ודרהם אחד | ||
|- | |- | ||
| Line 330: | Line 334: | ||
| | | | ||
:Subtract a square and two things from a square and three things; one thing remains equal to one dirham and this is the root of the square. | :Subtract a square and two things from a square and three things; one thing remains equal to one dirham and this is the root of the square. | ||
| + | |style="text-align:right;"|ותגרע אלגו ושני דברים מאלגו ושלשה דברים ישאר דבר אחד ישוה דרהם אחד והוא שרש האלגו | ||
| + | |- | ||
| + | | colspan="2"| | ||
:<math>\scriptstyle{\color{blue}{x^2+1+2x-\left(x^2+2x\right)=x^2+3x-\left(x^2+2x\right)\longrightarrow x=1}}</math> | :<math>\scriptstyle{\color{blue}{x^2+1+2x-\left(x^2+2x\right)=x^2+3x-\left(x^2+2x\right)\longrightarrow x=1}}</math> | ||
| − | |||
|- | |- | ||
| | | | ||
| Line 354: | Line 360: | ||
|- | |- | ||
| | | | ||
| − | + | :This is an indeterminate problem. | |
|style="text-align:right;"|זאת השאלה אלמת | |style="text-align:right;"|זאת השאלה אלמת | ||
|- | |- | ||
| | | | ||
| − | : | + | :Knowing this is that we assume the square is a square [<math>\scriptstyle{\color{blue}{x^2}}</math>]. |
|style="text-align:right;"|וידיעתה הוא שנניח האלגו אלגו | |style="text-align:right;"|וידיעתה הוא שנניח האלגו אלגו | ||
|- | |- | ||
| Line 368: | Line 374: | ||
| | | | ||
:Its root is less than a thing. | :Its root is less than a thing. | ||
| − | :<math>\scriptstyle n=\sqrt{x^2-6x}>x</math> | + | :<math>\scriptstyle{\color{OliveGreen}{n=\sqrt{x^2-6x}>x}}</math> |
|style="text-align:right;"|ושרשו‫<ref>marg.: ר"ל שרש הנשאר</ref> פחות מהדבר | |style="text-align:right;"|ושרשו‫<ref>marg.: ר"ל שרש הנשאר</ref> פחות מהדבר | ||
|- | |- | ||
| Line 376: | Line 382: | ||
|- | |- | ||
| | | | ||
| − | :Or minus five [dirham] [<math>\scriptstyle{\color{blue}{n=\sqrt{x^2+5}=x-5}}</math>]. | + | :Or, minus five [dirham] [<math>\scriptstyle{\color{blue}{n=\sqrt{x^2+5}=x-5}}</math>]. |
|style="text-align:right;"|או פחות חמשה | |style="text-align:right;"|או פחות חמשה | ||
|- | |- | ||
| | | | ||
| − | :Or minus three and a half [dirham] [<math>\scriptstyle{\color{blue}{n=\sqrt{x^2+5}=x-\left(3+\frac{1}{2}\right)}}</math>]. | + | :Or, minus three and a half [dirham] [<math>\scriptstyle{\color{blue}{n=\sqrt{x^2+5}=x-\left(3+\frac{1}{2}\right)}}</math>]. |
|style="text-align:right;"|או פחות שלשה וחצי | |style="text-align:right;"|או פחות שלשה וחצי | ||
|- | |- | ||
| | | | ||
| − | : | + | :Or, minus any number you want provided that it is greater than half the six roots that are subtracted from the square. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{a>\frac{1}{2}\sdot6\longrightarrow n=\sqrt{x^2+5}=x-a}}</math> | |
|style="text-align:right;"|או פחות מהמספר שתרצה אחר <s>זה</s> שיהיה יותר מהחצי מהששה דברים הנמשכים אל האלגו | |style="text-align:right;"|או פחות מהמספר שתרצה אחר <s>זה</s> שיהיה יותר מהחצי מהששה דברים הנמשכים אל האלגו | ||
|- | |- | ||
| Line 402: | Line 408: | ||
|- | |- | ||
| | | | ||
| + | :Confront them; the thing is eight. | ||
:<math>\scriptstyle{\color{blue}{x=8}}</math> | :<math>\scriptstyle{\color{blue}{x=8}}</math> | ||
|style="text-align:right;"|ותנכחהו עמו ויהיה הדבר שמנה | |style="text-align:right;"|ותנכחהו עמו ויהיה הדבר שמנה | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x^2=64}}</math> | + | :The square is sixty-four. |
| + | :<math>\scriptstyle{\color{blue}{x^2=64}}</math> | ||
|style="text-align:right;"|והאלגו <s>עשרה וששה ו</s>הוא ששים וארבעה | |style="text-align:right;"|והאלגו <s>עשרה וששה ו</s>הוא ששים וארבעה | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{n^2=x^2-6x=64-48=16}}</math> | + | :Subtract from it six times its root, which is 48; 16 remains. |
| + | :<math>\scriptstyle{\color{blue}{n^2=x^2-6x=64-48=16}}</math> | ||
|style="text-align:right;"|תגרע מהם שרשו ששה פעמים והוא מ"ח ישארו י"ו | |style="text-align:right;"|תגרע מהם שרשו ששה פעמים והוא מ"ח ישארו י"ו | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{n=\sqrt{x^2-6x}=4}}</math> | + | :Its root is four. |
| + | :<math>\scriptstyle{\color{blue}{n=\sqrt{x^2-6x}=4}}</math> | ||
|style="text-align:right;"|ושרשו ארבעה | |style="text-align:right;"|ושרשו ארבעה | ||
|- | |- | ||
| Line 423: | Line 433: | ||
|- | |- | ||
| | | | ||
| − | + | :This is an indeterminate problem. | |
|style="text-align:right;"|זאת השאלה אלמת | |style="text-align:right;"|זאת השאלה אלמת | ||
|- | |- | ||
| | | | ||
| − | : | + | :Knowing this is that you assume your square is a square [<math>\scriptstyle{\color{blue}{x^2}}</math>]. |
|style="text-align:right;"|וידיעתה שתניח האלגו שלך אלגו | |style="text-align:right;"|וידיעתה שתניח האלגו שלך אלגו | ||
|- | |- | ||
| Line 436: | Line 446: | ||
|- | |- | ||
| | | | ||
| − | :The root of it is a thing plus a number [<math>\scriptstyle n=\sqrt{x^2+10x+20}=x+a</math>]. | + | :The root of it is a thing plus a number [<math>\scriptstyle{\color{OliveGreen}{n=\sqrt{x^2+10x+20}=x+a}}</math>]. |
|style="text-align:right;"|יהיה שרש זה דבר ומספר | |style="text-align:right;"|יהיה שרש זה דבר ומספר | ||
|- | |- | ||
| | | | ||
| − | + | :Such that when it is multiplied by itself, the sum of the products by the things and the dirham is more than the twenty dirham, but no less and no more than the ten [things] and the twenty [dirham]. | |
| − | |||
| − | |||
|style="text-align:right;"|שכאשר הוכה בעצמו יהיה המתקבץ <s>ממנו הכאת נחבר <sup>מהכאת הדברים</sup></s> [מן ההכאה בדברי']‫<ref>marg.</ref> פחות מהעשרה ‫<ref>65r</ref>ובדרהמי יהיה יותר מהעשרים דרהמי | |style="text-align:right;"|שכאשר הוכה בעצמו יהיה המתקבץ <s>ממנו הכאת נחבר <sup>מהכאת הדברים</sup></s> [מן ההכאה בדברי']‫<ref>marg.</ref> פחות מהעשרה ‫<ref>65r</ref>ובדרהמי יהיה יותר מהעשרים דרהמי | ||
ולא יעבור שיהיה פחות משניהם רצוני מהעשרה ומהעשרים ולא יותר <s>ש</s>משניהם | ולא יעבור שיהיה פחות משניהם רצוני מהעשרה ומהעשרים ולא יותר <s>ש</s>משניהם | ||
|- | |- | ||
| − | | | + | | colspan="2"| |
| − | : | + | :<math>\scriptstyle{\color{OliveGreen}{\left(x+a\right)^2\longrightarrow\quad ax+a^2>20\quad ax+a^2\nless10x+20\quad ax+a^2\ngtr10x+20}}</math> |
| − | |||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{x^2+10x+20=\left(x+a\right)^2}}</math> | + | :Because then, when you confront it with the product of the square and ten things plus twenty dirham, a thing and one dirham remain equal to the thing. |
| − | |style="text-align:right;"|בעבור כי כאשר תכונוהו עם מה שיתקבץ מההכאה האלגו והעשרה דברים והעשרים דרהמי | + | :<math>\scriptstyle{\color{blue}{x^2+10x+20=\left(x+a\right)^2\longrightarrow x=x+1}}</math> |
| − | + | |style="text-align:right;"|בעבור כי כאשר תכונוהו עם מה שיתקבץ מההכאה האלגו והעשרה דברים והעשרים דרהמי ישאר דבר ודרהם אחד ישוה הדבר | |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| Line 472: | Line 476: | ||
|- | |- | ||
| | | | ||
| + | :Confront them; the thing is a quarter of a dirham and it is the root of the square. | ||
:<math>\scriptstyle{\color{blue}{x=\frac{1}{4}}}</math> | :<math>\scriptstyle{\color{blue}{x=\frac{1}{4}}}</math> | ||
|style="text-align:right;"|ותכונם עמו ויהיה הדבר הוא רביע דרהם והוא שרש האלגו | |style="text-align:right;"|ותכונם עמו ויהיה הדבר הוא רביע דרהם והוא שרש האלגו | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x^2=\frac{1}{2}\sdot\frac{1}{8}}}</math> | + | :The square is half an eighth. |
| + | :<math>\scriptstyle{\color{blue}{x^2=\frac{1}{2}\sdot\frac{1}{8}}}</math> | ||
|style="text-align:right;"|והאלגו חצי שמינית | |style="text-align:right;"|והאלגו חצי שמינית | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{n^2=x^2+10x+20=22+\frac{1}{2}+\left(\frac{1}{2}\sdot\frac{1}{8}\right)}}</math> | + | :If you add to it ten times its root and twenty dirham, it is twenty-two, a half and half an eighth. |
| + | :<math>\scriptstyle{\color{blue}{n^2=x^2+10x+20=22+\frac{1}{2}+\left(\frac{1}{2}\sdot\frac{1}{8}\right)}}</math> | ||
|style="text-align:right;"|ואם תוסיף עליו שרשו עשרה פעמים ועשרים דרהמי יהיה עשרים ושנים וחצי וחצי שמינית | |style="text-align:right;"|ואם תוסיף עליו שרשו עשרה פעמים ועשרים דרהמי יהיה עשרים ושנים וחצי וחצי שמינית | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{n=\sqrt{x^2+10x+20}=4+\frac{1}{2}+\frac{1}{4}}}</math> | + | :Its root is four dirham, a half and a quarter. |
| + | :<math>\scriptstyle{\color{blue}{n=\sqrt{x^2+10x+20}=4+\frac{1}{2}+\frac{1}{4}}}</math> | ||
|style="text-align:right;"|ושרש זה ארבעה דרהמי וחצי ורביע | |style="text-align:right;"|ושרש זה ארבעה דרהמי וחצי ורביע | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:x²-8x-30=n²|1124|67aP}}6) <math>\scriptstyle x^2-8x-30=n^2</math> | + | :{{#annot:x²-8x-30=n²|1124|67aP}}6) If you are told: a square that has a root, if you subtract from it eight times its root and thirty dirham, the remainder has a root. |
| + | :<math>\scriptstyle x^2-8x-30=n^2</math> | ||
|style="text-align:right;"|ו <big>ואם</big> יאמרו לך אלגו המחזיק שרש אם תגרע ממנו שרשו שמנה פעמי' ושלשים דרהמי יחזיק מה שישאר שרש{{#annotend:67aP}} | |style="text-align:right;"|ו <big>ואם</big> יאמרו לך אלגו המחזיק שרש אם תגרע ממנו שרשו שמנה פעמי' ושלשים דרהמי יחזיק מה שישאר שרש{{#annotend:67aP}} | ||
|- | |- | ||
| | | | ||
| − | + | :This is an indeterminate problem. | |
|style="text-align:right;"|זאת השאלה אלמת | |style="text-align:right;"|זאת השאלה אלמת | ||
|- | |- | ||
| | | | ||
| − | : | + | :Knowing this is that you assume your square is a square [<math>\scriptstyle{\color{blue}{x^2}}</math>]. |
|style="text-align:right;"|וידיעתה שתניח האלגו שלך אלגו | |style="text-align:right;"|וידיעתה שתניח האלגו שלך אלגו | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{n^2=x^2-8x-30}}</math> | + | :Subtract from it eight times its root and thirty dirham; the remainder is a square minus eight things and minus thirty dirham. |
| + | :<math>\scriptstyle{\color{blue}{n^2=x^2-8x-30}}</math> | ||
|style="text-align:right;"|תגרע ממנו שרשו שמנה פעמים ושלשים דרהמי וישאר אלגו פחות שמנה דברים ופחות שלשים דרהמי | |style="text-align:right;"|תגרע ממנו שרשו שמנה פעמים ושלשים דרהמי וישאר אלגו פחות שמנה דברים ופחות שלשים דרהמי | ||
|- | |- | ||
| | | | ||
| − | + | :It root is less than a thing [<math>\scriptstyle{\color{blue}{n=\sqrt{x^2-8x-30}<x}}</math>]. | |
|style="text-align:right;"|ושרשו פחות מדבר | |style="text-align:right;"|ושרשו פחות מדבר | ||
|- | |- | ||
| | | | ||
| − | : | + | :We suppose it is a thing minus five dirham [<math>\scriptstyle{\color{blue}{\sqrt{x^2-8x-30}=x-5}}</math>]. |
| − | |||
|style="text-align:right;"|וננחיהו דבר פחות חמשה דרהמי | |style="text-align:right;"|וננחיהו דבר פחות חמשה דרהמי | ||
|- | |- | ||
| | | | ||
| − | : | + | :Or, minus six [<math>\scriptstyle{\color{blue}{\sqrt{x^2-8x-30}=x-6}}</math>]. |
|style="text-align:right;"|או פחות ששה | |style="text-align:right;"|או פחות ששה | ||
|- | |- | ||
| | | | ||
| − | : | + | :Or, minus any number you want provided that it is greater than half the eight things that are subtracted from the square. |
| + | :<math>\scriptstyle{\color{OliveGreen}{a>\frac{1}{2}\sdot8\longrightarrow\sqrt{x^2-8x-30}=x-a}}</math> | ||
|style="text-align:right;"|או פחות מהמספר שתרצה שיהיה יותר מחצי השמנה דברים הנשנים באלגו | |style="text-align:right;"|או פחות מהמספר שתרצה שיהיה יותר מחצי השמנה דברים הנשנים באלגו | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you wish, suppose its root is a number minus a thing provided that the number is greater than half the eight things that are subtracted from the square. |
| + | :<math>\scriptstyle{\color{OliveGreen}{a>\frac{1}{2}\sdot8\longrightarrow\sqrt{x^2-8x-30}=a-x}}</math> | ||
|style="text-align:right;"|ואם תרצה תניח שרשו מספר פחות דבר ויהיה המספר יותר מחצי הדברים הנשנים באלגו | |style="text-align:right;"|ואם תרצה תניח שרשו מספר פחות דבר ויהיה המספר יותר מחצי הדברים הנשנים באלגו | ||
|- | |- | ||
| Line 531: | Line 542: | ||
:Multiply it by itself; it is a square and 25 dirham minus ten things equal a square minus eight things and thirty dirham. | :Multiply it by itself; it is a square and 25 dirham minus ten things equal a square minus eight things and thirty dirham. | ||
:<math>\scriptstyle{\color{blue}{\left(x-5\right)^2=x^2+25-10x=x^2-8x-30}}</math> | :<math>\scriptstyle{\color{blue}{\left(x-5\right)^2=x^2+25-10x=x^2-8x-30}}</math> | ||
| − | |style="text-align:right;"|ותכה אותו בעצמו ויהיה אלגו וכ"ה דרהמיש פחות עשרה דברים ישוה אלגו פחות שמנה דברים | + | |style="text-align:right;"|ותכה אותו בעצמו ויהיה אלגו וכ"ה דרהמיש פחות עשרה דברים ישוה אלגו פחות שמנה דברים ושלשים דרהמי |
| + | |- | ||
| + | | | ||
| + | :*<span style="color:Green>Confrontation:</span> | ||
| + | | | ||
|- | |- | ||
| | | | ||
| − | : | + | :Confront them; the thing is equal to 2[7] and a half; and this is the root of the square. |
| + | :<math>\scriptstyle{\color{blue}{x=2{\color{red}{7}}+\frac{1}{2}}}</math> | ||
|style="text-align:right;"|ותכונהו עמו ויהיה הדבר ישוה כ"ו וחצי והוא שרש האלגו | |style="text-align:right;"|ותכונהו עמו ויהיה הדבר ישוה כ"ו וחצי והוא שרש האלגו | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x^2=756+\frac{1}{4}}}</math> | + | :The square is 756 dirham and a quarter. |
| + | :<math>\scriptstyle{\color{blue}{x^2=756+\frac{1}{4}}}</math> | ||
|style="text-align:right;"|והאלגו תשנ"ו דרהמי ורביע | |style="text-align:right;"|והאלגו תשנ"ו דרהמי ורביע | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{n^2=x^2-8x-30=506+\frac{1}{4}}}</math> | + | :Subtract from it eight times its root and thirty dirham; 506 and a quarter remain. |
| + | :<math>\scriptstyle{\color{blue}{n^2=x^2-8x-30=506+\frac{1}{4}}}</math> | ||
|style="text-align:right;"|ותסיר ממנו שרשו שמנה פעמי' ושלשים דרהמי וישאר תק"ו ורביע | |style="text-align:right;"|ותסיר ממנו שרשו שמנה פעמי' ושלשים דרהמי וישאר תק"ו ורביע | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{n=\sqrt{x^2-8x-30}=2{\color{red}{2}}+\frac{1}{4}}}</math> | + | :Its root is 2[2] and a half. |
| + | :<math>\scriptstyle{\color{blue}{n=\sqrt{x^2-8x-30}=2{\color{red}{2}}+\frac{1}{4}}}</math> | ||
|style="text-align:right;"|ושרשו כ"ו וחצי | |style="text-align:right;"|ושרשו כ"ו וחצי | ||
|- | |- | ||
| | | | ||
| − | :7) <math>\scriptstyle\begin{cases}\scriptstyle x^2+x=n^2\\\scriptstyle x^2+2x=m^2\end{cases}</math> | + | :{{#annot:x²+x=n²,x²+2x=m²|1124|9AQM}}7) If you are told: a square that has a root, if you add its root to it, it has a root; and if you add its two roots to it, it has a root. |
| − | |style="text-align:right;"|ז <big>ואם</big> יאמרו לך אלגו שיחזיק שרש אם תוסיף עליו שרשו יחזיק שרש ואם תוסיף עליו אלגו שני שרשיו יחזיק שרש | + | :<math>\scriptstyle\begin{cases}\scriptstyle x^2+x=n^2\\\scriptstyle x^2+2x=m^2\end{cases}</math> |
| + | |style="text-align:right;"|ז <big>ואם</big> יאמרו לך אלגו שיחזיק שרש אם תוסיף עליו שרשו יחזיק שרש ואם תוסיף עליו אלגו שני שרשיו יחזיק שרש{{#annotend:9AQM}} | ||
|- | |- | ||
| | | | ||
| − | + | :This is an indeterminate problem. | |
|style="text-align:right;"|זאת השאלה אלמת | |style="text-align:right;"|זאת השאלה אלמת | ||
|- | |- | ||
| | | | ||
| − | : | + | :Solve it according to the following procedure: |
|style="text-align:right;"|ותעשה אותה כמעשה | |style="text-align:right;"|ותעשה אותה כמעשה | ||
|- | |- | ||
| − | | | + | |Look for a number that has a root [<math>\scriptstyle{\color{blue}{a^2}}</math>], such that when you add to it another number, it has a root [<math>\scriptstyle{\color{OliveGreen}{a^2+b=n^2}}</math>]; and when you add to it its two roots, it has a root [<math>\scriptstyle{\color{OliveGreen}{a^2+2b=m^2}}</math>]. |
| − | |||
| − | |||
|style="text-align:right;"|שתחפש מספר שיחזיק שרש ואם תוסיף עליו מספר אחר יחזיק שרש ואם תוסיף עליו שני שרשיו יחזיק שרש | |style="text-align:right;"|שתחפש מספר שיחזיק שרש ואם תוסיף עליו מספר אחר יחזיק שרש ואם תוסיף עליו שני שרשיו יחזיק שרש | ||
|- | |- | ||
| − | | | + | |When you find this number, divide it by the added number and the result is the root of the square. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{x=\frac{a^2}{b}}}</math> | |
|style="text-align:right;"|וכאשר מצאת אותו המספר תחלקהו על המספר הנוסף והעולה יהיה שרש האלגו | |style="text-align:right;"|וכאשר מצאת אותו המספר תחלקהו על המספר הנוסף והעולה יהיה שרש האלגו | ||
|- | |- | ||
| | | | ||
| − | :: | + | :The number that has a root is one, and the added numbers are 24 and 48. |
| − | |style="text-align:right;"|והמספר המחזיק שרש אחד< | + | :<math>\scriptstyle{\color{blue}{a^2=1\quad b=24\quad 2b=48}}</math> |
| − | והמספר הנוסף כ"ד ומ"ח | + | |style="text-align:right;"|והמספר המחזיק שרש אחד ‫<ref>65v</ref>והמספר הנוסף כ"ד ומ"ח |
|- | |- | ||
| | | | ||
| − | : | + | :You already know that when you add 48 to one, it is 49 and its root is seven. |
| + | :<math>\scriptstyle{\color{blue}{1+48=49=7^2}}</math> | ||
|style="text-align:right;"|וכבר ידעת כי בהוסיפך על אחד מ"ח יהיה מ"ט ושרשו שבעה | |style="text-align:right;"|וכבר ידעת כי בהוסיפך על אחד מ"ח יהיה מ"ט ושרשו שבעה | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you add 24 to one, it is 25 and its root is five. |
| + | :<math>\scriptstyle{\color{blue}{1+24=25=5^2}}</math> | ||
|style="text-align:right;"|ואם תוסיף כ"ד על האחד יהיה כ"ה ושרשו חמשה | |style="text-align:right;"|ואם תוסיף כ"ד על האחד יהיה כ"ה ושרשו חמשה | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you wish to know how much is the square, divide one by 24; the result is a third of an eighth and this is the root of the square. |
| + | :<math>\scriptstyle{\color{blue}{x=\frac{a^2}{b}=\frac{1}{24}=\frac{1}{3}\sdot\frac{1}{8}}}</math> | ||
|style="text-align:right;"|ואם בקשת לדעת כמה היה האלגו תחלק האחד על כ"ד ויעלה שלישית משמינית והוא שרש האלגו | |style="text-align:right;"|ואם בקשת לדעת כמה היה האלגו תחלק האחד על כ"ד ויעלה שלישית משמינית והוא שרש האלגו | ||
|- | |- | ||
| | | | ||
| − | : | + | :The square is one part of 576. |
| + | :<math>\scriptstyle{\color{blue}{x^2=\frac{1}{576}}}</math> | ||
|style="text-align:right;"|והאלגו חלק אחד מתקע"ו חלקים באחד | |style="text-align:right;"|והאלגו חלק אחד מתקע"ו חלקים באחד | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you add its root to it, it is a third of an eighth and one part of 576; it has a root and its root is five parts of 24. |
| + | :<math>\scriptstyle{\color{blue}{n^2=x^2+x=\frac{1}{576}+\left(\frac{1}{3}\sdot\frac{1}{8}\right)=\left(\frac{5}{24}\right)^2}}</math> | ||
|style="text-align:right;"|ואם תוסיף עליו שרשו והוא שלישית משמינית וחלק אחד מתקע"ו יחזיק שרש ושרשו חמשה חלקים מכ"ד | |style="text-align:right;"|ואם תוסיף עליו שרשו והוא שלישית משמינית וחלק אחד מתקע"ו יחזיק שרש ושרשו חמשה חלקים מכ"ד | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you add to the square its two roots, it is half a sixth and one part of 576; it has a root and its root is seven parts of 24. |
| + | :<math>\scriptstyle{\color{blue}{m^2=x^2+2x=\frac{1}{576}+\left(\frac{1}{2}\sdot\frac{1}{6}\right)=\left(\frac{7}{24}\right)^2}}</math> | ||
|style="text-align:right;"|ואם תוסיף באלגו שני שרשיו והוא חצי ששית וחלק אחד מתקע"ו ומחזיק שרש ושרשו שבעה חלקים מכ"ד | |style="text-align:right;"|ואם תוסיף באלגו שני שרשיו והוא חצי ששית וחלק אחד מתקע"ו ומחזיק שרש ושרשו שבעה חלקים מכ"ד | ||
|- | |- | ||
| | | | ||
| − | : | + | :The square is a [third] of an eighth [?]. |
|style="text-align:right;"|והאלגו ששית משמינית | |style="text-align:right;"|והאלגו ששית משמינית | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you wish, suppose the sought-after number that has a root is 49 and the added parts are 120 and 240. |
| − | + | :<math>\scriptstyle{\color{blue}{a^2=49\quad b=120\quad 2b=240}}</math> | |
| − | |style="text-align:right;"|ואם תרצה תניח המספר המושג או המחופש שמחזיק שרש מ"ט | + | |style="text-align:right;"|ואם תרצה תניח המספר המושג או המחופש שמחזיק שרש מ"ט והחלק הנוסף ק"כ ור"מ |
| − | והחלק הנוסף ק"כ ור"מ | ||
|- | |- | ||
| | | | ||
| − | : | + | :You know that when you add 120 to 49, it is 169 and its root is 13. |
| + | :<math>\scriptstyle{\color{blue}{49+120=169=13^2}}</math> | ||
|style="text-align:right;"|ואתה יודע כי כשתוסיף על מ"ט ק"כ יהיה קס"ט ושרשו י"ג | |style="text-align:right;"|ואתה יודע כי כשתוסיף על מ"ט ק"כ יהיה קס"ט ושרשו י"ג | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you add 240 to it, it is 289 and its root is 17. |
| + | :<math>\scriptstyle{\color{blue}{49+240=289=17^2}}</math> | ||
|style="text-align:right;"|ואם תוסיף עליו ר"מ יהיה רפ"ט ושרשו י"ז | |style="text-align:right;"|ואם תוסיף עליו ר"מ יהיה רפ"ט ושרשו י"ז | ||
|- | |- | ||
| | | | ||
| − | : | + | :When you wish to know the value of the square, divide 49 by 120; the quotient is a fifth, an eighth and half a sixth, and this is the root of the square. |
| + | :<math>\scriptstyle{\color{blue}{x=\frac{a^2}{b}=\frac{49}{120}=\frac{1}{5}+\frac{1}{8}+\left(\frac{1}{2}\sdot\frac{1}{6}\right)}}</math> | ||
|style="text-align:right;"|וכאשר תרצה לדעת שעור האלגו תחלק מ"ט על ק"כ ויעלה לחלק חומש אחד ושמינית אחד וחצי ששית והוא שרש האלגו | |style="text-align:right;"|וכאשר תרצה לדעת שעור האלגו תחלק מ"ט על ק"כ ויעלה לחלק חומש אחד ושמינית אחד וחצי ששית והוא שרש האלגו | ||
|- | |- | ||
| | | | ||
| − | : | + | :The square is two thousand and 401 parts of 14 thousand and 400. |
| + | :<math>\scriptstyle{\color{blue}{x^2=\frac{2401}{14400}}}</math> | ||
|style="text-align:right;"|והאלגו אלפיים ות"א חלקים מי"ד אלפים ות' באחד | |style="text-align:right;"|והאלגו אלפיים ות"א חלקים מי"ד אלפים ות' באחד | ||
|- | |- | ||
| | | | ||
| + | :If you add its root to it, which is five thousand and 880 [parts] of 14 thousand and 400, it is eight thousand and 281 [parts] of 14 thousand and 400, which has a root and its root is 91 parts of 120. | ||
|style="text-align:right;"|ואם תוסיף עליו שרשו והוא חמשת אלפים ותת"פ מי"ד אלפים ות' יהיה שמנת אלפים ורפ"א מי"ד אלפים ות' ויחזיק שרש ושרשו צ"א חלקים מק"כ | |style="text-align:right;"|ואם תוסיף עליו שרשו והוא חמשת אלפים ותת"פ מי"ד אלפים ות' יהיה שמנת אלפים ורפ"א מי"ד אלפים ות' ויחזיק שרש ושרשו צ"א חלקים מק"כ | ||
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| − | + | :<math>\scriptstyle{\color{blue}{n^2=x^2+x=\frac{2401}{14400}+\left[\frac{1}{5}+\frac{1}{8}+\left(\frac{1}{2}\sdot\frac{1}{6}\right)\right]=\frac{2401}{14400}+\frac{5880}{14400}=\frac{8281}{14400}=\left(\frac{91}{120}\right)^2}}</math> | |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ואם תוסיף על האלגו שני שרשיו והוא י"א אלפים ותש"ס מי"ד אלפים ות' יהיה י"ד אלפים ות' ויחזיק שרש ושרשו | + | :If you add to the square its two roots, which is 11 thousand and 760 [parts] of 14 thousand and 400, it is [14 thousand and 161 parts of] 14 thousand and 400, which has a root and its root is 1[1]9 parts of 120. |
| + | |style="text-align:right;"|ואם תוסיף על האלגו שני שרשיו והוא י"א אלפים ותש"ס מי"ד אלפים ות' יהיה י"ד אלפים ות' ויחזיק שרש ושרשו ק"ט מק"כ | ||
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| − | + | :<math>\scriptstyle{\color{blue}{m^2=x^2+2x=\frac{2401}{14400}+2\sdot\left[\frac{1}{5}+\frac{1}{8}+\left(\frac{1}{2}\sdot\frac{1}{6}\right)\right]=\frac{2401}{14400}+\frac{11760}{14400}=\frac{14161}{14400}=\left(\frac{1{\color{red}{1}}9}{120}\right)^2}}</math> | |
|- | |- | ||
| | | | ||
| − | :8) <math>\scriptstyle\begin{cases}\scriptstyle x^2+x=n^2\\\scriptstyle x^2+3x=m^2\end{cases}</math> | + | :{{#annot:x²+x=n²,x²+3x=m²|1124|5iEI}}8) If you are told: a square, if you add its root to it, it has a root; and if you add its three roots to it, it has a root. |
| − | |style="text-align:right;"|ח <big>וכן</big> אם יאמרו לך אלגו אם תוסיף עליו שרשו יחזיק שרש ואם תוסיף עליו שלשה שרשיו יחזיק שרש | + | :<math>\scriptstyle\begin{cases}\scriptstyle x^2+x=n^2\\\scriptstyle x^2+3x=m^2\end{cases}</math> |
| + | |style="text-align:right;"|ח <big>וכן</big> אם יאמרו לך אלגו אם תוסיף עליו שרשו יחזיק שרש ואם תוסיף עליו שלשה שרשיו יחזיק שרש{{#annotend:5iEI}} | ||
|- | |- | ||
| | | | ||
| Line 646: | Line 676: | ||
|- | |- | ||
| | | | ||
| − | :: | + | :The required number that has a root is one and the numbers added to it are eight and 24. |
| − | |style="text-align:right;"|והמספר הנשאל אשר מחזיק שרש אחד | + | :<math>\scriptstyle{\color{blue}{a^2=1\quad b=8\quad3b=24}}</math> |
| − | והמספר הנוסף עליו שמנה וכ"ד | + | |style="text-align:right;"|והמספר הנשאל אשר מחזיק שרש אחד והמספר הנוסף עליו שמנה וכ"ד |
|- | |- | ||
| | | | ||
| − | : | + | :Divide one by eight; the result is an eighth and this is the root of the square. |
| + | :<math>\scriptstyle{\color{blue}{x=\frac{a^2}{b}=\frac{1}{8}}}</math> | ||
|style="text-align:right;"|ותחלק האחד על שמנה יעלה שמינית אחד והוא שרש האלגו | |style="text-align:right;"|ותחלק האחד על שמנה יעלה שמינית אחד והוא שרש האלגו | ||
|- | |- | ||
| | | | ||
| − | : | + | :The square is an eighth of an eighth. |
| + | :<math>\scriptstyle{\color{blue}{x^2=\frac{1}{8}\sdot\frac{1}{8}}}</math> | ||
|style="text-align:right;"|והאלגו שמינית השמינית | |style="text-align:right;"|והאלגו שמינית השמינית | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you add to it its root, which is an eighth, it is an eighth and an eighth of an eighth, which has a root and its root is three-eighths. |
| + | :<math>\scriptstyle{\color{blue}{x^2+x=\left(\frac{1}{8}\sdot\frac{1}{8}\right)+\frac{1}{8}=\left(\frac{3}{8}\right)^2}}</math> | ||
|style="text-align:right;"|ואם תוסיף עליו שרשו והוא שמינית יהיה שמינית ושמינית השמינית ויחזיק שרש ושרשו שלשה שמיניות | |style="text-align:right;"|ואם תוסיף עליו שרשו והוא שמינית יהיה שמינית ושמינית השמינית ויחזיק שרש ושרשו שלשה שמיניות | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you add to it its three roots, which is three-eighths, it is three-eighths and an eighth of an eighth, which has a root and its root is five-eighths. |
| − | |style="text-align:right;"|ואם תוסיף עליו שלשה שרשים והוא שלשה שמיניות יהיה שלשה שמיניות ושמינית השמינית ויחזיק שרש ושרשו חמשה שמיניות | + | :<math>\scriptstyle{\color{blue}{x^2+3x=\left(\frac{1}{8}\sdot\frac{1}{8}\right)+\left(3\sdot\frac{1}{8}\right)=\left(\frac{1}{8}\sdot\frac{1}{8}\right)+\frac{3}{8}=\left(\frac{5}{8}\right)^2}}</math> |
| + | |style="text-align:right;"|ואם תוסיף עליו שלשה שרשים והוא שלשה שמיניות יהיה שלשה שמיניות ושמינית השמינית ויחזיק שרש<s>ו</s> ושרשו חמשה שמיניות | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you wish, suppose the number that has a root is 169 and the numbers added to it are 120 and 360. |
| − | + | :<math>\scriptstyle{\color{blue}{a^2=169\quad b=120\quad3b=360}}</math> | |
| − | |style="text-align:right;"|ואם תרצה תניח המספר המחזיק שרש קס"ט< | + | |style="text-align:right;"|ואם <sup>תרצה</sup> תניח המספר המחזיק <s>שרש קס"ט והמספר הנוסף עליו ק"כ וש"ס בעבור כי תוסיף על קס"ט ק"כ</s> ‫<ref>66r</ref>ואם תרצה תניח המספר המחזיק שרש קס"ט והמספר הנוסף עליו ק"כ וש"ס |
| − | והמספר הנוסף עליו ק"כ וש"ס | ||
|- | |- | ||
| | | | ||
| − | : | + | :Because, when you add 120 to 169, it is 289, which has a root and its root is seventeen. |
| − | |style="text-align:right;"|וזה כי כשתוסיף על קס"ט ק"כ יהיה רפ"ט ומחזיק | + | :<math>\scriptstyle{\color{blue}{a^2+b=169+120=289=17^2}}</math> |
| + | |style="text-align:right;"|וזה כי כשתוסיף על קס"ט ק"כ יהיה רפ"ט ומחזיק שרש ושרשו הוא שבעה עשר | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you add 360 to it, it is 529, which has a root and its root is twenty-three. |
| + | :<math>\scriptstyle{\color{blue}{a^2+3b=169+360=529=23^2}}</math> | ||
|style="text-align:right;"|ואם תוסיף עליו ש"ס יהיה תקכ"ט ומחזיק שרש ושרשו שלשה ועשרים | |style="text-align:right;"|ואם תוסיף עליו ש"ס יהיה תקכ"ט ומחזיק שרש ושרשו שלשה ועשרים | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you wish to know the value of the square, divide 169 by 120; the result is one and 49 parts of 120 and this is the root of the square. |
| + | :<math>\scriptstyle{\color{blue}{x=\frac{a^2}{b}=\frac{169}{120}=1+\frac{49}{120}}}</math> | ||
|style="text-align:right;"|ואם רצונך לדעת שעור המרובע תחלק קס"ט על ק"כ ויעלה אחד ומ"ט חלקים מק"כ והוא שרש המרובע | |style="text-align:right;"|ואם רצונך לדעת שעור המרובע תחלק קס"ט על ק"כ ויעלה אחד ומ"ט חלקים מק"כ והוא שרש המרובע | ||
|- | |- | ||
| | | | ||
| − | : | + | :The square is one integer and 14 thousand and 161 parts of 14 thousand and 400. |
| + | :<math>\scriptstyle{\color{blue}{x^2=1+\frac{14161}{14400}}}</math> | ||
|style="text-align:right;"|והמרובע יהיה אחד שלם וי"ד אלפים וקס"א חלקי' מי"ד אלפים ות' באחד | |style="text-align:right;"|והמרובע יהיה אחד שלם וי"ד אלפים וקס"א חלקי' מי"ד אלפים ות' באחד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ואם תוסיף עליו שרשו יעלה שלשה שלמים ותרע"ו אלפים ותתק"כ חלקים מאלף אלפים ותשכ"ח אלפים באחד ומחזיק שרש | + | :If you add its root to it, the result is three integers plus 676 thousand and 920 parts of a thousand of a thousand and 728 thousand, which has a root and its root is one and 101 parts of 120. |
| − | ושרשו הוא אחד וק"א חלקי' מק"כ באחד | + | |style="text-align:right;"|ואם תוסיף עליו שרשו יעלה שלשה שלמים ותרע"ו אלפים ותתק"כ חלקים מאלף אלפים ותשכ"ח אלפים באחד ומחזיק שרש ושרשו הוא אחד וק"א חלקי' מק"כ באחד |
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| − | + | :<math>\scriptstyle{\color{blue}{x^2+x=\left(1+\frac{14161}{14400}\right)+\left(1+\frac{49}{120}\right)=3+\frac{676920}{1728000}=\left(1+\frac{101}{120}\right)^2}}</math> | |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ואם תוסיף עליו שלשת שרשיו והם ארבעה דרהמי ועשרים ושבעה חלקים מק"כ באחד יהיה ששה דרהמי ושלשת אלפי' חלקי' מי"ד אלפים ות' ומחזיק שרש | + | :If you add its three roots to it, which is four dirham and twenty-seven parts of 120, it is six dirham plus three thousand [and one] parts of 14 thousand and 400, which has a root and its root is [two] and [5]9 [parts] of 120. |
| − | ושרשו אחד | + | |style="text-align:right;"|ואם תוסיף עליו שלשת שרשיו והם ארבעה דרהמי ועשרים ושבעה חלקים מק"כ באחד יהיה ששה דרהמי ושלשת אלפי' חלקי' מי"ד אלפים ות' ומחזיק שרש ושרשו אחד וצ"ט מק"כ |
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| − | + | :<math>\scriptstyle{\color{blue}{x^2+3x=\left(1+\frac{14161}{14400}\right)+3\sdot\left(1+\frac{49}{120}\right)=\left(1+\frac{14161}{14400}\right)+\left(4+\frac{27}{120}\right)=6+\frac{300{\color{red}{1}}}{14400}=\left({\color{red}{2}}+\frac{{\color{red}{5}}9}{120}\right)^2}}</math> | |
|- | |- | ||
| − | |There are many | + | |There are many solutions for this problem and its similar. |
|style="text-align:right;"|ולזאת השאלה ולדומים אליה דרכים רבים | |style="text-align:right;"|ולזאת השאלה ולדומים אליה דרכים רבים | ||
|- | |- | ||
| − | | | + | |Now, I will explain to you the reason for the extraction of these numbers, so that you will have scales for all these solutions. |
| − | |style="text-align:right;"|ועתה אבאר לך הסבה בהוצאת אלו המספרים | + | |style="text-align:right;"|ועתה אבאר לך הסבה בהוצאת אלו המספרים למען תהיה לך למאזנים לכל אלו האופנים |
|- | |- | ||
| − | | | + | |Know that for any square that has a root, if you add to it its two roots plus one dirham, [the sum] has a root and its root is as the root of the original square plus one. |
| − | : | + | :<math>\scriptstyle{\color{OliveGreen}{x^2+2x+1=\left(x+1\right)^2}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>דע</big> כי כל מרובע המחזיק שרש אם תקבץ עמו שני שרשיו ואדרהם אחד יחזיק שרש ושרשו הוא כמו שרש המרובע הראשון עם תוספת אחד |
|- | |- | ||
| − | | | + | |And when you wish to find a number that has a root, such that when you add to it another number, [their sum] has a root, and when you add to it twice the number that you added at the first time, or thrice, or four times, or as many times as you wish, the sum has a root. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכאשר תבקש למצוא מספר מהמספרים שיחזיק שרש וכאשר תוסיף עליו מספר מה אחר יחזיק שרש וכאשר תוסיף עליו שני פעמים המספר שהוספת בראשונה או שלשה פעמים או ארבעה פעמים או כמה שתרצה מהפעמים יהיה לאשר יתקבץ מהם שרש |
|- | |- | ||
| − | | | + | | |
| − | *<math>\scriptstyle\ | + | *<math>\scriptstyle{\color{OliveGreen}{\begin{cases}\scriptstyle a^2+b=n^2\\\scriptstyle a^2+2b=m^2\backslash\quad a^2+3b=m^2\backslash\quad a^2+4b=m^2\end{cases}}}</math> |
| − | + | | | |
| − | |||
| − | | | ||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Suppose your square is a square [<math>\scriptstyle{\color{blue}{x^2}}</math>]. |
|style="text-align:right;"|תניח המרובע שלך מרובע | |style="text-align:right;"|תניח המרובע שלך מרובע | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle x^2+2x+1=\left(x+1\right)^2</math> | + | :Sum it with two of its roots and one dirham; the result has a root and its root is a thing and one dirham. |
| + | :<math>\scriptstyle{\color{OliveGreen}{x^2+2x+1=\left(x+1\right)^2}}</math> | ||
|style="text-align:right;"|ותקבץ עמו שנים משרשיו ואדרהם אחד ויהיה העולה יחזיק שרש ושרשו הוא דבר ואדרהם אחד | |style="text-align:right;"|ותקבץ עמו שנים משרשיו ואדרהם אחד ויהיה העולה יחזיק שרש ושרשו הוא דבר ואדרהם אחד | ||
|- | |- | ||
| | | | ||
| − | + | :<math>\scriptstyle{\color{blue}{x^2+2\sdot\left(2x+1\right)=x^2+4x+2}}</math> | |
|style="text-align:right;"|ואם תרצה להוסיף על המרובע כשעור המספר הראשון תקבץ עמו ארבעה שרשים ושנים דרהמי ואם תרצה שלשה פעמים או ארבעה תקח כפי זה | |style="text-align:right;"|ואם תרצה להוסיף על המרובע כשעור המספר הראשון תקבץ עמו ארבעה שרשים ושנים דרהמי ואם תרצה שלשה פעמים או ארבעה תקח כפי זה | ||
|- | |- | ||
| | | | ||
:*[Defining: <math>\scriptstyle{\color{red}{\left(x+1+\frac{1}{2}\right)^2}}=x^2+4x+2</math>] | :*[Defining: <math>\scriptstyle{\color{red}{\left(x+1+\frac{1}{2}\right)^2}}=x^2+4x+2</math>] | ||
| − | |style="text-align:right;"|ואם לקחת כשעור המספר יהיה מרובע וארבעה שרשים ושנים דרהמי | + | |style="text-align:right;"|ואם לקחת כשעור המספר יהיה מרובע וארבעה שרשים ושנים דרהמי |
| + | |- | ||
| + | | | ||
| + | :*<span style="color:Green>Confrontation:</span> | ||
| + | | | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x=\frac{1}{4}}}</math> | + | :Confront them; the thing is equal to a quarter of a dirham; and this is the root of the square. |
| − | |style="text-align:right;"|ויהיה דבר ישוה רביעית אדרהם והוא שרש המרובע | + | :<math>\scriptstyle{\color{blue}{x=\frac{1}{4}}}</math> |
| + | |style="text-align:right;"|ותכונהו עמהם ויהיה דבר ישוה רביעית אדרהם והוא שרש המרובע | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x^2=\frac{1}{2}\sdot\frac{1}{8}}}</math> | + | :The square is half an eighth. |
| + | :<math>\scriptstyle{\color{blue}{x^2=\frac{1}{2}\sdot\frac{1}{8}}}</math> | ||
|style="text-align:right;"|והמרובע הוא חצי שמינית | |style="text-align:right;"|והמרובע הוא חצי שמינית | ||
|- | |- | ||
| | | | ||
| − | : | + | :It is as if we assume the number added to it is two things and one dirham; so it is one and a half dirham, because the thing is a quarter of a dirham. |
| − | |style="text-align:right;"|ונהיה כאלו הנחנו המספר הנוסף עליו שני דברים ודרהם אחד ויהיה אדרהם אחד וחצי בעבור כי הדבר הוא רביע דרהם | + | :<math>\scriptstyle{\color{blue}{2x+1=1+\frac{1}{2}}}</math> |
| + | |style="text-align:right;"|ונהיה כאלו הנחנו המספר הנוסף עליו שני דברים ודרהם אחד ויהיה אדרהם אחד וחצי בעבור ‫<ref>66v</ref>כי הדבר הוא רביע דרהם | ||
|- | |- | ||
| | | | ||
| − | : | + | :The dirham is sixteen. |
|style="text-align:right;"|והדרהם ששה עשר | |style="text-align:right;"|והדרהם ששה עשר | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{b=16\sdot\left(1+\frac{1}{2}\right)=24}}</math> | + | :Therefore, the one dirham and a half is twenty-four parts and this is the number that when you add it to one, it has a root. |
| − | |style="text-align:right;"|ויהיה מפני זה האדרהם וחצי עשרים וארבעה חלקים | + | :<math>\scriptstyle{\color{blue}{b=16\sdot\left(1+\frac{1}{2}\right)=24\longrightarrow1+24=n^2}}</math> |
| + | |style="text-align:right;"|ויהיה מפני זה האדרהם וחצי עשרים וארבעה חלקים והוא המספר שכאשר תוסיף <sup>אותו</sup> על האחד יחזיק שרש | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{1+ | + | :If you add it twice, which is 48, it also has a root. |
| − | |style="text-align:right;"|והוא | + | :<math>\scriptstyle{\color{blue}{1+\left(2\sdot24\right)=1+48=m^2}}</math> |
| + | |style="text-align:right;"|ואם תוסיפהו שני פעמים והוא מ"ח יחזיק גם כן שרש | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|ותשוה אחר זה כאשר בארתי לך | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |If you want, you can solve this question in another way: |
|style="text-align:right;"|<big>ואם</big> תרצה תעשה לזאת השאלה דרך אחרת | |style="text-align:right;"|<big>ואם</big> תרצה תעשה לזאת השאלה דרך אחרת | ||
|- | |- | ||
| | | | ||
| − | : | + | :Suppose the root of the square plus four things and two dirham is a thing, plus one dirham and two-thirds of a dirham. |
| + | :<math>\scriptstyle{\color{blue}{x+1+\frac{2}{3}=\sqrt{x^2+4x+2}}}</math> | ||
|style="text-align:right;"|תניח שרש המרובע והארבעה דברים ושני דרהמי דבר ודרהם אחד ושני שלישי דרהם | |style="text-align:right;"|תניח שרש המרובע והארבעה דברים ושני דרהמי דבר ודרהם אחד ושני שלישי דרהם | ||
|- | |- | ||
| | | | ||
| − | : | + | :Multiply this root by itself; it is a square, two dirham and seven-ninths of a dirham, plus three things and a third of a thing equal a square plus four things and two dirham. |
| − | |style="text-align:right;"|ותכה זה השרש בעצמו | + | |style="text-align:right;"|ותכה זה השרש בעצמו ויהיה מרובע ושני דרהמי ושבע תשיעיות מדרהם ושלשה דברים ושליש דבר ישוו מרובע וארבעה דברים ושני דרהמי |
|- | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\left(x+1+\frac{2}{3}\right)^2=x^2+4x+2=x^2+\left(3+\frac{1}{3}\right)x+\left(2+\frac{7}{9}\right)}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :*<span style="color:Green>Confrontation:</span> | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x=1+\frac{1}{6}}}</math> | + | :Confront them; the thing is one dirham and a sixth; and this is the root of the square. |
| − | |style="text-align:right;"|ויהיה הדבר דרהם אחד ושתות והוא שרש המרובע | + | :<math>\scriptstyle{\color{blue}{x=1+\frac{1}{6}}}</math> |
| + | |style="text-align:right;"|ותכונם יחד ויהיה הדבר דרהם אחד ושתות והוא שרש המרובע | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x^2=\frac{49}{36}}}</math> | + | :The square is 49 parts of 36 of one dirham; and this is the required part that has a root. |
| + | :<math>\scriptstyle{\color{blue}{x^2=\frac{49}{36}}}</math> | ||
|style="text-align:right;"|והמרובע יהיה מ"ט חלקים מל"ו חלקים באדרהם והוא החלק הנשאל שיחזיק שרש | |style="text-align:right;"|והמרובע יהיה מ"ט חלקים מל"ו חלקים באדרהם והוא החלק הנשאל שיחזיק שרש | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle\begin{cases}\scriptstyle49+a=n^2\\\scriptstyle49+2a=m^2\end{cases}</math> | + | *{{#annot:49+x=n²,49+2x=m²|1124|Y9EL}}If you wish to know the number that when you add it to 49, it has a root; and if you add it to it twice, it has a root. |
| − | |style="text-align:right;"|<big>ואם</big> רצית לדעת המספר שכאשר תוסיפהו על מ"ט יחזיק שרש ואם תוסיפהו עליו שני פעמים יחזיק שרש | + | :<math>\scriptstyle\begin{cases}\scriptstyle49+a=n^2\\\scriptstyle49+2a=m^2\end{cases}</math> |
| + | |style="text-align:right;"|<big>ואם</big> רצית לדעת המספר שכאשר תוסיפהו על מ"ט יחזיק שרש ואם תוסיפהו עליו שני פעמים יחזיק שרש{{#annotend:Y9EL}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{a=2x+1=2\sdot\left(1+\frac{1}{6}\right)+1=3+\frac{1}{3}}}</math> | + | :You know that the first added number is assumed to be two roots and one dirham and we have already explained that the root is one dirham and a sixth, and the dirham is 36 parts. So, the two roots and one dirham are three and a third. |
| − | + | :<math>\scriptstyle{\color{blue}{a=2x+1=2\sdot\left(1+\frac{1}{6}\right)+1=3+\frac{1}{3}}}</math> | |
|style="text-align:right;"|הנה ידעת שהנחת המספר הראשון הנוסף שני שרשים ודרהם אחד וכבר בארנו שהשרש הוא דרהם אחד ושתות והאדרהם ל"ו חלקים ויהיו שני השרשים ואדרהם שלשה ושליש | |style="text-align:right;"|הנה ידעת שהנחת המספר הראשון הנוסף שני שרשים ודרהם אחד וכבר בארנו שהשרש הוא דרהם אחד ושתות והאדרהם ל"ו חלקים ויהיו שני השרשים ואדרהם שלשה ושליש | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{a=36\sdot\left(3+\frac{1}{3}\right)=120}}</math> | + | :Multiply three and a third by 36; it is 120, and this is the number that when you add it to 49, it has a root. |
| − | |style="text-align:right;"|ותכה שלשה ושליש בל"ו ויהיה ק"כ | + | :<math>\scriptstyle{\color{blue}{a=36\sdot\left(3+\frac{1}{3}\right)=120\longrightarrow49+120=n^2}}</math> |
| + | |style="text-align:right;"|ותכה שלשה ושליש בל"ו ויהיה ק"כ והוא המספר שכאשר תוסיפהו על מ"ט יחזיק שרש | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{49+ | + | :If you add 120 twice to 49, it is 240, which has a root. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{49+\left(2\sdot120\right)=49+240=m^2}}</math> |
| + | |style="text-align:right;"|ואם תוסיף על מ"ט שני פעמים ק"כ והוא ר"מ יחזיק שרש | ||
|- | |- | ||
| | | | ||
| − | : | + | :Proceed as I explained. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ותעשה כאשר בארתי |
|- | |- | ||
| | | | ||
| − | :9) <math>\scriptstyle\begin{cases}\scriptstyle x^2-2x=n^2\\\scriptstyle x^2-3x=m^2\end{cases}</math> | + | :{{#annot:x²-2x=n²,x²-3x=m²|1124|65Jm}}9) If you are told: a square that has a root, if you subtract its two roots from it, the remainder has a root; and if you subtract its three roots from the square, the remainder has a root. |
| − | |style="text-align:right;"|ט <big>וכן</big> אם יאמרו לך מרובע המחזיק שרש אם תגרע ממנו שני שרשים יחזיק הנשאר שרש ואם תגרע מהמרובע שלשה שרשים יחזיק הנשאר שרש | + | :<math>\scriptstyle\begin{cases}\scriptstyle x^2-2x=n^2\\\scriptstyle x^2-3x=m^2\end{cases}</math> |
| + | |style="text-align:right;"|ט <big>וכן</big> אם יאמרו לך מרובע המחזיק שרש אם תגרע ממנו שני שרשים יחזיק הנשאר שרש ואם תגרע מהמרובע שלשה שרשים יחזיק הנשאר שרש{{#annotend:65Jm}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :This is an indeterminate problem. |
| − | |style="text-align:right;"|וזאת השאלה חרשת< | + | |style="text-align:right;"|וזאת השאלה חרשת<sup>נ' אלמת</sup> |
|- | |- | ||
| | | | ||
| − | : | + | :Solve it according to the following procedure: |
|style="text-align:right;"|ותעשה אותה כמעשה הזה | |style="text-align:right;"|ותעשה אותה כמעשה הזה | ||
|- | |- | ||
| | | | ||
| − | : | + | :Look for a number that has a root [<math>\scriptstyle{\color{blue}{a^2}}</math>], such that when you subtract from it another number, the remainder has a root [<math>\scriptstyle{\color{OliveGreen}{a^2-b=n^2}}</math>]; and when you subtract from it that number and its half, the remainder has a root [<math>\scriptstyle{\color{OliveGreen}{a^2-\left(b+\frac{1}{2}b\right)=m^2}}</math>] |
| − | |||
|style="text-align:right;"|שתבקש מספר שיחזיק שרש וכאשר תגרע ממנו מספר אחר יהיה הנשאר יחזיק שרש ואם תגרע ממנו אותו המספר וכמו חציו יהיה הנשאר יחזיק שרש | |style="text-align:right;"|שתבקש מספר שיחזיק שרש וכאשר תגרע ממנו מספר אחר יהיה הנשאר יחזיק שרש ואם תגרע ממנו אותו המספר וכמו חציו יהיה הנשאר יחזיק שרש | ||
|- | |- | ||
| | | | ||
| − | : | + | :Because the three roots are as twice the number and its half. |
| + | :<math>\scriptstyle{\color{blue}{3x=2x+\left(\frac{1}{2}\sdot2x\right)}}</math> | ||
|style="text-align:right;"|בעבור כי שלשת השרשים כמו שני המספרים וחצי יותר | |style="text-align:right;"|בעבור כי שלשת השרשים כמו שני המספרים וחצי יותר | ||
|- | |- | ||
| − | | | + | |When you find this number, divide it by the subtracted number and the quotient is the root of the square. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{x=\frac{a^2}{\frac{1}{2}b}}}</math> | |
| − | |style="text-align:right;"|וכאשר תמצא אותו המספר | + | |style="text-align:right;"|וכאשר תמצא אותו המספר תחלק<sup>הו</sup> על <sup>חצי</sup> המספר הנגרע והעולה לחלק יהיה שרש המרובע |
|- | |- | ||
| − | | | + | |You divide it by half the subtracted number, since the subtracted number represents the two roots of the square, and its half represents the root of the square. |
| − | |||
|style="text-align:right;"|וחלקת על חצי המספר הנגרע בעבור כי המספר הנגרע עלה במחשבת המספרים כפי שני שרשי המרובע והחצי עלה כפי שרש המרובע | |style="text-align:right;"|וחלקת על חצי המספר הנגרע בעבור כי המספר הנגרע עלה במחשבת המספרים כפי שני שרשי המרובע והחצי עלה כפי שרש המרובע | ||
|- | |- | ||
| | | | ||
| − | :: | + | :The minuend number that has a root is 25; the number subtracted from it is 16, which is generated from two roots, each root is eight; the other number, which is three roots, is 24. |
| − | |style="text-align:right;"|והמספר הנגרע שיחזיק שרש כ"ה | + | :<math>\scriptstyle{\color{blue}{a^2=25\quad b=2x=16\quad\frac{1}{2}b=x=8\quad3x=24}}</math> |
| − | והמספר הנגרע ממנו הוא י"ו | + | |style="text-align:right;"|והמספר הנגרע שיחזיק ‫<ref>67r</ref>שרש כ"ה והמספר הנגרע ממנו הוא י"ו והוא צמח ועלה משני שרשים כל שרש שמנה והמספר האחר שהוא שלשה שרשים כ"ד |
| − | והוא צמח ועלה משני שרשים כל שרש שמנה | ||
| − | והמספר האחר שהוא שלשה שרשים כ"ד | ||
|- | |- | ||
| | | | ||
| + | :When you subtract 16 from 25, nine remains, which has a root and it is three. | ||
:<math>\scriptstyle{\color{blue}{25-16=9=3^2}}</math> | :<math>\scriptstyle{\color{blue}{25-16=9=3^2}}</math> | ||
|style="text-align:right;"|כאשר תגרע מכ"ה י"ו ישאר תשעה ומחזיק שרש והוא שלשה | |style="text-align:right;"|כאשר תגרע מכ"ה י"ו ישאר תשעה ומחזיק שרש והוא שלשה | ||
|- | |- | ||
| | | | ||
| + | :When you subtract 24 from it, one remains, and its root is one. | ||
:<math>\scriptstyle{\color{blue}{25-24=1=1^2}}</math> | :<math>\scriptstyle{\color{blue}{25-24=1=1^2}}</math> | ||
|style="text-align:right;"|וכאשר תגרע מהם כ"ד ישאר אחד ושרשו אחד | |style="text-align:right;"|וכאשר תגרע מהם כ"ד ישאר אחד ושרשו אחד | ||
|- | |- | ||
| | | | ||
| + | :When you wish to know the value of the square, divide 25 by eight, the quotient is three and an eighth and this is the root of the square. | ||
:<math>\scriptstyle{\color{blue}{x=\frac{25}{8}=3+\frac{1}{8}}}</math> | :<math>\scriptstyle{\color{blue}{x=\frac{25}{8}=3+\frac{1}{8}}}</math> | ||
|style="text-align:right;"|וכאשר תרצה לדעת שעור המרובע תחלק כ"ה על שמנה ויעלה לחלק שלשה ושמינית והוא שרש המרובע | |style="text-align:right;"|וכאשר תרצה לדעת שעור המרובע תחלק כ"ה על שמנה ויעלה לחלק שלשה ושמינית והוא שרש המרובע | ||
|- | |- | ||
| | | | ||
| + | :The square is nine, six-eighths, and an eighth of an eighth. | ||
:<math>\scriptstyle{\color{blue}{x^2=9+\frac{6}{8}+\left(\frac{1}{8}\sdot\frac{1}{8}\right)}}</math> | :<math>\scriptstyle{\color{blue}{x^2=9+\frac{6}{8}+\left(\frac{1}{8}\sdot\frac{1}{8}\right)}}</math> | ||
|style="text-align:right;"|והמרובע תשעה וששה שמיניות ושמינית השמינית | |style="text-align:right;"|והמרובע תשעה וששה שמיניות ושמינית השמינית | ||
|- | |- | ||
| | | | ||
| + | :Subtract its two roots from it, which is six and a quarter; the remainder is three and an eighth of an eighth, which has a root and it is one and seven-eighths. | ||
|style="text-align:right;"|ותגרע ממנו שני שרשיו והוא ששה ורביע וישאר שלשה וחצי ושמינית השמינית ומחזיק שרש והוא אחד ושבעה שמיניות | |style="text-align:right;"|ותגרע ממנו שני שרשיו והוא ששה ורביע וישאר שלשה וחצי ושמינית השמינית ומחזיק שרש והוא אחד ושבעה שמיניות | ||
|- | |- | ||
| Line 872: | Line 924: | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ותגרע מהמרובע שלשת שרשיו שהם תשעה ושלשה שמיניות ישאר שלשה שמיניו' ושמינית השמינית ויחזיק שרש והוא חמשה שמיניות | + | :Subtract from the square its three roots, which is nine and three-eighths; the remainder is three-eighths and an eighth of an eighth, which has a root and it is five-eighths. |
| + | |style="text-align:right;"|ותגרע מהמרובע שלשת שרשיו שהם תשעה ושלשה שמיניות ישאר שלשה שמיניו' ושמינית השמינית <s>וישאר</s><sup>ויחזיק</sup> שרש והוא חמשה שמיניות | ||
|- | |- | ||
|colspan=2| | |colspan=2| | ||
:<math>\scriptstyle{\color{blue}{x^2-3x=\left[9+\frac{6}{8}+\left(\frac{1}{8}\sdot\frac{1}{8}\right)\right]-\left(9+\frac{3}{8}\right)=\frac{3}{8}+\left(\frac{1}{8}\sdot\frac{1}{8}\right)=\left(\frac{5}{8}\right)^2}}</math> | :<math>\scriptstyle{\color{blue}{x^2-3x=\left[9+\frac{6}{8}+\left(\frac{1}{8}\sdot\frac{1}{8}\right)\right]-\left(9+\frac{3}{8}\right)=\frac{3}{8}+\left(\frac{1}{8}\sdot\frac{1}{8}\right)=\left(\frac{5}{8}\right)^2}}</math> | ||
|- | |- | ||
| − | |The extraction of these numbers is already obvious from what | + | |The extraction of these numbers is already obvious from what we have shown to you. |
|style="text-align:right;"|והוצאת המספרים האלה כבר תהיה נגלית מאשר הראינו לך | |style="text-align:right;"|והוצאת המספרים האלה כבר תהיה נגלית מאשר הראינו לך | ||
|- | |- | ||
| − | | | + | |But, I will further teach you about it, in order that you will remember it properly. |
|style="text-align:right;"|אבל אשכילך עוד עליהם למען יכנס הדבר בלבך היטב | |style="text-align:right;"|אבל אשכילך עוד עליהם למען יכנס הדבר בלבך היטב | ||
|- | |- | ||
| − | |Know that for any number that has a root, if you subtract from it its two roots minus one dirham, the remainder has a root and its root is as the root of the original square minus one dirham.<math>\scriptstyle n^2-\left(2n-1\right)=\left(n-1\right)^2</math> | + | |Know that for any number that has a root, if you subtract from it its two roots minus one dirham, the remainder has a root and its root is as the root of the original square minus one dirham. |
| + | :<math>\scriptstyle{\color{OliveGreen}{n^2-\left(2n-1\right)=\left(n-1\right)^2}}</math> | ||
|style="text-align:right;"|<big>דע</big> כי כל מספר המחזיק שרש כאשר תגרע ממנו שני שרשיו פחות דרהם אחד יהיה לנשאר שרש ושרשו יהיה כמו שרש המרובע פחות דרהם אחד | |style="text-align:right;"|<big>דע</big> כי כל מספר המחזיק שרש כאשר תגרע ממנו שני שרשיו פחות דרהם אחד יהיה לנשאר שרש ושרשו יהיה כמו שרש המרובע פחות דרהם אחד | ||
|- | |- | ||
| − | | | + | | |
| − | + | *{{#annot:x²-y=n²,x²-1½y=m²|1124|CUHD}}When you wish to find a number that has a root, such that when you subtract from it another number, the remainder has a root; and if you subtract from it again the other number and its half, the remainder has a root. | |
| − | |style="text-align:right;"|וכאשר תרצה למצוא מספר המחזיק שרש שכאשר תגרע ממנו מספר אחר יהיה לנשאר שרש ואם תגרע עוד ממנו כמו המספר האחר וכמו חציו יהיה לנשאר שרש | + | :<math>\scriptstyle\begin{cases}\scriptstyle a^2-b=n^2\\\scriptstyle a^2-\left(1+\frac{1}{2}\right)b=m^2\end{cases}</math> |
| + | |style="text-align:right;"|וכאשר תרצה למצוא מספר המחזיק שרש שכאשר תגרע ממנו מספר אחר יהיה לנשאר שרש ואם תגרע עוד ממנו <sup>כמו</sup> המספר האחר וכמו חציו יהיה לנשאר שרש{{#annotend:CUHD}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :Suppose your square is a square [<math>\scriptstyle{\color{blue}{x^2}}</math>]. |
|style="text-align:right;"|תניח המרובע שלך מרובע | |style="text-align:right;"|תניח המרובע שלך מרובע | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle x^2-\left(2x-1\right)=x^2+1-2x=\left(x-1\right)^2</math> | + | :Subtract its two roots minus one dirham from it; the remainder is a square and one dirham minus two roots and its root is a thing minus one dirham. |
| − | |style="text-align:right;"|ותגרע ממנו שני שרשיו פחות דרהם אחד וישאר מרובע ודרהם אחד פחות שני שרשים ושרשו הוא דבר פחות דרהם אחד | + | :<math>\scriptstyle{\color{blue}{x^2-\left(2x-1\right)=x^2+1-2x=\left(x-1\right)^2}}</math> |
| + | |style="text-align:right;"|ותגרע ממנו שני שרשיו פחות דרהם אחד וישאר מרובע <s>פחות</s> ודרהם אחד פחות שני שרשים ושרשו הוא דבר פחות דרהם אחד | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x^2-\left(1+\frac{1}{2}\right)\sdot\left(2x-1\right)=x^2+\left(1+\frac{1}{2}\right)-3x}}</math> | + | :Subtract from this square its two roots minus one dirham and their half; the remainder is a square and one dirham and a half minus three roots. |
| + | :<math>\scriptstyle{\color{blue}{x^2-\left(1+\frac{1}{2}\right)\sdot\left(2x-1\right)=x^2+\left(1+\frac{1}{2}\right)-3x}}</math> | ||
|style="text-align:right;"|ותגרע מזה המרובע שני שרשיו פחות דרהם אחד ועוד כמו חציו וישאר מרובע ודרהם וחצי פחות שלשה דברים | |style="text-align:right;"|ותגרע מזה המרובע שני שרשיו פחות דרהם אחד ועוד כמו חציו וישאר מרובע ודרהם וחצי פחות שלשה דברים | ||
|- | |- | ||
| | | | ||
| − | : | + | :[<math>\scriptstyle{\color{OliveGreen}{\left(3-x\right)^2}}=x^2+\left(1+\frac{1}{2}\right)-3x</math>] |
| | | | ||
|- | |- | ||
| | | | ||
| − | :: | + | :*<span style="color:Green>Confrontation:</span> |
| − | | | + | | |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x | + | :Confront them as I explained to you; the thing is two dirham and a half; and this is the root of the square. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{x=2+\frac{1}{2}}}</math> |
| + | |style="text-align:right;"|ותכוין עמהם כמו שבארתי לך ויהיה הדבר שני דרהמי וחצי והוא שרש המרובע | ||
|- | |- | ||
| | | | ||
| − | : | + | :The square is six and a quarter and this is 25 parts of four [parts] of one dirham. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{x^2=6+\frac{1}{4}=\frac{25}{4}}}</math> |
| + | |style="text-align:right;"|והמרובע ששה ורביע והוא כ"ה חלקים מארבעה בדרהם | ||
|- | |- | ||
| | | | ||
| − | : | + | :We suppose the number subtracted from it is two roots minus one dirham, which is four dirham. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{2x-1=4}}</math> |
| + | |style="text-align:right;"|ונניח <s>החלק</s> <sup>המספר</sup> הנגרע ממנו שני שרשים פחות דרהם אחד והוא <s>חמשה</s> <sup>ארבעה</sup> דרהמי | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{b=4\sdot4=16}}</math> | + | :Each dirham is four parts, so they are sixteen |
| − | |style="text-align:right;"|ויהיו שש עשרה | + | :<math>\scriptstyle{\color{blue}{b=4\sdot4=16}}</math> |
| + | |style="text-align:right;"|וכל דרהם ארבעה חלקי' ויהיו שש עשרה | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{ | + | :The required number that has a root is 25; the number subtracted from it is 16; the other subtracted number is 24. |
| − | |style="text-align:right;"|והנה יהיה המספר המבוקש אשר יחזיק שרש כ"ה | + | :<math>\scriptstyle{\color{blue}{a^2=25\quad b=16\quad\left(1+\frac{1}{2}\right)b=24\quad3x=24}}</math> |
| − | והמספר הנגרע ממנו י"ו | + | |style="text-align:right;"|והנה יהיה המספר המבוקש אשר יחזיק שרש כ"ה והמספר הנגרע ממנו י"ו והמספר השני הנגרע כ"ד |
| − | והמספר השני הנגרע כ"ד | ||
|- | |- | ||
| | | | ||
| − | : | + | :Proceed in the rest of the problem as I have shown you. |
|style="text-align:right;"|ותעשה מהנשאר בשאלה כאשר הראיתיך | |style="text-align:right;"|ותעשה מהנשאר בשאלה כאשר הראיתיך | ||
|- | |- | ||
| | | | ||
| − | : | + | :As I explained to you, you can solve this problem and similar ones with other methods as you wish. |
|style="text-align:right;"|והנה תוכל לעשות לזאת השאלה ולדומים אליה דרכים אחרים כרצונך כאשר בארתי לך | |style="text-align:right;"|והנה תוכל לעשות לזאת השאלה ולדומים אליה דרכים אחרים כרצונך כאשר בארתי לך | ||
|- | |- | ||
| | | | ||
| − | :10) <math>\scriptstyle x-x^2=n^2</math> | + | :{{#annot:x-x²=n²|1124|hdU1}}10) If you are told: a square that has a root, if you subtract it from its root, the remainder has a root. |
| − | |style="text-align:right;"|י <big>ואם</big> יאמרו לך מרובע המחזיק שרש אם תגרעהו מן שרשו יהיה לנשאר שרש | + | :<math>\scriptstyle x-x^2=n^2</math> |
| + | |style="text-align:right;"|‫<ref>67v</ref>י <big>ואם</big> יאמרו לך מרובע המחזיק שרש אם תגרעהו מן שרשו יהיה לנשאר שרש{{#annotend:hdU1}} | ||
|- | |- | ||
| | | | ||
| − | + | :This is an indeterminate problem. | |
|style="text-align:right;"|זאת השאלה חרשת | |style="text-align:right;"|זאת השאלה חרשת | ||
|- | |- | ||
| | | | ||
| − | : | + | :We suppose the square is a square [<math>\scriptstyle{\color{blue}{x^2}}</math>]. |
| − | |style="text-align:right;"|ונניח המרובע מרובע | + | |style="text-align:right;"|‫[ונניח המרובע מרובע |
|- | |- | ||
| | | | ||
| − | : | + | :We subtract it from its root, which is a thing; a thing minus a square remains [<math>\scriptstyle{\color{blue}{n^2=x-x^2}}</math>] and it should have a root. |
| − | |style="text-align:right;"|ונגרעהו משרשו והוא דבר וישאר דבר פחות מרובע וצריך שיחזיק שרש | + | |style="text-align:right;"|ונגרעהו משרשו]‫<ref>marg.</ref> והוא דבר וישאר דבר פחות מרובע וצריך שיחזיק שרש |
|- | |- | ||
| | | | ||
| − | : | + | :Suppose its root is as many things as you want - if you wish, it can be a thing, or more, or less. |
|style="text-align:right;"|תניח שרשו איזה שתרצה מהדברים אם תרצה יהיה דבר או אם תרצה יהיה יותר או אם תרצה יהיה פחות | |style="text-align:right;"|תניח שרשו איזה שתרצה מהדברים אם תרצה יהיה דבר או אם תרצה יהיה יותר או אם תרצה יהיה פחות | ||
|- | |- | ||
| | | | ||
| − | : | + | :Suppose it is a thing [<math>\scriptstyle{\color{blue}{n=\sqrt{x-x^2}=x}}</math>]. |
|style="text-align:right;"|ותנחיהו דבר | |style="text-align:right;"|ותנחיהו דבר | ||
|- | |- | ||
| | | | ||
| − | : | + | :Multiply it by itself; it is a square equals a thing minus a square. |
| − | |style="text-align:right;"|ותכהו בעצמו | + | :<math>\scriptstyle{\color{blue}{x^2=x-x^2}}</math> |
| + | |style="text-align:right;"|ותכהו בעצמו ויהיה מרובע ישוה דבר פחות מרובע | ||
|- | |- | ||
| | | | ||
| − | ::: | + | :*<span style="color:Green>Confrontation:</span> |
| − | | | + | | |
|- | |- | ||
| | | | ||
| − | : | + | :Confront them as I instructed; the thing is equal to half a dirham; and this is the root of the square. |
| + | :<math>\scriptstyle{\color{blue}{x=\frac{1}{2}}}</math> | ||
|style="text-align:right;"|ותכוין עמו כאשר אמרתי ויהיה הדבר ישוה חצי דרהם והוא שרש המרובע | |style="text-align:right;"|ותכוין עמו כאשר אמרתי ויהיה הדבר ישוה חצי דרהם והוא שרש המרובע | ||
|- | |- | ||
| | | | ||
| − | : | + | :The square is a quarter of a dirham. |
| − | |style="text-align:right;"|והמרובע | + | :<math>\scriptstyle{\color{blue}{x^2=\frac{1}{4}}}</math> |
| + | |style="text-align:right;"|והמרובע <sup>רביע</sup> דרהם | ||
|- | |- | ||
| | | | ||
| − | : | + | :Suppose its root is two things [<math>\scriptstyle{\color{blue}{n=\sqrt{x-x^2}=2x}}</math>]. |
|style="text-align:right;"|ואם נניח שרשו שני דברים | |style="text-align:right;"|ואם נניח שרשו שני דברים | ||
|- | |- | ||
| | | | ||
| − | : | + | :The thing is equal to a fifth dirham. |
| − | |style="text-align:right;"|יעלה הדבר ישוה חמישית דרהם | + | :<math>\scriptstyle{\color{blue}{x=\frac{1}{5}}}</math> |
| + | |style="text-align:right;"|יעלה <s>המרובע</s> <sup>הדבר ישוה</sup> חמישית דרהם | ||
|- | |- | ||
| | | | ||
| − | : | + | :The square is a fifth of a fifth of a dirham. |
| + | :<math>\scriptstyle{\color{blue}{x^2=\frac{1}{5}\sdot\frac{1}{5}}}</math> | ||
|style="text-align:right;"|והמרובע חמישית מחמישית דרהם | |style="text-align:right;"|והמרובע חמישית מחמישית דרהם | ||
|- | |- | ||
| | | | ||
| − | : | + | :Subtract it from its root, which is a fifth of a dirham; the remainder is four-fifths of a fifth of a dirham, which has a root and it is two-fifths of a fifth of a dirham. |
| − | |style="text-align:right;"|ותגרעהו משרשו והוא חמישית דרהם וישאר ארבעה חֳמשים מחמישית דרהם | + | :<math>\scriptstyle{\color{blue}{x-x^2=\frac{1}{5}-\left(\frac{1}{5}\sdot\frac{1}{5}\right)=\frac{4}{5}\sdot\frac{1}{5}=\left(\frac{2}{5}\right)^2}}</math> |
| + | |style="text-align:right;"|ותגרעהו משרשו והוא חמישית דרהם וישאר ארבעה חֳמשים מחמישית דרהם ומחזיק שרש והוא שני חומשים מחמישית דרהם | ||
|- | |- | ||
| | | | ||
| − | :11) <math>\scriptstyle\begin{cases}\scriptstyle x+x^2=n^2\\\scriptstyle x-x^2=m^2\end{cases}</math> | + | :{{#annot:x+x²=n²,x-x²=m²|1124|Fofb}}11) If you are told: a square that has a root, if you add its root to it, it has a root; and if you subtract the square from its root, the remainder has a root. |
| − | |style="text-align:right;"|יא <big>ואם</big> יאמרו לך מרובע המחזיק שרש אם תוסיף עליו שרשו יחזיק שרש ואם תגרע המרובע משרשו יהיה לנשאר שרש | + | :<math>\scriptstyle\begin{cases}\scriptstyle x+x^2=n^2\\\scriptstyle x-x^2=m^2\end{cases}</math> |
| + | |style="text-align:right;"|יא <big>ואם</big> יאמרו לך מרובע המחזיק שרש אם תוסיף עליו שרשו יחזיק שרש ואם תגרע המרובע משרשו יהיה לנשאר שרש{{#annotend:Fofb}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :This is an indeterminate problem. |
|style="text-align:right;"|זאת השאלה חרשת | |style="text-align:right;"|זאת השאלה חרשת | ||
|- | |- | ||
| | | | ||
| − | : | + | :Solve it according to the following procedure: |
|style="text-align:right;"|ותעשה אותה כמעשה | |style="text-align:right;"|ותעשה אותה כמעשה | ||
|- | |- | ||
| | | | ||
| − | : | + | :Look for a number that has a root [<math>\scriptstyle{\color{blue}{a^2}}</math>], such that when you add another number to it, it has a root [<math>\scriptstyle{\color{OliveGreen}{a^2+b=n^2}}</math>]; and when you subtract the former number that has a root from the other number added, the remainder has a root [<math>\scriptstyle{\color{OliveGreen}{b-a^2=m^2}}</math>] |
| − | |||
|style="text-align:right;"|שתבקש מספר שיחזיק שרש ואם תקבץ עמו מספר אחר יחזיק שרש ואם תגרע המספר הראשון המחזיק שרש מהמספר האחר הנוסף יחזיק הנשאר שרש | |style="text-align:right;"|שתבקש מספר שיחזיק שרש ואם תקבץ עמו מספר אחר יחזיק שרש ואם תגרע המספר הראשון המחזיק שרש מהמספר האחר הנוסף יחזיק הנשאר שרש | ||
|- | |- | ||
| − | | | + | |When you find this number, divide it by the added number and the quotient is the root of the square. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{x=\frac{a^2}{b}}}</math> | |
|style="text-align:right;"|וכאשר תמצא זה המספר תחלקהו על המספר הנוסף והעולה לחלק יהיה שרש המרובע | |style="text-align:right;"|וכאשר תמצא זה המספר תחלקהו על המספר הנוסף והעולה לחלק יהיה שרש המרובע | ||
|- | |- | ||
| | | | ||
| − | :: | + | :The number that has a root is four and the added number is five. |
| − | |style="text-align:right;"|והמספר המחזיק שרש ארבעה | + | :<math>\scriptstyle{\color{blue}{a^2=4\quad b=5}}</math> |
| − | והמספר הנוסף חמשה | + | |style="text-align:right;"|והמספר המחזיק שרש ארבעה והמספר הנוסף חמשה |
|- | |- | ||
| | | | ||
| − | : | + | :When you add five to four, it is nine, which has a root and its root is three. |
| + | :<math>\scriptstyle{\color{blue}{4+5=9=3^2}}</math> | ||
|style="text-align:right;"|וכאשר תוסיף על ארבעה חמשה יהיה תשעה ומחזיק שרש ושרשו שלשה | |style="text-align:right;"|וכאשר תוסיף על ארבעה חמשה יהיה תשעה ומחזיק שרש ושרשו שלשה | ||
|- | |- | ||
| | | | ||
| − | : | + | :When you subtract four from five, one remains, which has a root and it is one. |
| + | :<math>\scriptstyle{\color{blue}{5-4=1=1^2}}</math> | ||
|style="text-align:right;"|וכאשר תגרע הארבעה מהחמשה ישאר אחד ומחזיק שרש והוא אחד | |style="text-align:right;"|וכאשר תגרע הארבעה מהחמשה ישאר אחד ומחזיק שרש והוא אחד | ||
|- | |- | ||
| | | | ||
| − | : | + | :When you want to know the value of the square, divide four by five; the quotient is four-fifths and it is the root of the square. |
| + | :<math>\scriptstyle{\color{blue}{x=\frac{4}{5}}}</math> | ||
|style="text-align:right;"|וכאשר תרצה לדעת שעור המרובע תחלק ארבעה על חמשה ויעלה לחלק ארבעה חומשים והוא שרש המרובע | |style="text-align:right;"|וכאשר תרצה לדעת שעור המרובע תחלק ארבעה על חמשה ויעלה לחלק ארבעה חומשים והוא שרש המרובע | ||
|- | |- | ||
| | | | ||
| − | : | + | :The square is three-fifths and a fifth of a fifth. |
| + | :<math>\scriptstyle{\color{blue}{x^2=\frac{3}{5}+\left(\frac{1}{5}\sdot\frac{1}{5}\right)}}</math> | ||
|style="text-align:right;"|והמרובע יהיה שלשה חומשים וחמישית מחמישית | |style="text-align:right;"|והמרובע יהיה שלשה חומשים וחמישית מחמישית | ||
|- | |- | ||
| − | | | + | |I explained to you the procedure of extracting these numbers. |
| − | |||
|style="text-align:right;"|ובארתי לך הנה המלאכה להוציא אלו המספרים | |style="text-align:right;"|ובארתי לך הנה המלאכה להוציא אלו המספרים | ||
|- | |- | ||
| − | | | + | |I have already explained in what preceded up to this point that if we suppose a square that has a root [<math>\scriptstyle{\color{OliveGreen}{x^2}}</math>] and if you subtract it from its two roots and one dirham, the remainder is two roots and one dirham minus a square. |
| − | : | + | :<math>\scriptstyle{\color{OliveGreen}{n^2=2x+1-x^2}}</math> |
| − | |style="text-align:right;"|וכבר בארתי במה שעבר עד הנה | + | |style="text-align:right;"|וכבר בארתי במה שעבר עד הנה שאם נניח מרובע שיחזיק שרש ואם תגרע<sup>ה</sup>ו משני שרשיו ואדרהם אחד ישאר שני שרשים ואדרהם אחד פחות מרובע |
|- | |- | ||
| | | | ||
| − | : | + | :Then, we suppose its root is a thing minus one dirham. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{n=\sqrt{2x+1-x^2}=x-1}}</math> |
| + | |style="text-align:right;"|<sup>אחר זה</sup> נניח שרשו דבר פחות דרהם אחד | ||
|- | |- | ||
| | | | ||
| − | : | + | :I subtract the dirham from the thing, because the product has one dirham and when you confront them, what remains is squares equal roots. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ושניתי האדרהם בדבר בעבור שיעלה בהכאה דרהם אחד וכאשר תכוין עמו ישאר מרובע ישוה דבר |
|- | |- | ||
| | | | ||
| − | : | + | :Then, multiply a thing minus one dirham by itself; it is a square and one dirham minus two things equal two things and one dirham minus a square. |
| − | |style="text-align:right;"|אחר זה | + | :<math>\scriptstyle{\color{blue}{\left(x-1\right)^2=x^2+1-2x=2x+1-x^2}}</math> |
| + | |style="text-align:right;"|אחר זה תכה דבר ‫<ref>68r</ref>פחות דרהם אחד בעצמו ויהיה מרובע ואדרהם אחד פחות שני דברים ישוה שני דברים ואדרהם אחד פחות מרובע | ||
|- | |- | ||
| | | | ||
| − | ::: | + | :*<span style="color:Green>Confrontation:</span> |
| − | |||
| − | |||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Confront them; the square equals two things. |
| − | |style="text-align:right;"|ויהיה מרובע | + | :<math>\scriptstyle{\color{blue}{x^2=2x}}</math> |
| + | |style="text-align:right;"|ותכוין עמו ויהיה מרובע ישוה שני דברי‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :The thing is two. |
| − | + | :<math>\scriptstyle{\color{blue}{x=2}}</math> | |
| − | |||
| − | |||
| − | |||
|style="text-align:right;"|והדבר שנים | |style="text-align:right;"|והדבר שנים | ||
|- | |- | ||
| | | | ||
| − | : | + | :The square is four; and this is the number that has a root. |
| + | :<math>\scriptstyle{\color{blue}{x^2=4}}</math> | ||
|style="text-align:right;"|והמרובע ארבעה והוא המספר המחזיק שרש | |style="text-align:right;"|והמרובע ארבעה והוא המספר המחזיק שרש | ||
|- | |- | ||
| | | | ||
| − | : | + | :The other number is four and one dirham, which is five. |
| + | :<math>\scriptstyle{\color{blue}{2x+1=4+1=5}}</math> | ||
|style="text-align:right;"|והמספר האחר הוא ארבעה ואדרהם אחד והוא חמשה | |style="text-align:right;"|והמספר האחר הוא ארבעה ואדרהם אחד והוא חמשה | ||
|- | |- | ||
| | | | ||
| − | : | + | :If we suppose the root of the square is half a thing plus one dirham. |
| + | :<math>\scriptstyle{\color{blue}{n=\sqrt{2x+1-x^2}=\frac{1}{2}x+1}}</math> | ||
|style="text-align:right;"|ואם נניח שרש המרובע חצי דבר ואדרהם אחד | |style="text-align:right;"|ואם נניח שרש המרובע חצי דבר ואדרהם אחד | ||
|- | |- | ||
| | | | ||
| − | ::: | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it by itself, then confront [the product] with the product of [the root of] two things and one dirham minus a square [by itself]. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}x+1\right)^2=\left(\sqrt{2x+1-x^2}\right)^2}}</math> | ||
|style="text-align:right;"|ותכהו בעצמו ותכונהו עם העולה מהכאת שני דברי' ודרהם אחד פחות מרובע | |style="text-align:right;"|ותכהו בעצמו ותכונהו עם העולה מהכאת שני דברי' ודרהם אחד פחות מרובע | ||
|- | |- | ||
| | | | ||
| − | : | + | :The result is the number that has a root, which is sixteen [parts of 25]. |
| + | :<math>\scriptstyle{\color{blue}{x^2=\frac{16}{{\color{red}{25}}}}}</math> | ||
|style="text-align:right;"|יעלה המספר המחזיק שרש ששה עשר | |style="text-align:right;"|יעלה המספר המחזיק שרש ששה עשר | ||
|- | |- | ||
| | | | ||
| − | + | :[?] | |
|style="text-align:right;"|והמספר האחר חמשה ושני דברים ויהיה שרש המרובע ששה עשר חלקים מס"ה חלקים באחד | |style="text-align:right;"|והמספר האחר חמשה ושני דברים ויהיה שרש המרובע ששה עשר חלקים מס"ה חלקים באחד | ||
|- | |- | ||
| − | | | + | |Whichever of this species that you have, apply the procedure on it in whatever method you choose. |
|style="text-align:right;"|וכל מה שיפול בידך מזה האופן תעשהו במעשה הזה ותקח הדרך אשר תבחר | |style="text-align:right;"|וכל מה שיפול בידך מזה האופן תעשהו במעשה הזה ותקח הדרך אשר תבחר | ||
|- | |- | ||
| − | | | + | |Now I will explain to you this question by understanding another way: |
|style="text-align:right;"|ועתה אבאר לך זאת השאלה בהבנת דרך אחרת | |style="text-align:right;"|ועתה אבאר לך זאת השאלה בהבנת דרך אחרת | ||
|- | |- | ||
| − | |Know that for every number that is divided into two parts, each of which has a root <math>\scriptstyle a^2+b^2=n</math>, and into two other parts that have no root: | + | |Know that for every number that is divided into two parts, each of which has a root [<math>\scriptstyle{\color{OliveGreen}{a^2+b^2=n}}</math>], and into two other parts that have no root: |
|style="text-align:right;"|<big>דע</big> כי כל מספר שיחלק לשני חלקים באופן שיהיה לכל חלק שרש ולשני חלקים אחרים שלא יהיה להם אטימו | |style="text-align:right;"|<big>דע</big> כי כל מספר שיחלק לשני חלקים באופן שיהיה לכל חלק שרש ולשני חלקים אחרים שלא יהיה להם אטימו | ||
|- | |- | ||
| − | | | + | |I will explain it to you, so that you will understand it: |
|style="text-align:right;"|ועתה אבאר לך זה למען תבינהו | |style="text-align:right;"|ועתה אבאר לך זה למען תבינהו | ||
|- | |- | ||
| | | | ||
| − | :12) <math>\scriptstyle a^2+b^2=5</math> | + | :{{#annot:x²+y²=n²|1124|Onda}}12) If you are told: divide five dirham into two parts, so that each part has a root. |
| − | |style="text-align:right;"|יב <big>אם</big> יאמרו לך חמשה דרהמי תחלקם לשני חלקים ולכל חלק שרש | + | :<math>\scriptstyle a^2+b^2=5</math> |
| + | |style="text-align:right;"|יב <big>אם</big> יאמרו לך חמשה דרהמי תחלקם לשני חלקים ולכל חלק שרש{{#annotend:Onda}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :You already know that one of the parts is one and the other is four. |
| + | :<math>\scriptstyle{\color{blue}{a_1^2=1\quad b_1^2=4}}</math> | ||
|style="text-align:right;"|וכבר ידעת שאחד החלקים הוא אחד והאחר ארבעה | |style="text-align:right;"|וכבר ידעת שאחד החלקים הוא אחד והאחר ארבעה | ||
|- | |- | ||
| | | | ||
| − | : | + | :When you want to divide it into two other parts, so that each has a root: |
|style="text-align:right;"|וכאשר תרצה לחלקם לשני חלקים אחרים בלעדי אלו החלקים באופן שיהיה לכל חלק שרש | |style="text-align:right;"|וכאשר תרצה לחלקם לשני חלקים אחרים בלעדי אלו החלקים באופן שיהיה לכל חלק שרש | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle a_2^2=\left(a_1+x\right)^2</math> | + | :Take the root of the first part, add a thing to it, and multiply it by itself; the result is the first part. |
| + | :<math>\scriptstyle{\color{OliveGreen}{a_2^2=\left(a_1+x\right)^2}}</math> | ||
|style="text-align:right;"|תקח שרש החלק האחד ותוסיף עליו דבר ותכהו בעצמו והיוצא יהיה החלק האחד | |style="text-align:right;"|תקח שרש החלק האחד ותוסיף עליו דבר ותכהו בעצמו והיוצא יהיה החלק האחד | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle b_2^2=5-a_2</math> | + | :Subtract it from five and the other part remains. |
| + | :<math>\scriptstyle{\color{OliveGreen}{b_2^2=5-a_2}}</math> | ||
|style="text-align:right;"|ותגרעהו מחמשה וישאר החלק האחר | |style="text-align:right;"|ותגרעהו מחמשה וישאר החלק האחר | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{a_1^2=1}}</math> | + | :We take the first part, which is one. |
| + | :<math>\scriptstyle{\color{blue}{a_1^2=1}}</math> | ||
|style="text-align:right;"|ולקחנו החלק האחד והוא אחד | |style="text-align:right;"|ולקחנו החלק האחד והוא אחד | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{a_1=1}}</math> | + | :Its root is one. |
| + | :<math>\scriptstyle{\color{blue}{a_1=1}}</math> | ||
|style="text-align:right;"|ושרשו אחד | |style="text-align:right;"|ושרשו אחד | ||
|- | |- | ||
| | | | ||
| − | : | + | :We add a thing to it; it is one and a thing. |
| − | |style="text-align:right;"|הוספנו עליו דבר ויהיה אחד ודבר אחד | + | |style="text-align:right;"|הוספנו עליו דבר ויהיה אחד ודבר אחד |
| − | |||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{b_2^2=5-\left(x^2+1+2x\right)=4-x^2-2x}}</math> | + | :We multiply it by itself; it is a square, one dirham and two things; and this is the first part of the two required parts. |
| + | :<math>\scriptstyle{\color{blue}{a_2^2=\left(1+x\right)^2=x^2+1+2x}}</math> | ||
| + | |style="text-align:right;"|הכינו אותו בעצמו והיה מרובע ודרהם אחד ושני דברים והוא החלק האחד משני החלקים המבוקשים | ||
| + | |- | ||
| + | | | ||
| + | :Subtract it from five; the remainder is four minus a square and minus two things; this is the other part and it should have a root. | ||
| + | :<math>\scriptstyle{\color{blue}{b_2^2=5-\left(x^2+1+2x\right)=4-x^2-2x}}</math> | ||
|style="text-align:right;"|ותגרעהו מן החמשה וישאר ארבעה פחות מרובע ופחות שני דברים והוא החלק האחר וצריך שיחזיק שרש | |style="text-align:right;"|ותגרעהו מן החמשה וישאר ארבעה פחות מרובע ופחות שני דברים והוא החלק האחר וצריך שיחזיק שרש | ||
|- | |- | ||
| | | | ||
| − | : | + | :We already know that its root should be less than two dirham. |
|style="text-align:right;"|וכבר ידענו ששרשו פחות משני דרהמי | |style="text-align:right;"|וכבר ידענו ששרשו פחות משני דרהמי | ||
|- | |- | ||
| | | | ||
| − | : | + | :We suppose it is two things minus [one and a third of a dirham]. |
| + | :<math>\scriptstyle b_2=\sqrt{4-x^2-2x}=2-\left(1+\frac{{\color{red}{1}}}{3}\right)x</math> | ||
|style="text-align:right;"|ונניחיהו שני דברי' פחות דבר ושני שלישי | |style="text-align:right;"|ונניחיהו שני דברי' פחות דבר ושני שלישי | ||
|- | |- | ||
| | | | ||
| − | : | + | :We multiply them by themselves; it is four dirham, a square and seven-ninths of a square minus five things and a third of a thing equal four dirham minus a square and minus two things. |
| − | |style="text-align:right;"|והכינום בעצמם | + | |style="text-align:right;"|והכינום בעצמם ויהיה ארבעה דרהמי ואלגו <s>ומאה</s> <sup>ושבעה</sup> תשיעיות מאלגו פחות חמשה דברים ושליש דבר ישוה ארבעה דרהמי פחות אלגו ופחות שני דברים |
|- | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\left[2-\left(1+\frac{1}{3}\right)x\right]^2=4+\left(1+\frac{7}{9}\right)x^2-\left(5+\frac{1}{3}\right)x=4-x^2-2x}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :*<span style="color:Green>Confrontation:</span> | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Confront them as you know; the thing is one dirham and one-fifth. |
| + | :<math>\scriptstyle{\color{blue}{x=1+\frac{1}{5}}}</math> | ||
|style="text-align:right;"|ותכוין עמו כאשר ידעת ויהיה הדבר דרהם אחד וחמישית אחד | |style="text-align:right;"|ותכוין עמו כאשר ידעת ויהיה הדבר דרהם אחד וחמישית אחד | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{a_2=x+1=2+\frac{1}{5}}}</math> | + | :We assumed the root of the first part of the two is a thing and one dirham, so the root of the first part is two dirham and a fifth. |
| − | |style="text-align:right;"|והנחנו שרש החלק האחד מהשנים דבר ודרהם אחד ויהיה שרש החלק האחד שני דרהמי וחמישית | + | :<math>\scriptstyle{\color{blue}{a_2=x+1=2+\frac{1}{5}}}</math> |
| + | |style="text-align:right;"|‫<ref>68v</ref>והנחנו שרש החלק האחד מהשנים דבר ודרהם אחד ויהיה שרש החלק האחד שני דרהמי וחמישית | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{a_2^2=\left(2+\frac{1}{5}\right)^2=4+\frac{4}{5}+\left(\frac{1}{5}\sdot\frac{1}{5}\right)}}</math> | + | :Multiply it by itself; it is four dirham, four-fifths, and a fifth of a fifth; this is the first part you are looking for. |
| − | + | |style="text-align:right;"|ותכהו בעצמו ויהיה ארבעה דרהמי <s>ורביע</s> וארבעה חומשים וחמישית החמישית והוא החלק האחד אשר בקשת | |
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{a_2^2=\left(2+\frac{1}{5}\right)^2=4+\frac{4}{5}+\left(\frac{1}{5}\sdot\frac{1}{5}\right)}}</math> | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{b_2^2=5-\left[4+\frac{4}{5}+\left(\frac{1}{5}\sdot\frac{1}{5}\right)\right]=\frac{4}{5}\sdot\frac{1}{5}}}</math> | + | :The other is what remains from five, which is four-fifths of a fifth. |
| + | :<math>\scriptstyle{\color{blue}{b_2^2=5-\left[4+\frac{4}{5}+\left(\frac{1}{5}\sdot\frac{1}{5}\right)\right]=\frac{4}{5}\sdot\frac{1}{5}}}</math> | ||
|style="text-align:right;"|והאחר הוא הנשאר מהחמשה והוא ארבעה חומשי החומש | |style="text-align:right;"|והאחר הוא הנשאר מהחמשה והוא ארבעה חומשי החומש | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you want to divide it into two other parts: |
|style="text-align:right;"|ואם תרצה לחלק אותו לשנים חלקים אחרים זולת אלו החלקים | |style="text-align:right;"|ואם תרצה לחלק אותו לשנים חלקים אחרים זולת אלו החלקים | ||
|- | |- | ||
| | | | ||
| − | : | + | :Suppose the smaller part, which is a root of four dirham minus a square and minus two things, is two dirham minus [one thing and a half]. |
| + | :<math>\scriptstyle b_2=\sqrt{4-x^2-2x}=2-\left({\color{red}{1+\frac{1}{2}}}\right)x</math> | ||
|style="text-align:right;"|תניח החלק הקטן שרש מארבעה דרהמי פחות אלגו ופחות שני דברים ושנים דרהמי פחות שני דברים | |style="text-align:right;"|תניח החלק הקטן שרש מארבעה דרהמי פחות אלגו ופחות שני דברים ושנים דרהמי פחות שני דברים | ||
|- | |- | ||
| | | | ||
| − | ::: | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Multiply them by themselves, then confront [the product] with the product of [the root of] four dirham minus a square minus two things [by itself]. | ||
| + | :<math>\scriptstyle{\color{blue}{\left[2-\left(1+\frac{1}{2}\right)x\right]^2=\left(\sqrt{4-x^2-2x}\right)^2}}</math> | ||
|style="text-align:right;"|ותכם בעצמם ותכונהו עם המתקבץ מהכאת ארבעה דרהמי פחות אלגו ופחות שני דברים | |style="text-align:right;"|ותכם בעצמם ותכונהו עם המתקבץ מהכאת ארבעה דרהמי פחות אלגו ופחות שני דברים | ||
|- | |- | ||
| | | | ||
| − | : | + | :Do as I told you. |
|style="text-align:right;"|ותעשה כאשר אמרתי לך | |style="text-align:right;"|ותעשה כאשר אמרתי לך | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{a_2=x+1=2+\frac{3}{13}}}</math> | + | :The root of one part is two dirham and three parts of thirteen. |
| + | :<math>\scriptstyle{\color{blue}{a_2=x+1=2+\frac{3}{13}}}</math> | ||
|style="text-align:right;"|ויהיה שרש החלק האחד שנים דרהמי ושלשה חלקים משלשה עשר | |style="text-align:right;"|ויהיה שרש החלק האחד שנים דרהמי ושלשה חלקים משלשה עשר | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{b_2=\frac{2}{13}}}</math> | + | :The root of the other part is two parts of 13. |
| + | :<math>\scriptstyle{\color{blue}{b_2=\frac{2}{13}}}</math> | ||
|style="text-align:right;"|ושרש החלק האחר שנים חלקים מי"ג | |style="text-align:right;"|ושרש החלק האחר שנים חלקים מי"ג | ||
|- | |- | ||
| | | | ||
| − | : | + | :Multiplying each of the two by itself: |
|style="text-align:right;"|ותכה כל אחד משניהם בעצמו | |style="text-align:right;"|ותכה כל אחד משניהם בעצמו | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{a_2^2=4+\frac{12}{13}+\frac{9}{169}}}</math> | + | :One part is four dirham, 12 parts of 13, and nine parts of 169. |
| + | :<math>\scriptstyle{\color{blue}{a_2^2=4+\frac{12}{13}+\frac{9}{169}}}</math> | ||
|style="text-align:right;"|ויהיה החלק האחד ארבעה דרהמי וי"ב חלקים מי"ג חלקים באחד ותשעה חלקים מקס"ט | |style="text-align:right;"|ויהיה החלק האחד ארבעה דרהמי וי"ב חלקים מי"ג חלקים באחד ותשעה חלקים מקס"ט | ||
|- | |- | ||
| − | | | + | |Do the same for all cases of this type that you solve in this procedure, and it will have as many parts as you wish. |
|style="text-align:right;"|וכן תעשה לכל אשר יבוא לך מזה המין תעשה עמו כמעשה הזה ויחזיק מהחלקים כמה שתרצה | |style="text-align:right;"|וכן תעשה לכל אשר יבוא לך מזה המין תעשה עמו כמעשה הזה ויחזיק מהחלקים כמה שתרצה | ||
|- | |- | ||
| − | | | + | |Always take the root of one of the two parts, add a thing to it and multiply it by itself [<math>\scriptstyle{\color{OliveGreen}{a_2^2=\left(a+x\right)^2}}</math>]; the result is one of the two parts you sought for [<math>\scriptstyle{\color{OliveGreen}{n=a_2^2+b_2^2}}</math>]. |
| − | |||
| − | |||
| − | |||
|style="text-align:right;"|והוא שלעולם תקח שרש האחד מהשני חלקים ותוסיף עליו דבר ותכה זה בעצמו והיוצא הוא האחד מהשני חלקים אשר בקשת | |style="text-align:right;"|והוא שלעולם תקח שרש האחד מהשני חלקים ותוסיף עליו דבר ותכה זה בעצמו והיוצא הוא האחד מהשני חלקים אשר בקשת | ||
|- | |- | ||
| − | | | + | |Subtract it from the number you want to divide into two parts, each of which has a root; the remainder is the other part [<math>\scriptstyle{\color{OliveGreen}{b_2^2=n-a_2^2}}</math>] and this is a number that has a root, from which a square and a root is subtracted [<math>\scriptstyle{\color{OliveGreen}{b_2^2=b^2-2ax-x^2}}</math>]. |
| − | + | |style="text-align:right;"|ותגרעהו מהמספר אשר תרצה לחלק לשני חלקים אשר לכל חלק מהם שרש והנשאר הוא החלק האחר והוא מספר שיחזיק שרש הנשנה לו אלגו ודבר | |
| − | |||
| − | |style="text-align:right;"|ותגרעהו מהמספר אשר תרצה לחלק לשני חלקים אשר לכל חלק מהם שרש והנשאר הוא החלק האחר | ||
| − | והוא מספר שיחזיק שרש הנשנה לו אלגו ודבר | ||
|- | |- | ||
| − | | | + | |Take the root of the number and subtract from it as many things as you want [<math>\scriptstyle{\color{OliveGreen}{b_2=\sqrt{b^2-2ax-x^2}=b-kx}}</math>], since they are not the same, when you multiply them by themselves [<math>\scriptstyle{\color{OliveGreen}{b^2-2ax-x^2=\left(b-kx\right)^2}}</math>]. |
| − | + | |style="text-align:right;"|ותקח שרש המספר ותשנה לו אשר תרצה מהדברים <sup>אחר</sup> אשר לא יכוונו כאשר תכם בעצמם | |
| − | |||
| − | |style="text-align:right;"|ותקח שרש המספר ותשנה לו אשר תרצה מהדברים אחר אשר לא יכוונו כאשר תכם בעצמם | ||
|- | |- | ||
| − | | | + | |Confront it with the part that has a root, which is subtracted from the square and the root; you receive parts endlessly. |
| − | :<math>\scriptstyle{\color{ | + | :<math>\scriptstyle{\color{OliveGreen}{\left(k^2+1\right)x^2=2\left(bk-a\right)x}}</math> |
|style="text-align:right;"|ותכוין עמו החלק שהוא מספר המחזיק שרש נשנה מהאלגו ודבר ויעלו לך מן החלקים עד אין להם תכלית | |style="text-align:right;"|ותכוין עמו החלק שהוא מספר המחזיק שרש נשנה מהאלגו ודבר ויעלו לך מן החלקים עד אין להם תכלית | ||
|- | |- | ||
| | | | ||
| − | :13) If you are told: divide ten into two parts. | + | :{{#annot:a+b=10,20+a=n²,50-b=m²|619|XtSS}}13) If you are told: divide ten into two parts. |
:Add the one part to twenty and the sum has a root. | :Add the one part to twenty and the sum has a root. | ||
:Subtract the other part from fifty and the remainder has a root. | :Subtract the other part from fifty and the remainder has a root. | ||
:<math>\scriptstyle\begin{cases}\scriptstyle a+b=10\\\scriptstyle20+a=n^2\\\scriptstyle50-b=m^2\end{cases}</math> | :<math>\scriptstyle\begin{cases}\scriptstyle a+b=10\\\scriptstyle20+a=n^2\\\scriptstyle50-b=m^2\end{cases}</math> | ||
| − | |style="text-align:right;"|יג <big>ואם</big> יאמרו לך עשרה תחלקהו לשני חלקים ותוסיף החלק האחד על עשרים ויהיה מה שיחזיק שרש ותגרע החלק האחר מחמשים ויחזיק הנשאר שרש | + | |style="text-align:right;"|יג <big>ואם</big> יאמרו לך עשרה תחלקהו לשני חלקים ותוסיף החלק האחד על עשרים ויהיה מה שיחזיק שרש ותגרע החלק האחר מחמשים ויחזיק הנשאר שרש{{#annotend:XtSS}} |
|- | |- | ||
| | | | ||
| − | : | + | :This is an indeterminate problem. |
|style="text-align:right;"|זאת השאלה חרשת | |style="text-align:right;"|זאת השאלה חרשת | ||
|- | |- | ||
| | | | ||
| − | : | + | :Its procedure is that we assume the first part is a square minus twenty [<math>\scriptstyle{\color{blue}{a=x^2-20}}</math>]. |
| − | |||
|style="text-align:right;"|ומעשהו שנניח החלק האחד אלגו פחות עשרים | |style="text-align:right;"|ומעשהו שנניח החלק האחד אלגו פחות עשרים | ||
|- | |- | ||
| | | | ||
| − | : | + | :So that when you add it to twenty, it has a root; it is a square. |
| + | :<math>\scriptstyle{\color{blue}{a+20=x^2}}</math> | ||
|style="text-align:right;"|באופן שכאשר תוסיפיהו על עשרים יחזיק שרש והוא אלגו | |style="text-align:right;"|באופן שכאשר תוסיפיהו על עשרים יחזיק שרש והוא אלגו | ||
|- | |- | ||
| | | | ||
| − | : | + | :Its root is a thing. |
| + | :<math>\scriptstyle{\color{blue}{\sqrt{a+20}=\sqrt{x^2}=x}}</math> | ||
|style="text-align:right;"|ושרשו דבר | |style="text-align:right;"|ושרשו דבר | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{b=10-a=30-x^2}}</math> | + | :The other part remaining from ten is thirty minus a square. |
| + | :<math>\scriptstyle{\color{blue}{b=10-a=30-x^2}}</math> | ||
|style="text-align:right;"|והחלק האחר יהיה הנשאר מהעשרה והוא שלשים פחות אלגו | |style="text-align:right;"|והחלק האחר יהיה הנשאר מהעשרה והוא שלשים פחות אלגו | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{50-b=50-\left(30-x^2\right)=x^2+20}}</math> | + | :Subtract it from fifty; a square and twenty dirham remain and this should have a root. |
| − | |style="text-align:right;"|ותגרעם מן החמשים וישאר אלגו ועשרים דרהמי וצריך שיחזיק שרש | + | :<math>\scriptstyle{\color{blue}{50-b=50-\left(30-x^2\right)=x^2+20}}</math> |
| + | |style="text-align:right;"|ותגרעם מן <s>השלשי</s> החמשים וישאר אלגו ועשרים דרהמי וצריך שיחזיק שרש | ||
|- | |- | ||
| | | | ||
| − | : | + | :When you want to find it, suppose the square is greater than twenty and less than thirty [<math>\scriptstyle{\color{blue}{20<x^2<30}}</math>], for this number has many [solutions]. |
| − | |style="text-align:right;"|וכאשר תבקש לדעתו תניח האלגו יותר מעשרים ופחות משלשים כי זה המספר לו אופנים רבים | + | |style="text-align:right;"|‫<ref>69r</ref>וכאשר תבקש לדעתו תניח האלגו <s>פחות</s> <sup>יותר</sup> מעשרים ופחות משלשים כי זה המספר לו אופנים רבים |
|- | |- | ||
| | | | ||
| − | : | + | :One solution is that you suppose the root of the square is five and a sixth. |
| + | :<math>\scriptstyle{\color{blue}{x=5+\frac{1}{6}}}</math> | ||
|style="text-align:right;"|והאופן האחד שתניח שרש האלגו חמשה ושתות | |style="text-align:right;"|והאופן האחד שתניח שרש האלגו חמשה ושתות | ||
|- | |- | ||
| | | | ||
| + | :The square is 26, two-thirds, and a quarter of a ninth. | ||
:<math>\scriptstyle{\color{blue}{x^2=26+\frac{2}{3}+\left(\frac{1}{4}\sdot\frac{1}{9}\right)}}</math> | :<math>\scriptstyle{\color{blue}{x^2=26+\frac{2}{3}+\left(\frac{1}{4}\sdot\frac{1}{9}\right)}}</math> | ||
|style="text-align:right;"|והאלגו יהיה כ"ו ושני שלישים ורביע תשיעית | |style="text-align:right;"|והאלגו יהיה כ"ו ושני שלישים ורביע תשיעית | ||
|- | |- | ||
| | | | ||
| + | :Subtract twenty dirham from it; the remainder is six, two-thirds, and a quarter of a ninth, and this is one of the two parts. | ||
|style="text-align:right;"|ותגרע ממנו עשרים דרהמי וישאר ששה ושני שלישים ורביע תשיעית והוא אחד מהשני חלקים | |style="text-align:right;"|ותגרע ממנו עשרים דרהמי וישאר ששה ושני שלישים ורביע תשיעית והוא אחד מהשני חלקים | ||
|- | |- | ||
| Line 1,290: | Line 1,393: | ||
|- | |- | ||
| | | | ||
| + | :When you add it to twenty, it has a root and its root is five and a sixth. | ||
:<math>\scriptstyle{\color{blue}{a+20=\left(5+\frac{1}{6}\right)^2}}</math> | :<math>\scriptstyle{\color{blue}{a+20=\left(5+\frac{1}{6}\right)^2}}</math> | ||
|style="text-align:right;"|וכאשר תוסיפהו על עשרים יחזיק שרש ושרשו חמשה ושתות | |style="text-align:right;"|וכאשר תוסיפהו על עשרים יחזיק שרש ושרשו חמשה ושתות | ||
|- | |- | ||
| | | | ||
| + | :The other part is what remains from ten, which is three dirham, a quarter and half a ninth. | ||
:<math>\scriptstyle{\color{blue}{b=10-a=10-\left[6+\frac{2}{3}+\left(\frac{1}{4}\sdot\frac{1}{9}\right)\right]=3+\frac{1}{4}+\left(\frac{1}{2}\sdot\frac{1}{9}\right)}}</math> | :<math>\scriptstyle{\color{blue}{b=10-a=10-\left[6+\frac{2}{3}+\left(\frac{1}{4}\sdot\frac{1}{9}\right)\right]=3+\frac{1}{4}+\left(\frac{1}{2}\sdot\frac{1}{9}\right)}}</math> | ||
|style="text-align:right;"|והחלק האחר יהיה מה שישאר מהעשרה והוא שלשה דרהמי ורביע וחצי תשיעית | |style="text-align:right;"|והחלק האחר יהיה מה שישאר מהעשרה והוא שלשה דרהמי ורביע וחצי תשיעית | ||
|- | |- | ||
| | | | ||
| + | :When you subtract it from fifty, the remainder is 46, two-thirds and a quarter of a ninth; this has a root and its root is six dirham, a half and a third. | ||
:<math>\scriptstyle{\color{blue}{50-b=46+\frac{2}{3}+\left(\frac{1}{4}\sdot\frac{1}{9}\right)=\left(6+\frac{1}{2}+\frac{1}{3}\right)^2}}</math> | :<math>\scriptstyle{\color{blue}{50-b=46+\frac{2}{3}+\left(\frac{1}{4}\sdot\frac{1}{9}\right)=\left(6+\frac{1}{2}+\frac{1}{3}\right)^2}}</math> | ||
|style="text-align:right;"|וכאשר תגרעם מחמשים ישאר מ"ו ושני שלישים ורביע תשיעית ומחזיק שרש ושרשו הוא ששה דרהמי וחצי ושליש | |style="text-align:right;"|וכאשר תגרעם מחמשים ישאר מ"ו ושני שלישים ורביע תשיעית ומחזיק שרש ושרשו הוא ששה דרהמי וחצי ושליש | ||
|- | |- | ||
| | | | ||
| − | : | + | :When we want to know how we assume the root of the square is five and a sixth: |
| + | :<math>\scriptstyle{\color{blue}{x=5+\frac{1}{6}}}</math> | ||
|style="text-align:right;"|וכאשר נרצה לדעת איך נניח שרש המרובע חמשה ושתות | |style="text-align:right;"|וכאשר נרצה לדעת איך נניח שרש המרובע חמשה ושתות | ||
|- | |- | ||
| | | | ||
| − | : | + | :Suppose the root of a square and twenty dirham is one dirham and two-thirds plus one thing. |
| + | :<math>\scriptstyle{\color{blue}{\sqrt{x^2+20}=\left(1+\frac{2}{3}\right)+x}}</math> | ||
|style="text-align:right;"|תניח שרש מאלגו ועשרים דרהמי דרהם אחד ושני שלישי ודבר אחד | |style="text-align:right;"|תניח שרש מאלגו ועשרים דרהמי דרהם אחד ושני שלישי ודבר אחד | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{\left[\left(1+\frac{2}{3}\right)+x\right]^2=\left(\ | + | :Multiply them by themselves; it is two dirham and seven-ninths, plus a square and three things and a third of a thing equal a square and twenty dirham. |
| − | + | |style="text-align:right;"|ותכם בעצמם ויהיה שני דרהמי ושבעה תשיעיות ואלגו ושלשה דברים ושלישית דבר ישוה אלגו ועשרים דרהמי | |
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\left[\left(1+\frac{2}{3}\right)+x\right]^2=\left(2+\frac{7}{9}\right)+x^2+\left(3+\frac{1}{3}\right)x=x^2+20}}</math> | ||
|- | |- | ||
| | | | ||
| − | ::: | + | :*<span style="color:Green>Confrontation:</span> |
| − | | | + | | |
|- | |- | ||
| | | | ||
| − | : | + | :Confront them; the thing is five and a sixth. |
| + | :<math>\scriptstyle{\color{blue}{x=5+\frac{1}{6}}}</math> | ||
|style="text-align:right;"|ותכוין עמו ויהיה הדבר חמשה ושתות | |style="text-align:right;"|ותכוין עמו ויהיה הדבר חמשה ושתות | ||
|- | |- | ||
| − | |If | + | |If you want a solution other than this, do as I told you before. |
|style="text-align:right;"|ואם תרצה אופן אחר זולת זה תעשה כאשר אמרתי לך קודם זה | |style="text-align:right;"|ואם תרצה אופן אחר זולת זה תעשה כאשר אמרתי לך קודם זה | ||
|- | |- | ||
| | | | ||
| − | :14) <math>\scriptstyle\begin{cases}\scriptstyle a+b=10\\\scriptstyle20+a=n^2\\\scriptstyle50+b=m^2\end{cases}</math> | + | :{{#annot:a+b=10, 20+a=n²,50+b=m²|619|OLcA}}14) If you are told: divide ten into two parts, add the first part to twenty and it has a root; add the other part to fifty, and it has a root. |
| − | |style="text-align:right;"|יד <big>ואם</big> יאמרו לך תחלק עשרה לשני חלקים ותוסיף החלק האחד על עשרים ויחזיק שרש ותוסיף החלק האחר על חמשים ויחזיק שרש | + | :<math>\scriptstyle\begin{cases}\scriptstyle a+b=10\\\scriptstyle20+a=n^2\\\scriptstyle50+b=m^2\end{cases}</math> |
| + | |style="text-align:right;"|יד <big>ואם</big> יאמרו לך תחלק עשרה לשני חלקים ותוסיף החלק האחד על עשרים ויחזיק שרש ותוסיף החלק האחר על חמשים ויחזיק שרש{{#annotend:OLcA}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :This is an indeterminate problem. |
|style="text-align:right;"|זאת השאלה חרשת | |style="text-align:right;"|זאת השאלה חרשת | ||
|- | |- | ||
| | | | ||
| − | : | + | :Its procedure is that we assume the first part is a square minus twenty [<math>\scriptstyle{\color{blue}{a=x^2-20}}</math>]. |
| − | |||
|style="text-align:right;"|ומלאכתו שנניח החלק האחד אלגו פחות עשרים דרהמי | |style="text-align:right;"|ומלאכתו שנניח החלק האחד אלגו פחות עשרים דרהמי | ||
|- | |- | ||
| | | | ||
| − | : | + | :So that when you add it to twenty, it has a root. |
| − | |style="text-align:right;"|באופן שכאשר | + | :<math>\scriptstyle{\color{blue}{a+20=x^2}}</math> |
| + | |style="text-align:right;"|באופן שכאשר תוסיפ<s>ם</s><sup>הו</sup> על עשרים יחזיק שרש | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{b=10-a=30-x^2}}</math> | + | :The other part that remains from ten is thirty minus a square. |
| + | :<math>\scriptstyle{\color{blue}{b=10-a=30-x^2}}</math> | ||
|style="text-align:right;"|והחלק האחר הנשאר מן העשרה יהיה שלשים פחות אלגו | |style="text-align:right;"|והחלק האחר הנשאר מן העשרה יהיה שלשים פחות אלגו | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{50+b=50+\left(30-x^2\right)=80-x^2}}</math> | + | :When you add it to fifty, it is eighty dirham minus a square, which should have a root. |
| + | :<math>\scriptstyle{\color{blue}{50+b=50+\left(30-x^2\right)=80-x^2}}</math> | ||
|style="text-align:right;"|וכשתוסיפם על החמשים יהיו שמנים דרהמי פחות אלגו וצריך שיחזיק שרש | |style="text-align:right;"|וכשתוסיפם על החמשים יהיו שמנים דרהמי פחות אלגו וצריך שיחזיק שרש | ||
|- | |- | ||
| | | | ||
| − | : | + | :As if it is said: divide eighty into two parts, each of which has a root. |
| − | + | :<math>\scriptstyle A^2+B^2=80</math> | |
|style="text-align:right;"|כאלו אמר תחלק שמנים לשני חלקים ולכל חלק שרש | |style="text-align:right;"|כאלו אמר תחלק שמנים לשני חלקים ולכל חלק שרש | ||
|- | |- | ||
| − | | | + | |When you have problems of this species, solve them by this procedure, so that you get a number that you divide into two parts, each of which has a root, provided that the given number can be divided. |
|style="text-align:right;"|וכאשר יפלו לפניך שאלות מזה המין תעשה כמעשה הזה עד שיעלה לך מספר שתחלקהו לשני חלקים ויחזיק כל חלק שרש אם הניח מספר שיוכל להתחלק | |style="text-align:right;"|וכאשר יפלו לפניך שאלות מזה המין תעשה כמעשה הזה עד שיעלה לך מספר שתחלקהו לשני חלקים ויחזיק כל חלק שרש אם הניח מספר שיוכל להתחלק | ||
|- | |- | ||
| − | | | + | |Otherwise the purpose cannot be achieved. |
|style="text-align:right;"|ואם לאו השאלה היא לא יתכן שתגיע אל תכלית | |style="text-align:right;"|ואם לאו השאלה היא לא יתכן שתגיע אל תכלית | ||
|- | |- | ||
| | | | ||
| − | : | + | :Divide eighty into two parts, each of which has a root. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{A^2+B^2=80}}</math> | |
| − | |style="text-align:right;"|ותחלק השמנים לשני חלקים ולכל חלק שרש | + | |style="text-align:right;"|ותחלק השמנים לשני חלקים ולכל חלק שרש |
| − | |||
| − | |||
|- | |- | ||
| − | |This problem has | + | | |
| + | :Suppose one of the parts is greater than twenty and less than thirty; and the other part is greater than fifty and less than sixty. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{20<A^2<30\quad50<B^2<60}}</math> | ||
| + | |style="text-align:right;"|ותניח אחד החלקים יותר מעשרי' ופחות משלשים והחלק האחר יותר מחמשים ופחות מששים | ||
| + | |- | ||
| + | |This problem has countless solutions. | ||
|style="text-align:right;"|ולזה הדרך אופנים רבים עד אין מספר | |style="text-align:right;"|ולזה הדרך אופנים רבים עד אין מספר | ||
|- | |- | ||
| − | |The procedure | + | |The procedure of extracting any solution you want is as I told you before. |
| − | |style="text-align:right;"|והמלאכה להוציא איזה אופן שתרצה מהם היא כפי מה שאמרתי לך קודם | + | |style="text-align:right;"|והמלאכה להוציא איזה אופן שתרצה מהם ‫<ref>69v</ref>היא כפי מה שאמרתי לך קודם |
|- | |- | ||
| | | | ||
| − | : | + | :One of the solutions is that you assume the first part is 26 dirham and 30 parts of 289. |
| − | |style="text-align:right;"|ואחד מהאופנים שתשים החלק האחד כ"ו דרהמי ול' חלקים | + | :<math>\scriptstyle{\color{blue}{A^2=26+\frac{30}{289}}}</math> |
| + | |style="text-align:right;"|ואחד מהאופנים שתשים החלק האחד כ"ו דרהמי ול' חלקים מ<sup>ר</sup>פ"ט | ||
|- | |- | ||
| | | | ||
| − | : | + | :The other part remaining from eighty is 53 and 259 parts of 289. |
| + | :<math>\scriptstyle{\color{blue}{B^2=80-\left(26+\frac{30}{289}\right)=53+\frac{259}{289}}}</math> | ||
|style="text-align:right;"|והחלק האחר הנשאר משמנים והוא נ"ג ורנ"ט חלקים מרפ"ט חלקים באחד | |style="text-align:right;"|והחלק האחר הנשאר משמנים והוא נ"ג ורנ"ט חלקים מרפ"ט חלקים באחד | ||
|- | |- | ||
| − | |When you | + | | |
| + | :When you want to know the measure of each part of ten: | ||
|style="text-align:right;"|וכאשר תרצה לדעת שעור כל חלק מן העשרה | |style="text-align:right;"|וכאשר תרצה לדעת שעור כל חלק מן העשרה | ||
|- | |- | ||
| | | | ||
| − | : | + | :Subtract twenty from the first part of eighty; the remainder is six dirham and [two hundred] and thirty parts of 289, and this is the first part of ten. |
| + | :<math>\scriptstyle{\color{blue}{a=A^2-20=\left(26+\frac{{\color{red}{2}}30}{289}\right)-20=6+\frac{{\color{red}{2}}30}{289}}}</math> | ||
|style="text-align:right;"|תגרע מהחלק ראשון מהשמנים עשרים וישאר ששה דרהמי ושלשים חלקים מרפ"ט והוא החלק ראשון מהעשרה | |style="text-align:right;"|תגרע מהחלק ראשון מהשמנים עשרים וישאר ששה דרהמי ושלשים חלקים מרפ"ט והוא החלק ראשון מהעשרה | ||
|- | |- | ||
| | | | ||
| − | : | + | :The other part is what remains from ten, which is three dirham and 259 parts of 289. |
| + | :<math>\scriptstyle{\color{blue}{b=10-a=10-\left(6+\frac{{\color{red}{2}}30}{289}\right)=3+\frac{{\color{red}{59}}}{289}}}</math> | ||
|style="text-align:right;"|והחלק האחר הוא הנשאר מעשרה והוא שלשה דרהמי ורנ"ט חלקים מרפ"ט חלקים באחד | |style="text-align:right;"|והחלק האחר הוא הנשאר מעשרה והוא שלשה דרהמי ורנ"ט חלקים מרפ"ט חלקים באחד | ||
|- | |- | ||
| | | | ||
| − | : | + | :When we add the greater of the two parts of ten to twenty, it is twenty-six and [two hundred and] thirty parts of 289 and it has a root, which is five dirham and three parts of seventeen. |
| + | :<math>\scriptstyle{\color{blue}{a+20=A^2=26+\frac{{\color{red}{2}}30}{289}=\left(5+\frac{3}{17}\right)^2}}</math> | ||
|style="text-align:right;"|וכאשר נוסיף החלק הגדול משני חלקי העשרה על עשרים יהיה עשרים ושש ושלשים חלקים מרפ"ט ומחזיק שרש והוא חמשה דרהמי ושלשה חלקים משבעה עשר | |style="text-align:right;"|וכאשר נוסיף החלק הגדול משני חלקי העשרה על עשרים יהיה עשרים ושש ושלשים חלקים מרפ"ט ומחזיק שרש והוא חמשה דרהמי ושלשה חלקים משבעה עשר | ||
|- | |- | ||
| | | | ||
| − | : | + | :When we add the smaller part of ten to fifty, it is fifty-three dirham and [59] parts of 289 and it has a root, which is seven dirham and five parts of seventeen. |
| − | |style="text-align:right;"|וכאשר נוסיף החלק | + | :<math>\scriptstyle{\color{blue}{b+50=B^2=53+\frac{{\color{red}{59}}}{289}=\left(7+\frac{5}{17}\right)^2}}</math> |
| + | |style="text-align:right;"|וכאשר נוסיף החלק הקטון מן העשרה על החמשים יהיה חמשים ושלשה דרהמי ורנ"ט חלקים מרפ"ט ומחזיק שרש והוא שבעה דרהמי וחמשה חלקים משבעה עשר | ||
|- | |- | ||
| | | | ||
| − | :15) <math>\scriptstyle\begin{cases}\scriptstyle3-x^2=n^2\\\scriptstyle2+x^2=m^2\end{cases}</math> | + | :{{#annot:3-x²=n²,2+x²=m²|1124|6Zd3}}15) If you are told: a square, if you subtract it from three dirham, the remainder has a root; and if you add it to two dirham, the sum has a root. |
| − | |style="text-align:right;"|טו <big>ואם</big> יאמרו לך מרובע אם תגרעהו משלשה דרהמי יחזיק הנשאר שרש ואם תוסיפהו על שני דרהמי יהיה למתקבץ שרש | + | :<math>\scriptstyle\begin{cases}\scriptstyle3-x^2=n^2\\\scriptstyle2+x^2=m^2\end{cases}</math> |
| + | |style="text-align:right;"|טו <big>ואם</big> יאמרו לך מרובע אם תגרעהו משלשה דרהמי יחזיק הנשאר שרש ואם תוסיפהו על שני דרהמי יהיה למתקבץ שרש{{#annotend:6Zd3}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :This is an indeterminate problem. |
|style="text-align:right;"|זאת השאלה חרשת | |style="text-align:right;"|זאת השאלה חרשת | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{3+2=5}}</math> | + | :Its procedure is that you sum two with three; they are five. |
| + | :<math>\scriptstyle{\color{blue}{3+2=5}}</math> | ||
|style="text-align:right;"|ומעשהו שתקבץ השנים עם השלשה ויהיו חמשה | |style="text-align:right;"|ומעשהו שתקבץ השנים עם השלשה ויהיו חמשה | ||
|- | |- | ||
| | | | ||
| − | : | + | :Divide them into two parts, each of which has a root. |
| + | :<math>\scriptstyle{\color{blue}{5=n^2+m^2}}</math> | ||
|style="text-align:right;"|תחלקם לשני חלקים יחזיק כל חלק שרש | |style="text-align:right;"|תחלקם לשני חלקים יחזיק כל חלק שרש | ||
|- | |- | ||
| | | | ||
| − | : | + | :Suppose the first part is greater than two and less than three. |
| + | :<math>\scriptstyle{\color{blue}{2<n^2<3}}</math> | ||
|style="text-align:right;"|ותניח החלק האחד יותר משנים ופחות משלשה | |style="text-align:right;"|ותניח החלק האחד יותר משנים ופחות משלשה | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x^2=m^2-2}}</math> | + | :Subtract two from the first part; what remains is a square. |
| + | :<math>\scriptstyle{\color{blue}{x^2=m^2-2}}</math> | ||
|style="text-align:right;"|ותגרע השנים מהחלק האחד ומה שישאר יהיה המרובע | |style="text-align:right;"|ותגרע השנים מהחלק האחד ומה שישאר יהיה המרובע | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you want, suppose the square is twenty-three parts of 169. |
| + | :<math>\scriptstyle{\color{blue}{x^2=\frac{23}{169}}}</math> | ||
|style="text-align:right;"|ואם תרצה תניח האלגו עשרים ושלשה חלקים מקס"ט חלקים באחד | |style="text-align:right;"|ואם תרצה תניח האלגו עשרים ושלשה חלקים מקס"ט חלקים באחד | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you want, suppose it is 146 [parts] of 169. |
| + | :<math>\scriptstyle{\color{blue}{x^2=\frac{146}{169}}}</math> | ||
|style="text-align:right;"|ואם תרצה תניחהו קמ"ו מקס"ט | |style="text-align:right;"|ואם תרצה תניחהו קמ"ו מקס"ט | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you want, suppose it is 431 parts of 625. |
| + | :<math>\scriptstyle{\color{blue}{x^2=\frac{431}{625}}}</math> | ||
|style="text-align:right;"|ואם תרצה תניחהו תל"א חלקים מתרכ"ה חלקים באחד | |style="text-align:right;"|ואם תרצה תניחהו תל"א חלקים מתרכ"ה חלקים באחד | ||
|- | |- | ||
| − | | | + | |You can solve this problem and similar ones, according to the methods you choose, as I explained to you before. |
| − | |||
|style="text-align:right;"|והנה תוכל לעשות זאת השאלה והדומים אליה כפי האופנים אשר תבחר כאשר בארתי לך לפנים | |style="text-align:right;"|והנה תוכל לעשות זאת השאלה והדומים אליה כפי האופנים אשר תבחר כאשר בארתי לך לפנים | ||
|- | |- | ||
| | | | ||
| − | :16) <math>\scriptstyle\begin{cases}\scriptstyle10-x^2=n^2\\\scriptstyle20-x^2=m^2\end{cases}</math> | + | :{{#annot:10-x²=n²,20-x²=m²|1124|EaVV}}16) If you are told: a square, if you subtract it from ten dirham, the remainder has a root; and if you subtract it from twenty, the remainder has a root. |
| − | |style="text-align:right;"|יו <big>ואם</big> יאמרו לך אלגו אם תגרעהו מעשרה דרהמי יחזיק הנשאר שרש ואם תגרעהו מעשרים יחזיק הנשאר שרש | + | :<math>\scriptstyle\begin{cases}\scriptstyle10-x^2=n^2\\\scriptstyle20-x^2=m^2\end{cases}</math> |
| + | |style="text-align:right;"|יו <big>ואם</big> יאמרו לך אלגו אם תגרעהו מעשרה דרהמי יחזיק הנשאר שרש ואם תגרעהו מעשרים יחזיק הנשאר שרש{{#annotend:EaVV}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :This is an indeterminate problem. |
|style="text-align:right;"|זאת השאלה חרשת | |style="text-align:right;"|זאת השאלה חרשת | ||
|- | |- | ||
| | | | ||
| − | : | + | :Its procedure is that you assume your square is ten dirham minus a square. |
| + | :<math>\scriptstyle{\color{blue}{n^2=10-x^2}}</math> | ||
|style="text-align:right;"|ומעשהו שתניח האלגו שלך עשרה דרהמי פחות אלגו | |style="text-align:right;"|ומעשהו שתניח האלגו שלך עשרה דרהמי פחות אלגו | ||
|- | |- | ||
| | | | ||
| − | : | + | :Or, if you want, twenty dirham minus a square. |
| + | :<math>\scriptstyle{\color{blue}{n^2=20-x^2}}</math> | ||
|style="text-align:right;"|ואם תרצה עשרים דרהמי פחות אלגו | |style="text-align:right;"|ואם תרצה עשרים דרהמי פחות אלגו | ||
|- | |- | ||
| | | | ||
| − | : | + | :Suppose it is so, in order that when you subtract it from one of them, a square remains and it has a root, which is a thing. |
|style="text-align:right;"|ותניחהו כן בעבור כי כאשר תגרעהו מאחד מהם ישאר אלגו ומחזיק שרש והוא דבר | |style="text-align:right;"|ותניחהו כן בעבור כי כאשר תגרעהו מאחד מהם ישאר אלגו ומחזיק שרש והוא דבר | ||
|- | |- | ||
| | | | ||
| − | : | + | :We suppose it is ten dirham minus a square. |
| + | :<math>\scriptstyle{\color{blue}{n^2=10-x^2}}</math> | ||
|style="text-align:right;"|ונניחהו עשרה דרהמי פחות אלגו | |style="text-align:right;"|ונניחהו עשרה דרהמי פחות אלגו | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{10-n^2=x^2}}</math> | + | :When you subtract it from ten, a square remains. |
| − | |style="text-align:right;"|וכאשר תגרעהו מן עשרה ישאר אלגו | + | :<math>\scriptstyle{\color{blue}{10-n^2=x^2}}</math> |
| + | |style="text-align:right;"|וכאשר תגרעהו מן עשרה ישאר ‫<ref>70r</ref>אלגו | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{20-n^2=x^2+10}}</math> | + | :If you subtract it from twenty, a square and ten dirham remain, which should have a root. |
| + | :<math>\scriptstyle{\color{blue}{20-n^2=x^2+10}}</math> | ||
|style="text-align:right;"|ואם תגרעהו מעשרים ישאר אלגו ועשרה דרהמי וצריך שיחזיק שרש | |style="text-align:right;"|ואם תגרעהו מעשרים ישאר אלגו ועשרה דרהמי וצריך שיחזיק שרש | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\sqrt{x^2+10}>x}}</math> | + | :You already know that its root is greater than a thing. |
| + | :<math>\scriptstyle{\color{blue}{\sqrt{x^2+10}>x}}</math> | ||
|style="text-align:right;"|וכבר ידעת ששרשו יותר מדבר | |style="text-align:right;"|וכבר ידעת ששרשו יותר מדבר | ||
|- | |- | ||
| | | | ||
| − | : | + | :We suppose its root is a thing plus two dirham. |
| − | : | + | :<math>\scriptstyle{\color{blue}{\sqrt{x^2+10}=x+2}}</math> |
|style="text-align:right;"|ונניח שרשו דבר ושני דרהמי | |style="text-align:right;"|ונניח שרשו דבר ושני דרהמי | ||
|- | |- | ||
| | | | ||
| − | : | + | :Or, a thing plus three |
| + | :<math>\scriptstyle{\color{blue}{\sqrt{x^2+10}=x+3}}</math> | ||
|style="text-align:right;"|או דבר ושלשה | |style="text-align:right;"|או דבר ושלשה | ||
|- | |- | ||
| | | | ||
| − | : | + | :Or, whichever numbers you wish, as I explained you before. |
| + | :<math>\scriptstyle{\color{blue}{\sqrt{x^2+10}=x+a}}</math> | ||
|style="text-align:right;"|או אשר תרצה מהמספרים כאשר בארתי לך לפנים | |style="text-align:right;"|או אשר תרצה מהמספרים כאשר בארתי לך לפנים | ||
|- | |- | ||
| | | | ||
| − | :: | + | :So that when we multiply it by itself and confront it with a square and ten dirham, the square is less than ten dirham. |
| − | |style="text-align:right;"|אחר שיהיה כאשר הכינו אותו בעצמו ונכוין עמהם אלגו ועשרה דרהמי | + | :<math>\scriptstyle{\color{blue}{\left(x+a\right)^2=x^2+10\longrightarrow x^2<10}}</math> |
| + | |style="text-align:right;"|אחר שיהיה כאשר הכינו אותו בעצמו ונכוין עמהם אלגו ועשרה דרהמי יהיה האלגו פחות מעשרה דרהמי | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x^2 | + | :Suppose it is a thing plus two dirham. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\sqrt{x^2+10}=x+2}}</math> |
| + | |style="text-align:right;"|ותניחהו דבר ושני דרהמי | ||
|- | |- | ||
| | | | ||
| − | : | + | :Multiply it by itself; it is a square and four dirham plus four things equal a square and ten dirham. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\left(x+2\right)^2=x^2+4+4x=x^2+10}}</math> |
| + | |style="text-align:right;"|ותכהו בעצמו יהיה אלגו וארבעה דרהמי וארבעה דברים ישוה אלגו ועשרה דרהמי | ||
|- | |- | ||
| | | | ||
| − | ::: | + | :*<span style="color:Green>Confrontation:</span> |
| − | |||
| − | |||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Confront them; the root of the square is one dirham and a half. |
| + | :<math>\scriptstyle{\color{blue}{x=1+\frac{1}{2}}}</math> | ||
|style="text-align:right;"|ותכוין עמו ויהיה שרש האלגו אדרהם אחד וחצי | |style="text-align:right;"|ותכוין עמו ויהיה שרש האלגו אדרהם אחד וחצי | ||
|- | |- | ||
| | | | ||
| − | : | + | :The square is two dirham and a quarter. |
| + | :<math>\scriptstyle{\color{blue}{x^2=2+\frac{1}{4}}}</math> | ||
|style="text-align:right;"|והאלגו שנים דרהמי ורביע | |style="text-align:right;"|והאלגו שנים דרהמי ורביע | ||
|- | |- | ||
| | | | ||
| − | : | + | :We assumed the square is ten dirham minus a square. |
| + | :<math>\scriptstyle{\color{blue}{n^2=10-x^2}}</math> | ||
|style="text-align:right;"|והנחנו האלגו עשרה דרהמי פחות אלגו | |style="text-align:right;"|והנחנו האלגו עשרה דרהמי פחות אלגו | ||
|- | |- | ||
| | | | ||
| − | : | + | :So, the required square is seven dirham, a half and a quarter. |
| + | :<math>\scriptstyle{\color{blue}{n^2=7+\frac{1}{2}+\frac{1}{4}}}</math> | ||
|style="text-align:right;"|ויהיה האלגו המבוקש שבעה דרהמי וחצי ורביע | |style="text-align:right;"|ויהיה האלגו המבוקש שבעה דרהמי וחצי ורביע | ||
|- | |- | ||
| | | | ||
| − | : | + | :If we subtract it from ten, two and a quarter remains and its root is one and a half. |
| + | :<math>\scriptstyle{\color{blue}{x^2=10-n^2=2+\frac{1}{4}}}</math> | ||
|style="text-align:right;"|ואם נגרעהו מעשרה ישאר שנים ורביע ושרשו אחד וחצי | |style="text-align:right;"|ואם נגרעהו מעשרה ישאר שנים ורביע ושרשו אחד וחצי | ||
|- | |- | ||
| | | | ||
| − | :: | + | :If we assume the root of the square and ten dirham is a thing and three dirham: |
| + | :<math>\scriptstyle{\color{blue}{\sqrt{x^2+10}=x+3}}</math> | ||
|style="text-align:right;"|ואם הנחנו שרש מאלגו מעשרה דרהמי דבר ושלשה דרהמי | |style="text-align:right;"|ואם הנחנו שרש מאלגו מעשרה דרהמי דבר ושלשה דרהמי | ||
|- | |- | ||
| | | | ||
| − | : | + | :The square is nine dirham, a half, a third, and five-sixths of a sixth. |
| + | :<math>\scriptstyle{\color{blue}{n^2=9+\frac{1}{2}+\frac{1}{3}+\left(\frac{5}{6}\sdot\frac{1}{6}\right)}}</math> | ||
|style="text-align:right;"|יהיה האלגו תשעה דרהמי וחצי ושליש וחמשה ששיות הששית | |style="text-align:right;"|יהיה האלגו תשעה דרהמי וחצי ושליש וחמשה ששיות הששית | ||
|- | |- | ||
| − | |The solutions | + | |The solutions you get in this problem and in its similar are more than any number. |
|style="text-align:right;"|והאופנים הם שתניח בזאת השאלה ובדומים אליה יותר מאשר הוא המספר | |style="text-align:right;"|והאופנים הם שתניח בזאת השאלה ובדומים אליה יותר מאשר הוא המספר | ||
|- | |- | ||
| | | | ||
| − | :17) <math>\scriptstyle\begin{cases}\scriptstyle20+x^2=n^2\\\scriptstyle30+x^2=m^2\end{cases}</math> | + | :{{#annot:20+x²=n²,30+x²=m²|1124|czE5}}17) If you are told: a square, if you add it to twenty, it has a root; and if you add it to thirty, it has a root. |
| − | |style="text-align:right;"|יז <big>ואם</big> יאמרו לך אלגו אם תוסיפהו על עשרים יחזיק שרש ואם תוסיפיהו על שלשים יחזיק שרש | + | :<math>\scriptstyle\begin{cases}\scriptstyle20+x^2=n^2\\\scriptstyle30+x^2=m^2\end{cases}</math> |
| + | |style="text-align:right;"|יז <big>ואם</big> יאמרו לך אלגו אם תוסיפהו על עשרים יחזיק שרש ואם תוסיפיהו על שלשים יחזיק שרש{{#annotend:czE5}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :This is an indeterminate problem. |
|style="text-align:right;"|זאת השאלה חרשת | |style="text-align:right;"|זאת השאלה חרשת | ||
|- | |- | ||
| | | | ||
| − | : | + | :Its procedure is that you assume the square is a square minus twenty dirham. |
| − | : | + | :<math>\scriptstyle{\color{blue}{a^2=x^2-20}}</math> |
|style="text-align:right;"|ומעשהו שנניח האלגו אלגו פחות עשרים דרהמי | |style="text-align:right;"|ומעשהו שנניח האלגו אלגו פחות עשרים דרהמי | ||
|- | |- | ||
| | | | ||
| − | : | + | :Or, if you want, a square minus thirty. |
| + | :<math>\scriptstyle{\color{blue}{a^2=x^2-30}}</math> | ||
|style="text-align:right;"|או אם תרצה אלגו פחות שלשים | |style="text-align:right;"|או אם תרצה אלגו פחות שלשים | ||
|- | |- | ||
| | | | ||
| − | : | + | :We suppose it is a square minus twenty dirham. |
| + | :<math>\scriptstyle{\color{blue}{a^2=x^2-20}}</math> | ||
|style="text-align:right;"|ונניחהו אלגו פחות עשרים דרהמי | |style="text-align:right;"|ונניחהו אלגו פחות עשרים דרהמי | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{a^2+20=x^2}}</math> | + | :When it is added to twenty, the result is a square. |
| − | |style="text-align:right;"|ובתוספתו על עשרים יעלה אלגו | + | :<math>\scriptstyle{\color{blue}{a^2+20=x^2}}</math> |
| + | |style="text-align:right;"|ובתוספתו על עשרים <s>יחזיק</s> יעלה אלגו | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\sqrt{a^2+20}=x}}</math> | + | :Its root is a thing. |
| + | :<math>\scriptstyle{\color{blue}{\sqrt{a^2+20}=x}}</math> | ||
|style="text-align:right;"|ושרשו דבר | |style="text-align:right;"|ושרשו דבר | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{a^2+{\color{red}{30}}=x^2+10}}</math> | + | :We add it to [thirty]; it is a square and ten dirham and this should have a root. |
| + | :<math>\scriptstyle{\color{blue}{a^2+{\color{red}{30}}=x^2+10}}</math> | ||
|style="text-align:right;"|ונוסיפהו על שנים ויהיה אלגו ועשרה דרהמי וצריך שיחזיק שרש | |style="text-align:right;"|ונוסיפהו על שנים ויהיה אלגו ועשרה דרהמי וצריך שיחזיק שרש | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x^2>20}}</math> | + | :The square is greater than twenty. |
| + | :<math>\scriptstyle{\color{blue}{x^2>20}}</math> | ||
|style="text-align:right;"|ויהיה האלגו יותר מעשרים | |style="text-align:right;"|ויהיה האלגו יותר מעשרים | ||
|- | |- | ||
| | | | ||
| − | : | + | :Suppose its root is a thing plus one dirham: |
| − | |style="text-align:right;"|ותניח שרשו דבר ואדרהם אחד' | + | :<math>\scriptstyle{\color{blue}{\sqrt{x^2+10}=x+1}}</math> |
| + | |style="text-align:right;"|ותניח שרשו דבר ואדרהם אחד‫' | ||
|- | |- | ||
| | | | ||
| − | ::: | + | :*<span style="color:Green>Confrontation:</span> |
| − | | | + | | |
|- | |- | ||
| | | | ||
| − | : | + | :Multiply them by themselves, then confront [the product] with the product of [the root of] a square and ten dirham [by itself]. |
| − | |style="text-align:right;"|ותכונהו עם המתקבץ מהכאת אלגו ועשרה דרהמי | + | :<math>\scriptstyle{\color{blue}{\left(x+1\right)^2=\left(\sqrt{x^2+10}\right)^2}}</math> |
| + | |style="text-align:right;"|ותכם בעצמם ותכונהו עם המתקבץ מהכאת אלגו ועשרה דרהמי | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{a^2=x^2-20=\frac{1}{4}}}</math> | + | :The square is twenty dirham and a quarter. |
| + | :<math>\scriptstyle{\color{blue}{x^2=20+\frac{1}{4}}}</math> | ||
| + | |style="text-align:right;"|ויהיה המרובע עשרים דרהמי ורביע | ||
| + | |- | ||
| + | | | ||
| + | :Subtract twenty dirham from it; one quarter remains and it is the required square. | ||
| + | :<math>\scriptstyle{\color{blue}{a^2=x^2-20=\frac{1}{4}}}</math> | ||
|style="text-align:right;"|ותגרע ממנו עשרי' דרהמי וישאר רביע אחד והוא המרובע המבוקש | |style="text-align:right;"|ותגרע ממנו עשרי' דרהמי וישאר רביע אחד והוא המרובע המבוקש | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you add it to twenty, it has a root. |
| + | :<math>\scriptstyle{\color{blue}{a^2+20}}</math> has a root | ||
|style="text-align:right;"|ואם תוסיפהו על עשרים יחזיק שרש | |style="text-align:right;"|ואם תוסיפהו על עשרים יחזיק שרש | ||
|- | |- | ||
| | | | ||
| − | ::: | + | :If you want, say: the square is 75 dirham and half an eighth. |
| + | :<math>\scriptstyle{\color{blue}{a^2=75+\left(\frac{1}{2}\sdot\frac{1}{8}\right)}}</math> | ||
|style="text-align:right;"|ואם תרצה תאמר האלגו ע"ה דרהמי וחצי שמינית | |style="text-align:right;"|ואם תרצה תאמר האלגו ע"ה דרהמי וחצי שמינית | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you add it to twenty, it has a root, and its root is nine dirham, a half and a quarter. |
| + | :<math>\scriptstyle{\color{blue}{a^2+20=\left(9+\frac{1}{2}+\frac{1}{4}\right)^2}}</math> | ||
|style="text-align:right;"|אם תוסיפהו על עשרים יחזיק שרש ושרשו תשעה דרהמי וחצי ורביע | |style="text-align:right;"|אם תוסיפהו על עשרים יחזיק שרש ושרשו תשעה דרהמי וחצי ורביע | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you add it to thirty, it has a root, which is ten dirham and a quarter. |
| + | :<math>\scriptstyle{\color{blue}{a^2+30=\left(10+\frac{1}{4}\right)^2}}</math> | ||
|style="text-align:right;"|ואם תוסיפהו על שלשים יחזיק שרש והוא עשרה דרהמי ורביע | |style="text-align:right;"|ואם תוסיפהו על שלשים יחזיק שרש והוא עשרה דרהמי ורביע | ||
|- | |- | ||
| Line 1,613: | Line 1,779: | ||
|- | |- | ||
| | | | ||
| − | :18) <math>\scriptstyle\begin{cases}\scriptstyle10+x^2=n^2\\\scriptstyle10-x^2=m^2\end{cases}</math> | + | :{{#annot:10+x²=n²,10-x²=m²|1124|rWQ1}}18) If you are told: a square, if you add it to ten, it has a root; and if you subtract it from ten, it has a root. |
| − | |style="text-align:right;"|יח <big>ואם</big> יאמרו לך אלגו אם תוסיפהו על עשרה יחזיק שרש ואם תגרעהו מעשרה יחזיק שרש | + | :<math>\scriptstyle\begin{cases}\scriptstyle10+x^2=n^2\\\scriptstyle10-x^2=m^2\end{cases}</math> |
| + | |style="text-align:right;"|יח <big>ואם</big> יאמרו לך אלגו אם תוסיפהו על עשרה יחזיק שרש ואם תגרעהו ‫<ref>70v</ref>מעשרה יחזיק שרש{{#annotend:rWQ1}} | ||
|- | |- | ||
| − | | | + | |If the two numbers in this problem are the same, and we suppose one of them can be divided into two parts, each of which has a root, then [the problem] has a countless number of solutions. |
| − | |||
|style="text-align:right;"|וכאשר ישתוו השני מספרים בזאת השאלה ונניח אחד מהשני מספרים שיחלקו לשני חלקים לכל חלק שרש אז תחזיק מהאופנים מה שלא יסופרו | |style="text-align:right;"|וכאשר ישתוו השני מספרים בזאת השאלה ונניח אחד מהשני מספרים שיחלקו לשני חלקים לכל חלק שרש אז תחזיק מהאופנים מה שלא יסופרו | ||
|- | |- | ||
| − | | | + | |If it cannot be divided into two parts, each of which has a root, the problem has no solution. |
| − | |||
|style="text-align:right;"|ואם לא יתחלק לשני חלקים שיחזיק כל חלק שרש תהיה השאלה חרשת זולת שום אופן | |style="text-align:right;"|ואם לא יתחלק לשני חלקים שיחזיק כל חלק שרש תהיה השאלה חרשת זולת שום אופן | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle10=a^2+b^2</math> | + | :Divide ten into two parts, each of which has a root: |
| + | :<math>\scriptstyle10=a^2+b^2</math> | ||
|style="text-align:right;"|ותחלק העשרה לשני חלקים ולכל חלק שרש | |style="text-align:right;"|ותחלק העשרה לשני חלקים ולכל חלק שרש | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{10=1+9}}</math> | + | :It is one and nine. |
| + | :<math>\scriptstyle{\color{blue}{10=1+9}}</math> | ||
|style="text-align:right;"|והוא אחד ותשעה | |style="text-align:right;"|והוא אחד ותשעה | ||
|- | |- | ||
| | | | ||
| − | : | + | :Take the roots of one and nine; they are four. |
| − | |style="text-align:right;"|ותקח שרשי אחד ותשעה ויהיו ארבעה ותכם בעצמם ויהיה ששה עשר | + | |style="text-align:right;"|ותקח שרשי אחד ותשעה ויהיו ארבעה |
| + | |- | ||
| + | | | ||
| + | :Multiply it by itself; it is sixteen. | ||
| + | |style="text-align:right;"|ותכם בעצמם ויהיה ששה עשר | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{ | + | :Subtract the ten from it; six remains and this is the square, which when you add it to ten, it has a root; and if you subtract it from ten, it has a root. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{x^2=\left(\sqrt{1}+\sqrt{9}\right)^2-10=4^2-10=16-10=6}}</math> |
| + | |style="text-align:right;"|תגרע מהם העשרה וישאר ששה והוא <s>החלק</s> <sup>האלגו</sup> אשר כאשר תוסיפהו על העשרה יחזיק שרש ואם תגרעהו מן העשרה יחזיק שרש | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\left(\sqrt{a^2}-\sqrt{b^2}\right)^2=\left(\sqrt{9}-\sqrt{1}\right)^2=4=m^2}}</math> | + | :The reason in this question is that when you subtract the square, which is six, from ten, four remains and four is the product of the excess of [the root of] one of the two parts over the root of the other part, so there is no mistake. |
| − | |style="text-align:right;"|והארבעה הם מהכאת העודף מאחד משני החלקים על שרש החלק האחר אין שיבוש | + | :<math>\scriptstyle{\color{blue}{m^2=10-x^2=10-6=4}}</math> |
| + | :<math>\scriptstyle{\color{blue}{\left(\sqrt{a^2}-\sqrt{b^2}\right)^2=\left(\sqrt{9}-\sqrt{1}\right)^2=4=m^2}}</math> | ||
| + | |style="text-align:right;"|והמשפט בזאת השאלה הוא עולה בעבור כי כאשר תגרע האלגו שהוא ששה מעשרה ישאר ארבעה והארבעה הם מהכאת העודף מאחד משני החלקים על שרש החלק האחר אין [שיבוש]‫<ref>Ma: שיבי שוש</ref> | ||
|- | |- | ||
| | | | ||
| − | :19) <math>\scriptstyle8x+109-x^2=n^2</math> | + | :{{#annot:8x+109-x²=n²|1124|tMRx}}19) If you are told: a square, if you subtract it from its eight roots plus 109 dirham, it has a root. |
| − | |style="text-align:right;"|יט <big>ואם</big> יאמרו לך אלגו אם תגרעהו משמנה שרשים וק"ט דרהמי יחזיק שרש | + | :<math>\scriptstyle8x+109-x^2=n^2</math> |
| + | |style="text-align:right;"|יט <big>ואם</big> יאמרו לך אלגו אם תגרעהו משמנה שרשים וק"ט דרהמי יחזיק שרש{{#annotend:tMRx}} | ||
|- | |- | ||
| − | | | + | |When you have such a question, multiply half [the number of] the roots by itself and add [the product] to the dirham. |
|style="text-align:right;"|וכאשר יקרה לפניך שאלה כזאת תכה מחצית השרשים בעצמם ותוסיפהו על האדרהמי | |style="text-align:right;"|וכאשר יקרה לפניך שאלה כזאת תכה מחצית השרשים בעצמם ותוסיפהו על האדרהמי | ||
|- | |- | ||
| − | | | + | |If their sum can be divided into two parts, each of which has a root, the problem is solvable and has a countless number of solutions. |
| − | + | |style="text-align:right;"|<sup>ואם</sup> יתחלק המקובץ מהם לשני חלקים ויחזיק כל חלק שרש תהיה השאלה נשלמת ותחזיק מהאופנים הרבה אשר לא יוכלו להמנות | |
| − | |style="text-align:right;"|ואם יתחלק המקובץ מהם לשני חלקים ויחזיק כל חלק שרש תהיה השאלה נשלמת ותחזיק מהאופנים הרבה אשר לא יוכלו להמנות | + | |- |
| + | |If their sum cannot be divided into two parts, each of which has a root, the problem has no solution and no solving procedure. | ||
| + | |style="text-align:right;"|ואם לא יתחלק המתקבץ מהם לשני חלקים ולכל חלק מהם שרש תהיה השאלה חרשת בזולת שום אופן | ||
|- | |- | ||
| | | | ||
| − | + | :Multiply half [the number of] the roots by itself; it is sixteen. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ותכה מחצית השרשים בעצמם ויהיו ששה עשר |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}\sdot8\right)^2+109=16+109=125}}</math> | + | :Add it to 109; it is 125. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}\sdot8\right)^2+109=16+109=125}}</math> |
| + | |style="text-align:right;"|ותוסיפם על ק"ט ויהיה קכ"ה | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{125=a^2+b^2}}</math> | + | :Divide 125 into two parts, each of which has a root: |
| + | :<math>\scriptstyle{\color{blue}{125=a^2+b^2}}</math> | ||
|style="text-align:right;"|ותחלק קכ"ה לשני חלקים שיחזיק כל חלק שרש | |style="text-align:right;"|ותחלק קכ"ה לשני חלקים שיחזיק כל חלק שרש | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you want, suppose the first part is 25 and the other is a hundred. |
| + | :<math>\scriptstyle{\color{blue}{125=25+100}}</math> | ||
|style="text-align:right;"|ואם תרצה תניח החלק האחד כ"ה והאחר מאה | |style="text-align:right;"|ואם תרצה תניח החלק האחד כ"ה והאחר מאה | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you want, suppose the first is four and the other is 121. |
| + | :<math>\scriptstyle{\color{blue}{125=4+121}}</math> | ||
|style="text-align:right;"|ואם תרצה תניח האחד ארבעה והאחר קכ"א | |style="text-align:right;"|ואם תרצה תניח האחד ארבעה והאחר קכ"א | ||
|- | |- | ||
| − | | | + | |If you want, you divide it into two other parts, as I instructed and explained. |
|style="text-align:right;"|ואם תרצה תחלקהו לשני חלקים אחרי' כאשר אמרתי ובארתי | |style="text-align:right;"|ואם תרצה תחלקהו לשני חלקים אחרי' כאשר אמרתי ובארתי | ||
|- | |- | ||
| − | | | + | |Then, take one of the two parts you want and add it to the square. |
| − | + | :<math>\scriptstyle{\color{blue}{x^2+a^2}}</math> | |
|style="text-align:right;"|ותשוב ותקח החלק האחד מאיזה משני חלקי' שתרצה ותוסיפהו על המרובע | |style="text-align:right;"|ותשוב ותקח החלק האחד מאיזה משני חלקי' שתרצה ותוסיפהו על המרובע | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Take 25 and add it to the square; it is a square and 25. |
| + | :<math>\scriptstyle{\color{blue}{x^2+25}}</math> | ||
|style="text-align:right;"|אחר תקח כ"ה ותוסיפהו על המרובע ויהיה אלגו וכ"ה | |style="text-align:right;"|אחר תקח כ"ה ותוסיפהו על המרובע ויהיה אלגו וכ"ה | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{x^2+25=8x+109}}</math> | + | :We say: a square and 25 dirham equal eight things and 109 dirham. |
| + | :<math>\scriptstyle{\color{blue}{x^2+25=8x+109}}</math> | ||
|style="text-align:right;"|ונאמר אלגו וכ"ה דרהמי ישוו שמנה דברים וק"ט דרהמי | |style="text-align:right;"|ונאמר אלגו וכ"ה דרהמי ישוו שמנה דברים וק"ט דרהמי | ||
|- | |- | ||
| | | | ||
| − | : | + | :Subtract 25 from 109; the square remains equal to eight things and 84 dirham. |
| − | |style="text-align:right;"|ותגרע כ"ה מק"ט | + | :<math>\scriptstyle{\color{blue}{x^2+25-25=8x+109-25\longrightarrow x^2=8x+84}}</math> |
| + | |style="text-align:right;"|ותגרע כ"ה מק"ט וישאר אלגו ישוה שמנה דברים ופ"ד דרהמי | ||
|- | |- | ||
| | | | ||
| − | : | + | :Half [the number of] the things is four. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומחצית הדברים יהיה ארבעה |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Multiply it by itself; it is sixteen. |
| − | + | |style="text-align:right;"|ת[כם] בעצמם ויהיו ששה עשר | |
| − | תוסיפם על פ"ד ויהיה מאה והם החלק האחד | + | |- |
| + | | | ||
| + | :Add it to 84; it is one hundred and it is one part. | ||
| + | |style="text-align:right;"|תוסיפם על פ"ד ויהיה מאה ‫<ref>71r</ref>והם החלק האחד | ||
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| − | + | :<math>\scriptstyle{\color{blue}{b^2=\left(\frac{1}{2}\sdot8\right)^2+84=4^2+84=16+84=100}}</math> | |
|- | |- | ||
| − | |The reason | + | |The reason we extract it by this procedure: |
|style="text-align:right;"|והמשפט למה הוצאנוה בזה המעשה | |style="text-align:right;"|והמשפט למה הוצאנוה בזה המעשה | ||
|- | |- | ||
| − | | | + | |We divide it into two parts, each of which has a root, because when we add the first part to the square and confront it with the things and the dirham in our number, then we halve [the number of] the things, multiply it by itself and add [the product] to the number, or subtract the number from it, the sum, or the remainder, is the other part. |
| − | |style="text-align:right;"|וחלקנום לשני חלקים לכל חלק שרש | + | :<math>\scriptstyle{\color{OliveGreen}{x^2+a^2=bx+c\longrightarrow\left(\frac{1}{2}\sdot b\right)^2\pm c}}</math> |
| + | |style="text-align:right;"|וחלקנום לשני חלקים לכל חלק שרש בעבור כי כשהוספנו החלק האחד על האלגו והשוינו עמו הדברים והדרהמי אשר במספרנו וחצינו הדברים והכינום בעצמם והוספנוהו על המספר או גרענו המספר ממנו יהיה המתקבץ ממנו או הנשאר הוא החלק האחר | ||
|- | |- | ||
| − | |is | + | |What remains from the roots and the dirham after subtracting the square from [them] is the part added to the square. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{a^2=bx+c-x^2}}</math> | |
| − | + | |style="text-align:right;"|ויהיה מה שישאר מהשרשים והדרהמי אחר גרעון האלגו מהמספר יהיה החלק הנוסף עליו אלגו | |
| − | |||
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | :Extract the root of one hundred; it is ten. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ותשוב ותקח שרש המאה והוא עשרה |
|- | |- | ||
| | | | ||
| − | : | + | :Add half [the number of] the root to it, which is four; it is fourteen and this is the root of the square. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{x=\sqrt{100}+\left(\frac{1}{2}\sdot8\right)=10+4=14}}</math> |
| + | |style="text-align:right;"|ותוסיף עליהם מחצית השרשים והוא ארבעה ויהיו ארבעה עשר והוא שרש האלגו | ||
|- | |- | ||
| | | | ||
| − | : | + | :The square is 196. |
| + | :<math>\scriptstyle{\color{blue}{x^2=196}}</math> | ||
|style="text-align:right;"|והאלגו קצ"ו | |style="text-align:right;"|והאלגו קצ"ו | ||
|- | |- | ||
| | | | ||
| − | : | + | :Subtract it from its eight roots and 109 dirham, which is 221; 25 remains and its root is five. |
| + | :<math>\scriptstyle{\color{blue}{n^2=8x+109-x^2=221-196=25=5^2}}</math> | ||
|style="text-align:right;"|ותגרעהו משמנה שרשיו וק"ט דרהמי והם רכ"א וישאר כ"ה ושרשו חמשה | |style="text-align:right;"|ותגרעהו משמנה שרשיו וק"ט דרהמי והם רכ"א וישאר כ"ה ושרשו חמשה | ||
|- | |- | ||
| | | | ||
| − | :: | + | :If we take the first part, which is one hundred dirham, and add it to the square: |
| + | :<math>\scriptstyle{\color{blue}{x^2+100}}</math> | ||
|style="text-align:right;"|ואם לקחנו החלק האחד והוא מאה דרהמי והוספנום על האלגו | |style="text-align:right;"|ואם לקחנו החלק האחד והוא מאה דרהמי והוספנום על האלגו | ||
|- | |- | ||
| | | | ||
| − | ::: | + | :*<span style="color:Green>Confrontation:</span> |
| − | | | + | | |
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{x^2=81}}</math> | + | :We confront the sum with its eight roots and 109 dirham. |
| + | :<math>\scriptstyle{\color{blue}{x^2+100=8x+109}}</math> | ||
| + | |style="text-align:right;"|ונכוין [המתקבץ]‫<ref>Ma.: המתתקבץ</ref> עם שמנה שרשיו וק"ט דרהמי | ||
| + | |- | ||
| + | | | ||
| + | :The square is 81. | ||
| + | :<math>\scriptstyle{\color{blue}{x^2=81}}</math> | ||
|style="text-align:right;"|יהיה האלגו פ"א | |style="text-align:right;"|יהיה האלגו פ"א | ||
|- | |- | ||
| | | | ||
| − | :: | + | :If we take the other part, which is four, and add it to the square: |
| + | :<math>\scriptstyle{\color{blue}{x^2+4}}</math> | ||
|style="text-align:right;"|ואם לקחנו החלק האחר והוא ארבעה והוספנוהו על האלגו | |style="text-align:right;"|ואם לקחנו החלק האחר והוא ארבעה והוספנוהו על האלגו | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{x^2+4=8x+109}}</math> | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :We confront it with its eight roots and 109. | ||
| + | :<math>\scriptstyle{\color{blue}{x^2+4=8x+109}}</math> | ||
|style="text-align:right;"|ונכוין עמו שמנה שרשיו וק"ט | |style="text-align:right;"|ונכוין עמו שמנה שרשיו וק"ט | ||
|- | |- | ||
| | | | ||
| − | : | + | :The square is 225. |
| + | :<math>\scriptstyle{\color{blue}{x^2=225}}</math> | ||
|style="text-align:right;"|יהיה המרובע רכ"ה | |style="text-align:right;"|יהיה המרובע רכ"ה | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you want it is 36, or if you want four. |
|style="text-align:right;"|ואם תרצה ל"ו ואם תרצה ארבעה | |style="text-align:right;"|ואם תרצה ל"ו ואם תרצה ארבעה | ||
|- | |- | ||
| Line 1,767: | Line 1,969: | ||
|- | |- | ||
| | | | ||
| − | :20) <math>\scriptstyle\begin{cases}\scriptstyle8x+x^2=n^2\\\scriptstyle2x-x^2=m^2\end{cases}</math> | + | :{{#annot:8x+x²=n²,2x-x²=m²|1124|62Ll}}20) If you are told: a square, if you add its eight roots to it, it has a root; and if you subtract it from its two roots, the remainder has a root. |
| − | |style="text-align:right;"|כ <big>ואם</big> יאמרו לך אלגו אם תוסיף עליו שמנה שרשיו יחזיק שרש ואם תגרעהו משני שרשיו יהיה לנשאר שרש | + | :<math>\scriptstyle\begin{cases}\scriptstyle8x+x^2=n^2\\\scriptstyle2x-x^2=m^2\end{cases}</math> |
| + | |style="text-align:right;"|כ <big>ואם</big> יאמרו לך אלגו אם תוסיף עליו שמנה שרשיו יחזיק שרש ואם תגרעהו משני שרשיו יהיה לנשאר שרש{{#annotend:62Ll}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :This is an indeterminate problem. |
|style="text-align:right;"|זאת השאלה חרשת | |style="text-align:right;"|זאת השאלה חרשת | ||
|- | |- | ||
| | | | ||
| − | : | + | :Its knowledge is that we assume the roots added to the square are sixteen things and 64 dirham. |
| − | + | :<math>\scriptstyle{\color{blue}{16X+64}}</math> | |
| − | + | |style="text-align:right;"|וחכמתה שנניח השמנה שרשים הנוספים באלגו ששה עשר דברים וס"ד דרהמי | |
| − | |||
| − | |||
| − | |style="text-align:right;"|שנניח השמנה שרשים הנוספים באלגו ששה עשר דברים וס"ד דרהמי | ||
|- | |- | ||
| | | | ||
| − | :: | + | :So that the sum has a root and its root is a thing and eight dirham. |
| + | :<math>\scriptstyle{\color{blue}{X^2+16X+64=\left(X+8\right)^2}}</math> | ||
|style="text-align:right;"|באופן שיהיה למתקבץ שרש ושרשו דבר ושמנה דרהמי | |style="text-align:right;"|באופן שיהיה למתקבץ שרש ושרשו דבר ושמנה דרהמי | ||
|- | |- | ||
| − | |If other | + | |If we assume other than this it is possible, provided that [the sum] has a root. |
|style="text-align:right;"|ואם הנחנו אחר זולת זה יעבר אחר זה שיהיה שיחזיק שרש | |style="text-align:right;"|ואם הנחנו אחר זולת זה יעבר אחר זה שיהיה שיחזיק שרש | ||
|- | |- | ||
| | | | ||
| − | : | + | :When we assume the eight roots are 16 things and 64 dirham, [<math>\scriptstyle{\color{blue}{16X+64}}</math>], then you know that each root is two things and eight dirham [<math>\scriptstyle{\color{blue}{2X+8}}</math>] and the two roots are four things and 16 dirham [<math>\scriptstyle{\color{blue}{4X+16}}</math>] |
| − | + | |style="text-align:right;"|וכאשר הנחנו השמנה שרשים י"ו דברים וס"ד דרהמי הנה אז תדע כי כל שרש שני דברים ושמנה דרהמי והשני שרשי' ארבעה דברים וי"ו דרהמי | |
| − | |||
| − | |||
| − | |||
| − | |style="text-align:right;"|הנה אז תדע כי כל שרש שני דברים ושמנה דרהמי | ||
|- | |- | ||
| | | | ||
| − | :: | + | :It is as if it is said: a square, if you subtract it from its four roots plus sixteen, the remainder has a root. |
| − | + | :<math>\scriptstyle4X+16-X^2=N^2</math> | |
| − | |||
| − | |||
| − | |||
|style="text-align:right;"|והוא כמו שיאמר אלגו אם תגרעהו מארבעה שרשיו וששה עשר יחזיק מה שישאר שרש | |style="text-align:right;"|והוא כמו שיאמר אלגו אם תגרעהו מארבעה שרשיו וששה עשר יחזיק מה שישאר שרש | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Proceed as I instructed: |
|style="text-align:right;"|ותעשה כאשר אמרתי | |style="text-align:right;"|ותעשה כאשר אמרתי | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X^2=36}}</math> | + | :The square is thirty-six. |
| + | :<math>\scriptstyle{\color{blue}{X^2=36}}</math> | ||
|style="text-align:right;"|ויהיה האלגו שלשים ושש והוא האלגו | |style="text-align:right;"|ויהיה האלגו שלשים ושש והוא האלגו | ||
|- | |- | ||
| | | | ||
| − | ::the root is | + | :Again, say: we assume the root of the square, which is two things and eight dirham, is twenty. |
| + | :<math>\scriptstyle{\color{blue}{2X+8=20}}</math> | ||
|style="text-align:right;"|ותשוב ותאמר כבר הנחנו שרש האלגו שני דברים ושמנה דרהמי ועשרים דברים | |style="text-align:right;"|ותשוב ותאמר כבר הנחנו שרש האלגו שני דברים ושמנה דרהמי ועשרים דברים | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x=\frac{36}{20}=1+\frac{4}{5}}}</math> | + | :Divide 36 by twenty; the result is one dirham and four-fifths and this is the root of the required square. |
| + | :<math>\scriptstyle{\color{blue}{x=\frac{36}{20}=1+\frac{4}{5}}}</math> | ||
|style="text-align:right;"|ותחלק ל"ו על עשרים יעלה דרהם אחד וארבעה חומשי' והוא שרש האלגו המבוקש | |style="text-align:right;"|ותחלק ל"ו על עשרים יעלה דרהם אחד וארבעה חומשי' והוא שרש האלגו המבוקש | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x^2=3+\frac{1}{5}+\left(\frac{1}{5}\sdot\frac{1}{5}\right)}}</math> | + | :The square is three dirham, a fifth, and a fifth of a fifth. |
| − | |style="text-align:right;"|והאלגו שלשה דרהמי | + | :<math>\scriptstyle{\color{blue}{x^2=3+\frac{1}{5}+\left(\frac{1}{5}\sdot\frac{1}{5}\right)}}</math> |
| + | |style="text-align:right;"|והאלגו שלשה דרהמי [וחמ]שית וחומש החמישית | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ואם תוסיף עליהם שמנה שרשיו והוא ארבעה עשר דרהמי ושני חומשים יהיה שבעה עשר ושלשה חומשים וחומש החמישית ושרשו ארבעה וחומש | + | :If you add its eight roots to it, which is fourteen dirham and two-fifths, it is seventeen, three-fifths, and a fifth of a fifth; and its root is four and a fifth. |
| + | |style="text-align:right;"|‫<ref>71v</ref>ואם תוסיף עליהם שמנה שרשיו והוא ארבעה עשר דרהמי ושני חומשים יהיה שבעה עשר ושלשה חומשים וחומש החמישית ושרשו ארבעה וחומש | ||
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| − | + | :<math>\scriptstyle{\color{blue}{8x+x^2=\left(14+\frac{2}{5}\right)+\left[3+\frac{1}{5}+\left(\frac{1}{5}\sdot\frac{1}{5}\right)\right]=17+\frac{3}{5}+\left(\frac{1}{5}\sdot\frac{1}{5}\right)=\left(4+\frac{1}{5}\right)^2}}</math> | |
|- | |- | ||
| | | | ||
| + | :If you subtract the square, which is three dirham, a fifth, and a fifth of a fifth, from its two roots, which is three dirham and three-fifths, the remainder is a fifth and four-fifths of a fifth; and its root is three-fifths. | ||
|style="text-align:right;"|ואם תגרע מהאלגו שהוא שלשה דרהמי וחמישית וחומש החמישית משני שרשיו שהם שלשה דרהמי ושלשה חומשים ישאר חומש אחד וארבעה חומשים מחמישית ושרשו שלשה חומשים | |style="text-align:right;"|ואם תגרע מהאלגו שהוא שלשה דרהמי וחמישית וחומש החמישית משני שרשיו שהם שלשה דרהמי ושלשה חומשים ישאר חומש אחד וארבעה חומשים מחמישית ושרשו שלשה חומשים | ||
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| − | + | :<math>\scriptstyle{\color{blue}{2x-x^2=\left(3+\frac{3}{5}\right)-\left[3+\frac{1}{5}+\left(\frac{1}{5}\sdot\frac{1}{5}\right)\right]=\frac{1}{5}+\left(\frac{4}{5}\sdot\frac{1}{5}\right)=\left(\frac{3}{5}\right)^2}}</math> | |
|- | |- | ||
| | | | ||
| − | : | + | :Do the same with the other part; the square will be one dirham. |
|style="text-align:right;"|ואם תעשה זה באופן החלק האחר יהיה האלגו דרהם אחד | |style="text-align:right;"|ואם תעשה זה באופן החלק האחר יהיה האלגו דרהם אחד | ||
|- | |- | ||
| − | | | + | |You can solve this problem in many ways. |
|style="text-align:right;"|והיטב תוכל לעשות לזאת השאלה באופנים רבים | |style="text-align:right;"|והיטב תוכל לעשות לזאת השאלה באופנים רבים | ||
|- | |- | ||
| | | | ||
| − | :21) <math>\scriptstyle2x+49-x^2=n^2</math> | + | :{{#annot:2x+49-x²=n²|1124|bkYa}}21) If you are told: a square, if you subtract it from its two roots plus 49 dirham, the remainder has a root. |
| − | |style="text-align:right;"|כא <big>ואם</big> יאמרו לך אלגו אם תגרעהו משני שרשיו ומ"ט דרהמי יחזיק הנשאר שרש | + | :<math>\scriptstyle2x+49-x^2=n^2</math> |
| + | |style="text-align:right;"|כא <big>ואם</big> יאמרו לך אלגו אם תגרעהו משני שרשיו ומ"ט דרהמי יחזיק הנשאר שרש{{#annotend:bkYa}} | ||
|- | |- | ||
| | | | ||
| − | + | :If you want, do as I instructed. | |
|style="text-align:right;"|אם תרצה תעשה כאשר אמרתי | |style="text-align:right;"|אם תרצה תעשה כאשר אמרתי | ||
|- | |- | ||
| | | | ||
| − | + | :If you want, multiply the two roots by themselves and the product of the square [<math>\scriptstyle{\color{blue}{x^2}}</math>]. | |
| − | |style="text-align:right;"|ואם תרצה תכה שני השרשים בעצמם והיוצא יהיה | + | |style="text-align:right;"|ואם תרצה תכה שני השרשים בעצמם והיוצא יהיה <sup>ה</sup>אלגו |
|- | |- | ||
| | | | ||
| − | : | + | :The reason why we did so is because 49 has a root. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והסבה <s>בהנחתנו זה</s> למה עשינו כזה בעבור כי המ"ט מחזיקים שרש |
|- | |- | ||
| − | |If the number have no root | + | |If the number have no root, return to the first procedure I explained to you before this question. |
|style="text-align:right;"|ואם לא יחזיק המספר שרש תשוב למעשה הראשון שבארתי לך קודם זאת השאלה | |style="text-align:right;"|ואם לא יחזיק המספר שרש תשוב למעשה הראשון שבארתי לך קודם זאת השאלה | ||
|- | |- | ||
| Line 1,870: | Line 2,071: | ||
|- | |- | ||
| | | | ||
| − | :22) <math>\scriptstyle\begin{cases}\scriptstyle x^2+x=n^2\\\scriptstyle x^2-x=m^2\end{cases}</math> | + | :{{#annot:x²+x=n²,x²-x=m²|1124|MUv8}}22) If you are told: a square, if you add its root to it, the sum has a root; and if you subtract its root from it, the remainder has a root. |
| − | |style="text-align:right;"|כב <big>ואם</big> יאמרו לך אלגו אם תוסיף עליו שרשו יהיה למתקבץ שרש ואם תגרע ממנו שרשו יחזיק הנשאר שרש | + | :<math>\scriptstyle\begin{cases}\scriptstyle x^2+x=n^2\\\scriptstyle x^2-x=m^2\end{cases}</math> |
| + | |style="text-align:right;"|כב <big>ואם</big> יאמרו לך אלגו אם תוסיף עליו שרשו יהיה למתקבץ שרש ואם תגרע ממנו שרשו יחזיק הנשאר שרש{{#annotend:MUv8}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :This is an indeterminate problem. |
|style="text-align:right;"|זאת השאלה חרשת | |style="text-align:right;"|זאת השאלה חרשת | ||
|- | |- | ||
| | | | ||
| − | : | + | :Solve it according to the following procedure: |
|style="text-align:right;"|ותעשה אותה כמעשה | |style="text-align:right;"|ותעשה אותה כמעשה | ||
|- | |- | ||
| | | | ||
| − | : | + | :Look for a number that has a root [<math>\scriptstyle{\color{blue}{a^2}}</math>], such that when you add to it another number, it has a root; and when you subtract that number from it, [the remainder] has a root. |
| − | |||
|style="text-align:right;"|שתבקש מספר שיחזיק שרש וכאשר תקבץ עמו מספר אחר יחזיק שרש ואם תגרע ממנו אותו המספר יחזיק שרש | |style="text-align:right;"|שתבקש מספר שיחזיק שרש וכאשר תקבץ עמו מספר אחר יחזיק שרש ואם תגרע ממנו אותו המספר יחזיק שרש | ||
|- | |- | ||
| − | | | + | |colspan=2| |
| − | : | + | :<math>\scriptstyle\begin{cases}\scriptstyle a^2+b=n^2\\\scriptstyle a^2-b=m^2\end{cases}</math> |
| − | |style="text-align:right;"|וכאשר מצאת אותו המספר תחלק אותו על המספר הנוסף ומה שיעלה לחלק יהיה שרש המרובע | + | |- |
| + | |When you find this number, divide it by the added number and the quotient is the root of the square. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\frac{a^2}{b}}}</math> | ||
| + | |style="text-align:right;"|וכאשר מצאת אותו המספר [תחלק]‫<ref>Ma.: כחלק</ref> אותו על המספר הנוסף ומה שיעלה לחלק יהיה שרש המרובע | ||
|- | |- | ||
| | | | ||
| − | :: | + | :The number that has a root is 25, the number added to it is 24, and the subtracted number is 24. |
| − | |style="text-align:right;"|והמספר המחזיק שרש הוא כ"ה | + | :<math>\scriptstyle{\color{blue}{a^2=25\quad b=24}}</math> |
| − | והמספר הנוסף עליו כ"ד והמספר הנגרע כ"ד | + | |style="text-align:right;"|והמספר המחזיק שרש הוא כ"ה והמספר הנוסף עליו כ"ד והמספר הנגרע כ"ד |
|- | |- | ||
| | | | ||
| − | : | + | :You already know that when you add 24 to 25, it is 49 and its root is seven. |
| − | |style="text-align:right;"|וכבר ידעת כי כאשר תוסיף כ"ד על כ"ה יהיה מ"ט ושרשו שבעה | + | :<math>\scriptstyle{\color{blue}{25+24=49=7^2}}</math> |
| + | |style="text-align:right;"|וכבר ידעת כי כאשר תוסיף כ"ד על כ"ה יהיה מ"ט ושרשו <s>מט</s> שבעה | ||
|- | |- | ||
| | | | ||
| − | : | + | :And when you subtract 24 from 25, one remains and its root is one. |
| + | :<math>\scriptstyle{\color{blue}{25-24=1=1^2}}</math> | ||
|style="text-align:right;"|וכאשר תגרע כ"ד מכ"ה ישאר אחד ושרשו אחד | |style="text-align:right;"|וכאשר תגרע כ"ד מכ"ה ישאר אחד ושרשו אחד | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you want to know the value of the square, divide 25 by 24; it is one and a third of an eighth and this is the root of the square. |
| + | :<math>\scriptstyle{\color{blue}{x=\frac{25}{24}=1+\left(\frac{1}{3}\sdot\frac{1}{8}\right)}}</math> | ||
|style="text-align:right;"|ואם תבקש לדעת שעור האלגו תחלק כ"ה על כ"ד ויהיה אחד ושלישית השמינית והוא שרש האלגו | |style="text-align:right;"|ואם תבקש לדעת שעור האלגו תחלק כ"ה על כ"ד ויהיה אחד ושלישית השמינית והוא שרש האלגו | ||
|- | |- | ||
| | | | ||
| − | : | + | :The square is one, two-thirds [of an eighth], and one part of 576. |
| + | :<math>\scriptstyle{\color{blue}{x^2=1+\left(\frac{2}{3}{\color{red}{\sdot\frac{1}{8}}}\right)+\frac{1}{576}}}</math> | ||
|style="text-align:right;"|והאלגו אחד ושני שלישים וחלק אחד מתקע"ו באחד | |style="text-align:right;"|והאלגו אחד ושני שלישים וחלק אחד מתקע"ו באחד | ||
|- | |- | ||
| | | | ||
| + | :If you add its root to it, which is one and [a third of an eighth], it is two dirham, an eighth, and one part of 576 and it has a root, which is one, a third and an eighth. | ||
|style="text-align:right;"|ואם תוסיף עליו שרשו והוא אחד ורביע ושתות יהיה שנים דרהמי ושמינית אחד וחלק אחד מתקע"ו ומחזיק שרש והוא אחד ושליש ושמינית | |style="text-align:right;"|ואם תוסיף עליו שרשו והוא אחד ורביע ושתות יהיה שנים דרהמי ושמינית אחד וחלק אחד מתקע"ו ומחזיק שרש והוא אחד ושליש ושמינית | ||
|- | |- | ||
| Line 1,918: | Line 2,127: | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ואם תגרע ממנו שרשו והוא אחד ושליש ושמינית ישאר כ"ה חלקים מתקע"ו חלקים באחד ומחזיק שרש והוא שמינית אחד וחצי ששית | + | :If you subtract its root from it, which is one and a third [of an eighth], 25 part of 576 remain and it has a root, which is an eighth and half a sixth. |
| + | |style="text-align:right;"|‫<ref>72r</ref>ואם תגרע ממנו שרשו והוא אחד ושליש ושמינית ישאר כ"ה חלקים מתקע"ו חלקים באחד ומחזיק שרש והוא שמינית אחד וחצי ששית | ||
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| Line 1,924: | Line 2,134: | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you want, suppose the required number that has a root is 169, the added number is 120, and the subtracted number is 120. |
| − | + | :<math>\scriptstyle{\color{blue}{a^2=169\quad b=120}}</math> | |
|style="text-align:right;"|ואם תרצה תניח המספר המבוקש המחזיק שרש קס"ט<br> | |style="text-align:right;"|ואם תרצה תניח המספר המבוקש המחזיק שרש קס"ט<br> | ||
והמספר הנוסף ק"כ והמספר הנגרע ממנו ק"כ | והמספר הנוסף ק"כ והמספר הנגרע ממנו ק"כ | ||
|- | |- | ||
| | | | ||
| − | : | + | :You know that when you add 120 to 169, the sum has a root, which is seventeen. |
| + | :<math>\scriptstyle{\color{blue}{169+120=17^2}}</math> | ||
|style="text-align:right;"|ואתה יודע כי כשתוסיף על קס"ט ק"כ יהיה למתקבץ שרש והוא שבעה עשר | |style="text-align:right;"|ואתה יודע כי כשתוסיף על קס"ט ק"כ יהיה למתקבץ שרש והוא שבעה עשר | ||
|- | |- | ||
| | | | ||
| − | : | + | :And when you subtract 120 from it, the remainder has a root, which is seven. |
| + | :<math>\scriptstyle{\color{blue}{169-120=7^2}}</math> | ||
|style="text-align:right;"|וכאשר תגרע מהם ק"כ יהיה לנשאר שרש והוא שבעה | |style="text-align:right;"|וכאשר תגרע מהם ק"כ יהיה לנשאר שרש והוא שבעה | ||
|- | |- | ||
| | | | ||
| − | : | + | :When you want to know the value of the square, divide 169 by 120; the quotient is one, a fifth, an eighth and half a sixth and this is the root of the square. |
| + | :<math>\scriptstyle{\color{blue}{x=\frac{169}{120}=1+\frac{1}{5}+\frac{1}{8}+\left(\frac{1}{2}\sdot\frac{1}{6}\right)}}</math> | ||
|style="text-align:right;"|וכאשר תרצה לדעת שעור האלגו תחלק קס"ט על ק"כ ויעלה לחלק אחד וחמישית אחד ושמינית אחד וחצי שתות והוא שרש האלגו | |style="text-align:right;"|וכאשר תרצה לדעת שעור האלגו תחלק קס"ט על ק"כ ויעלה לחלק אחד וחמישית אחד ושמינית אחד וחצי שתות והוא שרש האלגו | ||
|- | |- | ||
| | | | ||
| − | : | + | :The square is one and 14161 parts of 14400. |
| + | :<math>\scriptstyle{\color{blue}{x^2=\frac{169}{120}=1+\frac{14161}{14400}}}</math> | ||
|style="text-align:right;"|והאלגו אחד וי"ד אלפים וקס"א מי"ד אלפים ות' באחד | |style="text-align:right;"|והאלגו אחד וי"ד אלפים וקס"א מי"ד אלפים ות' באחד | ||
|- | |- | ||
| | | | ||
| + | :If you add its root to the square, which is one, a fifth, an eighth, and half a sixth, it is three dirham and 5641 parts of 14400 and it has a root, which is one and [101] parts of 120. | ||
|style="text-align:right;"|ואם תוסיף באלגו שרשו שהוא אחד וחמישית ושמינית וחצי שמינית ושתות אחד יהיה שלשה דרהמי וחמשת אלפים ותרמ"א חלקים מי"ד אלפים ות' חלקים באחד ומחזיק שרש והוא אחד ועשרה חלקים מק"כ | |style="text-align:right;"|ואם תוסיף באלגו שרשו שהוא אחד וחמישית ושמינית וחצי שמינית ושתות אחד יהיה שלשה דרהמי וחמשת אלפים ותרמ"א חלקים מי"ד אלפים ות' חלקים באחד ומחזיק שרש והוא אחד ועשרה חלקים מק"כ | ||
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| − | + | :<math>\scriptstyle{\color{blue}{x^2+x=\left(1+\frac{14161}{14400}\right)+\left[1+\frac{1}{5}+\frac{1}{8}+\left({\color{red}{\frac{1}{2}\sdot\frac{1}{6}}}\right)\right]=3+\frac{5641}{14400}=\left(1+\frac{{\color{red}{101}}}{120}\right)^2}}</math> | |
|- | |- | ||
| | | | ||
| + | :If you subtract its root from it, which is one, a fifth, an eighth, and half a sixth, 8281 parts of 14400 remain and it has a root, which is 91 parts of 120. | ||
|style="text-align:right;"|ואם תגרע ממנו שרשו שהוא אחד וחמישית ושמינית וחצי שתות ישארו שמנת אלפים ורפ"א מי"ד אלפים ות' ומחזיק שרש והוא צ"א חלקים מק"כ | |style="text-align:right;"|ואם תגרע ממנו שרשו שהוא אחד וחמישית ושמינית וחצי שתות ישארו שמנת אלפים ורפ"א מי"ד אלפים ות' ומחזיק שרש והוא צ"א חלקים מק"כ | ||
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| − | + | :<math>\scriptstyle{\color{blue}{x^2-x=\left(1+\frac{14161}{14400}\right)-\left[1+\frac{1}{5}+\frac{1}{8}+\left(\frac{1}{2}\sdot\frac{1}{6}\right)\right]=\frac{8281}{14400}=\left(\frac{91}{120}\right)^2}}</math> | |
|- | |- | ||
|This problem and its similar have many solutions. | |This problem and its similar have many solutions. | ||
|style="text-align:right;"|ולזאת השאלה ולדומים אליה אופנים רבים | |style="text-align:right;"|ולזאת השאלה ולדומים אליה אופנים רבים | ||
|- | |- | ||
| − | | | + | |I have already explained to you the procedure of extracting these numbers or whichever of them you want, because there are as many solutions as you wish. |
|style="text-align:right;"|וכבר בארתי לך המלאכה בהוצאת אלו המספרים או אשר תרצה מהם כי הם מחזיקים אופנים רבים כרצונך | |style="text-align:right;"|וכבר בארתי לך המלאכה בהוצאת אלו המספרים או אשר תרצה מהם כי הם מחזיקים אופנים רבים כרצונך | ||
|- | |- | ||
| − | | | + | |I will further teach you the extraction of these numbers, so that your heart will be wiser about them: |
| − | |style="text-align:right;"|ואני אשכילך עוד בהוצאת אלו המספרים למען יחכם בהם | + | |style="text-align:right;"|ואני אשכילך עוד בהוצאת <s>זה</s> <sup>אלו</sup> המספרים למען יחכם בהם לבך |
|- | |- | ||
| − | |<math>\scriptstyle x^2+2x+1</math> | + | |I have already shown you that for every square that has a root, when you add its two roots and one dirham to it, it has a root. |
| + | :<math>\scriptstyle x^2+2x+1</math> | ||
|style="text-align:right;"|<big>כבר</big> הודעתיך שכל אלגו שיחזיק שרש כאשר תוסיף עליו שני שרשיו ודרהם אחד יחזיק שרש | |style="text-align:right;"|<big>כבר</big> הודעתיך שכל אלגו שיחזיק שרש כאשר תוסיף עליו שני שרשיו ודרהם אחד יחזיק שרש | ||
|- | |- | ||
| − | | | + | |Then, subtract from the square its two roots and one dirham. |
| − | + | :<math>\scriptstyle{\color{blue}{x^2-\left(2x+1\right)}}</math> | |
|style="text-align:right;"|אחר זה תגרע מהאלגו שני שרשיו ודרהם אחד | |style="text-align:right;"|אחר זה תגרע מהאלגו שני שרשיו ודרהם אחד | ||
|- | |- | ||
| − | | | + | |Say: its two roots and one dirham [<math>\scriptstyle{\color{blue}{2x+1}}</math>] is the required number. |
| − | |||
|style="text-align:right;"|ותאמר שני שרשיו ודרהם אחד יהיה המספר המבוקש | |style="text-align:right;"|ותאמר שני שרשיו ודרהם אחד יהיה המספר המבוקש | ||
|- | |- | ||
| − | | | + | |The remainder is a square minus two roots and one dirham, so you already know that its root is less than a thing. |
| − | + | :<math>\scriptstyle{\color{blue}{\sqrt{x^2-\left(2x+1\right)}<x}}</math> | |
|style="text-align:right;"|וישאר אלגו פחות שני שרשים ודרהם אחד וכבר ידעת ששרשו פחות מדבר | |style="text-align:right;"|וישאר אלגו פחות שני שרשים ודרהם אחד וכבר ידעת ששרשו פחות מדבר | ||
|- | |- | ||
| | | | ||
| − | : | + | :We suppose it is a thing minus two dirham. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\sqrt{x^2-\left(2x+1\right)}=x-2}}</math> |
| + | |style="text-align:right;"|ונניחהו דבר פחות שני דרהמי | ||
|- | |- | ||
| | | | ||
| − | : | + | :Multiply it by itself; it is a square and four dirham minus four things equal a square minus two things and one dirham. |
| − | |style="text-align:right;"|ותכהו בעצמו | + | :<math>\scriptstyle{\color{blue}{\left(x-2\right)^2=x^2+4-4x=x^2-\left(2x+1\right)}}</math> |
| + | |style="text-align:right;"|ותכהו בעצמו ויהיה אלגו וארבעה דרהמי פחות ארבעה דברים ישוה אלגו פחות שני שרשים ודרהם אחד | ||
|- | |- | ||
| | | | ||
| − | ::: | + | :*<span style="color:Green>Confrontation:</span> |
| − | | | + | | |
|- | |- | ||
| | | | ||
| − | : | + | :Confront them; the thing is equal to two dirham and a half and this is the root of the square. |
| + | :<math>\scriptstyle{\color{blue}{x=2+\frac{1}{2}}}</math> | ||
|style="text-align:right;"|ותכונהו עמו ויהיה הדבר ישוה שני דרהמי וחצי והוא שרש האלגו | |style="text-align:right;"|ותכונהו עמו ויהיה הדבר ישוה שני דרהמי וחצי והוא שרש האלגו | ||
|- | |- | ||
| | | | ||
| − | : | + | :The square is six and a quarter. |
| + | :<math>\scriptstyle{\color{blue}{x^2=6+\frac{1}{4}}}</math> | ||
|style="text-align:right;"|והאלגו ששה ורביע | |style="text-align:right;"|והאלגו ששה ורביע | ||
|- | |- | ||
| | | | ||
| − | : | + | :We suppose the number added to it is two roots and one dirham, which is six, since the root is two and a half. |
| + | :<math>\scriptstyle{\color{blue}{2x+1=2\sdot\left(2+\frac{1}{2}\right)+1=6}}</math> | ||
|style="text-align:right;"|ונניח המספר הנוסף עליו שני שרשים ודרהם אחד והוא ששה בעבור כי השרש הוא שנים וחצי | |style="text-align:right;"|ונניח המספר הנוסף עליו שני שרשים ודרהם אחד והוא ששה בעבור כי השרש הוא שנים וחצי | ||
|- | |- | ||
| | | | ||
| − | :: | + | :The required number is six and a quarter, the added number is six, and the subtracted number is six. |
| + | :<math>\scriptstyle{\color{blue}{a^2=6+\frac{1}{4}\quad b=6}}</math> | ||
|style="text-align:right;"|ויהיה המספר המבוקש ששה ורביע והמספר הנוסף ששה והמספר הנגרע ששה | |style="text-align:right;"|ויהיה המספר המבוקש ששה ורביע והמספר הנוסף ששה והמספר הנגרע ששה | ||
|- | |- | ||
| | | | ||
| − | : | + | :Convert them into quarters; the required number is 25, the added number is 24, and the subtracted number is 24. |
| − | |style="text-align:right;"|תשיבם לרביעיות | + | :<math>\scriptstyle{\color{blue}{a^2=25\quad b=24}}</math> |
| + | |style="text-align:right;"|תשיבם לרביעיות ויהיה המספר המבוקש כ"ה והמספר הנוסף ‫<ref>72v</ref>כ"ד והמספר הנגרע כ"ד | ||
|- | |- | ||
| | | | ||
| − | :: | + | :We suppose the root of the square minus two things and one dirham is a thing minus dirham and a half. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\sqrt{x^2-\left(2x+1\right)}=x-\left(1+\frac{1}{2}\right)}}</math> |
| + | |style="text-align:right;"|ואם נניח שרש האלגו פחות שני דברים ודרהם אחד דבר פחות דרהם וחצי | ||
|- | |- | ||
| | | | ||
| − | : | + | :Multiply it by itself; it is a square and two dirham and a quarter minus three things equal a square minus a thing and one dirham. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{x^2+2+\frac{1}{4}-3x=x^2-\left({\color{red}{2}}x+1\right)}}</math> |
| + | |style="text-align:right;"|תכהו בעצמו ויהיה אלגו ושני דרהמי ורביע פחות שלשה דברים ישוה אלגו פחות דבר ודרהם אחד | ||
|- | |- | ||
| | | | ||
| − | : | + | :*<span style="color:Green>Confrontation:</span> |
| − | | | + | | |
|- | |- | ||
| | | | ||
| − | : | + | :Confront them; the thing is equal to three dirham and a quarter. |
| + | :<math>\scriptstyle{\color{blue}{x=3+\frac{1}{4}}}</math> | ||
|style="text-align:right;"|ותכונהו עמו ויהיה הדבר ישוה שלשה דרהמי ורביע | |style="text-align:right;"|ותכונהו עמו ויהיה הדבר ישוה שלשה דרהמי ורביע | ||
|- | |- | ||
| | | | ||
| − | : | + | :[Convert them into quarters], the thing is thirteen-[quarters] and this is the root of the required square. |
| + | :<math>\scriptstyle{\color{blue}{x=\frac{13}{{\color{red}{4}}}}}</math> | ||
|style="text-align:right;"|ותכנהו עמו ויהיה הדבר שלשה עשר והוא שרש האלגו המבוקש | |style="text-align:right;"|ותכנהו עמו ויהיה הדבר שלשה עשר והוא שרש האלגו המבוקש | ||
|- | |- | ||
| | | | ||
| − | : | + | :[The square] is 169, every sixteen of which are one unit. |
| + | :<math>\scriptstyle{\color{blue}{x^2=\frac{169}{16}}}</math> | ||
|style="text-align:right;"|שיחזיק שרש והוא קס"ט כל ששה עשר ממנו אחד | |style="text-align:right;"|שיחזיק שרש והוא קס"ט כל ששה עשר ממנו אחד | ||
|- | |- | ||
| | | | ||
| − | : | + | :The number added to it is two roots and one dirham, which is [seven] and a half, because the root is three dirham and a quarter. Every dirham is sixteen parts, so they are 120 parts. |
| − | |style="text-align:right;"|והמספר הנוסף עליו הוא שני שרשים ודרהם אחד והוא ששה וחצי בעבור כי השרש שלשה דרהמי ורביע | + | :<math>\scriptstyle{\color{blue}{2x+1=2\sdot\left(3+\frac{1}{4}\right)+1={\color{red}{7}}+\frac{1}{2}=\frac{120}{16}}}</math> |
| + | |style="text-align:right;"|והמספר הנוסף עליו הוא שני שרשים ודרהם אחד והוא ששה וחצי בעבור כי השרש שלשה דרהמי ורביע כל דרהם ששה עשר חלקים ויהיה זה ק"כ חלקים | ||
|- | |- | ||
| − | | | + | |In what follows, proceed as I have shown you. |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|style="text-align:right;"|ותעשה בנמשך כאשר הראיתי לך | |style="text-align:right;"|ותעשה בנמשך כאשר הראיתי לך | ||
|- | |- | ||
| | | | ||
| − | :23) <math>\scriptstyle\begin{cases}\scriptstyle x^2+2x=n^2\\\scriptstyle x^2-3x=m^2\end{cases}</math> | + | :{{#annot:x²+2x=n²,x²-3x=m²|1124|ltjx}}23) If you are told: a square, if you add its two roots to it, it has a root; and if you subtract its three roots from it, it has a root. |
| − | |style="text-align:right;"|כג <big>ואם</big> יאמרו לך אלגו אם תוסיף עליו שני שרשיו יחזיק שרש ואם תגרע ממנו שלשה שרשיו יחזיק שרש | + | :<math>\scriptstyle\begin{cases}\scriptstyle x^2+2x=n^2\\\scriptstyle x^2-3x=m^2\end{cases}</math> |
| + | |style="text-align:right;"|כג <big>ואם</big> יאמרו לך אלגו אם תוסיף עליו שני שרשיו יחזיק שרש ואם תגרע ממנו שלשה שרשיו יחזיק שרש{{#annotend:ltjx}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :You should look for a number that has a root [<math>\scriptstyle{\color{blue}{a^2}}</math>], such that when you add to it a certain number, it has a root; and when you subtract that number and its half from it, the remainder has a root. |
| − | |||
|style="text-align:right;"|וצריך שתבקש מספר שיחזיק שרש וכאשר תוסיף עליו מספר מה אחר יחזיק שרש וכאשר תגרע ממנו כמו המספר הנוסף וכמו חציו יחזיק הנשאר שרש | |style="text-align:right;"|וצריך שתבקש מספר שיחזיק שרש וכאשר תוסיף עליו מספר מה אחר יחזיק שרש וכאשר תגרע ממנו כמו המספר הנוסף וכמו חציו יחזיק הנשאר שרש | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a^2+b=n^2\\\scriptstyle a^2-\left(b+\frac{1}{2}b\right)=m^2\end{cases}</math> | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Since the three roots are two roots plus their half. |
| + | :<math>\scriptstyle{\color{blue}{3x=2x+\left(\frac{1}{2}\sdot2x\right)}}</math> | ||
|style="text-align:right;"|בעבור כי שלשת השרשים הם שני השרשים וכמו חציים | |style="text-align:right;"|בעבור כי שלשת השרשים הם שני השרשים וכמו חציים | ||
|- | |- | ||
| − | | | + | |When you find that number, divide it by half the additional number, or by a third of the subtracted number, and the quotient is the root of the square. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{x=\frac{a^2}{\frac{1}{2}b}=\frac{a^2}{\frac{1}{3}\left(b+\frac{1}{2}b\right)}}}</math> | |
| − | |style="text-align:right;"|וכאשר מצאת אותו המספר תחלקהו על חצי המספר הנוסף או על שלישית המספר הנגרע והעולה לחלק יהיה שרש המרובע | + | |style="text-align:right;"|וכאשר מצאת אותו המספר תחלקהו על <sup>חצי</sup> המספר הנוסף או על שלישית המספר הנגרע והעולה לחלק יהיה שרש המרובע |
|- | |- | ||
| | | | ||
| − | :: | + | :The number that has a root is 112, the number added to it is 48, and the subtracted number is 72. |
| − | |style="text-align:right;"|והמספר המחזיק שרש קכ"א | + | :<math>\scriptstyle{\color{blue}{a^2=121\quad b=48\quad b+\frac{1}{2}b=72}}</math> |
| − | והמספר הנוסף עליו מ"ח | + | |style="text-align:right;"|והמספר המחזיק שרש קכ"א והמספר הנוסף עליו מ"ח והמספר הנגרע ע"ב |
| − | והמספר הנגרע ע"ב | ||
|- | |- | ||
| | | | ||
| − | : | + | :When you want to know the [unknown] number, divide 121 by a half of 48 if you want, or by a third of 72 if you want, which is 24; the quotient is five and a third of an eighth and it is the root of the square. |
| − | |style="text-align:right;"|וכאשר תרצה לדעת שעור המספר תחלק קכ"א אם תרצה על מחצית המ"ח | + | :<math>\scriptstyle{\color{blue}{x=\frac{121}{\frac{1}{2}\sdot48}=\frac{121}{\frac{1}{3}\sdot72}=\frac{121}{24}=5+\left(\frac{1}{3}\sdot\frac{1}{8}\right)}}</math> |
| − | או אם תרצה על שליש הע"ב | + | |style="text-align:right;"|וכאשר תרצה לדעת שעור המספר תחלק קכ"א אם תרצה על <s>המח</s> מחצית המ"ח או אם תרצה על שליש הע"ב שהוא כ"ד ומה שיעלה לחלק יהיה חמשה ושלישית השמינית והוא שרש האלגו |
| − | שהוא כ"ד | ||
| − | ומה שיעלה לחלק יהיה חמשה ושלישית השמינית והוא שרש האלגו | ||
|- | |- | ||
| | | | ||
| − | : | + | :The square is 25, a quarter, a sixth, and one part of 576. |
| + | :<math>\scriptstyle{\color{blue}{x^2=25+\frac{1}{4}{\color{red}{+}}\frac{1}{6}+\frac{1}{576}}}</math> | ||
|style="text-align:right;"|והאלגו כ"ה ורביעית הששית וחלק אחד מתקע"ו באחד | |style="text-align:right;"|והאלגו כ"ה ורביעית הששית וחלק אחד מתקע"ו באחד | ||
|- | |- | ||
| | | | ||
| + | :If you add to it its two roots, which are ten dirham and half a sixth, it is 35 dirham, [a half] and one part of 576 and it has a root, which is five dirham, a half, a third, [and] an eighth. | ||
|style="text-align:right;"|ואם תוסיף עליו שני שרשיו שהם עשרה דרהמי וחצי ששית ויהיה ל"ה דרהמי ושלשה רביעי ששית וחלק אחד מתקע"ו ומחזיק שרש והוא חמשה דרהמי וחצי ושלישית השמינית | |style="text-align:right;"|ואם תוסיף עליו שני שרשיו שהם עשרה דרהמי וחצי ששית ויהיה ל"ה דרהמי ושלשה רביעי ששית וחלק אחד מתקע"ו ומחזיק שרש והוא חמשה דרהמי וחצי ושלישית השמינית | ||
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| − | + | :<math>\scriptstyle{\color{blue}{x^2+2x=\left(25+\frac{1}{4}{\color{red}{+}}\frac{1}{6}+\frac{1}{576}\right)+\left[10+\left(\frac{1}{2}\sdot\frac{1}{6}\right)\right]=35+{\color{red}{\frac{1}{2}}}+\frac{1}{576}=\left(5+\frac{1}{2}+\frac{1}{3}{\color{red}{+}}\frac{1}{8}\right)^2}}</math> | |
|- | |- | ||
| | | | ||
| + | :If you subtract from the square its three roots, which are 15 and one eighth, the remainder is ten plus a sixth, an eighth, and one part of 576 and it has a root, which is three plus an eighth and half a sixth. | ||
|style="text-align:right;"|ואם תגרע מהאלגו שלשת שרשיו שהם ט"ו ושמינית אחד ישאר עשרה ושתות ושמינית וחלק אחד מתקע"ו ומחזיק שרש והוא שלשה ושמינית וחצי ששית | |style="text-align:right;"|ואם תגרע מהאלגו שלשת שרשיו שהם ט"ו ושמינית אחד ישאר עשרה ושתות ושמינית וחלק אחד מתקע"ו ומחזיק שרש והוא שלשה ושמינית וחצי ששית | ||
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| − | + | :<math>\scriptstyle{\color{blue}{x^2-3x=\left(25+\frac{1}{4}{\color{red}{+}}\frac{1}{6}+\frac{1}{576}\right)-\left(15+\frac{1}{8}\right)=10+\frac{1}{6}+\frac{1}{8}+\frac{1}{576}=\left[3+\frac{1}{8}+\left(\frac{1}{2}\sdot\frac{1}{6}\right)\right]^2}}</math> | |
|- | |- | ||
| | | | ||
| − | : | + | :If you wish, assume the required number that has a root is 49 and the subtracted number is 48. |
| − | + | :<math>\scriptstyle{\color{blue}{a^2=49\quad b={\color{red}{32}}\quad b+\frac{1}{2}b=48}}</math> | |
| − | |style="text-align:right;"|ואם תרצה תניח המספר המבוקש שיחזיק שרש מ"ט | + | |style="text-align:right;"|ואם תרצה תניח המספר המבוקש <s>מ"ט</s> שיחזיק שרש מ"ט והמספר הנגרע מ"ח |
| − | והמספר הנגרע מ"ח | ||
|- | |- | ||
| | | | ||
| − | : | + | :Divide 49 by sixteen; the quotient is three and half an eighth and this is the root of the square. |
| − | |style="text-align:right;"|ותחלק מ"ט על ששה עשר | + | :<math>\scriptstyle{\color{blue}{x=\frac{49}{16}=3+\left(\frac{1}{2}\sdot\frac{1}{8}\right)}}</math> |
| + | |style="text-align:right;"|ותחלק מ"ט על ששה עשר ויעלה לחלק שלשה וחצי שמינית והוא שרש האלגו | ||
|- | |- | ||
| | | | ||
| − | : | + | :The square is nine plus three-eighth and one part of 256. |
| − | |style="text-align:right;"|והאלגו תשעה ושלשה שמיניות וחלק אחד מרנ"ו | + | :<math>\scriptstyle{\color{blue}{x^2=9+\frac{3}{8}+\frac{1}{256}}}</math> |
| + | |style="text-align:right;"|‫<ref>73r</ref>והאלגו תשעה ושלשה שמיניות וחלק אחד מרנ"ו | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you wish, assume the required number that has a root is 625, the added number is 336, and the subtracted number is 504. |
| − | + | :<math>\scriptstyle{\color{blue}{a^2=625\quad b=336\quad b+\frac{1}{2}b=504}}</math> | |
| − | |style="text-align:right;"|ואם תרצה תניח המספר המחזיק שרש תרכ"ה | + | |style="text-align:right;"|ואם תרצה תניח המספר המחזיק שרש תרכ"ה והמספר הנוסף של"ו והמספר הנגרע תק"ד |
| − | והמספר הנוסף של"ו | ||
| − | והמספר הנגרע תק"ד | ||
|- | |- | ||
| | | | ||
| + | :Divide 625 by a half of 336 or by a third of 504, which is 168; the quotient is three, two-thirds, a quarter of a seventh, and an eighth of a seventh, and this is the root of the square. | ||
|style="text-align:right;"|ותחלק תרכ"ה על מחצית של"ו או על שלישית תק"ד והוא קס"ח ויעלה לחלק שלשה ושני שלישים ורביע השביעית ושמינית השביעית והוא שרש האלגו | |style="text-align:right;"|ותחלק תרכ"ה על מחצית של"ו או על שלישית תק"ד והוא קס"ח ויעלה לחלק שלשה ושני שלישים ורביע השביעית ושמינית השביעית והוא שרש האלגו | ||
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| − | + | :<math>\scriptstyle{\color{blue}{x=\frac{625}{\frac{1}{2}\sdot336}=\frac{625}{\frac{1}{3}\sdot504}=\frac{625}{168}=3+\frac{2}{3}+\left(\frac{1}{4}\sdot\frac{1}{7}\right)+\left(\frac{1}{8}\sdot\frac{1}{7}\right)}}</math> | |
|- | |- | ||
| | | | ||
| − | : | + | :The square is thirteen dirham, 141 parts of 1[68] and 25 parts of 28224. |
| + | :<math>\scriptstyle{\color{blue}{x^2=13+\frac{141}{1{\color{red}{68}}}+\frac{25}{28224}}}</math> | ||
|style="text-align:right;"|והאלגו שלשה עשר דרהמי וקמ"א חלקים מקפ"ט וכ"ה חלקים מכ"ח אלפים ורכ"ד | |style="text-align:right;"|והאלגו שלשה עשר דרהמי וקמ"א חלקים מקפ"ט וכ"ה חלקים מכ"ח אלפים ורכ"ד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ואם תוסיף עליו שני שרשיו והם שבעה דרהמי וצ"ד חלקים מקפ"ט יהיה כ"א דרהמי ומ"ז חלקים מקס"ח חלקים וכ"ה חלקים מכ"ח אלפים | + | :If you add to it its two roots, which are seven dirham and [7]4 parts of 1[68], it is 21 dirham, 47 parts of 168, and 25 parts of 28224 and it has a root, which is four dirham and 103 parts of 16[8]. |
| + | |style="text-align:right;"|ואם תוסיף עליו שני שרשיו והם שבעה דרהמי וצ"ד חלקים מקפ"ט יהיה כ"א דרהמי ומ"ז <s>דרהמי</s> חלקים מקס"ח חלקים וכ"ה חלקים מכ"ח אלפים ו<sup>ר</sup>כ"ד חלקים ומחזיק שרש והוא ארבעה דרהמי וק"ג חלקים מקס"ג חלקים מאחד | ||
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| − | + | :<math>\scriptstyle{\color{blue}{x^2+2x=\left(13+\frac{141}{1{\color{red}{68}}}+\frac{25}{28224}\right)+\left(7+\frac{{\color{red}{74}}}{1{\color{red}{68}}}\right)=21+\frac{47}{168}+\frac{25}{28224}=\left(4+\frac{103}{16{\color{red}{8}}}\right)^2}}</math> | |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ואם תגרע מהאלגו שלשת שרשיו ישאר שני אדרהמי וקי"ד חלקים מקס"ח וכ"ה חלקים מכ"ח אלפים ורכ"ד חלקים מאחד ומחזיק שרש והוא דרהם אחד וק"ט מקס"ח חלקים באחד | + | :If you subtract from it its three roots, the remainder is two dirham, 114 parts of 168, and 25 parts of 28224 and it has a root, which is one dirham and 10[7] parts of 168. |
| + | |style="text-align:right;"|ואם תגרע מהאלגו שלשת שרשיו ישאר שני אדרהמי וקי"ד חלקים מקס"ח וכ"ה חלקים מכ"ח אלפים ורכ"ד חלקים מאחד ומחזיק שרש והוא דרהם אחד <s>וק</s> וק"ט מקס"ח חלקים באחד | ||
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| − | + | :<math>\scriptstyle{\color{blue}{x^2-3x=2+\frac{114}{168}+\frac{25}{28224}=\left(1+\frac{10{\color{red}{7}}}{168}\right)^2}}</math> | |
|- | |- | ||
| − | |The reason for the extraction of these numbers is explained above. | + | |The reason for the extraction of these numbers is clear from what we explained above. |
|style="text-align:right;"|וסבת הוצאת אלו המספרים תהיה מבוארת ממה שבארנו קודם | |style="text-align:right;"|וסבת הוצאת אלו המספרים תהיה מבוארת ממה שבארנו קודם | ||
|- | |- | ||
| − | |<math>\scriptstyle\sqrt{x^2+{\color{red}{2x+1}}}=x+1</math> | + | | |
| + | :I have already shown you that every square [plus its two roots and one dirham] has a root, which is one thing and one dirham. | ||
| + | :<math>\scriptstyle\sqrt{x^2+{\color{red}{2x+1}}}=x+1</math> | ||
|style="text-align:right;"|וכבר הראיתי לך שכל אלגו שיחזיק שרש והוא דבר ודרהם אחד | |style="text-align:right;"|וכבר הראיתי לך שכל אלגו שיחזיק שרש והוא דבר ודרהם אחד | ||
|- | |- | ||
| | | | ||
| − | : | + | :Suppose the number added to the square is two things and one dirham, and the number subtracted from it is its three roots and one and a half dirham. |
| − | + | |style="text-align:right;"|<s>תח</s> תניח המספר הנוסף על האלגו שני דברים ודרהם אחד והמספר הנגרע ממנו שלשת שרשיו ודרהם אחד וחצי | |
| − | + | |- | |
| + | |colspan=2| | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle x^2+2x+1=n^2\\\scriptstyle x^2-\left[3x+\left(1+\frac{1}{2}\right)\right]=m^2\end{cases}</math> | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x^2-\left[3x+\left(1+{\color{red}{\frac{1}{2}}}\right)\right]}}</math> | + | :Subtract from the square its three roots and one and a half dirham; the remainder is a square minus three roots and one dirham. |
| + | :<math>\scriptstyle{\color{blue}{x^2-\left[3x+\left(1+{\color{red}{\frac{1}{2}}}\right)\right]}}</math> | ||
|style="text-align:right;"|ותגרע מהאלגו שלשת שרשיו ודרהם וחצי וישאר אלגו פחות שלשה שרשים ודרהם אחד | |style="text-align:right;"|ותגרע מהאלגו שלשת שרשיו ודרהם וחצי וישאר אלגו פחות שלשה שרשים ודרהם אחד | ||
|- | |- | ||
| | | | ||
| − | : | + | :Suppose its root is [a thing] minus two dirham. |
| − | |style="text-align:right;"|ותניח שרשו | + | :<math>\scriptstyle{\color{blue}{\sqrt{x^2-\left[3x+\left(1+\frac{1}{2}\right)\right]}={\color{red}{x}}-2}}</math> |
| + | |style="text-align:right;"|ותניח שרשו שני דברים פחות שני דרהמי | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{\left(x-2\right)^2= | + | :Multiply it by itself; it is a square and four dirham minus four things equal a square minus three things plus one dirham and a half. |
| − | + | |style="text-align:right;"|ותכם בעצמם ויהיה אלגו וארבעה דרהמי פחות ארבעה דברים ישוה אלגו פחות שלשה דברים ודרהם אחד וחצי | |
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\left(x-2\right)^2=x^2+4-4x=x^2-\left[3x+\left(1+\frac{1}{2}\right)\right]}}</math> | ||
|- | |- | ||
| | | | ||
| − | ::: | + | :*<span style="color:Green>Confrontation:</span> |
| − | | | + | | |
|- | |- | ||
| | | | ||
| − | : | + | :Confront them; the thing is five and a half. |
| + | :<math>\scriptstyle{\color{blue}{x=5+\frac{1}{2}}}</math> | ||
|style="text-align:right;"|ותכונם עמהם ויהיה הדבר חמשה וחצי | |style="text-align:right;"|ותכונם עמהם ויהיה הדבר חמשה וחצי | ||
|- | |- | ||
| | | | ||
| − | : | + | :The square is thirty and a quarter. |
| + | :<math>\scriptstyle{\color{blue}{x^2=30+\frac{1}{4}}}</math> | ||
|style="text-align:right;"|והאלגו שלשים ורביע | |style="text-align:right;"|והאלגו שלשים ורביע | ||
|- | |- | ||
| | | | ||
| − | : | + | :We suppose the number added to it is two things and one dirham, which is twelve. |
| − | |style="text-align:right;"|ונניח המספר הנוסף עליו שני דברים ודרהם אחד והוא שנים עשר | + | :<math>\scriptstyle{\color{blue}{2x+1=12}}</math> |
| + | |style="text-align:right;"|ונניח המספר הנוסף עליו שני דברים ודרהם אחד <s>והם</s> <sup>והוא</sup> שנים עשר | ||
|- | |- | ||
| | | | ||
| − | : | + | :We suppose the number subtracted from it is three things plus one dirham and a half, which is eighteen. |
| + | :<math>\scriptstyle{\color{blue}{3x+\left(1+\frac{1}{2}\right)=18}}</math> | ||
|style="text-align:right;"|ונניח המספר הנגרע ממנו שלשה דברים ודרהם וחצי והוא שמנה עשר | |style="text-align:right;"|ונניח המספר הנגרע ממנו שלשה דברים ודרהם וחצי והוא שמנה עשר | ||
|- | |- | ||
| | | | ||
| − | : | + | :Convert them into quarters; The required number that has a root is 121, the additional number is 48, and the subtracted number is 72. |
| − | |style="text-align:right;"|השיבם רביעיות | + | :<math>\scriptstyle{\color{blue}{a^2=121\quad b=48\quad b+\frac{1}{2}b=72}}</math> |
| + | |style="text-align:right;"|השיבם רביעיות ויהיה המספר המבוקש שיחזיק שרש קכ"א והמספר הנוסף מ"ח והנגרע ע"ב | ||
|- | |- | ||
| − | | | + | |Proceed the procedure as I instructed you. |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|style="text-align:right;"|ותעשה בפעולתם כמו שאמרתי לך | |style="text-align:right;"|ותעשה בפעולתם כמו שאמרתי לך | ||
|- | |- | ||
| | | | ||
| − | :24) <math>\scriptstyle10x-8-x^2=n^2</math> | + | :{{#annot:10x-8-x²=n²|1124|JrVS}}24) If you are told: a square that has a root, if you subtract it from its ten roots minus eight dirham, the remainder has a root. |
| − | |style="text-align:right;"|כד <big>ואם</big> יאמרו לך אלגו שיחזיק שרש אם תגרעהו מעשרת שרשיו פחות שמנה דרהמי יחזיק מה שישאר שרש | + | :<math>\scriptstyle10x-8-x^2=n^2</math> |
| + | |style="text-align:right;"|כד <big>ואם</big> יאמרו לך אלגו שיחזיק שרש אם תגרעהו מעשרת שרשיו פחות שמנה <s>שרשיו</s> דרהמי יחזיק מה שישאר שרש{{#annotend:JrVS}} | ||
|- | |- | ||
| − | | | + | |When you have such a question, multiply half [the number of] the roots by itself and subtract the dirham from the product. |
|style="text-align:right;"|כאשר יקרה לך שאלות ככה תכה מחצית השרשים בעצמם והמתקבץ תגרע ממנו האדרהמי | |style="text-align:right;"|כאשר יקרה לך שאלות ככה תכה מחצית השרשים בעצמם והמתקבץ תגרע ממנו האדרהמי | ||
|- | |- | ||
| − | | | + | |If the remainder can be divided into two parts, each of which has a root, the problem has as many solutions as you wish. |
| − | + | |style="text-align:right;"|‫<ref>73v</ref>ומה שישאר אם יתחלק לשני חלקים שיחזיק כל חלק שרש הנה תחזיק השאלה רבים מהאופנים כמה שתרצה | |
| − | |style="text-align:right;"|ומה שישאר אם יתחלק לשני חלקים שיחזיק כל חלק שרש הנה תחזיק השאלה רבים מהאופנים כמה שתרצה | + | |- |
| + | |If the remainder cannot be divided into two parts, each of which has a root, the problem has no solution and no solving procedure. | ||
| + | |style="text-align:right;"|ואם לא יתחלק לשני חלקים שיחזיק כל חלק שרש הנה לא יהיה לשאלה אופן ולא מלאכה | ||
| + | |- | ||
| + | |If, when you multiply half [the number of] the roots by itself, the result is the same as the number of the dirham, or less, the problem is incorrect. | ||
| + | |style="text-align:right;"|ואם כאשר תכה מחצית השרשים בעצמו יצא כמו שעור הדרהמי או פחות מהם לא היתה השאלה נכונה | ||
|- | |- | ||
| | | | ||
| − | + | :We multiply half [the number of] the roots in this question, which is five, by itself; it is twenty-five. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונכה מחצית השרשים בעצמם בזאת השאלה והוא חמשה ויהיה עשרים וחמשה |
|- | |- | ||
| | | | ||
| − | + | :Subtract the eight dirham from it; seventeen remains. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תגרע מהם שמנה הדרהמי וישאר שבעה עשר |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Divide it into two parts, each of which has a root; they are sixteen and one. |
| − | + | |style="text-align:right;"|חלקם לשני חלקים ולכל חלק שרש והם ששה עשר ואחד | |
| − | חלקם לשני חלקים ולכל חלק שרש והם ששה עשר ואחד | ||
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| − | + | :<math>\scriptstyle{\color{blue}{a^2+b^2=\left(\frac{1}{2}\sdot10\right)^2-8=5^2-8=25-8=17=16+1}}</math> | |
|- | |- | ||
| | | | ||
| − | :: | + | :If you want, say: a square and sixteen dirham equal ten roots minus eight dirham. |
| + | :<math>\scriptstyle{\color{blue}{x^2+16=10x-8}}</math> | ||
|style="text-align:right;"|ואם תרצה תאמר אלגו וששה עשר דרהמי ישוה עשרה שרשים פחות שמנה דרהמי | |style="text-align:right;"|ואם תרצה תאמר אלגו וששה עשר דרהמי ישוה עשרה שרשים פחות שמנה דרהמי | ||
|- | |- | ||
| | | | ||
| − | :: | + | :If you want, say: a square and one dirham equal ten roots minus eight. |
| + | :<math>\scriptstyle{\color{blue}{x^2+1=10x-8}}</math> | ||
|style="text-align:right;"|ואם תרצה תאמר אלגו ודרהם אחד ישוה עשרה שרשים פחות שמנה | |style="text-align:right;"|ואם תרצה תאמר אלגו ודרהם אחד ישוה עשרה שרשים פחות שמנה | ||
|- | |- | ||
| | | | ||
| − | :: | + | :It is as if you say: a square and sixteen dirham equal ten roots minus eight dirham. |
| + | :<math>\scriptstyle{\color{blue}{x^2+16=10x-8}}</math> | ||
|style="text-align:right;"|והוא כאלו תאמר אלגו וששה עשר דרהמי ישוה עשרה שרשים פחות שמנה דרהמי | |style="text-align:right;"|והוא כאלו תאמר אלגו וששה עשר דרהמי ישוה עשרה שרשים פחות שמנה דרהמי | ||
|- | |- | ||
| | | | ||
| − | : | + | :The square is 36 if you wish, or [six]teen if you wish. |
| − | |style="text-align:right;"|ויהיה האלגו אם תרצה ל"ו | + | :<math>\scriptstyle{\color{blue}{x_1^2=36\quad x_2^2={1\color{red}{6}}}}</math> |
| + | |style="text-align:right;"|ויהיה האלגו אם תרצה ל"ו ואם תרצה יהיה שמנה עשר | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle | + | :{{#annot:x²+1=10x-8|1124|g7c7}}25) If you are told: a square and one dirham equal ten roots minus eight dirham. |
| − | |style="text-align:right;"|ואם | + | :<math>\scriptstyle x^2+1=10x-8</math> |
| + | |style="text-align:right;"|כה <big>ואם</big> יאמרו לך אלגו ודרהם אחד ישוו עשרה שרשים פחות שמנה דרהמי{{#annotend:g7c7}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :The square is one if you wish, or eighty-one if you wish. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{x_1^2=1\quad x_2^2=81}}</math> |
| + | |style="text-align:right;"|יהיה האלגו אם תרצה אחד ואם תרצה יהיה שמנים ואחד | ||
|- | |- | ||
| | | | ||
| − | : | + | :{{#annot:260-6x-x²=n²|1124|63F7}}26) If you are told: a square that has a root, if you subtract it from 260 minus six roots, the remainder has a root. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle260-6x-x^2=n^2</math> |
| + | |style="text-align:right;"|כו <big>ואם</big> יאמרו לך אלגו המחזיק שרש אם תגרעהו מר"ס פחות ששה שרשים יחזיק הנשאר שרש{{#annotend:63F7}} | ||
|- | |- | ||
| − | | | + | |When you have such a question, multiply half [the number of] the roots by itself and add it to the dirham. |
| − | + | |style="text-align:right;"|וכאשר תפול לפניך שאלה כזאת תכה מחצית השרשים בעצמם ותוסיפם על הדרהמי | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |If the sum can be divided into two parts, each of which has a root, the problem has as many solutions as you wish. |
| − | + | |style="text-align:right;"|ומה שיתקבץ אם יתחלק לשני חלקים שיחזיק כל חלק שרש תהיה השאלה אז בעלת אופנים כמה שתרצה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |If it cannot be divided like that, the problem is not solvable and has no solution. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם לא יתחלק ככה תהיה השאלה חרשת בזולת האופן |
|- | |- | ||
| | | | ||
| − | + | :The product of half [the number of] the roots by itself in this question is nine, because their half is three. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אחר זה הנה הכאת מחצית השרשים בעצמם בזאת השאלה היא תשעה כי מחציתם שלשה |
|- | |- | ||
| | | | ||
| − | + | :Add it to 260; it is 269. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תוסיפם על ר"ס ויהיה רס"ט |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Divide it into two parts, each of which has a root; they are 169 and one hundred. |
| − | + | |style="text-align:right;"|תחלקם לשני חלקים שיחזיק כל חלק שרש ויהיו קס"ט ומאה | |
| − | תחלקם לשני חלקים שיחזיק כל חלק שרש ויהיו קס"ט ומאה | ||
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| − | + | :<math>\scriptstyle{\color{blue}{a^2+b^2=\left(\frac{1}{2}\sdot6\right)^2+260=3^2+260=9+260=269=100+169}}</math> | |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x^2+169=260-6x}}</math> | + | :If you want, say: a square and 169 equal 260 dirham minus six things. |
| + | :<math>\scriptstyle{\color{blue}{x^2+169=260-6x}}</math> | ||
|style="text-align:right;"|ותאמר אם תרצה אלגו וקס"ט ישוו ר"ס דרהמי פחות ששה דברים | |style="text-align:right;"|ותאמר אם תרצה אלגו וקס"ט ישוו ר"ס דרהמי פחות ששה דברים | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{y^2+100=260-6y}}</math> | + | :If you want, say: a square and one hundred dirham equal 260 dirham minus six things. |
| + | :<math>\scriptstyle{\color{blue}{y^2+100=260-6y}}</math> | ||
|style="text-align:right;"|ואם תרצה תאמר אלגו ומאה דרהמי ישוו ר"ס פחות ששה דברים | |style="text-align:right;"|ואם תרצה תאמר אלגו ומאה דרהמי ישוו ר"ס פחות ששה דברים | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x^2=49}}</math> | + | :The square is 49 dirham. |
| + | :<math>\scriptstyle{\color{blue}{x^2+169=260-6x\longrightarrow x^2=49}}</math> | ||
|style="text-align:right;"|ויהיה האלגו מ"ט דרהמי | |style="text-align:right;"|ויהיה האלגו מ"ט דרהמי | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{y^2+100=260-6y}}</math> | + | :And if you said: a square and one hundred dirham equal 260 dirham minus six things, the square is one hundred dirham. |
| − | |style="text-align:right;"|ואם אמרת אלגו ומאה דרהמי ישוו ר"ס דרהמי פחות ששה דברים | + | :<math>\scriptstyle{\color{blue}{y^2+100=260-6y\longrightarrow y^2=100}}</math> |
| + | |style="text-align:right;"|ואם אמרת אלגו ומאה דרהמי ישוו ר"ס דרהמי פחות ששה דברים יהיה האלגו מאה דרהמי | ||
|- | |- | ||
| | | | ||
| − | : | + | :{{#annot:x²+2x=n²,x²+2x+3√(x²+2x)=m²|1124|Xpj1}}27) If you are told: a square that has a root, if you add its two roots to it, it has a root; and if you add to the sum its three roots, it has a root. |
| − | + | :<math>\scriptstyle\begin{cases}\scriptstyle x^2+2x=n^2\\\scriptstyle x^2+2x+3\sqrt{x^2+2x}=m^2\end{cases}</math> | |
| − | |||
| − | |||
| − | : | ||
|style="text-align:right;"|כז <big>ואם</big> יאמרו לך אלגו המחזיק שרש אם תוסיף עליו שני שרשיו יחזיק שרש<br> | |style="text-align:right;"|כז <big>ואם</big> יאמרו לך אלגו המחזיק שרש אם תוסיף עליו שני שרשיו יחזיק שרש<br> | ||
| − | ואם תוסיף על המקובץ שלשת שרשיו יחזיק שרש | + | ואם תוסיף על המקובץ שלשת שרשיו יחזיק שרש{{#annotend:Xpj1}} |
|- | |- | ||
| | | | ||
| − | : | + | :Its procedure is that you assume your square is a square [<math>\scriptstyle{\color{blue}{x^2}}</math>]. |
| − | + | |style="text-align:right;"|‫<ref>74r</ref>מלאכתו שתניח האלגו שלך אלגו | |
| − | |style="text-align:right;"|מלאכתו שתניח האלגו שלך אלגו | ||
|- | |- | ||
| | | | ||
| − | : | + | :Add its two roots to it; it is a square and two roots, which has a root and its root is a thing and a half. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\sqrt{x^2+2x}=\left(1+\frac{1}{2}\right)x}}</math> |
| + | |style="text-align:right;"|ותוסיף עליו שני שרשיו ויהיה אלגו ושני שרשים ויחזיק שרש והוא דבר וחצי | ||
|- | |- | ||
| | | | ||
| − | : | + | :The reason I assumed its root is a thing and a half is that the three roots are the same as the two roots plus their half. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{3\sqrt{x^2+2x}=2\sqrt{x^2+2x}+\left(\frac{1}{2}\sdot2\sqrt{x^2+2x}\right)}}</math> |
| + | |style="text-align:right;"|והסבה למה הנחתי שרשו דבר וחצי בעבור כי שלשת השרשים כמו שני השרשים וכמו חציים | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Multiply a thing and a half by it self; it is two squares and a quarter equal a square and two roots. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\left[\left(1+\frac{1}{2}\right)x\right]^2=\left(\sqrt{x^2+2x}\right)^2=\left(2+\frac{1}{4}\right)x^2=x^2+2x}}</math> |
| + | |style="text-align:right;"|ותכה דבר וחצי בעצמו ויהיה שני אלגו ורביע ישוה אלגו ושני שרשים | ||
|- | |- | ||
| | | | ||
| − | ::: | + | :*<span style="color:Green>Confrontation:</span> |
| − | |||
| − | |||
| | | | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Confront them; the root of the square is one dirham and three-fifths. |
| + | :<math>\scriptstyle{\color{blue}{x=1+\frac{3}{5}}}</math> | ||
|style="text-align:right;"|ותכונהו עמו ויהיה שרש האלגו דרהם אחד ושלשה חומשים | |style="text-align:right;"|ותכונהו עמו ויהיה שרש האלגו דרהם אחד ושלשה חומשים | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x^2=2+\frac{2}{5}+\left({\color{red}{\frac{4}{5}}}\sdot\frac{1}{5}\right)}}</math> | + | :The square is two dirham, two-fifths and [four-fifths] of a fifth. |
| + | :<math>\scriptstyle{\color{blue}{x^2=2+\frac{2}{5}+\left({\color{red}{\frac{4}{5}}}\sdot\frac{1}{5}\right)}}</math> | ||
|style="text-align:right;"|והאלגו שני דרהמי ושני חומשים ורביע חומש החומש | |style="text-align:right;"|והאלגו שני דרהמי ושני חומשים ורביע חומש החומש | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ואם תוסיף עליו שני שרשיו והם שלשה וחמשית יהיה חמשה דרהמי ושלשה חומשי' וארבעה חומשי החומש | + | :If you add to it its two roots, which is three and a fifth; it is five dirham, three-fifths and four-fifths of a fifth. |
| + | |style="text-align:right;"|ואם תוסיף עליו שני שרשיו והם שלשה וחמשית יהיה <s>חמשית</s> חמשה דרהמי ושלשה חומשי' וארבעה חומשי החומש | ||
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| − | + | :<math>\scriptstyle{\color{blue}{x^2+2x=\left[2+\frac{2}{5}+\left(\frac{4}{5}\sdot\frac{1}{5}\right)\right]+\left(3+\frac{1}{5}\right)=5+\frac{3}{5}+\left(\frac{4}{5}\sdot\frac{1}{5}\right)}}</math> | |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\sqrt{x^2+2x}=2+\frac{2}{5}=\left(1+\frac{1}{2}\right)x}}</math> | + | :It is known that its root is the same as the root of the primary square plus its half, which is two dirham and two-fifths. |
| + | :<math>\scriptstyle{\color{blue}{\sqrt{x^2+2x}=2+\frac{2}{5}=\left(1+\frac{1}{2}\right)x}}</math> | ||
|style="text-align:right;"|והוא ידוע כי שרשו כמו שרש האלגו הראשון וכמו חציו והוא שני דרהמי ושני חמישיות | |style="text-align:right;"|והוא ידוע כי שרשו כמו שרש האלגו הראשון וכמו חציו והוא שני דרהמי ושני חמישיות | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{2x=\left(1+\frac{1}{4}\right)x^2}}</math> | + | :The two roots are the same as the square plus its quarter. |
| + | :<math>\scriptstyle{\color{blue}{2x=\left(1+\frac{1}{4}\right)x^2}}</math> | ||
|style="text-align:right;"|ושני השרשים כמו האלגו וכמו רביעיתו | |style="text-align:right;"|ושני השרשים כמו האלגו וכמו רביעיתו | ||
|- | |- | ||
| | | | ||
| + | :Three roots of five dirham, three-fifths and [four-fifths] of a fifth are necessarily the same as the square plus its quarter, which is seven and a fifth. | ||
|style="text-align:right;"|והוא מחויב שיהיו שלשת שרשים מחמשה דרהמי ושלשה חומשים ורביע חומש מחמישית כמו שהוא האלגו ורביעיתו יותר והוא שבעה וחומש | |style="text-align:right;"|והוא מחויב שיהיו שלשת שרשים מחמשה דרהמי ושלשה חומשים ורביע חומש מחמישית כמו שהוא האלגו ורביעיתו יותר והוא שבעה וחומש | ||
|- | |- | ||
| Line 2,359: | Line 2,608: | ||
|- | |- | ||
| | | | ||
| + | :When you add them to five dirham, three-fifths and four-fifths of a fifth, it is twelve, four-fifths and four-fifths of a fifth. | ||
|style="text-align:right;"|וכאשר תוסיפם על חמשה דרהמי ושלשה חומשים וארבעה חומשי החומש יהיה שנים עשר וארבעה חומשים וארבעה חומשי החומש | |style="text-align:right;"|וכאשר תוסיפם על חמשה דרהמי ושלשה חומשים וארבעה חומשי החומש יהיה שנים עשר וארבעה חומשים וארבעה חומשי החומש | ||
|- | |- | ||
| Line 2,365: | Line 2,615: | ||
|- | |- | ||
| | | | ||
| + | :It is known that its root is the same as one and a half the root of five, three-fifths and four-fifths of a fifth, which is three dirham and three-fifths. | ||
|style="text-align:right;"|והוא ידוע כי שרשו הוא כמו שרש אחד וחצי מחמשה ושלשה חומשים וארבעה חומשי החומש והוא שלשה דרהמיש ושלשה חומשים | |style="text-align:right;"|והוא ידוע כי שרשו הוא כמו שרש אחד וחצי מחמשה ושלשה חומשים וארבעה חומשי החומש והוא שלשה דרהמיש ושלשה חומשים | ||
|- | |- | ||
| Line 2,371: | Line 2,622: | ||
|- | |- | ||
| | | | ||
| + | :Four and a half roots of 12 dirham, four-fifths and four-fifths of a fifth are necessarily the same as the square plus its quarter, which is 16 and a fifth. | ||
|style="text-align:right;"|והוא ראוי שיהיו ארבעה שרשים וחצי מי"ב וארבעה חומשים וארבעה חומשי החומש שיהיו כמו האלגו וכמו רביעיתו יותר והוא י"ו וחומש | |style="text-align:right;"|והוא ראוי שיהיו ארבעה שרשים וחצי מי"ב וארבעה חומשים וארבעה חומשי החומש שיהיו כמו האלגו וכמו רביעיתו יותר והוא י"ו וחומש | ||
|- | |- | ||
| Line 2,377: | Line 2,629: | ||
|- | |- | ||
| | | | ||
| − | : | + | :When you add them to 12 dirham, four-fifths and four-fifths of a fifth, it is 29 and four-fifths of a fifth. |
|style="text-align:right;"|וכאשר תוסיפם על י"ב וארבעה חומשים וארבעה חומשי החומש יהיה כ"ט וארבעה חומשי החומש | |style="text-align:right;"|וכאשר תוסיפם על י"ב וארבעה חומשים וארבעה חומשי החומש יהיה כ"ט וארבעה חומשי החומש | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\left(16+\frac{1}{5}\right)+\left[12+\frac{4}{5}+\left(\frac{4}{5}\sdot\frac{1}{5}\right)\right]=29+\left(\frac{4}{5}\sdot\frac{1}{5}\right)}}</math> | ||
|- | |- | ||
| | | | ||
| + | :It is known that its root is the same as one and a half the root of 12, four-fifths and four-fifths of a fifth, which is five dirham and two-fifths. | ||
|style="text-align:right;"|והוא ידוע כי שרשו הוא כמו שרש אחד וחצי מי"ב וארבעה חומשי' וארבעה חומשי החומש והוא חמשה דרהמי ושני חומשים | |style="text-align:right;"|והוא ידוע כי שרשו הוא כמו שרש אחד וחצי מי"ב וארבעה חומשי' וארבעה חומשי החומש והוא חמשה דרהמי ושני חומשים | ||
|- | |- | ||
| Line 2,386: | Line 2,642: | ||
:<math>\scriptstyle{\color{blue}{\sqrt{29+\left(\frac{4}{5}\sdot\frac{1}{5}\right)}=5+\frac{2}{5}=\left(1+\frac{1}{2}\right)\sdot\sqrt{12+\frac{4}{5}+\left(\frac{4}{5}\sdot\frac{1}{5}\right)}}}</math> | :<math>\scriptstyle{\color{blue}{\sqrt{29+\left(\frac{4}{5}\sdot\frac{1}{5}\right)}=5+\frac{2}{5}=\left(1+\frac{1}{2}\right)\sdot\sqrt{12+\frac{4}{5}+\left(\frac{4}{5}\sdot\frac{1}{5}\right)}}}</math> | ||
|- | |- | ||
| − | | | + | |Likewise, if you add as many of the roots as you like to what is related to them, the sum has a root as stated. |
|style="text-align:right;"|וכן אם תוסיף מה שתרצה מהשרשים על המתיחס אליו שאשר יתקבץ ממנו יחזיק שרש כמו שנאמר | |style="text-align:right;"|וכן אם תוסיף מה שתרצה מהשרשים על המתיחס אליו שאשר יתקבץ ממנו יחזיק שרש כמו שנאמר | ||
|- | |- | ||
| | | | ||
| + | :Because, when we add to 29 and four-fifths of a fifth its six roots and three-quarters of its root, the sum has a root and it is the same as one and a half of the root of 29 and four-fifths of a fifth, which is eight [and a tenth]. | ||
|style="text-align:right;"|כי כאשר נוסיף על כ"ט וארבעה חומשי החומש ששת שרשיו ושלשה רביעי השרש יהיה למתקבץ מהם שרש והוא כמו שרש וחצי מכ"ט וארבעה חומשים מחומש והוא שמנה עשר | |style="text-align:right;"|כי כאשר נוסיף על כ"ט וארבעה חומשי החומש ששת שרשיו ושלשה רביעי השרש יהיה למתקבץ מהם שרש והוא כמו שרש וחצי מכ"ט וארבעה חומשים מחומש והוא שמנה עשר | ||
|- | |- | ||
| Line 2,396: | Line 2,653: | ||
|- | |- | ||
| | | | ||
| + | :And when you add to the sum its ten roots and an eighth of its root, the sum has a root and it is the same as eight [and a tenth] plus its half, which is [12...] | ||
|style="text-align:right;"|וכאשר תוסיף על המתקבץ עשרת שרשיו ושמינית השרש יחזיק המתקבץ מהם שרש והוא כמו שמנה עשר וכמו חציו שהם כ"ז | |style="text-align:right;"|וכאשר תוסיף על המתקבץ עשרת שרשיו ושמינית השרש יחזיק המתקבץ מהם שרש והוא כמו שמנה עשר וכמו חציו שהם כ"ז | ||
|- | |- | ||
| Line 2,401: | Line 2,659: | ||
:<math>\scriptstyle{\color{blue}{\sqrt{\left(8+\frac{1}{10}\right)^2+\left(10+\frac{1}{8}\right)\sdot\sqrt{\left(8+\frac{1}{10}\right)^2}}={\color{red}{12+\frac{1}{10}+\left(\frac{1}{2}\sdot\frac{1}{10}\right)}}=\left(1+\frac{1}{2}\right)\sdot\left(8+\frac{1}{10}\right)}}</math> | :<math>\scriptstyle{\color{blue}{\sqrt{\left(8+\frac{1}{10}\right)^2+\left(10+\frac{1}{8}\right)\sdot\sqrt{\left(8+\frac{1}{10}\right)^2}}={\color{red}{12+\frac{1}{10}+\left(\frac{1}{2}\sdot\frac{1}{10}\right)}}=\left(1+\frac{1}{2}\right)\sdot\left(8+\frac{1}{10}\right)}}</math> | ||
|- | |- | ||
| − | | | + | |And so on for any addition you want in this way, when you assume an addition that relates to the question, as if we say that the ratio of the question is that its six roots are the same as two roots and their half, more and more are being added endlessly. |
| − | |style="text-align:right;"|וכן ימשכו כל אשר תרצה מההוספות כמו זה האופן כאשר תניח התוספת מתיחס לשאלה כאלו אמרנו שיחס השאלה הוא שששת שרשיו הם כמו שני השרשים וכמו חצים והולכים ומתוספים עד אין להם סוף | + | |style="text-align:right;"|וכן ימשכו כל אשר תרצה מההוספות כמו זה האופן כאשר תניח התוספת מתיחס לשאלה ‫<ref>74v</ref>כאלו אמרנו שיחס השאלה הוא שששת שרשיו הם כמו שני השרשים וכמו חצים והולכים ומתוספים עד אין להם סוף |
|- | |- | ||
| | | | ||
| − | :28) <math>\scriptstyle\begin{cases}\scriptstyle x^2+3x=n^2\\\scriptstyle x^2+3x+6\sqrt{x^2+3x}=m^2\end{cases}</math> | + | :{{#annot:x²+3x=n²,x²+3x+6√(x²+3x)=m²|1124|RKqA}}28) If you are told: a square that has a root, if you add its three roots to it, it has a root; and if you add to the sum its six roots, it has a root. |
| + | :<math>\scriptstyle\begin{cases}\scriptstyle x^2+3x=n^2\\\scriptstyle x^2+3x+6\sqrt{x^2+3x}=m^2\end{cases}</math> | ||
|style="text-align:right;"|כח <big>וכן</big> אם יאמרו לך אלגו המחזיק שרש אם תוסיף עליו שלשת שרשיו יחזיק שרש<br> | |style="text-align:right;"|כח <big>וכן</big> אם יאמרו לך אלגו המחזיק שרש אם תוסיף עליו שלשת שרשיו יחזיק שרש<br> | ||
| − | ואם תקבץ עם העולה ששת שרשיו יחזיק שרש | + | ואם תקבץ עם העולה ששת שרשיו יחזיק שרש{{#annotend:RKqA}} |
|- | |- | ||
| | | | ||
| − | : | + | :Its procedure is that you assume your square is a square [<math>\scriptstyle{\color{blue}{x^2}}</math>]. |
| − | |||
|style="text-align:right;"|מלאכתו שתניח האלגו שלך אלגו | |style="text-align:right;"|מלאכתו שתניח האלגו שלך אלגו | ||
|- | |- | ||
| | | | ||
| − | : | + | :Add its three roots to it; it is a square and three roots and its root is two things. |
| − | |style="text-align:right;"|תוסיף עליו שלשת שרשיו ויהיה | + | :<math>\scriptstyle{\color{blue}{\sqrt{x^2+3x}=2x}}</math> |
| + | |style="text-align:right;"|תוסיף עליו שלשת שרשיו ויהיה אלגו ושלשה שרשים ושרשו שני דברים | ||
|- | |- | ||
| | | | ||
| − | : | + | :The reason we assumed its root is two things is that six [things] are the same as three roots. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{3\sqrt{x^2+3x}=6x}}</math> |
| + | |style="text-align:right;"|והסבה בהניחנו שרשו שני <s>שרשים</s> דברים הוא בעבור כי ששת השרשים כמו שלשה שרשים | ||
|- | |- | ||
| | | | ||
| − | : | + | :Multiply two things by two things; it is four squares equal a square and three things. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{2x\sdot2x=4x^2=x^2+3x}}</math> |
| + | |style="text-align:right;"|ותכה שני דברים בשני דברים ויהיה ארבעה אלגוש ישוו אלגו ושלשה דברים | ||
|- | |- | ||
| | | | ||
| − | ::: | + | :*<span style="color:Green>Confrontation:</span> |
| − | |||
| − | |||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Confront them; the thing is equal to one dirham and this is the root of the square. |
| + | :<math>\scriptstyle{\color{blue}{x=\sqrt{x^2}=1}}</math> | ||
|style="text-align:right;"|ותכונהו עמו ויהיה הדבר ישוה דרהם אחד והוא שרש האלגו | |style="text-align:right;"|ותכונהו עמו ויהיה הדבר ישוה דרהם אחד והוא שרש האלגו | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x^2=1}}</math> | + | :The square is one dirham. |
| + | :<math>\scriptstyle{\color{blue}{x^2=1}}</math> | ||
|style="text-align:right;"|והאלגו דרהם אחד | |style="text-align:right;"|והאלגו דרהם אחד | ||
|- | |- | ||
| − | | | + | |And so on for any addition you want according to the ratio of the question: |
|style="text-align:right;"|וכמו זה ימשך מה שתרצה מההוספות כפי יחס השאלה | |style="text-align:right;"|וכמו זה ימשך מה שתרצה מההוספות כפי יחס השאלה | ||
|- | |- | ||
| | | | ||
| + | *We say that when you add to the sum [its] twelve roots, it has a root. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{X+12\sqrt{X}=n^2}}</math> | ||
|style="text-align:right;"|נאמר כי כאשר תוסיף על מה שהתקבץ שנים עשר שרשים יחזיק שרש | |style="text-align:right;"|נאמר כי כאשר תוסיף על מה שהתקבץ שנים עשר שרשים יחזיק שרש | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| + | *If you add to the sum its root, it has a root. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{X+\sqrt{X}=n^2}}</math> | ||
|style="text-align:right;"|ואם תוסיף על המקובץ שרשו יחזיק שרש | |style="text-align:right;"|ואם תוסיף על המקובץ שרשו יחזיק שרש | ||
|- | |- | ||
| | | | ||
| + | *If you add to this sum its twenty-four roots, the sum has a root. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{X+24\sqrt{X}=n^2}}</math> | ||
|style="text-align:right;"|וכאשר תוסיף על זה המקובץ עשרי' וארבעה שרשיו יחזיק המתקבץ שרש | |style="text-align:right;"|וכאשר תוסיף על זה המקובץ עשרי' וארבעה שרשיו יחזיק המתקבץ שרש | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle+48\sqrt{ | + | *If you add 48 roots, it has a root. |
| − | |style="text-align:right;"|ואם תוסיף מ"ח שרשים יחזיק | + | :<math>\scriptstyle{\color{OliveGreen}{X+48\sqrt{X}=n^2}}</math> |
| + | |style="text-align:right;"|ואם תוסיף מ"ח שרשים יחזיק שרש | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle+96\sqrt{ | + | *If you add 96 roots, it has a root. |
| + | :<math>\scriptstyle{\color{OliveGreen}{X+96\sqrt{X}=n^2}}</math> | ||
|style="text-align:right;"|ואם תוסיף צ"ו שרשים יחזיק שרש | |style="text-align:right;"|ואם תוסיף צ"ו שרשים יחזיק שרש | ||
|- | |- | ||
| − | | | + | |Whichever you have of this species, when the secondary roots are greater than the primary roots, is solved by this procedure. |
| − | |style="text-align:right;"|וכמעשה הזה יעלו כל מה שיפלו לפניך מזה המין כאשר יניחו השרשים השניים יותר מהראשונים | + | |style="text-align:right;"|וכמעשה הזה יעלו [כל מה]‫<ref>Ma.: כמה</ref> שיפלו לפניך מזה המין כאשר יניחו השרשים השניים יותר מהראשונים |
|- | |- | ||
| − | | | + | |However, if they are less than the primary roots they are not solved by this procedure, but they have other methods by which they are solved as follows: |
| − | |style="text-align:right;"|ואם יונחו פחות מהראשונים לא יצאו עם זה המעשה | + | |style="text-align:right;"|ואם יונחו פחות מהראשונים לא יצאו עם זה המעשה אבל להם דרכים אחרים יצאו בהם והם אלו |
|- | |- | ||
| | | | ||
| − | | | + | :{{#annot:x²+2x=n²,x²+2x+√(x²+2x)=m²|1124|Csxg}}29) If you are told: a square that has a root, if you add two roots to it, it has a root; and if you add to the sum its root, it has a root. |
| − | + | :<math>\scriptstyle\begin{cases}\scriptstyle x^2+2x=n^2\\\scriptstyle x^2+2x+\sqrt{x^2+2x}=m^2\end{cases}</math> | |
| − | |||
| − | : | ||
|style="text-align:right;"|כט <big>כאשר</big> יאמרו לך אלגו המחזיק שרש אם תוסיף עליו שני שרשים יחזיק שרש<br> | |style="text-align:right;"|כט <big>כאשר</big> יאמרו לך אלגו המחזיק שרש אם תוסיף עליו שני שרשים יחזיק שרש<br> | ||
| − | ואם תוסיף על המקובץ שרשו יחזיק שרש | + | ואם תוסיף על המקובץ שרשו יחזיק שרש{{#annotend:Csxg}} |
|- | |- | ||
| | | | ||
| − | :To | + | :To know this, assume your square is a square [<math>\scriptstyle{\color{blue}{x^2}}</math>]. |
|style="text-align:right;"|ולידיעתו תניח האלגו שלך אלגו | |style="text-align:right;"|ולידיעתו תניח האלגו שלך אלגו | ||
|- | |- | ||
| | | | ||
| − | : | + | :Add its two roots to it; it is a square and two roots [<math>\scriptstyle{\color{blue}{x^2+2x}}</math>]. |
| − | |style="text-align:right;"|ותוסיף עליו שני שרשיו ויהיה אלגו ושני שרשי' | + | |style="text-align:right;"|ותוסיף עליו שני שרשיו ויהיה אלגו ושני שרשי‫' |
|- | |- | ||
| | | | ||
| − | : | + | :Add to the sum its root, which is a root of a square and two roots; it is a square and two roots plus a root of a square and two roots [<math>\scriptstyle{\color{blue}{x^2+2x+\sqrt{x^2+2x}}}</math>], which should have a root. |
|style="text-align:right;"|ותוסיף על הנקבץ שרשו והוא שרש האלגו ושני שרשים ויהיה אלגו ושני שרשי' ושרש האלגו ושני שרשים וצריך שיחזיק שרש | |style="text-align:right;"|ותוסיף על הנקבץ שרשו והוא שרש האלגו ושני שרשים ויהיה אלגו ושני שרשי' ושרש האלגו ושני שרשים וצריך שיחזיק שרש | ||
|- | |- | ||
| | | | ||
| − | : | + | :We suppose its root is a thing and half a dirham [<math>\scriptstyle{\color{blue}{\sqrt{x^2+2x+\sqrt{x^2+2x}}=x+\frac{1}{2}}}</math>]. |
|style="text-align:right;"|ונניח שרשו דבר וחצי דרהם | |style="text-align:right;"|ונניח שרשו דבר וחצי דרהם | ||
|- | |- | ||
| | | | ||
| − | :Multiply | + | :Multiply it by itself; it is a square and a thing plus a quarter of a dirham equal a square and two things plus a root of a square and two roots. |
:<math>\scriptstyle{\color{blue}{\left(x+\frac{1}{2}\right)^2=x^2+x+\frac{1}{4}=x^2+2x+\sqrt{x^2+2x}}}</math> | :<math>\scriptstyle{\color{blue}{\left(x+\frac{1}{2}\right)^2=x^2+x+\frac{1}{4}=x^2+2x+\sqrt{x^2+2x}}}</math> | ||
|style="text-align:right;"|ותכהו בעצמו ויהיה אלגו ודבר ורביעית דרהם ישוה אלגו ושני דברים ושרש מאלגו ושני שרשים | |style="text-align:right;"|ותכהו בעצמו ויהיה אלגו ודבר ורביעית דרהם ישוה אלגו ושני דברים ושרש מאלגו ושני שרשים | ||
|- | |- | ||
| | | | ||
| − | : | + | :Subtract a square and a root from a square and two roots; a root and a root of a square and two roots remain equal to a quarter of a dirham. |
| − | |style="text-align:right;"|ותגרע אלגו ושרש מאלגו ושני שרשים | + | |style="text-align:right;"|ותגרע אלגו ושרש מאלגו ושני שרשים וישאר שרש ושרש מאלגו ושני שרשים ישוו רביעית דרהם |
|- | |- | ||
| − | | | + | |colspan=2| |
| − | + | :<math>\scriptstyle{\color{blue}{x^2+2x-\left(x^2+x\right)+\sqrt{x^2+2x}=x^2+x-\left(x^2+x\right)+\frac{1}{4}\longrightarrow x+\sqrt{x^2+2x}=\frac{1}{4}}}</math> | |
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Subtract a root from a quarter of a dirham; a quarter of a dirham minus a root remain equal to a root of a square and two roots. |
| − | |style="text-align:right;"|ותגרע שרש מרביעית דרהם | + | |style="text-align:right;"|ותגרע שרש ‫<ref>75r</ref>מרביעית דרהם ישאר רביעית דרהם פחות שרש ישוה שרש מאלגו ושני שרשי‫' |
|- | |- | ||
| − | | | + | |colspan=2| |
| − | + | :<math>\scriptstyle{\color{blue}{x-x+\sqrt{x^2+2x}=\frac{1}{4}-x\longrightarrow\frac{1}{4}-x=\sqrt{x^2+2x}}}</math> | |
| − | |||
|- | |- | ||
| | | | ||
| − | :Multiply a quarter of a dirham minus a root by | + | :Multiply a quarter of a dirham minus a root by itself; it is one part of 16 plus a square minus half a thing equal a square and two roots. |
:<math>\scriptstyle{\color{blue}{\left(\frac{1}{4}-x\right)^2=\frac{1}{16}+x^2-\frac{1}{2}x=x^2+2x}}</math> | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{4}-x\right)^2=\frac{1}{16}+x^2-\frac{1}{2}x=x^2+2x}}</math> | ||
|style="text-align:right;"|ותכה רביעית דרהם פחות שרש בעצמו ויהיה חלק מי"ו ואלגו פחות חצי דבר ישוה אלגו ושני שרשים | |style="text-align:right;"|ותכה רביעית דרהם פחות שרש בעצמו ויהיה חלק מי"ו ואלגו פחות חצי דבר ישוה אלגו ושני שרשים | ||
|- | |- | ||
| | | | ||
| − | :: | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Confront them; the root is a quarter of a tenth. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\frac{1}{4}\sdot\frac{1}{10}}}</math> | ||
|style="text-align:right;"|ותכונהו עמו ויהיה השרש רביעית העשירית | |style="text-align:right;"|ותכונהו עמו ויהיה השרש רביעית העשירית | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x^2=\frac{1}{1600}}}</math> | + | :The square is one part of 1600. |
| + | :<math>\scriptstyle{\color{blue}{x^2=\frac{1}{1600}}}</math> | ||
|style="text-align:right;"|והאלגו חלק אחד מאלף ות"ר | |style="text-align:right;"|והאלגו חלק אחד מאלף ות"ר | ||
|- | |- | ||
| | | | ||
| − | :We | + | :We assumed that the root is a thing and half a dirham [<math>\scriptstyle{\color{blue}{x+\frac{1}{2}}}</math>], so that the square will be consumed and the things remain equal to numbers. |
|style="text-align:right;"|והנחנו השרש דבר וחצי דרהם כדי שישחתו האלגוש וישארו דברים ישוו למספרים | |style="text-align:right;"|והנחנו השרש דבר וחצי דרהם כדי שישחתו האלגוש וישארו דברים ישוו למספרים | ||
|- | |- | ||
| | | | ||
| − | :30) <math>\scriptstyle\begin{cases}\scriptstyle x^2+4x=n^2\\\scriptstyle x^2+4x+2\sqrt{x^2+4x}=m^2\end{cases}</math> | + | :{{#annot:x²+4x=n²,x²+4x+2√(x²+4x)=m²|1124|2scj}}30) If you are told: a square that has a root, if you add its four roots to it, it has a root; and if you add to the sum its two roots, it has a root. |
| + | :<math>\scriptstyle\begin{cases}\scriptstyle x^2+4x=n^2\\\scriptstyle x^2+4x+2\sqrt{x^2+4x}=m^2\end{cases}</math> | ||
|style="text-align:right;"|ל <big>וכן</big> אם יאמרו לך אלגו המחזיק שרש<br> | |style="text-align:right;"|ל <big>וכן</big> אם יאמרו לך אלגו המחזיק שרש<br> | ||
אם תוסיף עליו ארבעת שרשיו יחזיק שרש<br> | אם תוסיף עליו ארבעת שרשיו יחזיק שרש<br> | ||
| − | ואם תוסיף על המתקבץ שני שרשיו יחזיק שרש | + | ואם תוסיף על המתקבץ שני שרשיו יחזיק שרש{{#annotend:2scj}} |
|- | |- | ||
| | | | ||
| Line 2,547: | Line 2,811: | ||
|- | |- | ||
| | | | ||
| − | : | + | :Add to it its four roots; it is a square and four things [<math>\scriptstyle{\color{blue}{x^2+4x}}</math>]. |
| − | |style="text-align:right;"|ותוסיף עליו ארבעת שרשיו ויהיה אלגו וארבעה דברים | + | |style="text-align:right;"|ותוסיף עליו ארבעת שרשיו ויהיה אלגו וארבעה <s>שרשים</s> <sup>דברים</sup> |
|- | |- | ||
| | | | ||
| − | : | + | :Add to the square and four things its two roots, which is a root of four squares and 16 things; it is a square and four things plus a root of four squares and 16 things [<math>\scriptstyle{\color{blue}{x^2+4x+2\sqrt{x^2+4x}=x^2+4x+\sqrt{4x^2+16x}}}</math>]. |
| − | |style="text-align:right;"|ותוסיף על אלגו וארבעה דברים שני שרשיו ושרש ארבעה אלגוש וי"ו דברים ויהיה אלגו וארבעה דברים ושרש מארבעה אלגוש וי"ו דברים | + | |style="text-align:right;"|ותוסיף על אלגו וארבעה דברים שני שרשיו ושרש ארבעה אלגוש וי"ו דברים ויהיה אלגו וארבעה דברים ושרש <s>מאלג</s> מארבעה אלגוש וי"ו דברים |
|- | |- | ||
| | | | ||
| − | : | + | :Subtract a square and two things from a square and four things; one dirham remains equal to two things and a root of four squares and 16 things. |
| − | |style="text-align:right;"|ותגרע | + | |style="text-align:right;"|ותגרע אלגו ושני דברים מאלגו וארבעה דברים וישאר דרהם אחד ישוה שני דברים ושרש מארבעה אלגוש וי"ו דברים |
|- | |- | ||
| − | | | + | |colspan=2| |
| − | + | :<math>\scriptstyle{\color{blue}{x^2+2x+1-\left(x^2+2x\right)=x^2+4x-\left(x^2+2x\right)+\sqrt{4x^2+16x}\longrightarrow1=2x+\sqrt{4x^2+16x}}}</math> | |
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Subtract the two things from the dirham; one dirham minus two things remain equal to a root of four squares and 16 things. |
| − | |style="text-align:right;"|ותגרע שני הדברים מהדרהם | + | |style="text-align:right;"|ותגרע שני הדברים מהדרהם וישאר דרהם אחד פחות שני דברים ישוה שרש מארבעה אלגוש וי"ו דברים |
|- | |- | ||
| − | | | + | |colspan=2| |
| − | + | :<math>\scriptstyle{\color{blue}{1-2x=2x-2x+\sqrt{4x^2+16x}\longrightarrow1-2x=\sqrt{4x^2+16x}}}</math> | |
| − | |||
|- | |- | ||
| | | | ||
:Multiply one dirham minus two things by themselves; they are one dirham plus four squares minus four roots equal four squares and 16 things. | :Multiply one dirham minus two things by themselves; they are one dirham plus four squares minus four roots equal four squares and 16 things. | ||
:<math>\scriptstyle{\color{blue}{\left(1-2x\right)^2=1+4x^2-4x=4x^2+16x}}</math> | :<math>\scriptstyle{\color{blue}{\left(1-2x\right)^2=1+4x^2-4x=4x^2+16x}}</math> | ||
| − | |style="text-align:right;"|ותכה דרהם אחד פחות שני דברים בעצמו ויהיה דרהם אחד וארבעה אלגוש פחות ארבעה שרשים ישוה ארבעה אלגוש וי"ו דברים | + | |style="text-align:right;"|ותכה דרהם אחד פחות שני דברים בעצמו ויהיה דרהם אחד וארבעה אלגוש פחות ארבעה שרשים ישוה ארבעה אלגוש <s>פחות ארבעה שרשים</s> וי"ו דברים |
| + | |- | ||
| + | | | ||
| + | :*<span style="color:Green>Confrontation:</span> | ||
| + | | | ||
|- | |- | ||
| | | | ||
| − | : | + | :Confront them; the thing is half a tenth of a dirham. |
| + | :<math>\scriptstyle{\color{blue}{x=\frac{1}{2}\sdot\frac{1}{10}}}</math> | ||
|style="text-align:right;"|ותכוין עמו ויהיה הדבר חצי עשירית מדרהם | |style="text-align:right;"|ותכוין עמו ויהיה הדבר חצי עשירית מדרהם | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x^2=\frac{1}{400}}}</math> | + | :The square is one part of four hundred. |
| + | :<math>\scriptstyle{\color{blue}{x^2=\frac{1}{400}}}</math> | ||
|style="text-align:right;"|והאלגו חלק אחד מארבע מאות | |style="text-align:right;"|והאלגו חלק אחד מארבע מאות | ||
|- | |- | ||
| − | |Whichever of this species that you have, apply the procedure on its root, until you find it by | + | |Whichever of this species that you have, apply the procedure on its root, until you find it by confrontation, when it remains that things are equal to numbers. |
|style="text-align:right;"|וכל אשר יפול בידך מזה המין תעשה מלאכה בשרשו עד שכאשר מצאת אותו עם הכוון ישאר דברים ישוו מספרים | |style="text-align:right;"|וכל אשר יפול בידך מזה המין תעשה מלאכה בשרשו עד שכאשר מצאת אותו עם הכוון ישאר דברים ישוו מספרים | ||
|- | |- | ||
| Line 2,590: | Line 2,858: | ||
|- | |- | ||
| | | | ||
| − | :31) <math>\scriptstyle\begin{cases}\scriptstyle x^2-4x=n^2\\\scriptstyle x^2-4x-2\sqrt{x^2-4x}=m^2\end{cases}</math> | + | :{{#annot:x²-4x=n²,x²-4x-2√(x²-4x)=m²|1124|vAyv}}31) If you are told: a square, if you subtract its four roots from it, it has a root; and if you subtract from the remainder its two roots, it has a root. |
| − | |style="text-align:right;"|לא <big>ואם</big> יאמרו לך אלגו אם תגרע ממנו ארבעה שרשיו יחזיק שרש<br> | + | :<math>\scriptstyle\begin{cases}\scriptstyle x^2-4x=n^2\\\scriptstyle x^2-4x-2\sqrt{x^2-4x}=m^2\end{cases}</math> |
| − | ואם תגרע מהנשאר שני שרשיו יחזיק שרש | + | |style="text-align:right;"|לא <big>ואם</big> יאמרו לך אלגו אם <s>תוסיף עליו</s> <sup>תגרע ממנו</sup> ארבעה שרשיו יחזיק שרש<br> |
| + | ואם תגרע ואם תגרע מהנשאר שני שרשיו יחזיק שרש{{#annotend:vAyv}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :Knowing this is that you assume your square is a square [<math>\scriptstyle{\color{blue}{x^2}}</math>]. |
|style="text-align:right;"|ולידיעת זה תניח האלגו שלך אלגו | |style="text-align:right;"|ולידיעת זה תניח האלגו שלך אלגו | ||
|- | |- | ||
| | | | ||
| − | : | + | :Subtract its four roots from it; a square minus four things remain and it has a root, which is half a thing [<math>\scriptstyle{\color{blue}{x^2-4x=\left(\frac{1}{2}x\right)^2}}</math>]. |
| − | |style="text-align:right;"|ותגרע ממנו ארבעה שרשיו וישאר אלגו פחות ארבעה דברים | + | |style="text-align:right;"|ותגרע ממנו ארבעה שרשיו וישאר אלגו פחות ארבעה דברים ויחזיק שרש והוא חצי דבר |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{ | + | :The reason I have assumed it is half a thing is that two roots are half of four. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{2x=\frac{1}{2}\sdot4x}}</math> |
| + | |style="text-align:right;"|והסבה למה הנחתי חצי הדבר בעבור כי היו שני שרשים חצי הארבעה | ||
|- | |- | ||
| | | | ||
| − | : | + | :Multiply half a square by itself; it is a quarter of a square equals a square minus four [things]. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}x\right)^2=\frac{1}{4}x^2=x^2-4{\color{red}{x}}}}</math> |
| + | |style="text-align:right;"|ותכה חצי דבר בעצמו ויהיה רביע אלגו ישוה אלגו ‫<ref>75v</ref>פחות ארבעה אלגוש | ||
|- | |- | ||
| | | | ||
| − | : | + | :*<span style="color:Green>Confrontation:</span> |
| − | + | | | |
| − | | | ||
|- | |- | ||
| | | | ||
| − | : | + | :Confront them; the root of the square is five and a third. |
| + | :<math>\scriptstyle{\color{blue}{x=\sqrt{x^2}=5+\frac{1}{3}}}</math> | ||
|style="text-align:right;"|ותכונהו עמו ויהיה שרש האלגו חמשה ושליש | |style="text-align:right;"|ותכונהו עמו ויהיה שרש האלגו חמשה ושליש | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x^2=28+\frac{4}{9}}}</math> | + | :The square is twenty-eight and four-ninths. |
| + | :<math>\scriptstyle{\color{blue}{x^2=28+\frac{4}{9}}}</math> | ||
|style="text-align:right;"|והאלגו עשרים ושמנה וארבעה תשיעיות | |style="text-align:right;"|והאלגו עשרים ושמנה וארבעה תשיעיות | ||
|- | |- | ||
| − | |The ratio of the roots in this question and its similar is treated as in the preceding question. | + | |The ratio of the roots in this question and its similar is treated as treated in the preceding question. |
|style="text-align:right;"|וזאת השאלה והדומים אליה יתנהג בה יחס השרשים כאשר נהגנו בשאלה שקדמה לה | |style="text-align:right;"|וזאת השאלה והדומים אליה יתנהג בה יחס השרשים כאשר נהגנו בשאלה שקדמה לה | ||
|- | |- | ||
| − | |We say that when you subtract from the remainder its root, it has a root; and if you subtract from that remainder half | + | |We say that when you subtract from the remainder its root, it has a root; and if you subtract from that remainder half its root, it has a root; then a quarter of its root; an eighth of its root; half the eighth of its root - each of the subtracted numbers are half the numbers that precede it, for two roots are half of four roots [<math>\scriptstyle{\color{blue}{2a=\frac{1}{2}\sdot4a}}</math>]. |
|style="text-align:right;"|נאמר כי כאשר תגרע מהנשאר שרשו יחזיק שרש ואם תגרע מהנשאר חצי שרשו יחזיק שרש וכן רביעית שרשו ושמינית שרשו וחצי שמינית שרשו כל אחד מאלו המספרים הנגרעים חצי המספרים הנגרעים שהם קודמים אליו בעבור כי שני השרשים חצי הארבעה השרשים | |style="text-align:right;"|נאמר כי כאשר תגרע מהנשאר שרשו יחזיק שרש ואם תגרע מהנשאר חצי שרשו יחזיק שרש וכן רביעית שרשו ושמינית שרשו וחצי שמינית שרשו כל אחד מאלו המספרים הנגרעים חצי המספרים הנגרעים שהם קודמים אליו בעבור כי שני השרשים חצי הארבעה השרשים | ||
|- | |- | ||
| Line 2,633: | Line 2,905: | ||
|- | |- | ||
| | | | ||
| − | :32) <math>\scriptstyle\begin{cases}\scriptstyle x^2+x=n^2\\\scriptstyle x^2+1=m^2\end{cases}</math> | + | :{{#annot:x²+x=n²,x²+1=m²|1124|eNRx}}32) If you are told: a square that has a root, if you add its root to it, it has a root; and if you add to one dirham to it, it has a root. |
| + | :<math>\scriptstyle\begin{cases}\scriptstyle x^2+x=n^2\\\scriptstyle x^2+1=m^2\end{cases}</math> | ||
|style="text-align:right;"|לב <big>ואם</big> יאמרו לך אלגו המחזיק שרש אם תוסיף עליו שרשו יחזיק שרשו<br> | |style="text-align:right;"|לב <big>ואם</big> יאמרו לך אלגו המחזיק שרש אם תוסיף עליו שרשו יחזיק שרשו<br> | ||
| − | ואם תוסיף עליו דרהם אחד יחזיק שרש | + | ואם תוסיף עליו דרהם אחד יחזיק שרש{{#annotend:eNRx}} |
|- | |- | ||
| | | | ||
| Line 2,642: | Line 2,915: | ||
|- | |- | ||
| | | | ||
| − | : | + | :Add its root to it; it is a square plus a thing [<math>\scriptstyle{\color{blue}{x^2+x}}</math>], and it has a root. |
| − | |style="text-align:right;"|ותוסיף עליו שרשו ויהיה אלגו ודבר ויחזיק | + | |style="text-align:right;"|ותוסיף עליו שרשו ויהיה אלגו ודבר ויחזיק שרש |
|- | |- | ||
| | | | ||
| − | : | + | :Add one dirham to the square; it is a square plus one dirham [<math>\scriptstyle{\color{blue}{x^2+1}}</math>], and it has a root. |
| − | |style="text-align:right;"|ותוסיף על האלגו אדרהם אחד ויהיה אלגו ודרהם אחד ויחזיק | + | |style="text-align:right;"|ותוסיף על האלגו אדרהם אחד ויהיה אלגו ודרהם אחד ויחזיק שרש |
|- | |- | ||
| | | | ||
| − | : | + | :Suppose a root of the square plus a thing [<math>\scriptstyle{\color{blue}{\sqrt{x^2+x}}}</math>] and a root of the square and one dirham minus half a dirham [<math>\scriptstyle{\color{blue}{\sqrt{x^2+1}-\frac{1}{2}}}</math>]. |
| − | + | |style="text-align:right;"|ותשוב ותניח שרש האלגו ודבר ושרש מאלגו ודרהם אחד פחות חצי דרהם | |
| − | |||
| − | |||
| − | |||
| − | |style="text-align:right;"|ושרש מאלגו ודרהם אחד פחות חצי דרהם | ||
|- | |- | ||
| | | | ||
| Line 2,665: | Line 2,934: | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{x^2-x^2}}</math> | + | :Subtract a square from a square; one dirham and a quarter minus a root of a square and one dirham remain equal to a root. |
| − | + | |style="text-align:right;"|ותגרע אלגו מאלגו וישאר דרהם אחד ורביע פחות שרש מאלגו ודרהם אחד ישוה שרש | |
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{x^2-x^2+1+\frac{1}{4}-\sqrt{x^2+1}=x^2-x^2+x\longrightarrow1+\frac{1}{4}-\sqrt{x^2+1}=x}}</math> | ||
|- | |- | ||
| | | | ||
| − | :< | + | :*<span style="color:Green>Restoration:</span> |
| − | | | + | | |
|- | |- | ||
| | | | ||
| − | : | + | :Restore the dirham and a quarter with the root of a square and one dirham by adding it to the root [of the square]; it is a root and a root of a square and one dirham equal one dirham and a quarter. |
| − | + | |style="text-align:right;"|ותאסוף האדרהם ורביע עם עם שרש מאלגו ודרהם אחד ותוסיפם על השרש ויהיה שרש ושרש מאלגו ודרהם אחד ישוה דרהם ורביע | |
| − | |style="text-align:right;"|ותאסוף האדרהם ורביע עם שרש מאלגו ודרהם אחד ותוסיפם על השרש | ||
|- | |- | ||
| − | | | + | |colspan=2| |
| − | :<math>\scriptstyle{\color{blue}{x+\sqrt{x^2+1}=1+\frac{1}{4}}}</math> | + | :<math>\scriptstyle{\color{blue}{1+\frac{1}{4}-\sqrt{x^2+1}+\sqrt{x^2+1}=x+\sqrt{x^2+1}\longrightarrow x+\sqrt{x^2+1}=1+\frac{1}{4}}}</math> |
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Subtract the root from one dirham and a quarter; one dirham and a quarter minus a thing remain equal to a root of a square and one dirham. |
| − | |style="text-align:right;"|ותגרע השרש מדרהם ורביע | + | |style="text-align:right;"|ותגרע השרש מדרהם ורביע ישאר דרהם ורביע פחות דבר ישוה שרש מאלגו ודרהם אחד |
|- | |- | ||
| − | | | + | |colspan=2| |
| − | :<math>\scriptstyle{\color{blue}{\left(1+\frac{1}{4}\right)-x=\sqrt{x^2+1}}}</math> | + | :<math>\scriptstyle{\color{blue}{x-x+\sqrt{x^2+1}=1+\frac{1}{4}-x\longrightarrow\left(1+\frac{1}{4}\right)-x=\sqrt{x^2+1}}}</math> |
| − | |||
|- | |- | ||
| | | | ||
| Line 2,697: | Line 2,966: | ||
|- | |- | ||
| | | | ||
| − | : | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Confront them; the thing equals nine parts of forty and this is the root of the square. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\sqrt{x^2}=\frac{9}{40}}}</math> | ||
|style="text-align:right;"|ותכונהו עמהם ויהיה הדבר ישוה תשעה חלקים מארבעים והוא שרש האלגו | |style="text-align:right;"|ותכונהו עמהם ויהיה הדבר ישוה תשעה חלקים מארבעים והוא שרש האלגו | ||
|- | |- | ||
| | | | ||
| + | :The square is 81 parts of 1600. | ||
:<math>\scriptstyle{\color{blue}{x^2=\frac{81}{1600}}}</math> | :<math>\scriptstyle{\color{blue}{x^2=\frac{81}{1600}}}</math> | ||
|style="text-align:right;"|והאלגו פ"א חלקים מאלף ות"ר | |style="text-align:right;"|והאלגו פ"א חלקים מאלף ות"ר | ||
|- | |- | ||
| | | | ||
| − | : | + | :We have assumed its root is a square and one dirham minus half a dirham [<math>\scriptstyle{\color{blue}{\sqrt{x^2+1}-\frac{1}{2}}}</math>] so that after the confrontation the roots will be equal to [dirham]. |
|style="text-align:right;"|והנחנו שרשו אלגו ודרהם פחות חצי דרהם למען ישאר אחר הכוון שרשים ישוו לדרהם אחד | |style="text-align:right;"|והנחנו שרשו אלגו ודרהם פחות חצי דרהם למען ישאר אחר הכוון שרשים ישוו לדרהם אחד | ||
|- | |- | ||
| | | | ||
| − | :33) <math>\scriptstyle\begin{cases}\scriptstyle x^2-5=n^2\\\scriptstyle x^2-5+\sqrt{x^2-5}=m^2\end{cases}</math> | + | :{{#annot:x²-5=n²,x²-5+√(x²-5)=m²|1124|6x8t}}33) If you are told: a square that has a root, if you subtract five dirham from it, the remainder has a root; and if you add to the remainder its root, the sum has a root. |
| + | :<math>\scriptstyle\begin{cases}\scriptstyle x^2-5=n^2\\\scriptstyle x^2-5+\sqrt{x^2-5}=m^2\end{cases}</math> | ||
|style="text-align:right;"|לג <big>ואם</big> יאמרו לך אלגו המחזיק שרש אם תגרע ממנו חמשה דרהמי יחזיק הנשאר שרש<br> | |style="text-align:right;"|לג <big>ואם</big> יאמרו לך אלגו המחזיק שרש אם תגרע ממנו חמשה דרהמי יחזיק הנשאר שרש<br> | ||
| − | ואם תוסיף על הנשאר שרשו יחזיק המקובץ שרש | + | ואם תוסיף על הנשאר שרשו יחזיק המקובץ שרש{{#annotend:6x8t}} |
|- | |- | ||
| | | | ||
:Knowing this is that you assume your square is a square [<math>\scriptstyle{\color{blue}{x^2}}</math>]. | :Knowing this is that you assume your square is a square [<math>\scriptstyle{\color{blue}{x^2}}</math>]. | ||
| − | |style="text-align:right;"|וידיעתו שתניח האלגו שלך אלגו | + | |style="text-align:right;"|‫<ref>76r</ref>וידיעתו שתניח האלגו שלך אלגו |
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{x^2-5}}</math> | + | :Subtract five dirham from it; a square minus five dirham [<math>\scriptstyle{\color{blue}{x^2-5}}</math>] remain. |
|style="text-align:right;"|ותגרע ממנו חמשה דרהמי וישאר אלגו פחות חמשה דרהמי | |style="text-align:right;"|ותגרע ממנו חמשה דרהמי וישאר אלגו פחות חמשה דרהמי | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{x^2-5+\sqrt{x^2-5}}}</math> | + | :Add to the square minus five dirham its root, which is a root of a square minus five dirham; it is a square minus five dirham plus a root of a square minus five dirham [<math>\scriptstyle{\color{blue}{x^2-5+\sqrt{x^2-5}}}</math>]. |
| − | |style="text-align:right;"|ותוסיף על אלגו פחות חמשה דרהמי שרשו והוא שרש האלגו פחות חמשה דרהמי ויהיה אלגו פחות חמשה דרהמי ושרש מהאלגו פחות חמשה דרהמי | + | |style="text-align:right;"|ותוסיף על אלגו פחות חמשה <s>שרשים</s> דרהמי שרשו והוא שרש האלגו פחות חמשה דרהמי ויהיה אלגו פחות חמשה דרהמי ושרש מהאלגו פחות חמשה דרהמי |
|- | |- | ||
| | | | ||
| − | : | + | :Suppose its root is a thing [minus half dirham]. [<math>\scriptstyle{\color{blue}{\sqrt{x^2-5+\sqrt{x^2-5}}=x{\color{red}{-\frac{1}{2}}}}}</math>] |
|style="text-align:right;"|ותניח שרשו דבר | |style="text-align:right;"|ותניח שרשו דבר | ||
|- | |- | ||
| Line 2,735: | Line 3,011: | ||
|- | |- | ||
| | | | ||
| − | : | + | :*<span style="color:Green>Restoration:</span> |
| − | : | + | | |
| − | | | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{\left(5+\frac{1}{4}\right){\color{red}{-x}}=\sqrt{x^2-5}}}</math> | + | :Restore the square and the root with five; add the five to the square and the quarter of a dirham [minus a thing]; it is five dirham and a quarter [minus a thing] equal a root of a square minus five dirham. |
| − | + | |style="text-align:right;"|ותאסוף זה האלגו עם החמשה וזה יהיה עם השרש ותוסיף החמשה על אלגו ורביע דרהם והשרש אלגו ויהיה השרש אלגו וחמשה דרהמי ורביע מהדברים ישוה שרש האלגו פחות חמשה דרהמי | |
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{x^2+\frac{1}{4}+5-x=x^2-5+5+\sqrt{x^2-5}\longrightarrow\left(5+\frac{1}{4}\right){\color{red}{-x}}=\sqrt{x^2-5}}}</math> | ||
|- | |- | ||
| | | | ||
| Line 2,751: | Line 3,029: | ||
|- | |- | ||
| | | | ||
| − | : | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Confront them; the thing is equal to three dirham and seventeen parts of 1[68] and this is the root of the square. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\sqrt{x^2}=3+\frac{17}{1{\color{red}{68}}}}}</math> | ||
|style="text-align:right;"|ותכוין עמהם ויהיה הדבר ישוה שלשה דרהמי ושבעה עשר חלקים מקפ"ט והוא שרש האלגו | |style="text-align:right;"|ותכוין עמהם ויהיה הדבר ישוה שלשה דרהמי ושבעה עשר חלקים מקפ"ט והוא שרש האלגו | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x^2=9+\frac{103}{168}+\frac{121}{28224}}}</math> | + | :The square is nine dirham, 103 parts of 168 , and 121 parts of 28224. |
| + | :<math>\scriptstyle{\color{blue}{x^2=9+\frac{103}{168}+\frac{121}{28224}}}</math> | ||
|style="text-align:right;"|והאלגו תשעה דרהמי וק"ג חלקים מקס"ח חלקים וקכ"א חלקים מכ"ח אלפים ורכ"ד חלקים באחד | |style="text-align:right;"|והאלגו תשעה דרהמי וק"ג חלקים מקס"ח חלקים וקכ"א חלקים מכ"ח אלפים ורכ"ד חלקים באחד | ||
|- | |- | ||
| − | |Check: | + | | |
| + | :*<span style="color:Green>'''Check:'''</span> | ||
| + | :When you subtract five from it, four dirham, 103 parts of 168 and 103 parts of 28224 remain. | ||
:<math>\scriptstyle{\color{blue}{x^2-5=4+\frac{103}{168}+\frac{121}{28224}}}</math> | :<math>\scriptstyle{\color{blue}{x^2-5=4+\frac{103}{168}+\frac{121}{28224}}}</math> | ||
|style="text-align:right;"|וכאשר תגרע ממנו חמשה ישאר ארבעה דרהמי וק"ג חלקים מקס"ח וקכ"א חלקים מכ"ח אלפים ורכ"ד | |style="text-align:right;"|וכאשר תגרע ממנו חמשה ישאר ארבעה דרהמי וק"ג חלקים מקס"ח וקכ"א חלקים מכ"ח אלפים ורכ"ד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ותוסיף עליו שרשו שהוא שני דרהמי וכ"ה חלקים מקס"ח וקכ"א חלקים מכ"ח אלפים ורכ"ד ויחזיק | + | :Add to it its root, which is two dirham and [25 parts of 168]; it has a root, which is 2 dirham and 10[1] parts of 168. |
| + | |style="text-align:right;"|ותוסיף עליו שרשו שהוא שני דרהמי וכ"ה חלקים מקס"ח וקכ"א חלקים מכ"ח אלפים ורכ"ד ויחזיק שרש והוא שני דרהמי ומאה דרהמי מקס"ח חלקים | ||
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| Line 2,769: | Line 3,056: | ||
|- | |- | ||
| | | | ||
| − | :34) <math>\scriptstyle\begin{cases}\scriptstyle x^2+4x=n^2\\\scriptstyle x^2-\left(2x+1\right)=m^2\end{cases}</math> | + | :{{#annot:x²+4x=n²,x²-(2x+1)=m²|1124|1vZf}}34) If you are told: a square that has a root, if you add its four roots to it, it has a root; and if you subtract its two roots plus one dirham from it, the remainder has a root. |
| − | |style="text-align:right;"|לד <big>ואם</big> יאמרו לך אלגו שיחזיק | + | :<math>\scriptstyle\begin{cases}\scriptstyle x^2+4x=n^2\\\scriptstyle x^2-\left(2x+1\right)=m^2\end{cases}</math> |
| − | ואם תגרע ממנו שני שרשיו ודרהם אחד יחזיק הנשאר שרש | + | |style="text-align:right;"|לד <big>ואם</big> יאמרו לך אלגו שיחזיק שרש אם תוסיף עליו ארבעת שרשיו יחזיק שרש<br> |
| + | ואם תגרע ממנו שני שרשיו ודרהם אחד יחזיק הנשאר שרש{{#annotend:1vZf}} | ||
|- | |- | ||
| | | | ||
| Line 2,778: | Line 3,066: | ||
|- | |- | ||
| | | | ||
| − | : | + | :Add its four roots to it; it is a square and four roots [<math>\scriptstyle{\color{blue}{x^2+4x}}</math>] that has a root. |
|style="text-align:right;"|ותוסיף עליו ארבעת שרשיו ויהיה אלגו וארבעה שרשי' ויחזיק שרש | |style="text-align:right;"|ותוסיף עליו ארבעת שרשיו ויהיה אלגו וארבעה שרשי' ויחזיק שרש | ||
|- | |- | ||
| | | | ||
| − | : | + | :Subtract from the square its two roots and one dirham; a square minus two roots and one dirham remain [<math>\scriptstyle{\color{blue}{x^2-\left(2x+1\right)}}</math>] and this has a root. |
|style="text-align:right;"|ותגרע מהאלגו שני שרשיו ודרהם אחד וישאר אלגו פחות שני שרשים ודרהם אחד ויחזיק שרש | |style="text-align:right;"|ותגרע מהאלגו שני שרשיו ודרהם אחד וישאר אלגו פחות שני שרשים ודרהם אחד ויחזיק שרש | ||
|- | |- | ||
| | | | ||
| − | : | + | :Suppose its root is a root of a square and four things minus three dirham [<math>\scriptstyle{\color{blue}{\sqrt{x^2+4x}-3}}</math>], as I explained to you. |
|style="text-align:right;"|ותניח שרשו כמו שאבאר לך בכוון שלא יתכן להיות שרש אחד מאלגו וארבעה דברים פחות שלשה דרהמי | |style="text-align:right;"|ותניח שרשו כמו שאבאר לך בכוון שלא יתכן להיות שרש אחד מאלגו וארבעה דברים פחות שלשה דרהמי | ||
|- | |- | ||
| | | | ||
| − | :Multiply | + | :Multiply it by itself; it is a square plus nine dirham and four roots minus six roots of a square and four things equal a square minus two roots and one dirham. |
|style="text-align:right;"|ותכהו בעצמו ויהיה אלגו ותשעה דרהמי וארבעה שרשים פחות ששה שרשים מאלגו וארבעה דברים ישוו אלגו פחות שני שרשים ודרהם אחד | |style="text-align:right;"|ותכהו בעצמו ויהיה אלגו ותשעה דרהמי וארבעה שרשים פחות ששה שרשים מאלגו וארבעה דברים ישוו אלגו פחות שני שרשים ודרהם אחד | ||
|- | |- | ||
| Line 2,797: | Line 3,085: | ||
|- | |- | ||
| | | | ||
| − | :: | + | :*<span style="color:Green>Confrontation:</span> |
| − | | | + | | |
|- | |- | ||
| | | | ||
| − | : | + | :Confront them; it is a square and six roots plus ten dirham equal a square and six roots of a square and four things. |
| − | |style="text-align:right;"|וששה שרשים מאלגו וארבעה | + | :<math>\scriptstyle{\color{blue}{x^2+6x+10=x^2+6\sdot\sqrt{x^2+4x}}}</math> |
| + | |style="text-align:right;"|ותכוין עמו ויהיה אלגו וששה שרשים ועשרה דרהמי ישוה אלגו וששה שרשים מאלגו וארבעה דברים | ||
|- | |- | ||
| | | | ||
| − | : | + | :Six roots of a square and four things is a root of 36 squares and 144 things. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{6\sdot\sqrt{x^2+4x}=\sqrt{36x^2+144x}}}</math> |
| + | |style="text-align:right;"|וששה שרשים מאלגו ‫<ref>76v</ref>וארבעה דברים שרש מל"ו אלגוש וקמ"ד דברים | ||
|- | |- | ||
| | | | ||
| − | : | + | :Subtract a square from a square; six roots and ten dirham remain equal to a root of 36 squares and 144 things. |
| − | |style="text-align:right;"|וישאר ששה שרשים ועשרה דרהמי ישוה שרש מל"ו אלגוש וקמ"ד דברים | + | |style="text-align:right;"|ותגרע אלגו מאלגו וישאר ששה שרשים ועשרה דרהמי ישוה שרש מל"ו אלגוש וקמ"ד דברים |
|- | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{x^2-x^2+6x+10=x^2-x^2+\sqrt{36x^2+144x}\longrightarrow6x+10=\sqrt{36x^2+144x}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :*<span style="color:Green>Confrontation:</span> | ||
| | | | ||
| − | : | + | |- |
| + | | | ||
| + | :Confront them; the thing is equal to four dirham plus a sixth and this is the root of the square. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\sqrt{x^2}=4+\frac{1}{6}}}</math> | ||
|style="text-align:right;"|ותכוין בהם ויהיה הדבר ישוה ארבעה דרהמי ושתות והוא שרש האלגו | |style="text-align:right;"|ותכוין בהם ויהיה הדבר ישוה ארבעה דרהמי ושתות והוא שרש האלגו | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x^2=17+\frac{1}{3}+\left(\frac{1}{4}\sdot\frac{1}{9}\right)}}</math> | + | :The square is seventeen dirham and a quarter of a ninth. |
| + | :<math>\scriptstyle{\color{blue}{x^2=17+\frac{1}{3}+\left(\frac{1}{4}\sdot\frac{1}{9}\right)}}</math> | ||
|style="text-align:right;"|והאלגו שבעה עשר דרהמיש ורביע התשיעית | |style="text-align:right;"|והאלגו שבעה עשר דרהמיש ורביע התשיעית | ||
|- | |- | ||
| | | | ||
| − | :35) <math>\scriptstyle\begin{cases}\scriptstyle x^2-2x=n^2\\\scriptstyle x^2-2x+\sqrt{x^2-2x}=m^2\end{cases}</math> | + | :{{#annot:x²-2x=n²,x²-2x+√(x²-2x)=m²|1124|65Ct}}35) If you are told: a square that has a root, if you subtract its two roots from it, it has a root; and if you add to the remainder its root, it has a root. |
| + | :<math>\scriptstyle\begin{cases}\scriptstyle x^2-2x=n^2\\\scriptstyle x^2-2x+\sqrt{x^2-2x}=m^2\end{cases}</math> | ||
|style="text-align:right;"|לה <big>ואם</big> יאמרו לך אלגו המחזיק שרש אם תגרע ממנו שני שרשיו יחזיק שרש<br> | |style="text-align:right;"|לה <big>ואם</big> יאמרו לך אלגו המחזיק שרש אם תגרע ממנו שני שרשיו יחזיק שרש<br> | ||
| − | ואם תוסיף על הנשאר שרשו יחזיק שרש | + | ואם תוסיף על הנשאר שרשו יחזיק שרש{{#annotend:65Ct}} |
|- | |- | ||
| | | | ||
| Line 2,830: | Line 3,130: | ||
|- | |- | ||
| | | | ||
| − | : | + | :Subtract its two roots from it; a square minus two roots [<math>\scriptstyle{\color{blue}{x^2-2x}}</math>] remain. |
|style="text-align:right;"|ותגרע ממנו שני שרשיו וישאר אלגו פחות שני שרשים | |style="text-align:right;"|ותגרע ממנו שני שרשיו וישאר אלגו פחות שני שרשים | ||
|- | |- | ||
| | | | ||
| − | : | + | :Add its root to it; it is a square minus two roots and a root of a square minus two roots [<math>\scriptstyle{\color{blue}{x^2-2x+\sqrt{x^2-2x}}}</math>] that has a root. |
| − | |style="text-align:right;"|ותוסיף עליו שרשו ויהיה אלגו פחות שני שרשים ושרש האלגו פחות שני שרשים ויחזיק שרש | + | |style="text-align:right;"|ותוסיף עליו שרשו <s>והוא</s> <sup>ויהיה</sup> אלגו פחות שני שרשים ושרש האלגו פחות שני שרשים ויחזיק שרש |
|- | |- | ||
| | | | ||
| − | : | + | :Suppose its root is a thing minus one and a half dirham [<math>\scriptstyle{\color{blue}{\sqrt{x^2-2x+\sqrt{x^2-2x}}=x-\left(1+\frac{1}{2}\right)}}</math>]. |
|style="text-align:right;"|ותניח שרשו דבר פחות דרהם אחד וחצי | |style="text-align:right;"|ותניח שרשו דבר פחות דרהם אחד וחצי | ||
|- | |- | ||
| Line 2,853: | Line 3,153: | ||
|- | |- | ||
| | | | ||
| − | :Confront them; | + | :Confront them; after the confrontation they are a thing and a root of a square minus two roots equal two dirham and a quarter. |
:<math>\scriptstyle{\color{blue}{x+\sqrt{x^2-2x}=2+\frac{1}{4}}}</math> | :<math>\scriptstyle{\color{blue}{x+\sqrt{x^2-2x}=2+\frac{1}{4}}}</math> | ||
|style="text-align:right;"|ותכוין בהם ויהיה אחר הכוון דבר ושרש מאלגו פחות שני שרשים ישוו שני דרהמי ורביע | |style="text-align:right;"|ותכוין בהם ויהיה אחר הכוון דבר ושרש מאלגו פחות שני שרשים ישוו שני דרהמי ורביע | ||
|- | |- | ||
| | | | ||
| − | : | + | :Subtract a thing from two dirham and a quarter; two dirham and a quarter minus a thing remain equal to a root of a square minus two roots. |
| − | + | :<math>\scriptstyle{\color{blue}{\left(2+\frac{1}{4}\right)-x=\sqrt{x^2-2x}}}</math> | |
| − | + | |style="text-align:right;"|ותגרע דבר משני דרהמי ורביע ישאר שני דרהמי ורביע פחות דבר ישוה שרש מאלגו פחות שני שרשים | |
| − | |||
| − | |||
| − | |style="text-align:right;"|ישאר שני דרהמי ורביע פחות דבר ישוה שרש מאלגו פחות שני שרשים | ||
|- | |- | ||
| | | | ||
| Line 2,879: | Line 3,176: | ||
:Confront them; the thing is two dirham and a quarter of a tenth and this is the root of the square. | :Confront them; the thing is two dirham and a quarter of a tenth and this is the root of the square. | ||
:<math>\scriptstyle{\color{blue}{x=2+\left(\frac{1}{4}\sdot\frac{1}{10}\right)}}</math> | :<math>\scriptstyle{\color{blue}{x=2+\left(\frac{1}{4}\sdot\frac{1}{10}\right)}}</math> | ||
| − | |style="text-align:right;"|ותכוין עמהם ויהיה הדבר שני דרהמי ורביע עשירית והוא | + | |style="text-align:right;"|ותכוין עמהם ויהיה הדבר שני דרהמי ורביע עשירית והוא שרש האלגו |
|- | |- | ||
| | | | ||
| Line 2,886: | Line 3,183: | ||
|style="text-align:right;"|והאלגו ארבעה ועשירית אחד מחלק אחד מאלף ת"ר | |style="text-align:right;"|והאלגו ארבעה ועשירית אחד מחלק אחד מאלף ת"ר | ||
|- | |- | ||
| − | |Check: | + | | |
| + | :*<span style="color:Green>'''Check:'''</span> | ||
| + | :Subtract from it its two roots that are four and half a tenth; half a tenth and one part of one thousand and 600 remain. | ||
|style="text-align:right;"|ותגרע ממנו שני שרשיו והם ארבעה וחצי עשירית ישאר חצי עשירית וחלק אחד מאלף ת"ר | |style="text-align:right;"|ותגרע ממנו שני שרשיו והם ארבעה וחצי עשירית ישאר חצי עשירית וחלק אחד מאלף ת"ר | ||
|- | |- | ||
| Line 2,893: | Line 3,192: | ||
|- | |- | ||
| | | | ||
| + | :Add to it its root, which is a fifth and a quarter of a tenth; it is a quarter, a quarter of a tenth and one part of a thousand and 600, which has a root and it is a half and a quarter of a tenth. | ||
|style="text-align:right;"|ותוסיף עליו שרשו והוא חומש אחד ורביעית העשירית יהיה רביע אחד ורובע העשירית וחלק אחד מאלף ת"ר<br> | |style="text-align:right;"|ותוסיף עליו שרשו והוא חומש אחד ורביעית העשירית יהיה רביע אחד ורובע העשירית וחלק אחד מאלף ת"ר<br> | ||
ומחזיק שרש והוא חצי ורביע העשירית | ומחזיק שרש והוא חצי ורביע העשירית | ||
| Line 2,900: | Line 3,200: | ||
|- | |- | ||
| | | | ||
| − | :36) <math>\scriptstyle\begin{cases}\scriptstyle x^2+3x+1=n^2\\\scriptstyle x^2-\left(3x-2\right)=m^2\end{cases}</math> | + | :{{#annot:x²+3x+1=n²,x²-(3x-2)=m²|1124|43ha}}36) If you are told: a square that has a root, if you add to it its three roots and one dirham, it has a root; and if you subtract its three roots minus two dirham from it, the remainder has a root. |
| + | :<math>\scriptstyle\begin{cases}\scriptstyle x^2+3x+1=n^2\\\scriptstyle x^2-\left(3x-2\right)=m^2\end{cases}</math> | ||
|style="text-align:right;"|לו <big>ואם</big> יאמרו לך אלגו שיחזיק שרש אם תוסיף עליו שלשת שרשיו ודרהם אחד יחזיק שרש<br> | |style="text-align:right;"|לו <big>ואם</big> יאמרו לך אלגו שיחזיק שרש אם תוסיף עליו שלשת שרשיו ודרהם אחד יחזיק שרש<br> | ||
| − | ואם תגרע ממנו שלשת שרשיו פחות שני דרהמי יחזיק מה שישאר שרש | + | ואם תגרע ממנו שלשת שרשיו פחות שני דרהמי יחזיק מה שישאר שרש{{#annotend:43ha}} |
|- | |- | ||
| | | | ||
| Line 2,909: | Line 3,210: | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{x^2+3x+1}}</math> has a root | + | :Add to it its three roots and one dirham. It is a square and three roots plus one dirham [<math>\scriptstyle{\color{blue}{x^2+3x+1}}</math>] that has a root. |
|style="text-align:right;"|ותוסיף עליו שלשת שרשיו ודרהם אחד ויהיה אלגו ושלשה שרשים ודרהם אחד ויחזיק שרש | |style="text-align:right;"|ותוסיף עליו שלשת שרשיו ודרהם אחד ויהיה אלגו ושלשה שרשים ודרהם אחד ויחזיק שרש | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{x^2-\left(3x-2\right)=x^2+2-3x}}</math> has a root | + | :Subtract from the square its three roots minus two dirham. A square and two dirham minus three roots remain [<math>\scriptstyle{\color{blue}{x^2-\left(3x-2\right)=x^2+2-3x}}</math>] and it has a root. |
| − | |style="text-align:right;"|ותגרע מהאלגו שלשת שרשיו פחות שני דרהמי וישאר אלגו ושני דרהמי פחות שלשה שרשים ומחזיק שרש | + | |style="text-align:right;"|ותגרע מהאלגו שלשת שרשיו פחות שני דרהמי וישאר אלגו ושני דרהמי פחות שלשה שרשים ומחזיק ‫<ref>77r</ref>שרש |
|- | |- | ||
| | | | ||
| − | : | + | :Suppose its root is a root of a square plus three roots and one dirham minus three dirham [<math>\scriptstyle{\color{blue}{\sqrt{x^2+2-3x}=\sqrt{x^2+3x+1}-3}}</math>]. |
|style="text-align:right;"|ותניח שרשו שרש מאלגו ושלשה שרשים ודרהם אחד פחות שלשה דרהמיש | |style="text-align:right;"|ותניח שרשו שרש מאלגו ושלשה שרשים ודרהם אחד פחות שלשה דרהמיש | ||
|- | |- | ||
| Line 2,932: | Line 3,233: | ||
|- | |- | ||
| | | | ||
| + | :Confront them; after the confrontation it is six things and eight dirham equal a root of 36 squares, 36 dirham and [1]08 things. | ||
:<math>\scriptstyle{\color{blue}{6x+8=\sqrt{36x^2+36+{\color{red}{1}}08x}}}</math> | :<math>\scriptstyle{\color{blue}{6x+8=\sqrt{36x^2+36+{\color{red}{1}}08x}}}</math> | ||
|style="text-align:right;"|ותכוין עמהם ויהיה אחר הכוון ששה דברים ושמנה דרהמי ישוה שרש מל"ו אלגוש ול"ו דרהמיש ור"ח דברים | |style="text-align:right;"|ותכוין עמהם ויהיה אחר הכוון ששה דברים ושמנה דרהמי ישוה שרש מל"ו אלגוש ול"ו דרהמיש ור"ח דברים | ||
| Line 2,945: | Line 3,247: | ||
|- | |- | ||
| | | | ||
| + | :Confront them; the thing is two dirham and a third and this is the root of the square. | ||
:<math>\scriptstyle{\color{blue}{x=\sqrt{x^2}=2+\frac{1}{3}}}</math> | :<math>\scriptstyle{\color{blue}{x=\sqrt{x^2}=2+\frac{1}{3}}}</math> | ||
|style="text-align:right;"|ותכוין עמהם ויהיה הדבר שני דרהמי ושליש אחד והוא שרש האלגו | |style="text-align:right;"|ותכוין עמהם ויהיה הדבר שני דרהמי ושליש אחד והוא שרש האלגו | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x^2=5+\frac{4}{9}}}</math> | + | :The square is five dirahm and four-ninths. |
| + | :<math>\scriptstyle{\color{blue}{x^2=5+\frac{4}{9}}}</math> | ||
|style="text-align:right;"|והאלגו חמשה דרהמי וארבעה תשיעיות | |style="text-align:right;"|והאלגו חמשה דרהמי וארבעה תשיעיות | ||
|- | |- | ||
| − | |Check: | + | | |
| + | :*<span style="color:Green>'''Check:'''</span> | ||
| + | :If you add to it its three roots and one dirham, it is thirteen dirham and four-ninths and its root is three dirham and two-thirds. | ||
:<math>\scriptstyle{\color{blue}{x^2+3x+1=13+\frac{4}{9}=\left(3+\frac{2}{3}\right)^2}}</math> | :<math>\scriptstyle{\color{blue}{x^2+3x+1=13+\frac{4}{9}=\left(3+\frac{2}{3}\right)^2}}</math> | ||
| − | |style="text-align:right;"|ואם תוסיף עליו שלשת שרשיו ודרהם אחד יהיה שלשה עשר דרהמי וארבעה תשיעיות ושרשו | + | |style="text-align:right;"|ואם תוסיף עליו שלשת שרשיו ודרהם אחד יהיה שלשה עשר דרהמי וארבעה תשיעיות ושרשו שלשה דרהמי ושני שלישים |
|- | |- | ||
| | | | ||
| + | :If you subtract from it its three roots minus two dirham, four-ninths remain and its root is two-thirds. | ||
:<math>\scriptstyle{\color{blue}{x^2-\left(3x-2\right)=\frac{4}{9}=\left(\frac{2}{3}\right)^2}}</math> | :<math>\scriptstyle{\color{blue}{x^2-\left(3x-2\right)=\frac{4}{9}=\left(\frac{2}{3}\right)^2}}</math> | ||
|style="text-align:right;"|ואם תגרע ממנו שלשת שרשיו פחות שנים דרהמי ישאר ארבעה תשיעיות ושרשו שנים שלישיות | |style="text-align:right;"|ואם תגרע ממנו שלשת שרשיו פחות שנים דרהמי ישאר ארבעה תשיעיות ושרשו שנים שלישיות | ||
|- | |- | ||
| | | | ||
| − | :37) If you are told: a square that has a root, if you subtract one dirham minus the root of the square from it, it has a root; [and if you subtract its root minus one dirham from it, it has a root]. | + | :{{#annot:x²-(x-1)=n²,x²-(1-x)=m²|1124|Wdk5}}37) If you are told: a square that has a root, if you subtract one dirham minus the root of the square from it, it has a root; [and if you subtract its root minus one dirham from it, it has a root]. |
:<math>\scriptstyle\begin{cases}\scriptstyle{\color{red}{x^2-\left(x-1\right)=n^2}}\\\scriptstyle x^2-\left(1-x\right)=m^2\end{cases}</math> | :<math>\scriptstyle\begin{cases}\scriptstyle{\color{red}{x^2-\left(x-1\right)=n^2}}\\\scriptstyle x^2-\left(1-x\right)=m^2\end{cases}</math> | ||
| − | |style="text-align:right;"|לז <big>ואם</big> יאמרו לך אלגו המחזיק שרש אם תגרע ממנו דרהם אחד פחות שרש האלגו יחזיק הנשאר שרש | + | |style="text-align:right;"|לז <big>ואם</big> יאמרו לך אלגו המחזיק שרש אם תגרע ממנו דרהם אחד פחות שרש האלגו יחזיק הנשאר שרש{{#annotend:Wdk5}} |
|- | |- | ||
| | | | ||
| Line 2,970: | Line 3,277: | ||
|- | |- | ||
| | | | ||
| − | :Add to it one dirham minus a root of the square. It is a square and one dirham minus a root of the square | + | :Add to it one dirham minus a root of the square. It is a square and one dirham minus a root of the square [<math>\scriptstyle{\color{blue}{x^2+1-x}}</math>] that has a root. |
| − | |||
|style="text-align:right;"|ותוסיף עליו דרהם אחד פחות שרש האלגו ויהיה אלגו ודרהם אחד פחות שרש האלגו ומחזיק שרש | |style="text-align:right;"|ותוסיף עליו דרהם אחד פחות שרש האלגו ויהיה אלגו ודרהם אחד פחות שרש האלגו ומחזיק שרש | ||
|- | |- | ||
| | | | ||
| − | :If you subtract one dirham minus a root of the square from the square, a square and a root minus one dirham | + | :If you subtract one dirham minus a root of the square from the square, a square and a root minus one dirham [<math>\scriptstyle{\color{blue}{x^2-\left(1-x\right)=x^2+x-1}}</math>] remains and it has a root. |
| − | |||
|style="text-align:right;"|ואם תגרע מהאלגו דרהם אחד פחות שרש האלגו ישאר אלגו ושרש פחות דרהם אחד ומחזיק שרש | |style="text-align:right;"|ואם תגרע מהאלגו דרהם אחד פחות שרש האלגו ישאר אלגו ושרש פחות דרהם אחד ומחזיק שרש | ||
|- | |- | ||
| Line 2,985: | Line 3,290: | ||
| | | | ||
:Multiply them by themselves; they are a square and a root minus two roots of a square and a root minus one dirham equal a square and one dirham minus a root. | :Multiply them by themselves; they are a square and a root minus two roots of a square and a root minus one dirham equal a square and one dirham minus a root. | ||
| + | |style="text-align:right;"|ותכהו בעצמו ויהיה אלגו ושרש פחות שני שרשים מאלגו ושרש פחות דרהם אחד ישוה אלגו ודרהם אחד פחות שרש | ||
| + | |- | ||
| + | |colspan=2| | ||
:<math>\scriptstyle{\color{blue}{\left(1-\sqrt{x^2+x-1}\right)^2=x^2+x-2\sqrt{x^2+x-1}=x^2+1-x}}</math> | :<math>\scriptstyle{\color{blue}{\left(1-\sqrt{x^2+x-1}\right)^2=x^2+x-2\sqrt{x^2+x-1}=x^2+1-x}}</math> | ||
| − | |||
|- | |- | ||
| | | | ||
| Line 3,006: | Line 3,313: | ||
| | | | ||
:It half, which is a thing minus half a dirham, is equal to a root of a square and a root minus one dirham. | :It half, which is a thing minus half a dirham, is equal to a root of a square and a root minus one dirham. | ||
| + | |style="text-align:right;"|וחציו שהוא דבר פחות חצי דרהם ישוה שרש מאלגו ושרש פחות דרהם אחד | ||
| + | |- | ||
| + | |colspan=2| | ||
:<math>\scriptstyle{\color{blue}{2x-1=2\sdot\sqrt{x^2+x-1}\quad/\sdot\frac{1}{2}\longrightarrow x-\frac{1}{2}=\sqrt{x^2+x-1}}}</math> | :<math>\scriptstyle{\color{blue}{2x-1=2\sdot\sqrt{x^2+x-1}\quad/\sdot\frac{1}{2}\longrightarrow x-\frac{1}{2}=\sqrt{x^2+x-1}}}</math> | ||
| − | |||
|- | |- | ||
| | | | ||
:Multiply a thing minus half a dirham by themselves; they are a square and a quarter of a dirham minus a thing equal a square and a root minus one dirham. | :Multiply a thing minus half a dirham by themselves; they are a square and a quarter of a dirham minus a thing equal a square and a root minus one dirham. | ||
:<math>\scriptstyle{\color{blue}{\left(x-\frac{1}{2}\right)^2=x^2+\frac{1}{4}-x=x^2+x-1}}</math> | :<math>\scriptstyle{\color{blue}{\left(x-\frac{1}{2}\right)^2=x^2+\frac{1}{4}-x=x^2+x-1}}</math> | ||
| − | |style="text-align:right;"|ותכה דבר פחות חצי דרהם בעצמו ויהיה אלגו ורביע דרהם פחות דבר ישוה אלגו ושרש פחות דרהם אחד | + | |style="text-align:right;"|ותכה דבר פחות חצי דרהם בעצמו ויהיה אלגו ורביע דרהם פחות דבר ישוה אלגו ושרש פחות <s>דבר</s> <sup>דרהם</sup> אחד |
|- | |- | ||
| | | | ||
| Line 3,021: | Line 3,330: | ||
:Confront them; the thing is five-eighths of a dirham and this is the root of the square. | :Confront them; the thing is five-eighths of a dirham and this is the root of the square. | ||
:<math>\scriptstyle{\color{blue}{x=\sqrt{x^2}=\frac{5}{8}}}</math> | :<math>\scriptstyle{\color{blue}{x=\sqrt{x^2}=\frac{5}{8}}}</math> | ||
| − | |style="text-align:right;"|ותכוין עמהם ויהיה הדבר חמשה שמיניות מדרהם והוא שרש האלגו | + | |style="text-align:right;"|ותכוין עמהם ויהיה הדבר חמשה שמיניות מדרהם ‫<ref>77v</ref>והוא שרש האלגו |
|- | |- | ||
| | | | ||
| Line 3,029: | Line 3,338: | ||
|- | |- | ||
| | | | ||
| − | :38) If you are told: a square that has a root, if you add two dirham minus the root of the square to it, it has a root; and if you subtract three minus the root of the square from it, it has a root. | + | :{{#annot:x²+(2-x)=n²,x²-(3-x)=m²|1124|xP6r}}38) If you are told: a square that has a root, if you add two dirham minus the root of the square to it, it has a root; and if you subtract three minus the root of the square from it, it has a root. |
:<math>\scriptstyle\begin{cases}\scriptstyle x^2+\left(2-x\right)=n^2\\\scriptstyle x^2-\left(3-x\right)=m^2\end{cases}</math> | :<math>\scriptstyle\begin{cases}\scriptstyle x^2+\left(2-x\right)=n^2\\\scriptstyle x^2-\left(3-x\right)=m^2\end{cases}</math> | ||
|style="text-align:right;"|לח <big>ואם</big> יאמרו לך אלגו המחזיק שרש אם תוסיף עליו שני דרהמי פחות שרש האלגו יחזיק שרש<br> | |style="text-align:right;"|לח <big>ואם</big> יאמרו לך אלגו המחזיק שרש אם תוסיף עליו שני דרהמי פחות שרש האלגו יחזיק שרש<br> | ||
| − | ואם תגרע ממנו שלשה פחות שרש האלגו יחזיק שרש | + | ואם תגרע ממנו שלשה פחות שרש האלגו יחזיק שרש{{#annotend:xP6r}} |
|- | |- | ||
| | | | ||
| Line 3,039: | Line 3,348: | ||
|- | |- | ||
| | | | ||
| − | :Add two dirham minus a root to it; it is a square and two dirham minus a root | + | :Add two dirham minus a root to it; it is a square and two dirham minus a root [<math>\scriptstyle{\color{blue}{x^2+2-x}}</math>] and it has a root. |
| − | |||
|style="text-align:right;"|ותוסיף עליו שני דרהמי פחות שרש ויהיה אלגו ושני דרהמי פחות שרש ויחזיק שרש | |style="text-align:right;"|ותוסיף עליו שני דרהמי פחות שרש ויהיה אלגו ושני דרהמי פחות שרש ויחזיק שרש | ||
|- | |- | ||
| | | | ||
| − | :Subtract three minus a root from it; the remainder is a square and a root minus three dirham | + | :Subtract three minus a root from it; the remainder is a square and a root minus three dirham [<math>\scriptstyle{\color{blue}{x^2-\left(3-x\right)=x^2+x-3}}</math>] and it has a root. |
| − | |||
|style="text-align:right;"|ותגרע ממנו שלשה פחות שרש וישאר אלגו ושרש פחות שלשה דרהמי ויחזיק שרש | |style="text-align:right;"|ותגרע ממנו שלשה פחות שרש וישאר אלגו ושרש פחות שלשה דרהמי ויחזיק שרש | ||
|- | |- | ||
| Line 3,066: | Line 3,373: | ||
| | | | ||
:Restore the square and two dirham with the root by adding it to the square and the root minus two dirham and subtract a square from a square; two roots and [two roots] of a square and a root minus three dirham remain equal four dirham. | :Restore the square and two dirham with the root by adding it to the square and the root minus two dirham and subtract a square from a square; two roots and [two roots] of a square and a root minus three dirham remain equal four dirham. | ||
| − | |style="text-align:right;"|ותאסוף האלגו ושני דרהמי עם השרש | + | |style="text-align:right;"|ותאסוף האלגו ושני דרהמי עם השרש ותוסיפהו עם האלגו ושרש פחות שני דרהמי ותגרע אלגו מאלגו וישאר שני שרשים ושרש מאלגו ושרש פחות שלשה דרהמי ישוה ארבעה דרהמי |
| − | ותגרע אלגו מאלגו וישאר שני שרשים ושרש מאלגו ושרש פחות שלשה דרהמי ישוה ארבעה דרהמי | ||
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| Line 3,079: | Line 3,385: | ||
| | | | ||
:Take its half; it is two dirham minus a root equal a root of a square and a root minus three dirham. | :Take its half; it is two dirham minus a root equal a root of a square and a root minus three dirham. | ||
| + | |style="text-align:right;"|ותקח חציו והוא שני דרהמי פחות שרש ישוה אלגו ושרש פחות שלשה דרהמי | ||
| + | |- | ||
| + | |colspan=2| | ||
:<math>\scriptstyle{\color{blue}{4-2x=2\sdot\sqrt{x^2+x-3}\quad/\sdot\frac{1}{2}\longrightarrow2-x=\sqrt{x^2+x-3}}}</math> | :<math>\scriptstyle{\color{blue}{4-2x=2\sdot\sqrt{x^2+x-3}\quad/\sdot\frac{1}{2}\longrightarrow2-x=\sqrt{x^2+x-3}}}</math> | ||
| − | |||
|- | |- | ||
| | | | ||
| Line 3,099: | Line 3,407: | ||
:The square is one dirham, four-fifths, and four-fifths of a fifth. | :The square is one dirham, four-fifths, and four-fifths of a fifth. | ||
:<math>\scriptstyle{\color{blue}{x^2=1+\frac{4}{5}+\left(\frac{4}{5}\sdot\frac{1}{5}\right)}}</math> | :<math>\scriptstyle{\color{blue}{x^2=1+\frac{4}{5}+\left(\frac{4}{5}\sdot\frac{1}{5}\right)}}</math> | ||
| − | |style="text-align:right;"|והאלגו דרהם אחד וארבעה | + | |style="text-align:right;"|והאלגו דרהם אחד וארבעה ח<sup>ו</sup>משים וארבעה חומשים מחומש |
|- | |- | ||
| | | | ||
| − | :39) If you are told: a square that has a root, if you add its root plus one dirham to it, it has a root; and if you add to the square its two roots plus two dirham, it has a root. | + | :{{#annot:x²+x+1=n²,x²+2x+2=m²|1124|YEF9}}39) If you are told: a square that has a root, if you add its root plus one dirham to it, it has a root; and if you add to the square its two roots plus two dirham, it has a root. |
:<math>\scriptstyle\begin{cases}\scriptstyle x^2+x+1=n^2\\\scriptstyle x^2+2x+2=m^2\end{cases}</math> | :<math>\scriptstyle\begin{cases}\scriptstyle x^2+x+1=n^2\\\scriptstyle x^2+2x+2=m^2\end{cases}</math> | ||
|style="text-align:right;"|לט <big>ואם</big> יאמרו לך אלגו המחזיק שרש אם תוסיף עליו שרשו ודרהם אחד יחזיק שרש<br> | |style="text-align:right;"|לט <big>ואם</big> יאמרו לך אלגו המחזיק שרש אם תוסיף עליו שרשו ודרהם אחד יחזיק שרש<br> | ||
| − | ואם תוסיף על האלגו שני שרשיו ושני דרהמי יחזיק שרש | + | ואם תוסיף על האלגו שני שרשיו ושני דרהמי יחזיק שרש{{#annotend:YEF9}} |
|- | |- | ||
| | | | ||
| Line 3,112: | Line 3,420: | ||
|- | |- | ||
| | | | ||
| − | :Add its root plus one dirham to it; it is a square and a root plus one dirham | + | :Add its root plus one dirham to it; it is a square and a root plus one dirham [<math>\scriptstyle{\color{blue}{x^2+x+1}}</math>] and it has a root. |
| − | |||
|style="text-align:right;"|ותוסיף עליו שרשו ודרהם אחד ויהיה אלגו ושרש ודרהם אחד ויחזיק שרש | |style="text-align:right;"|ותוסיף עליו שרשו ודרהם אחד ויהיה אלגו ושרש ודרהם אחד ויחזיק שרש | ||
|- | |- | ||
| | | | ||
| − | :Add to the square its two roots plus two dirham | + | :Add to the square its two roots plus two dirham [<math>\scriptstyle{\color{blue}{x^2+2x+2}}</math>]; it has a root. |
| − | |||
|style="text-align:right;"|ותוסיף על האלגו שני שרשיו ושני דרהמי ויחזיק שרש | |style="text-align:right;"|ותוסיף על האלגו שני שרשיו ושני דרהמי ויחזיק שרש | ||
|- | |- | ||
| Line 3,146: | Line 3,452: | ||
:Confront them; the thing is seven-eighths and this is the root of the square. | :Confront them; the thing is seven-eighths and this is the root of the square. | ||
:<math>\scriptstyle{\color{blue}{x=\sqrt{x^2}=\frac{7}{8}}}</math> | :<math>\scriptstyle{\color{blue}{x=\sqrt{x^2}=\frac{7}{8}}}</math> | ||
| − | |style="text-align:right;"|ותכוין בהם ויהיה הדבר שבעה שמיניות והוא שרש האלגו | + | |style="text-align:right;"|ותכוין בהם ויהיה הדבר שבעה שמיניות והוא שרש ‫<ref>78r</ref>האלגו |
|- | |- | ||
| | | | ||
| Line 3,159: | Line 3,465: | ||
== <span style="color:Green>Word Problems</span> == | == <span style="color:Green>Word Problems</span> == | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | === <span style="color:Green>If You Give Me Problems</span> === | ||
| | | | ||
| Line 3,169: | Line 3,480: | ||
:The fourth asked the three [others] for a fifth of what they have | :The fourth asked the three [others] for a fifth of what they have | ||
:How much is the price of the beast and how much money does each of them have? | :How much is the price of the beast and how much money does each of them have? | ||
| − | |style="text-align:right;"|מ | + | |style="text-align:right;"|מ <big>ואם</big> יאמרו לך ארבעה אנשים התקבצו יחד לקנות בהמה אחת<br> |
ואמר הראשון לשלשה אם תתנו לי החצי מאשר תחזיקו יהיה לי ערך הבהמה<br> | ואמר הראשון לשלשה אם תתנו לי החצי מאשר תחזיקו יהיה לי ערך הבהמה<br> | ||
ושאל השני לשלשה השלישית<br> | ושאל השני לשלשה השלישית<br> | ||
| Line 3,181: | Line 3,492: | ||
| | | | ||
:Knowing this is that you assume the first has a thing [<math>\scriptstyle{\color{blue}{x}}</math>], the second a dinar [<math>\scriptstyle{\color{blue}{y}}</math>], the third a dirham [<math>\scriptstyle{\color{blue}{u}}</math>], and the fourth a fals [<math>\scriptstyle{\color{blue}{v}}</math>] | :Knowing this is that you assume the first has a thing [<math>\scriptstyle{\color{blue}{x}}</math>], the second a dinar [<math>\scriptstyle{\color{blue}{y}}</math>], the third a dirham [<math>\scriptstyle{\color{blue}{u}}</math>], and the fourth a fals [<math>\scriptstyle{\color{blue}{v}}</math>] | ||
| − | |style="width:45%; text-align:right;"|וידיעת זה שתניח אל האחד דבר ולשני דינר אחד ולשלישי דרהם אחד ולרביעי פלס אחד | + | |style="width:45%; text-align:right;"|וידיעת זה שתניח <sup>אל</sup> האחד דבר ולשני דינר אחד ולשלישי דרהם אחד ולרביעי פלס אחד |
|- | |- | ||
| | | | ||
:The first, who has a thing, says to the three: give me half of what you have, I will add it to what I have and I will have the price of the beast. | :The first, who has a thing, says to the three: give me half of what you have, I will add it to what I have and I will have the price of the beast. | ||
| − | |style="text-align:right;"|ואמר הראשון שמחזיק דבר אל השלשה תנו לי החצי | + | |style="text-align:right;"|ואמר הראשון שמחזיק דבר אל השלשה תנו לי החצי <sup>מ</sup>אשר לכם ואקבצהו עם אשר לי ויהיה לי ערך הבהמה |
|- | |- | ||
| | | | ||
| Line 3,194: | Line 3,505: | ||
| | | | ||
:The second, who has one dinar, says to the three: give me a third of what you have, I will add it to what I have and I will have the price of the beast. | :The second, who has one dinar, says to the three: give me a third of what you have, I will add it to what I have and I will have the price of the beast. | ||
| − | |style="text-align:right;"|ואמר השני אשר לו דינר אחד לשלשה תנו לי | + | |style="text-align:right;"|ואמר השני אשר לו דינר אחד לשלשה תנו לי <sup>ה</sup>שלישית מאשר לכם ואקבצהו עם אשר לי ויהיה לי ערך הבהמה |
|- | |- | ||
| | | | ||
:A third of what they have is: a third of a thing, a third of a dirham, and a third of a fals. When they are added to the dinar that he has, he has in his hand one dinar, a third of a thing, a third of a dirham, and a third of a fals; this is the price of the beast. | :A third of what they have is: a third of a thing, a third of a dirham, and a third of a fals. When they are added to the dinar that he has, he has in his hand one dinar, a third of a thing, a third of a dirham, and a third of a fals; this is the price of the beast. | ||
:<math>\scriptstyle{\color{blue}{y+\frac{1}{3}x+\frac{1}{3}u+\frac{1}{3}v}}</math> | :<math>\scriptstyle{\color{blue}{y+\frac{1}{3}x+\frac{1}{3}u+\frac{1}{3}v}}</math> | ||
| − | |style="text-align:right;"|ושלישית אשר להם הוא שלישית דבר ושלישית דרהם | + | |style="text-align:right;"|ושלישית אשר להם הוא שלישית דבר ושלישית דרהם ושליש פלס וכאשר קובצו עם הדינר אשר לו יהיו בידו דינר אחד ושלישית דבר ושלישית דרהם ושלישית פלס והוא ערך הבהמה |
|- | |- | ||
| | | | ||
| Line 3,207: | Line 3,518: | ||
|- | |- | ||
| | | | ||
| − | :<span style="color:Green>Confrontation:</span> Confront them by subtracting a third of a thing from a whole thing; two-thirds of a thing remain. | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Confront them by subtracting a third of a thing from a whole thing; two-thirds of a thing remain. | ||
:<math>\scriptstyle{\color{blue}{x-\frac{1}{3}x=\frac{2}{3}x}}</math> | :<math>\scriptstyle{\color{blue}{x-\frac{1}{3}x=\frac{2}{3}x}}</math> | ||
|style="text-align:right;"|ותכוין בהם ויהיה זה שתגרע שלישית דבר מדבר שלם וישאר שני שלישי דבר | |style="text-align:right;"|ותכוין בהם ויהיה זה שתגרע שלישית דבר מדבר שלם וישאר שני שלישי דבר | ||
| Line 3,219: | Line 3,534: | ||
:Subtract a third of a fals from half a fals; one-sixth of a fals remains. | :Subtract a third of a fals from half a fals; one-sixth of a fals remains. | ||
:<math>\scriptstyle{\color{blue}{\frac{1}{2}v-\frac{1}{3}v=\frac{1}{6}v}}</math> | :<math>\scriptstyle{\color{blue}{\frac{1}{2}v-\frac{1}{3}v=\frac{1}{6}v}}</math> | ||
| − | |style="text-align:right;"|ותגרע שליש פלס מחצי פלס | + | |style="text-align:right;"|ותגרע שליש פלס מחצי פלס ישאר ששית פלס |
|- | |- | ||
| | | | ||
:Subtract half of [a dinar] from one dinar; half dinar remains, which is equal to two-thirds of a thing, a sixth of a dirham, and a sixth of a fals. | :Subtract half of [a dinar] from one dinar; half dinar remains, which is equal to two-thirds of a thing, a sixth of a dirham, and a sixth of a fals. | ||
:<math>\scriptstyle{\color{blue}{y-\frac{1}{2}y=\frac{1}{2}y=\frac{2}{3}x+\frac{1}{6}u+\frac{1}{6}v}}</math> | :<math>\scriptstyle{\color{blue}{y-\frac{1}{2}y=\frac{1}{2}y=\frac{2}{3}x+\frac{1}{6}u+\frac{1}{6}v}}</math> | ||
| − | |style="text-align:right;"|ותגרע חצי גאקיש מדינר אחד וישאר חצי דינר ישוה שני שלישי | + | |style="text-align:right;"|ותגרע חצי גאקיש מדינר אחד וישאר חצי דינר ישוה <sup>שני שלישי</sup> דבר <s>וחצי דרהם</s> ושתות דרהם ושתות <s>טורניש</s> <sup>פלס</sup> |
|- | |- | ||
| | | | ||
:The dinar equals a thing and one-third of a thing, one-third of a dirham, and one-third of a fals. | :The dinar equals a thing and one-third of a thing, one-third of a dirham, and one-third of a fals. | ||
:<math>\scriptstyle{\color{blue}{y=1\frac{1}{3}x+\frac{1}{3}u+\frac{1}{3}v}}</math> | :<math>\scriptstyle{\color{blue}{y=1\frac{1}{3}x+\frac{1}{3}u+\frac{1}{3}v}}</math> | ||
| − | |style="text-align:right;"|והדינר ישוה דבר ושליש דבר ושליש דרהם ושליש אלפלס | + | |style="text-align:right;"|<s>והגאקיש</s> <sup>והדינר</sup> ישוה דבר <s>ושני</s> ושליש דבר ושליש דרהם ושליש אלפלס |
|- | |- | ||
| | | | ||
:A quarter of a dinar equals one-third of a thing, half a sixth of a dirham, and half a sixth of a fals. | :A quarter of a dinar equals one-third of a thing, half a sixth of a dirham, and half a sixth of a fals. | ||
:<math>\scriptstyle{\color{blue}{\frac{1}{4}y=\frac{1}{3}x+\left(\frac{1}{2}\sdot\frac{1}{6}\right)u+\left(\frac{1}{2}\sdot\frac{1}{6}\right)v}}</math> | :<math>\scriptstyle{\color{blue}{\frac{1}{4}y=\frac{1}{3}x+\left(\frac{1}{2}\sdot\frac{1}{6}\right)u+\left(\frac{1}{2}\sdot\frac{1}{6}\right)v}}</math> | ||
| − | |style="text-align:right;"|ורביע דינר ישוה שליש דבר וחצי שתות דרהם וחצי שתות פלס | + | |style="text-align:right;"|‫[ורביע דינר ישוה שליש דבר וחצי שתות דרהם וחצי שתות פלס |
|- | |- | ||
| | | | ||
:A fifth of a dinar equals one-fifth of a thing and a fifth of a third of a thing, a fifth of a third of a dirham, and fifth of a third of a fals. | :A fifth of a dinar equals one-fifth of a thing and a fifth of a third of a thing, a fifth of a third of a dirham, and fifth of a third of a fals. | ||
:<math>\scriptstyle{\color{blue}{\frac{1}{5}y=\left[\frac{1}{5}+\left(\frac{1}{5}\sdot\frac{1}{3}\right)\right]x+\left(\frac{1}{5}\sdot\frac{1}{3}\right)u+\left(\frac{1}{5}\sdot\frac{1}{3}\right)v}}</math> | :<math>\scriptstyle{\color{blue}{\frac{1}{5}y=\left[\frac{1}{5}+\left(\frac{1}{5}\sdot\frac{1}{3}\right)\right]x+\left(\frac{1}{5}\sdot\frac{1}{3}\right)u+\left(\frac{1}{5}\sdot\frac{1}{3}\right)v}}</math> | ||
| − | |style="text-align:right;"|וחמישית דינר ישוה חמישית דבר וחמישית שלישית הדבר | + | |style="text-align:right;"|וחמישית דינר ישוה חמישית דבר וחמישית שלישית הדבר וחמי' שלישית דרהם וחמישית שלישית אלפלס]‫<ref>Ma. marg.</ref> |
|- | |- | ||
| | | | ||
| Line 3,247: | Line 3,562: | ||
| | | | ||
:Remove half the dinar from the price of the beast and put half the worth of the dinar instead, which is two-thirds of a thing, one-sixth of a dirham, and one-sixth of a fals. The price of the beast is a thing and two-thirds of a thing, two-thirds of a dirham and two-thirds of a fals. | :Remove half the dinar from the price of the beast and put half the worth of the dinar instead, which is two-thirds of a thing, one-sixth of a dirham, and one-sixth of a fals. The price of the beast is a thing and two-thirds of a thing, two-thirds of a dirham and two-thirds of a fals. | ||
| − | |style="text-align:right;"|ותגרע חצי הדינר מערך הבהמה ותניח במקומו חצי אשר ישוה הדינר והוא שני שלישי דבר ושתות דרהם ושתות אלפלס<br> | + | |style="text-align:right;"|ותגרע חצי הדינר מערך הבהמה ותניח במקומו <sup>חצי</sup> אשר ישוה <s>הגאקיש</s> <sup>הדינר</sup> והוא שני שלישי דבר ושתות דרהם ושתות אלפלס<br> |
ויהיה ערך הבהמה דבר ושני שלישים מדבר ושני שלישי דרהם ושני שלישי אלפלס | ויהיה ערך הבהמה דבר ושני שלישים מדבר ושני שלישי דרהם ושני שלישי אלפלס | ||
|- | |- | ||
| Line 3,255: | Line 3,570: | ||
| | | | ||
:We have already removed the dinar and placed a thing and a third of a thing, a third of a dirham and a third of a fals instead. | :We have already removed the dinar and placed a thing and a third of a thing, a third of a dirham and a third of a fals instead. | ||
| − | |style="text-align:right;"|וכבר הסירונו מספר הדינר והנחנו במקומו דבר ושליש דבר ושליש דרהם ושליש פלס | + | |style="text-align:right;"|וכבר <s>נסיר</s> <sup>הסירונו</sup> מספר הדינר <s>ונניח</s> <sup>והנחנו</sup> במקומו דבר ושליש דבר ושליש דרהם ושליש פלס‫<ref>Ma. marg.: ויהיה ערך הבהמה ישוב גם כן דבר ושני שלישי דבר ושני שלישי דרהם ושני שלישי פלס</ref> |
|- | |- | ||
| | | | ||
:The third, who has one dirham, says: give me a quarter of what you have, I will add it to what I have and I will have the price of the beast. | :The third, who has one dirham, says: give me a quarter of what you have, I will add it to what I have and I will have the price of the beast. | ||
| − | |style="text-align:right;"|ואמר השלישי אשר לו דרהם אחד תנו לי הרביעית | + | |style="text-align:right;"|ואמר השלישי אשר לו דרהם אחד תנו לי הרביעית <sup>מ</sup>אשר לכם ואקבצהו עם אשר לי ויהיה לי ערך הבהמה |
|- | |- | ||
| | | | ||
:A quarter of what they have is: a quarter of a thing, a quarter of a dinar, and a quarter of a fals. When you add them to the dirham that he has, then remove the amount of the dinar and put instead the value of a quarter of a dinar as explained above, what is in his hand is a third and a quarter of a thing, one dirham and half a sixth of a dirham, and a third of a fals; this is the price of the beast. It is equal to a thing and two-thirds of a thing, two-thirds of a dirham, and two-thirds of a fals. | :A quarter of what they have is: a quarter of a thing, a quarter of a dinar, and a quarter of a fals. When you add them to the dirham that he has, then remove the amount of the dinar and put instead the value of a quarter of a dinar as explained above, what is in his hand is a third and a quarter of a thing, one dirham and half a sixth of a dirham, and a third of a fals; this is the price of the beast. It is equal to a thing and two-thirds of a thing, two-thirds of a dirham, and two-thirds of a fals. | ||
| − | |style="text-align:right;"|ורביעית אשר להם הוא רביעית | + | |style="text-align:right;"|ורביעית אשר להם הוא <s>שלישית</s> ‫<ref>78v</ref>רביעית דבר ורביעית דינר ורביעית פלס וכאשר תקבצם עם האדרהם אשר לו יהיו ותסיר סך הדינר ותניח במקומו אשר ישוה רביעית הדינר [כמבואר למעלה]‫<ref>Ma. marg.</ref> יהיו אז מה שבידו שלישית דבר ורביעית דבר ודרהם וחצי שתות דרהם ושליש אלפלס והוא ערך הבהמה<br> |
ויהיה שוה לדבר ושני שלישי דבר ושני שלישי דרהם ושני שלישי אלפלס | ויהיה שוה לדבר ושני שלישי דבר ושני שלישי דרהם ושני שלישי אלפלס | ||
|- | |- | ||
| Line 3,270: | Line 3,585: | ||
|- | |- | ||
| | | | ||
| − | :<span style="color:Green>Confrontation:</span> Confront them by subtracting a third and a quarter of a thing from a thing and two-thirds of a thing; and a third of a fals from two-thirds of a fals; a thing and half a sixth plus one-third of a fals remain. | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Confront them by subtracting a third and a quarter of a thing from a thing and two-thirds of a thing; and a third of a fals from two-thirds of a fals; a thing and half a sixth plus one-third of a fals remain. | ||
| + | |style="text-align:right;"|ותכוין בהם והוא שתגרע שלישית דבר ורביעית דבר מדבר ושני שלישי דבר ושליש פלס משני שלישי פלס וישאר דבר וחצי שתות דבר ושליש פלס | ||
| + | |- | ||
| + | | colspan="2"| | ||
:<math>\scriptstyle{\color{blue}{\left[\left(1+\frac{2}{3}\right)-\left(\frac{1}{3}+\frac{1}{4}\right)\right]x+\left(\frac{2}{3}-\frac{1}{3}\right)v=\left[1+\left(\frac{1}{2}\sdot\frac{1}{6}\right)\right]x+\frac{1}{3}v}}</math> | :<math>\scriptstyle{\color{blue}{\left[\left(1+\frac{2}{3}\right)-\left(\frac{1}{3}+\frac{1}{4}\right)\right]x+\left(\frac{2}{3}-\frac{1}{3}\right)v=\left[1+\left(\frac{1}{2}\sdot\frac{1}{6}\right)\right]x+\frac{1}{3}v}}</math> | ||
| − | |||
|- | |- | ||
| | | | ||
:Subtract two-thirds of a dirham from a dirham and half a sixth; a quarter and a sixth of a dirham remain that are equal to a thing and half a sixth of a thing plus one-third of a fals. | :Subtract two-thirds of a dirham from a dirham and half a sixth; a quarter and a sixth of a dirham remain that are equal to a thing and half a sixth of a thing plus one-third of a fals. | ||
| + | |style="text-align:right;"|ותגרע שני שלישי דרהם מדרהם וחצי שתות וישאר רביע ושתות דרהם ישוה דבר וחצי שתות דבר ושליש פלס | ||
| + | |- | ||
| + | | colspan="2"| | ||
:<math>\scriptstyle{\color{blue}{\left[\left[1+\left(\frac{1}{2}\sdot\frac{1}{6}\right)\right]-\frac{2}{3}\right]u=\left(\frac{1}{4}+\frac{1}{6}\right)u=\left[1+\left(\frac{1}{2}\sdot\frac{1}{6}\right)\right]x+\frac{1}{3}v}}</math> | :<math>\scriptstyle{\color{blue}{\left[\left[1+\left(\frac{1}{2}\sdot\frac{1}{6}\right)\right]-\frac{2}{3}\right]u=\left(\frac{1}{4}+\frac{1}{6}\right)u=\left[1+\left(\frac{1}{2}\sdot\frac{1}{6}\right)\right]x+\frac{1}{3}v}}</math> | ||
| − | |||
|- | |- | ||
| | | | ||
:The dirham equals two things and three-fifths of a thing, plus four-fifths of a fals. | :The dirham equals two things and three-fifths of a thing, plus four-fifths of a fals. | ||
:<math>\scriptstyle{\color{blue}{u=\left(2+\frac{3}{5}\right)x+\frac{4}{5}v}}</math> | :<math>\scriptstyle{\color{blue}{u=\left(2+\frac{3}{5}\right)x+\frac{4}{5}v}}</math> | ||
| − | |style="text-align:right;"|והדרהם ישוה שני דברים ושלשה | + | |style="text-align:right;"|והדרהם ישוה שני דברים ושלשה חמשי דבר וארבעה חומשי אלפלס |
|- | |- | ||
| | | | ||
:Remove two-thirds of a dirham from the price of the beast and put two-thirds the value of the dirham instead, which is a thing, a third and two-fifths of a thing, plus one-third and one-fifth of a fals. The price of the beast is three things and two-fifths of a thing, plus one fals and a fifth of a fals. | :Remove two-thirds of a dirham from the price of the beast and put two-thirds the value of the dirham instead, which is a thing, a third and two-fifths of a thing, plus one-third and one-fifth of a fals. The price of the beast is three things and two-fifths of a thing, plus one fals and a fifth of a fals. | ||
| − | |style="text-align:right;"|ותסיר השני שלישי דרהם מערך הבהמה ותשים במקומם שני שלישים ממה ששוה הדרהם והוא דבר ושליש ושני | + | |style="text-align:right;"|ותסיר השני שלישי דרהם מערך הבהמה ותשים במקומם שני שלישים ממה ששוה הדרהם והוא דבר ושליש ושני ח<sup>ו</sup>משי דבר ושליש וחומש אלפלס<br> |
| − | ויהיה ערך הבהמה שלשה דברים ושני חומשי דבר ופלס אחד וחומש | + | ויהיה ערך הבהמה שלשה דברים <s>ושלשה</s> <sup>ושני</sup> חומשי דבר ופלס אחד וחומש |
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| Line 3,294: | Line 3,617: | ||
| | | | ||
:We have already removed the dirham and placed two things and three-fifths of a thing, plus four-fifths of a fals instead. | :We have already removed the dirham and placed two things and three-fifths of a thing, plus four-fifths of a fals instead. | ||
| − | |style="text-align:right;"|וכבר לקחנו הדרהם ושמנו במקומו שני דברים ושלשה חומשי דבר וארבעה חומשי | + | |style="text-align:right;"|וכבר לקחנו הדרהם ושמנו במקומו שני דברים ושלשה חומשי דבר וארבעה חומשי אלפלס |
|- | |- | ||
| | | | ||
:Remove a third of the dirham from the value of the dinar and put a third of the worth of the dirham instead, which is two-thirds and a fifth of a thing, plus a fifth and a third of a fifth of a fals. The value of the dinar is two things and a fifth, plus three-fifths of a fals. | :Remove a third of the dirham from the value of the dinar and put a third of the worth of the dirham instead, which is two-thirds and a fifth of a thing, plus a fifth and a third of a fifth of a fals. The value of the dinar is two things and a fifth, plus three-fifths of a fals. | ||
| − | |style="text-align:right;"|ותגרע שלישית הדרהם אשר הוא בערך הדינר ותניח במקומו שלישית מה שישוה הדרהם והוא שני שלישי דבר וחומש דבר וחומש | + | |style="text-align:right;"|ותגרע שלישית הדרהם אשר הוא בערך הדינר ותניח במקומו שלישית מה שישוה הדרהם והוא שני שלישי דבר וחומש דבר וחומש אלפלס ושלישית חומש אלפלס<br> |
| − | ויהיה ערך הדינר שני דברים וחומש ושלשה חומשי | + | ויהיה ערך <s>הבהמה</s> הדינר שני דברים וחומש ושלשה חומשי אלפלס |
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| Line 3,329: | Line 3,652: | ||
| | | | ||
:A fifth of what they have is: a thing and four-fifths of a fifth of a thing, plus a fifth of a fals and two-fifths of a fifth of a fals. When we add them to the fals that he has, he gets one fals, a fifth and two-fifths of a fifth of a fals, plus a thing and four-fifths of a fifth of a thing. All this is equal to the price of the beast, which is three things and two-fifths of a thing, plus one fals and a fifth of a fals. | :A fifth of what they have is: a thing and four-fifths of a fifth of a thing, plus a fifth of a fals and two-fifths of a fifth of a fals. When we add them to the fals that he has, he gets one fals, a fifth and two-fifths of a fifth of a fals, plus a thing and four-fifths of a fifth of a thing. All this is equal to the price of the beast, which is three things and two-fifths of a thing, plus one fals and a fifth of a fals. | ||
| − | |style="text-align:right;"|וחמישית אשר להם הוא דבר וארבעה חומשי חמישית דבר וחמישית אלפלס ושני חומשי חמישית אלפלס וכאשר קבצנום עם האלפלס אשר הוא מחזיק יהיה לו אלפלס אחד וחמישית אלפלס ושני חומשי חמישית | + | |style="text-align:right;"|וחמישית אשר להם הוא דבר וארבעה חומשי חמישית דבר וחמישית אלפלס ושני חומשי חמישית אלפלס וכאשר קבצנום עם האלפלס אשר הוא מחזיק יהיה לו אלפלס אחד וחמישית אלפלס ושני חומשי חמישית אלפלס ודבר וארבעה חומשי חמשית דבר<br> |
| − | וישוה כל זה ערך הבהמה שהוא שלשה דברים ושני חומשי דבר ופלס | + | וישוה כל זה ‫<ref>79r</ref>ערך הבהמה שהוא שלשה דברים ושני חומשי דבר ופלס וחומש |
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| Line 3,336: | Line 3,659: | ||
|- | |- | ||
| | | | ||
| − | :<span style="color:Green>Confrontation:</span> Subtract a fals and a fifth of a fals from a fals, a fifth, and two-fifth of a fifth of a fals; two-fifths of a fifth of a fals remain. | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Subtract a fals and a fifth of a fals from a fals, a fifth, and two-fifth of a fifth of a fals; two-fifths of a fifth of a fals remain. | ||
:<math>\scriptstyle{\color{blue}{\left[1+\frac{1}{5}+\left(\frac{2}{5}\sdot\frac{1}{5}\right)\right]v-1\frac{1}{5}v=\frac{2}{5}\sdot\frac{1}{5}v}}</math> | :<math>\scriptstyle{\color{blue}{\left[1+\frac{1}{5}+\left(\frac{2}{5}\sdot\frac{1}{5}\right)\right]v-1\frac{1}{5}v=\frac{2}{5}\sdot\frac{1}{5}v}}</math> | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ותגרע פלס וחומש מפלס וחומש ושני חמשי חמשית פלס וישאר שני חומשי חמישית פלס |
|- | |- | ||
| | | | ||
:Subtract a thing and four-fifths of a fifth of a thing from three things and two-fifths of a thing; two things, a fifth and a fifth of a fifth of a thing remain that are equal to two-fifths of a fifth of a fals. | :Subtract a thing and four-fifths of a fifth of a thing from three things and two-fifths of a thing; two things, a fifth and a fifth of a fifth of a thing remain that are equal to two-fifths of a fifth of a fals. | ||
| + | |style="text-align:right;"|ותגרע דבר וארבעה חומשי חמישית דבר משלשה דברים ושני חומשי דבר וישאר שני דברים וחומש וחמישית חמישית הדבר וישוה שני חומשי חמישית אלפלס | ||
| + | |- | ||
| + | | colspan="2"| | ||
:<math>\scriptstyle{\color{blue}{3\frac{2}{5}x-\left[1+\left(\frac{4}{5}\sdot\frac{1}{5}\right)\right]x=\left[2+\frac{1}{5}x+\left(\frac{1}{5}\sdot\frac{1}{5}\right)\right]x=\frac{2}{5}\sdot\frac{1}{5}v}}</math> | :<math>\scriptstyle{\color{blue}{3\frac{2}{5}x-\left[1+\left(\frac{4}{5}\sdot\frac{1}{5}\right)\right]x=\left[2+\frac{1}{5}x+\left(\frac{1}{5}\sdot\frac{1}{5}\right)\right]x=\frac{2}{5}\sdot\frac{1}{5}v}}</math> | ||
| − | |||
|- | |- | ||
| | | | ||
| Line 3,363: | Line 3,692: | ||
:The third [has] twenty-five, since it is four-fifths of what the fourth has plus twice of what the first has and three-fifths of it. | :The third [has] twenty-five, since it is four-fifths of what the fourth has plus twice of what the first has and three-fifths of it. | ||
:<math>\scriptstyle{\color{blue}{u=2\frac{3}{5}x+\frac{4}{5}v=\left[\left(2+\frac{3}{5}\right)\sdot1\right]+\left(\frac{4}{5}\sdot28\right)=25}}</math> | :<math>\scriptstyle{\color{blue}{u=2\frac{3}{5}x+\frac{4}{5}v=\left[\left(2+\frac{3}{5}\right)\sdot1\right]+\left(\frac{4}{5}\sdot28\right)=25}}</math> | ||
| − | |style="text-align:right;"|והשלישי עשרים וחמשה בעבור כי הוא ארבעה חומשי מאשר לרביעי וכמו הכפל מהראשון ושלשה | + | |style="text-align:right;"|והשלישי עשרים וחמשה בעבור כי הוא ארבעה חומשי מאשר לרביעי וכמו הכפל מהראשון ושלשה חומשים יותר |
|- | |- | ||
| | | | ||
:The price of the beast is thirty-seven, since it is as what the fourth has and a fifth of it plus three times of what the first has and [two-fifths] of it. | :The price of the beast is thirty-seven, since it is as what the fourth has and a fifth of it plus three times of what the first has and [two-fifths] of it. | ||
:<math>\scriptstyle{\color{blue}{3\frac{{\color{red}{2}}}{5}x+1\frac{1}{5}v=\left[\left(3+\frac{2}{5}\right)\sdot1\right]+\left(1+\frac{1}{5}\sdot28\right)=37}}</math> | :<math>\scriptstyle{\color{blue}{3\frac{{\color{red}{2}}}{5}x+1\frac{1}{5}v=\left[\left(3+\frac{2}{5}\right)\sdot1\right]+\left(1+\frac{1}{5}\sdot28\right)=37}}</math> | ||
| − | |style="text-align:right;"|וערך הבהמה שלשים ושבע בעבור כי הוא כמו אשר לרביעי וכמו החומש וכמו שלשה | + | |style="text-align:right;"|וערך הבהמה שלשים ושבע בעבור כי הוא כמו אשר לרביעי וכמו החומש וכמו שלשה דמיוני אשר לראשון וכמו חמישיתו |
|- | |- | ||
|This procedure is the one that is known to the arithmeticians and it is a complete understanding, except that it is very subtle and the number is extracted with great difficulty. | |This procedure is the one that is known to the arithmeticians and it is a complete understanding, except that it is very subtle and the number is extracted with great difficulty. | ||
| Line 3,374: | Line 3,703: | ||
|- | |- | ||
|I solve this with a direct procedure and full understanding: | |I solve this with a direct procedure and full understanding: | ||
| − | |style="text-align:right;"|וכבר | + | |style="text-align:right;"|<big>וכבר</big> אוציאהו לאור אני עם מלאכה ישרה והבנה שלמה |
|- | |- | ||
| | | | ||
| − | : | + | :We suppose all they have is one dirham [<math>\scriptstyle{\color{blue}{y}}</math>]. |
|style="text-align:right;"|וזה שנניח כל אשר יחזיקו דרהם אחד | |style="text-align:right;"|וזה שנניח כל אשר יחזיקו דרהם אחד | ||
|- | |- | ||
| | | | ||
| − | : | + | :We suppose the first has a thing [<math>\scriptstyle{\color{blue}{x}}</math>] of this dirham. |
|style="text-align:right;"|ונניח שיהיה לאחד מזה הדרהם דבר | |style="text-align:right;"|ונניח שיהיה לאחד מזה הדרהם דבר | ||
|- | |- | ||
| Line 3,406: | Line 3,735: | ||
:Subtract a third of a dirham from half a dirham; two-thirds of a dinar remain equal to half a thing plus a sixth of a dirham. | :Subtract a third of a dirham from half a dirham; two-thirds of a dinar remain equal to half a thing plus a sixth of a dirham. | ||
:<math>\scriptstyle{\color{blue}{\frac{2}{3}u=\frac{1}{2}x+\frac{1}{2}y-\frac{1}{3}y=\frac{1}{2}x+\frac{1}{6}y}}</math> | :<math>\scriptstyle{\color{blue}{\frac{2}{3}u=\frac{1}{2}x+\frac{1}{2}y-\frac{1}{3}y=\frac{1}{2}x+\frac{1}{6}y}}</math> | ||
| − | |style="text-align:right;"|ותגרע שליש דרהם מחצי דרהם וישאר שני שלישי דינר | + | |style="text-align:right;"|ותגרע שליש דרהם מחצי דרהם וישאר שני שלישי דינר ישוו חצי דבר וששית דרהם |
|- | |- | ||
| | | | ||
:The dinar is three-quarters of a thing plus a quarter of a dirham. | :The dinar is three-quarters of a thing plus a quarter of a dirham. | ||
:<math>\scriptstyle{\color{blue}{u=\frac{3}{4}x+\frac{1}{4}y}}</math> | :<math>\scriptstyle{\color{blue}{u=\frac{3}{4}x+\frac{1}{4}y}}</math> | ||
| − | |style="text-align:right;"|והדינר שלשה רביעי דבר ורביע דרהם | + | |style="text-align:right;"|והדינר ישוה שלשה רביעי דבר ורביע דרהם |
|- | |- | ||
| | | | ||
| Line 3,428: | Line 3,757: | ||
:When we add its quarter to what the third has, which is a dinar, the price of the beast is three-quarters of a dinar plus a quarter of a dirham. This is equal to the price of the beast in the first stage, which is half a thing plus half a dirham. | :When we add its quarter to what the third has, which is a dinar, the price of the beast is three-quarters of a dinar plus a quarter of a dirham. This is equal to the price of the beast in the first stage, which is half a thing plus half a dirham. | ||
:<math>\scriptstyle{\color{blue}{u+\left[\frac{1}{4}\sdot\left(y-u\right)\right]=\frac{3}{4}u+\frac{1}{4}y=\frac{1}{2}x+\frac{1}{2}y}}</math> | :<math>\scriptstyle{\color{blue}{u+\left[\frac{1}{4}\sdot\left(y-u\right)\right]=\frac{3}{4}u+\frac{1}{4}y=\frac{1}{2}x+\frac{1}{2}y}}</math> | ||
| − | |style="text-align:right;"|וכאשר קבצנו רביעיתו עם אשר לשלישי שהוא דינר אחד יהיה ערך הבהמה שלשה רביעי דינר ורביעית דרהם<br> | + | |style="text-align:right;"|וכאשר קבצנו רביעיתו עם אשר לשלישי שהוא דינר אחד יהיה ערך הבהמה שלשה רביעי דינר ורביעית <s>ורביעית</s> דרהם<br> |
וישוה ערך הבהמה מהחלק האחד שהוא חצי דבר וחצי דרהם | וישוה ערך הבהמה מהחלק האחד שהוא חצי דבר וחצי דרהם | ||
|- | |- | ||
| Line 3,434: | Line 3,763: | ||
:Subtract a quarter of a dirham from half a dirham; half a thing plus a quarter of a dirham remain equal to three-quarters of a dinar. | :Subtract a quarter of a dirham from half a dirham; half a thing plus a quarter of a dirham remain equal to three-quarters of a dinar. | ||
:<math>\scriptstyle{\color{blue}{\frac{3}{4}u=\frac{1}{2}x+\frac{1}{2}y-\frac{1}{4}y=\frac{1}{2}x+\frac{1}{4}y}}</math> | :<math>\scriptstyle{\color{blue}{\frac{3}{4}u=\frac{1}{2}x+\frac{1}{2}y-\frac{1}{4}y=\frac{1}{2}x+\frac{1}{4}y}}</math> | ||
| − | |style="text-align:right;"|ותגרע רביעית דרהם מחצי דרהם וישאר חצי דבר ורביע דרהם ישוה שלשה רביעי דינר | + | |style="text-align:right;"|ותגרע רביעית דרהם מחצי דרהם וישאר ‫<ref>79v</ref>חצי דבר ורביע דרהם ישוה שלשה רביעי דינר |
|- | |- | ||
| | | | ||
| Line 3,443: | Line 3,772: | ||
| | | | ||
:The three have a total of two things, a quarter and a sixth of a thing, plus a third and a quarter of a dirham. | :The three have a total of two things, a quarter and a sixth of a thing, plus a third and a quarter of a dirham. | ||
| − | |style="text-align:right;"|ויהיה כל אשר לשלשה שני דברים ורביע ושתות | + | |style="text-align:right;"|ויהיה כל אשר לשלשה שני דברים ורביע ושתות דבר ושליש ורביע דרהם |
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| Line 3,457: | Line 3,786: | ||
| | | | ||
:The fourth man says to the three that have two things, a quarter and a sixth of a thing, plus a third and a quarter of a dirham: give me a fifth of what you have, I will add it to what I have and I will have the price of the beast. | :The fourth man says to the three that have two things, a quarter and a sixth of a thing, plus a third and a quarter of a dirham: give me a fifth of what you have, I will add it to what I have and I will have the price of the beast. | ||
| − | |style="text-align:right;"|ואמר זה האיש הרביעי אל השלשה המחזיקים שני דברים ורביע ושתות דבר ושליש ורביע דרהם תנו לי החומש מאשר לכם | + | |style="text-align:right;"|ואמר זה האיש הרביעי אל השלשה המחזיקים שני דברים ורביע ושתות דבר ושליש ורביע דרהם תנו לי החומש מאשר לכם ואקבצהו עם אשר לי ויהיה לי ערך הבהמה |
|- | |- | ||
| | | | ||
| − | :A fifth of what they have is | + | :A fifth of what they have is twenty-nine parts of sixty parts of a thing and 7 parts of 60 of a dirham. |
| − | |style="text-align:right;"|והחומש | + | |style="text-align:right;"|והחומש מאשר להם הוא עשרים ותשעה חלקים מששים <sup>חלקים</sup> <s>באחד</s> בדבר [ושבעה חלקים מששים בדרהם]‫<ref>Ma. marg.</ref> |
|- | |- | ||
| | | | ||
:When you add this to what he has, which is a quarter and a sixth of a dirham minus two things, a quarter and a sixth of a thing, the fourth has 32 parts of sixty of a dirham minus a thing and fifty-six parts of sixty parts of the thing, which is the price of the beast. This is equal to the price in the first stage, which is half a thing plus half a dirham. | :When you add this to what he has, which is a quarter and a sixth of a dirham minus two things, a quarter and a sixth of a thing, the fourth has 32 parts of sixty of a dirham minus a thing and fifty-six parts of sixty parts of the thing, which is the price of the beast. This is equal to the price in the first stage, which is half a thing plus half a dirham. | ||
| − | |style="text-align:right;"|וכאשר תקבצהו עם אשר לו שהוא רביע ושתות מדרהם פחות שני דברים ורביע ושתות מדבר יהיה אשר יחזיק הרביעי ל"ב חלקים מששים בדרהם | + | |style="text-align:right;"|וכאשר תקבצהו עם אשר לו שהוא רביע ושתות מדרהם פחות שני דברים ורביע ושתות מדבר יהיה אשר יחזיק הרביעי ל"ב חלקים מששים בדרהם פחות דבר וחמשים ושש<s>ים</s><sup>ה</sup> חלקים מששים חלקים בדבר והוא ערך הבהמה<br> |
וישוה לערך מהחלק האחד שהוא חצי דבר וחצי דרהם | וישוה לערך מהחלק האחד שהוא חצי דבר וחצי דרהם | ||
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| − | :<math>\scriptstyle{\color{blue}{\begin{align}&\scriptstyle\left[\left(\frac{1}{4}+\frac{1}{6}\right)y-\left(2+\frac{1}{4}+\frac{1}{6}\right)x\right]+\left[\frac{1}{5}\sdot\left[\left(2+\frac{1}{4}+\frac{1}{6}\right)x+\left(\frac{1}{3}+\frac{1}{4}\right)y\right]\right]\\&\scriptstyle=\left[\left(\frac{1}{4}+\frac{1}{6}\right)y-\left(2+\frac{1}{4}+\frac{1}{6}\right)x\right]+\left(\frac{29}{60}x+\frac{7}{60}y\right) | + | :<math>\scriptstyle{\color{blue}{\begin{align}&\scriptstyle\left[\left(\frac{1}{4}+\frac{1}{6}\right)y-\left(2+\frac{1}{4}+\frac{1}{6}\right)x\right]+\left[\frac{1}{5}\sdot\left[\left(2+\frac{1}{4}+\frac{1}{6}\right)x+\left(\frac{1}{3}+\frac{1}{4}\right)y\right]\right]\\&\scriptstyle=\left[\left(\frac{1}{4}+\frac{1}{6}\right)y-\left(2+\frac{1}{4}+\frac{1}{6}\right)x\right]+\left(\frac{29}{60}x+\frac{7}{60}y\right)=\frac{32}{60}y-1\frac{56}{60}x=\frac{1}{2}x+\frac{1}{2}y\\\end{align}}}</math> |
|- | |- | ||
| | | | ||
| − | :<span style="color:Green>Confrontation:</span> Confront them and it becomes after the confrontation a third of a tenth of a dirham equals two things, a third and a tenth of a thing. | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Confront them and it becomes after the confrontation a third of a tenth of a dirham equals two things, a third and a tenth of a thing. | ||
:<math>\scriptstyle{\color{blue}{\left(\frac{1}{3}\sdot\frac{1}{10}\right)y=\left(2+\frac{1}{3}+\frac{1}{10}\right)x}}</math> | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{3}\sdot\frac{1}{10}\right)y=\left(2+\frac{1}{3}+\frac{1}{10}\right)x}}</math> | ||
| − | |style="text-align:right;"|ותכוין בהם ויהיה אחר | + | |style="text-align:right;"|ותכוין בהם ויהיה אחר הכוון שלישית העשירית מדרהם ישוה שני דברים ושלישית דבר ועשירית דבר |
|- | |- | ||
| | | | ||
| − | :The dirham is equal to | + | :The dirham is equal to seventy-three things. |
:<math>\scriptstyle{\color{blue}{y=73x}}</math> | :<math>\scriptstyle{\color{blue}{y=73x}}</math> | ||
| − | |style="text-align:right;"|והדרהם ישוה | + | |style="text-align:right;"|והדרהם ישוה שבעים ושלשה דברים |
|- | |- | ||
| | | | ||
| Line 3,494: | Line 3,827: | ||
:The second has nineteen, since what he has is equal to a quarter of a dirham and three-quarters of a thing. | :The second has nineteen, since what he has is equal to a quarter of a dirham and three-quarters of a thing. | ||
:<math>\scriptstyle{\color{blue}{\frac{3}{4}x+\frac{1}{4}y=19}}</math> | :<math>\scriptstyle{\color{blue}{\frac{3}{4}x+\frac{1}{4}y=19}}</math> | ||
| − | |style="text-align:right;"|ואשר לשני יהיה תשע עשרה בעבור כי היה לו כדי אשר | + | |style="text-align:right;"|ואשר לשני יהיה תשע עשרה בעבור כי היה [לו כדי אשר]‫<ref>Ma. marg.</ref> שוה רביע דרהם ושלשה רביעי דבר |
|- | |- | ||
| | | | ||
| Line 3,502: | Line 3,835: | ||
|- | |- | ||
| | | | ||
| − | :The fourth has | + | :The fourth has twenty-eight, since what he has is equal to a quarter and a sixth of a dirham minus two things, a quarter and a sixth of a thing. |
:<math>\scriptstyle{\color{blue}{\left(\frac{1}{4}+\frac{1}{6}\right)y-\left(2+\frac{1}{4}+\frac{1}{6}\right)x=28}}</math> | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{4}+\frac{1}{6}\right)y-\left(2+\frac{1}{4}+\frac{1}{6}\right)x=28}}</math> | ||
| − | |style="text-align:right;"|ולרביעי | + | |style="text-align:right;"|ולרביעי עשרים ושמנה בעבור כי היה לו כדי רביע ושתות דרהם פחות שני דברים ורביע ושתות דבר |
|- | |- | ||
| | | | ||
| − | :If you wish, sum up what the first, the second, and the third have; it is | + | :If you wish, sum up what the first, the second, and the third have; it is forty-five. Subtract it from 73; what remains is what the fourth man has, which is twenty-eight. |
:<math>\scriptstyle{\color{blue}{73-\left(1+19+25\right)=73-45=28}}</math> | :<math>\scriptstyle{\color{blue}{73-\left(1+19+25\right)=73-45=28}}</math> | ||
| − | |style="text-align:right;"|ואם תרצה תקבץ אשר לראשון | + | |style="text-align:right;"|ואם תרצה תקבץ אשר לראשון ולשני ולשלישי ויהיה ארבעים וחמשה ותגרעם מע"ג ויהיה מה שישאר אשר לאיש הרביעי והוא עשרים ושמנה |
|- | |- | ||
| | | | ||
| − | :The price of the beast is | + | :The price of the beast is thirty-seven, since it is assumed to be half of a dirham plus half of what the first has. |
:<math>\scriptstyle{\color{blue}{\frac{1}{2}x+\frac{1}{2}y=37}}</math> | :<math>\scriptstyle{\color{blue}{\frac{1}{2}x+\frac{1}{2}y=37}}</math> | ||
| − | |style="text-align:right;"|וערך הבהמה | + | |style="text-align:right;"|וערך הבהמה שלשים ושבעה בעבור כי הונח חצי מה ששוה הדרהם וחצי מה שיש לראשון |
|- | |- | ||
|From now on you will be able to apply this procedure to whatever that falls into your hands of this kind and its mysteries will be solved. | |From now on you will be able to apply this procedure to whatever that falls into your hands of this kind and its mysteries will be solved. | ||
| Line 3,520: | Line 3,853: | ||
|- | |- | ||
| | | | ||
| − | :41) If you are told: five people gathered to buy a beast. | + | :{{#annot:five people, beast|664|EAjC}}41) If you are told: five people gathered to buy a beast. |
:The first said to the second and the third: give me half of what you have and I will have enough to buy it. | :The first said to the second and the third: give me half of what you have and I will have enough to buy it. | ||
:The second said to the third and the fourth: give me a third of what you have and I will have the price of the beast. | :The second said to the third and the fourth: give me a third of what you have and I will have the price of the beast. | ||
| Line 3,527: | Line 3,860: | ||
:The fifth said to the first and the second: give me a sixth of what you have and I will have the price of the beast | :The fifth said to the first and the second: give me a sixth of what you have and I will have the price of the beast | ||
:How much is the price of the beast and how much does each of the five have? | :How much is the price of the beast and how much does each of the five have? | ||
| − | |style="text-align:right;"|מא | + | |style="text-align:right;"|מא <big>ואם</big> יאמרו לך חמשה אנשים התקבצו יחד לקנות אלפאגה אחת<br> |
| − | ואמר הראשון | + | ואמר הראשון לשני ולשלישי תנו לי החצי מאשר לכם ויהיה לי ‫<ref>80r</ref>כדי לקנותה<br> |
ואמר השני לשלישי ולרביעי תנו לי שלישית אשר לכם ויהיה לי ערך הבהמה<br> | ואמר השני לשלישי ולרביעי תנו לי שלישית אשר לכם ויהיה לי ערך הבהמה<br> | ||
| − | ואמר השלישי לרביעי ולחמישי תנו לי רביעית אשר לכם ויהיה לי ערך | + | ואמר השלישי לרביעי ולחמישי תנו לי רביעית אשר לכם ויהיה לי ערך האלפאגה<br> |
ואמר הרביעי לחמישי ולראשון תנו לי חמישית אשר לכם ויהיה לי ערך האלפאגה<br> | ואמר הרביעי לחמישי ולראשון תנו לי חמישית אשר לכם ויהיה לי ערך האלפאגה<br> | ||
| − | ואמר החמישי לראשון | + | ואמר החמישי לראשון ולשני תנו לי הששית מאשר לכם ויהיה לי ערך הבהמה<br> |
| − | כמה יהיה ערך הבהמה וכמה יהיה לכל אחד מהחמשה | + | כמה יהיה ערך הבהמה וכמה יהיה לכל אחד מהחמשה{{#annotend:EAjC}} |
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| Line 3,539: | Line 3,872: | ||
|- | |- | ||
| | | | ||
| − | :To know this we | + | :To know this we assume the first has one thing [<math>\scriptstyle{\color{blue}{x}}</math>], the second a dinar [<math>\scriptstyle{\color{blue}{y}}</math>], and the third a dirham [<math>\scriptstyle{\color{blue}{u}}</math>]. |
| − | |style="width:45%; text-align:right;"|ולדעת זה נניח לראשון דבר ולשני דינר אחד ולשלישי דרהם אחד | + | |style="width:45%; text-align:right;"|ולדעת זה נניח לראשון דבר <sup>אחד</sup> ולשני דינר אחד ולשלישי דרהם אחד |
|- | |- | ||
| | | | ||
:We do not need more than these three species in this problem and its similar, in order to extract the number. | :We do not need more than these three species in this problem and its similar, in order to extract the number. | ||
| − | |style="text-align:right;"|ולא נצטרך בזאת השאלה ולדומים אליה כדי להוציא החשבון ליותר | + | |style="text-align:right;"|ולא נצטרך בזאת השאלה ולדומים אליה כדי להוציא החשבון ליותר מאלו השלשה מינים |
|- | |- | ||
| | | | ||
| Line 3,557: | Line 3,890: | ||
| | | | ||
:The second, who has one dinar, says to the third and the fourth: give me a third of what you have, and with what I have, which is one dinar, I will have the price of the beast, which is one thing, half a dinar, and half a dirham. | :The second, who has one dinar, says to the third and the fourth: give me a third of what you have, and with what I have, which is one dinar, I will have the price of the beast, which is one thing, half a dinar, and half a dirham. | ||
| − | |style="text-align:right;"|ואמר השני אשר לו דינר אחד לשלישי ולרביעי תנו לי שלישית החלק מאשר לכם ועם אשר לי שהוא דינר אחד אחזיק ערך הבהמה | + | |style="text-align:right;"|ואמר השני אשר לו דינר אחד לשלישי ולרביעי תנו לי שלישית החלק מאשר לכם ועם אשר לי שהוא דינר אחד אחזיק ערך הבהמה <s>ויהיה ערך הבהמה דינר אחד ושלישית דבר ושלישית דרהם ויהיה שוה לחלק האחד</s> שיהיה דבר אחד וחצי דינר וחצי דרהם |
|- | |- | ||
| | | | ||
:Subtract one dinar from the price of the beast; one thing and half a dirham remain minus half a dinar and this is a third of what the third and the fourth have. | :Subtract one dinar from the price of the beast; one thing and half a dirham remain minus half a dinar and this is a third of what the third and the fourth have. | ||
:<math>\scriptstyle{\color{blue}{\left(x+\frac{1}{2}y+\frac{1}{2}u\right)-y=x+\frac{1}{2}u-\frac{1}{2}y}}</math> | :<math>\scriptstyle{\color{blue}{\left(x+\frac{1}{2}y+\frac{1}{2}u\right)-y=x+\frac{1}{2}u-\frac{1}{2}y}}</math> | ||
| − | |style="text-align:right;"|ותגרע מערך הבהמה דינר אחד וישאר דבר אחד וחצי דרהם פחות חצי דינר והוא שליש מה שיחזיקו השלישי והרביעי | + | |style="text-align:right;"|ותגרע <s>מחצי ה</s> מערך הבהמה דינר אחד וישאר דבר אחד וחצי דרהם פחות חצי דינר והוא <sup>שליש</sup> מה שיחזיקו השלישי והרביעי |
|- | |- | ||
| | | | ||
:Multiply it by three; it is three things, a dirham and a half minus a dinar and a half and this is all that the third and the fourth have. | :Multiply it by three; it is three things, a dirham and a half minus a dinar and a half and this is all that the third and the fourth have. | ||
:<math>\scriptstyle{\color{blue}{3\sdot\left(x+\frac{1}{2}u-\frac{1}{2}y\right)=3x+1\frac{1}{2}u-1\frac{1}{2}y}}</math> | :<math>\scriptstyle{\color{blue}{3\sdot\left(x+\frac{1}{2}u-\frac{1}{2}y\right)=3x+1\frac{1}{2}u-1\frac{1}{2}y}}</math> | ||
| − | |style="text-align:right;"|ותכהו בשלשה ויהיה שלשה דברים ודרהם אחד וחצי פחות דינר וחצי והוא כל אשר יחזיקו השלישי והרביעי | + | |style="text-align:right;"|‫[ותכהו בשלשה ויהיה שלשה דברים ודרהם אחד וחצי פחות דינר וחצי והוא כל אשר יחזיקו השלישי והרביעי |
|- | |- | ||
| | | | ||
:Subtract what the third has, which is one dirham; what the fourth has remains, which is three things and half a dirham minus one dinar and a half. | :Subtract what the third has, which is one dirham; what the fourth has remains, which is three things and half a dirham minus one dinar and a half. | ||
:<math>\scriptstyle{\color{blue}{\left(3x+1\frac{1}{2}u-1\frac{1}{2}y\right)-u=3x+\frac{1}{2}u-1\frac{1}{2}y}}</math> | :<math>\scriptstyle{\color{blue}{\left(3x+1\frac{1}{2}u-1\frac{1}{2}y\right)-u=3x+\frac{1}{2}u-1\frac{1}{2}y}}</math> | ||
| − | |style="text-align:right;"|ותגרע אשר לשלישי שהוא דרהם אחד וישאר מה שהוא לרביעי והוא שלשה דברים וחצי דרהם פחות דינר אחד וחצי | + | |style="text-align:right;"|ותגרע אשר לשלישי שהוא דרהם אחד וישאר מה שהוא לרביעי והוא שלשה דברים וחצי דרהם פחות דינר אחד וחצי]‫<ref>Ma. marg</ref> <s>ותגרע אשר לשלישי שהוא דרהם ישאר אשר לרביעי והוא דבר וחצי דרהם דרהם דבר אחד פחות חצי דרהם ופחות חצי דינר</s> |
|- | |- | ||
| | | | ||
| Line 3,581: | Line 3,914: | ||
:Subtract what the third has, which is one dinar, from the price of the beast; one thing and half a dinar remain minus half a dirham and this is a quarter of what the fourth and the fifth have. | :Subtract what the third has, which is one dinar, from the price of the beast; one thing and half a dinar remain minus half a dirham and this is a quarter of what the fourth and the fifth have. | ||
:<math>\scriptstyle{\color{blue}{\left(x+\frac{1}{2}y+\frac{1}{2}u\right)-u=x+\frac{1}{2}y-\frac{1}{2}u}}</math> | :<math>\scriptstyle{\color{blue}{\left(x+\frac{1}{2}y+\frac{1}{2}u\right)-u=x+\frac{1}{2}y-\frac{1}{2}u}}</math> | ||
| − | |style="text-align:right;"|ותגרע מערך הבהמה אשר לשלישי שהוא דרהם אחד וישאר דבר וחצי דינר פחות חצי דרהם והוא רביעית אשר לרביעי | + | |style="text-align:right;"|ותגרע מערך הבהמה אשר לשלישי שהוא דרהם אחד וישאר דבר וחצי דינר פחות חצי דרהם והוא רביעית אשר לרביעי ולחמישי |
|- | |- | ||
| | | | ||
| Line 3,590: | Line 3,923: | ||
| | | | ||
:Subtract from it what the fourth has, which is three things and half a dirham minus one dinar and a half; what the fifth has remains, which is one thing and three dinar and a half minus two dirham and a half. | :Subtract from it what the fourth has, which is three things and half a dirham minus one dinar and a half; what the fifth has remains, which is one thing and three dinar and a half minus two dirham and a half. | ||
| + | |style="text-align:right;"|ותגרע מהם מה שיש לרביעי שהוא שלשה דברים וחצי דרהם פחות דינר אחד וחצי וישאר מה שיש לחמישי דבר אחד ושלשה דינרים וחצי פחות שני דרהמי וחצי | ||
| + | |- | ||
| + | | colspan="2"| | ||
:<math>\scriptstyle{\color{blue}{\left(4x+2y-2u\right)-\left(3x+\frac{1}{2}u-1\frac{1}{2}y\right)=x+3\frac{1}{2}y-2\frac{1}{2}u}}</math> | :<math>\scriptstyle{\color{blue}{\left(4x+2y-2u\right)-\left(3x+\frac{1}{2}u-1\frac{1}{2}y\right)=x+3\frac{1}{2}y-2\frac{1}{2}u}}</math> | ||
| − | |||
|- | |- | ||
| | | | ||
:The fourth, who has three things and half a dirham minus one dinar and a half, says to the fifth and the first, who have two things and three dinar and a half minus two dirham and a half: give me a fifth of what you have, and with what I have, I will have the price of the beast. | :The fourth, who has three things and half a dirham minus one dinar and a half, says to the fifth and the first, who have two things and three dinar and a half minus two dirham and a half: give me a fifth of what you have, and with what I have, I will have the price of the beast. | ||
| − | |style="text-align:right;"|ואמר הרביעי המחזיק שלשה דברים וחצי דרהם פחות דינר אחד וחצי לחמישי ולראשון המחזיקים שני דברים ושלשה דינרים וחצי פחות שני דרהמי וחצי תנו לי החמישית מאשר לכם ועם אשר לי יהיה בידי כמו ערך הבהמה | + | |style="text-align:right;"|ואמר הרביעי המחזיק שלשה דברים וחצי דרהם ‫<ref>80v</ref>פחות דינר אחד וחצי לחמישי ולראשון המחזיקים שני דברים ושלשה דינרים וחצי פחות שני דרהמי וחצי תנו לי החמישית מאשר לכם ועם אשר לי יהיה בידי כמו ערך הבהמה |
|- | |- | ||
| | | | ||
:A fifth of what they have is two-fifths of a thing and seven-tenths of a dinar minus half a dirham. | :A fifth of what they have is two-fifths of a thing and seven-tenths of a dinar minus half a dirham. | ||
| − | |style="text-align:right;"|וחמישית אשר להם הוא שני חומשי דבר ושבעה עשיריות | + | |style="text-align:right;"|וחמישית אשר להם הוא שני חומשי דבר <s>וששה</s> <sup>ושבעה</sup> עשיריות מדינר פחות חצי דרהם |
|- | |- | ||
| | | | ||
:When you add this to what he has, it is three things and two-fifths minus four-fifths of a dinar. | :When you add this to what he has, it is three things and two-fifths minus four-fifths of a dinar. | ||
| − | |style="text-align:right;"|וכאשר תקבצם עם אשר לו | + | |style="text-align:right;"|וכאשר תקבצם עם אשר לו יהיו בידו שלשה דברים ושני חומשים פחות ארבעה חומשי דינר <s>חומש מדינר</s> |
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| Line 3,611: | Line 3,946: | ||
:This is equal to the price of the beast in the previous stage, which is a thing and half a dinar plus half a dirham. | :This is equal to the price of the beast in the previous stage, which is a thing and half a dinar plus half a dirham. | ||
:<math>\scriptstyle{\color{blue}{x+\frac{1}{2}y+\frac{1}{2}u=3\frac{2}{5}x-\frac{4}{5}y}}</math> | :<math>\scriptstyle{\color{blue}{x+\frac{1}{2}y+\frac{1}{2}u=3\frac{2}{5}x-\frac{4}{5}y}}</math> | ||
| − | |style="text-align:right;"|והוא שוה לערך הבהמה מהחלק האחר | + | |style="text-align:right;"|והוא שוה לערך הבהמה מהחלק האחר שהיה דבר וחצי דינר וחצי דרהם |
|- | |- | ||
| | | | ||
| − | :<span style="color:Green>Confrontation:</span> Confront them and the dirham becomes equal to four things and four-fifths of a thing minus two dinar and three-fifths of a dinar. | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Confront them and the dirham becomes equal to four things and four-fifths of a thing minus two dinar and three-fifths of a dinar. | ||
:<math>\scriptstyle{\color{blue}{u=4\frac{4}{5}x-2\frac{3}{5}y}}</math> | :<math>\scriptstyle{\color{blue}{u=4\frac{4}{5}x-2\frac{3}{5}y}}</math> | ||
|style="text-align:right;"|ותכוין בהם ויהיה הדרהם יהיה שוה ארבעה דברים וארבעה חומשי דבר פחות שני דינרים ושלשה חומשי דינר | |style="text-align:right;"|ותכוין בהם ויהיה הדרהם יהיה שוה ארבעה דברים וארבעה חומשי דבר פחות שני דינרים ושלשה חומשי דינר | ||
| Line 3,620: | Line 3,959: | ||
| | | | ||
:Subtract half a dirham from the price of the beast and replace it with half the value of the dirham, which is two things and two-fifths of a thing minus one dinar and three-tenths of a dinar; the price of the beast is three things and two-fifths of a thing minus four-fifths of a dinar. | :Subtract half a dirham from the price of the beast and replace it with half the value of the dirham, which is two things and two-fifths of a thing minus one dinar and three-tenths of a dinar; the price of the beast is three things and two-fifths of a thing minus four-fifths of a dinar. | ||
| − | |style="text-align:right;"|ותגרע חצי הדרהם אשר בערך הבהמה ותניח במקומו חצי מה ששוה הדרהם והוא שני דברים ושני חומשי דבר פחות דינר אחד ושלשה | + | |style="text-align:right;"|ותגרע חצי הדרהם אשר בערך הבהמה ותניח במקומו חצי מה ששוה הדרהם והוא שני דברים ושני חומשי דבר פחות דינר אחד ושלשה עשיריות מדינר ויהיה ערך הבהמה שלשה דברים ושני חומשי דבר פחות ארבעה חומשי דינר |
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| Line 3,649: | Line 3,988: | ||
:As we have explained, the fifth should have ten dinar minus eleven things. | :As we have explained, the fifth should have ten dinar minus eleven things. | ||
:<math>\scriptstyle{\color{blue}{x+3\frac{1}{2}y-2\frac{1}{2}u=10y-11x}}</math> | :<math>\scriptstyle{\color{blue}{x+3\frac{1}{2}y-2\frac{1}{2}u=10y-11x}}</math> | ||
| − | |style="text-align:right;"|וצריך הוא כפי מה | + | |style="text-align:right;"|וצריך הוא כפי מה שבארנו לחמישי עשרה דינרי' פחות אחד עשרה דברים |
|- | |- | ||
| | | | ||
:He says to the first and the second, who have one thing and one dinar: give me a sixth of what you have, I will add it to what I have, and I will have the price of the beast. | :He says to the first and the second, who have one thing and one dinar: give me a sixth of what you have, I will add it to what I have, and I will have the price of the beast. | ||
| − | |style="text-align:right;"|ואמר לראשון ולשני אשר להם דבר | + | |style="text-align:right;"|ואמר לראשון ולשני אשר להם דבר ודינר אחד תנו לי הששית מאשר לכם ואקבצהו עם אשר לי ויהיה לי ערך הבהמה |
|- | |- | ||
| | | | ||
| Line 3,669: | Line 4,008: | ||
:This is equal to the price of the beast in the previous stage, which is three things and two-fifths of a thing minus four-fifths of a dinar. | :This is equal to the price of the beast in the previous stage, which is three things and two-fifths of a thing minus four-fifths of a dinar. | ||
:<math>\scriptstyle{\color{blue}{3\frac{2}{5}x-\frac{4}{5}y=10\frac{1}{6}y-10\frac{5}{6}x}}</math> | :<math>\scriptstyle{\color{blue}{3\frac{2}{5}x-\frac{4}{5}y=10\frac{1}{6}y-10\frac{5}{6}x}}</math> | ||
| − | |style="text-align:right;"|וישוה לערך הבהמה מהחלק האחר שהוא | + | |style="text-align:right;"|וישוה לערך הבהמה מהחלק האחר שהוא שלשה דברים ושני חומשי דבר פחות ארבעה חומשי דינר |
|- | |- | ||
| | | | ||
| − | :<span style="color:Green>Confrontation:</span> Confront them and the thing becomes forty-seven after the confrontation, which is what the first has. | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Confront them and the thing becomes forty-seven after the confrontation, which is what the first has. | ||
:<math>\scriptstyle{\color{blue}{x=47}}</math> | :<math>\scriptstyle{\color{blue}{x=47}}</math> | ||
| − | |style="text-align:right;"|ותכוין בהם | + | |style="text-align:right;"|ותכוין בהם ויהיה אחרי זה <sup>ה</sup>כוון הדבר ארבעים ושבעה והוא אשר לראשון |
|- | |- | ||
| | | | ||
| Line 3,684: | Line 4,027: | ||
:The third has sixty-seven, because it is four times and four-fifths of what the first has minus two times and three-fifths of what the second has. | :The third has sixty-seven, because it is four times and four-fifths of what the first has minus two times and three-fifths of what the second has. | ||
:<math>\scriptstyle{\color{blue}{4\frac{4}{5}x-2\frac{3}{5}y=67}}</math> | :<math>\scriptstyle{\color{blue}{4\frac{4}{5}x-2\frac{3}{5}y=67}}</math> | ||
| − | |style="text-align:right;"|ואשר לשלישי ששים ושבעה בעבור כי הוא ארבעה | + | |style="text-align:right;"|ואשר לשלישי ששים ושבעה בעבור כי הוא ארבעה <s>כפלים</s><sup>דמיונים</sup> וארבעה חומשים ממה שיש לראשון פחות שני ‫<ref>81r</ref>דמיונים ושלשה חומשים ממה שיש לשני |
|- | |- | ||
| | | | ||
:The fourth has eighty-three, because he has five times and two-fifths of what the first has minus two times and four-fifths of what the second has. | :The fourth has eighty-three, because he has five times and two-fifths of what the first has minus two times and four-fifths of what the second has. | ||
:<math>\scriptstyle{\color{blue}{5\frac{2}{5}x-2\frac{4}{5}y=83}}</math> | :<math>\scriptstyle{\color{blue}{5\frac{2}{5}x-2\frac{4}{5}y=83}}</math> | ||
| − | |style="text-align:right;"|ולרביעי | + | |style="text-align:right;"|ולרביעי שמנים ושלשה בעבור כי לו חמשה דמיונים <s>וב</s> ושני חומשים ממה שיש לראשון פחות שני דמיונים וארבעה חומשים ממה שיש לשני |
|- | |- | ||
| | | | ||
:The fifth has ninety-three, because it is ten times of what the second has minus eleven times of what the first has. | :The fifth has ninety-three, because it is ten times of what the second has minus eleven times of what the first has. | ||
:<math>\scriptstyle{\color{blue}{10y-11x=93}}</math> | :<math>\scriptstyle{\color{blue}{10y-11x=93}}</math> | ||
| − | |style="text-align:right;"|ולחמישי תשעים ושלשה בעבור כי הוא עשרה | + | |style="text-align:right;"|ולחמישי תשעים ושלשה בעבור כי הוא עשרה דמיונים מאשר לשני פחות אחד עשר דמיוני <sup>אשר אל</sup> הראשון |
|- | |- | ||
| | | | ||
:The price of the beast is 111, because it is three times and two-fifths of what the first has minus four-fifths of what the second has. | :The price of the beast is 111, because it is three times and two-fifths of what the first has minus four-fifths of what the second has. | ||
:<math>\scriptstyle{\color{blue}{3\frac{2}{5}x-\frac{4}{5}y=111}}</math> | :<math>\scriptstyle{\color{blue}{3\frac{2}{5}x-\frac{4}{5}y=111}}</math> | ||
| − | |style="text-align:right;"|וערך הבהמה קי"א בעבור כי הוא שלשה | + | |style="text-align:right;"|וערך הבהמה קי"א בעבור כי הוא שלשה דמיונים ושני חומשים מאשר לראשון פחות ארבעה חומשים מאשר לשני |
|- | |- | ||
| | | | ||
| Line 3,712: | Line 4,055: | ||
:Subtract what the second has from the price of the beast; fifty remains. Multiply it by three; it is 150. Subtract from it what the third has, which is 67; the remainder is what the fourth has, which is 83. | :Subtract what the second has from the price of the beast; fifty remains. Multiply it by three; it is 150. Subtract from it what the third has, which is 67; the remainder is what the fourth has, which is 83. | ||
:<math>\scriptstyle{\color{blue}{\left[3\sdot\left(111-61\right)\right]-67=\left(3\sdot50\right)-67=150-67=83}}</math> | :<math>\scriptstyle{\color{blue}{\left[3\sdot\left(111-61\right)\right]-67=\left(3\sdot50\right)-67=150-67=83}}</math> | ||
| − | |style="text-align:right;"|ותגרע מה שיש לשני מערך הבהמה וישאר | + | |style="text-align:right;"|ותגרע מה שיש לשני מערך הבהמה וישאר חמשים ותכם בשלשה ויהיה ק"נ ותגרע מהם מה שיש לשלישי והוא ס"ז ישאר אשר לרביעי פ"ג |
|- | |- | ||
| | | | ||
:If you want to know what the fifth has: subtract what the third has from the price of the beast; 44 remains. Multiply it by four; it is 176. Subtract from it what the fourth has, which is 83; 93 remains and this is what the fifth has. | :If you want to know what the fifth has: subtract what the third has from the price of the beast; 44 remains. Multiply it by four; it is 176. Subtract from it what the fourth has, which is 83; 93 remains and this is what the fifth has. | ||
:<math>\scriptstyle{\color{blue}{\left[4\sdot\left(111-67\right)\right]-83=\left(4\sdot44\right)-83=176-83=93}}</math> | :<math>\scriptstyle{\color{blue}{\left[4\sdot\left(111-67\right)\right]-83=\left(4\sdot44\right)-83=176-83=93}}</math> | ||
| − | |style="text-align:right;"|ואם בקשת לדעת אשר לחמישי תגרע מה שיש לשלישי מערך הבהמה וישאר מ"ד ותכם | + | |style="text-align:right;"|ואם בקשת לדעת אשר לחמישי תגרע מה שיש לשלישי מערך הבהמה וישאר מ"ד ותכם בארבעה ויהיה קע"ו ותגרע מהם מה שיש לרביעי והוא פ"ג וישאר צ"ג והוא מה שיש לחמישי |
|- | |- | ||
| | | | ||
:If you wish, suppose the first has one thing [<math>\scriptstyle{\color{blue}{x}}</math>]; the second [has] one dinar [<math>\scriptstyle{\color{blue}{y}}</math>]; the third [has] one dirham [<math>\scriptstyle{\color{blue}{u}}</math>]; and the fourth [has] one fals [<math>\scriptstyle{\color{blue}{v}}</math>]. | :If you wish, suppose the first has one thing [<math>\scriptstyle{\color{blue}{x}}</math>]; the second [has] one dinar [<math>\scriptstyle{\color{blue}{y}}</math>]; the third [has] one dirham [<math>\scriptstyle{\color{blue}{u}}</math>]; and the fourth [has] one fals [<math>\scriptstyle{\color{blue}{v}}</math>]. | ||
| − | |style="text-align:right;"|ואם תרצה תניח לראשון דבר אחד ולשני דינר אחד ולשלישי דרהם אחד ולרביעי אלפלס אחד | + | |style="text-align:right;"|<big>ואם</big> תרצה תניח לראשון דבר אחד ולשני דינר אחד ולשלישי דרהם אחד ולרביעי אלפלס אחד |
|- | |- | ||
|In this question and [others] similar to it there is no need for more than these four [algebraic] species to extract the numbers. | |In this question and [others] similar to it there is no need for more than these four [algebraic] species to extract the numbers. | ||
| − | |style="text-align:right;"|ולא יצטרך לזאת השאלה ולדומים אליה כדי להוציא החשבון ליותר | + | |style="text-align:right;"|ולא יצטרך לזאת השאלה ולדומים אליה כדי להוציא החשבון ליותר מאלו הארבעה מינים |
|- | |- | ||
| | | | ||
:The first says to the second and the third: give me a half of what you have, I will add it to what I have, and I will have the price of the beast. | :The first says to the second and the third: give me a half of what you have, I will add it to what I have, and I will have the price of the beast. | ||
| − | |style="text-align:right;"|ואמר הראשון לשני ולשלישי תנו לי | + | |style="text-align:right;"|ואמר הראשון לשני ולשלישי תנו לי חצי אשר לכם ואקבצהו עם אשר לי ויהיה עמי ערך הבהמה |
|- | |- | ||
| | | | ||
:So, the price of the beast is a thing, half a dinar and half a dirham. | :So, the price of the beast is a thing, half a dinar and half a dirham. | ||
:<math>\scriptstyle{\color{blue}{x+\frac{1}{2}y+\frac{1}{2}u}}</math> | :<math>\scriptstyle{\color{blue}{x+\frac{1}{2}y+\frac{1}{2}u}}</math> | ||
| − | |style="text-align:right;"|ויהיה ערך הבהמה דבר וחצי דינר וחצי | + | |style="text-align:right;"|ויהיה ערך הבהמה דבר וחצי דינר וחצי דרהם |
|- | |- | ||
| | | | ||
:The second says to the third and the fourth: give me a third of what you have; with what I have I will have the price of the beast. | :The second says to the third and the fourth: give me a third of what you have; with what I have I will have the price of the beast. | ||
| − | |style="text-align:right;"|ואמר השני לשלישי ולרביעי תנו לי שלישית אשר לכם ועם אשר לי יהיה לי ערך הבהמה | + | |style="text-align:right;"|ואמר השני לשלישי ולרביעי תנו לי שלישית אשר לכם ועם אשר לי יהיה [לי]‫<ref>Ma. om.</ref> ערך הבהמה |
|- | |- | ||
| | | | ||
| Line 3,750: | Line 4,093: | ||
|- | |- | ||
| | | | ||
| − | :<span style="color:Green>Confrontation:</span> Confront them and the fals becomes equal to three things and half a dirham minus one dinar and a half, which is what the fourth has. | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Confront them and the fals becomes equal to three things and half a dirham minus one dinar and a half, which is what the fourth has. | ||
:<math>\scriptstyle{\color{blue}{v=3x+\frac{1}{2}u-1\frac{1}{2}y}}</math> | :<math>\scriptstyle{\color{blue}{v=3x+\frac{1}{2}u-1\frac{1}{2}y}}</math> | ||
|style="text-align:right;"|ותכוין בהם ויהיה האלפלס ישוה שלשה דברים וחצי דרהם פחות דינר וחצי והוא אשר לרביעי | |style="text-align:right;"|ותכוין בהם ויהיה האלפלס ישוה שלשה דברים וחצי דרהם פחות דינר וחצי והוא אשר לרביעי | ||
| Line 3,764: | Line 4,111: | ||
| | | | ||
:A quarter of what they have is three-quarters of a thing, a quarter of a fals and an eighth of a dinar minus 3-eighths of a dinar. | :A quarter of what they have is three-quarters of a thing, a quarter of a fals and an eighth of a dinar minus 3-eighths of a dinar. | ||
| − | |style="text-align:right;"|ורביעית אשר להם | + | |style="text-align:right;"|ורביעית אשר להם הוא שלשה רביעי דבר ורביעית אלפלס ושמינית דרהם פחות שלשה שמיניות מדינר |
|- | |- | ||
| | | | ||
| − | :When it is added to the dirham he has, [the sum] is the same as the price of the beast. So, the price of the beast in this stage is one dirham, an eighth of a dirham, a quarter of a fals, and three-quarters of a thing minus three-eighths of a dinar. | + | :When it is added to the dirham he has, [the sum] is [the same as] the price of the beast. So, the price of the beast in this stage is one dirham, an eighth of a dirham, a quarter of a fals, and three-quarters of a thing minus three-eighths of a dinar. |
| − | |style="text-align:right;"|וכאשר קובצו עם הדרהם אשר לו יהיה בידו | + | |style="text-align:right;"|וכאשר קובצו עם הדרהם אשר לו יהיה בידו ערך הבהמה והוא ויהיה ערך הבהמה מזה החלק דרהם אחד ‫<ref>81v</ref>ושמינית אחד מדרהם ורביע אלפלס ושלשה רביעי דבר פחות שלשה שמיניו' מדינר |
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| Line 3,776: | Line 4,123: | ||
:This is equal to the price of the beast in the first stage, which is a thing, half a dinar and half a dirham. | :This is equal to the price of the beast in the first stage, which is a thing, half a dinar and half a dirham. | ||
:<math>\scriptstyle{\color{blue}{x+\frac{1}{2}y+\frac{1}{2}u=1\frac{1}{8}u+\frac{1}{4}v+\frac{3}{4}x-\frac{3}{8}y}}</math> | :<math>\scriptstyle{\color{blue}{x+\frac{1}{2}y+\frac{1}{2}u=1\frac{1}{8}u+\frac{1}{4}v+\frac{3}{4}x-\frac{3}{8}y}}</math> | ||
| − | |style="text-align:right;"|ויהיה שוה לערך הבהמה מהחלק | + | |style="text-align:right;"|ויהיה שוה לערך הבהמה מהחלק ראשון שהוא דבר וחצי דינר וחצי דרהם |
|- | |- | ||
| | | | ||
| − | :<span style="color:Green>Confrontation:</span> Confront them and the fals becomes equal to a thing plus three dinar and a half minus two dirham and a half, which is what the fifth has. | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Confront them and the fals becomes equal to a thing plus three dinar and a half minus two dirham and a half, which is what the fifth has. | ||
:<math>\scriptstyle{\color{blue}{v=x+3\frac{1}{2}y-2\frac{1}{2}u}}</math> | :<math>\scriptstyle{\color{blue}{v=x+3\frac{1}{2}y-2\frac{1}{2}u}}</math> | ||
|style="text-align:right;"|ותכוין בהם ויהיה אלפלס ישוה דבר ושלשה דינרי' וחצי פחות שני דרהמי וחצי והוא אשר לחמישי | |style="text-align:right;"|ותכוין בהם ויהיה אלפלס ישוה דבר ושלשה דינרי' וחצי פחות שני דרהמי וחצי והוא אשר לחמישי | ||
| Line 3,785: | Line 4,136: | ||
| | | | ||
:Do as I showed you with the rest of the question. | :Do as I showed you with the rest of the question. | ||
| − | |style="text-align:right;"|ותעשה הנשאר מן השאלה כפי מה | + | |style="text-align:right;"|ותעשה הנשאר מן השאלה כפי מה שהראיתיך |
|- | |- | ||
| | | | ||
| Line 3,796: | Line 4,147: | ||
:How much is the price of the beast and how much does each of them have? | :How much is the price of the beast and how much does each of them have? | ||
:<math>\scriptstyle{\color{blue}{a+\frac{1}{2}b=b+\frac{1}{3}c=c+\frac{1}{4}d=d+\frac{1}{5}e=e+\frac{1}{6}a}}</math> | :<math>\scriptstyle{\color{blue}{a+\frac{1}{2}b=b+\frac{1}{3}c=c+\frac{1}{4}d=d+\frac{1}{5}e=e+\frac{1}{6}a}}</math> | ||
| − | |style="text-align:right;"|מב | + | |style="text-align:right;"|מב <big>ואם</big> יאמרו לך חמשה אנשים התקבצו ואמר הראשון לשני אם תתן לי החצי מאשר בידך יהיה לי ערך הבהמה<br> |
ואמר השני לשלישי אם תתן לי השלישית מאשר בידך יהיה לי ערך הבהמה<br> | ואמר השני לשלישי אם תתן לי השלישית מאשר בידך יהיה לי ערך הבהמה<br> | ||
ואמר השלישי לרביעי אם תתן לי הרביעית מאשר בידך יהיה לי ערך הבהמה<br> | ואמר השלישי לרביעי אם תתן לי הרביעית מאשר בידך יהיה לי ערך הבהמה<br> | ||
| Line 3,831: | Line 4,182: | ||
|- | |- | ||
| | | | ||
| − | :<span style="color:Green>Confrontation:</span> Confront them and the dirham becomes equal to three things minus one dinar and a half, which is what the third has. | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Confront them and the dirham becomes equal to three things minus one dinar and a half, which is what the third has. | ||
:<math>\scriptstyle{\color{blue}{u=3x-1\frac{1}{2}y}}</math> | :<math>\scriptstyle{\color{blue}{u=3x-1\frac{1}{2}y}}</math> | ||
|style="text-align:right;"|ותכוין בהם ויהיה הדרהם ישוה שלשה דברים פחות דינר אחד וחצי והוא אשר לשלישי | |style="text-align:right;"|ותכוין בהם ויהיה הדרהם ישוה שלשה דברים פחות דינר אחד וחצי והוא אשר לשלישי | ||
| Line 3,840: | Line 4,195: | ||
|- | |- | ||
| | | | ||
| − | :The third, who has three things minus a dinar and a half, says to the fourth, who has one dirham: if you give me a quarter of what you have, | + | :The third, who has three things minus a dinar and a half, says to the fourth, who has one dirham: if you give me a quarter of what you have, I will have the price of the beast. |
| − | |style="text-align:right;"|ואמר השלישי אשר לו שלשה דברים פחות דינר וחצי | + | |style="text-align:right;"|ואמר השלישי אשר לו שלשה דברים פחות דינר וחצי לרביעי אשר לו דרהם אחד אם תתן לי הרביעית מאשר לך יהיה לי ערך הבהמה |
|- | |- | ||
| | | | ||
| Line 3,851: | Line 4,206: | ||
:This is equal to the price of the beast in the first stage, which is a thing and half a dinar. | :This is equal to the price of the beast in the first stage, which is a thing and half a dinar. | ||
:<math>\scriptstyle{\color{blue}{x+\frac{1}{2}y=3x+\frac{1}{4}u-1\frac{1}{2}y}}</math> | :<math>\scriptstyle{\color{blue}{x+\frac{1}{2}y=3x+\frac{1}{4}u-1\frac{1}{2}y}}</math> | ||
| − | |style="text-align:right;"|וישוה ערך הבהמה | + | |style="text-align:right;"|וישוה ערך הבהמה מהחלק האחד שהוא דבר וחצי דינר |
| + | |- | ||
| + | | | ||
| + | :*<span style="color:Green>Confrontation:</span> | ||
| + | | | ||
|- | |- | ||
| | | | ||
| − | : | + | :Confront them and the dirham becomes equal to eight dinar minus eight things, which is what the fourth has. |
:<math>\scriptstyle{\color{blue}{u=8y-8x}}</math> | :<math>\scriptstyle{\color{blue}{u=8y-8x}}</math> | ||
| − | |style="text-align:right;"|ותכוין עמו ויהיה הדרהם | + | |style="text-align:right;"|ותכוין עמו ויהיה הדרהם ישוה שמנה דינרים פחות שמנה דברים והוא אשר לרביעי |
|- | |- | ||
| | | | ||
| Line 3,864: | Line 4,223: | ||
| | | | ||
:The fourth, who has eight dinar minus eight things, says to the fifth, who has one dirham: if you give me a fifth of what you have, I will have the price of the beast. | :The fourth, who has eight dinar minus eight things, says to the fifth, who has one dirham: if you give me a fifth of what you have, I will have the price of the beast. | ||
| − | |style="text-align:right;"|ואמר הרביעי אשר לו שמנה דינרים פחות שמנה דברים לחמישי אשר לו דרהם אחד אם תתן לי החמישית מאשר לך יהיה לי ערך הבהמה | + | |style="text-align:right;"|ואמר הרביעי אשר לו שמנה דינרים פחות שמנה דברים לחמישי אשר לו דרהם אחד אם תתן לי ‫<ref>82r</ref>החמישית מאשר לך יהיה לי ערך הבהמה |
|- | |- | ||
| | | | ||
:Then, the price of the beast in this stage is eight dinar and a fifth of a dirham minus eight things. | :Then, the price of the beast in this stage is eight dinar and a fifth of a dirham minus eight things. | ||
:<math>\scriptstyle{\color{blue}{\left(8y-8x\right)+\frac{1}{5}u=8y+\frac{1}{5}u-8x}}</math> | :<math>\scriptstyle{\color{blue}{\left(8y-8x\right)+\frac{1}{5}u=8y+\frac{1}{5}u-8x}}</math> | ||
| − | |style="text-align:right;"|ויהיה ערך הבהמה מזה החלק שמנה דינרים | + | |style="text-align:right;"|ויהיה ערך הבהמה מזה החלק שמנה דינרים וחמישית דרהם פחות שמנה דברים |
|- | |- | ||
| | | | ||
:This is equal to the price of the beast in the first stage, which is a thing and half a dinar. | :This is equal to the price of the beast in the first stage, which is a thing and half a dinar. | ||
:<math>\scriptstyle{\color{blue}{x+\frac{1}{2}y=8y+\frac{1}{5}u-8x}}</math> | :<math>\scriptstyle{\color{blue}{x+\frac{1}{2}y=8y+\frac{1}{5}u-8x}}</math> | ||
| − | |style="text-align:right;"|ויהיה שוה לערך | + | |style="text-align:right;"|ויהיה שוה לערך הב<sup>ה</sup>מה מהחלק האחר שהוא דבר וחצי דינר |
| + | |- | ||
| + | | | ||
| + | :*<span style="color:Green>Confrontation:</span> | ||
| + | | | ||
|- | |- | ||
| | | | ||
| − | : | + | :Confront them and the dirham becomes equal to 45 things minus 37 dinar and a half, which is what the fifth has. |
:<math>\scriptstyle{\color{blue}{u=45x-37\frac{1}{2}y}}</math> | :<math>\scriptstyle{\color{blue}{u=45x-37\frac{1}{2}y}}</math> | ||
|style="text-align:right;"|ותכוין בהם ויהיה הדרהם ישוה מ"ה דברים פחות ל"ז דינרים וחצי והוא מה שיש לחמישי | |style="text-align:right;"|ותכוין בהם ויהיה הדרהם ישוה מ"ה דברים פחות ל"ז דינרים וחצי והוא מה שיש לחמישי | ||
| Line 3,883: | Line 4,246: | ||
| | | | ||
:He says to the first, who has a thing: if you give me a sixth of what you have, I will have the price of the beast. | :He says to the first, who has a thing: if you give me a sixth of what you have, I will have the price of the beast. | ||
| − | |style="text-align:right;"|ואמר לראשון אשר לו דבר אם תתן לי הששית מאשר | + | |style="text-align:right;"|ואמר לראשון אשר לו דבר אם תתן לי הששית מאשר לך יהיה לי ערך הבהמה |
|- | |- | ||
| | | | ||
| Line 3,892: | Line 4,255: | ||
:We add it to what he has; the price of the beast in this stage is 45 things and a sixth of a thing minus 37 dinar and a half. | :We add it to what he has; the price of the beast in this stage is 45 things and a sixth of a thing minus 37 dinar and a half. | ||
:<math>\scriptstyle{\color{blue}{\left(45x-37\frac{1}{2}y\right)+\frac{1}{6}x=45x+\frac{1}{6}x-37\frac{1}{2}y}}</math> | :<math>\scriptstyle{\color{blue}{\left(45x-37\frac{1}{2}y\right)+\frac{1}{6}x=45x+\frac{1}{6}x-37\frac{1}{2}y}}</math> | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונקבצהו עם מה שמחזיק ויהיה ערך הבהמה מזה החלק מ"ה דברים וששית דבר פחות ל"ז דינרים וחצי |
|- | |- | ||
| | | | ||
| Line 3,900: | Line 4,263: | ||
|- | |- | ||
| | | | ||
| − | :<span style="color:Green>Confrontation:</span> Confront them and it becomes after the confrontation 44 things and a sixth equals 38 dinar. | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Confront them and it becomes after the confrontation 44 things and a sixth equals 38 dinar. | ||
:<math>\scriptstyle{\color{blue}{44\frac{1}{6}x=38y}}</math> | :<math>\scriptstyle{\color{blue}{44\frac{1}{6}x=38y}}</math> | ||
|style="text-align:right;"|ותכוין בהם ויהיה אחר הכוון מ"ד דברים וששית דבר ישוה ל"ח דינרים | |style="text-align:right;"|ותכוין בהם ויהיה אחר הכוון מ"ד דברים וששית דבר ישוה ל"ח דינרים | ||
| Line 3,916: | Line 4,283: | ||
:The dinar is 265 and this is what the second has. | :The dinar is 265 and this is what the second has. | ||
:<math>\scriptstyle{\color{blue}{y=265}}</math> | :<math>\scriptstyle{\color{blue}{y=265}}</math> | ||
| − | |style="text-align:right;"|והדינר רס"ה והוא אשר | + | |style="text-align:right;"|והדינר רס"ה והוא אשר לשני |
|- | |- | ||
| | | | ||
| Line 3,925: | Line 4,292: | ||
| | | | ||
:Convert all into halves: | :Convert all into halves: | ||
| − | |style="text-align:right;"|והשיבם כלם חצאים | + | |style="text-align:right;"|והשיבם כלם רביעים <sup>נ' חצאים</sup> |
|- | |- | ||
| | | | ||
| Line 3,962: | Line 4,329: | ||
:The third said to the fourth: if you give me three dirham I will have four times as much of what remains with you. | :The third said to the fourth: if you give me three dirham I will have four times as much of what remains with you. | ||
:The fourth said to the first: if you give me four dirham I will have five times as much of what remains with you. | :The fourth said to the first: if you give me four dirham I will have five times as much of what remains with you. | ||
| − | |style="text-align:right;"|מג | + | |style="text-align:right;"|מג <big>ואם</big> יאמרו לך ארבעה אנשים התקבצו לקנות בהמה אחת<br> |
ואמר הראשון לשני אם תתן לי דרהם אחד יהיה לי הכפל ממה שנשאר לך<br> | ואמר הראשון לשני אם תתן לי דרהם אחד יהיה לי הכפל ממה שנשאר לך<br> | ||
ואמר השני לשלישי אם תתן לי שני דרהמי יהיה לי שלשה כפלים ממה שנשאר לך<br> | ואמר השני לשלישי אם תתן לי שני דרהמי יהיה לי שלשה כפלים ממה שנשאר לך<br> | ||
| Line 3,977: | Line 4,344: | ||
|- | |- | ||
| | | | ||
| − | :the first | + | :When he first takes one dirham from the second, the first has a thing and one dirham; this should be the same as double what is left for the second, which is two dinar minus two dirham. |
| − | |style="text-align:right;"|וכאשר לקח הראשון מהשני דרהם אחד יהיה לראשון דבר ודרהם אחד ויהיה כמו הכפל ממה שנשאר לשני והוא שני | + | :<math>\scriptstyle{\color{blue}{x+1=2\sdot\left(y-1\right)=2y-2}}</math> |
| + | |style="text-align:right;"|וכאשר לקח הראשון מהשני דרהם אחד יהיה לראשון דבר ודרהם אחד ויהיה כמו הכפל ממה שנשאר לשני והוא שני דינרים פחות שני דרהמי | ||
|- | |- | ||
| | | | ||
| − | :<span style="color:Green>Confrontation:</span> Confront them and the dinar becomes equal to half a thing plus a dinar and a half, which is what the second has. | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Confront them and the dinar becomes equal to half a thing plus a dinar and a half, which is what the second has. | ||
:<math>\scriptstyle{\color{blue}{y=\frac{1}{2}x+1\frac{1}{2}}}</math> | :<math>\scriptstyle{\color{blue}{y=\frac{1}{2}x+1\frac{1}{2}}}</math> | ||
|style="text-align:right;"|ותכוין בהם ויהיה הדינר ישוה חצי דבר ודרהם וחצי והוא אשר לשני | |style="text-align:right;"|ותכוין בהם ויהיה הדינר ישוה חצי דבר ודרהם וחצי והוא אשר לשני | ||
| Line 3,990: | Line 4,362: | ||
|- | |- | ||
| | | | ||
| − | :the second | + | :When the second takes two dirham from the third, the second has [half] a thing plus three dirham and a half; this should be equal to three times what is left for the third, which is three dinar minus six dirham. |
| − | |style="text-align:right;"|וכאשר לקח ממנו השני שני דרהמי יהיה לשני חצי דבר ושלשה דרהמי וחצי ויהיה שוה לשלשה | + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}x+1\frac{1}{2}+2=\frac{1}{2}x+3\frac{1}{2}=3\sdot\left(y-2\right)=3y-6}}</math> |
| + | |style="text-align:right;"|וכאשר לקח ממנו השני שני דרהמי יהיה לשני שלישית <sup>נ' חצי</sup> דבר ושלשה דרהמי וחצי ‫<ref>82v</ref>ויהיה שוה לשלשה דמיוני מה שנשאר לשלישי והוא שלשה דינרים <sup>פחות</sup> ששה דרהמי | ||
|- | |- | ||
| | | | ||
| − | :<span style="color:Green>Confrontation:</span> Confront them and the dinar becomes equal to a sixth of a thing plus three dirham and a sixth, which is what the third has. | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Confront them and the dinar becomes equal to a sixth of a thing plus three dirham and a sixth, which is what the third has. | ||
:<math>\scriptstyle{\color{blue}{y=\frac{1}{6}x+3\frac{1}{6}}}</math> | :<math>\scriptstyle{\color{blue}{y=\frac{1}{6}x+3\frac{1}{6}}}</math> | ||
|style="text-align:right;"|ותכוין בהם וישוה הדינר שתות דבר ושלשה דרהמי ושתות והוא אשר לשלישי | |style="text-align:right;"|ותכוין בהם וישוה הדינר שתות דבר ושלשה דרהמי ושתות והוא אשר לשלישי | ||
| Line 4,003: | Line 4,380: | ||
|- | |- | ||
| | | | ||
| − | :the third | + | :When the third takes two dirham from him, he has a sixth of a thing plus six dirham and a sixth; this should be equal to four times what is left for the fourth, which is four dinar minus twelve dirham. |
| − | |style="text-align:right;"|וכאשר לקח ממנו השלישי שלשה דרהמי יהיה לו שתות דבר וששה דרהמי ושתות וישוה ארבעה | + | :<math>\scriptstyle{\color{blue}{\frac{1}{6}x+3\frac{1}{6}+3=\frac{1}{6}x+6\frac{1}{6}=4\sdot\left(y-3\right)=4y-12}}</math> |
| + | |style="text-align:right;"|וכאשר לקח ממנו השלישי שלשה דרהמי יהיה לו שתות דבר וששה דרהמי ושתות וישוה ארבעה דמיונים ממה שנשאר לרביעי והוא ארבעה דינרים פחות שנים עשר דרהמי | ||
|- | |- | ||
| | | | ||
| − | :<span style="color:Green>Confrontation:</span> Confront them and the dinar becomes equal to a third of an eighth of a thing plus four dirham, a quarter, a sixth, and an eighth of a dirham, which is what the fourth has. | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Confront them and the dinar becomes equal to a third of an eighth of a thing plus four dirham, a quarter, a sixth, and an eighth of a dirham, which is what the fourth has. | ||
:<math>\scriptstyle{\color{blue}{y=\left(\frac{1}{3}\sdot\frac{1}{8}\right)x+4\frac{1}{4}+\frac{1}{6}+\frac{1}{8}}}</math> | :<math>\scriptstyle{\color{blue}{y=\left(\frac{1}{3}\sdot\frac{1}{8}\right)x+4\frac{1}{4}+\frac{1}{6}+\frac{1}{8}}}</math> | ||
| − | |style="text-align:right;"|ותכוין בהם ויהיה שוה הדינר שלישית השמינית מדבר | + | |style="text-align:right;"|ותכוין בהם ויהיה שוה הדינר שלישית השמינית מדבר וארבעה דרהמי ורביע ושתות ושמינית דרהם והוא אשר לרביעי |
|- | |- | ||
| | | | ||
| − | :the fourth | + | :When [the fourth] takes four dirham from the first, he has eight dirham, 13 parts of 24 of a dirham, and a third of an eighth of a thing; this should be equal to five times what is left for the first, which is five things minus twenty dirham. |
| − | |style="text-align:right;"|וכאשר לקח מהראשון ארבעה דרהמי יהיה לו שמנה דרהמי וי"ג חלקים מכ"ד חלקים בדרהם ושלישית השמינית מדבר וישוה לחמשה | + | |style="text-align:right;"|וכאשר לקח מהראשון ארבעה דרהמי יהיה לו שמנה דרהמי וי"ג חלקים מכ"ד חלקים בדרהם ושלישית השמינית מדבר וישוה לחמשה דמיונים מאשר נשאר לראשון והוא חמשה דברים פחות עשרים דרהמי |
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| Line 4,019: | Line 4,401: | ||
|- | |- | ||
| | | | ||
| − | : | + | :*<span style="color:Green>Confrontation:</span> |
| − | | | + | | |
|- | |- | ||
| | | | ||
| − | :the second has | + | :Confront them; the thing is equal to five dirham and ninety parts of 119 and this is what the first has. |
| + | :<math>\scriptstyle{\color{blue}{x=5+\frac{90}{119}}}</math> | ||
| + | |style="text-align:right;"|ותכוין בהם יהיה הדבר ישוה לחמשה דרהמי ותשעים חלקים מקי"ט חלקים מאחד והוא אשר לראשון | ||
| + | |- | ||
| + | | | ||
| + | :The second has half of what the first has plus one dirham and a half more; it is four dirham and 45 parts of 119. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}x+1\frac{1}{2}=4+\frac{45}{119}}}</math> | ||
|style="text-align:right;"|ולשני החצי ממה שיש לראשון ודרהם אחד וחצי יותר ויהיה ארבעה דרהמי ומ"ה חלקים מקי"ט | |style="text-align:right;"|ולשני החצי ממה שיש לראשון ודרהם אחד וחצי יותר ויהיה ארבעה דרהמי ומ"ה חלקים מקי"ט | ||
|- | |- | ||
| | | | ||
| − | :the | + | :The third has a sixth of what the first has plus three dirham and a sixth more; it is four dirham and 15 parts of 119 of a dirham. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{6}x+3\frac{1}{6}=4+\frac{15}{119}}}</math> | ||
|style="text-align:right;"|ולשלישי הששית ממה שיש לראשון ושלשה דרהמי ושתות יותר והוא ארבעה דרהמי וט"ו חלקים מקי"ט חלקים בדרהם | |style="text-align:right;"|ולשלישי הששית ממה שיש לראשון ושלשה דרהמי ושתות יותר והוא ארבעה דרהמי וט"ו חלקים מקי"ט חלקים בדרהם | ||
|- | |- | ||
| | | | ||
| − | :the | + | :The fourth has a third of an eighth of what the first has plus four dirham and a sixth and an eighth; it is four dirham and 93 parts of 119 of a dirham. |
| + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{3}\sdot\frac{1}{8}\right)x+4\frac{1}{4}+\frac{1}{6}+\frac{1}{8}=4+\frac{95}{119}}}</math> | ||
|style="text-align:right;"|ולרביעי שליש השמינית מאשר לראשון וארבעה דרהמי ושתות ושמינית והוא ארבעה דרהמי וצ"ג חלקים מקי"ט חלקים בדרהם | |style="text-align:right;"|ולרביעי שליש השמינית מאשר לראשון וארבעה דרהמי ושתות ושמינית והוא ארבעה דרהמי וצ"ג חלקים מקי"ט חלקים בדרהם | ||
|- | |- | ||
| Line 4,042: | Line 4,432: | ||
:The one who has the sixth returned a sixth of his share. | :The one who has the sixth returned a sixth of his share. | ||
:They shared all their earning and divided it equally between the three of them and each received his proper share. | :They shared all their earning and divided it equally between the three of them and each received his proper share. | ||
| − | |style="text-align:right;"|מד | + | |style="text-align:right;"|מד <big>ואם</big> יאמרו לך אלגו בין שלשה אנשים לאחד החצי ולשני השליש ולשלישי השתות והרויחו האלגו ביניהם<br> |
| − | והחזיר אשר לו החצי המחצית מחלקו<br> | + | והחזיר אשר לו החצי [המחצית] מחלקו<br> |
והחזיר אשר לו השליש השלישית מחלקו<br> | והחזיר אשר לו השליש השלישית מחלקו<br> | ||
והשיב בעל השתות הששית מחלקו<br> | והשיב בעל השתות הששית מחלקו<br> | ||
| − | ושתפו כל אשר הרויחו שלשתם וחלקוהו בין שלשתם בשוה ועלה לכל אחד מהם | + | ושתפו [אי בולבירון]‫<ref>Ma. marg.</ref> כל אשר הרויחו שלשתם וחלקוהו בין שלשתם בשוה ועלה לכל אחד מהם |
|- | |- | ||
| | | | ||
| − | :To know this, we suppose the | + | :To know this, we suppose the amount of money is a thing [<math>\scriptstyle{\color{blue}{x}}</math>], one dinar [<math>\scriptstyle{\color{blue}{y}}</math>] and one dirham [<math>\scriptstyle{\color{blue}{u}}</math>]. |
| − | |style="text-align:right;"|ולדעת זה נניח האלגו דבר ודינר אחד ודרהם אחד | + | |style="text-align:right;"|ולדעת זה נניח האלגו דבר ודינר אחד ודרהם אחד אי דיו נ"א אי גאנו נראה לי |
|- | |- | ||
| | | | ||
| Line 4,070: | Line 4,460: | ||
| | | | ||
:The owner of the third returned a third of a dinar [<math>\scriptstyle{\color{blue}{\frac{1}{3}y}}</math>]. | :The owner of the third returned a third of a dinar [<math>\scriptstyle{\color{blue}{\frac{1}{3}y}}</math>]. | ||
| − | |style="text-align:right;"|והשיב בעל השליש שלישית | + | |style="text-align:right;"|והשיב בעל השליש שלישית דינר |
|- | |- | ||
| | | | ||
| Line 4,081: | Line 4,471: | ||
|- | |- | ||
| | | | ||
| − | :each received a | + | :They divided it between them into thirds; each received a sixth of a thing, a ninth of a dinar and half a ninth of a dirham. |
| − | |style="text-align:right;"|וחלקו אותו ביניהם לשליש | + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot\left(\frac{1}{2}x+\frac{1}{3}y+\frac{1}{6}u\right)=\frac{1}{6}x+\frac{1}{9}y+\left(\frac{1}{2}\sdot\frac{1}{9}\right)u}}</math> |
| + | |style="text-align:right;"|וחלקו אותו ביניהם ‫<ref>83r</ref>לשליש והגיע לכל אחד מהם שתות דבר ותשיעית דינר וחצי תשיעית דרהם | ||
|- | |- | ||
| | | | ||
| + | :Each summed it with what he had left. | ||
|style="text-align:right;"|וקבצוהו כל אחד מהם עם מה שנשאר להם | |style="text-align:right;"|וקבצוהו כל אחד מהם עם מה שנשאר להם | ||
|- | |- | ||
| | | | ||
| − | :the | + | :The owner of the half has two-thirds of a thing, a ninth of a dinar and half a ninth of a dirham. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}x+\frac{1}{6}x+\frac{1}{9}y+\left(\frac{1}{2}\sdot\frac{1}{9}\right)u=\frac{2}{3}x+\frac{1}{9}y+\left(\frac{1}{2}\sdot\frac{1}{9}\right)u}}</math> | ||
|style="text-align:right;"|ויהיה לבעל החצי שני שלישי דבר ותשיעית דינר וחצי תשיעית דרהם | |style="text-align:right;"|ויהיה לבעל החצי שני שלישי דבר ותשיעית דינר וחצי תשיעית דרהם | ||
|- | |- | ||
| | | | ||
| − | : | + | :This is equal to half the amount of money, which is half a thing, half a dinar, and half a dirham. |
| + | :<math>\scriptstyle{\color{blue}{\frac{2}{3}x+\frac{1}{9}y+\left(\frac{1}{2}\sdot\frac{1}{9}\right)u=\frac{1}{2}x+\frac{1}{2}y+\frac{1}{2}u}}</math> | ||
|style="text-align:right;"|וישוה לחצי האלגו שהוא חצי דבר וחצי דינר וחצי דרהם | |style="text-align:right;"|וישוה לחצי האלגו שהוא חצי דבר וחצי דינר וחצי דרהם | ||
|- | |- | ||
| | | | ||
| − | :<span style="color:Green>Confrontation:</span> Confront them and the thing becomes equal to two dinar and a third plus two dirham and two-thirds. | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Confront them and the thing becomes equal to two dinar and a third plus two dirham and two-thirds. | ||
:<math>\scriptstyle{\color{blue}{x=2\frac{1}{3}y+2\frac{2}{3}u}}</math> | :<math>\scriptstyle{\color{blue}{x=2\frac{1}{3}y+2\frac{2}{3}u}}</math> | ||
| − | |style="text-align:right;"|ותכוין בהם ויהיה הדבר ישוה שני דינרים ושליש ושני דרהמי ושני | + | |style="text-align:right;"|ותכוין בהם ויהיה הדבר ישוה שני דינרים ושני שלישים <sup>נ' ושליש</sup> ושני דרהמי ושני שלישי<sup>ו</sup>ת |
|- | |- | ||
| | | | ||
| − | : | + | :The amount of money should be three dinar and a third plus three dirham and two-thirds. |
| + | :<math>\scriptstyle{\color{blue}{x+y+u=3\frac{1}{3}y+3\frac{2}{3}u}}</math> | ||
|style="text-align:right;"|וצריך שיהיה האלגו שלשה דינרים ושליש ושלשה דרהמי ושני שלישים | |style="text-align:right;"|וצריך שיהיה האלגו שלשה דינרים ושליש ושלשה דרהמי ושני שלישים | ||
|- | |- | ||
| | | | ||
| − | : | + | :Its half was returned, which is one dinar and a sixth plus one dirham and a third. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}x=\frac{1}{2}\sdot\left(2\frac{1}{3}y+2\frac{2}{3}u\right)=1\frac{1}{6}y+1\frac{1}{3}u}}</math> | ||
|style="text-align:right;"|ושב חציו והוא דינר ושתות ודרהם ושליש | |style="text-align:right;"|ושב חציו והוא דינר ושתות ודרהם ושליש | ||
|- | |- | ||
| | | | ||
| − | : | + | :The total of what they returned is one dinar and a half plus one dirham and a half. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}x+\frac{1}{3}y+\frac{1}{6}u=1\frac{1}{2}y+1\frac{1}{2}u}}</math> | ||
|style="text-align:right;"|וכל מה שהשיבו דינר אחד וחצי ודרהם וחצי | |style="text-align:right;"|וכל מה שהשיבו דינר אחד וחצי ודרהם וחצי | ||
|- | |- | ||
| | | | ||
| − | :each received a | + | :They divided it into thirds; each received half a dinar and half a dirham. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot\left(1\frac{1}{2}y+1\frac{1}{2}u\right)=\frac{1}{2}y+\frac{1}{2}u}}</math> | ||
|style="text-align:right;"|וחלקוהו לשליש לכל אחד חצי דינר וחצי דרהם | |style="text-align:right;"|וחלקוהו לשליש לכל אחד חצי דינר וחצי דרהם | ||
|- | |- | ||
| | | | ||
| − | : | + | :The owner of the dinar, who has a third of the amount of money, has one dinar and a ninth of a dinar plus one dirham and two-ninths of a dirham. |
| − | |||
|style="text-align:right;"|ויהיה לבעל הדינר אשר לו שלישית האלגו והוא דינר ותשיעית דינר ודרהם ושני תשיעיות דרהם | |style="text-align:right;"|ויהיה לבעל הדינר אשר לו שלישית האלגו והוא דינר ותשיעית דינר ודרהם ושני תשיעיות דרהם | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\frac{2}{3}y+\frac{1}{2}y+\frac{1}{2}u=1\frac{1}{6}y+\frac{1}{2}u=}}{\color{blue}{\frac{1}{3}\sdot\left(3\frac{1}{3}y+3\frac{2}{3}u\right)=1\frac{1}{9}y+1\frac{2}{9}u}}</math> | ||
|- | |- | ||
| | | | ||
| − | :<span style="color:Green>Confrontation:</span> Confront them and the dinar becomes equal to | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Confront them and the dinar becomes equal to thirteen dirham, which is what the owner of the third earns. | ||
:<math>\scriptstyle{\color{blue}{y=13u}}</math> | :<math>\scriptstyle{\color{blue}{y=13u}}</math> | ||
| − | |style="text-align:right;"|ותכוין בהם ויהיה הדינר ישוה | + | |style="text-align:right;"|ותכוין בהם ויהיה הדינר ישוה שלשה עשר דרהמיש והוא מה שהרויח בעל השלישית |
|- | |- | ||
| | | | ||
| + | :The thing is two dinar and a third plus two dirham and two-thirds, which is thirty-three and this is what the owner of the half earns. | ||
:<math>\scriptstyle{\color{blue}{x=2\frac{1}{3}y+2\frac{2}{3}u=33u}}</math> | :<math>\scriptstyle{\color{blue}{x=2\frac{1}{3}y+2\frac{2}{3}u=33u}}</math> | ||
|style="text-align:right;"|והדבר שני דינרים ושליש ושני דרהמיש ושני שלישים והוא שלשים ושלשה והוא מה שהרויח בעל החצי | |style="text-align:right;"|והדבר שני דינרים ושליש ושני דרהמיש ושני שלישים והוא שלשים ושלשה והוא מה שהרויח בעל החצי | ||
|- | |- | ||
| | | | ||
| + | :The owner of the sixth earns one dirham, which is one. | ||
:<math>\scriptstyle{\color{blue}{u_1=1}}</math> | :<math>\scriptstyle{\color{blue}{u_1=1}}</math> | ||
|style="text-align:right;"|והרויח בעל השתות דרהם אחד והוא אחד | |style="text-align:right;"|והרויח בעל השתות דרהם אחד והוא אחד | ||
|- | |- | ||
| | | | ||
| − | : | + | :When you examine it without any hassle, you should multiply it six times: the owner of the half earns 198, the owner of the third - 78, the owner of the sixth - six, and the whole amount of money is 282. |
| − | |style="text-align:right;"|וצריך מבלי טורח כאשר תנסהו שתכהו בששה פעמים ויהיה מה שהרויח בעל החצי קצ"ח ובעל השלישית ע"ח ובעל השתות ששה והאלגו | + | |style="text-align:right;"|וצריך מבלי טורח כאשר תנסהו שתכהו בששה פעמים ויהיה מה שהרויח בעל החצי קצ"ח ובעל השלישית ע"ח ובעל השתות ששה והאלגו כלו רפ"ב |
|- | |- | ||
| − | | | + | | colspan="2"| |
| − | |style="text-align:right;"|וכמעשה הזה יעשוהו בעלי החשבון ביניהם והיא הבנה | + | :<math>\scriptstyle{\color{blue}{u=6\quad y=6\sdot13=78\quad x=6\sdot33=198\quad x+y+u=282}}</math> |
| + | |- | ||
| + | |This procedure is used by the arithmeticians and it is a complete understanding, but it is more correct for a large numbers, if many people are given [?]. | ||
| + | |style="text-align:right;"|וכמעשה הזה יעשוהו בעלי החשבון ביניהם והיא הבנה שלמה אלא שתתדקדק ביותר על מי שיעשהו במספר רב אם יניחו האנשים הרבה | ||
| + | |- | ||
| + | |I solve this with a direct procedure and full understanding: | ||
| + | |style="text-align:right;"|ואוציאהו לחפשי אני עם מלאכה ישרה [והבנה] מובנת | ||
|- | |- | ||
| | | | ||
| − | :the amount of money | + | :We suppose the amount of money is a thing [<math>\scriptstyle{\color{blue}{x}}</math>]. |
|style="text-align:right;"|והוא שנניח האלגו דבר | |style="text-align:right;"|והוא שנניח האלגו דבר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ואשר הרויח כל אחד מהם מן אלגו ואחר מה שהשיבו כפי הנחתנו מדרהמי שליש וחלקוהו | + | :Each of them earned [a share] from the amount of money; then, according to our assumption, they returned [some] of the dirham they had and divided [the returned amount] into a third. Each received [a third] and summed it with what he had left. |
| + | |style="text-align:right;"|ואשר הרויח כל אחד מהם מן אלגו ואחר מה שהשיבו כפי הנחתנו מדרהמי שליש וחלקוהו לשליש ועלה לכל אחד מהם וקבצהו עם מה שנשאר לו ויהיה לכל אחד | ||
|- | |- | ||
| | | | ||
| − | :the owner of the half returned half of | + | :You can easily know that what is left for the owner of the half, after he returned half of what he earned, is half a thing minus one dirham. |
| − | |style="text-align:right;"|ובנקלה תדע שאשר נשאר לבעל | + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}x-y}}</math> |
| + | |style="text-align:right;"|ובנקלה תדע שאשר נשאר לבעל <sup>ה</sup>חצי אחר שהשיב החצי ממה שהרויח חצי דבר פחות דרהם | ||
|- | |- | ||
| | | | ||
| − | : | + | :What he returned is half a thing minus one dirham. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}x-y}}</math> | ||
|style="text-align:right;"|ומה ששב הוא חצי דבר פחות דרהם אחד | |style="text-align:right;"|ומה ששב הוא חצי דבר פחות דרהם אחד | ||
|- | |- | ||
| | | | ||
| − | : | + | :The [owner of the] third returned a sixth of a thing minus [half] a dirham. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{6}x-{\color{red}{\frac{1}{2}}}y}}</math> | ||
|style="text-align:right;"|ולשלישי שב שתות דבר פחות דרהם אחד | |style="text-align:right;"|ולשלישי שב שתות דבר פחות דרהם אחד | ||
|- | |- | ||
| | | | ||
| − | : | + | :[The owner of the sixth] returned one part of thirty of a thing [minus a fifth of a] dirham. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{30}x-\frac{1}{5}y}}</math> | ||
|style="text-align:right;"|ומה ששב אחד משלשים בדבר חמשה דרהמי | |style="text-align:right;"|ומה ששב אחד משלשים בדבר חמשה דרהמי | ||
|- | |- | ||
| | | | ||
| − | :they returned a | + | :The total amount they returned is half a thing and a fifth of a thing minus one dirham and 7-tenths of a dirham. |
|style="text-align:right;"|ויהיה כל אשר השיבו חצי דבר וחמישית דבר פחות דרהם אחד וז' עשיריות מדרהם | |style="text-align:right;"|ויהיה כל אשר השיבו חצי דבר וחמישית דבר פחות דרהם אחד וז' עשיריות מדרהם | ||
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| − | <math>\scriptstyle{\color{blue}{\left(\frac{1}{2}x-y\right)+\left(\frac{1}{6}x-{\color{red}{\frac{1}{2}}}y\right)+\left(\frac{1}{30}x-\frac{1}{5}y\right)=\frac{1}{2}x+\frac{1}{5}x-y-\frac{7}{10}y=3y}}</math> | + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}x-y\right)+\left(\frac{1}{6}x-{\color{red}{\frac{1}{2}}}y\right)+\left(\frac{1}{30}x-\frac{1}{5}y\right)=\frac{1}{2}x+\frac{1}{5}x-y-\frac{7}{10}y=3y}}</math> |
|- | |- | ||
| | | | ||
| + | :The whole amount of money is 5-sevenths of a dirham. | ||
:<math>\scriptstyle{\color{blue}{x=6\frac{5}{7}y}}</math> | :<math>\scriptstyle{\color{blue}{x=6\frac{5}{7}y}}</math> | ||
|style="text-align:right;"|וה' שביעיות מדרהם והוא כל האלגו | |style="text-align:right;"|וה' שביעיות מדרהם והוא כל האלגו | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you want to know how much the owner of the half earned: it is a thing minus two dirham, which is four dirham and 5-sevenths of a dirham. |
:<math>\scriptstyle{\color{blue}{x-2y=4\frac{5}{7}y}}</math> | :<math>\scriptstyle{\color{blue}{x-2y=4\frac{5}{7}y}}</math> | ||
| − | |style="text-align:right;"|וכאשר תרצה לדעת כמה הרויח בעל החצי דבר פחות שני דרהמי והוא ארבעה דרהמי וה' | + | |style="text-align:right;"|וכאשר תרצה לדעת כמה הרויח בעל ‫<ref>83v</ref>החצי דבר פחות שני דרהמי והוא ארבעה דרהמי וה' שביעיו' מדרהם |
|- | |- | ||
| | | | ||
| Line 4,188: | Line 4,610: | ||
:The owner of the sixth earned a fifth of a thing minus one dirham and a fifth, which is a seventh of a dirham. | :The owner of the sixth earned a fifth of a thing minus one dirham and a fifth, which is a seventh of a dirham. | ||
:<math>\scriptstyle{\color{blue}{\frac{1}{5}x-1\frac{1}{5}y=\frac{1}{7}y}}</math> | :<math>\scriptstyle{\color{blue}{\frac{1}{5}x-1\frac{1}{5}y=\frac{1}{7}y}}</math> | ||
| − | |style="text-align:right;"|ומה שהרויח בעל השתות חמישית דבר פחות דרהם אחד וחומש והוא | + | |style="text-align:right;"|ומה שהרויח בעל השתות חמישית דבר פחות דרהם אחד וחומש והוא שביעי' דרהם |
|- | |- | ||
| | | | ||
| Line 4,216: | Line 4,638: | ||
|- | |- | ||
| | | | ||
| + | |||
=== <span style="color:Green>Garments</span> === | === <span style="color:Green>Garments</span> === | ||
| Line 4,221: | Line 4,644: | ||
|- | |- | ||
| | | | ||
| − | :45) If you are told: there are ten garments, the price of the first garment is one dirham and the price of each of the others exceeds one by the other by one dirham - the second exceeds the first by one dirham, the third exceeds the second by one dirham and so on for all the others, how much is the total price? | + | :{{#annot:10 garments|652|Gn4O}}45) If you are told: there are ten garments, the price of the first garment is one dirham and the price of each of the others exceeds one by the other by one dirham - the second exceeds the first by one dirham, the third exceeds the second by one dirham and so on for all the others, how much is the total price? |
| − | |style="text-align:right;"|מה | + | |style="text-align:right;"|מה <big>ואם</big> יאמרו לך עשרה בגדים ערך הבגד הראשון דרהם אחד וערך כל אחד מן האחרים יוסיף האחד על האחר דרהם אחד השני יעדיף על הראשון דרהם אחד והשלישי יעדיף על השני דרהם אחד וכן כל האחרים כמה יהיה ערך כלם{{#annotend:Gn4O}} |
|- | |- | ||
| | | | ||
| + | :You already know that the price of the first cloth is one dirham and of the tenth is ten dirham. | ||
:<math>\scriptstyle{\color{blue}{a_1+a_{10}=1+10}}</math> | :<math>\scriptstyle{\color{blue}{a_1+a_{10}=1+10}}</math> | ||
| − | |style="text-align:right;"|וכבר תדע כי ערך הבגד הראשון דרהם אחד והעשירי עשרה דרהמי | + | |style="text-align:right;"|וכבר <s>ידעת</s> <sup>תדע</sup> כי ערך הבגד הראשון דרהם אחד והעשירי עשרה דרהמי |
|- | |- | ||
| | | | ||
| + | :The price of the second is two and of the ninth is nine. | ||
:<math>\scriptstyle{\color{blue}{a_2+a_9=2+9}}</math> | :<math>\scriptstyle{\color{blue}{a_2+a_9=2+9}}</math> | ||
|style="text-align:right;"|וערך השני שנים והתשיעי תשעה | |style="text-align:right;"|וערך השני שנים והתשיעי תשעה | ||
|- | |- | ||
| | | | ||
| + | :The price of the third is three and of the eighth is eight. | ||
:<math>\scriptstyle{\color{blue}{a_3+a_8=3+8}}</math> | :<math>\scriptstyle{\color{blue}{a_3+a_8=3+8}}</math> | ||
| − | |style="text-align:right;"|וערך | + | |style="text-align:right;"|וערך השלישי שלשה והשמיני שמנה |
| + | |- | ||
| + | | | ||
| + | :So, the price of each of these pairs is eleven. | ||
| + | |style="text-align:right;"|והנה הערך [מכל אחד]‫<ref>Ma. marg.</ref> מאלו הזוגות אחד עשר | ||
|- | |- | ||
| | | | ||
| − | :the price of all | + | :Likewise, the price of all the pairs will be the same, and they are five pairs. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכמו כן יהיה ערך כל הזוגות כאלו והם <s>[.]</s> חמשה זוגות |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Multiply eleven by five; it is fifty-five and so is the price of the ten garments. |
| + | |style="text-align:right;"|ותכה אחד עשר בחמשה ויהיה חמשים לחמשה וככה ערך עשרת הבגדים | ||
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| Line 4,247: | Line 4,678: | ||
|- | |- | ||
| | | | ||
| − | :46) If you are told: the price of the first garment is one and the price of each of the rest exceeds the former by 2, how much is the total price? | + | :{{#annot:10 garments|652|Uboq}}46) If you are told: the price of the first garment is one and the price of each of the rest exceeds the former by 2, how much is the total price? |
| − | |style="text-align:right;"|מו | + | |style="text-align:right;"|מו <big>ואם</big> יאמרו לך ערך <sup>הבגד</sup> הראשון אחד ויוסיף <s>ערכם מהנשארים כל אחד</s> <sup>ערך כל אחד מהנשארים</sup> שנים על ערך חברו כמה יהיה ערך כלם{{#annotend:Uboq}} |
|- | |- | ||
| | | | ||
| + | :Let the price of the tenth cloth be 19. | ||
:<math>\scriptstyle{\color{blue}{a_{10}=19}}</math> | :<math>\scriptstyle{\color{blue}{a_{10}=19}}</math> | ||
|style="text-align:right;"|הנה יהיה ערך הבגד העשירי י"ט | |style="text-align:right;"|הנה יהיה ערך הבגד העשירי י"ט | ||
|- | |- | ||
| | | | ||
| + | :Since the second exceeds over the first by two; | ||
:<math>\scriptstyle{\color{blue}{a_2=a_1+2}}</math> | :<math>\scriptstyle{\color{blue}{a_2=a_1+2}}</math> | ||
|style="text-align:right;"|בעבור כי הוסיף השני על הראשון שנים | |style="text-align:right;"|בעבור כי הוסיף השני על הראשון שנים | ||
|- | |- | ||
| | | | ||
| + | :The third over the second by two; | ||
:<math>\scriptstyle{\color{blue}{a_3=a_2+2}}</math> | :<math>\scriptstyle{\color{blue}{a_3=a_2+2}}</math> | ||
|style="text-align:right;"|והשלישי על השני שנים | |style="text-align:right;"|והשלישי על השני שנים | ||
|- | |- | ||
| | | | ||
| + | :So, the third exceeds over the first by four. | ||
:<math>\scriptstyle{\color{blue}{a_3=a_1+4}}</math> | :<math>\scriptstyle{\color{blue}{a_3=a_1+4}}</math> | ||
|style="text-align:right;"|ויוסיף השלישי על הראשון ארבעה | |style="text-align:right;"|ויוסיף השלישי על הראשון ארבעה | ||
|- | |- | ||
| | | | ||
| + | :The fourth [exceeds] over the first by six. | ||
:<math>\scriptstyle{\color{blue}{a_4=a_1+6}}</math> | :<math>\scriptstyle{\color{blue}{a_4=a_1+6}}</math> | ||
|style="text-align:right;"|והרביעי על הראשון ששה | |style="text-align:right;"|והרביעי על הראשון ששה | ||
|- | |- | ||
| | | | ||
| + | :The tenth exceeds over the first by 18. | ||
:<math>\scriptstyle{\color{blue}{a_{10}=a_1+18}}</math> | :<math>\scriptstyle{\color{blue}{a_{10}=a_1+18}}</math> | ||
|style="text-align:right;"|והעשירי יוסיף על הראשון י"ח | |style="text-align:right;"|והעשירי יוסיף על הראשון י"ח | ||
|- | |- | ||
| | | | ||
| + | :Accordingly, the price of the first with the tenth is twenty. | ||
:<math>\scriptstyle{\color{blue}{a_1+a_{10}=20}}</math> | :<math>\scriptstyle{\color{blue}{a_1+a_{10}=20}}</math> | ||
|style="text-align:right;"|וכפי זה יהיה ערך הראשון עם העשירי מקובצים עשרים | |style="text-align:right;"|וכפי זה יהיה ערך הראשון עם העשירי מקובצים עשרים | ||
|- | |- | ||
| | | | ||
| + | :Multiply twenty by five; it is one hundred and this is the price of the ten garments. | ||
:<math>\scriptstyle{\color{blue}{\sum_{i=1}^{10} \left(2i-1\right)=20\sdot5=100}}</math> | :<math>\scriptstyle{\color{blue}{\sum_{i=1}^{10} \left(2i-1\right)=20\sdot5=100}}</math> | ||
| − | |style="text-align:right;"|ותכה עשרים בחמשה ויהיה מאה וככה | + | |style="text-align:right;"|ותכה עשרים בחמשה ויהיה מאה וככה ערך עשרת הבגדים |
|- | |- | ||
| | | | ||
| − | :47) If you are told: there are ten garments, the value of the first garment is three dirham and the price of each of the others exceeds one by the other by four, how much is the total price? | + | :{{#annot:10 garments|652|3M4y}}47) If you are told: there are ten garments, the value of the first garment is three dirham and the price of each of the others exceeds one by the other by four, how much is the total price? |
| − | |style="text-align:right;"|מז | + | |style="text-align:right;"|מז <big>ואם</big> יאמרו לך עשרה בגדים ערך הראשון שלשה דרהמים ויוסיף ערך האחרים האחד על האחר ארבעה ארבעה כמה יהיה ערך כלם{{#annotend:3M4y}} |
|- | |- | ||
| | | | ||
| + | :Its procedure, as I explained, is that you multiply the excess of one over the other, which is four, by the number of the garments minus one; it is thirty-six. Add three to it, which is the price of the first cloth; the sum is thirty-nine. | ||
| + | |style="text-align:right;"|והמעשה בזה כאשר בארתי הוא שתכה יתרון האחד על האחר והוא ארבעה במספר הבגדים פחות אחד ויהיה שלשים ושש ותוסיף עליהם שלשה שהוא ערך הבגד הראשון ויתקבץ שלשים ותשע | ||
| + | |- | ||
| + | | colspan="2"| | ||
:<math>\scriptstyle{\color{blue}{a_{10}=\left[d\sdot\left(n-1\right)+a_1\right]=\left[4\sdot\left(10-1\right)\right]+a_1=36+3=39}}</math> | :<math>\scriptstyle{\color{blue}{a_{10}=\left[d\sdot\left(n-1\right)+a_1\right]=\left[4\sdot\left(10-1\right)\right]+a_1=36+3=39}}</math> | ||
| − | |||
|- | |- | ||
| | | | ||
| + | :The price of the first plus the tenth is forty-two; similarly, the price of the ninth plus the second is forty-two; and so is the price of every pair of garments of them. Multiply 42 by five; it is two hundred and ten and this is the total price of the garments. | ||
|style="text-align:right;"|ויהיה ערך הראשון והעשירי ארבעים ‫<ref>84v</ref>ושנים וכן ערך התשיעי והשני יהיה ארבעים ושנים ובדרך הזה יהיה ערך כל שני בגדים מהם ותכה מ"ב בחמשה ויהיה מאתים ועשר וככה ערך כל הבגדים | |style="text-align:right;"|ויהיה ערך הראשון והעשירי ארבעים ‫<ref>84v</ref>ושנים וכן ערך התשיעי והשני יהיה ארבעים ושנים ובדרך הזה יהיה ערך כל שני בגדים מהם ותכה מ"ב בחמשה ויהיה מאתים ועשר וככה ערך כל הבגדים | ||
|- | |- | ||
| Line 4,303: | Line 4,746: | ||
|- | |- | ||
| | | | ||
| − | :48) If you are told: a group of people earned, the first earned one dirham, each of them earned one dirham more than the former, and they earned a total of 300. How many people were in the group? | + | :{{#annot:unknown number of partners|661|OYfN}}48) If you are told: a group of people earned, the first earned one dirham, each of them earned one dirham more than the former, and they earned a total of 300. How many people were in the group? |
| − | |style="text-align:right;"|מח | + | |style="text-align:right;"|מח <big>ואם</big> יאמרו לך חבורה מאנשים עלו להרויח והרויח הראשון דרהם אחד והרויח כל אחד מהם יותר מאשר לפניו דרהם אחד והיה כל אשר הרויחו שלש מאות כמה היו האנשים מהחבורה{{#annotend:OYfN}} |
|- | |- | ||
| | | | ||
| Line 4,321: | Line 4,764: | ||
|- | |- | ||
| | | | ||
| − | :<span style="color:Green>Confrontation:</span> Confront them; the thing becomes twenty-four and this is the number of people in the group. | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Confront them; the thing becomes twenty-four and this is the number of people in the group. | ||
:<math>\scriptstyle{\color{blue}{x=24}}</math> | :<math>\scriptstyle{\color{blue}{x=24}}</math> | ||
|style="text-align:right;"|ותכוין עמהם ויהיה הדבר עשרים וארבעה וככה מספר אנשי החבורה | |style="text-align:right;"|ותכוין עמהם ויהיה הדבר עשרים וארבעה וככה מספר אנשי החבורה | ||
|- | |- | ||
| | | | ||
| − | :49) If you are told: a group of people, the first earned two dirham, each of them exceed his former by three, and they earned a total of 126. How many people were in the group? | + | :{{#annot:unknown number of partners|661|Np0Q}}49) If you are told: a group of people, the first earned two dirham, each of them exceed his former by three, and they earned a total of 126. How many people were in the group? |
| − | |style="text-align:right;"|מט | + | |style="text-align:right;"|מט <big>ואם</big> יאמרו לך חבורה מאנשים והראשון הרויח שני דרהמי והוסיף כל אחד על אשר לפניו שלשה והיה כל מה שהרויחו מאה ועשרים ושש כמה יהיה מספר החבורה{{#annotend:Np0Q}} |
|- | |- | ||
| | | | ||
| + | :To know this, do as I explained to you regarding the garments: multiply the excess of each by the number of the [people in the] group minus one; it is three things minus three dirham. Add to it what the first earned, which is two; it is three things minus one dirham. | ||
|style="text-align:right;"|ולדעת זה תניח כמו שבארתי לך בבגדים שתכה מה שיותיר כל אחד במספר החבורה פחות אחד ויהיה שלשה דברים פחות שלשה דרהמי ותוסיף עליו מה שהרויח הראשון והוא שנים ויהיה שלשה דברים פחות דרהם אחד | |style="text-align:right;"|ולדעת זה תניח כמו שבארתי לך בבגדים שתכה מה שיותיר כל אחד במספר החבורה פחות אחד ויהיה שלשה דברים פחות שלשה דרהמי ותוסיף עליו מה שהרויח הראשון והוא שנים ויהיה שלשה דברים פחות דרהם אחד | ||
|- | |- | ||
| Line 4,336: | Line 4,784: | ||
|- | |- | ||
| | | | ||
| + | :The first and the last earned three things and one dirham and that is what each pair of them earned by the said order. | ||
:<math>\scriptstyle{\color{blue}{a_1+a_x=x_2+a_{x-1}=\ldots=3x+1}}</math> | :<math>\scriptstyle{\color{blue}{a_1+a_x=x_2+a_{x-1}=\ldots=3x+1}}</math> | ||
|style="text-align:right;"|ויהיה מה שהרויח הראשון והאחרון שלשה דברים ודרהם אחד והוא מה שהרויח כל <s>אחד</s> <sup>שנים</sup> מהם כפי הסדר האמור | |style="text-align:right;"|ויהיה מה שהרויח הראשון והאחרון שלשה דברים ודרהם אחד והוא מה שהרויח כל <s>אחד</s> <sup>שנים</sup> מהם כפי הסדר האמור | ||
|- | |- | ||
| | | | ||
| + | :Multiply this by the number of the [people in the] group; it is a square and a half plus half a thing and this is what the whole group earned, which is equal to 126 dirham. | ||
:<math>\scriptstyle{\color{blue}{\left(3x+1\right)\sdot{\color{red}{\frac{1}{2}}}x=1\frac{1}{2}x^2+\frac{1}{2}x=126}}</math> | :<math>\scriptstyle{\color{blue}{\left(3x+1\right)\sdot{\color{red}{\frac{1}{2}}}x=1\frac{1}{2}x^2+\frac{1}{2}x=126}}</math> | ||
|style="text-align:right;"|ותכה זה במספר החבורה ויהיה אלגו וחצי וחצי דבר והוא כל מה שהרויחה החבורה והוא ישוה קכ"ו דרהמי | |style="text-align:right;"|ותכה זה במספר החבורה ויהיה אלגו וחצי וחצי דבר והוא כל מה שהרויחה החבורה והוא ישוה קכ"ו דרהמי | ||
|- | |- | ||
| | | | ||
| − | :<span style="color:Green>Confrontation:</span> Confront them; the thing becomes nine and this in the number of [members in] the group. | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Confront them; the thing becomes nine and this in the number of [members in] the group. | ||
:<math>\scriptstyle{\color{blue}{x=9}}</math> | :<math>\scriptstyle{\color{blue}{x=9}}</math> | ||
|style="text-align:right;"|ותכוין עמהם ויהיה הדבר תשעה והוא מספר החבורה | |style="text-align:right;"|ותכוין עמהם ויהיה הדבר תשעה והוא מספר החבורה | ||
|- | |- | ||
| | | | ||
| − | :50) If you are told: a group of people, the first earned one dirham, each of them exceeded his former by one dirham. They summed the total of what they earned together then divided it equally and each got 10 dirham. | + | :{{#annot:unknown number of partners|661|JrhQ}}50) If you are told: a group of people, the first earned one dirham, each of them exceeded his former by one dirham. They summed the total of what they earned together then divided it equally and each got 10 dirham. |
| − | |style="text-align:right;"|נ | + | |style="text-align:right;"|נ <big>ואם</big> יאמרו לך חבורה מאנשים שהרויח הראשון דרהם אחד והוסיף כל אחד על אשר לפניו אחד דרהם וקבצו כל אשר הרויחו יחד וחלקוהו בשוה ועלה לחלק לכל אחד מהם עשרה דרהמי{{#annotend:JrhQ}} |
|- | |- | ||
| | | | ||
| − | : | + | :We already explained that all they earned is half a square plus half a thing. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}x^2+\frac{1}{2}x}}</math> | ||
|style="text-align:right;"|וכבר בארנו שכל אשר הרויחו הוא חצי אלגו וחצי דבר | |style="text-align:right;"|וכבר בארנו שכל אשר הרויחו הוא חצי אלגו וחצי דבר | ||
|- | |- | ||
| | | | ||
| + | :We divide it by the number of [members in] the group, which is a thing; the quotient is ten dirham. | ||
:<math>\scriptstyle{\color{blue}{\frac{\frac{1}{2}x^2+\frac{1}{2}x}{x}=10}}</math> | :<math>\scriptstyle{\color{blue}{\frac{\frac{1}{2}x^2+\frac{1}{2}x}{x}=10}}</math> | ||
|style="text-align:right;"|וחלקנוהו על מספר החבורה והוא דבר ויגיע לחלק עשרה דרהמי | |style="text-align:right;"|וחלקנוהו על מספר החבורה והוא דבר ויגיע לחלק עשרה דרהמי | ||
|- | |- | ||
| | | | ||
| − | :51) If you are told: a group, two thirds of which, the first earned one dirham, and each of them exceeded his former by two dirham. When they summed the total of what they earned together and divided it equally between the whole group each got 4 dirham. How many were in the group? | + | :{{#annot:unknown number of partners|661|VFiQ}}51) If you are told: a group, two thirds of which, the first earned one dirham, and each of them exceeded his former by two dirham. When they summed the total of what they earned together and divided it equally between the whole group each got 4 dirham. How many were in the group? |
| − | |style="text-align:right;"|‫<ref>84v</ref>נא | + | |style="text-align:right;"|‫<ref>84v</ref>נא <big>ואם</big> יאמרו לך חבורה אשר שני שלישיתיה הרויח הראשון דרהם אחד והוסיפו <s>בשלשה</s> בשנים דרהמי וכאשר שבו קבצו כל אשר הרויחו וחלקוהו בין כל החבורה בשוה והגיע לכל אחד מהם ארבעה דרהמי כמה יהיה מספר החבורה{{#annotend:VFiQ}} |
|- | |- | ||
| | | | ||
| − | :To know this, we suppose | + | :To know this, we suppose the number of [members in] the group is a thing [<math>\scriptstyle{\color{blue}{x}}</math>]. |
|style="text-align:right;"|ולדעת זה נשים מספר החבורה דבר | |style="text-align:right;"|ולדעת זה נשים מספר החבורה דבר | ||
|- | |- | ||
| | | | ||
| + | :Two thirds of them - the first earned one dirham, and [each of them exceeded his former] by two dirham. | ||
|style="text-align:right;"|ושני שלישם הרויח הראשון דרהם אחד והוסיפו בשני דרהם | |style="text-align:right;"|ושני שלישם הרויח הראשון דרהם אחד והוסיפו בשני דרהם | ||
|- | |- | ||
| | | | ||
| − | :the total | + | :According to what we explained, the total of what they earned should be four-ninths of a square. |
| + | :<math>\scriptstyle{\color{blue}{\frac{4}{9}x^2}}</math> | ||
|style="text-align:right;"|וצריך כפי מה שבארנו שיהיה כל אשר הרויחו ארבעה תשיעיות מאלגו | |style="text-align:right;"|וצריך כפי מה שבארנו שיהיה כל אשר הרויחו ארבעה תשיעיות מאלגו | ||
|- | |- | ||
| | | | ||
| + | :We divide it by the number of [members in] the group, which is a thing; the result [should be equal to] four dirham. | ||
:<math>\scriptstyle{\color{blue}{\frac{\frac{4}{9}x^2}{x}=4}}</math> | :<math>\scriptstyle{\color{blue}{\frac{\frac{4}{9}x^2}{x}=4}}</math> | ||
|style="text-align:right;"|ואנו נחלקם על מספר החבורה שהוא דבר ויצא ארבעה דרהמי | |style="text-align:right;"|ואנו נחלקם על מספר החבורה שהוא דבר ויצא ארבעה דרהמי | ||
|- | |- | ||
| | | | ||
| + | :Multiply four dirham by a thing; it is four dirham that are equal to four-ninths of a square. | ||
:<math>\scriptstyle{\color{blue}{\frac{4}{9}x^2=4x}}</math> | :<math>\scriptstyle{\color{blue}{\frac{4}{9}x^2=4x}}</math> | ||
|style="text-align:right;"|ותכה ארבעה דרהמי בדבר ויהיה ארבעה דברים ישוו ארבעה תשיעיות מאלגו | |style="text-align:right;"|ותכה ארבעה דרהמי בדבר ויהיה ארבעה דברים ישוו ארבעה תשיעיות מאלגו | ||
|- | |- | ||
| | | | ||
| + | :The square is equal to nine things. | ||
:<math>\scriptstyle{\color{blue}{x^2=9x}}</math> | :<math>\scriptstyle{\color{blue}{x^2=9x}}</math> | ||
|style="text-align:right;"|והאלגו ישוה <s>ארבעה</s> <sup>תשעה</sup> דברים | |style="text-align:right;"|והאלגו ישוה <s>ארבעה</s> <sup>תשעה</sup> דברים | ||
|- | |- | ||
| | | | ||
| + | :The thing is equal to nine and this is the number of [members in] the group. | ||
:<math>\scriptstyle{\color{blue}{x=9}}</math> | :<math>\scriptstyle{\color{blue}{x=9}}</math> | ||
|style="text-align:right;"|והדבר ישוה תשעה והם מספר החבורה | |style="text-align:right;"|והדבר ישוה תשעה והם מספר החבורה | ||
|- | |- | ||
| | | | ||
| − | :52) If you are told: a group, its three quarters earned, the first earned two dirham, and the others exceeded by three | + | :{{#annot:unknown number of partners|661|acDH}}52) If you are told: a group, its three quarters earned, the first earned two dirham, and the others exceeded by three [dirham]. When they divided the total of what they earned equally between the whole group each got 24 dirham. How many were in the group? |
| − | |style="text-align:right;"|נב | + | |style="text-align:right;"|נב <big>ואם</big> יאמרו לך חבורה ששלשת רביעיה עלו להרויח והרויח הראשון שני דרהמי והוסיפו האחרים בשלשה רביעים וכאשר שבו חלקום כל אשר הרויחו בין כל החבורה בשוה והגיע לכל אחד מהם עשרים וארבעה דרהמי כמה יהיה מספר החבורה{{#annotend:acDH}} |
|- | |- | ||
| | | | ||
| Line 4,400: | Line 4,862: | ||
|- | |- | ||
| | | | ||
| − | : | + | :Three-quarters of [the group] earned - they are three-quarters of a thing. |
| + | :<math>\scriptstyle{\color{blue}{\frac{3}{4}x}}</math> | ||
|style="text-align:right;"|ועלו שלשת רביעיה להרויח והם שלשה רביעי דבר | |style="text-align:right;"|ועלו שלשת רביעיה להרויח והם שלשה רביעי דבר | ||
|- | |- | ||
| | | | ||
| + | :The first earned two dirham, and [the others] exceeded by three [dirham]. | ||
|style="text-align:right;"|והרויח הראשון שנים דרהמי והוסיפו בשלשה שלשה רביעים | |style="text-align:right;"|והרויח הראשון שנים דרהמי והוסיפו בשלשה שלשה רביעים | ||
|- | |- | ||
| | | | ||
| − | :the total | + | :According to what we explained, the total of what the three-quarters [of the group] earned should be six-eighths of a square and three-quarters of an eighth of a square plus three-eighths of a thing. |
| + | :<math>\scriptstyle{\color{blue}{\frac{6}{8}x^2+\left(\frac{3}{4}\sdot\frac{1}{8}\right)x^2+\frac{3}{8}x}}</math> | ||
|style="text-align:right;"|וצריך כפי מה שבארנו שיהיה כל אשר הרויחו שלשת רביעי הדבר ששה שמיניות מאלגו ושלשת רביעי שמינית האלגו ושלשה שמיניות מדבר | |style="text-align:right;"|וצריך כפי מה שבארנו שיהיה כל אשר הרויחו שלשת רביעי הדבר ששה שמיניות מאלגו ושלשת רביעי שמינית האלגו ושלשה שמיניות מדבר | ||
|- | |- | ||
| | | | ||
| + | :Divide it by the number of [members in] the group, which is a thing. | ||
:<math>\scriptstyle{\color{blue}{\frac{\frac{6}{8}x^2+\left(\frac{3}{4}\sdot\frac{1}{8}\right)x^2+\frac{3}{8}x}{x}=24}}</math> | :<math>\scriptstyle{\color{blue}{\frac{\frac{6}{8}x^2+\left(\frac{3}{4}\sdot\frac{1}{8}\right)x^2+\frac{3}{8}x}{x}=24}}</math> | ||
|style="text-align:right;"|ותחלקהו על מספר החבורה שהוא דבר | |style="text-align:right;"|ותחלקהו על מספר החבורה שהוא דבר | ||
|- | |- | ||
| | | | ||
| + | :It is twenty-four things equal [six-eighths of a square and] three-quarters [of an eighth] of a square plus three-eighths of a thing. | ||
:<math>\scriptstyle{\color{blue}{\frac{6}{8}x^2+\left(\frac{3}{4}\sdot\frac{1}{8}\right)x^2+\frac{3}{8}x=24x}}</math> | :<math>\scriptstyle{\color{blue}{\frac{6}{8}x^2+\left(\frac{3}{4}\sdot\frac{1}{8}\right)x^2+\frac{3}{8}x=24x}}</math> | ||
|style="text-align:right;"|ויהיה עשרים וארבעה דברים ישוה שלשה רביעי אלגו ושלשה שמיניות מדבר | |style="text-align:right;"|ויהיה עשרים וארבעה דברים ישוה שלשה רביעי אלגו ושלשה שמיניות מדבר | ||
|- | |- | ||
| | | | ||
| − | :<span style="color:Green>Confrontation:</span> Confront them; the thing becomes twenty-eight and this is the number of [members in] the group. | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Confront them; the thing becomes twenty-eight and this is the number of [members in] the group. | ||
:<math>\scriptstyle{\color{blue}{x=28}}</math> | :<math>\scriptstyle{\color{blue}{x=28}}</math> | ||
|style="text-align:right;"|ותכוין עמהם ויהיה הדבר עשרים ושמנה והוא מספר החבורה | |style="text-align:right;"|ותכוין עמהם ויהיה הדבר עשרים ושמנה והוא מספר החבורה | ||
|- | |- | ||
| | | | ||
| − | :53) If you are told: a group, its two thirds earned, the first earned one dirham, and [the others] exceeded by two dirham. The remaining third - the first earned two dirham and [the others] exceeded by four [dirham]. Then the total earning of all people of the group was summed up and divided equally between them and each got 20 dirham. How many were in the group? | + | :{{#annot:unknown number of partners|661|K78Z}}53) If you are told: a group, its two thirds earned, the first earned one dirham, and [the others] exceeded by two dirham. The remaining third - the first earned two dirham and [the others] exceeded by four [dirham]. Then the total earning of all people of the group was summed up and divided equally between them and each got 20 dirham. How many were in the group? |
| − | |style="text-align:right;"|נג | + | |style="text-align:right;"|נג <big>ואם</big> יאמרו לך חבורה ששני שלישיה יצאו להרויח והרויח הראשון מהם דרהם אחד והוסיפו בשני דרהמי והשלישית הנשאר הרויח הראשון מהם שנים דרהמי והוסיפו בארבעה וקבצו כל מה שהרויחו כל אנשי החבורה וחלקוהו <s>בין שניהם</s> ביניהם בשוה והגיע לכל אחד מהם עשרים דרהמי כמה יהיה מספר החבורה{{#annotend:K78Z}} |
|- | |- | ||
| | | | ||
| Line 4,432: | Line 4,903: | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ויצאו ‫<ref>85r</ref>שני שלישיה להרויח והם שלישי דבר | + | :Two-thirds of [the group] earned - they are two-thirds of a thing. |
| + | :<math>\scriptstyle{\color{blue}{\frac{2}{3}x}}</math> | ||
| + | |style="text-align:right;"|ויצאו ‫<ref>85r</ref>שני שלישיה להרויח והם שלישי דבר | ||
|- | |- | ||
| | | | ||
| − | :the total | + | :The first earned one dirham, and [the others] exceeded by two dirham. |
| + | |style="text-align:right;"|והרויח הראשון דרהם אחד ונתוספו בשני דרהמי | ||
| + | |- | ||
| + | | | ||
| + | :According to what we explained, the total of what the two-thirds [of the group] earned should be four-ninths of a square. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{4}{9}x^2}}</math> | ||
|style="text-align:right;"|וצריך לפי מה שבארנו שיהיה מה שהרויחו שני השלישים ארבעה תשיעיות מאלגו | |style="text-align:right;"|וצריך לפי מה שבארנו שיהיה מה שהרויחו שני השלישים ארבעה תשיעיות מאלגו | ||
|- | |- | ||
| | | | ||
| + | :The first of the other third [of the group] earned two dirham, and [the others] exceeded by four [dirham]. | ||
|style="text-align:right;"|והשליש האחר הרויח הראשון מהם שני דרהמי ונתוספו הארבעה | |style="text-align:right;"|והשליש האחר הרויח הראשון מהם שני דרהמי ונתוספו הארבעה | ||
|- | |- | ||
| | | | ||
| − | :the total | + | :According to what we explained, the total of what the third [of the group] earned should be two-ninths of a square. |
| + | :<math>\scriptstyle{\color{blue}{\frac{2}{9}x^2}}</math> | ||
|style="text-align:right;"|וצריך כפי מה שבארנו שיהיה מה שהרויח השלישית שתי תשיעיות מאלגו | |style="text-align:right;"|וצריך כפי מה שבארנו שיהיה מה שהרויח השלישית שתי תשיעיות מאלגו | ||
|- | |- | ||
| | | | ||
| − | :the total | + | :We you sum it with what the two-thirds [of the group] earned, which is four-ninths, the total of the whole group earned is two-thirds of a square. |
| + | :<math>\scriptstyle{\color{blue}{\frac{4}{9}x^2+\frac{2}{9}x^2=\frac{2}{3}x^2}}</math> | ||
|style="text-align:right;"|ותקבצם עם אשר הרויחו השני שלישים והוא ארבעה תשיעיות ויהיה כל אשר הרויחו כל אנשי החבורה שני שלישיות מאלגו | |style="text-align:right;"|ותקבצם עם אשר הרויחו השני שלישים והוא ארבעה תשיעיות ויהיה כל אשר הרויחו כל אנשי החבורה שני שלישיות מאלגו | ||
|- | |- | ||
| | | | ||
| − | :Divide it by the number of [members in] the group, which is a thing; it | + | :Divide it by the number of [members in] the group, which is a thing; it [should be equal to] twenty. |
:<math>\scriptstyle{\color{blue}{\frac{\frac{2}{3}x^2}{x}=20}}</math> | :<math>\scriptstyle{\color{blue}{\frac{\frac{2}{3}x^2}{x}=20}}</math> | ||
|style="text-align:right;"|ותחלקהו על מספר החבורה שהוא דבר ויהיה עשרים | |style="text-align:right;"|ותחלקהו על מספר החבורה שהוא דבר ויהיה עשרים | ||
| Line 4,470: | Line 4,951: | ||
|- | |- | ||
| | | | ||
| − | :54) If you are told: a group of people earned, the first earned one dirham, and [each of them] exceeded by one dirham. Then they summed up the total earning of all and divided it equally between them and each got [an amount] equal to two thirds of the number of [members in] the group | + | :{{#annot:unknown number of partners|661|SKAs}}54) If you are told: a group of people earned, the first earned one dirham, and [each of them] exceeded by one dirham. Then they summed up the total earning of all and divided it equally between them and each got [an amount] equal to two thirds of the number of [members in] the group. |
| − | |style="text-align:right;"|נד | + | |style="text-align:right;"|נד <big>ואם</big> יאמרו לך חבורה מאנשים יצאו להרויח והרויח הראשון דרהם אחד ונתוספו באחד וכאשר שבו קבצו יחד כל מה שהרויחו כלם וחלקוהו ביניהם בשוה והגיע לכל אחד מהם כמו שני שלישי מספר החבורה{{#annotend:SKAs}} |
|- | |- | ||
| | | | ||
| Line 4,478: | Line 4,959: | ||
|- | |- | ||
| | | | ||
| − | :the total | + | :According to what we explained, the total of what the whole [group] earned is half a square and half a thing. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}x^2+\frac{1}{2}x}}</math> | ||
|style="text-align:right;"|שיהיה כמו שבארנו כל מה שהרויחו בם חצי אלגו וחצי דבר | |style="text-align:right;"|שיהיה כמו שבארנו כל מה שהרויחו בם חצי אלגו וחצי דבר | ||
|- | |- | ||
| | | | ||
| + | :We divide it by the number of [members in] the group, which is a thing; it [should be equal to] two-thirds of a thing. | ||
:<math>\scriptstyle{\color{blue}{\frac{\frac{1}{2}x^2+\frac{1}{2}x}{x}=\frac{2}{3}x}}</math> | :<math>\scriptstyle{\color{blue}{\frac{\frac{1}{2}x^2+\frac{1}{2}x}{x}=\frac{2}{3}x}}</math> | ||
|style="text-align:right;"|ונחלקהו על מספר החבורה והוא דבר ויהיה שני שלישי דבר | |style="text-align:right;"|ונחלקהו על מספר החבורה והוא דבר ויהיה שני שלישי דבר | ||
|- | |- | ||
| | | | ||
| + | :Multiply two-thirds of a thing by a thing; it is two-thirds of a thing equal half a square and half a thing. | ||
:<math>\scriptstyle{\color{blue}{\frac{1}{2}x^2+\frac{1}{2}x=\frac{2}{3}x^2}}</math> | :<math>\scriptstyle{\color{blue}{\frac{1}{2}x^2+\frac{1}{2}x=\frac{2}{3}x^2}}</math> | ||
|style="text-align:right;"|ותכה שני שלישי דבר בדבר ויהיה שני שלישי אלגו ישוה חצי אלגו וחצי דבר | |style="text-align:right;"|ותכה שני שלישי דבר בדבר ויהיה שני שלישי אלגו ישוה חצי אלגו וחצי דבר | ||
|- | |- | ||
| | | | ||
| + | :The thing is equal to three dirham and this is the number of [members in] the group. | ||
:<math>\scriptstyle{\color{blue}{x=3}}</math> | :<math>\scriptstyle{\color{blue}{x=3}}</math> | ||
|style="text-align:right;"|והדבר ישוה שלשה דרהמי והוא מספר החבורה | |style="text-align:right;"|והדבר ישוה שלשה דרהמי והוא מספר החבורה | ||
|- | |- | ||
| | | | ||
| − | :55) If you are told: a group, | + | :{{#annot:unknown number of partners|661|VeIc}}55) If you are told: a group, four and a half of its root earned, the first earned one dirham, and [each of them] exceeded by one dirham. Then they shared the total earning between all [members of] the group and each got ten dirham and a half. How many were in the group? |
| − | |style="text-align:right;"|נה | + | |style="text-align:right;"|נה <big>ואם</big> יאמרו לך חבורה שיצאו ארבעה שרשיה וחצי שרש להרויח והרויח הראשון דרהם אחד ונתוספו באדרהם אחד וכאשר שבו חלקו מה שהרויחו בין כל החבורה בשוה והגיע לכל אחד מהם עשרה דרהמי וחצי כמה יהיה מספר החבורה{{#annotend:VeIc}} |
|- | |- | ||
| | | | ||
| − | :To know this, suppose that the number of [members in] the group is a | + | :To know this, suppose that the number of [members in] the group is a square [<math>\scriptstyle{\color{blue}{x^2}}</math>]. |
|style="text-align:right;"|ולדעת זה תניח מספר החבורה <s>בצד</s> אלגו | |style="text-align:right;"|ולדעת זה תניח מספר החבורה <s>בצד</s> אלגו | ||
|- | |- | ||
| | | | ||
| − | : | + | :Its four roots and a half is four things and a half. |
| + | :<math>\scriptstyle{\color{blue}{4\frac{1}{2}\sqrt{x^2}=4\frac{1}{2}x}}</math> | ||
|style="text-align:right;"|ויצאו ארבעת שרשיו וחצי והוא ארבעה דברי' וחצי | |style="text-align:right;"|ויצאו ארבעת שרשיו וחצי והוא ארבעה דברי' וחצי | ||
|- | |- | ||
| | | | ||
| + | :The first earned one dirham, and [the others] exceeded by one dirham. | ||
|style="text-align:right;"|והריוח הראשון דרהם אחד ונתוספו באדרהם אחד | |style="text-align:right;"|והריוח הראשון דרהם אחד ונתוספו באדרהם אחד | ||
|- | |- | ||
| | | | ||
| − | :the total | + | :According to what we explained, the total of what they earned should be ten squares and an eighth of a square plus two things and a quarter of a thing. |
| + | :<math>\scriptstyle{\color{blue}{10\frac{1}{8}x^2+2\frac{1}{4}x}}</math> | ||
|style="text-align:right;"|וצריך שיהיה מה שהרויחו כפי מה שבארנו עשרה אלגוש ושמינית מאלגו ושני דברים ורביע דבר | |style="text-align:right;"|וצריך שיהיה מה שהרויחו כפי מה שבארנו עשרה אלגוש ושמינית מאלגו ושני דברים ורביע דבר | ||
|- | |- | ||
| | | | ||
| + | :We divide it by the number of [members in] the group, which is a square; the quotient [should be equal to] ten dirham and a half. | ||
:<math>\scriptstyle{\color{blue}{\frac{10\frac{1}{8}x^2+2\frac{1}{4}x}{x^2}=10\frac{1}{2}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{10\frac{1}{8}x^2+2\frac{1}{4}x}{x^2}=10\frac{1}{2}}}</math> | ||
|style="text-align:right;"|ונחלקם על מספר החבורה והוא אלגו ויצא לחלק עשרה דרהמי וחצי | |style="text-align:right;"|ונחלקם על מספר החבורה והוא אלגו ויצא לחלק עשרה דרהמי וחצי | ||
|- | |- | ||
| | | | ||
| + | :Multiply ten dirham and a half by a square; it is ten squares and a half equal ten squares and an eighth of a square plus two things and a quarter. | ||
:<math>\scriptstyle{\color{blue}{10\frac{1}{8}x^2+2\frac{1}{4}x=10\frac{1}{2}x^2}}</math> | :<math>\scriptstyle{\color{blue}{10\frac{1}{8}x^2+2\frac{1}{4}x=10\frac{1}{2}x^2}}</math> | ||
|style="text-align:right;"|ותכה עשרה דרהמי וחצי באלגו ויהיה עשרה אלגוש וחצי ישוו עשרה אלגוש ושמינית מאלגו ושני דברים ורביע | |style="text-align:right;"|ותכה עשרה דרהמי וחצי באלגו ויהיה עשרה אלגוש וחצי ישוו עשרה אלגוש ושמינית מאלגו ושני דברים ורביע | ||
|- | |- | ||
| | | | ||
| − | :<span style="color:Green>Confrontation:</span> Confront them; the thing becomes six. | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Confront them; the thing becomes six. | ||
:<math>\scriptstyle{\color{blue}{x=6}}</math> | :<math>\scriptstyle{\color{blue}{x=6}}</math> | ||
|style="text-align:right;"|ותכוין עמהם ויהיה הדבר ‫<ref>85v</ref>ששה | |style="text-align:right;"|ותכוין עמהם ויהיה הדבר ‫<ref>85v</ref>ששה | ||
| Line 4,531: | Line 5,025: | ||
|- | |- | ||
| | | | ||
| − | :56) If you are told: | + | :{{#annot:30 partners|661|L0wC}}56) If you are told: thirty people, the first earned one dirham, and [each of them] exceeded by one thing. Their total earning was 1335. By how much did each one exceed his former? |
| − | |style="text-align:right;"|נו | + | |style="text-align:right;"|נו <big>ואם</big> יאמרו לך שלשים אנשים הרויח הראשון דרהם אחד ונתוספו בדבר אחד והיה כל אשר הרויחו אלף ושלש מאות ושלשים וחמשה בכמה נתוספו כל אחד על האחר אשר לפניו{{#annotend:L0wC}} |
|- | |- | ||
| | | | ||
| Line 4,540: | Line 5,034: | ||
| | | | ||
:Do as I explained, that is, always multiply the excess, which is a thing, by the whole [number of members in the] group minus one, which is twenty-nine, then add to it what the first earned, which is one dirham; it is 29 things and one dirham and this is what the last earned. | :Do as I explained, that is, always multiply the excess, which is a thing, by the whole [number of members in the] group minus one, which is twenty-nine, then add to it what the first earned, which is one dirham; it is 29 things and one dirham and this is what the last earned. | ||
| + | |style="text-align:right;"|ותעשה בהם כאשר בארתי והוא שתכה מה <s>שיעדיף</s> שהוא ההעדף והוא דבר בחבורה כלה פחות אחד לעולם ויהיה עשרים ותשעה ותוסיף עליהם מה שהרויח הראשון והוא דרהם ויהיה כ"ט דברים ודרהם אחד והוא מה שהרויח האחרון | ||
| + | |- | ||
| + | | colspan="2"| | ||
:<math>\scriptstyle{\color{blue}{a_{30}=a_n=\left[d\sdot\left(n-1\right)\right]+a_1=\left[x\sdot\left(30-1\right)\right]+1=29x+1}}</math> | :<math>\scriptstyle{\color{blue}{a_{30}=a_n=\left[d\sdot\left(n-1\right)\right]+a_1=\left[x\sdot\left(30-1\right)\right]+1=29x+1}}</math> | ||
| − | |||
|- | |- | ||
| | | | ||
:Add to it what the first earned, which is one dirham; it is 29 things and two dirham and this is what every two of them earned according to the method we explained. | :Add to it what the first earned, which is one dirham; it is 29 things and two dirham and this is what every two of them earned according to the method we explained. | ||
| + | |style="text-align:right;"|ותוסיף עליו מה שהרויח הראשון והוא דרהם אחד ויהיה כ"ט דברים ושני דרהמי והוא מה שהרויח כל <s>אחד</s> <sup>שנים</sup> מהם כפי הסדר שבארנו | ||
| + | |- | ||
| + | | colspan="2"| | ||
:<math>\scriptstyle{\color{blue}{a_1+a_{30}=a_2+a_{30-1}=\ldots=29x+1+1=29x+2}}</math> | :<math>\scriptstyle{\color{blue}{a_1+a_{30}=a_2+a_{30-1}=\ldots=29x+1+1=29x+2}}</math> | ||
| − | |||
|- | |- | ||
| | | | ||
:Multiply it by fifteen, which is half the [number of members in the] group; it is thirty dirham plus 435 things, which is all they earned and it is one thousand, three hundred and thirty-five dirham. | :Multiply it by fifteen, which is half the [number of members in the] group; it is thirty dirham plus 435 things, which is all they earned and it is one thousand, three hundred and thirty-five dirham. | ||
| + | |style="text-align:right;"|ותכם בחמש עשרה שהוא מחצית החבורה ויהיה שלשים דרהמי ותל"ה דברים והוא כל אשר הרויחו א"י אלוגלו ויהיה אלף ושלש מאות ושלשים וחמשה דרהמי | ||
| + | |- | ||
| + | | colspan="2"| | ||
:<math>\scriptstyle{\color{blue}{\left(29x+2\right)\sdot\left(\frac{1}{2}\sdot30\right)=\left(29x+2\right)\sdot15=435x+30=1335}}</math> | :<math>\scriptstyle{\color{blue}{\left(29x+2\right)\sdot\left(\frac{1}{2}\sdot30\right)=\left(29x+2\right)\sdot15=435x+30=1335}}</math> | ||
| − | |||
|- | |- | ||
| | | | ||
| − | :<span style="color:Green>Confrontation:</span> Confront them; the thing becomes three and by this each one exceeds his former. | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Confront them; the thing becomes three and by this each one exceeds his former. | ||
:<math>\scriptstyle{\color{blue}{x=3}}</math> | :<math>\scriptstyle{\color{blue}{x=3}}</math> | ||
|style="text-align:right;"|ותכוין עמהם ויהיה הדבר שלשה והוא <s>כל</s> מה שהוסיף כל אחד מהם על אשר לפניו | |style="text-align:right;"|ותכוין עמהם ויהיה הדבר שלשה והוא <s>כל</s> מה שהוסיף כל אחד מהם על אשר לפניו | ||
| Line 4,565: | Line 5,069: | ||
|- | |- | ||
| | | | ||
| − | :57) If you are told: I sent a man from the city and ordered him to walk on the first day one parsa and add one parsa each day. He walked 81 days. | + | :{{#annot:two men|657|QZ2E}}57) If you are told: I sent a man from the city and ordered him to walk on the first day one parsa and add one parsa each day. He walked 81 days. |
:Then I sent another man and ordered him to walk on the first day one parsa and add two parsa each day. | :Then I sent another man and ordered him to walk on the first day one parsa and add two parsa each day. | ||
:In how many days he will meet the other one? | :In how many days he will meet the other one? | ||
| − | |style="text-align:right;"|נז | + | |style="text-align:right;"|נז <big>ואם</big> יאמרו לך שלחתי איש אחד מן העיר וצויתיו שילך [ביום ראשון]‫<ref>marg.</ref> <s>בכל יום</s> פרסא אחת ושיוסיף בכל יום פרסא אחת והלך שמנים וארבעה ימים<br> |
ואחר זה שלחתי איש אחר וצויתיו שילך [ביום ראשון]‫<ref>marg.</ref> <s>בכל יום</s> פרסא אחת ושיוסיף בכל יום שתי פרסאות<br> | ואחר זה שלחתי איש אחר וצויתיו שילך [ביום ראשון]‫<ref>marg.</ref> <s>בכל יום</s> פרסא אחת ושיוסיף בכל יום שתי פרסאות<br> | ||
| − | בכמה ימים יפגוש באחר | + | בכמה ימים יפגוש באחר{{#annotend:QZ2E}} |
|- | |- | ||
| | | | ||
| Line 4,588: | Line 5,092: | ||
| | | | ||
:The number of parsa, according to what we explained with regard to partnership, should be half a square plus seven thousand and fifty-six minus 168 things, and this is equal to half a square plus half a thing. | :The number of parsa, according to what we explained with regard to partnership, should be half a square plus seven thousand and fifty-six minus 168 things, and this is equal to half a square plus half a thing. | ||
| + | |style="text-align:right;"|וצריך שיהיה מספר הפרסאות כפי מה שבארנו בחבורה אלגו ושבעה אלפים וחמשים וששה פחות קס"ח דברים ויהיה שוה חצי אלגו וחצי דבר | ||
| + | |- | ||
| + | | colspan="2"| | ||
:<math>\scriptstyle{\color{blue}{\sum_{i=1}^{x-84} \left(2i-1\right)=x^2+7056-168x=\frac{1}{2}x^2+\frac{1}{2}x}}</math> | :<math>\scriptstyle{\color{blue}{\sum_{i=1}^{x-84} \left(2i-1\right)=x^2+7056-168x=\frac{1}{2}x^2+\frac{1}{2}x}}</math> | ||
| − | |||
|- | |- | ||
| | | | ||
| − | :<span style="color:Green>Confrontation:</span> Confront them; the thing becomes two hundred and eighty-eight and this is [the number of days] that the first walked. | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Confront them; the thing becomes two hundred and eighty-eight and this is [the number of days] that the first walked. | ||
:<math>\scriptstyle{\color{blue}{x=288}}</math> | :<math>\scriptstyle{\color{blue}{x=288}}</math> | ||
|style="text-align:right;"|ותכוין עמהם ויהיה הדבר מאתים ושמנים ושמנה והוא מה שהלך האיש הראשון | |style="text-align:right;"|ותכוין עמהם ויהיה הדבר מאתים ושמנים ושמנה והוא מה שהלך האיש הראשון | ||
| Line 4,602: | Line 5,112: | ||
|- | |- | ||
| | | | ||
| − | :58) If you are told: I ordered the first to walk on the first day one parsa and add one parsa each day. He walked 8 days. | + | :{{#annot:two men|657|KG1s}}58) If you are told: I ordered the first to walk on the first day one parsa and add one parsa each day. He walked 8 days. |
:I ordered the second to walk on the first day one parsa and add three parsa each day. | :I ordered the second to walk on the first day one parsa and add three parsa each day. | ||
:In how many days he will find him? | :In how many days he will find him? | ||
| − | |style="text-align:right;"|‫<ref>112r</ref>נח | + | |style="text-align:right;"|‫<ref>112r</ref>נח <big>ואם</big> יאמרו לך צויתי לראשון שילך ביום ראשון פרסא אחת ושיוסיף בכל יום פרסא אחת והלך שמנה ימים<br> |
וצויתי לשני שילך בכל יום הראשון פרסא אחת ושיוסיף בכל יום שלשה פרסאות<br> | וצויתי לשני שילך בכל יום הראשון פרסא אחת ושיוסיף בכל יום שלשה פרסאות<br> | ||
| − | בכמה ימים ימצאנו | + | בכמה ימים ימצאנו{{#annotend:KG1s}} |
|- | |- | ||
| | | | ||
| Line 4,625: | Line 5,135: | ||
| | | | ||
:The number of parsa [he walked] should be should be a square and a half plus one hundred minus 24 things and a half and this is equal to half a square plus half a thing. | :The number of parsa [he walked] should be should be a square and a half plus one hundred minus 24 things and a half and this is equal to half a square plus half a thing. | ||
| + | |style="text-align:right;"|וצריך שיהיה מספר פרסותיו אלגו וחצי ומאה דרהמי פחות כ"ד דברים וחצי והוא ישוה חצי אלגו וחצי דבר | ||
| + | |- | ||
| + | | colspan="2"| | ||
:<math>\scriptstyle{\color{blue}{\sum_{i=1}^{x-8} \left[1+\left(3i-3\right)\right]=1\frac{1}{2}x^2+100-24\frac{1}{2}x=\frac{1}{2}x^2+\frac{1}{2}x}}</math> | :<math>\scriptstyle{\color{blue}{\sum_{i=1}^{x-8} \left[1+\left(3i-3\right)\right]=1\frac{1}{2}x^2+100-24\frac{1}{2}x=\frac{1}{2}x^2+\frac{1}{2}x}}</math> | ||
| − | |||
|- | |- | ||
| | | | ||
| − | :<span style="color:Green>Confrontation:</span> Confront them; the thing becomes twenty days and this is [the number of days] that the first walked. | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Confront them; the thing becomes twenty days and this is [the number of days] that the first walked. | ||
:<math>\scriptstyle{\color{blue}{x=20}}</math> | :<math>\scriptstyle{\color{blue}{x=20}}</math> | ||
|style="text-align:right;"|ותכוין עמהם ויהיה הדבר עשרים ימים והוא מה שהלך הראשון | |style="text-align:right;"|ותכוין עמהם ויהיה הדבר עשרים ימים והוא מה שהלך הראשון | ||
| Line 4,639: | Line 5,155: | ||
|- | |- | ||
| | | | ||
| − | :59) If you are told: a man left the city and I ordered him to walk each day | + | :{{#annot:two men|657|JtEx}}59) If you are told: a man left the city and I ordered him to walk each day twenty parsa. He walked 15 days. |
:Then I sent another man after him and ordered him to walk each day the root of the number of parsa that the first one walked. | :Then I sent another man after him and ordered him to walk each day the root of the number of parsa that the first one walked. | ||
| − | |style="text-align:right;"|נט | + | |style="text-align:right;"|נט <big>ואם</big> יאמרו לך איש אחד עלה מן העיר <s>וצורתיו</s> וצויתיו שילך בכל יום עשרים פרסאות והלך ט"ו ימים<br> |
| − | וצויתי <sup>ושלחתי</sup> לאיש אחר אחר זה וצויתיו שילך בכל יום שרש מספר הפרסאות שיפגע בהם הראשון | + | וצויתי <sup>ושלחתי</sup> לאיש אחר אחר זה וצויתיו שילך בכל יום שרש מספר הפרסאות שיפגע בהם הראשון{{#annotend:JtEx}} |
|- | |- | ||
| | | | ||
| Line 4,666: | Line 5,182: | ||
|- | |- | ||
| | | | ||
| − | :<span style="color:Green>Confrontation:</span> Confront them; it is a square equals three hundred plus twenty things. | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Confront them; it is a square equals three hundred plus twenty things. | ||
:<math>\scriptstyle{\color{blue}{x^2=20x+300}}</math> | :<math>\scriptstyle{\color{blue}{x^2=20x+300}}</math> | ||
|style="text-align:right;"|ותכוין עמהם ויהיה אלגו ישוה שלש מאות דרהמי ועשרים דברים | |style="text-align:right;"|ותכוין עמהם ויהיה אלגו ישוה שלש מאות דרהמי ועשרים דברים | ||
| Line 4,686: | Line 5,206: | ||
|- | |- | ||
| | | | ||
| − | :60) If you are told: a man left the city and I ordered him to walk each day 20 parsa. He walked 4[4] days. | + | :{{#annot:two men|657|pBJB}}60) If you are told: a man left the city and I ordered him to walk each day 20 parsa. He walked 4[4] days. |
:Then I sent another man after him and ordered him to walk each day the root of the number of days that the first one walked. | :Then I sent another man after him and ordered him to walk each day the root of the number of days that the first one walked. | ||
| − | |style="text-align:right;"|ס | + | |style="text-align:right;"|ס <big>ואם</big> יאמרו לך איש אחד עלה מן העיר וצויתיו שילך בכל יום עשרים פרסאות והלך מ"ה ימים<br> |
| − | ושלחתי אחר זה אחר וצויתיו שילך בכל יום שרש מאשר הלך הראשון מהימים שימצאהו בהם | + | ושלחתי אחר זה אחר וצויתיו שילך בכל יום שרש מאשר הלך הראשון מהימים שימצאהו בהם{{#annotend:pBJB}} |
|- | |- | ||
| | | | ||
| Line 4,711: | Line 5,231: | ||
|- | |- | ||
| | | | ||
| − | :<span style="color:Green>Confrontation:</span> Confront them; the thing becomes twenty-two and it is the root of [the number of] days the first walked. | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Confront them; the thing becomes twenty-two and it is the root of [the number of] days the first walked. | ||
:<math>\scriptstyle{\color{blue}{x=22}}</math> | :<math>\scriptstyle{\color{blue}{x=22}}</math> | ||
|style="text-align:right;"|ותכוין עמהם ויהיה הדבר עשרים ושנים והוא שרש הימים שהלך הראשון | |style="text-align:right;"|ותכוין עמהם ויהיה הדבר עשרים ושנים והוא שרש הימים שהלך הראשון | ||
| Line 4,737: | Line 5,261: | ||
| | | | ||
| − | === <span style="color:Green> | + | === <span style="color:Green>Purchase Problems</span> === |
| | | | ||
|- | |- | ||
| | | | ||
| − | :61) If you are told: one ounce for 20 dirham, one ounce for 15 dirham and one ounce for 10, and you are told to take of all one ounce for 18 dirham | + | :{{#annot:three types of ounces|643|q40b}}61) If you are told: one ounce for 20 dirham, one ounce for 15 dirham and one ounce for 10, and you are told to take of all one ounce for 18 dirham |
| − | |style="text-align:right;"|סא <big>ואם</big> יאמרו לך אונקיא אחת בעד עשרים דרהמי ואונקיא אחת בעד ט"ו דרהמי ואונקיא בעד עשרה ואמרו לך תקח מהכל אונקי' אחת בעד י"ח דרהמי | + | |style="text-align:right;"|סא <big>ואם</big> יאמרו לך אונקיא אחת בעד עשרים דרהמי ואונקיא אחת בעד ט"ו דרהמי ואונקיא בעד עשרה ואמרו לך תקח מהכל אונקי' אחת בעד י"ח דרהמי{{#annotend:q40b}} |
|- | |- | ||
| | | | ||
| Line 4,750: | Line 5,274: | ||
|- | |- | ||
| | | | ||
| − | :Knowing this is that you take a thing [<math>\scriptstyle{\color{blue}{x}}</math>] of the ounce for ten at [ten] things | + | :Knowing this is that you take a thing [<math>\scriptstyle{\color{blue}{x}}</math>] of the ounce for ten at [ten] things [<math>\scriptstyle{\color{blue}{10x}}</math>] of the dirham and a thing [<math>\scriptstyle{\color{blue}{x}}</math>] of the ounce for 15 at 15 things [<math>\scriptstyle{\color{blue}{15x}}</math>] of the dirham. |
|style="text-align:right;"|וידיעתה שתקח דבר מהאונקיא שהיא בעד עשרה דברים מהדרהמי ודבר מהאונקיא שהיא בעד ט"ו דברים מהדרהמי | |style="text-align:right;"|וידיעתה שתקח דבר מהאונקיא שהיא בעד עשרה דברים מהדרהמי ודבר מהאונקיא שהיא בעד ט"ו דברים מהדרהמי | ||
|- | |- | ||
| Line 4,774: | Line 5,298: | ||
|- | |- | ||
| | | | ||
| − | :<span style="color:Green>Confrontation:</span> Confront them; the thing equals two-thirds of a fifth and so he bought of the ounce for 10 [dirham]. | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Confront them; the thing equals two-thirds of a fifth and so he bought of the ounce for 10 [dirham]. | ||
:<math>\scriptstyle{\color{blue}{x=\frac{2}{3}\sdot\frac{1}{5}}}</math> | :<math>\scriptstyle{\color{blue}{x=\frac{2}{3}\sdot\frac{1}{5}}}</math> | ||
|style="text-align:right;"|ותכוין עמהם ויהיה הדבר ישוה שני שלישי החומש <s>ומספר</s> <sup>וקנה מן</sup> האונקיא שהיא בעד עשרה | |style="text-align:right;"|ותכוין עמהם ויהיה הדבר ישוה שני שלישי החומש <s>ומספר</s> <sup>וקנה מן</sup> האונקיא שהיא בעד עשרה | ||
|- | |- | ||
| | | | ||
| − | : | + | :Of [the ounce] for 15 [dirham] [he bought] 2 [parts] of 15 of an ounce. |
|style="text-align:right;"|ומאשר היא בעד ט"ו מכל אחת ב' מט"ו מאונקיא | |style="text-align:right;"|ומאשר היא בעד ט"ו מכל אחת ב' מט"ו מאונקיא | ||
|- | |- | ||
| | | | ||
| − | : | + | :Of the ounce for twenty [dirham] he bought 11 [parts] of 15 of an ounce. |
|style="text-align:right;"|<s>ומספר</s> <sup>וקנה מן</sup> האונקיא שהיא בעד עשרים י"א מט"ו מאונקיא | |style="text-align:right;"|<s>ומספר</s> <sup>וקנה מן</sup> האונקיא שהיא בעד עשרים י"א מט"ו מאונקיא | ||
|- | |- | ||
| | | | ||
| − | *take | + | *If you want, take a thing [<math>\scriptstyle{\color{blue}{x}}</math>] of the ounce for ten at [ten] things [<math>\scriptstyle{\color{blue}{10x}}</math>] of the dirham and take two things [<math>\scriptstyle{\color{blue}{2x}}</math>] of the ounce for 15 at [30] things [<math>\scriptstyle{\color{blue}{30x}}</math>] of the dirham. |
| − | |style="text-align:right;"|ואם תרצה תקח דבר מהאונקיא שהיא בעד עשרה דברים מהדרהמי | + | |style="text-align:right;"|ואם תרצה תקח דבר מהאונקיא שהיא בעד עשרה דברים מהדרהמי ותקח שני דברים מהאונקיא שהיא בעד ט"ו דברים מהדרהמי |
|- | |- | ||
| | | | ||
| − | + | :18 dirham minus forty things remain from the price of the ounce [for 18]. | |
| − | + | :<math>\scriptstyle{\color{blue}{18-10x-30x=18-40x}}</math> | |
| − | |||
| − | |||
| − | |||
|style="text-align:right;"|וישאר ערך האונקיא י"ח דרהמי פחות ארבעים דברים | |style="text-align:right;"|וישאר ערך האונקיא י"ח דרהמי פחות ארבעים דברים | ||
|- | |- | ||
| | | | ||
| − | : | + | :One ounce minus three things remain from the ounce. |
| + | :<math>\scriptstyle{\color{blue}{1-x-2x=1-3x}}</math> | ||
|style="text-align:right;"|ומהאונקיא אונקיא פחות שלשה דברים | |style="text-align:right;"|ומהאונקיא אונקיא פחות שלשה דברים | ||
|- | |- | ||
| | | | ||
| − | : | + | :He bought such [a quantity] from the ounce at 20 dirham for twenty dirham minus [sixty] things. |
|style="text-align:right;"|וקנה בהם מהאונקיא שהיא בעד עשרים דרהמי בעד עשרים דרהמי פחות שני דברים | |style="text-align:right;"|וקנה בהם מהאונקיא שהיא בעד עשרים דרהמי בעד עשרים דרהמי פחות שני דברים | ||
|- | |- | ||
| | | | ||
| + | :The thing is equal to 1[8] dirham minus forty things. | ||
:<math>\scriptstyle{\color{blue}{1{\color{red}{8}}-40x=20-{\color{red}{60}}x}}</math> | :<math>\scriptstyle{\color{blue}{1{\color{red}{8}}-40x=20-{\color{red}{60}}x}}</math> | ||
|style="text-align:right;"|והדבר ישוה י"ו דרהמי פחות ארבעים דברים | |style="text-align:right;"|והדבר ישוה י"ו דרהמי פחות ארבעים דברים | ||
|- | |- | ||
| | | | ||
| − | :<span style="color:Green>Confrontation:</span> Confront them; the thing becomes [a tenth], so take one tenth of the ounce for 10 [dirham]. | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Confront them; the thing becomes [a tenth], so take one tenth of the ounce for 10 [dirham]. | ||
:<math>\scriptstyle{\color{blue}{x=\frac{1}{10}}}</math> | :<math>\scriptstyle{\color{blue}{x=\frac{1}{10}}}</math> | ||
|style="text-align:right;"|ותכוין עמהם ויהיה הדבר עשרה ותקח מהאונקיא שהיא בעד עשרה עשירית אחד מאונקיא | |style="text-align:right;"|ותכוין עמהם ויהיה הדבר עשרה ותקח מהאונקיא שהיא בעד עשרה עשירית אחד מאונקיא | ||
|- | |- | ||
| | | | ||
| − | : | + | :Of [the ounce] for 15 [dirham] [take] one fifth of an ounce. |
|style="text-align:right;"|ומאשר היא בעד ט"ו חמישית אחד מאונקיא | |style="text-align:right;"|ומאשר היא בעד ט"ו חמישית אחד מאונקיא | ||
|- | |- | ||
| | | | ||
| − | : | + | :Of [the ounce] for twenty [dirham] [take] seven tenths of an ounce, [which is] a half and a fifth of an ounce. |
| + | :<math>\scriptstyle{\color{blue}{\frac{7}{10}=\frac{1}{2}+\frac{1}{5}}}</math> | ||
|style="text-align:right;"|ומאשר היא בעד עשרים שבעה עשיריות מאונקיא וחצי וחומש מאונקיא | |style="text-align:right;"|ומאשר היא בעד עשרים שבעה עשיריות מאונקיא וחצי וחומש מאונקיא | ||
|- | |- | ||
| | | | ||
| − | :62) If you are told: one liṭra for 5 dirham, one liṭra for 4 dirham and 10 liṭra for one dirham - take from all one liṭra for 2 dirham | + | :{{#annot:three types of liṭra|643|HE6y}}62) If you are told: one liṭra for 5 dirham, one liṭra for 4 dirham and 10 liṭra for one dirham - take from all one liṭra for 2 dirham. |
| − | |style="text-align:right;"|‫<ref>113r</ref>סב <big>ואם</big> יאמרו לך ליט' אחת בעד חמשה דרהמי וליט' אחת בעד ארבעה דרהמי ועשרה ליט' בעד דרהם אחד תקח מהכל ליט' אחת בעד שני דרהמי | + | |style="text-align:right;"|‫<ref>113r</ref>סב <big>ואם</big> יאמרו לך ליט' אחת בעד חמשה דרהמי וליט' אחת בעד ארבעה דרהמי ועשרה ליט' בעד דרהם אחד תקח מהכל ליט' אחת בעד שני דרהמי{{#annotend:HE6y}} |
|- | |- | ||
| | | | ||
| Line 4,832: | Line 5,364: | ||
|- | |- | ||
| | | | ||
| − | + | :Knowing this is that you take a thing [<math>\scriptstyle{\color{blue}{x}}</math>] of the liṭra for five dirham at five things [<math>\scriptstyle{\color{blue}{5x}}</math>] of the two dirham and a thing [<math>\scriptstyle{\color{blue}{x}}</math>] of the liṭra for four dirham at four things [<math>\scriptstyle{\color{blue}{4x}}</math>] of the two dirham. | |
| − | |style="text-align:right;"|וידיעתו שתניח דבר מהליט' שהיא בעד חמשה דרהמי בעד חמשה דברים מהשני דרהמי | + | |style="text-align:right;"|וידיעתו שתניח דבר מהליט' שהיא בעד חמשה דרהמי בעד חמשה דברים מהשני דרהמי ודבר מהליט' שהיא בעד ארבעה דרהמי בעד ארבעה דברים משני הדרהמי |
|- | |- | ||
| | | | ||
| − | + | :Two dirham minus nine things remain from the two dirham, which equals a tenth minus a fifth of a thing. | |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{2-5x-4x=2-9x={\color{red}{\frac{1}{10}}}-\frac{1}{5}x}}</math> |
| + | |style="text-align:right;"|וישאר משני הדרהמי שני דרהמי פחות תשעה דברים ישוה עשרה דרהמי פחות חמישית דבר | ||
|- | |- | ||
| | | | ||
| − | : | + | :*<span style="color:Green>Confrontation:</span> |
| − | |||
| − | |||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Confront them; the thing becomes [a ninth] of 88 parts of a liṭra. |
:<math>\scriptstyle{\color{blue}{x=\frac{{\color{red}{1}}9}{88}}}</math> | :<math>\scriptstyle{\color{blue}{x=\frac{{\color{red}{1}}9}{88}}}</math> | ||
|style="text-align:right;"|ותכוין עמהם ויהיה הדבר ט' מפ"ח בליט‫' | |style="text-align:right;"|ותכוין עמהם ויהיה הדבר ט' מפ"ח בליט‫' | ||
|- | |- | ||
| | | | ||
| − | *take | + | *If you want, you take a thing [<math>\scriptstyle{\color{blue}{x}}</math>] of the liṭra for five [dirham] at five things [<math>\scriptstyle{\color{blue}{5x}}</math>] of the two dirham [and five things [<math>\scriptstyle{\color{blue}{5x}}</math>] of the liṭra for four dirham at twenty things [<math>\scriptstyle{\color{blue}{20x}}</math>] of the two dirham]. |
| − | |||
|style="text-align:right;"|ואם תרצה תקח מהליט' שהיא בעד חמשה דברים דבר בחמשה דברים משני הדרהמי | |style="text-align:right;"|ואם תרצה תקח מהליט' שהיא בעד חמשה דברים דבר בחמשה דברים משני הדרהמי | ||
|- | |- | ||
| | | | ||
| − | : | + | :Two dirham minus 25 things remain from the two dirham. |
| + | :<math>\scriptstyle{\color{blue}{2-5x-20x=2-25x}}</math> | ||
|style="text-align:right;"|וישאר משני הדרהמי שני דרהמי פחות כ"ה דברים | |style="text-align:right;"|וישאר משני הדרהמי שני דרהמי פחות כ"ה דברים | ||
|- | |- | ||
| | | | ||
| − | : | + | :One liṭra minus six things remain from the liṭra. |
| + | :<math>\scriptstyle{\color{blue}{1-x-5x=1-6x}}</math> | ||
|style="text-align:right;"|ומהליט' ליט' פחות ששה דברים | |style="text-align:right;"|ומהליט' ליט' פחות ששה דברים | ||
|- | |- | ||
| | | | ||
| − | : | + | :Take from the 10 liṭra for one dirham at a [tenth of a] dirham minus three-fifths of a thing. |
| + | :<math>\scriptstyle{\color{blue}{{\color{red}{\frac{1}{10}\sdot\left(1-6x\right)=\frac{1}{10}}}-\frac{3}{5}x}}</math> | ||
|style="text-align:right;"|ותקח מאשר הוא עשרה ליט' בעד דרהם אחד עם עשרה דרהמי פחות שלשה חומשי דבר | |style="text-align:right;"|ותקח מאשר הוא עשרה ליט' בעד דרהם אחד עם עשרה דרהמי פחות שלשה חומשי דבר | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{{\color{red}{\frac{1}{10}}}-\frac{{\color{red}{3}}}{5}x | + | :It is two dirham minus 25 things equals a [tenth of a] dirham minus [three]-fifths of a thing. |
| + | :<math>\scriptstyle{\color{blue}{2-25x={\color{red}{\frac{1}{10}}}-\frac{{\color{red}{3}}}{5}x}}</math> | ||
|style="text-align:right;"|ויהיה אז שני דרהמי פחות כ"ה דברי' ישוה עשרה דרהמי פחות שני חומשי דבר | |style="text-align:right;"|ויהיה אז שני דרהמי פחות כ"ה דברי' ישוה עשרה דרהמי פחות שני חומשי דבר | ||
|- | |- | ||
| | | | ||
| − | :<span style="color:Green>Confrontation:</span> Confront them; the thing is 19 parts of 244 and this is what he bought of the liṭra for | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Confront them; the thing is 19 parts of 244 and this is what he bought of the liṭra for five dirham. | ||
:<math>\scriptstyle{\color{blue}{x=\frac{19}{244}}}</math> | :<math>\scriptstyle{\color{blue}{x=\frac{19}{244}}}</math> | ||
|style="text-align:right;"|ותכוין עמו ויהיה הדבר י"ט מרמ"ד והוא מה שקנה מהליט' אשר בעד חמשה דרהמי | |style="text-align:right;"|ותכוין עמו ויהיה הדבר י"ט מרמ"ד והוא מה שקנה מהליט' אשר בעד חמשה דרהמי | ||
|- | |- | ||
| | | | ||
| − | : | + | :Of the liṭra for four dirham [he bought] 95 [parts] of 2[4]4 of a liṭra. |
| + | :<math>\scriptstyle{\color{blue}{5x=\frac{95}{244}}}</math> | ||
|style="text-align:right;"|וקנה מהליט' אשר בעד ארבעה דרהמי צ"ה מרפ"ד בליט‫' | |style="text-align:right;"|וקנה מהליט' אשר בעד ארבעה דרהמי צ"ה מרפ"ד בליט‫' | ||
|- | |- | ||
| | | | ||
| − | *take | + | *If you want, you take 3 [parts] of ten and two-fifths of a tenth [<math>\scriptstyle{\color{blue}{\frac{3}{10}+\left(\frac{2}{5}\sdot\frac{1}{10}\right)}}</math>] of the liṭra for five [dirham] at one dirham, a half and a fifth [<math>\scriptstyle{\color{blue}{1\frac{1}{2}+\frac{1}{5}}}</math>]; three [parts] of fifty [<math>\scriptstyle{\color{blue}{\frac{3}{50}}}</math>] of the liṭra for four dirham at a [fifth] and two [parts] of fifty [<math>\scriptstyle{\color{blue}{{\color{red}{\frac{1}{5}}}+\frac{2}{50}}}</math>] of a dirham; and three-fifths [<math>\scriptstyle{\color{blue}{\frac{3}{50}}}</math>] of the ten liṭra for one dirham at three [parts] of fifty [<math>\scriptstyle{\color{blue}{\frac{3}{50}}}</math>] of a dirham. |
| − | |style="text-align:right;"|ואם תרצה תקח מהליט' שהיא בעד חמשה ג' מעשרה בליט' ושני חומשים מעשירית הליט' בעד דרהם אחד וחצי וחומש | + | |style="text-align:right;"|ואם תרצה תקח מהליט' שהיא בעד חמשה ג' מעשרה בליט' ושני חומשים מעשירית הליט' בעד דרהם אחד וחצי וחומש ומהליט' שהיא בעד ארבעה דרהמי שלשה מחמשים בליט' בעד חמשה דרהמי ושנים מחמשים באדרהם ומהליט' שהיא עשרה ליט' בעד דרהם אחד שלשה חומשים מליט' בעד שלשה מחמשים בדרהם |
|- | |- | ||
| | | | ||
| − | + | === <span style="color:Green>Buy and Sell Problems</span> === | |
| − | + | ||
| − | |||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :63) If you are told: I bought wheat for ten dirham at the rate of [three] qafiz for one dirham and I bought sesame [الجلجلان] for three dirham at unknown rate, then I sold both of them at the price of the other and four dirham remained. How much was the price of the sesame? | + | :{{#annot:wheat and sesame|641|Wngu}}63) If you are told: I bought wheat for ten dirham at the rate of [three] qafiz for one dirham and I bought sesame [الجلجلان] for three dirham at unknown rate, then I sold both of them at the price of the other and four dirham remained. How much was the price of the sesame? |
|style="text-align:right;"|סג <big>ואם</big> יאמרו לך קניתי בעד עשרה דרהמי חטה לחשבון הקאפיזיש בעד דרהם אחד<br> | |style="text-align:right;"|סג <big>ואם</big> יאמרו לך קניתי בעד עשרה דרהמי חטה לחשבון הקאפיזיש בעד דרהם אחד<br> | ||
וקניתי עם שלשה דרהמי גולגולאן לחשבון בלתי ידוע<br> | וקניתי עם שלשה דרהמי גולגולאן לחשבון בלתי ידוע<br> | ||
ומכרתי מכל אחד מהם מהחטה כפי סוגו השעור מהגולגולאן ויותירו ארבעה דרהמי<br> | ומכרתי מכל אחד מהם מהחטה כפי סוגו השעור מהגולגולאן ויותירו ארבעה דרהמי<br> | ||
| − | כמה יהיה ערך הגולגלאן | + | כמה יהיה ערך הגולגלאן{{#annotend:Wngu}} |
|- | |- | ||
| | | | ||
| − | + | :Knowing this is that you buy 30 qafiz of wheat for ten dirham at three qafiz for one dirham. | |
| + | :<math>\scriptstyle{\color{blue}{10\times3=30}}</math> | ||
|style="text-align:right;"|וידיעתו שתקנה ל' קאפיזיש מחטה בעד עשרה דרהמי לחשבון שלשה קאפיזיש בעד דרהם אחד | |style="text-align:right;"|וידיעתו שתקנה ל' קאפיזיש מחטה בעד עשרה דרהמי לחשבון שלשה קאפיזיש בעד דרהם אחד | ||
|- | |- | ||
| | | | ||
| − | + | :I bought sesame for three dirham at one thing for one dirham; it is three things. | |
| + | :<math>\scriptstyle{\color{blue}{3\times x=3x}}</math> | ||
|style="text-align:right;"|וקניתי עם שלשה דרהמי גולגולאן לחשבון דבר אחד בעד דרהם אחד ויהיה שלשה דברים | |style="text-align:right;"|וקניתי עם שלשה דרהמי גולגולאן לחשבון דבר אחד בעד דרהם אחד ויהיה שלשה דברים | ||
|- | |- | ||
| | | | ||
| − | : | + | :The three things for three dirham are worth one thing. |
| + | :<math>\scriptstyle{\color{blue}{\frac{3x}{3}=x}}</math> | ||
|style="text-align:right;"|ושלשת הדברים לחשבון שלשה דרהמי ויעלה שוויו דבר | |style="text-align:right;"|ושלשת הדברים לחשבון שלשה דרהמי ויעלה שוויו דבר | ||
|- | |- | ||
| | | | ||
| − | :the value of the wheat sold at the price of the sesame: <math>\scriptstyle\left(10+3+4\right)-x=17-x</math> | + | :Seventeen minus a thing remain from fund and the profit, and this is the the value of the wheat sold at the price of the sesame: The [amount] of wheat is 30 qafiz at a price of one thing for one dirham; so it is 17 dirham minus a thing. |
| + | :<math>\scriptstyle{\color{blue}{\left(10+3+4\right)-x=17-x}}</math> | ||
|style="text-align:right;"|וישאר מהקרן ומהריוח שבעה עשר ‫<ref>113v</ref>פחות דבר והוא שווי החטה כאשר מכרוהו בכלל הגולגולאן <s>ויהיה אותו מהחטה</s> ותהיה החטה והיא ל' קאפיזיש לחשבון דבר אחד בעד דרהם אחד יהיה י"ז דרהמי פחות דבר | |style="text-align:right;"|וישאר מהקרן ומהריוח שבעה עשר ‫<ref>113v</ref>פחות דבר והוא שווי החטה כאשר מכרוהו בכלל הגולגולאן <s>ויהיה אותו מהחטה</s> ותהיה החטה והיא ל' קאפיזיש לחשבון דבר אחד בעד דרהם אחד יהיה י"ז דרהמי פחות דבר | ||
|- | |- | ||
| | | | ||
| − | :the amount of the wheat sold at the price of the sesame <math>\scriptstyle{\color{blue}{x\sdot\left(17-x\right)=17x-x^2=30}}</math> | + | :Multiply one thing by 17 dirham minus a thing; it is 17 things minus a square equals thirty. [= the amount of the wheat sold at the price of the sesame] |
| + | :<math>\scriptstyle{\color{blue}{x\sdot\left(17-x\right)=17x-x^2=30}}</math> | ||
|style="text-align:right;"|תכה דבר אחד בי"ז דרהמי פחות דבר ויהיה י"ז דברים פחות אלגו ישוה שלשים | |style="text-align:right;"|תכה דבר אחד בי"ז דרהמי פחות דבר ויהיה י"ז דברים פחות אלגו ישוה שלשים | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{x_1=15}}</math> | + | :*<span style="color:Green>Confrontation:</span> |
| − | + | | | |
| + | |- | ||
| + | | | ||
| + | :Confront them; the thing is 15 [<math>\scriptstyle{\color{blue}{x_1=15}}</math>], if you want, or two [<math>\scriptstyle{\color{blue}{x_2=2}}</math>], if you want. Say: the total amount of sesame is 15 qafiz, if you want, or two [qafiz], if you want. | ||
|style="text-align:right;"|וכוין עמו ויהיה הדבר אם תרצה ט"ו ואם תרצה שנים<br> | |style="text-align:right;"|וכוין עמו ויהיה הדבר אם תרצה ט"ו ואם תרצה שנים<br> | ||
ואמור הכלל מהגולגולאן אם תרצה ט"ו קאפיזיש ואם תרצה שנים | ואמור הכלל מהגולגולאן אם תרצה ט"ו קאפיזיש ואם תרצה שנים | ||
| Line 4,951: | Line 5,493: | ||
|- | |- | ||
| | | | ||
| − | :<span style="color:Green>Confrontation:</span> Confront; the thing is three and its the price of [one] qafiz. | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Confront; the thing is three and its the price of [one] qafiz. | ||
:<math>\scriptstyle{\color{blue}{x_1=3}}</math> | :<math>\scriptstyle{\color{blue}{x_1=3}}</math> | ||
|style="text-align:right;"|ותכוין ויהיה הדבר שלשה והוא שווי הקאפיז | |style="text-align:right;"|ותכוין ויהיה הדבר שלשה והוא שווי הקאפיז | ||
| Line 4,996: | Line 5,542: | ||
:Multiply 14 minus a thing by itself; it becomes 196 dirham and a square minus 28 things equal half a square plus half a thing. | :Multiply 14 minus a thing by itself; it becomes 196 dirham and a square minus 28 things equal half a square plus half a thing. | ||
:<math>\scriptstyle{\color{blue}{\left(14-x\right)^2=196+x^2-28x=\frac{1}{2}x^2+\frac{1}{2}x}}</math> | :<math>\scriptstyle{\color{blue}{\left(14-x\right)^2=196+x^2-28x=\frac{1}{2}x^2+\frac{1}{2}x}}</math> | ||
| − | |style="text-align:right;"|תכה י"ד פחות דבר בעצמו יהיה<ref>יהיה: MS | + | |style="text-align:right;"|תכה י"ד פחות דבר בעצמו יהיה<ref>יהיה: MS Mu ויעלה</ref> קצ"ו דרהמי' ואלגו פחות כ"ח דברים ישוה חצי אלגו וחצי דבר |
|- | |- | ||
| | | | ||
| − | :<span style="color:Green>Confrontation:</span> Confront them; the thing is eight and its is [the number of] the qafiz. | + | :*<span style="color:Green>Confrontation:</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Confront them; the thing is eight and its is [the number of] the qafiz. | ||
:<math>\scriptstyle{\color{blue}{x=8}}</math> | :<math>\scriptstyle{\color{blue}{x=8}}</math> | ||
|style="text-align:right;"|ותכוין בהם ויהיה הדבר שמנה והם הקאפיזיש | |style="text-align:right;"|ותכוין בהם ויהיה הדבר שמנה והם הקאפיזיש | ||
| Line 5,005: | Line 5,555: | ||
|} | |} | ||
| − | == | + | == Apparatus == |
| + | |||
| + | <div class="mw-collapsible mw-collapsed"><div class="mw-collapsible-content"> | ||
<references /> | <references /> | ||
| + | </div></div> | ||
== Appendix: Bibliography == | == Appendix: Bibliography == | ||
Latest revision as of 19:40, 1 May 2023
ContentsPrologue |
|
| In the name of God | [1]בשם השם |
| Abū Kāmil said: after we have completed inferring the summary of much of them | אמר אבו כאמל אחר שהשלמנו להוציא מה שהיה קצור מהרבה מהם |
| And commenting on words of the geometricians of our time and the ancients regarding to: | ונעיר על זה המהנדסים אשר בזמננו זה ומאשר חברו לנו דבריהם מהקודמים |
|
מהקוים המחתכים המחומש והמעושר אשר תקיף לכל אחד מהם עגולה ידועה או שתקיף לשניהם עגולה ידועה |
|
והוצאת קטר העגולה המקפת במחומש ובמעושר הידועים או כשיקיפו בעגולה מחומש ומעושר ידועים |
|
והוצאת שעור קו חלק אחד מחלקי מקיף העגולה הידועה |
|
והוצאת שעור צלעות המחומש והמעושר שוי הצלעות והזויות כאשר היה שעורם ידוע וצלעות המשולשים ידועי השעור כאשר היו במחומש או במעושר שוה הצלעו' והזיות |
| And more, that we explained in our book. | ויותר מזה שבארנו בספרנו זה |
| Now, I shall explain many of the indeterminate problems, that were inferred by the arithmeticians, each by itself. | ועתה אבאר הרבה מהשאלות החרשות נ' האלמות שהוציאום החשבנים כל דבר בעצמו |
| That is to say, they will be solved through many methods, by straight knowledge, in ways that consist of techniques used by the arithmeticians. | רצוני שהוצאתם היה בדרכים רבים וידיעה ישרה ובפנים מורכבים מאופנים נהוגים בין החשבנים |
| Those techniques were deprived of proofs, that should have been done. | והם אופנים משוללים ממופתים היה ראוי לעשות עליהם |
| Many of them are derived from proper speculation and good skill. | ומהם אשר הוצאו עם עיון טוב ומלאכה נאותה והרבה מהם |
| Praised be the Lord, for every praise, for he is informer of every knowledge. | ומשובח יהיה היוצר על כל שבח כי הוא המודיע כל ידיעה |
| I shall further explain much of what was given by the arithmeticians in their books, which they solved by the techniques of restoration and confrontation [algebra], in order that the reader will understand them properly. | ואבאר עוד הרבה מאשר הניחום החשבנים בספריהם ועשו אותם עם האופנים מאלגבר ואלמוקאבלה בעבור שיבינם הקורא הבנה טובה |
Indeterminate Problems |
|
|
א כאשר יאמרו לך אלגו שמחזיק שרש אם תוסיף עליו חמשה יחזיק שרש כמה הוא האלגו |
|
זאת השאלה אלמת |
|
ולה דרכים רבים ונאמר אנחנו שנים מהם ואיך ייעשו להיותם מובנים לך לעשות איזה מהם שתרצה |
|
והדרך האחת היא שתניח האלגו שלך אלגו המחזיק שרש ושרשו הוא דבר |
|
ותוסיף עליו חמשה יותר ויהיה אלגו וחמשה יותר וצריך שיחזיק שרש |
|
וכבר ידעת כי שרשו יותר מדבר בעבור כי האלגו היה שרשו דבר |
|
ותשים שרשו דבר ומספר |
|
יהיה מאותו המספר אלגו כשיוכה בעצמו יהיה פחות מהחמשה הנוספים |
|
ונשימיהו דבר ודרהם אחד |
|
ותכה זה בעצמו ויהיה אלגו ודרהם אחד ושני דברים ישוה אלגו וחמשה דרהמי |
|
[2]ותגרע אלגו ודרהם אחד מאלגו וחמשה דרהמי וישאר שני דברים ישוו ארבעה דרהמי |
|
והדבר שנים והם שרש האלגו |
|
והאלגו ארבעה דרהמי |
|
וכשתוסיף עליו חמשה יהיה תשעה דרהמי ושרשו שלשה |
|
ואם תניח שרשו דבר ושני דרהמי |
|
ותכהו בעצמו יהיה אלגו וארבעה דרהמי וארבעה דברים ישוו אלגו וחמשה דרהמי |
|
תגרע |
|
והדבר ישוה רביע דרהם והוא שרש האלגו |
|
והאלגו חצי שמינית מדרהם |
|
וכשתוסיף עליו חמשה דרהמי יהיה חמשה וחצי שמינית ושרשו שני דרהמי ורביע |
|
ואם תרצה תניח שרש האלגו דבר וחצי דרהם |
|
או דבר ודרהם אחד ושני שלישים |
|
או איזה מספר שתרצה אחר שיהיה המספר שתוסיף על הדבר כאשר הוכה בעצמו יהיה פחות מחמשה דרהמי |
|
וכן כל אשר תשאל מזה ייעשה כמעשה הזה |
|
ב וכאשר יאמרו לך אלגו המחזיק שרש אם תגרע ממנו עשרה דרהמי יחזיק מה שישאר שרש |
|
זאת השאלה אלמת |
|
וכבר ידעת כי שרש מאלגו דבר |
|
ושרש מאלגו פחות עשרה דרהמי הוא פחות מדבר |
|
ותניחהו דבר פחות דרהם אחד |
|
או פחות שני דרהמי |
|
או פחות אלגו |
|
תוסיף מהמספר והשברים או מהשברים |
|
ונניחהו דבר פחות דרהם אחד |
|
ותכה אותו בעצמו ויהיה אלגו ודרהם אחד פחות שני דברים |
|
ויהיה אלגו וי"א דרהמי פחות שני דברים ישוה אלגו |
|
|
|
איאימיינטה השני דברים ותוסיפם על האלגו ותגרע אלגו מאלגו וישאר י"א דרהמי ישוו שני דברים |
|
והדבר חמשה וחצי והוא שרש האלגו |
|
והאלגו שלשים ורביע |
|
וכאשר תגרע מהם עשרה דרהמי ישאר עשרים ורביע |
|
ושרשו ארבעה וחצי |
|
ואם תניח שרש האלגו שלך פחות עשרה דרהמי דבר פחות שני דרהמי |
|
|
|
ותכה אותו בעצמו ותכונהו עם המחובר מהכאת אלגו פחות עשרה דרהמי |
|
יהיה שרש האלגו שלשה וחצי |
|
והאלגו י"ב ורביע |
|
ואם תגרע מהם עשרה ישאר שנים ורביע |
|
ושרשו אחד וחצי |
|
ואם תניח המספר הנמשך לדבר כאשר תכה אותו בעצמו יתקבץ יותר מהדרהמי הנמשכים אל האלגו אחד |
|
תדע כי שרש האלגו [3]פחות אותו המספר יהיה אז אותו המספר מהדבר פחות אותו הדבר |
|
בעבור כי שרש האלגו פחות עשרה יהיה אותו המספר הנמשך לדבר פחות דבר |
|
וכן הכאת המספר מהדבר פחות מהמספר בעצמו כמו הכאת הדבר פחות אותו המספר בעצמו |
|
ג ואם יאמרו לך אלגו המחזיק שרש אם תוסיף עליו שרשו שלשה פעמים יחזיק שרש כמה יהיה האלגו |
|
זאת השאלה אלמת |
|
תניח שרש האלגו ושלשה דברים דבר ודרהם אחד |
|
או דבר וחצי דרהם |
|
או דבר ואדרהם אחד על האלגו והוא בזאת השאלה שלשה דברים |
|
ונניחהו דבר ודרהם אחד |
|
ותכה אותו בעצמו יהיה אלגו ודרהם אחד ושני דברים ישוה אלגו ושלשה דברים |
|
ותגרע אלגו ושני דברים מאלגו ושלשה דברים ישאר דבר אחד ישוה דרהם אחד והוא שרש האלגו |
|
| |
|
והאלגו דרהם אחד |
|
ואם תוסיף עליו שלשה פעמי' שרשו יהיה ארבעה דרהמי |
|
ושרשו שנים |
|
ד ואם יאמרו לך אלגו מחזיק שרש כאשר גרענו ממנו שרשו ששה פעמים יחזיק שרש |
|
זאת השאלה אלמת |
|
וידיעתה הוא שנניח האלגו אלגו |
|
ונגרע ממנו שרשו ששה פעמים וישאר אלגו פחות ששה שרשיו |
|
ושרשו[4] פחות מהדבר |
|
אם כן תניחהו דבר פחות ארבעה דרהמי |
|
או פחות חמשה |
|
או פחות שלשה וחצי |
|
או פחות מהמספר שתרצה אחר |
|
ונניחהו דבר פחות ארבעה דרהמי |
|
ונכה אותם בעצמם ויהיה אלגו וי"ו דרהמי פחות שמנה דברי' ישוה אלגו פחות ששה דברים |
|
|
|
ותנכחהו עמו ויהיה הדבר שמנה |
|
והאלגו |
|
תגרע מהם שרשו ששה פעמים והוא מ"ח ישארו י"ו |
|
ושרשו ארבעה |
|
ה ואם יאמרו לך אלגו המחזיק שרש אם תוסיף עליו עשרה פעמים שרשו ועשרה דרהמי יחזיק שרש |
|
זאת השאלה אלמת |
|
וידיעתה שתניח האלגו שלך אלגו |
|
ותוסיף עליו שרשו עשרה פעמי' ועשרי' דרהמי אחד |
|
יהיה שרש זה דבר ומספר |
|
שכאשר הוכה בעצמו יהיה המתקבץ ולא יעבור שיהיה פחות משניהם רצוני מהעשרה ומהעשרים ולא יותר |
|
| |
|
בעבור כי כאשר תכונוהו עם מה שיתקבץ מההכאה האלגו והעשרה דברים והעשרים דרהמי ישאר דבר ודרהם אחד ישוה הדבר |
|
אחר תניחהו דבר וארבעה דרהמי וחצי |
|
ותכה זה בעצמו ויהיה אלגו ותשעה דברים ועשרים דרהמי ורביע ישוה אלגו ועשרה דברים ועשרים דרהמי |
|
|
|
ותכונם עמו ויהיה הדבר הוא רביע דרהם והוא שרש האלגו |
|
והאלגו חצי שמינית |
|
ואם תוסיף עליו שרשו עשרה פעמים ועשרים דרהמי יהיה עשרים ושנים וחצי וחצי שמינית |
|
ושרש זה ארבעה דרהמי וחצי ורביע |
|
ו ואם יאמרו לך אלגו המחזיק שרש אם תגרע ממנו שרשו שמנה פעמי' ושלשים דרהמי יחזיק מה שישאר שרש |
|
זאת השאלה אלמת |
|
וידיעתה שתניח האלגו שלך אלגו |
|
תגרע ממנו שרשו שמנה פעמים ושלשים דרהמי וישאר אלגו פחות שמנה דברים ופחות שלשים דרהמי |
|
ושרשו פחות מדבר |
|
וננחיהו דבר פחות חמשה דרהמי |
|
או פחות ששה |
|
או פחות מהמספר שתרצה שיהיה יותר מחצי השמנה דברים הנשנים באלגו |
|
ואם תרצה תניח שרשו מספר פחות דבר ויהיה המספר יותר מחצי הדברים הנשנים באלגו |
|
ונניחהו דבר פחות חמשה דרהמי |
|
ותכה אותו בעצמו ויהיה אלגו וכ"ה דרהמיש פחות עשרה דברים ישוה אלגו פחות שמנה דברים ושלשים דרהמי |
|
|
|
ותכונהו עמו ויהיה הדבר ישוה כ"ו וחצי והוא שרש האלגו |
|
והאלגו תשנ"ו דרהמי ורביע |
|
ותסיר ממנו שרשו שמנה פעמי' ושלשים דרהמי וישאר תק"ו ורביע |
|
ושרשו כ"ו וחצי |
|
ז ואם יאמרו לך אלגו שיחזיק שרש אם תוסיף עליו שרשו יחזיק שרש ואם תוסיף עליו אלגו שני שרשיו יחזיק שרש |
|
זאת השאלה אלמת |
|
ותעשה אותה כמעשה |
Look for a number that has a root [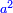 ], such that when you add to it another number, it has a root [ ], such that when you add to it another number, it has a root [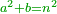 ]; and when you add to it its two roots, it has a root [ ]; and when you add to it its two roots, it has a root [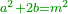 ]. ].
|
שתחפש מספר שיחזיק שרש ואם תוסיף עליו מספר אחר יחזיק שרש ואם תוסיף עליו שני שרשיו יחזיק שרש |
| When you find this number, divide it by the added number and the result is the root of the square.
|
וכאשר מצאת אותו המספר תחלקהו על המספר הנוסף והעולה יהיה שרש האלגו |
|
והמספר המחזיק שרש אחד [7]והמספר הנוסף כ"ד ומ"ח |
|
וכבר ידעת כי בהוסיפך על אחד מ"ח יהיה מ"ט ושרשו שבעה |
|
ואם תוסיף כ"ד על האחד יהיה כ"ה ושרשו חמשה |
|
ואם בקשת לדעת כמה היה האלגו תחלק האחד על כ"ד ויעלה שלישית משמינית והוא שרש האלגו |
|
והאלגו חלק אחד מתקע"ו חלקים באחד |
|
ואם תוסיף עליו שרשו והוא שלישית משמינית וחלק אחד מתקע"ו יחזיק שרש ושרשו חמשה חלקים מכ"ד |
|
ואם תוסיף באלגו שני שרשיו והוא חצי ששית וחלק אחד מתקע"ו ומחזיק שרש ושרשו שבעה חלקים מכ"ד |
|
והאלגו ששית משמינית |
|
ואם תרצה תניח המספר המושג או המחופש שמחזיק שרש מ"ט והחלק הנוסף ק"כ ור"מ |
|
ואתה יודע כי כשתוסיף על מ"ט ק"כ יהיה קס"ט ושרשו י"ג |
|
ואם תוסיף עליו ר"מ יהיה רפ"ט ושרשו י"ז |
|
וכאשר תרצה לדעת שעור האלגו תחלק מ"ט על ק"כ ויעלה לחלק חומש אחד ושמינית אחד וחצי ששית והוא שרש האלגו |
|
והאלגו אלפיים ות"א חלקים מי"ד אלפים ות' באחד |
|
ואם תוסיף עליו שרשו והוא חמשת אלפים ותת"פ מי"ד אלפים ות' יהיה שמנת אלפים ורפ"א מי"ד אלפים ות' ויחזיק שרש ושרשו צ"א חלקים מק"כ |
|
| |
|
ואם תוסיף על האלגו שני שרשיו והוא י"א אלפים ותש"ס מי"ד אלפים ות' יהיה י"ד אלפים ות' ויחזיק שרש ושרשו ק"ט מק"כ |
|
| |
|
ח וכן אם יאמרו לך אלגו אם תוסיף עליו שרשו יחזיק שרש ואם תוסיף עליו שלשה שרשיו יחזיק שרש |
|
ואם תוסיף עליו שלשה פעמים שעור האלגו יחזיק שרש ואמרנו זה בעבור כי משולש השרשים הוא משלשה שרשים |
|
והמספר הנשאל אשר מחזיק שרש אחד והמספר הנוסף עליו שמנה וכ"ד |
|
ותחלק האחד על שמנה יעלה שמינית אחד והוא שרש האלגו |
|
והאלגו שמינית השמינית |
|
ואם תוסיף עליו שרשו והוא שמינית יהיה שמינית ושמינית השמינית ויחזיק שרש ושרשו שלשה שמיניות |
|
ואם תוסיף עליו שלשה שרשים והוא שלשה שמיניות יהיה שלשה שמיניות ושמינית השמינית ויחזיק שרש |
|
ואם תרצה תניח המספר המחזיק |
|
וזה כי כשתוסיף על קס"ט ק"כ יהיה רפ"ט ומחזיק שרש ושרשו הוא שבעה עשר |
|
ואם תוסיף עליו ש"ס יהיה תקכ"ט ומחזיק שרש ושרשו שלשה ועשרים |
|
ואם רצונך לדעת שעור המרובע תחלק קס"ט על ק"כ ויעלה אחד ומ"ט חלקים מק"כ והוא שרש המרובע |
|
והמרובע יהיה אחד שלם וי"ד אלפים וקס"א חלקי' מי"ד אלפים ות' באחד |
|
ואם תוסיף עליו שרשו יעלה שלשה שלמים ותרע"ו אלפים ותתק"כ חלקים מאלף אלפים ותשכ"ח אלפים באחד ומחזיק שרש ושרשו הוא אחד וק"א חלקי' מק"כ באחד |
|
| |
|
ואם תוסיף עליו שלשת שרשיו והם ארבעה דרהמי ועשרים ושבעה חלקים מק"כ באחד יהיה ששה דרהמי ושלשת אלפי' חלקי' מי"ד אלפים ות' ומחזיק שרש ושרשו אחד וצ"ט מק"כ |
|
| |
| There are many solutions for this problem and its similar. | ולזאת השאלה ולדומים אליה דרכים רבים |
| Now, I will explain to you the reason for the extraction of these numbers, so that you will have scales for all these solutions. | ועתה אבאר לך הסבה בהוצאת אלו המספרים למען תהיה לך למאזנים לכל אלו האופנים |
| Know that for any square that has a root, if you add to it its two roots plus one dirham, [the sum] has a root and its root is as the root of the original square plus one.
|
דע כי כל מרובע המחזיק שרש אם תקבץ עמו שני שרשיו ואדרהם אחד יחזיק שרש ושרשו הוא כמו שרש המרובע הראשון עם תוספת אחד |
| And when you wish to find a number that has a root, such that when you add to it another number, [their sum] has a root, and when you add to it twice the number that you added at the first time, or thrice, or four times, or as many times as you wish, the sum has a root. | וכאשר תבקש למצוא מספר מהמספרים שיחזיק שרש וכאשר תוסיף עליו מספר מה אחר יחזיק שרש וכאשר תוסיף עליו שני פעמים המספר שהוספת בראשונה או שלשה פעמים או ארבעה פעמים או כמה שתרצה מהפעמים יהיה לאשר יתקבץ מהם שרש |
|
|
|
|
תניח המרובע שלך מרובע |
|
ותקבץ עמו שנים משרשיו ואדרהם אחד ויהיה העולה יחזיק שרש ושרשו הוא דבר ואדרהם אחד |
|
|
ואם תרצה להוסיף על המרובע כשעור המספר הראשון תקבץ עמו ארבעה שרשים ושנים דרהמי ואם תרצה שלשה פעמים או ארבעה תקח כפי זה |
|
ואם לקחת כשעור המספר יהיה מרובע וארבעה שרשים ושנים דרהמי |
|
|
|
ותכונהו עמהם ויהיה דבר ישוה רביעית אדרהם והוא שרש המרובע |
|
והמרובע הוא חצי שמינית |
|
ונהיה כאלו הנחנו המספר הנוסף עליו שני דברים ודרהם אחד ויהיה אדרהם אחד וחצי בעבור [9]כי הדבר הוא רביע דרהם |
|
והדרהם ששה עשר |
|
ויהיה מפני זה האדרהם וחצי עשרים וארבעה חלקים והוא המספר שכאשר תוסיף אותו על האחד יחזיק שרש |
|
ואם תוסיפהו שני פעמים והוא מ"ח יחזיק גם כן שרש |
| ותשוה אחר זה כאשר בארתי לך | |
| If you want, you can solve this question in another way: | ואם תרצה תעשה לזאת השאלה דרך אחרת |
|
תניח שרש המרובע והארבעה דברים ושני דרהמי דבר ודרהם אחד ושני שלישי דרהם |
|
ותכה זה השרש בעצמו ויהיה מרובע ושני דרהמי ושבע תשיעיות מדרהם ושלשה דברים ושליש דבר ישוו מרובע וארבעה דברים ושני דרהמי |
|
| |
|
|
|
ותכונם יחד ויהיה הדבר דרהם אחד ושתות והוא שרש המרובע |
|
והמרובע יהיה מ"ט חלקים מל"ו חלקים באדרהם והוא החלק הנשאל שיחזיק שרש |
|
ואם רצית לדעת המספר שכאשר תוסיפהו על מ"ט יחזיק שרש ואם תוסיפהו עליו שני פעמים יחזיק שרש |
|
הנה ידעת שהנחת המספר הראשון הנוסף שני שרשים ודרהם אחד וכבר בארנו שהשרש הוא דרהם אחד ושתות והאדרהם ל"ו חלקים ויהיו שני השרשים ואדרהם שלשה ושליש |
|
ותכה שלשה ושליש בל"ו ויהיה ק"כ והוא המספר שכאשר תוסיפהו על מ"ט יחזיק שרש |
|
ואם תוסיף על מ"ט שני פעמים ק"כ והוא ר"מ יחזיק שרש |
|
ותעשה כאשר בארתי |
|
ט וכן אם יאמרו לך מרובע המחזיק שרש אם תגרע ממנו שני שרשים יחזיק הנשאר שרש ואם תגרע מהמרובע שלשה שרשים יחזיק הנשאר שרש |
|
וזאת השאלה חרשתנ' אלמת |
|
ותעשה אותה כמעשה הזה |
|
שתבקש מספר שיחזיק שרש וכאשר תגרע ממנו מספר אחר יהיה הנשאר יחזיק שרש ואם תגרע ממנו אותו המספר וכמו חציו יהיה הנשאר יחזיק שרש |
|
בעבור כי שלשת השרשים כמו שני המספרים וחצי יותר |
| When you find this number, divide it by the subtracted number and the quotient is the root of the square.
|
וכאשר תמצא אותו המספר תחלקהו על חצי המספר הנגרע והעולה לחלק יהיה שרש המרובע |
| You divide it by half the subtracted number, since the subtracted number represents the two roots of the square, and its half represents the root of the square. | וחלקת על חצי המספר הנגרע בעבור כי המספר הנגרע עלה במחשבת המספרים כפי שני שרשי המרובע והחצי עלה כפי שרש המרובע |
|
והמספר הנגרע שיחזיק [10]שרש כ"ה והמספר הנגרע ממנו הוא י"ו והוא צמח ועלה משני שרשים כל שרש שמנה והמספר האחר שהוא שלשה שרשים כ"ד |
|
כאשר תגרע מכ"ה י"ו ישאר תשעה ומחזיק שרש והוא שלשה |
|
וכאשר תגרע מהם כ"ד ישאר אחד ושרשו אחד |
|
וכאשר תרצה לדעת שעור המרובע תחלק כ"ה על שמנה ויעלה לחלק שלשה ושמינית והוא שרש המרובע |
|
והמרובע תשעה וששה שמיניות ושמינית השמינית |
|
ותגרע ממנו שני שרשיו והוא ששה ורביע וישאר שלשה וחצי ושמינית השמינית ומחזיק שרש והוא אחד ושבעה שמיניות |
|
| |
|
ותגרע מהמרובע שלשת שרשיו שהם תשעה ושלשה שמיניות ישאר שלשה שמיניו' ושמינית השמינית |
|
| |
| The extraction of these numbers is already obvious from what we have shown to you. | והוצאת המספרים האלה כבר תהיה נגלית מאשר הראינו לך |
| But, I will further teach you about it, in order that you will remember it properly. | אבל אשכילך עוד עליהם למען יכנס הדבר בלבך היטב |
| Know that for any number that has a root, if you subtract from it its two roots minus one dirham, the remainder has a root and its root is as the root of the original square minus one dirham.
|
דע כי כל מספר המחזיק שרש כאשר תגרע ממנו שני שרשיו פחות דרהם אחד יהיה לנשאר שרש ושרשו יהיה כמו שרש המרובע פחות דרהם אחד |
|
וכאשר תרצה למצוא מספר המחזיק שרש שכאשר תגרע ממנו מספר אחר יהיה לנשאר שרש ואם תגרע עוד ממנו כמו המספר האחר וכמו חציו יהיה לנשאר שרש |
|
תניח המרובע שלך מרובע |
|
ותגרע ממנו שני שרשיו פחות דרהם אחד וישאר מרובע |
|
ותגרע מזה המרובע שני שרשיו פחות דרהם אחד ועוד כמו חציו וישאר מרובע ודרהם וחצי פחות שלשה דברים |
|
|
|
|
|
ותכוין עמהם כמו שבארתי לך ויהיה הדבר שני דרהמי וחצי והוא שרש המרובע |
|
והמרובע ששה ורביע והוא כ"ה חלקים מארבעה בדרהם |
|
ונניח |
|
וכל דרהם ארבעה חלקי' ויהיו שש עשרה |
|
והנה יהיה המספר המבוקש אשר יחזיק שרש כ"ה והמספר הנגרע ממנו י"ו והמספר השני הנגרע כ"ד |
|
ותעשה מהנשאר בשאלה כאשר הראיתיך |
|
והנה תוכל לעשות לזאת השאלה ולדומים אליה דרכים אחרים כרצונך כאשר בארתי לך |
|
[11]י ואם יאמרו לך מרובע המחזיק שרש אם תגרעהו מן שרשו יהיה לנשאר שרש |
|
זאת השאלה חרשת |
|
[ונניח המרובע מרובע |
|
ונגרעהו משרשו][12] והוא דבר וישאר דבר פחות מרובע וצריך שיחזיק שרש |
|
תניח שרשו איזה שתרצה מהדברים אם תרצה יהיה דבר או אם תרצה יהיה יותר או אם תרצה יהיה פחות |
|
ותנחיהו דבר |
|
ותכהו בעצמו ויהיה מרובע ישוה דבר פחות מרובע |
|
|
|
ותכוין עמו כאשר אמרתי ויהיה הדבר ישוה חצי דרהם והוא שרש המרובע |
|
והמרובע רביע דרהם |
|
ואם נניח שרשו שני דברים |
|
יעלה |
|
והמרובע חמישית מחמישית דרהם |
|
ותגרעהו משרשו והוא חמישית דרהם וישאר ארבעה חֳמשים מחמישית דרהם ומחזיק שרש והוא שני חומשים מחמישית דרהם |
|
יא ואם יאמרו לך מרובע המחזיק שרש אם תוסיף עליו שרשו יחזיק שרש ואם תגרע המרובע משרשו יהיה לנשאר שרש |
|
זאת השאלה חרשת |
|
ותעשה אותה כמעשה |
|
שתבקש מספר שיחזיק שרש ואם תקבץ עמו מספר אחר יחזיק שרש ואם תגרע המספר הראשון המחזיק שרש מהמספר האחר הנוסף יחזיק הנשאר שרש |
| When you find this number, divide it by the added number and the quotient is the root of the square.
|
וכאשר תמצא זה המספר תחלקהו על המספר הנוסף והעולה לחלק יהיה שרש המרובע |
|
והמספר המחזיק שרש ארבעה והמספר הנוסף חמשה |
|
וכאשר תוסיף על ארבעה חמשה יהיה תשעה ומחזיק שרש ושרשו שלשה |
|
וכאשר תגרע הארבעה מהחמשה ישאר אחד ומחזיק שרש והוא אחד |
|
וכאשר תרצה לדעת שעור המרובע תחלק ארבעה על חמשה ויעלה לחלק ארבעה חומשים והוא שרש המרובע |
|
והמרובע יהיה שלשה חומשים וחמישית מחמישית |
| I explained to you the procedure of extracting these numbers. | ובארתי לך הנה המלאכה להוציא אלו המספרים |
I have already explained in what preceded up to this point that if we suppose a square that has a root [ ] and if you subtract it from its two roots and one dirham, the remainder is two roots and one dirham minus a square. ] and if you subtract it from its two roots and one dirham, the remainder is two roots and one dirham minus a square.
|
וכבר בארתי במה שעבר עד הנה שאם נניח מרובע שיחזיק שרש ואם תגרעהו משני שרשיו ואדרהם אחד ישאר שני שרשים ואדרהם אחד פחות מרובע |
|
אחר זה נניח שרשו דבר פחות דרהם אחד |
|
ושניתי האדרהם בדבר בעבור שיעלה בהכאה דרהם אחד וכאשר תכוין עמו ישאר מרובע ישוה דבר |
|
אחר זה תכה דבר [13]פחות דרהם אחד בעצמו ויהיה מרובע ואדרהם אחד פחות שני דברים ישוה שני דברים ואדרהם אחד פחות מרובע |
|
|
|
ותכוין עמו ויהיה מרובע ישוה שני דברי' |
|
והדבר שנים |
|
והמרובע ארבעה והוא המספר המחזיק שרש |
|
והמספר האחר הוא ארבעה ואדרהם אחד והוא חמשה |
|
ואם נניח שרש המרובע חצי דבר ואדרהם אחד |
|
|
|
ותכהו בעצמו ותכונהו עם העולה מהכאת שני דברי' ודרהם אחד פחות מרובע |
|
יעלה המספר המחזיק שרש ששה עשר |
|
והמספר האחר חמשה ושני דברים ויהיה שרש המרובע ששה עשר חלקים מס"ה חלקים באחד |
| Whichever of this species that you have, apply the procedure on it in whatever method you choose. | וכל מה שיפול בידך מזה האופן תעשהו במעשה הזה ותקח הדרך אשר תבחר |
| Now I will explain to you this question by understanding another way: | ועתה אבאר לך זאת השאלה בהבנת דרך אחרת |
Know that for every number that is divided into two parts, each of which has a root [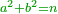 ], and into two other parts that have no root: ], and into two other parts that have no root:
|
דע כי כל מספר שיחלק לשני חלקים באופן שיהיה לכל חלק שרש ולשני חלקים אחרים שלא יהיה להם אטימו |
| I will explain it to you, so that you will understand it: | ועתה אבאר לך זה למען תבינהו |
|
יב אם יאמרו לך חמשה דרהמי תחלקם לשני חלקים ולכל חלק שרש |
|
וכבר ידעת שאחד החלקים הוא אחד והאחר ארבעה |
|
וכאשר תרצה לחלקם לשני חלקים אחרים בלעדי אלו החלקים באופן שיהיה לכל חלק שרש |
|
תקח שרש החלק האחד ותוסיף עליו דבר ותכהו בעצמו והיוצא יהיה החלק האחד |
|
ותגרעהו מחמשה וישאר החלק האחר |
|
ולקחנו החלק האחד והוא אחד |
|
ושרשו אחד |
|
הוספנו עליו דבר ויהיה אחד ודבר אחד |
|
הכינו אותו בעצמו והיה מרובע ודרהם אחד ושני דברים והוא החלק האחד משני החלקים המבוקשים |
|
ותגרעהו מן החמשה וישאר ארבעה פחות מרובע ופחות שני דברים והוא החלק האחר וצריך שיחזיק שרש |
|
וכבר ידענו ששרשו פחות משני דרהמי |
|
ונניחיהו שני דברי' פחות דבר ושני שלישי |
|
והכינום בעצמם ויהיה ארבעה דרהמי ואלגו |
|
| |
|
|
|
ותכוין עמו כאשר ידעת ויהיה הדבר דרהם אחד וחמישית אחד |
|
[14]והנחנו שרש החלק האחד מהשנים דבר ודרהם אחד ויהיה שרש החלק האחד שני דרהמי וחמישית |
|
ותכהו בעצמו ויהיה ארבעה דרהמי |
|
| |
|
והאחר הוא הנשאר מהחמשה והוא ארבעה חומשי החומש |
|
ואם תרצה לחלק אותו לשנים חלקים אחרים זולת אלו החלקים |
|
תניח החלק הקטן שרש מארבעה דרהמי פחות אלגו ופחות שני דברים ושנים דרהמי פחות שני דברים |
|
|
|
ותכם בעצמם ותכונהו עם המתקבץ מהכאת ארבעה דרהמי פחות אלגו ופחות שני דברים |
|
ותעשה כאשר אמרתי לך |
|
ויהיה שרש החלק האחד שנים דרהמי ושלשה חלקים משלשה עשר |
|
ושרש החלק האחר שנים חלקים מי"ג |
|
ותכה כל אחד משניהם בעצמו |
|
ויהיה החלק האחד ארבעה דרהמי וי"ב חלקים מי"ג חלקים באחד ותשעה חלקים מקס"ט |
| Do the same for all cases of this type that you solve in this procedure, and it will have as many parts as you wish. | וכן תעשה לכל אשר יבוא לך מזה המין תעשה עמו כמעשה הזה ויחזיק מהחלקים כמה שתרצה |
Always take the root of one of the two parts, add a thing to it and multiply it by itself [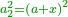 ]; the result is one of the two parts you sought for [ ]; the result is one of the two parts you sought for [ ]. ].
|
והוא שלעולם תקח שרש האחד מהשני חלקים ותוסיף עליו דבר ותכה זה בעצמו והיוצא הוא האחד מהשני חלקים אשר בקשת |
Subtract it from the number you want to divide into two parts, each of which has a root; the remainder is the other part [ ] and this is a number that has a root, from which a square and a root is subtracted [ ] and this is a number that has a root, from which a square and a root is subtracted [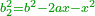 ]. ].
|
ותגרעהו מהמספר אשר תרצה לחלק לשני חלקים אשר לכל חלק מהם שרש והנשאר הוא החלק האחר והוא מספר שיחזיק שרש הנשנה לו אלגו ודבר |
Take the root of the number and subtract from it as many things as you want [ ], since they are not the same, when you multiply them by themselves [ ], since they are not the same, when you multiply them by themselves [ ]. ].
|
ותקח שרש המספר ותשנה לו אשר תרצה מהדברים אחר אשר לא יכוונו כאשר תכם בעצמם |
| Confront it with the part that has a root, which is subtracted from the square and the root; you receive parts endlessly.
|
ותכוין עמו החלק שהוא מספר המחזיק שרש נשנה מהאלגו ודבר ויעלו לך מן החלקים עד אין להם תכלית |
|
יג ואם יאמרו לך עשרה תחלקהו לשני חלקים ותוסיף החלק האחד על עשרים ויהיה מה שיחזיק שרש ותגרע החלק האחר מחמשים ויחזיק הנשאר שרש |
|
זאת השאלה חרשת |
|
ומעשהו שנניח החלק האחד אלגו פחות עשרים |
|
באופן שכאשר תוסיפיהו על עשרים יחזיק שרש והוא אלגו |
|
ושרשו דבר |
|
והחלק האחר יהיה הנשאר מהעשרה והוא שלשים פחות אלגו |
|
ותגרעם מן |
|
[15]וכאשר תבקש לדעתו תניח האלגו |
|
והאופן האחד שתניח שרש האלגו חמשה ושתות |
|
והאלגו יהיה כ"ו ושני שלישים ורביע תשיעית |
|
ותגרע ממנו עשרים דרהמי וישאר ששה ושני שלישים ורביע תשיעית והוא אחד מהשני חלקים |
|
| |
|
וכאשר תוסיפהו על עשרים יחזיק שרש ושרשו חמשה ושתות |
|
והחלק האחר יהיה מה שישאר מהעשרה והוא שלשה דרהמי ורביע וחצי תשיעית |
|
וכאשר תגרעם מחמשים ישאר מ"ו ושני שלישים ורביע תשיעית ומחזיק שרש ושרשו הוא ששה דרהמי וחצי ושליש |
|
וכאשר נרצה לדעת איך נניח שרש המרובע חמשה ושתות |
|
תניח שרש מאלגו ועשרים דרהמי דרהם אחד ושני שלישי ודבר אחד |
|
ותכם בעצמם ויהיה שני דרהמי ושבעה תשיעיות ואלגו ושלשה דברים ושלישית דבר ישוה אלגו ועשרים דרהמי |
|
| |
|
|
|
ותכוין עמו ויהיה הדבר חמשה ושתות |
| If you want a solution other than this, do as I told you before. | ואם תרצה אופן אחר זולת זה תעשה כאשר אמרתי לך קודם זה |
|
יד ואם יאמרו לך תחלק עשרה לשני חלקים ותוסיף החלק האחד על עשרים ויחזיק שרש ותוסיף החלק האחר על חמשים ויחזיק שרש |
|
זאת השאלה חרשת |
|
ומלאכתו שנניח החלק האחד אלגו פחות עשרים דרהמי |
|
באופן שכאשר תוסיפ |
|
והחלק האחר הנשאר מן העשרה יהיה שלשים פחות אלגו |
|
וכשתוסיפם על החמשים יהיו שמנים דרהמי פחות אלגו וצריך שיחזיק שרש |
|
כאלו אמר תחלק שמנים לשני חלקים ולכל חלק שרש |
| When you have problems of this species, solve them by this procedure, so that you get a number that you divide into two parts, each of which has a root, provided that the given number can be divided. | וכאשר יפלו לפניך שאלות מזה המין תעשה כמעשה הזה עד שיעלה לך מספר שתחלקהו לשני חלקים ויחזיק כל חלק שרש אם הניח מספר שיוכל להתחלק |
| Otherwise the purpose cannot be achieved. | ואם לאו השאלה היא לא יתכן שתגיע אל תכלית |
|
ותחלק השמנים לשני חלקים ולכל חלק שרש |
|
ותניח אחד החלקים יותר מעשרי' ופחות משלשים והחלק האחר יותר מחמשים ופחות מששים |
| This problem has countless solutions. | ולזה הדרך אופנים רבים עד אין מספר |
| The procedure of extracting any solution you want is as I told you before. | והמלאכה להוציא איזה אופן שתרצה מהם [16]היא כפי מה שאמרתי לך קודם |
|
ואחד מהאופנים שתשים החלק האחד כ"ו דרהמי ול' חלקים מרפ"ט |
|
והחלק האחר הנשאר משמנים והוא נ"ג ורנ"ט חלקים מרפ"ט חלקים באחד |
|
וכאשר תרצה לדעת שעור כל חלק מן העשרה |
|
תגרע מהחלק ראשון מהשמנים עשרים וישאר ששה דרהמי ושלשים חלקים מרפ"ט והוא החלק ראשון מהעשרה |
|
והחלק האחר הוא הנשאר מעשרה והוא שלשה דרהמי ורנ"ט חלקים מרפ"ט חלקים באחד |
|
וכאשר נוסיף החלק הגדול משני חלקי העשרה על עשרים יהיה עשרים ושש ושלשים חלקים מרפ"ט ומחזיק שרש והוא חמשה דרהמי ושלשה חלקים משבעה עשר |
|
וכאשר נוסיף החלק הקטון מן העשרה על החמשים יהיה חמשים ושלשה דרהמי ורנ"ט חלקים מרפ"ט ומחזיק שרש והוא שבעה דרהמי וחמשה חלקים משבעה עשר |
|
טו ואם יאמרו לך מרובע אם תגרעהו משלשה דרהמי יחזיק הנשאר שרש ואם תוסיפהו על שני דרהמי יהיה למתקבץ שרש |
|
זאת השאלה חרשת |
|
ומעשהו שתקבץ השנים עם השלשה ויהיו חמשה |
|
תחלקם לשני חלקים יחזיק כל חלק שרש |
|
ותניח החלק האחד יותר משנים ופחות משלשה |
|
ותגרע השנים מהחלק האחד ומה שישאר יהיה המרובע |
|
ואם תרצה תניח האלגו עשרים ושלשה חלקים מקס"ט חלקים באחד |
|
ואם תרצה תניחהו קמ"ו מקס"ט |
|
ואם תרצה תניחהו תל"א חלקים מתרכ"ה חלקים באחד |
| You can solve this problem and similar ones, according to the methods you choose, as I explained to you before. | והנה תוכל לעשות זאת השאלה והדומים אליה כפי האופנים אשר תבחר כאשר בארתי לך לפנים |
|
יו ואם יאמרו לך אלגו אם תגרעהו מעשרה דרהמי יחזיק הנשאר שרש ואם תגרעהו מעשרים יחזיק הנשאר שרש |
|
זאת השאלה חרשת |
|
ומעשהו שתניח האלגו שלך עשרה דרהמי פחות אלגו |
|
ואם תרצה עשרים דרהמי פחות אלגו |
|
ותניחהו כן בעבור כי כאשר תגרעהו מאחד מהם ישאר אלגו ומחזיק שרש והוא דבר |
|
ונניחהו עשרה דרהמי פחות אלגו |
|
וכאשר תגרעהו מן עשרה ישאר [17]אלגו |
|
ואם תגרעהו מעשרים ישאר אלגו ועשרה דרהמי וצריך שיחזיק שרש |
|
וכבר ידעת ששרשו יותר מדבר |
|
ונניח שרשו דבר ושני דרהמי |
|
או דבר ושלשה |
|
או אשר תרצה מהמספרים כאשר בארתי לך לפנים |
|
אחר שיהיה כאשר הכינו אותו בעצמו ונכוין עמהם אלגו ועשרה דרהמי יהיה האלגו פחות מעשרה דרהמי |
|
ותניחהו דבר ושני דרהמי |
|
ותכהו בעצמו יהיה אלגו וארבעה דרהמי וארבעה דברים ישוה אלגו ועשרה דרהמי |
|
|
|
ותכוין עמו ויהיה שרש האלגו אדרהם אחד וחצי |
|
והאלגו שנים דרהמי ורביע |
|
והנחנו האלגו עשרה דרהמי פחות אלגו |
|
ויהיה האלגו המבוקש שבעה דרהמי וחצי ורביע |
|
ואם נגרעהו מעשרה ישאר שנים ורביע ושרשו אחד וחצי |
|
ואם הנחנו שרש מאלגו מעשרה דרהמי דבר ושלשה דרהמי |
|
יהיה האלגו תשעה דרהמי וחצי ושליש וחמשה ששיות הששית |
| The solutions you get in this problem and in its similar are more than any number. | והאופנים הם שתניח בזאת השאלה ובדומים אליה יותר מאשר הוא המספר |
|
יז ואם יאמרו לך אלגו אם תוסיפהו על עשרים יחזיק שרש ואם תוסיפיהו על שלשים יחזיק שרש |
|
זאת השאלה חרשת |
|
ומעשהו שנניח האלגו אלגו פחות עשרים דרהמי |
|
או אם תרצה אלגו פחות שלשים |
|
ונניחהו אלגו פחות עשרים דרהמי |
|
ובתוספתו על עשרים |
|
ושרשו דבר |
|
ונוסיפהו על שנים ויהיה אלגו ועשרה דרהמי וצריך שיחזיק שרש |
|
ויהיה האלגו יותר מעשרים |
|
ותניח שרשו דבר ואדרהם אחד' |
|
|
|
ותכם בעצמם ותכונהו עם המתקבץ מהכאת אלגו ועשרה דרהמי |
|
ויהיה המרובע עשרים דרהמי ורביע |
|
ותגרע ממנו עשרי' דרהמי וישאר רביע אחד והוא המרובע המבוקש |
|
ואם תוסיפהו על עשרים יחזיק שרש |
|
ואם תרצה תאמר האלגו ע"ה דרהמי וחצי שמינית |
|
אם תוסיפהו על עשרים יחזיק שרש ושרשו תשעה דרהמי וחצי ורביע |
|
ואם תוסיפהו על שלשים יחזיק שרש והוא עשרה דרהמי ורביע |
| This problem and its similar have many solutions. | ולזאת השאלה ולדומים אליה אופנים רבים |
|
יח ואם יאמרו לך אלגו אם תוסיפהו על עשרה יחזיק שרש ואם תגרעהו [18]מעשרה יחזיק שרש |
| If the two numbers in this problem are the same, and we suppose one of them can be divided into two parts, each of which has a root, then [the problem] has a countless number of solutions. | וכאשר ישתוו השני מספרים בזאת השאלה ונניח אחד מהשני מספרים שיחלקו לשני חלקים לכל חלק שרש אז תחזיק מהאופנים מה שלא יסופרו |
| If it cannot be divided into two parts, each of which has a root, the problem has no solution. | ואם לא יתחלק לשני חלקים שיחזיק כל חלק שרש תהיה השאלה חרשת זולת שום אופן |
|
ותחלק העשרה לשני חלקים ולכל חלק שרש |
|
והוא אחד ותשעה |
|
ותקח שרשי אחד ותשעה ויהיו ארבעה |
|
ותכם בעצמם ויהיה ששה עשר |
|
תגרע מהם העשרה וישאר ששה והוא |
|
והמשפט בזאת השאלה הוא עולה בעבור כי כאשר תגרע האלגו שהוא ששה מעשרה ישאר ארבעה והארבעה הם מהכאת העודף מאחד משני החלקים על שרש החלק האחר אין [שיבוש][19] |
|
יט ואם יאמרו לך אלגו אם תגרעהו משמנה שרשים וק"ט דרהמי יחזיק שרש |
| When you have such a question, multiply half [the number of] the roots by itself and add [the product] to the dirham. | וכאשר יקרה לפניך שאלה כזאת תכה מחצית השרשים בעצמם ותוסיפהו על האדרהמי |
| If their sum can be divided into two parts, each of which has a root, the problem is solvable and has a countless number of solutions. | ואם יתחלק המקובץ מהם לשני חלקים ויחזיק כל חלק שרש תהיה השאלה נשלמת ותחזיק מהאופנים הרבה אשר לא יוכלו להמנות |
| If their sum cannot be divided into two parts, each of which has a root, the problem has no solution and no solving procedure. | ואם לא יתחלק המתקבץ מהם לשני חלקים ולכל חלק מהם שרש תהיה השאלה חרשת בזולת שום אופן |
|
ותכה מחצית השרשים בעצמם ויהיו ששה עשר |
|
ותוסיפם על ק"ט ויהיה קכ"ה |
|
ותחלק קכ"ה לשני חלקים שיחזיק כל חלק שרש |
|
ואם תרצה תניח החלק האחד כ"ה והאחר מאה |
|
ואם תרצה תניח האחד ארבעה והאחר קכ"א |
| If you want, you divide it into two other parts, as I instructed and explained. | ואם תרצה תחלקהו לשני חלקים אחרי' כאשר אמרתי ובארתי |
| Then, take one of the two parts you want and add it to the square.
|
ותשוב ותקח החלק האחד מאיזה משני חלקי' שתרצה ותוסיפהו על המרובע |
|
אחר תקח כ"ה ותוסיפהו על המרובע ויהיה אלגו וכ"ה |
|
ונאמר אלגו וכ"ה דרהמי ישוו שמנה דברים וק"ט דרהמי |
|
ותגרע כ"ה מק"ט וישאר אלגו ישוה שמנה דברים ופ"ד דרהמי |
|
ומחצית הדברים יהיה ארבעה |
|
ת[כם] בעצמם ויהיו ששה עשר |
|
תוסיפם על פ"ד ויהיה מאה [20]והם החלק האחד |
|
| |
| The reason we extract it by this procedure: | והמשפט למה הוצאנוה בזה המעשה |
| We divide it into two parts, each of which has a root, because when we add the first part to the square and confront it with the things and the dirham in our number, then we halve [the number of] the things, multiply it by itself and add [the product] to the number, or subtract the number from it, the sum, or the remainder, is the other part.
|
וחלקנום לשני חלקים לכל חלק שרש בעבור כי כשהוספנו החלק האחד על האלגו והשוינו עמו הדברים והדרהמי אשר במספרנו וחצינו הדברים והכינום בעצמם והוספנוהו על המספר או גרענו המספר ממנו יהיה המתקבץ ממנו או הנשאר הוא החלק האחר |
| What remains from the roots and the dirham after subtracting the square from [them] is the part added to the square.
|
ויהיה מה שישאר מהשרשים והדרהמי אחר גרעון האלגו מהמספר יהיה החלק הנוסף עליו אלגו |
|
ותשוב ותקח שרש המאה והוא עשרה |
|
ותוסיף עליהם מחצית השרשים והוא ארבעה ויהיו ארבעה עשר והוא שרש האלגו |
|
והאלגו קצ"ו |
|
ותגרעהו משמנה שרשיו וק"ט דרהמי והם רכ"א וישאר כ"ה ושרשו חמשה |
|
ואם לקחנו החלק האחד והוא מאה דרהמי והוספנום על האלגו |
|
|
|
ונכוין [המתקבץ][21] עם שמנה שרשיו וק"ט דרהמי |
|
יהיה האלגו פ"א |
|
ואם לקחנו החלק האחר והוא ארבעה והוספנוהו על האלגו |
|
|
|
ונכוין עמו שמנה שרשיו וק"ט |
|
יהיה המרובע רכ"ה |
|
ואם תרצה ל"ו ואם תרצה ארבעה |
| The solutions of this problem are numerous. | והאופנים לזאת השאלה הם רבים |
|
כ ואם יאמרו לך אלגו אם תוסיף עליו שמנה שרשיו יחזיק שרש ואם תגרעהו משני שרשיו יהיה לנשאר שרש |
|
זאת השאלה חרשת |
|
וחכמתה שנניח השמנה שרשים הנוספים באלגו ששה עשר דברים וס"ד דרהמי |
|
באופן שיהיה למתקבץ שרש ושרשו דבר ושמנה דרהמי |
| If we assume other than this it is possible, provided that [the sum] has a root. | ואם הנחנו אחר זולת זה יעבר אחר זה שיהיה שיחזיק שרש |
|
וכאשר הנחנו השמנה שרשים י"ו דברים וס"ד דרהמי הנה אז תדע כי כל שרש שני דברים ושמנה דרהמי והשני שרשי' ארבעה דברים וי"ו דרהמי |
|
והוא כמו שיאמר אלגו אם תגרעהו מארבעה שרשיו וששה עשר יחזיק מה שישאר שרש |
|
ותעשה כאשר אמרתי |
|
ויהיה האלגו שלשים ושש והוא האלגו |
|
ותשוב ותאמר כבר הנחנו שרש האלגו שני דברים ושמנה דרהמי ועשרים דברים |
|
ותחלק ל"ו על עשרים יעלה דרהם אחד וארבעה חומשי' והוא שרש האלגו המבוקש |
|
והאלגו שלשה דרהמי [וחמ]שית וחומש החמישית |
|
[22]ואם תוסיף עליהם שמנה שרשיו והוא ארבעה עשר דרהמי ושני חומשים יהיה שבעה עשר ושלשה חומשים וחומש החמישית ושרשו ארבעה וחומש |
|
| |
|
ואם תגרע מהאלגו שהוא שלשה דרהמי וחמישית וחומש החמישית משני שרשיו שהם שלשה דרהמי ושלשה חומשים ישאר חומש אחד וארבעה חומשים מחמישית ושרשו שלשה חומשים |
|
| |
|
ואם תעשה זה באופן החלק האחר יהיה האלגו דרהם אחד |
| You can solve this problem in many ways. | והיטב תוכל לעשות לזאת השאלה באופנים רבים |
|
כא ואם יאמרו לך אלגו אם תגרעהו משני שרשיו ומ"ט דרהמי יחזיק הנשאר שרש |
|
אם תרצה תעשה כאשר אמרתי |
|
ואם תרצה תכה שני השרשים בעצמם והיוצא יהיה האלגו |
|
והסבה |
| If the number have no root, return to the first procedure I explained to you before this question. | ואם לא יחזיק המספר שרש תשוב למעשה הראשון שבארתי לך קודם זאת השאלה |
| Since this procedure is possible only for a number that has a root, and it involves many solutions, not one. | כי זה המעשה לא יתכן כי אם במספר שיחזיק שרש ולא יחזיק אופן אחד כי אם רבים |
| The first solving procedure of this problem and its similar has many solutions. | והמעשה הראשון לזאת השאלה ולדומים אליה יהיה באופנים רבים |
|
כב ואם יאמרו לך אלגו אם תוסיף עליו שרשו יהיה למתקבץ שרש ואם תגרע ממנו שרשו יחזיק הנשאר שרש |
|
זאת השאלה חרשת |
|
ותעשה אותה כמעשה |
|
שתבקש מספר שיחזיק שרש וכאשר תקבץ עמו מספר אחר יחזיק שרש ואם תגרע ממנו אותו המספר יחזיק שרש |
|
| |
| When you find this number, divide it by the added number and the quotient is the root of the square.
|
וכאשר מצאת אותו המספר [תחלק][23] אותו על המספר הנוסף ומה שיעלה לחלק יהיה שרש המרובע |
|
והמספר המחזיק שרש הוא כ"ה והמספר הנוסף עליו כ"ד והמספר הנגרע כ"ד |
|
וכבר ידעת כי כאשר תוסיף כ"ד על כ"ה יהיה מ"ט ושרשו |
|
וכאשר תגרע כ"ד מכ"ה ישאר אחד ושרשו אחד |
|
ואם תבקש לדעת שעור האלגו תחלק כ"ה על כ"ד ויהיה אחד ושלישית השמינית והוא שרש האלגו |
|
והאלגו אחד ושני שלישים וחלק אחד מתקע"ו באחד |
|
ואם תוסיף עליו שרשו והוא אחד ורביע ושתות יהיה שנים דרהמי ושמינית אחד וחלק אחד מתקע"ו ומחזיק שרש והוא אחד ושליש ושמינית |
|
| |
|
[24]ואם תגרע ממנו שרשו והוא אחד ושליש ושמינית ישאר כ"ה חלקים מתקע"ו חלקים באחד ומחזיק שרש והוא שמינית אחד וחצי ששית |
|
| |
|
ואם תרצה תניח המספר המבוקש המחזיק שרש קס"ט והמספר הנוסף ק"כ והמספר הנגרע ממנו ק"כ |
|
ואתה יודע כי כשתוסיף על קס"ט ק"כ יהיה למתקבץ שרש והוא שבעה עשר |
|
וכאשר תגרע מהם ק"כ יהיה לנשאר שרש והוא שבעה |
|
וכאשר תרצה לדעת שעור האלגו תחלק קס"ט על ק"כ ויעלה לחלק אחד וחמישית אחד ושמינית אחד וחצי שתות והוא שרש האלגו |
|
והאלגו אחד וי"ד אלפים וקס"א מי"ד אלפים ות' באחד |
|
ואם תוסיף באלגו שרשו שהוא אחד וחמישית ושמינית וחצי שמינית ושתות אחד יהיה שלשה דרהמי וחמשת אלפים ותרמ"א חלקים מי"ד אלפים ות' חלקים באחד ומחזיק שרש והוא אחד ועשרה חלקים מק"כ |
|
| |
|
ואם תגרע ממנו שרשו שהוא אחד וחמישית ושמינית וחצי שתות ישארו שמנת אלפים ורפ"א מי"ד אלפים ות' ומחזיק שרש והוא צ"א חלקים מק"כ |
|
| |
| This problem and its similar have many solutions. | ולזאת השאלה ולדומים אליה אופנים רבים |
| I have already explained to you the procedure of extracting these numbers or whichever of them you want, because there are as many solutions as you wish. | וכבר בארתי לך המלאכה בהוצאת אלו המספרים או אשר תרצה מהם כי הם מחזיקים אופנים רבים כרצונך |
| I will further teach you the extraction of these numbers, so that your heart will be wiser about them: | ואני אשכילך עוד בהוצאת |
| I have already shown you that for every square that has a root, when you add its two roots and one dirham to it, it has a root.
|
כבר הודעתיך שכל אלגו שיחזיק שרש כאשר תוסיף עליו שני שרשיו ודרהם אחד יחזיק שרש |
| Then, subtract from the square its two roots and one dirham.
|
אחר זה תגרע מהאלגו שני שרשיו ודרהם אחד |
Say: its two roots and one dirham [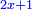 ] is the required number. ] is the required number.
|
ותאמר שני שרשיו ודרהם אחד יהיה המספר המבוקש |
| The remainder is a square minus two roots and one dirham, so you already know that its root is less than a thing.
|
וישאר אלגו פחות שני שרשים ודרהם אחד וכבר ידעת ששרשו פחות מדבר |
|
ונניחהו דבר פחות שני דרהמי |
|
ותכהו בעצמו ויהיה אלגו וארבעה דרהמי פחות ארבעה דברים ישוה אלגו פחות שני שרשים ודרהם אחד |
|
|
|
ותכונהו עמו ויהיה הדבר ישוה שני דרהמי וחצי והוא שרש האלגו |
|
והאלגו ששה ורביע |
|
ונניח המספר הנוסף עליו שני שרשים ודרהם אחד והוא ששה בעבור כי השרש הוא שנים וחצי |
|
ויהיה המספר המבוקש ששה ורביע והמספר הנוסף ששה והמספר הנגרע ששה |
|
תשיבם לרביעיות ויהיה המספר המבוקש כ"ה והמספר הנוסף [25]כ"ד והמספר הנגרע כ"ד |
|
ואם נניח שרש האלגו פחות שני דברים ודרהם אחד דבר פחות דרהם וחצי |
|
תכהו בעצמו ויהיה אלגו ושני דרהמי ורביע פחות שלשה דברים ישוה אלגו פחות דבר ודרהם אחד |
|
|
|
ותכונהו עמו ויהיה הדבר ישוה שלשה דרהמי ורביע |
|
ותכנהו עמו ויהיה הדבר שלשה עשר והוא שרש האלגו המבוקש |
|
שיחזיק שרש והוא קס"ט כל ששה עשר ממנו אחד |
|
והמספר הנוסף עליו הוא שני שרשים ודרהם אחד והוא ששה וחצי בעבור כי השרש שלשה דרהמי ורביע כל דרהם ששה עשר חלקים ויהיה זה ק"כ חלקים |
| In what follows, proceed as I have shown you. | ותעשה בנמשך כאשר הראיתי לך |
|
כג ואם יאמרו לך אלגו אם תוסיף עליו שני שרשיו יחזיק שרש ואם תגרע ממנו שלשה שרשיו יחזיק שרש |
|
וצריך שתבקש מספר שיחזיק שרש וכאשר תוסיף עליו מספר מה אחר יחזיק שרש וכאשר תגרע ממנו כמו המספר הנוסף וכמו חציו יחזיק הנשאר שרש |
|
| |
|
בעבור כי שלשת השרשים הם שני השרשים וכמו חציים |
| When you find that number, divide it by half the additional number, or by a third of the subtracted number, and the quotient is the root of the square.
|
וכאשר מצאת אותו המספר תחלקהו על חצי המספר הנוסף או על שלישית המספר הנגרע והעולה לחלק יהיה שרש המרובע |
|
והמספר המחזיק שרש קכ"א והמספר הנוסף עליו מ"ח והמספר הנגרע ע"ב |
|
וכאשר תרצה לדעת שעור המספר תחלק קכ"א אם תרצה על |
|
והאלגו כ"ה ורביעית הששית וחלק אחד מתקע"ו באחד |
|
ואם תוסיף עליו שני שרשיו שהם עשרה דרהמי וחצי ששית ויהיה ל"ה דרהמי ושלשה רביעי ששית וחלק אחד מתקע"ו ומחזיק שרש והוא חמשה דרהמי וחצי ושלישית השמינית |
|
| |
|
ואם תגרע מהאלגו שלשת שרשיו שהם ט"ו ושמינית אחד ישאר עשרה ושתות ושמינית וחלק אחד מתקע"ו ומחזיק שרש והוא שלשה ושמינית וחצי ששית |
|
| |
|
ואם תרצה תניח המספר המבוקש |
|
ותחלק מ"ט על ששה עשר ויעלה לחלק שלשה וחצי שמינית והוא שרש האלגו |
|
[26]והאלגו תשעה ושלשה שמיניות וחלק אחד מרנ"ו |
|
ואם תרצה תניח המספר המחזיק שרש תרכ"ה והמספר הנוסף של"ו והמספר הנגרע תק"ד |
|
ותחלק תרכ"ה על מחצית של"ו או על שלישית תק"ד והוא קס"ח ויעלה לחלק שלשה ושני שלישים ורביע השביעית ושמינית השביעית והוא שרש האלגו |
|
| |
|
והאלגו שלשה עשר דרהמי וקמ"א חלקים מקפ"ט וכ"ה חלקים מכ"ח אלפים ורכ"ד |
|
ואם תוסיף עליו שני שרשיו והם שבעה דרהמי וצ"ד חלקים מקפ"ט יהיה כ"א דרהמי ומ"ז |
|
| |
|
ואם תגרע מהאלגו שלשת שרשיו ישאר שני אדרהמי וקי"ד חלקים מקס"ח וכ"ה חלקים מכ"ח אלפים ורכ"ד חלקים מאחד ומחזיק שרש והוא דרהם אחד |
|
| |
| The reason for the extraction of these numbers is clear from what we explained above. | וסבת הוצאת אלו המספרים תהיה מבוארת ממה שבארנו קודם |
|
וכבר הראיתי לך שכל אלגו שיחזיק שרש והוא דבר ודרהם אחד |
|
|
|
| |
|
ותגרע מהאלגו שלשת שרשיו ודרהם וחצי וישאר אלגו פחות שלשה שרשים ודרהם אחד |
|
ותניח שרשו שני דברים פחות שני דרהמי |
|
ותכם בעצמם ויהיה אלגו וארבעה דרהמי פחות ארבעה דברים ישוה אלגו פחות שלשה דברים ודרהם אחד וחצי |
|
| |
|
|
|
ותכונם עמהם ויהיה הדבר חמשה וחצי |
|
והאלגו שלשים ורביע |
|
ונניח המספר הנוסף עליו שני דברים ודרהם אחד |
|
ונניח המספר הנגרע ממנו שלשה דברים ודרהם וחצי והוא שמנה עשר |
|
השיבם רביעיות ויהיה המספר המבוקש שיחזיק שרש קכ"א והמספר הנוסף מ"ח והנגרע ע"ב |
| Proceed the procedure as I instructed you. | ותעשה בפעולתם כמו שאמרתי לך |
|
כד ואם יאמרו לך אלגו שיחזיק שרש אם תגרעהו מעשרת שרשיו פחות שמנה |
| When you have such a question, multiply half [the number of] the roots by itself and subtract the dirham from the product. | כאשר יקרה לך שאלות ככה תכה מחצית השרשים בעצמם והמתקבץ תגרע ממנו האדרהמי |
| If the remainder can be divided into two parts, each of which has a root, the problem has as many solutions as you wish. | [27]ומה שישאר אם יתחלק לשני חלקים שיחזיק כל חלק שרש הנה תחזיק השאלה רבים מהאופנים כמה שתרצה |
| If the remainder cannot be divided into two parts, each of which has a root, the problem has no solution and no solving procedure. | ואם לא יתחלק לשני חלקים שיחזיק כל חלק שרש הנה לא יהיה לשאלה אופן ולא מלאכה |
| If, when you multiply half [the number of] the roots by itself, the result is the same as the number of the dirham, or less, the problem is incorrect. | ואם כאשר תכה מחצית השרשים בעצמו יצא כמו שעור הדרהמי או פחות מהם לא היתה השאלה נכונה |
|
ונכה מחצית השרשים בעצמם בזאת השאלה והוא חמשה ויהיה עשרים וחמשה |
|
תגרע מהם שמנה הדרהמי וישאר שבעה עשר |
|
חלקם לשני חלקים ולכל חלק שרש והם ששה עשר ואחד |
|
| |
|
ואם תרצה תאמר אלגו וששה עשר דרהמי ישוה עשרה שרשים פחות שמנה דרהמי |
|
ואם תרצה תאמר אלגו ודרהם אחד ישוה עשרה שרשים פחות שמנה |
|
והוא כאלו תאמר אלגו וששה עשר דרהמי ישוה עשרה שרשים פחות שמנה דרהמי |
|
ויהיה האלגו אם תרצה ל"ו ואם תרצה יהיה שמנה עשר |
|
כה ואם יאמרו לך אלגו ודרהם אחד ישוו עשרה שרשים פחות שמנה דרהמי |
|
יהיה האלגו אם תרצה אחד ואם תרצה יהיה שמנים ואחד |
|
כו ואם יאמרו לך אלגו המחזיק שרש אם תגרעהו מר"ס פחות ששה שרשים יחזיק הנשאר שרש |
| When you have such a question, multiply half [the number of] the roots by itself and add it to the dirham. | וכאשר תפול לפניך שאלה כזאת תכה מחצית השרשים בעצמם ותוסיפם על הדרהמי |
| If the sum can be divided into two parts, each of which has a root, the problem has as many solutions as you wish. | ומה שיתקבץ אם יתחלק לשני חלקים שיחזיק כל חלק שרש תהיה השאלה אז בעלת אופנים כמה שתרצה |
| If it cannot be divided like that, the problem is not solvable and has no solution. | ואם לא יתחלק ככה תהיה השאלה חרשת בזולת האופן |
|
אחר זה הנה הכאת מחצית השרשים בעצמם בזאת השאלה היא תשעה כי מחציתם שלשה |
|
תוסיפם על ר"ס ויהיה רס"ט |
|
תחלקם לשני חלקים שיחזיק כל חלק שרש ויהיו קס"ט ומאה |
|
| |
|
ותאמר אם תרצה אלגו וקס"ט ישוו ר"ס דרהמי פחות ששה דברים |
|
ואם תרצה תאמר אלגו ומאה דרהמי ישוו ר"ס פחות ששה דברים |
|
ויהיה האלגו מ"ט דרהמי |
|
ואם אמרת אלגו ומאה דרהמי ישוו ר"ס דרהמי פחות ששה דברים יהיה האלגו מאה דרהמי |
|
כז ואם יאמרו לך אלגו המחזיק שרש אם תוסיף עליו שני שרשיו יחזיק שרש ואם תוסיף על המקובץ שלשת שרשיו יחזיק שרש |
|
[28]מלאכתו שתניח האלגו שלך אלגו |
|
ותוסיף עליו שני שרשיו ויהיה אלגו ושני שרשים ויחזיק שרש והוא דבר וחצי |
|
והסבה למה הנחתי שרשו דבר וחצי בעבור כי שלשת השרשים כמו שני השרשים וכמו חציים |
|
ותכה דבר וחצי בעצמו ויהיה שני אלגו ורביע ישוה אלגו ושני שרשים |
|
|
|
ותכונהו עמו ויהיה שרש האלגו דרהם אחד ושלשה חומשים |
|
והאלגו שני דרהמי ושני חומשים ורביע חומש החומש |
|
ואם תוסיף עליו שני שרשיו והם שלשה וחמשית יהיה |
|
| |
|
והוא ידוע כי שרשו כמו שרש האלגו הראשון וכמו חציו והוא שני דרהמי ושני חמישיות |
|
ושני השרשים כמו האלגו וכמו רביעיתו |
|
והוא מחויב שיהיו שלשת שרשים מחמשה דרהמי ושלשה חומשים ורביע חומש מחמישית כמו שהוא האלגו ורביעיתו יותר והוא שבעה וחומש |
|
| |
|
וכאשר תוסיפם על חמשה דרהמי ושלשה חומשים וארבעה חומשי החומש יהיה שנים עשר וארבעה חומשים וארבעה חומשי החומש |
|
| |
|
והוא ידוע כי שרשו הוא כמו שרש אחד וחצי מחמשה ושלשה חומשים וארבעה חומשי החומש והוא שלשה דרהמיש ושלשה חומשים |
|
| |
|
והוא ראוי שיהיו ארבעה שרשים וחצי מי"ב וארבעה חומשים וארבעה חומשי החומש שיהיו כמו האלגו וכמו רביעיתו יותר והוא י"ו וחומש |
|
| |
|
וכאשר תוסיפם על י"ב וארבעה חומשים וארבעה חומשי החומש יהיה כ"ט וארבעה חומשי החומש |
|
| |
|
והוא ידוע כי שרשו הוא כמו שרש אחד וחצי מי"ב וארבעה חומשי' וארבעה חומשי החומש והוא חמשה דרהמי ושני חומשים |
|
| |
| Likewise, if you add as many of the roots as you like to what is related to them, the sum has a root as stated. | וכן אם תוסיף מה שתרצה מהשרשים על המתיחס אליו שאשר יתקבץ ממנו יחזיק שרש כמו שנאמר |
|
כי כאשר נוסיף על כ"ט וארבעה חומשי החומש ששת שרשיו ושלשה רביעי השרש יהיה למתקבץ מהם שרש והוא כמו שרש וחצי מכ"ט וארבעה חומשים מחומש והוא שמנה עשר |
|
| |
|
וכאשר תוסיף על המתקבץ עשרת שרשיו ושמינית השרש יחזיק המתקבץ מהם שרש והוא כמו שמנה עשר וכמו חציו שהם כ"ז |
|
| |
| And so on for any addition you want in this way, when you assume an addition that relates to the question, as if we say that the ratio of the question is that its six roots are the same as two roots and their half, more and more are being added endlessly. | וכן ימשכו כל אשר תרצה מההוספות כמו זה האופן כאשר תניח התוספת מתיחס לשאלה [29]כאלו אמרנו שיחס השאלה הוא שששת שרשיו הם כמו שני השרשים וכמו חצים והולכים ומתוספים עד אין להם סוף |
|
כח וכן אם יאמרו לך אלגו המחזיק שרש אם תוסיף עליו שלשת שרשיו יחזיק שרש ואם תקבץ עם העולה ששת שרשיו יחזיק שרש |
|
מלאכתו שתניח האלגו שלך אלגו |
|
תוסיף עליו שלשת שרשיו ויהיה אלגו ושלשה שרשים ושרשו שני דברים |
|
והסבה בהניחנו שרשו שני |
|
ותכה שני דברים בשני דברים ויהיה ארבעה אלגוש ישוו אלגו ושלשה דברים |
|
|
|
ותכונהו עמו ויהיה הדבר ישוה דרהם אחד והוא שרש האלגו |
|
והאלגו דרהם אחד |
| And so on for any addition you want according to the ratio of the question: | וכמו זה ימשך מה שתרצה מההוספות כפי יחס השאלה |
|
נאמר כי כאשר תוסיף על מה שהתקבץ שנים עשר שרשים יחזיק שרש |
|
ואם תוסיף על המקובץ שרשו יחזיק שרש |
|
וכאשר תוסיף על זה המקובץ עשרי' וארבעה שרשיו יחזיק המתקבץ שרש |
|
ואם תוסיף מ"ח שרשים יחזיק שרש |
|
ואם תוסיף צ"ו שרשים יחזיק שרש |
| Whichever you have of this species, when the secondary roots are greater than the primary roots, is solved by this procedure. | וכמעשה הזה יעלו [כל מה][30] שיפלו לפניך מזה המין כאשר יניחו השרשים השניים יותר מהראשונים |
| However, if they are less than the primary roots they are not solved by this procedure, but they have other methods by which they are solved as follows: | ואם יונחו פחות מהראשונים לא יצאו עם זה המעשה אבל להם דרכים אחרים יצאו בהם והם אלו |
|
כט כאשר יאמרו לך אלגו המחזיק שרש אם תוסיף עליו שני שרשים יחזיק שרש ואם תוסיף על המקובץ שרשו יחזיק שרש |
|
ולידיעתו תניח האלגו שלך אלגו |
|
ותוסיף עליו שני שרשיו ויהיה אלגו ושני שרשי' |
|
ותוסיף על הנקבץ שרשו והוא שרש האלגו ושני שרשים ויהיה אלגו ושני שרשי' ושרש האלגו ושני שרשים וצריך שיחזיק שרש |
|
ונניח שרשו דבר וחצי דרהם |
|
ותכהו בעצמו ויהיה אלגו ודבר ורביעית דרהם ישוה אלגו ושני דברים ושרש מאלגו ושני שרשים |
|
ותגרע אלגו ושרש מאלגו ושני שרשים וישאר שרש ושרש מאלגו ושני שרשים ישוו רביעית דרהם |
|
| |
|
ותגרע שרש [31]מרביעית דרהם ישאר רביעית דרהם פחות שרש ישוה שרש מאלגו ושני שרשי' |
|
| |
|
ותכה רביעית דרהם פחות שרש בעצמו ויהיה חלק מי"ו ואלגו פחות חצי דבר ישוה אלגו ושני שרשים |
|
|
|
ותכונהו עמו ויהיה השרש רביעית העשירית |
|
והאלגו חלק אחד מאלף ות"ר |
|
והנחנו השרש דבר וחצי דרהם כדי שישחתו האלגוש וישארו דברים ישוו למספרים |
|
ל וכן אם יאמרו לך אלגו המחזיק שרש אם תוסיף עליו ארבעת שרשיו יחזיק שרש |
|
וידיעתו שתניח האלגו שלך אלגו |
|
ותוסיף עליו ארבעת שרשיו ויהיה אלגו וארבעה |
|
ותוסיף על אלגו וארבעה דברים שני שרשיו ושרש ארבעה אלגוש וי"ו דברים ויהיה אלגו וארבעה דברים ושרש |
|
ותגרע אלגו ושני דברים מאלגו וארבעה דברים וישאר דרהם אחד ישוה שני דברים ושרש מארבעה אלגוש וי"ו דברים |
|
| |
|
ותגרע שני הדברים מהדרהם וישאר דרהם אחד פחות שני דברים ישוה שרש מארבעה אלגוש וי"ו דברים |
|
| |
|
ותכה דרהם אחד פחות שני דברים בעצמו ויהיה דרהם אחד וארבעה אלגוש פחות ארבעה שרשים ישוה ארבעה אלגוש |
|
|
|
ותכוין עמו ויהיה הדבר חצי עשירית מדרהם |
|
והאלגו חלק אחד מארבע מאות |
| Whichever of this species that you have, apply the procedure on its root, until you find it by confrontation, when it remains that things are equal to numbers. | וכל אשר יפול בידך מזה המין תעשה מלאכה בשרשו עד שכאשר מצאת אותו עם הכוון ישאר דברים ישוו מספרים |
| This procedure is very useful. | וזאת המלאכה גדולת התועלת די מוגו אפרוביגו |
|
לא ואם יאמרו לך אלגו אם ואם תגרע ואם תגרע מהנשאר שני שרשיו יחזיק שרש |
|
ולידיעת זה תניח האלגו שלך אלגו |
|
ותגרע ממנו ארבעה שרשיו וישאר אלגו פחות ארבעה דברים ויחזיק שרש והוא חצי דבר |
|
והסבה למה הנחתי חצי הדבר בעבור כי היו שני שרשים חצי הארבעה |
|
ותכה חצי דבר בעצמו ויהיה רביע אלגו ישוה אלגו [32]פחות ארבעה אלגוש |
|
|
|
ותכונהו עמו ויהיה שרש האלגו חמשה ושליש |
|
והאלגו עשרים ושמנה וארבעה תשיעיות |
| The ratio of the roots in this question and its similar is treated as treated in the preceding question. | וזאת השאלה והדומים אליה יתנהג בה יחס השרשים כאשר נהגנו בשאלה שקדמה לה |
We say that when you subtract from the remainder its root, it has a root; and if you subtract from that remainder half its root, it has a root; then a quarter of its root; an eighth of its root; half the eighth of its root - each of the subtracted numbers are half the numbers that precede it, for two roots are half of four roots [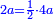 ]. ].
|
נאמר כי כאשר תגרע מהנשאר שרשו יחזיק שרש ואם תגרע מהנשאר חצי שרשו יחזיק שרש וכן רביעית שרשו ושמינית שרשו וחצי שמינית שרשו כל אחד מאלו המספרים הנגרעים חצי המספרים הנגרעים שהם קודמים אליו בעבור כי שני השרשים חצי הארבעה השרשים |
| Whichever of this species that you have, solve it by this method when the secondary roots are less than the primary roots. | וכל אשר יפול לפניך מזה המין תעשהו עם זה האופן כאשר הניחו השרשים השניים פחות מהראשונים |
|
לב ואם יאמרו לך אלגו המחזיק שרש אם תוסיף עליו שרשו יחזיק שרשו ואם תוסיף עליו דרהם אחד יחזיק שרש |
|
וידיעתו שתניח האלגו שלך אלגו |
|
ותוסיף עליו שרשו ויהיה אלגו ודבר ויחזיק שרש |
|
ותוסיף על האלגו אדרהם אחד ויהיה אלגו ודרהם אחד ויחזיק שרש |
|
ותשוב ותניח שרש האלגו ודבר ושרש מאלגו ודרהם אחד פחות חצי דרהם |
|
ותכם בעצמם ויהיה אלגו ודרהם ורביע פחות שרש מאלגו ודרהם אחד ישוה אלגו ודבר |
|
| |
|
ותגרע אלגו מאלגו וישאר דרהם אחד ורביע פחות שרש מאלגו ודרהם אחד ישוה שרש |
|
| |
|
|
|
ותאסוף האדרהם ורביע עם עם שרש מאלגו ודרהם אחד ותוסיפם על השרש ויהיה שרש ושרש מאלגו ודרהם אחד ישוה דרהם ורביע |
|
| |
|
ותגרע השרש מדרהם ורביע ישאר דרהם ורביע פחות דבר ישוה שרש מאלגו ודרהם אחד |
|
| |
|
ותכה האדרהם ורביע פחות דבר בעצמו ויהיה אדרהם וחצי וחצי שמינית פחות שני שרשים וחצי ישוה אלגו ודרהם אחד |
|
| |
|
|
|
ותכונהו עמהם ויהיה הדבר ישוה תשעה חלקים מארבעים והוא שרש האלגו |
|
והאלגו פ"א חלקים מאלף ות"ר |
|
והנחנו שרשו אלגו ודרהם פחות חצי דרהם למען ישאר אחר הכוון שרשים ישוו לדרהם אחד |
|
לג ואם יאמרו לך אלגו המחזיק שרש אם תגרע ממנו חמשה דרהמי יחזיק הנשאר שרש ואם תוסיף על הנשאר שרשו יחזיק המקובץ שרש |
|
[33]וידיעתו שתניח האלגו שלך אלגו |
|
ותגרע ממנו חמשה דרהמי וישאר אלגו פחות חמשה דרהמי |
|
ותוסיף על אלגו פחות חמשה |
|
ותניח שרשו דבר |
|
ותכהו בעצמו ויהיה אלגו ורביע דרהם פחות שרש ישוה אלגו פחות חמשה דרהמי והוא שרש מאלגו פחות חמשה דרהמי |
|
|
|
ותאסוף זה האלגו עם החמשה וזה יהיה עם השרש ותוסיף החמשה על אלגו ורביע דרהם והשרש אלגו ויהיה השרש אלגו וחמשה דרהמי ורביע מהדברים ישוה שרש האלגו פחות חמשה דרהמי |
|
| |
|
ותכה חמשה דרהמי ורביע הדברי' בעצמם ויהיה עשרים ושבעה דרהמי וחצי וחצי שמינית ואלגו פחות עשרה שרשים וחצי שרש ישוה אלגו פחות חמשה דרהמי |
|
| |
|
|
|
ותכוין עמהם ויהיה הדבר ישוה שלשה דרהמי ושבעה עשר חלקים מקפ"ט והוא שרש האלגו |
|
והאלגו תשעה דרהמי וק"ג חלקים מקס"ח חלקים וקכ"א חלקים מכ"ח אלפים ורכ"ד חלקים באחד |
|
וכאשר תגרע ממנו חמשה ישאר ארבעה דרהמי וק"ג חלקים מקס"ח וקכ"א חלקים מכ"ח אלפים ורכ"ד |
|
ותוסיף עליו שרשו שהוא שני דרהמי וכ"ה חלקים מקס"ח וקכ"א חלקים מכ"ח אלפים ורכ"ד ויחזיק שרש והוא שני דרהמי ומאה דרהמי מקס"ח חלקים |
|
| |
|
לד ואם יאמרו לך אלגו שיחזיק שרש אם תוסיף עליו ארבעת שרשיו יחזיק שרש ואם תגרע ממנו שני שרשיו ודרהם אחד יחזיק הנשאר שרש |
|
וידיעתו שתניח האלגו שלך אלגו |
|
ותוסיף עליו ארבעת שרשיו ויהיה אלגו וארבעה שרשי' ויחזיק שרש |
|
ותגרע מהאלגו שני שרשיו ודרהם אחד וישאר אלגו פחות שני שרשים ודרהם אחד ויחזיק שרש |
|
ותניח שרשו כמו שאבאר לך בכוון שלא יתכן להיות שרש אחד מאלגו וארבעה דברים פחות שלשה דרהמי |
|
ותכהו בעצמו ויהיה אלגו ותשעה דרהמי וארבעה שרשים פחות ששה שרשים מאלגו וארבעה דברים ישוו אלגו פחות שני שרשים ודרהם אחד |
|
| |
|
|
|
ותכוין עמו ויהיה אלגו וששה שרשים ועשרה דרהמי ישוה אלגו וששה שרשים מאלגו וארבעה דברים |
|
וששה שרשים מאלגו [34]וארבעה דברים שרש מל"ו אלגוש וקמ"ד דברים |
|
ותגרע אלגו מאלגו וישאר ששה שרשים ועשרה דרהמי ישוה שרש מל"ו אלגוש וקמ"ד דברים |
|
| |
|
|
|
ותכוין בהם ויהיה הדבר ישוה ארבעה דרהמי ושתות והוא שרש האלגו |
|
והאלגו שבעה עשר דרהמיש ורביע התשיעית |
|
לה ואם יאמרו לך אלגו המחזיק שרש אם תגרע ממנו שני שרשיו יחזיק שרש ואם תוסיף על הנשאר שרשו יחזיק שרש |
|
וידיעתו שתניח האלגו שלך אלגו |
|
ותגרע ממנו שני שרשיו וישאר אלגו פחות שני שרשים |
|
ותוסיף עליו שרשו |
|
ותניח שרשו דבר פחות דרהם אחד וחצי |
|
ותכם בעצמם ויהיה אלגו ושני דרהמי ורביע פחות שלשה שרשים ישוה אלגו פחות שני שרשים ושרש מאלגו פחות שני שרשים |
|
| |
|
|
|
ותכוין בהם ויהיה אחר הכוון דבר ושרש מאלגו פחות שני שרשים ישוו שני דרהמי ורביע |
|
ותגרע דבר משני דרהמי ורביע ישאר שני דרהמי ורביע פחות דבר ישוה שרש מאלגו פחות שני שרשים |
|
ותכה שני דרהמי ורביע פחות דבר בעצמו ויהיה חמשה דרהמי וחצי שמינית ואלגו פחות ארבעה דברים וחצי דבר ישוה אלגו פחות שני שרשים |
|
| |
|
|
|
ותכוין עמהם ויהיה הדבר שני דרהמי ורביע עשירית והוא שרש האלגו |
|
והאלגו ארבעה ועשירית אחד מחלק אחד מאלף ת"ר |
|
ותגרע ממנו שני שרשיו והם ארבעה וחצי עשירית ישאר חצי עשירית וחלק אחד מאלף ת"ר |
|
| |
|
ותוסיף עליו שרשו והוא חומש אחד ורביעית העשירית יהיה רביע אחד ורובע העשירית וחלק אחד מאלף ת"ר ומחזיק שרש והוא חצי ורביע העשירית |
|
| |
|
לו ואם יאמרו לך אלגו שיחזיק שרש אם תוסיף עליו שלשת שרשיו ודרהם אחד יחזיק שרש ואם תגרע ממנו שלשת שרשיו פחות שני דרהמי יחזיק מה שישאר שרש |
|
וידיעתו שתניח האלגו שלך אלגו |
|
ותוסיף עליו שלשת שרשיו ודרהם אחד ויהיה אלגו ושלשה שרשים ודרהם אחד ויחזיק שרש |
|
ותגרע מהאלגו שלשת שרשיו פחות שני דרהמי וישאר אלגו ושני דרהמי פחות שלשה שרשים ומחזיק [35]שרש |
|
ותניח שרשו שרש מאלגו ושלשה שרשים ודרהם אחד פחות שלשה דרהמיש |
|
ותכם בעצמם ויבא אלגו ושלשה שרשים ועשרה דרהמי פחות שרש מל"ו אלגוש ול"ו דרהמי וק"ח דברים ישוו אלגו ושני דרהמי פחות שלשה שרשים |
|
| |
|
|
|
ותכוין עמהם ויהיה אחר הכוון ששה דברים ושמנה דרהמי ישוה שרש מל"ו אלגוש ול"ו דרהמיש ור"ח דברים |
|
ותכה ששה דברים ושמנה דרהמי בעצמם ויהיה ל"ו אלגוש וצ"ו דברי' וס"ד דרהמי ישוו ל"ו אלגו ול"ו דרהמי וק"ח דברים |
|
|
|
ותכוין עמהם ויהיה הדבר שני דרהמי ושליש אחד והוא שרש האלגו |
|
והאלגו חמשה דרהמי וארבעה תשיעיות |
|
ואם תוסיף עליו שלשת שרשיו ודרהם אחד יהיה שלשה עשר דרהמי וארבעה תשיעיות ושרשו שלשה דרהמי ושני שלישים |
|
ואם תגרע ממנו שלשת שרשיו פחות שנים דרהמי ישאר ארבעה תשיעיות ושרשו שנים שלישיות |
|
לז ואם יאמרו לך אלגו המחזיק שרש אם תגרע ממנו דרהם אחד פחות שרש האלגו יחזיק הנשאר שרש |
|
וידיעתו שתניח האלגו שלך אלגו |
|
ותוסיף עליו דרהם אחד פחות שרש האלגו ויהיה אלגו ודרהם אחד פחות שרש האלגו ומחזיק שרש |
|
ואם תגרע מהאלגו דרהם אחד פחות שרש האלגו ישאר אלגו ושרש פחות דרהם אחד ומחזיק שרש |
|
ותניח שרש האלגו אלגו ודרהם אחד פחות דבר ודרהם אחד פחות שרש האלגו ושרש פחות דרהם אחד |
|
ותכהו בעצמו ויהיה אלגו ושרש פחות שני שרשים מאלגו ושרש פחות דרהם אחד ישוה אלגו ודרהם אחד פחות שרש |
|
| |
|
|
|
ותאספם עם השרש ותוסיפהו עם האלגו ושרש ותאסוף האחד עם שני שרשים מאלגו ושרש פחות דרהם אחד ותוסיפהו על האלגו ודרהם אחד ותגרע אלגו מאלגו וישאר דרהם אחד ושרש מאלגו ושרש פחות דרהם אחד ישוו שני דברים |
|
| |
|
ותגרע דרהם אחד משני דברים וישאר שני דברים פחות דרהם אחד ישוה שני שרשים מאלגו ושרש פחות דרהם אחד |
|
וחציו שהוא דבר פחות חצי דרהם ישוה שרש מאלגו ושרש פחות דרהם אחד |
|
| |
|
ותכה דבר פחות חצי דרהם בעצמו ויהיה אלגו ורביע דרהם פחות דבר ישוה אלגו ושרש פחות |
|
|
|
ותכוין עמהם ויהיה הדבר חמשה שמיניות מדרהם [36]והוא שרש האלגו |
|
והאלגו שלשה שמיניות ושמינית אחד משמינית |
|
לח ואם יאמרו לך אלגו המחזיק שרש אם תוסיף עליו שני דרהמי פחות שרש האלגו יחזיק שרש ואם תגרע ממנו שלשה פחות שרש האלגו יחזיק שרש |
|
ולידיעת זה תשים האלגו שלך אלגו |
|
ותוסיף עליו שני דרהמי פחות שרש ויהיה אלגו ושני דרהמי פחות שרש ויחזיק שרש |
|
ותגרע ממנו שלשה פחות שרש וישאר אלגו ושרש פחות שלשה דרהמי ויחזיק שרש |
|
ותניח שרש האלגו ושני דרהמי פחות שרש מדרהם אחד ושרש מאלגו ושרש פחות שלשה דרהמי |
|
ותכהו בעצמו ויהיה אלגו ושרש פחות שני דרהמי ושני שרשים מאלגו פחות שלשה דרהמי ישוה אלגו ושנים דרהמי פחות שרש |
|
| |
|
|
|
ותאסוף האלגו ושני דרהמי עם השרש ותוסיפהו עם האלגו ושרש פחות שני דרהמי ותגרע אלגו מאלגו וישאר שני שרשים ושרש מאלגו ושרש פחות שלשה דרהמי ישוה ארבעה דרהמי |
|
| |
|
ותגרע שני השרשים מהארבעה דרהמיש ישאר ארבעה דרהמי פחות שני שרשים ישוה שנים שרשים מאלגו ושרש פחות שלשה דרהמי |
|
ותקח חציו והוא שני דרהמי פחות שרש ישוה אלגו ושרש פחות שלשה דרהמי |
|
| |
|
ותכה שני דרהמי פחות דבר בעצמו ויהיה ארבעה דרהמי ואלגו פחות ארבעה דברים ישוה אלגו ושרש פחות שלשה דרהמי |
|
|
|
ותכוין בהם ויהיה הדבר ישוה דרהם אחד ושני חומשים |
|
והאלגו דרהם אחד וארבעה חומשים וארבעה חומשים מחומש |
|
לט ואם יאמרו לך אלגו המחזיק שרש אם תוסיף עליו שרשו ודרהם אחד יחזיק שרש ואם תוסיף על האלגו שני שרשיו ושני דרהמי יחזיק שרש |
|
וידיעתו שתניח האלגו שלך אלגו |
|
ותוסיף עליו שרשו ודרהם אחד ויהיה אלגו ושרש ודרהם אחד ויחזיק שרש |
|
ותוסיף על האלגו שני שרשיו ושני דרהמי ויחזיק שרש |
|
ותניח שרשו חצי דרהם ושרש מאלגו ושרש ודרהם אחד ישוה אלגו ושני שרשים ושני דרהמי |
|
ותגרע אלגו ושרש ודרהם אחד ורביע מאלגו ושני שרשים ושני דרהמי וישאר דבר ושלשה רביעי דרהם |
|
ותכהו בעצמו ויהיה אלגו ודבר וחצי וחצי דרהם וחצי שמינית ישוה אלגו ושרש ושני דרהמי |
|
| |
|
|
|
ותכוין בהם ויהיה הדבר שבעה שמיניות והוא שרש [37]האלגו |
|
והאלגו חמשה שמיניות ושמינית השמינית |
Word Problems |
|
If You Give Me Problems |
|
|
מ ואם יאמרו לך ארבעה אנשים התקבצו יחד לקנות בהמה אחת ואמר הראשון לשלשה אם תתנו לי החצי מאשר תחזיקו יהיה לי ערך הבהמה |
|
| |
|
וידיעת זה שתניח אל האחד דבר ולשני דינר אחד ולשלישי דרהם אחד ולרביעי פלס אחד |
|
ואמר הראשון שמחזיק דבר אל השלשה תנו לי החצי מאשר לכם ואקבצהו עם אשר לי ויהיה לי ערך הבהמה |
|
ומחצית אשר להם הוא חצי דינר וחצי דרהם וחצי פלס וכאשר קובצו עם הדבר אשר הוא מחזיק יהיה דבר וחצי דינר וחצי דרהם וחצי פלס והוא ערך הבהמה |
|
ואמר השני אשר לו דינר אחד לשלשה תנו לי השלישית מאשר לכם ואקבצהו עם אשר לי ויהיה לי ערך הבהמה |
|
ושלישית אשר להם הוא שלישית דבר ושלישית דרהם ושליש פלס וכאשר קובצו עם הדינר אשר לו יהיו בידו דינר אחד ושלישית דבר ושלישית דרהם ושלישית פלס והוא ערך הבהמה |
|
וזה יהיה שוה לדבר וחצי דינר וחצי דרהם וחצי פלס |
|
|
|
ותכוין בהם ויהיה זה שתגרע שלישית דבר מדבר שלם וישאר שני שלישי דבר |
|
ותגרע שליש דרהם מחצי דרהם וישאר ששית דרהם |
|
ותגרע שליש פלס מחצי פלס ישאר ששית פלס |
|
ותגרע חצי גאקיש מדינר אחד וישאר חצי דינר ישוה שני שלישי דבר |
|
|
|
[ורביע דינר ישוה שליש דבר וחצי שתות דרהם וחצי שתות פלס |
|
וחמישית דינר ישוה חמישית דבר וחמישית שלישית הדבר וחמי' שלישית דרהם וחמישית שלישית אלפלס][38] |
|
ותניח ערך הבהמה דבר וחצי דינר וחצי דרהם וחצי אלפלס |
|
ותגרע חצי הדינר מערך הבהמה ותניח במקומו חצי אשר ישוה ויהיה ערך הבהמה דבר ושני שלישים מדבר ושני שלישי דרהם ושני שלישי אלפלס |
|
| |
|
וכבר |
|
ואמר השלישי אשר לו דרהם אחד תנו לי הרביעית מאשר לכם ואקבצהו עם אשר לי ויהיה לי ערך הבהמה |
|
ורביעית אשר להם הוא ויהיה שוה לדבר ושני שלישי דבר ושני שלישי דרהם ושני שלישי אלפלס |
|
| |
|
|
|
ותכוין בהם והוא שתגרע שלישית דבר ורביעית דבר מדבר ושני שלישי דבר ושליש פלס משני שלישי פלס וישאר דבר וחצי שתות דבר ושליש פלס |
|
| |
|
ותגרע שני שלישי דרהם מדרהם וחצי שתות וישאר רביע ושתות דרהם ישוה דבר וחצי שתות דבר ושליש פלס |
|
| |
|
והדרהם ישוה שני דברים ושלשה חמשי דבר וארבעה חומשי אלפלס |
|
ותסיר השני שלישי דרהם מערך הבהמה ותשים במקומם שני שלישים ממה ששוה הדרהם והוא דבר ושליש ושני חומשי דבר ושליש וחומש אלפלס ויהיה ערך הבהמה שלשה דברים |
|
| |
|
וכבר לקחנו הדרהם ושמנו במקומו שני דברים ושלשה חומשי דבר וארבעה חומשי אלפלס |
|
ותגרע שלישית הדרהם אשר הוא בערך הדינר ותניח במקומו שלישית מה שישוה הדרהם והוא שני שלישי דבר וחומש דבר וחומש אלפלס ושלישית חומש אלפלס ויהיה ערך |
|
| |
|
הנה התבאר שיחזיק הראשון דבר |
|
והשני והוא אותו מהדינר יחזיק שני דברים וחומש ושלשה חומשי אלפלס |
|
והשלישי והוא אותו מהדרהם יחזיק שני דברים ושלשה חומשי דבר וארבעה חומשי אלפלס |
|
וערך הבהמה יהיה שלשה דברים ושני חומשי דבר ופלס אחד וחומש |
|
ואמר האיש הרביעי המחזיק אלפלס אחד אל השלשה תנו לי החמישית ממה שבידכם ואקבצהו עם אשר לי ויהיה לי ערך הבהמה |
|
וחמישית אשר להם הוא דבר וארבעה חומשי חמישית דבר וחמישית אלפלס ושני חומשי חמישית אלפלס וכאשר קבצנום עם האלפלס אשר הוא מחזיק יהיה לו אלפלס אחד וחמישית אלפלס ושני חומשי חמישית אלפלס ודבר וארבעה חומשי חמשית דבר וישוה כל זה [42]ערך הבהמה שהוא שלשה דברים ושני חומשי דבר ופלס וחומש |
|
| |
|
|
|
ותגרע פלס וחומש מפלס וחומש ושני חמשי חמשית פלס וישאר שני חומשי חמישית פלס |
|
ותגרע דבר וארבעה חומשי חמישית דבר משלשה דברים ושני חומשי דבר וישאר שני דברים וחומש וחמישית חמישית הדבר וישוה שני חומשי חמישית אלפלס |
|
| |
|
והפלס ישוה עשרים ושמנה דברים |
|
ונניח הדבר אחד והוא מה שמחזיק הראשון |
|
והשני תשעה עשר בעבור כי הוא שלשה חומשים ממה שמחזיק הרביעי וכמו הכפל מאשר יחזיק הראשון ושני חומשיו יותר |
|
והשלישי עשרים וחמשה בעבור כי הוא ארבעה חומשי מאשר לרביעי וכמו הכפל מהראשון ושלשה חומשים יותר |
|
וערך הבהמה שלשים ושבע בעבור כי הוא כמו אשר לרביעי וכמו החומש וכמו שלשה דמיוני אשר לראשון וכמו חמישיתו |
| This procedure is the one that is known to the arithmeticians and it is a complete understanding, except that it is very subtle and the number is extracted with great difficulty. | וזה המעשה הוא אשר ידעו אותו בעלי המספר ביניהם והיא הבנה שלימה זולת שהיא מאד דקה ובקושי גדול יצא החשבון |
| I solve this with a direct procedure and full understanding: | וכבר אוציאהו לאור אני עם מלאכה ישרה והבנה שלמה |
|
וזה שנניח כל אשר יחזיקו דרהם אחד |
|
ונניח שיהיה לאחד מזה הדרהם דבר |
|
וישאר לשלשה דרהם פחות דבר |
|
וכאשר נקבץ חציים עם אשר לראשון שהוא דבר יהיה ערך הבהמה חצי דבר וחצי דרהם |
|
ותניח לשני דינר אחד |
|
וכאשר נקבץ שלישית הדרהם עם הדינר יהיה ערך הבהמה שני שלישיות מדינר ושלישית אדרהם וישוה ערך הבהמה מהחלק האחד שהיה חצי דבר וחצי דרהם |
|
ותגרע שליש דרהם מחצי דרהם וישאר שני שלישי דינר ישוו חצי דבר וששית דרהם |
|
והדינר ישוה שלשה רביעי דבר ורביע דרהם |
|
והוסר שם הדינר ונשאר במקומו שלשה רביעי דבר ורביע דרהם |
|
ותניח לשלישי דינר אחד |
|
וישאר לשלשה דרהם אחד פחות דינר |
|
וכאשר קבצנו רביעיתו עם אשר לשלישי שהוא דינר אחד יהיה ערך הבהמה שלשה רביעי דינר ורביעית וישוה ערך הבהמה מהחלק האחד שהוא חצי דבר וחצי דרהם |
|
ותגרע רביעית דרהם מחצי דרהם וישאר [43]חצי דבר ורביע דרהם ישוה שלשה רביעי דינר |
|
והדינר ישוה שני שלישי דבר ושליש דרהם |
|
ויהיה כל אשר לשלשה שני דברים ורביע ושתות דבר ושליש ורביע דרהם |
|
| |
|
וכבר הנחנו לארבעתם דרהם אחד ומפני זה ישאר לרביעי רביע ושתות מדרהם פחות שני דברים ורביע ושתות דבר |
|
| |
|
ואמר זה האיש הרביעי אל השלשה המחזיקים שני דברים ורביע ושתות דבר ושליש ורביע דרהם תנו לי החומש מאשר לכם ואקבצהו עם אשר לי ויהיה לי ערך הבהמה |
|
והחומש מאשר להם הוא עשרים ותשעה חלקים מששים חלקים |
|
וכאשר תקבצהו עם אשר לו שהוא רביע ושתות מדרהם פחות שני דברים ורביע ושתות מדבר יהיה אשר יחזיק הרביעי ל"ב חלקים מששים בדרהם פחות דבר וחמשים ושש וישוה לערך מהחלק האחד שהוא חצי דבר וחצי דרהם |
|
| |
|
|
|
ותכוין בהם ויהיה אחר הכוון שלישית העשירית מדרהם ישוה שני דברים ושלישית דבר ועשירית דבר |
|
והדרהם ישוה שבעים ושלשה דברים |
|
ותניח שווי הדרהם ע"ג והוא כל אשר מחזיקים ארבעתם |
|
ושווי הדבר אחד והוא מה שיש לראשון |
|
ואשר לשני יהיה תשע עשרה בעבור כי היה [לו כדי אשר][45] שוה רביע דרהם ושלשה רביעי דבר |
|
ולשלישי עשרים וחמשה בעבור כי היה לו כדי שליש דרהם ושני שלישי דבר |
|
ולרביעי עשרים ושמנה בעבור כי היה לו כדי רביע ושתות דרהם פחות שני דברים ורביע ושתות דבר |
|
ואם תרצה תקבץ אשר לראשון ולשני ולשלישי ויהיה ארבעים וחמשה ותגרעם מע"ג ויהיה מה שישאר אשר לאיש הרביעי והוא עשרים ושמנה |
|
וערך הבהמה שלשים ושבעה בעבור כי הונח חצי מה ששוה הדרהם וחצי מה שיש לראשון |
| From now on you will be able to apply this procedure to whatever that falls into your hands of this kind and its mysteries will be solved. | ומעתה תוכל לעשות כמעשה הזה כל אשר יפול בידך מזה האופן ויצא לאור תעלומותיו |
|
מא ואם יאמרו לך חמשה אנשים התקבצו יחד לקנות אלפאגה אחת ואמר הראשון לשני ולשלישי תנו לי החצי מאשר לכם ויהיה לי [46]כדי לקנותה |
|
| |
|
ולדעת זה נניח לראשון דבר אחד ולשני דינר אחד ולשלישי דרהם אחד |
|
ולא נצטרך בזאת השאלה ולדומים אליה כדי להוציא החשבון ליותר מאלו השלשה מינים |
|
אחר כן אמר הראשון המחזיק דבר לשני ולשלישי המחזיקים דינר ודרהם תנו לי החצי מאשר לכם ואקבצהו עם אשר לי ויהיה לי ערך הבהמה |
|
והחצי מאשר מחזיקים הוא חצי דינר וחצי דרהם ולכן יהיה לו דבר אחד וחצי דינר וחצי דרהם והוא ערך הבהמה |
|
ואמר השני אשר לו דינר אחד לשלישי ולרביעי תנו לי שלישית החלק מאשר לכם ועם אשר לי שהוא דינר אחד אחזיק ערך הבהמה |
|
ותגרע |
|
[ותכהו בשלשה ויהיה שלשה דברים ודרהם אחד וחצי פחות דינר וחצי והוא כל אשר יחזיקו השלישי והרביעי |
|
ותגרע אשר לשלישי שהוא דרהם אחד וישאר מה שהוא לרביעי והוא שלשה דברים וחצי דרהם פחות דינר אחד וחצי][47] |
|
ואמר השלישי המחזיק דרהם אחד לרביעי ולחמישי תנו לי רביעית אשר לכם ועם אשר לי יהיה לי ערך הבהמה והוא דבר אחד וחצי דינר וחצי דרהם |
|
ותגרע מערך הבהמה אשר לשלישי שהוא דרהם אחד וישאר דבר וחצי דינר פחות חצי דרהם והוא רביעית אשר לרביעי ולחמישי |
|
ותכהו בארבעה ויהיה ארבעה דברים ושני דינרים פחות שני דרהמי והוא כל אשר לרביעי ולחמישי |
|
ותגרע מהם מה שיש לרביעי שהוא שלשה דברים וחצי דרהם פחות דינר אחד וחצי וישאר מה שיש לחמישי דבר אחד ושלשה דינרים וחצי פחות שני דרהמי וחצי |
|
| |
|
ואמר הרביעי המחזיק שלשה דברים וחצי דרהם [48]פחות דינר אחד וחצי לחמישי ולראשון המחזיקים שני דברים ושלשה דינרים וחצי פחות שני דרהמי וחצי תנו לי החמישית מאשר לכם ועם אשר לי יהיה בידי כמו ערך הבהמה |
|
וחמישית אשר להם הוא שני חומשי דבר |
|
וכאשר תקבצם עם אשר לו יהיו בידו שלשה דברים ושני חומשים פחות ארבעה חומשי דינר |
|
| |
|
והוא שוה לערך הבהמה מהחלק האחר שהיה דבר וחצי דינר וחצי דרהם |
|
|
|
ותכוין בהם ויהיה הדרהם יהיה שוה ארבעה דברים וארבעה חומשי דבר פחות שני דינרים ושלשה חומשי דינר |
|
ותגרע חצי הדרהם אשר בערך הבהמה ותניח במקומו חצי מה ששוה הדרהם והוא שני דברים ושני חומשי דבר פחות דינר אחד ושלשה עשיריות מדינר ויהיה ערך הבהמה שלשה דברים ושני חומשי דבר פחות ארבעה חומשי דינר |
|
| |
|
והנה אשר לראשון הוא דבר |
|
ואשר לשני הוא דינר אחד |
|
ולשלישי ארבעה דברים וארבעה חומשי דבר פחות שני דינרים ושלשה חומשי דינר |
|
ולרביעי חמשה דברים ושני חומשי דבר פחות שני דינרים וארבעה חומשי דינר |
|
והסבה למה הנחנו ככה לרביעי הוא בעבור שהיה לרביעי שלשה דברים וחצי פחות דינר אחד וחצי ותגרע חצי דרהם מאשר בידו ותניח במקומו החצי ממה ששוה הדרהם |
|
וצריך הוא כפי מה שבארנו לחמישי עשרה דינרי' פחות אחד עשרה דברים |
|
ואמר לראשון ולשני אשר להם דבר ודינר אחד תנו לי הששית מאשר לכם ואקבצהו עם אשר לי ויהיה לי ערך הבהמה |
|
והששית מאשר להם הוא ששית דבר וששית דינר |
|
ויהיה מה שיחזיק החמישי עשרה דינרים ושתות פחות עשרה דברים וחמשה ששיות הדבר והוא ערך הבהמה |
|
| |
|
וישוה לערך הבהמה מהחלק האחר שהוא שלשה דברים ושני חומשי דבר פחות ארבעה חומשי דינר |
|
|
|
ותכוין בהם ויהיה אחרי זה הכוון הדבר ארבעים ושבעה והוא אשר לראשון |
|
והדינר ששים ואחד והוא אשר לשני |
|
ואשר לשלישי ששים ושבעה בעבור כי הוא ארבעה |
|
ולרביעי שמנים ושלשה בעבור כי לו חמשה דמיונים |
|
ולחמישי תשעים ושלשה בעבור כי הוא עשרה דמיונים מאשר לשני פחות אחד עשר דמיוני אשר אל הראשון |
|
וערך הבהמה קי"א בעבור כי הוא שלשה דמיונים ושני חומשים מאשר לראשון פחות ארבעה חומשים מאשר לשני |
|
ואם בקשת להוציא אשר לרביעי ואשר לחמישי אחר זה המעשה |
|
הנה כבר ידעת שאשר לראשון מ"ז ואשר לשני ס"א ואשר לשלישי ס"ז |
|
ותגרע מה שיש לשני מערך הבהמה וישאר חמשים ותכם בשלשה ויהיה ק"נ ותגרע מהם מה שיש לשלישי והוא ס"ז ישאר אשר לרביעי פ"ג |
|
ואם בקשת לדעת אשר לחמישי תגרע מה שיש לשלישי מערך הבהמה וישאר מ"ד ותכם בארבעה ויהיה קע"ו ותגרע מהם מה שיש לרביעי והוא פ"ג וישאר צ"ג והוא מה שיש לחמישי |
|
ואם תרצה תניח לראשון דבר אחד ולשני דינר אחד ולשלישי דרהם אחד ולרביעי אלפלס אחד |
| In this question and [others] similar to it there is no need for more than these four [algebraic] species to extract the numbers. | ולא יצטרך לזאת השאלה ולדומים אליה כדי להוציא החשבון ליותר מאלו הארבעה מינים |
|
ואמר הראשון לשני ולשלישי תנו לי חצי אשר לכם ואקבצהו עם אשר לי ויהיה עמי ערך הבהמה |
|
ויהיה ערך הבהמה דבר וחצי דינר וחצי דרהם |
|
ואמר השני לשלישי ולרביעי תנו לי שלישית אשר לכם ועם אשר לי יהיה [לי][50] ערך הבהמה |
|
ויהיה ערכה מזה החלק דינר ושליש דרהם ושליש אלפלס |
|
וישוה לערך הבהמה מהחלק האחר שהוא דבר וחצי דינר וחצי דרהם |
|
|
|
ותכוין בהם ויהיה האלפלס ישוה שלשה דברים וחצי דרהם פחות דינר וחצי והוא אשר לרביעי |
|
ותניח לחמישי אלפלס אחד |
|
ואמר השלישי אשר לו דרהם אחד לרביעי ולחמישי אשר להם אלפלס אחד ושלשה דברים וחצי דרהם פחות שני דינרים וחצי תנו לי רביעית אשר לכם ועם אשר לי יהיה ערך הבהמה |
|
ורביעית אשר להם הוא שלשה רביעי דבר ורביעית אלפלס ושמינית דרהם פחות שלשה שמיניות מדינר |
|
וכאשר קובצו עם הדרהם אשר לו יהיה בידו ערך הבהמה והוא ויהיה ערך הבהמה מזה החלק דרהם אחד [51]ושמינית אחד מדרהם ורביע אלפלס ושלשה רביעי דבר פחות שלשה שמיניו' מדינר |
|
| |
|
ויהיה שוה לערך הבהמה מהחלק ראשון שהוא דבר וחצי דינר וחצי דרהם |
|
|
|
ותכוין בהם ויהיה אלפלס ישוה דבר ושלשה דינרי' וחצי פחות שני דרהמי וחצי והוא אשר לחמישי |
|
ותעשה הנשאר מן השאלה כפי מה שהראיתיך |
|
מב ואם יאמרו לך חמשה אנשים התקבצו ואמר הראשון לשני אם תתן לי החצי מאשר בידך יהיה לי ערך הבהמה ואמר השני לשלישי אם תתן לי השלישית מאשר בידך יהיה לי ערך הבהמה |
|
ולדעת זה תניח לראשון דבר ולשני דינר אחד ולשלישי דרהם אחד |
|
ואמר הראשון אשר בידו דבר אחד לשני אשר בידו דינר אחד אם תתן לי החצי מאשר לך יהיה לי ערך האלפאגה |
|
ויהיה ערך הבהמה דבר וחצי דינר |
|
ואמר השני אשר בידו דינר אחד לשלישי אשר בידו דרהם אחד אם תתן לי השלישית מאשר לך יהיה לי ערך הבהמה |
|
ויהיה ערך הבהמה מזה החלק דינר אחד ושלישית דרהם |
|
ויהיה שוה לערך הבהמה מהחלק האחד שהיה דבר וחצי דינר |
|
|
|
ותכוין בהם ויהיה הדרהם ישוה שלשה דברים פחות דינר אחד וחצי והוא אשר לשלישי |
|
ותניח לרביעי דרהם אחד |
|
ואמר השלישי אשר לו שלשה דברים פחות דינר וחצי לרביעי אשר לו דרהם אחד אם תתן לי הרביעית מאשר לך יהיה לי ערך הבהמה |
|
ויהיה ערך הבהמה מזה החלק שלשה דברים ורביע דרהם פחות דינר וחצי |
|
וישוה ערך הבהמה מהחלק האחד שהוא דבר וחצי דינר |
|
|
|
ותכוין עמו ויהיה הדרהם ישוה שמנה דינרים פחות שמנה דברים והוא אשר לרביעי |
|
ותניח לחמישי דרהם אחד |
|
ואמר הרביעי אשר לו שמנה דינרים פחות שמנה דברים לחמישי אשר לו דרהם אחד אם תתן לי [52]החמישית מאשר לך יהיה לי ערך הבהמה |
|
ויהיה ערך הבהמה מזה החלק שמנה דינרים וחמישית דרהם פחות שמנה דברים |
|
ויהיה שוה לערך הבהמה מהחלק האחר שהוא דבר וחצי דינר |
|
|
|
ותכוין בהם ויהיה הדרהם ישוה מ"ה דברים פחות ל"ז דינרים וחצי והוא מה שיש לחמישי |
|
ואמר לראשון אשר לו דבר אם תתן לי הששית מאשר לך יהיה לי ערך הבהמה |
|
והששית מהראשון הוא ששית דבר |
|
ונקבצהו עם מה שמחזיק ויהיה ערך הבהמה מזה החלק מ"ה דברים וששית דבר פחות ל"ז דינרים וחצי |
|
ויהיה שוה לערך הבהמה מהחלק האחר שהוא דבר וחצי דינר |
|
|
|
ותכוין בהם ויהיה אחר הכוון מ"ד דברים וששית דבר ישוה ל"ח דינרים |
|
והשיבם כלם ששיות |
|
ויהיה הדבר רכ"ח והוא אשר לראשון |
|
והדינר רס"ה והוא אשר לשני |
|
ולשלישי שלשה דברים פחות דינר אחד וחצי והוא רפ"ו וחצי |
|
והשיבם כלם רביעים נ' חצאים |
|
ויהיה הדבר והוא אשר לראשון תנ"ו |
|
ויהיה הדינר והוא מה שיש לשני תק"ל |
|
ויהיה אשר לשלישי תקע"ג |
|
ולרביעי שמנה דינרים פחות שמנה דברים ויהיה תקצ"ב |
|
ולחמישי מ"ה דברים פחות ל"ז דינרים וחצי והוא תרמ"ה |
|
ויהיה ערך הבהמה תתקכ"א |
|
מג ואם יאמרו לך ארבעה אנשים התקבצו לקנות בהמה אחת ואמר הראשון לשני אם תתן לי דרהם אחד יהיה לי הכפל ממה שנשאר לך |
|
|
|
|
ולדעת זה תניח לראשון דבר ולשני דינר אחד |
|
וכאשר לקח הראשון מהשני דרהם אחד יהיה לראשון דבר ודרהם אחד ויהיה כמו הכפל ממה שנשאר לשני והוא שני דינרים פחות שני דרהמי |
|
|
|
ותכוין בהם ויהיה הדינר ישוה חצי דבר ודרהם וחצי והוא אשר לשני |
|
ותניח לשלישי דינר אחד |
|
וכאשר לקח ממנו השני שני דרהמי יהיה לשני שלישית נ' חצי דבר ושלשה דרהמי וחצי [53]ויהיה שוה לשלשה דמיוני מה שנשאר לשלישי והוא שלשה דינרים פחות ששה דרהמי |
|
|
|
ותכוין בהם וישוה הדינר שתות דבר ושלשה דרהמי ושתות והוא אשר לשלישי |
|
ונניח לרביעי דינר אחד |
|
וכאשר לקח ממנו השלישי שלשה דרהמי יהיה לו שתות דבר וששה דרהמי ושתות וישוה ארבעה דמיונים ממה שנשאר לרביעי והוא ארבעה דינרים פחות שנים עשר דרהמי |
|
|
|
ותכוין בהם ויהיה שוה הדינר שלישית השמינית מדבר וארבעה דרהמי ורביע ושתות ושמינית דרהם והוא אשר לרביעי |
|
וכאשר לקח מהראשון ארבעה דרהמי יהיה לו שמנה דרהמי וי"ג חלקים מכ"ד חלקים בדרהם ושלישית השמינית מדבר וישוה לחמשה דמיונים מאשר נשאר לראשון והוא חמשה דברים פחות עשרים דרהמי |
|
| |
|
|
|
ותכוין בהם יהיה הדבר ישוה לחמשה דרהמי ותשעים חלקים מקי"ט חלקים מאחד והוא אשר לראשון |
|
ולשני החצי ממה שיש לראשון ודרהם אחד וחצי יותר ויהיה ארבעה דרהמי ומ"ה חלקים מקי"ט |
|
ולשלישי הששית ממה שיש לראשון ושלשה דרהמי ושתות יותר והוא ארבעה דרהמי וט"ו חלקים מקי"ט חלקים בדרהם |
|
ולרביעי שליש השמינית מאשר לראשון וארבעה דרהמי ושתות ושמינית והוא ארבעה דרהמי וצ"ג חלקים מקי"ט חלקים בדרהם |
|
מד ואם יאמרו לך אלגו בין שלשה אנשים לאחד החצי ולשני השליש ולשלישי השתות והרויחו האלגו ביניהם והחזיר אשר לו החצי [המחצית] מחלקו |
|
ולדעת זה נניח האלגו דבר ודינר אחד ודרהם אחד אי דיו נ"א אי גאנו נראה לי |
|
והרויח בעל החצי דבר |
|
ובעל השליש דינר אחד |
|
ובעל השתות דרהם אחד |
|
והשיב בעל החצי חצי דבר |
|
והשיב בעל השליש שלישית דינר |
|
והשיב בעל השתות ששית דרהם |
|
והתקבץ מאשר השיבו חצי דבר ושלישית דינר וששית דרהם |
|
וחלקו אותו ביניהם [55]לשליש והגיע לכל אחד מהם שתות דבר ותשיעית דינר וחצי תשיעית דרהם |
|
וקבצוהו כל אחד מהם עם מה שנשאר להם |
|
ויהיה לבעל החצי שני שלישי דבר ותשיעית דינר וחצי תשיעית דרהם |
|
וישוה לחצי האלגו שהוא חצי דבר וחצי דינר וחצי דרהם |
|
|
|
ותכוין בהם ויהיה הדבר ישוה שני דינרים ושני שלישים נ' ושליש ושני דרהמי ושני שלישיות |
|
וצריך שיהיה האלגו שלשה דינרים ושליש ושלשה דרהמי ושני שלישים |
|
ושב חציו והוא דינר ושתות ודרהם ושליש |
|
וכל מה שהשיבו דינר אחד וחצי ודרהם וחצי |
|
וחלקוהו לשליש לכל אחד חצי דינר וחצי דרהם |
|
ויהיה לבעל הדינר אשר לו שלישית האלגו והוא דינר ותשיעית דינר ודרהם ושני תשיעיות דרהם |
|
| |
|
|
|
ותכוין בהם ויהיה הדינר ישוה שלשה עשר דרהמיש והוא מה שהרויח בעל השלישית |
|
והדבר שני דינרים ושליש ושני דרהמיש ושני שלישים והוא שלשים ושלשה והוא מה שהרויח בעל החצי |
|
והרויח בעל השתות דרהם אחד והוא אחד |
|
וצריך מבלי טורח כאשר תנסהו שתכהו בששה פעמים ויהיה מה שהרויח בעל החצי קצ"ח ובעל השלישית ע"ח ובעל השתות ששה והאלגו כלו רפ"ב |
|
| |
| This procedure is used by the arithmeticians and it is a complete understanding, but it is more correct for a large numbers, if many people are given [?]. | וכמעשה הזה יעשוהו בעלי החשבון ביניהם והיא הבנה שלמה אלא שתתדקדק ביותר על מי שיעשהו במספר רב אם יניחו האנשים הרבה |
| I solve this with a direct procedure and full understanding: | ואוציאהו לחפשי אני עם מלאכה ישרה [והבנה] מובנת |
|
והוא שנניח האלגו דבר |
|
ואשר הרויח כל אחד מהם מן אלגו ואחר מה שהשיבו כפי הנחתנו מדרהמי שליש וחלקוהו לשליש ועלה לכל אחד מהם וקבצהו עם מה שנשאר לו ויהיה לכל אחד |
|
ובנקלה תדע שאשר נשאר לבעל החצי אחר שהשיב החצי ממה שהרויח חצי דבר פחות דרהם |
|
ומה ששב הוא חצי דבר פחות דרהם אחד |
|
ולשלישי שב שתות דבר פחות דרהם אחד |
|
ומה ששב אחד משלשים בדבר חמשה דרהמי |
|
ויהיה כל אשר השיבו חצי דבר וחמישית דבר פחות דרהם אחד וז' עשיריות מדרהם |
|
| |
|
וה' שביעיות מדרהם והוא כל האלגו |
|
וכאשר תרצה לדעת כמה הרויח בעל [56]החצי דבר פחות שני דרהמי והוא ארבעה דרהמי וה' שביעיו' מדרהם |
|
והרויח בעל השליש חצי דבר פחות דרהם אחד וחצי והוא דרהם אחד וו' שביעיות מדרהם |
|
ומה שהרויח בעל השתות חמישית דבר פחות דרהם אחד וחומש והוא שביעי' דרהם |
|
ותניח כל דרהם מ"ב חלקים |
|
ויהיה האלגו רפ"ב |
|
ומה שהרויח בעל החצי קצ"ח |
|
ומה שהרויח בעל השליש ע"ח |
|
ומה שהרויח בעל השתות ששה |
Garments |
|
|
מה ואם יאמרו לך עשרה בגדים ערך הבגד הראשון דרהם אחד וערך כל אחד מן האחרים יוסיף האחד על האחר דרהם אחד השני יעדיף על הראשון דרהם אחד והשלישי יעדיף על השני דרהם אחד וכן כל האחרים כמה יהיה ערך כלם |
|
וכבר |
|
וערך השני שנים והתשיעי תשעה |
|
וערך השלישי שלשה והשמיני שמנה |
|
והנה הערך [מכל אחד][57] מאלו הזוגות אחד עשר |
|
וכמו כן יהיה ערך כל הזוגות כאלו והם |
|
ותכה אחד עשר בחמשה ויהיה חמשים לחמשה וככה ערך עשרת הבגדים |
|
| |
|
מו ואם יאמרו לך ערך הבגד הראשון אחד ויוסיף |
|
הנה יהיה ערך הבגד העשירי י"ט |
|
בעבור כי הוסיף השני על הראשון שנים |
|
והשלישי על השני שנים |
|
ויוסיף השלישי על הראשון ארבעה |
|
והרביעי על הראשון ששה |
|
והעשירי יוסיף על הראשון י"ח |
|
וכפי זה יהיה ערך הראשון עם העשירי מקובצים עשרים |
|
ותכה עשרים בחמשה ויהיה מאה וככה ערך עשרת הבגדים |
|
מז ואם יאמרו לך עשרה בגדים ערך הראשון שלשה דרהמים ויוסיף ערך האחרים האחד על האחר ארבעה ארבעה כמה יהיה ערך כלם |
|
והמעשה בזה כאשר בארתי הוא שתכה יתרון האחד על האחר והוא ארבעה במספר הבגדים פחות אחד ויהיה שלשים ושש ותוסיף עליהם שלשה שהוא ערך הבגד הראשון ויתקבץ שלשים ותשע |
|
| |
|
ויהיה ערך הראשון והעשירי ארבעים [58]ושנים וכן ערך התשיעי והשני יהיה ארבעים ושנים ובדרך הזה יהיה ערך כל שני בגדים מהם ותכה מ"ב בחמשה ויהיה מאתים ועשר וככה ערך כל הבגדים |
|
| |
Group of People |
|
|
מח ואם יאמרו לך חבורה מאנשים עלו להרויח והרויח הראשון דרהם אחד והרויח כל אחד מהם יותר מאשר לפניו דרהם אחד והיה כל אשר הרויחו שלש מאות כמה היו האנשים מהחבורה |
|
ולדעת זה תניח מספר אנשי החבורה דבר |
|
וצריך שירויחו בין כל שנים מהם כפי הסדר האמור בבגדי' דבר ודרהם אחד |
|
ותכה דבר ודרהם אחד בחצי דבר ויהיה חצי אלגו וחצי דבר והוא כל מה שהרויחו ויהיה שוה שלש מאות דרהמי |
|
|
|
ותכוין עמהם ויהיה הדבר עשרים וארבעה וככה מספר אנשי החבורה |
|
מט ואם יאמרו לך חבורה מאנשים והראשון הרויח שני דרהמי והוסיף כל אחד על אשר לפניו שלשה והיה כל מה שהרויחו מאה ועשרים ושש כמה יהיה מספר החבורה |
|
ולדעת זה תניח כמו שבארתי לך בבגדים שתכה מה שיותיר כל אחד במספר החבורה פחות אחד ויהיה שלשה דברים פחות שלשה דרהמי ותוסיף עליו מה שהרויח הראשון והוא שנים ויהיה שלשה דברים פחות דרהם אחד |
|
| |
|
ויהיה מה שהרויח הראשון והאחרון שלשה דברים ודרהם אחד והוא מה שהרויח כל |
|
ותכה זה במספר החבורה ויהיה אלגו וחצי וחצי דבר והוא כל מה שהרויחה החבורה והוא ישוה קכ"ו דרהמי |
|
|
|
ותכוין עמהם ויהיה הדבר תשעה והוא מספר החבורה |
|
נ ואם יאמרו לך חבורה מאנשים שהרויח הראשון דרהם אחד והוסיף כל אחד על אשר לפניו אחד דרהם וקבצו כל אשר הרויחו יחד וחלקוהו בשוה ועלה לחלק לכל אחד מהם עשרה דרהמי |
|
וכבר בארנו שכל אשר הרויחו הוא חצי אלגו וחצי דבר |
|
וחלקנוהו על מספר החבורה והוא דבר ויגיע לחלק עשרה דרהמי |
|
[59]נא ואם יאמרו לך חבורה אשר שני שלישיתיה הרויח הראשון דרהם אחד והוסיפו |
|
ולדעת זה נשים מספר החבורה דבר |
|
ושני שלישם הרויח הראשון דרהם אחד והוסיפו בשני דרהם |
|
וצריך כפי מה שבארנו שיהיה כל אשר הרויחו ארבעה תשיעיות מאלגו |
|
ואנו נחלקם על מספר החבורה שהוא דבר ויצא ארבעה דרהמי |
|
ותכה ארבעה דרהמי בדבר ויהיה ארבעה דברים ישוו ארבעה תשיעיות מאלגו |
|
והאלגו ישוה |
|
והדבר ישוה תשעה והם מספר החבורה |
|
נב ואם יאמרו לך חבורה ששלשת רביעיה עלו להרויח והרויח הראשון שני דרהמי והוסיפו האחרים בשלשה רביעים וכאשר שבו חלקום כל אשר הרויחו בין כל החבורה בשוה והגיע לכל אחד מהם עשרים וארבעה דרהמי כמה יהיה מספר החבורה |
|
ולדעת זה תניח מספר החבורה דבר |
|
ועלו שלשת רביעיה להרויח והם שלשה רביעי דבר |
|
והרויח הראשון שנים דרהמי והוסיפו בשלשה שלשה רביעים |
|
וצריך כפי מה שבארנו שיהיה כל אשר הרויחו שלשת רביעי הדבר ששה שמיניות מאלגו ושלשת רביעי שמינית האלגו ושלשה שמיניות מדבר |
|
ותחלקהו על מספר החבורה שהוא דבר |
|
ויהיה עשרים וארבעה דברים ישוה שלשה רביעי אלגו ושלשה שמיניות מדבר |
|
|
|
ותכוין עמהם ויהיה הדבר עשרים ושמנה והוא מספר החבורה |
|
נג ואם יאמרו לך חבורה ששני שלישיה יצאו להרויח והרויח הראשון מהם דרהם אחד והוסיפו בשני דרהמי והשלישית הנשאר הרויח הראשון מהם שנים דרהמי והוסיפו בארבעה וקבצו כל מה שהרויחו כל אנשי החבורה וחלקוהו |
|
ולדעת זה תניח מספר החבורה דבר |
|
ויצאו [60]שני שלישיה להרויח והם שלישי דבר |
|
והרויח הראשון דרהם אחד ונתוספו בשני דרהמי |
|
וצריך לפי מה שבארנו שיהיה מה שהרויחו שני השלישים ארבעה תשיעיות מאלגו |
|
והשליש האחר הרויח הראשון מהם שני דרהמי ונתוספו הארבעה |
|
וצריך כפי מה שבארנו שיהיה מה שהרויח השלישית שתי תשיעיות מאלגו |
|
ותקבצם עם אשר הרויחו השני שלישים והוא ארבעה תשיעיות ויהיה כל אשר הרויחו כל אנשי החבורה שני שלישיות מאלגו |
|
ותחלקהו על מספר החבורה שהוא דבר ויהיה עשרים |
|
ותכה עשרים בדבר ויהיה עשרים דברים ישוו שני שלישי אלגו |
|
והאלגו ישוה שלשים דברים |
|
והדבר שלשים והוא מספר החבורה |
|
נד ואם יאמרו לך חבורה מאנשים יצאו להרויח והרויח הראשון דרהם אחד ונתוספו באחד וכאשר שבו קבצו יחד כל מה שהרויחו כלם וחלקוהו ביניהם בשוה והגיע לכל אחד מהם כמו שני שלישי מספר החבורה |
|
וצריך |
|
שיהיה כמו שבארנו כל מה שהרויחו בם חצי אלגו וחצי דבר |
|
ונחלקהו על מספר החבורה והוא דבר ויהיה שני שלישי דבר |
|
ותכה שני שלישי דבר בדבר ויהיה שני שלישי אלגו ישוה חצי אלגו וחצי דבר |
|
והדבר ישוה שלשה דרהמי והוא מספר החבורה |
|
נה ואם יאמרו לך חבורה שיצאו ארבעה שרשיה וחצי שרש להרויח והרויח הראשון דרהם אחד ונתוספו באדרהם אחד וכאשר שבו חלקו מה שהרויחו בין כל החבורה בשוה והגיע לכל אחד מהם עשרה דרהמי וחצי כמה יהיה מספר החבורה |
|
ולדעת זה תניח מספר החבורה |
|
ויצאו ארבעת שרשיו וחצי והוא ארבעה דברי' וחצי |
|
והריוח הראשון דרהם אחד ונתוספו באדרהם אחד |
|
וצריך שיהיה מה שהרויחו כפי מה שבארנו עשרה אלגוש ושמינית מאלגו ושני דברים ורביע דבר |
|
ונחלקם על מספר החבורה והוא אלגו ויצא לחלק עשרה דרהמי וחצי |
|
ותכה עשרה דרהמי וחצי באלגו ויהיה עשרה אלגוש וחצי ישוו עשרה אלגוש ושמינית מאלגו ושני דברים ורביע |
|
|
|
ותכוין עמהם ויהיה הדבר [61]ששה |
|
והאלגו שלשים וששה והוא מספר החבורה |
|
נו ואם יאמרו לך שלשים אנשים הרויח הראשון דרהם אחד ונתוספו בדבר אחד והיה כל אשר הרויחו אלף ושלש מאות ושלשים וחמשה בכמה נתוספו כל אחד על האחר אשר לפניו |
|
ולדעת זה תניח השני שהוסיף על הראשון בדבר אחד והשלישי על השני בדבר אחד והרביעי על השלישי בדבר אחד וכן עד האחרון |
|
ותעשה בהם כאשר בארתי והוא שתכה מה |
|
| |
|
ותוסיף עליו מה שהרויח הראשון והוא דרהם אחד ויהיה כ"ט דברים ושני דרהמי והוא מה שהרויח כל |
|
| |
|
ותכם בחמש עשרה שהוא מחצית החבורה ויהיה שלשים דרהמי ותל"ה דברים והוא כל אשר הרויחו א"י אלוגלו ויהיה אלף ושלש מאות ושלשים וחמשה דרהמי |
|
| |
|
|
|
ותכוין עמהם ויהיה הדבר שלשה והוא |
Motion Problems |
|
|
נז ואם יאמרו לך שלחתי איש אחד מן העיר וצויתיו שילך [ביום ראשון][62] ואחר זה שלחתי איש אחר וצויתיו שילך [ביום ראשון][63] |
|
וידיעת זה היא שתניח מספר הימי' הראשונים שהלך האיש הראשון דבר |
|
וצריך כפי מה שבארנו בחבורה שיהיה מספר הפרסאות שהלך חצי אלגו וחצי דבר |
|
ויהיה מה שהלך השני מהימים דבר פחות פ"ד ימים |
|
וצריך שיהיה מספר הפרסאות כפי מה שבארנו בחבורה אלגו ושבעה אלפים וחמשים וששה פחות קס"ח דברים ויהיה שוה חצי אלגו וחצי דבר |
|
| |
|
|
|
ותכוין עמהם ויהיה הדבר מאתים ושמנים ושמנה והוא מה שהלך האיש הראשון |
|
והלך השני מאתים וארבעה ימים |
|
[64]נח ואם יאמרו לך צויתי לראשון שילך ביום ראשון פרסא אחת ושיוסיף בכל יום פרסא אחת והלך שמנה ימים וצויתי לשני שילך בכל יום הראשון פרסא אחת ושיוסיף בכל יום שלשה פרסאות |
|
ולדעת זה תניח מספר הימים שהלך הראשון דבר |
|
וצריך כפי מה שבארנו בחבורה שיהיה מספר הפרסאות חצי אלגו וחצי דבר |
|
ויהיה מה שהלך השני מן הימים דבר פחות שמנה |
|
וצריך שיהיה מספר פרסותיו אלגו וחצי ומאה דרהמי פחות כ"ד דברים וחצי והוא ישוה חצי אלגו וחצי דבר |
|
| |
|
|
|
ותכוין עמהם ויהיה הדבר עשרים ימים והוא מה שהלך הראשון |
|
ומה שהלך השני הוא שנים עשר ימים |
|
נט ואם יאמרו לך איש אחד עלה מן העיר וצויתי ושלחתי לאיש אחר אחר זה וצויתיו שילך בכל יום שרש מספר הפרסאות שיפגע בהם הראשון |
|
וידיעתו שתניח מה שהלך הראשון מן הפרסאות אלגו |
|
וצריך שיהיה מספר הימים שהלך חצי עשירית מאלגו |
|
והאיש השני הלך פחות מהראשון בחמשה עשר ימים ויהיה מספר ימיו חצי עשירית מאלגו פחות ט"ו ימים |
|
ותכם במה שהלך בכל יום והוא דבר בעבור שצוה ללכת בכל יום שרש מספר הפרסאות שימצאהו בהם ומספר הפרסאות הוא אלגו ושרשו דבר ויהיה חצי עשירית ממעוקב דיקוביקא בלעז פחות חמשה עשר דברים ישוה אלגו |
|
| |
|
|
|
ותכוין עמהם ויהיה אלגו ישוה שלש מאות דרהמי ועשרים דברים |
|
והדבר ישוה שלשים והוא שרש מספר הפרסאות שהלך הראשון |
|
ותכם בעצמם ויהיה תשע מאות פרסאות |
|
ותחלקם על הפרסאות שהלך בכל יום והם עשרים ויעלה לחלק מ"ה ימים והם ימי השני |
|
ס ואם יאמרו לך איש אחד עלה מן העיר וצויתיו שילך בכל יום עשרים פרסאות והלך מ"ה ימים ושלחתי אחר זה אחר וצויתיו שילך בכל יום שרש מאשר הלך הראשון מהימים שימצאהו בהם |
|
וידיעתו שתניח בכל יום שרש מאשר הלך הראשון מהימים אלגו |
|
ויהיה מספר פרסותיו שהלך עשרים אלגוש |
|
[65]ויהיה מספר ימי השני אלגו פחות מ"ה ימים |
|
ותכם במה שהלך כל יום והוא דבר והנה קוביקא פחות מ"ה דברים ישוה עשרים אלגו |
|
|
|
ותכוין עמהם ויהיה הדבר עשרים ושנים והוא שרש הימים שהלך הראשון |
|
ותכם בעצמם ויהיה תפ"ד והם הימים שהלך הראשון |
|
ותכם בעשרים ויהיה תשעה אלפים ותר"פ והוא מספר הפרסאות שהלך |
|
ומספר אשר מהשני ת"מ |
|
ותכם בפרסאות שהלך כל יום והם כ"ב ויהיו תשעה אלפים ותר"פ |
Purchase Problems |
|
|
סא ואם יאמרו לך אונקיא אחת בעד עשרים דרהמי ואונקיא אחת בעד ט"ו דרהמי ואונקיא בעד עשרה ואמרו לך תקח מהכל אונקי' אחת בעד י"ח דרהמי |
|
זאת השאלה היא חרשת |
|
וידיעתה שתקח דבר מהאונקיא שהיא בעד עשרה דברים מהדרהמי ודבר מהאונקיא שהיא בעד ט"ו דברים מהדרהמי |
|
וישאר מהאונקיא אונקיא פחות שני דברים |
|
ומהדרהמי י"ח דרהמי פחות כ"ה דברים |
|
ותקח מאונקיא שהיא בעד עשרים אונקיא פחות שני דברים בעד עשרים דרהמי פחות ארבעים דברים |
|
ויהיה י"ח דרהמי פחות כ"ה דברים ישוה עשרים דרהמי פחות ארבעים דברים |
|
|
|
ותכוין עמהם ויהיה הדבר ישוה שני שלישי החומש |
|
ומאשר היא בעד ט"ו מכל אחת ב' מט"ו מאונקיא |
|
|
|
ואם תרצה תקח דבר מהאונקיא שהיא בעד עשרה דברים מהדרהמי ותקח שני דברים מהאונקיא שהיא בעד ט"ו דברים מהדרהמי |
|
וישאר ערך האונקיא י"ח דרהמי פחות ארבעים דברים |
|
ומהאונקיא אונקיא פחות שלשה דברים |
|
וקנה בהם מהאונקיא שהיא בעד עשרים דרהמי בעד עשרים דרהמי פחות שני דברים |
|
והדבר ישוה י"ו דרהמי פחות ארבעים דברים |
|
|
|
ותכוין עמהם ויהיה הדבר עשרה ותקח מהאונקיא שהיא בעד עשרה עשירית אחד מאונקיא |
|
ומאשר היא בעד ט"ו חמישית אחד מאונקיא |
|
ומאשר היא בעד עשרים שבעה עשיריות מאונקיא וחצי וחומש מאונקיא |
|
[66]סב ואם יאמרו לך ליט' אחת בעד חמשה דרהמי וליט' אחת בעד ארבעה דרהמי ועשרה ליט' בעד דרהם אחד תקח מהכל ליט' אחת בעד שני דרהמי |
|
זאת השאלה היא חרשת |
|
וידיעתו שתניח דבר מהליט' שהיא בעד חמשה דרהמי בעד חמשה דברים מהשני דרהמי ודבר מהליט' שהיא בעד ארבעה דרהמי בעד ארבעה דברים משני הדרהמי |
|
וישאר משני הדרהמי שני דרהמי פחות תשעה דברים ישוה עשרה דרהמי פחות חמישית דבר |
|
|
|
ותכוין עמהם ויהיה הדבר ט' מפ"ח בליט' |
|
ואם תרצה תקח מהליט' שהיא בעד חמשה דברים דבר בחמשה דברים משני הדרהמי |
|
וישאר משני הדרהמי שני דרהמי פחות כ"ה דברים |
|
ומהליט' ליט' פחות ששה דברים |
|
ותקח מאשר הוא עשרה ליט' בעד דרהם אחד עם עשרה דרהמי פחות שלשה חומשי דבר |
|
ויהיה אז שני דרהמי פחות כ"ה דברי' ישוה עשרה דרהמי פחות שני חומשי דבר |
|
|
|
ותכוין עמו ויהיה הדבר י"ט מרמ"ד והוא מה שקנה מהליט' אשר בעד חמשה דרהמי |
|
וקנה מהליט' אשר בעד ארבעה דרהמי צ"ה מרפ"ד בליט' |
|
ואם תרצה תקח מהליט' שהיא בעד חמשה ג' מעשרה בליט' ושני חומשים מעשירית הליט' בעד דרהם אחד וחצי וחומש ומהליט' שהיא בעד ארבעה דרהמי שלשה מחמשים בליט' בעד חמשה דרהמי ושנים מחמשים באדרהם ומהליט' שהיא עשרה ליט' בעד דרהם אחד שלשה חומשים מליט' בעד שלשה מחמשים בדרהם |
Buy and Sell Problems |
|
|
סג ואם יאמרו לך קניתי בעד עשרה דרהמי חטה לחשבון הקאפיזיש בעד דרהם אחד וקניתי עם שלשה דרהמי גולגולאן לחשבון בלתי ידוע |
|
וידיעתו שתקנה ל' קאפיזיש מחטה בעד עשרה דרהמי לחשבון שלשה קאפיזיש בעד דרהם אחד |
|
וקניתי עם שלשה דרהמי גולגולאן לחשבון דבר אחד בעד דרהם אחד ויהיה שלשה דברים |
|
ושלשת הדברים לחשבון שלשה דרהמי ויעלה שוויו דבר |
|
וישאר מהקרן ומהריוח שבעה עשר [67]פחות דבר והוא שווי החטה כאשר מכרוהו בכלל הגולגולאן |
|
תכה דבר אחד בי"ז דרהמי פחות דבר ויהיה י"ז דברים פחות אלגו ישוה שלשים |
|
|
|
וכוין עמו ויהיה הדבר אם תרצה ט"ו ואם תרצה שנים ואמור הכלל מהגולגולאן אם תרצה ט"ו קאפיזיש ואם תרצה שנים |
| וכאשר יקרה לפניך שאלה כזאת או דומה אליה אם יחלק הקרן והריוח או הקרן פחות | |
|
סד ואם יאמרו לך קאפיזיש בלתי ידועים שוויים צ"ג דרהמי אלא |
|
וידיעתו שתניח שווי הקאפיז דבר |
|
ישאר מספר הקאפיזיש ל"ד פחות דבר |
|
תכה דבר בל"ד פחות דבר יהיה ל"ד דברים פחות אלגו ישוה צ"ג דרהמי |
|
|
|
ותכוין ויהיה הדבר שלשה והוא שווי הקאפיז |
|
והקאפיזיש ל"א |
|
ואם תרצה תניח הקאפיזיש שלשה |
|
ושווי הקאפיז ל"א |
|
סה ואם יאמרו לך קאפיזיש בלתי ידועים מכר הראשון בעד דרהם אחד והוסיפו בדרהם אחד ולקח שרש הכל והוסיפהו על מספרו ויצא י"ד |
|
חכמתו שתניח מספר הקאפיזיש דבר |
|
וצריך שיהיה שוויו כפי מה שבארנו בחבורות חצי אלגו וחצי דבר |
|
ושרשו נוסף על דבר יהיה י"ד |
|
ותגרע דבר מי"ד ישאר י"ד פחות דבר ישוה שרש מחצי אלגו וחצי דבר |
|
תכה י"ד פחות דבר בעצמו יהיה[68] קצ"ו דרהמי' ואלגו פחות כ"ח דברים ישוה חצי אלגו וחצי דבר |
|
|
|
ותכוין בהם ויהיה הדבר שמנה והם הקאפיזיש |
Apparatus
- ↑ 63v
- ↑ 64r
- ↑ 64v
- ↑ marg.: ר"ל שרש הנשאר
- ↑ marg.
- ↑ 65r
- ↑ 65v
- ↑ 66r
- ↑ 66v
- ↑ 67r
- ↑ 67v
- ↑ marg.
- ↑ 68r
- ↑ 68v
- ↑ 69r
- ↑ 69v
- ↑ 70r
- ↑ 70v
- ↑ Ma: שיבי שוש
- ↑ 71r
- ↑ Ma.: המתתקבץ
- ↑ 71v
- ↑ Ma.: כחלק
- ↑ 72r
- ↑ 72v
- ↑ 73r
- ↑ 73v
- ↑ 74r
- ↑ 74v
- ↑ Ma.: כמה
- ↑ 75r
- ↑ 75v
- ↑ 76r
- ↑ 76v
- ↑ 77r
- ↑ 77v
- ↑ 78r
- ↑ Ma. marg.
- ↑ Ma. marg.: ויהיה ערך הבהמה ישוב גם כן דבר ושני שלישי דבר ושני שלישי דרהם ושני שלישי פלס
- ↑ 78v
- ↑ Ma. marg.
- ↑ 79r
- ↑ 79v
- ↑ Ma. marg.
- ↑ Ma. marg.
- ↑ 80r
- ↑ Ma. marg
- ↑ 80v
- ↑ 81r
- ↑ Ma. om.
- ↑ 81v
- ↑ 82r
- ↑ 82v
- ↑ Ma. marg.
- ↑ 83r
- ↑ 83v
- ↑ Ma. marg.
- ↑ 84v
- ↑ 84v
- ↑ 85r
- ↑ 85v
- ↑ marg.
- ↑ marg.
- ↑ 112r
- ↑ 112v
- ↑ 113r
- ↑ 113v
- ↑ יהיה: MS Mu ויעלה
Appendix: Bibliography
Kitāb Masā’il allatī hiya ghair Maḥdūda (The third section on indeterminate equations) / by Abū Kāmil Shujāʽ Ibn Aslam Ibn Muḥammad ibn Shujāʽ (Egypt, ca. 850-930)
– Hebrew translation –
by Mordecai (Angelo) Finzi (Mantua, d. 1475)
Ḥeshbon ha-ʽAgulah (ha-Sheʼelot ha-Ḥershot ve-ha-ʼIlmot)
Manuscripts:
- 1) Mantova, Comunita Israelitica MS ebr. 17/3 (IMHM: f 797), ff. 63v-85v; 112r-113v (15th century; autograph)
- Abu Kamil Shudeya, Algebra
- 2) München, Bayerische Staatsbibliothek, Cod. hebr. 225/3 (IMHM: f 1118), ff. 166v-191v (15th century)
- Mathematische Abhandlungen, vielleicht von Mordechai Finzi (1445-1473)
The transcript is based mainly on manuscript Mantova 17
Bibliography:
- Lévy, Tony. 2007. L’algèbre arabe dans les textes hébraïques (II). Dans l’Italie des XVe et XVIe siècles, sources arabes et sources vernaculaires, Arabic Sciences and Philosophy 17, pp. 81-107.
- Schub, Pincus and Martin Levey. 1968. Book on Indeterminate Problems of abū Kāmil (850-930), Centaurus 13, pp. 91-94.
- ———. 1970. Indeterminate Problems of abū Kāmil (850-930), Atti della Accademia Nazionale dei Lincei, Memorie della classe di scienze fisiche, matematiche e naturali, ser. VIII, vol. X, no.2, pp. 23-96.
- Sesiano, Jacques. 1977. Les Méthodes d'analyse indéterminée chez Abū Kāmil, Centaurus 21, pp. 89-105.
- Yadegari, Mohammad. 1978. The Use of Mathematical Induction by Abū Kāmil Shujā‘ Ibn Aslam (850-930), Isis, vol. 69, no. 2 (Jun., 1978), pp. 259-262.
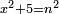
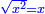 ].
].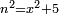
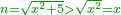

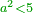

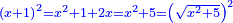
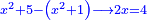

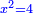
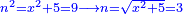
 ].
].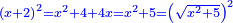

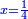
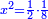

 ].
]. ].
].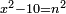

 ].
].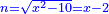 ].
].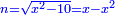 ]
]
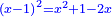
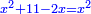

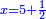
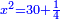
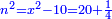
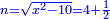
 ].
].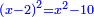
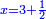
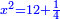
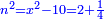
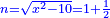
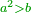 ].
].

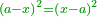
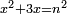
 ].
].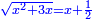 ].
]. ].
].

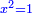

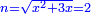

 ].
].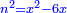

 ].
]. ].
].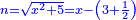 ].
].
 ].
].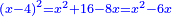
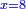


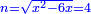
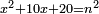
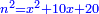
 ].
].
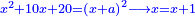
 ].
].![\scriptstyle{\color{blue}{\left[x+\left(4+\frac{1}{2}\right)\right]^2=x^2+9x+\left(20+\frac{1}{4}\right)=x^2+10x+20}}](/mediawiki/images/math/d/6/1/d61c27001c69cfd61e32bde797def6d8.png)

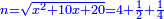
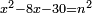
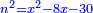
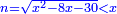 ].
].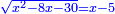 ].
].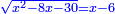 ].
].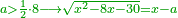
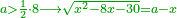
 ].
].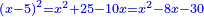


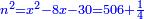
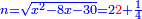
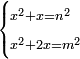







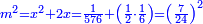
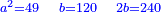
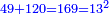

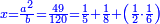

![\scriptstyle{\color{blue}{n^2=x^2+x=\frac{2401}{14400}+\left[\frac{1}{5}+\frac{1}{8}+\left(\frac{1}{2}\sdot\frac{1}{6}\right)\right]=\frac{2401}{14400}+\frac{5880}{14400}=\frac{8281}{14400}=\left(\frac{91}{120}\right)^2}}](/mediawiki/images/math/7/3/f/73f3712df4c06c99e4c853174d081e93.png)
![\scriptstyle{\color{blue}{m^2=x^2+2x=\frac{2401}{14400}+2\sdot\left[\frac{1}{5}+\frac{1}{8}+\left(\frac{1}{2}\sdot\frac{1}{6}\right)\right]=\frac{2401}{14400}+\frac{11760}{14400}=\frac{14161}{14400}=\left(\frac{1{\color{red}{1}}9}{120}\right)^2}}](/mediawiki/images/math/4/5/3/4530152c3a593663fa00ad6e78bbffca.png)

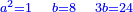
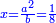
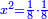
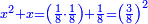
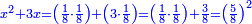
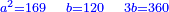
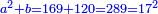
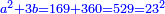

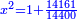
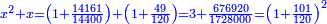
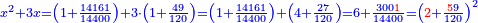
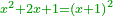
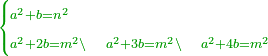
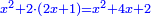
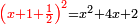 ]
]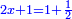
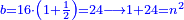
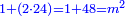
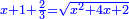
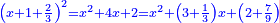

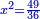

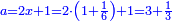

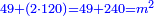
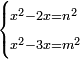
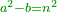 ]; and when you subtract from it that number and its half, the remainder has a root [
]; and when you subtract from it that number and its half, the remainder has a root [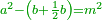 ]
]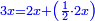


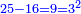
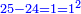
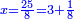
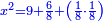
![\scriptstyle{\color{blue}{x^2-2x=\left[9+\frac{6}{8}+\left(\frac{1}{8}\sdot\frac{1}{8}\right)\right]-\left(6+\frac{1}{4}\right)=3+\frac{1}{2}+\left(\frac{1}{8}\sdot\frac{1}{8}\right)=\left(1+\frac{7}{8}\right)^2}}](/mediawiki/images/math/c/0/3/c03a1cf27555e36dcbecb26ac89bc566.png)
![\scriptstyle{\color{blue}{x^2-3x=\left[9+\frac{6}{8}+\left(\frac{1}{8}\sdot\frac{1}{8}\right)\right]-\left(9+\frac{3}{8}\right)=\frac{3}{8}+\left(\frac{1}{8}\sdot\frac{1}{8}\right)=\left(\frac{5}{8}\right)^2}}](/mediawiki/images/math/2/f/f/2ffcf247f479965c00a42cb9a8e7da3e.png)
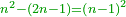
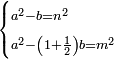
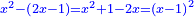
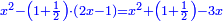
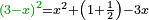 ]
]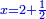
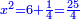
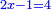
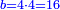
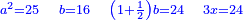
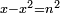
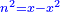 ] and it should have a root.
] and it should have a root. ].
].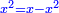
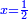
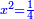
 ].
].

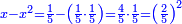
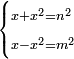
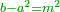 ]
]
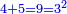
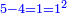



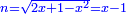
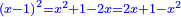

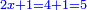
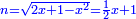
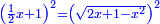
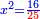
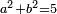

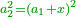


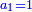
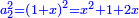
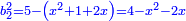
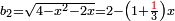
![\scriptstyle{\color{blue}{\left[2-\left(1+\frac{1}{3}\right)x\right]^2=4+\left(1+\frac{7}{9}\right)x^2-\left(5+\frac{1}{3}\right)x=4-x^2-2x}}](/mediawiki/images/math/9/b/7/9b7e0a4026ebdb50fdbcc7cc6f657579.png)
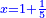
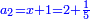
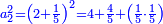
![\scriptstyle{\color{blue}{b_2^2=5-\left[4+\frac{4}{5}+\left(\frac{1}{5}\sdot\frac{1}{5}\right)\right]=\frac{4}{5}\sdot\frac{1}{5}}}](/mediawiki/images/math/6/4/e/64e0e6c4fa14315a7a248466f7d88902.png)
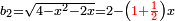
![\scriptstyle{\color{blue}{\left[2-\left(1+\frac{1}{2}\right)x\right]^2=\left(\sqrt{4-x^2-2x}\right)^2}}](/mediawiki/images/math/d/5/2/d5242221c565a5101c58589c2a027d2b.png)
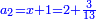
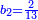
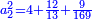
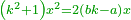
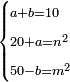
 ].
].
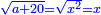
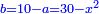

 ], for this number has many [solutions].
], for this number has many [solutions].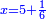

![\scriptstyle{\color{blue}{a=x^2-20=\left[26+\frac{2}{3}+\left(\frac{1}{4}\sdot\frac{1}{9}\right)\right]-20=6+\frac{2}{3}+\left(\frac{1}{4}\sdot\frac{1}{9}\right)}}](/mediawiki/images/math/8/5/5/85534b870bf3086a19ef6900f48dd7e2.png)

![\scriptstyle{\color{blue}{b=10-a=10-\left[6+\frac{2}{3}+\left(\frac{1}{4}\sdot\frac{1}{9}\right)\right]=3+\frac{1}{4}+\left(\frac{1}{2}\sdot\frac{1}{9}\right)}}](/mediawiki/images/math/d/8/f/d8f46212272c5cee1c22a34e2aafc751.png)
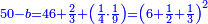
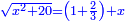
![\scriptstyle{\color{blue}{\left[\left(1+\frac{2}{3}\right)+x\right]^2=\left(2+\frac{7}{9}\right)+x^2+\left(3+\frac{1}{3}\right)x=x^2+20}}](/mediawiki/images/math/d/3/4/d34d66eca4f46d4e0db30bf1fe034158.png)
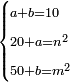

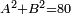
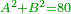

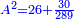



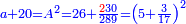
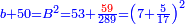
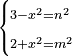

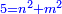
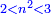

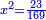

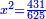
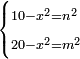

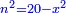

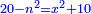
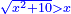



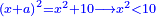
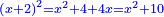


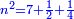
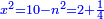

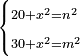
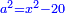
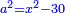





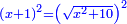


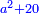 has a root
has a root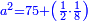
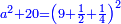
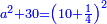
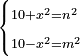

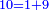
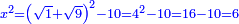
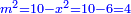
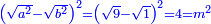
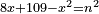
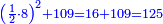


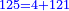
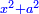
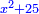
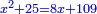
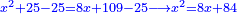
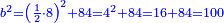

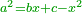

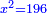
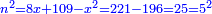
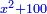
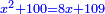
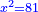

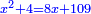
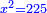
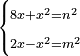


 ] and the two roots are four things and 16 dirham [
] and the two roots are four things and 16 dirham [ ]
]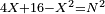
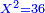

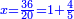
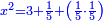
![\scriptstyle{\color{blue}{8x+x^2=\left(14+\frac{2}{5}\right)+\left[3+\frac{1}{5}+\left(\frac{1}{5}\sdot\frac{1}{5}\right)\right]=17+\frac{3}{5}+\left(\frac{1}{5}\sdot\frac{1}{5}\right)=\left(4+\frac{1}{5}\right)^2}}](/mediawiki/images/math/8/6/1/861834c2507163fc3c2737d99ce02c8f.png)
![\scriptstyle{\color{blue}{2x-x^2=\left(3+\frac{3}{5}\right)-\left[3+\frac{1}{5}+\left(\frac{1}{5}\sdot\frac{1}{5}\right)\right]=\frac{1}{5}+\left(\frac{4}{5}\sdot\frac{1}{5}\right)=\left(\frac{3}{5}\right)^2}}](/mediawiki/images/math/c/f/a/cfa723873960216653ec6f59eda554bd.png)
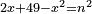

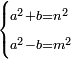

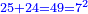
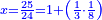
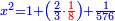
![\scriptstyle{\color{blue}{x^2+x=\left[1+\left(\frac{2}{3}{\color{red}{\sdot\frac{1}{8}}}\right)+\frac{1}{576}\right]+\left[1+\left({\color{red}{\frac{1}{3}\sdot\frac{1}{8}}}\right)\right]=2+\frac{1}{8}+\frac{1}{576}=\left(1+\frac{1}{3}+\frac{1}{8}\right)^2}}](/mediawiki/images/math/f/f/a/ffa71821370a9825ecc3837e29ab06ee.png)
![\scriptstyle{\color{blue}{x^2-x=\left[1+\left(\frac{2}{3}{\color{red}{\sdot\frac{1}{8}}}\right)+\frac{1}{576}\right]-\left[1+\left({\color{red}{\frac{1}{3}\sdot\frac{1}{8}}}\right)\right]=\frac{25}{576}=\left[\frac{1}{8}+\left(\frac{1}{2}\sdot\frac{1}{6}\right)\right]^2}}](/mediawiki/images/math/3/a/c/3ac5a85142988d84b5408ff369da3bfc.png)
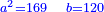
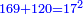
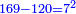
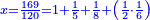
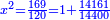
![\scriptstyle{\color{blue}{x^2+x=\left(1+\frac{14161}{14400}\right)+\left[1+\frac{1}{5}+\frac{1}{8}+\left({\color{red}{\frac{1}{2}\sdot\frac{1}{6}}}\right)\right]=3+\frac{5641}{14400}=\left(1+\frac{{\color{red}{101}}}{120}\right)^2}}](/mediawiki/images/math/5/f/c/5fc6fd6e9b2c0106bbea702b9143a332.png)
![\scriptstyle{\color{blue}{x^2-x=\left(1+\frac{14161}{14400}\right)-\left[1+\frac{1}{5}+\frac{1}{8}+\left(\frac{1}{2}\sdot\frac{1}{6}\right)\right]=\frac{8281}{14400}=\left(\frac{91}{120}\right)^2}}](/mediawiki/images/math/4/d/2/4d29ed2a234190752a72bbff0b85ddea.png)
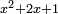

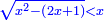
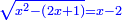
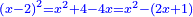
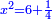
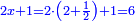
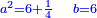
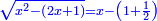


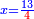
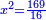
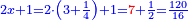
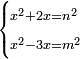
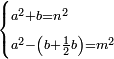
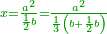
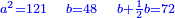
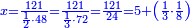

![\scriptstyle{\color{blue}{x^2+2x=\left(25+\frac{1}{4}{\color{red}{+}}\frac{1}{6}+\frac{1}{576}\right)+\left[10+\left(\frac{1}{2}\sdot\frac{1}{6}\right)\right]=35+{\color{red}{\frac{1}{2}}}+\frac{1}{576}=\left(5+\frac{1}{2}+\frac{1}{3}{\color{red}{+}}\frac{1}{8}\right)^2}}](/mediawiki/images/math/0/2/3/02376f4630c644e6b455f78d4028fbe8.png)
![\scriptstyle{\color{blue}{x^2-3x=\left(25+\frac{1}{4}{\color{red}{+}}\frac{1}{6}+\frac{1}{576}\right)-\left(15+\frac{1}{8}\right)=10+\frac{1}{6}+\frac{1}{8}+\frac{1}{576}=\left[3+\frac{1}{8}+\left(\frac{1}{2}\sdot\frac{1}{6}\right)\right]^2}}](/mediawiki/images/math/3/4/9/34973b92cc89f94c2dd83a45f67fc787.png)

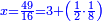

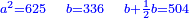
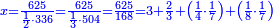
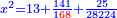
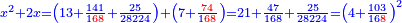
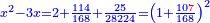

![\scriptstyle\begin{cases}\scriptstyle x^2+2x+1=n^2\\\scriptstyle x^2-\left[3x+\left(1+\frac{1}{2}\right)\right]=m^2\end{cases}](/mediawiki/images/math/0/f/8/0f807ab541011f16cb21bd6c3494d4f7.png)
![\scriptstyle{\color{blue}{x^2-\left[3x+\left(1+{\color{red}{\frac{1}{2}}}\right)\right]}}](/mediawiki/images/math/b/d/a/bdae3ffbff309541b227e49897d292a2.png)
![\scriptstyle{\color{blue}{\sqrt{x^2-\left[3x+\left(1+\frac{1}{2}\right)\right]}={\color{red}{x}}-2}}](/mediawiki/images/math/0/2/9/029ebb28798fbc22c3e2a27a27934f82.png)
![\scriptstyle{\color{blue}{\left(x-2\right)^2=x^2+4-4x=x^2-\left[3x+\left(1+\frac{1}{2}\right)\right]}}](/mediawiki/images/math/d/7/5/d75f2296670d877cd7c3134b78dd0156.png)
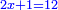
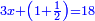
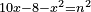
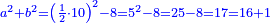



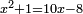




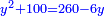
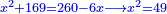

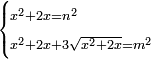

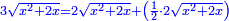
![\scriptstyle{\color{blue}{\left[\left(1+\frac{1}{2}\right)x\right]^2=\left(\sqrt{x^2+2x}\right)^2=\left(2+\frac{1}{4}\right)x^2=x^2+2x}}](/mediawiki/images/math/d/b/a/dba26db6a10a1b307757d237da47e8c1.png)
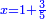
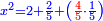
![\scriptstyle{\color{blue}{x^2+2x=\left[2+\frac{2}{5}+\left(\frac{4}{5}\sdot\frac{1}{5}\right)\right]+\left(3+\frac{1}{5}\right)=5+\frac{3}{5}+\left(\frac{4}{5}\sdot\frac{1}{5}\right)}}](/mediawiki/images/math/0/c/e/0ce207462169165bac229f56bebe1530.png)
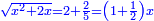
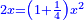

![\scriptstyle{\color{blue}{x^2+2x+3\sqrt{x^2+2x}=\left[5+\frac{3}{5}+\left(\frac{4}{5}\sdot\frac{1}{5}\right)\right]+\left(7+\frac{1}{5}\right)=12+\frac{4}{5}+\left(\frac{4}{5}\sdot\frac{1}{5}\right)}}](/mediawiki/images/math/2/3/d/23dc373d6e5d726525de55348156a214.png)

![\scriptstyle{\color{blue}{\left(4+\frac{1}{2}\right)\sdot\sqrt{12+\frac{4}{5}+\left(\frac{4}{5}\sdot\frac{1}{5}\right)}=16+\frac{1}{5}=\left(1+\frac{1}{4}\right)\sdot\left[12+\frac{4}{5}+\left(\frac{4}{5}\sdot\frac{1}{5}\right)\right]}}](/mediawiki/images/math/0/f/b/0fb00edbe0c49653c269bc806b09ba81.png)
![\scriptstyle{\color{blue}{\left(16+\frac{1}{5}\right)+\left[12+\frac{4}{5}+\left(\frac{4}{5}\sdot\frac{1}{5}\right)\right]=29+\left(\frac{4}{5}\sdot\frac{1}{5}\right)}}](/mediawiki/images/math/e/e/6/ee60d81ab41aa4bb558d48486fc39b85.png)
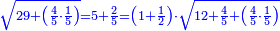
![\scriptstyle{\color{blue}{\sqrt{\left[29+\left(\frac{4}{5}\sdot\frac{1}{5}\right)\right]+\left(6+\frac{3}{4}\right)\sdot\sqrt{29+\left(\frac{4}{5}\sdot\frac{1}{5}\right)}}={\color{red}{8+\frac{1}{10}}}=\left(1+\frac{1}{2}\right)\sdot\sqrt{29+\left(\frac{4}{5}\sdot\frac{1}{5}\right)}}}](/mediawiki/images/math/8/2/2/8223f615278100b4cc79700048344b9d.png)
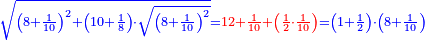
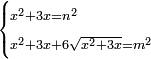

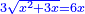
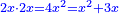

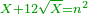

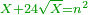
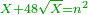
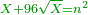
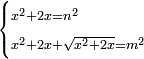
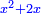 ].
].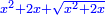 ], which should have a root.
], which should have a root.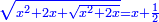 ].
].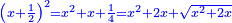
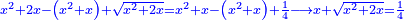
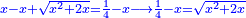
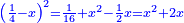
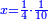
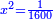
 ], so that the square will be consumed and the things remain equal to numbers.
], so that the square will be consumed and the things remain equal to numbers.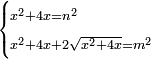
 ].
]. ].
].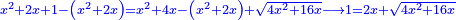
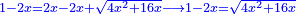

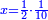
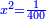
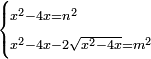
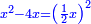 ].
].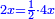
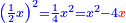
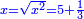


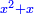 ], and it has a root.
], and it has a root.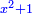 ], and it has a root.
], and it has a root.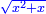 ] and a root of the square and one dirham minus half a dirham [
] and a root of the square and one dirham minus half a dirham [ ].
].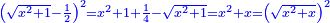

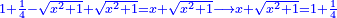
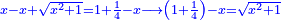
![\scriptstyle{\color{blue}{\left[\left(1+\frac{1}{4}\right)-x\right]^2=\left[1+\frac{1}{2}+\left(\frac{1}{2}\sdot\frac{1}{8}\right)\right]+{\color{red}{x^2}}-\left(2+\frac{1}{2}\right)x=x^2+1}}](/mediawiki/images/math/5/e/2/5e27627f6c6c937822a771e33d0d599e.png)
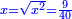
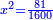
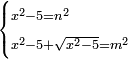
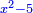 ] remain.
] remain.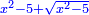 ].
].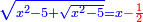 ]
]
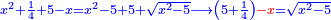
![\scriptstyle{\color{blue}{\left[\left(5+\frac{1}{4}\right){\color{red}{-x}}\right]^2=\left[27+\frac{1}{2}+\left(\frac{1}{2}\sdot\frac{1}{8}\right)\right]+x^2-\left(10+\frac{1}{2}\right)x=x^2-5}}](/mediawiki/images/math/1/a/1/1a162a3608f582636e951adab50f7268.png)
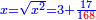
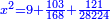
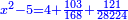

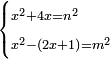
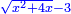 ], as I explained to you.
], as I explained to you. 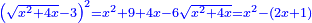
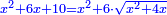

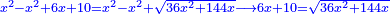
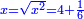
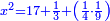
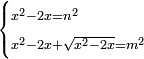
 ] remain.
] remain.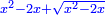 ] that has a root.
] that has a root. ].
].![\scriptstyle{\color{blue}{\left[x-\left(1+\frac{1}{2}\right)\right]^2=x^2+\left(2+\frac{1}{4}\right)-3x=x^2-2x+\sqrt{x^2-2x}}}](/mediawiki/images/math/5/7/8/578cde2a1b1a382f626a79f854011398.png)

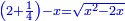
![\scriptstyle{\color{blue}{\left[\left(2+\frac{1}{4}\right)-x\right]^2=\left[5+\left(\frac{1}{2}\sdot\frac{1}{8}\right)\right]+x^2-\left(4+\frac{1}{2}\right)x=x^2-2x}}](/mediawiki/images/math/3/a/b/3ab8e3fd1c823ed525fe1f8fc11a01fb.png)
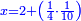
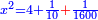
![\scriptstyle{\color{blue}{x^2-2x=\left(4+\frac{1}{10}+\frac{1}{1600}\right)-\left[4+\left(\frac{1}{2}\sdot\frac{1}{10}\right)\right]=\left(\frac{1}{2}\sdot\frac{1}{10}\right)+\frac{1}{1600}}}](/mediawiki/images/math/2/4/b/24b69a44800458f373d2fac0a331be86.png)
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle x^2-2x+\sqrt{x^2-2x}&\scriptstyle=\left[\left(\frac{1}{2}\sdot\frac{1}{10}\right)+\frac{1}{1600}\right]+\left[\frac{1}{5}+\left(\frac{1}{4}\sdot\frac{1}{10}\right)\right]=\frac{1}{4}+\left(\frac{1}{4}\sdot\frac{1}{10}\right)+\frac{1}{1600}\\&\scriptstyle=\left[\frac{1}{2}+\left(\frac{1}{4}\sdot\frac{1}{10}\right)\right]^2\\\end{align}}}](/mediawiki/images/math/0/2/7/0279c76dbd17cad87de002f3e29b422a.png)
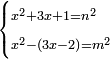
 ] that has a root.
] that has a root.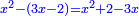 ] and it has a root.
] and it has a root. ].
].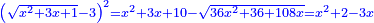

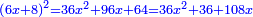
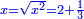
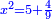
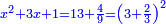
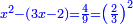
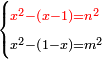
 ] that has a root.
] that has a root.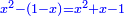 ] remains and it has a root.
] remains and it has a root.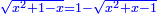 ]
]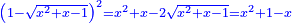
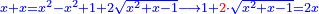



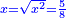
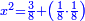
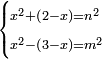
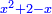 ] and it has a root.
] and it has a root. ] and it has a root.
] and it has a root.
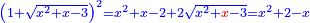
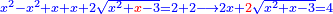


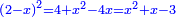
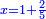
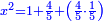
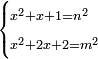
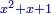 ] and it has a root.
] and it has a root.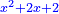 ]; it has a root.
]; it has a root.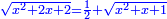
![\scriptstyle{\color{blue}{\left(x^2+2x+2\right)-\left[\left(x^2+x+1\right)+\frac{1}{4}\right]=x+\frac{3}{4}}}](/mediawiki/images/math/0/0/1/001debd2f5a29140f6dcd1edd66bd010.png)
![\scriptstyle{\color{blue}{\left(x+\frac{3}{4}\right)^2\longrightarrow x^2+\left(1+\frac{1}{2}\right)x+\left[\frac{1}{2}+\left(\frac{1}{2}\sdot\frac{1}{8}\right)\right]=x^2+x+{\color{red}{1}}}}](/mediawiki/images/math/7/3/f/73f31418f3f186d2bd7040380625d920.png)
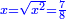

![\scriptstyle{\color{blue}{a+\left[\frac{1}{2}\sdot\left(b+c+d\right)\right]=b+\left[\frac{1}{3}\sdot\left(a+c+d\right)\right]=c+\left[\frac{1}{4}\sdot\left(a+b+d\right)\right]=d+\left[\frac{1}{5}\sdot\left(a+b+c\right)\right]}}](/mediawiki/images/math/a/e/4/ae49ae680d8337711b8246237b23fc23.png)
 ], the second a dinar [
], the second a dinar [ ], the third a dirham [
], the third a dirham [ ], and the fourth a fals [
], and the fourth a fals [ ]
]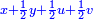
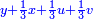
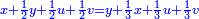
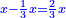
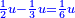
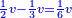

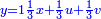
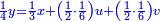
![\scriptstyle{\color{blue}{\frac{1}{5}y=\left[\frac{1}{5}+\left(\frac{1}{5}\sdot\frac{1}{3}\right)\right]x+\left(\frac{1}{5}\sdot\frac{1}{3}\right)u+\left(\frac{1}{5}\sdot\frac{1}{3}\right)v}}](/mediawiki/images/math/8/2/c/82ca068b5c2d020ba1dd90ba8e01b1c4.png)
![\scriptstyle{\color{blue}{x+\frac{1}{2}y+\frac{1}{2}u+\frac{1}{2}v=x+\left[\frac{2}{3}x+\frac{1}{6}u+\frac{1}{6}v\right]+\frac{1}{2}u+\frac{1}{2}v=1\frac{2}{3}x+\frac{2}{3}u+\frac{2}{3}v}}](/mediawiki/images/math/6/a/4/6a4db9ea14a01086466f9c2a966b7df0.png)
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle u+\frac{1}{4}x+\frac{1}{4}y+\frac{1}{4}v=u+\frac{1}{4}x+\left[\frac{1}{3}x+\left(\frac{1}{2}\sdot\frac{1}{6}\right)u+\left(\frac{1}{2}\sdot\frac{1}{6}\right)v\right]+\frac{1}{4}v&\scriptstyle=\left(\frac{1}{3}+\frac{1}{4}\right)x+\left[1+\left(\frac{1}{2}\sdot\frac{1}{6}\right)\right]u+\frac{1}{3}v\\&\scriptstyle=1\frac{2}{3}x+\frac{2}{3}u+\frac{2}{3}v\\\end{align}}}](/mediawiki/images/math/4/2/e/42e6cf6349bc90828f1577908f11ce24.png)
![\scriptstyle{\color{blue}{\left[\left(1+\frac{2}{3}\right)-\left(\frac{1}{3}+\frac{1}{4}\right)\right]x+\left(\frac{2}{3}-\frac{1}{3}\right)v=\left[1+\left(\frac{1}{2}\sdot\frac{1}{6}\right)\right]x+\frac{1}{3}v}}](/mediawiki/images/math/0/f/a/0fa8392cf6b231033609e8643afb272a.png)
![\scriptstyle{\color{blue}{\left[\left[1+\left(\frac{1}{2}\sdot\frac{1}{6}\right)\right]-\frac{2}{3}\right]u=\left(\frac{1}{4}+\frac{1}{6}\right)u=\left[1+\left(\frac{1}{2}\sdot\frac{1}{6}\right)\right]x+\frac{1}{3}v}}](/mediawiki/images/math/1/5/7/157283267fca1c6514a6d9c2f28bdd9e.png)
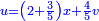
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle1\frac{2}{3}x+\frac{2}{3}u+\frac{2}{3}v &\scriptstyle=1\frac{2}{3}x+\frac{2}{3}\sdot\left[\left(2+\frac{3}{5}\right)x+\frac{4}{5}v\right]+\frac{2}{3}v=1\frac{2}{3}x+\left[\left(1+\frac{1}{3}+\frac{2}{5}\right)x+\left(\frac{1}{3}+\frac{1}{5}\right)v\right]+\frac{2}{3}z \\&\scriptstyle=3\frac{2}{5}x+1\frac{1}{5}v\\\end{align}}}](/mediawiki/images/math/9/9/d/99dda9531039c278f1e8c8473c4e92a8.png)
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle y &\scriptstyle=1\frac{1}{3}x+\frac{1}{3}u+\frac{1}{3}v=1\frac{1}{3}x+\frac{1}{3}\sdot\left[\left(2+\frac{3}{5}\right)x+\frac{4}{5}v\right]+\frac{1}{3}v=1\frac{1}{3}x+\left[\left(\frac{2}{3}+\frac{1}{5}\right)x+\left[\frac{1}{5}+\left(\frac{1}{3}\sdot\frac{1}{5}\right)\right]v\right]+\frac{1}{3}v\\&\scriptstyle=2\frac{1}{5}x+\frac{3}{5}v\\\end{align}}}](/mediawiki/images/math/c/9/5/c958ed1d1e58b4ca07d09d15f3c71122.png)
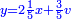
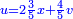
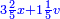
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle v+\frac{1}{5}x+\frac{1}{5}y+\frac{1}{5}u&\scriptstyle=v+\frac{1}{5}\sdot\left[x+\left(2\frac{1}{5}x+\frac{3}{5}v\right)+\left(2\frac{3}{5}x+\frac{4}{5}v\right)\right]=v+\left[\left[1+\left(\frac{4}{5}\sdot\frac{1}{5}\right)\right]x+\left[\frac{1}{5}+\left(\frac{2}{5}\sdot\frac{1}{5}\right)\right]v\right]\\&\scriptstyle=\left[1+\left(\frac{4}{5}\sdot\frac{1}{5}\right)\right]x+\left[1+\frac{1}{5}+\left(\frac{2}{5}\sdot\frac{1}{5}\right)\right]v=3\frac{2}{5}x+1\frac{1}{5}v\\\end{align}}}](/mediawiki/images/math/7/d/d/7dd362ed87d4a2270322a9c0f05c344b.png)
![\scriptstyle{\color{blue}{\left[1+\frac{1}{5}+\left(\frac{2}{5}\sdot\frac{1}{5}\right)\right]v-1\frac{1}{5}v=\frac{2}{5}\sdot\frac{1}{5}v}}](/mediawiki/images/math/0/9/9/09989998647008c8d2372f3168f32be9.png)
![\scriptstyle{\color{blue}{3\frac{2}{5}x-\left[1+\left(\frac{4}{5}\sdot\frac{1}{5}\right)\right]x=\left[2+\frac{1}{5}x+\left(\frac{1}{5}\sdot\frac{1}{5}\right)\right]x=\frac{2}{5}\sdot\frac{1}{5}v}}](/mediawiki/images/math/b/1/6/b16ac80f3e90ed3b72162ad72172972d.png)

![\scriptstyle{\color{blue}{y=2\frac{{\color{red}{1}}}{5}x+\frac{3}{5}v=\left[\left(2+\frac{1}{5}\right)\sdot1\right]+\left(\frac{3}{5}\sdot28\right)=19}}](/mediawiki/images/math/4/1/6/41672b3160b07d1f0444759c9e134669.png)
![\scriptstyle{\color{blue}{u=2\frac{3}{5}x+\frac{4}{5}v=\left[\left(2+\frac{3}{5}\right)\sdot1\right]+\left(\frac{4}{5}\sdot28\right)=25}}](/mediawiki/images/math/4/b/d/4bd1b033a6e077153401f1c04decaf98.png)
![\scriptstyle{\color{blue}{3\frac{{\color{red}{2}}}{5}x+1\frac{1}{5}v=\left[\left(3+\frac{2}{5}\right)\sdot1\right]+\left(1+\frac{1}{5}\sdot28\right)=37}}](/mediawiki/images/math/b/7/3/b7370c63a604bb49053b22f5ca109738.png)
 ].
].![\scriptstyle{\color{blue}{x+\left[\frac{1}{2}\sdot\left(y-x\right)\right]=\frac{1}{2}x+\frac{1}{2}y}}](/mediawiki/images/math/2/4/0/24025f6b2232950c37bb11369a0f6810.png)
![\scriptstyle{\color{blue}{u+\left[\frac{1}{3}\sdot\left(y-u\right)\right]=\frac{2}{3}u+\frac{1}{3}y=\frac{1}{2}x+\frac{1}{2}y}}](/mediawiki/images/math/a/0/b/a0b98375081da140457151a8e0b019f6.png)


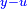 ].
].![\scriptstyle{\color{blue}{u+\left[\frac{1}{4}\sdot\left(y-u\right)\right]=\frac{3}{4}u+\frac{1}{4}y=\frac{1}{2}x+\frac{1}{2}y}}](/mediawiki/images/math/1/9/d/19dec3eaf471ac9e8daaf84403d733e4.png)
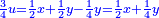
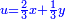
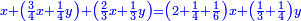
![\scriptstyle{\color{blue}{y-\left[\left(2+\frac{1}{4}+\frac{1}{6}\right)x+\left(\frac{1}{3}+\frac{1}{4}\right)y\right]=\left(\frac{1}{4}+\frac{1}{6}\right)y-\left(2+\frac{1}{4}+\frac{1}{6}\right)x}}](/mediawiki/images/math/a/2/6/a2691d3f0fb50d154b99ca2c08db9a88.png)
![\scriptstyle{\color{blue}{\begin{align}&\scriptstyle\left[\left(\frac{1}{4}+\frac{1}{6}\right)y-\left(2+\frac{1}{4}+\frac{1}{6}\right)x\right]+\left[\frac{1}{5}\sdot\left[\left(2+\frac{1}{4}+\frac{1}{6}\right)x+\left(\frac{1}{3}+\frac{1}{4}\right)y\right]\right]\\&\scriptstyle=\left[\left(\frac{1}{4}+\frac{1}{6}\right)y-\left(2+\frac{1}{4}+\frac{1}{6}\right)x\right]+\left(\frac{29}{60}x+\frac{7}{60}y\right)=\frac{32}{60}y-1\frac{56}{60}x=\frac{1}{2}x+\frac{1}{2}y\\\end{align}}}](/mediawiki/images/math/2/d/c/2dc5bef5eeb9337bf03fc1f10845b284.png)
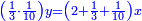
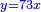
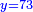
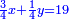
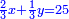
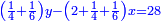
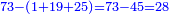

![\scriptstyle{\color{blue}{a+\left[\frac{1}{2}\sdot\left(b+c\right)\right]=b+\left[\frac{1}{3}\sdot\left(c+d\right)\right]=c+\left[\frac{1}{4}\sdot\left(d+e\right)\right]=d+\left[\frac{1}{5}\sdot\left(e+a\right)\right]=e+\left[\frac{1}{6}\sdot\left(a+b\right)\right]}}](/mediawiki/images/math/5/8/5/585b26c7e0cd60e4dccb5bca434fdf76.png)
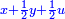
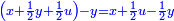
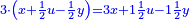
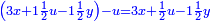
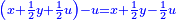
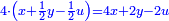
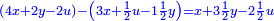
![\scriptstyle{\color{blue}{\left(3x+\frac{1}{2}u-1\frac{1}{2}y\right)+\left[\frac{1}{5}\sdot\left(2x+3\frac{1}{2}y-2\frac{1}{2}u\right)\right]=\left(3x+\frac{1}{2}u-1\frac{1}{2}y\right)+\left(\frac{2}{5}x+\frac{7}{10}y-\frac{1}{2}u\right)=3\frac{2}{5}x-\frac{4}{5}y}}](/mediawiki/images/math/c/c/2/cc24eac01997a563f9ab3c068a225348.png)
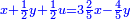
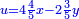
![\scriptstyle{\color{blue}{x+\frac{1}{2}y+\left[\frac{1}{2}\sdot\left(4\frac{4}{5}x-2\frac{3}{5}y\right)\right]=x+\frac{1}{2}y+2\frac{2}{5}x-1\frac{3}{10}y=3\frac{2}{5}x-\frac{4}{5}y}}](/mediawiki/images/math/1/c/6/1c6511ff0c9f871f25351150b0c944b9.png)
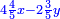 ].
]. ].
].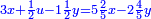
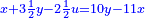
![\scriptstyle{\color{blue}{\left(10y-11x\right)+\left[\frac{1}{6}\sdot\left(x+y\right)\right]=\left(10y-11x\right)+\left(\frac{1}{6}x+\frac{1}{6}y\right)=10\frac{1}{6}y-10\frac{5}{6}x}}](/mediawiki/images/math/4/b/5/4b5bff47c6fa51131d2d5b61515724f9.png)
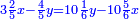
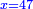
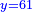
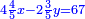

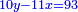
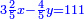
![\scriptstyle{\color{blue}{\left[3\sdot\left(111-61\right)\right]-67=\left(3\sdot50\right)-67=150-67=83}}](/mediawiki/images/math/1/a/b/1ab76e2903cc032f3b28c3647428efbb.png)
![\scriptstyle{\color{blue}{\left[4\sdot\left(111-67\right)\right]-83=\left(4\sdot44\right)-83=176-83=93}}](/mediawiki/images/math/8/e/a/8ea766d7ea4583c39e7fbb524c2be9b2.png)
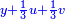
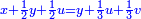
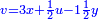
![\scriptstyle{\color{blue}{u+\left[\frac{1}{4}\sdot\left[\left(3x+\frac{1}{2}u-{\color{red}{1}}\frac{1}{2}y\right)+v\right]\right]=u+\left(\frac{3}{4}x+\frac{1}{4}v+\frac{1}{8}u-\frac{3}{8}y\right)=1\frac{1}{8}u+\frac{1}{4}v+\frac{3}{4}x-\frac{3}{8}y}}](/mediawiki/images/math/b/1/7/b1747cf13f2963a349c298e4df5d443d.png)
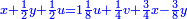
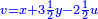
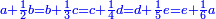

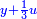
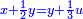
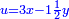
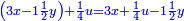
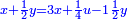
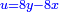
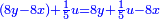
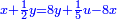
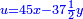

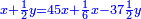
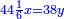

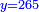
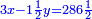

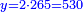


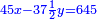

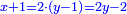
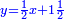
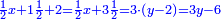
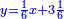
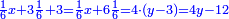

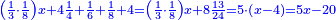
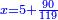
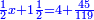
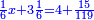
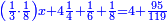
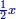 ].
]. ].
].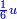 ].
].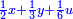 ].
].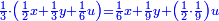
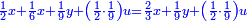
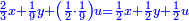
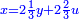
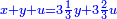
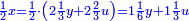
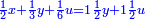
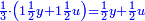
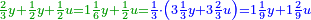
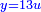
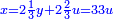
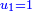
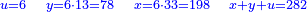
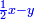

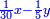
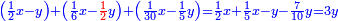
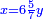

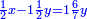


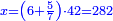

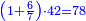
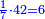
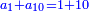
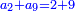
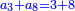
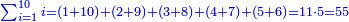
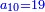

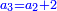

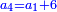



![\scriptstyle{\color{blue}{a_{10}=\left[d\sdot\left(n-1\right)+a_1\right]=\left[4\sdot\left(10-1\right)\right]+a_1=36+3=39}}](/mediawiki/images/math/e/9/0/e905399335c1776803fe8d7e6d16fa25.png)
![\scriptstyle{\color{blue}{\sum_{i=1}^{10} \left[3+4\sdot\left(i-1\right)\right]=\left(a_1+a_{10}\right)+\left(a_2+a_9\right)+\ldots=42+42+\ldots=42\sdot5=210}}](/mediawiki/images/math/7/4/8/748bdd83320ff5515cfa7a4468aa3fb0.png)
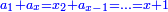
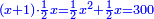
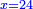
![\scriptstyle{\color{blue}{a_x=\left[d\sdot\left(n-1\right)\right]+a_1=\left[3\sdot\left(x-1\right)\right]+2=\left(3x-3\right)+2=3x-1}}](/mediawiki/images/math/7/4/d/74d1079352eaf54969dbae4659e12d62.png)

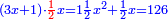
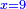
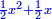
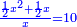
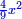
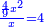
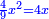
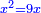
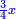
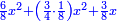
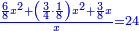
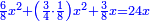
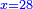

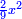


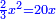
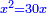
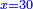

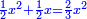
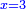
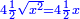

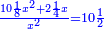
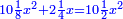

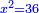
![\scriptstyle{\color{blue}{a_{30}=a_n=\left[d\sdot\left(n-1\right)\right]+a_1=\left[x\sdot\left(30-1\right)\right]+1=29x+1}}](/mediawiki/images/math/0/6/1/061949fcfa835cf5dc3c4f08f1a38a6a.png)

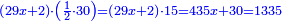
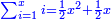




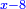
![\scriptstyle{\color{blue}{\sum_{i=1}^{x-8} \left[1+\left(3i-3\right)\right]=1\frac{1}{2}x^2+100-24\frac{1}{2}x=\frac{1}{2}x^2+\frac{1}{2}x}}](/mediawiki/images/math/f/b/5/fb500d80de828336751b348a7084bab6.png)
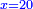
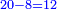
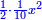

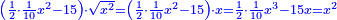
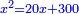
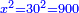

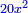
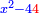

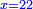
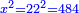
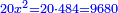


 ] of the dirham and a thing [
] of the dirham and a thing [ ] of the dirham.
] of the dirham.

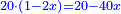
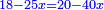

 ] of the ounce for 15 at [30] things [
] of the ounce for 15 at [30] things [ ] of the dirham.
] of the dirham.

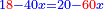
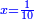
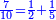
 ] of the two dirham and a thing [
] of the two dirham and a thing [ ] of the two dirham.
] of the two dirham.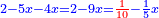
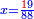
 ] of the two dirham].
] of the two dirham].



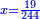
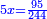
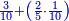 ] of the liṭra for five [dirham] at one dirham, a half and a fifth [
] of the liṭra for five [dirham] at one dirham, a half and a fifth [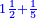 ]; three [parts] of fifty [
]; three [parts] of fifty [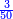 ] of the liṭra for four dirham at a [fifth] and two [parts] of fifty [
] of the liṭra for four dirham at a [fifth] and two [parts] of fifty [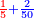 ] of a dirham; and three-fifths [
] of a dirham; and three-fifths [
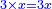
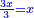
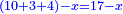
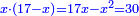
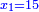 ], if you want, or two [
], if you want, or two [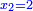 ], if you want. Say: the total amount of sesame is 15 qafiz, if you want, or two [qafiz], if you want.
], if you want. Say: the total amount of sesame is 15 qafiz, if you want, or two [qafiz], if you want.