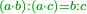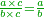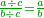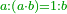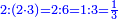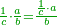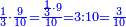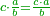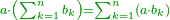Difference between revisions of "קצת מענייני חכמת המספר"
From mispar
(→Methods of checking) |
(→Other Propositions) |
||
| (163 intermediate revisions by the same user not shown) | |||
| Line 55: | Line 55: | ||
|- | |- | ||
| | | | ||
| − | *Numbers that are summed successively and the result is a known number. | + | *{{#annot:aₙ, 1, 55|669|2wMi}}Numbers that are summed successively and the result is a known number. |
:<math>\scriptstyle\sum_{i=1}^n i =a</math> | :<math>\scriptstyle\sum_{i=1}^n i =a</math> | ||
|style="text-align:right;"|חשבון שחובר על הסדר ועלה מספר ידוע | |style="text-align:right;"|חשבון שחובר על הסדר ועלה מספר ידוע | ||
| Line 62: | Line 62: | ||
::Such as the number 55, how much is the last number? | ::Such as the number 55, how much is the last number? | ||
::<math>\scriptstyle{\color{blue}{\sum_{i=1}^n i =55}}</math> | ::<math>\scriptstyle{\color{blue}{\sum_{i=1}^n i =55}}</math> | ||
| − | |style="text-align:right;"|כגון מספר נ"ה כמה הוא מספר האחרון | + | |style="text-align:right;"|כגון מספר נ"ה כמה הוא מספר האחרון{{#annotend:2wMi}} |
|- | |- | ||
| | | | ||
| Line 79: | Line 79: | ||
|- | |- | ||
| | | | ||
| − | *If the numbers that are given in succession do not start from 1. | + | *{{#annot:5-10|669|6eLr}}If the numbers that are given in succession do not start from 1. |
|style="text-align:right;"|ואם המספרים המונחים על סדר המספר לא יתחילו מהא‫' | |style="text-align:right;"|ואם המספרים המונחים על סדר המספר לא יתחילו מהא‫' | ||
|- | |- | ||
| Line 89: | Line 89: | ||
::As saying: from 5 to 10. | ::As saying: from 5 to 10. | ||
::<math>\scriptstyle\sum_{i=5}^{10} i</math> | ::<math>\scriptstyle\sum_{i=5}^{10} i</math> | ||
| − | |style="text-align:right;"|כאמרך מה' עד י‫' | + | |style="text-align:right;"|כאמרך מה' עד י‫'{{#annotend:6eLr}} |
|- | |- | ||
| | | | ||
| Line 97: | Line 97: | ||
|- | |- | ||
| | | | ||
| − | *Numbers that are summed from a known number. | + | *{{#annot:aₙ, 5, 45|669|xRiD}}Numbers that are summed from a known number. |
:<math>\scriptstyle\sum_{i=m}^n i=a</math> | :<math>\scriptstyle\sum_{i=m}^n i=a</math> | ||
|style="text-align:right;"|חשבון שחובר מתחלת מספר ידוע | |style="text-align:right;"|חשבון שחובר מתחלת מספר ידוע | ||
| Line 104: | Line 104: | ||
::As saying: from 5 and the sum is 45, how much is the last number? | ::As saying: from 5 and the sum is 45, how much is the last number? | ||
::<math>\scriptstyle{\color{blue}{\sum_{i=5}^n i=45}}</math> | ::<math>\scriptstyle{\color{blue}{\sum_{i=5}^n i=45}}</math> | ||
| − | |style="text-align:right;"|כאמרך מה' ועלה מ"ה כמה הוא סוף המספר | + | |style="text-align:right;"|כאמרך מה' ועלה מ"ה כמה הוא סוף המספר{{#annotend:xRiD}} |
|- | |- | ||
| | | | ||
| Line 112: | Line 112: | ||
|- | |- | ||
| | | | ||
| − | ::We know the 10, which is [the last] summed number, and that the sum is 45, but the 5 is unknown. | + | ::{{#annot:a₁, 10, 45|669|Sm0l}}We know the 10, which is [the last] summed number, and that the sum is 45, but the 5 is unknown. |
::<math>\scriptstyle{\color{blue}{\sum_{i=m}^{10} i=45}}</math> | ::<math>\scriptstyle{\color{blue}{\sum_{i=m}^{10} i=45}}</math> | ||
| − | |style="text-align:right;"|ידענו הי' שהוא המספר שהנקבץ עד מ"ה ונעלם הה‫' | + | |style="text-align:right;"|ידענו הי' שהוא המספר שהנקבץ עד מ"ה ונעלם הה‫'{{#annotend:Sm0l}} |
|- | |- | ||
| | | | ||
| Line 131: | Line 131: | ||
|- | |- | ||
| | | | ||
| + | |||
=== <span style=color:green>Sums of Squares</span> === | === <span style=color:green>Sums of Squares</span> === | ||
| | | | ||
| Line 226: | Line 227: | ||
|- | |- | ||
| | | | ||
| − | * | + | *To know the sum of the cube numbers [that are set] in succession until whichever number you wish. |
:<math>\scriptstyle\sum_{k=1}^n k^3 </math> | :<math>\scriptstyle\sum_{k=1}^n k^3 </math> | ||
| − | |style="text-align:right;"|לידע מעוקב המספרים על הסדר ר"ל קבוצם על הסדר עד איזה מספר שתרצה | + | |style="text-align:right;"|לידע מעוקב המספרים על הסדר ר"ל קבוצם על הסדר עד איזה מספר שתרצה |
|- | |- | ||
| | | | ||
| Line 251: | Line 252: | ||
|- | |- | ||
| | | | ||
| − | ::As if you wish to know [the sum of] the cube numbers from 5 to 10. | + | ::{{#annot:5-10|673|JGRe}}As if you wish to know [the sum of] the cube numbers from 5 to 10. |
| − | |style="text-align:right;"|כגון שרצית לידע מעוקבי מספרים מה' עד הי‫' | + | |style="text-align:right;"|כגון שרצית לידע מעוקבי מספרים מה' עד הי‫'{{#annotend:JGRe}} |
|- | |- | ||
| | | | ||
| Line 271: | Line 272: | ||
| | | | ||
::Know the cubes from 1 to 4; they are 100. Add them to 2[0]25; they are [3025]. Know its root; it is 55. Know up to which number it is the sum according to the previous way; it is 10 and this is the required. | ::Know the cubes from 1 to 4; they are 100. Add them to 2[0]25; they are [3025]. Know its root; it is 55. Know up to which number it is the sum according to the previous way; it is 10 and this is the required. | ||
| − | |||
|style="text-align:right;"|תדע המעוקבים שמהא' עד ד' והם 100 ותוסיפם על 2925 יהיו [3025] תדע גדרם בנ"ה ותדע עד איזה מספר נקבץ על דרך הקודם והוא י' והוא המבוקש | |style="text-align:right;"|תדע המעוקבים שמהא' עד ד' והם 100 ותוסיפם על 2925 יהיו [3025] תדע גדרם בנ"ה ותדע עד איזה מספר נקבץ על דרך הקודם והוא י' והוא המבוקש | ||
| + | |} | ||
| + | ::<math>\scriptstyle{\color{blue}{\sum_{k=1}^n k=\sqrt{2{\color{red}{0}}25+\sum_{k=1}^4 k^3}=\sqrt{2{\color{red}{0}}25+100}=\sqrt{3025}=55=\sum_{k=1}^{10}}}</math> | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| Line 281: | Line 284: | ||
| | | | ||
::Know [the sum] from 1 to 10; it is 3025. Subtract the number that you know, which is 2[0]25; 100 remains. Extract its root; it is ten. Know up to which number it is the sum according to the previous way; it is 4 and the number that follows it, which is 5, is the required. | ::Know [the sum] from 1 to 10; it is 3025. Subtract the number that you know, which is 2[0]25; 100 remains. Extract its root; it is ten. Know up to which number it is the sum according to the previous way; it is 4 and the number that follows it, which is 5, is the required. | ||
| − | + | |style="width:45%; text-align:right;"|תדע מא' עד י' והם 3025 ותגרע מהם המספר הידוע לך והוא 2925 וישארו ק' קח גדרם בעשר ודע עד איזה מספר חובר ע"ד הקודם והוא הד' והמספר שלאחריו שהוא ה' הוא המבוקש | |
| − | |style="text-align:right;"|תדע מא' עד י' והם 3025 ותגרע מהם המספר הידוע לך והוא 2925 וישארו ק' קח גדרם בעשר ודע עד איזה מספר חובר ע"ד הקודם והוא הד' והמספר שלאחריו שהוא ה' הוא המבוקש | ||
| − | |||
| − | |||
|} | |} | ||
| − | + | ::<math>\scriptstyle{\color{blue}{\scriptstyle\sum_{k=1}^{m-1} k=\sqrt{\sum_{k=1}^{10} k^3-2{\color{red}{0}}25}=\sqrt{3025-2{\color{red}{0}}25}=\sqrt{100}=10=\sum_{k=1}^4 k=\sum_{k=1}^{5-1} k}}</math> | |
{| | {| | ||
|- | |- | ||
| Line 295: | Line 295: | ||
|- | |- | ||
| | | | ||
| − | * | + | *To know the sum of the odd numbers [that are set] in succession. |
:<math>\scriptstyle\sum_{k=1}^n\left(2k-1\right)</math> | :<math>\scriptstyle\sum_{k=1}^n\left(2k-1\right)</math> | ||
| − | |style="text-align:right;"|לידע קבוץ הנפרדים על הסדר | + | |style="text-align:right;"|לידע קבוץ הנפרדים על הסדר |
|- | |- | ||
| | | | ||
| Line 373: | Line 373: | ||
|- | |- | ||
| | | | ||
| − | : | + | :<math>\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n\left(2k-1\right)^2=\left[\left[\sum_{k=1}^n\left(2k-1\right)\right]\sdot\left[\left[\frac{2}{3}\sdot\left(2k - 1\right)\right]+\frac{2}{3}\right]\right]-\left(\frac{1}{3}\sdot{n}\right)}}</math> |
| + | | | ||
| + | |- | ||
| | | | ||
| + | ::Know the sum of the odd numbers according to the previous way; it is 25. | ||
| + | |style="width:45%; text-align:right;"|דע חבור הנפרדי' ע"ד הקודם והם כ"ה | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Take 2-thirds of nine, which is the last number, plus two-thirds; it is six and 2-thirds. |
| − | |style=" | + | |style="text-align:right;"|וקח ב' שלישי התשעה שהוא מספר האחרון בתוספת שני שלישיות אחד יהיו ששה וב' שלישיות אחד |
|- | |- | ||
| | | | ||
| − | + | ::Multiply it by 25; the result is 166 and 2-thirds. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הכם עם כ"ה יעלה קס"ו וב' שלישיות א‫' |
|- | |- | ||
| | | | ||
| − | : | + | ::Know how many odd numbers there are from one to nine, which is the last number; they are 5. |
| − | + | |style="text-align:right;"|ודע כמה הנפרדים שמאחד עד תשעה שהוא המספר האחרון הם ה‫' | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | ::Take one-third for each integer; the result is five thirds, which are 1 and 2-thirds. | |
| − | + | |style="text-align:right;"|קח לכל שלם שליש אחד יעלה חמשה שלישים שהם א' וב' שלישיות | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| + | | | ||
| + | ::Subtract it from 166 and 2-thirds; 165 remain and this is their sum. | ||
| + | |style="text-align:right;"|חסרם מהקס"ו וב' שלישי אח' ישאר קס"ה וככה קבוצם | ||
|} | |} | ||
| − | + | ::<math>\scriptstyle{\color{blue}{1^2+3^2+5^2+7^2+9^2=\left[25\sdot\left[\left(\frac{2}{3}\sdot9\right)+\frac{2}{3}\right]\right]-\left(\frac{1}{3}\sdot5\right)=\left[25\sdot\left(6+\frac{2}{3}\right)\right]-\frac{5}{3}=\left(166+\frac{2}{3}\right)-\left(1+\frac{2}{3}\right)}}</math> | |
{| | {| | ||
|- | |- | ||
| | | | ||
| − | + | *<math>\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n\left(2k-1\right)^2=\left[\frac{2}{3}\sdot\left(2n-1\right)\right]\sdot\left[\left(\frac{1}{2}\sdot{2n}\right)\sdot\left[\frac{1}{2}\sdot\left(2n+1\right)\right]\right]}}</math> | |
| + | | | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you want, take two-thirds of the last odd number, and multiply it by the product of half the even number that follows it by half the odd number that follows it. |
| − | + | |style="width:45%; text-align:right;"|ואם תרצה קח שני שלישי הנפרד האחרון והכהו בשטח ההווה מהכאת חצי הזוג שאחריו בחצי נפרד שלאחריו | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | * | + | :*In the example: two thirds of 9 are 6; the next even number is 10. |
| − | + | |style="text-align:right;"|והמשל בזה שני שלישי הט' הם ו' הזוג שלאחריו הוא י‫' | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | ::Take its half; it is 5. Keep it. | |
| − | : | + | |style="text-align:right;"|קח חציים בה' ושמרם |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | | |
| − | + | ::The nest odd number is 11. | |
| − | :: | + | |style="text-align:right;"|והנפרד שלאחריו הוא י"א |
| − | |style="text-align:right;"| | + | |- |
| + | | | ||
| + | ::Take its half; it is 5 and a half. | ||
| + | |style="text-align:right;"|קח חציים בה' וחצי | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | ::Multiply it by the reserved 5; the result is 27 and a half. |
| + | |style="text-align:right;"|והכם בה' השמורים יעלו כ"ז וחצי | ||
|- | |- | ||
| | | | ||
| + | ::Multiply it by six; it is 165 and this is their sum. | ||
| + | |style="text-align:right;"|הכם בששה יהיו קס"ה וככה קבוצם | ||
|} | |} | ||
| + | ::<math>\scriptstyle{\color{blue}{1^2+3^2+5^2+7^2+9^2=\left(\frac{2}{3}\sdot{9}\right)\sdot\left[\left(\frac{1}{2}\sdot{10}\right)\sdot\left(\frac{1}{2}\sdot{11}\right)\right]=6\sdot\left[5\sdot\left(5+\frac{1}{2}\right)\right]=6\sdot\left(27+\frac{1}{2}\right)}}</math> | ||
{| | {| | ||
|- | |- | ||
| | | | ||
| − | + | *<math>\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n\left(2k-1\right)^2=\left[\left[\frac{1}{3}\sdot\left(2n-1\right)\right]+\frac{1}{3}\right]\sdot\left[\left[\frac{1}{2}\sdot\left(2n-1\right)\right]\sdot\left(2n+1\right)\right]}}</math> | |
| − | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | * | + | :*If you want, take a third of the last odd number plus one-third; it is 3 and one-third. Keep it. |
| − | + | |style="width:45%; text-align:right;"|ואם תרצה תקח שליש הנפרד האחרון בתוספת שליש אחד והוא ‫<ref>29v</ref>ג' ושליש ושמרם | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Then, multiply half the last odd number that is 9, which is 4 and a half, by the next odd number, which is 11; the result is 49 and a half. |
| − | + | |style="text-align:right;"|אח"כ הכה חצי הנפרד האחרון והוא הט' שהוא ד' וחצי בנפרד שלאחריו שהוא הי"א יעלו מ"ט וחצי | |
| − | |style=" | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Multiply it by 3 and one-third; the result is 165. |
| − | ::<math>\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n\left(2k-1\right)^ | + | |style="text-align:right;"|הכם בג' ושליש השמורים יעלו קס"ה |
| − | | | + | |} |
| + | ::<math>\scriptstyle{\color{blue}{1^2+3^2+5^2+7^2+9^2=\left[\left(\frac{1}{3}\sdot{9}\right)+\frac{1}{3}\right]\sdot\left[\left(\frac{1}{2}\sdot{9}\right)\sdot{11}\right]=\left(3+\frac{1}{3}\right)\sdot\left[\left(4+\frac{1}{2}\right)\sdot{11}\right]=\left(3+\frac{1}{3}\right)\sdot\left(49+\frac{1}{2}\right)}}</math> | ||
| + | |||
| + | *<math>\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n\left(2k-1\right)^2=\left[\left[\sum_{k=1}^n\left(2k-1\right)\right]-\left[\left[\frac{1}{3}\sdot\left[\sum_{k=1}^n\left(2k-1\right)\right]\right]+\frac{1}{6}\right]\right]\sdot\left[\left(2n-1\right)+1\right]}}</math> | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | + | :If you want, subtract from the sum of the odd numbers its third plus one-sixth and keep the remainder, then add one to the last odd number and multiply the sum by the reserved. | |
| − | + | |style="width:45%; text-align:right;"|ואם תרצה חסר מקבוץ נפרדיו שלישיתו וששית אח' ושמור הנשאר והוסיף על הנפרד האחרון אח' והכה הכל בשמור | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::In our example: we sum up the odd numbers; the sum is 25. |
| − | + | |style="text-align:right;"|במשלינו זה קבצינו נפרדיו ועלו כ"ה | |
| − | |style="text-align:right;"| | + | |- |
| − | |- | ||
| | | | ||
| − | + | ::We subtract from it its third plus one-sixth; 16 and a half remains. We keep it. | |
| − | + | |style="text-align:right;"|חסרנו מהם שלישיתו וששית אחד נשאר י"ו וחצי ושמרנום | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We add one to 9; it is 10. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הוספנו על הט' אחד יהיו י‫' |
|- | |- | ||
| | | | ||
| + | ::Multiply it by the reserved 16 and a half; the result is 165. | ||
| + | |style="text-align:right;"|הכם <s>בחצי הט' שהוא ד' וחצי</s> עם י"ו וחצי השמורים יעלה קס"ה | ||
|} | |} | ||
| + | ::<math>\scriptstyle{\color{blue}{1^2+3^2+5^2+7^2+9^2=\left[25-\left[\left(\frac{1}{3}\sdot25\right)+\frac{1}{6}\right]\right]\sdot\left(9+1\right)=\left(16+\frac{1}{2}\right)\sdot10}}</math> | ||
| + | |||
| + | *<math>\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n\left(2k-1\right)^2=\left[\left[\left[\frac{1}{3}\sdot\left(2n-1\right)\right]+\frac{2}{3}\right]\sdot\left[\frac{1}{2}\sdot\left(2n-1\right)\right]\right]\sdot\left[\left(2n-1\right)+1\right]}}</math> | ||
{| | {| | ||
|- | |- | ||
| | | | ||
| − | + | ::If you want, take a third of 9 plus [2-thirds]; it is 3 and [2-thirds]. | |
| − | + | |style="width:45%; text-align:right;"|ואם תרצה קח שליש הט' בתוספת ב' ג' א' אח' יהיו ג' וב' ג' אח‫' | |
| + | |- | ||
| | | | ||
| + | ::Multiply it by a half of 9, which is 4 and a half; the result is 16 and a half. | ||
| + | |style="text-align:right;"|הכם בחצי הט' שהוא ד' וחצי יעלה י"ו וחצי | ||
|- | |- | ||
| | | | ||
| − | + | ::Add 1 to 9; it is 10. | |
| − | : | + | |style="text-align:right;"|הוסיף א' על הט' יהיו י‫' |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Multiply it by 16 and a half; the result is 165. |
| − | ::<math>\scriptstyle{\color{ | + | |style="text-align:right;"|הכם בי"ו וחצי יעלה קס"ה |
| − | + | |} | |
| − | | | + | ::<math>\scriptstyle{\color{blue}{1^2+3^2+5^2+7^2+9^2=\left[\left[\left(\frac{1}{3}\sdot9\right)+\frac{2}{3}\right]\sdot\left(\frac{1}{2}\sdot9\right)\right]\sdot\left(9+1\right)=\left[\left(3+\frac{2}{3}\right)\sdot\left(4+\frac{1}{2}\right)\right]\sdot{10}=\left(16+\frac{1}{2}\right)\sdot10}}</math> |
| + | {| | ||
|- | |- | ||
| | | | ||
| − | + | *<math>\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n\left(2k-1\right)^2=\left[\frac{1}{6}\sdot\left(2n-1\right)\right]\sdot\left[\left[\left(2n-1\right)+1\right]\sdot\left[\left(2n-1\right)+2\right]\right]}}</math> | |
| − | |||
| − | |||
| − | |||
| − | |||
| | | | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | ::If you want an easier way, take one-sixth of 9; it is one and a half. Keep it. |
| − | + | |style="width:45%; text-align:right;"|ואם תרצה בדרך יותר נקל קח ששית הט' באח' וחצי ושמרהו | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | ::Then, multiply the numbers that follow it by each other, which are 10 and 11; the result is 110. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אח"כ הכה {{#annot:term|2630,404|JKKR}}המספרים הנמשכים אחריו{{#annotend:JKKR}} זה בזה והם י' והי"א יעלה ק"י |
|- | |- | ||
| | | | ||
| − | :: | + | ::Multiply it by one and a half; the result is 165. |
| − | ::<math>\scriptstyle{\color{ | + | |style="text-align:right;"|הכם באחד וחצי יעלה קס"ה |
| − | |style="text-align:right;"| | + | |} |
| + | ::<math>\scriptstyle{\color{blue}{1^2+3^2+5^2+7^2+9^2=\left(\frac{1}{6}\sdot9\right)\sdot\left[\left(9+1\right)\sdot\left(9+2\right)\right]=\left(1+\frac{1}{2}\right)\sdot\left(10\sdot11\right)=\left(1+\frac{1}{2}\right)\sdot110}}</math> | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | *<math>\scriptstyle\sum_{k=m}^n\left (2k-1\right)^2</math> | ||
| + | :*To know the [sum of the] squares of the odd numbers from 5 to 9: | ||
| + | |style="text-align:right;"|לידע מרובעי הנפרדים שיש ממספ' ה' עד ט‫' | ||
|- | |- | ||
| | | | ||
| − | + | ::Know the sum of the squares of the odd numbers from 1 to 9. | |
| − | + | |style="width:45%; text-align:right;"|תדע חבור מרובעי הנפרדים שיש מא' עד ט‫' | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | ::Know the sum of the | + | ::Know the sum of the squares of the odd numbers from one to the odd number that precedes 5, which is 3. |
| − | + | |style="text-align:right;"|ותדע חבור מרובעי הנפרדים שמאח' עד המספר הנפרד שקודם הה' והוא ג‫' | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | ::Subtract this from that and the remainder is the required. | |
| − | + | |style="text-align:right;"|והפיל זה מזה והנשאר הוא המבוקש | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | ::<math>\scriptstyle{\color{blue}{5^2+\cdots+9^2=\left(1^2+\cdots+9^2\right)-\left(1^2+\cdots+3^2\right)}}</math> | |
| − | ::<math>\scriptstyle{\color{ | + | | |
| − | |style="text-align:right;"| | + | |- |
| + | |The ancients did not talk about the opposite questions. | ||
| + | |style="text-align:right;"|בהפך שאלות אלו לא דברו הראשונים | ||
|- | |- | ||
| | | | ||
| Line 541: | Line 552: | ||
| | | | ||
| − | ==== <span style=color:green>Sums of | + | ==== <span style=color:green>Sums of Cubes of Odd Numbers</span> ==== |
| | | | ||
|- | |- | ||
| | | | ||
| − | *To | + | *To sum the cubes of the odd numbers until a known odd number. |
| − | :<math>\scriptstyle\sum_{k=1}^n\left(2k\right)^ | + | :<math>\scriptstyle\sum_{k=1}^n\left(2k-1\right)^3</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|לקבץ מעוקבי הנפרדים על הסדר עד נפרד ידוע |
|- | |- | ||
| | | | ||
| − | :* | + | :*Multiply the sum of the odd numbers by its double minus one. |
| − | ::<math>\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n\left(2k\right)^ | + | ::<math>\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n\left(2k-1\right)^3=\left[2\sdot\left[\sum_{k=1}^n\left(2k-1\right)\right]^2\right]-\left[\sum_{k=1}^n\left(2k-1\right)\right]}}</math> |
| − | |style="width:45%; text-align:right;"| | + | |style="width:45%; text-align:right;"|הכה קבוץ נפרדיו בכפל וחסר אחת |
|- | |- | ||
| | | | ||
| − | :* | + | :*Or, subtract one half from the sum of the odd numbers, then multiply the remainder by double the sum of the odd numbers. |
| − | ::<math>\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n\left(2k\right)^ | + | ::<math>\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n\left(2k-1\right)^3=\left[\left[\sum_{k=1}^n\left(2k-1\right)\right]-\frac{1}{2}\right]\sdot\left[2\sdot\left[\sum_{k=1}^n\left(2k-1\right)\right]\right]}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|או חסר חצי אחת מקבוץ נפרדיו והנשאר הכהו בכפל קבוץ נפרדיו |
|- | |- | ||
| | | | ||
| − | + | *Numbers that are summed by the succession of the cubes of odd numbers and you wish to know [how much] is the last odd number. | |
| − | + | :<math>\scriptstyle\sum_{k=1}^n\left(2k-1\right)^3=a</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|חשבון שנתקבץ ממעוקבי הנפרדים על הסדר ורצית לידע נפרד האחרון |
|- | |- | ||
| | | | ||
| − | + | ::Take half the sum; add to it a half of an eighth; extract the root of the total; add to the result one quarter, which is the root of a half of an eighth; multiply all by 4; extract the root of the result; subtract one from it and the remainder is the required. | |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{OliveGreen}{2n-1=\sqrt{4\sdot\left[\sqrt{\left(\frac{1}{2}\sdot{a}\right)+\left(\frac{1}{2}\sdot\frac{1}{8}\right)}+\sqrt{\frac{1}{2}\sdot\frac{1}{8}}\right]}-1}}</math> |
| + | |style="text-align:right;"|קח חצי החשבו' הנתקב' והוסיף עליו חצי שמנית וקח גדר הכל והוסיף על היוצא רביע אחד שהוא שורש חצי שמנית והכה הכל בד' וקח השרש מהעולה וחסר אחת ממנו והנשאר הוא המבוקש | ||
|- | |- | ||
| | | | ||
| − | + | *We know the last odd number and the sum, but the first odd number is unknown. | |
| − | + | :<math>\scriptstyle\sum_{k=m}^n\left(2k-1\right)^3=a</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ידענו נפרד האחרון והסך ונעלם הנפרד האחד |
|- | |- | ||
| − | | | + | | |
| − | + | ::Know [their sum] from 1 to the last odd number, take the difference between this sum and the known sum, find the last odd number according to the previous method and the odd number that follows it is the required. | |
| − | + | ::<math>\scriptstyle{\color{OliveGreen}{\left[\sum_{k=1}^n\left(2k-1\right)^3\right]-a=\sum_{k=1}^{m-1}\left(2k-1\right)^3}}</math> | |
| − | + | |style="text-align:right;"|דע מהא' עד הנפרד האחרון וקח היתרון שבין זה הסך ובין הסך הידוע לך ודע הנפרד האחרון ממנו ע"ד הקודם והנפרד שלאחריו הוא המבוקש | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| Line 585: | Line 596: | ||
| | | | ||
| − | + | === <span style=color:green>Sums of Even Numbers</span> === | |
| | | | ||
|- | |- | ||
| | | | ||
| − | *To know | + | *To know the sum of the even numbers [that are set] in succession. |
| − | :<math>\scriptstyle\sum_{k=1}^n | + | :<math>\scriptstyle\sum_{k=1}^n 2k </math> |
| − | |style="text-align:right;"|לידע | + | |style="text-align:right;"|לידע קבוץ הזוגות על הסדר |
|- | |- | ||
| | | | ||
| − | : | + | :*Take half the last even number plus one; or add to it 2 and then take half the total. Multiply it by half the last even number and this is the required. |
| − | ::<math>\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n\left( | + | ::<math>\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n 2k =\left[\left(\frac{1}{2}\sdot{2n}\right)+1\right]\sdot\left(\frac{1}{2}\sdot{2n}\right)}}</math> |
| − | |style="width:45%; text-align:right;"| | + | ::<math>\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n 2k =\left[\frac{1}{2}\sdot\left(2n+2\right)\right]\sdot\left(\frac{1}{2}\sdot{2n}\right)}}</math> |
| + | |style="width:45%; text-align:right;"|קח חצי הזוג האחרון בתוספת אח' או הוסיף עליו ב' וקח חצי הכל והכהו בחצי הזוג האחרון והוא המבוקש | ||
|- | |- | ||
| | | | ||
| − | * | + | :*If you wish, take a quarter of the last even number and multiply it by the even number that follows it; or take a quarter of the even number that follows it and multiply it by the last even number. |
| − | :<math>\scriptstyle\sum_{k=1}^n\left( | + | ::<math>\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n 2k=\left(\frac{1}{4}\sdot{2n}\right)\sdot\left[2\sdot\left(n+1\right)\right]}}</math> |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n 2k=\left[\frac{1}{4}\sdot\left[2\sdot\left(n+1\right)\right]\right]\sdot{2n}}}</math> |
| + | |style="text-align:right;"|ואם תרצה קח רביע הזוג האחרו' והכהו בזוג שלאחריו או קח רביע הזוג שלאחריו והכהו בזוג האחרון | ||
|- | |- | ||
| | | | ||
| − | + | *Numbers that are summed by the succession of the even numbers and the result is a known sum, how much is the last even number? | |
| − | + | :<math>\scriptstyle\sum_{k=1}^n 2k=a</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|חשבון שחובר על הסדר הזוגות ועלה סך ידוע איזה הוא הזוג האחרון |
|- | |- | ||
| | | | ||
| − | + | ::Multiply the known sum by 4 and add 1 to the result; the root of the total minus one is the required. | |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{OliveGreen}{2n=\sqrt{4a+1}-1}}</math> |
| + | |style="text-align:right;"|הכה הסך הידוע בד' והוסף על העולה א' ושרש הכל בחסרון אח' הוא המבוקש | ||
|- | |- | ||
| | | | ||
| − | + | *If the even numbers, whose sum we wish to know, do not start from 2. | |
| − | + | |style="text-align:right;"|ואם הזוגות אשר רצינו לידע קבוצם ולא יתחילו מב‫' | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | ::Sum up from 2 to the last even number, then subtract from the result the sum from 2 up to the even number that precedes the first even number and the remainder is the required. | |
| − | :<math>\scriptstyle\sum_{k=m}^n\ | + | ::<math>\scriptstyle{\color{OliveGreen}{\sum_{k=m}^{n}2k=\sum_{k=1}^{n}2k-\sum_{k=1}^{m-1}2k}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|קבץ מב' עד הזוג האחרון וחסר מהמקובץ העולה מב' עד הזוג שלפני הזוג האח' והנשאר הוא המבוקש |
| + | |- | ||
| + | | | ||
| + | *We sum up from a known even number and the result is a known amount, how much is the last even number? | ||
| + | :<math>\scriptstyle\sum_{k=m}^n 2k=a</math> | ||
| + | |style="text-align:right;"|חברנו מזוג ידוע ועלה סך ידוע איזה הוא הזוג האחרון | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Know the sum of the even numbers successively up to the even number that precedes the first even number, add it to the known amount, then know up to what even number the sum is according to the previous way. |
| − | ::<math>\scriptstyle{\color{OliveGreen}{ | + | ::<math>\scriptstyle{\color{OliveGreen}{\sum_{k=1}^{m-1}2k+a=\sum_{k=1}^{n}2k}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|דע חבור הזוגות על הסדר עד הזוגות שקודם הראשון הידוע והוסיפהו על הסך הידוע ותדע עד איזה זוגות נתחבר ע"ד הקודם |
|- | |- | ||
| | | | ||
| − | *We know the last | + | *We know the last even number and the sum, but the first even number is unknown. |
| − | :<math>\scriptstyle\sum_{k=m}^n | + | :<math>\scriptstyle\sum_{k=m}^n 2k=a</math> |
| − | |style="text-align:right;"|ידענו האחרון והסך | + | |style="text-align:right;"|ידענו הזוג האחרון והסך הנעלם הזוג הא‫' |
|- | |- | ||
| | | | ||
| − | :: | + | ::Know the sum of the even numbers from 2 up to the last known [number] and take the difference between it and the sum that is known to you; know up to which even number it is summed and the even number that follows it is the first unknown number. |
| − | ::<math>\scriptstyle{\color{OliveGreen}{ | + | ::<math>\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n 2k-a=\sum_{k=1}^{m-1}2k}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|דע חבור הזוגות שמב' עד האחרון הידוע וקח יתרון שבינם ובין הסך הידוע לך ודע עד איזה זוג נתחבר והזוג שלאחריו הוא הזוג הא' הנעלם |
|- | |- | ||
| + | | | ||
|} | |} | ||
{| | {| | ||
| Line 642: | Line 661: | ||
| | | | ||
| − | === <span style=color:green> | + | ==== <span style=color:green>Sums of Squares of Even Numbers</span> ==== |
| | | | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | *To know the sum of the squares of the even numbers [that are set] in succession. |
| + | :<math>\scriptstyle\sum_{k=1}^n\left(2k\right)^2</math> | ||
| + | |style="text-align:right;"|לדעת מרובעי הזוגות על הסדר | ||
|- | |- | ||
| | | | ||
| − | + | :*Take two thirds of the last even number plus 2-thirds of 1 and multiply it by the sum of the even numbers. | |
| − | === | + | ::<math>\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n\left(2k\right)^2=\left[\left[\frac{2}{3}\sdot\left(2n\right)\right]+\frac{2}{3}\right]\sdot\left[\sum_{k=1}^n 2k\right]}}</math> |
| − | + | |style="width:45%; text-align:right;"|קח שני שלישי הזוג האחרון בתוספת ב' שלישי א' והכהו בעולה מחבור הזוגות | |
| − | | | ||
|- | |- | ||
| | | | ||
| − | * | + | :*If you wish, take 2-thirds of the sum of the even numbers and multiply it by the last even number, to which you add 1. |
| − | :<math>\scriptstyle\sum_{k=1}^n | + | ::<math>\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n\left(2k\right)^2=\left[\frac{2}{3}\sdot\left[\sum_{k=1}^n 2k\right]\right]\sdot\left(2n+1\right)}}</math> |
| − | |style=" | + | |style="text-align:right;"|ואם תרצה קח מחבור זוגותיהם ב' שלישיות והכהו בזוג האחרון שתוסיף עליו א‫' |
|- | |- | ||
| | | | ||
| − | :* | + | :*If you wish, take one-sixth of the last even number and multiply it by the product of the two numbers that follows it. |
| − | ::<math>\scriptstyle\sum_{k=1}^n 2 | + | ::<math>\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n\left(2k\right)^2=\left[\frac{1}{6}\sdot\left(2k\right)\right]\sdot\left[\left(2n+1\right)\sdot\left(2n+2\right)\right]}}</math> |
| − | + | |style="text-align:right;"|ואם תרצה קח ששית הזוג האחרון והכהו בשטח ההווה מב' {{#annot:term|2630,404|lqnv}}המספרים הנמשכים אחריו{{#annotend:lqnv}} | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | *If they do not start from 2. | |
| − | + | |style="text-align:right;"|ואם לא יתחילו מהב‫' | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Know [the sum of] the squares from 2 up to the last even number and subtract from it the sum [of the squares] from 2 up to the even number that precedes the first. |
| − | ::<math>\scriptstyle{\color{OliveGreen}{\sum_{k= | + | ::<math>\scriptstyle{\color{OliveGreen}{\sum_{k=m}^{n}\left(2k\right)^2=\sum_{k=1}^{n}\left(2k\right)^2-\sum_{k=1}^{m-1}\left(2k\right)^2}}</math> |
| − | + | |style="text-align:right;"|תדע מרובעים מהב' עד הזוג האחרון ותחסר מהמקובץ העולה מב' עד הזוג שלפני הראשון | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |Understand this. |
| − | : | + | |style="text-align:right;"|והבן |
| − | |style="text-align:right;"| | + | |- |
| + | |The ancients did not talk about the opposite questions. | ||
| + | |style="text-align:right;"|בהפך שאלו אלו לא דברו הראשונים | ||
|- | |- | ||
| | | | ||
| − | + | |} | |
| − | + | {| | |
| − | |||
|- | |- | ||
| | | | ||
| − | + | ||
| − | + | ==== <span style=color:green>Sums of Cubes of Even Numbers</span> ==== | |
| + | | | ||
|- | |- | ||
| | | | ||
| − | + | *To know [the sum of] the cubes of the even numbers [that are set] in succession. | |
| − | + | :<math>\scriptstyle\sum_{k=1}^n\left(2k\right)^3</math> | |
| − | + | |style="text-align:right;"|לידע מעוקבי הזוגות על הסדר | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Multiply the sum of the even numbers by their double and this is the required. |
| − | ::<math>\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n | + | ::<math>\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n\left(2k\right)^3=2\sdot\left[\sum_{k=1}^n 2k\right]^2}}</math> |
| − | + | |style="width:45%; text-align:right;"|תכה העולה מקבוץ זוגי אחדיו בכפלם והוא המבוקש | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | *<math>\ | + | *[The sum of] the cubes of the even numbers is a known amount, and you wish to know [how much is] the last even number? |
| − | + | :<math>\scriptstyle\sum_{k=1}^n\left(2k\right)^3=a</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|מעוקבי זוגות שעלה סך ידוע ותרצה לידע הזוג האחרון |
|- | |- | ||
| | | | ||
| − | + | ::Take the root of half the amount and know up to what even number it is summed. | |
| − | + | ::<math>\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n 2k=\sqrt{\frac{1}{2}\sdot{a}}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תקח גדר חצי הסך ותדע עד איזה זוג חובר |
|- | |- | ||
| | | | ||
| − | * | + | *To know [the sum of] the cubes of the even numbers that do not start from 2. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|לידע מעוקבי זוגות שלא יתחילו מב‫' |
|- | |- | ||
| | | | ||
| − | :: | + | ::Know [the sum of] the cubes up to the last even number and subtract from it the sum [of the cubes] from 2 up to the even number that precedes the first. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{OliveGreen}{\sum_{k=m}^{n}\left(2k\right)^3=\sum_{k=1}^{n}\left(2k\right)^3-\sum_{k=1}^{m-1}\left(2k\right)^3}}</math> |
| + | |style="text-align:right;"|דע מעוקבם עד הזוג האחרון וחסר מהמקובץ העולה מב' עד הזוג שלפני הראשון | ||
|- | |- | ||
| | | | ||
| − | *<math>\ | + | *We know the first even number and the sum, but the last even number is unknown. |
| − | :: | + | :<math>\scriptstyle\sum_{k=m}^n\left(2k\right)^3=a</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ידענו הזוג האחד והסך ונעלם הזוג האחרון |
| + | |- | ||
| + | | | ||
| + | ::Sum up [the cubes] from 2 to the even number that precedes the first and add it to the known sum; know up to which even number it is summed. | ||
| + | ::<math>\scriptstyle{\color{OliveGreen}{\left[\sum_{k=1}^{m-1}\left(2k\right)^3\right]+a=\sum_{k=1}^n\left(2k\right)^3}}</math> | ||
| + | |style="text-align:right;"|תקבץ מהב' עד הזוג שקודם הא' ותוסיפהו על הסך הידוע ותדע עד איזה זוג חובר | ||
| + | |- | ||
| + | | | ||
| + | *We know the last [even number] and the sum, but the first [even number] is unknown. | ||
| + | :<math>\scriptstyle\sum_{k=m}^n\left(2k\right)^3=a</math> | ||
| + | |style="text-align:right;"|ידענו האחרון והסך ונעלם הא‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Sum up [the cubes] from 2 to the last odd number and take the difference between it and the known sum; know up to which even number it is summed according to the previous way and the even number that follows it is the unknown. | ||
| + | ::<math>\scriptstyle{\color{OliveGreen}{\left[\sum_{k=1}^n\left(2k\right)^3\right]-a=\sum_{k=1}^{m-1}\left(2k\right)^3}}</math> | ||
| + | |style="text-align:right;"|תקבץ מהב' עד הזוג האחרון ותקח היתרון שבינו ובין הסך הידוע ותדע עד איזה זוג חובר ע"ד הקודם והזוג שלאחריו הוא הנעלם | ||
|- | |- | ||
|} | |} | ||
| − | |||
{| | {| | ||
|- | |- | ||
| | | | ||
| − | + | === <span style=color:green>Progression</span> === | |
| | | | ||
| + | |- | ||
| + | |The sum of numbers that exceed each other by a known increment. | ||
| + | |style="text-align:right;"|<big>קבוץ מספרים</big> נוספים אלו על אלו בתוספת ידוע | ||
|- | |- | ||
| | | | ||
| − | + | ||
| − | + | ==== <span style=color:green>Geometric progression</span> ==== | |
| − | + | ||
| | | | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | *Whether by geometric progression and it is of two types: |
| − | + | :<math>\scriptstyle\sum_{k=1}^n q^k</math> | |
| − | |style="text-align:right;"| | + | |style="width:45%; text-align:right;"|אם על יחס הנדסי וזה על שני מיני‫' |
|- | |- | ||
| | | | ||
| − | :: | + | :*Either by the power of 2, starting from one or not. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle\sum_{k=1}^n 2^k</math> |
| + | ::<math>\scriptstyle\sum_{k=m}^n 2^k</math> | ||
| + | |style="text-align:right;"|אם על יחס הכפל יתחילו מהאח' או לא יתחילו | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Such as the numbers 2; 4; 8; 16; 32; 64; 128. |
| − | ::<math>\scriptstyle{\color{ | + | ::<math>\scriptstyle{\color{blue}{\sum_{k=1}^7 2^k}}</math> |
| − | + | |style="text-align:right;"|כגון מספרי ב'ד'ח' י"ו ל"ב ס"ד קכ"ח | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::The way to know their sum is by doubling 128, which is the last number; the result is 256. Subtract from it the first number, which is 2; 254 remains and this is their sum. |
| − | ::<math>\scriptstyle{\color{OliveGreen}{ | + | ::<math>\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n 2^k=\left(2\sdot{2^n}\right)-2}}</math> |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{2+4+8+16+32+64+128=\left(2\sdot{128}\right)-2=256-2}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הדרך לדעת קבוצם שתכפול הקכ"ח שהוא המספר האחרון יעלה רנ"ו וגרע ממנו המספר הראשון שהוא הב' ישאר רנ"ד וככה קבוצם |
|- | |- | ||
| | | | ||
| − | :* | + | :*Or by ratio, even if it is double ratio or triple ratio. |
| − | + | |style="text-align:right;"|ואם הוא יחס אחר וגם אם יהיה יחס הכפל או השליש | |
| − | |style="text-align:right;"|ואם | ||
|- | |- | ||
| | | | ||
| − | ::Such as the numbers | + | ::Such as the numbers 3; 9; 27; 81; 243; 729; that are powers of three. |
| − | ::<math>\scriptstyle | + | ::<math>\scriptstyle\sum_{k=1}^6 3^k</math> |
| − | |style="text-align:right;"|כגון | + | |style="text-align:right;"|כגון מספרי ג' ט' כ"ז פ"א רמ"ג תשכ"ט שהם הולכים על יחס השליש |
|- | |- | ||
| | | | ||
| − | :: | + | ::It has two ways: |
| − | + | |style="text-align:right;"|יש בזה ב' דרכים | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | ::* | + | ::*<math>\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n q^k=q^n+\left[\frac{1}{q-1}\sdot\left(q^n-q\right)\right]}}</math> |
| − | + | | | |
| − | |||
| − | | | ||
|- | |- | ||
| | | | ||
| − | : | + | ::The first way is that you know the number from which the name of the progression is derived: two for a half, 3 for a third, 4 for a quarter; then we subtract one from it. As our number for instance, for which the increment of one over the other is three; we subtract one from it; two remains. The fraction that is derived from two is half. We take half the last number, after we subtract the first from it; it is 363. We add it to the last number, which is 729; the result is 1089 and this is their sum. |
| − | ::<math>\scriptstyle{\color{ | + | |style="text-align:right;"|הדרך הראשון שתדע המספר אשר יגזור שם היחס שנים לחצי ג' לשליש ד' לרביע ונגרע ממנו אחד כגון מספרינו זה שתוספת קצת על קצת הוא שליש נגרע ממנו האחד ישארו שנים והשבר הנגזר משנים הוא החצי ונקח החצי מהמספר האחרון אחר שנגרע ממנו הראשון יהיה שס"ג הוספנום על המספר האחרון שהוא תשכ"ט יעלה אלף ‫<ref>30r</ref>ופ"ט וככה קבוצם |
| − | | | + | |} |
| + | ::<math>\scriptstyle{\color{blue}{3+9+27+81+243+729=729+\left[\frac{1}{3-1}\sdot\left(729-3\right)\right]=729+\left[\frac{1}{2}\sdot\left(729-3\right)\right]=729+363}}</math> | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::*<math>\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n q^k=q^n+\frac{q\sdot\left(q^n-q\right)}{q^2-q}}}</math> |
| − | |||
| − | |||
| − | |||
| | | | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | ::The second way is that you multiply the first number, which is 3, by 729, which is the last number, after you subtract the first from it; the result is 2178. We divide it by the difference between the second and the first, which is six; the quotient is 363. We add it to the last number, which is 729; the result is 1089 and this is their sum. |
| − | + | |style="width:45%; text-align:right;"|הדרך הב' שתכה המספר הראשון שהוא הג' עם התשכ"ט שהוא המספר האחרון אחר שתגרע ממנו הראשון יעלה שני אלפים וקע"ח חלקנום על המותר שבין הב' לראשון שהוא ששה יעלה שס"ג הוספנום על המספר האחרון שהוא התשכ"ט יעלה אלף ופ"ט וככה קבוצם | |
| − | ::<math>\scriptstyle{\color{blue}{ | + | |} |
| − | | | + | ::<math>\scriptstyle{\color{blue}{3+9+27+81+243+729=729+\frac{3\sdot\left(729-3\right)}{9-3}=729+\frac{2178}{6}=729+363}}</math> |
| + | {| | ||
|- | |- | ||
| | | | ||
| − | + | *<math>\scriptstyle2^n=\left(2^\frac{n}{2}\right)^2</math> | |
| − | |||
| − | |||
| − | |||
| − | |||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | ::If their beginning is from one and they are related in the double ratio, as the numbers 1, 2, 4, 8, 16, 32, 64, and you you wish to know the number of the last term, or whichever term. |
| − | |style="text-align:right;"|כגון מספרי | + | |style="width:45%; text-align:right;"|ואם התחלתם מהאחד והם מיוחסים יחס הכפל כגון מספרי א"ב ד"ח י"ו ל"ב ס"ד ורצית לדעת מספר המדרגה האחרונה או איזו מדרגה שתהיה |
|- | |- | ||
| | | | ||
| − | : | + | ::Multiply the mean [term] by itself, as 8 in our example; the result is 64. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{64=8^2}}</math> |
| + | |style="text-align:right;"|תכה האמצעית בעצמה כגון הח' במשלינו זה יעלה ס"ד | ||
|- | |- | ||
| | | | ||
| − | *<math>\ | + | *<math>\scriptstyle2^n=2^\left(\frac{n}{2}-a\right)\sdot{2^\left(\frac{n}{2}+a\right)}</math> |
| − | |||
| − | |||
| | | | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Or, the two mean terms that are at equal interval [from the extremes], such as 4 and 16, or 2 and 32. |
| + | ::<math>\scriptstyle{\color{blue}{64=4\sdot{16}=2\sdot{32}}}</math> | ||
| + | |style="text-align:right;"|או שני המדרגות הרחוקות מהאמצעית ריחוק שוה כגון הד' עם הי"ו או השנים עם הל"ב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *<math>\scriptstyle2^n=2^\left(\frac{2m}{2}-1\right)\sdot{2^\frac{2m}{2}}</math> |
| + | :If the number of the terms is even, multiply the two means. | ||
| + | |style="text-align:right;"|ואם היה מספר המדרגות זוג תכה השני אמצעים | ||
|- | |- | ||
| | | | ||
| − | + | ::For instance: you wish to know the number of the sixth term. Multiply the third term by the fourth, which is 4 by 8; the result is 32. | |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{32=4\sdot{8}}}</math> |
| + | |style="text-align:right;"|כגון שרצית לדעת מספר המדרגה הו' תכה המדרגה השלישית עם הרביעית שהוא הד' עם הח' ויעלה ל"ב | ||
|- | |- | ||
| | | | ||
| − | *<math>\ | + | *<math>\scriptstyle2^n=2^\left(\frac{2m}{2}-1-a\right)\sdot{2^\left(\frac{2m}{2}+1\right)}</math> |
| − | |||
| − | |||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Or, the two terms that are at equal interval [from the extremes], such as 2 and 16; the result is 32 and this is the sixth term. |
| + | ::<math>\scriptstyle{\color{blue}{32=2\sdot{16}}}</math> | ||
| + | |style="text-align:right;"|או ב' המדרגות הרחוקות שהם ריחוק שוה כגון הב' עם הי"ו ויעלה ל"ב וזהו מספר המדרגה הששית | ||
|- | |- | ||
| − | |||
|} | |} | ||
| Line 863: | Line 878: | ||
| | | | ||
| − | == | + | ==== <span style=color:green>Arithmetic progression</span> ==== |
| − | + | | | |
|- | |- | ||
| − | | | + | | |
| − | + | *Or they are in an arithmetic progression and it is in three types: either their beginning is equal to their increment, or less, or more. | |
| − | |style=" | + | |style="width:45%; text-align:right;"|ואם הם על יחס מספרי וזה על ג' מינים או התחלתם שוה לתוספתם או פחות או יותר |
|- | |- | ||
| − | | | + | | |
| − | :<math>\scriptstyle{\color{OliveGreen}{ | + | :*If their beginning is equal to their increment, they either start from one, or not. |
| − | |style="text-align:right;"|או | + | ::<math>\scriptstyle{\color{OliveGreen}{a_1=d}}</math> |
| + | |style="text-align:right;"|ואם התחלתם שוה לתוספתם יתחילו מהאח' או לא יתחילו | ||
|- | |- | ||
| − | | | + | | |
| − | :<math>\scriptstyle{\color{ | + | ::Such as the numbers 3; 6; 9; 12; 15; 18; 21; whose increment one over the other is equal to the first number, which is 3. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\sum_{k=1}^7 3k}}</math> |
| − | + | |style="text-align:right;"|כגון מספרי ג' ו' ט' י"ב ט"ו י"ח כ"א שתוספת אלו על אלו שוה למספר הראשון והוא הג‫' | |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | ::It has two ways: |
| − | : | + | |style="text-align:right;"|יש בזה ב' דרכים |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| + | ::*<math>\scriptstyle{\color{OliveGreen}{S_n=\left[\left(\frac{1}{2}\sdot n\right)+\frac{1}{2}\right]\sdot a_n}}</math> | ||
| | | | ||
| − | |||
|- | |- | ||
| − | |<math>\scriptstyle\ | + | | |
| − | | | + | ::The first is that you take half the number of the terms, plus a half of 7, which is 4 in our example, and multiply it by the last number, which is 21; the result is 84. |
| + | ::<math>\scriptstyle{\color{blue}{3+6+9+12+15+18+21=\left[\left(\frac{1}{2}\sdot7\right)+\frac{1}{2}\right]\sdot21=4\sdot21=84}}</math> | ||
| + | |style="text-align:right;"|הראשון שתקח חצי כמות המדרגות בתוספת חצי ז' שהוא במשלינו זה ד' ותכהו במספר האחרון שהוא הכ"א יעלה פ"ד | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::*<math>\scriptstyle{\color{OliveGreen}{S_n=\left[\frac{1}{2}\sdot\left(a_1+a_n\right)\right]\sdot n}}</math> |
| − | + | | | |
| − | | | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::The second is that you sum the first with the last; it is 24. Take its half, which is 12, and multiply it by the number of the terms, which is 7; the result is 84. |
| − | ::<math>\scriptstyle{\color{blue}{2\ | + | ::<math>\scriptstyle{\color{blue}{3+6+9+12+15+18+21=\left[\frac{1}{2}\sdot\left(3+21\right)\right]\sdot7=\left(\frac{1}{2}\sdot24\right)\sdot7=12\sdot7=84}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והב' שתחבר הראשון עם האחרון יהיו כ"ד ותקח חצים והוא י"ב ותכם במנין המדרגות והוא ז' יעלה פ"ד |
| + | |- | ||
| + | | | ||
| + | :*If their beginning is less than their increment. | ||
| + | ::<math>\scriptstyle{\color{OliveGreen}{a_1<d}}</math> | ||
| + | |style="text-align:right;"|ואם התחלתם פחות מתוספתם | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Such as the numbers 1; 3; 5; 7; 9; 11; 13; 15; whose increment is two and their beginning is one. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{\sum_{k=1}^8 \left[\left[2\sdot\left(k-1\right)\right]+1\right]}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כגון א' ג' ה' ז' ט' י"א י"ג ט"ו שתוספתם שנים והתחלתם מהאחד |
|- | |- | ||
| | | | ||
| − | :: | + | ::*<math>\scriptstyle{\color{OliveGreen}{S_n=\left[\left[\left(d-a_1\right)+a_n\right]\sdot\left[\left(\frac{1}{2}\sdot n\right)+\frac{1}{2}\right]\right]-\left[\left(d-a_1\right)\sdot n\right]}}</math> |
| − | + | | | |
| − | | | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We add the deficit, which is one, to the last number, which is 15; it is 16. |
| − | + | |style="text-align:right;"|נוסיף המגרעת שהוא אחד על המספר האחרון שהוא ט"ו יהיו י"ו | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | ::We multiply it by half the [number of the] terms plus one half, which is 4½; the result is 72. |
| − | + | |style="text-align:right;"|ונכם בחצי המדרגות בתוספת חצי שהם ד' וחצי יעלה ע"ב | |
| − | |||
| − | |||
|- | |- | ||
| − | | | + | | |
| − | |style=" | + | ::We subtract from it the product of the deficit by the [number of the] terms, which is the product of one by 8 that is the number of the terms; the product is 8. |
| + | |style="text-align:right;"|נגרע מהם הכאת המגרעת עם כל המדרגות שהוא הכאת האח' בח' שהם מנין המדרגות יעלה ח‫' | ||
|- | |- | ||
| + | | | ||
| + | ::We subtract it from 72; 64 remains and this is their sum. | ||
| + | |style="text-align:right;"|נגרעם מהע"ב ישארו ס"ד וככה קבוצם | ||
|} | |} | ||
| − | :<math> | + | ::<math>{\color{blue}{\begin{align}\scriptstyle1+3+5+7+9+11+13+15&\scriptstyle=\left[\left[\left(2-1\right)+15\right]\sdot\left[\left(\frac{1}{2}\sdot8\right)+\frac{1}{2}\right]\right]-\left[\left(2-1\right)\sdot8\right]=\left[\left(1+15\right)\sdot\left(4+\frac{1}{2}\right)\right]-\left(1\sdot8\right)\\&\scriptstyle=\left[16\sdot\left(4+\frac{1}{2}\right)\right]-8=72-8=64\\\end{align}}}</math> |
{| | {| | ||
|- | |- | ||
| | | | ||
| − | : | + | ::*<math>\scriptstyle{\color{OliveGreen}{S_n=\left[\frac{1}{2}\sdot\left(a_1+a_n\right)\right]\sdot n}}</math> |
| − | :<math>\scriptstyle{\color{ | + | | |
| − | | | ||
|- | |- | ||
| | | | ||
| − | : | + | ::If you want to use the second way: we sum 1 with 15; it is 16. We take its half, which is 8, and multiply it by the number of the terms, which are 8; it is 64 and this is their sum. |
| − | |style="width:45%; text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{1+3+5+7+9+11+13+15=\left[\frac{1}{2}\sdot\left(1+15\right)\right]\sdot8=\left(\frac{1}{2}\sdot{16}\right)\sdot8=8\sdot8}}</math> |
| + | |style="width: 45%; text-align:right;"|ואם תרצה להשתמש בדרך הב' שנחבר הא' עם הט"ו יהיו י"ו ונקח חציים שהוא ח' ותכם במנין המדרגות שהם ח' יהיו ז' ס"ד וככה קבוצם | ||
|- | |- | ||
| | | | ||
| + | :*If their beginning is greater than their increment. | ||
| + | ::<math>\scriptstyle{\color{OliveGreen}{a_1>d}}</math> | ||
| + | |style="text-align:right;"|ואם יותר מתוספתם | ||
| + | |- | ||
| | | | ||
| − | {| | + | ::Such as the numbers 5; 8; 11; 14; 17; 20; 23. |
| + | ::<math>\scriptstyle{\color{blue}{\sum_{k=1}^7 \left(3k+2\right)}}</math> | ||
| + | |style="text-align:right;"|כגון מספרי ה' ח' י"א י"ד י"ז כ' כ"ג | ||
|- | |- | ||
| − | |<math>\scriptstyle\ | + | | |
| − | | | + | ::*<math>\scriptstyle{\color{OliveGreen}{S_n=\left[\left[\left(a_1-d\right)+a_n\right]\sdot\left[\left(\frac{1}{2}\sdot{n}\right)+\frac{1}{2}\right]\right]-\left(a_1-d\right)}}</math> |
| + | | | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Add the excess over the increment, which is 2, to the last number, which is 23; it is 25. |
| − | + | |style="text-align:right;"|תוסיף היתר על התוספת שהוא ב' על המספר האחרון שהוא כ"ג יהיו כ"ה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | ::Multiply | + | ::Multiply it by half the number of the terms plus one-half; it is 100. |
| − | + | |style="text-align:right;"|הכם בחצי המדרגות בתוספת חצי יהיו ק‫' | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Subtract the excess, which is 2; 96 remains and it is their sum. |
| − | ::<math>\scriptstyle{\ | + | |style="text-align:right;"|גרע התוספת שהוא ב' ישאר צ"ח וככה קבוצם |
| − | | | + | |} |
| + | ::<math>{\color{blue}{\begin{align}\scriptstyle5+8+11+14+17+20+23&\scriptstyle=\left[\left[\left(5-3\right)+23\right]\sdot\left[\left(\frac{1}{2}\sdot{7}\right)+\frac{1}{2}\right]\right]-\left(5-3\right)=\left[\left(2+23\right)\sdot\left[\left(\frac{1}{2}\sdot{7}\right)+\frac{1}{2}\right]\right]-2\\&\scriptstyle=\left[25\sdot\left[\left(\frac{1}{2}\sdot{7}\right)+\frac{1}{2}\right]\right]-2=100-2\\\end{align}}}</math> | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::*<math>\scriptstyle{\color{OliveGreen}{S_n=\left[\frac{1}{2}\sdot\left[\frac{a_n-a_1}{d}+1\right]\right]\sdot\left(a_1+a_n\right)}}</math> |
| − | + | | | |
| − | | | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::If you wish, subtract the first, which is 5, from the last, which is 23; 18 remains. |
| − | + | |style="width:45%; text-align:right;"|ואם תרצה חסר הראשון שהו' הה' מן האחרון שהוא כ"ג ישארו י"ח | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Divide it by the increment, which is 3, then add 1 to the result; it is seven. Keep it. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | |style="text-align:right;"|תחלקהו על מספר תוספתם שהוא ג' והיוצא תוסיף עליו א' יהיו שבעה ושמרם |
| − | | | + | |- |
| + | | | ||
| + | ::Sum up the first and the last and multiply [the sum] by half the seven. | ||
| + | |style="text-align:right;"|אח"כ חבר הראשון והאחרון והכם בחצי השבעה | ||
| + | |} | ||
| + | ::<math>\scriptstyle{\color{blue}{5+8+11+14+17+20+23=\left[\frac{1}{2}\sdot\left[\frac{23-5}{3}+1\right]\right]\sdot\left(5+23\right)=\left[\frac{1}{2}\sdot\left[\frac{18}{3}+1\right]\right]\sdot\left(5+23\right)=\left(\frac{1}{2}\sdot{7}\right)\sdot\left(5+23\right)}}</math> | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::*<math>\scriptstyle{\color{OliveGreen}{S_n=\left[\frac{a_n-a_1}{d}+1\right]\sdot\left[\frac{1}{2}\sdot\left(a_1+a_n\right)\right]}}</math> |
| − | + | | | |
| − | | | ||
|- | |- | ||
| | | | ||
| + | ::Or, take its half and multiply it by seven and so is their sum. | ||
| + | |style="width:45%; text-align:right;"|או תקח חציים ותכם עם השבעה וככה קבוצם | ||
|} | |} | ||
| + | ::<math>\scriptstyle{\color{blue}{5+8+11+14+17+20+23=\left(\frac{23-5}{3}+1\right)\sdot\left[\frac{1}{2}\sdot\left(5+23\right)\right]=\left(\frac{18}{3}+1\right)\sdot\left[\frac{1}{2}\sdot\left(5+23\right)\right]=7\sdot\left[\frac{1}{2}\sdot\left(5+23\right)\right]}}</math> | ||
{| | {| | ||
|- | |- | ||
| | | | ||
| − | + | *If you wish to know [their sum] when the first number is equal to the increment. | |
| − | = | + | :<math>\scriptstyle{\color{OliveGreen}{a_1=d}}</math> |
| − | + | |style="width:45%; text-align:right;"|ואם תרצה תדע אם המספר שממנו צמיחתם שוה לתוספתם | |
|- | |- | ||
| − | | | + | | |
| − | :<math>\scriptstyle{\color{ | + | :*As the numbers: 6, 8, 10, 12, 14, 16, 18, 20. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\sum_{k=3}^{10} 2k}}</math> |
| + | |style="text-align:right;"|כגון מספרי ו' ח' י' י"ב י"ד י"ו י"ח כ‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :*<math>\scriptstyle\sum_{k=m}^n a_k=\sum_{k=1}^n a_k-\sum_{k=1}^{m-1} a_k</math> |
| − | | | + | | |
|- | |- | ||
| | | | ||
| − | : | + | :Know the number of the terms from the first number to the last number. |
| − | + | |style="text-align:right;"|תדע מנין המדרגות שממספר שממנו צמיחתם עד המספר האחרון | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | | |
| − | + | :Know the sum of the numbers according to the previous way. Keep it. | |
| − | + | |style="text-align:right;"|ותדע קבוץ המספרים על דרך הקודם ושמרהו | |
| − | |||
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | :Then, know the number of the terms from the first number to the number that precedes the first by one term. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואח"כ תדע מנין המדרגות שהם מהמספר שממנו צמיחתם עד המספר הקודם לראשון מדרגה אחת |
|- | |- | ||
| | | | ||
| − | : | + | :Know their sum according to the previous way and subtract it from the reserved. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ותדע קבוצם על דרך הקודם ותגרע זה מהשמור |
|- | |- | ||
| | | | ||
| − | + | *If the first number is smaller than the increment. | |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{OliveGreen}{a_1<d}}</math> |
| + | |style="text-align:right;"|ואם המספר שממנו צמיחתם פחות מתוספתם | ||
|- | |- | ||
| | | | ||
| − | :: | + | :*As the numbers: 5, 8, 11, 14, 17. |
| − | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left[\left( | + | ::<math>\scriptstyle{\color{blue}{\sum_{k=1}^5 \left(3k+2\right)}}</math> |
| − | | | + | |style="text-align:right;"|כגון מספרי ה'ח' י"א י"ד י"ז |
| + | |} | ||
| + | ::<math>{\color{OliveGreen}{\begin{align}\scriptstyle\sum_{k=m}^n a_k&\scriptstyle=\left[\left[\left[\left(\frac{1}{2}\sdot{n}\right)+\frac{1}{2}\right]\sdot\left[a_n+\left(d-a_1\right)\right]\right]-\left[\left(d-a_1\right)\sdot{n}\right]\right]\\&\scriptstyle-\left[\left[\left[\left[\frac{1}{2}\sdot\left(m-1\right)\right]+\frac{1}{2}\right]\sdot\left[a_{m-1}+\left(d-a_1\right)\right]\right]-\left[\left(d-a_1\right)\sdot\left(m-1\right)\right]\right]\\\end{align}}}</math> | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | : | + | :Know the number of terms from the first number to the last number. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תדע מנין המדרגות שמהמספר שממנו צמיחתם עד המספר האחרון |
|- | |- | ||
| | | | ||
| + | :Add the deficit to the last number and know their sum according to the previous method. | ||
| + | |style="text-align:right;"|ותוסיף המגרעת על המספר האחרון ותדע מנין קבוצם ע"ד הקודם | ||
| + | |- | ||
| | | | ||
| − | + | :Multiply it by half the number of the terms plus one-half. | |
| + | |style="text-align:right;"|תכה חצי כמות המדרגות בתוספת חצי עמו | ||
|- | |- | ||
| − | | | + | | |
| − | | | + | :Subtract from the result the product of the deficit by the number of the terms from the first number to the last number and keep the remainder. |
| + | |style="text-align:right;"|ותגרע מהעולה הכאת המגרע' עם מנין המדרגות שמהמספר שממנו צמיחתם עד המספר האחרון ושמור הנשאר | ||
|- | |- | ||
| − | | | + | | |
| − | + | :Then, know the number of terms from the first number to the number that precedes the first number. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אח"כ תדע מנין המדרגות שמהמספר שממנו צמיחתם עד המדרגה הקודמת למספר המדרגה הראשונה |
|- | |- | ||
| | | | ||
| − | + | :Add the deficit to [the number that precedes the first number]. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ותחבר עמו מספר המגרעת <s>עם כל המדרגות</s> |
|- | |- | ||
| | | | ||
| − | : | + | :Multiply it by half the number of the terms plus one-half. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ותכהו עם חצי המדרגות בתוספת חצי |
|- | |- | ||
| | | | ||
| − | : | + | :Subtract from the result the product of the deficit by the number of the terms from the first number to the number that precedes the first number. |
| − | + | |style="text-align:right;"|ותגרע מהעולה הכאת המגרעת עם כל המדרגות שמהמספר שממנו צמיחתם עד המדרגה הקודמת למספר הראשון | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Subtract the remainder from the reserved and it is the required. |
| + | |style="text-align:right;"|והנשאר תגרעהו מהשמור והוא המבוקש | ||
|- | |- | ||
| | | | ||
| + | *To know the number of the terms and to know if the first number is equal to the increment: | ||
| + | |style="text-align:right;"|ולידע מנין המדרגות וגם לידע אם המספר הא' שממנו צמיחתם שוה לתוספתם | ||
| + | |- | ||
| | | | ||
| − | + | :Divide the last number by the increment. | |
| − | + | |style="text-align:right;"|תחלק המספר האחרון על מספר תוספתם | |
| − | |||
| − | | | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{ | + | :*If there is no remainder, the result of division is the number of the terms from the first number to the last number and the first number is equal to the increment. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{OliveGreen}{a_1=d\longrightarrow\frac{a_n}{d}=n}}</math> |
| + | |style="text-align:right;"|ואם יצא בחילוק שוה מה שיצא בחילוק הוא מספר המדרגות שמהמספר שממנו צמיחתם עד המספר האחרון והתחלתם שוה לתוספתם | ||
|- | |- | ||
| | | | ||
| − | : | + | *If there is a remainder, the result of division plus one is the number of the terms from the first number to the last number and the remainder is the first number. |
| − | |style="text-align:right;"|ואם | + | ::<math>\scriptstyle{\color{OliveGreen}{a_1\neq{d}\longrightarrow\frac{a_n-a_1}{d}+1=n}}</math> |
| + | |style="text-align:right;"|ואם נותר שום מספר מה שיצא בחילוק בתוספת אחת הוא מספר המדרגות שמהמספר שממנו צמיחתם עד המספר האחרון והנותר הוא המספר שממנו צמיחתם | ||
|- | |- | ||
| − | | | + | |Understand this. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והבן |
|- | |- | ||
| | | | ||
| − | + | *If the last number is what you wish to know, multiply the increment by the number of the terms minus one, then add the first number to the product. | |
| − | {| | + | :<math>\scriptstyle{\color{OliveGreen}{a_n=d\sdot\left(n-1\right)+a_1}}</math> |
| + | |style="text-align:right;"|ואם המספר האחרון הוא אשר רצית לידע הכה המותר עם מספר המדרגות חסר אחת והוסיף על העולה מספר הראשון | ||
|- | |- | ||
| − | | | + | |Understand this. |
| − | | | + | |style="text-align:right;"|והבן |
|- | |- | ||
| − | | | + | |There are many specific method for [this] specific issue and also other issues of arithmetical problems etc. |
| − | + | |style="text-align:right;"|ויש דרכים אחרים מיוחדים בנושא מיוחד וג"כ עניינים אחרים לידע שאלות החשבון וכו‫' | |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| Line 1,104: | Line 1,136: | ||
| | | | ||
| − | == | + | == Multiplication of Fractions == |
| − | + | |style="text-align:right;"|<big>הכאת השברים</big> | |
| + | |- | ||
| + | |Multiply the numerator by the numerator, then relate [the product] to the product of the denominator by the denominator. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}\times\frac{c}{d}=\frac{a\sdot c}{b\sdot d}}}</math> | ||
| + | |style="Width:45%; text-align:right;"|שתכה ה{{#annot:term|570,1857|p9Nv}}כמות{{#annotend:p9Nv}} עם הכמות וניחסהו אל הכאת ה{{#annot:term|571,2269|A7GN}}איכות{{#annotend:A7GN}} עם האיכות | ||
| + | |- | ||
| + | |Or we multiply the product of the denominators by itself, then we relate to it the result of multiplication of the products of the numerator of one by the denominator of the other. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}\times\frac{c}{d}=\frac{\left(a\sdot d\right)\sdot\left(c\sdot b\right)}{\left(b\sdot d\right)^2}}}</math> | ||
| + | |style="text-align:right;"|או נכה העולה מהכאת האיכות בפני עצמו ונייחס אליו הכאת העולה מהכאת כמות האח' עם איכות חבירו זה בזה | ||
| + | |- | ||
| + | |Likewise, if fractions of fractions, and fractions of fractions of fractions and so on endlessly are related to integers: we multiply all the numerators by each other, then the product by the integers, whether they are integers or fractions of fractions of integers, then multiply the denominators by their order and relate the product to the product of the numerators by each other. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a_1}{b_1}\sdot\frac{a_2}{b_2}\cdots\frac{a_n}{b_n}\sdot{n}=\frac{a_1\sdot{a_2}\cdots{a_n}\sdot{n}}{b_1\sdot{b_2}\cdots{b_n}}}}</math> | ||
| + | |style="text-align:right;"|וכן אם היו מיוחסים אל שלמים שברי שברים ושברי שברי שברים וכן עד אין תכלית נכה כל האיכיות זה בזה ומה ‫<ref>30v</ref>שיתקבץ עם השלימים ואם היו שלמים אחדים או שברי שברים של שלמים תחזור ותכה כל הכמיות על הסדר ותיחס <s>ואם תרצה</s> המקובץ אל הכאת האיכות זה בזה | ||
|- | |- | ||
| − | | | + | |If you want to sum products of numbers: |
| − | + | |style="text-align:right;"|ואם תרצה לקבץ מספרים מוכים | |
| − | |style=" | ||
|- | |- | ||
| | | | ||
| − | :: | + | :{{#annot:(²/₄ײ/₆)+(⅘×⅚)|17|shmO}}For instance: you wish to know the sum of the product of two quarters by 2-sixths with the product of 4-fifths by 5-sixths, without having to multiply first then to sum, and still the total result is exact. |
| − | + | :<math>\scriptstyle{\color{blue}{\left(\frac{2}{4}\times\frac{2}{6}\right)+\left(\frac{4}{5}\times\frac{5}{6}\right)}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כגון שרצית לידע קבוץ הכאת שני רביעיות עם ב' ששיות עם קבוץ הכאת ד' חומשיות עם ה' ששיות מבלתי שתצטרך תחלה להכות ואח"כ לקבץ אבל יצא הכל {{#annot:term|2447,1386|i968}}מתוקן{{#annotend:i968}} ביחד{{#annotend:shmO}} |
| − | | | ||
| − | | | ||
| − | :: | ||
| − | |||
|- | |- | ||
| | | | ||
| Line 1,124: | Line 1,163: | ||
{|class="wikitable" style="margin-left: auto; margin-right: 0px;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | |<math>\scriptstyle\frac{ | + | |<math>\scriptstyle\frac{5}{6}\;\frac{4}{5}</math>||<math>\scriptstyle\frac{2}{6}\;\frac{2}{4}</math> |
|} | |} | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Multiply all the denominators by each other; the result is 720, keep it. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{4\sdot6\sdot5\sdot6=720}}</math> |
| − | |style="text-align:right;"|תכה | + | |style="text-align:right;"|תכה האיכיות כלם זה בזה יעלה תש"כ ותשמרם |
|- | |- | ||
| | | | ||
| − | :: | + | ::Multiply the numerator of the first by the denominator of the second; it is 120. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{2\sdot2\sdot5\sdot6=120}}</math> |
| − | |style="text-align:right;"|אח"כ תכה כמות | + | |style="text-align:right;"|אח"כ תכה כמות הראשונים עם איכות השניים יהיו ק"ך |
|- | |- | ||
| | | | ||
| − | ::Multiply | + | ::[Multiply] the numerator of the second by the denominator of the first; the result is 480. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{4\sdot5\sdot4\sdot6=480}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכמות השניים עם איכות הראשונים יעלה ת"ף |
|- | |- | ||
| | | | ||
| − | :: | + | ::Add it to 120; it is 600. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{120+480=600}}</math> |
| − | |style="text-align:right;"|חברם | + | |style="text-align:right;"|חברם אל ק"ך יהיו ת"ר |
|- | |- | ||
| | | | ||
| − | :: | + | ::Relate it to 720; it is 600 parts of 720, which is 5-sixths and so is the sum of the product of these with the product of these. |
| − | ::<math>\scriptstyle{\color{blue}{4\ | + | ::<math>\scriptstyle{\color{blue}{\left(\frac{2}{4}\times\frac{2}{6}\right)+\left(\frac{4}{5}\times\frac{5}{6}\right)=600:720=\frac{600}{720}=\frac{5}{6}}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|יחסם אל תש"כ יהיו ת"ר חלקים מתש"כ והם ה' שישיות וככה העולה מקבוץ הכאת אלו עם קבוץ הכאת אלו |
| + | |- | ||
| + | |In this way, as many as the numbers are, you multiply the numerators of the whole number by all the denominators. | ||
| + | |style="text-align:right;"|וכן על דרך זה ירבו המספרים מה שירבו תכה כמיות כל המספר עם כל האיכיות | ||
| + | |} | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(\frac{a_1}{b_1}\times\frac{a_2}{b_2}\right)+\left(\frac{a_3}{b_3}\times\frac{a_4}{b_4}\right)+\cdots+\left(\frac{a_{n-1}}{b_{n-1}}\times\frac{a_n}{b_n}\right)=\frac{\left(a_1\sdot{a_2}\sdot{b_3}\cdots{b_n}\right)+\left(a_3\sdot{a_4}\sdot{b_1}\sdot{b_2}\sdot{b_5}\cdots{b_n}\right)+\cdots+\left(a_{n-1}\sdot{a_n}\sdot{b_1}\cdots{b_{n-2}}\right)}{\prod_{k=1}^n b_k}}}</math> | ||
| + | {| | ||
| + | |- | ||
| + | |If you want by way of addition, i.e. you wish to multiply this sum by that sum. | ||
| + | |style="width:45%; text-align:right;"|ואם תרצה ע"ד הקיבוץ שרצית להכות קבוץ זה עם קבוץ זה | ||
| + | |- | ||
| + | |} | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(\frac{a_1}{b_1}+\frac{a_2}{b_2}\right)\times\left(\frac{a_3}{b_3}+\frac{a_4}{b_4}\right)=\frac{\left(a_1\sdot{b_2}\sdot{b_3}\sdot{a_4}\right)+\left(a_1\sdot{b_2}\sdot{a_3}\sdot{b_4}\right)+\left(a_2\sdot{b_1}\sdot{b_3}\sdot{a_4}\right)+\left(a_2\sdot{b_1}\sdot{a_3}\sdot{b_4}\right)}{\prod_{k=1}^4 b_k}}}</math> | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{2}{4}+\frac{ | + | :For instance: you wish to know the product of [the sum of] two quarters with two sixths by the sum of 4-fifths with 5-sixths, without having to sum them, then to multiply them, and still the total result is exact. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\left(\frac{2}{4}+\frac{2}{6}\right)\times\left(\frac{4}{5}+\frac{5}{6}\right)}}</math> |
| + | |style="text-align:right;"|כגון שרצית לידע כמה העולה מהכאת הב' רביעיות עם שני ששיות עם קבוץ ד' חומשיות עם ה' ששיות בזול' שתקבצם ואח"כ להכותם אבל יצא הכל מתוקן בפעם אחת | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :Arrange them as follows: |
| + | |style="width:45%; text-align:right;"|תסדרם ככה | ||
|- | |- | ||
| | | | ||
| Line 1,163: | Line 1,217: | ||
{|class="wikitable" style="margin-left: auto; margin-right: 0px;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | |<math>\scriptstyle\frac{ | + | |<math>\scriptstyle\frac{5}{6}\;\frac{4}{5}</math>||<math>\scriptstyle\frac{2}{6}\;\frac{2}{4}</math> |
|} | |} | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Multiply the numerator of the first by the denominator of the second, the denominator of the third, and the numerator of the fourth; it is 300, keep it. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{2\sdot6\sdot5\sdot5=300}}</math> |
| − | + | |style="text-align:right;"|תכה כמות הראשון עם אכות הב' ואיכות הג' וכמות הד' יהיו ש' ושמרם | |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | ::Multiply the numerator of the first by the denominator of the second, the numerator of the third, and the denominator of the fourth; it is 288, keep it. | |
| − | | | + | ::<math>\scriptstyle{\color{blue}{2\sdot6\sdot4\sdot6=288}}</math> |
| + | |style="text-align:right;"|אח"כ תכה כמות הראשון עם איכות הב' וכמות הג' ואיכות הד' יהיו רפ"ח ושמרם | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Multiply the numerator of the second by the denominator of the first, the denominator of the third, and the numerator of the fourth; it is 200, keep it. |
| − | :<math>\scriptstyle{\color{ | + | ::<math>\scriptstyle{\color{blue}{2\sdot4\sdot5\sdot5=200}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אח"כ תכה כמות הב' עם איכות הראשון ועם איכות הג' וכמות הד' יהיו ק"ק ושמרם |
|- | |- | ||
| | | | ||
| − | :: | + | ::Multiply the numerator of the second by the denominator of the first, the numerator of the third, and the denominator of the fourth; it is 192. |
| − | ::<math>\scriptstyle{\color{blue}{\ | + | ::<math>\scriptstyle{\color{blue}{2\sdot4\sdot4\sdot6=192}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אח"כ תכה כמות הב' עם איכות הראשון וכמות הג' ואיכות הד' יהיו קצ"ב |
|- | |- | ||
| | | | ||
| − | :: | + | ::Sum the four reserved; the result is 980. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{300+288+200+192=980}}</math> |
| + | |style="text-align:right;"|חבר הד' השמורים יעלה תתק"ף | ||
|- | |- | ||
| | | | ||
| + | ::Multiply the denominators by each other; the result is 720. | ||
| + | ::<math>\scriptstyle{\color{blue}{4\sdot6\sdot5\sdot6=720}}</math> | ||
| + | |style="text-align:right;"|ותכה המורים זה בזה עד כלותם יעלה תש"כ | ||
| + | |- | ||
| | | | ||
| − | {| | + | ::Relate the 980 to it and this is the product of the sum of 2-quarters with two sixths by the sum of 4-fifths with five sixths. |
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{2}{4}+\frac{2}{6}\right)\times\left(\frac{4}{5}+\frac{5}{6}\right)=980:720}}</math> | ||
| + | |style="text-align:right;"|יחס התתק"ף אליהם הוא העולה מהכאת קבוץ ב' רביעיות עם שני ששיות עם קבוץ ד' חומשיות עם חמשה ששיות | ||
|- | |- | ||
| − | | | + | | |
|} | |} | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | + | ||
| − | + | ===Methods of checking=== | |
| + | !style="width:45%; text-align:right;"|מאזנים | ||
|- | |- | ||
| − | | | + | |Divide the result of the multiplication by one of the multiplied fractions, whichever you want, and the result of division is the other fraction. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{\left(\frac{a}{b}\times\frac{c}{d}\right)\div\frac{a}{b}=\frac{c}{d}}}</math> | |
| − | :<math>\scriptstyle{\color{OliveGreen}{\left( | + | |style="text-align:right;"|שתחלק העולה מההכאה על השב' אחד מהמוכים איזה שתרצה ויעלה השבר האחר בחלוקה |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Like this: we multiply 2-quarters by 2-sixths; the result is 4 parts of 24. |
| − | | | + | |style="text-align:right;"|כיצד הכינו ב' רביעיות עם ב' ששיות ועלה ד' חלקים מכ"ד |
|- | |- | ||
| | | | ||
| − | :: | + | ::We divide them by the 2-quarters; the result of division is two sixths. |
| − | ::<math>\scriptstyle{\color{blue}{\frac{4}{ | + | ::<math>\scriptstyle{\color{blue}{\left(\frac{2}{4}\times\frac{2}{6}\right)\div\frac{2}{4}=\frac{4}{24}\div\frac{2}{4}=\frac{2}{6}}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נחלקם על הב' רביעיות יצא בחילוק שני ששיות |
|- | |- | ||
| − | | | + | |The division procedure will be explained in its place with God's help. |
| − | | | + | |style="text-align:right;"|ודרך החלוק יתבאר במקומו בעה"ו |
| − | |||
|- | |- | ||
| − | |<math>\scriptstyle\frac{ | + | |Another scale: multiply the difference between the smaller fraction and 1 by the other. We sum the result with the primary product and [the sum] is equal to the greater fraction. |
| − | | | + | :<math>\scriptstyle{\color{OliveGreen}{\left[\left(1-\frac{c}{d}\right)\times\frac{a}{b}\right]+\left(\frac{a}{b}\times\frac{c}{d}\right)=\frac{a}{b}}}</math> |
| + | |style="text-align:right;"|מאזנים אחרים שתכה ההב' שבין השבר הקטן עד תשלום הא' שלם עם חבירו ונקבץ העולה עם העולה מהכאה ראשונה וישוה לשבר הגדול | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::In this example, we multiply 2-quarters by 2-sixths; the result is 4 parts of 24. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|במשל זה הכינו ב' רביעיות עם ב' ששיות ועלה ד' חלקים מכ"ד |
|- | |- | ||
| | | | ||
| − | :: | + | ::We take the smaller fraction, which is 2-sixths, the difference between it and the unit is 4-sixths. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|לקחנו השבר הקטן והוא הב' ששיות וההבדל שבינו ובין השלם ד' ששיות |
|- | |- | ||
| | | | ||
| − | + | ::We multiply it by 2-quarters, which is the greater fraction; the result is 8 parts of 24. | |
| − | + | |style="text-align:right;"|הכינום עם ב' רביעיות שהוא השבר הגדול ועלה ח' חלקים מכ"ד | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{ | + | ::We sum it with the 4 parts of 24, which is the primary product; [the sum] is 12 parts of 24 that are 2-quarters. |
| + | |style="text-align:right;"|קבצנו אותם עם הד' חלקים מכ"ד שהיא ההכאה הראשונה והם י"ב חלקים מכ"ד והם הב' רביעיות | ||
| + | |} | ||
| + | |||
| + | ::<math>\scriptstyle{\color{blue}{\left[\left(1-\frac{2}{6}\right)\times\frac{2}{4}\right]+\left(\frac{2}{4}\times\frac{2}{6}\right)=\left(\frac{4}{6}\times\frac{2}{4}\right)+\frac{4}{24}=\frac{8}{24}+\frac{4}{24}=\frac{12}{24}=\frac{2}{4}}}</math> | ||
| + | {| | ||
| + | |- | ||
| | | | ||
| + | ::If you want to verify that they are indeed 2-quarters, arrange them as follows: | ||
| + | |style="width:45%; text-align:right;"|ואם תרצה לאמת אם הם בעצמם הב' רביעיות סדרם ככה | ||
|- | |- | ||
| | | | ||
| − | |||
| − | |||
| − | |||
| − | |||
| | | | ||
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px;" | |
| − | |||
|- | |- | ||
| − | | | + | |<math>\scriptstyle\frac{2}{4}</math>||<math>\scriptstyle\frac{1}{2}\;\frac{2}{4}</math> |
| − | | | ||
|} | |} | ||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |Cross-multiply the numerator of the one by the denominator of the other and [the result] is equal to the product of the denominator of the one by the numerator of the other. Understand [this]. |
| − | :<math>\scriptstyle{\color{OliveGreen}{ | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}=\frac{c}{d}\longleftrightarrow b\times{c}=d\times{a}}}</math> |
| − | |style=" | + | |style="text-align:right;"|ו{{#annot:term|2626,2627|Cj00}}תכה אלכסונות{{#annotend:Cj00}} כמות הא' עם איכות חבירו וישו' העולה מהכאת כמות האחד עם איכות חבירו והבן |
|- | |- | ||
| − | | | + | |Another scale: we take the numerator of the fraction resulting from the multiplication of the fractions. We look for a number whose ratio to it is the ratio of whichever fraction you wish of the multiplied fractions. If the ratio of the required number to the numerator of the fraction resulting from the multiplication of the fractions is equal to the ratio of the remaining fraction, you were right. |
| − | :<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b} | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}\times\frac{c}{d}=\frac{a\sdot{c}}{b\sdot{d}}\longrightarrow\left(a\sdot{c}\right):x=\frac{a}{b}\longleftrightarrow x:\left(b\sdot{d}\right)=\frac{c}{d}}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|מאזנים אחרים נקח כמות השבר היוצא מהכאת השברים המוכים ונבקש מספר שיהי' יחסו אליו יחס השבר האח' מהשברים המוכים איזה שתרצה ואם היחס מספר המבוקש אל איכות השבר היוצא מהכאת השברים המוכים שוה אל יחס השבר הנשאר צדקת |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::In our example: we multiply the 2-quarters by 2-sixths; the result is 4 parts of 24. |
| + | |style="text-align:right;"|במשלינו זה הכינו הב' רביעיות עם הב' ששיות ועלה ד' חלקים מכ"ד | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{ | + | ::We look for a number, to which the 4 that is the numerator resulting from the product of the multiplied fractions is related by the ratio of 2-quarters or 2-sixths. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{2}{4}\times\frac{2}{6}=\frac{4}{24}\longrightarrow 4:x=\frac{2}{4}\longleftrightarrow x:24=\frac{2}{6}}}</math> |
| + | |style="text-align:right;"|נבקש מספר שיהיה מיוחס אליו הד' שהוא הכמות היוצא מהכאת השברים המוכי' יחס ב' רביעיות או ב' ששיות | ||
|- | |- | ||
| | | | ||
| − | : | + | ::The way to know it is that we arrange them like this: |
| − | + | |style="text-align:right;"|ודרך ידיעת זה הוא שנסדרם ככה | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| Line 1,287: | Line 1,340: | ||
{|class="wikitable" style="margin-left: auto; margin-right: 0px;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | |<math>\scriptstyle\frac{4}{ | + | |<math>\scriptstyle\frac{4}{8}\;\frac{2}{4}</math> |
|} | |} | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We cross-multiply 4 by 4; the result is 16. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ו{{#annot:term|2626,2627|PyIk}}נכה האלכסונות{{#annotend:PyIk}} שהם ד' עם ד' יעלה י"ו |
|- | |- | ||
| | | | ||
| − | + | ::We divide it by two, which is the [numerator] of 2-quarters; the result is 8. | |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{x=\frac{4\sdot{4}}{2}=\frac{16}{2}=8}}</math> |
| + | |style="text-align:right;"|נחלקם על השנים שהוא איכות הב' רביעיות יעלה ח‫' | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{ | + | ::We place it beneath the second 4; we find that for to 8 is the ratio of 2-quarters. |
| + | ::<math>\scriptstyle{\color{blue}{4:8=\frac{2}{4}}}</math> | ||
| + | |style="text-align:right;"|ונשים אותו תחת הד' הב' נמצא שארבעה מח' הוא יחס הב' רביעיות | ||
| + | |- | ||
| + | | | ||
| + | ::We relate the required number, which is 8, to the denominator of the fraction resulting from the multiplication, which is 24; we find that it is 2-sixths of it and this is the other remaining fraction. | ||
| + | ::<math>\scriptstyle{\color{blue}{8:24=\frac{2}{6}}}</math> | ||
| + | |style="text-align:right;"|וניחס מספר המבוק' שהוא הח' אל איכות השבר היוצא מההכאה שהוא כ"ד ונמצא שהם ב' ששיות ממנו וזהו השבר האחד הנשאר | ||
| + | |- | ||
| | | | ||
| + | :*If you wish to apply the second scale, look for a number, to which the ratio of 4 is as the ratio of 2-sixths. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{2}{4}\times\frac{2}{6}=\frac{4}{24}\longrightarrow 4:x=\frac{2}{6}\longleftrightarrow x:24=\frac{2}{4}}}</math> | ||
| + | |style="text-align:right;"|ואם תרצה לעשות המאזנים בהב' שתבקש מספר שיהיה יחסו הד' אליו כיחס הב' שישיות | ||
|- | |- | ||
| | | | ||
| − | : | + | ::This is by that we arrange them like this: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וזה בשנסדרם ככה |
|- | |- | ||
| | | | ||
| Line 1,310: | Line 1,375: | ||
{|class="wikitable" style="margin-left: auto; margin-right: 0px;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | |<math>\scriptstyle\frac{ | + | |<math>\scriptstyle\frac{4}{12}\;\frac{2}{6}</math> |
|} | |} | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Cross-multiply the 6 by the 4; the result is 24. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ו{{#annot:term|2626,2627|9prx}}תכה האלכסון{{#annotend:9prx}} שהוא הו' עם הד' יעלה כ"ד |
|- | |- | ||
| | | | ||
| − | ::<math>{\color{blue}{ | + | ::Divide it by 2; the quotient is 12. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{x=\frac{6\sdot{4}}{2}=\frac{24}{2}=12}}</math> |
| + | |style="text-align:right;"|חלקם על הב' יעלה י"ב בחלוקה | ||
|- | |- | ||
| | | | ||
| − | + | ::Write it beneath the 4. Take the 12 and relate it to the 24, of which it is 2-quarter, and this is the other fraction itself. | |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{12:24=\frac{2}{4}}}</math> |
| + | |style="text-align:right;"|ותשימים תחת הד' ותקח הי"ב ותיחסם אל הכ"ד והם ב' רביעיו' ממנו והוא בעצמו השבר האחר | ||
|- | |- | ||
| | | | ||
| − | + | |} | |
| − | + | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | + | ||
| − | |style="text-align:right;"| | + | == Addition of Fractions == |
| + | |style="text-align:right;"|<big>קבוץ השברים</big> | ||
|- | |- | ||
| | | | ||
| − | + | *<math>\scriptstyle{\color{OliveGreen}{\frac{a_1}{b_1}+\frac{a_2}{b_2}+\cdots+\frac{a_n}{b_n}=\frac{\sum_{k=1}^n \left[a_k\sdot\left(\prod_{i=1,i\neq{k}}^n b_i\right)\right]}{\prod_{k=1}^n b_k}}}</math> | |
| − | |||
| − | |||
| | | | ||
| − | |||
|- | |- | ||
| − | | | + | |The second species is the addition of fractions, which is that if you wish to sum up known fractions, multiply the numerator of the first by all the denominators, except that which beneath it, then relate it to the product of the denominators one by the other, or divide it by it; reduce it, meaning examine by which of them it is divided first and divide it by it. Likewise for the second [numerator and so on] until they are complete. |
| − | + | |style="width:45%; text-align:right;"|המין הב' הוא קבוץ השברים והוא שאם תרצה לקבץ שברים ידועים תכה כמות האח' עם כל האכיות זולת מה שתחתיו ותיחסהו אל הכאת האיכיות זה בזה או תחלקהו עליהם ותעשה אותם כלילת יופי רצוני שתראה לאיזה מהם מתחלק ראשונה ותחלקהו עליו ואח"כ על הב' עד תומם | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | :*Example: we wish to sum two-quarters, 4-eighths, and 3-ninths. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{2}{4}+\frac{4}{8}+\frac{3}{9}}}</math> |
| + | |style="text-align:right;"|דמיו' רצינו לקבץ שני רביעיות וד' שמניות וג' תשיעיות | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We arrange them like this: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נסדרם ככה |
|- | |- | ||
| | | | ||
| − | |||
| − | |||
| − | |||
| | | | ||
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px;" | |
| − | |style=" | ||
|- | |- | ||
| − | | | + | |<math>\scriptstyle\frac{3}{9}\;\frac{4}{8}\;\frac{2}{4}</math> |
| − | + | |} | |
| − | | | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle | + | ::Cross-multiply 2, which is the numerator of the first, by 8, and the product by 9, which is the denominator of the third; the result is 144. Keep it. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{2\sdot8\sdot9=144}}</math> |
| + | |style="text-align:right;"|תכה הב' שהוא הכמות הא' עם הח' שבאלכסונות ומה שיתקבץ עם הט' שהוא איכות הג' יעלה קמ"ד ושמרם | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Then, cross-multiply the numerator of the second, which is 4, by 4, which is the denominator of the first, and the product by 9, which is the denominator of the third; the result is 144. Keep it. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{4\sdot4\sdot9=144}}</math> |
| + | |style="text-align:right;"|אח"כ תכה כמות השני והוא הד' עם הד' שבאלכסונות שהוא איכות הראשו' ומה שיתקבץ עם הט' שהוא איכות הג' יעלה קמ"ד ושמרם | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Multiply also the numerator of 9, which is 3, by the denominator of 4, which is 8, and the product by the denominator of the second, which is 4; the result is 96. |
| − | :<math>\scriptstyle\ | + | ::<math>\scriptstyle{\color{blue}{3\sdot8\sdot4=96}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ג"כ תכה כמות הט' והוא הג' עם איכות הד' והוא הח' ומה שיתקבץ עם איכות הב' והוא הד' יעלה [.] צ"ו |
|- | |- | ||
| | | | ||
| − | : | + | ::Sum it with the two reserved number; the result is 384. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{144+144+96=384}}</math> |
| + | |style="text-align:right;"|חברם עם הב' המספרים השמורים יעלה שפ"ד | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Then, we multiply all the denominators be each other; the result is 288. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{4\sdot8\sdot9=288}}</math> |
| + | |style="text-align:right;"|אח"כ נכה כל האכיות זה בזה יעלה רפ"ח | ||
|- | |- | ||
| | | | ||
| − | : | + | ::384 of them are 1 integer and 96 parts of 288. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והם השפ"ד מהם א' שלם ‫<ref>31r</ref>וצ"ו חלקים מרפ"ח |
|- | |- | ||
| | | | ||
| − | : | + | ::If you wish to divide 384 by the denominators, divide it first by 8, then by 4, then by 9, so that you will get reduced fractions; the result of division is 1 integer and 3-ninths, which are 96 parts of 288. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{2}{4}+\frac{4}{8}+\frac{3}{9}=\frac{384}{288}=1+\frac{96}{288}=1+\frac{3}{9}}}</math> |
| + | |style="text-align:right;"|ואם תרצה לחלק השפ"ד על המורים כשתחלקהו תחלה על הח' אח"כ לד' אח"כ לט' כדי שיצאו לך החלקים נאותים ויעלה בחלוקה א' שלם וג' תשיעיות והם הצ"ו חלקים מרפ"ח | ||
| + | |- | ||
| + | |The proof is that you arrange them like this: | ||
| + | |style="text-align:right;"|והמופת שתסדרם ככה | ||
|- | |- | ||
| | | | ||
| − | + | | | |
| − | |style=" | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px;" |
| − | |||
|- | |- | ||
| − | | | + | |<math>\scriptstyle\frac{96}{288}\;\frac{3}{9}</math> |
| − | + | |} | |
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | ::Cross-multiply and [the products] are equal. This is the first method. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{96\sdot{9}=288\sdot{3}}}</math> |
| + | |style="text-align:right;"|ותכה האלכסונו' ויהיו שוים זהו הדרך הא‫' | ||
|- | |- | ||
| | | | ||
| − | + | *<math>\scriptstyle{\color{OliveGreen}{\left(1-\frac{a}{b}\right)>\frac{c}{d}}}</math> | |
| − | |||
| − | |||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |The second method: take the complement of the first fraction for a whole unit. We subtract from it the other fraction that is summed with it, if the complement is greater than it. Then, take the complement for the whole unit and this is the sum of the two fractions. |
| − | : | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}+\frac{c}{d}=1-\left[\left(1-\frac{a}{b}\right)-\frac{c}{d}\right]}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והדרך הב' שתקח החסרון אשר יחסר מהשבר הא' עד תשלומו לא' שלם ונחסר ממנו השבר הא' הנקבץ עמו אם היה החסרון גדול ממנו והיוצא קח החסר ממנו עד תשלום הא' שלם והוא סך הב' שברים |
|- | |- | ||
| | | | ||
| − | : | + | ::Example: you wish to sum two-quarters with 2-ninths. |
| − | + | ::<math>\scriptstyle{\color{blue}{\frac{2}{4}+\frac{2}{9}}}</math> | |
| − | + | |style="text-align:right;"|דמיון רצית לקבץ שני רביעיות עם ב' תשיעיו‫' | |
| − | |||
| − | |||
| − | ::<math>\scriptstyle{\color{blue}{\frac{2}{4}+\frac{ | ||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
::Arrange them like this: | ::Arrange them like this: | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תסדרם ככה |
|- | |- | ||
| | | | ||
| Line 1,435: | Line 1,495: | ||
{|class="wikitable" style="margin-left: auto; margin-right: 0px;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | |<math>\scriptstyle\frac{ | + | |<math>\scriptstyle\frac{2}{9}\;\frac{2}{4}</math> |
|} | |} | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Take the complement of 2-quarters for a whole unit; it is the other two-quarters. |
| − | + | |style="text-align:right;"|ותקח החסר מהב' רביעיות עד תשלום א' שלם והם שני רביעיות אחרים | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Subtract the 2-ninths from it; 10 parts of 36 remain. |
| − | + | |style="text-align:right;"|ותחסר מהם הב' תסיעיות ישארו י' חלקים מל"ו | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Take their complement for a whole unit; it is 26 parts of 36 and this is their sum. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{\frac{2}{4}+\frac{2}{9}=1-\left[\left(1-\frac{2}{4}\right)-\frac{2}{9}\right]=1-\left(\frac{2}{4}-\frac{2}{9}\right)=1-\frac{10}{36}=\frac{26}{36}}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וקח החסר מהם עד תשלום האחד שלם והם כ"ו חלקים מל"ו וככה קבוצם |
|- | |- | ||
| | | | ||
| − | + | *<math>\scriptstyle{\color{OliveGreen}{\left(1-\frac{a}{b}\right)<\frac{c}{d}}}</math> | |
| − | |||
| − | |||
| − | |||
| | | | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |If the complement from a whole unit is smaller than the second fraction, so you cannot subtract the other fraction from it. |
| − | + | |style="text-align:right;"|ואם מה שחסר לא' שלם הוא יותר קטן מהשבר הב' באופן שלא תוכל מחסר ממנו השבר הא‫' | |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | = | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}+\frac{c}{d}=\left[\frac{c}{d}-\left(1-\frac{a}{b}\right)\right]+1}}</math> |
| − | |||
| − | |||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | ::Such as 4-ninths with 5-sixths. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{4}{9}+\frac{5}{6}}}</math> |
| + | |style="text-align:right;"|כגו' ד' תשיעיו' עם ה' ששיות | ||
|- | |- | ||
| | | | ||
| Line 1,487: | Line 1,531: | ||
{|class="wikitable" style="margin-left: auto; margin-right: 0px;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | |<math>\scriptstyle\frac{ | + | |<math>\scriptstyle\frac{5}{6}\;\frac{4}{9}</math> |
|} | |} | ||
|- | |- | ||
| | | | ||
| − | ::::<math>\scriptstyle{\color{blue}{\left(\frac | + | ::The complement of 4-ninths for a whole unit is 5-ninths, which is less than 5-sixths. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\left(1-\frac{4}{9}\right)=\frac{5}{9}<\frac{5}{6}}}</math> |
| + | |style="text-align:right;"|שחסר לד' תשיעיות לתשלום אח' שלם הוא ה' תשיעיו' והם פחות מה' ששיות | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle\left[\left(\frac{a}{b}+\frac{c}{d}\right)+\left | + | ::We subtract 5-ninths from 5-sixths; 15 parts of 54 remain and this is the excess over the one integer. So, their sum is 1 integer and 15 parts of 54. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{4}{9}+\frac{5}{6}=\left[\frac{5}{6}-\left(1-\frac{4}{9}\right)\right]+1=\left(\frac{5}{6}-\frac{5}{9}\right)+1=\frac{15}{54}+1}}</math> |
| + | |style="text-align:right;"|הנה נחסר הה' תשיעיות מהה' ששיות ישאר ט"ו חלקים מנ"ד והם התוספת על הא' השלם וקבוצם הוא א' שלם וט"ו חלקים מנ"ד | ||
| + | |- | ||
| + | |If you wish to add a third fraction. | ||
| + | |style="text-align:right;"|ואם תרצה לקבץ עוד שב' ג‫' | ||
| + | |- | ||
| + | | | ||
| + | *<math>\scriptstyle{\color{OliveGreen}{\left[1-\left[\left(\frac{a}{b}+\frac{c}{d}\right)-1\right]\right]<\frac{e}{f}}}</math> | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}+\frac{c}{d}+\frac{e}{f}=\left[\frac{e}{f}-\left[1-\left[\left(\frac{a}{b}+\frac{c}{d}\right)-1\right]\right]\right]+1+1}}</math> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | ::As for example 6-sevenths. | ||
| + | ::<math>{\color{blue}{\scriptstyle\frac{4}{9}+\frac{5}{6}+\frac{6}{7}}}</math> | ||
| + | |style="text-align:right;"|כאלו משל ו' שביעיות | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Apply carefully the previous method, that you complete the 15 parts to a whole unit; it is 39 parts of 54. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הנה תעשה הדרך הקודם בעיון שתשלים הט"ו חלקים מנ"ד לא' שלם והוא ל"ט חלקים מנ"ד |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\left[\left(\frac | + | ::The six-sevenths are more than 39 parts of 54. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{1-\left[\left(\frac{4}{9}+\frac{5}{6}\right)-1\right]=1-\frac{15}{54}=\frac{39}{54}<\frac{6}{7}}}</math> |
| + | |style="text-align:right;"|ובעבור שהשש שביעיות יותר מל"ט חלקים מנ"ד | ||
|- | |- | ||
| | | | ||
| + | ::Hence, we subtract 39 parts of 54 from them; 51 parts of 378 remain and it is the excess over 1 integer, plus the 1 that is kept in our hand, they are 2; which is 2 and 58 parts of 378. | ||
| + | |style="text-align:right;"|הנה נחסר מהם ל"ט חלקים מנ"ד ישאר נ"א חלקים משע"ח והם התוספת על הא' השלם והא' השמור שבידינו הם ב' והם ב' ונ"[א] חלקים משע"ח | ||
|} | |} | ||
| − | + | ::<math>\scriptstyle{\color{blue}{\frac{4}{9}+\frac{5}{6}+\frac{6}{7}=\left[\frac{6}{7}-\left[1-\left[\left(\frac{4}{9}+\frac{5}{6}\right)-1\right]\right]\right]+1+1=\left[\frac{6}{7}-\left(1-\frac{15}{54}\right)\right]+1+1=\left(\frac{6}{7}-\frac{39}{54}\right)+1+1=\frac{51}{378}+1+1=2+\frac{51}{378}}}</math> | |
{| | {| | ||
|- | |- | ||
| | | | ||
| − | + | *<math>\scriptstyle{\color{OliveGreen}{\left[1-\left[\left(\frac{a}{b}+\frac{c}{d}\right)-1\right]\right]>\frac{e}{f}}}</math> | |
| − | + | | | |
| − | |||
|- | |- | ||
| − | | | + | |Likewise, if you have to subtract the fraction from it, for instance, when the complement of the result is greater than [the third fraction], take the complement of what remains and it is the sum of the three fractions. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}+\frac{c}{d}+\frac{e}{f}=1-\left[\left[1-\left[\left(\frac{a}{b}+\frac{c}{d}\right)-1\right]\right]-\frac{e}{f}\right]+1}}</math> |
| + | |style="width:45%; text-align:right;"|וכן אם היית צריך לחסר השבר האחד ממנו כגון שהיה אשר יחסר ליוצא גדול ממנו הנה ממה שישאר תשלימהו לא' והוא סך הג' שברים הנקבצים | ||
|- | |- | ||
| − | | | + | |In this way you can sum endlessly. |
| − | + | |style="text-align:right;"|וכן בזה הדרך תוכל לקבץ עד אין תכלית | |
| − | | | ||
|- | |- | ||
| − | | | + | |If you want to use another method, take the result before you subtract or add it. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם תרצה להשתמש בדרך אחרת והוא שתקח היוצא טרם שתחסרהו או תוסיפהו |
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}-\frac{c}{d} | + | *<math>\scriptstyle{\color{OliveGreen}{\left[\left(1-\frac{a}{b}\right)-\frac{c}{d}\right]<\frac{e}{f}}}</math> |
| | | | ||
|- | |- | ||
| − | | | + | |If the result is an excess over one and it is smaller than the third [fraction] that you want to sum with the two others, subtract it from that fraction and the result is the excess over the one integer. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}+\frac{c}{d}+\frac{e}{f}=\left[\frac{e}{f}-\left[\left(1-\frac{a}{b}\right)-\frac{c}{d}\right]\right]+1}}</math> |
| + | |style="text-align:right;"|ואם היה היוצא מאשר יצטרך להשלים לאחד וג"כ היה קטן מהג' אשר תרצה לקבץ עם הב' האחרים הנה תחסרהו מהשבר ההוא והיוצא הוא התוספת על השלם הא‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | ::For example: if you wish to sum 2-quarters with 2-sixths and 4-sevenths. |
| − | ::<math>\scriptstyle{\color{blue}{\frac{4}{6} | + | ::<math>\scriptstyle{\color{blue}{\frac{2}{4}+\frac{2}{6}+\frac{4}{7}}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ד"מ ואם רצית לקבץ ב' רביעיות עם ב' שישיות וד' שביעיו‫' |
|- | |- | ||
| | | | ||
| − | :: | + | ::Arrange them like this: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|סדרם ככה |
|- | |- | ||
| | | | ||
| Line 1,546: | Line 1,608: | ||
{|class="wikitable" style="margin-left: auto; margin-right: 0px;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | |<math>\scriptstyle\frac{4}{6}\;\frac{ | + | |<math>\scriptstyle\frac{4}{7}\;\frac{2}{6}\;\frac{2}{4}</math> |
|} | |} | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Take the complement of 2-quarters for one unit and subtract two-sixths from it; 4 parts of 24 remain. |
| − | + | |style="text-align:right;"|הנה תקח החסר מב' רביעיות עד הא' השלם ונחסר ממנו שני ששיות נשאר ד' חלקים מכ"ד | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We have to take the complement for one and by that the sum of the three fractions is known, but do not take the complement, take it as it is and subtract it from the third fraction, which is 4-sevenths; 68 parts of 168 remain and this is the excess over one integer. You find that their total sum is 1 and 68 parts of 198. |
| − | ::<math>\scriptstyle{\color{blue}{4\ | + | |style="text-align:right;"|ונצטרך להשלים לאחד ובזה יודע סך הג' שברים הנה לא תשלימהו לאחד אלא תקחם כמות שהם ונחסרם מהשבר הג' שהוא ד' שביעיו' ישאר ס"ח חלקים מקס"ח והם התוספת על אח' שלם תמצא סך קבצם עולה א' ס"ח חלקים מקצ"ח |
| − | | | + | |} |
| + | ::<math>\scriptstyle{\color{blue}{\frac{2}{4}+\frac{2}{6}+\frac{4}{7}=\left[\frac{4}{7}-\left[\left(1-\frac{2}{4}\right)-\frac{2}{6}\right]\right]+1=\left(\frac{4}{7}-\frac{4}{24}\right)+1=\frac{68}{168}+1}}</math> | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | + | *<math>\scriptstyle{\color{OliveGreen}{\left[\left(1-\frac{a}{b}\right)-\frac{c}{d}\right]>\frac{e}{f}}}</math> | |
| − | + | | | |
| − | |style="text-align:right;"| | + | |- |
| + | |If the result is greater than the fraction that you want to add. | ||
| + | |style="width:45%; text-align:right;"|ואם היוצא גדול מהשבר אשר תרצה לקבצו | ||
|- | |- | ||
| | | | ||
| − | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}+\frac{c}{d}+\frac{e}{f}=1-\left[\left[\left(1-\frac{a}{b}\right)-\frac{c}{d}\right]-\frac{e}{f}\right]}}</math> | |
| − | + | | | |
| − | | | ||
|- | |- | ||
| | | | ||
| − | : | + | :*As when we add one-tenth instead of the 4-sevenths. |
| − | ::<math>\scriptstyle{\color{blue}{\frac{ | + | ::<math>\scriptstyle{\color{blue}{\frac{2}{4}+\frac{2}{6}+\frac{1}{10}}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כגון שהוספנו במקום הד' שביעיות עשירית |
|- | |- | ||
| | | | ||
| − | :: | + | ::According to this diagram: |
| − | + | |style="text-align:right;"|כזה הצורה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| Line 1,583: | Line 1,646: | ||
{|class="wikitable" style="margin-left: auto; margin-right: 0px;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | |<math>\scriptstyle\frac{2}{6}\;\frac{2}{ | + | |<math>\scriptstyle\frac{1}{10}\;\frac{2}{6}\;\frac{2}{4}</math> |
|} | |} | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::The 4 parts of 24 are more than one-tenth. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\left[\left(1-\frac{2}{4}\right)-\frac{2}{6}\right]=\frac{4}{24}>\frac{1}{10}}}</math> |
| + | |style="text-align:right;"|שהנה הד' חלקים מכ"ד הם יותר גדול מעשירית | ||
|- | |- | ||
| | | | ||
| − | + | ::We subtract the one-tenth from them; 16 parts of 240 remain. | |
| − | | | + | |style="text-align:right;"|הנה נחסר העשירית מהם ישארו י"ו חלקים מר"ם |
|- | |- | ||
| | | | ||
| − | :: | + | ::We take their complement for one units; it is 224 parts of 240 and this is the sum of the three fractions. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הם נשלימם לא' שלם רכ"ד חלקים מר"ם וככה קבוצם של ג' השברים |
| + | |} | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{2}{4}+\frac{2}{6}+\frac{1}{10}=1-\left[\left[\left(1-\frac{2}{4}\right)-\frac{2}{6}\right]-\frac{1}{10}\right]=1-\left(\frac{4}{24}-\frac{1}{10}\right)=1-\frac{16}{240}=\frac{224}{240}}}</math> | ||
| + | {| | ||
|- | |- | ||
| − | | | + | |If the result is an excess over one integer, we keep the one and look for the complement of the result for one unit. |
| − | + | |style="width:45%; text-align:right;"|ואם היוצא מאשר נצטרך להוסיף על השלם האחד הנה נשמור השלם ונבקש החסרון אשר יחסר מהיוצא עד תשלום הא' השלם | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | *<math>\scriptstyle{\color{OliveGreen}{\left[1-\left[\frac{c}{d}-\left(1-\frac{a}{b}\right)\right]\right]<\frac{e}{f}}}</math> | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| | | | ||
|- | |- | ||
| − | | | + | |If this complement is smaller than the third fraction that is summed with the others, we subtract it from it, then add one to the result, and with the one that is kept in our hand it is the total sum. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}+\frac{c}{d}+\frac{e}{f}=\left[\left[\frac{e}{f}-\left[1-\left[\frac{c}{d}-\left(1-\frac{a}{b}\right)\right]\right]\right]+1\right]+1}}</math> | |
| − | |style="text-align:right;"|ואם | + | |style="text-align:right;"|ואם היה החסרון ההוא קטן מהשבר הג' הנקבץ עם האחרים הנה נחסרהו ממנו היוצא נוסיף עליו אחד ועם הא' השמור בידינו והוא סך השבר |
|- | |- | ||
| | | | ||
| − | + | *<math>\scriptstyle{\color{OliveGreen}{\left[1-\left[\frac{c}{d}-\left(1-\frac{a}{b}\right)\right]\right]>\frac{e}{f}}}</math> | |
| − | |||
| − | |||
| | | | ||
| − | :: | + | |- |
| − | |style="text-align:right;"| | + | |If [the complement] is greater than the third summed fraction, subtract the fraction from it, and take the complement of the remainder for a whole unit, then add to it the one that is kept in our hand and it is the sum of the three fractions. |
| + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}+\frac{c}{d}+\frac{e}{f}=\left[1-\left[\left[1-\frac{c}{d}-\left(1-\frac{a}{b}\right)\right]-\frac{e}{f}\right]\right]+1}}</math> | ||
| + | |style="text-align:right;"|ואם היה החשבון גדול מהשבר הג' הנקבץ הנה תחסר השבר ממנו והיוצא תשלימהו לא' שלם והוסיף עליו הא' השמור אשר בידנו והוא סך הג' שברים | ||
| + | |- | ||
| + | |Understand this. | ||
| + | |style="text-align:right;"|והבן | ||
| + | |- | ||
| + | |The third addition method | ||
| + | |style="text-align:right;"|והדרך הג' לקבוץ | ||
| + | |- | ||
| + | |One should know one proposition, which is that there are two types of numbers: relatively prime and relatively composite. | ||
| + | |style="text-align:right;"|צריך לידע {{#annot:term|2632,2633|Q627}}הקדמה{{#annotend:Q627}} א' והוא שהמספרים ב' מנים נבדלים משותפים | ||
| + | |- | ||
| + | |The relatively prime numbers are those that are not counted by any number [= not share a common divisor] other than 1. | ||
| + | |style="text-align:right;"|הנבדלים הם שלא ימנה לשנים מספר זולת הא‫' | ||
| + | |- | ||
| + | |The relatively composite numbers are of two types: either one counts [= is a divisor of] the other, or not, but there is another number that counts both [= a common divisor of both]. | ||
| + | |style="text-align:right;"|והמשותפים שני מינים או ימנה האח' את חברו או לא ימנהו אלא שיש מספר אח' ימנה את שניהם | ||
| + | |- | ||
| + | |The way to identify these types is that you divide the greater by the smaller and the quotient resulting from the division indicates the reduced ratio. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{a\sdot{b}\div{a}=b}}</math> | ||
| + | |style="text-align:right;"|והדרך אל ידיעת אלו המנים בשתחלק הגדול על הקטן והחלק היוצא בחלוק מורה על {{#annot:term|2624,2463|bkdb}}קטן היחס{{#annotend:bkdb}} | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::For, if the two proportional numbers are ten and 100, divide 100 by 10 and the quotient is 10, and this 10 indicates that 100 is 10 times 10. |
| − | ::<math>\scriptstyle{\color{blue}{\ | + | ::<math>\scriptstyle{\color{blue}{100\div{10}=10\longleftrightarrow 100=10\times{10}}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כי אם היו שני המספרים המתיחסים עשרה וק' תחלק הק' על הי' ויצא בחלוקה י' ואלה <s>הם</s> <sup>הי'</sup> מורים שק' י' פעמים כפל הי‫' |
| + | |- | ||
| + | |If something remains, divide the smaller number by the remainder, then the first remainder by the second remainder and so on do not cease from dividing the remainder by the remainder until the number ends, so that no remainder is left, and [the last remainder] is the greatest number that counts both of them [= their greatest common divisor]. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(a\sdot b\right)\div\left(c\sdot b\right)=\left[\left(a\sdot b\right)\div b\right]\div\left[\left(c\sdot b\right)\div b\right]=a\div c}}</math> | ||
| + | |style="text-align:right;"|ואם ישאר כלום תחלק המספר הקטן על המותר אח"כ המותר האח' על המותר הב' ולא תסור מחלוק המותר על המותר עד שיכלה המספר שלא ישאר בו מותר והוא גדול המספר אשר ימנם יחד | ||
|- | |- | ||
| − | | | + | |Afterwards, divide the smaller number by it and keep the result. |
| − | + | |style="text-align:right;"|אח"כ חלק המספר הקטן עליו והעולה שמרהו | |
| − | |||
|- | |- | ||
| − | | | + | |Look also for the number of times that it counts the greater, by dividing it by it, and keep the result. |
| − | + | |style="text-align:right;"|גם בקש מספר הפעמים אשר ימנהו הגדול וזה כשתחלקהו עליו והעולה שמרהו | |
| − | | | ||
|- | |- | ||
| − | | | + | |The two reserved [numbers] are the smallest proportional numbers of this ratio. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והב' שמורים הם קטני היחס ההוא |
|- | |- | ||
| | | | ||
| − | : | + | ::Example for relatively composite numbers: 12 and [the greater] number 27. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|המשל השות' י"ב והמספר הקטן כ"ז |
|- | |- | ||
| | | | ||
| − | :: | + | ::Divide 27 by 12; 3 remain. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|חלקהו הכ"ז על הי"ב נשארו ג‫' |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | ::We divide 12 be it; nothing remains. So we find that the greatest number that counts both together [= their greatest common divisor] is 3. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|חלקנו עליהם הי"ב ולא נשאר כלום ולכן מצינו שגדול המספר אשר ימנם יחד הוא ג‫' |
|- | |- | ||
| | | | ||
| + | ::We look for the number of times that it counts the 12, by dividing 12 by 3; the result is 4. We keep it. | ||
| + | |style="text-align:right;"|בקשנו מספר הפעמים אשר ימנה הי"ב הוא בשחלקנו הי"ב על הג' ועלה ד' ושמרנום | ||
| + | |- | ||
| | | | ||
| − | + | ::We divide 27 by 3; the result is 9. We keep it. | |
| + | |style="text-align:right;"|חלקנו הכ"ז על הג' ועלה ט' ושמרנום | ||
|- | |- | ||
| − | |<math>\scriptstyle\frac{ | + | | |
| − | |} | + | ::We know that 4 and 9 are the smallest proportional numbers of this ratio, so we know that the ratio of 12 to 27 is 4-ninths. |
| + | ::<math>\scriptstyle{\color{blue}{12:27=12\div27=\left(12\div 3\right)\div\left(27\div 3\right)=4\div 9=\frac{4}{9}}}</math> | ||
| + | |style="text-align:right;"|וידענו שהד' והט' הם {{#annot:term|2624,2463|Cyg5}}קטני היחס{{#annotend:Cyg5}} ההוא ולכן ידענו שהי"ב יחסו אל הכ"ז ד' תשיעיות | ||
| + | |- | ||
| + | |Therefore, when you want to find the smallest number in which given fractions are found, whichever they are, if their denominators are relatively prime, they are multiplied by each other and the product is the smallest number, in which these given fractions are found. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{LCM\left(p,q\right)=p\sdot q}}</math> | ||
| + | |style="text-align:right;"|ולכן כשתרצה לדעת קטן המספרים אשר ימצאו בו השברים המונחים איזה שברים שיהיו עם איכיותיהם נבדלים זה מזה יוכו זה עם זה והעולה הוא קטן המספר אשר מצאו בו אלו החלקים ההם המונחים | ||
| + | |- | ||
| + | |If they are relatively composite and one counts the other, the greater number is the lowest common multiple, in which these given fractions are found. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{LCM\left[\left(a\sdot b\right),b\right]=a\sdot b}}</math> | ||
| + | |style="text-align:right;"|אם יהיו משותפים והאח' מונה אחר המספר הגדול ‫<ref>31v</ref>הוא קטן היחס אשר ימצאו השברי' המונחים | ||
| + | |- | ||
| + | |If it does not count it, but there is a number that counts them together, we look for their lowest common multiple according to the previous way. | ||
| + | |style="text-align:right;"|ואם לא ימנהו אלא שיש מספר ימנם יחד נבקש {{#annot:term|2624,2628|Gu26}}קטן יחסם{{#annotend:Gu26}} ע"ד הקודם | ||
| + | |- | ||
| + | |Then, we multiply the greater number among the two given denominators by the smaller number of their ratio in the lowest term. | ||
| + | |style="text-align:right;"|ונכה המספר הגדול מב' האיכות המונחים עם המספר הקטון מב' איכות קטן היחס | ||
| + | |- | ||
| + | |Or, We multiply the smaller number among the two given denominators by the greater number of their ratio in the lowest term. | ||
| + | |style="text-align:right;"|או נכה המספר הקטן מב' איכיות המונחים עם המספר הגדול מב' איכיות קטן היחס | ||
| + | |- | ||
| + | |The result is the smallest number, in which the two given fractions are found [= LCM]. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{LCM\left[\left(a\sdot b\right),\left(c\sdot b\right)\right]=\left(a\sdot b\right)\sdot c=a\sdot\left(c\sdot b\right)}}</math> | ||
| + | |style="text-align:right;"|והעולה הוא קטן המספר אשר ימצאו בו ב' שברים ההם | ||
| + | |- | ||
| + | |If there are numerous fractions apply this same method: find the lowest common multiple, in which these two fractions are found, according to what preceded. | ||
| + | |style="text-align:right;"|ואם היו השברים רבים תעש' בזה הדרך עצמו תמצא {{#annot:term|2624,2628|BsIH}}קטן היחס{{#annotend:BsIH}} אשר ימצאו בו ב' שברים ההם לפי מה שקדם | ||
| + | |- | ||
| + | |If the third fraction counts it, the lowest common multiple, in which the former fractions are found, is also the lowest common multiple of the three fractions. | ||
| + | |style="text-align:right;"|עם השבר הג' מונהו הנה קטן היחס אשר ימצאו בו השברים הקודמים בו ג"כ ימצא קטון היחס של הג' שברים | ||
| + | |- | ||
| + | |If they are relatively composite, we multiply it by it. | ||
| + | |style="text-align:right;"|ואם הם נבדלים נכהו עמו | ||
| + | |- | ||
| + | |If another number counts both of them, we multiply [the smaller number of] their ratio in the lowest term by the greater number, which is the lowest common multiple, in which the two former fractions are found. | ||
| + | |style="text-align:right;"|ואם מספר אחר ימנם יחד נכה {{#annot:term|2624,2628|qAyd}}המספר הקטון יחסם{{#annotend:qAyd}} עם המספר הגדול שהוא המספר קטן היחס אשר נמצא בו הב' שברים הקודמים | ||
|- | |- | ||
| − | | | + | |The same way for more fractions |
| − | |style=" | + | |style="text-align:right;"|וכן ע"ז הדרך ירבו השברי‫' |
|- | |- | ||
| | | | ||
| − | + | :<math>\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n \frac{a_k}{b_k}=\frac{\sum_{k=1}^n \left[\frac{a_k}{b_k}\sdot LCM\left(b_1,b_2,\cdots,b_n\right)\right]}{LCM\left(b_1,b_2,\cdots,b_n\right)}}}</math> | |
| − | |||
| − | |||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |Therefore, if you wish to sum up some fractions, we take their lowest common multiple according to the said way, divide it by all the denominators as the number of their numerators and keep each separately, then we sum up [the reserved numbers] and divide [the sum] by their lowest common multiple and this is the result. |
| − | + | |style="text-align:right;"|ולכן אם תרצה לקבץ שברים מה הנה ניקח קטון היחסם ע"ד האמור ונחלקהו על כל האיכיות כפי מנין כמותם ונשמור כל אחד בפני עצמו אח"כ נקבצם ונחלקם על מספר קטון יחסם וזהו העולה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | :*For example: we wish to sum 2-quarters, 4-sixths, 8-ninths and 5-eighths. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{2}{4}+\frac{4}{6}+\frac{8}{9}+\frac{5}{8}}}</math> |
| + | |style="text-align:right;"|ד"מ בזה רצינו לקבץ ב' רביעיות וד' ששיות וח' תשיעיות וה' שמניות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Arrange them like this: |
| + | |style="text-align:right;"|סדרם ככה | ||
|- | |- | ||
| | | | ||
| Line 1,687: | Line 1,802: | ||
{|class="wikitable" style="margin-left: auto; margin-right: 0px;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | |<math>\scriptstyle\frac{ | + | |<math>\scriptstyle\frac{5}{8}\;\frac{8}{9}\;\frac{4}{6}\;\frac{2}{4}</math> |
|} | |} | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::4 and 6 are counted by 2. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והד' והו' ימנם מספר הב‫' |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Apply the said procedure: we divide 6 by 4; 2 remains. |
| + | |style="text-align:right;"|כשתעשה הדרך האמור שנחלק הו' על הד' וישאר ב‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Then, divide 4 by 2; there is no remainder, so we know that two is the number that counts both of them [= their greatest common divisor] |
| + | |style="text-align:right;"|אח"כ תחלק הד' על הב' ולא ישא' כלום ידענו שהשנים הוא מספר ימנם יחד | ||
|- | |- | ||
| − | | | + | | |
| − | + | ::Hence, we multiply it by 6, or 3, which is the number of times that 2 counts 6, by 4; the result is 12 and this is the lowest common multiple that has a quarter and a sixth. | |
| − | {| | + | ::<math>\scriptstyle{\color{blue}{ LCM\left(4,6\right)=LCM\left[\left(2\sdot2\right),\left(3\sdot2\right)\right]=2\sdot6=3\sdot4=12}}</math> |
| + | |style="text-align:right;"|ולכן נכהו עם הו' או נכה הג' שהוא מספר הפעמים שישנה הו' הב' עם ד' יעלה י"ב וזהו קטון היחס אשר יש בו רביעית וששית | ||
|- | |- | ||
| | | | ||
| − | + | ::Thereupon, we know that 9 is relatively prime to 12 [sic], so we multiply it by 9; the result is 108. | |
| − | = | + | |style="text-align:right;"|אח"כ ידענו שהט' הוא מספר נבדל מהי"ב ולכן הכנו אותו עם הט' יעלה ק"ח |
| − | | | + | |- |
| − | :<math> | + | | |
| − | + | ::According to the previous method, we find that 4 counts both 8 and 108, so we multiply 4 by 108; the result is 432 and this is the smallest number [sic] that has a quarter, a sixth, a ninth, and an eighth. | |
| + | ::<math>\scriptstyle{\color{blue}{\left(12\sdot9\right)\sdot4=108\sdot4=432}}</math> | ||
| + | |style="text-align:right;"|וע"ד הקודם מצאנו שהד' ימנה לח' ולק"ח והכנו הד' עם הק"ח ועלה תל"ב וזהו קטון המספר אשר ימצא בו רביעית וששית ותשיעית ושמנית | ||
|- | |- | ||
| | | | ||
| − | |style=" | + | ::Then, we divide 432 by 6 four times as its numerator; the result is 288. |
| + | ::<math>\scriptstyle{\color{blue}{4\sdot\left(432\div6\right)=288}}</math> | ||
| + | |style="text-align:right;"|ואח"כ חלקנו התל"ב על הו' ד' פעמים כמנין כמותם ועלה רפ"ח | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Then, we divide 432 by 9 eight times as its numerator; the result is 384. We keep it. |
| + | ::<math>\scriptstyle{\color{blue}{8\sdot\left(432\div9\right)=384}}</math> | ||
| + | |style="text-align:right;"|אח"כ חלקנו התל"ב על הט' ח' פעמים כמנין כמותם ועלה שפ"ד ושמרנום | ||
| + | |- | ||
| | | | ||
| − | + | ::Then, we divide 432 by 8 five times as its numerator; the result is 270. | |
| − | + | ::<math>\scriptstyle{\color{blue}{5\sdot\left(432\div8\right)=270}}</math> | |
| − | + | |style="text-align:right;"|אח"כ חלקנו התל"ב על הח' ה' פעמים כמנין כמותם ועלה ר"ע | |
| − | | | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We sum up the four reserved [numbers]; the result is 1158. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{216+288+384+270=1158}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|חברנו הד' שמורים יעלה אלף וקנ"ח |
|- | |- | ||
| | | | ||
| − | : | + | ::Its ratio to 432 is two integers and 294 parts of 432. |
| − | ::<math>\scriptstyle{\color{blue}{\ | + | ::<math>\scriptstyle{\color{blue}{\frac{2}{4}+\frac{4}{6}+\frac{8}{9}+\frac{5}{8}=\frac{1158}{432}=2+\frac{294}{432}}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|יחסם אל תל"ב יהיו שנים שלמים ורצ"ד חלקים מתל"ב |
|- | |- | ||
| − | | | + | |Q.E.D. |
| − | + | |style="text-align:right;"|וזה מה שרצינו לבאר | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | |||
| − | |||
| − | |||
|} | |} | ||
| − | + | ||
{| | {| | ||
|- | |- | ||
| | | | ||
| − | |style="width:45%; text-align:right;"| | + | ===Methods of checking=== |
| + | |style="width:45%; text-align:right;"|<big>מאזנים</big> | ||
|- | |- | ||
| | | | ||
| − | + | *<math>\scriptstyle{\color{OliveGreen}{\left(\frac{a}{b}+\frac{c}{d}\right)-\frac{a}{b}=\frac{c}{d}}}</math> | |
| − | |style="text-align:right;"| | + | | |
| + | |- | ||
| + | |We subtract whichever of the [summed] fractions you wish and the other remains. | ||
| + | |style="text-align:right;"|שנחסר השבר הנשאר איזה מהם שתרצה וישאר האחר | ||
| + | |- | ||
| + | | | ||
| + | :*Example: we sum up two-quarters with 2-sixths. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{2}{4}+\frac{2}{6}}}</math> | ||
| + | |style="text-align:right;"|המשל קבצנו שני רביעיות עם ב' ששיות | ||
| + | |- | ||
| + | | | ||
| + | ::Like this: | ||
| + | |style="text-align:right;"|כזה | ||
|- | |- | ||
| | | | ||
| Line 1,755: | Line 1,887: | ||
{|class="wikitable" style="margin-left: auto; margin-right: 0px;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | |<math>\scriptstyle\frac{ | + | |<math>\scriptstyle\frac{2}{6}\;\frac{2}{4}</math> |
|} | |} | ||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | ::The result is twenty parts of 24. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ועלה עשרים חלקים מכ"ד |
| + | |- | ||
| + | | | ||
| + | ::Subtract 2-quarters from it; two-sixths remain. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{2}{4}+\frac{2}{6}\right)-\frac{2}{4}=\frac{20}{24}-\frac{2}{4}=\frac{2}{6}}}</math> | ||
| + | |style="text-align:right;"|חסר מהם הב' רביעיות ונשארו שני ששיות | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Or, subtract 2-sixths; 2-quarters remain. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\left(\frac{2}{4}+\frac{2}{6}\right)-\frac{2}{6}=\frac{20}{24}-\frac{2}{6}=\frac{2}{4}}}</math> |
| + | |style="text-align:right;"|או חסר הב' ששיות וישארו הב' רביעיות | ||
|- | |- | ||
| | | | ||
| − | + | *<math>\scriptstyle{\color{OliveGreen}{\left[\left(\frac{a}{b}+\frac{c}{d}\right)+\left(1-\frac{a}{b}\right)\right]-1=\frac{c}{d}}}</math> | |
| − | |style="text-align:right;"| | + | | |
| + | |- | ||
| + | |Another scale: add to the sum the complement for one of whichever of the summed fractions you wish, then subtract one from the result, and if the remainder is equal to [the sum of] the remaining summed [fractions], you were right. | ||
| + | |style="text-align:right;"|מאזנים אחרים שתקבץ עם המקובץ החסר משלימו איזה שבר שתרצה מהנקבצין עד האחד השלם והעולה תשליך ממנו אחד ואם הנשאר שוה לעולה מהנקבצין הנשארים צדקת | ||
|- | |- | ||
| | | | ||
| − | : | + | ::As when we take 2-quarters, which is its [own] complement for one unit, we sum it with 20 parts of 24; the result is 1 and 2-sixths. We subtract one; the remainder is 2-sixths. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\left[\left(\frac{2}{4}+\frac{2}{6}\right)+\left(1-\frac{2}{4}\right)\right]-1=\left(\frac{20}{24}+\frac{2}{4}\right)-1=\left(1+\frac{2}{6}\right)-1=\frac{2}{6}}}</math> |
| + | |style="text-align:right;"|כגון שלקחנו הב' רביעיות שחסר משלמותך השלם וקבצנום עם כ' חלקים מכ"ד יעלה א' וב' ששיות השלכנו האח' נשאר הב' ששיות | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Or, we sum the complement of two-sixths, which is 4-sixths, with 20 parts of 24; the result is one and 3-sixths. We subtract one; the remainder is 3-sixths, which are two-quarters. |
| − | |style="text-align:right;"|אח | + | ::<math>\scriptstyle{\color{blue}{\left[\left(\frac{2}{4}+\frac{2}{6}\right)+\left(1-\frac{2}{6}\right)\right]-1=\left(\frac{20}{24}+\frac{4}{6}\right)-1=\left(1+\frac{3}{6}\right)-1=\frac{3}{6}=\frac{2}{4}}}</math> |
| + | |style="text-align:right;"|או נקבץ החסר משני ששיות שהם ד' ששיות עם כ' חלקים מכ"ד יעלה אח' וג' שישיות והשלכנו האחד ונשאר ג' ששיות והם שני רביעיות | ||
| + | |- | ||
| + | |Understand this. | ||
| + | |style="text-align:right;"|והבן | ||
|- | |- | ||
| | | | ||
| − | |||
| − | |||
|} | |} | ||
| − | + | ||
{| | {| | ||
|- | |- | ||
| | | | ||
| − | |style="width:45%; text-align:right;"| | + | |
| + | ==Subtraction of Fractions== | ||
| + | |style="width:45%; text-align:right;"|<big>דרך החסור</big> | ||
|- | |- | ||
| − | | | + | |Now we start to explain the method of subtraction by the help of God. |
| − | + | |style="text-align:right;"|ועתה נתחיל לבאר דרך החסור בע"ה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| + | *<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}-\frac{c}{d}=\frac{\left(a\sdot d\right)-\left(c\sdot b\right)}{b\sdot d}}}</math> | ||
| | | | ||
| − | |||
|- | |- | ||
| − | | | + | |I say that the most common method is that you multiply the numerator of the one by the denominator of the other and the numerator of the other by the denominator of the one, then subtract one from the other and relate the remainder to the product of the denominators. |
| − | + | |style="text-align:right;"|ואו' שהדרך היותר כולל הוא שתכה כמות האחד עם איכות חבירו וכמות האחר עם איכות חבירו וחסר זה מזה והנשאר [תיחס]<ref>marg.</ref> אל הכאת האיכות | |
|- | |- | ||
| | | | ||
| − | + | *<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}-\frac{c}{d}=\frac{\frac{\left(a\sdot d\right)-\left(c\sdot b\right)}{b}}{d}}}</math> | |
| − | |||
| − | |||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |Or, divide by the denominators according to the previous way, by reducing them and this is the required. |
| − | + | |style="text-align:right;"|או תחלקהו על המורים ע"ד הקודם בשתעשה להם כלילת יופי והוא המבוקש | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | :*<math>\scriptstyle{\color{blue}{ | + | :*Example: we wish to subtract 3-eighths from 4-sixths. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{4}{6}-\frac{3}{8}}}</math> |
| − | + | |style="text-align:right;"|דרך משל רצינו לחסר ג' שמניות מד' ששיות | |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | ::As this diagram: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כזה הצורה |
|- | |- | ||
| | | | ||
| Line 1,827: | Line 1,964: | ||
{|class="wikitable" style="margin-left: auto; margin-right: 0px;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | |<math>\scriptstyle\frac{4}{ | + | |<math>\scriptstyle\frac{4}{6}\;\frac{3}{8}</math> |
|} | |} | ||
|- | |- | ||
| | | | ||
| − | :<math>{\color{blue | + | ::Multiply the numerator of 3 by the denominator of 4; it is 18. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{3\sdot6=18}}</math> |
| + | |style="text-align:right;"|תכה כמות הג' עם איכות הד' יהיו י"ח | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Multiply the numerator of the other by the denominator of the first; it is 32. |
| + | ::<math>\scriptstyle{\color{blue}{4\sdot8=32}}</math> | ||
| + | |style="text-align:right;"|ותכה הכמות האחר עם איכות חבירו יהיו ל"ב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Subtract 18 from it; 14 remain. |
| + | ::<math>\scriptstyle{\color{blue}{32-18=14}}</math> | ||
| + | |style="text-align:right;"|תחסר מהם י"ח ישאר י"ד | ||
|- | |- | ||
| | | | ||
| − | + | ::Multiply the denominators, which are 8 by 6; it is 48. | |
| − | + | ::<math>\scriptstyle{\color{blue}{6\sdot8=48}}</math> | |
| − | :<math> | + | |style="text-align:right;"|ותכה האיכיות והם הח' בו' יהיו מ"ח |
| − | |||
|- | |- | ||
| | | | ||
| − | |style=" | + | ::It is 14 parts of 48. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{4}{6}-\frac{3}{8}=\frac{14}{48}}}</math> | ||
| + | |style="text-align:right;"|והם י"ד חלקים ממ"ח | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Or, divide 14 by 6; the result is 2 and 2 remain, which are two-eighths and 2-sixths and this is their diagram: |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{4}{6}-\frac{3}{8}=\frac{14}{48}=\frac{2}{8}+\left(\frac{2}{6}\sdot\frac{1}{8}\right)}}</math> |
| + | |style="text-align:right;"|או תחלק הי"ד על הו' יעלה ב' וישארו ב' והם שני שמניות וב' ששיות וככה צורתם | ||
|- | |- | ||
| | | | ||
| Line 1,857: | Line 2,001: | ||
{|class="wikitable" style="margin-left: auto; margin-right: 0px;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | |<math>\scriptstyle | + | |<math>\scriptstyle\frac{2}{6}\;\frac{2}{8}</math> |
|} | |} | ||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | ::This is the remainder of the subtraction of 3-eighths from 4-sixths. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והוא הנשאר מחסור הג' שמניות מד' ששיות |
|- | |- | ||
| | | | ||
| − | + | *<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}-\frac{c}{d}=1-\left[\frac{c}{d}+\left(1-\frac{a}{b}\right)\right]}}</math> | |
| − | | | + | | |
|- | |- | ||
| | | | ||
| − | :: | + | ::Another way is that you complete the greater fraction, which is 4-sixths, into one integer. We complete it by two-sixths. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|דרך אחרת שתשלים השבר הגדול שהוא ד' ששיות לא' שלם והנה השלמנום בשני ששיות |
|- | |- | ||
| | | | ||
| − | : | + | ::We sum it up with the smaller fraction, which is 3-eighths; the result is 34 parts of 48. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|קבצנום עם השבר הקטן והוא הג' שמניות יעלה ל"ד חלקים ממ"ח |
|- | |- | ||
| | | | ||
| − | :: | + | ::We complete it into one integer by 14 parts of 48 and this is the required. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{4}{6}-\frac{3}{8}=1-\left[\frac{3}{8}+\left(1-\frac{4}{6}\right)\right]=1-\left(\frac{3}{8}+\frac{2}{6}\right)=1-\frac{34}{48}=\frac{14}{48}}}</math> |
| + | |style="text-align:right;"|השלמנום לאח' שלם בי"ד חלקי' ממ"ח והוא המבוקש | ||
|- | |- | ||
| | | | ||
| − | + | *<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}-\frac{c}{d}=\left[\frac{a}{b}+\left(1-\frac{c}{d}\right)\right]-1}}</math> | |
| − | | | + | | |
|- | |- | ||
| | | | ||
| − | : | + | ::If you wish, sum up the complement of the smaller fraction, which is 3-eighths. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם תרצה קבץ החסר מהשבר הקטן והוא הג' שמניות |
|- | |- | ||
| | | | ||
| − | : | + | ::We complete it into one integer by 5-eighths. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|השלמנום לאח' שלם בה' שמניות |
|- | |- | ||
| − | | | + | | |
| − | + | ::We sum it up with 4-sixths; the result is 1 integer and 14 parts of 48. | |
| − | + | |style="text-align:right;"|נקבצו עם הד' ששיות ועלה א' שלם וי"ד חלקים ממ"ח | |
|- | |- | ||
| | | | ||
| − | + | ::We subtract one integer and the remainder is the required. | |
| − | == | + | ::<math>\scriptstyle{\color{blue}{\frac{4}{6}-\frac{3}{8}=\left[\frac{4}{6}+\left(1-\frac{3}{8}\right)\right]-1=\left(\frac{4}{6}+\frac{5}{8}\right)-1=\left(1+\frac{14}{48}\right)-1=\frac{14}{48}}}</math> |
| + | |style="text-align:right;"|והשלכנו האחד השלם ומה שישאר הוא המבוקש | ||
| + | |- | ||
| | | | ||
| + | === Methods of checking === | ||
| + | |style="width:45%; text-align:right;"|<big>מאזנים</big> | ||
|- | |- | ||
| | | | ||
| − | + | *<math>\scriptstyle{\color{OliveGreen}{\left[\frac{a}{b}+\left(\frac{a}{b}-\frac{c}{d}\right)\right]-\frac{c}{d}=2\sdot\left(\frac{a}{b}-\frac{c}{d}\right)}}</math> | |
| − | |||
| | | | ||
|- | |- | ||
| − | | | + | |Sum up the remainder from the subtraction of the fraction from the fraction with the greater fraction, then subtract the smaller [fraction] from the sum, and if the remainder is double the remainder from the [original] subtraction, you were right. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|שתקבץ הנשאר מחיסור השבר מהשבר עם השבר הגדול והעולה מקבוצם חסר ממנו הקטן והנשאר ממנו אם היה כפל הנשאר מהחסור צדקת |
|- | |- | ||
| | | | ||
| − | : | + | :*In our example: we sum up the 14 parts of 48 with the 4-sixths; the result is 276 parts of 288. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|במשלינו זה קבצנו הי"ד חלקים ממ"ח עם הד' ששיות ועלה רע"ו חלקי' מרפ"ח |
|- | |- | ||
| | | | ||
| − | ::::<math>\scriptstyle{\color{blue}{\ | + | ::We subtract 3-eighth from them; the remainder is 1344 parts of 2304 and it is double the 14 parts of 48. |
| − | | | + | |style="text-align:right;"|חסרנו מהם הג' שמניות ונשאר אלף ושמ"ד חלקים מהאלפים וש"ד והם כפל הי"ד חלקים ממ"ח |
| + | |} | ||
| + | ::<math>\scriptstyle{\color{blue}{\left[\frac{4}{6}+\left(\frac{4}{6}-\frac{3}{8}\right)\right]-\frac{3}{8}=\left(\frac{4}{6}+\frac{14}{48}\right)-\frac{3}{8}=\frac{276}{288}-\frac{3}{8}=\frac{1344}{2304}=2\sdot\frac{14}{48}=2\sdot\left(\frac{4}{6}-\frac{3}{8}\right)}}</math> | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The proof is that you write the [2]4 parts of 48 and the 1344 parts of 2304 in this diagram: |
| + | ::<math>\scriptstyle{\color{blue}{1344\sdot48=2304\sdot28}}</math> | ||
| + | |style="text-align:right;"|והמופת שתשי' ה[כ"]ד חלקים ממ"ח והאלף ושמ"ד חלקים מאלפים וש"ד בזאת הצורה | ||
|- | |- | ||
| | | | ||
| − | = | + | | |
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px;" | |
|- | |- | ||
| − | | | + | |<math>\scriptstyle\frac{1344}{2304}\;\frac{28}{48}</math> |
| − | | | + | |} |
|- | |- | ||
| | | | ||
| − | + | ::Then, cross multiply and [the products] are equal. | |
| − | |style="text-align:right;"| | + | |style="width:45%; text-align:right;"|ותכה האלכסונות ויהיו שוים |
|- | |- | ||
| | | | ||
| − | + | *<math>\scriptstyle{\color{OliveGreen}{\left(\frac{a}{b}-\frac{c}{d}\right)+\frac{c}{d}=\frac{a}{b}}}</math> | |
| − | |style="text-align:right;"| | + | | |
| + | |- | ||
| + | |Another scale: sum up the remainder from the subtraction of the fraction from the fraction with the smaller fraction, then if [the remainder] is equal to the greater [fraction], you were right. | ||
| + | |style="text-align:right;"|<big>מאזנים</big> אחרים שתקבץ הנשאר מחיסור השבר מהשבר עם השבר הקטן ואם ישוה לגדול צדקת | ||
|- | |- | ||
| | | | ||
| − | * | + | :*We sum 14 parts of 48 with 3-eighths; the result is 4-sixths. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\left(\frac{4}{6}-\frac{3}{8}\right)+\frac{3}{8}=\frac{14}{48}+\frac{3}{8}=\frac{4}{6}}}</math> |
| + | |style="text-align:right;"|הרי קבצנו הי"ד חלקים ממ"ח עם הג' שמניות ועלה ד' ששיות | ||
|- | |- | ||
| | | | ||
| − | + | *<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}-\left(\frac{a}{b}-\frac{c}{d}\right)=\frac{c}{d}}}</math> | |
| − | |||
| − | |||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |Another scale: subtract the remainder from the greater fraction, then if the remainder is equal to the smaller [fraction], know that you were right. |
| − | + | |style="text-align:right;"|מאזנים אחרים חסר הנשאר מהשבר הגדול והנשאר אם ישוה לקטן דע שצדקת | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | :*In our example: we subtract fourteen parts of 48 from 4-sixths; 108 parts of 288 remain and they are the 3-eighths. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{4}{6}-\left(\frac{4}{6}-\frac{3}{8}\right)=\frac{4}{6}-\frac{14}{48}=\frac{108}{288}=\frac{3}{8}}}</math> |
| + | |style="text-align:right;"|במשלינו זה חסרנו הארבעה עשר חלקים ‫<ref>32r</ref>ממ"ח מד' ששיות ישארו ק"ח חלקים מרפ"ח והם הם הג' שמיניות | ||
|- | |- | ||
| | | | ||
| − | ::: | + | ::The proof is that you arrange them as follows: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והמופת שתסדרם ככה |
|- | |- | ||
| | | | ||
| Line 1,964: | Line 2,116: | ||
{|class="wikitable" style="margin-left: auto; margin-right: 0px;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | |<math>\scriptstyle\frac{ \; | + | |<math>\scriptstyle\frac{3}{8}\;\frac{108}{288}</math> |
|} | |} | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | ::Then, cross multiply and [the products] are equal. |
| + | ::<math>\scriptstyle{\color{OliveGreen}{3\sdot288=8\sdot108}}</math> | ||
| + | |style="text-align:right;"|ותכה האלכסונות ויהיו שוים | ||
|- | |- | ||
| − | | | + | |It is enough for the one who understands. |
| − | + | |style="text-align:right;"|ודי למבין | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | *<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}-\left(\frac{a}{b}-\frac{c}{d}\right)=\frac{c}{d}}}</math> | |
| − | |||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |Another scale: subtract the remainder from the greater fraction, then if the remainder is equal to the smaller [fraction], know that you were right. |
| − | + | |style="text-align:right;"|<big>מאזנים</big> אחרים חסר הנשאר מהשבר הגדול והנשאר אם שוה לקטן דע שצדקת | |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle{\color{ | + | :*In our example: we subtract 14 parts of 48 from 4-sixths; 108 parts of 288 remain and they are the [3]-eighths. |
| − | | | + | ::<math>\scriptstyle{\color{blue}{\frac{4}{6}-\left(\frac{4}{6}-\frac{3}{8}\right)=\frac{4}{6}-\frac{14}{48}=\frac{108}{288}=\frac{3}{8}}}</math> |
| + | |style="text-align:right;"|במשלינו זה חסרנו הי"ד חלקים ממ"ח מד' ששיות ישארו ק"ח חלקים מרפ"ח והם הם הו' שמיניות | ||
|- | |- | ||
| − | | | + | |} |
| − | + | ||
| − | + | {| | |
| − | |||
|- | |- | ||
| | | | ||
| + | |||
| + | === <span style=color:green>Combined Subtraction</span> === | ||
| + | |} | ||
| + | :<math>{\color{OliveGreen}{\begin{align}&\scriptstyle\left(\frac{a_1}{b_2}+\frac{a_2}{b_2}+\cdots+\frac{a_n}{b_n}\right)-\left(\frac{c_1}{d_2}+\frac{c_2}{d_2}+\cdots+\frac{c_m}{d_m}\right)\\&\scriptstyle=\frac{\sum_{k=1}^n \left[a_k\sdot\left(\prod_{i=1,i\neq k}^n b_i\right)\sdot\left(\prod_{i=1}^m d_i\right)\right]-\sum_{k=1}^m \left[c_k\sdot\left(\prod_{i=1,i\neq k}^m d_i\right)\sdot\left(\prod_{i=1}^n b_i\right)\right]}{\prod_{k=1}^n b_k\sdot\prod_{i=1}^m d_i}\\\end{align}}}</math> | ||
| + | {| | ||
| + | |- | ||
| + | |If you want to subtract numerous fractions from numerous fractions without having to sum up first and then subtract, you can use the following method: | ||
| + | |style="width:45%; text-align:right;"|ואולם אם רצית לחסר שברים רבים משברים רבים מבלתי שתצטרך לקבץ תחלה ואח"כ לחסרם הנה תוכל להשתמש בזה הדרך | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle{\color{blue}{\left(\frac{4}{5}+\frac{5}{6}+\frac{1}{7}\right)-\left(\frac{1}{2}+\frac{1}{3}+\frac{3}{4}\right)}}</math> | ||
| | | | ||
{|class="wikitable" style="margin-left: auto; margin-right: 0px;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | |<math>\scriptstyle\frac{ | + | |<math>\scriptstyle\frac{1}{7}\;\frac{5}{6}\;\frac{4}{5}</math>||<math>\scriptstyle\frac{3}{4}\;\frac{1}{3}\;\frac{1}{2}</math> |
|} | |} | ||
|- | |- | ||
| | | | ||
| − | ::Multiply | + | ::Multiply all the denominators by each other; the result is 5040. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{5\sdot6\sdot7\sdot2\sdot3\sdot4=5040}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תכה כל האיכיות זה בזה יעלה 5040 |
|- | |- | ||
| | | | ||
| − | :: | + | ::Multiply the [each] numerator of the subtrahend by all the denominators except its denominator; the result is 7980 |
| − | ::<math>\scriptstyle{\color{blue}{3\sdot5= | + | ::<math>\scriptstyle{\color{blue}{\left(1\sdot3\sdot4\sdot5\sdot6\sdot7\right)+\left(1\sdot2\sdot4\sdot5\sdot6\sdot7\right)+\left(3\sdot2\sdot3\sdot5\sdot6\sdot7\right)=7980}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ותכה כמות השבר הנחסר עם כל איכיות זולת איכותו וכן כל איכות הכמות מהנחסר יעלה 7980 |
|- | |- | ||
| | | | ||
| − | :: | + | ::Do the same with all the numerators [of the minuend]; the result is 8952 |
| − | ::<math>\scriptstyle{\color{blue}{\ | + | ::<math>\scriptstyle{\color{blue}{\left(4\sdot6\sdot7\sdot2\sdot3\sdot4\right)+\left(5\sdot5\sdot7\sdot2\sdot3\sdot4\right)+\left(1\sdot5\sdot6\sdot2\sdot3\sdot4\right)=8952}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכן תעשה לכל הכמיות אשר יוחסרו השברים מהם ויעלה ח' אלפים ותתקנ"ב |
|- | |- | ||
| | | | ||
| − | :: | + | ::Subtract 7980 from it; 972 remain and they are 972 parts of 5040. |
| − | ::<math>\scriptstyle{\color{blue}{\frac{7}{4\ | + | ::<math>\scriptstyle{\color{blue}{\left(\frac{4}{5}+\frac{5}{6}+\frac{1}{7}\right)-\left(\frac{1}{2}+\frac{1}{3}+\frac{3}{4}\right)=\frac{8952-7980}{5040}=\frac{972}{5040}}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ותחסר מהם הז' אלפים ותתק"ף ישארו תתקע"ב והם תתקע"ב חלקים מה' אלפים ומ‫' |
| + | |} | ||
| + | :<math>{\color{OliveGreen}{\begin{align}&\scriptstyle\left(\frac{a_1}{b_1}-\frac{c_1}{d_1}\right)+\left(\frac{a_2}{b_2}-\frac{c_2}{d_2}\right)+\cdots+\left(\frac{a_n}{b_n}-\frac{c_n}{d_n}\right)\\&\scriptstyle=\frac{\sum_{k=1}^n \left[a_k\sdot\left(\prod_{i=1,i\neq k}^n b_i\right)\sdot\left(\prod_{i=1}^m d_i\right)\right]-\sum_{k=1}^n \left[c_k\sdot\left(\prod_{i=1,i\neq k}^n d_i\right)\sdot\left(\prod_{i=1}^n b_i\right)\right]}{\prod_{k=1}^n b_k\sdot\prod_{i=1}^n d_i}\\\end{align}}}</math> | ||
| + | {| | ||
|- | |- | ||
| − | | | + | |If you want to know the sum of all the remainders from the subtractions of numerous fractions from numerous fractions without having to subtract first and then sum up. |
| − | + | |style="width:45%; text-align:right;"|ואולם אם רצית לדעת העולה מכל הנשארים מחסרונו' שברים רבים משברים רבים מבלתי שתצטרך לחסרם תחלה ואח"כ לקבצם | |
| − | | | ||
|- | |- | ||
| | | | ||
| − | : | + | :*To subtract a half from 2-thirds and 3-quarters from 4-fifths. |
| − | ::<math> | + | ::<math>{\color{blue}{\scriptstyle\left(\frac{2}{3}-\frac{1}{2}\right)+\left(\frac{4}{5}-\frac{3}{4}\right)}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|לחסר החצי מב' שלישיות וג' רביעיות מד' חומשיות |
|- | |- | ||
| | | | ||
| − | + | | | |
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px;" | |
| − | |||
|- | |- | ||
| − | | | + | |<math>\scriptstyle\frac{4}{5}\;\frac{3}{4}</math>||<math>\scriptstyle\frac{2}{3}\;\frac{1}{2}</math> |
| − | + | |} | |
| − | | | + | |
|- | |- | ||
| | | | ||
| − | :: | + | ::We multiply all the denominators [by each other], the result is 120. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{3\sdot2\sdot5\sdot4=120}}</math> |
| + | |style="text-align:right;"|נכה האיכיות יעלו ק"כ | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We multiply the numerator of the first subtrahend by all the denominators except its denominator; it is 60. |
| − | ::<math>\scriptstyle{\color{blue}{\ | + | ::<math>\scriptstyle{\color{blue}{1\sdot3\sdot5\sdot4=60}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נכה כמות השבר הנחסר מהראשון עם כל האיכיות חוץ מאיכותו והם ס‫' |
|- | |- | ||
| | | | ||
| − | :: | + | ::We also multiply the numerator of the second subtrahend by all the denominators except its denominator; it is 90. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{3\sdot5\sdot3\sdot2=90}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|גם נכה כמות השבר הנחסר מהב' עם כל האיכיות זולת אכותו והם צ‫' |
|- | |- | ||
| | | | ||
| − | ::We | + | ::We sum it with 60; it is 150. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{60+90=150}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|קבצם עם הס' הם ק"נ |
|- | |- | ||
| | | | ||
| − | :: | + | ::Then, multiply [the numerator] of the greater fraction of the first type, from which the smaller is subtracted, by all the denominators except its denominator; and likewise the numerator of the greater fraction of the second type, from which the smaller is subtracted, and sum [the second product] with the first [product]; it is 176. |
| − | ::<math>\scriptstyle{\color{blue}{\ | + | ::<math>\scriptstyle{\color{blue}{\left(2\sdot2\sdot5\sdot4\right)+\left(4\sdot4\sdot3\sdot2\right)=176}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אח"כ הכה השבר הגדול אשר ממנו יחוסר הקטן מהמין הראשון עם כל איכות זולת מאיכותו וכן כמות השבר הגדול אשר ממנו ויחוסר הקטן מהמין הב' וקבצהו עם הראשון והם קע"ו |
|- | |- | ||
| | | | ||
| − | + | ::We subtract 150 from it; 26 remain and they are 26 parts of 150. | |
| − | | | + | ::<math>\scriptstyle{\color{blue}{\left(\frac{2}{3}-\frac{1}{2}\right)+\left(\frac{4}{5}-\frac{3}{4}\right)=\frac{176-150}{150}=\frac{26}{150}}}</math> |
| + | |style="text-align:right;"|נחסר מהם הק"נ וישארו כ"ו והם כ"ו חלקים מק"נ | ||
| + | |} | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(\frac{a_1}{b_1}\times\frac{a_2}{b_2}\times\cdots\times\frac{a_n}{b_n}\right)-\left(\frac{c_1}{c_1}\times\frac{c_2}{c_2}\times\cdots\times\frac{c_m}{d_m}\right)=\frac{\left[\left(\prod_{i=1}^n a_i\right)\sdot\left(\prod_{j=1}^m d_j\right)\right]-\left[\left(\prod_{j=1}^m c_j\right)\sdot\left(\prod_{i=1}^n b_i\right)\right]}{\prod_{i=1}^n b_i\sdot\prod_{j=1}^m d_j}}}</math> | ||
| + | {| | ||
|- | |- | ||
| − | | | + | |If you want to know the remainder from the subtraction of products of fractions by fractions without having to know the products of these fractions. |
| − | + | |style="width:45%; text-align:right;"|ואולם אם רצית לדעת הנשאר מחסור העולה מהכאת השברים אחדים עם שברים מבלתי שתצטרך לדעת העולה מהכאת השברים ההם | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | :: | + | :*For example: you wish to know [the remainder] from the subtraction of the product of a half by 2-thirds from the product of 3-quarters by 5-fifths, without having to multiply a half by 2-thirds and 3-quarters by 5-fifths. |
| − | ::<math> | + | ::<math>{\color{blue}{\scriptstyle\left(\frac{3}{4}\times\frac{4}{5}\right)-\left(\frac{1}{2}\times\frac{2}{3}\right)}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כמו עד"מ רצית לדעת מחיסור העולה מהכאת החצי עם ב' שלישיות מהעולה מהכאת הג' רביעיות עם ד' חומשיות מבלתי שתצטרך להכות החצי עם הב' שלישיות והג' רביעיות עם הד' חומשיות |
|- | |- | ||
| | | | ||
| − | :: | + | ::They are arranged as follows: |
| − | + | |style="text-align:right;"|הנה יסודרו בזה הדרך | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |||
| − | |||
| − | |||
| | | | ||
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px;" | |
| − | |||
|- | |- | ||
| − | | | + | |<math>\scriptstyle\frac{4}{5}\;\frac{3}{4}</math>||<math>\scriptstyle\frac{2}{3}\;\frac{1}{2}</math> |
| − | + | |} | |
| − | | | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We multiply all the denominators [by each other]; it is 120. |
| − | + | ::<math>\scriptstyle{\color{blue}{4\sdot5\sdot2\sdot3=120}}</math> | |
| − | + | |style="text-align:right;"|נכה כל האיכיו' והם ק"כ | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | ::We multiply the subtracted fractions, numerator by numerator, then the product by the denominators of the greater fractions, from which the smaller are subtracted; it is 40. | |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{1\sdot2\sdot4\sdot5=40}}</math> |
| + | |style="text-align:right;"|ונכה השברים הנחסרים הכמות עם הכמות והעולה עם איכות השברים הגדולים אשר יוחסרו הקטנים מהם והם מ‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We multiply the numerators of the greater fractions, numerator by numerator, then the product by the denominators of the smaller fractions; it is 72. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{3\sdot4\sdot2\sdot3=72}}</math> |
| + | |style="text-align:right;"|ונכה כמות השברים הגדולים הכמות עם הכמות והעולה עם כל איכיות השברים הקטנים והם ע"ב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We subtract 40 from it; 32 remain and they are 32 parts of 120. |
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{3}{4}\times\frac{4}{5}\right)-\left(\frac{1}{2}\times\frac{2}{3}\right)=\frac{72-40}{120}=\frac{32}{120}}}</math> | ||
| + | |style="text-align:right;"|נחסר מהם מ' ישארו ל"ב והם ל"ב חלקים מק"כ | ||
|- | |- | ||
| | | | ||
| − | + | *<math>{\color{OliveGreen}{\begin{align}&\scriptstyle\left(\frac{a_1}{b_1}-\frac{c_1}{d_1}\right)\times\left(\frac{a_2}{b_2}-\frac{c_2}{d_2}\right)\\&\scriptstyle=\frac{\left[\left(a_1\sdot d_1\right)-\left(c_1\sdot b_1\right)\right]\times\left[\left(a_2\sdot d_2\right)-\left(c_2\sdot b_2\right)\right]}{b_1\sdot d_1\sdot b_2\sdot d_2}\\\end{align}}}</math> | |
| − | |||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |If you want to know the product of the remainder from the subtraction of some fractions from some fractions by the remainder from the subtraction of some fractions from some fractions without having to know the remainders from these subtractions at all. |
| − | + | |style="text-align:right;"|ואולם אם רצית לדעת העול' מהכאת הנשאר מחיסור שברים מה משברים מה עם הנשאר מחיסור השברים מה משברים מה מבלתי שתצטרך לדעת הנשארים מהחסורים ההם כלל | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | :*For example: you wish to know the product of the remainder from the subtraction of a half from 2-thirds by the remainder from the subtraction of 3-quarters from 4-fifths. | |
| − | = | + | ::<math>{\color{blue}{\scriptstyle\left(\frac{2}{3}-\frac{1}{2}\right)\times\left(\frac{4}{5}-\frac{3}{4}\right)}}</math> |
| − | | | + | |style="text-align:right;"|כמו עד"מ אם רצית לדעת העולה מהכאת הנשאר מחסור החצי מב' שלישיו' עם הנשאר מחיסור הג' רביעיות מהד' חמשיות |
|- | |- | ||
| − | |||
| | | | ||
| − | + | ::They are arranged as follows: | |
| − | + | |style="text-align:right;"|הנה יסודרו ע"ז הדרך | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| Line 2,139: | Line 2,292: | ||
{|class="wikitable" style="margin-left: auto; margin-right: 0px;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | |<math>\scriptstyle\frac{ | + | |<math>\scriptstyle\frac{4}{5}\;\frac{3}{4}</math>||<math>\scriptstyle\frac{2}{3}\;\frac{1}{2}</math> |
|} | |} | ||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | ::We multiply all the denominators [by each other]; it is 120. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{3\sdot2\sdot5\sdot4}}</math> |
| + | |style="text-align:right;"|נכה האיכיות והם ק"כ | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We subtract the cross product 1 by 3 from the cross product 2 by 2 and keep the remainder. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\left(2\sdot2\right)-\left(1\sdot3\right)}}</math> |
| + | |style="text-align:right;"|אח"ז נחסר העולה מ{{#annot:term|2625,2521|eZiE}}הכאת אלכסון{{#annotend:eZiE}} א"ג מהכאת אלכסון ב"ב והנשאר נשמרהו | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We also subtract the cross product 3 by 5 from the cross product 4 by 4 and keep the remainder. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\left(4\sdot4\right)-\left(3\sdot5\right)}}</math> |
| + | |style="text-align:right;"|גם נחס' העול' מהכאת אלכסון ג"ה מהעולה מהכאת אלכסון ד"ד והנשאר נשמרהו | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Then we multiply the remainder by the remainder and relate the product to the first reserved and the result is the product of the remainder from the subtraction of a half from 2-thirds by the remainder from the subtraction of 3-quarters from 4-fifths. |
| − | |style="text-align:right;"| | + | ::<math>{\color{blue}{\scriptstyle\left(\frac{2}{3}-\frac{1}{2}\right)\times\left(\frac{4}{5}-\frac{3}{4}\right)=\frac{\left[\left(2\sdot2\right)-\left(1\sdot3\right)\right]\times\left[\left(4\sdot4\right)-\left(3\sdot5\right)\right]}{3\sdot2\sdot5\sdot4}}}</math> |
| + | |style="text-align:right;"|ואח"ז נכה הנשאר עם הנשאר וההווה נייחסהו אל השמור הראשון וההוה הוא העולה מהכאת הנשאר מחסור החצי מהב' שלישיו' עם הנשאר מחסור הג' רביעיות מד' חמשיות | ||
| + | |} | ||
| + | :<math>{\color{OliveGreen}{\begin{align}&\scriptstyle\left[\left(\frac{a_1}{b_1}\times\frac{c_1}{d_1}\right)+\left(\frac{a_2}{b_2}\times\frac{c_2}{d_2}\right)+\cdots+\left(\frac{a_n}{b_n}\times\frac{c_n}{d_n}\right)\right]-\left[\left(\frac{a_{n+1}}{b_{n+1}}\times\frac{c_{n+1}}{d_{n+1}}\right)+\left(\frac{a_{n+2}}{b_{n+2}}\times\frac{c_{n+2}}{d_{n+2}}\right)+\cdots+\left(\frac{a_{n+m}}{b_{n+m}}\times\frac{c_{n+m}}{d_{n+m}}\right)\right]\\&\scriptstyle=\frac{\left[\sum_{k=1}^n \left[\left(a_k\sdot c_k\right)\sdot\prod_{i=1,i\neq k}^{n+m} \left(b_i\sdot d_i\right)\right]\right]-\left[\sum_{k={n+1}}^{n+m} \left[\left(a_k\sdot c_k\right)\sdot\prod_{i=1,i\neq k}^{n+m} \left(b_i\sdot d_i\right)\right]\right]}{\prod_{i=1}^{n+m} b_i\sdot\prod_{i=1}^{n+m} d_i}\\\end{align}}}</math> | ||
| + | {| | ||
| + | |- | ||
| + | |If you want to know the remainder from the subtraction of the sum of products of fractions from the sum of products of fractions without having to multiply, then sum, then subtract, but to apply all three at the same time. | ||
| + | |style="width:45%; text-align:right;"|ואולם אם רצית לדעת הנשאר מחסור העולה מקבוץ העולה מהכאות שברים מהעולה מקבוץ העולים מהכאת שברים מה מבלתי שתצטרך להכות ואח"ז לקבץ ואח"ז לחסר אבל יצאו לך שלשתן בפעם אחת | ||
|- | |- | ||
| | | | ||
| − | :: | + | :*For example: you wish to multiply a half by 2-thirds, 3-quaters by 4-fifths, 3-sevenths by 4-fifths, and 2-thirds by 3-quarters; then to sum up the product of a half by 2-thirds with the product of 3-quaters by 4-fifths, and the product of 3-sevenths by 4-fifths with the product of 2-thirds by 3-quarters; then to subtract the sum from the sum and know the remainder. |
| − | |style="text-align:right;"| | + | ::<math>{\color{blue}{\scriptstyle\left[\left(\frac{1}{2}\times\frac{2}{3}\right)+\left(\frac{3}{4}\times\frac{4}{5}\right)\right]-\left[\left(\frac{3}{7}\times\frac{4}{5}\right)+\left(\frac{2}{3}\times\frac{1}{4}\right)\right]}}</math> |
| + | |style="text-align:right;"|עד"מ רצית להכות חצי עם ב' שלישיו' וג' רביעיות עם ד' חמשיות וג' שביעיות עם ד' חמשיות וב' שלישיות עם ג' רביעיות ואח"ז לקבץ העולה מהכאת החצי עם הב' שלישיות עם העולה מהכאת ג' רביעיות עם ד' חמשיו' והעולה מהכאת הג' שביעיות עם ד' חמשיות עם העולה מהכאת הב' שלישיות עם הרביע אח"ז לחסר ההוה מהקבוץ מההוה מהקבוץ לדעת הנשאר | ||
|- | |- | ||
| | | | ||
| − | : | + | ::They are arranged as follows: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הנה יסודרו על זה הסדר |
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle | + | | |
| − | | | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px;" |
| + | |- | ||
| + | |<math>\scriptstyle\frac{1}{4}\;\frac{2}{3}</math>||<math>\scriptstyle\frac{4}{5}\;\frac{3}{7}</math>||<math>\scriptstyle\frac{4}{5}\;\frac{3}{4}</math>||<math>\scriptstyle\frac{2}{3}\;\frac{1}{2}</math> | ||
| + | |} | ||
| + | |||
|- | |- | ||
| − | |||
| | | | ||
| + | ::We multiply all the denominators [by each other]; it is 50400. | ||
| + | ::<math>\scriptstyle{\color{blue}{2\sdot3\sdot4\sdot5\sdot7\sdot5\sdot3\sdot4=50400}}</math> | ||
| + | |style="text-align:right;"|נכה כל האיכיות והם נ' אלף ות‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We multiply the numerators of the two first fractions by each other, then the product by all the denominators successively except their own denominators; it is 16800. |
| + | ::<math>\scriptstyle{\color{blue}{1\sdot2\sdot4\sdot5\sdot7\sdot5\sdot3\sdot4=16800}}</math> | ||
| + | |style="text-align:right;"|נכה כמות הב' שברים הראשונים זה עם זה והעולה עם כל האיכיות על הסדר הזה חוץ מאיכיותיו והם י"ו אלף ות"ת | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We also multiply the numerators of the two second fractions [by each other], then the product by all the denominators successively except their own denominators; it is 30240. |
| − | |style="text-align:right;"|נכה כמות | + | ::<math>\scriptstyle{\color{blue}{3\sdot4\sdot2\sdot3\sdot7\sdot5\sdot3\sdot4=30240}}</math> |
| + | |style="text-align:right;"|גם נכה כמות הב' שברים השניי' העול' עם כל האכיות על הסדר חוץ אכיותיו והם ל' אלף ור"מ | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We sum it with 16800; it is 47040. We keep it. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{16800+30240=47040}}</math> |
| + | |style="text-align:right;"|ונקבצם עם י"ו אלפים ות"ת והם מ"ז אלף ומ' ונשמרם | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We multiply [the numerators of] the two third fractions by each other, then the product by all the denominators except their own denominators; it is 17280. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{3\sdot4\sdot3\sdot4\sdot2\sdot3\sdot4\sdot5=17280}}</math> |
| + | |style="text-align:right;"|אח"ז נכה הב' שברים מהשלישיי' זה עם זה והעולה עם האכיות כולם חוץ מאכיותיהם והם י"ז אלף ור"פ | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We also multiply the numerators of the two fourth fractions by each other, then the product by all the denominators except their own denominators; it is 8400. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{2\sdot1\sdot7\sdot5\sdot2\sdot3\sdot4\sdot5=8400}}</math> |
| + | |style="text-align:right;"|גם נכה כמות הב' שברים הרביעיים זה עם זה והעולה עם כל האיכיות חוץ מאכיותיהן והם ח' אלפים ‫<ref>32v</ref>ות‫' | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{ | + | ::We sum it with 1[7]280; it is 25680. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{17280+8400=25680}}</math> |
| + | |style="text-align:right;"|נקבצם עם הי' אלף ור"פ והם כ"ה אלף ותר"פ | ||
|- | |- | ||
| − | |||
| | | | ||
| + | ::We subtract it from the second reserved; 21360 remain and they are 21360 [parts] of 50800. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left[\left(\frac{1}{2}\times\frac{2}{3}\right)+\left(\frac{3}{4}\times\frac{4}{5}\right)\right]-\left[\left(\frac{3}{7}\times\frac{4}{5}\right)+\left(\frac{2}{3}\times\frac{1}{4}\right)\right]=\frac{47040-25680}{50400}=\frac{21360}{50400}}}</math> | ||
| + | |style="text-align:right;"|נחסרם מהשמור הב' וישארו כ"א אלף ש"ס והם כ"א אלף ש"ס מנ' אלף ות"ת | ||
| + | |- | ||
| + | |} | ||
| + | |||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | + | ||
| − | + | == <span style=color:green>Division</span> == | |
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | === <span style=color:green>Dividing a large number by a smaller number</span> === |
| − | + | ||
| − | |||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| − | |< | + | |When you wish to divide a certain amount among people, giving each one twice as much as his friend. |
| − | + | |style="width:45%; text-align:right;"|<big>כאשר</big> תרצה לחלק מספר מה אל אנשים מה ולתת לכל אח' כפל חבירו | |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*As when you wish to divide 100 among four people, giving each one twice as much as his friend. |
| + | |style="text-align:right;"|כגון שתרצה לחלק ק' אל ד' אנשים ולתת לכל אחד כפל חבירו | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Do as follows: know the sum, that is we take the share of the first as it is - one; the share [of the second] 2, so it is 3; the share of the third is 4, so it is 7; the share of the fourth is 8, so it is 15. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{1+2+4+8=3+4+8=7+8=15}}</math> |
| + | |style="text-align:right;"|ככה תעשה דע א' כמה יתחבר וזה שחלק הא' נקחהו כמו שהוא אח' והחלק ב' הרי ג' והחלק ג' ד' הרי ז' והחלק הד' ח' הרי ט"ו | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Divide 100 by 15; the result is the share of the first. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{100\div15}}</math> |
| + | |style="text-align:right;"|חלק ק' על ט"ו והיוצא הוא חלק הראשון | ||
|- | |- | ||
| | | | ||
| − | : | + | ::To know [the share of] the second, double it. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ולדעת הב' כפלהו בו |
|- | |- | ||
| − | | | + | |And so on. |
| − | | | + | |style="text-align:right;"|כן לעולם |
|- | |- | ||
| − | | | + | |From this you understand all the questions of this type. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומזה תבין לכל השאלות שבזה המין |
|- | |- | ||
| − | | | + | |Understand this. |
| − | + | |style="text-align:right;"|והבן | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | |
| − | + | === <span style=color:green>Dividing a small number by a greater number</span> === | |
| − | |||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |To divide a small number by a greater number, divide the greater by the smaller. |
| − | + | |style="text-align:right;"|<big>לחלק</big> מספר מעט על מספר רב תחלק הרב על המעט | |
|- | |- | ||
| − | | | + | |If it is divided into integers no more and no less, the reduced result of division is the denominator of the quotient, which are all the required fractions of the integer together. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{OliveGreen}{a\div\left(a\sdot b\right)=\frac{1}{\left(a\sdot b\right)\div a}}}</math> |
| + | |style="text-align:right;"|אם יתחלק לו לשלמים בלתי תוספת ומגרע' הנה היוצא בחלק בצמצום הוא מורה החלק אשר הם כל השברים הנשאלים יחד מהשלם | ||
|- | |- | ||
| | | | ||
| − | :*<math>\scriptstyle{\color{blue}{ | + | :*I.e., if the result of division is 4, it is a quarter of 1. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{1\div4=\frac{1}{4\div1}}}</math> |
| + | |style="text-align:right;"|ר"ל שאם יצא בחלוק ד' הנה יבא לו רביעית הא‫' | ||
|- | |- | ||
| − | | | + | |And the like. |
| − | :: | + | |style="text-align:right;"|ובדומה לזה |
| − | |style="text-align:right;"|אח | + | |- |
| + | |If it is not divided into integers, but a certain number remains, we add one to the [integer] resulting from the division, then subtract the remainder from the dividend and what remains are the parts of all the denominators. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{a\div\left[\left(a\sdot b\right)+1\right]=\frac{1}{\left[\left(a\sdot b\right)\div a\right]+1}+\left(\frac{1}{\left[\left(a\sdot b\right)\div a\right]+1}\sdot\frac{a-1}{\left(a\sdot b\right)+1}\right)}}</math> | ||
| + | |style="text-align:right;"|ואם לא יתחלק כלו לשלמים וישאר שום מספר הנה נוסי' אח' ועלה יוצא בחלוק ונחסר הנשאר מהמספר אשר חלקו ועליו ומה שישא' הם חלקים מכל המורים | ||
|- | |- | ||
| | | | ||
| − | :: | + | :*Example: we wish to divide 7 by 29. |
| − | |style="text-align:right;"| | + | ::<math>{\color{blue}{\scriptstyle7\div29}}</math> |
| + | |style="text-align:right;"|המשל רצינו לחלק ז' על כ"ט | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We do the opposite: we divide 29 by 7; the result of division is 4. Since 1 remains, we add 1 to 4; it is 5. We subtract 1 from 7; 6 remain and they are 6 parts of 29 of one-fifth that are added to the fifth that we have in our hand. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נעשה ההפך נחלק הכ"ט על הז' יצא בחלוק ד' ובשביל שנשאר א' בלא נוסי' על הד' א' ויהיה ה' והוא ה' ונחסר הא' מז' ישארו ו' והם ו' חלקים מכ"ט בחמישית נוסף שהעלה חמישי' שיש בידינו |
| + | |} | ||
| + | ::<math>\scriptstyle{\color{blue}{7\div29=\frac{1}{\left[\left(29-1\right)\div7\right]+1}+\left(\frac{1}{\left[\left(29-1\right)\div7\right]+1}\sdot\frac{7-1}{29}\right)=\frac{1}{4+1}+\left(\frac{1}{4+1}\sdot\frac{6}{29}\right)=\frac{1}{5}+\left(\frac{1}{5}\sdot\frac{6}{29}\right)}}</math> | ||
| + | {| | ||
| + | |- | ||
| + | |If you want to have more parts. | ||
| + | |style="width:45%; text-align:right;"|ואם תרצה שיצאו לך חלקים יותר | ||
|- | |- | ||
| | | | ||
| − | :<math> | + | :*Divide 29 by 6. |
| − | |style="text-align:right;"| | + | ::<math>{\color{blue}{\scriptstyle29\div6}}</math> |
| + | |style="text-align:right;"|חלק הכ"ט על הו‫' | ||
|- | |- | ||
| + | | | ||
| + | ::The result of division is 4 and 5 remains. We add 1 to 4; it is 5. We subtract 5 from 6 as said; 1 remains. So, the result is one-fifth, one-fifth of one-fifth, and one part of 29 of one-fifth of one-fifth. | ||
| + | |style="text-align:right;"|יצא בחלוק ד' וישארו ה' נוסי' א' על הד' ויהיו ה' ונחסר הה' אל הו' כאמור וישאר א' והעולה הוא ה' א' וחמישית ה' א' וחלק מכ"ט מחמישית חמישית אחד | ||
|} | |} | ||
| − | + | ::<math>\scriptstyle{\color{blue}{7\div29=\frac{1}{5}+\left(\frac{1}{5}\sdot\frac{6}{29}\right)=\frac{1}{5}+\left[\frac{1}{5}\sdot\frac{1}{4+1}+\left(\frac{1}{4+1}\sdot\frac{6-5}{29}\right)\right]=\frac{1}{5}+\left(\frac{1}{5}\sdot\frac{1}{5}\right)+\left(\frac{1}{5}\sdot\frac{1}{5}\sdot\frac{1}{5}\right)}}</math> | |
{| | {| | ||
|- | |- | ||
| | | | ||
| − | + | ::This is their diagram: | |
| − | + | |style="width:45%; text-align:right;"|וזו צורתם | |
| − | | | ||
|- | |- | ||
| | | | ||
| − | |||
| − | |||
| − | |||
| − | |||
| | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | | | + | |<math>\scriptstyle\frac{ \;1\;1\;1}{29\;5\;5}</math> |
| − | | | + | |} |
|- | |- | ||
| − | | | + | |The proof is by changing the denominator. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{1}{b}+\left(\frac{1}{b}\sdot\frac{1}{d}\right)=\frac{\left(1\times d\right)+1}{b\sdot d}}}</math> |
| + | |style="text-align:right;"|והמופת שנעשם פריטה | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | ::Multiply 1 by 5 and add what is above it. Then multiply the sum by 29 and add what is above it. Divide the total by all the denominators except for 29; you get a whole 7 no more and no less. |
| + | ::<math>{\color{blue}{\scriptstyle\frac{1}{5}+\left(\frac{1}{5}\sdot\frac{1}{5}\right)+\left(\frac{1}{5}\sdot\frac{1}{5}\sdot\frac{1}{5}\right)=\frac{\left[\left[\left(1\times 5\right)+1\right]\sdot29\right]+1}{5\sdot5}\div29=\frac{7}{29}}}</math> | ||
| + | |style="text-align:right;"|שתכה הא' בה' ותשא מה שעל ראש' ותכה הכל עם הכ"ט ותשא מה שעל ראשם ותחלק הכל לכל המורים זולת הכ"ט ויצא לך מספ' הז' שלם בלי תוספת ומגרעת | ||
|- | |- | ||
| − | | | + | | |
| − | + | |style="text-align:right;"|ואם לא יהיה במורים המספר אשר תרצה לחלק עליו המספר האחד | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | | |
| − | :< | + | ::<span style=color:red>the last sentence is unclear</span> |
| − | |style="text-align:right;"|אח"ז | + | |style="text-align:right;"|כגון אם רצית לחלק ו' לכ"ז שיבא לו חמישי' אח' בכ"ז ותחלקהו על כל המורי' זולתו ויצא לך בשוה המספר |
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | |
| + | == Division of Fractions == | ||
| + | |style="text-align:right;"|<big>מין החלוק מן השברים</big> | ||
|- | |- | ||
| − | | | + | | |
| − | | | + | *<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}\div\frac{c}{d}=\frac{a\sdot d}{b\sdot c}}}</math> |
| + | | | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :*{{#annot:¾÷⅖|552|GaRS}}When you wish to divide 3-quarters by two-fifths. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{3}{4}\div\frac{2}{5}}}</math> | ||
| + | |style="text-align:right;"|רצית לחלק ג' רביעיות על שני חמישיות{{#annotend:GaRS}} | ||
|- | |- | ||
| − | | | + | | |
| − | |style=" | + | | |
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | | | + | |<math>\scriptstyle\frac{2}{5}\;\frac{3}{4}</math> |
| − | | | + | |} |
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | ::Multiply 2 by 4; it is 8. |
| + | ::<math>\scriptstyle{\color{blue}{4\sdot2=8}}</math> | ||
| + | |style="text-align:right;"|הכה הב' עם הד' יהיו ח‫' | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | ::Then multiply 3 by 5; it is 15. |
| + | ::<math>\scriptstyle{\color{blue}{3\sdot5=15}}</math> | ||
| + | |style="text-align:right;"|אח"כ הכה הג' עם הה' יהיו ט"ו | ||
| + | |- | ||
| + | | | ||
| + | ::Divide 15 by 8; the result is 1 integer, which is two-fifths, and 7 remain. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{3}{4}\div\frac{2}{5}=\frac{15}{8}=1+\frac{7}{8}}}</math> | ||
| + | |style="text-align:right;"|חלק הט"ו על הח' יצא א' שלם הרי יצא לנו שני חמישיות ונשארו ז‫' | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | ::Relate it to 20, which is the product of the denominator by the denominator; the result is 7 parts of 20. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{7}{4\sdot5}=\frac{7}{20}}}</math> | ||
| + | |style="text-align:right;"|יחסם על הכ' שהו' הכאת האיכות עם האיכות יעלה ז' מכ‫' | ||
|- | |- | ||
| | | | ||
| − | + | *<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}=\frac{c}{d}\sdot\frac{a\sdot d}{b\sdot c}}}</math> | |
| | | | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | ::Then, divide it into fifths; the result is one fifth and 3-quarters of a fifth. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{7}{20}=\frac{1}{5}+\left(\frac{3}{4}\sdot\frac{1}{5}\right)}}</math> | ||
| + | |style="text-align:right;"|אח"כ חלקם על החומש ויצא חומש אח' וג' רביעיות חומש | ||
|- | |- | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| | | | ||
| − | {| | + | ::Add it to 2-fifths and the result is the required, which is 3-fifths and 3-quarters of one fifth. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{3}{4}=\frac{2}{5}+\left[\frac{1}{5}+\left(\frac{3}{4}\sdot\frac{1}{5}\right)\right]=\frac{3}{5}+\left(\frac{3}{4}\sdot\frac{1}{5}\right)}}</math> | ||
| + | |style="text-align:right;"|חברהו עם הב' חמישיות והיוצא הוא המבוקש והוא ג' חמישיות וג' רביעיות החומש האח‫' | ||
|- | |- | ||
| − | | | + | | |
| − | | | + | *<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}=\frac{\frac{\left(a\sdot d\right)\sdot d}{b\sdot d}}{d}}}</math> |
| − | + | | | |
| − | |||
|- | |- | ||
| − | | | + | | |
| − | + | ::Another way: we multiply 3 by 5, the numerator of the divisor by the denominator of the divisor; the result is 15. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|דרך אחר נכה הג' עם הה' כמות המחלק עם איכות המחלק יעלה ט"ו |
|- | |- | ||
| − | |<math>\scriptstyle\ | + | | |
| − | |style="text-align:right;"| | + | ::Multiply 15 by the denominator of the divisor, which is 5; the result is 75 and this is the divisor. |
| + | ::<math>\scriptstyle{\color{blue}{\left(3\sdot5\right)\sdot5=15\sdot5=75}}</math> | ||
| + | |style="text-align:right;"|והכה הט"ו עם איכות המחלק שהוא הה' יעלה ע"ה וזהו המחולק | ||
|- | |- | ||
| − | |<math>\scriptstyle\ | + | | |
| − | |style="text-align:right;"| | + | ::Then we multiply the denominators; it is 20. |
| + | ::<math>\scriptstyle{\color{blue}{4\sdot5=20}}</math> | ||
| + | |style="text-align:right;"|אח"כ נכה האיכיות והם כ‫' | ||
|- | |- | ||
| − | |<math>\scriptstyle\frac{ | + | | |
| − | |style="text-align:right;"| | + | ::We divide 75 by it; the result is 3 and 3-quarters. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{75}{20}=3+\frac{3}{4}}}</math> | ||
| + | |style="text-align:right;"|נחלק עליהם הע"ה יצאו ג' וג' רביעיות | ||
|- | |- | ||
| − | |<math>\scriptstyle\frac{ | + | | |
| − | |style="text-align:right;"| | + | ::Since the dividend is fifths, we know that the 3 and 3-quarters [are 3-fifths] and 3-quarters of a fifth. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{3}{4}=\frac{3+\frac{3}{4}}{5}=\frac{3}{5}+\left(\frac{3}{4}\sdot\frac{1}{5}\right)}}</math> | ||
| + | |style="text-align:right;"|ולהיות שהמחולק הוא חמישיות ידענו שהג' וג' רביעיות חמשיות וג' רביעיות החומש | ||
|- | |- | ||
| − | | | + | | |
| − | + | *<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}=\frac{\frac{a\sdot d}{b}}{d}}}</math> | |
| − | + | | | |
| − | |||
| − | | | ||
|- | |- | ||
| − | |<math>\scriptstyle\ | + | | |
| − | |style="text-align:right;"| | + | ::Another way: we multiply the numerator of the dividend by the denominator of the divisor; it is 15. |
| + | ::<math>\scriptstyle{\color{blue}{3\sdot5=15}}</math> | ||
| + | |style="text-align:right;"|דרך אחר נכה כמות המחולק עם איכות המחלק יהיו ט"ו | ||
|- | |- | ||
| − | |<math>\scriptstyle | + | | |
| − | |style="text-align:right;"| | + | ::We divide it by the denominator of the dividend; the result is 3 and 3-quarters. |
| − | + | ::<math>\scriptstyle{\color{blue}{\frac{15}{4}=3+\frac{3}{4}}}</math> | |
| + | |style="text-align:right;"|נחלקם על איכות המחולק יצאו ג' וג' רביעיות | ||
|- | |- | ||
| − | |<math>\scriptstyle\ | + | | |
| − | |style="text-align:right;"| | + | ::Since the dividend is fifths, we know that the 3 and 3-quarters are 3-fifths and 3-quarters of a fifth. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{3}{4}=\frac{3+\frac{3}{4}}{5}=\frac{3}{5}+\left(\frac{3}{4}\sdot\frac{1}{5}\right)}}</math> | ||
| + | |style="text-align:right;"|ולהיות שהמחול' הוא חמישי' ידענו שהג' וג' רביעיות הם ג' חמשיות וג' רביעיות החומש | ||
|- | |- | ||
| | | | ||
| − | + | ==== Methods of checking ==== | |
| − | === | + | |style="text-align:right;"|<big>מאזנים</big> |
| − | |||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle{\color{OliveGreen}{\ | + | *Multiply the quotient by the divisor, if [the result] is equal to the dividend, you were right. |
| − | |style="text-align:right;"|אם | + | :<math>\scriptstyle{\color{OliveGreen}{\left(a\div b\right)\times b=a}}</math> |
| + | |style="text-align:right;"|תכה ה{{#annot:term|783,1259|Ej2y}}חלק{{#annotend:Ej2y}} עם ה{{#annot:term|604,1228|XDL2}}מחלק{{#annotend:XDL2}} אם הוא שוה ל{{#annot:term|605,1227|NPOJ}}מחולק{{#annotend:NPOJ}} צדקת | ||
|- | |- | ||
| | | | ||
| − | : | + | :*In our example: we multiply 3 and 3-quarters by one-fifth; the result is 3-quarters and it is the dividend itself. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\left(3+\frac{3}{4}\right)\sdot\frac{1}{5}=\frac{3}{4}}}</math> |
| + | |style="text-align:right;"|במשלינו זה הכינו הג' וג' רביעיו' עם החמישי' ועלה ג' רביעיות והוא הוא בעצמו המחולק | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle{\color{ | + | :*Or, we multiply 1 and 7-eighths by 2-fifths; the result is 3-quarters itself. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\left(1+\frac{7}{8}\right)\sdot\frac{2}{5}=\frac{3}{4}}}</math> |
| + | |style="text-align:right;"|או נכה א' וז' שמיניות עם ב' חמישיות ויעלה בעצמו הג' רביעיות | ||
|- | |- | ||
| | | | ||
| − | + | ::Because, when 1 and 7-eighths are related to 2-fifths, which is 1 and 7-eighths as its amount, i.e. 1 is 2-fifths, and 7-eighths are 7-eighths of 2-fifths, which is one-fifth and 3-quarters of one-fifth; with the 2-fifths in our hand it is 3-[fifths] and 3-quarters of the fifth. | |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\left(1+\frac{7}{8}\right)\sdot\frac{2}{5}=\frac{2}{5}+\left(\frac{7}{8}\sdot\frac{2}{5}\right)=\frac{2}{5}+\left[\frac{1}{5}+\left(\frac{3}{4}\sdot\frac{1}{5}\right)\right]=\frac{3}{5}+\left(\frac{3}{4}\sdot\frac{1}{5}\right)}}</math> |
| + | |style="text-align:right;"|וזה שא' וז' שמיניות כשיוחס אל הב' חמישיות שהם א' וז' שמיניות כמותם ר"ל הא' הוא ב' חמישיות וז' שמיניו' הם ז' שמיניות של ב' חמישיו' שהוא חומש א' וג' רביעיו' החומש עם הב' חומשים שבידינו הם ג' רביעיות וג' רביעיו' החומש | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | ::Therefore, our saying: "we relate 3 and 3-quarters to one-fifth", or "we relate 1 and 7-eighths to 2-fifths" is the same [sic?]. |
| + | ::<math>\scriptstyle{\color{blue}{\left(3+\frac{3}{4}\right):\frac{1}{5}=\left(1+\frac{7}{8}\right):\frac{2}{5}}}</math> | ||
| + | |style="text-align:right;"|וא"כ הכל שוה לאמרנו ג' וג' רביעיות ויחסנו אותם לחומש או א' וז' שמיניות ויחסנו אותם לב' חמישיו‫' | ||
|- | |- | ||
| − | | | + | |It is clear to the intellect. Understand this. |
| − | + | |style="text-align:right;"|וזה מבואר בעין השכל והבן | |
| − | |||
|- | |- | ||
| | | | ||
| − | + | *Another scale: find a number, such that numerator of the [dividend] is related to it by the ratio of the numerator of the divisor to its denominator. If that number is related to the denominator of the dividend by the ratio of the numerator of the quotient to its denominator, know that you were right. | |
| − | == | + | :<math>\scriptstyle{\color{OliveGreen}{a:x=\frac{c}{d}\longleftrightarrow x:b=\left(\frac{a}{b}\div\frac{c}{d}\right)}}</math> |
| + | |style="text-align:right;"|<big>מאזנים אחרים</big> שתמצא המספ' המתיחס כמות המחלק אליו יחס כמות המחלק אל איכותו ואם המספר ההוא מתיחס אל איכות המחולק יחס כמו' החלק אל איכותו דע שצדקת | ||
| + | |- | ||
| | | | ||
| + | :*In our example: we divide 3-quarters by 2-fifths; the result is 1 and 7-eighths, which is 15-eighths. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{3}{4}\div\frac{2}{5}=\frac{15}{8}=1+\frac{7}{8}}}</math> | ||
| + | |style="text-align:right;"|במשלינו זה חלקנו הג' רביעיות על הב' חמשיות ויצא א' וז' שמיניות שהם ט"ו שמיניות | ||
|- | |- | ||
| | | | ||
| − | + | ::We look for a number, such that when you relate to it the numerator of the dividend, which is 3, it is as the ratio of the numerator of the divisor to its denominator, which is 2 to 5. We find that it is 7 and a half. | |
| − | + | ::<math>\scriptstyle{\color{blue}{3:\left(7+\frac{1}{2}\right)=\frac{2}{5}}}</math> | |
| + | |style="text-align:right;"|בקשנו מספר שתיחס כמות המחולק שהוא הג' אליו יחס כמות המחלק אל איכותו שהוא הב' אל הה' ומצאנו שהוא ז' וחצי | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | ::The ratio of 7 and a half to 4, which is the denominator of the dividend, is as the ratio of the numerator of the quotient, which is 15, to its denominator, which is 8. |
| + | ::<math>\scriptstyle{\color{blue}{\left(7+\frac{1}{2}\right):4=\frac{15}{8}=\left(\frac{3}{4}\div\frac{2}{5}\right)}}</math> | ||
| + | |style="text-align:right;"|והנה יחס הז' וחצי אל הד' שהוא איכות המחולק כיחס כמות החלק שהוא הט"ו אל איכותו שהוא ח‫' | ||
|- | |- | ||
| − | | | + | |It is clear to the intellect. |
| − | + | |style="text-align:right;"|וזה מבואר בעין השכל | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Also if you want to apply this method on 3 and 3-quarters, look for the ratio of one-fifth alone. ''You shall do, and you shall prevail'' [Samuel I 26, 25], for it is all the same thing as we have explained and revealed. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וג"כ אם תרצה לעשותו בזה הדרך בג' וג' רביעיות ותבקש ביחס החמישית לבד גם ''עשה תעשה ויכול תוכל''‫<ref group=note>שמואל א כ"ו, כ"ה</ref> כי הכל דבר א' כמו שביארנו וגלינו |
|- | |- | ||
| | | | ||
| − | * | + | *If you want you can apply the opposite scale: look for a number, such that the ratio of the numerator of the dividend to it is as the ratio of the numerator of the [quotient] to its denominator. If that number is related to the denominator of the dividend by the ratio of the numerator of the divisor to its denominator, we know that we were right. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{OliveGreen}{a:x=\left(\frac{a}{b}\div\frac{c}{d}\right)\longleftrightarrow x:b=\frac{c}{d}}}</math> |
| + | |style="text-align:right;"|ואם תרצה לעשות המאזנים בהפך והוא שתראה המסופר שיהיה יחס כמות המחולק אליו כיחס כמות המחלק אל איכותו ‫<ref>33r</ref>ואם המספר ההוא מת[ייחס] אל איכות המחולק כיחס כמות המחלק אל איכותו ידענו שצדקנו | ||
|- | |- | ||
| | | | ||
| − | + | ::In our example: we look for a number, such that the ratio of the numerator of the dividend, which is 3, to it is as the ratio of the numerator of the quotient to its denominator, which is 15 to 8. We find that it is 1 and 3-fifths, which is 8-[fifths]. | |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{3:\left(1+\frac{3}{5}\right)=3:\frac{8}{{\color{red}{5}}}=\frac{15}{8}=\left(\frac{3}{4}\div\frac{2}{5}\right)}}</math> |
| + | |style="text-align:right;"|במשלינו זה בקשנו מספר שיהיה יחס כמות מחולק אליו שהוא ג' כיחס כמות החלק אל איכותו שהוא הט"ו עם הח' ומצאנו שהוא א' וג' חמישיות שהם ח' שמיניות | ||
|- | |- | ||
| | | | ||
| − | + | ::Its ratio to 4 is as the ratio of 2 to 5, which is as [the ratio] of the numerator of the divisor to its denominator. | |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\left(1+\frac{3}{5}\right):4=\frac{8}{{\color{red}{5}}}:4=\frac{2}{5}}}</math> |
| + | |style="text-align:right;"|ויחסם אל הד' הוא כיחס הב' אל הה' שהוא כמות המחלק אל אכותו | ||
|- | |- | ||
| − | | | + | |Understand this, for it is absolutely clear. |
| − | + | |style="text-align:right;"|והבן זה מאד כי הובן בתכלית | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | ==== | + | |
| + | ==== <span style=color:green>Combined Division</span> ==== | ||
| | | | ||
|- | |- | ||
| | | | ||
| − | * | + | *<math>\scriptstyle{\color{OliveGreen}{\left(\frac{a_1}{b_1}+\frac{c_1}{d_1}\right)\div\left(\frac{a_2}{b_2}+\frac{c_2}{d_2}\right)=\frac{\left(a_1\sdot d_1\sdot b_2\sdot d_2\right)+\left(b_1\sdot c_1\sdot b_2\sdot d_2\right)}{\left(b_1\sdot d_1\sdot b_2\sdot c_2\right)+\left(b_1\sdot d_1\sdot a_2\sdot d_2\right)}}}</math> |
| | | | ||
|- | |- | ||
| | | | ||
| − | :* | + | :*{{#annot:(⅔+¾)÷(²/₇+⅙)|552|8XVT}}If you wish to divide the sum of 2-thirds and 3-quarters by the sum of two-sevenths and one-sixth, without having to sum up first, then to divide. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\left(\frac{2}{3}+\frac{3}{4}\right)\div\left(\frac{2}{7}+\frac{1}{6}\right)}}</math> |
| + | |style="text-align:right;"|ואולם אם רצית לחלק קבוץ הב' שלישיות וג' רביעיו' על קבוץ שני שביעיות וששית מבלתי שתצטרך לקבץ תחלה ואח"כ לחל‫'{{#annotend:8XVT}} | ||
|- | |- | ||
| | | | ||
| − | : | + | ::They are arranged this way: |
| − | |style="text-align:right;"| | + | |style="width:45%; text-align:right;"|הנה יסודרו על זה הדרך |
|- | |- | ||
| | | | ||
| − | + | | | |
| − | |style=" | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px;" |
|- | |- | ||
| − | | | + | |<math>\scriptstyle\frac{1}{6}\;\frac{2}{7}</math>||<math>\scriptstyle\frac{3}{4}\;\frac{2}{3}</math> |
| − | + | |} | |
| − | + | ||
| − | | | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We multiply the denominator of the first fraction by the denominator of the second fraction, then the product by the denominator of the third, then the product by the numerator of the fourth; it is 84. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{3\sdot4\sdot7\sdot1=84}}</math> |
| + | |style="text-align:right;"|ונכה איכות השבר הראשון עם איכות השבר הב' והעולה עם איכות הג' והעולה עם כמו' הד' והם פ"ד | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We also multiply the denominator of the first fraction by the denominator of the second fraction, then the product by the numerator of the third, then the product by the denominator of the fourth; it is 144. We keep it. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{3\sdot4\sdot2\sdot6=144}}</math> |
| + | |style="text-align:right;"|גם נכה איכות השבר הראשון עם איכות השבר הב' והעול' עם כמות הג' והעול' עם איכות הד' והם קמ"ד ונשמרם | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We add it to 84; it is 228. We keep it. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{84+144=228}}</math> |
| + | |style="text-align:right;"|נחברם אל הפ"ד והם רכ"ח ונשמרם | ||
|- | |- | ||
| | | | ||
| − | + | ::We multiply the denominator of the fourth by the denominator of the third, then the product by the denominator of the second, then the product by the numerator of the first; it is 336. | |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{2\sdot4\sdot7\sdot6=336}}</math> |
| + | |style="text-align:right;"|אח"ז נכה איכות הד' עם איכות הג' והעולה עם אכות הב' והעולה עם כמות הראשון והם של"ו | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We also multiply the denominator of the fourth by the denominator of the third, then the product by the numerator of the second, then the product by the denominator of the first; it is 378. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{3\sdot3\sdot7\sdot6=378}}</math> |
| + | |style="text-align:right;"|גם נכה איכות הרביעי עם איכות הג' והעולה עם כמות הב' והעולה עם איכות הראשו' והם שע"ח | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Add it to 336; it is 714. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{336+378=714}}</math> |
| + | |style="text-align:right;"|חברם אל השל"ו הם תשי"ד | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We divide it by the reserved 228; the quotient is the result of division of [the sum of] 2-thirds and 3-quarters by [the sum of] 2-sevenths and one-sixth |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\left(\frac{2}{3}+\frac{3}{4}\right)\div\left(\frac{2}{7}+\frac{1}{6}\right)=\frac{714}{228}}}</math> |
| + | |style="text-align:right;"|נחלקם על הרכ"ח השמורים והיוצא הוא ההווה מחלוק הב' שלישיו' וג' רביעיו' על הב' שביעיות וששית | ||
|- | |- | ||
| + | |<math>\scriptstyle{\color{OliveGreen}{\left(\frac{a_1}{b_1}\div\frac{c_1}{d_1}\right)+\left(\frac{a_2}{b_2}\div\frac{c_2}{d_2}\right)=\frac{\left(a_1\sdot d_1\sdot b_2\sdot c_2\right)+\left(b_1\sdot c_1\sdot a_2\sdot d_2\right)}{b_1\sdot c_1\sdot b_2\sdot c_2}}}</math> | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle\frac{ | + | :*{{#annot:(¾÷⅔)+(²/₇÷⅙)|552|Vj8S}}If you wish to sum up the result of division of 3-quarters by 2-thirds with the result of division of 2-sevenths by [one-sixth], without having to divide first, then sum up. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\left(\frac{3}{4}\div\frac{2}{3}\right)+\left(\frac{2}{7}\div\frac{1}{6}\right)}}</math> |
| + | |style="text-align:right;"|ואולם אם רצית לקבץ היוצא מחלוק הג' רביעיות על הב' שלישיות עם היוצא מחלוק הב' שביעיו' על הו' מבלתי שתצטרך לחלק תחלה ואח"כ לקבץ{{#annotend:Vj8S}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle\ | + | ::We multiply the numerator of the first fraction by the denominator of the second, then by the denominator of the third, then by the numerator of the fourth; it is 56. We keep it. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{4\sdot2\sdot7\sdot1=56}}</math> |
| + | |style="text-align:right;"|נכה כמות השבר הראשון עם איכות הב' ועם איכות הג' ועם כמות הד' והם נ"ו ונשמרם | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle\frac{a}{60^3}=thirds</math> | + | ::We multiply the numerator of the first fraction by the denominator of the second, then by the numerator of the third, then by the denominator of the fourth; it is 96. |
| − | |style="text-align:right;"|ואם ג' שלישיים | + | ::<math>\scriptstyle{\color{blue}{2\sdot6\sdot4\sdot2=96}}</math> |
| + | |style="text-align:right;"|אח"ז נכה כמות השבר הראשון עם איכות הב' וכמות הג' ואיכות הד' והם צ"ו | ||
| + | |- | ||
| + | | | ||
| + | ::We also multiply the numerator of the fourth by the denominator of the third, then by the numerator of the second, then by the denominator of the first; it is 63. | ||
| + | ::<math>\scriptstyle{\color{blue}{3\sdot3\sdot7\sdot1=63}}</math> | ||
| + | |style="text-align:right;"|גם נכה כמות השבר הד' עם איכות הג' וכמות הב' ואיכות הראשו' והם ס"ג | ||
| + | |- | ||
| + | | | ||
| + | ::We add it to 96; it is 159. | ||
| + | ::<math>\scriptstyle{\color{blue}{63+96=159}}</math> | ||
| + | |style="text-align:right;"|נחברם אל הצ"ו והם קנ"ט | ||
| + | |- | ||
| + | | | ||
| + | ::We divide it by the reserved 56; the result is 2 integers and 4[7] parts of 56 and this is the required sum of the result of division of 3-quarters by 2-thirds with the result of division of 2-sevenths by [one-sixth]. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{3}{4}\div\frac{2}{3}\right)+\left(\frac{2}{7}\div\frac{1}{6}\right)=\frac{159}{56}=2+\frac{4{\color{red}{7}}}{56}}}</math> | ||
| + | |style="text-align:right;"|נחלקם על הנ"ו השמורים והיוצא הוא ב' שלמים ומ"ה חלקים מנ"ו והוא המבוקש מקבוץ היוצאים מחלוק הג' רביעיות על הב' שלישיו' ומחלוק הב' שביעיו' על הו‫' | ||
| + | |- | ||
| + | |<math>\scriptstyle{\color{OliveGreen}{\left(\frac{a_1}{b_1}\times\frac{c_1}{d_1}\right)\div\left(\frac{a_2}{b_2}\times\frac{c_2}{d_2}\right)=\frac{a_1\sdot c_1\sdot b_2\sdot d_2}{b_1\sdot d_1\sdot a_2\sdot c_2}}}</math> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :*{{#annot:(⅔×¾)÷(²/₇×⅙)|552|TQ16}}If you wish to divide the product of 2-thirds by 3-quarters by the product of 2-sevenths by one-sixth, without having to multiply first, then divide. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{2}{3}\times\frac{3}{4}\right)\div\left(\frac{2}{7}\times\frac{1}{6}\right)}}</math> | ||
| + | |style="text-align:right;"|ואולם אם רצית לחלק העולה מהכאת הב' שלישיו' עם הג' רביעי' על העולה מהכאת הב' שביעיו' עם הששי' מבלתי שתצטר' להכות תחלה ואח"כ לחלק{{#annotend:TQ16}} | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply the numerator of the first by [the numerator of] the second, then the product by the denominator of the third, then by the denominator of the fourth; it is 2[5]2. We keep it. | ||
| + | ::<math>\scriptstyle{\color{blue}{2\sdot3\sdot7\sdot6=2{\color{red}{5}}2}}</math> | ||
| + | |style="text-align:right;"|נכה כמות הראשו' עם הב' והעול' עם איכות הג' ואיכות הד' והם רל"ב ונשמרם | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply the numerator of the fourth fraction by the numerator of the third fraction, then the product by the denominator of the first, then by [the denominator of] the second; it is 24. | ||
| + | ::<math>\scriptstyle{\color{blue}{3\sdot4\sdot2\sdot1=24}}</math> | ||
| + | |style="text-align:right;"|אח"ז נכה כמות השבר הד' עם כמו' השבר הג' והעולה עם איכות הראשו' והב' והם כ"ד | ||
| + | |- | ||
| + | | | ||
| + | ::Divide 2[5]2 by it; the quotient is the result of division of the product of 2-thirds by 3-quarters by the product of 2-sevenths by [one-sixth]. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{2}{3}\times\frac{3}{4}\right)\div\left(\frac{2}{7}\times\frac{1}{6}\right)=\frac{2{\color{red}{5}}2}{24}}}</math> | ||
| + | |style="text-align:right;"|תחלק עליהם הרל"ב והיוצא הוא ההווה מחלוק העולה מהכאת הב' שלישיו' עם הג' רביעיו' על העולה מהכאת הב' שביעי' על הו‫' | ||
| + | |- | ||
| + | |<math>\scriptstyle{\color{OliveGreen}{\left(\frac{a_1}{b_1}\div\frac{c_1}{d_1}\right)\times\left(\frac{a_2}{b_2}\div\frac{c_2}{d_2}\right)=\frac{a_1\sdot d_1\sdot a_2\sdot d_2}{b_1\sdot c_1\sdot b_2\sdot c_2}}}</math> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :*{{#annot:(¾÷⅔)×(²/₇÷⅙)|552|Rr95}}If you wish to multiply the result of division of 3-quarters by 2-thirds by the result of division of 2-sevenths by [one-sixth], without having to divide first, then multiply. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{3}{4}\div\frac{2}{3}\right)\times\left(\frac{2}{7}\div\frac{1}{6}\right)}}</math> | ||
| + | |style="text-align:right;"|ואולם אם רצית להכות היוצא מחלוק הג' רביעיות על הב' שלישיות עם היוצא מחלוק הב' שביעית על הו' מבלתי שתצטרך לחלק תחלה ואח"כ להכות{{#annotend:Rr95}} | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply the numerator of the first by the denominator of the second, then by the denominator of the third, then by the numerator of the fourth; it is 56. We keep it. | ||
| + | ::<math>\scriptstyle{\color{blue}{4\sdot2\sdot7\sdot1=56}}</math> | ||
| + | |style="text-align:right;"|נכה כמות הראשון עם איכות הב' ואיכות הג' וכמות הד' והם נ"ו ונשמרם | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply the denominator of the first by the numerator of the second, then by the numerator of the third, then by the denominator of the fourth; it is 108. | ||
| + | ::<math>\scriptstyle{\color{blue}{3\sdot3\sdot2\sdot6=108}}</math> | ||
| + | |style="text-align:right;"|אח"ז נכה האכות הראשון עם כמות הב' ועם כמות הג' ועם איכות הד' והם ק"ח | ||
| + | |- | ||
| + | | | ||
| + | ::We divide it by the reserved 56; the result is the product of the result of division of 3-quarters by 2-thirds by the result of division of 2-sevenths by one-sixth. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{3}{4}\div\frac{2}{3}\right)\times\left(\frac{2}{7}\div\frac{1}{6}\right)=\frac{108}{56}}}</math> | ||
| + | |style="text-align:right;"|נחלקם על הנ"ו השמורים והיוצא הוא ההווה מהכאת היוצא מחלוק הג' רביעיו' על הב' שלישיו' עם היוצא מחלוק הב' שביעיו' על הששית | ||
| + | |- | ||
| + | |<math>\scriptstyle{\color{OliveGreen}{\left(\frac{a_1}{b_1}-\frac{c_1}{d_1}\right)\div\left(\frac{a_2}{b_2}-\frac{c_2}{d_2}\right)=\frac{\left[\left(a_1\sdot d_1\right)-\left(c_1\sdot b_1\right)\right]\times\left(b_2\sdot d_2\right)}{\left[\left(a_2\sdot d_2\right)-\left(c_2\sdot b_2\right)\right]\times\left(b_1\sdot d_1\right)}}}</math> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :*{{#annot:(¾-⅔)÷(²/₇-⅙)|552|C9my}}If you wish to divide the remainder from subtraction of 2-thirds from 3-quarters by the remainder from subtraction of one-sixth from 2-sevenths, without having to subtract first then divide. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{3}{4}-\frac{2}{3}\right)\div\left(\frac{2}{7}-\frac{1}{6}\right)}}</math> | ||
| + | |style="text-align:right;"|ואולם אם רצית לחלק הנשאר מחצור הב' שלישיות מג' רביעיות על הנשאר מחסור הששית מהב' שביעיות מבלתי שתצטרך לחסר ראשונה ואח"כ לחלק{{#annotend:C9my}} | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply the numerator of the first by the denominator of the second, then we subtract the product from the product of the numerator of the second by the denominator of the first, and multiply the remainder by the denominator of the third, then by the denominator of the fourth; it is 42. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left[\left(3\sdot3\right)-\left(4\sdot2\right)\right]\times\left(7\sdot6\right)=42}}</math> | ||
| + | |style="text-align:right;"|נכה כמות הראשון עם איכות הב' והעולה נחסרהו מהכאת כמות השני עם איכות הראשון והנשאר נכהו עם איכות הג' ואיכות הד' והם מ"ב | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply the numerator of the fourth by the denominator of the third, then we subtract the product from the product of the numerator of the third by the denominator of the fourth, and multiply the remainder by the denominator of the second, then by the denominator of the first; it is 60. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left[\left(2\sdot6\right)-\left(7\sdot1\right)\right]\times\left(4\sdot3\right)=60}}</math> | ||
| + | |style="text-align:right;"|אח"ז נכה כמות הד' עם איכות הג' והעולה נחסרהו מהעולה מהכאת כמות הג' עם איכות הד' והנשאר נכהו עם איכות הב' ועם איכות הראשון והם ס‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We divide it by the reserved 42; the quotient is the result of division of the remainder from subtraction of 2-thirds from 3-quarters by the remainder from subtraction of [one-sixth] from 2-sevenths. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{3}{4}-\frac{2}{3}\right)\div\left(\frac{2}{7}-\frac{1}{6}\right)=\frac{42}{60}}}</math> | ||
| + | |style="text-align:right;"|נחלקם על המ"ב השמורים והיוצא הוא ההווה מחלוק הנשאר מחסור הב' שלישיו' מהג' רביעיות על הנשאר מחיסור הו' מהב' שביעיות | ||
| + | |- | ||
| + | |<math>\scriptstyle{\color{OliveGreen}{\left(\frac{a_1}{b_1}\div\frac{c_1}{d_1}\right)-\left(\frac{a_2}{b_2}\div\frac{c_2}{d_2}\right)=\frac{\left(a_1\sdot d_1\sdot b_2\sdot c_2\right)-\left(b_1\sdot c_1\sdot a_2\sdot d_2\right)}{b_1\sdot c_1\sdot b_2\sdot c_2}}}</math> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :*{{#annot:(²/₇÷⅙)-(¾÷⅔)|552|8JsO}}If you wish to subtract the result of division of 3-quarters by 2-thirds from the result of division of 2-sevenths by [one-sixth], without having to divide first, then subtract. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{2}{7}\div\frac{1}{6}\right)-\left(\frac{3}{4}\div\frac{2}{3}\right)}}</math> | ||
| + | |style="text-align:right;"|ואולם אם רצית לחסר היוצא מחלוק הג' רביעיו' על הב' שלישיו' מהיוצא מחילוק הב' שביעיו' על הו' מבלתי שתצטרך לחלק ראשונה ואח"כ לחסרם{{#annotend:8JsO}} | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply the numerator of the first by the denominator of the second, then by the denominator of the third, then by the numerator of the fourth; it is 56 and this is the first reserved. | ||
| + | ::<math>\scriptstyle{\color{blue}{7\sdot1\sdot4\sdot2=56}}</math> | ||
| + | |style="text-align:right;"|נכה כמות הראשון עם איכות הב' ואיכות הג' וכמו' הד' והם נ"ו וזהו השמור הראשון | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply the denominator of the first by the [denominator] of the second, then the product by the denominator of the third, then by the [numerator] of the fourth; it is 63. We keep it and this is the second reserved. | ||
| + | ::<math>\scriptstyle{\color{blue}{3\sdot3\sdot7\sdot1=63}}</math> | ||
| + | |style="text-align:right;"|אח"כ נכה איכות הראשון עם הב' והעולה עם איכות הג' ועם איכות הד' והם ס"ג ונשמרם וזהו השמור הב‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply the denominator of the fourth by the numerator of the third, then by the denominator of the second, then by the numerator of the first; it is 96. | ||
| + | ::<math>\scriptstyle{\color{blue}{2\sdot6\sdot4\sdot2=96}}</math> | ||
| + | |style="text-align:right;"|אח"ז נכה איכות הד' עם כמות הג' ועם איכות הב' ועם כמות הראשו' והם צ"ו | ||
| + | |- | ||
| + | | | ||
| + | ::We subtract from it 63, which is the second reserved; the remainder is 33. | ||
| + | ::<math>\scriptstyle{\color{blue}{96-63=33}}</math> | ||
| + | |style="text-align:right;"|נחסר מהם הס"ג שהוא השמור הב' והנשאר שהוא הל"ג | ||
| + | |- | ||
| + | | | ||
| + | ::We relate it to the first reserved, which is 56; the result is the remainder from the subtraction of the result of division of 3-quarters by 2-thirds from the result of division of 2-sevenths by one-sixth. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{2}{7}\div\frac{1}{6}\right)-\left(\frac{3}{4}\div\frac{2}{3}\right)=\frac{33}{56}}}</math> | ||
| + | |style="text-align:right;"|ניחסם אל השמור הראשון שהוא הנ"ו וההווה הוא ההוה מחסור היוצא מחלוק הג' רביעיות על הב' שלישיות מהיוצא מחלוק הב' שביעיות על הששית | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|וש'מ'ל‫' | ||
| + | |- | ||
| + | |} | ||
| + | |||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | == <span style=color:green>Roots</span> == | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | === The Roots of Integers === | ||
| + | |style="width:45%; text-align:right;"|<big>גדר השלמים</big> | ||
| + | |- | ||
| + | |<span style=color:green>Description of the extraction procedure:</span> | ||
| + | | | ||
| + | |- | ||
| + | |We arrange the required number in a line each [digit] according to its rank. | ||
| + | |style="text-align:right;"|נסדר המספר הדרוש בטור אח' כל אח' לפי מדרגתו | ||
| + | |- | ||
| + | |If the number of ranks is odd, we start from the last rank. | ||
| + | |style="text-align:right;"|ואם המספר המדרגות נפרדות נתחיל מהמדרגה האחרונה | ||
| + | |- | ||
| + | |If its is even, we start from the rank that precedes the last rank, while considering the last rank as tens and the one that precedes it as units. | ||
| + | |style="text-align:right;"|ואם זוג נתחיל ממדרגה הקודמת למדרגה האחרונה בעוד המדרגה האחרונה שתחשוב המדרגה האחרונה לעשרות והקודמת לה לאחדים | ||
| + | |- | ||
| + | |We seek for the closest root and write it beneath the required rank, i.e. the last or the one that precedes it; it is called the first root. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{a^2+r}\approx a}}</math> | ||
| + | |style="text-align:right;"|ונבקש שרש הקרוב ונכתבהו תחת המדרגה הנדרשת ר"ל האחרונה או הקודמת לה ויקרא השרש הא‫' | ||
| + | |- | ||
| + | |Then, we seek for a number, such that twice its product by the first root plus once by itself is enough to be subtracted from the rank that precedes the rank of the first root and from the one that precedes it; it is called the second root. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{100a^2+20ab+b^2}=\sqrt{\left(10a+b\right)^2}=10a+b}}</math> | ||
| + | |style="text-align:right;"|אח"ז נבק' ‫<ref>33v</ref>מספר שיספיק העולה מהכאתו עם השורש הראשון פעמים ועם עצמו אחת שיחוסר מהמדרגה הקודמת למדרגת השרש הראשון ומהקודמת לה ויקרא השרש הב‫' | ||
| + | |- | ||
| + | |We write it in the rank that precedes the [rank] of the first root. | ||
| + | |style="text-align:right;"|ונכתבהו במדרגה הקודמת לשרש הראשון | ||
| + | |- | ||
| + | |We further seek for a number, such that twice its product by the first and the second roots plus once by itself is enough to be subtracted from the rank that precedes [the rank of] the second root and from the two that precede it; it is called the third root. | ||
| + | |style="text-align:right;"|עוד אח"ז נבקש מספר שיספיק העולה מהעולה מהכאתו עם השרש הא' והב' פעמים ועם עצמו פעם אחת שיחוסר מהמדרגה הקודמת לשרש הב' ומהשתים הקודמת לה ויקרא השרש הג‫' | ||
| + | |- | ||
| + | |We write it in the rank that precedes the [rank] of the second root. | ||
| + | |style="text-align:right;"|כתבנהו במדרגה הקודמת לשרש הב‫' | ||
| + | |- | ||
| + | |We seek again for a number, such that twice its product by the first, the second, and the third roots plus once by itself is enough to be subtracted from the rank that precedes [the rank of the third root] and from the three that precede it; it is called the fourth root. | ||
| + | |style="text-align:right;"|עוד אח"ז תבקש מספר שיספיק העולה מהכאתו עם השרש הא' והב' והג' פעמים ועם עצמו פעם שיחוסר מהמדרגה הקודמת ומהג' הקודמת לה ויקרא שרש ד‫' | ||
| + | |- | ||
| + | |We write it in the rank that precedes the [rank] of the third root. | ||
| + | |style="text-align:right;"|וכתבנוהו במדרגה הקודמת לשרש הג‫' | ||
| + | |- | ||
| + | |And so on always until the subtracted rank is the first rank. | ||
| + | |style="text-align:right;"|וכן תמיד עד אשר תגיע שיהיה המדרג' הנחסרת המדרגה הראשונה | ||
| + | |- | ||
| + | |The numbers that are written beneath the ranks are themselves the root. | ||
| + | |style="text-align:right;"|והמספרים הכתובים תחת המדרגות הם השרש בעצמם | ||
| + | |- | ||
| + | |But, if there is a remainder from the required number that cannot be divided, know that the required number does not have a root. | ||
| + | |style="text-align:right;"|<big>ואולם</big> אם נשאר מהמספר הדרוש בלתי מתחלק דע שהמספר הדרוש הוא בלתי נגדר | ||
| + | |- | ||
| + | | | ||
| + | ==== <span style=color:green>Approximating the root of a non-square number</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | |The way of knowing the approximate root is that you add to the required number whatever even number of zeros you wish, then apply this same procedure itself, until you come to subtract from the first rank, as I write: | ||
| + | |style="text-align:right;"|והדרך בידיעת שרש הקרו' הוא זה שתוסיף על המספר הדרוש איזה זוג תפרש שתרצה ותנהיג זה הדרך בעצמו עד שתגיע לחסר מהמדרג' הראשונה כזה שאני כותב | ||
| + | |- | ||
| + | |<span style=color:green>Exammple:</span> <math>\scriptstyle{\color{blue}{\sqrt{356870000}}}</math> | ||
| + | :<span style=color:green>the solving procedure is not given, but it seems that it is based on the following calculations:</span> | ||
| + | :<math>\scriptstyle{\color{blue}{1^2\longrightarrow3-1^2=3-1=2}}</math> | ||
| + | :<math>\scriptstyle{\color{blue}{\left[\left[2\sdot\left(10\sdot1\right)\right]+8\right]\sdot8=\left(20\sdot8\right)+8^2\longrightarrow\begin{cases}\scriptstyle25-\left(2\sdot8\right)=25-16=9\\\scriptstyle96-8^2=96-64=32\\\end{cases}}}</math> | ||
| + | :<math>\scriptstyle{\color{blue}{\left[\left[2\sdot\left(10\sdot18\right)\right]+8\right]\sdot8=\left(360\sdot8\right)+8^2\longrightarrow\begin{cases}\scriptstyle32-\left(3\sdot8\right)=32-24=8\\\scriptstyle88-\left(6\sdot8\right)=88-48=40\\\scriptstyle407-8^2=343\\\end{cases}}}</math> | ||
| + | :<math>\scriptstyle{\color{blue}{\left[\left[2\sdot\left(10\sdot188\right)\right]+9\right]\sdot9=\left(3760\sdot9\right)+9^2\longrightarrow\begin{cases}\scriptstyle34-\left(3\sdot9\right)=34-27=7\\\scriptstyle73-\left(7\sdot9\right)=73-63=10\\\scriptstyle100-\left(6\sdot9\right)=100-54=46\\\scriptstyle460-9^2=379\\\end{cases}}}</math> | ||
| + | :<math>\scriptstyle{\color{blue}{\left[\left[2\sdot\left(10\sdot1889\right)\right]+1\right]\sdot1=\left(37780\sdot1\right)+1^2\longrightarrow\begin{cases}\scriptstyle3-\left(3\sdot1\right)=0\\\scriptstyle7-\left(7\sdot1\right)=0\\\scriptstyle9-\left(7\sdot1\right)=9-7=2\\\scriptstyle20-\left(8\sdot1\right)=20-8=12\\\scriptstyle20-1^2=19\\\end{cases}}}</math> | ||
| + | :<span style=color:green>Hence the root is 18891 and the remainder is 119</span> | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | <br> <br> <br>0<br>2<br>3||style="text-align:right;"|0<br><span style=color:red>9</span><br>04<br>38<br>92<br>56||00<br>1<span style=color:red>7</span><br>74<br>40<br>03<br>87|| <br> <br><span style=color:red>2</span>1<br><span style=color:red>9</span>2<br>69<br>00|| <br> <br> <br>1<br>29<br>00 | ||
| + | |-style="text-align:right;" | ||
| + | |1||8||8||9||1 | ||
| + | |} | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | |Then, multiply the result by sixty and again by sixty, until the number of zeros at the beginning of the product is half the number of the zeros that were added, and the denominator of the result is the number of times that it is multiplied by 60. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{a}=\frac{\sqrt{60^{2n}\sdot a}}{60^n}}}</math> | ||
| + | |style="width:45%; text-align:right;"|אח"ז תכה היוצא בששים והעולה בששים עד שיהיו תפארש שבראש טור העולה בהכאת כמות חצי התפראש הנוספות והעולה יהיה איכותו במספר הפעמים שהוכה בס‫' | ||
| + | |- | ||
| + | |I.e. if it was multiplied once, they are minutes. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a}{60}=a^\prime}}</math> | ||
| + | |style="text-align:right;"|ר"ל אם הוכה פעם אחת הם ראשונים | ||
| + | |- | ||
| + | |If twice, they are seconds. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a}{60^2}=a^{\prime\prime}}}</math> | ||
| + | |style="text-align:right;"|ואם ב' פעמים הם שניים | ||
| + | |- | ||
| + | |If three times, they are thirds. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a}{60^3}=a^{\prime\prime\prime}}}</math> | ||
| + | |style="text-align:right;"|ואם ג' פעמים הם שלישים | ||
| + | |- | ||
| + | |If four, they are fourths. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a}{60^4}=a^{iv}}}</math> | ||
| + | |style="text-align:right;"|ואם ארבעה הם רביעים | ||
| + | |- | ||
| + | |Then, erase the zeros [that were added in the first step] from the resulting number and divide what remains by sixty; the result is raised by one rank. | ||
| + | |style="text-align:right;"|אח"ז השלך מהמספר העולה מהמספר הכאת הספראש והנשאר תחלקהו על ששים והיוצא יעלה מדרגה א‫' | ||
| + | |- | ||
| + | |I.e. if they are fourths, the result is thirds and the remainder that cannot be divided are fourths. Keep them. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(a_1+60\sdot a_2\right)^{iv}=a_1^{iv}+a_2^{\prime\prime\prime}}}</math> | ||
| + | |style="text-align:right;"|ר"ל שאם היו רביעיי' היוצא היה שלישים והנשארים בלתי נחלקם הם רביעיים ושמרם | ||
| + | |- | ||
| + | |Divide also the result of division, which are thirds, by 60; the result are seconds and the remainder that cannot be divided are thirds. Keep them. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{a_1^{iv}+\left(a_2+60\sdot a_3\right)^{\prime\prime\prime}=a_1^{iv}+a_2^{\prime\prime\prime}+a_3^{\prime\prime}}}</math> | ||
| + | |style="text-align:right;"|עוד חלק היוצא מהחלוק שהם שלישים על ס' והיוצאים בחילוק הם שניים והנשארים בלתי נחלקים הם שלישיים ושמרם | ||
| + | |- | ||
| + | |Divide also the result of division, which are seconds, by 60; the result are minutes and the remainder that cannot be divided are seconds. Keep them. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{a_1^{iv}+a_2^{\prime\prime\prime}+\left(a_3+60\sdot a_4\right)^{\prime\prime}=a_1^{iv}+a_2^{\prime\prime\prime}+a_3^{\prime\prime}+a_4^{\prime}}}</math> | ||
| + | |style="text-align:right;"|עוד חלק היוצאים בחלוק שהם ב' על ס' והיוצא בחלוק הם ראשונים הנשארים בלתי נחלקי' הם שניים ושמרם | ||
| + | |||
| + | |- | ||
| + | |Then, divide the minutes by 60; the result are degrees, i.e. integers and the remainder are minutes. So, the result are degrees, minutes, seconds, thirds, and fourths. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{a_1^{iv}+a_2^{\prime\prime\prime}+a_3^{\prime\prime}+\left(a_4+60\sdot a_5\right)^{\prime}=a_1^{iv}+a_2^{\prime\prime\prime}+a_3^{\prime\prime}+a_4^{\prime}+a_5}}</math> | ||
| + | |style="text-align:right;"|אח"ז חלק הראשונים על ס' והיוצא הם מעלות ר"ל שלמים והנשארים הם ראשונים וככה הוא היוצא מהמעלות והראשונים והשניים והשלישים והרביעיים | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === The Roots of Fractions === | ||
| + | |style="text-align:right;"|<big>שרש השברים</big> | ||
| + | |- | ||
| + | |If its numerator and its denominator have a root, take the root of its numerator and relate it to the root of its denominator. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{\frac{a^2}{b^2}}=\frac{\sqrt{a^2}}{\sqrt{b^2}}=\frac{a}{b}}}</math> | ||
| + | |style="text-align:right;"|אם היה כמותם ואכותם נגדר תקח גדר כמותם ותיחסהו לגדר אכותם | ||
| + | |- | ||
| + | | | ||
| + | :*As 4-ninths. | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{\frac{4}{9}}}}</math> | ||
| + | |style="text-align:right;"|כגון ד' תשעיות | ||
| + | |- | ||
| + | | | ||
| + | ::The root of 4 is two; the root of 9 is 3; so, the root of 4-ninths is two-thirds. | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{\frac{4}{9}}=\sqrt{\frac{2^2}{3^2}}=\frac{\sqrt{2^2}}{\sqrt{3^2}}=\frac{2}{3}}}</math> | ||
| + | |style="text-align:right;"|גדר הד' שנים וגדר הט' ג' א"כ גדר הד' תשעיות הם שני שלישיות | ||
| + | |- | ||
| + | |If its numerator and its denominator do not have a root, or only its denominator, multiply the denominator by itself, then multiply the numerator by the denominator and relate it to the first product. | ||
| + | |style="text-align:right;"|ואם אכותם וכמותם בלתי נגדר או איכותם לבד תכה האיכות בעצמו ותכה הכמות עם האיכות ותיחסהו לההכאה הראשונה | ||
| + | |- | ||
| + | |Apply the same method: take the root of the numerator, i.e. the product of the numerator by the denominator, if it has an integer root, or approximately, if it does not have an integer root, and relate it to the root of the product of the denominator by the denominator and this is the approximate root. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{\frac{a^2}{b}}=\sqrt{\frac{a^2\sdot b}{b^2}}=\frac{\sqrt{a^2\sdot b}}{\sqrt{b^2}}}}</math> | ||
| + | |style="text-align:right;"|ותעשה זה הדרך בעצמו שתקח גדר הכמות ר"ל שהוא הכאת הכמות עם האיכות שהוא כמות הכאת האיכות עם האיכות אם יש לו גדר שלם או {{#annot:term|1613,2072|lC3Q}}על דרך קרוב{{#annotend:lC3Q}} אם אין לו גדר שלם ותיחסהו לגדר העולה מהכאת האיכות עם האיכות וזהו השרש הקרוב | ||
| + | |- | ||
| + | |If its numerator alone does not have a root and its denominator has a root, take the approximate root of the numerator and relate it to the denominator. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{\frac{a^2+r}{b^2}}=\frac{\sqrt{a^2+r}}{\sqrt{b^2}}\approx\frac{a}{b}}}</math> | ||
| + | |style="text-align:right;"|ואם כמותם לבד בלתי נגדר ואיכותם הוא המספר הנגדר קח שרש הקרוב לכמות ותיחסהו אל האיכות | ||
| + | |- | ||
| + | |If there are integers and fractions, decompose the integers to fractions, then relate [the numerator] to the denominator according the same preceding method. | ||
| + | |style="text-align:right;"|ואם היה שלמים ושברים תתיך השלמים עד שיהו שברים ותיחסם אל האיכות על דרך בעינו שהקדמנו | ||
| + | |- | ||
| + | |} | ||
| + | |||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | == <span style=color:green>Cubic Roots</span> == | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | === Cubic Roots of Integers === | ||
| + | |style="width:45%; text-align:right;"|<big>מעוקב השלמים</big> | ||
| + | |- | ||
| + | |<span style=color:green>Description of the extraction procedure:</span> | ||
| + | | | ||
| + | |- | ||
| + | |Arrange the required line each according to its rank. | ||
| + | |style="text-align:right;"|תסדר הטור הדרוש כל אח' כפי מדרגתו | ||
| + | |- | ||
| + | |After every three digits draw a line. | ||
| + | |style="text-align:right;"|ובסוף כל ג' אותיות תשים קו | ||
| + | |- | ||
| + | |Beggin from the last line [= the leftmost dividing line]. | ||
| + | |style="text-align:right;"|ותתחיל מהקו האחרון | ||
| + | |- | ||
| + | |We write there a number to be cubed and subtracted from the number that is above it and its preceding ranks. It is called the first cube root. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt[3]{a^3+r}\approx a}}</math> | ||
| + | |style="text-align:right;"|ונכתוב שם מספר שיוכה בדרך עקוב ויחוסר מהמספר שעליו עם עזר המדרגות הקודמו' ויקרא היסוד הראשון | ||
| + | |- | ||
| + | |Then, write a second cube root at the place of the preceding line, to be multiplied by the product of the first [cube root] by 3 multiplied by the product of the first and the second cube roots together, so that the result is subtracted from the second digit and its preceding digits. Cube also the second cube root and subtract the product from the number that is above it and its preceding digits. | ||
| + | |style="text-align:right;"|אח"ז תכתוב במקום הקו הקודמת יסוד ב' ושיוכה עם העולה מהכאת הראשון בג' והעול' עם היסוד הראשון והב' יחד והעולה שיחוסר מהמספר שני לו עם עזר הנמשכים לו גם תכה היסוד הב' באופ' מעוקב והעולה תחסרהו מהמספר שעליו עם עזר המספרים הקודמים לו | ||
| + | |} | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt[3]{\left(10a\right)^3+3\sdot\left[\left(10a\right)^2\sdot b\right]+3\sdot\left(10a\sdot b^2\right)+b^3}=\sqrt[3]{\left(10a+b\right)^3}=10a+b}}</math> | ||
| + | {| | ||
| + | |- | ||
| + | |Then, we write a third cube root at the place of the preceding line, to be multiplied by the product of the first and second [cube roots] together by 3 multiplied by the product of the first the second and the third cube roots together, so that [the result] is subtracted from the second [digit] and its preceding digits. We also cube the third cube root and subtract the product from the number that is above it and its preceding digits. | ||
| + | |style="width:45%; text-align:right;"|אח"ז נכתו' במקום הקו הקודמת יסו' ג' שיוכה עם העולה מהכאת הראשון והב' יחד עם מספר הג' והעולה עם היסוד הראשון והב' והג' ויחוס' מהמרב' הב' עם עזר המדרגות הנמשכים לו גם נכה היסוד הג' באופן מעוקב והעולה נחסרהו מן המספר שעליו עם עזר המספרים הקודמים לו | ||
| + | |- | ||
| + | |And so on in that order. | ||
| + | |style="text-align:right;"|וכן תמיד בסדר הזה | ||
| + | |- | ||
| + | | | ||
| + | ==== <span style=color:green>Approximating the root of a non-cubic number</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | |When we reach the rank of units, but there are digits that are still left of the required number and you want to know its approximate cube root, we add to the ranks that precede the units three zeroes or six, or nine, or as many as you wish, as long as you add them in threes. | ||
| + | |style="text-align:right;"|<big>וכאשר</big> הגענו אל מדרגת האחדים ממנו ונשארו מספרים על המספר הדרוש ותרצה לדעת עוד יסודו הקרוב נוסיף על המדרגו' המונחות מצד האחדים ג' סיפראש או ששה או ט' או מה שתרצה לבד שתוסיפהו ג'ג‫' | ||
| + | |- | ||
| + | |Then, we apply the preceding method for finding the cube root until reaching the first rank of the zeroes. | ||
| + | |style="text-align:right;"|ואח"ז נעשה מה שקד' מהדרך למציאות היסוד עד שתגיע למדרגה הראשונה מהסיפראש | ||
| + | |- | ||
| + | |We take all the cube roots, arrange them in one line according the ranks, and erase one third of the number of zeros [added in the first step]. | ||
| + | |style="text-align:right;"|אח"ז נקח כל היסודות ונסדרם בטור אח' על סדר המדרגות ונשליך מהם במספר שליש הסיפראש | ||
| + | |- | ||
| + | |We take the integer cube roots; they are degrees. | ||
| + | |style="text-align:right;"|‫<ref>34r</ref>ונקח היסודות האחדות והם מעלות | ||
| + | |- | ||
| + | |Then, we multiply what remains by 60, erase three zeros from [the product] and take the remainder; they are minutes. | ||
| + | |style="text-align:right;"|אח"ז נכה הנשלכים בס' והעולה נשליך מהם כמספר ג' הסיפראס ונקח הנשארים והם ראשונים | ||
| + | |- | ||
| + | |We proceed like this until all the zeros [added in the first step] are erased. | ||
| + | |style="text-align:right;"|וכן נעשה תמיד עד שיכלה הסיפראש | ||
| + | |- | ||
| + | |Then, we sum up all and it is the approximate cubic root. | ||
| + | |style="text-align:right;"|ואז נחבר הכל יהיה הוא היסוד הקרוב | ||
| + | |- | ||
| + | !Another method for a number that has no [integer] cube root | ||
| + | |style="text-align:right;"|דרך אחר למספר שאין בו יסוד מעוקב | ||
| + | |- | ||
| + | |Add zeros as many as you wish, as long as they are added in threes. | ||
| + | |style="text-align:right;"|שתוסיף סיפראש כמה שתרצה ובלבד שיהיו נוספים בתוספת ג'ג‫' | ||
| + | |- | ||
| + | |We apply the previous procedure until reaching the first zero. | ||
| + | |style="text-align:right;"|ונשתמש עם הדרך הקודם בעינו עד שנגיע לסיפרא הראשונה | ||
| + | |- | ||
| + | |Afterwards, we take all the cube roots that are arranged beneath the required number, multiply them by 60, then the product by 60, and so on until the number of the zeroes that appear at the beginning of the resulting line is a third of the number of the zeroes [added in the first step]. | ||
| + | |style="text-align:right;"|אח"ז נקח כל היסודות המסודרות תחת מספר הדרוש ונכהו בס' והעולה בס' וזה עד שתהיינ' הסיפראש היוצאות בראש הטור העולה מההכאות בכמות שליש הסיפראש | ||
| + | |- | ||
| + | |We erase the zeros [added in the first step] and divide the result by 60. The remainder is [of the type] of the denominator of the divided fractions. | ||
| + | |style="text-align:right;"|אח"ז נשליך הסיפראש ונחלק הנשאר על ס' והנשאר יהיה מאיכות השברים המחולקים | ||
| + | |- | ||
| + | |I.e. if it was multiplied only once, they are minutes. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a}{60}=a^\prime}}</math> | ||
| + | |style="text-align:right;"|ר"ל שאם הוכו פעם אחת לבד יהיו ראשונים | ||
| + | |- | ||
| + | |If twice, they are seconds. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a}{60^2}=a^{\prime\prime}}}</math> | ||
| + | |style="text-align:right;"|ואם ב' שניים | ||
| + | |- | ||
| + | |If three [times], they are thirds. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a}{60^2}=a^{\prime\prime\prime}}}</math> | ||
| + | |style="text-align:right;"|ואם ג' שלישיים | ||
| + | |- | ||
| + | |The result is of the type that precedes the type of the dividend, i.e. if they were minutes, the result of division are degrees; if they were seconds, the result of division are minutes; and so on. | ||
| + | |style="text-align:right;"|והיוצא הוא ממין הקודם למין ז' המחולקים ר"ל שאם היו ראשונים היוצא מהחלוקה מעלות ואם היו שניים היוצא מהחלוקה ראשונים וכן תמיד | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === Cubic Roots of Fractions === | ||
| + | |style="text-align:right;"|<big>יסוד השברים המעוקבים</big> | ||
| + | |- | ||
| + | |If the numerator and the denominator are cube numbers, take the cube [root] of the numerator and relate it to the cube [root] of the denominator. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt[3]{\frac{a^3}{b^3}}=\frac{\sqrt[3]{a^3}}{\sqrt[3]{b^3}}=\frac{a}{b}}}</math> | ||
| + | |style="text-align:right;"|אם הכמות והאיכות מעוקב תקח מעוקב הכמות ותיחסהו אל מעוקב האיכות | ||
| + | |- | ||
| + | |If they are not cube numbers, or the denominator alone is not a cube number, we cube the denominator and multiply the numerator by the product of the denominator by itself, then we relate the cube root of the second reserved, which is the product of the numerator by the product of the denominator by itself, to the first reserved, which is the cube of the denominator. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt[3]{\frac{a^3}{b}}=\sqrt[3]{\frac{a^3\sdot b^2}{b^3}}=\frac{\sqrt[3]{a^3\sdot b^2}}{\sqrt[3]{b^3}}}}</math> | ||
| + | |style="text-align:right;"|ואם אינם מעוקבים או האיכות לבד בלתי מעוקב נכה האיכות בדרך עקוב ונכה הכמות עם העולה מהכאת האיכות בעצמו אח"ז ניחס יסוד השמור הב' שהוא הכאת הכמות עם העולה מהכאת האיכות בעצמו אל השמור הראשון שהוא העולה מהכאת האיכות בדרך עקוב | ||
| + | |- | ||
| + | |If the denominator is a cube, we look for the approximate cube root of the numerator and relate it to the cube root of the denominator. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt[3]{\frac{a^3+r}{b^3}}=\frac{\sqrt[3]{a^3+r}}{\sqrt[3]{b^3}}\approx\frac{a}{b}}}</math> | ||
| + | |style="text-align:right;"|ואם איכותם נעקב נבקש יסוד הכמות הקרוב והיוצא ניחסהו אל מעוקב האיכות | ||
| + | |- | ||
| + | | | ||
| + | ::I.e. if its cube [root] is 3, the ratio is a third and so on. | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt[3]{\frac{a^3+r}{3^3}}=\frac{\sqrt[3]{a^3+r}}{\sqrt[3]{3^3}}\approx\frac{1}{3}a}}</math> | ||
| + | |style="text-align:right;"|ר"ל שאם היה מעוקבו ג' היה היחס הוא השליש וכן תמי‫' | ||
| + | |- | ||
| + | |If there are integers and fractions together, we decompose the integers to fractions, then proceed according to the previous method. | ||
| + | |style="text-align:right;"|ואולם בשלמים ושברים יחד נתיך השלמים לשברים ונעשה הדרך הקודם בעינו | ||
| + | |- | ||
| + | |} | ||
| + | |||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | == <span style=color:green>Divisibility of a Number</span> == | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | === <span style=color:green>3; 6; 9</span> === | ||
| + | | | ||
| + | |- | ||
| + | |To know if a certain number has a third, a sixth, or a ninth without fractions [= if 3, 6, or 9 are divisors of the number]: | ||
| + | |style="width:45%; text-align:right;"|<big>לידע</big> אם יש שלישי' או ששי' או ט' מספר מה מבלי שברים | ||
| + | |- | ||
| + | |First, you should know if it has a ninth, and this is known by summing the numerals of the number as if they were units. | ||
| + | |style="text-align:right;"|תדע ראשונה אם יש לו תשיעי' וזה יודע כשתחבר רשמי מספר החשבון כאלו הם אחדים | ||
| + | |- | ||
| + | |If it is cast out by nines, it is known that is has a ninth, as well as a third [= divisible by 9 and 3]. | ||
| + | |style="text-align:right;"|ואם יושלך לט"ט בידוע שיש לו תשיעיות וג"כ שלישי‫' | ||
| + | |- | ||
| + | |Also a sixth, if the number is even [= divisible by 6], but not if it is odd. | ||
| + | |style="text-align:right;"|וג"כ ששית אם המספר זוג ואם נפרד לאו | ||
|- | |- | ||
| − | | | + | |If 6 or 3 remain, it has a third [= divisible by 3], and, if it is an even number, a sixth also [= divisible by 6], but not a ninth [= not divisible by 9]. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם ישארו ו' או ג' יהיה לו שלישי' וג"כ ששית אם הוא זוג אבל לא תשיעית |
|- | |- | ||
| | | | ||
| − | === | + | === <span style=color:green>2; 4; 8</span> === |
| − | + | | | |
| + | |- | ||
| + | |If you wish to know if it has a half, a quarter, or an eighth [= if 2, 4, or 8 are divisors of a the number]: | ||
| + | |style="text-align:right;"|ואם תרצה לידע אם יש לו מחצית או ד' או שמינית | ||
| + | |- | ||
| + | |If the number is odd, it does not have any of them [= not divisible by 2, 4, or 8]. | ||
| + | |style="text-align:right;"|אם חשבון נפרד אין לו שום אחד מהם | ||
| + | |- | ||
| + | |If it is even, it has a half [= divisible by 2]. | ||
| + | |style="text-align:right;"|ואם זוג בידוע שיש לו חצי | ||
| + | |- | ||
| + | |To know if [it also has] a quarter and an eighth: | ||
| + | |style="text-align:right;"|ולידע אם לא ג"כ רביעית ושמיני‫' | ||
|- | |- | ||
| | | | ||
| − | + | :Take the number that is in the first rank to the right [= units] as it is. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|קח המספר אשר במעלה הראשונה לצד ימין כמו שהיא |
|- | |- | ||
| | | | ||
| − | + | :Double the one that is in the second [rank] [= tens], but if there is no number there, do not take any thing. | |
| − | |style="text-align:right;"|ואם | + | |style="text-align:right;"|ואשר בשנייה כפול ואם אין שם מספר לא תקח כלום |
|- | |- | ||
| | | | ||
| − | + | :If the one that is in the third [rank] [= hundreds] is an odd number, multiply it by 4, if it is an even number or a zero, do not take any thing. | |
| − | |style="text-align:right;"|ואם | + | |style="text-align:right;"|ואשר בשלישית אם הוא נפרד כפלהו בד' ואם הוא זוג או סיפרא לא תקח כלום |
|- | |- | ||
| | | | ||
| − | : | + | :From the [fourth] rank onward leave [the digits] and do not take any thing. Sum up all that you have gathered up to the third rank according to this procedure. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכן מהמעלה הג' ולמעלה תניח אותו לא תקח כלום וקבץ כל מה שקבצת עד המעלה הג' עד"ז |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | :<math>\scriptstyle{\color{OliveGreen}{2a+10b+\left[\left(2c-1\right)\sdot100\right]\longrightarrow 2a+2b+4\sdot\left(2c-1\right)}}</math> | |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{2a+10b+\left(2c\sdot100\right)\longrightarrow2a+2b}}</math> | |
| | | | ||
|- | |- | ||
| − | | | + | |If it is cast out by eights it has an eighth [= divisible by 8], the more so a quarter [= divisible by 4]. |
| − | == | + | |style="text-align:right;"|אם יושלך באלו לח' יש לו שמינית וכ"ש רביעי‫' |
| − | |||
|- | |- | ||
| − | | | + | |If 4 remains, it has a quarter [= divisible by 4] alone. |
| − | |style=" | + | |style="text-align:right;"|ואם ישארו ד' יש לו ד' לבד |
|- | |- | ||
| − | | | + | |If another number remains, it does not have even a quarter [= not divisible by 4]. |
| − | + | |style="text-align:right;"|ואם נותר מספר אחר אין לו אפי' רביעית | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |style="text-align:right;"|ואם | ||
|- | |- | ||
| | | | ||
| − | === <span style=color:green> | + | === <span style=color:green>7</span> === |
| | | | ||
|- | |- | ||
| − | |If you wish to know if it has a | + | |If you wish to know if it has a seventh [= if 7 is a divisor of a given number] |
| − | |style="text-align:right;"|ואם תרצה לידע אם יש לו | + | |style="text-align:right;"|ואם תרצה לידע אם יש לו שביעי‫' |
|- | |- | ||
| − | |If | + | |If has a few methods: |
| − | + | |style="text-align:right;"|יש בזה דרכים | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | *The first is that you set these numerals 1, 3, 2, 6, 4, 5 by the order of the ranks, then multiply each of these numerals by its corresponding rank, consider all as units, and cast out the sevens. If it is cast out by sevens it has a seventh [= divisible by 7], otherwise it has none. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הראשון שתניח אלו האותיות א'ג'ב'ו'ד'ה' על סדר המדרגות חלילה ותכה כל א' מאלו האותיות במדרגה שכנגדה וחשב כל האחדים והשלך השביעיות ואם יושלך לז' יש לו שביעית ואם לאו לאו |
|- | |- | ||
| | | | ||
| − | + | *The second is that you multiply the leftmost digit by 3, add [the product] to what you find in the rank that precedes it and cast out the sevens, then multiply the remainder from casting out the sevens by 3, add [the product] to what you find preceding it, and so on repeatedly, up to the first rank. If it is cast out by sevens, it has a seventh [= divisible by 7]. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>הב'</big> שתכה הרושם האחרון שלצד שמאל בג' וחברהו לאשר תמצא במעלה שלפניו ותשליכה לז' והנשאר תחברהו לאשר לפניו ותכה הנשאר מהשלכת השביעיו' בג' וחברהו לאשר תמצא לפניו וכן תמיד עד המדרגה הראשונה ואם יושלך ז'ז' יש לו שביעית |
|- | |- | ||
| | | | ||
| − | :If | + | :If you do not find there any number but a zero, for every zero, multiply the remainder by 3, until all the zeros end and so on [the procedure continues as described]. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם לא תמצא שם מספר כי אם סיפרא תכה הנשאר בג' וכן בכל ספרא וספרא תכה הנשאר בג' עד שיכלו כל סיפראים וכן תמיד |
|- | |- | ||
| | | | ||
| − | + | ||
| − | + | === <span style=color:green>11</span> === | |
| − | |||
| − | |||
| − | |||
| − | : | ||
| | | | ||
|- | |- | ||
| − | | | + | |To know if it has an eleventh, i.e. if it is cast out by elevens [= if 11 is a divisor of a given number]: |
| − | |style="text-align:right;"|אם יושלך | + | |style="text-align:right;"|ולידע אם לו י"א ר"ל אם יושלך לי"א |
|- | |- | ||
| − | + | |Subtract the leftmost [numeral] from the one that precedes it, then the remainder from the one that precedes the preceding and so on repeatedly. | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |Subtract the leftmost [numeral] from the one that precedes it, then the remainder from the one that precedes the preceding and so on repeatedly. | ||
|style="text-align:right;"|תחסר האחרון שלצד שמאל מאשר לפניו והנשאר מאשר לפני פניו וכן תמיד | |style="text-align:right;"|תחסר האחרון שלצד שמאל מאשר לפניו והנשאר מאשר לפני פניו וכן תמיד | ||
|- | |- | ||
| Line 2,695: | Line 3,300: | ||
| | | | ||
| − | == Square Numbers == | + | == <span style=color:green>Square Numbers</span> == |
| | | | ||
|- | |- | ||
| Line 2,702: | Line 3,307: | ||
|- | |- | ||
|Take its third, multiply it by itself and rise it to the next rank - if it is in the units, the next rank is the tens; if it is in the tens, the next rank is the hundreds. Subtract from it the [square] of the third and the remainder is the square you wish to know. | |Take its third, multiply it by itself and rise it to the next rank - if it is in the units, the next rank is the tens; if it is in the tens, the next rank is the hundreds. Subtract from it the [square] of the third and the remainder is the square you wish to know. | ||
| − | :<math>\scriptstyle a^2=\left[10\sdot\left(\frac{1}{3}\sdot a\right)^2\right]-\left(\frac{1}{3}\sdot a\right)^2</math> | + | :<math>\scriptstyle{\color{OliveGreen}{a^2=\left[10\sdot\left(\frac{1}{3}\sdot a\right)^2\right]-\left(\frac{1}{3}\sdot a\right)^2}}</math> |
|style="text-align:right;"|קח שלישיתו והכהו בעצמו והעלהו למדרגה הקרובה אם היא אחדים המדרגה הקרובה היא העשרות ואם היא עשרות המדרגה הקרובה היא המאות וחסר מהם הכאת השליש ומה שישאר הוא המרובע שרצית לידע | |style="text-align:right;"|קח שלישיתו והכהו בעצמו והעלהו למדרגה הקרובה אם היא אחדים המדרגה הקרובה היא העשרות ואם היא עשרות המדרגה הקרובה היא המאות וחסר מהם הכאת השליש ומה שישאר הוא המרובע שרצית לידע | ||
|- | |- | ||
| | | | ||
| − | ::Example: if you wish to know how much is the square of 9. | + | ::{{#annot:9²|857|2n2J}}Example: if you wish to know how much is the square of 9. |
| − | |style="text-align:right;"|המשל אם תרצה לידע כמה הוא מרובע הט‫' | + | |style="text-align:right;"|המשל אם תרצה לידע כמה הוא מרובע הט‫'{{#annotend:2n2J}} |
|- | |- | ||
| | | | ||
::Take its third, which is 3; multiply it by itself; the result is 9. Rise it to the next rank; it is ninety. Subtract from it the [square] of the third, which is 9; eighty-one remains and it is the square of 9. | ::Take its third, which is 3; multiply it by itself; the result is 9. Rise it to the next rank; it is ninety. Subtract from it the [square] of the third, which is 9; eighty-one remains and it is the square of 9. | ||
| − | |style="text-align:right;"|קח שלישיתו והוא הג' והכה אותו בעצמו ויעלה ט' עלהו למדרגה הקרובה והיא תשעים חסר מהם הכאת השליש והוא ט' ישאר א' ושמונים והוא מרובע הט‫' | + | |style="text-align:right;"|קח שלישיתו והוא הג' והכה אותו בעצמו ויעלה ט' ‫<ref>34v</ref>עלהו למדרגה הקרובה והיא תשעים חסר מהם הכאת השליש והוא ט' ישאר א' ושמונים והוא מרובע הט‫' |
|} | |} | ||
::<math>\scriptstyle{\color{blue}{9^2=\left[10\sdot\left(\frac{1}{3}\sdot9\right)^2\right]-\left(\frac{1}{3}\sdot9\right)^2=\left(10\sdot3^2\right)-3^2=\left(10\sdot9\right)-9=90-9=81}}</math> | ::<math>\scriptstyle{\color{blue}{9^2=\left[10\sdot\left(\frac{1}{3}\sdot9\right)^2\right]-\left(\frac{1}{3}\sdot9\right)^2=\left(10\sdot3^2\right)-3^2=\left(10\sdot9\right)-9=90-9=81}}</math> | ||
| Line 2,721: | Line 3,326: | ||
|- | |- | ||
| | | | ||
| − | ::Example: if you wish to know the square of 8. | + | ::{{#annot:8²|857|IvM9}}Example: if you wish to know the square of 8. |
| − | |style="text-align:right;"|המשל אם תרצה לידע מרובע הח‫' | + | |style="text-align:right;"|המשל אם תרצה לידע מרובע הח‫'{{#annotend:IvM9}} |
|- | |- | ||
| | | | ||
| Line 2,736: | Line 3,341: | ||
|- | |- | ||
| | | | ||
| − | ::Such as: you wish to know the square of 7. | + | ::{{#annot:7²|857|kNhC}}Such as: you wish to know the square of 7. |
| − | |style="text-align:right;"|כגון רצית לידע מרובע ז‫' | + | |style="text-align:right;"|כגון רצית לידע מרובע ז‫'{{#annotend:kNhC}} |
|- | |- | ||
| | | | ||
| Line 2,749: | Line 3,354: | ||
| | | | ||
| − | == | + | == <span style=color:green>Multiplicative Supplementing</span> == |
| | | | ||
|- | |- | ||
|Numerical operation called jaber in Arabic | |Numerical operation called jaber in Arabic | ||
| − | + | |style="width:45%; text-align:right;"|<big>מין מספר הנקרא בערבי {{#annot:term|695,1307|ul5O}}ג'בר{{#annotend:ul5O}}</big> | |
|- | |- | ||
| − | | | + | |I do not know its name in Hebrew. |
|style="text-align:right;"|ולא ידעתי לו שם בלשוננו | |style="text-align:right;"|ולא ידעתי לו שם בלשוננו | ||
|- | |- | ||
| − | |In foreign language '''reducir''' | + | |In foreign language [it is] '''reducir'''. |
| − | |style="text-align:right;"|ובלע"ז | + | |style="text-align:right;"|ובלע"ז רידוזיר |
|- | |- | ||
| − | | | + | |Its meaning is that if you have any number and you want to find by which number we multiply this fractionor these fractions, so that the resulting [product] will be that fraction or those fractions [= the complement number]. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{a\sdot x=b}}</math> | |
| − | :<math>\scriptstyle a\sdot x=b</math> | ||
|style="text-align:right;"|וענינו שאם יש לך שום מספ' ורצית לומ' באיזה מספר נכה זה השבר או אלו השברים עד שיעלה זה השבר או אלו השברים | |style="text-align:right;"|וענינו שאם יש לך שום מספ' ורצית לומ' באיזה מספר נכה זה השבר או אלו השברים עד שיעלה זה השבר או אלו השברים | ||
|- | |- | ||
| | | | ||
| − | :Example: <math>\scriptstyle{\color{blue}{\frac{2}{4}\sdot x=1+\frac{4}{5}}}</math> | + | :*Example: by what number we multiply 2-quarters, so that they become 1 and 4-fifths. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{2}{4}\sdot x=1+\frac{4}{5}}}</math> | ||
|style="text-align:right;"|במשל ב' רביעיות באיזה מספר נכו אותם עד שישובו א' וד' חמישיו‫' | |style="text-align:right;"|במשל ב' רביעיות באיזה מספר נכו אותם עד שישובו א' וד' חמישיו‫' | ||
|- | |- | ||
| − | | | + | |The method is that you divide this number by the specific fraction. |
| − | + | ::<math>\scriptstyle{\color{OliveGreen}{x=b\div a}}</math> | |
|style="text-align:right;"|והדרך בזה שתחלק זה המספר על השבר המיוחד | |style="text-align:right;"|והדרך בזה שתחלק זה המספר על השבר המיוחד | ||
|- | |- | ||
| − | | | + | |The result, as explained in the division of integers and fractions by fractions, is called [in Arabic] al-majbur [= complement, multiplicative supplement]. |
| − | + | |style="text-align:right;"|ומה שיעלה כמו שמבואר בחלוק השלמים ושברים על שברים הוא אלמגבור | |
| − | |style="text-align:right;"|ומה שיעלה כמו שמבואר בחלוק השלמים ושברים על שברים הוא | ||
|- | |- | ||
| | | | ||
| − | == | + | == <span style=color:green>Decomposing to a Fraction</span> == |
| | | | ||
|- | |- | ||
| − | | | + | |There is a numerical operation that is opposite to it, which is called '''bāb al-ḥaṭ''' [in Arabic]. |
| − | + | |style="text-align:right;"|ויש מין אחר הפכי לזה והוא הנקרא באב אלחט | |
|- | |- | ||
| | | | ||
| − | : | + | :*It is: by what number do we multiply 1 and four-fifth, so that it becomes 2-quarters, which lowers the value of the original. |
| + | ::<math>\scriptstyle{\color{blue}{\left(1+\frac{4}{5}\right)\sdot x=\frac{2}{4}}}</math> | ||
|style="text-align:right;"|והוא א' וארבע' חמישיות באיזה מספר נכה אותם עד שישובו ב' רביעיות שהוא חסרון בערך הראשון | |style="text-align:right;"|והוא א' וארבע' חמישיות באיזה מספר נכה אותם עד שישובו ב' רביעיות שהוא חסרון בערך הראשון | ||
|- | |- | ||
| | | | ||
| − | + | ::Do the opposite: divide 2-quarters by 1 and 4-fifths. | |
| − | + | ::<math>\scriptstyle{\color{blue}{x=\frac{2}{4}\div\left(1+\frac{4}{5}\right)}}</math> | |
|style="text-align:right;"|ותעשה ההפך שתחלק הב' רביעיו' על א' וד' חמישיות | |style="text-align:right;"|ותעשה ההפך שתחלק הב' רביעיו' על א' וד' חמישיות | ||
|- | |- | ||
| − | |Indication that the treatise relies on another work: | + | |<span style=color:green>[Indication that the treatise relies on another work:]</span> Although the author wrote two chapters concerning these [two issues], it seems that they can be included in one chapter. |
| − | |style="text-align:right;"|ואעפ"י | + | |style="text-align:right;"|ואעפ"י שהמחבר חברם בב' שערים נ"ל שהם מכללו בשער אח‫' |
| + | |- | ||
| + | |Understand this. | ||
| + | |style="text-align:right;"|והבן | ||
|- | |- | ||
|} | |} | ||
| Line 2,803: | Line 3,411: | ||
| | | | ||
| − | == Geometric Shapes == | + | == <span style=color:green>Geometric Shapes</span> == |
| | | | ||
|- | |- | ||
| | | | ||
| − | === Square === | + | === <span style=color:green>Square</span> === |
| + | | | ||
| + | |- | ||
| | | | ||
| + | :*If a square whose sides are ten by ten, for instance, and you wish to know its area or it diagonal. | ||
| + | |style="text-align:right;"|<big>אם {{#annot:term|305,2062|MiTK}}מרובע נכון{{#annotend:MiTK}}</big> שיש בצלעיו עשר על עשר ד"מ ורצית לידע שטחו או אלכסונו | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Do as follows: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תעשה בזה הדרך |
|- | |- | ||
| | | | ||
| − | + | ::Multiply the side by the other [side] and it is its area. | |
| − | :<math>\scriptstyle S_{square}=side\times side</math> | + | ::<math>\scriptstyle{\color{OliveGreen}{S_{square}=side\times side}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תכה הצלע עם חבירו כאח' וזהו שטחו |
|- | |- | ||
| | | | ||
| − | + | ::Multiply the two sides each by itself and the root of the sum [of their products] is the diagonal. | |
| + | ::<math>\scriptstyle{\color{OliveGreen}{d_{square}=\sqrt{side^2+side^2}}}</math> | ||
|style="text-align:right;"|ותכה הב' צלעות כל אחת בפני עצמו וגדר של מקובצם הוא האלכסון | |style="text-align:right;"|ותכה הב' צלעות כל אחת בפני עצמו וגדר של מקובצם הוא האלכסון | ||
|- | |- | ||
| | | | ||
| − | * | + | :*If you know the area, its root is the side. |
| + | ::<math>\scriptstyle{\color{OliveGreen}{side_{square}=\sqrt{S}}}</math> | ||
|style="text-align:right;"|ואם ידעת השטח גדרו והוא הצלע | |style="text-align:right;"|ואם ידעת השטח גדרו והוא הצלע | ||
|- | |- | ||
| | | | ||
| − | * | + | :*If you know the diagonal, the root of half its product by itself is the side. |
| + | ::<math>\scriptstyle{\color{OliveGreen}{side_{square}=\sqrt{\frac{1}{2}\sdot d^2}}}</math> | ||
|style="text-align:right;"|ואם ידעת האלכסון גדר חצי הכאתו הוא הצלע | |style="text-align:right;"|ואם ידעת האלכסון גדר חצי הכאתו הוא הצלע | ||
|- | |- | ||
| | | | ||
| − | === Rectangle === | + | === <span style=color:green>Rectangle</span> === |
| | | | ||
|- | |- | ||
| | | | ||
| − | : | + | :*A rectangle, whose sides are 10 in length and 5 in width, for instance. |
|style="text-align:right;"|אבל במרובע ארוך שיש לצלעיו י' באורך וה' ברוחב עד"מ | |style="text-align:right;"|אבל במרובע ארוך שיש לצלעיו י' באורך וה' ברוחב עד"מ | ||
|- | |- | ||
| | | | ||
| − | + | ::When knowing its two sides, multiply them by each other and [the product] is its area. | |
| − | :<math>\scriptstyle S_{rectangle}=side_1\times side_2</math> | + | ::<math>\scriptstyle{\color{OliveGreen}{S_{rectangle}=side_1\times side_2}}</math> |
|style="text-align:right;"|בידיעת ב' צלעיו תכה זה עם זה כאחת זהו שטחו | |style="text-align:right;"|בידיעת ב' צלעיו תכה זה עם זה כאחת זהו שטחו | ||
|- | |- | ||
| | | | ||
| − | + | ::When knowing its area and its one side, divide the area by the known side; the result is the unknown side. | |
| + | ::<math>\scriptstyle{\color{OliveGreen}{side_1=S_{rectangle}\div side_2}}</math> | ||
|style="text-align:right;"|ובידיעת שטחו וצלע א' תחלק השטח על הצלע הידוע ויצא הצלע הנעלם | |style="text-align:right;"|ובידיעת שטחו וצלע א' תחלק השטח על הצלע הידוע ויצא הצלע הנעלם | ||
|- | |- | ||
| | | | ||
| − | + | ::When knowing its two sides, multiply each by itself and the root of the sum of [their products] is the diagonal. | |
| + | ::<math>\scriptstyle{\color{OliveGreen}{d_{rectangle}=\sqrt{side_1^2+side_2^2}}}</math> | ||
|style="text-align:right;"|ובידיעת ב' צלעיו ותכה זה בפני עצמו וזה בפני עצמו וגדר מקובצם הוא האלכסון | |style="text-align:right;"|ובידיעת ב' צלעיו ותכה זה בפני עצמו וזה בפני עצמו וגדר מקובצם הוא האלכסון | ||
|- | |- | ||
| | | | ||
| − | + | ::When knowing the diagonal and its one side, subtract the product of the side [by itself] from the product of the diagonal [by itself], and the root of the remainder is the other side. | |
| + | ::<math>\scriptstyle{\color{OliveGreen}{side_1=\sqrt{d_{rectangle}^2-side_2^2}}}</math> | ||
|style="text-align:right;"|ובידיע' האלכסון וצלע א' תוציא הכאת הצלע מהכאת האלכסון וגדר הנשאר הוא הצלע האחר | |style="text-align:right;"|ובידיע' האלכסון וצלע א' תוציא הכאת הצלע מהכאת האלכסון וגדר הנשאר הוא הצלע האחר | ||
|- | |- | ||
| | | | ||
| − | === Triangle === | + | === On Measuring the Area of the Triangle === |
| − | + | |style="text-align:right;"|<big>בשבירת המשולש</big> | |
|- | |- | ||
| − | | | + | |There are many ways, but what seems to be more general is what is clear from the words of Euclid the Wise in one proposition and by applying this proposition we will generate the required by the help of God. |
| − | |style="text-align:right;"|יש דרכים רבים אבל מה שנ"ר יותר כולל הוא מה שנתבא' בכח דברי אק<span style=color:red>ד</span>ידס החכם מתוך הקדמה אח' ובקיום זאת ההקדמה נוליד המבוקש בע"ה | + | |style="width:45%; text-align:right;"|יש דרכים רבים אבל מה שנ"ר יותר כולל הוא מה שנתבא' בכח דברי אק<span style=color:red>ד</span>ידס החכם מתוך הקדמה אח' ובקיום זאת ההקדמה נוליד המבוקש בע"ה |
|- | |- | ||
| − | | | + | |It is that it is explained there that [the square] of the diagonal of the rectangle is equal to the sum of the squares of both sides when each is multiplied by itself. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{d_{rectangle}^2=side_1^2+side_2^2}}</math> | |
|style="text-align:right;"|וזה ששם נתבאר שקוטר המרובע ר"ל אלכסונו הוא כפל מרובע ב' הצלעים כשיוכה זה בפני עצמו וזה בפני עצמו הוא שוה להכאת האלכסון בעצמו | |style="text-align:right;"|וזה ששם נתבאר שקוטר המרובע ר"ל אלכסונו הוא כפל מרובע ב' הצלעים כשיוכה זה בפני עצמו וזה בפני עצמו הוא שוה להכאת האלכסון בעצמו | ||
|- | |- | ||
| − | | | + | |From this proposition it is clear to you that when there is a triangle of this form: |
| − | : | ||
|style="text-align:right;"|ובזה ההקדמה יתבאר לך כשיש משולש בצורה הזאת | |style="text-align:right;"|ובזה ההקדמה יתבאר לך כשיש משולש בצורה הזאת | ||
|- | |- | ||
| | | | ||
| − | :[[File:Mispar - triangle.png|thumb| | + | :[[File:Mispar - triangle.png|thumb|170px|left]] |
| − | |[[File:קצת מענייני חכמת המספר - משולש.png|thumb| | + | |[[File:קצת מענייני חכמת המספר - משולש.png|thumb|170px]] |
| + | |- | ||
| + | |We agree that it is equilateral and each side is 10. | ||
| + | |style="text-align:right;"|ונסכים שהוא שוה הג' הצלעות ושיש בכל צלע עשר | ||
| + | |- | ||
| + | |Finding its area is by that you divide the triangle in your thought into two identical triangles. | ||
| + | |style="text-align:right;"|הנה ידיעת שבריו הוא שתחלקהו במחשבתך לב' משולשים שוים | ||
| + | |- | ||
| + | |This is by drawing a straight line from vertex A and this line necessarily divides the line, i.e. line GB, into two halves. | ||
| + | |style="text-align:right;"|וזה שתרשום בזוית א' קו ישר ובהכרח יחלוק הקו ר"ל קו ג"ב לשני חצאין | ||
| + | |- | ||
| + | |So, we know that one side is 5 and the hypotenuse is ten as given. | ||
| + | |style="text-align:right;"|ובזה הרי ידענו שצלע אחד יש בו ה' והאלכסון עשר כפי מה שהונח | ||
| + | |- | ||
| + | |The extracting the other side, following from the proposition we have presented, is by that you subtract the product of the side [by itself] from the product of the hypotenuse [by itself] and the square of the remainder is the other side. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{side_1=\sqrt{d_{rectangle}^2-side_2^2}}}</math> | ||
| + | |style="text-align:right;"|ובהוצאת הצלע האח' יתחייב כפי ההקדמ' שהנחנו שתוציא הכאת הצלע מהכאת האלכסון וגדר הנשאר הוא הצלע האחר | ||
| + | |- | ||
| + | |The quality of the conclusion is clear since the product of the diagonal [by itself] is equal to the sum of the products of both sides, each by itself. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{d_{rectangle}^2=side_1^2+side_2^2}}</math> | ||
| + | |style="text-align:right;"|ואיכות החיוב מבואר כיון שהאלכסון בהכאתו שוה להכאת שני צלעיו כל אח' בפני עצמו | ||
| + | |- | ||
| + | |Therefore, it necessarily follows that when you subtract the product of one side [by itself] from [the product of] the diagonal [by itself], the root of the remainder is the [other side]. | ||
| + | |style="text-align:right;"|א"כ יתחייב בהכרח כשתוציא הכאת הצלע האח' מהאלכסון שגדר הנשאר הוא האלכסו‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We do so: we multiply 5 [by itself]; it is 25. |
| + | |style="text-align:right;"|ועשינו כן שהכינו הה' והיה כ"ה | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | ::We multiply 10 [by itself]; it is 100. |
| + | |style="text-align:right;"|והכינו הי' והיה ק‫' | ||
|- | |- | ||
| | | | ||
| − | + | ::We subtract 25 from 100; 75 remains. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|חסרנו הכ"ה מהק' ונשארו ע"ה |
|- | |- | ||
| | | | ||
| − | + | ::Its root is the other side. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וגדרם הוא הצלע האחר |
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{side=\sqrt{10^2-5^2}=\sqrt{100-25}=\sqrt{75}\approx8+\frac{2}{4}+\left(\frac{3}{4}\sdot\frac{1}{4}\right)}}</math> | + | ::We look for its root by approximation, as according the easier and more accurate method that I have presented to you in the previous chapter. We find that its root is 8 and 2-quarters and 3-quarters of one-quarter. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{side=\sqrt{10^2-5^2}=\sqrt{100-25}=\sqrt{75}\approx8+\frac{2}{4}+\left(\frac{3}{4}\sdot\frac{1}{4}\right)}}</math> |
| + | |style="text-align:right;"|בקשנו גדרם ע"ד הקירוב כמו שהודעתיך בשער הקידום הדרך היותו נקל כזו והיותר מדוייק ומצאנו ששרשם הוא הוא ח' וב' רביעיות וג' רביעיות רביעית | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{S_{triangle}=5\sdot\left[8+\frac{2}{4}+\left(\frac{3}{4}\sdot\frac{1}{4}\right)\right]=43+\frac{1}{4}+\left(\frac{3}{4}\sdot\frac{1}{4}\right)}}</math> | + | ::We multiply it by 5, which is the one side; the result is 43 integers, one-quarter, and 3-quarters of a quarter, and this is the area of the triangle. |
| + | ::<math>\scriptstyle{\color{blue}{S_{triangle}=5\sdot\left[8+\frac{2}{4}+\left(\frac{3}{4}\sdot\frac{1}{4}\right)\right]=43+\frac{1}{4}+\left(\frac{3}{4}\sdot\frac{1}{4}\right)}}</math> | ||
|style="text-align:right;"|הכינום עם ה' שהוא הצלע הא' ויצא מ"ג שלמים ורביעי' וג' רביעיות רביעית והוא שטח המשולש | |style="text-align:right;"|הכינום עם ה' שהוא הצלע הא' ויצא מ"ג שלמים ורביעי' וג' רביעיות רביעית והוא שטח המשולש | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|לפי שהאלכסון חולק המרובע לב' חצאים וחצי זהו חצי המשולש וחציו האחר הוא חצי המשולש ר"ל החלקים מהמשולש אשר חלקנו צלעו בזוית אח' ודי למבין | + | ::Since the diagonal divides the rectangle into two halves, their half is half the triangle and the other half is half the triangle, i.e. the parts of the triangle, whose side we have divided at one vertex. |
| + | |style="text-align:right;"|לפי שהאלכסון חולק המרובע לב' חצאים וחצי זהו חצי המשולש וחציו האחר הוא חצי המשולש ר"ל החלקים מהמשולש אשר חלקנו צלעו בזוית אח‫' | ||
| + | |- | ||
| + | |This is enough for the one who understands. | ||
| + | |style="text-align:right;"|ודי למבין | ||
|- | |- | ||
|} | |} | ||
| Line 2,916: | Line 3,564: | ||
|- | |- | ||
!Propositions that are of great need for arithmetic from the words of Euclid the wise in Book II and they are 9 propositions | !Propositions that are of great need for arithmetic from the words of Euclid the wise in Book II and they are 9 propositions | ||
| − | |style="width:45%; text-align:right;"|<big>הקדמות צריכין</big> צורך גדול למספר מדברי אקלידס החכם במא' הב' והם ט' הקדמות | + | |style="width:45%; text-align:right;"|<big>{{#annot:term|2632,2633|KZo5}}הקדמות{{#annotend:KZo5}} צריכין</big> צורך גדול למספר מדברי אקלידס החכם במא' הב' והם ט' הקדמות |
|- | |- | ||
| | | | ||
:1) [[ספר_היסודות_לאקלידס#Elements_II_2|<span style=color:blue>'''Euclid, Elements, Book II, propositions 2'''</span>]]: {{#annot:∑ₖ((∑ᵢaᵢ)·aₖ)=(∑ᵢaᵢ)²|250|4EHv}}If you divide any number into parts as you wish, [the sum of] the products of each of the parts by the whole number is equal to the square of the whole number. | :1) [[ספר_היסודות_לאקלידס#Elements_II_2|<span style=color:blue>'''Euclid, Elements, Book II, propositions 2'''</span>]]: {{#annot:∑ₖ((∑ᵢaᵢ)·aₖ)=(∑ᵢaᵢ)²|250|4EHv}}If you divide any number into parts as you wish, [the sum of] the products of each of the parts by the whole number is equal to the square of the whole number. | ||
| − | :<math>\scriptstyle\sum_{k=1}^n \left[\left(\sum_{i=1}^n a_i\right)\sdot a_k\right]=\left(\sum_{i=1}^n a_i\right)^2</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n \left[\left(\sum_{i=1}^n a_i\right)\sdot a_k\right]=\left(\sum_{i=1}^n a_i\right)^2}}</math> |
|style="text-align:right;"|<big>אחת</big> כל מספר שחלקת אותו לחלקים איך שרצית הנה הכאת כל אח' מהחלקי' בכל המספר השוה למרובע הכל{{#annotend:4EHv}} | |style="text-align:right;"|<big>אחת</big> כל מספר שחלקת אותו לחלקים איך שרצית הנה הכאת כל אח' מהחלקי' בכל המספר השוה למרובע הכל{{#annotend:4EHv}} | ||
|- | |- | ||
| Line 2,929: | Line 3,577: | ||
| | | | ||
:2) [[ספר_היסודות_לאקלידס#Elements_II_3|<span style=color:blue>'''Euclid, Elements, Book II, proposition 3'''</span>]]: {{#annot:(a+b)·b=(a·b)+b²|251|PVyt}}For any number divided into two parts as you wish, the product of the whole number by any of its two parts is equal to the product of the one part by the other plus the square of the part by which you multiplied the whole number. | :2) [[ספר_היסודות_לאקלידס#Elements_II_3|<span style=color:blue>'''Euclid, Elements, Book II, proposition 3'''</span>]]: {{#annot:(a+b)·b=(a·b)+b²|251|PVyt}}For any number divided into two parts as you wish, the product of the whole number by any of its two parts is equal to the product of the one part by the other plus the square of the part by which you multiplied the whole number. | ||
| − | :<math>\scriptstyle\left(a+b\right)\sdot b= \left(a\sdot b\right)+b^2</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\left(a+b\right)\sdot b=\left(a\sdot b\right)+b^2}}</math> |
|style="text-align:right;"|<big>ב</big> כל מספר שחלקת אותו לב' חלקים איך שקרה הנה הכאת המספר כלו עם כל אחד מב' חלקיו איזה שיהיה שוה להכאת החלק האחד עם האחר ולמרובע החלק אשר בו הכית כל המספר{{#annotend:PVyt}} | |style="text-align:right;"|<big>ב</big> כל מספר שחלקת אותו לב' חלקים איך שקרה הנה הכאת המספר כלו עם כל אחד מב' חלקיו איזה שיהיה שוה להכאת החלק האחד עם האחר ולמרובע החלק אשר בו הכית כל המספר{{#annotend:PVyt}} | ||
|- | |- | ||
| | | | ||
:Proof: the whole, when you multiply it by whichever of its parts it is as if you multiply this same part by itself and by the other part, since the whole consists of both of them. | :Proof: the whole, when you multiply it by whichever of its parts it is as if you multiply this same part by itself and by the other part, since the whole consists of both of them. | ||
| − | :<math>\scriptstyle\left(a+b\right)\sdot b=\left(a\sdot b\right)+\left(b\sdot b\right)</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\left(a+b\right)\sdot b=\left(a\sdot b\right)+\left(b\sdot b\right)}}</math> |
|style="text-align:right;"|‫<ref>35r</ref><big>מופתו</big> כי הכלל כשהכית אותו עם איזה חלק הוא כאלו הכית אותו החלק בעצמו ועם החלק האחר כיון שזה הכלל מורכב מהם | |style="text-align:right;"|‫<ref>35r</ref><big>מופתו</big> כי הכלל כשהכית אותו עם איזה חלק הוא כאלו הכית אותו החלק בעצמו ועם החלק האחר כיון שזה הכלל מורכב מהם | ||
|- | |- | ||
| Line 2,953: | Line 3,601: | ||
| | | | ||
:3) [[ספר_היסודות_לאקלידס#Elements_II_4|<span style=color:blue>'''Euclid, Elements, Book II, proposition 4'''</span>]]: {{#annot:(a+b)²=a²+b²+2(a·b)|252|v7Dx}}For any number divided into two parts as you wish, the square of the whole number is equal to [the sum of] the squares of the two parts and twice the product of the one part by the other. | :3) [[ספר_היסודות_לאקלידס#Elements_II_4|<span style=color:blue>'''Euclid, Elements, Book II, proposition 4'''</span>]]: {{#annot:(a+b)²=a²+b²+2(a·b)|252|v7Dx}}For any number divided into two parts as you wish, the square of the whole number is equal to [the sum of] the squares of the two parts and twice the product of the one part by the other. | ||
| − | :<math>\scriptstyle\left(a+b\right)^2=a^2+b^2+\left[2\sdot\left(a\sdot b\right)\right]</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\left(a+b\right)^2=a^2+b^2+\left[2\sdot\left(a\sdot b\right)\right]}}</math> |
|style="text-align:right;"|<big>ג</big> כל מספר שחלקת אותו לשני חלקים איך שקרה הנה מרובע כל המספר שוה לב' המרובעים ההווים מב' החלקים ולהכאת החלק האחד עם חבירו ב' פעמים{{#annotend:v7Dx}} | |style="text-align:right;"|<big>ג</big> כל מספר שחלקת אותו לשני חלקים איך שקרה הנה מרובע כל המספר שוה לב' המרובעים ההווים מב' החלקים ולהכאת החלק האחד עם חבירו ב' פעמים{{#annotend:v7Dx}} | ||
|- | |- | ||
| Line 2,960: | Line 3,608: | ||
|style="text-align:right;"|<big>מופתו</big> כי הכאת הכלל עם הכלל כאלו הכית הב' חלקים עם הכלל והכאת החלק והא' עם הכלל הוא כאלו הכית החלק בעצמו ועם החלק האח' וג"כ בחלק האח' שהכית אותו בכלל כאלו הכית בעצמו ועם החלק הא' כיון שאין בכלל זולת חלקיו והרי אלו החלקים הם חלקי זה המספר הגדול | |style="text-align:right;"|<big>מופתו</big> כי הכאת הכלל עם הכלל כאלו הכית הב' חלקים עם הכלל והכאת החלק והא' עם הכלל הוא כאלו הכית החלק בעצמו ועם החלק האח' וג"כ בחלק האח' שהכית אותו בכלל כאלו הכית בעצמו ועם החלק הא' כיון שאין בכלל זולת חלקיו והרי אלו החלקים הם חלקי זה המספר הגדול | ||
|} | |} | ||
| − | :<math>\scriptstyle\left(a+b\right)^2=\left[a\sdot\left(a+b\right)\right]+\left[b\sdot\left(a+b\right)\right]=\left[a^2+\left(a\sdot b\right)\right]+\left[b^2+\left(a\sdot b\right)\right]=a^2+b^2+\left[2\sdot\left(a\sdot b\right)\right]</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\left(a+b\right)^2=\left[a\sdot\left(a+b\right)\right]+\left[b\sdot\left(a+b\right)\right]=\left[a^2+\left(a\sdot b\right)\right]+\left[b^2+\left(a\sdot b\right)\right]=a^2+b^2+\left[2\sdot\left(a\sdot b\right)\right]}}</math> |
{| | {| | ||
|- | |- | ||
| Line 2,988: | Line 3,636: | ||
| | | | ||
:4) [[ספר_היסודות_לאקלידס#Elements_II_5|<span style=color:blue>'''Euclid, Elements, Book II, proposition 5'''</span>]]: {{#annot:(½(a+b))²=(a·b)+(b-½(a+b))²=(a·b)+(½(a+b)-a)²|253|9QNa}}For any number divided into two equal parts and into two unequal parts, [the sum of] the product of one of the unequal parts by the other and the square of the difference between the two parts, i.e. between the equal part [= the half of the whole number] and the unequal [part] is equal to the square of half the [whole] number. | :4) [[ספר_היסודות_לאקלידס#Elements_II_5|<span style=color:blue>'''Euclid, Elements, Book II, proposition 5'''</span>]]: {{#annot:(½(a+b))²=(a·b)+(b-½(a+b))²=(a·b)+(½(a+b)-a)²|253|9QNa}}For any number divided into two equal parts and into two unequal parts, [the sum of] the product of one of the unequal parts by the other and the square of the difference between the two parts, i.e. between the equal part [= the half of the whole number] and the unequal [part] is equal to the square of half the [whole] number. | ||
| − | :<math>\scriptstyle\left(a\sdot b\right)+\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]^2=\left[\frac{1}{2}\sdot\left(a+b\right)\right]^2</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\left(a\sdot b\right)+\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]^2=\left[\frac{1}{2}\sdot\left(a+b\right)\right]^2}}</math> |
|style="text-align:right;"|<big>ד</big> כל מספר כאשר תחלקהו לב' חלקים שוים ולב' חלקים בלתי שוים הנה הכאת החלק הא' עם חבירו מהחלקים הבלתי שוים ומרוב' מה שבין ב' חלקים ר"ל בין החלק השוה ובלתי שוה שוה למרובע חצי המספר{{#annotend:9QNa}} | |style="text-align:right;"|<big>ד</big> כל מספר כאשר תחלקהו לב' חלקים שוים ולב' חלקים בלתי שוים הנה הכאת החלק הא' עם חבירו מהחלקים הבלתי שוים ומרוב' מה שבין ב' חלקים ר"ל בין החלק השוה ובלתי שוה שוה למרובע חצי המספר{{#annotend:9QNa}} | ||
|- | |- | ||
| | | | ||
:Proof: [when] the number is divided into two unequal parts, the excess of its half over the smaller [part] is the excess of the greater [part] over the half. | :Proof: [when] the number is divided into two unequal parts, the excess of its half over the smaller [part] is the excess of the greater [part] over the half. | ||
| − | :<math>\scriptstyle\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a=b-\left[\frac{1}{2}\sdot\left(a+b\right)\right]</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a=b-\left[\frac{1}{2}\sdot\left(a+b\right)\right]}}</math> |
|style="width:45%; text-align:right;"|<big>מופתו</big> המספר שנחלק לב' חלקים בלתי שוים מה שנגרע מהמספר הקטן מהחצי הוא מיותר במספר הגדול על החצי | |style="width:45%; text-align:right;"|<big>מופתו</big> המספר שנחלק לב' חלקים בלתי שוים מה שנגרע מהמספר הקטן מהחצי הוא מיותר במספר הגדול על החצי | ||
|- | |- | ||
| Line 3,007: | Line 3,655: | ||
| | | | ||
:Hence, when we multiply the smaller number by the greater it is as if we multiply the smaller number by half the number plus the excess, since the excess and the half are the parts of the greater part. | :Hence, when we multiply the smaller number by the greater it is as if we multiply the smaller number by half the number plus the excess, since the excess and the half are the parts of the greater part. | ||
| − | :<math>\begin{align}\scriptstyle a\sdot b&\scriptstyle=a\sdot\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]+\left[b-\left[\frac{1}{2}\sdot\left(a+b\right)\right]\right]\right]\\&\scriptstyle=a\sdot\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]+\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]\right]\\\end{align}</math> | + | :<math>{\color{OliveGreen}{\begin{align}\scriptstyle a\sdot b&\scriptstyle=a\sdot\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]+\left[b-\left[\frac{1}{2}\sdot\left(a+b\right)\right]\right]\right]\\&\scriptstyle=a\sdot\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]+\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]\right]\\\end{align}}}</math> |
|style="text-align:right;"|וא"כ כשאנו מכים המספר הקטן בגדול הוא כאלו הכינו המספר הקטן בחצי המספר והתוספת כיון שהתוספת והחצי הם חלקי החלק הגדול | |style="text-align:right;"|וא"כ כשאנו מכים המספר הקטן בגדול הוא כאלו הכינו המספר הקטן בחצי המספר והתוספת כיון שהתוספת והחצי הם חלקי החלק הגדול | ||
|- | |- | ||
| | | | ||
:Also, the product of the half by itself is as we multiply the half by the smaller number plus the excess, since the excess and the smaller number are the parts of the half. | :Also, the product of the half by itself is as we multiply the half by the smaller number plus the excess, since the excess and the smaller number are the parts of the half. | ||
| − | :<math>\scriptstyle\left[\frac{1}{2}\sdot\left(a+b\right)\right]^2=\left[\frac{1}{2}\sdot\left(a+b\right)\right]\sdot\left[a+\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]\right]</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\left[\frac{1}{2}\sdot\left(a+b\right)\right]^2=\left[\frac{1}{2}\sdot\left(a+b\right)\right]\sdot\left[a+\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]\right]}}</math> |
|style="text-align:right;"|והכאת החצי עצמו הוא כאלו הכינו החצי עם המספר הקטן ועם התוספת כיון שהתוספת והמספר הקטן הם חלקי החצי | |style="text-align:right;"|והכאת החצי עצמו הוא כאלו הכינו החצי עם המספר הקטן ועם התוספת כיון שהתוספת והמספר הקטן הם חלקי החצי | ||
|- | |- | ||
| Line 3,019: | Line 3,667: | ||
|style="text-align:right;"|והוא מבואר שההכא' בין הכאת החצי בתוספת בין הכאת המספר הקטן בתוספת הוא הכאת התוספת בתוספת וע"כ כהכאת התוספת בעצמו | |style="text-align:right;"|והוא מבואר שההכא' בין הכאת החצי בתוספת בין הכאת המספר הקטן בתוספת הוא הכאת התוספת בתוספת וע"כ כהכאת התוספת בעצמו | ||
|} | |} | ||
| − | :<math>\scriptstyle\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]^2=\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]\sdot\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]\right]-\left[a\sdot\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]\right]</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]^2=\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]\sdot\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]\right]-\left[a\sdot\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]\right]}}</math> |
{| | {| | ||
|- | |- | ||
| | | | ||
:If we add it to the product of the smaller by the greater, it is the square of the half. | :If we add it to the product of the smaller by the greater, it is the square of the half. | ||
| − | :<math>\scriptstyle\left[\frac{1}{2}\sdot\left(a+b\right)\right]^2=\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]^2+\left(a\sdot b\right)</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\left[\frac{1}{2}\sdot\left(a+b\right)\right]^2=\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]^2+\left(a\sdot b\right)}}</math> |
|style="width:45%; text-align:right;"|ואם נוסיפהו על מרובע הקטן בגדול הוא מרוב' החצי בהכאת | |style="width:45%; text-align:right;"|ואם נוסיפהו על מרובע הקטן בגדול הוא מרוב' החצי בהכאת | ||
|- | |- | ||
| Line 3,044: | Line 3,692: | ||
| | | | ||
:5) [[ספר_היסודות_לאקלידס#Elements_II_6|<span style=color:blue>'''Euclid, Elements, Book II, proposition 6'''</span>]]: {{#annot:(a+b)·b+(½a)²=(½a+b)²|254|nmaV}}If we divide any number into half and add to it another number, [the sum of] the product of the whole number plus the additional [number] by the additional [number] and the square of half the number is equal to the square of half the number and the additional [number] together. | :5) [[ספר_היסודות_לאקלידס#Elements_II_6|<span style=color:blue>'''Euclid, Elements, Book II, proposition 6'''</span>]]: {{#annot:(a+b)·b+(½a)²=(½a+b)²|254|nmaV}}If we divide any number into half and add to it another number, [the sum of] the product of the whole number plus the additional [number] by the additional [number] and the square of half the number is equal to the square of half the number and the additional [number] together. | ||
| − | :<math>\scriptstyle\left[\left(a+b\right)\sdot b\right]+\left(\frac{1}{2}\sdot a\right)^2=\left[\left(\frac{1}{2}\sdot a\right)+b\right]^2</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\left[\left(a+b\right)\sdot b\right]+\left(\frac{1}{2}\sdot a\right)^2=\left[\left(\frac{1}{2}\sdot a\right)+b\right]^2}}</math> |
|style="text-align:right;"|<big>ה</big> כל מספר כאשר חלקנו אותו לחצי והוספת עליו מספר אחר הנה הכאת המספר כלו מחובר עם התוספ' בתוספת ומרובע חצי המספר שוה למרובע חצי המספר והתוספת ביחד{{#annotend:nmaV}} | |style="text-align:right;"|<big>ה</big> כל מספר כאשר חלקנו אותו לחצי והוספת עליו מספר אחר הנה הכאת המספר כלו מחובר עם התוספ' בתוספת ומרובע חצי המספר שוה למרובע חצי המספר והתוספת ביחד{{#annotend:nmaV}} | ||
|- | |- | ||
| | | | ||
:Proof: when we add any number to the half, the product of the excess by the half and the product of the excess by the half plus the excess are added to the square of the half. | :Proof: when we add any number to the half, the product of the excess by the half and the product of the excess by the half plus the excess are added to the square of the half. | ||
| − | :<math>\scriptstyle\left[\left(\frac{1}{2}\sdot a\right)+b\right]^2=\left(\frac{1}{2}\sdot a\right)^2+\left[b\sdot\left(\frac{1}{2}\sdot a\right)\right]+\left[b\sdot\left[\left(\frac{1}{2}\sdot a\right)+b\right]\right]</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\left[\left(\frac{1}{2}\sdot a\right)+b\right]^2=\left(\frac{1}{2}\sdot a\right)^2+\left[b\sdot\left(\frac{1}{2}\sdot a\right)\right]+\left[b\sdot\left[\left(\frac{1}{2}\sdot a\right)+b\right]\right]}}</math> |
|style="width:45%; text-align:right;"|<big>מופתו</big> כי כשהוספנו על החצי מספר מה הנה יתוסף על מרובע החצי הכאת התוספת בחצי והכאת התוס' בחצי מחובר עם התוספת | |style="width:45%; text-align:right;"|<big>מופתו</big> כי כשהוספנו על החצי מספר מה הנה יתוסף על מרובע החצי הכאת התוספת בחצי והכאת התוס' בחצי מחובר עם התוספת | ||
|- | |- | ||
| Line 3,059: | Line 3,707: | ||
| | | | ||
:This itself is what we do when we add the excess to the whole number, since the number is [as] its half and the excess [is] what we add to it, and the product of the excess by the half and by the sum of the half and the excess [is the same as] its product by the whole number together with the excess. Understand this. | :This itself is what we do when we add the excess to the whole number, since the number is [as] its half and the excess [is] what we add to it, and the product of the excess by the half and by the sum of the half and the excess [is the same as] its product by the whole number together with the excess. Understand this. | ||
| − | :<math>\scriptstyle b\sdot\left[\left(\frac{1}{2}\sdot a\right)+\left(\frac{1}{2}\sdot a\right)+b\right]=b\sdot\left(a+b\right)</math> | + | :<math>\scriptstyle{\color{OliveGreen}{b\sdot\left[\left(\frac{1}{2}\sdot a\right)+\left(\frac{1}{2}\sdot a\right)+b\right]=b\sdot\left(a+b\right)}}</math> |
|style="text-align:right;"|וזהו בעצמו מה שאנו עושין כשאנו מוסיפין התוספת במספר כלו לפי שהמספ' חציו ועם התוספת שאנו מוסיפין עליו ומה להכאת התוספת עם החצי ואע"כ עם החצי והתוספת מחובר או הכאתו עם כל המספר והתוספת ביחד והבן | |style="text-align:right;"|וזהו בעצמו מה שאנו עושין כשאנו מוסיפין התוספת במספר כלו לפי שהמספ' חציו ועם התוספת שאנו מוסיפין עליו ומה להכאת התוספת עם החצי ואע"כ עם החצי והתוספת מחובר או הכאתו עם כל המספר והתוספת ביחד והבן | ||
|- | |- | ||
| | | | ||
:6) [[ספר_היסודות_לאקלידס#Elements_II_7|<span style=color:blue>'''Euclid, Elements, Book II, proposition 7'''</span>]]: {{#annot:(a+b)²+a²=(2·(a+b)·a)+b²|255|DEFA}}For any number divided into two parts, the sum of the square of the whole number and the square of one of the parts is equal to twice the product of this part by the whole number plus the product of the other part by itself<br> | :6) [[ספר_היסודות_לאקלידס#Elements_II_7|<span style=color:blue>'''Euclid, Elements, Book II, proposition 7'''</span>]]: {{#annot:(a+b)²+a²=(2·(a+b)·a)+b²|255|DEFA}}For any number divided into two parts, the sum of the square of the whole number and the square of one of the parts is equal to twice the product of this part by the whole number plus the product of the other part by itself<br> | ||
| − | :<math>\scriptstyle\left(a+b\right)^2+a^2=2\sdot\left[\left(a+b\right)\sdot a\right]+b^2</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\left(a+b\right)^2+a^2=2\sdot\left[\left(a+b\right)\sdot a\right]+b^2}}</math> |
|style="text-align:right;"|<big>ו</big> כל מספר שתחלקהו בב' חלקים איך שקרה המרובע ההווה מהמספר כלו והמרובע ההווה מא' מב' אלו החלקים כאשר התקבצו שוה להכאת החלק הנזכ' עם המספר כלו ולהכאת החלק הב' הנשאר בעצמו{{#annotend:DEFA}} | |style="text-align:right;"|<big>ו</big> כל מספר שתחלקהו בב' חלקים איך שקרה המרובע ההווה מהמספר כלו והמרובע ההווה מא' מב' אלו החלקים כאשר התקבצו שוה להכאת החלק הנזכ' עם המספר כלו ולהכאת החלק הב' הנשאר בעצמו{{#annotend:DEFA}} | ||
|- | |- | ||
| | | | ||
:Proof: it has already proven in proposition 3 that the product of the whole by itself is [as the sum of] the products of each of its parts by itself and twice the product of one part by the other. | :Proof: it has already proven in proposition 3 that the product of the whole by itself is [as the sum of] the products of each of its parts by itself and twice the product of one part by the other. | ||
| − | :<math>\scriptstyle\left(a+b\right)^2=a^2+b^2+\left[2\sdot\left(a+b\right)\right]</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\left(a+b\right)^2=a^2+b^2+\left[2\sdot\left(a+b\right)\right]}}</math> |
|style="text-align:right;"|<big>מופתו</big> כבר התבאר בהקדמה ג' שהכאת הכלל בעצמו הוא הכאת חלקיו כל אחת בפני עצמו והכאת החלק הא' עם חבירו ב' פעמים | |style="text-align:right;"|<big>מופתו</big> כבר התבאר בהקדמה ג' שהכאת הכלל בעצמו הוא הכאת חלקיו כל אחת בפני עצמו והכאת החלק הא' עם חבירו ב' פעמים | ||
|- | |- | ||
| Line 3,088: | Line 3,736: | ||
|style="text-align:right;"|הרי נותר הנה הכאת חלק אחד בעצמו פעם אח' אנו מוסיפין זה על מרובע הכלל כדי שיהיה שוה | |style="text-align:right;"|הרי נותר הנה הכאת חלק אחד בעצמו פעם אח' אנו מוסיפין זה על מרובע הכלל כדי שיהיה שוה | ||
|} | |} | ||
| − | :<math>\ | + | :<math>\scriptstyle{\color{OliveGreen}{2\sdot\left[\left(a+b\right)\sdot a\right]+b^2=2a^2+\left[2\sdot\left(a\sdot b\right)\right]+b^2=a^2+\left[a^2+\left[2\sdot\left(a\sdot b\right)\right]+b^2\right]=\left(a+b\right)^2+a^2}}</math> |
{| | {| | ||
|- | |- | ||
| Line 3,110: | Line 3,758: | ||
| | | | ||
:7) [[ספר_היסודות_לאקלידס#Elements_II_8|<span style=color:blue>'''Euclid, Elements, Book II, proposition 8'''</span>]]: {{#annot:(4·(a+b)·a)+b²=((a+b)+a)²|256|R68t}}For any number divided into two parts as you wish, if you multiply the whole number by one of the parts four times, the sum of the product with the square of the other part is equal to the [square] of the whole number plus the one part | :7) [[ספר_היסודות_לאקלידס#Elements_II_8|<span style=color:blue>'''Euclid, Elements, Book II, proposition 8'''</span>]]: {{#annot:(4·(a+b)·a)+b²=((a+b)+a)²|256|R68t}}For any number divided into two parts as you wish, if you multiply the whole number by one of the parts four times, the sum of the product with the square of the other part is equal to the [square] of the whole number plus the one part | ||
| − | :<math>\ | + | :<math>\scriptstyle{\color{OliveGreen}{4\sdot\left[\left(a+b\right)\sdot a\right]+b^2=\left[\left(a+b\right)+a\right]^2}}</math> |
|style="text-align:right;"|<big>ז</big> כל מספר שחלקת אותו לב' חלקים איך שקרה אם הכית המספר כלו עם חלק אח' מהם ד' פעמים וקבצת הכל עם מרובע החלק הב' הנשאר היה שוה להכאת מספרו החלק הנזכר כאשר תחברם יחד{{#annotend:R68t}} | |style="text-align:right;"|<big>ז</big> כל מספר שחלקת אותו לב' חלקים איך שקרה אם הכית המספר כלו עם חלק אח' מהם ד' פעמים וקבצת הכל עם מרובע החלק הב' הנשאר היה שוה להכאת מספרו החלק הנזכר כאשר תחברם יחד{{#annotend:R68t}} | ||
|- | |- | ||
| Line 3,119: | Line 3,767: | ||
| | | | ||
*The first: for any number divided into two parts as you wish, [the sum of] the product of one part by the whole number and the [square] of the other part is equal to the sum of the product of the other part by the whole number and the [square] of the one part. | *The first: for any number divided into two parts as you wish, [the sum of] the product of one part by the whole number and the [square] of the other part is equal to the sum of the product of the other part by the whole number and the [square] of the one part. | ||
| − | :<math>\scriptstyle\left[a\sdot\left(a+b\right)\right]+b^2=\left[b\sdot\left(a+b\right)\right]+a^2</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\left[a\sdot\left(a+b\right)\right]+b^2=\left[b\sdot\left(a+b\right)\right]+a^2}}</math> |
|style="text-align:right;"|אחת כל מספר שחלקת אותו לב' חלקים כי איך שקרה הנה הכאת החלק עם כל הכלל והחלק הנשאר בעצמו כאשר תקבצם שוה להכאת החלק הנשאר בכלל והכאת החלק הא' בעצמו כאשר תקבצם | |style="text-align:right;"|אחת כל מספר שחלקת אותו לב' חלקים כי איך שקרה הנה הכאת החלק עם כל הכלל והחלק הנשאר בעצמו כאשר תקבצם שוה להכאת החלק הנשאר בכלל והכאת החלק הא' בעצמו כאשר תקבצם | ||
|- | |- | ||
| Line 3,133: | Line 3,781: | ||
| | | | ||
:This is because the product of the part by the whole is as if you multiply [the part] by itself and with the other part that remains, for the whole is nothing but its parts. | :This is because the product of the part by the whole is as if you multiply [the part] by itself and with the other part that remains, for the whole is nothing but its parts. | ||
| − | :<math>\scriptstyle a\sdot\left(a+b\right)=a^2+\left(a\sdot b\right)</math> | + | :<math>\scriptstyle{\color{OliveGreen}{a\sdot\left(a+b\right)=a^2+\left(a\sdot b\right)}}</math> |
|style="text-align:right;"|וזה כי הכאת החלק בכלל הוא כאלו הכית אותו בעצמו ועם החלק האחד הנשאר כי אין הכלל זולת חלקיו | |style="text-align:right;"|וזה כי הכאת החלק בכלל הוא כאלו הכית אותו בעצמו ועם החלק האחד הנשאר כי אין הכלל זולת חלקיו | ||
|- | |- | ||
| | | | ||
:Then, when we multiply the part that remains by itself, we carry out three products: the product of each part by itself and the product of one part by the other. | :Then, when we multiply the part that remains by itself, we carry out three products: the product of each part by itself and the product of one part by the other. | ||
| − | :<math>\scriptstyle\left[a\sdot\left(a+b\right)\right]+b^2=a^2+\left(a\sdot b\right)+b^2</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\left[a\sdot\left(a+b\right)\right]+b^2=a^2+\left(a\sdot b\right)+b^2}}</math> |
|style="text-align:right;"|ואח"כ כשאנו מכין החלק הנשא' בעצמו הרי עשינו ג' הכאות הכאת כל חלק בפני עצמו והכאת החלק הא' באחר | |style="text-align:right;"|ואח"כ כשאנו מכין החלק הנשא' בעצמו הרי עשינו ג' הכאות הכאת כל חלק בפני עצמו והכאת החלק הא' באחר | ||
|- | |- | ||
| Line 3,161: | Line 3,809: | ||
| | | | ||
*The second proposition: for any number whose square is known and we want to know the square of another number, we add [to the known square] twice the product of difference [between the two numbers] by the number [whose square is] known plus [the square] of the difference. | *The second proposition: for any number whose square is known and we want to know the square of another number, we add [to the known square] twice the product of difference [between the two numbers] by the number [whose square is] known plus [the square] of the difference. | ||
| − | :<math>\scriptstyle b^2=a^2+2\sdot\left[a\sdot\left(b-a\right)\right]+\left(b-a\right)^2</math> | + | :<math>\scriptstyle{\color{OliveGreen}{b^2=a^2+2\sdot\left[a\sdot\left(b-a\right)\right]+\left(b-a\right)^2}}</math> |
|style="text-align:right;"|ההקדמה הב' כי כל מספר שנודע לנו מרובעו ונרצה לידע מרובע מספר אחר הנה נוסף עליו הכאת התוספת במספ' הנודע ב' פעמים והתוספת בעצמו פעם אחת | |style="text-align:right;"|ההקדמה הב' כי כל מספר שנודע לנו מרובעו ונרצה לידע מרובע מספר אחר הנה נוסף עליו הכאת התוספת במספ' הנודע ב' פעמים והתוספת בעצמו פעם אחת | ||
|- | |- | ||
| Line 3,197: | Line 3,845: | ||
| | | | ||
:By this, the words of the mentioned proposition are understood: when you multiply the part four times by the whole, then the remaining part by itself, [the sum of the products] is equal to the [square] of the [whole] number plus the addition. | :By this, the words of the mentioned proposition are understood: when you multiply the part four times by the whole, then the remaining part by itself, [the sum of the products] is equal to the [square] of the [whole] number plus the addition. | ||
| − | :<math>\ | + | :<math>\scriptstyle{\color{OliveGreen}{4\sdot\left[\left(a+b\right)\sdot a\right]+b^2=\left[\left(a+b\right)+a\right]^2}}</math> |
|style="text-align:right;"|בזה יובנו דברי ההקדמה הנזכ' הנה כשהכית החלק בכלל ד' פעמים ואח"כ החלק הנשאר בעצמו שוה להכאת המספר והתוספת כשיחוברו | |style="text-align:right;"|בזה יובנו דברי ההקדמה הנזכ' הנה כשהכית החלק בכלל ד' פעמים ואח"כ החלק הנשאר בעצמו שוה להכאת המספר והתוספת כשיחוברו | ||
|- | |- | ||
| | | | ||
::This is because [the sum of] the product of the remaining part by itself, when it is added to the product of the one part by the whole, is the same as when we switch [the parts] and proceed vice versa. | ::This is because [the sum of] the product of the remaining part by itself, when it is added to the product of the one part by the whole, is the same as when we switch [the parts] and proceed vice versa. | ||
| − | ::<math>\scriptstyle b^2+\left[a\sdot\left(a+b\right)\right]=a^2+\left[b\sdot\left(a+b\right)\right]</math> | + | ::<math>\scriptstyle{\color{OliveGreen}{b^2+\left[a\sdot\left(a+b\right)\right]=a^2+\left[b\sdot\left(a+b\right)\right]}}</math> |
|style="text-align:right;"|וזה כי הכאת החלק הנשאר בעצמו כאשר יחובר אל הכאת החלק האח' בכלל שוה כאשר המירונו שנעשה ההפך | |style="text-align:right;"|וזה כי הכאת החלק הנשאר בעצמו כאשר יחובר אל הכאת החלק האח' בכלל שוה כאשר המירונו שנעשה ההפך | ||
|- | |- | ||
| Line 3,209: | Line 3,857: | ||
|style="text-align:right;"|וא"כ הד' פעמים שהכינו בכלל נקח אחד מהם וישארו ג' ותחבר זאת ההכאת אל הכאת החלק הנשאר בעצמו הוא כאלו החלק האח' בכלל וזה החלק שאנו מכין אותו בכלל נכה אותו בעצמו וא"כ יש לו ג' הכאות החלק בכלל והכאת אחרת של החלק האחר בכלל והכאת החלק האח' בעצמו | |style="text-align:right;"|וא"כ הד' פעמים שהכינו בכלל נקח אחד מהם וישארו ג' ותחבר זאת ההכאת אל הכאת החלק הנשאר בעצמו הוא כאלו החלק האח' בכלל וזה החלק שאנו מכין אותו בכלל נכה אותו בעצמו וא"כ יש לו ג' הכאות החלק בכלל והכאת אחרת של החלק האחר בכלל והכאת החלק האח' בעצמו | ||
|} | |} | ||
| − | :<math>\ | + | :<math>\scriptstyle{\color{OliveGreen}{4\sdot\left[\left(a+b\right)\sdot a\right]+b^2=3\sdot\left[a\sdot\left(a+b\right)\right]+\left[a\sdot\left(a+b\right)\right]+b^2=3\sdot\left[a\sdot\left(a+b\right)\right]+\left[b\sdot\left(a+b\right)\right]+a^2}}</math> |
{| | {| | ||
|- | |- | ||
| | | | ||
::We have already explained in the first proposition [Elements, Book II, propositions 1-2] that the product of the dividend number by itself is as if we multiply it by each of its parts. | ::We have already explained in the first proposition [Elements, Book II, propositions 1-2] that the product of the dividend number by itself is as if we multiply it by each of its parts. | ||
| − | ::<math>\scriptstyle\sum_{k=1}^n \left[\left(\sum_{i=1}^n a_i\right)\sdot a_k\right]=\left(\sum_{i=1}^n a_i\right)^2</math> | + | ::<math>\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n \left[\left(\sum_{i=1}^n a_i\right)\sdot a_k\right]=\left(\sum_{i=1}^n a_i\right)^2}}</math> |
|style="width:45%; text-align:right;"|וכבר ביארנו בהקדמה הראשונה שהכאת המספר שנתחלק בעצמו כאלו הכינו אותו עם כל חלקיו | |style="width:45%; text-align:right;"|וכבר ביארנו בהקדמה הראשונה שהכאת המספר שנתחלק בעצמו כאלו הכינו אותו עם כל חלקיו | ||
|- | |- | ||
| Line 3,222: | Line 3,870: | ||
|- | |- | ||
|} | |} | ||
| − | :<math>\begin{align}\scriptstyle4\sdot\left[\left(a+b\right)\sdot a\right]+b^2&\scriptstyle=3\sdot\left[a\sdot\left(a+b\right)\right]+\left[a\sdot\left(a+b\right)\right]+b^2=3\sdot\left[a\sdot\left(a+b\right)\right]+\left[b\sdot\left(a+b\right)\right]+a^2\\&\scriptstyle=2\sdot\left[a\sdot\left(a+b\right)\right]+\left[a\sdot\left(a+b\right)\right]+\left[b\sdot\left(a+b\right)\right]+a^2=2\sdot\left[a\sdot\left(a+b\right)\right]+\left(a+b\right)^2+a^2\\&\scriptstyle=\left[\left(a+b\right)+a\right]^2\\\end{align}</math> | + | :<math>{\color{OliveGreen}{\begin{align}\scriptstyle4\sdot\left[\left(a+b\right)\sdot a\right]+b^2&\scriptstyle=3\sdot\left[a\sdot\left(a+b\right)\right]+\left[a\sdot\left(a+b\right)\right]+b^2=3\sdot\left[a\sdot\left(a+b\right)\right]+\left[b\sdot\left(a+b\right)\right]+a^2\\&\scriptstyle=2\sdot\left[a\sdot\left(a+b\right)\right]+\left[a\sdot\left(a+b\right)\right]+\left[b\sdot\left(a+b\right)\right]+a^2=2\sdot\left[a\sdot\left(a+b\right)\right]+\left(a+b\right)^2+a^2\\&\scriptstyle=\left[\left(a+b\right)+a\right]^2\\\end{align}}}</math> |
{| | {| | ||
|- | |- | ||
| Line 3,231: | Line 3,879: | ||
|- | |- | ||
|1) For parts whose products are equal, the ratio of a [product] to a [product] is equal to the ratio of a [part] to a [apr]. | |1) For parts whose products are equal, the ratio of a [product] to a [product] is equal to the ratio of a [part] to a [apr]. | ||
| − | :<math>\scriptstyle\left(a\sdot b\right):\left(a\sdot c\right)=b:c</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\left(a\sdot b\right):\left(a\sdot c\right)=b:c}}</math> |
|style="text-align:right;"|החלקים אשר כפליהם שוים הנה ייחס קצתם אל קצתם כיחס חלק קצתם אל קצת | |style="text-align:right;"|החלקים אשר כפליהם שוים הנה ייחס קצתם אל קצתם כיחס חלק קצתם אל קצת | ||
|- | |- | ||
|Hence, it follows from this that, for any fraction, when its numerator and its denominator are multiplied the same number of times, [the result] is the same original fraction. | |Hence, it follows from this that, for any fraction, when its numerator and its denominator are multiplied the same number of times, [the result] is the same original fraction. | ||
| − | :<math>\scriptstyle\frac{a\times c}{b\times c}=\frac{a}{b}</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a\times c}{b\times c}=\frac{a}{b}}}</math> |
|style="text-align:right;"|וא"כ יתחייב מזה שכל חלק מהשברים כאשר יכפל כמותו ואיכותו שוה הפעמי' הנה הוא השבר הראשון בעינו | |style="text-align:right;"|וא"כ יתחייב מזה שכל חלק מהשברים כאשר יכפל כמותו ואיכותו שוה הפעמי' הנה הוא השבר הראשון בעינו | ||
|- | |- | ||
|Also, if you divide its numerator and its denominator into the same parts, then relate the quotient of the numerator to the quotient of the denominator, [the result] is the same original fraction. | |Also, if you divide its numerator and its denominator into the same parts, then relate the quotient of the numerator to the quotient of the denominator, [the result] is the same original fraction. | ||
| − | :<math>\scriptstyle\frac{a\div c}{b\div c}=\frac{a}{b}</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a\div c}{b\div c}=\frac{a}{b}}}</math> |
|style="text-align:right;"|וכן אם תחלק כמותו ואכותו לחלקים שוים ותיחס חלק הכמות עם חלק האיכות הוא השבר הראשון בעינו | |style="text-align:right;"|וכן אם תחלק כמותו ואכותו לחלקים שוים ותיחס חלק הכמות עם חלק האיכות הוא השבר הראשון בעינו | ||
|- | |- | ||
| Line 3,246: | Line 3,894: | ||
|- | |- | ||
|3) For any number multiplied by any number, the ratio of the multiplied number to the product is equal to the ratio of one to the multiplier. | |3) For any number multiplied by any number, the ratio of the multiplied number to the product is equal to the ratio of one to the multiplier. | ||
| − | :<math>\scriptstyle a:\left(a\sdot b\right)=1:b</math> | + | :<math>\scriptstyle{\color{OliveGreen}{a:\left(a\sdot b\right)=1:b}}</math> |
|style="text-align:right;"|<big>ג</big> שכל מספר נכפל בכפלים כמ' ש<sup>י</sup>היו המספר הנכפל יחסו אל המספר העולה מכפליו כערך הא' אל הכפלים שבו נכפל | |style="text-align:right;"|<big>ג</big> שכל מספר נכפל בכפלים כמ' ש<sup>י</sup>היו המספר הנכפל יחסו אל המספר העולה מכפליו כערך הא' אל הכפלים שבו נכפל | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Such as the number 2, when it is multiplied by 3 and the product is 6. Its ratio to 6 is as the ratio of one to three, which is a third. |
| + | ::<math>\scriptstyle{\color{blue}{2:\left(2\sdot3\right)=2:6=1:3=\frac{1}{3}}}</math> | ||
|style="text-align:right;"|כמו מספר הב' שהוכה בג' ועלה ו' יחסו אל הו' כיחס האחד אל שלשה שהוא שליש | |style="text-align:right;"|כמו מספר הב' שהוכה בג' ועלה ו' יחסו אל הו' כיחס האחד אל שלשה שהוא שליש | ||
|- | |- | ||
| − | |4) <math>\scriptstyle\frac{1}{c}\sdot\frac{a}{b}=\frac{\frac{1}{c}\sdot a}{b}</math> | + | |4) When you want to take any part of some fractions, we take the required part of the numerator of the given fractions and relate it to [their] denominator. |
| + | :<math>\scriptstyle{\color{OliveGreen}{\frac{1}{c}\sdot\frac{a}{b}=\frac{\frac{1}{c}\sdot a}{b}}}</math> | ||
|style="text-align:right;"|<big>ד</big> כאשר תרצה לקחת מהשברים מה חלק מה הנה נקח מכמות השברים המונחים לפני החלק הדרוש וניחסהו אל האיכות | |style="text-align:right;"|<big>ד</big> כאשר תרצה לקחת מהשברים מה חלק מה הנה נקח מכמות השברים המונחים לפני החלק הדרוש וניחסהו אל האיכות | ||
|- | |- | ||
| | | | ||
| − | : | + | ::If you wish to take a third of 9-tenths, take a third of 9, which is 3, relate it to the denominator, which is ten; it is 3-tenths. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot\frac{9}{10}=\frac{\frac{1}{3}\sdot9}{10}=3:10=\frac{3}{10}}}</math> | ||
|style="text-align:right;"|אם רצית שליש הט' עשיריות קח שליש הט' והם ג' יחסים אל האיכו' והם עשיריות יהיו ג' עשיריות | |style="text-align:right;"|אם רצית שליש הט' עשיריות קח שליש הט' והם ג' יחסים אל האיכו' והם עשיריות יהיו ג' עשיריות | ||
|- | |- | ||
| − | |5) <math>\scriptstyle c\sdot\frac{a}{b}=\frac{c\sdot a}{b}</math> | + | |5) When you want to multiply given fractions by any multiples, we multiply the numerator and relate it to the denominator. |
| + | :<math>\scriptstyle{\color{OliveGreen}{c\sdot\frac{a}{b}=\frac{c\sdot a}{b}}}</math> | ||
|style="text-align:right;"|<big>ה</big> שכאשר תרצה לכפול שברים מונחים באיזה כפלים שיהיו נכפול הכמו' וניחסהו אל האיכות | |style="text-align:right;"|<big>ה</big> שכאשר תרצה לכפול שברים מונחים באיזה כפלים שיהיו נכפול הכמו' וניחסהו אל האיכות | ||
|- | |- | ||
| | | | ||
| − | : | + | ::You wish to multiply 2-ninths by 3. Multiply 2 by 3; it is 6. Relate it to the denominator; it is 6-ninths. |
| + | ::<math>\scriptstyle{\color{blue}{3\sdot\frac{2}{9}=\frac{3\sdot2}{9}=6:9=\frac{6}{9}}}</math> | ||
|style="text-align:right;"|רצית להכות ב' תשיעיות בג' תכה הב' בג' הם ו' יחסם אל האיכות יהיו ו' תשיעיות | |style="text-align:right;"|רצית להכות ב' תשיעיות בג' תכה הב' בג' הם ו' יחסם אל האיכות יהיו ו' תשיעיות | ||
|- | |- | ||
|6) [[ספר_היסודות_לאקלידס#Elements_VII_17|<span style=color:blue>'''Euclid, Elements, Book VII, proposition 17'''</span>]]: For any number multiplied by two numbers, the ratio of the two products one to the other is equal to the ratio of the two numbers one to the other. | |6) [[ספר_היסודות_לאקלידס#Elements_VII_17|<span style=color:blue>'''Euclid, Elements, Book VII, proposition 17'''</span>]]: For any number multiplied by two numbers, the ratio of the two products one to the other is equal to the ratio of the two numbers one to the other. | ||
| − | :<math>\scriptstyle\left(a\sdot b\right):\left(a\sdot c\right)=b:c</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\left(a\sdot b\right):\left(a\sdot c\right)=b:c}}</math> |
|style="text-align:right;"|‫<ref>36r</ref><big>ו</big> כל מספר יוכו בו ב' מספרים יחס השטח האחד אל האח' כיחס הב' מספרים בעצמם הא' מהם אל הב‫' | |style="text-align:right;"|‫<ref>36r</ref><big>ו</big> כל מספר יוכו בו ב' מספרים יחס השטח האחד אל האח' כיחס הב' מספרים בעצמם הא' מהם אל הב‫' | ||
|- | |- | ||
|7) [[ספר_היסודות_לאקלידס#Elements_VII_19|<span style=color:blue>'''Euclid, Elements, Book VII, proposition 19'''</span>]]: For any four proportional numbers, the product of the first by the fourth is equal to the product of the second by the third. | |7) [[ספר_היסודות_לאקלידס#Elements_VII_19|<span style=color:blue>'''Euclid, Elements, Book VII, proposition 19'''</span>]]: For any four proportional numbers, the product of the first by the fourth is equal to the product of the second by the third. | ||
| − | :<math>\scriptstyle a_1:a_2=a_3:a_4\longrightarrow a_1\sdot a_4=a_2\sdot a_3</math> | + | :<math>\scriptstyle{\color{OliveGreen}{a_1:a_2=a_3:a_4\longrightarrow a_1\sdot a_4=a_2\sdot a_3}}</math> |
|style="text-align:right;"|<big>ז</big> ד' מספרים מתיחסם הכאת הראשון בד' שוה להכאת הב' בג‫' | |style="text-align:right;"|<big>ז</big> ד' מספרים מתיחסם הכאת הראשון בד' שוה להכאת הב' בג‫' | ||
|- | |- | ||
|8) <span style=color:blue>'''Euclid, Elements, Book II, proposition 1'''</span>: For any number multiplied by any number, their product is equal to [the sum of] the products of the multiplied number by each part of the multiplier number, divided into parts as you wish. | |8) <span style=color:blue>'''Euclid, Elements, Book II, proposition 1'''</span>: For any number multiplied by any number, their product is equal to [the sum of] the products of the multiplied number by each part of the multiplier number, divided into parts as you wish. | ||
| − | :<math>\scriptstyle a\sdot\left(\sum_{k=1}^n b_k\right)=\sum_{k=1}^n \left(a\sdot b_k\right)</math> | + | :<math>\scriptstyle{\color{OliveGreen}{a\sdot\left(\sum_{k=1}^n b_k\right)=\sum_{k=1}^n \left(a\sdot b_k\right)}}</math> |
|style="text-align:right;"|<big>ח</big> כל מספר יוכה עם מספר מה איזה מספר היה הנה העולה מהם שוה לעולה מהכאת המספר המוכה עם כל אחד מחלקי המספר המכה על איזה חלקים שיחלק | |style="text-align:right;"|<big>ח</big> כל מספר יוכה עם מספר מה איזה מספר היה הנה העולה מהם שוה לעולה מהכאת המספר המוכה עם כל אחד מחלקי המספר המכה על איזה חלקים שיחלק | ||
|- | |- | ||
| Line 3,283: | Line 3,936: | ||
|- | |- | ||
|Reminder: the mean with regard to itself is not opposing, but with regard to its quality it is opposing to each of the extremes. It is hidden as the center of the circle for those who know geometry. It is mean in relation to us, but not in relation to the thing, therefore it is hidden. For, the mean in relation to the thing is known to the geometricians and the improvement is by removing the less from the extreme. You cannot measure it through a general way, but by the divine holy Torah that cannot be measured by the human intellect and this is true anyway. | |Reminder: the mean with regard to itself is not opposing, but with regard to its quality it is opposing to each of the extremes. It is hidden as the center of the circle for those who know geometry. It is mean in relation to us, but not in relation to the thing, therefore it is hidden. For, the mean in relation to the thing is known to the geometricians and the improvement is by removing the less from the extreme. You cannot measure it through a general way, but by the divine holy Torah that cannot be measured by the human intellect and this is true anyway. | ||
| − | |style="text-align:right;"|<big>זכירה</big> האמצעי בבחינתו בעצמו אינו נגדיי ובבחינות טובו הוא נגדיי לא' מהקצוות והוא נסתר כמו {{#annot: | + | |style="text-align:right;"|<big>זכירה</big> האמצעי בבחינתו בעצמו אינו נגדיי ובבחינות טובו הוא נגדיי לא' מהקצוות והוא נסתר כמו {{#annot:center of a circle|1108,2234|gyB1}}מרכז העגולה{{#annotend:gyB1}} אף ליודעי חכמת השיעור והוא האמצעי בערך אלינו ולא בערך הדבר ועל כן הוא נסתר כי האמצעי בערך הדבר ידוע לבעלי חכמת השיעור והתיקון בהרחיק מהקצווי הפחות ולא תשער זה ע"ד כולל זולת התורה האלהית הקדושה שאינה משוערת מהשכל האינושי וזה אמת על כל פנים |
|- | |- | ||
|} | |} | ||
== Notes == | == Notes == | ||
| + | |||
| + | <div style="text-align: right;"><div class="mw-collapsible mw-collapsed"><div class="mw-collapsible-content"> | ||
| + | <br> | ||
| + | <references group=note/> | ||
| + | </div></div></div> | ||
| + | |||
| + | == Apparatus == | ||
<div class="mw-collapsible mw-collapsed"><div class="mw-collapsible-content"> | <div class="mw-collapsible mw-collapsed"><div class="mw-collapsible-content"> | ||
<references /> | <references /> | ||
Latest revision as of 16:22, 1 October 2022
קצת מענייני חכמת המספר
Qeṣat mi-ʽInyanei Ḥoḵmat ha-Mispar
Contents
- 1 Sums
- 2 Multiplication of Fractions
- 3 Addition of Fractions
- 4 Subtraction of Fractions
- 5 Division
- 6 Division of Fractions
- 7 Roots
- 8 Cubic Roots
- 9 Divisibility of a Number
- 10 Square Numbers
- 11 Multiplicative Supplementing
- 12 Decomposing to a Fraction
- 13 Geometric Shapes
- 14 Euclidean Propositions
- 15 Notes
- 16 Apparatus
- 17 Appendix: Bibliography
| [1]קצת מענייני חכמת המספר | |
Sums |
|
Sums of Natural Numbers |
|
|
לידע מספרים מונחים על סדר המספר |
|
כגון אבג"ד ה"ו זחט"י |
|
|
|
|
תכה מהמספר האחרון שהוא העשרה בחציו וחצי א' |
|
|
|
|
או הוסיף עליו אח' והכהו בחציו בלי תוספ' ויהיו נ"ה וככה קבוצם |
|
|
|
|
ואם תרצה הכה המספר האחרון בעצמו שהוא י' יעלה ק' הוסיף עליו מספר השרש שהוא הי' יהיו ק"י קח חציים והוא המבוקש |
|
חשבון שחובר על הסדר ועלה מספר ידוע |
|
כגון מספר נ"ה כמה הוא מספר האחרון |
|
|
|
|
תכפלהו בב' יהיו ק"י תמצא בו מרובע וגדרו באחת ואז השאלה אמתית וגדרו הוא סוף המספר |
|
ואם תרצה הוסיף רביע אחד על כפלו וקח גדר הכל ותפיל גדר הרביע והוא החצי והנשאר הוא המבוקש |
|
ואם המספרים המונחים על סדר המספר לא יתחילו מהא' |
|
|
|
|
כאמרך מה' עד י' |
|
דע קבוצם מא' עד עשרה הוא נ"ה ודע קבוצם מא' עד ד' שהוא קודם ה' יהיו י' הפלים מהנ"ה ישארו מ"ה וככה קבוצם |
|
חשבון שחובר מתחלת מספר ידוע |
|
כאמרך מה' ועלה מ"ה כמה הוא סוף המספר |
|
דע קבוץ האחדים שמא' עד המספר הקודם לה' שהוא הד' יהיו י' הוסיפם על המ"ה יהיו נ"ה דע מאיזה מספר נתחברו ע"ד הקודם והוא י' וזהו סוף המספר |
|
ידענו הי' שהוא המספר שהנקבץ עד מ"ה ונעלם הה' |
|
תדע מהא' עד י' שהוא נ"ה |
|
ותדע עד איזה מספר נקבץ קבוץ היתרון על המ"ה שהוא י' ע"ד הקודם והוא ד' והמספר שלאחריו הוא המבוקש |
Sums of Squares |
|
|
לידע מרובעי המספרים הטבעיי' על הסדר עד איזה מספר שתרצה |
|
כגון מהא' עד י' |
|
|
|
|
תדע קבוצם ע"ד הקודם והוא נ"ה |
|
ותקח ב' שלישיות הי' בתוספת שליש א' יהיו ז' |
|
ותכם עם הנ"ה יהיו שפ"ה וככה קבוצם |
|
ואם אין למספר שליש שלם אם בתוספת א' |
|
כמו הי' |
|
|
|
|
חסר א' וקח ב' שלישיות הנשאר והוסף עליו אח' שלם והכם בקבוץ אחדיו |
|
ואם בחסרון אחד |
|
כמו הי"א |
|
|
|
|
הוסיף אח' וקח ב' שלישיות ותחסר מהמקובץ שליש א' ותכהו בקבוץ אחדיו והוא המבוקש |
|
ואם לא יתחילו מהא' |
|
כאמרך מה' עד י' |
|
|
|
|
דע חבור המרובעים שמהא' עד י' ע"ד הנקדם ואח"כ דע קבוץ המרובעים שמהא' עד ד' שקודם הה' והפיל זה מזה והנשאר הוא המבוקש |
| The ancients did not talk about the opposite questions. | בהפך שאלות אלו לא דברו הראשונים |
Sums of Cubes |
|
|
לידע מעוקב המספרים על הסדר ר"ל קבוצם על הסדר עד איזה מספר שתרצה |
|
תכה קבוץ אחדיהם בעצמו והוא המבוקש |
|
מעוקבי' מספרים נעלמים שעלה סך ידוע ותרצה לדעת איזהו היסוד האחרון |
|
קח גדר הכל ודע עד איזה מספר חובר והוא המבוקש |
|
ואם לא יתחילו מהא' |
|
כגון שרצית לידע מעוקבי מספרים מה' עד הי' |
|
תדע כמה מעוקבי' שמא' עד י' ע"ד הקודם ותדע המעוקבים שמא' עד הד' שהוא הקודם לה' והפיל זה מזה והנשאר הוא המבוקש |
|
קבצנו ממעוקב מספר ידוע עד מספר נעלם ועלה סך ידוע ורצית לידע עד איזה מספר נקבץ |
|
כאמרך מה' עד מספר נעלם עלה 2925 |
|
תדע המעוקבים שמהא' עד ד' והם 100 ותוסיפם על 2925 יהיו [3025] תדע גדרם בנ"ה ותדע עד איזה מספר נקבץ על דרך הקודם והוא י' והוא המבוקש |
|
ידענו הי' ונעלם הה' ועלה 2925 |
|
תדע מא' עד י' והם 3025 ותגרע מהם המספר הידוע לך והוא 2925 וישארו ק' קח גדרם בעשר ודע עד איזה מספר חובר ע"ד הקודם והוא הד' והמספר שלאחריו שהוא ה' הוא המבוקש |
Sums of Odd Numbers |
|
|
לידע קבוץ הנפרדים על הסדר |
|
קח חצי הנפרד האחרון בתוספת חצי והכהו בעצמו |
|
או הוסיף אחד על הנפרד האחרון וקח חצי הכל בלא תוספת והכהו בעצמו |
|
חשבון שחובר על סדר הנפרדים ועלה קבוצם חשבון ידוע ותרצה לידע עד איזה נפרד חובר |
|
קח גדר המספר כי בהכרח יהיה לו גדר שלם אם השאלה אמתית וכפלהו והפיל א' מהנקבץ והנשאר הוא המבוקש |
|
ואם תרצה הכה חשבון הידוע בד' והפיל א' מגדר הכל |
|
ואם הנפרדים המונחים אשר רצינו לדעת קבוצם לא יתחילו מהא' |
|
דע קבוצם מהא' עד נפרד האחרון ע"ד הקודם וחסר מהמבוקש העולה מא' עד הנפרד שקודם הנפרד הא' והנשאר הוא המבוקש |
|
חשבון שחובר מנפרד ידוע על סדר הנפרדים ועלה סך ידוע כמה הוא הנפרד האחרון |
|
חבר מהא' עד הנפרד הא' שקודם הנפרד הידוע והוספיהו על הסך הידוע ותדע עד איזה נפרדים הגיע הכל והוא המבוקש |
|
ידענו הנפרד האחרון והסך ונעלם הנפרד האח' |
|
חבר קבוץ הנפרדים שמהא' עד הנפרד האחרון וראה היתרון שבינו ובין הסך ידוע ודע עד איזה מספר חובר והנפרד שאחריו הוא הנפרד הראשון |
Sums of Squares of Odd Numbers |
|
|
לידע מרובעי הנפרדים על הסדר |
|
כאלו תאמר א' ג' ה' ז' ט' |
|
|
|
|
דע חבור הנפרדי' ע"ד הקודם והם כ"ה |
|
וקח ב' שלישי התשעה שהוא מספר האחרון בתוספת שני שלישיות אחד יהיו ששה וב' שלישיות אחד |
|
הכם עם כ"ה יעלה קס"ו וב' שלישיות א' |
|
ודע כמה הנפרדים שמאחד עד תשעה שהוא המספר האחרון הם ה' |
|
קח לכל שלם שליש אחד יעלה חמשה שלישים שהם א' וב' שלישיות |
|
חסרם מהקס"ו וב' שלישי אח' ישאר קס"ה וככה קבוצם |
|
|
|
|
ואם תרצה קח שני שלישי הנפרד האחרון והכהו בשטח ההווה מהכאת חצי הזוג שאחריו בחצי נפרד שלאחריו |
|
והמשל בזה שני שלישי הט' הם ו' הזוג שלאחריו הוא י' |
|
קח חציים בה' ושמרם |
|
והנפרד שלאחריו הוא י"א |
|
קח חציים בה' וחצי |
|
והכם בה' השמורים יעלו כ"ז וחצי |
|
הכם בששה יהיו קס"ה וככה קבוצם |
|
|
|
|
ואם תרצה תקח שליש הנפרד האחרון בתוספת שליש אחד והוא [2]ג' ושליש ושמרם |
|
אח"כ הכה חצי הנפרד האחרון והוא הט' שהוא ד' וחצי בנפרד שלאחריו שהוא הי"א יעלו מ"ט וחצי |
|
הכם בג' ושליש השמורים יעלו קס"ה |
|
ואם תרצה חסר מקבוץ נפרדיו שלישיתו וששית אח' ושמור הנשאר והוסיף על הנפרד האחרון אח' והכה הכל בשמור |
|
במשלינו זה קבצינו נפרדיו ועלו כ"ה |
|
חסרנו מהם שלישיתו וששית אחד נשאר י"ו וחצי ושמרנום |
|
הוספנו על הט' אחד יהיו י' |
|
הכם |
|
ואם תרצה קח שליש הט' בתוספת ב' ג' א' אח' יהיו ג' וב' ג' אח' |
|
הכם בחצי הט' שהוא ד' וחצי יעלה י"ו וחצי |
|
הוסיף א' על הט' יהיו י' |
|
הכם בי"ו וחצי יעלה קס"ה |
|
|
|
|
ואם תרצה בדרך יותר נקל קח ששית הט' באח' וחצי ושמרהו |
|
אח"כ הכה המספרים הנמשכים אחריו זה בזה והם י' והי"א יעלה ק"י |
|
הכם באחד וחצי יעלה קס"ה |
|
לידע מרובעי הנפרדים שיש ממספ' ה' עד ט' |
|
תדע חבור מרובעי הנפרדים שיש מא' עד ט' |
|
ותדע חבור מרובעי הנפרדים שמאח' עד המספר הנפרד שקודם הה' והוא ג' |
|
והפיל זה מזה והנשאר הוא המבוקש |
|
|
|
| The ancients did not talk about the opposite questions. | בהפך שאלות אלו לא דברו הראשונים |
Sums of Cubes of Odd Numbers |
|
|
לקבץ מעוקבי הנפרדים על הסדר עד נפרד ידוע |
|
הכה קבוץ נפרדיו בכפל וחסר אחת |
|
או חסר חצי אחת מקבוץ נפרדיו והנשאר הכהו בכפל קבוץ נפרדיו |
|
חשבון שנתקבץ ממעוקבי הנפרדים על הסדר ורצית לידע נפרד האחרון |
|
קח חצי החשבו' הנתקב' והוסיף עליו חצי שמנית וקח גדר הכל והוסיף על היוצא רביע אחד שהוא שורש חצי שמנית והכה הכל בד' וקח השרש מהעולה וחסר אחת ממנו והנשאר הוא המבוקש |
|
ידענו נפרד האחרון והסך ונעלם הנפרד האחד |
|
דע מהא' עד הנפרד האחרון וקח היתרון שבין זה הסך ובין הסך הידוע לך ודע הנפרד האחרון ממנו ע"ד הקודם והנפרד שלאחריו הוא המבוקש |
Sums of Even Numbers |
|
|
לידע קבוץ הזוגות על הסדר |
|
קח חצי הזוג האחרון בתוספת אח' או הוסיף עליו ב' וקח חצי הכל והכהו בחצי הזוג האחרון והוא המבוקש |
|
ואם תרצה קח רביע הזוג האחרו' והכהו בזוג שלאחריו או קח רביע הזוג שלאחריו והכהו בזוג האחרון |
|
חשבון שחובר על הסדר הזוגות ועלה סך ידוע איזה הוא הזוג האחרון |
|
הכה הסך הידוע בד' והוסף על העולה א' ושרש הכל בחסרון אח' הוא המבוקש |
|
ואם הזוגות אשר רצינו לידע קבוצם ולא יתחילו מב' |
|
קבץ מב' עד הזוג האחרון וחסר מהמקובץ העולה מב' עד הזוג שלפני הזוג האח' והנשאר הוא המבוקש |
|
חברנו מזוג ידוע ועלה סך ידוע איזה הוא הזוג האחרון |
|
דע חבור הזוגות על הסדר עד הזוגות שקודם הראשון הידוע והוסיפהו על הסך הידוע ותדע עד איזה זוגות נתחבר ע"ד הקודם |
|
ידענו הזוג האחרון והסך הנעלם הזוג הא' |
|
דע חבור הזוגות שמב' עד האחרון הידוע וקח יתרון שבינם ובין הסך הידוע לך ודע עד איזה זוג נתחבר והזוג שלאחריו הוא הזוג הא' הנעלם |
Sums of Squares of Even Numbers |
|
|
לדעת מרובעי הזוגות על הסדר |
|
קח שני שלישי הזוג האחרון בתוספת ב' שלישי א' והכהו בעולה מחבור הזוגות |
|
ואם תרצה קח מחבור זוגותיהם ב' שלישיות והכהו בזוג האחרון שתוסיף עליו א' |
|
ואם תרצה קח ששית הזוג האחרון והכהו בשטח ההווה מב' המספרים הנמשכים אחריו |
|
ואם לא יתחילו מהב' |
|
תדע מרובעים מהב' עד הזוג האחרון ותחסר מהמקובץ העולה מב' עד הזוג שלפני הראשון |
| Understand this. | והבן |
| The ancients did not talk about the opposite questions. | בהפך שאלו אלו לא דברו הראשונים |
Sums of Cubes of Even Numbers |
|
|
לידע מעוקבי הזוגות על הסדר |
|
תכה העולה מקבוץ זוגי אחדיו בכפלם והוא המבוקש |
|
מעוקבי זוגות שעלה סך ידוע ותרצה לידע הזוג האחרון |
|
תקח גדר חצי הסך ותדע עד איזה זוג חובר |
|
לידע מעוקבי זוגות שלא יתחילו מב' |
|
דע מעוקבם עד הזוג האחרון וחסר מהמקובץ העולה מב' עד הזוג שלפני הראשון |
|
ידענו הזוג האחד והסך ונעלם הזוג האחרון |
|
תקבץ מהב' עד הזוג שקודם הא' ותוסיפהו על הסך הידוע ותדע עד איזה זוג חובר |
|
ידענו האחרון והסך ונעלם הא' |
|
תקבץ מהב' עד הזוג האחרון ותקח היתרון שבינו ובין הסך הידוע ותדע עד איזה זוג חובר ע"ד הקודם והזוג שלאחריו הוא הנעלם |
Progression |
|
| The sum of numbers that exceed each other by a known increment. | קבוץ מספרים נוספים אלו על אלו בתוספת ידוע |
Geometric progression |
|
|
אם על יחס הנדסי וזה על שני מיני' |
|
אם על יחס הכפל יתחילו מהאח' או לא יתחילו |
|
כגון מספרי ב'ד'ח' י"ו ל"ב ס"ד קכ"ח |
|
הדרך לדעת קבוצם שתכפול הקכ"ח שהוא המספר האחרון יעלה רנ"ו וגרע ממנו המספר הראשון שהוא הב' ישאר רנ"ד וככה קבוצם |
|
ואם הוא יחס אחר וגם אם יהיה יחס הכפל או השליש |
|
כגון מספרי ג' ט' כ"ז פ"א רמ"ג תשכ"ט שהם הולכים על יחס השליש |
|
יש בזה ב' דרכים |
|
|
|
|
הדרך הראשון שתדע המספר אשר יגזור שם היחס שנים לחצי ג' לשליש ד' לרביע ונגרע ממנו אחד כגון מספרינו זה שתוספת קצת על קצת הוא שליש נגרע ממנו האחד ישארו שנים והשבר הנגזר משנים הוא החצי ונקח החצי מהמספר האחרון אחר שנגרע ממנו הראשון יהיה שס"ג הוספנום על המספר האחרון שהוא תשכ"ט יעלה אלף [3]ופ"ט וככה קבוצם |
|
|
|
|
הדרך הב' שתכה המספר הראשון שהוא הג' עם התשכ"ט שהוא המספר האחרון אחר שתגרע ממנו הראשון יעלה שני אלפים וקע"ח חלקנום על המותר שבין הב' לראשון שהוא ששה יעלה שס"ג הוספנום על המספר האחרון שהוא התשכ"ט יעלה אלף ופ"ט וככה קבוצם |
|
|
|
|
ואם התחלתם מהאחד והם מיוחסים יחס הכפל כגון מספרי א"ב ד"ח י"ו ל"ב ס"ד ורצית לדעת מספר המדרגה האחרונה או איזו מדרגה שתהיה |
|
תכה האמצעית בעצמה כגון הח' במשלינו זה יעלה ס"ד |
|
|
|
|
או שני המדרגות הרחוקות מהאמצעית ריחוק שוה כגון הד' עם הי"ו או השנים עם הל"ב |
|
ואם היה מספר המדרגות זוג תכה השני אמצעים |
|
כגון שרצית לדעת מספר המדרגה הו' תכה המדרגה השלישית עם הרביעית שהוא הד' עם הח' ויעלה ל"ב |
|
|
|
|
או ב' המדרגות הרחוקות שהם ריחוק שוה כגון הב' עם הי"ו ויעלה ל"ב וזהו מספר המדרגה הששית |
Arithmetic progression |
|
|
ואם הם על יחס מספרי וזה על ג' מינים או התחלתם שוה לתוספתם או פחות או יותר |
|
ואם התחלתם שוה לתוספתם יתחילו מהאח' או לא יתחילו |
|
כגון מספרי ג' ו' ט' י"ב ט"ו י"ח כ"א שתוספת אלו על אלו שוה למספר הראשון והוא הג' |
|
יש בזה ב' דרכים |
|
|
|
|
הראשון שתקח חצי כמות המדרגות בתוספת חצי ז' שהוא במשלינו זה ד' ותכהו במספר האחרון שהוא הכ"א יעלה פ"ד |
|
|
|
|
והב' שתחבר הראשון עם האחרון יהיו כ"ד ותקח חצים והוא י"ב ותכם במנין המדרגות והוא ז' יעלה פ"ד |
|
ואם התחלתם פחות מתוספתם |
|
כגון א' ג' ה' ז' ט' י"א י"ג ט"ו שתוספתם שנים והתחלתם מהאחד |
|
|
|
|
נוסיף המגרעת שהוא אחד על המספר האחרון שהוא ט"ו יהיו י"ו |
|
ונכם בחצי המדרגות בתוספת חצי שהם ד' וחצי יעלה ע"ב |
|
נגרע מהם הכאת המגרעת עם כל המדרגות שהוא הכאת האח' בח' שהם מנין המדרגות יעלה ח' |
|
נגרעם מהע"ב ישארו ס"ד וככה קבוצם |
|
|
|
|
ואם תרצה להשתמש בדרך הב' שנחבר הא' עם הט"ו יהיו י"ו ונקח חציים שהוא ח' ותכם במנין המדרגות שהם ח' יהיו ז' ס"ד וככה קבוצם |
|
ואם יותר מתוספתם |
|
כגון מספרי ה' ח' י"א י"ד י"ז כ' כ"ג |
|
|
|
|
תוסיף היתר על התוספת שהוא ב' על המספר האחרון שהוא כ"ג יהיו כ"ה |
|
הכם בחצי המדרגות בתוספת חצי יהיו ק' |
|
גרע התוספת שהוא ב' ישאר צ"ח וככה קבוצם |
|
|
|
|
ואם תרצה חסר הראשון שהו' הה' מן האחרון שהוא כ"ג ישארו י"ח |
|
תחלקהו על מספר תוספתם שהוא ג' והיוצא תוסיף עליו א' יהיו שבעה ושמרם |
|
אח"כ חבר הראשון והאחרון והכם בחצי השבעה |
|
|
|
|
או תקח חציים ותכם עם השבעה וככה קבוצם |
|
ואם תרצה תדע אם המספר שממנו צמיחתם שוה לתוספתם |
|
כגון מספרי ו' ח' י' י"ב י"ד י"ו י"ח כ' |
|
|
|
|
תדע מנין המדרגות שממספר שממנו צמיחתם עד המספר האחרון |
|
ותדע קבוץ המספרים על דרך הקודם ושמרהו |
|
ואח"כ תדע מנין המדרגות שהם מהמספר שממנו צמיחתם עד המספר הקודם לראשון מדרגה אחת |
|
ותדע קבוצם על דרך הקודם ותגרע זה מהשמור |
|
ואם המספר שממנו צמיחתם פחות מתוספתם |
|
כגון מספרי ה'ח' י"א י"ד י"ז |
|
תדע מנין המדרגות שמהמספר שממנו צמיחתם עד המספר האחרון |
|
ותוסיף המגרעת על המספר האחרון ותדע מנין קבוצם ע"ד הקודם |
|
תכה חצי כמות המדרגות בתוספת חצי עמו |
|
ותגרע מהעולה הכאת המגרע' עם מנין המדרגות שמהמספר שממנו צמיחתם עד המספר האחרון ושמור הנשאר |
|
אח"כ תדע מנין המדרגות שמהמספר שממנו צמיחתם עד המדרגה הקודמת למספר המדרגה הראשונה |
|
ותחבר עמו מספר המגרעת |
|
ותכהו עם חצי המדרגות בתוספת חצי |
|
ותגרע מהעולה הכאת המגרעת עם כל המדרגות שמהמספר שממנו צמיחתם עד המדרגה הקודמת למספר הראשון |
|
והנשאר תגרעהו מהשמור והוא המבוקש |
|
ולידע מנין המדרגות וגם לידע אם המספר הא' שממנו צמיחתם שוה לתוספתם |
|
תחלק המספר האחרון על מספר תוספתם |
|
ואם יצא בחילוק שוה מה שיצא בחילוק הוא מספר המדרגות שמהמספר שממנו צמיחתם עד המספר האחרון והתחלתם שוה לתוספתם |
|
ואם נותר שום מספר מה שיצא בחילוק בתוספת אחת הוא מספר המדרגות שמהמספר שממנו צמיחתם עד המספר האחרון והנותר הוא המספר שממנו צמיחתם |
| Understand this. | והבן |
|
ואם המספר האחרון הוא אשר רצית לידע הכה המותר עם מספר המדרגות חסר אחת והוסיף על העולה מספר הראשון |
| Understand this. | והבן |
| There are many specific method for [this] specific issue and also other issues of arithmetical problems etc. | ויש דרכים אחרים מיוחדים בנושא מיוחד וג"כ עניינים אחרים לידע שאלות החשבון וכו' |
Multiplication of Fractions |
הכאת השברים | ||
| Multiply the numerator by the numerator, then relate [the product] to the product of the denominator by the denominator.
|
שתכה הכמות עם הכמות וניחסהו אל הכאת האיכות עם האיכות | ||
| Or we multiply the product of the denominators by itself, then we relate to it the result of multiplication of the products of the numerator of one by the denominator of the other.
|
או נכה העולה מהכאת האיכות בפני עצמו ונייחס אליו הכאת העולה מהכאת כמות האח' עם איכות חבירו זה בזה | ||
| Likewise, if fractions of fractions, and fractions of fractions of fractions and so on endlessly are related to integers: we multiply all the numerators by each other, then the product by the integers, whether they are integers or fractions of fractions of integers, then multiply the denominators by their order and relate the product to the product of the numerators by each other.
|
וכן אם היו מיוחסים אל שלמים שברי שברים ושברי שברי שברים וכן עד אין תכלית נכה כל האיכיות זה בזה ומה [4]שיתקבץ עם השלימים ואם היו שלמים אחדים או שברי שברים של שלמים תחזור ותכה כל הכמיות על הסדר ותיחס | ||
| If you want to sum products of numbers: | ואם תרצה לקבץ מספרים מוכים | ||
|
כגון שרצית לידע קבוץ הכאת שני רביעיות עם ב' ששיות עם קבוץ הכאת ד' חומשיות עם ה' ששיות מבלתי שתצטרך תחלה להכות ואח"כ לקבץ אבל יצא הכל מתוקן ביחד | ||
| |||
|
תכה האיכיות כלם זה בזה יעלה תש"כ ותשמרם | ||
|
אח"כ תכה כמות הראשונים עם איכות השניים יהיו ק"ך | ||
|
וכמות השניים עם איכות הראשונים יעלה ת"ף | ||
|
חברם אל ק"ך יהיו ת"ר | ||
|
יחסם אל תש"כ יהיו ת"ר חלקים מתש"כ והם ה' שישיות וככה העולה מקבוץ הכאת אלו עם קבוץ הכאת אלו | ||
| In this way, as many as the numbers are, you multiply the numerators of the whole number by all the denominators. | וכן על דרך זה ירבו המספרים מה שירבו תכה כמיות כל המספר עם כל האיכיות |
| If you want by way of addition, i.e. you wish to multiply this sum by that sum. | ואם תרצה ע"ד הקיבוץ שרצית להכות קבוץ זה עם קבוץ זה |
|
כגון שרצית לידע כמה העולה מהכאת הב' רביעיות עם שני ששיות עם קבוץ ד' חומשיות עם ה' ששיות בזול' שתקבצם ואח"כ להכותם אבל יצא הכל מתוקן בפעם אחת | ||
|
תסדרם ככה | ||
| |||
|
תכה כמות הראשון עם אכות הב' ואיכות הג' וכמות הד' יהיו ש' ושמרם | ||
|
אח"כ תכה כמות הראשון עם איכות הב' וכמות הג' ואיכות הד' יהיו רפ"ח ושמרם | ||
|
אח"כ תכה כמות הב' עם איכות הראשון ועם איכות הג' וכמות הד' יהיו ק"ק ושמרם | ||
|
אח"כ תכה כמות הב' עם איכות הראשון וכמות הג' ואיכות הד' יהיו קצ"ב | ||
|
חבר הד' השמורים יעלה תתק"ף | ||
|
ותכה המורים זה בזה עד כלותם יעלה תש"כ | ||
|
יחס התתק"ף אליהם הוא העולה מהכאת קבוץ ב' רביעיות עם שני ששיות עם קבוץ ד' חומשיות עם חמשה ששיות | ||
Methods of checking |
מאזנים |
|---|---|
| Divide the result of the multiplication by one of the multiplied fractions, whichever you want, and the result of division is the other fraction.
|
שתחלק העולה מההכאה על השב' אחד מהמוכים איזה שתרצה ויעלה השבר האחר בחלוקה |
|
כיצד הכינו ב' רביעיות עם ב' ששיות ועלה ד' חלקים מכ"ד |
|
נחלקם על הב' רביעיות יצא בחילוק שני ששיות |
| The division procedure will be explained in its place with God's help. | ודרך החלוק יתבאר במקומו בעה"ו |
| Another scale: multiply the difference between the smaller fraction and 1 by the other. We sum the result with the primary product and [the sum] is equal to the greater fraction.
|
מאזנים אחרים שתכה ההב' שבין השבר הקטן עד תשלום הא' שלם עם חבירו ונקבץ העולה עם העולה מהכאה ראשונה וישוה לשבר הגדול |
|
במשל זה הכינו ב' רביעיות עם ב' ששיות ועלה ד' חלקים מכ"ד |
|
לקחנו השבר הקטן והוא הב' ששיות וההבדל שבינו ובין השלם ד' ששיות |
|
הכינום עם ב' רביעיות שהוא השבר הגדול ועלה ח' חלקים מכ"ד |
|
קבצנו אותם עם הד' חלקים מכ"ד שהיא ההכאה הראשונה והם י"ב חלקים מכ"ד והם הב' רביעיות |
|
ואם תרצה לאמת אם הם בעצמם הב' רביעיות סדרם ככה | ||
| |||
| Cross-multiply the numerator of the one by the denominator of the other and [the result] is equal to the product of the denominator of the one by the numerator of the other. Understand [this].
|
ותכה אלכסונות כמות הא' עם איכות חבירו וישו' העולה מהכאת כמות האחד עם איכות חבירו והבן | ||
| Another scale: we take the numerator of the fraction resulting from the multiplication of the fractions. We look for a number whose ratio to it is the ratio of whichever fraction you wish of the multiplied fractions. If the ratio of the required number to the numerator of the fraction resulting from the multiplication of the fractions is equal to the ratio of the remaining fraction, you were right.
|
מאזנים אחרים נקח כמות השבר היוצא מהכאת השברים המוכים ונבקש מספר שיהי' יחסו אליו יחס השבר האח' מהשברים המוכים איזה שתרצה ואם היחס מספר המבוקש אל איכות השבר היוצא מהכאת השברים המוכים שוה אל יחס השבר הנשאר צדקת | ||
|
במשלינו זה הכינו הב' רביעיות עם הב' ששיות ועלה ד' חלקים מכ"ד | ||
|
נבקש מספר שיהיה מיוחס אליו הד' שהוא הכמות היוצא מהכאת השברים המוכי' יחס ב' רביעיות או ב' ששיות | ||
|
ודרך ידיעת זה הוא שנסדרם ככה | ||
| |||
|
ונכה האלכסונות שהם ד' עם ד' יעלה י"ו | ||
|
נחלקם על השנים שהוא איכות הב' רביעיות יעלה ח' | ||
|
ונשים אותו תחת הד' הב' נמצא שארבעה מח' הוא יחס הב' רביעיות | ||
|
וניחס מספר המבוק' שהוא הח' אל איכות השבר היוצא מההכאה שהוא כ"ד ונמצא שהם ב' ששיות ממנו וזהו השבר האחד הנשאר | ||
|
ואם תרצה לעשות המאזנים בהב' שתבקש מספר שיהיה יחסו הד' אליו כיחס הב' שישיות | ||
|
וזה בשנסדרם ככה | ||
| |||
|
ותכה האלכסון שהוא הו' עם הד' יעלה כ"ד | ||
|
חלקם על הב' יעלה י"ב בחלוקה | ||
|
ותשימים תחת הד' ותקח הי"ב ותיחסם אל הכ"ד והם ב' רביעיו' ממנו והוא בעצמו השבר האחר | ||
Addition of Fractions |
קבוץ השברים | |
|
|
||
| The second species is the addition of fractions, which is that if you wish to sum up known fractions, multiply the numerator of the first by all the denominators, except that which beneath it, then relate it to the product of the denominators one by the other, or divide it by it; reduce it, meaning examine by which of them it is divided first and divide it by it. Likewise for the second [numerator and so on] until they are complete. | המין הב' הוא קבוץ השברים והוא שאם תרצה לקבץ שברים ידועים תכה כמות האח' עם כל האכיות זולת מה שתחתיו ותיחסהו אל הכאת האיכיות זה בזה או תחלקהו עליהם ותעשה אותם כלילת יופי רצוני שתראה לאיזה מהם מתחלק ראשונה ותחלקהו עליו ואח"כ על הב' עד תומם | |
|
דמיו' רצינו לקבץ שני רביעיות וד' שמניות וג' תשיעיות | |
|
נסדרם ככה | |
| ||
|
תכה הב' שהוא הכמות הא' עם הח' שבאלכסונות ומה שיתקבץ עם הט' שהוא איכות הג' יעלה קמ"ד ושמרם | |
|
אח"כ תכה כמות השני והוא הד' עם הד' שבאלכסונות שהוא איכות הראשו' ומה שיתקבץ עם הט' שהוא איכות הג' יעלה קמ"ד ושמרם | |
|
ג"כ תכה כמות הט' והוא הג' עם איכות הד' והוא הח' ומה שיתקבץ עם איכות הב' והוא הד' יעלה [.] צ"ו | |
|
חברם עם הב' המספרים השמורים יעלה שפ"ד | |
|
אח"כ נכה כל האכיות זה בזה יעלה רפ"ח | |
|
והם השפ"ד מהם א' שלם [5]וצ"ו חלקים מרפ"ח | |
|
ואם תרצה לחלק השפ"ד על המורים כשתחלקהו תחלה על הח' אח"כ לד' אח"כ לט' כדי שיצאו לך החלקים נאותים ויעלה בחלוקה א' שלם וג' תשיעיות והם הצ"ו חלקים מרפ"ח | |
| The proof is that you arrange them like this: | והמופת שתסדרם ככה | |
| ||
|
ותכה האלכסונו' ויהיו שוים זהו הדרך הא' | |
|
|
||
| The second method: take the complement of the first fraction for a whole unit. We subtract from it the other fraction that is summed with it, if the complement is greater than it. Then, take the complement for the whole unit and this is the sum of the two fractions.
|
והדרך הב' שתקח החסרון אשר יחסר מהשבר הא' עד תשלומו לא' שלם ונחסר ממנו השבר הא' הנקבץ עמו אם היה החסרון גדול ממנו והיוצא קח החסר ממנו עד תשלום הא' שלם והוא סך הב' שברים | |
|
דמיון רצית לקבץ שני רביעיות עם ב' תשיעיו' | |
|
תסדרם ככה | |
| ||
|
ותקח החסר מהב' רביעיות עד תשלום א' שלם והם שני רביעיות אחרים | |
|
ותחסר מהם הב' תסיעיות ישארו י' חלקים מל"ו | |
|
וקח החסר מהם עד תשלום האחד שלם והם כ"ו חלקים מל"ו וככה קבוצם | |
|
|
||
| If the complement from a whole unit is smaller than the second fraction, so you cannot subtract the other fraction from it. | ואם מה שחסר לא' שלם הוא יותר קטן מהשבר הב' באופן שלא תוכל מחסר ממנו השבר הא' | |
|
|
||
|
כגו' ד' תשיעיו' עם ה' ששיות | |
| ||
|
שחסר לד' תשיעיות לתשלום אח' שלם הוא ה' תשיעיו' והם פחות מה' ששיות | |
|
הנה נחסר הה' תשיעיות מהה' ששיות ישאר ט"ו חלקים מנ"ד והם התוספת על הא' השלם וקבוצם הוא א' שלם וט"ו חלקים מנ"ד | |
| If you wish to add a third fraction. | ואם תרצה לקבץ עוד שב' ג' | |
|
|
||
|
כאלו משל ו' שביעיות | |
|
הנה תעשה הדרך הקודם בעיון שתשלים הט"ו חלקים מנ"ד לא' שלם והוא ל"ט חלקים מנ"ד | |
|
ובעבור שהשש שביעיות יותר מל"ט חלקים מנ"ד | |
|
הנה נחסר מהם ל"ט חלקים מנ"ד ישאר נ"א חלקים משע"ח והם התוספת על הא' השלם והא' השמור שבידינו הם ב' והם ב' ונ"[א] חלקים משע"ח |
|
|
||
| Likewise, if you have to subtract the fraction from it, for instance, when the complement of the result is greater than [the third fraction], take the complement of what remains and it is the sum of the three fractions.
|
וכן אם היית צריך לחסר השבר האחד ממנו כגון שהיה אשר יחסר ליוצא גדול ממנו הנה ממה שישאר תשלימהו לא' והוא סך הג' שברים הנקבצים | |
| In this way you can sum endlessly. | וכן בזה הדרך תוכל לקבץ עד אין תכלית | |
| If you want to use another method, take the result before you subtract or add it. | ואם תרצה להשתמש בדרך אחרת והוא שתקח היוצא טרם שתחסרהו או תוסיפהו | |
|
|
||
| If the result is an excess over one and it is smaller than the third [fraction] that you want to sum with the two others, subtract it from that fraction and the result is the excess over the one integer.
|
ואם היה היוצא מאשר יצטרך להשלים לאחד וג"כ היה קטן מהג' אשר תרצה לקבץ עם הב' האחרים הנה תחסרהו מהשבר ההוא והיוצא הוא התוספת על השלם הא' | |
|
ד"מ ואם רצית לקבץ ב' רביעיות עם ב' שישיות וד' שביעיו' | |
|
סדרם ככה | |
| ||
|
הנה תקח החסר מב' רביעיות עד הא' השלם ונחסר ממנו שני ששיות נשאר ד' חלקים מכ"ד | |
|
ונצטרך להשלים לאחד ובזה יודע סך הג' שברים הנה לא תשלימהו לאחד אלא תקחם כמות שהם ונחסרם מהשבר הג' שהוא ד' שביעיו' ישאר ס"ח חלקים מקס"ח והם התוספת על אח' שלם תמצא סך קבצם עולה א' ס"ח חלקים מקצ"ח |
|
|
||
| If the result is greater than the fraction that you want to add. | ואם היוצא גדול מהשבר אשר תרצה לקבצו | |
|
|
||
|
כגון שהוספנו במקום הד' שביעיות עשירית | |
|
כזה הצורה | |
| ||
|
שהנה הד' חלקים מכ"ד הם יותר גדול מעשירית | |
|
הנה נחסר העשירית מהם ישארו י"ו חלקים מר"ם | |
|
הם נשלימם לא' שלם רכ"ד חלקים מר"ם וככה קבוצם של ג' השברים |
| If the result is an excess over one integer, we keep the one and look for the complement of the result for one unit. | ואם היוצא מאשר נצטרך להוסיף על השלם האחד הנה נשמור השלם ונבקש החסרון אשר יחסר מהיוצא עד תשלום הא' השלם | |
|
|
||
| If this complement is smaller than the third fraction that is summed with the others, we subtract it from it, then add one to the result, and with the one that is kept in our hand it is the total sum.
|
ואם היה החסרון ההוא קטן מהשבר הג' הנקבץ עם האחרים הנה נחסרהו ממנו היוצא נוסיף עליו אחד ועם הא' השמור בידינו והוא סך השבר | |
|
|
||
| If [the complement] is greater than the third summed fraction, subtract the fraction from it, and take the complement of the remainder for a whole unit, then add to it the one that is kept in our hand and it is the sum of the three fractions.
|
ואם היה החשבון גדול מהשבר הג' הנקבץ הנה תחסר השבר ממנו והיוצא תשלימהו לא' שלם והוסיף עליו הא' השמור אשר בידנו והוא סך הג' שברים | |
| Understand this. | והבן | |
| The third addition method | והדרך הג' לקבוץ | |
| One should know one proposition, which is that there are two types of numbers: relatively prime and relatively composite. | צריך לידע הקדמה א' והוא שהמספרים ב' מנים נבדלים משותפים | |
| The relatively prime numbers are those that are not counted by any number [= not share a common divisor] other than 1. | הנבדלים הם שלא ימנה לשנים מספר זולת הא' | |
| The relatively composite numbers are of two types: either one counts [= is a divisor of] the other, or not, but there is another number that counts both [= a common divisor of both]. | והמשותפים שני מינים או ימנה האח' את חברו או לא ימנהו אלא שיש מספר אח' ימנה את שניהם | |
| The way to identify these types is that you divide the greater by the smaller and the quotient resulting from the division indicates the reduced ratio.
|
והדרך אל ידיעת אלו המנים בשתחלק הגדול על הקטן והחלק היוצא בחלוק מורה על קטן היחס | |
|
כי אם היו שני המספרים המתיחסים עשרה וק' תחלק הק' על הי' ויצא בחלוקה י' ואלה | |
| If something remains, divide the smaller number by the remainder, then the first remainder by the second remainder and so on do not cease from dividing the remainder by the remainder until the number ends, so that no remainder is left, and [the last remainder] is the greatest number that counts both of them [= their greatest common divisor].
|
ואם ישאר כלום תחלק המספר הקטן על המותר אח"כ המותר האח' על המותר הב' ולא תסור מחלוק המותר על המותר עד שיכלה המספר שלא ישאר בו מותר והוא גדול המספר אשר ימנם יחד | |
| Afterwards, divide the smaller number by it and keep the result. | אח"כ חלק המספר הקטן עליו והעולה שמרהו | |
| Look also for the number of times that it counts the greater, by dividing it by it, and keep the result. | גם בקש מספר הפעמים אשר ימנהו הגדול וזה כשתחלקהו עליו והעולה שמרהו | |
| The two reserved [numbers] are the smallest proportional numbers of this ratio. | והב' שמורים הם קטני היחס ההוא | |
|
המשל השות' י"ב והמספר הקטן כ"ז | |
|
חלקהו הכ"ז על הי"ב נשארו ג' | |
|
חלקנו עליהם הי"ב ולא נשאר כלום ולכן מצינו שגדול המספר אשר ימנם יחד הוא ג' | |
|
בקשנו מספר הפעמים אשר ימנה הי"ב הוא בשחלקנו הי"ב על הג' ועלה ד' ושמרנום | |
|
חלקנו הכ"ז על הג' ועלה ט' ושמרנום | |
|
וידענו שהד' והט' הם קטני היחס ההוא ולכן ידענו שהי"ב יחסו אל הכ"ז ד' תשיעיות | |
| Therefore, when you want to find the smallest number in which given fractions are found, whichever they are, if their denominators are relatively prime, they are multiplied by each other and the product is the smallest number, in which these given fractions are found.
|
ולכן כשתרצה לדעת קטן המספרים אשר ימצאו בו השברים המונחים איזה שברים שיהיו עם איכיותיהם נבדלים זה מזה יוכו זה עם זה והעולה הוא קטן המספר אשר מצאו בו אלו החלקים ההם המונחים | |
| If they are relatively composite and one counts the other, the greater number is the lowest common multiple, in which these given fractions are found.
|
אם יהיו משותפים והאח' מונה אחר המספר הגדול [6]הוא קטן היחס אשר ימצאו השברי' המונחים | |
| If it does not count it, but there is a number that counts them together, we look for their lowest common multiple according to the previous way. | ואם לא ימנהו אלא שיש מספר ימנם יחד נבקש קטן יחסם ע"ד הקודם | |
| Then, we multiply the greater number among the two given denominators by the smaller number of their ratio in the lowest term. | ונכה המספר הגדול מב' האיכות המונחים עם המספר הקטון מב' איכות קטן היחס | |
| Or, We multiply the smaller number among the two given denominators by the greater number of their ratio in the lowest term. | או נכה המספר הקטן מב' איכיות המונחים עם המספר הגדול מב' איכיות קטן היחס | |
| The result is the smallest number, in which the two given fractions are found [= LCM].
|
והעולה הוא קטן המספר אשר ימצאו בו ב' שברים ההם | |
| If there are numerous fractions apply this same method: find the lowest common multiple, in which these two fractions are found, according to what preceded. | ואם היו השברים רבים תעש' בזה הדרך עצמו תמצא קטן היחס אשר ימצאו בו ב' שברים ההם לפי מה שקדם | |
| If the third fraction counts it, the lowest common multiple, in which the former fractions are found, is also the lowest common multiple of the three fractions. | עם השבר הג' מונהו הנה קטן היחס אשר ימצאו בו השברים הקודמים בו ג"כ ימצא קטון היחס של הג' שברים | |
| If they are relatively composite, we multiply it by it. | ואם הם נבדלים נכהו עמו | |
| If another number counts both of them, we multiply [the smaller number of] their ratio in the lowest term by the greater number, which is the lowest common multiple, in which the two former fractions are found. | ואם מספר אחר ימנם יחד נכה המספר הקטון יחסם עם המספר הגדול שהוא המספר קטן היחס אשר נמצא בו הב' שברים הקודמים | |
| The same way for more fractions | וכן ע"ז הדרך ירבו השברי' | |
|
|
||
| Therefore, if you wish to sum up some fractions, we take their lowest common multiple according to the said way, divide it by all the denominators as the number of their numerators and keep each separately, then we sum up [the reserved numbers] and divide [the sum] by their lowest common multiple and this is the result. | ולכן אם תרצה לקבץ שברים מה הנה ניקח קטון היחסם ע"ד האמור ונחלקהו על כל האיכיות כפי מנין כמותם ונשמור כל אחד בפני עצמו אח"כ נקבצם ונחלקם על מספר קטון יחסם וזהו העולה | |
|
ד"מ בזה רצינו לקבץ ב' רביעיות וד' ששיות וח' תשיעיות וה' שמניות | |
|
סדרם ככה | |
| ||
|
והד' והו' ימנם מספר הב' | |
|
כשתעשה הדרך האמור שנחלק הו' על הד' וישאר ב' | |
|
אח"כ תחלק הד' על הב' ולא ישא' כלום ידענו שהשנים הוא מספר ימנם יחד | |
|
ולכן נכהו עם הו' או נכה הג' שהוא מספר הפעמים שישנה הו' הב' עם ד' יעלה י"ב וזהו קטון היחס אשר יש בו רביעית וששית | |
|
אח"כ ידענו שהט' הוא מספר נבדל מהי"ב ולכן הכנו אותו עם הט' יעלה ק"ח | |
|
וע"ד הקודם מצאנו שהד' ימנה לח' ולק"ח והכנו הד' עם הק"ח ועלה תל"ב וזהו קטון המספר אשר ימצא בו רביעית וששית ותשיעית ושמנית | |
|
ואח"כ חלקנו התל"ב על הו' ד' פעמים כמנין כמותם ועלה רפ"ח | |
|
אח"כ חלקנו התל"ב על הט' ח' פעמים כמנין כמותם ועלה שפ"ד ושמרנום | |
|
אח"כ חלקנו התל"ב על הח' ה' פעמים כמנין כמותם ועלה ר"ע | |
|
חברנו הד' שמורים יעלה אלף וקנ"ח | |
|
יחסם אל תל"ב יהיו שנים שלמים ורצ"ד חלקים מתל"ב | |
| Q.E.D. | וזה מה שרצינו לבאר |
Methods of checking |
מאזנים | |
|
|
||
| We subtract whichever of the [summed] fractions you wish and the other remains. | שנחסר השבר הנשאר איזה מהם שתרצה וישאר האחר | |
|
המשל קבצנו שני רביעיות עם ב' ששיות | |
|
כזה | |
| ||
|
ועלה עשרים חלקים מכ"ד | |
|
חסר מהם הב' רביעיות ונשארו שני ששיות | |
|
או חסר הב' ששיות וישארו הב' רביעיות | |
|
|
||
| Another scale: add to the sum the complement for one of whichever of the summed fractions you wish, then subtract one from the result, and if the remainder is equal to [the sum of] the remaining summed [fractions], you were right. | מאזנים אחרים שתקבץ עם המקובץ החסר משלימו איזה שבר שתרצה מהנקבצין עד האחד השלם והעולה תשליך ממנו אחד ואם הנשאר שוה לעולה מהנקבצין הנשארים צדקת | |
|
כגון שלקחנו הב' רביעיות שחסר משלמותך השלם וקבצנום עם כ' חלקים מכ"ד יעלה א' וב' ששיות השלכנו האח' נשאר הב' ששיות | |
|
או נקבץ החסר משני ששיות שהם ד' ששיות עם כ' חלקים מכ"ד יעלה אח' וג' שישיות והשלכנו האחד ונשאר ג' ששיות והם שני רביעיות | |
| Understand this. | והבן | |
Subtraction of Fractions |
דרך החסור | |
| Now we start to explain the method of subtraction by the help of God. | ועתה נתחיל לבאר דרך החסור בע"ה | |
|
|
||
| I say that the most common method is that you multiply the numerator of the one by the denominator of the other and the numerator of the other by the denominator of the one, then subtract one from the other and relate the remainder to the product of the denominators. | ואו' שהדרך היותר כולל הוא שתכה כמות האחד עם איכות חבירו וכמות האחר עם איכות חבירו וחסר זה מזה והנשאר [תיחס][7] אל הכאת האיכות | |
|
|
||
| Or, divide by the denominators according to the previous way, by reducing them and this is the required. | או תחלקהו על המורים ע"ד הקודם בשתעשה להם כלילת יופי והוא המבוקש | |
|
דרך משל רצינו לחסר ג' שמניות מד' ששיות | |
|
כזה הצורה | |
| ||
|
תכה כמות הג' עם איכות הד' יהיו י"ח | |
|
ותכה הכמות האחר עם איכות חבירו יהיו ל"ב | |
|
תחסר מהם י"ח ישאר י"ד | |
|
ותכה האיכיות והם הח' בו' יהיו מ"ח | |
|
והם י"ד חלקים ממ"ח | |
|
או תחלק הי"ד על הו' יעלה ב' וישארו ב' והם שני שמניות וב' ששיות וככה צורתם | |
| ||
|
והוא הנשאר מחסור הג' שמניות מד' ששיות | |
|
|
||
|
דרך אחרת שתשלים השבר הגדול שהוא ד' ששיות לא' שלם והנה השלמנום בשני ששיות | |
|
קבצנום עם השבר הקטן והוא הג' שמניות יעלה ל"ד חלקים ממ"ח | |
|
השלמנום לאח' שלם בי"ד חלקי' ממ"ח והוא המבוקש | |
|
|
||
|
ואם תרצה קבץ החסר מהשבר הקטן והוא הג' שמניות | |
|
השלמנום לאח' שלם בה' שמניות | |
|
נקבצו עם הד' ששיות ועלה א' שלם וי"ד חלקים ממ"ח | |
|
והשלכנו האחד השלם ומה שישאר הוא המבוקש | |
Methods of checking |
מאזנים | |
|
|
||
| Sum up the remainder from the subtraction of the fraction from the fraction with the greater fraction, then subtract the smaller [fraction] from the sum, and if the remainder is double the remainder from the [original] subtraction, you were right. | שתקבץ הנשאר מחיסור השבר מהשבר עם השבר הגדול והעולה מקבוצם חסר ממנו הקטן והנשאר ממנו אם היה כפל הנשאר מהחסור צדקת | |
|
במשלינו זה קבצנו הי"ד חלקים ממ"ח עם הד' ששיות ועלה רע"ו חלקי' מרפ"ח | |
|
חסרנו מהם הג' שמניות ונשאר אלף ושמ"ד חלקים מהאלפים וש"ד והם כפל הי"ד חלקים ממ"ח |
|
והמופת שתשי' ה[כ"]ד חלקים ממ"ח והאלף ושמ"ד חלקים מאלפים וש"ד בזאת הצורה | |
| ||
|
ותכה האלכסונות ויהיו שוים | |
|
|
||
| Another scale: sum up the remainder from the subtraction of the fraction from the fraction with the smaller fraction, then if [the remainder] is equal to the greater [fraction], you were right. | מאזנים אחרים שתקבץ הנשאר מחיסור השבר מהשבר עם השבר הקטן ואם ישוה לגדול צדקת | |
|
הרי קבצנו הי"ד חלקים ממ"ח עם הג' שמניות ועלה ד' ששיות | |
|
|
||
| Another scale: subtract the remainder from the greater fraction, then if the remainder is equal to the smaller [fraction], know that you were right. | מאזנים אחרים חסר הנשאר מהשבר הגדול והנשאר אם ישוה לקטן דע שצדקת | |
|
במשלינו זה חסרנו הארבעה עשר חלקים [8]ממ"ח מד' ששיות ישארו ק"ח חלקים מרפ"ח והם הם הג' שמיניות | |
|
והמופת שתסדרם ככה | |
| ||
|
ותכה האלכסונות ויהיו שוים | |
| It is enough for the one who understands. | ודי למבין | |
|
|
||
| Another scale: subtract the remainder from the greater fraction, then if the remainder is equal to the smaller [fraction], know that you were right. | מאזנים אחרים חסר הנשאר מהשבר הגדול והנשאר אם שוה לקטן דע שצדקת | |
|
במשלינו זה חסרנו הי"ד חלקים ממ"ח מד' ששיות ישארו ק"ח חלקים מרפ"ח והם הם הו' שמיניות |
Combined Subtraction |
| If you want to subtract numerous fractions from numerous fractions without having to sum up first and then subtract, you can use the following method: | ואולם אם רצית לחסר שברים רבים משברים רבים מבלתי שתצטרך לקבץ תחלה ואח"כ לחסרם הנה תוכל להשתמש בזה הדרך | ||
|
|
| ||
|
תכה כל האיכיות זה בזה יעלה 5040 | ||
|
ותכה כמות השבר הנחסר עם כל איכיות זולת איכותו וכן כל איכות הכמות מהנחסר יעלה 7980 | ||
|
וכן תעשה לכל הכמיות אשר יוחסרו השברים מהם ויעלה ח' אלפים ותתקנ"ב | ||
|
ותחסר מהם הז' אלפים ותתק"ף ישארו תתקע"ב והם תתקע"ב חלקים מה' אלפים ומ' |
| If you want to know the sum of all the remainders from the subtractions of numerous fractions from numerous fractions without having to subtract first and then sum up. | ואולם אם רצית לדעת העולה מכל הנשארים מחסרונו' שברים רבים משברים רבים מבלתי שתצטרך לחסרם תחלה ואח"כ לקבצם | ||
|
לחסר החצי מב' שלישיות וג' רביעיות מד' חומשיות | ||
| |||
|
נכה האיכיות יעלו ק"כ | ||
|
נכה כמות השבר הנחסר מהראשון עם כל האיכיות חוץ מאיכותו והם ס' | ||
|
גם נכה כמות השבר הנחסר מהב' עם כל האיכיות זולת אכותו והם צ' | ||
|
קבצם עם הס' הם ק"נ | ||
|
אח"כ הכה השבר הגדול אשר ממנו יחוסר הקטן מהמין הראשון עם כל איכות זולת מאיכותו וכן כמות השבר הגדול אשר ממנו ויחוסר הקטן מהמין הב' וקבצהו עם הראשון והם קע"ו | ||
|
נחסר מהם הק"נ וישארו כ"ו והם כ"ו חלקים מק"נ |
| If you want to know the remainder from the subtraction of products of fractions by fractions without having to know the products of these fractions. | ואולם אם רצית לדעת הנשאר מחסור העולה מהכאת השברים אחדים עם שברים מבלתי שתצטרך לדעת העולה מהכאת השברים ההם | ||
|
כמו עד"מ רצית לדעת מחיסור העולה מהכאת החצי עם ב' שלישיות מהעולה מהכאת הג' רביעיות עם ד' חומשיות מבלתי שתצטרך להכות החצי עם הב' שלישיות והג' רביעיות עם הד' חומשיות | ||
|
הנה יסודרו בזה הדרך | ||
| |||
|
נכה כל האיכיו' והם ק"כ | ||
|
ונכה השברים הנחסרים הכמות עם הכמות והעולה עם איכות השברים הגדולים אשר יוחסרו הקטנים מהם והם מ' | ||
|
ונכה כמות השברים הגדולים הכמות עם הכמות והעולה עם כל איכיות השברים הקטנים והם ע"ב | ||
|
נחסר מהם מ' ישארו ל"ב והם ל"ב חלקים מק"כ | ||
|
|
|||
| If you want to know the product of the remainder from the subtraction of some fractions from some fractions by the remainder from the subtraction of some fractions from some fractions without having to know the remainders from these subtractions at all. | ואולם אם רצית לדעת העול' מהכאת הנשאר מחיסור שברים מה משברים מה עם הנשאר מחיסור השברים מה משברים מה מבלתי שתצטרך לדעת הנשארים מהחסורים ההם כלל | ||
|
כמו עד"מ אם רצית לדעת העולה מהכאת הנשאר מחסור החצי מב' שלישיו' עם הנשאר מחיסור הג' רביעיות מהד' חמשיות | ||
|
הנה יסודרו ע"ז הדרך | ||
| |||
|
נכה האיכיות והם ק"כ | ||
|
אח"ז נחסר העולה מהכאת אלכסון א"ג מהכאת אלכסון ב"ב והנשאר נשמרהו | ||
|
גם נחס' העול' מהכאת אלכסון ג"ה מהעולה מהכאת אלכסון ד"ד והנשאר נשמרהו | ||
|
ואח"ז נכה הנשאר עם הנשאר וההווה נייחסהו אל השמור הראשון וההוה הוא העולה מהכאת הנשאר מחסור החצי מהב' שלישיו' עם הנשאר מחסור הג' רביעיות מד' חמשיות |
| If you want to know the remainder from the subtraction of the sum of products of fractions from the sum of products of fractions without having to multiply, then sum, then subtract, but to apply all three at the same time. | ואולם אם רצית לדעת הנשאר מחסור העולה מקבוץ העולה מהכאות שברים מהעולה מקבוץ העולים מהכאת שברים מה מבלתי שתצטרך להכות ואח"ז לקבץ ואח"ז לחסר אבל יצאו לך שלשתן בפעם אחת | ||||
|
עד"מ רצית להכות חצי עם ב' שלישיו' וג' רביעיות עם ד' חמשיות וג' שביעיות עם ד' חמשיות וב' שלישיות עם ג' רביעיות ואח"ז לקבץ העולה מהכאת החצי עם הב' שלישיות עם העולה מהכאת ג' רביעיות עם ד' חמשיו' והעולה מהכאת הג' שביעיות עם ד' חמשיות עם העולה מהכאת הב' שלישיות עם הרביע אח"ז לחסר ההוה מהקבוץ מההוה מהקבוץ לדעת הנשאר | ||||
|
הנה יסודרו על זה הסדר | ||||
| |||||
|
נכה כל האיכיות והם נ' אלף ות' | ||||
|
נכה כמות הב' שברים הראשונים זה עם זה והעולה עם כל האיכיות על הסדר הזה חוץ מאיכיותיו והם י"ו אלף ות"ת | ||||
|
גם נכה כמות הב' שברים השניי' העול' עם כל האכיות על הסדר חוץ אכיותיו והם ל' אלף ור"מ | ||||
|
ונקבצם עם י"ו אלפים ות"ת והם מ"ז אלף ומ' ונשמרם | ||||
|
אח"ז נכה הב' שברים מהשלישיי' זה עם זה והעולה עם האכיות כולם חוץ מאכיותיהם והם י"ז אלף ור"פ | ||||
|
גם נכה כמות הב' שברים הרביעיים זה עם זה והעולה עם כל האיכיות חוץ מאכיותיהן והם ח' אלפים [9]ות' | ||||
|
נקבצם עם הי' אלף ור"פ והם כ"ה אלף ותר"פ | ||||
|
נחסרם מהשמור הב' וישארו כ"א אלף ש"ס והם כ"א אלף ש"ס מנ' אלף ות"ת |
Division |
|
Dividing a large number by a smaller number |
|
| When you wish to divide a certain amount among people, giving each one twice as much as his friend. | כאשר תרצה לחלק מספר מה אל אנשים מה ולתת לכל אח' כפל חבירו |
|
כגון שתרצה לחלק ק' אל ד' אנשים ולתת לכל אחד כפל חבירו |
|
ככה תעשה דע א' כמה יתחבר וזה שחלק הא' נקחהו כמו שהוא אח' והחלק ב' הרי ג' והחלק ג' ד' הרי ז' והחלק הד' ח' הרי ט"ו |
|
חלק ק' על ט"ו והיוצא הוא חלק הראשון |
|
ולדעת הב' כפלהו בו |
| And so on. | כן לעולם |
| From this you understand all the questions of this type. | ומזה תבין לכל השאלות שבזה המין |
| Understand this. | והבן |
Dividing a small number by a greater number |
|
| To divide a small number by a greater number, divide the greater by the smaller. | לחלק מספר מעט על מספר רב תחלק הרב על המעט |
| If it is divided into integers no more and no less, the reduced result of division is the denominator of the quotient, which are all the required fractions of the integer together.
|
אם יתחלק לו לשלמים בלתי תוספת ומגרע' הנה היוצא בחלק בצמצום הוא מורה החלק אשר הם כל השברים הנשאלים יחד מהשלם |
|
ר"ל שאם יצא בחלוק ד' הנה יבא לו רביעית הא' |
| And the like. | ובדומה לזה |
| If it is not divided into integers, but a certain number remains, we add one to the [integer] resulting from the division, then subtract the remainder from the dividend and what remains are the parts of all the denominators.
|
ואם לא יתחלק כלו לשלמים וישאר שום מספר הנה נוסי' אח' ועלה יוצא בחלוק ונחסר הנשאר מהמספר אשר חלקו ועליו ומה שישא' הם חלקים מכל המורים |
|
המשל רצינו לחלק ז' על כ"ט |
|
נעשה ההפך נחלק הכ"ט על הז' יצא בחלוק ד' ובשביל שנשאר א' בלא נוסי' על הד' א' ויהיה ה' והוא ה' ונחסר הא' מז' ישארו ו' והם ו' חלקים מכ"ט בחמישית נוסף שהעלה חמישי' שיש בידינו |
| If you want to have more parts. | ואם תרצה שיצאו לך חלקים יותר |
|
חלק הכ"ט על הו' |
|
יצא בחלוק ד' וישארו ה' נוסי' א' על הד' ויהיו ה' ונחסר הה' אל הו' כאמור וישאר א' והעולה הוא ה' א' וחמישית ה' א' וחלק מכ"ט מחמישית חמישית אחד |
|
וזו צורתם | ||
| |||
| The proof is by changing the denominator.
|
והמופת שנעשם פריטה | ||
|
שתכה הא' בה' ותשא מה שעל ראש' ותכה הכל עם הכ"ט ותשא מה שעל ראשם ותחלק הכל לכל המורים זולת הכ"ט ויצא לך מספ' הז' שלם בלי תוספת ומגרעת | ||
| ואם לא יהיה במורים המספר אשר תרצה לחלק עליו המספר האחד | |||
|
כגון אם רצית לחלק ו' לכ"ז שיבא לו חמישי' אח' בכ"ז ותחלקהו על כל המורי' זולתו ויצא לך בשוה המספר | ||
Division of Fractions |
מין החלוק מן השברים | ||
|
|
|||
|
רצית לחלק ג' רביעיות על שני חמישיות | ||
| |||
|
הכה הב' עם הד' יהיו ח' | ||
|
אח"כ הכה הג' עם הה' יהיו ט"ו | ||
|
חלק הט"ו על הח' יצא א' שלם הרי יצא לנו שני חמישיות ונשארו ז' | ||
|
יחסם על הכ' שהו' הכאת האיכות עם האיכות יעלה ז' מכ' | ||
|
|
|||
|
אח"כ חלקם על החומש ויצא חומש אח' וג' רביעיות חומש | ||
|
חברהו עם הב' חמישיות והיוצא הוא המבוקש והוא ג' חמישיות וג' רביעיות החומש האח' | ||
|
|
|||
|
דרך אחר נכה הג' עם הה' כמות המחלק עם איכות המחלק יעלה ט"ו | ||
|
והכה הט"ו עם איכות המחלק שהוא הה' יעלה ע"ה וזהו המחולק | ||
|
אח"כ נכה האיכיות והם כ' | ||
|
נחלק עליהם הע"ה יצאו ג' וג' רביעיות | ||
|
ולהיות שהמחולק הוא חמישיות ידענו שהג' וג' רביעיות חמשיות וג' רביעיות החומש | ||
|
|
|||
|
דרך אחר נכה כמות המחולק עם איכות המחלק יהיו ט"ו | ||
|
נחלקם על איכות המחולק יצאו ג' וג' רביעיות | ||
|
ולהיות שהמחול' הוא חמישי' ידענו שהג' וג' רביעיות הם ג' חמשיות וג' רביעיות החומש | ||
Methods of checking |
מאזנים | ||
|
תכה החלק עם המחלק אם הוא שוה למחולק צדקת | ||
|
במשלינו זה הכינו הג' וג' רביעיו' עם החמישי' ועלה ג' רביעיות והוא הוא בעצמו המחולק | ||
|
או נכה א' וז' שמיניות עם ב' חמישיות ויעלה בעצמו הג' רביעיות | ||
|
וזה שא' וז' שמיניות כשיוחס אל הב' חמישיות שהם א' וז' שמיניות כמותם ר"ל הא' הוא ב' חמישיות וז' שמיניו' הם ז' שמיניות של ב' חמישיו' שהוא חומש א' וג' רביעיו' החומש עם הב' חומשים שבידינו הם ג' רביעיות וג' רביעיו' החומש | ||
|
וא"כ הכל שוה לאמרנו ג' וג' רביעיות ויחסנו אותם לחומש או א' וז' שמיניות ויחסנו אותם לב' חמישיו' | ||
| It is clear to the intellect. Understand this. | וזה מבואר בעין השכל והבן | ||
|
מאזנים אחרים שתמצא המספ' המתיחס כמות המחלק אליו יחס כמות המחלק אל איכותו ואם המספר ההוא מתיחס אל איכות המחולק יחס כמו' החלק אל איכותו דע שצדקת | ||
|
במשלינו זה חלקנו הג' רביעיות על הב' חמשיות ויצא א' וז' שמיניות שהם ט"ו שמיניות | ||
|
בקשנו מספר שתיחס כמות המחולק שהוא הג' אליו יחס כמות המחלק אל איכותו שהוא הב' אל הה' ומצאנו שהוא ז' וחצי | ||
|
והנה יחס הז' וחצי אל הד' שהוא איכות המחולק כיחס כמות החלק שהוא הט"ו אל איכותו שהוא ח' | ||
| It is clear to the intellect. | וזה מבואר בעין השכל | ||
|
וג"כ אם תרצה לעשותו בזה הדרך בג' וג' רביעיות ותבקש ביחס החמישית לבד גם עשה תעשה ויכול תוכל[note 1] כי הכל דבר א' כמו שביארנו וגלינו | ||
|
ואם תרצה לעשות המאזנים בהפך והוא שתראה המסופר שיהיה יחס כמות המחולק אליו כיחס כמות המחלק אל איכותו [10]ואם המספר ההוא מת[ייחס] אל איכות המחולק כיחס כמות המחלק אל איכותו ידענו שצדקנו | ||
|
במשלינו זה בקשנו מספר שיהיה יחס כמות מחולק אליו שהוא ג' כיחס כמות החלק אל איכותו שהוא הט"ו עם הח' ומצאנו שהוא א' וג' חמישיות שהם ח' שמיניות | ||
|
ויחסם אל הד' הוא כיחס הב' אל הה' שהוא כמות המחלק אל אכותו | ||
| Understand this, for it is absolutely clear. | והבן זה מאד כי הובן בתכלית | ||
Combined Division |
|||
|
|
|||
|
ואולם אם רצית לחלק קבוץ הב' שלישיות וג' רביעיו' על קבוץ שני שביעיות וששית מבלתי שתצטרך לקבץ תחלה ואח"כ לחל' | ||
|
הנה יסודרו על זה הדרך | ||
| |||
|
ונכה איכות השבר הראשון עם איכות השבר הב' והעולה עם איכות הג' והעולה עם כמו' הד' והם פ"ד | ||
|
גם נכה איכות השבר הראשון עם איכות השבר הב' והעול' עם כמות הג' והעול' עם איכות הד' והם קמ"ד ונשמרם | ||
|
נחברם אל הפ"ד והם רכ"ח ונשמרם | ||
|
אח"ז נכה איכות הד' עם איכות הג' והעולה עם אכות הב' והעולה עם כמות הראשון והם של"ו | ||
|
גם נכה איכות הרביעי עם איכות הג' והעולה עם כמות הב' והעולה עם איכות הראשו' והם שע"ח | ||
|
חברם אל השל"ו הם תשי"ד | ||
|
נחלקם על הרכ"ח השמורים והיוצא הוא ההווה מחלוק הב' שלישיו' וג' רביעיו' על הב' שביעיות וששית | ||
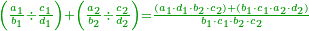
|
|||
|
ואולם אם רצית לקבץ היוצא מחלוק הג' רביעיות על הב' שלישיות עם היוצא מחלוק הב' שביעיו' על הו' מבלתי שתצטרך לחלק תחלה ואח"כ לקבץ | ||
|
נכה כמות השבר הראשון עם איכות הב' ועם איכות הג' ועם כמות הד' והם נ"ו ונשמרם | ||
|
אח"ז נכה כמות השבר הראשון עם איכות הב' וכמות הג' ואיכות הד' והם צ"ו | ||
|
גם נכה כמות השבר הד' עם איכות הג' וכמות הב' ואיכות הראשו' והם ס"ג | ||
|
נחברם אל הצ"ו והם קנ"ט | ||
|
נחלקם על הנ"ו השמורים והיוצא הוא ב' שלמים ומ"ה חלקים מנ"ו והוא המבוקש מקבוץ היוצאים מחלוק הג' רביעיות על הב' שלישיו' ומחלוק הב' שביעיו' על הו' | ||
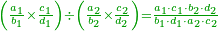
|
|||
|
ואולם אם רצית לחלק העולה מהכאת הב' שלישיו' עם הג' רביעי' על העולה מהכאת הב' שביעיו' עם הששי' מבלתי שתצטר' להכות תחלה ואח"כ לחלק | ||
|
נכה כמות הראשו' עם הב' והעול' עם איכות הג' ואיכות הד' והם רל"ב ונשמרם | ||
|
אח"ז נכה כמות השבר הד' עם כמו' השבר הג' והעולה עם איכות הראשו' והב' והם כ"ד | ||
|
תחלק עליהם הרל"ב והיוצא הוא ההווה מחלוק העולה מהכאת הב' שלישיו' עם הג' רביעיו' על העולה מהכאת הב' שביעי' על הו' | ||
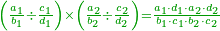
|
|||
|
ואולם אם רצית להכות היוצא מחלוק הג' רביעיות על הב' שלישיות עם היוצא מחלוק הב' שביעית על הו' מבלתי שתצטרך לחלק תחלה ואח"כ להכות | ||
|
נכה כמות הראשון עם איכות הב' ואיכות הג' וכמות הד' והם נ"ו ונשמרם | ||
|
אח"ז נכה האכות הראשון עם כמות הב' ועם כמות הג' ועם איכות הד' והם ק"ח | ||
|
נחלקם על הנ"ו השמורים והיוצא הוא ההווה מהכאת היוצא מחלוק הג' רביעיו' על הב' שלישיו' עם היוצא מחלוק הב' שביעיו' על הששית | ||
![\scriptstyle{\color{OliveGreen}{\left(\frac{a_1}{b_1}-\frac{c_1}{d_1}\right)\div\left(\frac{a_2}{b_2}-\frac{c_2}{d_2}\right)=\frac{\left[\left(a_1\sdot d_1\right)-\left(c_1\sdot b_1\right)\right]\times\left(b_2\sdot d_2\right)}{\left[\left(a_2\sdot d_2\right)-\left(c_2\sdot b_2\right)\right]\times\left(b_1\sdot d_1\right)}}}](/mediawiki/images/math/d/e/1/de19fec67919b87194e6104febf0c88d.png)
|
|||
|
ואולם אם רצית לחלק הנשאר מחצור הב' שלישיות מג' רביעיות על הנשאר מחסור הששית מהב' שביעיות מבלתי שתצטרך לחסר ראשונה ואח"כ לחלק | ||
|
נכה כמות הראשון עם איכות הב' והעולה נחסרהו מהכאת כמות השני עם איכות הראשון והנשאר נכהו עם איכות הג' ואיכות הד' והם מ"ב | ||
|
אח"ז נכה כמות הד' עם איכות הג' והעולה נחסרהו מהעולה מהכאת כמות הג' עם איכות הד' והנשאר נכהו עם איכות הב' ועם איכות הראשון והם ס' | ||
|
נחלקם על המ"ב השמורים והיוצא הוא ההווה מחלוק הנשאר מחסור הב' שלישיו' מהג' רביעיות על הנשאר מחיסור הו' מהב' שביעיות | ||
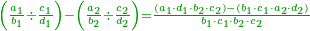
|
|||
|
ואולם אם רצית לחסר היוצא מחלוק הג' רביעיו' על הב' שלישיו' מהיוצא מחילוק הב' שביעיו' על הו' מבלתי שתצטרך לחלק ראשונה ואח"כ לחסרם | ||
|
נכה כמות הראשון עם איכות הב' ואיכות הג' וכמו' הד' והם נ"ו וזהו השמור הראשון | ||
|
אח"כ נכה איכות הראשון עם הב' והעולה עם איכות הג' ועם איכות הד' והם ס"ג ונשמרם וזהו השמור הב' | ||
|
אח"ז נכה איכות הד' עם כמות הג' ועם איכות הב' ועם כמות הראשו' והם צ"ו | ||
|
נחסר מהם הס"ג שהוא השמור הב' והנשאר שהוא הל"ג | ||
|
ניחסם אל השמור הראשון שהוא הנ"ו וההווה הוא ההוה מחסור היוצא מחלוק הג' רביעיות על הב' שלישיות מהיוצא מחלוק הב' שביעיות על הששית | ||
| וש'מ'ל' |
Roots |
|||||||||||
The Roots of Integers |
גדר השלמים | ||||||||||
| Description of the extraction procedure: | |||||||||||
| We arrange the required number in a line each [digit] according to its rank. | נסדר המספר הדרוש בטור אח' כל אח' לפי מדרגתו | ||||||||||
| If the number of ranks is odd, we start from the last rank. | ואם המספר המדרגות נפרדות נתחיל מהמדרגה האחרונה | ||||||||||
| If its is even, we start from the rank that precedes the last rank, while considering the last rank as tens and the one that precedes it as units. | ואם זוג נתחיל ממדרגה הקודמת למדרגה האחרונה בעוד המדרגה האחרונה שתחשוב המדרגה האחרונה לעשרות והקודמת לה לאחדים | ||||||||||
| We seek for the closest root and write it beneath the required rank, i.e. the last or the one that precedes it; it is called the first root.
|
ונבקש שרש הקרוב ונכתבהו תחת המדרגה הנדרשת ר"ל האחרונה או הקודמת לה ויקרא השרש הא' | ||||||||||
| Then, we seek for a number, such that twice its product by the first root plus once by itself is enough to be subtracted from the rank that precedes the rank of the first root and from the one that precedes it; it is called the second root.
|
אח"ז נבק' [11]מספר שיספיק העולה מהכאתו עם השורש הראשון פעמים ועם עצמו אחת שיחוסר מהמדרגה הקודמת למדרגת השרש הראשון ומהקודמת לה ויקרא השרש הב' | ||||||||||
| We write it in the rank that precedes the [rank] of the first root. | ונכתבהו במדרגה הקודמת לשרש הראשון | ||||||||||
| We further seek for a number, such that twice its product by the first and the second roots plus once by itself is enough to be subtracted from the rank that precedes [the rank of] the second root and from the two that precede it; it is called the third root. | עוד אח"ז נבקש מספר שיספיק העולה מהעולה מהכאתו עם השרש הא' והב' פעמים ועם עצמו פעם אחת שיחוסר מהמדרגה הקודמת לשרש הב' ומהשתים הקודמת לה ויקרא השרש הג' | ||||||||||
| We write it in the rank that precedes the [rank] of the second root. | כתבנהו במדרגה הקודמת לשרש הב' | ||||||||||
| We seek again for a number, such that twice its product by the first, the second, and the third roots plus once by itself is enough to be subtracted from the rank that precedes [the rank of the third root] and from the three that precede it; it is called the fourth root. | עוד אח"ז תבקש מספר שיספיק העולה מהכאתו עם השרש הא' והב' והג' פעמים ועם עצמו פעם שיחוסר מהמדרגה הקודמת ומהג' הקודמת לה ויקרא שרש ד' | ||||||||||
| We write it in the rank that precedes the [rank] of the third root. | וכתבנוהו במדרגה הקודמת לשרש הג' | ||||||||||
| And so on always until the subtracted rank is the first rank. | וכן תמיד עד אשר תגיע שיהיה המדרג' הנחסרת המדרגה הראשונה | ||||||||||
| The numbers that are written beneath the ranks are themselves the root. | והמספרים הכתובים תחת המדרגות הם השרש בעצמם | ||||||||||
| But, if there is a remainder from the required number that cannot be divided, know that the required number does not have a root. | ואולם אם נשאר מהמספר הדרוש בלתי מתחלק דע שהמספר הדרוש הוא בלתי נגדר | ||||||||||
Approximating the root of a non-square number |
|||||||||||
| The way of knowing the approximate root is that you add to the required number whatever even number of zeros you wish, then apply this same procedure itself, until you come to subtract from the first rank, as I write: | והדרך בידיעת שרש הקרו' הוא זה שתוסיף על המספר הדרוש איזה זוג תפרש שתרצה ותנהיג זה הדרך בעצמו עד שתגיע לחסר מהמדרג' הראשונה כזה שאני כותב | ||||||||||
Exammple: 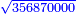
|
|
| Then, multiply the result by sixty and again by sixty, until the number of zeros at the beginning of the product is half the number of the zeros that were added, and the denominator of the result is the number of times that it is multiplied by 60.
|
אח"ז תכה היוצא בששים והעולה בששים עד שיהיו תפארש שבראש טור העולה בהכאת כמות חצי התפראש הנוספות והעולה יהיה איכותו במספר הפעמים שהוכה בס' |
| I.e. if it was multiplied once, they are minutes.
|
ר"ל אם הוכה פעם אחת הם ראשונים |
| If twice, they are seconds.
|
ואם ב' פעמים הם שניים |
| If three times, they are thirds.
|
ואם ג' פעמים הם שלישים |
| If four, they are fourths.
|
ואם ארבעה הם רביעים |
| Then, erase the zeros [that were added in the first step] from the resulting number and divide what remains by sixty; the result is raised by one rank. | אח"ז השלך מהמספר העולה מהמספר הכאת הספראש והנשאר תחלקהו על ששים והיוצא יעלה מדרגה א' |
| I.e. if they are fourths, the result is thirds and the remainder that cannot be divided are fourths. Keep them.
|
ר"ל שאם היו רביעיי' היוצא היה שלישים והנשארים בלתי נחלקם הם רביעיים ושמרם |
| Divide also the result of division, which are thirds, by 60; the result are seconds and the remainder that cannot be divided are thirds. Keep them.
|
עוד חלק היוצא מהחלוק שהם שלישים על ס' והיוצאים בחילוק הם שניים והנשארים בלתי נחלקים הם שלישיים ושמרם |
| Divide also the result of division, which are seconds, by 60; the result are minutes and the remainder that cannot be divided are seconds. Keep them.
|
עוד חלק היוצאים בחלוק שהם ב' על ס' והיוצא בחלוק הם ראשונים הנשארים בלתי נחלקי' הם שניים ושמרם |
| Then, divide the minutes by 60; the result are degrees, i.e. integers and the remainder are minutes. So, the result are degrees, minutes, seconds, thirds, and fourths.
|
אח"ז חלק הראשונים על ס' והיוצא הם מעלות ר"ל שלמים והנשארים הם ראשונים וככה הוא היוצא מהמעלות והראשונים והשניים והשלישים והרביעיים |
The Roots of Fractions |
שרש השברים |
| If its numerator and its denominator have a root, take the root of its numerator and relate it to the root of its denominator.
|
אם היה כמותם ואכותם נגדר תקח גדר כמותם ותיחסהו לגדר אכותם |
|
כגון ד' תשעיות |
|
גדר הד' שנים וגדר הט' ג' א"כ גדר הד' תשעיות הם שני שלישיות |
| If its numerator and its denominator do not have a root, or only its denominator, multiply the denominator by itself, then multiply the numerator by the denominator and relate it to the first product. | ואם אכותם וכמותם בלתי נגדר או איכותם לבד תכה האיכות בעצמו ותכה הכמות עם האיכות ותיחסהו לההכאה הראשונה |
| Apply the same method: take the root of the numerator, i.e. the product of the numerator by the denominator, if it has an integer root, or approximately, if it does not have an integer root, and relate it to the root of the product of the denominator by the denominator and this is the approximate root.
|
ותעשה זה הדרך בעצמו שתקח גדר הכמות ר"ל שהוא הכאת הכמות עם האיכות שהוא כמות הכאת האיכות עם האיכות אם יש לו גדר שלם או על דרך קרוב אם אין לו גדר שלם ותיחסהו לגדר העולה מהכאת האיכות עם האיכות וזהו השרש הקרוב |
| If its numerator alone does not have a root and its denominator has a root, take the approximate root of the numerator and relate it to the denominator.
|
ואם כמותם לבד בלתי נגדר ואיכותם הוא המספר הנגדר קח שרש הקרוב לכמות ותיחסהו אל האיכות |
| If there are integers and fractions, decompose the integers to fractions, then relate [the numerator] to the denominator according the same preceding method. | ואם היה שלמים ושברים תתיך השלמים עד שיהו שברים ותיחסם אל האיכות על דרך בעינו שהקדמנו |
Cubic Roots |
|
Cubic Roots of Integers |
מעוקב השלמים |
| Description of the extraction procedure: | |
| Arrange the required line each according to its rank. | תסדר הטור הדרוש כל אח' כפי מדרגתו |
| After every three digits draw a line. | ובסוף כל ג' אותיות תשים קו |
| Beggin from the last line [= the leftmost dividing line]. | ותתחיל מהקו האחרון |
| We write there a number to be cubed and subtracted from the number that is above it and its preceding ranks. It is called the first cube root.
|
ונכתוב שם מספר שיוכה בדרך עקוב ויחוסר מהמספר שעליו עם עזר המדרגות הקודמו' ויקרא היסוד הראשון |
| Then, write a second cube root at the place of the preceding line, to be multiplied by the product of the first [cube root] by 3 multiplied by the product of the first and the second cube roots together, so that the result is subtracted from the second digit and its preceding digits. Cube also the second cube root and subtract the product from the number that is above it and its preceding digits. | אח"ז תכתוב במקום הקו הקודמת יסוד ב' ושיוכה עם העולה מהכאת הראשון בג' והעול' עם היסוד הראשון והב' יחד והעולה שיחוסר מהמספר שני לו עם עזר הנמשכים לו גם תכה היסוד הב' באופ' מעוקב והעולה תחסרהו מהמספר שעליו עם עזר המספרים הקודמים לו |
| Then, we write a third cube root at the place of the preceding line, to be multiplied by the product of the first and second [cube roots] together by 3 multiplied by the product of the first the second and the third cube roots together, so that [the result] is subtracted from the second [digit] and its preceding digits. We also cube the third cube root and subtract the product from the number that is above it and its preceding digits. | אח"ז נכתו' במקום הקו הקודמת יסו' ג' שיוכה עם העולה מהכאת הראשון והב' יחד עם מספר הג' והעולה עם היסוד הראשון והב' והג' ויחוס' מהמרב' הב' עם עזר המדרגות הנמשכים לו גם נכה היסוד הג' באופן מעוקב והעולה נחסרהו מן המספר שעליו עם עזר המספרים הקודמים לו |
| And so on in that order. | וכן תמיד בסדר הזה |
Approximating the root of a non-cubic number |
|
| When we reach the rank of units, but there are digits that are still left of the required number and you want to know its approximate cube root, we add to the ranks that precede the units three zeroes or six, or nine, or as many as you wish, as long as you add them in threes. | וכאשר הגענו אל מדרגת האחדים ממנו ונשארו מספרים על המספר הדרוש ותרצה לדעת עוד יסודו הקרוב נוסיף על המדרגו' המונחות מצד האחדים ג' סיפראש או ששה או ט' או מה שתרצה לבד שתוסיפהו ג'ג' |
| Then, we apply the preceding method for finding the cube root until reaching the first rank of the zeroes. | ואח"ז נעשה מה שקד' מהדרך למציאות היסוד עד שתגיע למדרגה הראשונה מהסיפראש |
| We take all the cube roots, arrange them in one line according the ranks, and erase one third of the number of zeros [added in the first step]. | אח"ז נקח כל היסודות ונסדרם בטור אח' על סדר המדרגות ונשליך מהם במספר שליש הסיפראש |
| We take the integer cube roots; they are degrees. | [12]ונקח היסודות האחדות והם מעלות |
| Then, we multiply what remains by 60, erase three zeros from [the product] and take the remainder; they are minutes. | אח"ז נכה הנשלכים בס' והעולה נשליך מהם כמספר ג' הסיפראס ונקח הנשארים והם ראשונים |
| We proceed like this until all the zeros [added in the first step] are erased. | וכן נעשה תמיד עד שיכלה הסיפראש |
| Then, we sum up all and it is the approximate cubic root. | ואז נחבר הכל יהיה הוא היסוד הקרוב |
| Another method for a number that has no [integer] cube root | דרך אחר למספר שאין בו יסוד מעוקב |
|---|---|
| Add zeros as many as you wish, as long as they are added in threes. | שתוסיף סיפראש כמה שתרצה ובלבד שיהיו נוספים בתוספת ג'ג' |
| We apply the previous procedure until reaching the first zero. | ונשתמש עם הדרך הקודם בעינו עד שנגיע לסיפרא הראשונה |
| Afterwards, we take all the cube roots that are arranged beneath the required number, multiply them by 60, then the product by 60, and so on until the number of the zeroes that appear at the beginning of the resulting line is a third of the number of the zeroes [added in the first step]. | אח"ז נקח כל היסודות המסודרות תחת מספר הדרוש ונכהו בס' והעולה בס' וזה עד שתהיינ' הסיפראש היוצאות בראש הטור העולה מההכאות בכמות שליש הסיפראש |
| We erase the zeros [added in the first step] and divide the result by 60. The remainder is [of the type] of the denominator of the divided fractions. | אח"ז נשליך הסיפראש ונחלק הנשאר על ס' והנשאר יהיה מאיכות השברים המחולקים |
| I.e. if it was multiplied only once, they are minutes.
|
ר"ל שאם הוכו פעם אחת לבד יהיו ראשונים |
| If twice, they are seconds.
|
ואם ב' שניים |
| If three [times], they are thirds.
|
ואם ג' שלישיים |
| The result is of the type that precedes the type of the dividend, i.e. if they were minutes, the result of division are degrees; if they were seconds, the result of division are minutes; and so on. | והיוצא הוא ממין הקודם למין ז' המחולקים ר"ל שאם היו ראשונים היוצא מהחלוקה מעלות ואם היו שניים היוצא מהחלוקה ראשונים וכן תמיד |
Cubic Roots of Fractions |
יסוד השברים המעוקבים |
| If the numerator and the denominator are cube numbers, take the cube [root] of the numerator and relate it to the cube [root] of the denominator.
|
אם הכמות והאיכות מעוקב תקח מעוקב הכמות ותיחסהו אל מעוקב האיכות |
| If they are not cube numbers, or the denominator alone is not a cube number, we cube the denominator and multiply the numerator by the product of the denominator by itself, then we relate the cube root of the second reserved, which is the product of the numerator by the product of the denominator by itself, to the first reserved, which is the cube of the denominator.
|
ואם אינם מעוקבים או האיכות לבד בלתי מעוקב נכה האיכות בדרך עקוב ונכה הכמות עם העולה מהכאת האיכות בעצמו אח"ז ניחס יסוד השמור הב' שהוא הכאת הכמות עם העולה מהכאת האיכות בעצמו אל השמור הראשון שהוא העולה מהכאת האיכות בדרך עקוב |
| If the denominator is a cube, we look for the approximate cube root of the numerator and relate it to the cube root of the denominator.
|
ואם איכותם נעקב נבקש יסוד הכמות הקרוב והיוצא ניחסהו אל מעוקב האיכות |
|
ר"ל שאם היה מעוקבו ג' היה היחס הוא השליש וכן תמי' |
| If there are integers and fractions together, we decompose the integers to fractions, then proceed according to the previous method. | ואולם בשלמים ושברים יחד נתיך השלמים לשברים ונעשה הדרך הקודם בעינו |
Divisibility of a Number |
|
3; 6; 9 |
|
| To know if a certain number has a third, a sixth, or a ninth without fractions [= if 3, 6, or 9 are divisors of the number]: | לידע אם יש שלישי' או ששי' או ט' מספר מה מבלי שברים |
| First, you should know if it has a ninth, and this is known by summing the numerals of the number as if they were units. | תדע ראשונה אם יש לו תשיעי' וזה יודע כשתחבר רשמי מספר החשבון כאלו הם אחדים |
| If it is cast out by nines, it is known that is has a ninth, as well as a third [= divisible by 9 and 3]. | ואם יושלך לט"ט בידוע שיש לו תשיעיות וג"כ שלישי' |
| Also a sixth, if the number is even [= divisible by 6], but not if it is odd. | וג"כ ששית אם המספר זוג ואם נפרד לאו |
| If 6 or 3 remain, it has a third [= divisible by 3], and, if it is an even number, a sixth also [= divisible by 6], but not a ninth [= not divisible by 9]. | ואם ישארו ו' או ג' יהיה לו שלישי' וג"כ ששית אם הוא זוג אבל לא תשיעית |
2; 4; 8 |
|
| If you wish to know if it has a half, a quarter, or an eighth [= if 2, 4, or 8 are divisors of a the number]: | ואם תרצה לידע אם יש לו מחצית או ד' או שמינית |
| If the number is odd, it does not have any of them [= not divisible by 2, 4, or 8]. | אם חשבון נפרד אין לו שום אחד מהם |
| If it is even, it has a half [= divisible by 2]. | ואם זוג בידוע שיש לו חצי |
| To know if [it also has] a quarter and an eighth: | ולידע אם לא ג"כ רביעית ושמיני' |
|
קח המספר אשר במעלה הראשונה לצד ימין כמו שהיא |
|
ואשר בשנייה כפול ואם אין שם מספר לא תקח כלום |
|
ואשר בשלישית אם הוא נפרד כפלהו בד' ואם הוא זוג או סיפרא לא תקח כלום |
|
וכן מהמעלה הג' ולמעלה תניח אותו לא תקח כלום וקבץ כל מה שקבצת עד המעלה הג' עד"ז |
|
|
|
| If it is cast out by eights it has an eighth [= divisible by 8], the more so a quarter [= divisible by 4]. | אם יושלך באלו לח' יש לו שמינית וכ"ש רביעי' |
| If 4 remains, it has a quarter [= divisible by 4] alone. | ואם ישארו ד' יש לו ד' לבד |
| If another number remains, it does not have even a quarter [= not divisible by 4]. | ואם נותר מספר אחר אין לו אפי' רביעית |
7 |
|
| If you wish to know if it has a seventh [= if 7 is a divisor of a given number] | ואם תרצה לידע אם יש לו שביעי' |
| If has a few methods: | יש בזה דרכים |
|
הראשון שתניח אלו האותיות א'ג'ב'ו'ד'ה' על סדר המדרגות חלילה ותכה כל א' מאלו האותיות במדרגה שכנגדה וחשב כל האחדים והשלך השביעיות ואם יושלך לז' יש לו שביעית ואם לאו לאו |
|
הב' שתכה הרושם האחרון שלצד שמאל בג' וחברהו לאשר תמצא במעלה שלפניו ותשליכה לז' והנשאר תחברהו לאשר לפניו ותכה הנשאר מהשלכת השביעיו' בג' וחברהו לאשר תמצא לפניו וכן תמיד עד המדרגה הראשונה ואם יושלך ז'ז' יש לו שביעית |
|
ואם לא תמצא שם מספר כי אם סיפרא תכה הנשאר בג' וכן בכל ספרא וספרא תכה הנשאר בג' עד שיכלו כל סיפראים וכן תמיד |
11 |
|
| To know if it has an eleventh, i.e. if it is cast out by elevens [= if 11 is a divisor of a given number]: | ולידע אם לו י"א ר"ל אם יושלך לי"א |
| Subtract the leftmost [numeral] from the one that precedes it, then the remainder from the one that precedes the preceding and so on repeatedly. | תחסר האחרון שלצד שמאל מאשר לפניו והנשאר מאשר לפני פניו וכן תמיד |
| If all is cast out, it has an eleventh [= divisible by 11], otherwise it has none. | ואם יושלך הכל יש לו י"א ואם לאו לאו |
| If you do not find there a number, or if the digit is smaller than [the subtrahend], so that you cannot subtract from it, add 11 to it, then subtract from the total and so on [the procedure continues as described]. | ואם לא תמצא שם מספר או מספר קטן ממנו שלא תוכל לחס' ממנו והנשאר תוסיף עליו הי"א ותחסר מהכל זה המספר וכן תמיד |
13 |
|
| If it has a thirteenth [= if 13 is a divisor of a given number] | אם יש לו י"ג |
| Multiply the last numeral by 3, cast out the thirteens, subtract the remainder from what you find in the preceding rank, then multiply the remainder by 3 again, cast out the thirteens, subtract the remainder from what precedes it, and so on until their end. | תכה הרושם האחרון בג' והוציאוהו י"ג י"ג והנשאר הוציאוהו מאשר תמצא במעלה אשר לפניו והנשאר כפלהו שנית בג' והוציאוהו י"ג י"ג והנשאר הוציאוהו מאשר לפניו וכן תמיד עד תכליתם |
| If all is gone, it is cast out by thirteens [= divisible by 13]. | ואם יצא הכל יושלך לי"ג |
| If there is not enough in a certain rank, so that you do not find there enough to subtract as I have instructed you, add 13, then subtract from the sum what you should subtract, multiply the remainder by 3, cast it out by thirteens [the procedure continues as described] and if it is cast out, it has a thirteenth, otherwise it has none. | ואם יחסר בשום מעלה שלא תמצא שם די להוציא אשר צויתיך הוסיף י"ג והוציא מהנתחבר אשר עליך להוציא והנשאר כפלהו בג' והוציאוהו י"ג י"ג ואם יצא יש לו י"ג ואם לאו לאו |
General technique |
דרך כולל כל |
|---|---|
| Adding the leftmost digit to the second leftmost digit and casting out the [potential divisor] in question from the sum, then adding the remainder to the digit in the preceding rank as tens and casting out the [potential divisor] again from the sum, and so on | שתחבר המספר האחרון עם אשר לפניו בשתחברהו לעשרות והב' לאחדים ותשליכהו למספר אשר תרצה להשליכו והנשאר חשבהו לעשרות וחברהו לאשר תמצא במעלה שלפניו והוציאהו למספר אשר תרצה להוציא וכן תמיד על הסדר הזה |
Square Numbers |
|
| When you want to know the square of a number in an easy way: | כשתרצה לידע מרובע שום מספר ע"ד הקל |
| Take its third, multiply it by itself and rise it to the next rank - if it is in the units, the next rank is the tens; if it is in the tens, the next rank is the hundreds. Subtract from it the [square] of the third and the remainder is the square you wish to know.
|
קח שלישיתו והכהו בעצמו והעלהו למדרגה הקרובה אם היא אחדים המדרגה הקרובה היא העשרות ואם היא עשרות המדרגה הקרובה היא המאות וחסר מהם הכאת השליש ומה שישאר הוא המרובע שרצית לידע |
|
המשל אם תרצה לידע כמה הוא מרובע הט' |
|
קח שלישיתו והוא הג' והכה אותו בעצמו ויעלה ט' [13]עלהו למדרגה הקרובה והיא תשעים חסר מהם הכאת השליש והוא ט' ישאר א' ושמונים והוא מרובע הט' |
| If it does not have a third, but is one less than [having] a third, add one.
|
ואם יהיה חסר אחד לשליש שאין לו שליש הוסף א' |
|
המשל אם תרצה לידע מרובע הח' |
|
וכשתרצה לקחת השליש אין לו שליש הוסף אח' ויהיו ט' ושלישים הוא ג' הכם בעצמם כמו שאמרנו בזה המשל הקודם והעלהו למדרגה הקרובה ויהיה תשעים חסר מהם מרובע הג' ישאר פ"א ובשביל הא' שהוספת חסר הח' והט' שהוא י"ז מהפ"א ישארו ס"ד וככה מרובע השמונה |
| If it exceeds, meaning that it does not have a third, but an excess remains.
|
ואם היה בתוספת רצוני שאין לו שליש אלא שישאר תוספת |
|
כגון רצית לידע מרובע ז' |
|
וכשתרצה לקחת שלישיתו תעש' ע"ז הדרך שתחסר א' וישארו ששה שלישית הוא שניים עשה הדרך בעצמו שאמרנו ויעלה ל"ו אח"כ בשביל הא' שחסרת הוסיף הז' והששה הם י"ג עם ל"ו יעלה מ"ט וככה מרובע הז' |
Multiplicative Supplementing |
|
| Numerical operation called jaber in Arabic | מין מספר הנקרא בערבי ג'בר |
| I do not know its name in Hebrew. | ולא ידעתי לו שם בלשוננו |
| In foreign language [it is] reducir. | ובלע"ז רידוזיר |
| Its meaning is that if you have any number and you want to find by which number we multiply this fractionor these fractions, so that the resulting [product] will be that fraction or those fractions [= the complement number].
|
וענינו שאם יש לך שום מספ' ורצית לומ' באיזה מספר נכה זה השבר או אלו השברים עד שיעלה זה השבר או אלו השברים |
|
במשל ב' רביעיות באיזה מספר נכו אותם עד שישובו א' וד' חמישיו' |
| The method is that you divide this number by the specific fraction.
|
והדרך בזה שתחלק זה המספר על השבר המיוחד |
| The result, as explained in the division of integers and fractions by fractions, is called [in Arabic] al-majbur [= complement, multiplicative supplement]. | ומה שיעלה כמו שמבואר בחלוק השלמים ושברים על שברים הוא אלמגבור |
Decomposing to a Fraction |
|
| There is a numerical operation that is opposite to it, which is called bāb al-ḥaṭ [in Arabic]. | ויש מין אחר הפכי לזה והוא הנקרא באב אלחט |
|
והוא א' וארבע' חמישיות באיזה מספר נכה אותם עד שישובו ב' רביעיות שהוא חסרון בערך הראשון |
|
ותעשה ההפך שתחלק הב' רביעיו' על א' וד' חמישיות |
| [Indication that the treatise relies on another work:] Although the author wrote two chapters concerning these [two issues], it seems that they can be included in one chapter. | ואעפ"י שהמחבר חברם בב' שערים נ"ל שהם מכללו בשער אח' |
| Understand this. | והבן |
Geometric Shapes |
|
Square |
|
|
אם מרובע נכון שיש בצלעיו עשר על עשר ד"מ ורצית לידע שטחו או אלכסונו |
|
תעשה בזה הדרך |
|
תכה הצלע עם חבירו כאח' וזהו שטחו |
|
ותכה הב' צלעות כל אחת בפני עצמו וגדר של מקובצם הוא האלכסון |
|
ואם ידעת השטח גדרו והוא הצלע |
|
ואם ידעת האלכסון גדר חצי הכאתו הוא הצלע |
Rectangle |
|
|
אבל במרובע ארוך שיש לצלעיו י' באורך וה' ברוחב עד"מ |
|
בידיעת ב' צלעיו תכה זה עם זה כאחת זהו שטחו |
|
ובידיעת שטחו וצלע א' תחלק השטח על הצלע הידוע ויצא הצלע הנעלם |
|
ובידיעת ב' צלעיו ותכה זה בפני עצמו וזה בפני עצמו וגדר מקובצם הוא האלכסון |
|
ובידיע' האלכסון וצלע א' תוציא הכאת הצלע מהכאת האלכסון וגדר הנשאר הוא הצלע האחר |
On Measuring the Area of the Triangle |
בשבירת המשולש |
| There are many ways, but what seems to be more general is what is clear from the words of Euclid the Wise in one proposition and by applying this proposition we will generate the required by the help of God. | יש דרכים רבים אבל מה שנ"ר יותר כולל הוא מה שנתבא' בכח דברי אקדידס החכם מתוך הקדמה אח' ובקיום זאת ההקדמה נוליד המבוקש בע"ה |
| It is that it is explained there that [the square] of the diagonal of the rectangle is equal to the sum of the squares of both sides when each is multiplied by itself.
|
וזה ששם נתבאר שקוטר המרובע ר"ל אלכסונו הוא כפל מרובע ב' הצלעים כשיוכה זה בפני עצמו וזה בפני עצמו הוא שוה להכאת האלכסון בעצמו |
| From this proposition it is clear to you that when there is a triangle of this form: | ובזה ההקדמה יתבאר לך כשיש משולש בצורה הזאת |
|
|
|
| We agree that it is equilateral and each side is 10. | ונסכים שהוא שוה הג' הצלעות ושיש בכל צלע עשר |
| Finding its area is by that you divide the triangle in your thought into two identical triangles. | הנה ידיעת שבריו הוא שתחלקהו במחשבתך לב' משולשים שוים |
| This is by drawing a straight line from vertex A and this line necessarily divides the line, i.e. line GB, into two halves. | וזה שתרשום בזוית א' קו ישר ובהכרח יחלוק הקו ר"ל קו ג"ב לשני חצאין |
| So, we know that one side is 5 and the hypotenuse is ten as given. | ובזה הרי ידענו שצלע אחד יש בו ה' והאלכסון עשר כפי מה שהונח |
| The extracting the other side, following from the proposition we have presented, is by that you subtract the product of the side [by itself] from the product of the hypotenuse [by itself] and the square of the remainder is the other side.
|
ובהוצאת הצלע האח' יתחייב כפי ההקדמ' שהנחנו שתוציא הכאת הצלע מהכאת האלכסון וגדר הנשאר הוא הצלע האחר |
| The quality of the conclusion is clear since the product of the diagonal [by itself] is equal to the sum of the products of both sides, each by itself.
|
ואיכות החיוב מבואר כיון שהאלכסון בהכאתו שוה להכאת שני צלעיו כל אח' בפני עצמו |
| Therefore, it necessarily follows that when you subtract the product of one side [by itself] from [the product of] the diagonal [by itself], the root of the remainder is the [other side]. | א"כ יתחייב בהכרח כשתוציא הכאת הצלע האח' מהאלכסון שגדר הנשאר הוא האלכסו' |
|
ועשינו כן שהכינו הה' והיה כ"ה |
|
והכינו הי' והיה ק' |
|
חסרנו הכ"ה מהק' ונשארו ע"ה |
|
וגדרם הוא הצלע האחר |
|
בקשנו גדרם ע"ד הקירוב כמו שהודעתיך בשער הקידום הדרך היותו נקל כזו והיותר מדוייק ומצאנו ששרשם הוא הוא ח' וב' רביעיות וג' רביעיות רביעית |
|
הכינום עם ה' שהוא הצלע הא' ויצא מ"ג שלמים ורביעי' וג' רביעיות רביעית והוא שטח המשולש |
|
לפי שהאלכסון חולק המרובע לב' חצאים וחצי זהו חצי המשולש וחציו האחר הוא חצי המשולש ר"ל החלקים מהמשולש אשר חלקנו צלעו בזוית אח' |
| This is enough for the one who understands. | ודי למבין |
Euclidean Propositions |
|
Euclid's Elements, Book II, Propositions 2-8 - [Arithmetical Version] |
|
| Propositions that are of great need for arithmetic from the words of Euclid the wise in Book II and they are 9 propositions | הקדמות צריכין צורך גדול למספר מדברי אקלידס החכם במא' הב' והם ט' הקדמות |
|---|---|
|
אחת כל מספר שחלקת אותו לחלקים איך שרצית הנה הכאת כל אח' מהחלקי' בכל המספר השוה למרובע הכל |
|
המופת שאין בכלל זולת חלקיו והכאת הכלל בכלל הוא כהכאת הכלל בכל חלקיו וזה מבואר |
|
ב כל מספר שחלקת אותו לב' חלקים איך שקרה הנה הכאת המספר כלו עם כל אחד מב' חלקיו איזה שיהיה שוה להכאת החלק האחד עם האחר ולמרובע החלק אשר בו הכית כל המספר |
|
[14]מופתו כי הכלל כשהכית אותו עם איזה חלק הוא כאלו הכית אותו החלק בעצמו ועם החלק האחר כיון שזה הכלל מורכב מהם |
|
כמשל מספר הי' נחלק לב' חלקים לז' ולג' |
|
הנה הכאת הי' בז' כאלו הכית הז' בעצמו ועם |
|
וכן כאשר הכית הי' בג' הרי הוא כאלו הכית הג' עם הז' ועמו בעצמו כגון שהם ר"ל הג' והז' הם הם בעצמם מקובצים מספר הי' וזה מבואר מאד |
|
ג כל מספר שחלקת אותו לשני חלקים איך שקרה הנה מרובע כל המספר שוה לב' המרובעים ההווים מב' החלקים ולהכאת החלק האחד עם חבירו ב' פעמים |
|
מופתו כי הכאת הכלל עם הכלל כאלו הכית הב' חלקים עם הכלל והכאת החלק והא' עם הכלל הוא כאלו הכית החלק בעצמו ועם החלק האח' וג"כ בחלק האח' שהכית אותו בכלל כאלו הכית בעצמו ועם החלק הא' כיון שאין בכלל זולת חלקיו והרי אלו החלקים הם חלקי זה המספר הגדול |
|
כמשל הי' נחלק לז' ולג' |
|
הכאת הי' בעצמו הוא כאלו הכית הז' עם הי' והג' עם הי' |
|
הכאת הז' עם הי' כאלו הכית הז' בעצמו ועם הג' כיון שהז' והג' הם חלקי הי' |
|
והכאת הג' בי' כאלו הכית הג' בז' ועם הג' |
|
הרי ב' פעמים הכינו הג' עם הז' וג"כ הכינו הז' בעצמו והג' בעצמו ושוה הכל למרובע הי' ומבואר הוא מאד למסתכל בעין השכל |
|
ד כל מספר כאשר תחלקהו לב' חלקים שוים ולב' חלקים בלתי שוים הנה הכאת החלק הא' עם חבירו מהחלקים הבלתי שוים ומרוב' מה שבין ב' חלקים ר"ל בין החלק השוה ובלתי שוה שוה למרובע חצי המספר |
|
מופתו המספר שנחלק לב' חלקים בלתי שוים מה שנגרע מהמספר הקטן מהחצי הוא מיותר במספר הגדול על החצי |
|
במש' הי' שנחלק לז' ולג' |
|
מה שנגרע הג' מהה' שהוא ב' הז' יותר מהה' זה המספר בעצמו שהם הב' |
|
וא"כ כשאנו מכים המספר הקטן בגדול הוא כאלו הכינו המספר הקטן בחצי המספר והתוספת כיון שהתוספת והחצי הם חלקי החלק הגדול |
|
והכאת החצי עצמו הוא כאלו הכינו החצי עם המספר הקטן ועם התוספת כיון שהתוספת והמספר הקטן הם חלקי החצי |
|
והוא מבואר שההכא' בין הכאת החצי בתוספת בין הכאת המספר הקטן בתוספת הוא הכאת התוספת בתוספת וע"כ כהכאת התוספת בעצמו |
|
ואם נוסיפהו על מרובע הקטן בגדול הוא מרוב' החצי בהכאת |
|
במשל הכאת הז' בג' הוא כאלו הכינו הג' בה' ובב' |
|
והכאת הה' בעצמו הוא כאלו הכינו הג' בה' והה' בב' שהם התוספת |
|
וההבדל שבין זאת ההכאה להכאת הג' בב' הוא הכאת הב' בעצמם וזה מבואר מאד |
|
ה כל מספר כאשר חלקנו אותו לחצי והוספת עליו מספר אחר הנה הכאת המספר כלו מחובר עם התוספ' בתוספת ומרובע חצי המספר שוה למרובע חצי המספר והתוספת ביחד |
|
מופתו כי כשהוספנו על החצי מספר מה הנה יתוסף על מרובע החצי הכאת התוספת בחצי והכאת התוס' בחצי מחובר עם התוספת |
|
וזה כי אמרנו ה' פעמים ה' הם כ"ה ואמרנו ז' שלז' הרי נתוסף הכאת הב' שהם התוספת בה' והכאת הב' בז' שהוא מחובר עם התוספת והחצי |
|
וזהו בעצמו מה שאנו עושין כשאנו מוסיפין התוספת במספר כלו לפי שהמספ' חציו ועם התוספת שאנו מוסיפין עליו ומה להכאת התוספת עם החצי ואע"כ עם החצי והתוספת מחובר או הכאתו עם כל המספר והתוספת ביחד והבן |
|
ו כל מספר שתחלקהו בב' חלקים איך שקרה המרובע ההווה מהמספר כלו והמרובע ההווה מא' מב' אלו החלקים כאשר התקבצו שוה להכאת החלק הנזכ' עם המספר כלו ולהכאת החלק הב' הנשאר בעצמו |
|
מופתו כבר התבאר בהקדמה ג' שהכאת הכלל בעצמו הוא הכאת חלקיו כל אחת בפני עצמו והכאת החלק הא' עם חבירו ב' פעמים |
|
וא"כ כשאנו מכין החלק הנז' אשר הוספנו מרובעו למרובע המספר כלו עם המספר כלו ב' פעמים הרי אנו מכין אותו בעצמו ב' פעמים ועם החלק הא' ב' פעמי' |
|
ואח"כ אנו מוסיפין מרובע החלק הב' הנשאר |
|
והכאת הכלל בעצמו הוא הכאת החלק בעצמו פעם א' וכל אח' עם חבירו ב' פעמים |
|
הרי נותר הנה הכאת חלק אחד בעצמו פעם אח' אנו מוסיפין זה על מרובע הכלל כדי שיהיה שוה |
|
במשל הרי הי' נחלק לז' ולג' |
|
הנה הכאת הג' בי' ב' פעמים הוא הכאת הג' בז' ב' פעמים והכאת הג' בעצמו ב' פעמים |
|
ואח"כ אנו מכין הז' בעצמו |
|
נותר לנו על מרובע הכלל הכאת [15]הג' בעצמו פעם אחד לכן אנו מוסיפין אותו על מרובע הכלל כדי שישוו |
|
ז כל מספר שחלקת אותו לב' חלקים איך שקרה אם הכית המספר כלו עם חלק אח' מהם ד' פעמים וקבצת הכל עם מרובע החלק הב' הנשאר היה שוה להכאת מספרו החלק הנזכר כאשר תחברם יחד |
|
המופת יתבאר בב' הקדמות |
|
אחת כל מספר שחלקת אותו לב' חלקים כי איך שקרה הנה הכאת החלק עם כל הכלל והחלק הנשאר בעצמו כאשר תקבצם שוה להכאת החלק הנשאר בכלל והכאת החלק הא' בעצמו כאשר תקבצם |
|
במשל הי' נחלק לז' ולג' |
|
הנה הכאת הז' בי' והכאת הג' בעצמו שוה להכאת הג' בי' והכאת הז' בעצמו |
|
וזה כי הכאת החלק בכלל הוא כאלו הכית אותו בעצמו ועם החלק האחד הנשאר כי אין הכלל זולת חלקיו |
|
ואח"כ כשאנו מכין החלק הנשא' בעצמו הרי עשינו ג' הכאות הכאת כל חלק בפני עצמו והכאת החלק הא' באחר |
|
וג"כ כאשר הפכנו הענין זה בעצמו אנו עושין |
|
המשל כי הכאת הג' בי' הוא כאלו הג' בז' ועמו בעצמו |
|
וכשהכינו הז' בעצמם עשינו בזה ג' הכאות הכאת הז' בעצמו והכאת הג' בעצמו והכאת הג' בז' |
|
כן ג"כ כשאנו מכין הז' בי' ואח"כ הג' בעצמו אלו הג' הכאות בעצמם אנו עושין הכאת הג' בעצמם והכאת הז' בעצמם והכאת הג' בז' וזה מבואר |
|
ההקדמה הב' כי כל מספר שנודע לנו מרובעו ונרצה לידע מרובע מספר אחר הנה נוסף עליו הכאת התוספת במספ' הנודע ב' פעמים והתוספת בעצמו פעם אחת |
|
במשל נודע לנו מרובע הי' ורצינו לידע מרובע הי"ז |
|
הנה נכה הז' בי' ב' פעמים ועם עצמו פעם אחת ונוסיפהו על מרובע הי' והוא העולה מהכאת י"ז בעצמו |
|
והסב' כשאנו אומרים י"ז פעמים הוא כאמרנו י' פעמים י"ז וז' פעמים י"ז |
|
וכשאנו אומרים י' פעמים י"ז כאלו אמרנו ז' פעמים י' וי' פעמים ז' |
|
וכשאנו אומרי' ז' פעמים י"ז כאלו אמרנום ז' פעמים י' וז' פעמים ז' |
|
נמצאו בזה ד' הכאות הכאת הי' בי' והכאת הז' בי' ב' פעמים והכאת הז' בעצמם |
|
וכשיש לנו י' פעמים י' חסר לנו הכאת הז' שהוא התוספת בי' שהוא המספר אשר נודע מרובעו ב' פעמים והכאת הז' שהוא התוספת בעצמו |
|
בזה יובנו דברי ההקדמה הנזכ' הנה כשהכית החלק בכלל ד' פעמים ואח"כ החלק הנשאר בעצמו שוה להכאת המספר והתוספת כשיחוברו |
|
וזה כי הכאת החלק הנשאר בעצמו כאשר יחובר אל הכאת החלק האח' בכלל שוה כאשר המירונו שנעשה ההפך |
|
וא"כ הד' פעמים שהכינו בכלל נקח אחד מהם וישארו ג' ותחבר זאת ההכאת אל הכאת החלק הנשאר בעצמו הוא כאלו החלק האח' בכלל וזה החלק שאנו מכין אותו בכלל נכה אותו בעצמו וא"כ יש לו ג' הכאות החלק בכלל והכאת אחרת של החלק האחר בכלל והכאת החלק האח' בעצמו |
|
וכבר ביארנו בהקדמה הראשונה שהכאת המספר שנתחלק בעצמו כאלו הכינו אותו עם כל חלקיו |
|
וא"כ מהג' פעמים שהכינו החלק בכלל נחסר אחת ונחבר עמה הכאת החלק האח' במספר שנתחל' והרי יש לנו מרובע המספר שנתחלק כלו נשאר לנו ב' פעמים הכאת החלק במספר שנתחלק ב' פעמים והכאת זה החלק בעצמו וזהו מה שאנו צריכין להוסיף על מרובע המספר שנתחלק כמו שביארנו |
Other Propositions |
הקדמות אחרות |
| 1) For parts whose products are equal, the ratio of a [product] to a [product] is equal to the ratio of a [part] to a [apr].
|
החלקים אשר כפליהם שוים הנה ייחס קצתם אל קצתם כיחס חלק קצתם אל קצת |
| Hence, it follows from this that, for any fraction, when its numerator and its denominator are multiplied the same number of times, [the result] is the same original fraction.
|
וא"כ יתחייב מזה שכל חלק מהשברים כאשר יכפל כמותו ואיכותו שוה הפעמי' הנה הוא השבר הראשון בעינו |
| Also, if you divide its numerator and its denominator into the same parts, then relate the quotient of the numerator to the quotient of the denominator, [the result] is the same original fraction.
|
וכן אם תחלק כמותו ואכותו לחלקים שוים ותיחס חלק הכמות עם חלק האיכות הוא השבר הראשון בעינו |
| 2) the product of a fraction by a fraction is as we say: we take the fraction of the fraction. | ב הכאת השבר עם השבר הוא כאמרנו נקח השבר השבר |
| 3) For any number multiplied by any number, the ratio of the multiplied number to the product is equal to the ratio of one to the multiplier.
|
ג שכל מספר נכפל בכפלים כמ' שיהיו המספר הנכפל יחסו אל המספר העולה מכפליו כערך הא' אל הכפלים שבו נכפל |
|
כמו מספר הב' שהוכה בג' ועלה ו' יחסו אל הו' כיחס האחד אל שלשה שהוא שליש |
| 4) When you want to take any part of some fractions, we take the required part of the numerator of the given fractions and relate it to [their] denominator.
|
ד כאשר תרצה לקחת מהשברים מה חלק מה הנה נקח מכמות השברים המונחים לפני החלק הדרוש וניחסהו אל האיכות |
|
אם רצית שליש הט' עשיריות קח שליש הט' והם ג' יחסים אל האיכו' והם עשיריות יהיו ג' עשיריות |
| 5) When you want to multiply given fractions by any multiples, we multiply the numerator and relate it to the denominator.
|
ה שכאשר תרצה לכפול שברים מונחים באיזה כפלים שיהיו נכפול הכמו' וניחסהו אל האיכות |
|
רצית להכות ב' תשיעיות בג' תכה הב' בג' הם ו' יחסם אל האיכות יהיו ו' תשיעיות |
| 6) Euclid, Elements, Book VII, proposition 17: For any number multiplied by two numbers, the ratio of the two products one to the other is equal to the ratio of the two numbers one to the other.
|
[16]ו כל מספר יוכו בו ב' מספרים יחס השטח האחד אל האח' כיחס הב' מספרים בעצמם הא' מהם אל הב' |
| 7) Euclid, Elements, Book VII, proposition 19: For any four proportional numbers, the product of the first by the fourth is equal to the product of the second by the third.
|
ז ד' מספרים מתיחסם הכאת הראשון בד' שוה להכאת הב' בג' |
| 8) Euclid, Elements, Book II, proposition 1: For any number multiplied by any number, their product is equal to [the sum of] the products of the multiplied number by each part of the multiplier number, divided into parts as you wish.
|
ח כל מספר יוכה עם מספר מה איזה מספר היה הנה העולה מהם שוה לעולה מהכאת המספר המוכה עם כל אחד מחלקי המספר המכה על איזה חלקים שיחלק |
| A short paragraph – philosophical observation concerning the meaning of the mean term: | |
| Reminder: the mean with regard to itself is not opposing, but with regard to its quality it is opposing to each of the extremes. It is hidden as the center of the circle for those who know geometry. It is mean in relation to us, but not in relation to the thing, therefore it is hidden. For, the mean in relation to the thing is known to the geometricians and the improvement is by removing the less from the extreme. You cannot measure it through a general way, but by the divine holy Torah that cannot be measured by the human intellect and this is true anyway. | זכירה האמצעי בבחינתו בעצמו אינו נגדיי ובבחינות טובו הוא נגדיי לא' מהקצוות והוא נסתר כמו מרכז העגולה אף ליודעי חכמת השיעור והוא האמצעי בערך אלינו ולא בערך הדבר ועל כן הוא נסתר כי האמצעי בערך הדבר ידוע לבעלי חכמת השיעור והתיקון בהרחיק מהקצווי הפחות ולא תשער זה ע"ד כולל זולת התורה האלהית הקדושה שאינה משוערת מהשכל האינושי וזה אמת על כל פנים |
Notes
- ↑ שמואל א כ"ו, כ"ה
Apparatus
Appendix: Bibliography
Anonymous
Qeṣat mi ʽInyanei Ḥoḵmat ha-Mispar
Manuscripts:
- Paris, Bibliothèque Nationale heb. 1007/2 (IMHM: f 15710), ff. 29r-36r (1572)
Bibliography:
- Steinschneider, Moritz. 1906. Mathematik bei den Juden, Band II: 1551-1840. Monatsschrift für die Geschichte und Wissenschaft des Judenthums 50, p. 349. repr.: ed. Gad Freudenthal, Hildesheim, Zürich, New York: Olms, 2014, p. 153.
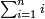
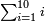
![\scriptstyle{\color{OliveGreen}{\sum_{i=1}^{n} i=n\sdot\left[\left(\frac{1}{2}\sdot n\right)+\frac{1}{2}\right]}}](/mediawiki/images/math/1/5/c/15c33ef94f6705e49da83967f0dddc21.png)
![\scriptstyle{\color{blue}{\sum_{i=1}^{10} i=10\sdot\left[\left(\frac{1}{2}\sdot10\right)+\frac{1}{2}\right]}}](/mediawiki/images/math/c/0/f/c0fdc71237cda523c5f4225f91df85b5.png)

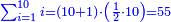
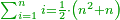
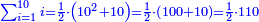
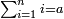

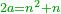
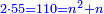
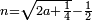

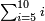

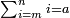
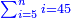
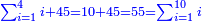
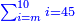
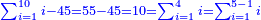


![\scriptstyle{\color{OliveGreen}{\sum_{i=1}^{n} i^2=\left(\sum_{i=1}^{n} i\right)\sdot\left[\left(\frac{2}{3}\sdot n\right)+\frac{1}{3}\right]}}](/mediawiki/images/math/3/d/e/3de704868bfb9b9a142ddf62d83bc112.png)
![\scriptstyle{\color{blue}{\sum_{i=1}^{10} i^2=\left(\sum_{i=1}^{10} i\right)\sdot\left[\left(\frac{2}{3}\sdot10\right)+\frac{1}{3}\right]=55\sdot7=385}}](/mediawiki/images/math/2/b/a/2ba3acc98bcbd3f147ab927dd9b48811.png)
![\scriptstyle{\color{OliveGreen}{\sum_{i=1}^n i^2=\left(\sum_{i=1}^n i\right)\sdot\left[\left[\frac{2}{3}\sdot\left(n-1\right)\right]+1\right]}}](/mediawiki/images/math/e/d/f/edf214156fd7c4c3ddb575a93911d0d1.png)
![\scriptstyle{\color{blue}{\sum_{k=1}^{10} k^2=\left(\sum_{k=1}^{10} k\right)\sdot\left[\left[\frac{2}{3}\sdot\left(10-1\right)\right]+1\right]}}](/mediawiki/images/math/8/b/c/8bcfe13faadb2a38abc83f12f8d0a1f5.png)
![\scriptstyle{\color{OliveGreen}{\sum_{i=1}^{11} i^2 =\left(\sum_{i=1}^{n} i\right)\sdot\left[\left[\frac{2}{3}\sdot \left(n+1\right)\right]-\frac{1}{3}\right]}}](/mediawiki/images/math/f/2/0/f20ca72a0b35535ef76c3017d54e7fa1.png)
![\scriptstyle{\color{blue}{\sum_{i=1}^{11} i^2 =\left(\sum_{i=1}^{11} i\right)\sdot\left[\left[\frac{2}{3}\sdot \left(11+1\right)\right]-\frac{1}{3}\right]}}](/mediawiki/images/math/e/a/c/eacacb7ee0f3d70ddd583f3e4ece1220.png)
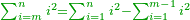
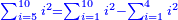
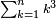
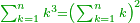
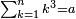
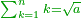
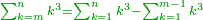
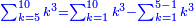
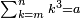
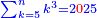

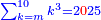
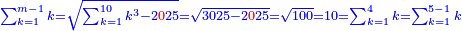
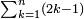
![\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n \left(2k-1\right)=\left[\left[\frac{1}{2}\sdot\left(2n-1\right)\right]+\frac{1}{2}\right]^2}}](/mediawiki/images/math/c/d/6/cd6076d16bb3b48675f41c7fe4e4fe82.png)
![\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n \left(2k-1\right)=\left[\frac{1}{2}\sdot\left[\left(2n-1\right)+1\right]\right]^2}}](/mediawiki/images/math/4/8/0/480e7eba8f067679d3ce243a9d7beec2.png)
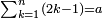
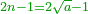
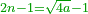
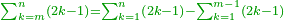
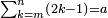
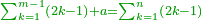

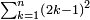
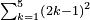
![\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n\left(2k-1\right)^2=\left[\left[\sum_{k=1}^n\left(2k-1\right)\right]\sdot\left[\left[\frac{2}{3}\sdot\left(2k - 1\right)\right]+\frac{2}{3}\right]\right]-\left(\frac{1}{3}\sdot{n}\right)}}](/mediawiki/images/math/9/f/8/9f8bf60b5acf28afbcefa11cd414ecef.png)
![\scriptstyle{\color{blue}{1^2+3^2+5^2+7^2+9^2=\left[25\sdot\left[\left(\frac{2}{3}\sdot9\right)+\frac{2}{3}\right]\right]-\left(\frac{1}{3}\sdot5\right)=\left[25\sdot\left(6+\frac{2}{3}\right)\right]-\frac{5}{3}=\left(166+\frac{2}{3}\right)-\left(1+\frac{2}{3}\right)}}](/mediawiki/images/math/a/d/2/ad2d9ef19a6fe9da0c11ec31b5e771a0.png)
![\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n\left(2k-1\right)^2=\left[\frac{2}{3}\sdot\left(2n-1\right)\right]\sdot\left[\left(\frac{1}{2}\sdot{2n}\right)\sdot\left[\frac{1}{2}\sdot\left(2n+1\right)\right]\right]}}](/mediawiki/images/math/e/0/3/e03f72674ae2318246b87ae1bd9e5d7d.png)
![\scriptstyle{\color{blue}{1^2+3^2+5^2+7^2+9^2=\left(\frac{2}{3}\sdot{9}\right)\sdot\left[\left(\frac{1}{2}\sdot{10}\right)\sdot\left(\frac{1}{2}\sdot{11}\right)\right]=6\sdot\left[5\sdot\left(5+\frac{1}{2}\right)\right]=6\sdot\left(27+\frac{1}{2}\right)}}](/mediawiki/images/math/a/1/d/a1d61054ac3ee8175fcb1d41e2074c13.png)
![\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n\left(2k-1\right)^2=\left[\left[\frac{1}{3}\sdot\left(2n-1\right)\right]+\frac{1}{3}\right]\sdot\left[\left[\frac{1}{2}\sdot\left(2n-1\right)\right]\sdot\left(2n+1\right)\right]}}](/mediawiki/images/math/c/8/9/c8991a20cc95f0d4af55673ee29dc8d6.png)
![\scriptstyle{\color{blue}{1^2+3^2+5^2+7^2+9^2=\left[\left(\frac{1}{3}\sdot{9}\right)+\frac{1}{3}\right]\sdot\left[\left(\frac{1}{2}\sdot{9}\right)\sdot{11}\right]=\left(3+\frac{1}{3}\right)\sdot\left[\left(4+\frac{1}{2}\right)\sdot{11}\right]=\left(3+\frac{1}{3}\right)\sdot\left(49+\frac{1}{2}\right)}}](/mediawiki/images/math/e/d/2/ed2403d4f1ae29d06a3035240a670334.png)
![\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n\left(2k-1\right)^2=\left[\left[\sum_{k=1}^n\left(2k-1\right)\right]-\left[\left[\frac{1}{3}\sdot\left[\sum_{k=1}^n\left(2k-1\right)\right]\right]+\frac{1}{6}\right]\right]\sdot\left[\left(2n-1\right)+1\right]}}](/mediawiki/images/math/4/b/a/4ba8a54d07a9d03cbf095f661181b9ab.png)
![\scriptstyle{\color{blue}{1^2+3^2+5^2+7^2+9^2=\left[25-\left[\left(\frac{1}{3}\sdot25\right)+\frac{1}{6}\right]\right]\sdot\left(9+1\right)=\left(16+\frac{1}{2}\right)\sdot10}}](/mediawiki/images/math/4/c/b/4cbf2b88ab0801682a6ba38af8339840.png)
![\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n\left(2k-1\right)^2=\left[\left[\left[\frac{1}{3}\sdot\left(2n-1\right)\right]+\frac{2}{3}\right]\sdot\left[\frac{1}{2}\sdot\left(2n-1\right)\right]\right]\sdot\left[\left(2n-1\right)+1\right]}}](/mediawiki/images/math/8/4/3/843e4d299bd314d20f5e4a052f934462.png)
![\scriptstyle{\color{blue}{1^2+3^2+5^2+7^2+9^2=\left[\left[\left(\frac{1}{3}\sdot9\right)+\frac{2}{3}\right]\sdot\left(\frac{1}{2}\sdot9\right)\right]\sdot\left(9+1\right)=\left[\left(3+\frac{2}{3}\right)\sdot\left(4+\frac{1}{2}\right)\right]\sdot{10}=\left(16+\frac{1}{2}\right)\sdot10}}](/mediawiki/images/math/a/3/a/a3a64c7b5349464cfc77edc1aed2eea9.png)
![\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n\left(2k-1\right)^2=\left[\frac{1}{6}\sdot\left(2n-1\right)\right]\sdot\left[\left[\left(2n-1\right)+1\right]\sdot\left[\left(2n-1\right)+2\right]\right]}}](/mediawiki/images/math/e/e/e/eee087ab888607bae5982c088a63c70f.png)
![\scriptstyle{\color{blue}{1^2+3^2+5^2+7^2+9^2=\left(\frac{1}{6}\sdot9\right)\sdot\left[\left(9+1\right)\sdot\left(9+2\right)\right]=\left(1+\frac{1}{2}\right)\sdot\left(10\sdot11\right)=\left(1+\frac{1}{2}\right)\sdot110}}](/mediawiki/images/math/a/f/7/af76e963ae748b99d5a6d1fb4b24d543.png)
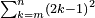


![\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n\left(2k-1\right)^3=\left[2\sdot\left[\sum_{k=1}^n\left(2k-1\right)\right]^2\right]-\left[\sum_{k=1}^n\left(2k-1\right)\right]}}](/mediawiki/images/math/a/b/b/abbc57b6822d7919d2943970484e1da2.png)
![\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n\left(2k-1\right)^3=\left[\left[\sum_{k=1}^n\left(2k-1\right)\right]-\frac{1}{2}\right]\sdot\left[2\sdot\left[\sum_{k=1}^n\left(2k-1\right)\right]\right]}}](/mediawiki/images/math/b/4/c/b4c8d7fb68379d8a737051638a672b40.png)
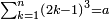
![\scriptstyle{\color{OliveGreen}{2n-1=\sqrt{4\sdot\left[\sqrt{\left(\frac{1}{2}\sdot{a}\right)+\left(\frac{1}{2}\sdot\frac{1}{8}\right)}+\sqrt{\frac{1}{2}\sdot\frac{1}{8}}\right]}-1}}](/mediawiki/images/math/1/0/7/107f72cc443f8a9a890e0b0f6ab95a12.png)
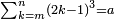
![\scriptstyle{\color{OliveGreen}{\left[\sum_{k=1}^n\left(2k-1\right)^3\right]-a=\sum_{k=1}^{m-1}\left(2k-1\right)^3}}](/mediawiki/images/math/1/9/e/19e5063197238ee4cd7b664cae09ffca.png)
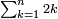
![\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n 2k =\left[\left(\frac{1}{2}\sdot{2n}\right)+1\right]\sdot\left(\frac{1}{2}\sdot{2n}\right)}}](/mediawiki/images/math/d/4/f/d4f5e1321400e450d63e5bc6f484802c.png)
![\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n 2k =\left[\frac{1}{2}\sdot\left(2n+2\right)\right]\sdot\left(\frac{1}{2}\sdot{2n}\right)}}](/mediawiki/images/math/e/a/4/ea4778ea0d8c0eed8278c154fe446ec7.png)
![\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n 2k=\left(\frac{1}{4}\sdot{2n}\right)\sdot\left[2\sdot\left(n+1\right)\right]}}](/mediawiki/images/math/7/a/1/7a17825bd5a429db6435e493f4f3189f.png)
![\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n 2k=\left[\frac{1}{4}\sdot\left[2\sdot\left(n+1\right)\right]\right]\sdot{2n}}}](/mediawiki/images/math/b/6/2/b62a6b448fd35a31747ce8e6b14bec30.png)

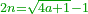
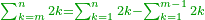
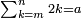
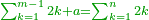

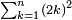
![\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n\left(2k\right)^2=\left[\left[\frac{2}{3}\sdot\left(2n\right)\right]+\frac{2}{3}\right]\sdot\left[\sum_{k=1}^n 2k\right]}}](/mediawiki/images/math/b/3/9/b3997cee3ad2d10139de5c77fb587836.png)
![\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n\left(2k\right)^2=\left[\frac{2}{3}\sdot\left[\sum_{k=1}^n 2k\right]\right]\sdot\left(2n+1\right)}}](/mediawiki/images/math/a/6/b/a6b6869b9254dcd2cc0325bee50cace6.png)
![\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n\left(2k\right)^2=\left[\frac{1}{6}\sdot\left(2k\right)\right]\sdot\left[\left(2n+1\right)\sdot\left(2n+2\right)\right]}}](/mediawiki/images/math/0/d/2/0d2c8d638501fd303b9d189a29a75b96.png)
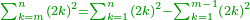
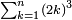
![\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n\left(2k\right)^3=2\sdot\left[\sum_{k=1}^n 2k\right]^2}}](/mediawiki/images/math/1/1/6/1161fd2908109fe574eb5793ff85d15f.png)


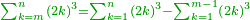
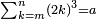
![\scriptstyle{\color{OliveGreen}{\left[\sum_{k=1}^{m-1}\left(2k\right)^3\right]+a=\sum_{k=1}^n\left(2k\right)^3}}](/mediawiki/images/math/a/4/8/a48b98299a1570bef1bb299afcfe14df.png)
![\scriptstyle{\color{OliveGreen}{\left[\sum_{k=1}^n\left(2k\right)^3\right]-a=\sum_{k=1}^{m-1}\left(2k\right)^3}}](/mediawiki/images/math/4/1/a/41ab89d9b5edc29c8098763696448946.png)
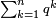

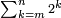
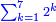
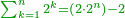
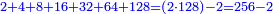

![\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n q^k=q^n+\left[\frac{1}{q-1}\sdot\left(q^n-q\right)\right]}}](/mediawiki/images/math/c/1/5/c1595839d93ed1d418d9efa7115fc2fb.png)
![\scriptstyle{\color{blue}{3+9+27+81+243+729=729+\left[\frac{1}{3-1}\sdot\left(729-3\right)\right]=729+\left[\frac{1}{2}\sdot\left(729-3\right)\right]=729+363}}](/mediawiki/images/math/9/8/c/98cae9e9e05b42148f13037eaf34b28e.png)
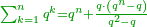
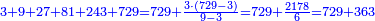

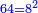
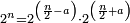
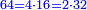
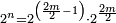
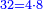


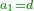
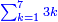
![\scriptstyle{\color{OliveGreen}{S_n=\left[\left(\frac{1}{2}\sdot n\right)+\frac{1}{2}\right]\sdot a_n}}](/mediawiki/images/math/e/9/e/e9e84c2521033a8bd08e6d2a0a1be2fe.png)
![\scriptstyle{\color{blue}{3+6+9+12+15+18+21=\left[\left(\frac{1}{2}\sdot7\right)+\frac{1}{2}\right]\sdot21=4\sdot21=84}}](/mediawiki/images/math/8/8/f/88f89feca622e0b9a15aee4d7b152ef8.png)
![\scriptstyle{\color{OliveGreen}{S_n=\left[\frac{1}{2}\sdot\left(a_1+a_n\right)\right]\sdot n}}](/mediawiki/images/math/1/8/a/18a8fe8fe49a6e4bf4ad363577843324.png)
![\scriptstyle{\color{blue}{3+6+9+12+15+18+21=\left[\frac{1}{2}\sdot\left(3+21\right)\right]\sdot7=\left(\frac{1}{2}\sdot24\right)\sdot7=12\sdot7=84}}](/mediawiki/images/math/5/a/9/5a99660d4189ad6756ee63ee891fac64.png)
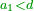
![\scriptstyle{\color{blue}{\sum_{k=1}^8 \left[\left[2\sdot\left(k-1\right)\right]+1\right]}}](/mediawiki/images/math/7/a/d/7ad45aa9fb63dddfd4194e85bbaac96c.png)
![\scriptstyle{\color{OliveGreen}{S_n=\left[\left[\left(d-a_1\right)+a_n\right]\sdot\left[\left(\frac{1}{2}\sdot n\right)+\frac{1}{2}\right]\right]-\left[\left(d-a_1\right)\sdot n\right]}}](/mediawiki/images/math/d/0/2/d02aa23b1e57fdae10f291131d3bf5c5.png)
![{\color{blue}{\begin{align}\scriptstyle1+3+5+7+9+11+13+15&\scriptstyle=\left[\left[\left(2-1\right)+15\right]\sdot\left[\left(\frac{1}{2}\sdot8\right)+\frac{1}{2}\right]\right]-\left[\left(2-1\right)\sdot8\right]=\left[\left(1+15\right)\sdot\left(4+\frac{1}{2}\right)\right]-\left(1\sdot8\right)\\&\scriptstyle=\left[16\sdot\left(4+\frac{1}{2}\right)\right]-8=72-8=64\\\end{align}}}](/mediawiki/images/math/3/6/d/36ddcc9cea6ce102795ec521e3f62083.png)
![\scriptstyle{\color{blue}{1+3+5+7+9+11+13+15=\left[\frac{1}{2}\sdot\left(1+15\right)\right]\sdot8=\left(\frac{1}{2}\sdot{16}\right)\sdot8=8\sdot8}}](/mediawiki/images/math/a/4/d/a4dd2e5c9a830cd49efad146fe6fbb04.png)
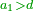

![\scriptstyle{\color{OliveGreen}{S_n=\left[\left[\left(a_1-d\right)+a_n\right]\sdot\left[\left(\frac{1}{2}\sdot{n}\right)+\frac{1}{2}\right]\right]-\left(a_1-d\right)}}](/mediawiki/images/math/b/f/6/bf648a3f3bd37547dd46b22becc408ca.png)
![{\color{blue}{\begin{align}\scriptstyle5+8+11+14+17+20+23&\scriptstyle=\left[\left[\left(5-3\right)+23\right]\sdot\left[\left(\frac{1}{2}\sdot{7}\right)+\frac{1}{2}\right]\right]-\left(5-3\right)=\left[\left(2+23\right)\sdot\left[\left(\frac{1}{2}\sdot{7}\right)+\frac{1}{2}\right]\right]-2\\&\scriptstyle=\left[25\sdot\left[\left(\frac{1}{2}\sdot{7}\right)+\frac{1}{2}\right]\right]-2=100-2\\\end{align}}}](/mediawiki/images/math/3/3/5/3352c6787268f28827204f83db28e0d6.png)
![\scriptstyle{\color{OliveGreen}{S_n=\left[\frac{1}{2}\sdot\left[\frac{a_n-a_1}{d}+1\right]\right]\sdot\left(a_1+a_n\right)}}](/mediawiki/images/math/6/4/9/6499ec6e4ec6368272a0ea8c6ae25b36.png)
![\scriptstyle{\color{blue}{5+8+11+14+17+20+23=\left[\frac{1}{2}\sdot\left[\frac{23-5}{3}+1\right]\right]\sdot\left(5+23\right)=\left[\frac{1}{2}\sdot\left[\frac{18}{3}+1\right]\right]\sdot\left(5+23\right)=\left(\frac{1}{2}\sdot{7}\right)\sdot\left(5+23\right)}}](/mediawiki/images/math/a/1/f/a1f13967b94903ce0179a5d4aacb7a92.png)
![\scriptstyle{\color{OliveGreen}{S_n=\left[\frac{a_n-a_1}{d}+1\right]\sdot\left[\frac{1}{2}\sdot\left(a_1+a_n\right)\right]}}](/mediawiki/images/math/7/5/0/75033430ddf50c931ca3e3782f3ef57b.png)
![\scriptstyle{\color{blue}{5+8+11+14+17+20+23=\left(\frac{23-5}{3}+1\right)\sdot\left[\frac{1}{2}\sdot\left(5+23\right)\right]=\left(\frac{18}{3}+1\right)\sdot\left[\frac{1}{2}\sdot\left(5+23\right)\right]=7\sdot\left[\frac{1}{2}\sdot\left(5+23\right)\right]}}](/mediawiki/images/math/a/f/d/afd67bad6710bc17988cad744968d1c5.png)
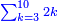

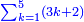
![{\color{OliveGreen}{\begin{align}\scriptstyle\sum_{k=m}^n a_k&\scriptstyle=\left[\left[\left[\left(\frac{1}{2}\sdot{n}\right)+\frac{1}{2}\right]\sdot\left[a_n+\left(d-a_1\right)\right]\right]-\left[\left(d-a_1\right)\sdot{n}\right]\right]\\&\scriptstyle-\left[\left[\left[\left[\frac{1}{2}\sdot\left(m-1\right)\right]+\frac{1}{2}\right]\sdot\left[a_{m-1}+\left(d-a_1\right)\right]\right]-\left[\left(d-a_1\right)\sdot\left(m-1\right)\right]\right]\\\end{align}}}](/mediawiki/images/math/8/8/1/8815ae3e40ce89650a8b77d64fdf713f.png)

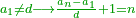
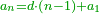
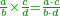
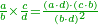
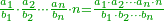
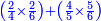

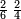
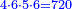
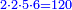
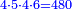

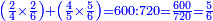
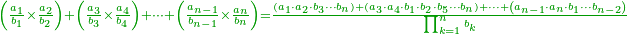
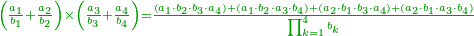
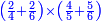


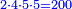
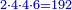
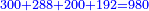
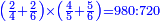
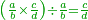
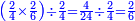
![\scriptstyle{\color{OliveGreen}{\left[\left(1-\frac{c}{d}\right)\times\frac{a}{b}\right]+\left(\frac{a}{b}\times\frac{c}{d}\right)=\frac{a}{b}}}](/mediawiki/images/math/7/4/4/744b88e33933bda6d8cf81e3fa9253ba.png)
![\scriptstyle{\color{blue}{\left[\left(1-\frac{2}{6}\right)\times\frac{2}{4}\right]+\left(\frac{2}{4}\times\frac{2}{6}\right)=\left(\frac{4}{6}\times\frac{2}{4}\right)+\frac{4}{24}=\frac{8}{24}+\frac{4}{24}=\frac{12}{24}=\frac{2}{4}}}](/mediawiki/images/math/0/4/3/043b50bab6492edc057a0a9bec3007bb.png)

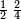
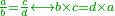
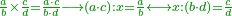
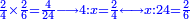
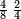
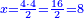

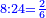
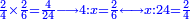
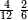


![\scriptstyle{\color{OliveGreen}{\frac{a_1}{b_1}+\frac{a_2}{b_2}+\cdots+\frac{a_n}{b_n}=\frac{\sum_{k=1}^n \left[a_k\sdot\left(\prod_{i=1,i\neq{k}}^n b_i\right)\right]}{\prod_{k=1}^n b_k}}}](/mediawiki/images/math/1/3/3/1337a9ba8d106c26c238d334902374d3.png)
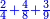
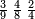



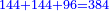

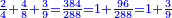
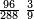
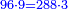

![\scriptstyle{\color{OliveGreen}{\frac{a}{b}+\frac{c}{d}=1-\left[\left(1-\frac{a}{b}\right)-\frac{c}{d}\right]}}](/mediawiki/images/math/2/d/1/2d1285622bda360093311cf198cb01c7.png)
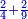
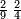
![\scriptstyle{\color{blue}{\frac{2}{4}+\frac{2}{9}=1-\left[\left(1-\frac{2}{4}\right)-\frac{2}{9}\right]=1-\left(\frac{2}{4}-\frac{2}{9}\right)=1-\frac{10}{36}=\frac{26}{36}}}](/mediawiki/images/math/0/e/2/0e2cedbf95ca5b6bddddcae39fa9909d.png)
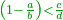
![\scriptstyle{\color{OliveGreen}{\frac{a}{b}+\frac{c}{d}=\left[\frac{c}{d}-\left(1-\frac{a}{b}\right)\right]+1}}](/mediawiki/images/math/2/4/5/245b516ec65d38dae667441d3e7a67fa.png)
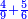
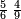

![\scriptstyle{\color{blue}{\frac{4}{9}+\frac{5}{6}=\left[\frac{5}{6}-\left(1-\frac{4}{9}\right)\right]+1=\left(\frac{5}{6}-\frac{5}{9}\right)+1=\frac{15}{54}+1}}](/mediawiki/images/math/e/8/c/e8c618ad53c37930baa7e805c91823ab.png)
![\scriptstyle{\color{OliveGreen}{\left[1-\left[\left(\frac{a}{b}+\frac{c}{d}\right)-1\right]\right]<\frac{e}{f}}}](/mediawiki/images/math/4/9/7/49741b4b26c398c46bba2d79665b68f5.png)
![\scriptstyle{\color{OliveGreen}{\frac{a}{b}+\frac{c}{d}+\frac{e}{f}=\left[\frac{e}{f}-\left[1-\left[\left(\frac{a}{b}+\frac{c}{d}\right)-1\right]\right]\right]+1+1}}](/mediawiki/images/math/f/c/b/fcb0eb459b9784e504e2dec9e0e88939.png)
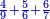
![\scriptstyle{\color{blue}{1-\left[\left(\frac{4}{9}+\frac{5}{6}\right)-1\right]=1-\frac{15}{54}=\frac{39}{54}<\frac{6}{7}}}](/mediawiki/images/math/f/8/5/f85b885efdc3f0514b4f44ae45b3fa1d.png)
![\scriptstyle{\color{blue}{\frac{4}{9}+\frac{5}{6}+\frac{6}{7}=\left[\frac{6}{7}-\left[1-\left[\left(\frac{4}{9}+\frac{5}{6}\right)-1\right]\right]\right]+1+1=\left[\frac{6}{7}-\left(1-\frac{15}{54}\right)\right]+1+1=\left(\frac{6}{7}-\frac{39}{54}\right)+1+1=\frac{51}{378}+1+1=2+\frac{51}{378}}}](/mediawiki/images/math/3/c/5/3c53f8bd51094f381f9214bcf58e2d15.png)
![\scriptstyle{\color{OliveGreen}{\left[1-\left[\left(\frac{a}{b}+\frac{c}{d}\right)-1\right]\right]>\frac{e}{f}}}](/mediawiki/images/math/9/e/4/9e4c647186f98c74f1173a45bcc754ff.png)
![\scriptstyle{\color{OliveGreen}{\frac{a}{b}+\frac{c}{d}+\frac{e}{f}=1-\left[\left[1-\left[\left(\frac{a}{b}+\frac{c}{d}\right)-1\right]\right]-\frac{e}{f}\right]+1}}](/mediawiki/images/math/6/6/a/66ab6f43cb1fbb9902fb689dcfa7e433.png)
![\scriptstyle{\color{OliveGreen}{\left[\left(1-\frac{a}{b}\right)-\frac{c}{d}\right]<\frac{e}{f}}}](/mediawiki/images/math/a/b/4/ab40c2486fd0660c201a9aa5223f2357.png)
![\scriptstyle{\color{OliveGreen}{\frac{a}{b}+\frac{c}{d}+\frac{e}{f}=\left[\frac{e}{f}-\left[\left(1-\frac{a}{b}\right)-\frac{c}{d}\right]\right]+1}}](/mediawiki/images/math/8/9/c/89cb72abf38f100492c3c9efd4055bd9.png)


![\scriptstyle{\color{blue}{\frac{2}{4}+\frac{2}{6}+\frac{4}{7}=\left[\frac{4}{7}-\left[\left(1-\frac{2}{4}\right)-\frac{2}{6}\right]\right]+1=\left(\frac{4}{7}-\frac{4}{24}\right)+1=\frac{68}{168}+1}}](/mediawiki/images/math/e/a/d/eadb28b1a729b17a4e53e6081f44fc3d.png)
![\scriptstyle{\color{OliveGreen}{\left[\left(1-\frac{a}{b}\right)-\frac{c}{d}\right]>\frac{e}{f}}}](/mediawiki/images/math/8/d/a/8da11b61d38bb1eb29aed009a03f201e.png)
![\scriptstyle{\color{OliveGreen}{\frac{a}{b}+\frac{c}{d}+\frac{e}{f}=1-\left[\left[\left(1-\frac{a}{b}\right)-\frac{c}{d}\right]-\frac{e}{f}\right]}}](/mediawiki/images/math/2/6/0/26078bc0f2e73e40af71e1c992c4bd71.png)
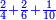
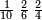
![\scriptstyle{\color{blue}{\left[\left(1-\frac{2}{4}\right)-\frac{2}{6}\right]=\frac{4}{24}>\frac{1}{10}}}](/mediawiki/images/math/9/5/9/95982f33557fe5e67d22f1647f93e6d1.png)
![\scriptstyle{\color{blue}{\frac{2}{4}+\frac{2}{6}+\frac{1}{10}=1-\left[\left[\left(1-\frac{2}{4}\right)-\frac{2}{6}\right]-\frac{1}{10}\right]=1-\left(\frac{4}{24}-\frac{1}{10}\right)=1-\frac{16}{240}=\frac{224}{240}}}](/mediawiki/images/math/a/c/b/acbf199d2d384493d028785ab5ac8d2d.png)
![\scriptstyle{\color{OliveGreen}{\left[1-\left[\frac{c}{d}-\left(1-\frac{a}{b}\right)\right]\right]<\frac{e}{f}}}](/mediawiki/images/math/0/3/b/03b2a79f8c9e216c7e01dceb671b4cd6.png)
![\scriptstyle{\color{OliveGreen}{\frac{a}{b}+\frac{c}{d}+\frac{e}{f}=\left[\left[\frac{e}{f}-\left[1-\left[\frac{c}{d}-\left(1-\frac{a}{b}\right)\right]\right]\right]+1\right]+1}}](/mediawiki/images/math/b/4/d/b4d2143c02b591331c82d1bee2bcd5aa.png)
![\scriptstyle{\color{OliveGreen}{\left[1-\left[\frac{c}{d}-\left(1-\frac{a}{b}\right)\right]\right]>\frac{e}{f}}}](/mediawiki/images/math/3/6/c/36c9435c0bc4ac0a0bfd593902016b68.png)
![\scriptstyle{\color{OliveGreen}{\frac{a}{b}+\frac{c}{d}+\frac{e}{f}=\left[1-\left[\left[1-\frac{c}{d}-\left(1-\frac{a}{b}\right)\right]-\frac{e}{f}\right]\right]+1}}](/mediawiki/images/math/1/f/8/1f8e30b3a1efb536ff52d21b8973f74c.png)
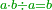

![\scriptstyle{\color{OliveGreen}{\left(a\sdot b\right)\div\left(c\sdot b\right)=\left[\left(a\sdot b\right)\div b\right]\div\left[\left(c\sdot b\right)\div b\right]=a\div c}}](/mediawiki/images/math/3/7/6/376be9fc42af66ce74baba7149d68422.png)

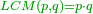
![\scriptstyle{\color{OliveGreen}{LCM\left[\left(a\sdot b\right),b\right]=a\sdot b}}](/mediawiki/images/math/7/7/f/77fcd8d9e1011ee22803f7cb9564d730.png)
![\scriptstyle{\color{OliveGreen}{LCM\left[\left(a\sdot b\right),\left(c\sdot b\right)\right]=\left(a\sdot b\right)\sdot c=a\sdot\left(c\sdot b\right)}}](/mediawiki/images/math/3/d/3/3d3cc36556d06c370448a1e33eadca87.png)
![\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n \frac{a_k}{b_k}=\frac{\sum_{k=1}^n \left[\frac{a_k}{b_k}\sdot LCM\left(b_1,b_2,\cdots,b_n\right)\right]}{LCM\left(b_1,b_2,\cdots,b_n\right)}}}](/mediawiki/images/math/3/0/5/30543199c8c7a189501c979c0555c3fa.png)
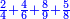

![\scriptstyle{\color{blue}{ LCM\left(4,6\right)=LCM\left[\left(2\sdot2\right),\left(3\sdot2\right)\right]=2\sdot6=3\sdot4=12}}](/mediawiki/images/math/9/0/5/9056c902fb8aa7dc6a2a29b8851ab72a.png)


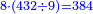
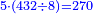
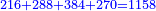
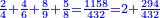
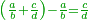
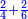
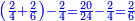

![\scriptstyle{\color{OliveGreen}{\left[\left(\frac{a}{b}+\frac{c}{d}\right)+\left(1-\frac{a}{b}\right)\right]-1=\frac{c}{d}}}](/mediawiki/images/math/7/b/f/7bf138f2330ac1057a2d595508120c86.png)
![\scriptstyle{\color{blue}{\left[\left(\frac{2}{4}+\frac{2}{6}\right)+\left(1-\frac{2}{4}\right)\right]-1=\left(\frac{20}{24}+\frac{2}{4}\right)-1=\left(1+\frac{2}{6}\right)-1=\frac{2}{6}}}](/mediawiki/images/math/8/6/4/864f6f2c6f571bc0ab5f55cc4be9614b.png)
![\scriptstyle{\color{blue}{\left[\left(\frac{2}{4}+\frac{2}{6}\right)+\left(1-\frac{2}{6}\right)\right]-1=\left(\frac{20}{24}+\frac{4}{6}\right)-1=\left(1+\frac{3}{6}\right)-1=\frac{3}{6}=\frac{2}{4}}}](/mediawiki/images/math/0/7/b/07b6760ec6933235d33f343d19528d64.png)
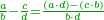
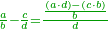
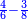
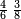
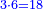
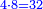

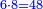
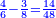
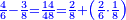
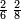
![\scriptstyle{\color{OliveGreen}{\frac{a}{b}-\frac{c}{d}=1-\left[\frac{c}{d}+\left(1-\frac{a}{b}\right)\right]}}](/mediawiki/images/math/a/7/0/a703d02fc788987ffa06c6c170609d3e.png)
![\scriptstyle{\color{blue}{\frac{4}{6}-\frac{3}{8}=1-\left[\frac{3}{8}+\left(1-\frac{4}{6}\right)\right]=1-\left(\frac{3}{8}+\frac{2}{6}\right)=1-\frac{34}{48}=\frac{14}{48}}}](/mediawiki/images/math/e/6/c/e6c3bf2ae9c4471daa25e36009354632.png)
![\scriptstyle{\color{OliveGreen}{\frac{a}{b}-\frac{c}{d}=\left[\frac{a}{b}+\left(1-\frac{c}{d}\right)\right]-1}}](/mediawiki/images/math/7/7/0/77004881ca11ceb95d4dbecb56642539.png)
![\scriptstyle{\color{blue}{\frac{4}{6}-\frac{3}{8}=\left[\frac{4}{6}+\left(1-\frac{3}{8}\right)\right]-1=\left(\frac{4}{6}+\frac{5}{8}\right)-1=\left(1+\frac{14}{48}\right)-1=\frac{14}{48}}}](/mediawiki/images/math/c/8/e/c8e205993be6c43b6e513b1a1e954a51.png)
![\scriptstyle{\color{OliveGreen}{\left[\frac{a}{b}+\left(\frac{a}{b}-\frac{c}{d}\right)\right]-\frac{c}{d}=2\sdot\left(\frac{a}{b}-\frac{c}{d}\right)}}](/mediawiki/images/math/1/c/7/1c7430dc2a05bf3d8be0e978a847889b.png)
![\scriptstyle{\color{blue}{\left[\frac{4}{6}+\left(\frac{4}{6}-\frac{3}{8}\right)\right]-\frac{3}{8}=\left(\frac{4}{6}+\frac{14}{48}\right)-\frac{3}{8}=\frac{276}{288}-\frac{3}{8}=\frac{1344}{2304}=2\sdot\frac{14}{48}=2\sdot\left(\frac{4}{6}-\frac{3}{8}\right)}}](/mediawiki/images/math/8/d/1/8d1c158cc81e9beb646e99071183d591.png)

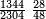
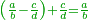
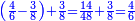
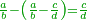
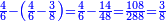
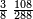
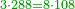
![{\color{OliveGreen}{\begin{align}&\scriptstyle\left(\frac{a_1}{b_2}+\frac{a_2}{b_2}+\cdots+\frac{a_n}{b_n}\right)-\left(\frac{c_1}{d_2}+\frac{c_2}{d_2}+\cdots+\frac{c_m}{d_m}\right)\\&\scriptstyle=\frac{\sum_{k=1}^n \left[a_k\sdot\left(\prod_{i=1,i\neq k}^n b_i\right)\sdot\left(\prod_{i=1}^m d_i\right)\right]-\sum_{k=1}^m \left[c_k\sdot\left(\prod_{i=1,i\neq k}^m d_i\right)\sdot\left(\prod_{i=1}^n b_i\right)\right]}{\prod_{k=1}^n b_k\sdot\prod_{i=1}^m d_i}\\\end{align}}}](/mediawiki/images/math/4/d/c/4dcd5d89beb5c0da5e72607633288b70.png)
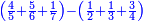
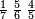
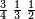

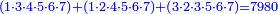
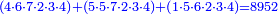
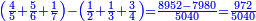
![{\color{OliveGreen}{\begin{align}&\scriptstyle\left(\frac{a_1}{b_1}-\frac{c_1}{d_1}\right)+\left(\frac{a_2}{b_2}-\frac{c_2}{d_2}\right)+\cdots+\left(\frac{a_n}{b_n}-\frac{c_n}{d_n}\right)\\&\scriptstyle=\frac{\sum_{k=1}^n \left[a_k\sdot\left(\prod_{i=1,i\neq k}^n b_i\right)\sdot\left(\prod_{i=1}^m d_i\right)\right]-\sum_{k=1}^n \left[c_k\sdot\left(\prod_{i=1,i\neq k}^n d_i\right)\sdot\left(\prod_{i=1}^n b_i\right)\right]}{\prod_{k=1}^n b_k\sdot\prod_{i=1}^n d_i}\\\end{align}}}](/mediawiki/images/math/8/d/3/8d3aec6a4bbf87b62d0cbba13d6ac7d2.png)
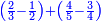
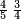
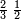
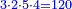





![\scriptstyle{\color{OliveGreen}{\left(\frac{a_1}{b_1}\times\frac{a_2}{b_2}\times\cdots\times\frac{a_n}{b_n}\right)-\left(\frac{c_1}{c_1}\times\frac{c_2}{c_2}\times\cdots\times\frac{c_m}{d_m}\right)=\frac{\left[\left(\prod_{i=1}^n a_i\right)\sdot\left(\prod_{j=1}^m d_j\right)\right]-\left[\left(\prod_{j=1}^m c_j\right)\sdot\left(\prod_{i=1}^n b_i\right)\right]}{\prod_{i=1}^n b_i\sdot\prod_{j=1}^m d_j}}}](/mediawiki/images/math/2/e/d/2ed5cf4c36db342e7f27474ababdd393.png)
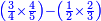




![{\color{OliveGreen}{\begin{align}&\scriptstyle\left(\frac{a_1}{b_1}-\frac{c_1}{d_1}\right)\times\left(\frac{a_2}{b_2}-\frac{c_2}{d_2}\right)\\&\scriptstyle=\frac{\left[\left(a_1\sdot d_1\right)-\left(c_1\sdot b_1\right)\right]\times\left[\left(a_2\sdot d_2\right)-\left(c_2\sdot b_2\right)\right]}{b_1\sdot d_1\sdot b_2\sdot d_2}\\\end{align}}}](/mediawiki/images/math/a/5/7/a57b58619cb3cdaff8f457f98a6a022f.png)
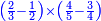

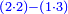

![{\color{blue}{\scriptstyle\left(\frac{2}{3}-\frac{1}{2}\right)\times\left(\frac{4}{5}-\frac{3}{4}\right)=\frac{\left[\left(2\sdot2\right)-\left(1\sdot3\right)\right]\times\left[\left(4\sdot4\right)-\left(3\sdot5\right)\right]}{3\sdot2\sdot5\sdot4}}}](/mediawiki/images/math/3/c/c/3cc16132d344c4acfadd35fb7d6cbd3a.png)
![{\color{OliveGreen}{\begin{align}&\scriptstyle\left[\left(\frac{a_1}{b_1}\times\frac{c_1}{d_1}\right)+\left(\frac{a_2}{b_2}\times\frac{c_2}{d_2}\right)+\cdots+\left(\frac{a_n}{b_n}\times\frac{c_n}{d_n}\right)\right]-\left[\left(\frac{a_{n+1}}{b_{n+1}}\times\frac{c_{n+1}}{d_{n+1}}\right)+\left(\frac{a_{n+2}}{b_{n+2}}\times\frac{c_{n+2}}{d_{n+2}}\right)+\cdots+\left(\frac{a_{n+m}}{b_{n+m}}\times\frac{c_{n+m}}{d_{n+m}}\right)\right]\\&\scriptstyle=\frac{\left[\sum_{k=1}^n \left[\left(a_k\sdot c_k\right)\sdot\prod_{i=1,i\neq k}^{n+m} \left(b_i\sdot d_i\right)\right]\right]-\left[\sum_{k={n+1}}^{n+m} \left[\left(a_k\sdot c_k\right)\sdot\prod_{i=1,i\neq k}^{n+m} \left(b_i\sdot d_i\right)\right]\right]}{\prod_{i=1}^{n+m} b_i\sdot\prod_{i=1}^{n+m} d_i}\\\end{align}}}](/mediawiki/images/math/c/7/8/c7894db688edfad179acf4a04c9ddb0c.png)
![{\color{blue}{\scriptstyle\left[\left(\frac{1}{2}\times\frac{2}{3}\right)+\left(\frac{3}{4}\times\frac{4}{5}\right)\right]-\left[\left(\frac{3}{7}\times\frac{4}{5}\right)+\left(\frac{2}{3}\times\frac{1}{4}\right)\right]}}](/mediawiki/images/math/b/b/d/bbd1d7fd145240388f8000054956bb7f.png)

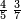



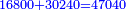
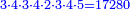

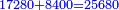
![\scriptstyle{\color{blue}{\left[\left(\frac{1}{2}\times\frac{2}{3}\right)+\left(\frac{3}{4}\times\frac{4}{5}\right)\right]-\left[\left(\frac{3}{7}\times\frac{4}{5}\right)+\left(\frac{2}{3}\times\frac{1}{4}\right)\right]=\frac{47040-25680}{50400}=\frac{21360}{50400}}}](/mediawiki/images/math/e/d/2/ed2d7d05282e05bd340152ee2627e47b.png)
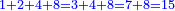
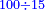
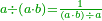
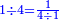
![\scriptstyle{\color{OliveGreen}{a\div\left[\left(a\sdot b\right)+1\right]=\frac{1}{\left[\left(a\sdot b\right)\div a\right]+1}+\left(\frac{1}{\left[\left(a\sdot b\right)\div a\right]+1}\sdot\frac{a-1}{\left(a\sdot b\right)+1}\right)}}](/mediawiki/images/math/0/9/7/09769dd3d54c39584ffb905810b951db.png)
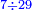
![\scriptstyle{\color{blue}{7\div29=\frac{1}{\left[\left(29-1\right)\div7\right]+1}+\left(\frac{1}{\left[\left(29-1\right)\div7\right]+1}\sdot\frac{7-1}{29}\right)=\frac{1}{4+1}+\left(\frac{1}{4+1}\sdot\frac{6}{29}\right)=\frac{1}{5}+\left(\frac{1}{5}\sdot\frac{6}{29}\right)}}](/mediawiki/images/math/e/e/b/eebe310736551b77afcfdf6b5068c881.png)
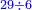
![\scriptstyle{\color{blue}{7\div29=\frac{1}{5}+\left(\frac{1}{5}\sdot\frac{6}{29}\right)=\frac{1}{5}+\left[\frac{1}{5}\sdot\frac{1}{4+1}+\left(\frac{1}{4+1}\sdot\frac{6-5}{29}\right)\right]=\frac{1}{5}+\left(\frac{1}{5}\sdot\frac{1}{5}\right)+\left(\frac{1}{5}\sdot\frac{1}{5}\sdot\frac{1}{5}\right)}}](/mediawiki/images/math/1/9/a/19a0ef396e0d42a7b2dd5c4e5e52dc71.png)
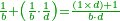
![{\color{blue}{\scriptstyle\frac{1}{5}+\left(\frac{1}{5}\sdot\frac{1}{5}\right)+\left(\frac{1}{5}\sdot\frac{1}{5}\sdot\frac{1}{5}\right)=\frac{\left[\left[\left(1\times 5\right)+1\right]\sdot29\right]+1}{5\sdot5}\div29=\frac{7}{29}}}](/mediawiki/images/math/e/c/5/ec5c691bfcfdd99a3362cecb334b8bc3.png)
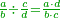
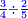
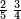

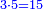
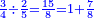
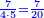
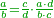
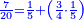
![\scriptstyle{\color{blue}{\frac{3}{4}=\frac{2}{5}+\left[\frac{1}{5}+\left(\frac{3}{4}\sdot\frac{1}{5}\right)\right]=\frac{3}{5}+\left(\frac{3}{4}\sdot\frac{1}{5}\right)}}](/mediawiki/images/math/2/5/1/2511f3fde3e808c168403400f37179f8.png)
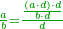
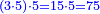
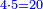
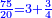
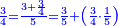
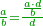
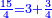
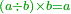
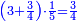
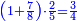
![\scriptstyle{\color{blue}{\left(1+\frac{7}{8}\right)\sdot\frac{2}{5}=\frac{2}{5}+\left(\frac{7}{8}\sdot\frac{2}{5}\right)=\frac{2}{5}+\left[\frac{1}{5}+\left(\frac{3}{4}\sdot\frac{1}{5}\right)\right]=\frac{3}{5}+\left(\frac{3}{4}\sdot\frac{1}{5}\right)}}](/mediawiki/images/math/0/4/c/04c80c01b3285380c20c3366d7777367.png)
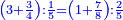
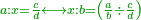
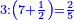

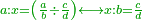
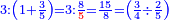
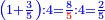
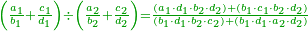
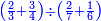

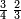
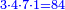
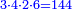
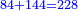
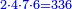
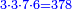

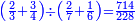

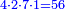

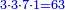
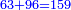

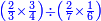

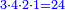
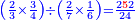
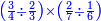
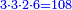

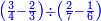
![\scriptstyle{\color{blue}{\left[\left(3\sdot3\right)-\left(4\sdot2\right)\right]\times\left(7\sdot6\right)=42}}](/mediawiki/images/math/0/d/8/0d89936b5ff51b49bdc00487eb7c653f.png)
![\scriptstyle{\color{blue}{\left[\left(2\sdot6\right)-\left(7\sdot1\right)\right]\times\left(4\sdot3\right)=60}}](/mediawiki/images/math/f/5/3/f53c691e6d1f9e0d0e83791f40284745.png)
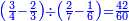



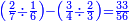


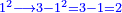
![\scriptstyle{\color{blue}{\left[\left[2\sdot\left(10\sdot1\right)\right]+8\right]\sdot8=\left(20\sdot8\right)+8^2\longrightarrow\begin{cases}\scriptstyle25-\left(2\sdot8\right)=25-16=9\\\scriptstyle96-8^2=96-64=32\\\end{cases}}}](/mediawiki/images/math/5/5/1/551b3dfbac610d5a2fad8b46d52c2b14.png)
![\scriptstyle{\color{blue}{\left[\left[2\sdot\left(10\sdot18\right)\right]+8\right]\sdot8=\left(360\sdot8\right)+8^2\longrightarrow\begin{cases}\scriptstyle32-\left(3\sdot8\right)=32-24=8\\\scriptstyle88-\left(6\sdot8\right)=88-48=40\\\scriptstyle407-8^2=343\\\end{cases}}}](/mediawiki/images/math/6/e/2/6e2720ff045bcc18eff284a72c77c882.png)
![\scriptstyle{\color{blue}{\left[\left[2\sdot\left(10\sdot188\right)\right]+9\right]\sdot9=\left(3760\sdot9\right)+9^2\longrightarrow\begin{cases}\scriptstyle34-\left(3\sdot9\right)=34-27=7\\\scriptstyle73-\left(7\sdot9\right)=73-63=10\\\scriptstyle100-\left(6\sdot9\right)=100-54=46\\\scriptstyle460-9^2=379\\\end{cases}}}](/mediawiki/images/math/c/8/9/c8916fa5c83d0d03105a2c160a63b297.png)
![\scriptstyle{\color{blue}{\left[\left[2\sdot\left(10\sdot1889\right)\right]+1\right]\sdot1=\left(37780\sdot1\right)+1^2\longrightarrow\begin{cases}\scriptstyle3-\left(3\sdot1\right)=0\\\scriptstyle7-\left(7\sdot1\right)=0\\\scriptstyle9-\left(7\sdot1\right)=9-7=2\\\scriptstyle20-\left(8\sdot1\right)=20-8=12\\\scriptstyle20-1^2=19\\\end{cases}}}](/mediawiki/images/math/2/e/9/2e9e55586cf560cc44c5388c7ee1283b.png)
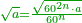

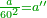
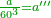

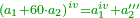
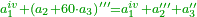
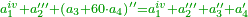
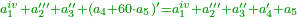
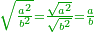

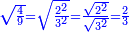

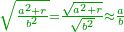
![\scriptstyle{\color{OliveGreen}{\sqrt[3]{a^3+r}\approx a}}](/mediawiki/images/math/d/0/8/d08276a0299fcd67809bb7e55f5c8068.png)
![\scriptstyle{\color{OliveGreen}{\sqrt[3]{\left(10a\right)^3+3\sdot\left[\left(10a\right)^2\sdot b\right]+3\sdot\left(10a\sdot b^2\right)+b^3}=\sqrt[3]{\left(10a+b\right)^3}=10a+b}}](/mediawiki/images/math/d/5/9/d598b308f04b6a0d930b9301f3c9d44c.png)
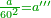
![\scriptstyle{\color{OliveGreen}{\sqrt[3]{\frac{a^3}{b^3}}=\frac{\sqrt[3]{a^3}}{\sqrt[3]{b^3}}=\frac{a}{b}}}](/mediawiki/images/math/3/2/3/323955c8b1c8bc2687db4477d0d0157e.png)
![\scriptstyle{\color{OliveGreen}{\sqrt[3]{\frac{a^3}{b}}=\sqrt[3]{\frac{a^3\sdot b^2}{b^3}}=\frac{\sqrt[3]{a^3\sdot b^2}}{\sqrt[3]{b^3}}}}](/mediawiki/images/math/6/0/0/600e0c41edfbcc6126fdda0a03ba2e05.png)
![\scriptstyle{\color{OliveGreen}{\sqrt[3]{\frac{a^3+r}{b^3}}=\frac{\sqrt[3]{a^3+r}}{\sqrt[3]{b^3}}\approx\frac{a}{b}}}](/mediawiki/images/math/a/6/3/a63687c30d63ae602120884f039beea2.png)
![\scriptstyle{\color{blue}{\sqrt[3]{\frac{a^3+r}{3^3}}=\frac{\sqrt[3]{a^3+r}}{\sqrt[3]{3^3}}\approx\frac{1}{3}a}}](/mediawiki/images/math/8/2/0/820c534a20149d3bc62b29e9d072e91d.png)
![\scriptstyle{\color{OliveGreen}{2a+10b+\left[\left(2c-1\right)\sdot100\right]\longrightarrow 2a+2b+4\sdot\left(2c-1\right)}}](/mediawiki/images/math/7/4/a/74ac340f2b4f98ca34b24a2531e2302a.png)
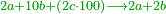
![\scriptstyle{\color{OliveGreen}{a^2=\left[10\sdot\left(\frac{1}{3}\sdot a\right)^2\right]-\left(\frac{1}{3}\sdot a\right)^2}}](/mediawiki/images/math/2/a/8/2a871e5e8b235962639d9cd1ccfcb09a.png)
![\scriptstyle{\color{blue}{9^2=\left[10\sdot\left(\frac{1}{3}\sdot9\right)^2\right]-\left(\frac{1}{3}\sdot9\right)^2=\left(10\sdot3^2\right)-3^2=\left(10\sdot9\right)-9=90-9=81}}](/mediawiki/images/math/a/0/3/a03c74cf98d310605915985de36e3e6f.png)
![\scriptstyle{\color{OliveGreen}{\left(a-1\right)^2=\left[\left[10\sdot\left(\frac{1}{3}\sdot a\right)^2\right]-\left(\frac{1}{3}\sdot a\right)^2\right]-\left(a-1\right)-a}}](/mediawiki/images/math/1/4/e/14eb1193ccd4cee1d7623918f2cb1ec0.png)
![\scriptstyle{\color{blue}{8^2=\left[\left[10\sdot\left(\frac{1}{3}\sdot9\right)^2\right]-\left(\frac{1}{3}\sdot9\right)^2\right]-8-9=\left[\left(10\sdot3^2\right)-3^2\right]-17=\left[\left(10\sdot9\right)-9\right]-17=\left(90-9\right)-17=81-17=64}}](/mediawiki/images/math/4/a/9/4a9170531abe4bb61de20ca612f8dbd8.png)
![\scriptstyle{\color{OliveGreen}{\left(a+1\right)^2=\left[\left[10\sdot\left(\frac{1}{3}\sdot a\right)^2\right]-\left(\frac{1}{3}\sdot a\right)^2\right]+\left(a+1\right)+a}}](/mediawiki/images/math/c/f/f/cff5e4f72245765135347088704def19.png)
![\scriptstyle{\color{blue}{7^2=\left[\left[10\sdot\left(\frac{1}{3}\sdot6\right)^2\right]-\left(\frac{1}{3}\sdot6\right)^2\right]+7+6=\left[\left(10\sdot2^2\right)-2^2\right]+13=36+13=49}}](/mediawiki/images/math/5/8/6/586dc70b947f2bac5cb81a9c3d344f77.png)
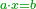
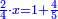
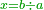
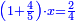
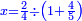
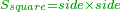
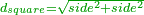


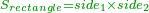
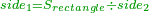
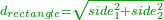

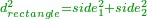

![\scriptstyle{\color{blue}{S_{triangle}=5\sdot\left[8+\frac{2}{4}+\left(\frac{3}{4}\sdot\frac{1}{4}\right)\right]=43+\frac{1}{4}+\left(\frac{3}{4}\sdot\frac{1}{4}\right)}}](/mediawiki/images/math/3/7/4/374d0caf4ae58e88ea8696d9aec06b3a.png)
![\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n \left[\left(\sum_{i=1}^n a_i\right)\sdot a_k\right]=\left(\sum_{i=1}^n a_i\right)^2}}](/mediawiki/images/math/9/9/f/99fd3737e42a1acf970a92fb7a977c13.png)

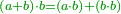
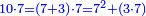

![\scriptstyle{\color{OliveGreen}{\left(a+b\right)^2=a^2+b^2+\left[2\sdot\left(a\sdot b\right)\right]}}](/mediawiki/images/math/d/f/2/df26d1cdaf50844081193e48666b1eeb.png)
![\scriptstyle{\color{OliveGreen}{\left(a+b\right)^2=\left[a\sdot\left(a+b\right)\right]+\left[b\sdot\left(a+b\right)\right]=\left[a^2+\left(a\sdot b\right)\right]+\left[b^2+\left(a\sdot b\right)\right]=a^2+b^2+\left[2\sdot\left(a\sdot b\right)\right]}}](/mediawiki/images/math/4/3/d/43dbec2db5f1c80447e9f0af87976ef0.png)
![\scriptstyle{\color{blue}{10^2=\left(7\sdot10\right)+\left(3\sdot10\right)=\left[7^2+\left(7\sdot3\right)\right]+\left[3^2+\left(3\sdot7\right)\right]=3^2+7^2+\left[2\sdot\left(3\sdot7\right)\right]}}](/mediawiki/images/math/b/5/1/b510258a370852d22a0003c32e3bed54.png)
![\scriptstyle{\color{OliveGreen}{\left(a\sdot b\right)+\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]^2=\left[\frac{1}{2}\sdot\left(a+b\right)\right]^2}}](/mediawiki/images/math/9/3/3/9338487b12c6a0e6ee74fab15e061cb9.png)
![\scriptstyle{\color{OliveGreen}{\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a=b-\left[\frac{1}{2}\sdot\left(a+b\right)\right]}}](/mediawiki/images/math/9/a/5/9a59ace7ae57674d3df572c35727b09e.png)

![{\color{OliveGreen}{\begin{align}\scriptstyle a\sdot b&\scriptstyle=a\sdot\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]+\left[b-\left[\frac{1}{2}\sdot\left(a+b\right)\right]\right]\right]\\&\scriptstyle=a\sdot\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]+\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]\right]\\\end{align}}}](/mediawiki/images/math/4/b/d/4bd89e9f911311caa6748e6aacfd672f.png)
![\scriptstyle{\color{OliveGreen}{\left[\frac{1}{2}\sdot\left(a+b\right)\right]^2=\left[\frac{1}{2}\sdot\left(a+b\right)\right]\sdot\left[a+\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]\right]}}](/mediawiki/images/math/3/9/f/39fe9a439bdf2a3adb3b02ada030575e.png)
![\scriptstyle{\color{OliveGreen}{\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]^2=\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]\sdot\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]\right]-\left[a\sdot\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]\right]}}](/mediawiki/images/math/b/2/1/b2198ff177bc9365f80ecd40e7d73911.png)
![\scriptstyle{\color{OliveGreen}{\left[\frac{1}{2}\sdot\left(a+b\right)\right]^2=\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]^2+\left(a\sdot b\right)}}](/mediawiki/images/math/b/1/d/b1df07fd2b6d805c43d1d7c34056a087.png)
![\scriptstyle{\color{blue}{5^2=\left(5\sdot3\right)+\left(5\sdot2\right)=\left(3\sdot5\right)+\left[\left(3\sdot2\right)+2^2\right]=\left(3\sdot7\right)+2^2=\left(3\sdot7\right)+\left(5-3\right)^2}}](/mediawiki/images/math/9/4/0/9403b526c051834da85753a590c1ea2d.png)
![\scriptstyle{\color{OliveGreen}{\left[\left(a+b\right)\sdot b\right]+\left(\frac{1}{2}\sdot a\right)^2=\left[\left(\frac{1}{2}\sdot a\right)+b\right]^2}}](/mediawiki/images/math/a/9/2/a92046fe5abf282c91d09104586c53a7.png)
![\scriptstyle{\color{OliveGreen}{\left[\left(\frac{1}{2}\sdot a\right)+b\right]^2=\left(\frac{1}{2}\sdot a\right)^2+\left[b\sdot\left(\frac{1}{2}\sdot a\right)\right]+\left[b\sdot\left[\left(\frac{1}{2}\sdot a\right)+b\right]\right]}}](/mediawiki/images/math/f/1/3/f1382ff0307bb5048c4a3910ceeb89ce.png)
![\scriptstyle{\color{blue}{\left(5+2\right)^2=5^2+\left(2\sdot5\right)+\left[2\sdot\left(5+2\right)\right]}}](/mediawiki/images/math/d/8/b/d8bb7e60d8daff23fdecbf3b19ed61ea.png)
![\scriptstyle{\color{OliveGreen}{b\sdot\left[\left(\frac{1}{2}\sdot a\right)+\left(\frac{1}{2}\sdot a\right)+b\right]=b\sdot\left(a+b\right)}}](/mediawiki/images/math/0/d/d/0dd6b4e4d2fa61e4f29daafcc9e7a071.png)
![\scriptstyle{\color{OliveGreen}{\left(a+b\right)^2+a^2=2\sdot\left[\left(a+b\right)\sdot a\right]+b^2}}](/mediawiki/images/math/0/3/a/03a8d96192812ea7db90d5166ad5177a.png)
![\scriptstyle{\color{OliveGreen}{\left(a+b\right)^2=a^2+b^2+\left[2\sdot\left(a+b\right)\right]}}](/mediawiki/images/math/f/3/8/f3810bc19cef88758119891a7736368f.png)
![\scriptstyle{\color{OliveGreen}{2\sdot\left[\left(a+b\right)\sdot a\right]+b^2=2a^2+\left[2\sdot\left(a\sdot b\right)\right]+b^2=a^2+\left[a^2+\left[2\sdot\left(a\sdot b\right)\right]+b^2\right]=\left(a+b\right)^2+a^2}}](/mediawiki/images/math/9/4/a/94ae3ec37d2b163425be43142d0bd222.png)
![\scriptstyle{\color{blue}{\left[2\sdot\left(3\sdot10\right)\right]+7^2=\left[2\sdot\left(3\sdot7\right)\right]+\left(2\sdot3^2\right)+7^2=10^2+3^2}}](/mediawiki/images/math/4/6/c/46cd1b2dd9f1a4f4f4d7dbed0a0fde5b.png)
![\scriptstyle{\color{OliveGreen}{4\sdot\left[\left(a+b\right)\sdot a\right]+b^2=\left[\left(a+b\right)+a\right]^2}}](/mediawiki/images/math/c/a/f/caf2f89aa2a00ea5e45e6eed3d4bc694.png)
![\scriptstyle{\color{OliveGreen}{\left[a\sdot\left(a+b\right)\right]+b^2=\left[b\sdot\left(a+b\right)\right]+a^2}}](/mediawiki/images/math/a/9/b/a9be984156d0d11db6b9d6ed625842c5.png)
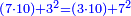

![\scriptstyle{\color{OliveGreen}{\left[a\sdot\left(a+b\right)\right]+b^2=a^2+\left(a\sdot b\right)+b^2}}](/mediawiki/images/math/0/0/0/00073be87d5d026d5c247dec9f5f4510.png)
![\scriptstyle{\color{OliveGreen}{\left[b\sdot\left(a+b\right)\right]+a^2=b^2+\left(a\sdot b\right)+a^2}}](/mediawiki/images/math/4/9/a/49aae3b2f9171ac4618ff5a79d047a74.png)
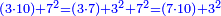
![\scriptstyle{\color{OliveGreen}{b^2=a^2+2\sdot\left[a\sdot\left(b-a\right)\right]+\left(b-a\right)^2}}](/mediawiki/images/math/3/0/5/30537d5c04a4f45d815a67832bf6b960.png)
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle17^2&\scriptstyle=10^2+2\sdot\left[10\sdot\left(17-10\right)\right]+\left(17-10\right)^2\\&\scriptstyle=10^2+2\sdot\left(10\sdot7\right)+7^2\\\end{align}}}](/mediawiki/images/math/6/7/7/67731d828dc38dc51514d914a547e881.png)
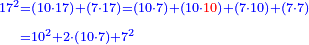
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle10^2&\scriptstyle=17^2-2\sdot\left(10\sdot7\right)-7^2\\&\scriptstyle=17^2-2\sdot\left[10\sdot\left(17-10\right)\right]-\left(17-10\right)^2\\\end{align}}}](/mediawiki/images/math/9/a/4/9a4a30b469c46bcbb14480005742377f.png)
![\scriptstyle{\color{OliveGreen}{b^2+\left[a\sdot\left(a+b\right)\right]=a^2+\left[b\sdot\left(a+b\right)\right]}}](/mediawiki/images/math/6/e/8/6e89b28d3c841f78d9945f500125f0e2.png)
![\scriptstyle{\color{OliveGreen}{4\sdot\left[\left(a+b\right)\sdot a\right]+b^2=3\sdot\left[a\sdot\left(a+b\right)\right]+\left[a\sdot\left(a+b\right)\right]+b^2=3\sdot\left[a\sdot\left(a+b\right)\right]+\left[b\sdot\left(a+b\right)\right]+a^2}}](/mediawiki/images/math/9/7/1/971671405efd53d6a68abf685626fea3.png)
![{\color{OliveGreen}{\begin{align}\scriptstyle4\sdot\left[\left(a+b\right)\sdot a\right]+b^2&\scriptstyle=3\sdot\left[a\sdot\left(a+b\right)\right]+\left[a\sdot\left(a+b\right)\right]+b^2=3\sdot\left[a\sdot\left(a+b\right)\right]+\left[b\sdot\left(a+b\right)\right]+a^2\\&\scriptstyle=2\sdot\left[a\sdot\left(a+b\right)\right]+\left[a\sdot\left(a+b\right)\right]+\left[b\sdot\left(a+b\right)\right]+a^2=2\sdot\left[a\sdot\left(a+b\right)\right]+\left(a+b\right)^2+a^2\\&\scriptstyle=\left[\left(a+b\right)+a\right]^2\\\end{align}}}](/mediawiki/images/math/7/9/c/79ce585b73cc09a6ae55f685e16c252f.png)