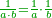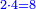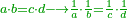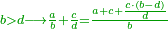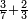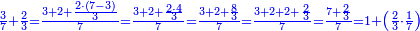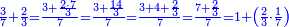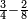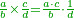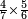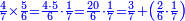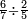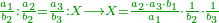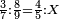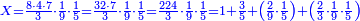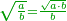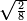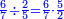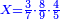Difference between revisions of "בר נותן טעם"
(→Chapter Two: Subtraction) |
(→Written Subtraction) |
||
| (490 intermediate revisions by the same user not shown) | |||
| Line 6: | Line 6: | ||
| − | =Prologue= | + | == <span style=color:Green>Prologue</span> == |
{|style="margin-left: auto; margin-right: 0px; text-align:center;" | {|style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| Line 14: | Line 14: | ||
|- | |- | ||
|אהבת נפש חשקתיך | |אהבת נפש חשקתיך | ||
| − | |ואני אהבתיך‫<ref>ישעיהו מג, ד</ref> | + | |ואני אהבתיך‫<ref group=note>ישעיהו מג, ד</ref> |
|- | |- | ||
|יפה פה תאר [ונחמד] מראה | |יפה פה תאר [ונחמד] מראה | ||
| Line 21: | Line 21: | ||
|colspan="2" style="text-align: center;" |ר' יואל ן' דאוד | |colspan="2" style="text-align: center;" |ר' יואל ן' דאוד | ||
|- | |- | ||
| − | |כל בניינה ''בנוי לתלפיות''‫<ref>שיר השירים ד, ד</ref> | + | |כל בניינה ''בנוי לתלפיות''‫<ref group=note>שיר השירים ד, ד</ref> |
|אשר נפשך חשקה בחכמת הלימודיות | |אשר נפשך חשקה בחכמת הלימודיות | ||
|- | |- | ||
| − | |מרחקת ''שוא ודבר כזב''<ref>משלי ל, ח</ref> תפל והתול | + | |מרחקת ''שוא ודבר כזב''<ref group=note>משלי ל, ח</ref> תפל והתול |
| − | |אין בה ''עקש ופתלתול''‫<ref>דברים לב, ה</ref> | + | |אין בה ''עקש ופתלתול''‫<ref group=note>דברים לב, ה</ref> |
|- | |- | ||
| − | |מעוות לא תוכל ''לתקן וחסרון''‫<ref>קהלת א, טו</ref> לא תמלא | + | |מעוות לא תוכל ''לתקן וחסרון''‫<ref group=note>קהלת א, טו</ref> לא תמלא |
|צדק תצדיק ויושר תעלה | |צדק תצדיק ויושר תעלה | ||
|- | |- | ||
|אומרת על הן הן ועל לאו לאו | |אומרת על הן הן ועל לאו לאו | ||
| − | |תחת לשונה דבש וחלב ‫<ref>שיר השירים ד, י"א</ref> | + | |תחת לשונה דבש וחלב ‫<ref group=note>שיר השירים ד, י"א</ref> |
|- | |- | ||
|חכמת המספר בתה ואחותה | |חכמת המספר בתה ואחותה | ||
| Line 39: | Line 39: | ||
|וזה כמה כתבתי עליה | |וזה כמה כתבתי עליה | ||
|- | |- | ||
| − | |colspan="2"|ולהטעים דרכיה דרכי נועם ‫<ref>משלי ג, י"ז</ref> | + | |colspan="2"|ולהטעים דרכיה דרכי נועם ‫<ref group=note>משלי ג, י"ז</ref> |
|- | |- | ||
| − | |colspan="2"|בלשון עם נועז‫<ref>ישעיה ל"ג, י"ט</ref> | + | |colspan="2"|בלשון עם נועז‫<ref group=note>ישעיה ל"ג, י"ט</ref> |
|- | |- | ||
| − | |colspan="2"|ללעוזות בלעז‫<ref>מועד, מגילה, ב, א</ref> | + | |colspan="2"|ללעוזות בלעז‫<ref group=note>מועד, מגילה, ב, א</ref> |
|- | |- | ||
|colspan="2"|בלי דופי ולעז | |colspan="2"|בלי דופי ולעז | ||
| Line 68: | Line 68: | ||
|אמרתי אשר מן האנשים חיו | |אמרתי אשר מן האנשים חיו | ||
|- | |- | ||
| − | |colspan="2"|יעזרם אלהים צור בו חציו חסיו‫<ref>דברים ל"ב, ל"ז</ref> | + | |colspan="2"|יעזרם אלהים צור בו חציו חסיו‫<ref group=note>דברים ל"ב, ל"ז</ref> |
|- | |- | ||
| − | |colspan="2"|ואליהם אהלים ישליו‫<ref>איוב י"ב, ו</ref> | + | |colspan="2"|ואליהם אהלים ישליו‫<ref group=note>איוב י"ב, ו</ref> |
|- | |- | ||
| − | |colspan="2"|ושנות מספר יאתיו‫<ref>איוב טז, כ"ב</ref> | + | |colspan="2"|ושנות מספר יאתיו‫<ref group=note>איוב טז, כ"ב</ref> |
|- | |- | ||
|רזה ודלה | |רזה ודלה | ||
| Line 88: | Line 88: | ||
|colspan="2"|ישבחוהו לאומים ותומכם מאושר | |colspan="2"|ישבחוהו לאומים ותומכם מאושר | ||
|- | |- | ||
| − | |ולהיות על שתי הסעיפים פוצח [פוסח]‫<ref>מלכים א י"ח, כ"א</ref> | + | |ולהיות על שתי הסעיפים פוצח [פוסח]‫<ref>marg.</ref><ref group=note>מלכים א י"ח, כ"א</ref> |
|בהם יוכל להשתרר ולנצח | |בהם יוכל להשתרר ולנצח | ||
|- | |- | ||
| − | |colspan="2"|ללכת כרצונו אנה ואנה‫<ref>מלכים א ב, מ"ב</ref> | + | |colspan="2"|ללכת כרצונו אנה ואנה‫<ref group=note>מלכים א ב, מ"ב</ref> |
|- | |- | ||
|וכמקשה המלונה | |וכמקשה המלונה | ||
| − | |כאשר בתוך המים ינוד הקנה‫<ref>מלכים א י"ד, ט"ו</ref> | + | |כאשר בתוך המים ינוד הקנה‫<ref group=note>מלכים א י"ד, ט"ו</ref> |
|- | |- | ||
|פעם לאסור ופעם להתיר | |פעם לאסור ופעם להתיר | ||
| Line 102: | Line 102: | ||
|בחכמת הטבע ודומיהן | |בחכמת הטבע ודומיהן | ||
|- | |- | ||
| − | |ובלומדי אזן [לא יפתחו] לשמוע כלימודים | + | |ובלומדי אזן [לא יפתחו]‫<ref>marg.</ref> לשמוע כלימודים |
|לכן קצה נפשם בכל חכמת הלימודיות | |לכן קצה נפשם בכל חכמת הלימודיות | ||
|- | |- | ||
| Line 129: | Line 129: | ||
|כי יהיה להם לשחוק | |כי יהיה להם לשחוק | ||
|- | |- | ||
| − | |[כי יהיה להם לשחוק] | + | |‫[כי יהיה להם לשחוק]‫<ref>marg.</ref> |
|ולמחוייב ולנמנע לא ישימו חוק | |ולמחוייב ולנמנע לא ישימו חוק | ||
|- | |- | ||
| Line 138: | Line 138: | ||
|כי אין עסקם בפשוטות | |כי אין עסקם בפשוטות | ||
|- | |- | ||
| − | |ומה ''יתרון לבעל הלשון''‫<ref>קהלת י, י"א</ref> | + | |ומה ''יתרון לבעל הלשון''‫<ref group=note>קהלת י, י"א</ref> |
|מושגות בבבת עין ואישון | |מושגות בבבת עין ואישון | ||
|- | |- | ||
| Line 144: | Line 144: | ||
|ואם כה יאמרו חכמים | |ואם כה יאמרו חכמים | ||
|- | |- | ||
| − | |והאלהים עשה אותם ישר‫<ref>קהלת ז, כ"ט</ref> | + | |והאלהים עשה אותם ישר‫<ref group=note>קהלת ז, כ"ט</ref> |
|מה יעשו הסכלים אשר לא ידעו אי זה יכשר | |מה יעשו הסכלים אשר לא ידעו אי זה יכשר | ||
|- | |- | ||
| Line 157: | Line 157: | ||
|- | |- | ||
|למאות לאלפים ולרבבות | |למאות לאלפים ולרבבות | ||
| − | |''מאליפות מרובבות''‫<ref>תהילים קמד, י"ג</ref> | + | |''מאליפות מרובבות''‫<ref group=note>תהילים קמד, י"ג</ref> |
|- | |- | ||
|מדרגה תחת מדרגה חונים | |מדרגה תחת מדרגה חונים | ||
| Line 169: | Line 169: | ||
|- | |- | ||
|וברצונם יעקרו שוד וישנו את תפקידם | |וברצונם יעקרו שוד וישנו את תפקידם | ||
| − | |יען באפם יגזלו מאנשי ה[ה]ודם ומאודם | + | |יען באפם יגזלו מאנשי ה‫[ה]‫<ref>marg.</ref>ודם ומאודם |
|- | |- | ||
|ומהם בפולי ועדשים וכל מיני זרעונים | |ומהם בפולי ועדשים וכל מיני זרעונים | ||
| Line 183: | Line 183: | ||
|זה ישכיב וזה יחריד | |זה ישכיב וזה יחריד | ||
|- | |- | ||
| − | |colspan="2"|זה ישפיל וזה ירים‫<ref>תהילים ע"ה, ח</ref> | + | |colspan="2"|זה ישפיל וזה ירים‫<ref group=note>תהילים ע"ה, ח</ref> |
|- | |- | ||
|colspan="2"|זה יגנוב הדינרים | |colspan="2"|זה יגנוב הדינרים | ||
|- | |- | ||
| − | |colspan="2"|וזה יאכל ''ב' ג' גרגרים''‫<ref>ישעיה י"ז, ו</ref> | + | |colspan="2"|וזה יאכל ''ב' ג' גרגרים''‫<ref group=note>ישעיה י"ז, ו</ref> |
|- | |- | ||
|ירא וחרד על דברו | |ירא וחרד על דברו | ||
|ואשר על יי שברו | |ואשר על יי שברו | ||
|- | |- | ||
| − | |[מהדק] | + | |‫[מהדק]‫<ref>marg.</ref> מה<sup>מ</sup>דקדקים |
|להחזיק ולהקים | |להחזיק ולהקים | ||
|- | |- | ||
| Line 211: | Line 211: | ||
|colspan="2"|וחלק לו בבינה | |colspan="2"|וחלק לו בבינה | ||
|- | |- | ||
| − | |colspan="2"|הבדילו מן התועים ומעשה ידיהו כוננה‫<ref>תהילים צ, י"ז</ref> | + | |colspan="2"|הבדילו מן התועים ומעשה ידיהו כוננה‫<ref group=note>תהילים צ, י"ז</ref> |
|- | |- | ||
|colspan="2"|והיו ידיו אמונה | |colspan="2"|והיו ידיו אמונה | ||
| Line 221: | Line 221: | ||
|colspan="2"|וכל אחד את רעהו יונה | |colspan="2"|וכל אחד את רעהו יונה | ||
|- | |- | ||
| − | |colspan="2"|והוא שומר דרכי אל ומי כמוהו מונה‫<ref>איוב ל"ו, כ"ב</ref> | + | |colspan="2"|והוא שומר דרכי אל ומי כמוהו מונה‫<ref group=note>איוב ל"ו, כ"ב</ref> |
|- | |- | ||
| | | | ||
| Line 234: | Line 234: | ||
|colspan="2"|''מולל ברגליו'' | |colspan="2"|''מולל ברגליו'' | ||
|- | |- | ||
| − | |colspan="2"|''מורה באצבעותיו''‫<ref>משלי ו, יג</ref> | + | |colspan="2"|''מורה באצבעותיו''‫<ref group=note>משלי ו, יג</ref> |
|- | |- | ||
|ורוע מזלו | |ורוע מזלו | ||
| Line 244: | Line 244: | ||
|colspan="2"|לבל יחליף וימיר | |colspan="2"|לבל יחליף וימיר | ||
|- | |- | ||
| − | |colspan="2"|ולא יאכל ''גרגרים מראש אמיר''‫<ref>ישעיה י"ז, ו</ref> | + | |colspan="2"|ולא יאכל ''גרגרים מראש אמיר''‫<ref group=note>ישעיה י"ז, ו</ref> |
|- | |- | ||
|colspan="2"|לחלוק לחקוק אותו בלוח ברזל בצפורן שמיר | |colspan="2"|לחלוק לחקוק אותו בלוח ברזל בצפורן שמיר | ||
|- | |- | ||
|לנצח יוכל להבחן במופתיו | |לנצח יוכל להבחן במופתיו | ||
| − | |אהל בל יצען בל יסע יתדותיו‫<ref>ישעיה לג, כ</ref> | + | |אהל בל יצען בל יסע יתדותיו‫<ref group=note>ישעיה לג, כ</ref> |
|- | |- | ||
|בכל מיני אזון וחקור | |בכל מיני אזון וחקור | ||
| − | |יתברר ויתלבן בבחינה [ובכור] | + | |יתברר ויתלבן בבחינה [ובכור]‫<ref>marg.</ref> ובקור |
|- | |- | ||
|colspan="2"|הן כל אלה הדברי' מחלישים דעתי | |colspan="2"|הן כל אלה הדברי' מחלישים דעתי | ||
| Line 260: | Line 260: | ||
|colspan="2"|מרפים ידי לשמור משמרתי | |colspan="2"|מרפים ידי לשמור משמרתי | ||
|- | |- | ||
| − | |אהבה מקלקלת את השורה | + | |אהבה מקלקלת <sup>את השורה</sup> |
|וחבתן על כולם גברה | |וחבתן על כולם גברה | ||
|- | |- | ||
| Line 275: | Line 275: | ||
|colspan="2"|להעשר סידורה | |colspan="2"|להעשר סידורה | ||
|- | |- | ||
| − | |colspan="2"|כמשפט הבכורה‫<ref>דברים כ"א, י"ז</ref> | + | |colspan="2"|כמשפט הבכורה‫<ref group=note>דברים כ"א, י"ז</ref> |
|- | |- | ||
| | | | ||
|- | |- | ||
| − | |colspan="2"|וחברתי קיצור זה בדרכי המספר | + | |colspan="2"|וחברתי קיצור זה בדרכי המספר וטעמי<sup>הם</sup> |
|- | |- | ||
| | | | ||
| Line 295: | Line 295: | ||
|ולהורות הוראות | |ולהורות הוראות | ||
|- | |- | ||
| − | |כאיש אשר לא שומע [יודע] | + | |כאיש אשר לא <s>שומע</s> ‫[יודע]‫<ref>marg.</ref> ומ<sup>י</sup>מיו לא ראה מאורות |
| − | |אבל כמק<s>ט</s>לט שבלים‫<ref>ישעיה י"ז, ה</ref> ואורות | + | |אבל כמק<s>ט</s>לט שבלים‫<ref group=note>ישעיה י"ז, ה</ref> ואורות |
|- | |- | ||
|colspan="2"|ואם מדעתי אמציא המצאות | |colspan="2"|ואם מדעתי אמציא המצאות | ||
| Line 313: | Line 313: | ||
|לרבים אותם יודיעו | |לרבים אותם יודיעו | ||
|- | |- | ||
| − | |וגם חוכמות שרותיה תענינה | + | |וגם חוכמות <s>שרותיה תענינה</s> |
|ויאמרו אמת ואמונה | |ויאמרו אמת ואמונה | ||
|- | |- | ||
| Line 322: | Line 322: | ||
|colspan="2"|עזב את הגבירות מרבות השררה והגדולה | |colspan="2"|עזב את הגבירות מרבות השררה והגדולה | ||
|- | |- | ||
| − | |ו''רבות בנות עשו חיל''‫<ref>משלי לא, כט</ref> ופירות | + | |ו''רבות בנות עשו חיל''‫<ref group=note>משלי לא, כט</ref> ופירות |
|אשר להם סנסנים ופארות | |אשר להם סנסנים ופארות | ||
|- | |- | ||
| Line 335: | Line 335: | ||
|- | |- | ||
|והניח את הספק צפון ונעלם | |והניח את הספק צפון ונעלם | ||
| − | |אחז את [...] המפורסם ובריא אולם‫<ref>תהילים עג, ד</ref> | + | |אחז את [...] המפורסם ובריא אולם‫<ref group=note>תהילים עג, ד</ref> |
|- | |- | ||
| Line 355: | Line 355: | ||
|אשר הוא קוצר השגתי | |אשר הוא קוצר השגתי | ||
|- | |- | ||
| − | |לא נקטף עודנו באבו‫<ref>איוב ח, יב</ref> | + | |לא נקטף עודנו באבו‫<ref group=note>איוב ח, יב</ref> |
|יתד שהכל תלוי בו | |יתד שהכל תלוי בו | ||
|- | |- | ||
| Line 363: | Line 363: | ||
|colspan="2"|אכן יודע האמת ידע כי כוונתי לשום שמים | |colspan="2"|אכן יודע האמת ידע כי כוונתי לשום שמים | ||
|- | |- | ||
| − | |colspan="2"|לפתוח פתח ''בריח ודלתים''‫<ref>איוב לח, י</ref> | + | |colspan="2"|לפתוח פתח ''בריח ודלתים''‫<ref group=note>איוב לח, י</ref> |
|- | |- | ||
|colspan="2"|ולהציע הצעות ולהבין הבנות למלאכת השמים | |colspan="2"|ולהציע הצעות ולהבין הבנות למלאכת השמים | ||
| Line 374: | Line 374: | ||
|colspan="2"|במיעוט שכלי המפורסם להעמיד הבית והחדרים אשר עליהם נשענים | |colspan="2"|במיעוט שכלי המפורסם להעמיד הבית והחדרים אשר עליהם נשענים | ||
|- | |- | ||
| − | |colspan="2"|וזה אחל לעשות בעזרת שוכן מרומים מעונים | + | |colspan="2"|וזה אחל לעשות בעזרת שוכן <s>מרומים</s> מעונים |
|} | |} | ||
| Line 381: | Line 381: | ||
| | | | ||
| − | = Introduction: The Positional Decimal System = | + | == <span style=color:Green>Introduction: The Positional Decimal System</span> == |
| | | | ||
|- | |- | ||
| − | |The numerals | + | |The numerals [...] in the books of the Gentile sages are these [corresponding to Hebrew letters]: |
| − | |style="text-align:right;"|הרשמים ה[...] במספר בספרי חכמי הגוים הם אלו | + | |style="width:45%; text-align:right;"|הרשמים ה[...] במספר בספרי חכמי הגוים הם אלו |
|- | |- | ||
| | | | ||
| Line 422: | Line 422: | ||
|} | |} | ||
|- | |- | ||
| − | | | + | |The last numeral is called '''Sifra''' [= zero], which is not a number. |
|style="text-align:right;"|וזה ה{{#annot:term|204,1823|O5d7}}רושם{{#annotend:O5d7}} האחרון הנקרא {{#annot:term|205,1554|E3nK}}סיפרא{{#annotend:E3nK}} אינננו מספר | |style="text-align:right;"|וזה ה{{#annot:term|204,1823|O5d7}}רושם{{#annotend:O5d7}} האחרון הנקרא {{#annot:term|205,1554|E3nK}}סיפרא{{#annotend:E3nK}} אינננו מספר | ||
|- | |- | ||
| − | |The meaning of the decimal places | + | |<span style=color:Green>The meaning of the decimal places:</span> Each rank is given in this science its numerical role, as will be explained, to indicate the numerical ranks that follow it. |
| − | |style="text-align:right;"|אכן הונח בזאת החכמה בכל מעלה ומעלה חלקה ממספר כמו שיתבאר כדי להראות מעלות המספרים הבאים אחריה | + | |style="text-align:right;"|אכן הונח בזאת החכמה בכל מעלה <s>ומעלה</s> חלקה ממספר כמו שיתבאר כדי להראות מעלות המספרים הבאים אחריה |
|- | |- | ||
| − | | | + | |These ranks start from the right. |
|style="text-align:right;"|ואלו ה{{#annot:term|203,1316|Ze8E}}מעלות{{#annotend:Ze8E}} מתחילות מהימין | |style="text-align:right;"|ואלו ה{{#annot:term|203,1316|Ze8E}}מעלות{{#annotend:Ze8E}} מתחילות מהימין | ||
|- | |- | ||
| − | | | + | |Each rank to the left is ten times the preceding [rank]. |
|style="text-align:right;"|וכל {{#annot:term|203,1316|Yypw}}מעלה{{#annotend:Yypw}} העולה היא לצד שמאל עולה עשר ידות מאשר לפניה | |style="text-align:right;"|וכל {{#annot:term|203,1316|Yypw}}מעלה{{#annotend:Yypw}} העולה היא לצד שמאל עולה עשר ידות מאשר לפניה | ||
|- | |- | ||
| − | !The written ranks [= decimal places] | + | !<span style=color:Green>The written ranks [= decimal places]</span> |
| | | | ||
|- | |- | ||
| | | | ||
| − | * | + | *I.e. the numeral that is in the first rank are the units. |
|style="text-align:right;"|ר"ל שרושם המספר אשר במעלה הא' יהיו אחדים | |style="text-align:right;"|ר"ל שרושם המספר אשר במעלה הא' יהיו אחדים | ||
|- | |- | ||
| | | | ||
| − | * | + | *In the second - the tens. |
|style="text-align:right;"|ובשנית עשרות | |style="text-align:right;"|ובשנית עשרות | ||
|- | |- | ||
| | | | ||
| − | * | + | *In the third - the hundreds. |
|style="text-align:right;"|ובג' מאות | |style="text-align:right;"|ובג' מאות | ||
|- | |- | ||
| | | | ||
| − | * | + | *In the fourth - the thousands |
|style="text-align:right;"|ובד' אלפים | |style="text-align:right;"|ובד' אלפים | ||
|- | |- | ||
| − | | | + | |And so on, so that these numerals: 30678002 are thirty thousands of thousands that are called millions, six hundred and seventy-eight thousand, and two. |
|style="text-align:right;"|וכן לעולם בענין ש{{#annot:term|204,1823|RC87}}רשמים{{#annotend:RC87}} אלו 30678002 עולים שלשים אלפי אלפים הנקראים {{#annot:term|350,1200|PJuV}}חשבונות{{#annotend:PJuV}} ‫<ref>4r</ref>ושש מאות ושבעים ושמונת אלפים ושנים | |style="text-align:right;"|וכן לעולם בענין ש{{#annot:term|204,1823|RC87}}רשמים{{#annotend:RC87}} אלו 30678002 עולים שלשים אלפי אלפים הנקראים {{#annot:term|350,1200|PJuV}}חשבונות{{#annotend:PJuV}} ‫<ref>4r</ref>ושש מאות ושבעים ושמונת אלפים ושנים | ||
|- | |- | ||
| − | |The significance of the zeros as a place holders | + | |<span style=color:Green>The significance of the zeros as a place holders:</span> Since there are no tens and hundreds, here, as well as units of millions, the zeros are written instead of them, to indicate the rest of the numerals, because without them these numerals would indicat thirty-six thousand, seven hundred, and eighty-two [36782]. Deduce from this. |
|style="text-align:right;"|ולפי שאין בכאן עשרות ומאות גם אח<sup>ד</sup>י חשבונות הושמו ה{{#annot:term|205,1554|p3vj}}ספרות{{#annotend:p3vj}} במקומם להורות מעלות שאר המספרים כי זולתם לא היו עולים רשמים אלו כי אם שלשים ושש אלף ושבע מאות ושמונים ושנים והקש על זה | |style="text-align:right;"|ולפי שאין בכאן עשרות ומאות גם אח<sup>ד</sup>י חשבונות הושמו ה{{#annot:term|205,1554|p3vj}}ספרות{{#annotend:p3vj}} במקומם להורות מעלות שאר המספרים כי זולתם לא היו עולים רשמים אלו כי אם שלשים ושש אלף ושבע מאות ושמונים ושנים והקש על זה | ||
|- | |- | ||
| Line 465: | Line 465: | ||
| | | | ||
| − | = Table of Contents = | + | == <span style=color:Green>Table of Contents</span> == |
| | | | ||
|- | |- | ||
| − | | | + | |I have divided this book into two sections: |
| − | |style="text-align:right;"|וחלקתי הספר לב' חלקים | + | |style="width:45%; text-align:right;"|וחלקתי הספר לב' חלקים |
|- | |- | ||
| − | | | + | |The first section of integers |
|style="text-align:right;"|<big>החלק הא' בשלמים</big> | |style="text-align:right;"|<big>החלק הא' בשלמים</big> | ||
| + | |- | ||
| + | |The second section on fractions and in it six chapters, including an introduction, in which there are three parts. | ||
| + | |style="text-align:right;"|<big>החלק הב'</big> [בשברים]‫<ref>marg.</ref> <s>בשברים</s> ובו ששה פרקים ובו הקדמה ובה ג' שערי‫' | ||
|- | |- | ||
| | | | ||
| − | | | + | {| class="wikitable" |
|- | |- | ||
| − | | | + | |The first part on decomposing to a fraction |
| + | |The first chapter on addition | ||
| + | |- | ||
| + | |The second part on multiplication [= fractions of fractions] | ||
| + | |The first chapter on subtraction | ||
| + | |- | ||
| + | |The third part on expansion to a common denominator | ||
| + | |The third chapter on multiplication | ||
| + | |- | ||
| + | |In it also are six chapters on fractions | ||
| + | |The fourth chapter on division | ||
| + | |- | ||
| + | |The first chapter on addition | ||
| + | |The fifth chapter on proportions | ||
| + | |- | ||
| + | |The five remaining chapters are as stated on integers | ||
| + | |The sixth chapter on roots | ||
| + | |} | ||
| | | | ||
{| class="wikitable" style="margin-left: auto; margin-right: auto; border: none;" | {| class="wikitable" style="margin-left: auto; margin-right: auto; border: none;" | ||
| Line 500: | Line 520: | ||
|} | |} | ||
|- | |- | ||
| − | | | + | |Discussion on conversion and summing. |
| − | |style="text-align:right;"|‫[מאמ' ההמרה גם מאמר האחדות‫] | + | |style="text-align:right;"|‫[מאמ' ההמרה גם מאמר האחדות‫]‫<ref>marg.</ref> |
| + | |- | ||
| + | |There is in it also a rule that includes matters that are useful for all chapters of the fractions. | ||
| + | |style="text-align:right;"|ועוד <sup>בו</sup> כלל אחד כולל עניינים מועילים לכל פרקי השברים | ||
|- | |- | ||
|} | |} | ||
| Line 819: | Line 842: | ||
| | | | ||
*When the whole procedure is complete, if there are still digits on the upper row, under which there is no number, nor 0, or a dot, since all is complete, put them as a remainder under the line, as they are. | *When the whole procedure is complete, if there are still digits on the upper row, under which there is no number, nor 0, or a dot, since all is complete, put them as a remainder under the line, as they are. | ||
| − | |style="text-align:right;"|וכאשר כלית כל מלאכתך אם נשארו עוד רשמים בשורה העליונה אשר אין תחתיהן לא מספר ולא 0 ולא נקודה שכבר נשלם הכל תשימם ל{{#annot:term| | + | |style="text-align:right;"|וכאשר כלית כל מלאכתך אם נשארו עוד רשמים בשורה העליונה אשר אין תחתיהן לא מספר ולא 0 ולא נקודה שכבר נשלם הכל תשימם ל{{#annot:term|184,2454|fYDP}}שארית{{#annotend:fYDP}} תחת הקו כמות שהן |
|- | |- | ||
| | | | ||
| Line 897: | Line 920: | ||
:*<span style="color:Green>Fourth rank:</span> We go to the fourth rank and we find there a dot, but there is no number in the fourth rank on the bottom row to add to it, only 0, so we subtract this one alone from what is found in the fourth rank on the upper row. Yet, we cannot since there is no number there but 0, so we borrow one from the fifth rank and put a dot beneath it. The 1 becomes a ten, we subtract from it 1, 9 remain. We put them under the line, corresponding to the [fourth] rank. | :*<span style="color:Green>Fourth rank:</span> We go to the fourth rank and we find there a dot, but there is no number in the fourth rank on the bottom row to add to it, only 0, so we subtract this one alone from what is found in the fourth rank on the upper row. Yet, we cannot since there is no number there but 0, so we borrow one from the fifth rank and put a dot beneath it. The 1 becomes a ten, we subtract from it 1, 9 remain. We put them under the line, corresponding to the [fourth] rank. | ||
::<math>\scriptstyle{\color{blue}{10-\left(1+0\right)=10-1=9}}</math> | ::<math>\scriptstyle{\color{blue}{10-\left(1+0\right)=10-1=9}}</math> | ||
| − | |style="text-align:right;"|ונלך למעלה הרביעית ונמצא שם נקדה ואין מספר במעלה הרביעית ההיא בשורה התחתונה ההיא לחברו עמו כי אם 0 לכן נחסר זה האחד לבדו מהנמצא במעלה הרביעית ההיא בשורה העליונה ולא נוכל כי אין שם מספר כי אם 0 לכן נקרא <s>ראשון</s> <sup>אחד</sup> מהמעלה החמישית ונשים תחתיה נקדה וזה הא' יהיה לנו לעשר | + | |style="text-align:right;"|ונלך למעלה הרביעית ונמצא שם נקדה ואין מספר במעלה הרביעית ההיא בשורה התחתונה ההיא לחברו עמו כי אם 0 לכן נחסר זה האחד לבדו מהנמצא במעלה הרביעית ההיא בשורה העליונה ולא נוכל כי אין שם מספר כי אם 0 לכן נקרא <s>ראשון</s> <sup>אחד</sup> מהמעלה החמישית ונשים תחתיה נקדה וזה הא' יהיה לנו לעשר נסי<sup>ר</sup>ם ממנו ה 1 ישארו 9 נשימם תחת הקו כנגד המעלה ההיא |
|- | |- | ||
| | | | ||
| Line 958: | Line 981: | ||
|- | |- | ||
|When you want to multiply a number by a number, i.e. to see how much are the multiples of one number when multiplied by the multiples of the other number, write the two forms of these numbers one above the other by the order and draw an ink line beneath them. | |When you want to multiply a number by a number, i.e. to see how much are the multiples of one number when multiplied by the multiples of the other number, write the two forms of these numbers one above the other by the order and draw an ink line beneath them. | ||
| − | |style="text-align:right;"|כאשר תרצה לכפול מספר במספר ר"ל לראות כמה יעלו כפלי המספר האחד כשיוכפל כפלים בחשבון המספר האחר שיש [שים] שתי צורות מספרים אלו זו על זו על הסדר ותרשום קו דיו תחתיהן | + | |style="text-align:right;"|כאשר תרצה לכפול מספר במספר ר"ל לראות כמה יעלו כפלי המספר האחד כשיוכפל כפלים בחשבון המספר האחר שיש [שים]‫<ref>marg.</ref> שתי צורות מספרים אלו זו על זו על הסדר ותרשום קו דיו תחתיהן |
|- | |- | ||
| | | | ||
*Multiply the first upper digit by each of the bottom digits and always write the units of the result of its product by each of the bottom digit beneath the line, under that bottom digit and keep the tens to add them as units to its product by the following digit to the left. | *Multiply the first upper digit by each of the bottom digits and always write the units of the result of its product by each of the bottom digit beneath the line, under that bottom digit and keep the tens to add them as units to its product by the following digit to the left. | ||
| − | |style="text-align:right;"|וכפול המספר הראשון העליון בכל אחד מהמספרים התחתונים [וכאשר תעלה מכפלו עם כל אחד מהמספרים התחתונים תשים לעולם] תשים לעולם האחדים תחת הקו כנגד המספר התחתון ההוא והעשרות תשמור והיו לאחדים בידך לחברם עם העולה מכפלו עם המספר הנמשך אליו לצד שמאלי | + | |style="text-align:right;"|וכפול המספר הראשון העליון בכל אחד מהמספרים התחתונים [וכאשר תעלה מכפלו עם כל אחד מהמספרים התחתונים תשים לעולם]‫<ref>marg.</ref> תשים לעולם האחדים תחת הקו כנגד המספר התחתון ההוא והעשרות תשמור והיו לאחדים בידך לחברם עם העולה מכפלו עם המספר הנמשך אליו לצד שמאלי |
|- | |- | ||
| | | | ||
:*If there is no number in the following rank of the bottom line since it is already completed or since there is a zero there, write the [reserved] number that you have in the following rank under the line. | :*If there is no number in the following rank of the bottom line since it is already completed or since there is a zero there, write the [reserved] number that you have in the following rank under the line. | ||
| − | |style="text-align:right;"|ואם לא ‫<ref>8r</ref>יהיה במעלה שאחר <s>או</s> <sup>זו</sup> שום מספר בשורה התחתונה שנשלמה כבר או שיש שם [סיפרא] תשים במעלה שאחר זו תחת הקו מספר האחדים אשר בידך | + | |style="text-align:right;"|ואם לא ‫<ref>8r</ref>יהיה במעלה שאחר <s>או</s> <sup>זו</sup> שום מספר בשורה התחתונה שנשלמה כבר או שיש שם [סיפרא]‫<ref>marg.</ref> תשים במעלה שאחר זו תחת הקו מספר האחדים אשר בידך |
|- | |- | ||
| | | | ||
| Line 988: | Line 1,011: | ||
|- | |- | ||
|Accordingly, the decimal place of the units of the product of any upper digit by any bottom digit is always as the sum of the decimal places of both digits minus one. | |Accordingly, the decimal place of the units of the product of any upper digit by any bottom digit is always as the sum of the decimal places of both digits minus one. | ||
| − | |style="text-align:right;"|עד שיצא לנו מזה שלעולם מספר מעלות מקום אחדי כפל שום מספר עליון עם שום מספר תחתון יהיה כמנין מעלות שני המספרים {{#annot:term| | + | |style="text-align:right;"|עד שיצא לנו מזה שלעולם מספר מעלות מקום אחדי כפל שום מספר עליון עם שום מספר תחתון יהיה כמנין מעלות שני המספרים {{#annot:term|178,2083|2zri}}מחוברות יחד{{#annotend:2zri}} חסר אחת |
|- | |- | ||
| | | | ||
| Line 1,008: | Line 1,031: | ||
| | | | ||
| − | ==== Example ==== | + | ==== <span style=color:Green>Example</span> ==== |
| − | |||
|- | |- | ||
| | | | ||
| − | *{{#annot:9007500×5400920|156|Anhy}} | + | *{{#annot:9007500×5400920|156|Anhy}}Example: we wish to multiply the number 9007500 by another number 5400920. |
:<math>\scriptstyle9007500\times5400920</math> | :<math>\scriptstyle9007500\times5400920</math> | ||
| − | |style="text-align:right;"|רצינו לכפול מספר 9007500 במספר אחר שהוא 5400920{{#annotend:Anhy}} | + | |style="text-align:right;"|המשל רצינו לכפול מספר 9007500 במספר אחר שהוא 5400920{{#annotend:Anhy}} |
|- | |- | ||
| | | | ||
| Line 1,081: | Line 1,103: | ||
::*Multiply the 5 by the zero that is in the first rank of the bottom number; it is zero. Place it after the two mentioned zeros, which is the third rank that [precedes] the fourth rank. It follows that its row starts from the third rank. | ::*Multiply the 5 by the zero that is in the first rank of the bottom number; it is zero. Place it after the two mentioned zeros, which is the third rank that [precedes] the fourth rank. It follows that its row starts from the third rank. | ||
:::<math>\scriptstyle{\color{blue}{5\times0=0}}</math> | :::<math>\scriptstyle{\color{blue}{5\times0=0}}</math> | ||
| − | |style="text-align:right;"|ותכפול ה5 בסיפרא שהיא אשר במעלה הראשונה מהמספר התחתון ויהיה סיפרא ותשימנה אחר השתי סיפרות הנזכרות שהיא המעלה השלישית הדומה למעלה הרביעית והנה בא על מתכונתו ששורתו מתחלת במעלה הג' שהיא מעלתו | + | |style="text-align:right;"|ותכפול ה5 בסיפרא שהיא אשר במעלה הראשונה מהמספר התחתון ויהיה סיפרא ותשימנה ‫<ref>9r</ref>אחר השתי סיפרות הנזכרות שהיא המעלה השלישית הדומה למעלה הרביעית והנה בא על מתכונתו ששורתו מתחלת במעלה הג' שהיא מעלתו |
|- | |- | ||
| | | | ||
| Line 1,091: | Line 1,113: | ||
::*Keep the 10 as one, to add it to the product of 5 by 9, which is 45; the total sum is 46. Place the 6 after all the zeros and keep the 4. | ::*Keep the 10 as one, to add it to the product of 5 by 9, which is 45; the total sum is 46. Place the 6 after all the zeros and keep the 4. | ||
:::<math>\scriptstyle{\color{blue}{1+\left(5\times9\right)=1+45=46}}</math> | :::<math>\scriptstyle{\color{blue}{1+\left(5\times9\right)=1+45=46}}</math> | ||
| − | |style="text-align:right;"|ותשמור ה-10 לאחד לחברו עם כפל 5 ב-9 שהוא 45 ויהיה הכל | + | |style="text-align:right;"|ותשמור ה-10 לאחד לחברו עם כפל 5 ב-9 שהוא 45 ויהיה הכל <sup>4</sup>6 ותשים ה6 אחר כל הסיפרות ותשמ<sup>ו</sup>ר ה-4 |
|- | |- | ||
| | | | ||
| Line 1,137: | Line 1,159: | ||
::*Say also: the product of 7 by 2 is 14. Place 4, which are the units, after the mentioned zeros and keep the 1. | ::*Say also: the product of 7 by 2 is 14. Place 4, which are the units, after the mentioned zeros and keep the 1. | ||
:::<math>\scriptstyle{\color{blue}{7\times2=14}}</math> | :::<math>\scriptstyle{\color{blue}{7\times2=14}}</math> | ||
| − | |style="text-align:right;"|ותאמר עוד כפל 7 [ב2 ויעלו 14 תשים 4 שהם האחדים אחר הסיפרות הנזכרות ותשמור הא' | + | |style="text-align:right;"|ותאמר עוד כפל 7 ‫[ב2 ויעלו 14 תשים 4 שהם האחדים אחר הסיפרות הנזכרות ותשמור הא‫' |
|- | |- | ||
| | | | ||
::*Say also: the product of 7 by 9 is 63; plus the one that we have the result is 64. We put the 4 and keep the 6 as units. | ::*Say also: the product of 7 by 9 is 63; plus the one that we have the result is 64. We put the 4 and keep the 6 as units. | ||
:::<math>\scriptstyle{\color{blue}{1+\left(7\times9\right)=1+63=64}}</math> | :::<math>\scriptstyle{\color{blue}{1+\left(7\times9\right)=1+63=64}}</math> | ||
| − | |style="text-align:right;"|ותאמר עוד כפל 7 ב9] ב9 ויעלה 63 ועוד האחד אשר בידינו יעלה 64 נשים ה4 ונשמר ה6 לאחדים בידינו | + | |style="text-align:right;"|ותאמר עוד כפל 7 ב9]‫<ref>marg.</ref> ב9 ויעלה 63 ועוד האחד אשר בידינו יעלה 64 נשים ה4 ונשמר ה6 לאחדים בידינו |
|- | |- | ||
| | | | ||
::*We say also: the product of 7 by zero is zero. Since we have a 6, we do not put a zero, but we put the 6 that we have in its place. | ::*We say also: the product of 7 by zero is zero. Since we have a 6, we do not put a zero, but we put the 6 that we have in its place. | ||
:::<math>\scriptstyle{\color{blue}{6+\left(7\times0\right)=6+0=6}}</math> | :::<math>\scriptstyle{\color{blue}{6+\left(7\times0\right)=6+0=6}}</math> | ||
| − | |style="text-align:right;"|ונאמר עוד כפל 7 בסיפרא היה עולה סיפרא אכן להיות בידינו ה6 לא נשים סיפרא אבל נשים ה6 אשר בידינו למקומה | + | |style="text-align:right;"|‫<ref>9v</ref>ונאמר עוד כפל 7 בסיפרא היה עולה סיפרא אכן להיות בידינו ה6 לא נשים סיפרא אבל נשים ה6 אשר בידינו למקומה |
|- | |- | ||
| | | | ||
| Line 1,171: | Line 1,193: | ||
::*Again we say: the product of 9 by zero is zero. Put a zero after the two zeros that we placed at the beginning of this line. | ::*Again we say: the product of 9 by zero is zero. Put a zero after the two zeros that we placed at the beginning of this line. | ||
:::<math>\scriptstyle{\color{blue}{9\times0=0}}</math> | :::<math>\scriptstyle{\color{blue}{9\times0=0}}</math> | ||
| − | |style="text-align:right;"|ושוב נאמ' כפל 9 בסיפרא הוא סיפרא ותשים סיפרא אחר השתי סיפרות כאשר שמנו | + | |style="text-align:right;"|ושוב נאמ' כפל 9 בסיפרא הוא סיפרא ותשים סיפרא אחר השתי סיפרות כאשר שמנו ב<s>ג</s>[ת]‫<ref>marg.</ref>חלת שורה זו |
|- | |- | ||
| | | | ||
| Line 1,201: | Line 1,223: | ||
::*We say: the product of 9 by 5 is 45; plus the 3 that we have the result is 48. We put the 8 and after it the 4, because the multiplication of the 9 by all the bottom digits is complete. | ::*We say: the product of 9 by 5 is 45; plus the 3 that we have the result is 48. We put the 8 and after it the 4, because the multiplication of the 9 by all the bottom digits is complete. | ||
:::<math>\scriptstyle{\color{blue}{3+\left(9\times5\right)=3+45=48}}</math> | :::<math>\scriptstyle{\color{blue}{3+\left(9\times5\right)=3+45=48}}</math> | ||
| − | |style="text-align:right;"|ונאמר כפל 9 ב5 עולה 45 ו3 אשר בידינו יעלו 48 ונשים 8 ואחריהן 7 [4] כי כבר נשלם כפל 9 זה בכל הרשמים התחתונים | + | |style="text-align:right;"|ונאמר כפל 9 ב5 עולה 45 ו3 אשר בידינו יעלו 48 ונשים 8 ואחריהן <s>7</s> [4]‫<ref>marg.</ref> כי כבר נשלם כפל 9 זה בכל הרשמים התחתונים |
|- | |- | ||
| | | | ||
:*Since the upper digits were already multiplied by all the bottom [digits], we draw a line beneath all the lines and sum all the lines that were generated from their products, i.e. the three lines between the lines. | :*Since the upper digits were already multiplied by all the bottom [digits], we draw a line beneath all the lines and sum all the lines that were generated from their products, i.e. the three lines between the lines. | ||
| − | |style="text-align:right;"|ואחרי אשר כבר נכפלו ר"ל הרשמים העליונים עם כל התחתונים נרשום תחת כל השורות קו דיו ונחבר כל השורות שנתחדשו מכפליהן ר"ל ה3 שורות אשר בין הקוים | + | |style="text-align:right;"|ואחרי אשר כבר נכפלו ר"ל הרשמים העליונים עם כל התחתונים נרשום תחת כל השורות קו דיו ונחבר כל השורות שנתחדשו מכפליהן ר"ל ה3 שורות אשר ‫<ref>10r</ref>בין הקוים <sup>ו</sup>הנה |
|- | |- | ||
| | | | ||
| Line 1,230: | Line 1,252: | ||
| | | | ||
:*Because, e.g. if the upper number is of the hundreds, which is the third rank, when we multiply it by the units of the bottom number, the result is of the hundreds. | :*Because, e.g. if the upper number is of the hundreds, which is the third rank, when we multiply it by the units of the bottom number, the result is of the hundreds. | ||
| − | |style="text-align:right;"|כי על ד"מ אם המספר העליון הוא מאות שהוא במעלה הג' כשנכפלם באחדי המספר התחתון יהיה העולה מאות | + | |style="text-align:right;"|כי על ד"מ אם המספר העליון <sup>הוא</sup> מאות שהוא במעלה הג' כשנכפלם באחדי המספר התחתון יהיה העולה מאות |
|- | |- | ||
| | | | ||
| Line 1,250: | Line 1,272: | ||
| | | | ||
:And so on, forever it rises rank by rank, until it is clear from what is said that the place of the units resulting from the multiplication of each upper digit by a bottom [digit] is the rank whose decimal position is as the [sum] of the ranks of both upper and bottom digits that are multiplied by each other minus one. | :And so on, forever it rises rank by rank, until it is clear from what is said that the place of the units resulting from the multiplication of each upper digit by a bottom [digit] is the rank whose decimal position is as the [sum] of the ranks of both upper and bottom digits that are multiplied by each other minus one. | ||
| − | |style="text-align:right;"|וכן לעולם כאשר יתרחק יעלה מעלה אחר מעלה עד שיצא לנו מזה ברור מה שאמרנו כי אחדי כפל כל מספר עליון בתחתון יהיה מקום האחדים העולים מהכפל ההוא במעלה אשר מנין מדרגותיה כמנין מעלות שני רשמים האלו הנכפלים זה בזה העליון והתחתון יחד <sup>חסר</sup> אחת | + | |style="text-align:right;"|וכן לעולם כאשר יתרחק יעלה מעלה אחר מעלה עד שיצא לנו מזה ברור מה שאמרנו כי אחדי כפל כל מספר עליון בתחתון יהיה מקום האחדים העולים מהכפל ההוא במעלה אשר מנין מדרגותיה כמנין מעלות שני רשמים ‫<ref>10v</ref>האלו הנכפלים זה בזה העליון והתחתון יחד <sup>חסר</sup> אחת |
|- | |- | ||
| | | | ||
| Line 1,327: | Line 1,349: | ||
== Chapter Four: Division == | == Chapter Four: Division == | ||
| − | |style="width:45%; text-align:right;"|<big>הפרק הרביעי בחילוק</big> | + | |style="width:45%; text-align:right;"|‫<ref>11r</ref><big>הפרק הרביעי בחילוק</big> |
|- | |- | ||
| | | | ||
| Line 1,367: | Line 1,389: | ||
| | | | ||
:*When there is a remainder from the last digit or digits, consider them as tens or hundreds, according to their relation to the present rank, [subtract from them] as the multiples that you subtract the last bottom digit from the last upper digit or digits. | :*When there is a remainder from the last digit or digits, consider them as tens or hundreds, according to their relation to the present rank, [subtract from them] as the multiples that you subtract the last bottom digit from the last upper digit or digits. | ||
| − | |style="text-align:right;"|‫[וכאשר נשאר מהאחרון או מהאחרונים בקחתך אותם לעשרות ולמאות כפי ערכם אל המעלה הזאת ככפלים אשר הוצאת האחרון התחתון מן האחרון העליון או האחרונים‫] | + | |style="text-align:right;"|‫[וכאשר נשאר מהאחרון או מהאחרונים בקחתך אותם לעשרות ולמאות כפי ערכם אל המעלה הזאת ככפלים אשר הוצאת האחרון התחתון מן האחרון העליון או האחרונים‫]‫<ref>marg.</ref> |
|- | |- | ||
| | | | ||
:*If there is not enough, do not subtract from the last digit as much as these multiples, for you always have to subtract each [bottom digit] from its corresponding upper digit as many times as you subtract the last [bottom digit] from the last [upper] digit or digits. | :*If there is not enough, do not subtract from the last digit as much as these multiples, for you always have to subtract each [bottom digit] from its corresponding upper digit as many times as you subtract the last [bottom digit] from the last [upper] digit or digits. | ||
| − | |style="text-align:right;"|‫[ואם אין בו‫] לא תוציא <sup>לאחרון</sup> ככל הכפלים ההם כי לעולם יש לך להוציא כל אחד כל פעמים מהעליון הראוי לו כפעמים אשר תוציא האחרון מן האחרון או מן האחרונים | + | |style="text-align:right;"|‫[ואם אין בו‫]‫<ref>marg.</ref> לא תוציא <sup>לאחרון</sup> ככל הכפלים ההם כי לעולם יש לך להוציא כל אחד כל פעמים מהעליון הראוי לו כפעמים אשר תוציא האחרון מן האחרון או מן האחרונים |
|- | |- | ||
| | | | ||
:*You should know that whenever you wish to subtract the bottom digit from the upper digit and you do not find in its corresponding rank enough [to subtract] its multiples, if there is in the one that follows the consecutive [rank], you can subtract from it, provided that you keep the positional value of the ranks, so that every digit is tens to its preceding and hundreds to the one that precedes its preceding and so on according to this relation. | :*You should know that whenever you wish to subtract the bottom digit from the upper digit and you do not find in its corresponding rank enough [to subtract] its multiples, if there is in the one that follows the consecutive [rank], you can subtract from it, provided that you keep the positional value of the ranks, so that every digit is tens to its preceding and hundreds to the one that precedes its preceding and so on according to this relation. | ||
| − | |style="text-align:right;"|א[כן] יש לך לדעת כי בכל עת שתרצה להוציא <sup>ה</sup>תחתון מ<sup>ה</sup>עליון ואין דיו לכופליו כאשר תמצא במעלה הראויה לו שאם יש בנמשך אחר הנמשך תוכל להוציא ממנו ובלבד שתשמור לעולם ערך המעלות שכל מספר הוא במעלה שלפניו לעשרות ואשר לפני פניו למאות וכן לעולם על הערך הזה | + | |style="text-align:right;"|א[כן] יש לך לדעת כי בכל עת שתרצה להוציא <sup>ה</sup>תחתון מ<sup>ה</sup>עליון ואין דיו לכופליו כאשר תמצא במעלה הראויה לו שאם יש בנמשך אחר הנמשך תוכל להוציא ממנו ובלבד שתשמור לעולם ערך המעלות שכל מספר הוא במעלה שלפניו לעשרות ואשר לפני פניו למאות וכן לעולם ‫<ref>11v</ref>על הערך הזה |
|- | |- | ||
| | | | ||
| Line 1,415: | Line 1,437: | ||
| | | | ||
*<span style="color:Green">The decimal place of the interim result:</span> Always remember to place each time the result of division of that time, i.e. the multiples that you subtract at that time, corresponding to the rank from which the first bottom digit is subtracted, i.e. the [digit] that is in the first rank, if there is a number there, or if there is only a zero there - see from where it should be subtracted, if there is a number there, and place there the result of division of that time. | *<span style="color:Green">The decimal place of the interim result:</span> Always remember to place each time the result of division of that time, i.e. the multiples that you subtract at that time, corresponding to the rank from which the first bottom digit is subtracted, i.e. the [digit] that is in the first rank, if there is a number there, or if there is only a zero there - see from where it should be subtracted, if there is a number there, and place there the result of division of that time. | ||
| − | |style="text-align:right;"|וזכור לעולם שתשים בכל פעם היוצא בחילוק בפעם ההיא ר"ל לפעמים הכפלים אשר תוציא בפעם ההיא כנגד המעלה אשר משם [יקח] המספר הראשון התחתון ר"ל אשר יהיה במעלה הראשונה אם יהיה שם מספר [ואף אם לא יהיה שם מספר] כי אם סיפרא תראה מהיכן היה לו ראוי ליקח אם היה שם מספר <s>כי אם סיפרא</s> ושם תשים היוצא בחילוק בפעם ההיא | + | |style="text-align:right;"|וזכור ‫<ref>12r</ref>לעולם שתשים בכל פעם היוצא בחילוק בפעם ההיא ר"ל לפעמים הכפלים אשר תוציא בפעם ההיא כנגד המעלה אשר משם [יקח]‫<ref>marg.</ref> המספר הראשון התחתון ר"ל אשר יהיה במעלה הראשונה אם יהיה שם מספר [ואף אם לא יהיה שם מספר]‫<ref>marg.</ref> כי אם סיפרא תראה מהיכן היה לו ראוי ליקח אם היה שם מספר <s>כי אם סיפרא</s> ושם תשים היוצא בחילוק בפעם ההיא |
|- | |- | ||
| | | | ||
:It turns out that when we want to know from which rank a certain bottom digit should be subtracted, we should see from which rank the last bottom digit should be subtracted at that time, then count the number of the ranks from there to the right, as the number of the ranks that this bottom digit is far to the right from the last bottom digit - where they end it should be subtracted from the upper digit. | :It turns out that when we want to know from which rank a certain bottom digit should be subtracted, we should see from which rank the last bottom digit should be subtracted at that time, then count the number of the ranks from there to the right, as the number of the ranks that this bottom digit is far to the right from the last bottom digit - where they end it should be subtracted from the upper digit. | ||
| − | |style="text-align:right;"|ויצא לנו מכך כי כאשר נרצה לידע אי זה מקום ראוי לקחת ממנו שום מספר מהתחתונים בשום פנים שנראה מאי זו מעלה לקחת לאחרון שבתחתונים בפעם ההיא ותמנה משם לצד ימין מנין מעלות כמספר מעלות מרחק המספר ההוא התחתון [לצד ימין מהמספר האחרון התחתון] ובמקום שיכלו מהעליונות משם יקח | + | |style="text-align:right;"|ויצא לנו מכך כי כאשר נרצה לידע אי זה מקום ראוי לקחת ממנו שום מספר מהתחתונים בשום פנים שנראה מאי זו מעלה לקחת לאחרון שבתחתונים בפעם ההיא ותמנה משם לצד ימין מנין מעלות כמספר מעלות מרחק המספר ההוא התחתון [לצד ימין מהמספר האחרון התחתון]‫<ref>marg.</ref> ובמקום שיכלו מהעליונות משם יקח |
|- | |- | ||
| | | | ||
| Line 1,430: | Line 1,452: | ||
|style="text-align:right;"|<big>המשל</big> | |style="text-align:right;"|<big>המשל</big> | ||
|- | |- | ||
| − | |We wish to divide 4380408998 by a smaller number, which is 46079. | + | |{{#annot:4380408998÷46079|157|5u2A}}We wish to divide 4380408998 by a smaller number, which is 46079. |
:<math>\scriptstyle4380408998\div46079</math> | :<math>\scriptstyle4380408998\div46079</math> | ||
| − | |style="text-align:right;"|רצינו לחלק 4380408998 על מספר קטן ממנו והוא 46079 | + | |style="text-align:right;"|רצינו לחלק 4380408998 על מספר קטן ממנו והוא 46079{{#annotend:5u2A}} |
|- | |- | ||
| | | | ||
| Line 1,468: | Line 1,490: | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|[אמ' משה זה טעות אבל האמת הוא כי היוצא בחלוק לכל א' [...] הוא זה 95000 שלמים נוסף על השברים] | + | |style="text-align:right;"|‫[אמ' משה זה טעות אבל האמת הוא כי היוצא בחלוק לכל א' [...] הוא זה 95000 שלמים נוסף על השברים‫]‫<ref>marg.</ref> |
|- | |- | ||
| | | | ||
| Line 1,476: | Line 1,498: | ||
| | | | ||
:But, we cannot subtract 6 that precedes the last bottom digit from 4 that precedes the last upper digit | :But, we cannot subtract 6 that precedes the last bottom digit from 4 that precedes the last upper digit | ||
| − | |style="text-align:right;"|אכן מד' אשר לפני האחרון העליון שהוא אשר לפני האחרון התחתון לא נוכל לצאת 6 שהוא אשר לפני האחרון התחתון | + | |style="text-align:right;"|אכן מד' אשר לפני האחרון העליון שהוא אשר לפני האחרון התחתון ‫<ref>12v</ref>לא נוכל לצאת 6 שהוא אשר לפני האחרון התחתון |
|- | |- | ||
| | | | ||
:Therefore, we do not subtract from there [= the last upper digit], but we subtract from the last two [upper] digits, which are 43. | :Therefore, we do not subtract from there [= the last upper digit], but we subtract from the last two [upper] digits, which are 43. | ||
| − | |style="text-align:right;"|לכן לא נוציא משום [משם] דבר אבל נוציא מהשנים האחרונים שהם 43 | + | |style="text-align:right;"|לכן לא נוציא משום [משם]‫<ref>marg.</ref> דבר אבל נוציא מהשנים האחרונים שהם 43 |
|- | |- | ||
| | | | ||
| Line 1,496: | Line 1,518: | ||
| | | | ||
:The bottom line has 5 digits, hence we count 5 ranks to the right from the upper 3, from which we subtract. They end in the zero and we place beneath it the 9 resulting from the division, which is the number of times that we have to subtract the bottom digits from the upper digits at this time, each from its corresponding rank, as mentioned. | :The bottom line has 5 digits, hence we count 5 ranks to the right from the upper 3, from which we subtract. They end in the zero and we place beneath it the 9 resulting from the division, which is the number of times that we have to subtract the bottom digits from the upper digits at this time, each from its corresponding rank, as mentioned. | ||
| − | |style="text-align:right;"|והנה השורה התחתונה היא 5 רשמים לכן לא נמנה מהג [3] העליון אשר לוקח משם 5 מעלות לצד ימין ויכלו בסיפרא ונשים תחתיה אלו ה9 היוצאים בחילוק שהוא מספר הכפלים אשר לנו להוציא התחתונים מהעליונים בפעם הזאת כל אחד מהמעלה הראויה לו כנזכר | + | |style="text-align:right;"|והנה השורה התחתונה היא 5 רשמים לכן לא נמנה מהג [3]‫<ref>marg.</ref> העליון אשר לוקח משם 5 מעלות לצד ימין ויכלו בסיפרא ונשים תחתיה אלו ה9 היוצאים בחילוק שהוא מספר הכפלים אשר לנו להוציא התחתונים מהעליונים בפעם הזאת כל אחד מהמעלה הראויה לו כנזכר |
|} | |} | ||
| Line 1,556: | Line 1,578: | ||
::*We say also: 9 times 6 are 54. | ::*We say also: 9 times 6 are 54. | ||
:::<math>\scriptstyle{\color{blue}{9\times6=54}}</math> | :::<math>\scriptstyle{\color{blue}{9\times6=54}}</math> | ||
| − | |style="text-align:right;"|ועוד נאמר 9 [פעמים] 6 הם 54 | + | |style="text-align:right;"|ועוד נאמר 9 [פעמים]‫<ref>marg.</ref> 6 הם 54 |
|- | |- | ||
| | | | ||
| Line 1,570: | Line 1,592: | ||
| | | | ||
::*Also 9 times 7, because we do not pay attention to the bottom zero, except for keeping the ranks, i.e. it indicates for us that the multiple of 7 that precedes it should not be subtracted from the rank of the upper zero that precedes the 8, from which we subtract the 6, but from the rank of the 4 that precedes the 8 by 2 ranks, as the 7 precedes the 6 by 2 ranks. | ::*Also 9 times 7, because we do not pay attention to the bottom zero, except for keeping the ranks, i.e. it indicates for us that the multiple of 7 that precedes it should not be subtracted from the rank of the upper zero that precedes the 8, from which we subtract the 6, but from the rank of the 4 that precedes the 8 by 2 ranks, as the 7 precedes the 6 by 2 ranks. | ||
| − | |style="text-align:right;"|עוד 9 פעמים 7 כי לא נחוש לסיפרא התחתונה כי אם ל{{#annot:term|2453,2452|UO8O}}שמירת המדרגות{{#annotend:UO8O}} ר"ל שהיא מורה לנו שאין להוציא כפל 7 זה אשר לפניה ממקום הסיפרא העליונה אשר לפני ה8 אשר לקחנו משם ל6 אבל ממקום ה4 שהוא לפני ה8 2 מעלות כדרך שה7 לפני ה6 2 מעלות | + | |style="text-align:right;"|עוד 9 פעמים 7 <sup>כי</sup> לא נחוש ‫<ref>13r</ref>לסיפרא התחתונה כי אם ל{{#annot:term|2453,2452|UO8O}}שמירת המדרגות{{#annotend:UO8O}} ר"ל שהיא מורה לנו שאין להוציא כפל 7 זה אשר לפניה ממקום הסיפרא העליונה אשר לפני ה8 אשר לקחנו משם ל6 אבל ממקום ה4 שהוא לפני ה8 2 מעלות כדרך שה7 לפני ה6 2 מעלות |
|- | |- | ||
| | | | ||
| Line 1,618: | Line 1,640: | ||
:::The one becomes a ten above the 4, from which we subtract the 8 tens and each unit of these tens is ten in relation to the rank of the zero, from which the [1] is subtracted. We subtract the 8 tens from these ten tens; 2 remain. We place them above the 4, i.e. above the one that is above the 4. | :::The one becomes a ten above the 4, from which we subtract the 8 tens and each unit of these tens is ten in relation to the rank of the zero, from which the [1] is subtracted. We subtract the 8 tens from these ten tens; 2 remain. We place them above the 4, i.e. above the one that is above the 4. | ||
:::<math>\scriptstyle{\color{blue}{\left(10\sdot10\right)-80=2\sdot10}}</math> | :::<math>\scriptstyle{\color{blue}{\left(10\sdot10\right)-80=2\sdot10}}</math> | ||
| − | |style="text-align:right;"|וזה האחד יהיה לעשר על ה4 אשר משם נקח ה8 עשרות וכל אחד מאלו העשרה הוא עשר כערך מקום הסיפרא אשר משם לקח ה9 ונסיר ה8 [עשרות מעשר] עשרות אלו ישארו 2 ונשימם על ה4 ר"ל על האחד שהיה על ה4 | + | |style="text-align:right;"|וזה האחד יהיה לעשר על ה4 אשר משם נקח ה8 עשרות וכל אחד מאלו העשרה הוא עשר כערך מקום הסיפרא אשר משם לקח ה9 ונסיר ה8 [עשרות מעשר]‫<ref>marg.</ref> עשרות אלו ישארו 2 ונשימם על ה4 ר"ל על האחד ‫<ref>13v</ref>שהיה על ה4 |
|- | |- | ||
| | | | ||
| Line 1,633: | Line 1,655: | ||
|- | |- | ||
|} | |} | ||
| + | ::<span style=color:Green>[Illustration of the procedure:]</span> | ||
::{| | ::{| | ||
|- | |- | ||
| Line 1,684: | Line 1,707: | ||
:::We cannot subtract the 3 tens from the following rank, therefore we take one from the 3 that follows; 2 remain. We place them above it. | :::We cannot subtract the 3 tens from the following rank, therefore we take one from the 3 that follows; 2 remain. We place them above it. | ||
:::<math>\scriptstyle{\color{blue}{3-1=2}}</math> | :::<math>\scriptstyle{\color{blue}{3-1=2}}</math> | ||
| − | |style="text-align:right;"|והג' עשרות נקחם מה<s>ב' אשר עליהם כי שם מקום לקיחתם</s> הבא אחריו ולא נוכל לכן נקח אחד מהג' הבא אחריו וישארו 2 נשימם עליו | + | |style="text-align:right;"|והג' עשרות נקחם מה<s>ב' אשר עליהם כי שם מקום לקיחתם</s> הבא אחריו ולא נוכל לכן נקח אחד מהג' הבא ‫<ref>14r</ref>אחריו וישארו 2 נשימם עליו |
|- | |- | ||
| | | | ||
| Line 1,714: | Line 1,737: | ||
|style="text-align:right;"|ונשוב לחלקם להם ונרשום על זאת השארית קו דיו כדי שלא נתבלבל | |style="text-align:right;"|ונשוב לחלקם להם ונרשום על זאת השארית קו דיו כדי שלא נתבלבל | ||
|} | |} | ||
| + | ::<span style=color:Green>[Illustration of the procedure:]</span> | ||
::{| | ::{| | ||
|- | |- | ||
| Line 1,783: | Line 1,807: | ||
::*We say also: 6 times 7 are 42. | ::*We say also: 6 times 7 are 42. | ||
:::<math>\scriptstyle{\color{blue}{6\times7=42}}</math> | :::<math>\scriptstyle{\color{blue}{6\times7=42}}</math> | ||
| − | |style="text-align:right;"|עוד נאמר 6 פעמים 7 הם 42 | + | |style="text-align:right;"|עוד נאמר ‫<ref>14v</ref>6 פעמים 7 הם 42 |
|- | |- | ||
| | | | ||
| Line 1,821: | Line 1,845: | ||
|style="text-align:right;"|לכן נשוב לחלקם עליהם נרשום קו דיו עליהם על השארית הנזכר | |style="text-align:right;"|לכן נשוב לחלקם עליהם נרשום קו דיו עליהם על השארית הנזכר | ||
|} | |} | ||
| + | ::<span style=color:Green>[Illustration of the procedure:]</span> | ||
::{| | ::{| | ||
|- | |- | ||
| Line 1,912: | Line 1,937: | ||
::All were subtracted and 1021 remain, which is less than the bottom [number], therefore cannot be divided by it into integers. | ::All were subtracted and 1021 remain, which is less than the bottom [number], therefore cannot be divided by it into integers. | ||
::<math>\scriptstyle{\color{blue}{1021<46079}}</math> | ::<math>\scriptstyle{\color{blue}{1021<46079}}</math> | ||
| − | |style="text-align:right;"|הנה כבר לקחו כלם ונשארו 1021 והוא פחות מהתחתון ולא יוכל להתחלק עליו לשלמים | + | |style="text-align:right;"|הנה כבר לקחו כלם ונשארו 1021 והוא פחות מהתחתון ולא יוכל להתחלק ‫<ref>15r</ref>עליו לשלמים |
|- | |- | ||
| | | | ||
| Line 1,920: | Line 1,945: | ||
| | | | ||
:*The result of division is 95063 for each. | :*The result of division is 95063 for each. | ||
| − | |style="text-align:right;"|ושיצא בחילוק לכל אחד | + | |style="text-align:right;"|ושיצא בחילוק לכל אחד 95<sup>0</sup>63 |
|- | |- | ||
| | | | ||
| Line 1,940: | Line 1,965: | ||
:Multiply the result of division, i.e. 95063, by the divisor, i.e. 46079, add the remainder, i.e. 1021, to the product and you will receive the dividend, i.e. 4380408998, which is the number that you divided. | :Multiply the result of division, i.e. 95063, by the divisor, i.e. 46079, add the remainder, i.e. 1021, to the product and you will receive the dividend, i.e. 4380408998, which is the number that you divided. | ||
:<math>\scriptstyle{\color{blue}{\left(95063\times46079\right)+1021=4380408998}}</math> | :<math>\scriptstyle{\color{blue}{\left(95063\times46079\right)+1021=4380408998}}</math> | ||
| − | |style="text-align:right;"|כפול היוצא בחילוק ר"ל | + | |style="text-align:right;"|כפול היוצא בחילוק ר"ל 950<s>9</s>63 במספר אשר חלקנו עליו ר"ל 46079 ותוסיף השארית ר"ל ה1021 על העולה מכפל[ם] ויצא לך החשבון המתחלק ר"ל 4380408998 שזה המספר אשר חלקת עליהם |
|- | |- | ||
| | | | ||
| Line 1,953: | Line 1,978: | ||
*In order that you will learn it, I write here the check of the example that I have brought in the third chapter of multiplication, that is we divide the result of that multiplication, which is 486463564860000 by one of the two multiplied numbers, first it will be by 5400920. | *In order that you will learn it, I write here the check of the example that I have brought in the third chapter of multiplication, that is we divide the result of that multiplication, which is 486463564860000 by one of the two multiplied numbers, first it will be by 5400920. | ||
:<math>\scriptstyle486463564860000\div5400920</math> | :<math>\scriptstyle486463564860000\div5400920</math> | ||
| − | |style="text-align:right;"|<big>וכדי</big> שתתלמד ארשום כאן בחינת המשל אשר הבאתי בפרק הג' בכפל והוא שנחלק העולה מהכפל ההוא והוא 486463564860000 [על אחד מהב' מספרים הנכפלים ויהיה תחלה | + | |style="text-align:right;"|<big>וכדי</big> שתתלמד ארשום כאן בחינת המשל אשר הבאתי בפרק הג' בכפל והוא שנחלק העולה מהכפל ההוא והוא 486463564860000 [על אחד מהב' מספרים הנכפלים ויהיה תחלה ל‫5400920]‫<ref>marg.</ref> |
|- | |- | ||
| | | | ||
| Line 1,982: | Line 2,007: | ||
|} | |} | ||
|} | |} | ||
| − | + | ::<span style=color:Green>[Illustration of the procedure:]</span> | |
:{| | :{| | ||
|- | |- | ||
| Line 2,019: | Line 2,044: | ||
::*We say also: 9 by 4 are 36. | ::*We say also: 9 by 4 are 36. | ||
:::<math>\scriptstyle{\color{blue}{9\times4=36}}</math> | :::<math>\scriptstyle{\color{blue}{9\times4=36}}</math> | ||
| − | |style="text-align:right;"|[עוד נאמר 9 ב4 הם 36 | + | |style="text-align:right;"|‫[עוד נאמר 9 ב4 הם 36 |
|- | |- | ||
| | | | ||
| Line 2,029: | Line 2,054: | ||
:::We subtract the 3 from the 3; nothing remains also. | :::We subtract the 3 from the 3; nothing remains also. | ||
:::<math>\scriptstyle{\color{blue}{3-3=0}}</math> | :::<math>\scriptstyle{\color{blue}{3-3=0}}</math> | ||
| − | |style="text-align:right;"|ונסיר] ונסיר ה3 מה3 לא ישאר ג"כ דבר | + | |style="text-align:right;"|ונסיר]‫<ref>marg.</ref> ונסיר ה3 מה3 לא ישאר ג"כ דבר |
|} | |} | ||
| + | ::<span style=color:Green>[Illustration of the procedure:]</span> | ||
::::{| | ::::{| | ||
|- | |- | ||
| Line 2,052: | Line 2,078: | ||
| | | | ||
:::To know from which rank we subtract, do one of the two things: | :::To know from which rank we subtract, do one of the two things: | ||
| − | |style="text-align:right;"|ולדעת מאיזו מדרגה וקח [נקח] תעשה אחד מ2 דברים | + | |style="text-align:right;"|ולדעת מאיזו מדרגה וקח [נקח]‫<ref>marg.</ref> תעשה אחד מ2 דברים |
|- | |- | ||
| | | | ||
:::*Count from the rank where you put the result of division, which is the 6, three ranks to the left, as the distance of the 9 from the first rank. They end in the upper 3 and from it we subtract the one resulted in the multiplication. | :::*Count from the rank where you put the result of division, which is the 6, three ranks to the left, as the distance of the 9 from the first rank. They end in the upper 3 and from it we subtract the one resulted in the multiplication. | ||
| − | |style="text-align:right;"|או תמנה מהמעלה [אשר שמת שם היוצא בחלוק שהוא ה6 לצד שמאל 3 מעלות כמרחק זה ה9 מהמעלה הא'] הא' ויכלו ב[3] העליון ומשם נקח זה האחד שעלה בכפל | + | |style="text-align:right;"|או תמנה מהמעלה [אשר שמת שם היוצא בחלוק שהוא ה6 לצד שמאל 3 מעלות כמרחק זה ה9 מהמעלה הא']‫<ref>marg.</ref> הא' ויכלו ב[3] העליון ומשם נקח זה האחד שעלה בכפל |
|- | |- | ||
| | | | ||
:::*If you want, count from the rank from which the last bottom digit was subtracted, which is the upper 8, five ranks to the right, as the distance of the 9 from the last digit 5. They end also in 3. | :::*If you want, count from the rank from which the last bottom digit was subtracted, which is the upper 8, five ranks to the right, as the distance of the 9 from the last digit 5. They end also in 3. | ||
| − | |style="text-align:right;"|ואם תרצה תמנה מהמקום אשר משם לקח המספר האחרון התחתון והוא ה8 [העליון 5] מעלות לצד ימין כמרחק זה ה9 מה5 המספר האחרון לצד ימין ויכלו ג"כ ג' | + | |style="text-align:right;"|ואם תרצה תמנה מהמקום אשר משם לקח המספר האחרון התחתון ‫<ref>15v</ref>והוא ה8 [העליון 5]‫<ref>marg.</ref> מעלות לצד ימין כמרחק זה ה9 מה5 המספר האחרון לצד ימין ויכלו ג"כ ג‫' |
|- | |- | ||
| | | | ||
| Line 2,068: | Line 2,094: | ||
|- | |- | ||
| | | | ||
| + | :::We subtract the 8 tens from the 6; there is not enough. [We] take [1] from the 4; 3 remains. The 1 is as ten and with the 6 they are 16. We subtract the 8 from it; 8 remains. | ||
| + | :::<math>\scriptstyle{\color{blue}{16-8=8}}</math> | ||
|style="text-align:right;"|ונקח ה8 עשרות מה6 ואין די ויקחו מה4 וישארו 3 וזה הראשון הוא לעשרה ועם ה6 יהיו 16 נסיר מהם ה8 ישארו 8 | |style="text-align:right;"|ונקח ה8 עשרות מה6 ואין די ויקחו מה4 וישארו 3 וזה הראשון הוא לעשרה ועם ה6 יהיו 16 נסיר מהם ה8 ישארו 8 | ||
|- | |- | ||
| Line 2,076: | Line 2,104: | ||
|- | |- | ||
| | | | ||
| + | :::8 cannot be subtracted from 5, so we take 1 from the 2. We subtract another 1 for the ten and nothing remains. | ||
| + | :::<math>\scriptstyle{\color{blue}{1-1=0}}</math> | ||
|style="text-align:right;"|ומה5 לא יוכל לצאת ה8 לכן נקח 1 מה2 ועוד נקח משם 1 לעשר ולא ישאר דבר | |style="text-align:right;"|ומה5 לא יוכל לצאת ה8 לכן נקח 1 מה2 ועוד נקח משם 1 לעשר ולא ישאר דבר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|וזה ה1 הראשון אשר לקחנו יהיה עשרה ועם ה5 יהיו 9 נסיר מהם 8 ישארו 7 [ישאר 7] | + | :::The first 1 that we took becomes ten and with the 5 they are [15]. We subtract 8 from it; 7 remains. |
| + | :::<math>\scriptstyle{\color{blue}{{\color{red}{15}}-8=27}}</math> | ||
| + | |style="text-align:right;"|וזה ה1 הראשון אשר לקחנו יהיה עשרה ועם ה5 יהיו 9 נסיר מהם 8 ישארו 7 [ישאר 7‫]‫<ref>marg.</ref> | ||
|- | |- | ||
| | | | ||
| + | ::All the digits were subtracted, because you do not take anything for the 0. | ||
|style="text-align:right;"|וכבר לקחו כל המספרים כי ה0 ולא תקח דבר | |style="text-align:right;"|וכבר לקחו כל המספרים כי ה0 ולא תקח דבר | ||
|} | |} | ||
| + | ::<span style=color:Green>[Illustration of the procedure:]</span> | ||
::::{| | ::::{| | ||
|- | |- | ||
| Line 2,101: | Line 2,135: | ||
|- | |- | ||
| | | | ||
| + | ::3807648606000 remains in the upper line and it is much greater than the bottom number, so we divide it by it again and draw a line above them. | ||
|style="width:45%; text-align:right;"|והנה נשאר בעליון 3807648606000 והוא רב מאד מהמספר התחתון לכן נשוב נחלקנו עליו ונרשום קו דיו עליהם | |style="width:45%; text-align:right;"|והנה נשאר בעליון 3807648606000 והוא רב מאד מהמספר התחתון לכן נשוב נחלקנו עליו ונרשום קו דיו עליהם | ||
|- | |- | ||
| | | | ||
| + | :*We say: in 3 there is not enough for 5, so we take from 38. There is 7 times 5 in it. Place the 7 resulting in the division beneath the 6, for the four ranks to the right of the 8 end there, which is as the number of the ranks of the bottom line. | ||
|style="text-align:right;"|ונאמ' מ3 אין די ל5 ונקח מ38 ויהיה בהם 7 פעמים 5 תשים זה ה7 היוצא בחילוק תחת ה6 כי שם יכלו הד' מעלות מזה ה8 לצד ימין אשר הם כמנין מעלות השורה התחתונה | |style="text-align:right;"|ונאמ' מ3 אין די ל5 ונקח מ38 ויהיה בהם 7 פעמים 5 תשים זה ה7 היוצא בחילוק תחת ה6 כי שם יכלו הד' מעלות מזה ה8 לצד ימין אשר הם כמנין מעלות השורה התחתונה | ||
|- | |- | ||
| Line 2,127: | Line 2,163: | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|וה8 לא יוכלו לצאת | + | :::8 cannot be subtracted from 0, so we take 1 from the 3; it becomes [ten]. We subtract the 8 and 2 remains. We write it there |
| + | :::<math>\scriptstyle{\color{blue}{10-8=2}}</math> | ||
| + | |style="text-align:right;"|וה8 לא יוכלו לצאת מה0 ונקח 1 מה3 ויהיה 34 נקח ה8 וישארו ב' ונשימם עליו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ונקח עוד מה3 | + | :::We subtract the 2 tens from the 3 also; nothing remains. |
| + | :::<math>\scriptstyle{\color{blue}{3-1-2=0}}</math> | ||
| + | |style="text-align:right;"|ונקח עוד מה3 <s>ויהיה 4</s> הב' עשרות ולא ישאר דבר | ||
|} | |} | ||
| + | ::<span style=color:Green>[Illustration of the procedure:]</span> | ||
::::{| | ::::{| | ||
|- | |- | ||
| Line 2,154: | Line 2,195: | ||
|- | |- | ||
| | | | ||
| + | :::We subtract the 3 from the 4, which is [in] the corresponding rank, as we mentioned in one of the two methods, whether as third to the left, or as fifth to the right like the 9; 1 remains from the 4. We write it above it. | ||
| + | :::<math>\scriptstyle{\color{blue}{4-3=1}}</math> | ||
|style="text-align:right;"|ונקח ה3 מה4 שהיא מדרגה הראויה לו כמו שהזכרנו באחד מהב' דרכים אם להיותה שלישית לשמאל ואם להיותה חמישית לימין כמו שהוא ה9 וישאר א' מה4 ונשימהו עליו | |style="text-align:right;"|ונקח ה3 מה4 שהיא מדרגה הראויה לו כמו שהזכרנו באחד מהב' דרכים אם להיותה שלישית לשמאל ואם להיותה חמישית לימין כמו שהוא ה9 וישאר א' מה4 ונשימהו עליו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|והו' עשרות נסירם מה6 ולא ישאר דבר והנה לקחו כלם | + | :::We subtract the 6 tens from the 6; nothing remains. |
| + | :::<math>\scriptstyle{\color{blue}{6-6=0}}</math> | ||
| + | |style="text-align:right;"|והו' עשרות נסירם מה6 ולא ישאר דבר <s>והנה לקחו כלם</s> | ||
|- | |- | ||
| | | | ||
| Line 2,172: | Line 2,217: | ||
:::We subtract the 1 from the 1; nothing remains. | :::We subtract the 1 from the 1; nothing remains. | ||
:::<math>\scriptstyle{\color{blue}{1-1=0}}</math> | :::<math>\scriptstyle{\color{blue}{1-1=0}}</math> | ||
| − | |style="text-align:right;"|ונסיר ה1 מ1 ולא ישאר דבר | + | |style="text-align:right;"|ונסיר ה1 מ1 ולא ‫<ref>16r</ref>ישאר דבר |
|- | |- | ||
| | | | ||
| + | ::All are subtracted and 2700460000 remains in the upper line. | ||
|style="text-align:right;"|והנה לקחו כלם ונשאר בעליון 2700460000 | |style="text-align:right;"|והנה לקחו כלם ונשאר בעליון 2700460000 | ||
|- | |- | ||
| | | | ||
| + | ::Since the 7 is first beneath the drawn line and you might forget it, write it above the drawn line. Draw also a line above all and divide them again, as they are much more than the bottom number. | ||
|style="text-align:right;"|וה7 לפי שהוא תחת הקו הרשום תחלה ואולי תשכחהו שימהו על הקו הרשום ועוד תרשום קו על הכל ותשוב לחלקים אחרי היותם יותר מהתחתון | |style="text-align:right;"|וה7 לפי שהוא תחת הקו הרשום תחלה ואולי תשכחהו שימהו על הקו הרשום ועוד תרשום קו על הכל ותשוב לחלקים אחרי היותם יותר מהתחתון | ||
|} | |} | ||
| − | + | ::<span style=color:Green>[Illustration of the procedure:]</span> | |
::::{| | ::::{| | ||
|- | |- | ||
| Line 2,200: | Line 2,247: | ||
|- | |- | ||
| | | | ||
| − | |style="width:45%; text-align:right;"|ונאמר ב2 אין די ל5 ניקח מ27 ויהיו בו ה' פעמים ונשימהו תחת השלישית כי שם יכלו ה7 מעלות לצד ימין שהם כמנין מעלות השורה התחתונה | + | :*We say: in 2 there is not enough for 5, so we take from 27. There is 5 times in it. We place it beneath the third [rank], for the seven ranks end there. |
| + | |style="width:45%; text-align:right;"|ונאמר ב2 אין די ל5 ניקח מ27 ויהיו בו ה' פעמים ונשימהו תחת השלישית כי שם יכלו ה7 מעלות לצד ימין <sup>שהם</sup> כמנין מעלות השורה התחתונה | ||
|- | |- | ||
| | | | ||
| Line 2,223: | Line 2,271: | ||
|- | |- | ||
| | | | ||
| + | :::We do not subtract units. | ||
|style="text-align:right;"|ולא נקח אחדים | |style="text-align:right;"|ולא נקח אחדים | ||
|- | |- | ||
| | | | ||
| + | :::The 2 are tens, so we subtract them from the 2 that we wrote now above the 7; nothing remains. | ||
| + | :::<math>\scriptstyle{\color{blue}{2-2=0}}</math> | ||
|style="text-align:right;"|אכן הב' עשרות נקחם מהב' אשר שמנו עתה על הז' ולא ישאר דבר | |style="text-align:right;"|אכן הב' עשרות נקחם מהב' אשר שמנו עתה על הז' ולא ישאר דבר | ||
|- | |- | ||
| Line 2,234: | Line 2,285: | ||
|- | |- | ||
| | | | ||
| + | :::We subtract the 5 from the 6, as it is the corresponding rank as stated; 1 remains. | ||
| + | :::<math>\scriptstyle{\color{blue}{6-5=1}}</math> | ||
|style="text-align:right;"|נסיר הה' מהו' כי היא מעלה הראויה לו כנזכר וישאר א‫' | |style="text-align:right;"|נסיר הה' מהו' כי היא מעלה הראויה לו כנזכר וישאר א‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ונסיר הד' מהד' ולא ישאר דבר | + | :::We subtract the 4 from the 4; nothing remains. |
| + | :::<math>\scriptstyle{\color{blue}{4-4=0}}</math> | ||
| + | |style="text-align:right;"|ונסיר הד' מהד' ולא ישאר דבר | ||
|- | |- | ||
|<span style=color:red>[the last step is missing <math>\scriptstyle{\color{red}{2\times5=10\longrightarrow1-1=0}}</math>]</span> | |<span style=color:red>[the last step is missing <math>\scriptstyle{\color{red}{2\times5=10\longrightarrow1-1=0}}</math>]</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :The whole number is gone. | ||
| + | |style="text-align:right;"|הנה לנו שכלה כל החשבון | ||
| + | |- | ||
| + | | | ||
| + | :Since we have empty ranks in the result of division, both at the beginning and in the middle, we write zeros in these places, because this is the role and the value of the zeros, as we have mentioned. | ||
|style="text-align:right;"|ולהיות לנו בזה היוצא בחלוק {{#annot:term|2138,2137|6jNM}}מעלות חלקות מהמספר{{#annotend:6jNM}} הן בתחלה הן באמצע נשים ספרות במקומם כי זה מעשה הסיפרות ותועלתם כאשר הזכרנו | |style="text-align:right;"|ולהיות לנו בזה היוצא בחלוק {{#annot:term|2138,2137|6jNM}}מעלות חלקות מהמספר{{#annotend:6jNM}} הן בתחלה הן באמצע נשים ספרות במקומם כי זה מעשה הסיפרות ותועלתם כאשר הזכרנו | ||
|- | |- | ||
| − | | | + | |We receive that when we divide the product by one of the multiplicands, the second results no more and no less, with no remainder at all. |
|style="text-align:right;"|והנה יצא לנו שכאשר חלקנו העולה מהכפל באחת הנכפלים יצא השני בלי תוספת ומגרעת ובלי שארית כלל | |style="text-align:right;"|והנה יצא לנו שכאשר חלקנו העולה מהכפל באחת הנכפלים יצא השני בלי תוספת ומגרעת ובלי שארית כלל | ||
|- | |- | ||
| − | | | + | |Likewise, if you divide by the other, the one results in the division, as appears in the following diagram: |
| − | |||
|style="text-align:right;"|וכן אם תחלקנה לחבירתה תצא חבירתה בחילוק כאשר בא בזאת הצורה | |style="text-align:right;"|וכן אם תחלקנה לחבירתה תצא חבירתה בחילוק כאשר בא בזאת הצורה | ||
|- | |- | ||
| | | | ||
| + | *<math>\scriptstyle486463564860000\div90070500</math> | ||
| | | | ||
{|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| Line 2,274: | Line 2,337: | ||
|} | |} | ||
|- | |- | ||
| − | | | + | |I do not want to elaborate it any further and all is clear to the one who understands. |
|style="text-align:right;"|ולא ראיתי להאריך בזה עוד והכל מבואר למבין | |style="text-align:right;"|ולא ראיתי להאריך בזה עוד והכל מבואר למבין | ||
|- | |- | ||
| Line 2,282: | Line 2,345: | ||
| | | | ||
|- | |- | ||
| − | | | + | |<span style=color:Green>The reason for the decimal place of the interim result of division:</span> |
| − | |style="text-align:right;"| | + | | |
| + | |- | ||
| + | |The reason for the writing place of the result of division is that according to the rank [of the dividend] from which the first digit [of the divisor] is subtracted, so is the [rank of the number of] times that it is subtracted from it. | ||
| + | |style="text-align:right;"|‫<ref>16v</ref><big>וטעם מקום הנחת</big> היוצא בחילוק הוא כי כפי המדרגה אשר ממנה לקח המספר הראשון הם הפעמים אשר לקחו | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle600\div3</math> | + | *I.e. if we divide six hundred by 3 units: |
| + | :<math>\scriptstyle600\div3</math> | ||
|style="text-align:right;"|ר"ל שאם חלקנו שש מאות לג' אחדים | |style="text-align:right;"|ר"ל שאם חלקנו שש מאות לג' אחדים | ||
|- | |- | ||
| | | | ||
| + | :Each unit receives 2 and the rank from which it is subtracted is the rank of hundreds, so the two resulting from the division are of the same rank and they are two hundred. | ||
:<math>\scriptstyle{\color{blue}{600\div3=200}}</math> | :<math>\scriptstyle{\color{blue}{600\div3=200}}</math> | ||
| − | |style="text-align:right;"|הנה יגיע לכל אחד ב' והמדרגה אשר ממנה לקח היא מדרגת המאות הנה השנים אשר יצאו בחלוק היא הם מאותה המדרגה והם מאתים | + | |style="text-align:right;"|הנה יגיע לכל אחד ב' והמדרגה אשר ממנה לקח היא מדרגת המאות הנה השנים אשר יצאו בחלוק <s>היא</s> הם מאותה המדרגה והם מאתים |
|- | |- | ||
| − | |<math>\scriptstyle a00\div b=c00\longrightarrow a000\div b0=c00</math> | + | | |
| + | :If there were thousands in the upper [line] and tens in the bottom [line], then as the units receive from the hundreds, so the tens would receive from the thousands. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{a00\div b=c00\longrightarrow a000\div b0=c00}}</math> | ||
|style="text-align:right;"|ואם היו שם עוד אלפים בעליון ועשרות בתחתון הנה הפעמים אשר יגיעו לאחדים מהמאות יגיעו לעשרות מהאלפים | |style="text-align:right;"|ואם היו שם עוד אלפים בעליון ועשרות בתחתון הנה הפעמים אשר יגיעו לאחדים מהמאות יגיעו לעשרות מהאלפים | ||
|- | |- | ||
| | | | ||
| − | : | + | :Because, as the tens are tens in relation to the units, so the thousands are tens in relation to the hundreds; and as the hundreds are in relation to the units, so the thousands are in relation to the tens, and the tens of thousands are in relation to the hundreds; and so on, as those are in relation to those, so are these in relation to these. |
| − | |style="text-align:right;"|כי כמו שהעשרות הם עשרות לאחדים [ככה האלפים הם עשרות למאות הנה כאשר הגיע לאחדים מהמאות] מהמאות יגיעו לעשרות מהאלפים וכן למאות מהעשרות אלפים וכן לעולם כי כמו שזה עולה לאשר כמותו כן עולה זה | + | |style="text-align:right;"|כי כמו שהעשרות הם עשרות לאחדים [ככה האלפים הם עשרות למאות הנה כאשר הגיע לאחדים מהמאות]‫<ref>marg.</ref> מהמאות יגיעו לעשרות מהאלפים וכן למאות מהעשרות אלפים וכן לעולם כי כמו שזה עולה לאשר כמותו כן עולה זה |
|- | |- | ||
| | | | ||
| − | : | + | :We have already explained that the rank of the [number of] times that the units [are subtracted] is the rank from which they are subtracted |
| − | |style="text-align:right;"|כבר ביארנו כי מדרגת הפעמים אשר הגיעו לאחדים היא מרוחק המדרגה אשר ממנו לקח | + | |style="text-align:right;"|כבר ביארנו כי מדרגת הפעמים אשר הגיעו לאחדים היא מרוחק המדרגה אשר ממנו לקח |
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"|ר"ל שכל אחד מהתחתונות מדרגתה הראויה לקחת ממנה היא | + | :[Thus, since the units are subtracted from the hundreds], the tens are subtracted from the thousands, and the hundreds from the tens of thousands and so on. |
| + | |style="text-align:right;"|והיא בעצמה המדרגה אשר לקחו העשרות מהאלפים והמאות מהעשרות אלפים וכן כולם | ||
| + | |- | ||
| + | |I.e. each of the bottom [digits] should be extracted from the rank [of the dividend] that is as far to the left of the rank, from which the [divisor's] units was extracted, as the number of ranks that it is far from the [rank of] units, i.e. as the number [of ranks] that the bottom [digit] is far to the left from the units [of the divisor]. | ||
| + | |style="text-align:right;"|ר"ל שכל אחד מהתחתונות מדרגתה הראויה לקחת ממנה היא מרו<sup>ח</sup>קת לצד שמאל [מהמדרגה אשר לקחו ממנה האחדים כמספר המדרגות אשר היא מהאחדים]‫<ref>marg.</ref> מהאחדים ר"ל כמספר אשר זה התחתון לצד שמאל מהאחדים <s>ר"ל כמספר אשר זה התחתון לצד שמאל מהאחדים</s> | ||
|- | |- | ||
| | | | ||
| − | : | + | :The rank of the hundreds is third to the left of the units, so when the units are taken from the hundreds, as we mentioned, the tens should be taken from the thousands that are third [in relation to the tens] to the left of the rank of hundreds, from which the units are taken. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"| שהרי המאות מדרגתם היא שלישית לצד שמאל מהאחדים וכן העשרות אלפים אשר ראוי לו לקחת מהם בקחת האחדים מהמאות כאשר זכרנו גם הם שלישיים לצד שמאל מהמאות אשר היא <sup>ה</sup>מדרגה אשר ממנה לקחו האחדים |
| + | |- | ||
| + | |Apply this. | ||
| + | |style="text-align:right;"|והקש על זה | ||
| + | |- | ||
| + | |This includes the reason of the whole procedure. | ||
| + | |style="text-align:right;"|ובזה נכלל טעם כל המעשה | ||
|- | |- | ||
| | | | ||
| − | ==== | + | ==== reason: check ==== |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|‫<ref>17r</ref><big>וטעם הבחינה</big> |
|- | |- | ||
| − | | | + | |For the multiplication is the inverse operation of division. |
|style="text-align:right;"|כי הכפל הוא הפך החילוק | |style="text-align:right;"|כי הכפל הוא הפך החילוק | ||
| + | |- | ||
| + | |I.e. [the meaning of] division is to know how many times the small number is in the larger number. | ||
| + | |style="text-align:right;"|ר"ל שהחילוק הוא לידע כמה פעמים המספר הקטון במספר הגדול | ||
| + | |- | ||
| + | |Whereas [the meaning of] multiplication is [to know] how much is the sum of the multiples of a given number for a given number of times. | ||
| + | |style="text-align:right;"|והכפל הוא כמה סך כפלי מספר ידוע פעמים ידועים | ||
|- | |- | ||
| | | | ||
| − | * | + | *If we divide 20 by 5, each one receives 4; if by 4, each one [receives] 5. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכן אם בחלקנו כ' לה' יעלה לכל אחד ד' או לד' לכל אחד ה‫' |
| + | |- | ||
| + | | | ||
| + | :So, 20 is a product of 4 by 5, which are the number by which we divide and the result of division. | ||
| + | |style="text-align:right;"|הנה כ' הוא כפל ד' בה' שהם המספר אשר חלקנו עליו והיוצא בחילוק | ||
|- | |- | ||
| | | | ||
| − | + | :<math>\scriptstyle{\color{blue}{\begin{cases}\scriptstyle20\div5=4\\\scriptstyle20\div4=5\end{cases}\longrightarrow20=4\times5}}</math> | |
| − | | | + | | |
|- | |- | ||
| | | | ||
| − | * | + | *If we divide 21 by 4, each one receives 5 and 1 remains. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם חלקנו כ"א לד' [..] יעלה לכל אחד ה' וישאר א‫' |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | :Therefore, when we multiply 4 by 5, the result is 20. We add to it the remaining 1; the total result is 21, which is the same as the dividend. | |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{21\div4=5+\frac{1}{4}\longrightarrow21=\left(4\times5\right)+1}}</math> |
| − | ולזה כאשר כפלנו ד' בה' ויעלה כ' הוספנו עליהם הא' הנשאר יעלה הכל כ"א שהוא כמספר המתחלק וכל זה ברור | + | |style="text-align:right;"|ולזה כאשר כפלנו ד' בה' ויעלה כ' הוספנו עליהם הא' הנשאר יעלה הכל כ"א שהוא כמספר המתחלק |
| + | |- | ||
| + | |All this is clear. | ||
| + | |style="text-align:right;"|וכל זה ברור | ||
|- | |- | ||
| − | |Finding the proper fraction of the remainder from division | + | |<span style=color:Green>Finding the proper fraction of the remainder from division:</span> When you wish to add the smaller [number] that remains above the bottom number that is greater than it, or any small number that is above a greater number, you will find in the discussion on summing fractions chapter 1, section 2, a general method for all the numbers, whether they have divisors, or they are prime. |
| − | |style="text-align:right;"|וכאשר תרצה לחבר המעט הנשאר על המספר התחתון | + | |style="text-align:right;"|<big>וכאשר</big> תרצה לחבר המעט הנשאר על המספר התחתון שהוא גדול ממנו או שום אחד מספר קטן על מספר אחד גדול ממנו תמצא בחלק הב' בפרק הא' במאמ' האחדות אשר בו דרך כולל לכל המספרים בין יהיו להם מורים בין אם יהיו {{#annot:term|76,2029|O61W}}פשוטים{{#annotend:O61W}} |
|} | |} | ||
{| | {| | ||
| Line 2,349: | Line 2,440: | ||
|- | |- | ||
|To give you an inclusive method for dividing a large number by a smaller number and vice versa, I use the technique of the ancients: to extract the denominators of the number by which you want to divide, whether it is the smaller number or the greater number; that is to consider the numbers of which it is composed, if it is not a prime number. | |To give you an inclusive method for dividing a large number by a smaller number and vice versa, I use the technique of the ancients: to extract the denominators of the number by which you want to divide, whether it is the smaller number or the greater number; that is to consider the numbers of which it is composed, if it is not a prime number. | ||
| − | |style="width:45%; text-align:right;"|אכן לתת לך דרך כולל בין לחלק רב למעט או בהפך דרכתי דרך הראשונים והוא שתוציא המורים מהחשבון אשר תרצה לחלק עליו אם מועט אם הרבה והוא ל[ראו'] המספרים אשר הוא {{#annot:term|2491,1961|4sLD}}מורכב מהם{{#annotend:4sLD}} אם איננו {{#annot:term|76,2029|2DxW}}פשוט{{#annotend:2DxW}} | + | |style="width:45%; text-align:right;"|<big>אכן לתת לך דרך כולל</big> בין לחלק רב למעט או בהפך דרכתי דרך הראשונים והוא שתוציא המורים מהחשבון אשר תרצה לחלק עליו אם מועט אם הרבה והוא ל[ראו'] המספרים אשר הוא {{#annot:term|2491,1961|4sLD}}מורכב מהם{{#annotend:4sLD}} אם איננו {{#annot:term|76,2029|2DxW}}פשוט{{#annotend:2DxW}} |
|- | |- | ||
| | | | ||
| Line 2,356: | Line 2,447: | ||
|- | |- | ||
|First, if you want to know if it has a third, a sixth, or a ninth [= if 3, 6, or 9 are divisors of the number]: | |First, if you want to know if it has a third, a sixth, or a ninth [= if 3, 6, or 9 are divisors of the number]: | ||
| − | |style="text-align:right;"|ראשונה אם תרצה לדעת אם יש לו שלישית או שישית או תשיעית מבלי שברים | + | |style="text-align:right;"|<big>ראשונה</big> אם תרצה לדעת אם יש לו שלישית או שישית או תשיעית מבלי שברים |
|- | |- | ||
| | | | ||
| Line 2,368: | Line 2,459: | ||
| | | | ||
*<span style="color:Green>nine and three:</span> Every [number] that it has a ninth [= divisible by 9], has also a third [= divisible by 3], but not vice versa. | *<span style="color:Green>nine and three:</span> Every [number] that it has a ninth [= divisible by 9], has also a third [= divisible by 3], but not vice versa. | ||
| − | |style="text-align:right;"|וכל שיש לו תשיעית יש לו ג"כ שלישית ולא יתהפך | + | |style="text-align:right;"|‫<ref>17v</ref>וכל שיש לו תשיעית יש לו ג"כ שלישית ולא יתהפך |
|- | |- | ||
| | | | ||
| Line 2,396: | Line 2,487: | ||
| | | | ||
*We say that if the first digit is an odd number, it does not have a sixth [= 6 is not a divisor of the number], since the whole number is odd, and an odd number is indivisible by an even number, i.e. it is not a product of an even number multiplied by an odd number and all the more so by an even number. | *We say that if the first digit is an odd number, it does not have a sixth [= 6 is not a divisor of the number], since the whole number is odd, and an odd number is indivisible by an even number, i.e. it is not a product of an even number multiplied by an odd number and all the more so by an even number. | ||
| − | |style="text-align:right;"|ואמרנו שאם הרושם הראשון הוא מספר נפרד שאין לו שישית הוא לפי שכל | + | |style="text-align:right;"|ואמרנו שאם הרושם הראשון הוא מספר נפרד שאין לו שישית הוא לפי שכל <sup>ה</sup>חשבון בכללו <s>הוא נפרד שאין לו שישית הוא לפי שכל החשבון בכללו</s> הוא נפרד והחשבון הנפרד לא {{#annot:term|2187,1226|sEuM}}נחלק לשלמים{{#annotend:sEuM}} אל חשבון זוג ר"ל שאינו מורכב מחשבון זוג אפי' עם הנפרד כ"ש עם הזוג |
|- | |- | ||
| | | | ||
| Line 2,407: | Line 2,498: | ||
| | | | ||
|- | |- | ||
| − | | | + | |If you want to find out if it has a half, a quarter, or an eighth [= if 2, 4, or 8 are divisors of a the number] |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>ואם תרצה</big> לדעת אם יש לו מחצית או רביעית או שמינית |
|- | |- | ||
| − | | | + | |Consider its first numeral [its units]: |
| − | |style="text-align:right;"|ראה הרושם הראשון | + | |style="text-align:right;"|‫<ref>18r</ref>ראה הרושם הראשון |
|- | |- | ||
| − | |If it is an odd number, it does not have | + | |If it is an odd number, it does not have any of them [not divisible by 2, 4, or 8] - from the reason we have mentioned concerning the sixth [= 6 as a divisor]. |
| − | |style="text-align:right;"|אם הוא חשבון נפרד | + | |style="text-align:right;"|אם הוא חשבון נפרד הנה אין לו אח' מהם מהטעם שאמרנו בשישית |
|- | |- | ||
| | | | ||
| − | *two: if it is an even number or 0 - then the whole number is even, for the tens and up are even - therefore it has a half [= divisible by 2] | + | *<span style="color:Green>two:</span> if it is an even number or 0 - then the whole number is even, for the tens and up are even - therefore it is known that it has a half [= divisible by 2]. |
| − | |style="text-align:right;"|ואם הוא זוג | + | |style="text-align:right;"|ואם הוא זוג או 0' הרי כל החשבון בכללו זוג כי העשרות ומהם ולמ[ע]‫<ref>marg.</ref>לה כלם זוג אחדים וא"כ בידוע שיש לו מחצית |
|- | |- | ||
| | | | ||
| − | *four and eight: if it | + | *<span style="color:Green>four and eight:</span> to know if it has also a quarter and an eighth: |
|style="text-align:right;"|ולדעת אם יש לו ג"כ רביעית ושמינית | |style="text-align:right;"|ולדעת אם יש לו ג"כ רביעית ושמינית | ||
|- | |- | ||
| − | |The digits of the whole number are summed according to the following procedure: | + | | |
| − | + | :<span style="color:Green>The digits of the whole number are summed according to the following procedure:</span> | |
| − | + | | | |
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :*Take the numeral that is in the first rank as it is. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|קח המספר אשר במעלה הראשונה כמו שהוא |
|- | |- | ||
| | | | ||
| − | :* | + | :*Double what is in the second [rank], if there is a number there. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואשר בשנייה כפול אם יש שם מספר |
|- | |- | ||
| | | | ||
| − | :* | + | :*If what is in the third [rank] is an odd number, take 4 units for it; if it is an even number or 0, do not take any thing for it. |
| − | |style="text-align:right;"|ואם | + | |style="text-align:right;"|ואשר בשלישי' אם הוא נפרד קח בעבורו ד' אחדים ואם הוא זוג או 0 לא תקח בעבורו מאומה |
|- | |- | ||
| | | | ||
| − | :* | + | :*Likewise from the third rank up, do not take any thing. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכן מהמעלה השלישית ולמעלה לא תקח דבר |
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :Sum up all that you took and cast out the eights. |
| + | |style="text-align:right;"|וחבר כל אשר לקחת ו{{#annot:term|1560,1265|07O7}}השלך אותו ח' ח‫'{{#annotend:07O7}} | ||
|- | |- | ||
| − | | | + | | |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{2a+10b+\left[100\sdot\left(2c-1\right)\right]\longrightarrow2a+2b+4}}</math> | |
| + | :<math>\scriptstyle{\color{OliveGreen}{2a+10b+\left(100\sdot2c\right)\longrightarrow2a+2b}}</math> | ||
| + | | | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :*If all is gone [= no remainder], it has an eighth and a quarter [= divisible by 8 and 4]. |
| + | |style="text-align:right;"|ואם {{#annot:term|1560,1231|293n}}יצא הכל{{#annotend:293n}} הנה יש לו שמינית ורביעית | ||
|- | |- | ||
| − | |'''No need to take any thing for the ranks that are higher than the hundreds | + | | |
| − | |style="text-align:right;"|ומהמעלה השלישית ולמעלה לא תקח דבר | + | :*If four remains, it has a quarter [= divisible by 4]. |
| + | |style="text-align:right;"|ואם ישאר [ארבעה]‫<ref>marg.</ref> יש לו רביעית לבד | ||
| + | |- | ||
| + | | | ||
| + | :*If another number remains, it does not have an eighth nor a quarter [=not divisible by 4 or 8] | ||
| + | |style="text-align:right;"|ואם ישאר חשבון אחר אין לו לא שמינית ולא רביעית | ||
| + | |- | ||
| + | |<span style="color:Green>The reason for doubling the digit of the tens in the sum:</span> | ||
| + | | | ||
| + | |- | ||
| + | |The reason we say that we take what is in the second rank as doubled is that they are tens and when you subtract 8 from each ten, 2 remains. So, we are left with two from each ten, therefore we double all the tens and take them doubled. | ||
| + | :<math>\scriptstyle{\color{blue}{10-8=2}}</math> | ||
| + | |style="text-align:right;"|<big>וטעם</big> אומרנו שנקח אשר במעלה השנית כפול הוא לפי שהם עשרות ומכל עשר כאשר תסיר ח' ישארו ב' הרי שישאר לנו מכל עשר שנים לכן אנו כופלים כל העשרות ולכך אנו לוקחים אותם כפולות | ||
| + | |- | ||
| + | |<span style="color:Green>The reason for not taking any digit for an even number of hundreds in the sum:</span> | ||
| + | | | ||
| + | |- | ||
| + | |What is in the third rank are hundreds and every even number of hundreds has an eighth [= is divisible by 8]. For the eighth of two hundred is 25. So, we do not take any thing for an even number of hundreds. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{8}\sdot200=25}}</math> | ||
| + | |style="text-align:right;"|ואשר במעלה השלישית הם מאות וכל זוגי מאות יש להם שמינית כי שמינית מאתים הוא כ"ה לכן לא נקח בעבור זוגי המאות דבר | ||
| + | |- | ||
| + | |<span style="color:Green>The reason for taking 4 for an odd number of hundreds in the sum:</span> | ||
| + | | | ||
| + | |- | ||
| + | |But, if there is an odd number of hundreds, after subtracting the even number of hundreds, we take 4 for it. Because, when subtracting the eights from one hundred, which are 12 eights that are 96, 4 remains. | ||
| + | :<math>\scriptstyle{\color{blue}{100-\left(12\sdot8\right)=100-96=4}}</math> | ||
| + | |style="text-align:right;"|אך אם יש שם מאה נפרד אחר הסרת זוגי המאות נקח בעבורו ד' כי בהסיר שמיניות המאה שהם י"ב שמיניות שהם עולים לצ"ו ישארו ד‫' | ||
| + | |- | ||
| + | |<span style="color:Green>No need to take any thing for the ranks that are higher than the hundreds</span> | ||
| + | | | ||
| + | |- | ||
| + | |You do not take any thing for the third rank up, because all of them are an even number of hundreds, so they have an eighth [= are divisible by 8], as we have explained. | ||
| + | |style="text-align:right;"|ומהמעלה השלישית ולמעלה לא תקח דבר כי כלם הם זוגי מאות ויש להם שמינית כמו שביארנו | ||
|- | |- | ||
| | | | ||
| Line 2,465: | Line 2,588: | ||
| | | | ||
|- | |- | ||
| − | | | + | |If you want to find out if it has a seventh [= if 7 is a divisor of a given number] |
|style="text-align:right;"|ואם תרצה לדעת אם יש לו שביעית | |style="text-align:right;"|ואם תרצה לדעת אם יש לו שביעית | ||
|- | |- | ||
| − | | | + | |See the final digit to the right and multiply it by 3, add [the product] to what you find in the preceding [rank], and cast out the sevens [from the sum]. Multiply the remainder by 3 and add [the product] to what you find in the preceding [rank]. If you do not find there any number, but 0, multiply [the product] again by 3, likewise for every 0, and add [the product] to what you find in the preceding [rank]. Then, cast out the sevens [from the sum, and so on repeatedly]. |
| − | |style="text-align:right;"|ראה הרושם האחרון אשר לצד שמאל וכפלהו בג' וחברהו לאשר תמצא במעלה לאשר לפניה והסר לעולם השביעיות והנשאר כפלהו בג' וחברהו עם אשר תמצא אשר לפניה ואם לא תמצא שם מספר כי אם 0' | + | |style="text-align:right;"|ראה הרושם האחרון אשר לצד שמאל וכפלהו בג' וחברהו לאשר תמצא במעלה לאשר לפניה והסר לעולם ‫<ref>18v</ref>השביעיות והנשאר כפלהו בג' וחברהו עם אשר תמצא אשר לפניה ואם לא תמצא שם מספר כי אם 0' כפלהו פעם שנית בג' וכן על כל 0' וחברהו עם אשר תמצא לפניו ו{{#annot:term|1560,1265|yHcy}}השלך לעולם הז' ז‫'{{#annotend:yHcy}} |
|- | |- | ||
| | | | ||
| − | + | *If all is cast out by the sevens, we know that it has a seventh [= divisible by 7]. | |
| − | |style="text-align:right;"|ואם {{#annot:term| | + | |style="text-align:right;"|ואם {{#annot:term|1560,1231|jc6j}}יצא הכל לשביעיות{{#annotend:jc6j}} הרי ידענו שיש לו שביעית |
|- | |- | ||
| | | | ||
| − | + | *Otherwise - it does not. | |
|style="text-align:right;"|ואם לאו לאו | |style="text-align:right;"|ואם לאו לאו | ||
|- | |- | ||
| − | | | + | |<span style="color:Green>The reason for multiplying each rank by 3 and adding the product to the preceding rank:</span> |
| − | |style="text-align:right;"| | + | | |
| + | |- | ||
| + | |The reason that we multiply every rank by 3 [and add the product] to the preceding rank is that every rank is ten with regard to the preceding [rank] and when subtracting 7 from [10], 3 remains. Therefore, each unit is valued three with regard to the preceding [rank], after subtracting the 7 [from it]. | ||
| + | |style="text-align:right;"|<big>הטעם</big> מה שאנו כופלים כל מעלה בג' לחברו לאש' לפניה הוא לפי שכל מעלה היא עשר בערך אשר לפניה ובהסר מהם הז' [ישארו ג' הנה כל אחד הוא כשלש בערך אשר לפניו אחרי הסרת הז‫']‫<ref>marg.</ref> | ||
|- | |- | ||
| | | | ||
| Line 2,487: | Line 2,613: | ||
| | | | ||
|- | |- | ||
| − | | | + | |If you want to find out if it has a tenth, or a fifth [= if 10, or 5 are divisors of a given number] |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>ואם תרצה</big> לדעת אם יש לו עשירית או חמישית |
|- | |- | ||
| | | | ||
| − | + | *If the first digit is 0, the whole [number] is tens, because the hundreds and up are all tens also, therefore it has a tenth and a fifth [= divisible by 10 and 5]. | |
| − | |style="text-align:right;"|אם הרושם הראשון הוא 0 | + | |style="text-align:right;"|אם הרושם הראשון הוא 0 הנה הכל עשרות [כי אם המאות ומשם ולמעלה הכל הוא עשרות]‫<ref>marg.</ref> והנה יש לו עשירית גם חמישית |
|- | |- | ||
| | | | ||
| − | + | *If it is 5, it has only a fifth [= divisible by 5]. | |
| − | |style="text-align:right;"|ואם הוא ה' | + | |style="text-align:right;"|ואם הוא ה' הנה יש לו חמישית לבד |
|- | |- | ||
| | | | ||
| − | + | *If it is another number, it does not have even a fifth [= not divisible by 5]. | |
|style="text-align:right;"|ואם הוא מספר אחר גם חמישית אין לו | |style="text-align:right;"|ואם הוא מספר אחר גם חמישית אין לו | ||
|- | |- | ||
| Line 2,507: | Line 2,633: | ||
| | | | ||
|- | |- | ||
| − | | | + | |If you want to find out if it has 11th [= if 11 is a divisor of a given number] |
|style="text-align:right;"|ואם תרצה לדעת אם יש לו י"א | |style="text-align:right;"|ואם תרצה לדעת אם יש לו י"א | ||
|- | |- | ||
| − | | | + | |I.e. if it is completely divisible by 11, meaning that it is all cast out by elevens and nothing is left, the same as what we have said in all the preceding divisors. |
| − | |style="text-align:right;"|פי' אם {{#annot:term|2187,1225|sJQw}}יתחלק ל{{#annotend:sJQw}}י"א על השלימות והוא ש{{#annot:term| | + | |style="text-align:right;"|פי' אם {{#annot:term|2187,1225|sJQw}}יתחלק ל{{#annotend:sJQw}}י"א על השלימות והוא ש{{#annot:term|1560,2017|Cxib}}יושלך כלו י"א <sup>י"א</sup>{{#annotend:Cxib}} ולא ישאר דבר וכיוצא בזה הוא מה שאמרנו בכל המורים העוברים |
|- | |- | ||
| − | | | + | |See the last digit and subtract 1 from what you find in the preceding rank. Subtract the remainder from what you find in the further preceding rank and so on repeatedly, until reaching to the beginning. |
| − | |style="text-align:right;"|ראה הרושם האחרון והוצא ה1 | + | |style="text-align:right;"|ראה הרושם האחרון והוצא ה1 מאשר תמצא במעלה אשר לפניו והנשאר הוציאנו מה שתמצא מאשר לפני פניו וכן עד הגיעו לראש |
|- | |- | ||
| | | | ||
| − | + | *If all is cast out, it has an 11th [= divisible by 11]. | |
| − | |style="text-align:right;"|ואם יצא הכל יש לו י"א י"א | + | |style="text-align:right;"|ואם יצא הכל יש לו י"א <sup>י"א</sup> |
|- | |- | ||
| | | | ||
| − | + | *Otherwise - it does not | |
|style="text-align:right;"|ואם לאו לאו | |style="text-align:right;"|ואם לאו לאו | ||
|- | |- | ||
| − | |If | + | |If you find a zero anywhere or any small number, from which you cannot subtract what I have instructed you, add 11 to what is found there, whether [it is] a zero or a small number, and subtract from the sum as I have instructed you, then [subtract] the remainder from what precedes and so on. |
| − | |style="text-align:right;"|ואם בשום מקום תמצא סיפרא | + | |style="text-align:right;"|ואם בשום מקום תמצא <sup>סיפרא</sup> או שום מספר קטן במנין שלא תוכל להוציא ממנו אשר צוויתיך {{#annot:term|178,1206|2EI3}}הוסיף{{#annotend:2EI3}} י"א על הנמצא שם סיפרא 0 <s>וכן</s> או <s>שמונה</s> חשבון קטן ומהכל תוציא אשר ציויתיך והנשאר מאשר לפניו כן לעולם |
|- | |- | ||
| − | | | + | |<span style="color:Green>The reason for the procedure:</span> For every number is ten times with regard to the preceding rank, therefore when you take it as tens and what precedes it as units, it is as if all that you take are tens and units. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>הטעם</big> כי כל מספר הוא עשרה בערך במעלה אשר לפניו לכן כאשר תקחנו לעשרות ואשר לפניו לאחדים זה בזה הרי כל מה שלקחת הם י"א י"א |
|- | |- | ||
| − | | | + | |<span style="color:Green>The reason for adding 11 to a small number:</span> Our saying to add 11 to what precedes, when you do not find there enough to subtract, is that if we add some 11s to our number it neither raises nor decreases, for it is cast out by elevens anyway, either with the addition or without, and this is clear. |
| − | |style="text-align:right;"|ואשר הוא לפי אמרנו ולהוסיף אות י"א באשר לפניו | + | |style="text-align:right;"|ואשר <s>הוא לפי</s> אמרנו ולהוסיף ‫<ref>19r</ref><s>אות</s> י"א באשר לפניו כאשר לא תמצא שם די מחסורו הוא לפי שאם נוסיף כמה י"א י"א בחשבוננו לא יעלה ולא יוריד כי הוא בעצמו {{#annot:term|1560,2017|DNT7}}יושלך לי"א י"א{{#annotend:DNT7}} <s>ר</s> ג"כ יצא אחר התוספת ואם לאו <sup>לאו</sup> וזה מבואר |
|- | |- | ||
| | | | ||
| Line 2,538: | Line 2,664: | ||
| | | | ||
|- | |- | ||
| − | | | + | |If you want to find out if it has 13th [= if 13 is a divisor of a given number] |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>ואם תרצה</big> לדעת אם יש לו י"ג |
|- | |- | ||
| − | | | + | |See the last digit and multiply it by 3, then cast out the thirteens that are in [the product] and subtract the remainder from what you find in the preceding rank. Multiply the remainder again by 3, then cast out the thirteens that are in [the product] and subtract the remainder from the preceding [rank] and so on until the [digits] end. |
|style="text-align:right;"|ראה הרושם האחרון וכפלהו בג' ו{{#annot:term|1560,1232|tDVX}}הוצא הי"ג י"ג{{#annotend:tDVX}} אשר בו והנשאר הוציאהו מאשר תמצא במעלה אשר לפניו והנשאר כפלהו שנית בג' והוצא הי"ג אשר בו <s>והנשאר כפלהו שנית בג' והוצא הג' אשר בו</s> והנשאר הוציאהו מאשר לפניו וכן לעולם עד תכליתם | |style="text-align:right;"|ראה הרושם האחרון וכפלהו בג' ו{{#annot:term|1560,1232|tDVX}}הוצא הי"ג י"ג{{#annotend:tDVX}} אשר בו והנשאר הוציאהו מאשר תמצא במעלה אשר לפניו והנשאר כפלהו שנית בג' והוצא הי"ג אשר בו <s>והנשאר כפלהו שנית בג' והוצא הג' אשר בו</s> והנשאר הוציאהו מאשר לפניו וכן לעולם עד תכליתם | ||
|- | |- | ||
| | | | ||
| − | + | *If all is cast out, it has a 13th [= divisible by 13]. | |
|style="text-align:right;"|ואם יצא הכל יש לו י"ג | |style="text-align:right;"|ואם יצא הכל יש לו י"ג | ||
|- | |- | ||
| | | | ||
| − | + | *Otherwise - it does not. | |
|style="text-align:right;"|ואם לאו לאו | |style="text-align:right;"|ואם לאו לאו | ||
|- | |- | ||
| − | |If | + | |If you do not find not enough in a certain rank to subtract as I have instructed you, add 13 and subtract from the sum what you need to subtract. Then multiply the remainder by 3 and cast out the thirteens. Subtract the remainder from the preceding [rank] and so on. |
| − | |style="text-align:right;"|וכאשר יחסר בשום פנים מעלה | + | |style="text-align:right;"|וכאשר יחסר בשום <s>פנים</s> מעלה שלא תמצא די להוציא אשר ציויתיך הוסף י"ג והוצא [מ{{#annot:term|178,1215|PVjr}}המתחבר{{#annotend:PVjr}} אשר עליך להוציא והנשאר כפלהו בג' והוצא]‫<ref>marg.</ref> הי"ג י"ג והנ' הוציאהו מאשר לפניו וכן לעולם |
|- | |- | ||
| − | | | + | |<span style="color:Green>The reason for the procedure:</span> Because every number is ten times its value with regard to the preceding rank. Hence, when you subtract it and you subtract 3 times as much as it in the preceding rank, which is as units with regard to it, all that you subtract is as tens and 3 units for each ten. So each number are 13s. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>הטעם</big> כי כל מספ' הוא עשרה כערך אשר במעלה הקודמת וכאשר תסירנו ותסיר ג' שכמותו מהמעלה הקודמת שהי' לה לאחדים הרי כל אשר הוצאת הוא עשרות וג' <s>ו</s>אחדים על כל עשר <s>מספר</s> הנה כל מספר הוא י"ג י"ג |
|- | |- | ||
| − | | | + | |Furthermore, the addition of the 13 that I have instructed you to add does not harm the extraction procedure of 13, as we have noted concerning the addition of 11 in the extraction procedure of 11 as a divisor. This is clear. |
| − | |style="text-align:right;"|גם התוספת אש' ציויתיך להוסיף מהי"ג לא יזיק בהוצאת הי"ג | + | |style="text-align:right;"|גם התוספת אש' ציויתיך להוסיף מהי"ג לא יזיק בהוצאת הי"ג כאשר הזכרנו ב{{#annot:term|154,2511|b7h5}}הוספת ה{{#annotend:b7h5}}י"א בהמצאת מורה הי"א וזה מבואר |
|- | |- | ||
| | | | ||
| Line 2,567: | Line 2,693: | ||
|- | |- | ||
| | | | ||
| − | * | + | *For a number that you do not find any of the aforesaid divisors [2-11, 13] and you want to know if it has any other divisor, the divisor that you look for cannot have any of these divisors [= cannot be divisible by any of the numbers 2-11, 13]. |
| − | |style="text-align:right;"|והמספר אשר לא תמצא לו אחד מה{{#annot:term| | + | |style="text-align:right;"|והמספר אשר לא תמצא לו אחד מה{{#annot:term|604,1239|gIva}}מורים {{#annotend:gIva}}הנזכרים ותרצה לידע אם יש לו מורה אחר המורה הזה אשר תבקש הוא שלא יהיה לו שום מורה מהמורים |
| + | |- | ||
| + | | | ||
| + | :The reason is that if it has any divisor of them, then it is known that it cannot be a divisor of the [given] number. For if it were a divisor of the [given] number, then the [given] number also would have had the same divisor that this divisor had, but you have not found it. | ||
| + | |style="text-align:right;"|הטעם שאם היה לו שום {{#annot:term|604,1239|TmQF}}מורה{{#annotend:TmQF}} מהם בידוע שאינו מורה לזה החשבון שאם הוא מורה לזה החשבון הנה לחשבון ג"כ יש לו <sup>ה</sup>מורה אשר לזה המורה ואתה לא מצאתו | ||
| + | |- | ||
| + | | | ||
| + | :*Example: when one takes 21, if 21 is a divisor of a number, then this number also has the divisors of this divisors, i.e. [it has] a seventh and a third, but you already know that your number does not have any of them, so it does not have 21 either. | ||
| + | |style="text-align:right;"|המשל אם יקח כ"א הנה אם ‫<ref>19v</ref>כ"א הוא מורה לחשבון הנה יש לחשבון מורי זה המורה ר"ל שביעית ושלישית וכבר ידעת שאין לחשבו<sup>נ</sup>ך אחד מהם לכן לא יהיה לו כ"א | ||
|- | |- | ||
| | | | ||
| − | + | *In addition, when you examine if a number has all the previous divisors one after the other and you do not find, if it is less than the square of the next divisor that you want to examine, you do not need to examine it, because it is not its divisor, neither does any other number, for it is known that it is a prime number. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|גם אם מספר אשר בקשת לו כל המורים העוברים זה אחר זה ולא מצאתם אם הוא פחות ממרובע המורה הסמוך אשר תרצה לבקש אינך צריך לבקשו כי איננו לו מורה אם לא מורה אחר בעולם כי בידוע שהוא {{#annot:term|76,2029|LVhT}}מספר פשוט{{#annotend:LVhT}} |
|- | |- | ||
| | | | ||
| − | : | + | :The reason is that if it had this next divisor that you seek for, needless to say another greater than it, then since your number is less than its square, the number of times that this divisor can be in it should be less than the divisor itself, and this number of times itself should be its divisor. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הטעם שאם היה לו זה המורה <s>הפשוט</s> הסמוך אשר אתה מבקש ואין צריך לומר אחר גדול ממנו הנה להיות חשבונך פחות ממרובעו הנה הפעמים אשר יהיה בו המורה ההוא יהיה מספרם פחות ממספר המורה בעצמו וגם מספר הפעמים יהיה לו למורה |
|- | |- | ||
| | | | ||
| − | + | :Since, for any [number] that is divided by any number, so that the result of division is a certain number and nothing remains, both are its divisors. Because, when it is divided by the current result of the division, the result of this division is the former divisor and nothing remains. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כי כל דבר הנחלק למספר מה ויצא בחילוק מספר מה ולא נשאר דבר הנה שניהם לו מורים כי כאשר יתחלק לאשר יוצא עתה בחילוק <sup>יצא בחלוק</sup> אשר נחלקו עליו עתה ולא ישאר דבר |
|- | |- | ||
| | | | ||
| − | : | + | :Yet, since this number of times is less than the required divisor, it is among one of the preceding divisors, therefore it has one of the preceding divisors, but you did not find them as such, so this is a contradiction. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואולם להיות מספר פעמים אלו פחות ממספר מורה המב<sup>ו</sup>קש הלא הם כאחד המורים הקודמים וא"כ היה לו אחד מהמורים הקודמים ואתה לא מצאתם <s>הרי</s> <sup>זה</sup> שקר |
|- | |- | ||
| | | | ||
| − | :* | + | :*Example: if you seek until 17, but you do not find and you want to see if it has a 17th. If your number is less than 289, which is the square of 17, it is known that if it is cast out by 17s, then the number of times that 17 is in it is less than 17. For if it were 17 or more then your number were as the square of 17 or more, but it is smaller. However, if this number of times that 17 is in it is less than 17, for instance 16 and down, then it has a quarter or any of the preceding divisors, for it is divisible also by this number of times, but this is a contradiction, because you did not find any of the preceding [divisors]. |
| − | |style="text-align:right;"|המשל אם בקשת עד י"ז ולא מצאת ותרצה לבקש אם יש לו י"ז ומספרך | + | |style="text-align:right;"|המשל אם בקשת עד י"ז ולא מצאת ותרצה לבקש אם יש לו י"ז ומספרך <s>ש</s>הוא פחות מרפ"ט שהוא <s>מ</s>מרובע י"ז הנה בידוע שאם היה יוצא לי"ז י"ז שהפעמים אשר בו י"ז י"ז הם פחות מי"ז שאם היו י"ז <s>לא</s> <sup>או</sup> יותר הרי חשבונך היה כמרובע י"ז או גדול ממנו והוא קטן<br> |
| − | ואם הפעמים האלה אשר י"ז בו הם פחות מי"ז | + | ואם הפעמים האלה אשר י"ז בו הם פחות מי"ז המשל י"ו ומשם ולמטה הנה היה לו רביעית או אחד מהמורים הקודמים שהרי יתחלק למספר פעמים אלו ג"כ ויצא בחילוף הי"ז וזה שקר ‫<ref>20r</ref>שהרי לא מצאת לו אחד מהעוברים |
|- | |- | ||
| | | | ||
| − | * | + | *When your number is as the square of the next divisor that you want to examine, or greater than it and you wish to know if this [potential] next divisor is indeed its divisor: |
| − | |style="text-align:right;"|ואולם כאשר יהיה חשבונך כמרובע המורה הנמשך אשר תבקש | + | |style="text-align:right;"|<big>ואולם</big> כאשר יהיה חשבונך כמרובע המורה הנמשך אשר תבקש או גדול ממנו ותרצה לדעת אם תמצא לו זה המורה הסמוך |
|- | |- | ||
| | | | ||
| − | :*If | + | :*If it is divisible by it to integers without a remainder, then it is its true divisor and so is the result of division. |
|style="text-align:right;"|ואם {{#annot:term|2187,1225|SD1U}}יתחלק אליו לשלימים מבלי שארית{{#annotend:SD1U}} הוא לו ל{{#annot:term|2135,2134|uyDf}}מורה צדק{{#annotend:uyDf}} גם היוצא בחילוק | |style="text-align:right;"|ואם {{#annot:term|2187,1225|SD1U}}יתחלק אליו לשלימים מבלי שארית{{#annotend:SD1U}} הוא לו ל{{#annot:term|2135,2134|uyDf}}מורה צדק{{#annotend:uyDf}} גם היוצא בחילוק | ||
|- | |- | ||
| | | | ||
| − | :*Otherwise - | + | :*Otherwise - it is not. |
|style="text-align:right;"|ואם לאו לאו | |style="text-align:right;"|ואם לאו לאו | ||
|} | |} | ||
| Line 2,610: | Line 2,744: | ||
| | | | ||
| + | |- | ||
| + | |When you know that it has a divisor, divide the whole number by this divisor, and the result of division will be an integer. | ||
| + | |style="width:45%; text-align:right;"|וכאשר ידעת שיש לו שום מורה חלק המספר כולו לזה המורה יתחלק אליו לשלימים והיוצא בחילוק | ||
| + | |- | ||
| + | |If you do not want to seek for more, then these two numbers, i.e. the divisor, by which you divide, and the result of the division are both its divisors. | ||
| + | |style="text-align:right;"|ואם <sup>לא</sup> תרצה לבקש יותר הנה אלו השני מספרים ר"ל המורה אשר חלקת עליו והיוצא בחילוק הם הם מוריו | ||
| + | |- | ||
| + | |If the result of division is a large number and you want to seek its divisor, proceed according to the mentioned methods. | ||
| + | |style="text-align:right;"|אכן אם היוצא בחילוק הוא חשבון גדול ותרצה לבקש לו מורה ג"כ עשה כדרכים הנזכרים | ||
| + | |- | ||
| + | |But, know that [the numbers] you find that are not divisors of the large number, you will find that they are not divisors of the result of division and this is clear. You should not seek for one of [these numbers], only for those that are similar to the one that you have found or greater than it. | ||
| + | |style="text-align:right;"|ואולם דע שהמורים אשר לא מצאת לחשבון הגדול לא תמצאם ג"כ לזה היוצא בחילוק וזה ברור ואין לך לבקש אחד מהם כי אם הדומה לאשר מצאת או למעלה ממנו | ||
| + | |- | ||
| + | |If you find its divisor, divide it by it and the result of division is a third divisor. | ||
| + | |style="text-align:right;"|אם מצאת לו מורה חלקנו עליו והיוצא בחילוק יהיה מורה שלישי | ||
| + | |- | ||
| + | |If this [result] is also a large [number] and you want to seek its divisor also, proceed as mentioned, divide it by the divisor that you have found to be its divisor and the result of division is its fourth divisor. | ||
| + | |style="text-align:right;"|ואם זה ג"כ גדול ותרצה לבקש לו ג"כ מורה אחר עשה כנזכר וחלקנו על המורה אשר ידעת אשר הוא למורה לו והיוצא בחילוק יהיה לו למורה רביעי | ||
| + | |- | ||
| + | |If you want, you can seek also for its fifth, or sixth, or other [divisors]. | ||
| + | |style="text-align:right;"|ואם תרצה תוכל ל[...] לבקש עוד חמישי או שישי או זולתם | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:2447235÷50335084800|157|khi4}}Example: we wish to divide 2447235 by 50335084800. | ||
| + | :<math>\scriptstyle2447235\div50335084800</math> | ||
| + | |style="text-align:right;"|המשל רצינו לחלק 2447235 על 50335084800{{#annotend:khi4}} | ||
|- | |- | ||
| | | | ||
| − | + | :We extract the divisors of this large number, by which we want to divide. | |
| − | |style=" | + | |style="text-align:right;"|נוציא [....] המורים לזה החשבון הגדול אשר רצינו לחלק עליו |
|- | |- | ||
| | | | ||
| − | : | + | :*First, we see if 3, or 6, or 9 are [its divisors]: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונראה ראשונה אם יש לו ג' או ששה או ט‫' |
|- | |- | ||
| | | | ||
| − | + | ::Since the first digit is 0, we know that if 3 or 9 [are its divisors], then 6 is also its [divisor]. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואחר שהרושם הראשון הוא 0 ידענו שאם יש לו ג' או ט' יש לו ג"כ ששה |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::To know if 3 or 9 [are its divisors], we sum up all [the digits] as if they were units. |
| + | |style="text-align:right;"|ולדעת אם יש לו ג' או ט' נחברם כלם כאלו הם אחדים | ||
|- | |- | ||
| | | | ||
| − | + | ::We say: 8 with 4 is 12. We subtract from it 9; 3 remains. | |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{4+8=12\equiv_93}}</math> |
| + | |style="text-align:right;"|ונאמ' ח' עם ד' הם י"ב נוציא מהם הט' ישארו [.] ג‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::With 8 it is 11. We subtract the 9; 2 remains. |
| + | ::<math>\scriptstyle{\color{blue}{3+8=11\equiv_92}}</math> | ||
| + | |style="text-align:right;"|ועם <sup>8</sup> הם י"א נסיר הט' ישארו ב‫' | ||
|- | |- | ||
| | | | ||
| − | + | ::With 5 it is 7 and with 3 it is ten. We subtract 9; 1 remains. | |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\left(5+2\right)+3=7+3=10\equiv_91}}</math> |
| + | |style="text-align:right;"|ועם ה' יהיו ז' ‫<ref>20v</ref>ועם הג' יהיו עשרה נסיר ט' ישארו <s>ב'</s> <sup>א‫'</sup> | ||
|- | |- | ||
| | | | ||
| − | : | + | ::With 3 it is 4 and with 5 it is 9. So, the number is cast out by nines. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\left(1+3\right)+5=4+5=9\equiv_90}}</math> |
| + | |style="text-align:right;"|ועם הג' יהיו ד' ועם הה' יהיו כולם <sup>ט'</sup> <s>ט'</s> הרי {{#annot:term|1560,1231|D5Ek}}יצא החשבון לט' ט‫'{{#annotend:D5Ek}} | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::<math>\scriptstyle{\color{blue}{5+0+3+3+5+0+8+4+8+0+0\equiv_90}}</math> |
| − | | | + | | |
|- | |- | ||
| | | | ||
| − | : | + | :Hence, we know that 9, 3 and 6 are its [divisors] for the reason we have mentioned. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וידענו שיש לו ט' וג' גם ו' לסבה שזכרנו |
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :We take whichever of them we want. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונקח מהם אשר תרצה |
|- | |- | ||
| | | | ||
| + | :*For instance, we take 6 as its divisor. We divide it by 6; the result of division is 8389180800. | ||
::<math>\scriptstyle{\color{blue}{\frac{50335084800}{6}=8389180800}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{50335084800}{6}=8389180800}}</math> | ||
| − | |style="text-align:right;"|ועל דרך משל | + | |style="text-align:right;"|ועל דרך משל נקח ל{{#annot:term|604,1239|P3U8}}מורה{{#annotend:P3U8}} ו' ונחלקנו על ו' ויצא בחלוקם 8389180800 |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{8+3+8+9+1+8+0+8+0+0\equiv_90}}</math | + | ::Since the result of division is a large number, we seek its divisor. We do as we did with the original number; it is all cast out by nines. |
| − | + | ::<math>\scriptstyle{\color{blue}{8+3+8+9+1+8+0+8+0+0\equiv_90}}</math> | |
| − | + | |style="text-align:right;"|ואחר שהיוצא בחילוק הוא חשבון גדול נבקש לו מורה ונעשה לזה כאשר לחשבון הראשון ו{{#annot:term|1560,1231|sB7E}}יצא הכל לט' ט{{#annotend:sB7E}}‫' | |
| − | |style="text-align:right;"|ואחר שהיוצא בחילוק הוא חשבון גדול | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| + | ::Since the first digit is a zero and 9 is its divisor, 6 is also its divisor and all the more so is 3. | ||
| + | |style="text-align:right;"|ואחר שהרושם הראשון <sup>סיפרא</sup>ויש לו ט' יש לו ג"כ ו' וכ"ש ג‫' | ||
| + | |- | ||
| + | | | ||
| + | :*We take 9 as its divisor and divide this number by 9; the result of divison is 932131200. | ||
::<math>\scriptstyle{\color{blue}{\frac{8389180800}{9}=932131200}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{8389180800}{9}=932131200}}</math> | ||
|style="text-align:right;"|ונקח ט' למורה ונחלק זה החשבון לט' ויצא בחילו' 932131200 | |style="text-align:right;"|ונקח ט' למורה ונחלק זה החשבון לט' ויצא בחילו' 932131200 | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{9+3+2+1+3+1+2+0+0\equiv_93}}</math | + | ::We do with it as we have done with the formers; 3 remains. |
| − | + | ::<math>\scriptstyle{\color{blue}{9+3+2+1+3+1+2+0+0\equiv_93}}</math> | |
| − | + | |style="text-align:right;"|ונעשה לזה כאשר עשינו לקודמים וישארו ג‫' | |
| − | |style="text-align:right;"|ונעשה לזה כאשר עשינו לקודמים וישארו ג‫' | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| + | ::So, 3 is its [divisor] and 6 also, since the first [digit] is a zero. | ||
| + | |style="text-align:right;"|הנה יש לו ג' ג"כ ו' להיות הראשונה <sup>סיפרא</sup> | ||
| + | |- | ||
| + | | | ||
| + | :*We take 3 as its divisor and divide it by it; the result of divison is 310710400. | ||
::<math>\scriptstyle{\color{blue}{\frac{932131200}{3}=310710400}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{932131200}{3}=310710400}}</math> | ||
|style="text-align:right;"|ונקח למורה ג' ונחלקנו עליו ויצא בחילוקם 310710400 | |style="text-align:right;"|ונקח למורה ג' ונחלקנו עליו ויצא בחילוקם 310710400 | ||
|- | |- | ||
| | | | ||
| − | :Since 9 is not a [divisor] of | + | :Since 9 is not a [divisor] of the former, it is known that even 3 is not [divisor] of this [number]. |
| − | |style="text-align:right;"|ואחר שהקודם לא היה לו ט' | + | |style="text-align:right;"|ואחר שהקודם לא היה לו ט' בידוע שלזה אין לו אפי' ג‫' |
| − | הטעם לפי שזה שלישית הקודם ואם לזה היה לו שלישית הקודם ואם לזה היה לו שלישית | + | |- |
| + | | | ||
| + | ::The reason is that this [number] is a third of the former [number], so if this [number] had a third, its third, i.e. the third of the [number] that is a third of the former [number], is a third of a third of the former [number], which is a ninth, but we have found that it does not [have a ninth]. | ||
| + | |style="text-align:right;"|הטעם לפי שזה שלישית הקודם ואם לזה היה לו שלישית <s>הקודם ואם לזה היה לו שלישית</s> הנה שלישיתו ר"ל שלישית זה שהיה שלישית הראשון הוא לראשון שלישית שלישית שהוא תשיעית ולא מצאנוהו | ||
|- | |- | ||
| | | | ||
| + | ::So it is truly, when you sum up [the digits], then cast out the nines, 7 remains. | ||
::<math>\scriptstyle{\color{blue}{3+1+0+7+1+0+4+0+0\equiv_97}}</math> | ::<math>\scriptstyle{\color{blue}{3+1+0+7+1+0+4+0+0\equiv_97}}</math> | ||
| − | |style="text-align:right;"|וכן הוא האמת | + | |style="text-align:right;"|וכן הוא האמת כאשר תחברם ותוציא הט' ט' ישארו ז‫' |
|- | |- | ||
| | | | ||
| − | : | + | :We see if 2, 4, or 8 are its [divisors]. |
| − | |style="text-align:right;"|ונעיין אם יש לו ב' וד' | + | |style="text-align:right;"|ונעיין אם יש לו ב' וד' או ח‫' |
|- | |- | ||
| | | | ||
| − | ::the first digit is | + | ::Since the first [digit] is a zero, it is known that 2 is its [divisor]. |
| − | |style="text-align:right;"|ואחר שהראשון סיפרא | + | |style="text-align:right;"|ואחר שהראשון <sup>סיפרא</sup> בידוע שיש [...] לו <sup>ב‫'</sup> |
|- | |- | ||
| | | | ||
| − | ::the first | + | ::To know if 4, or 8 are its [divisors], we should take what is in the first rank. We find there only 0, so we take nothing. We take what is in the second [rank]. We find there only 0, so we take nothing. Since the third [rank] is an even [number], we do not have to take anything for it or for the higher [ranks]. So, 8, 4, and 2 are its [divisors]. |
| − | ::<math>\scriptstyle{\color{blue}{0+0+0\equiv_80}}</math> | + | ::<math>\scriptstyle{\color{blue}{0+0+0\equiv_80}}</math> |
| − | + | |style="text-align:right;"|<sup>ולדעת</sup> אם יש לו <sup>ד'</sup> או ח' היה לנו לקחת אשר [במעלה] הראשונה ולא מצאנו שם כי אם 0 ולא נקח דבר כי אם בשנית היה לנו לכופלו ולא מצאנו שם כי אם 0 לא נקח דבר והשלישית אחר שהוא זוג אין לנו לקחת בעבורה דבר ולא ממנה ולמעלה<br> | |
| − | |style="text-align:right;"|ולדעת אם יש לו ד' | ||
הנה יש לו ח' וד' וב‫' | הנה יש לו ח' וד' וב‫' | ||
|- | |- | ||
| | | | ||
| + | :We take whichever of them we want as a divisor. | ||
| + | |style="text-align:right;"|ונקח למורה אשר נחפוץ מהם | ||
| + | |- | ||
| + | | | ||
| + | :*For instance, 8. We divide it by 8; the result of division is 38838800. | ||
::<math>\scriptstyle{\color{blue}{\frac{310710400}{8}=38838800}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{310710400}{8}=38838800}}</math> | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|המשל ח' ונחלקנו לח' ויצא <s>בח</s> בחילוק 38838800 |
|- | |- | ||
| | | | ||
| − | ::the | + | ::It also has an eighth, a quarter and a half, for the reason mentioned. |
| − | ::<math>\scriptstyle{\color{blue}{0+0+0\equiv_80}}</math> | + | ::<math>\scriptstyle{\color{blue}{0+0+0\equiv_80}}</math> |
| − | |||
|style="text-align:right;"|ולזאת ג"כ יש לה שמינית ורביעית וחצי לסבה הנזכרת | |style="text-align:right;"|ולזאת ג"כ יש לה שמינית ורביעית וחצי לסבה הנזכרת | ||
|- | |- | ||
| | | | ||
| + | :We take one of them. | ||
| + | |style="text-align:right;"|ונקח א' מהם | ||
| + | |- | ||
| + | | | ||
| + | :*For instance, 4. We divide by it; the result of division is 9709700. | ||
::<math>\scriptstyle{\color{blue}{\frac{38838800}{4}=9709700}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{38838800}{4}=9709700}}</math> | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|המשל ד' וחלקנו עליו ויצא בחילוק 9709700 |
|- | |- | ||
| | | | ||
| − | ::the first | + | ::It does not have an eighth, because the first [rank] and the second [rank] are 0, so we take nothing for them; but the third [rank] is 7, which is an odd number, so we take 4 for it. Hence, only 4 and 2 are its [divisors]. |
| − | ::<math>\scriptstyle{\color{blue}{0+0+4\equiv_84}}</math> | + | ::<math>\scriptstyle{\color{blue}{0+0+4\equiv_84}}</math> |
| − | + | |style="text-align:right;"|‫<ref>21r</ref>ולזאת אין לה שמינית כי הראשונה והשנית הם 0 ולא נקח בעבורם דבר והשלישית היא ז' שהוא נפרד ונקח בעבורו ד‫'<br> | |
| − | |style="text-align:right;"|ולזאת אין לה שמינית | ||
הנה שאין לו כי אם ד' וב‫' | הנה שאין לו כי אם ד' וב‫' | ||
|- | |- | ||
| | | | ||
| + | :We take one of them. | ||
| + | |style="text-align:right;"|ונקח אחד מהם | ||
| + | |- | ||
| + | | | ||
| + | :*For instance, 2. We divide it by it; the result of division is 4854850. | ||
::<math>\scriptstyle{\color{blue}{\frac{9709700}{2}=4854850}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{9709700}{2}=4854850}}</math> | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|המשל ב' ונחלקנו עליו ויצא בחילוק 4854850 |
|- | |- | ||
| | | | ||
| − | :Since | + | ::Since this [number] is half the former [number], it does not have a quarter, because the former [number] does not have an eighth, as a quarter of this [number] is a quarter of half the former, which is an eighth. |
| − | |style="text-align:right;"|מאחר שזו היא מחצית הראשונה | + | |style="text-align:right;"|מאחר שזו היא מחצית הראשונה אין לנו רביעית לפי שלא היה לראשונה שמינית כי רביעית זו היא רביעית חצי הראשונה שהוא שמינית |
|- | |- | ||
| | | | ||
| + | ::You will also find by examination that it does not have a quarter: since there is no number in the first [rank], the second is ten, and the third is an even number, so we take nothing for it. Therefore, we have only ten. We subtract 8; 2 remains. Hence, it has only a half. | ||
::<math>\scriptstyle{\color{blue}{0+\left(2\sdot5\right)+0=10\equiv_82}}</math> | ::<math>\scriptstyle{\color{blue}{0+\left(2\sdot5\right)+0=10\equiv_82}}</math> | ||
| − | |style="text-align:right;"|וכן תמצאנו בבחינה שאין לו רביעית | + | |style="text-align:right;"|וכן תמצאנו בבחינה שאין לו רביעית כי בראשונה אין מספר וכל השניה היא עשרה והשלישית היא זוג ולא נקח דבר בעבורה הרי שאין בידינו כי אם <sup>עשר</sup> נסיר ח' ישארו ב' הרי שאין לו כי אם חצי |
| + | |- | ||
| + | | | ||
| + | :If you do not want to take it again as a divisor, see if 5 or 10 are its [divisors]. | ||
| + | |style="text-align:right;"|ואם לא תרצה לקחתו שנית למורה [עיין] אם יש לו <sup>עשר</sup> או ה‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Since the first [digit] is 0, it is known that 10 as well as 5 are its [divisors]. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואחר שהראשונה 0 בידוע שיש לו [עשר וגם]‫<ref>marg.</ref> ה‫' |
|- | |- | ||
| | | | ||
| − | : | + | :We take one of them. |
| − | + | |style="text-align:right;"|ונקח אחד מהם | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| + | :*For instance, 5. We divide it by it; the result of division is 970970. | ||
::<math>\scriptstyle{\color{blue}{\frac{4854850}{5}=970970}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{4854850}{5}=970970}}</math> | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|המשל ה' ונחלקנו עליו ויצא בחילוק 970970 |
|- | |- | ||
| | | | ||
| − | ::the first digit is 0 | + | ::Since the first [digit] is 0, 10 and 5 are its [divisors] also. |
| − | + | |style="text-align:right;"|וגם זה אחר שהראשונה 0 יש לה י' גם ה‫' | |
| − | |style="text-align:right;"|וגם זה | ||
|- | |- | ||
| | | | ||
| + | :We take the ten, for instance, and divide it by 10; the result of division is 97097. | ||
::<math>\scriptstyle{\color{blue}{\frac{970970}{10}=97097}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{970970}{10}=97097}}</math> | ||
|style="text-align:right;"|ונקח עד"מ העשרה ונחלקנו לי' ויצא בחילוק 97097 | |style="text-align:right;"|ונקח עד"מ העשרה ונחלקנו לי' ויצא בחילוק 97097 | ||
|- | |- | ||
| | | | ||
| − | ::the first digit is not 0 | + | ::Since the first [digit] is not 5 nor 0, neither 5, nor 10 are its [divisors]. |
| − | + | |style="text-align:right;"|ואחר שהראשונה אינה לא ה' ולא 0 אין לו לא ה' ולא עשרה | |
| − | |style="text-align:right;"|ואחר שהראשונה אינה לא ה' ולא 0 | ||
|- | |- | ||
| | | | ||
| − | : | + | :We see if it has a seventh. |
|style="text-align:right;"|ונראה אם יש לו שביעית | |style="text-align:right;"|ונראה אם יש לו שביעית | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::We subtract seven from the last 9; two remains. |
| − | + | ::<math>\scriptstyle{\color{blue}{9\equiv_72}}</math> | |
| − | |style="text-align:right;"|ומהט' האחרון נסיר שבעה | + | |style="text-align:right;"|ומהט' האחרון נסיר שבעה ישארו שנים |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\ | + | ::We multiply it by 3; it is 6. We add it to the 7 that precedes it; it is 13. We subtract 7; 6 remains. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\left(2\sdot3\right)+7=6+7=13\equiv_76}}</math> |
| + | |style="text-align:right;"|ונכפלהו בג' יהיו ו' ונחברם לז' אשר לפניו יהיו י"ג נסיר הז' ישארו ו‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We multiply it by 3; it is 18. We subtract 14, which are two sevens, from it; 4 remains. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{6\sdot3=18\equiv_74}}</math> |
| + | |style="text-align:right;"|נכפלהו בג' יהיו י"ח נסיר מהם י"ד שני שביעיות ישארו ד‫' | ||
|- | |- | ||
| | | | ||
| − | ::the | + | ::Since there is no number in the preceding rank, only 0, we multiply the 4 by 3; it is 12. We subtract 7; five remains. |
| − | + | ::<math>\scriptstyle{\color{blue}{4\sdot3=12\equiv_75}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואחר שבמעלה שלפני זאת אין שם חשבון כי אם 0 נכפול אלו הד' בג' יהיו י"ב נסיר ז' ישארו חמשה |
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | ::We add it to the preceding [digit]; it is 14, which are sevens. The first [digit] is also 7; so all is cast out by sevens. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{5+9=14\equiv_70}}</math> |
| + | ::<math>\scriptstyle{\color{blue}{0+7=7\equiv_70}}</math> | ||
| + | |style="text-align:right;"|נחברם לט' שלפני זאת יהיו י"ד והם שביעיות גם הראשונה ז' הנה יצא הכל ז' ז‫' | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::It has a seventh. |
| − | + | |style="text-align:right;"|הנה יש לו שביעית | |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{ | + | :*We take it as a divisor and divide it by it; the result of divison is 13871. |
| − | |style="text-align:right;"|ונקחנו למורה ונחלקנו עליו ויצא בחילוק 1261 | + | ::<math>\scriptstyle{\color{blue}{\frac{97097}{7}=13871}}</math> |
| + | |style="text-align:right;"|ונקחנו למורה ונחלקנו עליו ויצא בחילוק <s>1261</s> <sup>13871</sup> | ||
|- | |- | ||
| | | | ||
| − | : | + | :We see if 7 is its [divisor] also. |
| − | |style="text-align:right;"|ונשוב לראות אם יש | + | |style="text-align:right;"|<s>ונשוב לראות</s> <sup>ונעיין</sup> אם יש לזה ‫<ref>21v</ref>ג"כ ז‫' |
|- | |- | ||
| | | | ||
| − | :: | + | ::We find that 4 remains, so 7 is not its [divisor]. |
| − | + | |style="text-align:right;"|ונמצא שישארו ד' הנה אין לו ז‫' | |
| − | |style="text-align:right;"|ונמצא שישארו ז‫' | ||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :We see if 11 is its [divisor]. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונראה אם יש לו י"א |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::We subtract the last 1 from the 3 that precedes it; 2 remains. |
| − | + | ::<math>\scriptstyle{\color{blue}{3-1=2}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונחסר הא' האחרון מהג' שלפניו וישארו ב‫' |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::We subtract it from the 8 that precedes it; 6 remains. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{8-2=6}}</math> |
| + | |style="text-align:right;"|נסירם מהח' שלפניהם ישארו ו‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::We subtract it from the 7 that precedes it; 1 remains. |
| − | + | ::<math>\scriptstyle{\color{blue}{7-6=1}}</math> | |
| − | + | |style="text-align:right;"|נסירם מהז' שלפניהם ישאר א‫' | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We subtract it from the 1 that precedes it; nothing remains. |
| + | ::<math>\scriptstyle{\color{blue}{1-1=0}}</math> | ||
| + | |style="text-align:right;"|נסירם מהא' שלפניו ולא ישאר דבר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::11 is its [divisor]. |
| + | |style="text-align:right;"|הרי יש לו י"א | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{ | + | :*We take it as a divisor and divide it by it; the result of divison is 1261. |
| − | |style="text-align:right;"|ויצא | + | ::<math>\scriptstyle{\color{blue}{\frac{13871}{11}=1261}}</math> |
| − | + | |style="text-align:right;"|ונקחנו למורה ונחלקנו עליו ויצא בחילוק 1261 | |
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| + | :We see if 11 is its [divisor]. | ||
| + | |style="text-align:right;"|ונשוב לראות אם יש לו הי"א | ||
| + | |- | ||
| | | | ||
| − | {| border="1" style="margin-left: auto; margin-right: 0px; text-align:center;" | + | ::We find that 7 remains, so 11 is not its [divisor]. |
| + | |style="text-align:right;"|ונמצא שישארו ז' הנה אין לו י"א | ||
| + | |- | ||
| + | | | ||
| + | :We see if 13 is its [divisor]. | ||
| + | |style="text-align:right;"|ונעיין אם יש לו י"ג | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply the last 1 by 3, but we cannot subtract it from the 2 that precedes it, so we add 13 to it; it is 15. We subtract the 3; 12 remains. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(2+13\right)-\left(3\sdot1\right)=15-3=12}}</math> | ||
| + | |style="text-align:right;"|ונכפול הא' אחרון בג' ולא נוכל להסירם מהב' שלפניהם לכן {{#annot:term|178,1206|fbiZ}}נוסיף עליהם{{#annotend:fbiZ}} י"ג ויהיו ט"ו נסיר הג' ישארו י"ב | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply it by 3; it is 36. We subtract 26, which are 13 and 13; 10 remains. | ||
| + | ::<math>\scriptstyle{\color{blue}{12\sdot3=36\equiv_{13}10}}</math> | ||
| + | |style="text-align:right;"|ונכפלם בג' ויהיו ל"ו נסיר כ"ו שהם י"ג י"ג ישארו י‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We cannot subtract it from the 6 that precedes it, so we add 13 to it; it is 19. We subtract the 10 from it; 9 remains. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(6+13\right)-10=19-10=9}}</math> | ||
| + | |style="text-align:right;"|‫[ולא נוכל להסירם מהו' שלפניהם ונוסיף עליהם י"ג ויהיה י"ט נסיר מהם י' ישארו‫]‫<ref>marg.</ref> ישארו ט‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply it by 3; it is 27. We subtract 26; 1 remains. | ||
| + | ::<math>\scriptstyle{\color{blue}{9\sdot3=27\equiv_{13}1}}</math> | ||
| + | |style="text-align:right;"|נכפלם בג' יהיו כ"ז נסיר כ"ו ישאר א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We subtract it from the first 1; nothing remains. | ||
| + | ::<math>\scriptstyle{\color{blue}{1-1=0}}</math> | ||
| + | |style="text-align:right;"|נסירנו מהא' הראשון ולא ישאר דבר | ||
| + | |- | ||
| + | | | ||
| + | :Hence, 13 is its [divisor]. | ||
| + | |style="text-align:right;"|הרי יש לו י"ג | ||
| + | |- | ||
| + | | | ||
| + | :*We take it as a divisor and divide it by it; the result of divison is 97. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1261}{13}=97}}</math> | ||
| + | |style="text-align:right;"|נקחנו למורה ונחלקנו עליו ויצא בחילוק 97 | ||
| + | |- | ||
| + | | | ||
| + | ::Since this number is smaller that the square of 13, you do not need to see if 13 is its [divisor], all the more so a greater divisor, or one of the preceding [divisors], as they are not [divisors] of the former [number], so we take it as a divisor itself. | ||
| + | ::<math>\scriptstyle{\color{blue}{97<13^2}}</math> | ||
| + | |style="text-align:right;"|ואחר שזה החשבון הוא פחות ממרובע י"ג אינך צריך לעיין עוד אם יש לו [הי"ג] י"ג וכ"ש מורה גדול ממנו ולא אחד מהקודמים א<s>ש</s><sup>ח</sup>ר שלא נמצא לראשונים לכן נקחנו בעצמו למורה | ||
| + | |- | ||
| + | | | ||
| + | :We have received the divisors of this number. | ||
| + | |style="text-align:right;"|הנה יצאו לנו מורים לזה החשבון | ||
| + | |- | ||
| + | | | ||
| + | :The smaller number that we want to divide by this greater number is 2447235. | ||
| + | |style="text-align:right;"|והחשבון הקטן אשר רצינו לחלק לזה החשבון הגדול היה 2447<sup>2</sup>35 | ||
| + | |- | ||
| + | |We divide it by these divisors and write them one after another as we wish, for it does not matter, because there is no former and last in the divisors. | ||
| + | |style="text-align:right;"|ונחלקנו לאלו המורים ונשימם זה אחר זה כרצוננו כי זה לא יזיק כי אין מוקדם ומאוחר במורים | ||
| + | |- | ||
| + | |Divide the whole number by the last divisor to the left and write the remainder beneath it, then divide the result of divison by the one that precedes it; and so on, until the number is gone, [or] it reaches a phase in which the result of division is smaller than the divisor that precedes it. Then, you write the result beneath this preceding divisor and your procedure is complete. | ||
| + | |style="text-align:right;"|ותחלק כל החשבון למורה האחרון אשר לצד שמאל והנשאר שים תחתיו והיוצא בחילוק חלק לאשר לפניו וכן לעולם עד אשר יכלה המספר ויגיע למקום שהיוצא בחילוק יהיה פחות מהמורה אשר לפניו כי אז תשים זה היוצא תחת המורה הזה אשר לפניו וכבר כלית כל מלאכתך | ||
| + | |- | ||
| + | |If the divisors are all gone, but the number is not gone, and this happens when the dividend is greater than the number by which we wnat to divide that consists of these divisors, meaning that the divisors are derived from it, then write aside the result of division by the first divisor; these are integers; and as we did in this procedure, i.e. when we divided our number by these divisors, since the dividend is smaller than the number by which we want to divide that is above the divisors, the number is gone, but they are not gone. | ||
| + | |style="text-align:right;"|ואם יכלו המורים והמספר לא יכלה וזה יקרה כאשר היה המספר המתחלק גדול ‫<ref>22r</ref>מהמספר אשר רצינו לחלק עליו אשר {{#annot:term|2491,1961|8YuF}}הורכב{{#annotend:8YuF}} מהמורים <sup>ההם</sup> פי' שיצאו ממנו המורים ההם אז <sup>ה</sup>יוצא בחילוק בחלקך למורה הראשון תשימנו מבחוץ והם שלמים וכאשר עשינו זה המעש' ר"ל כשחלקנו מספרינו למורים אלו להיות המתחלק קטן מהמספר אשר רצינו לחלק עליו אשר הוא [מע]ל המורים יכלה המספר והמה לא יכלו | ||
| + | |- | ||
| + | | | ||
| + | :We receive that when we divide 2447235 by [50335084800], the result of division is two-fifths of a half of a quarter of an eighth of a third of a ninth of a sixth, i.e. when we decompose one unit to 6 parts, then 1 of these 6 [parts] to 9, then 1 of these 9 [parts] to 3, then 1 of these 3 [parts] to 8, then 1 of these 8 [parts] to 4, then 1 of these 4 [parts] to 2, then 1 of these 2 [parts] to five, each of the units of the large number, by which we divide, receives two parts of the former parts. | ||
| + | |style="text-align:right;"|ויצא לנו <sup>כי</sup> כאשר נחלק 2447235 על 5035<sup>0</sup>800 שהיוצא בחילוק הוא שני חמישיות חצי רביעית שמינית שלישית תשיעית שישית פי' כי כאשר עשינו האח' השלם ו' חלקים וא' מאלו הו' ט' וא' מאלו ה[.] <sup>ה</sup>ט' ג' וא' מאלו הג' ח' וא' מהח' א' ד' וא מהד' ב' וא' מב' אלו חמשה שיוצא לכל אחד מאחדי המספר הגדול אשר חלקנו עליו שתי חלקים חלק זה מהחלקים האחרונים האלו ה[.....] | ||
| + | |- | ||
| + | | | ||
| + | :We also get from the division 5-tenths of these parts, i.e. of a fifth of a half of a quarter of an eighth of a third of a ninth of a sixth. | ||
| + | |style="text-align:right;"|ועוד יצא בחלוקנו זה ה' עשיריות חלק זה <sup>פי'</sup> [ה'][עשיריות]‫<ref>marg.</ref> ה[חמיש]ית חצי רביעית שמינית שלישית תשיעית שישית | ||
| + | |- | ||
| + | | | ||
| + | :Also one-seventh of a tenth of a fifth of a half of a quarter etc. | ||
| + | |style="text-align:right;"|ועוד שביעית עשירית חמישית חצי רביעית וכו‫' | ||
| + | |- | ||
| + | | | ||
| + | :Also 4 parts of 11 of a seventh of a tenth of a fifth etc. | ||
| + | |style="text-align:right;"|ועוד ד' חלקים מי"א מז' מי' מה' וכו‫' | ||
| + | |- | ||
| + | | | ||
| + | :Also 9 parts of 13 of 11 [parts] of a seventh of a tenth of a fifth etc. | ||
| + | |style="text-align:right;"|ועוד ‫[ט' חלקים מי"ג מי"א מז' מי' מה' וכו‫' | ||
| + | |- | ||
| + | | | ||
| + | :Also 22 parts of 97 of 13 [parts] of 11 [parts] of a seventh of a tenth of a fifth etc. | ||
| + | |style="text-align:right;"|ועוד כ"ב‫]‫<ref>marg.</ref> כ"ב חלקים מצ"ז מי"ג מי"א מז' מי' מה' וכו‫' | ||
| + | |- | ||
| + | | | ||
| + | | | ||
| + | {| border="1" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| 97 || 13 || 11 || 7 || 10 || 5 || 2 || 4 || 8 || 3 || 9 || 6 | | 97 || 13 || 11 || 7 || 10 || 5 || 2 || 4 || 8 || 3 || 9 || 6 | ||
| Line 2,870: | Line 3,136: | ||
| 22 || 9 || 4 || 1 || 5 || 2 || colspan=6| | | 22 || 9 || 4 || 1 || 5 || 2 || colspan=6| | ||
|} | |} | ||
| + | |} | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle2447235\div50335084800&\scriptstyle=\left(\frac{2}{5}\sdot\frac{1}{2}\sdot\frac{1}{4}\sdot\frac{1}{8}\sdot\frac{1}{3}\sdot\frac{1}{9}\sdot\frac{1}{6}\right)+\left(\frac{5}{10}\sdot\frac{1}{5}\sdot\frac{1}{2}\sdot\frac{1}{4}\sdot\frac{1}{8}\sdot\frac{1}{3}\sdot\frac{1}{9}\sdot\frac{1}{6}\right)+\left(\frac{1}{7}\sdot\frac{1}{10}\sdot\frac{1}{5}\sdot\frac{1}{2}\sdot\frac{1}{4}\sdot\frac{1}{8}\sdot\frac{1}{3}\sdot\frac{1}{9}\sdot\frac{1}{6}\right)\\&\scriptstyle+\left(\frac{4}{11}\sdot\frac{1}{7}\sdot\frac{1}{10}\sdot\frac{1}{5}\sdot\frac{1}{2}\sdot\frac{1}{4}\sdot\frac{1}{8}\sdot\frac{1}{3}\sdot\frac{1}{9}\sdot\frac{1}{6}\right)+\left(\frac{9}{13}\sdot\frac{1}{11}\sdot\frac{1}{7}\sdot\frac{1}{10}\sdot\frac{1}{5}\sdot\frac{1}{2}\sdot\frac{1}{4}\sdot\frac{1}{8}\sdot\frac{1}{3}\sdot\frac{1}{9}\sdot\frac{1}{6}\right)\\&\scriptstyle+\left(\frac{22}{97}\sdot\frac{1}{13}\sdot\frac{1}{11}\sdot\frac{1}{7}\sdot\frac{1}{10}\sdot\frac{1}{5}\sdot\frac{1}{2}\sdot\frac{1}{4}\sdot\frac{1}{8}\sdot\frac{1}{3}\sdot\frac{1}{9}\sdot\frac{1}{6}\right)\\\end{align}}}</math> | ||
| + | {| | ||
| + | |- | ||
| + | |If you wish to receive more proper fractions, always see, when you divide the [divisor] into divisors, if the [dividend] has any of these divisors. | ||
| + | |style="width:45%; text-align:right;"|<big>ואם</big> תרצה שיצאו לך חלקים נאותים יותר תעיין לעולם כאשר תחלק המספר ל{{#annot:term|604,1239|Qr95}}מורים{{#annotend:Qr95}} אם למספר הזה יש שום אחד מהמורים ההם | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | ::Example in our number: the dividend has a ninth. Write this divisor last to the left and divide by it first; nothing remains to write beneath it. |
| + | |style="text-align:right;"|המשל <s>כאומרנו</s> במספרינו וזה המתחלק שיש לו תשיעית ותשים המורה ההוא האחרון לצד שמאל ותחלק עליו ראשונה ולא ישאר דבר לשום תחתיו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We see if the result of division has also one of the remaining divisors. Write it before the one by which we have divided and divide by it; nothing remains. |
| + | |style="text-align:right;"|וגם ליוצא בחילוק נעיין אם יש לו א' מהמורים הנותרים ונשימנו לפני זה אשר חלקנו עליו ונחלק ‫[עליו ולא ישאר [.] דבר | ||
|- | |- | ||
| | | | ||
| − | : | + | :Thus we always do in this matter, so that we get, when we divide the mentioned small number by the greater number, the result that each unit receives a half part of 13 of [a part of] 11 of a quarter of a third of a sixth, plus 9 parts of 97 of a tenth of an eighth of a half etc. as it is in this diagram: |
| − | |style="text-align:right;"|וכן נעשה לעולם בענין שיצא לנו בחלקינו זה] זה המספר הקטן הנזכר לגדול | + | |style="text-align:right;"|וכן נעשה לעולם בענין שיצא לנו בחלקינו זה‫]‫<ref>marg.</ref> זה המספר הקטן הנזכר לגדול שהיוצא לכל א' הוא חצי חלק מי"ג מי"א מד' מג' מו' ועוד ט' חלקים ‫<ref>22v</ref>מצ"ז מי' מח' מחצי וכו' הכל כמו שהוא בצורה הזאת |
|- | |- | ||
| | | | ||
| Line 2,889: | Line 3,163: | ||
| colspan=3| || 9 || || || 1 || colspan=5| | | colspan=3| || 9 || || || 1 || colspan=5| | ||
|} | |} | ||
| + | |} | ||
| + | :<math>\scriptstyle{\color{blue}{2447235\div50335084800=\left(\frac{1}{2}\sdot\frac{1}{13}\sdot\frac{1}{11}\sdot\frac{1}{4}\sdot\frac{1}{3}\sdot\frac{1}{6}\right)+\left(\frac{9}{97}\sdot\frac{1}{10}\sdot\frac{1}{8}\sdot\frac{1}{2}\sdot\frac{1}{13}\sdot\frac{1}{11}\sdot\frac{1}{4}\sdot\frac{1}{3}\sdot\frac{1}{6}\right)}}</math> | ||
| + | {| | ||
| + | |- | ||
| + | |These two [division] procedures are the same, yet the second procedure that is based on division by the divisors [of the given number] involves more proper fractions and this reduction is called '''perfect beauty''', as it means making the units nice and proper general amounts. | ||
| + | |style="width:45%; text-align:right;"|ואלו שני המעשים הכל אחד אלא שבמעשה השני חלקים יותר נאותים ולחלוקה על המורים עליו השגחה זו נקרא לו <big>{{#annot:term|1555,2488|ea69}}כלילת יופי{{#annotend:ea69}}</big> לפי שהוא לעשות מהפרטים כללים יפים ונאותים | ||
|- | |- | ||
| − | | | + | |In order to elaborate the matter and explain it in detail, I give an example for our division: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכ[די להרחיב הענין ו]לבארו בפי' אמשול משל לחלוקנו זה |
|- | |- | ||
| | | | ||
| + | ::The dividend has a ninth, therefore we place the 9 last and divide by it; nothing remains, so we do not write any thing beneath it. The result of division is 271915. | ||
::<math>\scriptstyle{\color{blue}{\frac{2447235}{9}=271915}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{2447235}{9}=271915}}</math> | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והוא כי המספר המתחלק יש לו תשיעית לכן שמנו הט' האחרון וחלקנוהו עליו ולא נשאר דבר על כן לא שמנו תחתיו דבר ויצא לנו בחלוק 271915 |
|- | |- | ||
| | | | ||
| + | ::This result of division has a fifth, therefore we place the 5 before the 9 and divide by it; nothing remains. The result of division is 54383. | ||
::<math>\scriptstyle{\color{blue}{\frac{271915}{5}=54383}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{271915}{5}=54383}}</math> | ||
| − | |style="text-align:right;"|ויש לזה היוצא בחילוק חמישית | + | |style="text-align:right;"|ויש לזה היוצא בחילוק חמישית לכן שמנו הה' לפני הט' וחלקנו עליו ולא נשאר דבר ויצא [לזה] היוצא בחלוק 54383 |
|- | |- | ||
| | | | ||
| + | ::It has a seventh, therefore we place the 7 immediately before the mentioned divisors and divide by it; nothing remains. The result of division is 7769. | ||
::<math>\scriptstyle{\color{blue}{\frac{54383}{7}=7769}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{54383}{7}=7769}}</math> | ||
| − | |style="text-align:right;"|ויש לו שביעית | + | |style="text-align:right;"|ויש לו שביעית לכן שמנו מיד ל[פני] המורים הנזכרים הז' וחלקנוהו עליו ולא נשאר דבר ויצא בחילוק ‫[7769]‫<ref>marg.</ref> |
|- | |- | ||
| | | | ||
| + | ::It has none of the remaining divisors, therefore we place whichever we want. We place the greater, which is 97, and divide by it; 9 remains. We place it beneath and the result of division is 80. | ||
::<math>\scriptstyle{\color{blue}{\frac{7769}{97}=80+\frac{9}{97}}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{7769}{97}=80+\frac{9}{97}}}</math> | ||
| − | |style="text-align:right;"|ואין לו שום אחד מהמורים הנותרים | + | |style="text-align:right;"|ואין לו שום אחד מהמורים הנותרים לכן נשים אשר נרצה ושמנו היותר גדול והוא הצ"ז וחלקנו עליו ונשאר ט' ושמנוהו תחתיו ויצא בחילוק 80 |
|- | |- | ||
| | | | ||
| + | ::It has a tenth, therefore we place the 10 immediately and divide by it; nothing remains. The result of division is 8. | ||
::<math>\scriptstyle{\color{blue}{\frac{80+\frac{9}{97}}{10}=8+\left(\frac{9}{97}\sdot\frac{1}{10}\right)}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{80+\frac{9}{97}}{10}=8+\left(\frac{9}{97}\sdot\frac{1}{10}\right)}}</math> | ||
|style="text-align:right;"|ויש לו עשירית לכן שמנו מיד ה10 וחלקנום עליו ולא נשאר דבר ויצא בחלוק ח‫' | |style="text-align:right;"|ויש לו עשירית לכן שמנו מיד ה10 וחלקנום עליו ולא נשאר דבר ויצא בחלוק ח‫' | ||
|- | |- | ||
| | | | ||
| + | ::It has an eighth, therefore we place the 8 immediately and divide by it; nothing remains. | ||
::<math>\scriptstyle{\color{blue}{\frac{8+\left(\frac{9}{97}\sdot\frac{1}{10}\right)}{8}=1+\left(\frac{9}{97}\sdot\frac{1}{10}\sdot\frac{1}{8}\right)}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{8+\left(\frac{9}{97}\sdot\frac{1}{10}\right)}{8}=1+\left(\frac{9}{97}\sdot\frac{1}{10}\sdot\frac{1}{8}\right)}}</math> | ||
| − | |style="text-align:right;"|ויש לו שמינית | + | |style="text-align:right;"|ויש לו שמינית לכן שמנו מיד הח' וחלקנום עליו ולא נשאר דבר ויצא בחלוק |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::Since it is smaller than any of the remaining divisors, we should not divise further, but we write it beneath the divisor that we write immediately after the mentioned written [divisors]: We write 2 and beneath it the 1 that results lastly in the division. Then: we write the remaining divisors randomly. |
| − | | | + | |style="text-align:right;"|ואחר שהוא פחות משום אחד מהמורים הנותרים אין לנו עוד לחלק אבל נשימהו תחת המורה אשר נשים מיד לפני המושמים הנזכרים ושמנו הב' ושמנו תחתיו זה הא' אשר יצא באחרונה בחלוק ואחר שמנו המורים הנותרים כאשר הזדמן |
| + | |} | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1+\left(\frac{9}{97}\sdot\frac{1}{10}\sdot\frac{1}{8}\right)}{2\sdot13\sdot11\sdot4\sdot3\sdot6}=\left(\frac{1}{2}\sdot\frac{1}{13}\sdot\frac{1}{11}\sdot\frac{1}{4}\sdot\frac{1}{3}\sdot\frac{1}{6}\right)+\left(\frac{9}{97}\sdot\frac{1}{10}\sdot\frac{1}{8}\sdot\frac{1}{2}\sdot\frac{1}{13}\sdot\frac{1}{11}\sdot\frac{1}{4}\sdot\frac{1}{3}\sdot\frac{1}{6}\right)}}</math> | ||
| + | {| | ||
|- | |- | ||
| − | | | + | |The rule that follows from this discussion: when we wish to divide any great or small number by another number, greater or smaller than it, we extract the divisors of the number by which we want to divide and write them randomly one after the other, or if one wishes, he can write them intentionally, in order to get more proper fractions, as we explained. Then, we divide the dividend by the last divisor to the left and write the remainder beneath it and we divide the result by the one that precedes it and so on. |
| − | |style="text-align:right;"| | + | |style="width:45%; text-align:right;"|<big>הכלל</big> העולה מהדברים הוא שכאשר נרצה לחלק ‫<ref>23r</ref>שום מספר גדול או קטן על מספר אחר גדול ממנו או קטן ממנו שנוציא מורה המספר אשר רצינו לחלק עליו ונשים אותם כפי המזדמן זה אחר זה או אם ירצה ישגיח בהנחתם יען יצאו החלקים יותר נאותים כאשר ביארנו ונחלק המספר המתחלק על המורה האחרון אשר לצד שמאל והנשאר נשים תחתיו והיוצא נחלק לאשר לפניו וכן לעולם |
|- | |- | ||
| − | | | + | |If the dividend is smaller than the one by which we divide it, the number is completely consumed, but [the divisors] are not gone. |
| − | + | |style="text-align:right;"|ואם היה המתחלק קטן מאשר חלקנו עליו יכלה המספר והמה לא יכלו | |
| − | |style="text-align:right;"|ואם היה המתחלק קטן מאשר חלקנו עליו | + | |- |
| + | |When it is consumed, the result of division is smaller than the divisor that precedes the divisors by which we have already divided, therefore we should not divide this smaller result by the divisor that is greater than it, but write it beneath it. | ||
| + | |style="text-align:right;"|וכאשר יכלה יהיה היוצא בחילוק פחות מהמורה אשר לפני המורים אשר חלקנו כבר עליהם לכן אין לנו לחלק זה היוצא המעט על זה המורה הרב ממנו אבל שימהו תחתיו | ||
|- | |- | ||
| − | | | + | |If the dividend is greater than the one by which we want to divide it, the divisors are all gone, but the number is not completely consumed. We write the result of the last divison aside the diagram and they are the integers resulting from the division. What is in the diagram beneath the divisors are the fractions and the fractions of fractions resulting from the division that are added to the mentioned integers. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>ואם</big> היה המספר המתחלק גדול מאשר רצינו לחלק עליו יכלו המורים והמספר לא יכלה והיוצא מן החלוק האחרון [....] נשימהו חוץ לצורה והם השלמים אשר יצאו בחלוק ואשר בתוך הצורה תחת המורים הם השברים ושברי שברים אשר יצאו בחלוק מוסף על השלימים הנזכרים |
|- | |- | ||
| − | | | + | |In order to elaborate the matter, I present another example: |
| − | + | |style="text-align:right;"|וכדי להרחיב הענין אעשה משל אחר | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle3123740520\div216</math> | + | *{{#annot:3123740520÷216|157|Z5A9}}We wish to divide 3123740520 by 216. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle3123740520\div216</math> |
| + | |style="text-align:right;"|<big>המשל</big> רצינו לחלק 3123740520 על 216{{#annotend:Z5A9}} | ||
|- | |- | ||
| | | | ||
| + | ::The divisors of the smaller number, by which we want to divide, are 9, 8, 3. | ||
::<math>\scriptstyle{\color{blue}{216=3\sdot8\sdot9}}</math> | ::<math>\scriptstyle{\color{blue}{216=3\sdot8\sdot9}}</math> | ||
| − | |style="text-align:right;"|והנה מורה זה המספר הקטן | + | |style="text-align:right;"|והנה מורה זה המספר הקטן אשר רצינו לחלק עליו הם אלו | 3 | 8 | 9 |
|- | |- | ||
| | | | ||
| + | ::We divide our greater number by 9; nothing remains and the result of division is 347082280. | ||
::<math>\scriptstyle{\color{blue}{\frac{3123740520}{9}=347082280}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{3123740520}{9}=347082280}}</math> | ||
|style="text-align:right;"|וחלקנו מספרינו זה הגדול על הט' ולא נשאר דבר ויצא בחילוק 347082280 | |style="text-align:right;"|וחלקנו מספרינו זה הגדול על הט' ולא נשאר דבר ויצא בחילוק 347082280 | ||
|- | |- | ||
| | | | ||
| + | ::We divide it by 8; nothing remains and the result of division is 43385285. | ||
::<math>\scriptstyle{\color{blue}{\frac{347082280}{8}=43385285}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{347082280}{8}=43385285}}</math> | ||
| − | |style="text-align:right;"|וחלקנום [על הח'] ולא נשאר דבר ויצא בחילוק 43385285 | + | |style="text-align:right;"|וחלקנום [על הח']‫<ref>marg.</ref> ולא נשאר דבר ויצא בחילוק 43385285 |
|- | |- | ||
| | | | ||
| + | ::We divide it by 3; the result of division of the last [number] is 14461761, which are the integers and 2 remain. | ||
| + | |style="text-align:right;"|‫[וחלקנום על הג' ויצא לנו בחלוק הזה האחרון 14461761 והם]‫<ref>marg.</ref> והם השלמים ונשארו ב‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We write it beneath the 3 and these are the fractions resulting from the division that are added to the integers. | ||
::<math>\scriptstyle{\color{blue}{\frac{43385285}{3}=14461761+\frac{2}{3}}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{43385285}{3}=14461761+\frac{2}{3}}}</math> | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ושמנום תחת הג' והם השברים היוצאים בחילוק הנוספים על השלמים |
| + | |- | ||
| + | | | ||
| + | :If now in this last division nothing would have remained also, we would not have written anything beneath it, and since nothing would have been found beneath the divisors, no fractions would have been resulted in division at all, only integers. | ||
| + | |style="text-align:right;"|ואם <sup>גם</sup> עתה בזה הח<sup>י</sup>לוק האחרון ‫<ref>23v</ref>לא היה נשאר דבר לא היינו שמים תחתיו דבר וכיון שלא נמצא דבר תחת המורים לא היו יוצאים בחילוק שברים כלל כי אם השלמים לבד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :This happen when the number by which we divide is a divisor of the original number. |
| + | |style="text-align:right;"|וזה יקרה כאשר החשבון אשר חלקנו עליו יהיה ראוי להיות מורה לחשבון המתחלה | ||
|- | |- | ||
| | | | ||
| + | :But, in our example, since 2 remains in the last division, it have also fractions. | ||
:<math>\scriptstyle{\color{blue}{3123740520\div216=14461761+\frac{2}{3}}}</math> | :<math>\scriptstyle{\color{blue}{3123740520\div216=14461761+\frac{2}{3}}}</math> | ||
| − | |style="text-align:right;"|ואולם במשלנו זה אח' אשר נשארו בחלוק האחרון ב' | + | |style="text-align:right;"|ואולם במשלנו זה אח' אשר נשארו בחלוק האחרון ב' יש לו ג"כ שברים |
| + | |- | ||
| + | | | ||
| + | :We write it beneath the 3 and we get that when we divide 3123740520 by 216, each unit receives 14461761 integers and 2-thirds, according to the following diagram: | ||
| + | |style="text-align:right;"|ושמנום תחת הג' ויצא לנו כי כאשר חלקנו 3123740520 על 216 <s>שיות</s> עד שיגיע לכל אחד מהם 14461761 שלמים וב' שלישיות כאשר בא בזאת הצורה | ||
|- | |- | ||
| | | | ||
| Line 2,971: | Line 3,280: | ||
|} | |} | ||
|- | |- | ||
| − | + | !<span style="color:Green>Check</span> | |
| − | |||
| − | |||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |When you want to check if the extraction of the divisors was correct: multiply the first by the second, then the product by the third, then by the fourth and so on until they are gone. If you receive the original number no more and no less from this calculation, know that the [divisors] are correct, and if not, they are not. |
| − | |style="text-align:right;"|ואם | + | |style="text-align:right;"|<big>ואם</big> רצית לבחון הוצאת המורים ההיתה כתקנה כפול הראשון בשני והעולה בשלישי והעולה ברביעי וכן לכלם עד כלותם ואם יצא לך מזה החשבון המספר הראשון בלי תוספת ומגרעת תדע שיצאו כתקנם ואם לאו לאו |
|- | |- | ||
| | | | ||
| − | + | :In the mentioned example, if we want to know if the resulting divisors are correct: we multiply 9 by 8; it is 72. We multiply it by 3; the product is 216. So, the divisors are all gone and the result is the number itself that have these divisors. | |
| − | |style="text-align:right;"|אם | + | :<math>\scriptstyle{\color{blue}{9\sdot8\sdot3=72\sdot3=216}}</math> |
| + | |style="text-align:right;"|<big>המשל</big> בצורה הנזכרת אם רצינו לידע אם המורי' יצאו על היושר נכפול ט' בח' יהיו ע"ב נכפלים בג' יעלו 216 והנה כלו המורים ויצא החשבון בעל המורים בעינו | ||
|- | |- | ||
| − | | | + | |When you want to know if you divide the number by the divisors correctly: |
| − | + | |style="text-align:right;"|<big>ואם</big> תרצה לידע אם חלקת המספר על המורים על היוש‫' | |
| − | |style="text-align:right;"|ואם | ||
|- | |- | ||
| − | | | + | |If there are integers [in the final result], multiply the integers by the first divisor, then multiply the whole product by the second divisor and add what you find beneath it to the product, then multiply the sum by the third divisor and add to it what you find beneath it. Continue like this until they are gone. If, when the divisors are gone, we get the dividend, your calculation is correct, and if not, it is not. |
| − | + | |style="text-align:right;"|אם יש שם שלמים כפול השלמים במורה הא' ההוא וכל {{#annot:term|241,1217|F3u6}}המקובץ{{#annotend:F3u6}} כפלהו במורה השני והוסף על העולה אשר תמצא תחתיו וכפול הכל על המורה [..] השלישי והוסף עליו אשר תמצא תחתיו וכן תעשה לעולם עד כלותם ואם ככלות המורים יצא לנו המספר המתחלק הלא מעשיך אמת ויציב ואם לאו לאו | |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |If there are no integers [in the final result], take what you find first beneath the first divisor that you find something beneath it and multiply it by the divisor that is next to it to the left. Add what is beneath it to the product and multiply the sum by the divisor that follows. Add what is beneath it and so on until they are gone. If the result is the dividend itself [the calculation] is correct, if not, it is not. |
| − | + | |style="text-align:right;"|ואם אין שם שלמים קח אשר תמצא ראשונה תחת המורה הקודם אשר תמצא תחתיו דבר וכפלהו במורה הסמוך לו לצד שמאל ‫<ref>24r</ref>וחבר הנמצא תחתיו עם העולה וכפול הכל על המורה הנמשך <s>הנמשך</s> והוסף אשר תחתיו וכן לעולם עד כלותם ואם אז יצא החשבון המתחלק בעינו הנה [נכון] ואם לאו לאו | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|המשל בתמונה | + | *Example in the following diagram: |
| + | |style="text-align:right;"|<big>המשל</big> בתמונה הזו | ||
|- | |- | ||
| | | | ||
| Line 3,012: | Line 3,312: | ||
| colspan=3| || 9 || colspan=2| || 1 || colspan=5| | | colspan=3| || 9 || colspan=2| || 1 || colspan=5| | ||
|} | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply the integers by 3, which is the first divisor; the result is 43385283. | ||
| + | |style="text-align:right;"|ונכפול השלמים בג' שהוא המורה הראשון ויעלה 43385283 | ||
| + | |- | ||
| + | | | ||
| + | ::We add to it the 2 that is beneath this divisor; the total is 43385285. | ||
| + | |style="text-align:right;"|נחבר לזה הב' אשר תחת המורה הזה ויעלה הכל 43385285 | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply it by 8, which is the second divisor; the result is 347082280. | ||
| + | |style="text-align:right;"|נכפלם על הח' שהוא המורה השני ויעלה 347082280 | ||
| + | |- | ||
| + | | | ||
| + | ::Since we do not find anything beneath this divisor, we do not add anything to it. | ||
| + | |style="text-align:right;"|ואחר שלא נמצא תחת זה המורה דבר לא נוסיף עליהם דבר | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply it again by 9, which is the third divisor; we receive the dividend itself, which is 3123740520. | ||
| + | |style="text-align:right;"|ונשוב ונכפלם בט' שהוא המורה השלישי ויצא לנו החשבון המתחלק בעינו שהוא 31237<sup>4</sup>0520 | ||
| + | |} | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left(14461761+\frac{2}{3}\right)\sdot3\sdot8\sdot9&\scriptstyle=\left[\left(14461761\sdot3\right)+2\right]\sdot8\sdot9=\left(43385283+2\right)\sdot8\sdot9=43385285\sdot8\sdot9\\&\scriptstyle=347082280\sdot9=3123740520\\\end{align}}}</math> | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | *I shall present it briefly in the example that precedes this one, in which there are no integers in the result of division, only fractions: | ||
| + | :<math>\scriptstyle2447235\div50335084800</math> | ||
| + | |style="width:45%; text-align:right;"|<big>עוד</big> אמשול זה בדרך קצרה בצורה הקודמת לזאת אשר אין שם שלמים ביוצא בחילוק כי אם שברים לבד | ||
| + | |- | ||
| + | | | ||
| + | ::We take the one that is beneath the 2, which is the first divisor beneath which there is something, and we multiply it by 8, which is the next divisor; the result is 8. | ||
| + | |style="text-align:right;"|ונקח הראשון אשר תחת הב' שהוא המורה הראשון אשר נמצא תחתיו דבר ונכפלהו בח' שהוא המורה הסמוך ויעלה ח‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Since there is nothing beneath it, we do not add anything to it and multiply it by 10, which is the next divisor; the result is 80. | ||
| + | |style="text-align:right;"|ואחר שאין תחתיו דבר לא נוסיף עליהם דבר ונכפלם בי' שהוא המורה הסמוך ויעלה פ‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Since nothing is found beneath it, we multiply it without adding by 97, which is the next divisor; the result is 7760. | ||
| + | |style="text-align:right;"|ואחר שלא נמצא תחתיו דבר נשוב ונכפלם בלי תוספת על הצ"ז שהוא המורה <sup>ה</sup>סמוך ויעלה 7760 | ||
| + | |- | ||
| + | | | ||
| + | ::We add to it the 9 that is beneath it; the result is 77[69]. | ||
| + | |style="text-align:right;"|נחבר אליהם הט' אשר תמצא תחתיו ויעלה 77669 | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply it by 7; the result is 54383. | ||
| + | |style="text-align:right;"|ונכפלם בז' ויעלה 54383 | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply it by 5; the result is 271915. | ||
| + | |style="text-align:right;"|ונשוב ונכפלם בה' ויעלה 271915 | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply it by 9, which is the last divisor; we receive the dividend itself, which is 2447235, so it is correct. | ||
| + | |style="text-align:right;"|ונכפלם בט' שהוא המורה האחרון ויצא לנו החשבון המתחלק בעינו והוא 2447235 והנה ‫[נכון הנה אמת | ||
| + | |} | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left[\left(1\sdot8\sdot10\sdot97\right)+9\right]\sdot7\sdot5\sdot9&\scriptstyle=\left[\left(8\sdot10\sdot97\right)+9\right]\sdot7\sdot5\sdot9=\left[\left(80\sdot97\right)+9\right]\sdot7\sdot5\sdot9=\left(7760+9\right)\sdot7\sdot5\sdot9\\&\scriptstyle=7769\sdot7\sdot5\sdot9=54383\sdot5\sdot9=271915\sdot9=2447235\\\end{align}}}</math> | ||
| + | ::<math>\scriptstyle{\color{blue}{\left[\left(\frac{1}{2}\sdot\frac{1}{13}\sdot\frac{1}{11}\sdot\frac{1}{4}\sdot\frac{1}{3}\sdot\frac{1}{6}\right)+\left(\frac{9}{97}\sdot\frac{1}{10}\sdot\frac{1}{8}\sdot\frac{1}{2}\sdot\frac{1}{13}\sdot\frac{1}{11}\sdot\frac{1}{4}\sdot\frac{1}{3}\sdot\frac{1}{6}\right)\right]\sdot50335084800=2447235}}</math> | ||
| + | {| | ||
| + | |- | ||
| + | ! | ||
| + | ==== <span style="color:Green>reason</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | |The reason for extracting the divisors and dividing the [dividend] by them: because the number by which we want to divide consists of these divisors. | ||
| + | |style="width:45%; text-align:right;"|וטעם הוצאת המורים וחלקנו עליהם המספר כנזכר הוא כי אחר שהמספר‫]‫<ref>marg.</ref> <s>עליהם המספר כנזכר הוא כי אחר שהמספר</s> אשר רצינו לחלק עליו הוא מורכב מאלו ‫<ref>24v</ref>המורים כמוהו כמוהם | ||
|- | |- | ||
| | | | ||
| − | + | *I.e. if 1 is divided by one hundred, for instance, each 1 receives from the hundred one part of the hundred that is in it. | |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle1\div100</math> |
| + | |style="text-align:right;"|ר"ל כי מי שיחלק א' על מאה עד"מ הנה יגיע ממנו לכל א' מהמאה חלק עליו ממאה שבו | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | ::Meaning, we decompose the one unit that we want to divide to one hundred equal parts and each 1 of the hundred, by which we want to divide the original 1, receives one part of them. |
| + | |style="text-align:right;"|פי' שנעשה הא' השלם אשר רצינו לחלק [מאה חלקים שוים ויגיע לכל א' מהמאה אשר רצינו לחלק עליהם‫]‫<ref>marg.</ref> עליהם <sup>הא'</sup> הראשון חלק א' מהם | ||
|- | |- | ||
| | | | ||
| − | + | ::Since the 100, by which we want to divide, has a fifth and its fifth is 20, we find that each 1 of the hundred is one part of 20 of a fifth of a hundred. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואחר שחשבון הק' אשר רצינו לחלק <s>[עליהם] הא' חלק א' מהם ואחר שחשבון</s> יש לו חמישית וחמישיתו הוא כ' נמצא שכל א' מהמאה הוא חלק א' מכ' מחמישית שבמאה |
|- | |- | ||
| | | | ||
| + | :When we decompose the whole unit to 100 parts, each one of them is one part of 100 of the whole and it is also one part of twenty of a fifth of the whole. So our saying one part of 100 of the whole is as our saying one part of 20 of a fifth of the whole. | ||
:<math>\scriptstyle{\color{blue}{1\div100=\frac{1}{20}\sdot\frac{1}{5}}}</math> | :<math>\scriptstyle{\color{blue}{1\div100=\frac{1}{20}\sdot\frac{1}{5}}}</math> | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכאשר נעשה א' שלם ק' <s>אקלימים</s> חלקים הנה כל אחד מהם הוא חלק אחד <sup>מק'</sup> <s>שבשלם</s> <sup>שבשלם</sup> וגם הוא חלק א' מעשרים מחמישית השלם הרי שאמרנו חלק א' מק' שבשלם כאומרנו חלק אחד מכ' מחמישית שבשלם |
|- | |- | ||
| | | | ||
| + | ::For this reason itself, our saying a quarter of a fifth is as our saying one part of 20. Since the twenty has a fifth and its fifth is 4. We find that twenty consists of 5 and 4 and one [part of twenty] is a fifth of a quarter, or a quarter of a fifth, because it is the same. Hence, our saying a quarter of a fifth is as our saying one part of 20 of the whole. | ||
::<math>\scriptstyle{\color{blue}{1\div20=\frac{1}{4}\sdot\frac{1}{5}}}</math> | ::<math>\scriptstyle{\color{blue}{1\div20=\frac{1}{4}\sdot\frac{1}{5}}}</math> | ||
| − | |style="text-align:right;"|ולסבה זו בעינה יהיה אומרנו רביעית חמישית כאומרנו חלק א' מכ' | + | |style="text-align:right;"|ולסבה זו בעינה יהיה אומרנו רביעית חמישית כאומרנו חלק א' מכ' לפי שעשרים יש לו חמישית וחמישיתו ד' נמצא שעשרים מורכב מה' וד' ושהאחד הוא חמישית רביעית או רביעית חמישית כי הכל א' הרי לנו ש שאמרנו רביעית חמישית כאומרנו חלק א' מכ' שבשלם |
|- | |- | ||
| | | | ||
| + | :But, our saying one part of 20 of a fifth of the whole is as our saying one part of 100 of the whole, as we have explained. So, we get that our saying a quarter of a fifth of a fifth is as our saying one part of 100 of the whole. | ||
:<math>\scriptstyle{\color{blue}{1\div100=\frac{1}{20}\sdot\frac{1}{5}=\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{5}}}</math> | :<math>\scriptstyle{\color{blue}{1\div100=\frac{1}{20}\sdot\frac{1}{5}=\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{5}}}</math> | ||
| − | |style="text-align:right;"|[ואולם אמרנו חלק אחד מכ' מה' שבשלם הוא כאמרנו חלק א' מק' שבשלם כאשר] כאשר ביארנו | + | |style="text-align:right;"|‫[ואולם אמרנו חלק אחד מכ' מה' שבשלם הוא כאמרנו חלק א' מק' שבשלם כאשר‫]‫<ref>marg.</ref> כאשר ביארנו הנה יצא לנו שאומרנו רביעית חמישית חמישית הוא כאומרנו חלק א' מק' שבשלם |
|- | |- | ||
| − | | | + | |Likewise for any number consisting of divisors, as many as they may be. |
|style="text-align:right;"|ובדמיון זה בכל חשבון מורכב ממורים כמה שיהיו | |style="text-align:right;"|ובדמיון זה בכל חשבון מורכב ממורים כמה שיהיו | ||
|- | |- | ||
| | | | ||
| + | :Thus, if we divide the whole unit by 100, each 1 of them receives one part of 100 of the whole, which is a quarter of a fifth of a fifth. | ||
:<math>\scriptstyle{\color{blue}{1\div100=\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{5}}}</math> | :<math>\scriptstyle{\color{blue}{1\div100=\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{5}}}</math> | ||
| − | |style="text-align:right;"|ואם כאשר חלקנו א' שלם לק' | + | |style="text-align:right;"|ואם כאשר חלקנו א' שלם לק' הגיע לכל א' מהם חלק אחד מק' שבשלם שהוא רביעית חמישית החמישית |
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle2\div100</math> | + | *If we divide 2 integers by 100. |
| + | :<math>\scriptstyle2\div100</math> | ||
|style="text-align:right;"|ואם חלקנו על ק' ב' שלמים | |style="text-align:right;"|ואם חלקנו על ק' ב' שלמים | ||
|- | |- | ||
| | | | ||
| + | :Each one receives 2-quarters of a fifth of a fifth. | ||
:<math>\scriptstyle{\color{blue}{2\div100=\frac{2}{4}\sdot\frac{1}{5}\sdot\frac{1}{5}}}</math> | :<math>\scriptstyle{\color{blue}{2\div100=\frac{2}{4}\sdot\frac{1}{5}\sdot\frac{1}{5}}}</math> | ||
|style="text-align:right;"|יגיע לכל א' ב' רביעיות חמישיות חמישית | |style="text-align:right;"|יגיע לכל א' ב' רביעיות חמישיות חמישית | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle3\div100</math | + | *If 3, 3. |
| + | :<math>\scriptstyle3\div100</math> | ||
:<math>\scriptstyle{\color{blue}{3\div100=\frac{3}{4}\sdot\frac{1}{5}\sdot\frac{1}{5}}}</math> | :<math>\scriptstyle{\color{blue}{3\div100=\frac{3}{4}\sdot\frac{1}{5}\sdot\frac{1}{5}}}</math> | ||
|style="text-align:right;"|ואם ג' ג‫' | |style="text-align:right;"|ואם ג' ג‫' | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle n\div100</math | + | *Thus, as the number of integers divided by 100, so is the number of quarters of a fifth of a fifth that each of them receives, and this is clear. |
| + | :<math>\scriptstyle n\div100</math> | ||
:<math>\scriptstyle{\color{blue}{n\div100=\frac{n}{4}\sdot\frac{1}{5}\sdot\frac{1}{5}}}</math> | :<math>\scriptstyle{\color{blue}{n\div100=\frac{n}{4}\sdot\frac{1}{5}\sdot\frac{1}{5}}}</math> | ||
| − | |style="text-align:right;"|הרי [הרי] כי מספר השלמים אשר נחלק אל ק' מספר הרביעיות חמישיות חמישית שיגיעו לכל אחד מהם וזה ברור | + | |style="text-align:right;"|הרי [הרי‫]‫<ref>marg.</ref> כי מספר השלמים אשר נחלק אל ק' מספר הרביעיות חמישיות חמישית שיגיעו לכל אחד מהם וזה ברור |
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle70\div100</math> | + | *{{#annot:70÷100|157|rm2i}}If we wish to divide 70 by 100. |
| − | |style="text-align:right;"|ולזה אם רצינו לחלק ע' על ק‫' | + | :<math>\scriptstyle70\div100</math> |
| + | |style="text-align:right;"|ולזה אם רצינו לחלק <sup>ע'</sup> על ק‫'{{#annotend:rm2i}} | ||
|- | |- | ||
| | | | ||
| + | :We know that each of them receives 70 quarters of a fifth of a fifth. It is as if we write the denominators by this order and place the 70 beneath the last denominator. | ||
:<math>\scriptstyle{\color{blue}{70\div100=\frac{70}{4}\sdot\frac{1}{5}\sdot\frac{1}{5}}}</math> | :<math>\scriptstyle{\color{blue}{70\div100=\frac{70}{4}\sdot\frac{1}{5}\sdot\frac{1}{5}}}</math> | ||
| − | |style="text-align:right;"|ידענו שיגיע לכל אחד מהם ע' רביעיות חמישיות חמישית והרי הוא כאלו שמנו המורים כזה הסדר | + | |style="text-align:right;"|ידענו שיגיע לכל אחד מהם ע' רביעיות חמישיות חמישית והרי הוא כאלו שמנו המורים כזה הסדר ושמנו הע' <s>רביעיות</s> תחת המורה האחרון |
|- | |- | ||
| | | | ||
| Line 3,080: | Line 3,459: | ||
|- | |- | ||
| | | | ||
| + | :Since you have 70 quarters of a fifth of a fifth, if we want to know how many fifths of a fifth they are, it is as if we have 70 quarters and we want to know how many integers they are. | ||
| + | |style="text-align:right;"|ואחר שיש בידיך 70 ‫<ref>25r</ref>רביעיות חמשיות חמשית אם בקשנו לדעת כמה חמשיות חמישית הם הרי הוא כאלו היו בידינו ע' רביעיות ורצינו לדעת כמה שלמים הם | ||
| + | |- | ||
| + | | | ||
| + | :This is known by dividing them into 4, because every 4 quarters are one integer and also every 4 quarters of a fifth of a fifth are one-fifth of a fifth. So, we divide these 70 quarters of a fifth of a fifth and the remainder is of the first type, i.e. quarters of a fifth of a fifth. | ||
| + | |style="text-align:right;"|וזה יודע בחלקנו אותם לד' לפי שכל ד' רביעיות הם א' שלם וכן כל ד' רביעיות חמישית [חמישית חמישית הם חמישית חמישית שלם לכן נחלק אלו הע' רביעיות חמישית‫]‫<ref>marg.</ref> חמישית והנשאר יהיה מהמין הראשון ר"ל רביעיות חמישיות חמישית | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, we should write the result of division, which is 17, beneath the 5, which is the preceding divisor and the remainder, which is 2, beneath the 4, which is the last divisor by which we divide, like this: | ||
:<math>\scriptstyle{\color{blue}{70\div100=\frac{70}{4}\sdot\frac{1}{5}\sdot\frac{1}{5}=\left(\frac{17}{5}\sdot\frac{1}{5}\right)+\left(\frac{2}{4}\sdot\frac{1}{5}\sdot\frac{1}{5}\right)}}</math> | :<math>\scriptstyle{\color{blue}{70\div100=\frac{70}{4}\sdot\frac{1}{5}\sdot\frac{1}{5}=\left(\frac{17}{5}\sdot\frac{1}{5}\right)+\left(\frac{2}{4}\sdot\frac{1}{5}\sdot\frac{1}{5}\right)}}</math> | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ולזה ראוי לנו לשים היוצא בחלוק שהוא י"ז תחת הה' אשר הוא המורה אשר לפניו <s>והנשאר שהוא תחת הד' שהוא המורה אשר לפניו</s> והנשאר שהוא ב' תחת הד' שהוא המורה האחרון אשר חלקנו עליו כזה |
| + | |- | ||
| + | | | ||
| + | | | ||
{| border="1" style="margin-left: auto; margin-right: 0px; text-align:center;" | {| border="1" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| Line 3,087: | Line 3,478: | ||
|- | |- | ||
| 2 || 17 || | | 2 || 17 || | ||
| − | |} | + | |} |
| − | הנה ידענו כי כאשר חלקנו ע' על ק' | + | |- |
| + | | | ||
| + | :We know that when we divide 70 by 100, each one of them receives 17 fifths of a fifth and 2-quarters of a fifth of a fifth. | ||
| + | |style="text-align:right;"|הנה ידענו כי כאשר חלקנו ע' על <sup>ק'</sup> שהגיע לכל אחד מהם י"ז חמישיות חמישית וב' רביעיות חמשית חמישית | ||
| + | |- | ||
| + | | | ||
| + | :Since we have 17 fifths of a fifth, we know that they are three-fifths and more, because every five fifths of a fifth are one-fifth, as five-fifths are one integer. | ||
| + | |style="text-align:right;"|ואחר שיש בידינו י"ז חמישיות חמישית ידענו שהם שלשה חמישיות שלמות ויותר לפי שכל חמש חמשיות חמישית הם חמישית אחד כמו שחמש חמשיות שלם הן שלם | ||
|- | |- | ||
| | | | ||
| + | :We should know how many fifths they are, so we divide them by 5; the result of division is 3, which is 3-fifths, so we write it beneath the first 5, and the 2, [which is the] remainder is of the same type as the first, i.e. fifths of a fifth, so we write it beneth the second 5; like this: | ||
| + | |style="text-align:right;"|וראוי לנו לידע כמה חמישיות שלמות הן לכן נחלקם על הה' ויצא לנו בחלוק ג' שהוא ג' חמישיות שלימות לכן נשימם תחת הה' הראשון והב' השני והנשארים הם ממין במינם כבתחלה ר"ל חמישיות חמישית לכן שמנום תחת הה' השני כזה | ||
| + | |} | ||
:<math>\scriptstyle{\color{blue}{70\div100=\frac{70}{4}\sdot\frac{1}{5}\sdot\frac{1}{5}=\left(\frac{17}{5}\sdot\frac{1}{5}\right)+\left(\frac{2}{4}\sdot\frac{1}{5}\sdot\frac{1}{5}\right)=\frac{3}{5}+\left(\frac{2}{5}\sdot\frac{1}{5}\right)+\left(\frac{2}{4}\sdot\frac{1}{5}\sdot\frac{1}{5}\right)}}</math> | :<math>\scriptstyle{\color{blue}{70\div100=\frac{70}{4}\sdot\frac{1}{5}\sdot\frac{1}{5}=\left(\frac{17}{5}\sdot\frac{1}{5}\right)+\left(\frac{2}{4}\sdot\frac{1}{5}\sdot\frac{1}{5}\right)=\frac{3}{5}+\left(\frac{2}{5}\sdot\frac{1}{5}\right)+\left(\frac{2}{4}\sdot\frac{1}{5}\sdot\frac{1}{5}\right)}}</math> | ||
| − | | | + | {| |
| + | |- | ||
| + | | | ||
| + | | | ||
{| border="1" style="margin-left: auto; margin-right: 0px; text-align:center;" | {| border="1" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| Line 3,101: | Line 3,505: | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ואם אלו הג' אשר תחת המורה הראשון היה כמותו | + | :If this 3 that is beneath the first divisor was the same as it or greater, i.e. if it was 5 or more, the result would have been an integer, for every five fifths are one integer, so we should divide them by 5; the result of division would have been an integer and the remainder would have been fifths as at first. |
| + | |style="width:45%; text-align:right;"|ואם אלו הג' אשר תחת המורה הראשון היה כמותו או גדול ממנו ר"ל שהיה ה' או יותר היה העולה לשלם או לשלימים כי כל חמש חמשיות הן שלם אחד והיה ראוי לנו לחלק אותן על ה' והיוצא בחילוק היו שלימים והנשאר היה חמישיות כאשר בתחלה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|אכן אחר שהוא קטן מהמורה | + | :Since it is smaller than the divisor, i.e. it is less than 5, which is the first divisor, there is no integer at all and we should not divide [further], as we already achieved our goal, that is, when we divide 70 by 100, each one of them receives 3-fifths, 2-fifths of a fifth, and 2-quarters of a fifth of a fifth. |
| + | |style="text-align:right;"|אכן אחר שהוא קטן מהמורה ר"ל שהוא פחות מה' שהוא המורה שהוא המורה הראשון אין כאן שלם כלל ואין לנו לעשות שום חלוק אבל כבר השגנו מבוקשנו והוא כי כאשר ‫<ref>25v</ref>חלקנו ע' על ק' שהגיע לכל אחד מהם ג' חמישיות וב' חמישיות חמישית וב' רביעיות חמישית חמישית | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|הכלל העולה מאלו | + | :The rule that follows from this discussion is that if 70 is divided by 100, each one receives 70 parts of 100 parts of the whole. |
| + | |style="text-align:right;"|הכלל העולה <s>מאלו</s> <sup>מ</sup>הדברים שהמחלק ע' על ק' יגיע לכל אחד ע' חלקים מק' חלקים בשלם | ||
|- | |- | ||
| − | | | + | | |
| + | :Since one hundred consists of these three numbers, i.e. from 5, 5, and 4. This is because when we multiply the one by the other and the product by the remaining, the result is 100, meaning the product of 5 by 5 is 25 and when we multiply it by 4, the result is 100. | ||
:<math>\scriptstyle{\color{blue}{5\sdot5\sdot4=25\sdot4=100}}</math> | :<math>\scriptstyle{\color{blue}{5\sdot5\sdot4=25\sdot4=100}}</math> | ||
| − | |style="text-align:right;"|ואחר שמאה הוא מורכב מאלו השלשה מספרים | + | |style="text-align:right;"|ואחר שמאה הוא מורכב מאלו השלשה מספרים ר"ל מה' וה' וד' וזה כי כאשר כפלנו הא' בחבירו והעולה בנשאר עולה ק' פי' כי כפל ה' בה' הוא כ"ה וכאשר כפלנום בד' יעלו ק‫' |
| + | |- | ||
| + | |This is the check of the extraction of the divisors that we mentioned above, for when multiplying one by the other, then the product by another and so on until they end and we get the original number, we know that this number consists of these numbers. | ||
| + | |style="text-align:right;"|וזאת היא בחינת הוצאת המורים אשר הזכרנו למעלה כי בכפול זה בזה והעולה באחר וכן לעולם עד כלותם ויצא לנו החשבון הראשון ידענו שהחשבון ההוא מורכב מאלו ה[ב'‫]‫<ref>marg.</ref> מספרים | ||
|- | |- | ||
| | | | ||
| + | :Since 100 consists of 5 and 4, our saying one part of 100 of the whole is as our saying a quarter of a fifth of a fifth, or our saying a fifth of a fifth of a quarter, because all is the same. | ||
:<math>\scriptstyle{\color{blue}{\frac{1}{100}=\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{5}=\frac{1}{5}\sdot\frac{1}{5}\sdot\frac{1}{4}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{1}{100}=\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{5}=\frac{1}{5}\sdot\frac{1}{5}\sdot\frac{1}{4}}}</math> | ||
| − | |style="text-align:right;"|ואחר היות הק' מורכב מה' הד' | + | |style="text-align:right;"|ואחר היות הק' מורכב מה' הד' כך הוא אומרנו חלק מק' שבשלם כאומרנו רביעית חמישית חמישית רביעית חמישית או כאומרנו חמישית חמישית רביעית כי הכל אחד |
|- | |- | ||
| − | | | + | |For another reason we say that we can arrange the divisors randomly one after the other, or intentionally, in order to receive reduced fractions that are as proper as possible. |
| − | |style="text-align:right;"|ומטעם אחר על זה אמרנו שבידינו לסדר המורים זה אחר זה כפי המזדמן | + | |style="text-align:right;"|ומטעם אחר על זה אמרנו שבידינו לסדר המורים זה אחר זה כפי המזדמן אם בהשגחה כדי שיצאו השברים היותר שלמים שיוכל והיותר נאותים |
|- | |- | ||
| | | | ||
| + | :Because, when we say that if 70 is divided by one hundred, each one receives 70 quarters of a fifth of a fifth, we could say 70 fifths of a quarter of a fifth, or 70 fifths of a fifth of a quarter. | ||
:<math>\scriptstyle{\color{blue}{70\div100=\frac{70}{4}\sdot\frac{1}{5}\sdot\frac{1}{5}=\frac{70}{5}\sdot\frac{1}{4}\sdot\frac{1}{5}=\frac{70}{5}\sdot\frac{1}{5}\sdot\frac{1}{4}}}</math> | :<math>\scriptstyle{\color{blue}{70\div100=\frac{70}{4}\sdot\frac{1}{5}\sdot\frac{1}{5}=\frac{70}{5}\sdot\frac{1}{4}\sdot\frac{1}{5}=\frac{70}{5}\sdot\frac{1}{5}\sdot\frac{1}{4}}}</math> | ||
| − | |style="text-align:right;"|וזה כי כאשר אמרנו שהמחלק ע' על המאה יגיע לכל אחד ע' רביעיות חמישית חמישית | + | |style="text-align:right;"|וזה כי כאשר אמרנו שהמחלק ע' על המאה יגיע לכל אחד ע' רביעיות חמישית חמישית היינו יכולים לומר ע' חמשיות רביעית חמישית או ע' חמישיות חמישית רביעית |
| + | |- | ||
| + | |Since we can arrange them as we wish, it is appropriate to arrange them and keep them in order. | ||
| + | |style="text-align:right;"|ואחר שבידינו לסדרם כחפצנו ראוי לסדרם ולהשגיח בסדורו | ||
|- | |- | ||
| | | | ||
| + | :This is because when we want to divide the 70 by these divisors, since 70 has a fifth, which is one of these divisors, we should divide it first by 5 and place it last, so that nothing is left to write beneath it. The result of division is 14. | ||
| + | |style="text-align:right;"|וזה כי כאשר רצינו לחלק אלו הע' לאלו המורים אחר שהעין יש לו חמישית שהוא א' מאלו המורים ראוי לנו לחלקם ראשונה על הה' ונשימנו אחרון כדי שלא ישאר דבר לשים תחתנו ויצא בחילוק י"ד | ||
| + | |- | ||
| + | | | ||
| + | ::If it would have had a quartet, it would have been appropriate to divide it by 4 and place it before the last. | ||
| + | |style="text-align:right;"|ואם היה להם רביעית היה ראוי לחלקם על ד' ולשומו לפני האחרון | ||
| + | |- | ||
| + | | | ||
| + | ::If a fifth, by 5. | ||
| + | |style="text-align:right;"|ואם היה לו ה' לה‫' | ||
| + | |- | ||
| + | | | ||
| + | :Since it does not have one of them, we arrange the remaining divisors randomly. | ||
| + | |style="text-align:right;"|אכן ‫<ref>26r</ref>שאין לו אחד מהם נסדר אלו השני המורים הנשארים כפי המזדמן | ||
| + | |- | ||
| + | | | ||
| + | :For instance, we divide the 14 by 4 and place it before the last; the result of division is 3. Since it is less than the 5, which is the other divisor, we write it beneath it and we write the remaining 2 beneath the 4, which is the second divisor by which we divide. Like this: | ||
:<math>\scriptstyle{\color{blue}{70\div100=\frac{70}{5}\sdot\frac{1}{4}\sdot\frac{1}{5}=\frac{14}{4}\sdot\frac{1}{5}=\frac{3}{5}+\left(\frac{2}{4}\sdot\frac{1}{5}\right)}}</math> | :<math>\scriptstyle{\color{blue}{70\div100=\frac{70}{5}\sdot\frac{1}{4}\sdot\frac{1}{5}=\frac{14}{4}\sdot\frac{1}{5}=\frac{3}{5}+\left(\frac{2}{4}\sdot\frac{1}{5}\right)}}</math> | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וע'ד'מ' נחלק אלו הי"ד לד' ונשימהו לפני האחרון ויצא בחילוק ג' ואחר שהוא פחות מהה' שהוא המורה האח' נשימהו תחתיו והב' הנשארים נשימם תחת הד' שהוא המורה השני [אשר‫]‫<ref>marg.</ref> נחלקנו עליו כזה |
| − | |||
| − | |||
| − | |||
| − | |||
| − | וע'ד'מ' נחלק אלו | ||
|- | |- | ||
| | | | ||
| Line 3,143: | Line 3,571: | ||
|- | |- | ||
| | | | ||
| + | :Thus, we get more proper fractions, because it is more appropriate to say 3-fifths and two-quarters of a fifth, which is half a fifth, as appears in this diagram, than saying 3-fifths, two-fifths of a fifth, and 2-quarters of a fifth of a fifth. | ||
:<math>\scriptstyle{\color{blue}{70\div100=\frac{3}{5}+\left(\frac{2}{5}\sdot\frac{1}{5}\right)+\left(\frac{2}{4}\sdot\frac{1}{5}\sdot\frac{1}{5}\right)=\frac{3}{5}+\left(\frac{2}{4}\sdot\frac{1}{5}\right)=\frac{3}{5}+\left(\frac{1}{2}\sdot\frac{1}{5}\right)}}</math> | :<math>\scriptstyle{\color{blue}{70\div100=\frac{3}{5}+\left(\frac{2}{5}\sdot\frac{1}{5}\right)+\left(\frac{2}{4}\sdot\frac{1}{5}\sdot\frac{1}{5}\right)=\frac{3}{5}+\left(\frac{2}{4}\sdot\frac{1}{5}\right)=\frac{3}{5}+\left(\frac{1}{2}\sdot\frac{1}{5}\right)}}</math> | ||
| − | |style="text-align:right;"|והנה יצאו לנו חלקים יותר נאותים | + | |style="text-align:right;"|והנה יצאו לנו חלקים יותר נאותים כי יותר נאות הוא לומר ג' חמישיות [ושני רביעיות חמישית‫]‫<ref>marg.</ref> שהן חצי חמישית כאשר בא בצורה הזאת מאומרנו ג' חמישיות ושני חמישיות חמישית וב' רביעיות חמישית חמישית |
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"|וידוע הוא כי בחינת זה הוא להשיבם כלם מהמין הראשון | + | :It is known that the check of this is to convert all of them to the first type, i.e. fifths of a quarter of a fifth, as the last diagram, or quarters of a fifth of a fifth, as the preceding diagram, and this is called "decomposing to a fraction", as will be explained in the second section. |
| + | |style="text-align:right;"|וידוע הוא כי בחינת זה הוא להשיבם כלם מהמין הראשון ר"ל חמישיות רביעית חמישית כפי צורה זו האחרונה או רביעית חמישית חמישית כפי הצורה הקודמת וזה יקרא פריט<sup>ה</sup> כאשר יתבאר בחלק השני | ||
|- | |- | ||
| | | | ||
| − | : | + | :Since it has whole fifths and also quarters of a fifth, we convert all of them first to quarters of a fifth. |
| − | |style="text-align:right;"|ואחר שיש לו חמישיות שלמות | + | |style="text-align:right;"|ואחר שיש לו חמישיות שלמות גם רביעיות חמישית נשיבם כלם ראשונה רביעיות חמישית |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :It is known that every whole fifth is 4-quarters, meaning 4-quarters of a fifth, as every integer is 4-quarters of the whole. |
| + | |style="text-align:right;"|וידוע כי כל חמישית שלמה היא ד' רביעיותיה פי' ד' רביעית חמישית כמו שכל שלם ד' רביעיות שלם | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We get that each one of these 3 whole fifths is 4-quarters of a fifth. |
| + | |style="text-align:right;"|הרי לנו כי כל אחד מאלו הג' חמישיות שלימות היא ד' רביעיות חמישית | ||
|- | |- | ||
| | | | ||
| − | + | :To know how many are they, we multiply 3, which is the number of the fifths, by 4, which is the next divisor; the result is 12. We get that 3-fifths are 12 quarters of a fifth. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ולדעת כמה הם נכפול ג' שהוא מספר החמישיות בד' שהוא המורה הבא אחריו ויעלה י"ב הרי לנו שהג' חמישיות הם י"ב רביעיות חמישית |
|- | |- | ||
| | | | ||
| − | : | + | :We find two beneath it, which is of this type, meaning quarters of fifths. We add it to them; they are 14 quarters of a fifth. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומצאנו תחתיו שנים שהם מזה המין פי' שהם רביעיות חמישיות נחברם אליהם ויהיו י"ד רביעיות חמישית |
|- | |- | ||
| | | | ||
| − | : | + | :When we want to know how many fifths of a quarter of a fifth are they, we multiply them by 5, which is the divisor that follows them; the total result is 70. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכאש' נרצה לדעת כמה חמישיות רביעית חמישית הם נכפלם בה' שהוא המורה הבא אחריהם ויעלו כלם <sup>ע‫'</sup> |
|- | |- | ||
| | | | ||
| + | :If there was anything beneath this divisor, it would have been also fifths of a quarter of a fifth and we would have add it to them. | ||
| + | |style="text-align:right;"|ואם תחת זה המורה היה נמצא דבר זה היה ג"כ חמישיות רביעית חמישית ‫<ref>26v</ref>והיינו מחברים אותם אליהם | ||
| + | |- | ||
| + | | | ||
| + | :Since nothing is found beneath it and the divisors are all gone, we have completed our procedure | ||
| + | |style="text-align:right;"|אכן אחר שלא נמצא תחתיו דבר וכבר כלו המורים כבר כלינו מעשינו | ||
| + | |- | ||
| + | | | ||
| + | :Since the result is our original number, meaning 70, which is the small number that we wanted to divide by 100, which has these divisors no more and no less, we know that our procedure is right. | ||
| + | |style="text-align:right;"|ואחר שעלה לחשבוננו הראשון פי' לעין [ע']‫<ref>marg.</ref> שהוא החשבון הקטן אשר רצינו לחלק על הק' שהוא בעל אלו המורים מבלי תוספת ומגרעת ידענו כי כל מעשי<sup>נ</sup>ו בצדק ובמשפט | ||
| + | |} | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{3}{5}+\left(\frac{2}{4}\sdot\frac{1}{5}\right)=\frac{\left(3\sdot4\right)+2}{4}\sdot\frac{1}{5}=\frac{12+2}{4}\sdot\frac{1}{5}=\frac{14}{4}\sdot\frac{1}{5}=\frac{14\sdot5}{5}\sdot\frac{1}{4}\sdot\frac{1}{5}=\frac{70}{5}\sdot\frac{1}{4}\sdot\frac{1}{5}=70\div100}}</math> | ||
| + | {| | ||
| + | |- | ||
| + | |Thus, the reasons of the all that was mentioned - the procedure as well as the examinations - are clear, and all is explained generally and particularly. | ||
| + | |style="width:45%; text-align:right;"|הרי לנו מבוארים טעמי' כל הנזכר גם המעשה גם הבחינות וביאור הכל בכלל ובפרט | ||
| + | |- | ||
| + | !<span style="color:Green>Division of a large number by a smaller number, with a result of integers and fractions</span> | ||
| + | | | ||
| + | |- | ||
| + | |In order to train I bring another example, in which the divided by the 100 is greater than it, so the result are fractions as well as integers. | ||
| + | |style="text-align:right;"|<big>וכדי להתלמד</big> אביא משל אחר שיהיה <sup>ה</sup>מתחלק על אלו הק' גדול מהם כדי שיצאו שם שברים גם שלמים | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:140÷100|157|ftSN}}We wish to divide 140 by 100. | ||
| + | :<math>\scriptstyle140\div100</math> | ||
| + | |style="text-align:right;"|<big>המשל</big> רצינו לחלק ק"מ על ק‫'{{#annotend:ftSN}} | ||
| + | |- | ||
| + | | | ||
| + | :*Since the 140, which is the number that we want to divide, has all these divisors, we place whichever we want last and divide by it; by 5, for instance. The result of division is 28 and nothing remains. | ||
| + | ::<math>\scriptstyle{\color{blue}{140\div5=28}}</math> | ||
| + | |style="text-align:right;"|ואחר שאלו הק"מ שהם החשבון אש' רצינו לחלק יש לה כל אלו המורים נשים אשר נחפוץ אחרון ונחלקנו עליו המשל על הה' <sup>ויצא</sup> <s>ושבא</s> בחילוק כ"ח ולא נשאר דבר | ||
| + | |- | ||
| + | | | ||
| + | :*Since it has a quarter, we divide it by 4 and place it before the last. The result of division is 7. | ||
| + | ::<math>\scriptstyle{\color{blue}{28\div4=7}}</math> | ||
| + | |style="text-align:right;"|ואחר שיש להם רביעית נחלקם על הד' ונשימנו לפני האחרון ויצא בחילוק ז‫' | ||
| + | |- | ||
| + | | | ||
| + | :*Since it is greater than the remaining divisor, we divide it by it; the result of division is 1, which is an integer, as the divisors are gone, we write it aside and beneath it we write the remaining 2. Like this: | ||
::<math>\scriptstyle{\color{blue}{7\div5=1+\frac{2}{5}}}</math> | ::<math>\scriptstyle{\color{blue}{7\div5=1+\frac{2}{5}}}</math> | ||
| − | |style="text-align:right;"|ואחר שהם כמורה הנשאר וגדול | + | |style="text-align:right;"|ואחר שהם כמורה הנשאר וגדול מ<s>ו</s>מנו נחלקם עליו ויצא בחילוק א' שהוא א' שלם כי כבר שלמו המורים ונשימנו מחוץ והב' הנשארים נשימם תחתיו כזה |
|- | |- | ||
| | | | ||
| Line 3,191: | Line 3,657: | ||
|- | |- | ||
| | | | ||
| + | :Hence, when we divide 140 by 100, each one of the one-hundred is 140 parts of one-hundred, which are 140-fifths of a quarter of a fifth, which are 28-quarters of a fifth, which are 7-fifths, which are 1 integer and 2-fifths. | ||
:<math>\scriptstyle{\color{blue}{140\div100=\frac{140}{5}\sdot\frac{1}{4}\sdot\frac{1}{5}=\frac{28}{4}\sdot\frac{1}{5}=\frac{7}{5}=1+\frac{2}{5}}}</math> | :<math>\scriptstyle{\color{blue}{140\div100=\frac{140}{5}\sdot\frac{1}{4}\sdot\frac{1}{5}=\frac{28}{4}\sdot\frac{1}{5}=\frac{7}{5}=1+\frac{2}{5}}}</math> | ||
| − | |style="text-align:right;"|הרי לנו כי כאשר חלקנו ק"מ על ק' | + | |style="text-align:right;"|הרי לנו כי כאשר חלקנו ק"מ על ק' שעולה לכל 1 ואחד מהמאה ק"מ חלקים ממאה שבשלם שהם ק"מ חמישיות רביעית חמישית שהם כ"ח רביעיות חמישיות שהם ז' חמישיות שלמות שהם א' שלם וב' חמישיות |
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{140\ | + | :As this dividend is double the former dividend, so the result of division, which is 1 integer and 2-fifths, which are 7-fifths, is double the former result of division, which is 3-fifths, 2-quarters of a fifth and half a fifth. |
| − | |style="text-align:right;"|וכמו שזה החשבון המתחלק הזה כפל החשבון המתחלק ראשונה | + | :<math>\scriptstyle{\color{blue}{140=2\sdot70}}</math> |
| + | :<math>\scriptstyle{\color{blue}{1+\frac{2}{5}=\frac{7}{5}=2\sdot\left[\frac{3}{5}+\left(\frac{2}{4}\sdot\frac{1}{5}\right)\right]=2\sdot\left[\frac{3}{5}+\left(\frac{1}{2}\sdot\frac{1}{5}\right)\right]}}</math> | ||
| + | |style="text-align:right;"|וכמו שזה החשבון המתחלק הזה כפל החשבון המתחלק ראשונה כן היוצא בחילוק שהוא א' שלם וב' חמישיות שהם ז' חמישיות הוא כפל היוצא בחילוק ראשונה שהיה ג' חמישיות וב' רביעיות חמישית פי' ג' ‫<ref>27r</ref>חמישיות <s>וב' רביעיות חמישית פי' שלשה חמישיות</s> וחצי חמישית | ||
| + | |- | ||
| + | |The whole procedure is clear and explained also by checking, which is to restore everything to its former state. | ||
| + | |style="text-align:right;"|הנה כל המעשה ברור ומבורר גם הבחינה והיא להשיב הכל לקדמותו | ||
|- | |- | ||
| | | | ||
| − | : | + | :Because, we decompose the one to fifths by multiplying it by 5, which is the denominator of the fifths; it is 5. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וזה כי האחד נשיבהו חמשיות שלמות וזה בכופלנו אותו בה' שהוא המורה על החמישיות ויהיו ה‫' |
| + | |- | ||
| + | | | ||
| + | :We add to it the 2 that is beneath it, which are also fifths. | ||
| + | |style="text-align:right;"|<s>ונחבר אליהם הב' הנמצא תחתיו שהם ג"כ חמישיות שלימות וזה בכפלנו אותו בה' שהוא המורה על החמישיות ויהיו ה'</s> ונחבר אליהם הב' הנמצא תחתיו שהם ג"כ חמשיות שלמות [יהיו כלם ז' חמישיות שלמות‫]‫<ref>marg.</ref> | ||
| + | |- | ||
| + | | | ||
| + | :When we want to decompose them to quarters of a fifth, we multiply them by 4; they are 28 quarters of a fifth, and since there is nothing beneath them, we do not add to them anything. | ||
| + | |style="text-align:right;"|וכאשר נרצה להשיבם רביעיות חמישית נכפלם בד' ויהיו כ"ח רביעיות חמישית ואחר שלא נמצא תחתיו דבר לא נחבר אליהם דבר | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{ | + | :We decompose them to fifths of a quarter of a fifth by multiplying them by 5; the result is 140 fifths of a quarter of a fifth. The divisors are all gone and there is nothing beneath them to add to the result. |
| − | | | + | |style="text-align:right;"|עוד נשיבם חמישיות רביעית חמישית וזה בכפלנו אותו בה' ויעלה ק"מ חמישיות רביעית חמישית והנה כלו המורים ואין תחתיו דבר לחבר על העולה |
| − | + | |} | |
| − | + | :<math>\scriptstyle{\color{blue}{1+\frac{2}{5}=\frac{\left(1\sdot5\right)+2}{5}=\frac{7}{5}=\frac{7\sdot4}{4}\sdot\frac{1}{5}=\frac{28}{4}\sdot\frac{1}{5}=\frac{28\sdot5}{5}\sdot\frac{1}{4}\sdot\frac{1}{5}=\frac{140}{5}\sdot\frac{1}{4}\sdot\frac{1}{5}=140\div100}}</math> | |
| + | {| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ויצא לנו כחשבוננו הראשון שוה בשוה וב[דרך זה] תעשה כפל המספרי' הנשארים | + | :We get the same result as our original number. |
| + | |style="width: 45%; text-align:right;"|ויצא לנו כחשבוננו הראשון שוה בשוה | ||
| + | |- | ||
| + | |Multiply the rest of the numbers by this method in the procedure or in the check. | ||
| + | |style="text-align:right;"|וב[דרך זה] תעשה כפל המספרי' הנשארים הן למעשה הן לבחינה | ||
|- | |- | ||
| | | | ||
| Line 3,219: | Line 3,704: | ||
== Chapter Five: <span style="color:Green>Proportions</span> == | == Chapter Five: <span style="color:Green>Proportions</span> == | ||
| − | + | |style="width:45%; text-align:right;"|<big>הפרק הה‫'</big> | |
|- | |- | ||
| | | | ||
| Line 3,225: | Line 3,710: | ||
| | | | ||
|- | |- | ||
| − | |For the ratio that a known number | + | |For the ratio that a known number is to [a known number], if you want to know for another known number, to which number it has this same ratio: |
:<math>\scriptstyle{\color{OliveGreen}{a_1:a_2=a_3:a_4}}</math> | :<math>\scriptstyle{\color{OliveGreen}{a_1:a_2=a_3:a_4}}</math> | ||
|style="text-align:right;"|אם תרצה לדעת הערך שיש למספר ידוע למספר ידוע אחר אצל איזה מספר יש לו אותו הערך בעצמו | |style="text-align:right;"|אם תרצה לדעת הערך שיש למספר ידוע למספר ידוע אחר אצל איזה מספר יש לו אותו הערך בעצמו | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|<s><big>המשל</big> הערך לה' אצל איזה מספר יש לו אותו הערך בעצמו</s> | |
| − | |||
| − | |style="text-align:right;"|המשל הערך לה' אצל איזה מספר יש לו אותו הערך בעצמו | ||
|- | |- | ||
| | | | ||
| − | :*Example: For the ratio that 5 is to 7, 10 | + | :*{{#annot:5÷7=10÷X|567|rfV6}}Example: For the ratio that 5 is to 7, 10 has the same ratio to which number? |
::<math>\scriptstyle{\color{blue}{5:7=10:x}}</math> | ::<math>\scriptstyle{\color{blue}{5:7=10:x}}</math> | ||
| − | |style="text-align:right;"|המשל הערך שיש לה' אצל ז' אצל מי יש לי' זה הערך | + | |style="text-align:right;"|המשל הערך שיש לה' אצל ז' אצל מי יש לי' זה הערך{{#annotend:rfV6}} |
|- | |- | ||
| | | | ||
| Line 3,261: | Line 3,744: | ||
|- | |- | ||
|This is the rule: the related of those that are first and of those that are last is called first, and that to which it is related, is called second. | |This is the rule: the related of those that are first and of those that are last is called first, and that to which it is related, is called second. | ||
| − | |style="text-align:right;"|זה הכלל כי לנערך הן מן הראשונים הן מן האחרונים נקרא ראשון ולאשר מעריך אצלו נקרא שני | + | |style="text-align:right;"|זה הכלל כי לנערך ‫<ref>27v</ref>הן מן הראשונים הן מן האחרונים נקרא ראשון ולאשר מעריך אצלו נקרא שני |
|- | |- | ||
| | | | ||
| Line 3,270: | Line 3,753: | ||
:*If you have the first of the two that are last, but the second of those that are last is unknown: we multiply the first [of those that are last] by the second of the first two that are known, then divide by that which remains of the three that are known, i.e. by the first of those that are first; the result of division is the unknown. | :*If you have the first of the two that are last, but the second of those that are last is unknown: we multiply the first [of those that are last] by the second of the first two that are known, then divide by that which remains of the three that are known, i.e. by the first of those that are first; the result of division is the unknown. | ||
::<math>\scriptstyle{\color{OliveGreen}{a_4=\frac{a_2\sdot a_3}{a_1}}}</math> | ::<math>\scriptstyle{\color{OliveGreen}{a_4=\frac{a_2\sdot a_3}{a_1}}}</math> | ||
| − | |style="text-align:right;"|אם יש בידך הראשון מן השנים האחרונים ונעלם השני שבאחרונים נכפול אותו הראשון [בשני] מן השנים הראשונים הידועים וחלקנו לנשאר מהג' הידועים ר"ל לראשון שבראשונים והיוצא בחילוק הוא הנעלם | + | |style="text-align:right;"|אם יש בידך הראשון מן השנים האחרונים ונעלם השני שבאחרונים נכפול אותו הראשון [בשני]‫<ref>marg.</ref> מן השנים הראשונים הידועים וחלקנו לנשאר מהג' הידועים ר"ל לראשון שבראשונים והיוצא בחילוק הוא הנעלם |
|- | |- | ||
| | | | ||
| Line 3,278: | Line 3,761: | ||
|- | |- | ||
| | | | ||
| + | ::When you divide the product by the second of those that are first, or by the first [of those that are second], if you want, extract the divisors, if this first or second, by which you divide, is a large number. The result, whether integers or fractions, is the required unknown. | ||
|style="text-align:right;"|וכאשר תחלק העולה על השני שבראשונים או על הראשון אם תרצה תוציא המורים אם זה הראשון או השני אשר תחלק עליו הוא חשבון גדול והיוצא בין שלמים ושברים הוא המבוקש הנעלם | |style="text-align:right;"|וכאשר תחלק העולה על השני שבראשונים או על הראשון אם תרצה תוציא המורים אם זה הראשון או השני אשר תחלק עליו הוא חשבון גדול והיוצא בין שלמים ושברים הוא המבוקש הנעלם | ||
|- | |- | ||
|This is the rule: always multiply the first of these by the second of the others and divide the product by what remains of those that are known; the result of division is the unknown. | |This is the rule: always multiply the first of these by the second of the others and divide the product by what remains of those that are known; the result of division is the unknown. | ||
| − | |style="text-align:right;"|זה הכלל לעולם תכפול הראשון מאלו בשני מאלו והעולה תחלק על הנשאר מהידועים והיוצא בחילוק הוא הנעלם | + | |style="text-align:right;"|זה הכלל לעולם תכפול הראשון מאלו בשני מאלו והעולה תחלק על [הנשאר] מהידועים והיוצא בחילוק הוא הנעלם |
|- | |- | ||
| | | | ||
| − | :*Example: if we say: the ratio | + | :*{{#annot:3÷7=5÷X|567|Mvdt}}Example: if we say: the ratio that 3 is to 7 - to whom does 5 have this ratio? |
::<math>\scriptstyle{\color{blue}{3:7=5:x}}</math> | ::<math>\scriptstyle{\color{blue}{3:7=5:x}}</math> | ||
| − | |style="text-align:right;"|המשל אם אמרנו הערך שיש לג' אצל הז' לה' אצל מי יש לו זה הערך בעצמו | + | |style="text-align:right;"|<big>המשל</big> אם אמרנו הערך שיש לג' אצל הז' לה' אצל מי יש לו זה הערך בעצמו{{#annotend:Mvdt}} |
|- | |- | ||
| | | | ||
| + | ::We write them as follows: | ||
|style="text-align:right;"|ונשימם לו על זה כזה | |style="text-align:right;"|ונשימם לו על זה כזה | ||
|- | |- | ||
| Line 3,303: | Line 3,788: | ||
::We know the related of those that are last, which is called first, it is 5. We multiply it by the second of those that are first, which is 7; the result is 35. We divide it by what remains of those that are known, which is 3; the result of division is 11 integers and 2-thirds and this is the required unknown. | ::We know the related of those that are last, which is called first, it is 5. We multiply it by the second of those that are first, which is 7; the result is 35. We divide it by what remains of those that are known, which is 3; the result of division is 11 integers and 2-thirds and this is the required unknown. | ||
::<math>\scriptstyle{\color{blue}{x=\frac{5\sdot7}{3}=\frac{35}{3}=11+\frac{2}{3}}}</math> | ::<math>\scriptstyle{\color{blue}{x=\frac{5\sdot7}{3}=\frac{35}{3}=11+\frac{2}{3}}}</math> | ||
| − | |style="text-align:right;"|הנה ידענו הנערך שבאחרונים והוא הנקרא ראשון והוא הה' ונכפלנו בשני שבראשונים והוא הז' ויעלו ל"ה ונחלקנו לנשאר מהידועים והוא הג' ויצא בחילוק י"א שלמים [וב' שלישיות וזהו הנעלם המבוקש | + | |style="text-align:right;"|הנה ידענו הנערך שבאחרונים והוא הנקרא ראשון והוא הה' ונכפלנו בשני שבראשונים והוא הז' ויעלו ל"ה ונחלקנו לנשאר מהידועים והוא הג' ויצא בחילוק י"א שלמים ‫[וב' שלישיות וזהו הנעלם המבוקש |
|- | |- | ||
| | | | ||
| − | ::Meaning: the ratio that 3 is to 7 is | + | ::Meaning: the ratio that 3 is to 7 is this same ratio that 5 is to 11 and 2-thirds. |
::<math>\scriptstyle{\color{blue}{3:7=5:\left(11+\frac{2}{3}\right)}}</math> | ::<math>\scriptstyle{\color{blue}{3:7=5:\left(11+\frac{2}{3}\right)}}</math> | ||
| − | |style="text-align:right;"|פי' כי הערך אשר לג' אצל הז' הוא הערך בעצמו אשר לה' אצל י"א וב' שלישיות] וב' שלישיות | + | |style="text-align:right;"|פי' כי הערך אשר לג' אצל הז' הוא הערך בעצמו אשר לה' אצל י"א וב' שלישיות‫]‫<ref>marg.</ref> וב' שלישיות |
|- | |- | ||
| | | | ||
| Line 3,318: | Line 3,803: | ||
|- | |- | ||
| | | | ||
| − | :*<math>\scriptstyle3:7=x:\left(11+\frac{2}{3}\right)</math> | + | :*{{#annot:3÷7=X÷11⅔|567|SYHL}}If it is said: the ratio that 3 is to 7 - to 11 and 2-thirds who has this ratio? |
| − | |style="text-align:right;"|ואם אמרו הערך אשר לג' אצל ז' אצל י"א וב' שלישיות למי יש לו זה הערך | + | ::<math>\scriptstyle3:7=x:\left(11+\frac{2}{3}\right)</math> |
| + | |style="text-align:right;"|ואם אמרו הערך אשר לג' אצל ז' אצל י"א וב' שלישיות למי יש לו זה הערך{{#annotend:SYHL}} | ||
|- | |- | ||
| | | | ||
| + | ::We write them in this diagram: | ||
|style="text-align:right;"|נשימם בצורה הזאת | |style="text-align:right;"|נשימם בצורה הזאת | ||
|- | |- | ||
| Line 3,336: | Line 3,823: | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{x=\frac{\left(11+\frac{2}{3}\right)\sdot3}{7}=\frac{35}{7}=5}}</math> | + | ::The unknown is the related term that is first of the latter and the known of them is 11 and 2-thirds, which is the second of them. So we multiply it by the first of the formers, which is 3; the result is 35. We divide it by the remaining of the knowns, which is 7; the result of division [is 5]. |
| − | |style="text-align:right;"|הנה הנעלם הוא הנערך שהוא הראשון שבאחרונים והידוע שבהם הי"א וב' שלישיות והוא השני שבהם לכן נכפלנו בראשון שבראשונים שהוא הג' ויעלה ל"ה ונחלקם לנשאר מהידועי' והוא הז' ויצא בחלוקה | + | ::<math>\scriptstyle{\color{blue}{x=\frac{\left(11+\frac{2}{3}\right)\sdot3}{7}=\frac{35}{7}=5}}</math> |
| + | |style="text-align:right;"|‫<ref>28r</ref>הנה הנעלם הוא הנערך שהוא הראשון שבאחרונים והידוע שבהם הי"א וב' שלישיות והוא השני שבהם לכן נכפלנו בראשון שבראשונים שהוא הג' ויעלה ל"ה ונחלקם לנשאר מהידועי' והוא הז' ויצא בחלוקה | ||
| + | |- | ||
| + | !<span style="color:Green>Written calculation</span> | ||
| + | | | ||
|- | |- | ||
| − | | | + | |This is the rule: we call them by their names and arrange each type beneath its own type, then we multiply each type by the one that is not of its type, which are the diagonals, and divide by its type. The result of division is the required unknown. |
|style="text-align:right;"|זה הכלל נקרא להם שם ונסדרם מין תחת מינו ונכפלם מין בשאינו מינו שהם האלכסונים ונחלקנו למינו היוצא בחילוק הוא הנעלם המבוקש | |style="text-align:right;"|זה הכלל נקרא להם שם ונסדרם מין תחת מינו ונכפלם מין בשאינו מינו שהם האלכסונים ונחלקנו למינו היוצא בחילוק הוא הנעלם המבוקש | ||
|- | |- | ||
| + | |? | ||
| + | |style="text-align:right;"|ואם הם בעצמם היה להם שינוי בשמות אשר בהם נודע איזה מינו או שאינו מינו לא נצטרך לקרוא להם שם חדש | ||
| + | |- | ||
| + | !<span style="color:Green>Exchange Problem - Currencies</span> | ||
| | | | ||
| − | |||
|- | |- | ||
| | | | ||
| − | * | + | *{{#annot:two currencies|632|R34u}}Example: If 3 gold dinar are worth 50 silver dinar, how many silver dinar will 11 gold dinar be worth? |
:<math>\scriptstyle3:50=11:x</math> | :<math>\scriptstyle3:50=11:x</math> | ||
| − | |style="text-align:right;"|המשל אם ג' דינרי זהב שוים נ' דינרי כסף י"א דינרי זהב כמה דינרי כסף שוים | + | |style="text-align:right;"|<big>המשל</big> אם ג' דינרי זהב שוים נ' דינרי כסף י"א דינרי זהב כמה דינרי כסף שוים{{#annotend:R34u}} |
|- | |- | ||
| | | | ||
| + | ::We arrange them, each type beneath its own type, the gold beneath the gold, as follows: | ||
|style="text-align:right;"|נסדרם מין תחת מינו הזהב תחת הזהב כזה | |style="text-align:right;"|נסדרם מין תחת מינו הזהב תחת הזהב כזה | ||
|- | |- | ||
| Line 3,363: | Line 3,858: | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{x=\frac{11\sdot50}{3}=\frac{550}{3}=183+\frac{1}{3}}}</math> | + | ::We multiply each type by the one that is not of its type, which are the gold and the silver, i.e. the diagonals, 11 by 50; the product is 550. We divide it by 3 that is of the gold type; the result of division is 183 and one-third that are the unknown silver dinar. |
| + | ::<math>\scriptstyle{\color{blue}{x=\frac{11\sdot50}{3}=\frac{550}{3}=183+\frac{1}{3}}}</math> | ||
|style="text-align:right;"|ונכפול למין בשאינו מינו שהם הזהב והכסף והם הי"א בנ' שהם האלכסונים ויעלה 550 ונחלקם על מינו פי' על ג' של זהב ויצא בחילוק 183 ושליש שהם הדינרי כסף הנעלמים | |style="text-align:right;"|ונכפול למין בשאינו מינו שהם הזהב והכסף והם הי"א בנ' שהם האלכסונים ויעלה 550 ונחלקם על מינו פי' על ג' של זהב ויצא בחילוק 183 ושליש שהם הדינרי כסף הנעלמים | ||
|- | |- | ||
| | | | ||
| + | ::We receive that if 3 gold dinar are worth 50 silver [dinar], then 11 gold dinar are worth 183 silver dinar and one-third of a dinar, like this: | ||
::<math>\scriptstyle{\color{blue}{3:50=11:\left(183+\frac{1}{3}\right)}}</math> | ::<math>\scriptstyle{\color{blue}{3:50=11:\left(183+\frac{1}{3}\right)}}</math> | ||
|style="text-align:right;"|הרי לנו שאם שלשה דינרי זהב שוים נ' של כסף י"א דינרי זהב שוים 183 דינרי כסף ועוד שליש דינר כזה | |style="text-align:right;"|הרי לנו שאם שלשה דינרי זהב שוים נ' של כסף י"א דינרי זהב שוים 183 דינרי כסף ועוד שליש דינר כזה | ||
| Line 3,381: | Line 3,878: | ||
|} | |} | ||
|- | |- | ||
| + | !<span style="color:Green>Exchange Problem - Currencies:</span> | ||
| | | | ||
| − | + | |- | |
| + | |If the question is vice versa, that the gold is unknown to us, as our saying: | ||
| + | |style="text-align:right;"|<big>ואם השאלה</big> היתה לה<sup>פ</sup>ך שנעלם לנו הזהב כאומרנו | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:two currencies|632|VOY0}}If 3 gold dinar are worth 50 silver dinar, how many [gold dinar] will 183⅓ silver dinar be worth? | ||
:<math>\scriptstyle3:50=x:\left(183+\frac{1}{3}\right)</math> | :<math>\scriptstyle3:50=x:\left(183+\frac{1}{3}\right)</math> | ||
| − | |style="text-align:right;"|ואם | + | |style="text-align:right;"|ואם <sup>ג'</sup> דינרי זהב שוים נ' של כסף 183 דינרי כסף ושליש דינר כמה שוים{{#annotend:VOY0}} |
|- | |- | ||
| | | | ||
| + | ::We write them each type above its own type, as follows: | ||
|style="text-align:right;"|נשימם מין על מינו כזה | |style="text-align:right;"|נשימם מין על מינו כזה | ||
|- | |- | ||
| Line 3,399: | Line 3,903: | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{x=\frac{\left(183+\frac{1}{3}\right)\sdot3}{50}=\frac{550}{50}=11}}</math> | + | ::We multiply each type by the one that is not of its type, meaning the silver and the gold that are the diagonals; the product is 550. We divide it by the remaining of the silver type, which is 50; the result of division is 11 and they are the unknown gold dinar. |
| − | |style="text-align:right;"|ונכפול מין בשאינו מינו פי' הכסף בזהב שהם האלכסונים ויעלו 550 ונחלקם על מינו שהוא הכסף הנשאר והוא הנ' ויצא בחילוק י"א והם דינרי זהב הנעלמים | + | ::<math>\scriptstyle{\color{blue}{x=\frac{\left(183+\frac{1}{3}\right)\sdot3}{50}=\frac{550}{50}=11}}</math> |
| + | |style="text-align:right;"|ונכפול מין בשאינו מינו פי' הכסף בזהב שהם האלכסונים ויעלו 550 ונחלקם על מינו שהוא הכסף הנשאר והוא הנ' ויצא בחילוק י"א והם דינרי זהב הנעלמים | ||
|- | |- | ||
| − | | | + | |It all comes down to the same thing. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והכל עולה לענין אחד |
|- | |- | ||
| | | | ||
| − | :< | + | ==== <span style="color:Green>Reasons</span> ==== |
| − | |style="text-align:right;"| | + | |
| + | | | ||
| + | |- | ||
| + | !<span style="color:Green>The reason for the solution of the first example:</span> | ||
| + | | | ||
| + | |- | ||
| + | |The reason is that when we say: the ratio that 3 has to 7 - to whom does 5 have this ratio? | ||
| + | :<math>\scriptstyle3:7=5:x</math> | ||
| + | |style="text-align:right;"|‫<ref>28v</ref><big>הטעם</big> כי כאשר אמרנו הערך שיש לג' אצל ז' לה' אצל מי שיש לו זה הערך | ||
|- | |- | ||
| − | | | + | |We know that as the ratio that 3 is to 7, one, which is a third of 3, is to a third of 7, meaning that if 3 is, for instance, a third of 7, then one is a third of a third of 7, and this is clear. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הנה ידענו שהערך שיש לג' אצל ז' יש לאחד שהוא שליש הג' אצל שליש ‫[הז' פי' שאם הג' על דרך משל שליש הז' הנה האחד הוא שליש שלישית הז' וזה ברור |
|- | |- | ||
| − | | | + | |Since we know that the ratio that 1 is to a third of 7 is as the ratio that 3 is to 7, which is the required ratio, and that a third of 7 is 7-thirds, then we know that 5 has that same ratio to 5 times 7-thirds. |
| − | :<math>\scriptstyle{\color{blue}{3:7=\left(\frac{1}{3}\sdot3\right):\left(\frac{1}{3}\sdot7\right)=1: | + | :<math>\scriptstyle{\color{blue}{3:7=\left(\frac{1}{3}\sdot3\right):\left(\frac{1}{3}\sdot7\right)=1:\left(\frac{1}{3}\sdot7\right)=1:\frac{7}{3}=5:\left(\frac{7}{3}\sdot5\right)=5:\frac{5\sdot7}{3}}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואחר שידענו שערך א' אצל שליש ז' הוא‫]‫<ref>marg.</ref> ז' הוא כערך ג' אצל ז' שהוא הערך הנשאל ושליש ז' הוא ז' שלישיות הנה ידענו שזה הערך בעצמו יש לה' אצל ה' פעמים ז' שלישיות |
|- | |- | ||
| − | | | + | |To know how many thirds they are, we have to multiply 5 by 7 and the result are thirds |
| − | + | |style="text-align:right;"|ולדעת כמה שלישיות הם יש לנו לכפול ה' בז' והעולה הם שלישיות | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |To know how many integers they are, we divide them by 3. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ולדעת כמה שלמים הם חלקנום על הג‫' |
|- | |- | ||
| + | !<span style="color:Green>The reason for the solution of the second example:</span> | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |Likewise in the second example: we know the ratio that 3 is to 7 and we wish to know who has this same ratio to 11 and 2-thirds. |
| − | :<math>\ | + | :<math>\scriptstyle3:7=x:\left(11+\frac{2}{3}\right)</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכן בדמיון השני כי אחר שידענו ערך ג' אצל ז' ורצינו לידע למי יש לו זה הערך בעצמו אצל י"א וב' שלישיות |
|- | |- | ||
| − | | | + | |It is as if we know the ratio that 7 is to 3 and we wish to know to whom does 11 and 2-thirds has this ratio. |
| − | + | :<math>\scriptstyle7:3=\left(11+\frac{2}{3}\right):x</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הרי הוא כאלו ידענו ערך ז' אצל ג' ונרצה לידע לי"א וב' שלישיות אצל מי יש לו זה הערך |
|- | |- | ||
| − | | | + | |The reason is clear, because it becomes as the first example it self. |
| − | + | |style="text-align:right;"|והנה הטעם ברור שהרי שב כדמיון הראשון בעינו | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |In order to elaborate the explanation I will explain from the beginning: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אכן כדי להרחיב ביאור אבארנו בעודו בעינו |
|- | |- | ||
| − | | | + | |I say that we know that the ratio that 3 is to 7 is the same ratio that one, which is a third of 3, is to 7-thirds, which are a third of 7, as we explained. |
| − | :<math>\scriptstyle{\color{blue}{3:7= | + | :<math>\scriptstyle{\color{blue}{3:7=\left(\frac{1}{3}\sdot3\right):\left(\frac{1}{3}\sdot7\right)=1:\frac{7}{3}}}</math> |
| − | |style="text-align:right;"|כי | + | |style="text-align:right;"|ואומר כי אחר שידענו שהערך שיש לג' אצל ז' הוא הערך בעצמו שיש לאחד שהוא שליש הג' אצל ז' שלישיות שהם שליש הז' כאשר ביארנו |
|- | |- | ||
| − | | | + | |Hence, for every seven-thirds that are in 11 and 2-thirds, one is the related and as the number of 7-thirds that are in them, so is the number of units of the unknown that is related to them. |
| − | + | |style="text-align:right;"|א"כ לכל שבעה שלישיות אשר בי"א וב' שלישיות הנערך אליהם הוא א' וכמספר כמה <sup>ז'</sup> שלישיות יש בהם כך הוא המספר אחדי הנערך אליהם הנעלם | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |To know how many whole 7-thirds there are in 11 and 2-thirds, we should know first how many thirds are they and this is known by multiplying them by 3. We multiply them by 3; the result is 35, so we know that there are 35 thirds in them. |
| − | + | |style="text-align:right;"|ולדעת כמה שלמים ז' שלישיות יש בי"א וב' שלישיות נדע תחלה כמה שלישיות הוא <sup>וזה</sup> יודע בכפלהו אותם בג' לכן כפלנום בג' ועלה ל"ה הנה ידענו שיש בהם [ל"ה‫]‫<ref>marg.</ref> שלישיות | |
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | |To know how many times 7-thirds are in them, we divide them by 7; we get 5 and this is the number of times that 7-thirds are in 11 and 2-thirds. |
| − | :<math>\scriptstyle{\color{ | + | :<math>\scriptstyle{\color{blue}{\left(11+\frac{2}{3}\right):\frac{7}{3}=\frac{\left(11+\frac{2}{3}\right)\sdot3}{7}=\frac{35}{7}=5}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ולדעת כמה פעמים יש בהם ז' שלישיות חלקנום על ז' ויצא לנו ה' והוא המספר הפעמים אשר יש ז' שלישיות בי"א וב' שלישיות |
|- | |- | ||
| − | |We | + | |We already know that 1 integer is the related to every 7-thirds, so the related to 5 times 7-thirds is 5 integers. |
| − | + | |style="text-align:right;"|וכבר ידענו שהנערך אצל כל ז' שלישיות הוא א' שלם א"כ הנערך אצל ה' פעמים ז' שלישיות הוא ה' שלמים | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |But, we know that 11 and 2-thirds is 5 times 7-thirds, so the related to them is 5 integers. |
| − | :<math>\scriptstyle{\color{ | + | :<math>\scriptstyle{\color{blue}{3:7=1:\frac{7}{3}=5:\left(\frac{7}{3}\sdot5\right)=5:\left(11+\frac{2}{3}\right)}}</math> |
| − | |style="text-align:right;"|ואולם | + | |style="text-align:right;"|ואולם ידענו שהי"א וב' שלישיות הוא ה' ‫<ref>29r</ref>פעמים ז' שלישיות א"כ הנערך אליהם הוא ה' שלמים |
|- | |- | ||
| + | !<span style="color:Green>The reasons for the solutions of the exchange problems:</span> | ||
| | | | ||
| − | *When the mean and one of the others are known, the unknown is extracted, for we multiply the mean by itself , then divide [the product] by the other that is known and the result is the unknown. | + | |- |
| − | :*<math>\scriptstyle{\color{ | + | |In the examples of the dinar: |
| − | :*<math>\scriptstyle{\color{ | + | |style="text-align:right;"|<big>ועוד</big> במשלי הדינרים |
| − | |style="text-align:right;"|ולזה בהודע האמצעי ואחד מן האחרים יודע הנעלם כי נכפול האמצעי בעצמו ונחלקנו לאחר הנודע ויצא הנעלם | + | |- |
| + | | | ||
| + | *We know that 3 gold dinar are worth 50 silver [dinar]. | ||
| + | :<math>\scriptstyle3:50=11:x</math> | ||
| + | |style="text-align:right;"|כי כאשר ידענו שג' דינרי זהב שוים נ' של כסף | ||
| + | |- | ||
| + | |It is known that one gold dinar is worth one-third of 50 silver dinar, which is fifty-thirds of a dinar. | ||
| + | |style="text-align:right;"|נודע שדינר זהב אחד שהוא שוה שליש נ' דינרים של כסף שהוא חמישים שלישי דינר | ||
| + | |- | ||
| + | |It is known from this that 11 gold dinar are worth 11 times 50-thirds of a silver dinar. | ||
| + | |style="text-align:right;"|ונודע מזה שהי"א דינרי זהב שוים י"א פעמים נ' שלישי דינר כסף | ||
| + | |- | ||
| + | |To know how many thirds they are, we multiply 11 by 50; the result is 550, so we know that 11 gold dinar are worth 550-thirds of a silver dinar. | ||
| + | |style="text-align:right;"|ולדעת כמה שלישים הם כפלנו הי"א בנ' ועלה 550 הנה ידענו שהי"א דינרי זהב שוים 550 שלישי דינר כסף | ||
| + | |- | ||
| + | |To know how many silver dinar they are, we divide them by 3; the result is 183 and one-third and they are the silver dinar that are worth 11 gold dinar and this is clear. | ||
| + | |style="text-align:right;"|ולדעת כמה דינרי כסף הם חלקנום על ג' ויצא 183 ושליש והם הדינרי כסף ששוים הי"א דינרי זהב וזה ברור | ||
| + | |} | ||
| + | :<math>\scriptstyle{\color{blue}{3:50=1:\left(\frac{1}{3}\sdot50\right)=1:\frac{50}{3}=11:\left(11\sdot\frac{50}{3}\right)=11:\frac{11\sdot50}{3}=11:\frac{550}{3}=11:\left(183+\frac{1}{3}\right)}}</math> | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | *I will explain it also in the second example: since we know that 3 gold dinar are worth 50 silver dinar, each gold dinar is worth 50-thirds of a silver dinar, as we explained. | ||
| + | :<math>\scriptstyle3:50=x:\left(183+\frac{1}{3}\right)</math> | ||
| + | |style="width:45%; text-align:right;"|<big>ועוד</big> אבארנו במשל השני והוא כי ביודעינו שג' דינרי זהב שוים נ' דינרי כסף כל דינרי זהב שוה נ' שלישי דינר כסף כמו שביארנו | ||
| + | |- | ||
| + | |Therefore, every 50-thirds of a dinar that is in 183 and one-third is worth one gold dinar. | ||
| + | |style="text-align:right;"|וא"כ כל נ' שלישי דינר אשר בק'פ'ג' ושליש שוה דינר זהב | ||
| + | |- | ||
| + | |To know how many 50-thirds of a dinar there are in them, we should know first how many thirds of a dinar they are and this is known by multiplying them by 3 as we did; the result is 550, which are thirds of a dinar. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(183+\frac{1}{3}\right):\frac{50}{3}=\frac{\left(183+\frac{1}{3}\right)\sdot3}{50}=\frac{550}{50}=11}}</math> | ||
| + | |style="text-align:right;"|ולדעת כמה פעמים יש בהם נ' שלישי דינר נדע תחלה כמה שלישי דינר הם וזה יודע בכפלנו אותם בג' כאש' עשינו ועלו 550 והם שלישי דינר | ||
| + | |- | ||
| + | |Every 50 of them are worth one gold dinar, so when we divide them by them, as we did, we know how many gold dinar they are worth and this is as the result of division, which is 11. All this is clear. | ||
| + | |style="text-align:right;"|וכל נ' מהם שוים דינר זהב א"כ בחלקנום אותם על נ' כאשר עשינו נדע כמה דינרי זהב שוים שהוא כמספר היוצא בחלוקו הוא י"א וכל זה ברור | ||
| + | |- | ||
| + | |It becomes clear from all that is mentioned with a little investigation that for every four proportional numbers, the product of the first of the formers by the second of the latter is as the product of the second of the formers by the first of the latter. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{a_1:a_2=a_3:a_4\longrightarrow a_1\sdot a_4=a_2\sdot a_3}}</math> | ||
| + | |style="text-align:right;"|והנה יתבאר מכל הנזכר במעט עיון כי כל ד' מספרים נערכים כפל הראשון מאלו בשני מן האחרים ככפל השני בראשון מן האחרים | ||
| + | |- | ||
| + | | | ||
| + | :*Because, in the first example, the product of 5 by 7, which is 35, is as the product of 3 by 11 and 2-thirds, which is the unknown. | ||
| + | ::<math>\scriptstyle{\color{blue}{3:7=5:\left(11+\frac{2}{3}\right)\longrightarrow5\sdot7=35=3\sdot\left(11+\frac{2}{3}\right)}}</math> | ||
| + | |style="text-align:right;"|כי בדמיון הראשון כפל הה' בז' שהוא ל"ה ככפל הג' בי"א וב' שלישיות אשר היה הנעלם | ||
| + | |- | ||
| + | |Therefore, when one of them is unknown, whichever it may be, we multiply the knowns, first of these by the second of those, and we know that it is itself the product of the unknown by the one that remains of the knowns. Therefore, when we divide it by the known, the result is the unknown. | ||
| + | |style="text-align:right;"|ולזה כאש' נעלם אחד מהם איזה מהם שיהיה כפלנו מהנודעים הראשון מאלו בשני מאלו וידענו שזה בעצמו הוא כפל הנעלם בנשאר מהנודעים ולזה בחלקנו אותו לנודע יצא הנעלם | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === <span style="color:Green>Proportional Triad</span> === | ||
| + | | | ||
| + | |- | ||
| + | |Sometimes the proportional numbers are only three, i.e. the mean is the first of those that are last and the second of those that are first. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{a_1:a_2=a_2:a_3}}</math> | ||
| + | |style="text-align:right;"|‫<ref>29v</ref><big>ולפעמים</big> לא יהיו המספרים הנערכים כי אם ג' פי' שהאמצעי יהיה ראשון לאחרונים ושני לראשונים | ||
| + | |- | ||
| + | |We have already explained that for every four proportional numbers the product of the first of those by the second of those is as the product of the first of those by the second of thos, i.e. the product of the first by the last is as the product of the two means by each other. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{a_1:a_2=a_3:a_4\longrightarrow a_1\sdot a_4=a_2\sdot a_3}}</math> | ||
| + | |style="text-align:right;"|ואולם כבר ביארנו שכל ד' מספרים נערכים כפל הראשון מאלו בשני מאלו <s>בשני מ </s>ככפל הראשון מאלו בשני מאלו פי' כפל הראשון באחרון ככפל הב' האמצעיים זה בזה | ||
| + | |- | ||
| + | |When they are only three, the mean stands instead of the two means, which are the second of those that are first and the first of those that are last, so the product of the first by the third, which is the second of those that are last, is as the product of the mean by itself, which is both first [of those that are last] and second [of those that are first], as we explained. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{a_1:a_2=a_2:a_3\longrightarrow a_1\sdot a_3=\left(a_2\right)^2}}</math> | ||
| + | |style="text-align:right;"|ואולם כשהם ג' לבד האמצעי עומד במקום השנים האמצעיי' שהוא שני לראשונים וראשון לאחרונים א"כ כפל הראשון בשלישי שהוא השני מהאחרונים ככפל האמצעי בעצמו שהוא ראשון ושני כאשר ביארנו | ||
| + | |- | ||
| + | | | ||
| + | *When the mean and one of the others are known, the unknown is extracted, for we multiply the mean by itself , then divide [the product] by the other that is known and the result is the unknown. | ||
| + | :*<math>\scriptstyle{\color{OliveGreen}{a_1=\frac{\left(a_2\right)^2}{a_3}}}</math> | ||
| + | :*<math>\scriptstyle{\color{OliveGreen}{a_3=\frac{\left(a_2\right)^2}{a_1}}}</math> | ||
| + | |style="text-align:right;"|ולזה בהודע האמצעי ואחד מן האחרים יודע הנעלם כי נכפול האמצעי בעצמו ונחלקנו לאחר הנודע ויצא הנעלם | ||
|- | |- | ||
| | | | ||
*When the two [extremes] are known, the mean is extracted, by multiplying the two that are known [one by the other] and the product is as the product of the mean by itself, i.e. as its square. | *When the two [extremes] are known, the mean is extracted, by multiplying the two that are known [one by the other] and the product is as the product of the mean by itself, i.e. as its square. | ||
| − | :*<math>\scriptstyle{\color{ | + | :*<math>\scriptstyle{\color{OliveGreen}{\left(a_2\right)^2=a_1\sdot a_3}}</math> |
|style="text-align:right;"|גם בהודע השנים יודע האמצעי וזה בהכפל השנים הנודעים והעולה הוא ככפל האמצעי בעצמו פי' שהוא כמרובע | |style="text-align:right;"|גם בהודע השנים יודע האמצעי וזה בהכפל השנים הנודעים והעולה הוא ככפל האמצעי בעצמו פי' שהוא כמרובע | ||
|- | |- | ||
| | | | ||
:The mean is the root - we extract the root of this number by finding a number whose product by itself is as this product and the resulting root is the unknown mean. | :The mean is the root - we extract the root of this number by finding a number whose product by itself is as this product and the resulting root is the unknown mean. | ||
| − | :*<math>\scriptstyle{\color{ | + | :*<math>\scriptstyle{\color{OliveGreen}{a_2=\sqrt{a_1\sdot a_3}}}</math> |
|style="text-align:right;"|והאמצעי הוא השרש ונוציא שורש זה המספר שהוא לבקש מספר שכפלו על עצמו עולה כפי החשבון והשרש אשר יצא הוא האמצעי הנעלם | |style="text-align:right;"|והאמצעי הוא השרש ונוציא שורש זה המספר שהוא לבקש מספר שכפלו על עצמו עולה כפי החשבון והשרש אשר יצא הוא האמצעי הנעלם | ||
|- | |- | ||
| Line 3,487: | Line 4,063: | ||
|- | |- | ||
|Example for the three proportional numbers: | |Example for the three proportional numbers: | ||
| − | |style="text-align:right;"|דמיון | + | |style="text-align:right;"|<big>דמיון</big> זה ג' מספרים נערכים |
|- | |- | ||
| | | | ||
| − | *As we say: the ratio of 2 to 4 is as the ratio of 4 to 8 | + | *{{#annot:2÷4=4÷8|567|wusI}}As we say: the ratio of 2 to 4 is as the ratio of 4 to 8 |
:<math>\scriptstyle{\color{blue}{2:4=4:8}}</math> | :<math>\scriptstyle{\color{blue}{2:4=4:8}}</math> | ||
| − | |style="text-align:right;"|הוא כאומרנו הערך אשר לב' אצל הד' כערך ד' אצל ח' | + | |style="text-align:right;"|הוא כאומרנו הערך אשר לב' אצל הד' כערך ד' אצל ח‫'{{#annotend:wusI}} |
|- | |- | ||
| | | | ||
| Line 3,501: | Line 4,077: | ||
:*If one of the extremes is unknown, such as 2, whereas 4 and 8 are known: | :*If one of the extremes is unknown, such as 2, whereas 4 and 8 are known: | ||
::<math>\scriptstyle{\color{blue}{x:4=4:8}}</math> | ::<math>\scriptstyle{\color{blue}{x:4=4:8}}</math> | ||
| − | |style="text-align:right;"|ואם הנעלם מהקצוות המשל הב' ונודע הד' והח' | + | |style="text-align:right;"|ואם הנעלם מהקצוות המשל הב' ונודע הד' והח‫' |
|- | |- | ||
| | | | ||
| Line 3,507: | Line 4,083: | ||
|style="text-align:right;"|כלומר ששאל השואל למי יש ערך אצל ד' כערך אשר לד' אצל שמונה | |style="text-align:right;"|כלומר ששאל השואל למי יש ערך אצל ד' כערך אשר לד' אצל שמונה | ||
|- | |- | ||
| + | !<span style="color:Green>Exchange Problem - Currencies:</span> | ||
| | | | ||
| − | ::* | + | |- |
| − | |style="text-align:right;"|או שאמ' כמה דינרי זהב שוים ד' דינרי כסף אם ד' דינרי זהב שוים אחד דינרי כסף | + | | |
| + | ::*{{#annot:two currencies|632|AGlb}}Or if he says: How many golden dinar are worth 4 silver dinar, if 4 golden dinar are worth [8] silver dinar? | ||
| + | |style="text-align:right;"|או שאמ' כמה דינרי זהב שוים ד' דינרי כסף אם ד' דינרי זהב שוים אחד דינרי כסף{{#annotend:AGlb}} | ||
|- | |- | ||
| | | | ||
::We know that the product of 4 by 4, which is the mean, that is 16 is as the product of the known 8 by the unknown | ::We know that the product of 4 by 4, which is the mean, that is 16 is as the product of the known 8 by the unknown | ||
::<math>\scriptstyle{\color{blue}{4\sdot4=16=8\sdot x}}</math> | ::<math>\scriptstyle{\color{blue}{4\sdot4=16=8\sdot x}}</math> | ||
| − | |style="text-align:right;"|הנה ידענו שכפל ד' בד' שהוא האמצעי שהוא שהם י"ו שהוא ככפל ח' הידוע בנעלם | + | |style="text-align:right;"|הנה ידענו שכפל ד' בד' שהוא האמצעי <s>שהוא</s> שהם י"ו שהוא ככפל <sup>ח'</sup> הידוע ‫<ref>30r</ref>בנעלם |
|- | |- | ||
| | | | ||
::Thus, we divide it by 8 and the result of division, which is 2, is the unknown. | ::Thus, we divide it by 8 and the result of division, which is 2, is the unknown. | ||
::<math>\scriptstyle{\color{blue}{x=\frac{16}{8}=2}}</math> | ::<math>\scriptstyle{\color{blue}{x=\frac{16}{8}=2}}</math> | ||
| − | |style="text-align:right;"|לכן נחלקם על הח' והיוצא והוצרך בחלוק והוא ב' הוא הנעלם | + | |style="text-align:right;"|לכן נחלקם על הח' <sup>והיוצא</sup> <s>והוצרך</s> בחלוק והוא ב' הוא הנעלם |
|- | |- | ||
| | | | ||
:*If 2 and 4 are known, and 8 is unknown: | :*If 2 and 4 are known, and 8 is unknown: | ||
::<math>\scriptstyle2:4=4:x</math> | ::<math>\scriptstyle2:4=4:x</math> | ||
| − | |style="text-align:right;"|וכן אם נודעו השנים והד' ונעלם הח' | + | |style="text-align:right;"|וכן אם נודעו השנים והד' ונעלם הח‫' |
|- | |- | ||
| | | | ||
| Line 3,530: | Line 4,109: | ||
|style="text-align:right;"|ששאל השואל הערך אשר לב' אצל ד' אצל מי יש לד' זה הערך | |style="text-align:right;"|ששאל השואל הערך אשר לב' אצל ד' אצל מי יש לד' זה הערך | ||
|- | |- | ||
| + | !<span style="color:Green>Exchange Problem - Currencies:</span> | ||
| | | | ||
| − | ::* | + | |- |
| − | |style="text-align:right;"|או ששאל אם שני דינרי זהב שוים ד' דינרי כסף ד' דינרי זהב כמה דינרי כסף שוים | + | | |
| + | ::*{{#annot:two currencies|632|eMkS}}Or, if he asks: If 2 golden dinar are worth 4 silver dinar, how many silver dinar will 4 golden dinar be worth? | ||
| + | |style="text-align:right;"|או ששאל אם שני דינרי זהב שוים ד' דינרי כסף ד' דינרי זהב כמה דינרי כסף שוים{{#annotend:eMkS}} | ||
|- | |- | ||
| | | | ||
::We multiply 4 by itself; the result is 16. We divide it by [2]; the result of division is [8] and this is the unknown. | ::We multiply 4 by itself; the result is 16. We divide it by [2]; the result of division is [8] and this is the unknown. | ||
::<math>\scriptstyle{\color{blue}{x=\frac{4\sdot4}{{\color{red}{2}}}=\frac{16}{{\color{red}{2}}}={\color{red}{8}}}}</math> | ::<math>\scriptstyle{\color{blue}{x=\frac{4\sdot4}{{\color{red}{2}}}=\frac{16}{{\color{red}{2}}}={\color{red}{8}}}}</math> | ||
| − | |style="text-align:right;"|נכפול הד' בעצמו ויעלה י"ו ונחלקם על | + | |style="text-align:right;"|נכפול הד' בעצמו ויעלה י"ו ונחלקם על הח' ויצא בחילוק ב' והוא הנעלם |
|- | |- | ||
| | | | ||
| Line 3,567: | Line 4,149: | ||
== Chapter Six: Roots == | == Chapter Six: Roots == | ||
| − | + | |style="text-align:right;"|<big>הפרק השישי</big> בהוצאת השרשים | |
|- | |- | ||
| | | | ||
| − | === written extraction of roots === | + | === <span style=color:Green>written extraction of roots</span> === |
| | | | ||
|- | |- | ||
| | | | ||
| − | ==== description of the procedure ==== | + | ==== <span style=color:Green>description of the procedure</span> ==== |
| | | | ||
|- | |- | ||
|When you wish to extract the root of a certain number, count the number of the ranks, whether it is even or odd. | |When you wish to extract the root of a certain number, count the number of the ranks, whether it is even or odd. | ||
| − | |style="text-align:right;"|כאשר תרצה להוציא שורש שום מספר תמנה מספר מעלות ההוא אם זוג ואם נפרד | + | |style="width:45%; text-align:right;"|כאשר תרצה להוציא שורש שום מספר תמנה מספר מעלות ההוא אם זוג ואם נפרד |
|- | |- | ||
| | | | ||
| Line 3,594: | Line 4,176: | ||
| | | | ||
:Then seek a number whose square is the same as these tens and units or as great as possible to be subtract from them and write this number you find beneath the rank that precedes the last rank. | :Then seek a number whose square is the same as these tens and units or as great as possible to be subtract from them and write this number you find beneath the rank that precedes the last rank. | ||
| − | |style="text-align:right;"|ותבקש מספר שיהיה מרובעו בכל אלו העשרות והאחדים אשר לקחת או היותר שתוכל להוציאו מהם וזה המספר אשר מצאת תשימהו תחת המעלה אשר לפני המעלה האחרונה | + | |style="text-align:right;"|ותבקש מספר שיהיה מרובעו בכל אלו העשרות והאחדים אשר לקחת או היותר שתוכל להוציאו מהם וזה המספר אשר מצאת תשימהו ‫<ref>30v</ref>תחת המעלה אשר לפני המעלה האחרונה |
|- | |- | ||
| | | | ||
| Line 3,635: | Line 4,217: | ||
| | | | ||
:Multiply [the number] by itself and subtract [the product] from the rank above it. | :Multiply [the number] by itself and subtract [the product] from the rank above it. | ||
| − | |style="text-align:right;"|ותכפלנו <s>המספרים הראשונים מלבד אשר שמת ראשון שעבר עליו הקולמוס</s> על עצמו ותוציאנו מהמעלה אשר על ראשו | + | |style="text-align:right;"|ותכפלנו <s>המספרים הראשונים מלבד אשר שמת ראשון שעבר עליו הקולמוס</s> על עצמו ותוציאנו ‫<ref>31r</ref>מהמעלה אשר על ראשו |
|- | |- | ||
| | | | ||
| Line 3,642: | Line 4,224: | ||
|- | |- | ||
| | | | ||
| − | * | + | *When you double the number and write its double in the preceding rank, if the digit that is above it is not enough to subtract [the double] even once, and that enough remains in the preceding rank to subtract from it the product of the other digit by itself, write 0 before it and lower it by one rank, whether there is a 0 there or any other digit. Then seek a number to write before it, so that you multiply it by each of the [digits of the root] and by itself and subtract every [product] from what is above it. |
|style="text-align:right;"|ואם כאשר כפלת המספר ושמת כפלו במעלה אשר לפניו אם אין ברשמים אשר עליהם כדי להוציאם אפי' פעם אחת ושישאר במעלה אשר לפניהם אחד להוציא ממנו כפל האחר בעצמו אז תשים 0 לפניהם ותורידם מעלה אחת גם ל0 גם לכל רושם מהם ותבקש מספר שתשים לפניהם ותכפלנו בכל אחד מהם ובעצמו ותוציא כל דבר מאשר על ראשו | |style="text-align:right;"|ואם כאשר כפלת המספר ושמת כפלו במעלה אשר לפניו אם אין ברשמים אשר עליהם כדי להוציאם אפי' פעם אחת ושישאר במעלה אשר לפניהם אחד להוציא ממנו כפל האחר בעצמו אז תשים 0 לפניהם ותורידם מעלה אחת גם ל0 גם לכל רושם מהם ותבקש מספר שתשים לפניהם ותכפלנו בכל אחד מהם ובעצמו ותוציא כל דבר מאשר על ראשו | ||
|- | |- | ||
| − | | | + | | |
| + | :Write the remainder above the digit that is above it and lower again the [digits of the root] by one rank, as long as you lower them, whenever you lower them, as they are, without doubling, except for the last digit that is generated at that same phase that you double it. | ||
|style="text-align:right;"|והנותר תשים על הרושם אשר על ראשו ותורידם עוד מעלה אחרת ובלבד שתורידם לעולם בכל הורדה שתורידם שיורדו כמות שהם בלי כפל כלל זולתי המספר האחרון שנתחדש בפעם ההיא שתכפלנו | |style="text-align:right;"|והנותר תשים על הרושם אשר על ראשו ותורידם עוד מעלה אחרת ובלבד שתורידם לעולם בכל הורדה שתורידם שיורדו כמות שהם בלי כפל כלל זולתי המספר האחרון שנתחדש בפעם ההיא שתכפלנו | ||
|- | |- | ||
| | | | ||
| − | * | + | *If no tens are generated [in double the digit of the root], write it in the rank that precedes the ranks in which you write the other digits as they are lowered. |
|style="text-align:right;"|ואם לא נתחדש שם עשר תשימנו במעלה שלפני המעלות אשר תשים הרשמי' האחרים בהורדתם | |style="text-align:right;"|ואם לא נתחדש שם עשר תשימנו במעלה שלפני המעלות אשר תשים הרשמי' האחרים בהורדתם | ||
|- | |- | ||
| | | | ||
| − | * | + | *If a ten is generated, add it to the digit that you write first to the right, and if this digit is only 0, write the 1, i.e. the ten instead of it. Write the units that are generated with the ten from this double, in the preceding rank. |
|style="text-align:right;"|ואם מהכפל ההוא יתחדש עשר תחברנו עם הרושם אשר שמת ראשון לצד ימין <s>ואם מהכפל ההוא יתחדש עשר תחברנו עם הרושם אשר שמת ראשון לצד ימין</s> ואם לא היה כי אם <sup>0</sup> תסירנה ותשים הא' ר"ל העשר במקומה והאחדים אשר נתחדשו מהכפל עם זה העשרה שימם במעלה שלפניהם | |style="text-align:right;"|ואם מהכפל ההוא יתחדש עשר תחברנו עם הרושם אשר שמת ראשון לצד ימין <s>ואם מהכפל ההוא יתחדש עשר תחברנו עם הרושם אשר שמת ראשון לצד ימין</s> ואם לא היה כי אם <sup>0</sup> תסירנה ותשים הא' ר"ל העשר במקומה והאחדים אשר נתחדשו מהכפל עם זה העשרה שימם במעלה שלפניהם | ||
|- | |- | ||
| | | | ||
| − | * | + | *If no tens are generated [in double the digit of the root], as when the last digit is a five and its double is a ten without units, write the ten as I said instead of the 0, or add it to what you find in the rank to the right, and since there are no units, write 0 before these ranks. |
|style="text-align:right;"|ואם לא נתחדשו שם אחדים כגון שהרושם האחרון היה חמשה וכפלו יהיה עשרה שלם בלתי אחדים תשים הי' כאשר אמרתי במקום ה0 או תחברנו עם אשר תמצא במעלה לצד ימין ואחר שאין אחדים שם תשים 0 לפני המעלות ההם | |style="text-align:right;"|ואם לא נתחדשו שם אחדים כגון שהרושם האחרון היה חמשה וכפלו יהיה עשרה שלם בלתי אחדים תשים הי' כאשר אמרתי במקום ה0 או תחברנו עם אשר תמצא במעלה לצד ימין ואחר שאין אחדים שם תשים 0 לפני המעלות ההם | ||
|- | |- | ||
| − | |Repeating the process | + | |<span style=color:Green>Repeating the process:</span> seek for another number as stated and proceed like this until their end. |
|style="text-align:right;"|ותבקש עוד מספר כמו שנזכר וכן תעשה עד תומם | |style="text-align:right;"|ותבקש עוד מספר כמו שנזכר וכן תעשה עד תומם | ||
|- | |- | ||
| − | |The | + | |The root consists of all the numbers you seek for in all the phases without their doubling. |
| − | |style="text-align:right;"|והשרש הוא כל המספרים אשר בקשת בכל עת בלי כפל | + | |style="text-align:right;"|‫<ref>31v</ref>והשרש הוא כל המספרים אשר בקשת בכל עת בלי כפל |
|- | |- | ||
| | | | ||
| − | ==== examples ==== | + | ==== <span style=color:Green>examples</span> ==== |
| | | | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle\sqrt{344680129066}</math> | + | *{{#annot:√344680129066|439|lfu5}}We wish to seek the root of 344680129066. |
| − | |style="text-align:right;"|<big>המשל</big> רצינו לבקש שרש 344680129066 | + | :<math>\scriptstyle\sqrt{344680129066}</math> |
| + | |style="text-align:right;"|<big>המשל</big> רצינו לבקש שרש 344680129066{{#annotend:lfu5}} | ||
|- | |- | ||
| | | | ||
| Line 3,706: | Line 4,290: | ||
|} | |} | ||
|} | |} | ||
| − | ::<span style=color: | + | ::<span style=color:Green>[Illustration of the procedure:]</span> |
::{| | ::{| | ||
|- | |- | ||
| Line 3,724: | Line 4,308: | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|והנה מעלות מספר זה הם י"ב והם זוג נקח השני רשמים האחרונים האחרון אחרון לעשרות ואשר לפניו לאחדים ויהיו 34 | + | :The ranks of this number are 12 and it is an even number. We take the two last digits - the last as tens and the one that precedes it as units; they are 34. |
| − | ונבקש מספר שנכפלנו על עצמו ויוציא כל ה34 או היותר שאפשר והוא ה' ונשימנו תחת הד' ונאמר ה' פעמים <sup>ה'</sup> הם כ"ה {{#annot:term|181,1232|F2P9}}נוציאם מ{{#annotend:F2P9}}הל"ד ישארו ט' ונעביר קולמוס על הג' ונשים הט' על הד‫' | + | |style="width:45%; text-align:right;"|והנה מעלות מספר זה הם י"ב והם זוג נקח השני רשמים האחרונים האחרון אחרון לעשרות ואשר לפניו לאחדים ויהיו 34 |
| − | ונכפול הה' ואחר שאין ונעביר עליו הקולמוס והנה כופלו הוא י' נשים א' תחת הה' ואחר שאין עם עשר זה אחדים כלל נשים לפני זה האחד 0 | + | |- |
| − | + | | | |
| + | :*We seek for a number, such that when we multiply it by itself, it will consume the whole 34, or as much as possible of it; it is 5. We write it beneath the 4. | ||
| + | |style="text-align:right;"|ונבקש מספר שנכפלנו על עצמו ויוציא כל ה34 או היותר שאפשר והוא ה' ונשימנו תחת הד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We say: 5 times 5 is 25. We subtract it from 34; 9 remains. | ||
| + | :::<math>\scriptstyle{\color{blue}{34-5^2=34-25=9}}</math> | ||
| + | |style="text-align:right;"|ונאמר ה' פעמים <sup>ה'</sup> הם כ"ה {{#annot:term|181,1232|F2P9}}נוציאם מ{{#annotend:F2P9}}הל"ד ישארו ט‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We cross the 3 with a pen and write 9 above the 4. | ||
| + | |style="text-align:right;"|ונעביר קולמוס על הג' ונשים הט' על הד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We double the 5 and cross it with a pen. Its double is 10. We write 1 beneath the 5 and since there are no units with the ten at all, we write 0 before the one. | ||
| + | |style="text-align:right;"|ונכפול הה' <s>ואחר שאין</s> ונעביר עליו הקולמוס והנה כופלו הוא י' נשים א' תחת הה' ואחר שאין עם עשר זה אחדים כלל נשים לפני זה האחד 0 | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*We seek for a number to write in the rank that precedes the 0, multiply by 1 and by itself, and to subtract as much as we can from what remains above the 4 and from the 48 before it that are the ranks that are above [this number]. It is 8. We write it before them. |
| + | |style="text-align:right;"|ונבקש מספר נשימהו במעלה שלפני ה0' ונכפלנו <s>ב</s> בא' ובעצמו ונוציא כל היותר שנוכל מאשר נשאר על הד' <sup>גם</sup> מהמ"ו אשר לפניו שהם <s>השלימות</s> המעלות אשר עליהן ויהיה ח' ונשימנו לפניהם | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ונוציא הד' האחדים מהו' אשר על ראשו ישארו ב' נשימם על הו‫'< | + | ::*We multiply 8 by 1; it is 8. We subtract it from the 9 that is above it; one remains. We write it above it. |
| − | והס' שהם ו' עשרות לא נוכל להוציאם מהד' שאחר הו' שהוא עשרות נגדו ונקרא הא' אשר אחריהם ונעביר עליו הקולמוס ויהיה עשר במעלת הד' הנזכר ונחבר אליהם הד' עצמו יהיו כלם י"ד {{#annot:term|181,1232|J2LS}}נוציא מהם{{#annotend:J2LS}} הס' אשר הם ו' עשרות נשארו ח' ונשימנו על הד‫'< | + | :::<math>\scriptstyle{\color{blue}{9-\left(1\times8\right)=9-8=1}}</math> |
| − | אחר זה נורידם מעלה אחת ונכפול הח' אשר נתחדש בפעם הזאת ואחר אשר נתחדש מכפלו אחדים ועשר לא נשים ה0' אבל נשים א' בעד העשר במקומה ונשים הו' אחדים לפניו | + | |style="text-align:right;"|ונכפול ח' בא' יהיו ח' נוציאם מהט' אשר עליו ישאר אחד נשימנו עליו |
| + | |- | ||
| + | | | ||
| + | ::*We also multiply 8 by itself; the result is 64. | ||
| + | :::<math>\scriptstyle{\color{blue}{8^2=64}}</math> | ||
| + | |style="text-align:right;"|עוד נכפול ח' על עצמו ויעלה ‫<ref>32r</ref>ס"ד | ||
| + | |- | ||
| + | | | ||
| + | :::We subtract the 4 units from the 6 that is above it; 2 remains. We write it above the 6. | ||
| + | :::<math>\scriptstyle{\color{blue}{6-4=2}}</math> | ||
| + | |style="text-align:right;"|ונוציא הד' האחדים מהו' אשר על ראשו ישארו ב' נשימם על הו‫' | ||
| + | |- | ||
| + | | | ||
| + | :::We cannot subtract the 6 tens from the 4 that is after the 6, which is tens with respect to it. We take the 1 that is after it and cross it with a pen; it becomes ten in the rank of the mentioned 4. We add to it the 4 itself; they are 14 all together. We subtract from it the 60, which are 6 tens; 8 remains. We write it above the 4. | ||
| + | :::<math>\scriptstyle{\color{blue}{14-6=8}}</math> | ||
| + | |style="text-align:right;"|והס' שהם ו' עשרות לא נוכל להוציאם מהד' שאחר הו' שהוא עשרות נגדו ונקרא הא' אשר אחריהם ונעביר עליו הקולמוס ויהיה עשר במעלת הד' הנזכר ונחבר אליהם הד' עצמו יהיו כלם י"ד {{#annot:term|181,1232|J2LS}}נוציא מהם{{#annotend:J2LS}} הס' אשר הם ו' עשרות נשארו ח' ונשימנו על הד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Then, we lower it by one rank and double the 8 that is generated at that phase. Since units and a ten result from its double, we do not write 0, but we write 1 for the ten instead of it and the 6 units before it. | ||
| + | :::<math>\scriptstyle{\color{OliveGreen}{2\times8=16}}</math> | ||
| + | |style="text-align:right;"|אחר זה נורידם מעלה אחת ונכפול הח' אשר נתחדש בפעם הזאת ואחר אשר נתחדש מכפלו אחדים ועשר לא נשים ה0' אבל נשים א' בעד העשר במקומה ונשים הו' אחדים לפניו | ||
|} | |} | ||
| − | + | ::<span style=color:Green>[Illustration of the procedure:]</span> | |
:{| | :{| | ||
|- | |- | ||
| Line 3,760: | Line 4,380: | ||
| | | | ||
:*We look for a number to place before them, to multiply by them and by itself and subtract as much as possible from what is above them; it is 7. We write it before them. | :*We look for a number to place before them, to multiply by them and by itself and subtract as much as possible from what is above them; it is 7. We write it before them. | ||
| − | |style="text-align:right;"|ונבקש מספר שנשים לפניהם שנכפלנו בהם ובעצמו ונוציא היותר שאפש' מאשר עליהם ויהיה ז' ונשימנו לפניהם | + | |style="width:45%; text-align:right;"|ונבקש מספר שנשים לפניהם שנכפלנו בהם ובעצמו ונוציא היותר שאפש' מאשר עליהם ויהיה ז' ונשימנו לפניהם |
|- | |- | ||
| | | | ||
| − | ::*We say: seven times 1 is 7. | + | ::*We say: seven times 1 is 7. We subtract it from the 8 that is above it; 1 remains. We write it above it. |
| − | :::<math>\scriptstyle{\color{blue}{1\times7=7}}</math> | + | :::<math>\scriptstyle{\color{blue}{8-\left(1\times7\right)=8-7=1}}</math> |
| − | |style="text-align:right;"|ונאמ' שבעה פעמים א' הם ז' | + | |style="text-align:right;"|ונאמ' שבעה פעמים א' הם ז' נוציאם מהח' אשר על ראשו וישאר א' ונשימנו עליו |
|- | |- | ||
| | | | ||
| − | + | ::*We also say: 7 times 1, by the 1 that is before it, is 7. | |
| − | + | :::<math>\scriptstyle{\color{blue}{1\times7=7}}</math> | |
| − | + | |style="text-align:right;"|עוד נאמר ז' פעמים א' על הא' אשר לפניו הם ז‫' | |
| − | |style="text-align:right;"|עוד נאמר ז' פעמים א' על הא' אשר לפניו הם ז' | ||
|- | |- | ||
| | | | ||
| + | :::We cannot subtract it from the 2 that is above it. We take the 1 that we wrote above the 8 just now, and cross it with a pen; it becomes ten and with the 2 it is 12. We subtract the 7 from it; 5 remains. We write it above the 2. | ||
| + | :::<math>\scriptstyle{\color{blue}{12-7=5}}</math> | ||
|style="text-align:right;"|ולא נוכל להוציאם מהב' אשר על ראשם נקח הא' אשר שמנו עתה על הח' ונעביר עליו הקולמוס ויהיו לעשרה ועם הב' יהיו י"ב נוציא מהם הז' ישארו ה' נשימם על הב‫' | |style="text-align:right;"|ולא נוכל להוציאם מהב' אשר על ראשם נקח הא' אשר שמנו עתה על הח' ונעביר עליו הקולמוס ויהיו לעשרה ועם הב' יהיו י"ב נוציא מהם הז' ישארו ה' נשימם על הב‫' | ||
|- | |- | ||
| | | | ||
| + | ::*We also multiply 7 by 6; the result is 42. | ||
| + | :::<math>\scriptstyle{\color{blue}{6\times7=42}}</math> | ||
|style="text-align:right;"|עוד נכפול הז' בו' יעלו מ"ב | |style="text-align:right;"|עוד נכפול הז' בו' יעלו מ"ב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|נסיר הב' מהח' ואש' עליהם ישארו ו' נשימם עליהם | + | :::We subtract the 2 from the 8 that is above it; 6 remains. We write it above it. |
| + | :::<math>\scriptstyle{\color{blue}{8-2=6}}</math> | ||
| + | |style="text-align:right;"|נסיר הב' מהח' ואש' עליהם ישארו ו' נשימם עליהם | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::We subtract the 4 tens from the 5 that is in the rank that follows; 1 remains. We write it above it. |
| + | :::<math>\scriptstyle{\color{blue}{5-4=1}}</math> | ||
| + | |style="text-align:right;"|ונסיר הד' עשרות מהה' אשר במעלה שאחריהם וישאר א' ונשימנו עליו | ||
|- | |- | ||
| | | | ||
| + | ::*We also multiply 7 by itself; the result is 49. | ||
| + | :::<math>\scriptstyle{\color{blue}{7^2=49}}</math> | ||
| + | |style="text-align:right;"|עוד נכפול הז' על עצמו ויעלה מ"ט | ||
| + | |- | ||
| + | | | ||
| + | :::We cannot subtract even the units from the 0 that is above it. So, we take [5] from the 6 that is after the 5; 1 remains and [the 5] becomes 50 in the rank of the 0. We subtract from it the 49; 1 remains. We write it above it. | ||
| + | :::<math>\scriptstyle{\color{blue}{50-49=1}}</math> | ||
|style="text-align:right;"|ומה0 אשר עליו לא נוכל להסיר אפילו האחדים לכן נסיר מהו' שאחרי הה' וישאר א' ויהיה נ' במעלתם ה0' נסיר מהם המ"ט וישארו א' ונשימנו עליהם | |style="text-align:right;"|ומה0 אשר עליו לא נוכל להסיר אפילו האחדים לכן נסיר מהו' שאחרי הה' וישאר א' ויהיה נ' במעלתם ה0' נסיר מהם המ"ט וישארו א' ונשימנו עליהם | ||
|- | |- | ||
| | | | ||
| + | ::We lower them again by one rank and double the 7 that is generated at that phase; it is 14. We add the ten to the 1 with the 6 that follows it to the left; it is 7. Then, we write the 4, which is the units, before the 7. | ||
| + | :::<math>\scriptstyle{\color{OliveGreen}{2\times7=14}}</math> | ||
|style="text-align:right;"|עוד נורידם מעלה אחת ונכפול הז' אשר נתחדש בזאת הפעם ויהיו י"ד ונחבר העשר לא' עם הו' אשר אחריו לצד שמאל ויהיו ז' אחרי כן נשים הד' שהם האחדים לפני הז‫' | |style="text-align:right;"|עוד נורידם מעלה אחת ונכפול הז' אשר נתחדש בזאת הפעם ויהיו י"ד ונחבר העשר לא' עם הו' אשר אחריו לצד שמאל ויהיו ז' אחרי כן נשים הד' שהם האחדים לפני הז‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :*We look for a number to multiply by all of them and by itself, as in the other phases. We do not find even one, as they cannot be subtracted from what is above them even once. So, we write a zero before them. |
| − | |style="text-align:right;"|ונבקש מספר לכפול על כולם ועל עצמו כבשאר הפעמים ולא נמצא | + | |style="text-align:right;"|‫<ref>32v</ref>ונבקש מספר לכפול על כולם ועל עצמו כבשאר הפעמים ולא נמצא כי אין גם אחד לפי שלא יוכלו לצאת מאשר על ראשם אפי' פעם אחת לכן נשים [סיפרא]‫<ref>marg.</ref> לפניהם |
| + | |- | ||
| + | | | ||
| + | ::We lower them by one rank again, but we do not double any number, since no number is generated at this phase and the 0 is not a number to be doubled. | ||
| + | |style="text-align:right;"|ונורידם עוד מעלה אחת ולא נכפול שום מספר כי לא נתחדש מספ' בפעם הזאת וה0' אינה מספר לכפלה | ||
| + | |- | ||
| + | | | ||
| + | :*We look for a number to place before them; it is 9. | ||
| + | |style="text-align:right;"|ונבקש מספר שנשים לפניהם ויהיה ט‫' | ||
| + | |- | ||
| + | | | ||
| + | ::*We multiply it by each of them and subtract [the product] from what is above it, also by itself and subtract [the product] from what is above it; as you see in the written diagram. | ||
| + | |style="text-align:right;"|ונכפלנו בכל אחד ונוציאנו מאשר ימצא על ראשו וגם בעצמו ונוציאנו מאשר על ראשו כאשר תראה בצורה הרשומה | ||
| + | |- | ||
| + | | | ||
| + | ::We lower them again and double the 9 that is generated at that phase; it is 18. Since a ten is generated here with the units, we do not write the 0, in this lowering, but we write 1 for the ten instead of it. We write the 8 that are the units before it. | ||
| + | :::<math>\scriptstyle{\color{OliveGreen}{2\times9=18}}</math> | ||
| + | |style="text-align:right;"|עוד נורידם ונכפול הט' שנתחדש עתה בפעם הזאת ויהיו י"ח ואחר שנתחדש כאן עשר עם האחדים לא נשים ה0' בהורדה זו אבל נשים א' לעשר במקומה ונשים הח' שהם אחדים לפניו | ||
| + | |- | ||
| + | | | ||
| + | :*We look for a number to place before them as in the previous phases; it is 4. | ||
| + | |style="text-align:right;"|ונבקש מספר נשים לפניהם כפעם בפעם ויהיו ד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::*We multiply it by each of them and by itself and subtract [each product] from its corresponding place as mentioned. | ||
| + | |style="text-align:right;"|ונכפלנו בכל אחד גם בעצמו ונוציא כל דבר ממקומו הראוי לו כנזכר | ||
| + | |- | ||
| + | | | ||
| + | ::Now, we reached the first rank, so we should not lower them. | ||
| + | |style="text-align:right;"|והנה הגענו למעלה הראשונה לכן אין לנו להורידם | ||
|- | |- | ||
| | | | ||
| − | : | + | :The root are the digits that are generated in each phase, and they are: 587094. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ויהיה השרש המספר שחדשנו בכל פעם אחד והם 587094 |
|- | |- | ||
| | | | ||
| − | : | + | :If nothing were left, this root would have been a proper root, but since something remains, which is 764230, this root is not a proper root, but approximate. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם לא היה נשאר דבר היה זה השרש אמיתי אבל אחר שנשאר דבר והוא 764230 אין השרש הזה אמיתי כי אם בקרוב |
|- | |- | ||
| | | | ||
::<math>\scriptstyle\longrightarrow{\color{Violet}{\begin{align}&\scriptstyle587094\ the\ root\\&\scriptstyle764230\ the\ remainder\\\end{align}}}</math> | ::<math>\scriptstyle\longrightarrow{\color{Violet}{\begin{align}&\scriptstyle587094\ the\ root\\&\scriptstyle764230\ the\ remainder\\\end{align}}}</math> | ||
| − | | | + | | |
| − | |||
|- | |- | ||
| − | | | + | |It will be explained afterwards how we come closer to the truth, even if the truth is absent. |
|style="text-align:right;"|ועוד נתבאר אחר זה איך {{#annot:term|1612,1874|Odqz}}נתקרב יותר אל האמת{{#annotend:Odqz}} ואם האמת נעדרת | |style="text-align:right;"|ועוד נתבאר אחר זה איך {{#annot:term|1612,1874|Odqz}}נתקרב יותר אל האמת{{#annotend:Odqz}} ואם האמת נעדרת | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle\sqrt{10375}</math> | + | *{{#annot:√10375|439|WnAI}}Another example: we wish to know the root of the number 10375. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle\sqrt{10375}</math> |
| + | |style="text-align:right;"|<big>משל</big> אחר רצינו לדעת שרש מספר זה 10375{{#annotend:WnAI}} | ||
|- | |- | ||
| | | | ||
| Line 3,827: | Line 4,492: | ||
|}<br> | |}<br> | ||
::<math>\scriptstyle\longrightarrow{\color{Violet}{\begin{align}&\scriptstyle101\ the\ root\\&\scriptstyle174\ the\ remainder\\\end{align}}}</math> | ::<math>\scriptstyle\longrightarrow{\color{Violet}{\begin{align}&\scriptstyle101\ the\ root\\&\scriptstyle174\ the\ remainder\\\end{align}}}</math> | ||
| − | |style="text-align:right;"|ואחר שמספר המעלות נפרד שהן ה' נקח הא' אשר נמצא במעלה האחרונה ונבקש מספר שנכפלנו בעצמו ונוציאנו כלו או היותר שאיפשר ויהיה א' ונשימנו תחתיו ונכפול לא' זה על עצמו ונוציאנו מהא' אשר על אשר על ראשו ונעביר עליו קולמוס | + | | |
| − | ונכפלנו ונורידנו ולא נוכל להוציאם מה0' אשר עליהם אפי' פעם אחת גם על האחד לא נותר דבר לכן נשים 0 לפניו עוד נורידם ולא נכפול דבר כי לא נתחדש מספר בזה הפעם | + | |- |
| − | ונבקש מספר אשר נשים לפניהם כנזכר ויהיה א' ונשימנו לפניהם ונכפול הא' על הב' ויהיו ב' נסירם מהג' אשר עליהם ישאר א' ונשימנו עליו ונכפול הא' על עצמו ויעלה א' נסירנו מהה' אשר עליו וישארו ד' נשימם עליו | + | | |
| − | וכבר שלמו המעלות ולא נוריד עוד והנה ה{{#annot:term|204,1332|PBaR}}אותיות{{#annotend:PBaR}} והם שנתחדשו פעם בפעם | + | :Since the number of the ranks is odd, which is 5, we take the 1 that is in the last rank. |
| + | |style="text-align:right;"|ואחר שמספר המעלות נפרד שהן ה' נקח הא' אשר נמצא במעלה האחרונה | ||
| + | |- | ||
| + | | | ||
| + | :*We seek for a number to multiply by itself so that all of it will be cast out, or as much as possible of it; it is 1. We write it beneath it. | ||
| + | |style="text-align:right;"|ונבקש מספר שנכפלנו בעצמו ונוציאנו כלו או היותר שאיפשר ויהיה א' ונשימנו תחתיו | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply 1 by itself and subtract it from the 1 that is above it. We cross it with a pen. | ||
| + | :::<math>\scriptstyle{\color{blue}{1-1^2}}</math> | ||
| + | |style="text-align:right;"|ונכפול לא' זה על עצמו ונוציאנו מהא' ‫<ref>33r</ref>אשר על אשר על ראשו ונעביר עליו קולמוס | ||
| + | |- | ||
| + | | | ||
| + | ::We double it and lower it, but we cannot subtract it even once from the 0 that is above it and above the 1 there is nothing left. So we write 0 before it and lower it again, but we do not double anything, since no number is generated at this phase. | ||
| + | |style="text-align:right;"|ונכפלנו ונורידנו ולא נוכל להוציאם מה0' אשר עליהם אפי' פעם אחת גם על האחד לא נותר דבר <sup>לכן</sup> נשים 0 לפניו עוד נורידם ולא נכפול דבר כי לא נתחדש מספר בזה הפעם | ||
| + | |- | ||
| + | | | ||
| + | :*We seek for a number to write before it as mentioned; it is 1. We write it before it. | ||
| + | |style="text-align:right;"|ונבקש מספר אשר נשים לפניהם כנזכר ויהיה א' ונשימנו לפניהם | ||
| + | |- | ||
| + | | | ||
| + | ::*We multiply 1 by 2; it is 2. We subtract it from the 3 that is above it; 1 remains. We write it above it. | ||
| + | :::<math>\scriptstyle{\color{blue}{3\left(1\times2\right)=3-2=1}}</math> | ||
| + | |style="text-align:right;"|ונכפול הא' על הב' ויהיו ב' נסירם מהג' אשר עליהם ישאר א' ונשימנו עליו | ||
| + | |- | ||
| + | | | ||
| + | ::*We multiply 1 by itself; the result is 1. We subtract it from the 5 that is above it; 4 remains. We write it above it. | ||
| + | :::<math>\scriptstyle{\color{blue}{5-1^2=5-1=4}}</math> | ||
| + | |style="text-align:right;"|ונכפול הא' על עצמו ויעלה א' נסירנו מהה' אשר עליו וישארו ד' נשימם עליו | ||
| + | |- | ||
| + | | | ||
| + | :The ranks are completed, so we do not lower again. | ||
| + | |style="text-align:right;"|וכבר שלמו המעלות ולא נוריד <sup>עוד</sup> | ||
| + | |- | ||
| + | | | ||
| + | :The digits that are generated in each phase are the root, which is: 101. | ||
| + | |style="text-align:right;"|והנה ה{{#annot:term|204,1332|PBaR}}אותיות{{#annotend:PBaR}} והם שנתחדשו פעם בפעם הם השרש והוא 101 | ||
| + | |- | ||
| + | | | ||
| + | | | ||
{|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| Line 3,847: | Line 4,551: | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ולפי שנשאר ולפי שנשאר שם מספר מה אין זה שרש אמיתי אבל הקרוב ועוד נתבאר איך נתקרב יותר אל האמת | + | :Since a number is left there, this is not a proper root, but approximate. |
| + | |style="text-align:right;"|ולפי שנשאר ולפי שנשאר שם מספר מה אין זה שרש אמיתי אבל הקרוב | ||
| + | |- | ||
| + | |It will be explained how we come closer to the truth. | ||
| + | |style="text-align:right;"|ועוד נתבאר איך נתקרב יותר אל האמת | ||
|- | |- | ||
| | | | ||
| − | ==== reason: procedure ==== | + | ==== <span style=color:Green>reason: procedure</span> ==== |
| | | | ||
|- | |- | ||
| − | |The reason we say that if the number of the ranks is an odd number we take the last digit alone and seek a number to put beneath it and if it is an even number we take the two last digits, the last as tens and the one that precedes it as units: for every product of a rank | + | |The reason we say that if the number of the ranks is an odd number we take the last digit alone and seek a number to put beneath it and if it is an even number we take the two last digits, the last as tens and the one that precedes it as units: because for every product of a non-units rank by itself, the rank of the units of this product [<math>\scriptstyle{\color{OliveGreen}{\left(a\sdot10^n\right)^2}}</math>] is always an odd number [<math>\scriptstyle{\color{OliveGreen}{\left(2n-1\right)}}</math>]. |
| − | <math>\scriptstyle{\color{ | ||
|style="text-align:right;"|<big>וטעם</big> אמרנו שאם מספר מעלות החשבון נפרד שנקח האחרון לבד ונבקש מספר נשים תחתיו וכו' ואם הם זוג שנקח השני רשמים האחרונים האחרון לעשרות ושלפניו לאחדים הוא לפי שכל כפל כלל בעצמו הנה מעלת האחדים העולים בכפל ההוא היא נפרד לעולם | |style="text-align:right;"|<big>וטעם</big> אמרנו שאם מספר מעלות החשבון נפרד שנקח האחרון לבד ונבקש מספר נשים תחתיו וכו' ואם הם זוג שנקח השני רשמים האחרונים האחרון לעשרות ושלפניו לאחדים הוא לפי שכל כפל כלל בעצמו הנה מעלת האחדים העולים בכפל ההוא היא נפרד לעולם | ||
|- | |- | ||
| − | |Since the | + | |Since the decimal place of the product of every two digits, i.e. the rank of this product is as [the sum of] the ranks of both digits minus one, as we have explained in chapter three. |
| − | |style="text-align:right;"|לפי שמקום הנחת כפל כל שני מספרים | + | |style="text-align:right;"|לפי שמקום הנחת כפל כל שני מספרים ר"ל שמדרגת הכפל ההוא כמדרגות שני המספרים יחד חסר אחד כמו שביארנו בפרק הג‫' |
|- | |- | ||
| − | | | + | |Hence, the rank of the units of the product of every number by itself is double its rank minus one, and this is always an odd number. |
| − | + | |style="text-align:right;"|ולזה מדרגת אחדי מספר כפל מספר על עצמו והיא כפל מדרגותיו חסר אחד והנה הם הנפרדים לעולם | |
| + | |- | ||
| + | |So, when the number of ranks of the number is odd, we subtract from the last rank [= the leftmost rank] the square of the root, i.e. the square of the digit that we write beneath it, which is part of the root. | ||
|style="text-align:right;"|ולזה כשהמספר מעלות המספר נפרד אנו מוציאים מהמעלה האחרונה שהיא נפרדת מרובע השרש ר"ל מרובע המספרים אשר שמנו תחתיו שהוא חלק השרש | |style="text-align:right;"|ולזה כשהמספר מעלות המספר נפרד אנו מוציאים מהמעלה האחרונה שהיא נפרדת מרובע השרש ר"ל מרובע המספרים אשר שמנו תחתיו שהוא חלק השרש | ||
|- | |- | ||
| + | |But, if the number [of ranks] is even, we subtract [the square of the last rank of the root from] the two last ranks [of the number] one as tens and the other as units. For the units of the product of a number by itself is always placed in an odd rank and the tens in an even rank, and this is clear. | ||
| + | |style="text-align:right;"|ואם הם זוג לקחנו השתים האחרונות זו לעשרות וזו לאחדי' ‫<ref>33v</ref>בענין שלעולם אחדי כפל כל מספר בעצמו יצאו ממעלה נפרדת והעשרות ממעלת זוג וזה ברור | ||
| + | |- | ||
| + | |Since we have explained that the rank of the units of the product [of a number by itself] is double the ranks of the root, which is the number that we multiply by itself, minus one, we find that if the root is in the first [rank], the product is also in the first [rank]; if the root is in the second [rank] the square is in the third [rank]; if [the root is in] the third, [the square is] in the fifth; if [the root is in] the fourth, [the square is] in the seventh; and so on. | ||
| + | |style="text-align:right;"|ואחר שביארנו שמדרגות אחדי הכפל הם כפל מעלות השרש שהוא המספר שכפלנוהו על עצמו חסר אחת נמצא שאם השרש<s>ם</s> הוא בראשונה [הכפל ג"כ בראשונה]‫<ref>marg.</ref> ואם השרש בשנית המרובע בשלישית ואם בשלישית בחמישית ואם ברביעית בשביעית וכן לעולם | ||
| + | |- | ||
| + | |Thus, the addition of one rank in the root requires an addition of two ranks in the square. | ||
| + | |style="text-align:right;"|הנה כי תוספת מעלה אחת בשרש יחייב תוספת א"כ ב' מעלות במרובע | ||
| + | |- | ||
| + | |We do accordingly in the procedure: for every two ranks added to the number, we add one [rank] to the root, by lowering the root each time by one rank and adding one rank to it. | ||
| + | |style="text-align:right;"|וכן נעשה במעשה <s>כ</s> <sup>כי</sup> לכל ב' מעלות מתוספת בחשבון אנו מוסיפים אחד בשרש וזה שאנו מורידין השרש מעלה אחת בכל פעם ומוסיפים עליו מעלה אחת והוא המספר אשר אנו שמים לפניהם בכל פעם | ||
| + | |- | ||
| + | |We find that tnumber of the shifting phases [in the procedure] is as the number of the even ranks of the [given] number from the first decimal position as well as the number of ranks of the root from the first rank. You will see all this explained in the diagram. | ||
| + | |style="text-align:right;"|נמצא שכמספר פעמי ההורדה כך הוא מספר זוגי מעלות החשבון על מקום ההנחה הראשונה וכמספר <sup>זה הוא</sup> <s>זהו</s> מספר מעלות השרש על המעלה האחת הראשונה וכל זה תראה מפורש בצורה | ||
| + | |- | ||
| + | !<span style=color:Green>The reason for shifting the subtrahend one rank to the right each phase:</span> | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |The reason for lowering by one rank each time is that what is added in the root at that time is one rank lower than what was [before], therefore, the rank of the [square] is also lower by one rank, i.e. what is added now in the root multiplied by what is already given to the root in the previous phase or phases. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>והטעם</big> הורדת מעלה אחת בכל פעם הוא לפי שהמתוסף בשרש בפעם הזאת הוא מעלה אחת פחות מאשר {{#annot:term|2510,178|cJCp}}נתוסף{{#annotend:cJCp}} בתחלה וא"כ מעלת הכפל יהיה ג"כ מעלה אחת פחות ר"ל כפול זה המתוסף עתה בשרש באשר היה כבר המונח לשרש בפעם או <sup>ב</sup>פעמים העוברים |
|- | |- | ||
| − | | | + | |Because if what is given at first is, for instance, a product of the digit by itself, what we add now in the root is less than the former by one rank, and when we multiply it by the former, [the product] is subtracted from the rank that [the former] is subtracted. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כי ע'ד'מ' המושם בתחלה הוא מכפל המספר בעצמו וכאשר אנחנו מוסיפים עתה בשרש זה המתוסף הוא פחות מעלה אחת מהראשון וכאש' כפלנוהו בראשון יגרע זאת המעלה אשר גרע זה ממנו |
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :Example: if the product of [the last digit of the root] is subtracted from the fifth rank, then the rank of [that digit] is in the third rank, and therefore [its product] is subtracted from the fifth rank, which is double its rank minus one [5=(3+3)-1]. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{\left(a00\right)^2=\left(a^2\right)0000}}</math> | |
| + | |style="text-align:right;"|כי המשל אם כפל השרש הראשון בעצמו היה לוקח מהמעלה החמישית הוא היה מן המעלה השלישית ולזה לקח ‫<ref>34r</ref>מהחמישית שהוא כפל מעלותיו חסר אחת | ||
|- | |- | ||
| | | | ||
| − | : | + | :When we add now to the root, what is added is in the second rank, and when we multiply what is in the second rank by what is in the third rank, the rank of this product is in the fourth rank, which is the number of the ranks of both digits minus one [4=(3+2)-1]. Therefore, we shift what was first one rank lower, as it should be subtracted from there. |
| − | + | |style="text-align:right;"|וכאשר נוסיף זה עתה בשרש יהיה המתוסף מהמעלה השנית וכאשר כפלנו אשר מהמעלה השנית על אשר במעלה הג' ר"ל כאשר אנו כופלים זה המתחדש עתה שהוא במעלה הב' באשר היה בתחלה שהוא מהמעלה הג' יהיה מדרגת זה הכפל במדרגת הד' שהם מספר מעלות שני המספרים חסר אחת ולזה שמנו אשר בתחלה מעלה אחת למטה כי משם הוא ראוי לקחתו | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | :When we multiply the added root by itself, [the product] is subtracted from the third rank only, for the product of [a digit in the second rank] by [a digit in the second rank] should be subtracted from the third [rank], which is as [the sum of] the ranks of the two digits minus one [3=(2+2)-1]. Therefore, we shift [the former digit of the root] one rank lower, as [its product] should be subtracted from there. |
| − | |style="text-align:right;"|וכאשר | + | :<math>\scriptstyle{\color{OliveGreen}{\left(ab0\right)^2=\left(a^2\right)0000+\left[2\sdot\left[\left(a\sdot b\right)000\right]\right]+\left(b^2\right)00}}</math> |
| + | |style="text-align:right;"|השרש המתוסף כאשר כפלנוהו בעצמו יגרע מעלה אחרת ואין לו לקח' כי אם מהמעלה השלישית כי כפל בעל שתי מעלות בבעל שתי מעלות יש לו לקחת מהשלישית שהוא כ{{#annot:term|203,1344|LiSQ}}מדרגות{{#annotend:LiSQ}} שני המספרים חסר אחת לכן שמנוהו מעלה אחת לפניהם כי משם ראוי לו לקחת | ||
| + | |- | ||
| + | |In each phase, the rank, from which the product of [the digit added in the root] by the former [digits] is to be subtracted, is lower by one than [the rank of] the product of [the digit added in the root] in the previous phase by the [former digits], and [the rank of] its product by itself is lower by two. | ||
| + | |style="text-align:right;"|וכן בכל פעם יחסר מעלה ממקום הראוי לקחת עתה בכפל המתחדש בראשונים מאשר היה מכפל המתחדש בפעם העובר עמהם וכפלו בעצמו יחסר שתים | ||
| + | |- | ||
| + | |All this is explained in reason and in diagram. | ||
| + | |style="text-align:right;"|וכל זה מבואר בטעם ובצורה | ||
| + | |- | ||
| + | |When we cannot subtract [the product] even once, we shift [the digit added in the root] once, and what is added in the root is lower by two ranks from [the former digit], so we lower it by two ranks, to be subtracted from the rank that is lower by two and the product of what is added in the root by itself is subtracted from the rank that is lower by four ranks [from where the product of the former digit was subtracted], since [the added digit] is lower by two ranks. | ||
| + | |style="text-align:right;"|וכאשר אין אנו יכולים להוציאם אפי' פעם אחת אנו שמים ומורידים אותם פעם אחרת כי כאשר תוסף בשרש יהיה פחות ב' מעלות מאשר בתחלה לכן הורדנום ב' מעלות שיקחו מב' מעלות פחות וכפל השרש המתוסף בעצמו יקח מד' מעלות פחות לפי שירד שני מעלות | ||
|- | |- | ||
| | | | ||
| − | : | + | :Example: if the first [digit of the root] is in the fourth [rank], its product by itself is subtracted from the seventh rank, and if [the preceding digit of the root] is a zero, what is added [after it in the root] is in the second [rank] and its product by itself should be subtracted from the third [rank], so we subtract four ranks. |
| − | :<math>\scriptstyle\left( | + | :<math>\scriptstyle{\color{OliveGreen}{\left(a0b0\right)^2=\left(a^2\right)000000+\left[2\sdot\left[\left(a\sdot b\right)00000\right]\right]+\left(b^2\right)00}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כי המשל אם הראשון היה ברביעית היה לו ליקח כפלתו בעצמו מהמעלה השביעית ואשר מתוסף עתה כשהיה 0 בפעם אשר בנתים יהיה בשנית וראוי לקחת כפלו בעצמו מהשלישית הרי כשנגרע ד' מעלות |
|- | |- | ||
| − | | | + | |All this is also explained in reason and in diagram. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|גם כל זה הוא מבואר בטעם ובצורה |
|- | |- | ||
| + | !<span style=color:Green>The reason for doubling the digits of the root:</span> | ||
| | | | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |The reason for doubling the root, i.e. that in every [phase of the procedure] we multiply [the digit that is added to the root] by double the former [digit of the] root and by itself, is because every thing that is added to the root is added to both sides [= multiplicands] of the square. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>וטעם</big> הכפל השרש ר"ל שבכל הולדה אנו כופלים אשר <s>הו</s> נתחדש אז ונמצא ‫<ref>34v</ref>שאנו כופלים המתחדש בכפל השרש הראשון ובעצמו הוא לפי שכאשר {{#annot:term|2510,178|ELza}}ניתוסף{{#annotend:ELza}} דבר בשרש הוא ניתוסף בשתי צלעות המרובע |
|- | |- | ||
| | | | ||
| − | + | :I.e. if the root at the beginning is 30, the square is 900. | |
| − | |style="text-align:right;"|ר"ל שאם מתחלה היה השרש 30 | + | :<math>\scriptstyle{\color{blue}{\left(30\right)^2=900}}</math> |
| + | |style="text-align:right;"|ר"ל שאם מתחלה היה השרש 30 הנה המרובע היה 900 | ||
|- | |- | ||
| | | | ||
| − | + | :If we add 5 to it, it becomes 35 and its square is 35 by 35, which is as saying: the product of 30 by 30, the product of 5 by 30, the product of 30 by 5, and the product of 5 by 5. | |
| − | |style="text-align:right;"|ואם אנו מוסיפים עליו ה' יהיוה ל"ה ומרובעו הוא כפל ל"ה על ל"ה שהוא כאומרנו לכפל ל' בל' וכפל ה' [בל' וכפל ל' בה' וכפל ה'] בה' | + | |style="text-align:right;"|ואם אנו מוסיפים עליו ה' יהיוה ל"ה ומרובעו הוא כפל ל"ה על ל"ה שהוא כאומרנו לכפל ל' בל' וכפל ה' [בל' וכפל ל' בה' וכפל ה']‫<ref>marg.</ref> בה‫' |
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"|ולזה אנו כופלים השרש וכשאנו מורידים אין אנו כופלים אלא אשר מתוסף בפעם העובר בסמוך שלא נכפל אבל כל אחדים כבר נכפלו לכן אין אנו כופלים אותו פעם אחרת כלל ומכל זה תדע כי השרש הוא המספרים המתחדשים בכל פעם פשוטים בלי כפל כלל | + | :We find that because of the addition of 5 [to the root], [the square] increases by twice the product of 5 by 30, and once the product of 5 by 5. |
| + | |style="text-align:right;"|נמצא שנתוסף בסבת תוספת הה' כפל ה' על ל' פעמים ר"ל ה' בה' פעם אחת ובעצמו פעם אחת | ||
| + | |} | ||
| + | *<math>\scriptstyle{\color{blue}{\left(30+5\right)^2=\left(35\right)^2=35\sdot35=\left(30\sdot30\right)+\left(5\sdot30\right)+\left(30\sdot5\right)+\left(5\sdot5\right)=\left(30\right)^2+\left[\left[2\sdot\left(5\sdot30\right)+5^2\right]\right]}}</math> | ||
| + | {| | ||
| + | |- | ||
| + | |Therefore, we double the root, but when we lower [the digits of the root], we double only the [digit] the was added in the preceding phase that was not doubled yet, as all the [other digits] are already doubled, therefore we do not double them again, since the root consists of the digits that are added in each phase that are not doubled at all. | ||
| + | |style="width:45%; text-align:right;"|ולזה אנו כופלים השרש וכשאנו מורידים אין אנו כופלים אלא אשר מתוסף בפעם העובר בסמוך שלא נכפל אבל כל אחדים כבר נכפלו לכן אין אנו כופלים אותו פעם אחרת <sup>כלל</sup> ומכל זה תדע כי השרש הוא המספרים המתחדשים בכל פעם פשוטים בלי כפל כלל | ||
|- | |- | ||
|} | |} | ||
| Line 3,941: | Line 4,680: | ||
*<span style=color:Green>Second approximation:</span> | *<span style=color:Green>Second approximation:</span> | ||
:If you wish to come closer to the truth, even if the truth is hidden from the eyes of all living [Job 28, 21], as '''Euclid''' proved, multiply the integer and fractions by themselves, as I will explain in the chapter on multiplication, in the section on fractions and the result will exceed or fall short of the initial number [whose root is being extracted]. | :If you wish to come closer to the truth, even if the truth is hidden from the eyes of all living [Job 28, 21], as '''Euclid''' proved, multiply the integer and fractions by themselves, as I will explain in the chapter on multiplication, in the section on fractions and the result will exceed or fall short of the initial number [whose root is being extracted]. | ||
| − | |style="text-align:right;"|ואם תרצה להתקרב עוד אל האמת ואם האמת ''נעלמה מעיני כל חי''<ref>איוב כח, כא</ref> כאשר ביאר אוקלידס במופת כפול אלו השלמים והשברים על עצמם כאשר אבאר בחלק השברים בפרק הכפל ויעלה פחות או יותר מהחשבון הראשון | + | |style="text-align:right;"|ואם תרצה להתקרב עוד אל האמת ואם האמת ''נעלמה מעיני כל חי''‫<ref group=note>איוב כח, כא</ref> כאשר ביאר אוקלידס במופת כפול אלו השלמים והשברים על עצמם כאשר אבאר בחלק השברים בפרק הכפל ויעלה פחות או יותר מהחשבון הראשון |
|- | |- | ||
| | | | ||
:Double the root, as stated, and divide the excess or deficit by [the result]. | :Double the root, as stated, and divide the excess or deficit by [the result]. | ||
| − | |style="text-align:right;"|וכפול השרש כאשר אמרנו וחלק אליו זה העודף או חסרון | + | |style="text-align:right;"|וכפול השרש כאשר אמרנו וחלק אליו זה העודף ‫<ref>35r</ref>או חסרון |
|- | |- | ||
| | | | ||
:*Subtract the result from the preceding fractions if the number [whose root is being extracted] is less than the square of the root that you extracted in the previous stage. | :*Subtract the result from the preceding fractions if the number [whose root is being extracted] is less than the square of the root that you extracted in the previous stage. | ||
|style="text-align:right;"|והיוצא הוציאנו מהשברים הראשונים אם המספר היה פחות ממרובע השרש אשר הוצאת בפעם הקודמת | |style="text-align:right;"|והיוצא הוציאנו מהשברים הראשונים אם המספר היה פחות ממרובע השרש אשר הוצאת בפעם הקודמת | ||
| − | | | + | |} |
| − | |||
::<math>\scriptstyle{\color{OliveGreen}{\left(a+\frac{b}{2a}\right)^2>a^2+b\longrightarrow\sqrt{a^2+b}\approx\left(a+\frac{b}{2a}\right)-\frac{\left(a+\frac{b}{2a}\right)^2-\left(a^2+b\right)}{2\sdot\left(a+\frac{b}{2a}\right)}}}</math> | ::<math>\scriptstyle{\color{OliveGreen}{\left(a+\frac{b}{2a}\right)^2>a^2+b\longrightarrow\sqrt{a^2+b}\approx\left(a+\frac{b}{2a}\right)-\frac{\left(a+\frac{b}{2a}\right)^2-\left(a^2+b\right)}{2\sdot\left(a+\frac{b}{2a}\right)}}}</math> | ||
| − | | | + | {| |
|- | |- | ||
| | | | ||
:*If the square is less than the [given] number, add the result to the preceding fractions. | :*If the square is less than the [given] number, add the result to the preceding fractions. | ||
| − | |style="text-align:right;"|ואם היה המרובע פחות מהמספר תוסיף זה היוצא על השברים הראשונים | + | |style="width:45%; text-align:right;"|ואם היה המרובע פחות מהמספר תוסיף זה היוצא על השברים הראשונים |
| − | | | + | |} |
| − | + | ||
::<math>\scriptstyle{\color{OliveGreen}{\left(a+\frac{b}{2a}\right)^2<a^2+b\longrightarrow\sqrt{a^2+b}\approx\left(a+\frac{b}{2a}\right)+\frac{\left(a^2+b\right)-\left(a+\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}}}</math> | ::<math>\scriptstyle{\color{OliveGreen}{\left(a+\frac{b}{2a}\right)^2<a^2+b\longrightarrow\sqrt{a^2+b}\approx\left(a+\frac{b}{2a}\right)+\frac{\left(a^2+b\right)-\left(a+\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}}}</math> | ||
| − | | | + | {| |
|- | |- | ||
| | | | ||
:The sum or the remainder will be the fraction added in the root to the initial integer. | :The sum or the remainder will be the fraction added in the root to the initial integer. | ||
| − | |style="text-align:right;"|והעולה או הנותר יהיו השברים העודפי' בשרש על השלמים הראשונים | + | |style="width:45%; text-align:right;"|והעולה או הנותר יהיו השברים העודפי' בשרש על השלמים הראשונים |
|- | |- | ||
| | | | ||
:*The example for this is 174 in the second diagram. | :*The example for this is 174 in the second diagram. | ||
| − | ::<math>\scriptstyle{\color{ | + | ::<math>\scriptstyle{\color{OliveGreen}{\sqrt{10375}}}</math> |
|style="text-align:right;"|המשל בזה הוא בצורה השנית 174 | |style="text-align:right;"|המשל בזה הוא בצורה השנית 174 | ||
|- | |- | ||
| | | | ||
::*<span style=color:Green>First approximation:</span> | ::*<span style=color:Green>First approximation:</span> | ||
| − | + | ::If it were less than the root, we would have divide it by double the root, which is 202, without adding one. | |
|style="text-align:right;"|ואם היה פחות מהשרש היינו מחלקים אותו לכפל השרש שהוא 202 בלי תוספת אחד | |style="text-align:right;"|ואם היה פחות מהשרש היינו מחלקים אותו לכפל השרש שהוא 202 בלי תוספת אחד | ||
|- | |- | ||
| | | | ||
| − | + | ::Since it is more than the root, we double the root, which is 101; so it is 202. We add one to it; they are 203. | |
| − | + | ::<math>{\color{blue}{\scriptstyle174>101\longrightarrow\left(2\sdot101\right)+1=202+1=203}}</math> | |
|style="text-align:right;"|וכן עתה שהוא יותר מהשרש נכפול השרש שהנו 101 ויהא 202 ונוסיף עליו א' ויהיו 203 | |style="text-align:right;"|וכן עתה שהוא יותר מהשרש נכפול השרש שהנו 101 ויהא 202 ונוסיף עליו א' ויהיו 203 | ||
|- | |- | ||
| | | | ||
| − | + | ::We extract its denominators: we find that it is divisible by seven and its seventh is 29. Hence, these are its denominators, i.e. 7 and 29. | |
| − | + | ::<math>\scriptstyle{\color{blue}{203=7\sdot29}}</math> | |
|style="text-align:right;"|ונוציא מוריו ונמצא שיש לו שביעית ושביעיתו 29 ואלו הם מוריו ר"ל ז' כ"ט | |style="text-align:right;"|ונוציא מוריו ונמצא שיש לו שביעית ושביעיתו 29 ואלו הם מוריו ר"ל ז' כ"ט | ||
|- | |- | ||
| | | | ||
| − | + | ::We divide the remainder, which is 174, by them; the result of division is 6 sevenths and these are the fractions that are added to 101, which are the first integers, in the root. | |
| − | + | ::<math>\scriptstyle{\color{blue}{\sqrt{10375}\approx101+\frac{174}{203}\approx101+\frac{6}{7}}}</math> | |
|style="text-align:right;"|ונחלק אליהם השארית שהוא 174 ויצא בחילוק ו' שביעיות שלמות ואלו הם השברים העודפים בשרש על הק"א השלמים הראשונים | |style="text-align:right;"|ונחלק אליהם השארית שהוא 174 ויצא בחילוק ו' שביעיות שלמות ואלו הם השברים העודפים בשרש על הק"א השלמים הראשונים | ||
|- | |- | ||
| | | | ||
::*<span style=color:Green>Second approximation:</span> | ::*<span style=color:Green>Second approximation:</span> | ||
| − | + | ::If we wish to come closer to the truth, we multiply this root, i.e. 101 integers and 6 sevenths, by itself; they are 10374 integers, 6 sevenths and a seventh of a seventh; as will be explained in the third chapter of the second section. | |
| − | + | ::<math>\scriptstyle{\color{blue}{\left(101+\frac{6}{7}\right)^2=10374+\frac{6}{7}+\left(\frac{1}{7}\sdot\frac{1}{7}\right)}}</math> | |
| − | |style="text-align:right;"|ואם נרצה להתקרב עוד אל האמת נכפול זה השרש ר"ל ק"א שלמים וו' שביעיות על עצמו 10374 שלמים וו' שביעיות שלימות ושביעית שביעית כאשר יתבאר בחלק הב' בפרק הג' | + | |style="text-align:right;"|ואם נרצה להתקרב עוד אל האמת נכפול זה השרש ר"ל ק"א שלמים וו' שביעיות על עצמו 10374 שלמים וו' שביעיות שלימות ושביעית שביעית כאשר יתבאר בחלק הב' בפרק הג‫' |
|- | |- | ||
| | | | ||
| − | + | ::This is less than the requested number by 6 sevenths of a seventh. | |
| − | + | ::<math>\scriptstyle{\color{blue}{10375-\left(101+\frac{6}{7}\right)^2=\frac{6}{7}\sdot\frac{1}{7}}}</math> | |
|style="text-align:right;"|וזהו פחות מהחשבון הנשאל בו' שביעיות שביעית | |style="text-align:right;"|וזהו פחות מהחשבון הנשאל בו' שביעיות שביעית | ||
|- | |- | ||
| | | | ||
| − | + | ::Therefore, if you wish to come closer to the truth, you have to double the root, i.e. the 101 integers and 6 sevenths, and divide the 6 sevenths of a seventh by [this product]; then you should add the result to the previous root, which is 101 integers and 6 sevenths and so on. | |
| − | + | ::<math>\scriptstyle{\color{blue}{\left(101+\frac{6}{7}\right)^2<10375\longrightarrow\sqrt{10375}\approx\left(101+\frac{6}{7}\right)+\frac{\frac{6}{7}\sdot\frac{1}{7}}{2\sdot\left(101+\frac{6}{7}\right)}}}</math> | |
|style="text-align:right;"|לכן אם אתה רוצה להתקרב עוד אל האמת יש לך לכפול השרש ר"ל הק"א שלימים וו' שביעיות שלימות ולחלק אליהם אלו הו' שביעיות שביעית <s>לכן אם אתה רוצה להתקרב עוד אל האמת יש לך לכפול השרש ר"ל הק"א שלימים וו' שביעיות שלמות ולחלק אליהם אלו הו' שביעיות שביעית</s> והיוצא היה לך להוסיף אותו על השרש הקודם שהיה ק"א שלימים וו' שביעיות וכן לעולם | |style="text-align:right;"|לכן אם אתה רוצה להתקרב עוד אל האמת יש לך לכפול השרש ר"ל הק"א שלימים וו' שביעיות שלימות ולחלק אליהם אלו הו' שביעיות שביעית <s>לכן אם אתה רוצה להתקרב עוד אל האמת יש לך לכפול השרש ר"ל הק"א שלימים וו' שביעיות שלמות ולחלק אליהם אלו הו' שביעיות שביעית</s> והיוצא היה לך להוסיף אותו על השרש הקודם שהיה ק"א שלימים וו' שביעיות וכן לעולם | ||
|- | |- | ||
| Line 4,017: | Line 4,755: | ||
| | | | ||
:If it is less, do not add 1. | :If it is less, do not add 1. | ||
| − | |style="text-align:right;"|ואם פחות לא תוסיף א' | + | |style="text-align:right;"|‫<ref>35v</ref>ואם פחות לא תוסיף א‫' |
|- | |- | ||
| | | | ||
| Line 4,024: | Line 4,762: | ||
|- | |- | ||
| | | | ||
| − | :*<math>\scriptstyle{\color{ | + | :*<math>\scriptstyle{\color{OliveGreen}{b\ge a\longrightarrow\sqrt{a^2+b}\approx a+\frac{b}{2a+1}}}</math> |
| − | :*<math>\scriptstyle{\color{ | + | :*<math>\scriptstyle{\color{OliveGreen}{b<a\longrightarrow\sqrt{a^2+b}\approx a+\frac{b}{2a}}}</math> |
| | | | ||
|- | |- | ||
| Line 4,034: | Line 4,772: | ||
| | | | ||
:If the product is more than the first number, divide the excess by double the root and subtract [the quotient] from [the first approximate root]. | :If the product is more than the first number, divide the excess by double the root and subtract [the quotient] from [the first approximate root]. | ||
| − | |style="text-align:right;"|ואם יעלה יותר מהחשבון הראשון תחלק העודף ההוא על כפל השרש ותחסרנו ממנו | + | |style="text-align:right;"|ואם <s>יצא</s> יעלה יותר מהחשבון הראשון תחלק העודף ההוא על כפל השרש ותחסרנו ממנו |
| − | | | + | |} |
| − | + | ||
| − | :*<math>\scriptstyle{\color{ | + | :*<math>\scriptstyle{\color{OliveGreen}{\left(a+\frac{b}{2a}\right)^2>a^2+b\longrightarrow\sqrt{a^2+b}\approx\left(a+\frac{b}{2a}\right)-\frac{\left(a+\frac{b}{2a}\right)^2-\left(a^2+b\right)}{2\sdot\left(a+\frac{b}{2a}\right)}}}</math> |
| − | | | + | {| |
|- | |- | ||
| | | | ||
:If the product is less than the number, see by how much, divide [the excess] also by double the root, then add [the quotient] to [the first approximate] root. | :If the product is less than the number, see by how much, divide [the excess] also by double the root, then add [the quotient] to [the first approximate] root. | ||
| − | |style="text-align:right;"|ואם היה העולה פחות מהחשבון תראה בכמה הוא ותחלקנו לכפל השרש ג"כ ותוסיפנו על השרש הקודם | + | |style="width:45%; text-align:right;"|ואם היה העולה פחות מהחשבון תראה בכמה הוא ותחלקנו לכפל השרש ג"כ ותוסיפנו על השרש הקודם |
| − | | | + | |} |
| − | + | ||
| − | :*<math>\scriptstyle{\color{ | + | :*<math>\scriptstyle{\color{OliveGreen}{\left(a+\frac{b}{2a}\right)^2<a^2+b\longrightarrow\sqrt{a^2+b}\approx\left(a+\frac{b}{2a}\right)+\frac{\left(a^2+b\right)-\left(a+\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}}}</math> |
| − | | | + | {| |
|- | |- | ||
|And so on. | |And so on. | ||
| − | |style="text-align:right;"|וכן לעולם | + | |style="width:45%; text-align:right;"|וכן לעולם |
|- | |- | ||
|You come ever closer to the truth, but you will never attain it. | |You come ever closer to the truth, but you will never attain it. | ||
|style="text-align:right;"|ולעולם תתקרב יותר אל האמת ולא תשיגנה לעולם | |style="text-align:right;"|ולעולם תתקרב יותר אל האמת ולא תשיגנה לעולם | ||
|- | |- | ||
| − | !Shortcuts | + | !<span style=color:Green>Shortcuts</span> |
| | | | ||
|- | |- | ||
| Line 4,064: | Line 4,802: | ||
|- | |- | ||
| | | | ||
| − | *<span style=color: | + | *<span style=color:Green>The first approximation</span> |
| + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{a^2+b}\approx a+\frac{b}{2a+1}}}</math> | ||
| + | :<span style=color:Green>The error of the approximation</span> | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(a^2+b\right)-\left(a+\frac{b}{2a+1}\right)^2=\frac{b}{2a+1}\sdot\left(1-\frac{b}{2a+1}\right)}}</math> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
:If it is an addition [to the integer received through the extraction algorithm] and it was produced by adding 1 to twice the root [in the denominator], find the product of the fraction attained in this step by its complement [with respect to 1]. This product will be the deficit when you multiply the [approximate] root by itself, with respect to the initial number [whose root is extracted]. | :If it is an addition [to the integer received through the extraction algorithm] and it was produced by adding 1 to twice the root [in the denominator], find the product of the fraction attained in this step by its complement [with respect to 1]. This product will be the deficit when you multiply the [approximate] root by itself, with respect to the initial number [whose root is extracted]. | ||
| − | |||
| − | |||
| − | |||
|style="text-align:right;"|ואם היו לתוספת ונעשה בתוספת א' על כפל השרש<br> | |style="text-align:right;"|ואם היו לתוספת ונעשה בתוספת א' על כפל השרש<br> | ||
ראה כמה כפל השברים המתחדשים ההם בפעם ההיא במה שיש מהשברים ההם עד תשלום<br> | ראה כמה כפל השברים המתחדשים ההם בפעם ההיא במה שיש מהשברים ההם עד תשלום<br> | ||
| Line 4,074: | Line 4,815: | ||
|- | |- | ||
| | | | ||
| − | :<span style=color: | + | :<span style=color:Green>The second approximation</span> |
| + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{a^2+b}\approx\left(a+\frac{b}{2a+1}\right)+\frac{\frac{b}{2a+1}\sdot\left(1-\frac{b}{2a+1}\right)}{2\sdot\left(a+\frac{b}{2a+1}\right)}}}</math> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
:You should, therefore, divide it by double the [approximate] root itself and added to this root. | :You should, therefore, divide it by double the [approximate] root itself and added to this root. | ||
| − | |||
|style="text-align:right;"|והוא אשר יש לך לחלק עוד על כפל השרש בעצמו ולהוסיפו עליו | |style="text-align:right;"|והוא אשר יש לך לחלק עוד על כפל השרש בעצמו ולהוסיפו עליו | ||
|- | |- | ||
| Line 4,086: | Line 4,830: | ||
::Its complement [with respect to one] is one seventh, and when you multiply them by this complement the product is six sevenths of a seventh; this is indeed the deficit we found with respect to the original number [whose root was extracted], and so we instructed to divide it by double the [approximate] root and add [the result] to this root. | ::Its complement [with respect to one] is one seventh, and when you multiply them by this complement the product is six sevenths of a seventh; this is indeed the deficit we found with respect to the original number [whose root was extracted], and so we instructed to divide it by double the [approximate] root and add [the result] to this root. | ||
::<math>\scriptstyle{\color{blue}{10375-\left(101+\frac{6}{7}\right)^2=\frac{6}{7}\sdot\left(1-\frac{6}{7}\right)=\frac{6}{7}\sdot\frac{1}{7}}}</math> | ::<math>\scriptstyle{\color{blue}{10375-\left(101+\frac{6}{7}\right)^2=\frac{6}{7}\sdot\left(1-\frac{6}{7}\right)=\frac{6}{7}\sdot\frac{1}{7}}}</math> | ||
| − | |style="text-align:right;"|והנה {{#annot:term|598,1422|bcFr}}השלמתם לשלם{{#annotend:bcFr}} הוא שביעית אחת וכאשר תכפלם ב{{#annot:term|598,1422|xbp0}}השלמה{{#annotend:xbp0}} זו יעלה ו' שביעיות שביעית וזה בעצמו הוא שמצינו חסר בכפל השרש מהחשבון [הא'] וצוינו לחלקו לכפל השרש ולהוסיפו על השרש | + | |style="text-align:right;"|והנה {{#annot:term|598,1422|bcFr}}השלמתם לשלם{{#annotend:bcFr}} הוא שביעית אחת וכאשר תכפלם ב{{#annot:term|598,1422|xbp0}}השלמה{{#annotend:xbp0}} זו יעלה ו' שביעיות שביעית וזה בעצמו הוא שמצינו חסר בכפל השרש מהחשבון [הא']‫<ref>marg.</ref> וצוינו לחלקו לכפל השרש ולהוסיפו על השרש |
|- | |- | ||
| | | | ||
| − | *<span style=color: | + | *<span style=color:Green>The first approximation</span> |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{a^2+b}\approx a+\frac{b}{2a}}}</math> | |
| − | :<math>\scriptstyle\sqrt{a^2+b}\approx a+\frac{b}{2a}</math> | + | :<span style=color:Green>The error of the approximation</span> |
| − | :<span style=color: | + | :<math>\scriptstyle{\color{OliveGreen}{\left(a+\frac{b}{2a}\right)^2-\left(a^2+b\right)=\left(\frac{b}{2a}\right)^2}}</math> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :But if the additional [fraction] does not involve adding 1 [in the denominator] and falling short [of the initial number whose root is extracted], then we multiply the [additional] fraction by itself, and divide by double the [approximate] root, because this is the excess of the square of the [approximate] root over the original number [whose root is extracted]. | ||
|style="text-align:right;"|אך אם היה לתוספת בלי תוספת א' שהיו למגרעת<br> | |style="text-align:right;"|אך אם היה לתוספת בלי תוספת א' שהיו למגרעת<br> | ||
נראה כפל השברים אשר נתחדשו על עצמם ונחלקם לכפול השרש<br> | נראה כפל השברים אשר נתחדשו על עצמם ונחלקם לכפול השרש<br> | ||
| Line 4,098: | Line 4,846: | ||
|- | |- | ||
| | | | ||
| − | + | *<span style=color:Green>The second approximation</span> | |
| + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{a^2+b}\approx\left(a+\frac{b}{2a}\right)-\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}}}</math> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
:We subtract the result from the previous [approximate] root, and so on. | :We subtract the result from the previous [approximate] root, and so on. | ||
| − | |||
|style="text-align:right;"|והיוצא נחסרנו לעולם מהשרש הקודם וכן לעולם | |style="text-align:right;"|והיוצא נחסרנו לעולם מהשרש הקודם וכן לעולם | ||
|- | |- | ||
| − | |Therefore, if you wish to repeat the procedure in order to | + | |Therefore, if you wish to repeat the procedure in order to come closer to the truth, [do this], because the more you repeat, the nearer you come to the truth, even if you can never attain it, as we have explained. |
| − | |style="text-align:right;"|ולזה אם רצונך להכפל זה המעשה כדי להתקרב אל האמת כי כל מה שתוסיף להכפל זה הענין תוסיף להתקרב אל האמת ואם לא תשיגנה לעולם כמו שביארנו | + | |style="text-align:right;"|ולזה אם רצונך להכפל זה המעשה כדי להתקרב אל האמת כי כל מה שתוסיף להכפל זה הענין ‫<ref>36r</ref>תוסיף להתקרב אל האמת ואם לא תשיגנה לעולם כמו שביארנו |
|- | |- | ||
|[If you repeat the procedure], never add 1 to double the root, even if the remainder is very large with respect to the [approximate] root, so as to avoid confusion, for [adding 1] was instructed only for a single [approximation] step. | |[If you repeat the procedure], never add 1 to double the root, even if the remainder is very large with respect to the [approximate] root, so as to avoid confusion, for [adding 1] was instructed only for a single [approximation] step. | ||
| − | |style="text-align:right;"|לא תוסיף א' לעולם על כפל השרש [ואף אם יהיה הנשאר הרבה מאד על השורש] כדי שלא יבלבל עליך כי לא ציויתיו אלא למסתפק בפעם אחת | + | |style="text-align:right;"|לא תוסיף א' לעולם על כפל השרש [ואף אם יהיה הנשאר הרבה מאד על השורש]‫<ref>marg.</ref> כדי שלא יבלבל עליך כי לא ציויתיו אלא למסתפק בפעם אחת |
|- | |- | ||
| − | |Adding 1 [<math>\scriptstyle a+\frac{b}{2a+1}</math>] when the remainder is the same as the [approximate] root or greater, improves the approximation, as I explained, but if one repeats the procedure [<math>\scriptstyle a+\frac{b}{2a}</math>], one does not need this addition, because by repeating the procedure one approaches [the truth] very closely even without adding 1. It is better not to add it, so as to maintain a standard form of procedure and prevent confusion. | + | |Adding 1 [<math>\scriptstyle{\color{OliveGreen}{a+\frac{b}{2a+1}}}</math>] when the remainder is the same as the [approximate] root or greater, improves the approximation, as I explained, but if one repeats the procedure [<math>\scriptstyle{\color{OliveGreen}{a+\frac{b}{2a}}}</math>], one does not need this addition, because by repeating the procedure one approaches [the truth] very closely even without adding 1. It is better not to add it, so as to maintain a standard form of procedure and prevent confusion. |
| − | |style="text-align:right;"|ובתוספת הא' כשהנשאר כשרש או יותר הוא {{#annot:term| | + | |style="text-align:right;"|ובתוספת הא' כשהנשאר כשרש או יותר הוא {{#annot:term|1612,1874|05Nl}}מתקרב יותר{{#annotend:05Nl}} כמו שכתבתי<br> |
אבל המכפיל פעמי המעשה אינך צריך לתוספת זה<br> | אבל המכפיל פעמי המעשה אינך צריך לתוספת זה<br> | ||
כי בהכפל המעשה {{#annot:term|1612,1874|Kx0D}}יתקרב מאד מאד{{#annotend:Kx0D}} אף מבלי תוספת הא‫'<br> | כי בהכפל המעשה {{#annot:term|1612,1874|Kx0D}}יתקרב מאד מאד{{#annotend:Kx0D}} אף מבלי תוספת הא‫'<br> | ||
וטוב שלא נוסיפנו כדי שיהיה כל מעשהו בסגנון אחד ולא יתבלבל | וטוב שלא נוסיפנו כדי שיהיה כל מעשהו בסגנון אחד ולא יתבלבל | ||
| + | |- | ||
| + | !<span style=color:Green>The reason that if the remainder is smaller than the approximate root <math>\scriptstyle{\color{OliveGreen}{b<a}}</math>, it is divided by double the root <math>\scriptstyle{\color{OliveGreen}{\frac{b}{2a}}}</math>: | ||
| + | | | ||
|- | |- | ||
|The reason we say that if there is a remainder that is smaller than the [approximate] root, then we should divide it by double the root, is because that which is added to the root will add to the square its product by twice the previous root and its product by itself, as we explained with regard to integers. | |The reason we say that if there is a remainder that is smaller than the [approximate] root, then we should divide it by double the root, is because that which is added to the root will add to the square its product by twice the previous root and its product by itself, as we explained with regard to integers. | ||
| − | :<math>\scriptstyle\left(a+b\right)^2-a^2=2ab+b^2</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\left(a+b\right)^2-a^2=2ab+b^2}}</math> |
| − | |style="text-align:right;"|וטעם אומרנו שאם ישאר דבר והוא פחו' מהשרש שנחלקנו לכפל השרש<br> | + | |style="text-align:right;"|<big>וטעם</big> אומרנו שאם ישאר דבר והוא פחו' מהשרש שנחלקנו לכפל השרש<br> |
| − | הוא לפי שאש' {{#annot:term|1212, | + | הוא לפי שאש' {{#annot:term|1212,178|g91P}}יתוסף{{#annotend:g91P}} בשורש יוסיף במרובע כפלו בשורש הראשון פעמים גם כפלו בעצמו כאשר ביארנו בשלמים |
| + | |- | ||
| + | | | ||
| + | :*<span style=color:Green>The excess of the first approximation:</span> | ||
| + | ::<math>\scriptstyle{\color{OliveGreen}{\left(a+\frac{b}{2a}\right)^2\approx a^2+\left[2\sdot\left(a\sdot\frac{b}{2a}\right)\right]=a^2+b}}</math> | ||
| + | | | ||
|- | |- | ||
|But, we proceed as if it only adds its product by twice the root. If this were true, i.e. that what is added to the root adds to the square only the product of what is added to the root by twice the root, then we would have this product, which equals the excess of the number [whose root is extracted] over the square of the integer [received through the extraction algorithm]. | |But, we proceed as if it only adds its product by twice the root. If this were true, i.e. that what is added to the root adds to the square only the product of what is added to the root by twice the root, then we would have this product, which equals the excess of the number [whose root is extracted] over the square of the integer [received through the extraction algorithm]. | ||
| − | |||
| − | |||
|style="text-align:right;"|ואנו עושים מעשינו כאלו אינו מוסיף כי אם כפלו בשרש פעמים<br> | |style="text-align:right;"|ואנו עושים מעשינו כאלו אינו מוסיף כי אם כפלו בשרש פעמים<br> | ||
ואם היה זה האמת ר"ל שהמתוסף על השרש לא היה מוסיף על המרובע כי אם כפל זה המתוסף בכפל השרש לבד<br> | ואם היה זה האמת ר"ל שהמתוסף על השרש לא היה מוסיף על המרובע כי אם כפל זה המתוסף בכפל השרש לבד<br> | ||
הנה היה בידינו המספר העולה מהכפל הזה והוא השארית הנזכרת שהיא נוספת בחשבון על מרובע השלימים | הנה היה בידינו המספר העולה מהכפל הזה והוא השארית הנזכרת שהיא נוספת בחשבון על מרובע השלימים | ||
|- | |- | ||
| − | | | + | |Hence, when we add in the root what is equal to its product by twice the root, as this excess itself, we reach the required result. |
| − | |||
| − | |||
|style="text-align:right;"|וכאשר נוסיף בשרש דבר מה שיהיה שוה כפלו בכפל השרש כזה התוספת בעצמו הגענו אל מבוקשנו | |style="text-align:right;"|וכאשר נוסיף בשרש דבר מה שיהיה שוה כפלו בכפל השרש כזה התוספת בעצמו הגענו אל מבוקשנו | ||
| + | |- | ||
| + | |Altough this addition is unknown to us, as we know the result of the multiplication, which is the mentioned remainder, and we also know one of the multiplicands, which is double the root, when we divide the product by double the root, the result is the unknown, which is the addition, i.e. by multiplying this addition by double the root, the result is the mentioned remainder and this is clear. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{2a\sdot\frac{b}{2a}=b}}</math> | ||
| + | |style="text-align:right;"|ועם היות שנעלם ממנו תוספת זה ומ"מ אחר שידענו העולה מהכפל ההוא והיא השארית הנזכרת גם ידענו אחד מהנכפלים והוא כפל השרש [הנה בחלקנו זה העולה לכפל השורש יצא]‫<ref>marg.</ref> יצא הנעלם שהוא התוספת ר"ל כי בכפול זה התוספת בכפול השרש יעלה כנשאר הנזכר וזה ברור | ||
| + | |- | ||
| + | |Since, that which is added to the root, adds also to the square its product by itself, i.e. the product of this addition by itself. | ||
| + | |style="text-align:right;"|אכן לפי שהמתוסף על השרש מוסיף עוד במרובע כפלו בעצמו ר"ל כפל התוספת הזה בעצמו | ||
| + | |- | ||
| + | |Therefore, when we multiply the root by itself after this addition is added to it, the square will exceed the initial number [whose root is extracted] by the square of the addition. This is what we explained above. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(a+\frac{b}{2a}\right)^2-\left(a^2+b\right)=\left(\frac{b}{2a}\right)^2}}</math> | ||
| + | |style="text-align:right;"|לכן כאשר נכפול השרש בעצמו אחר הוסיף ‫<ref>36v</ref>עליו זה התוספת יעלה המרובע מוסף על החשבון הראשון כפל התוספת הזה בעצמו וכן ביארנוהו למעלה | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:Green>The second approximation</span> | |
| + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{a^2+b}\approx\left(a+\frac{b}{2a}\right)-\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}}}</math> | ||
| + | | | ||
|- | |- | ||
| − | | | + | | |
| − | + | :If we want to come even closer, we divide this addition by double the root and the result is subtracted from the [approximate] root, as we explained. | |
| − | + | |style="text-align:right;"|ואם היינו רוצים להתקרב עוד ואנו מחלקים זה התוספת לכפל השרש הזה והיוצא {{#annot:term|181,2030|t72G}}יחסר{{#annotend:t72G}} מזה השרש כאשר ביארנו למעלה | |
| − | |||
| − | |||
| − | |||
| − | |style="text-align:right;"|ואם היינו רוצים להתקרב עוד ואנו מחלקים זה התוספת לכפל השרש הזה והיוצא {{#annot:term| | ||
|} | |} | ||
| − | :*The excess of the second approximation: | + | :*<span style=color:Green>The excess of the second approximation:</span> |
| − | ::<math>\scriptstyle\left(a+\frac{b}{2a}\right)^2-\left[\left(a+\frac{b}{2a}\right)-\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\right]^2=\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\sdot\left[\left[2\sdot\left(a+\frac{b}{2a}\right)\right] | + | ::<math>\scriptstyle{\color{OliveGreen}{\left(a+\frac{b}{2a}\right)^2-\left[\left(a+\frac{b}{2a}\right)-\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\right]^2=\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\sdot\left[2\sdot\left[\left(a+\frac{b}{2a}\right)-\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\right]\right]+\left[\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\right]^2}}</math> |
{| | {| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|הנה זה שאנו מחסרים היה מוסיף על המרובע ככפלו על כפל השרש {{#annot:term|789,1674|MPXZ}}המחוסר{{#annotend:MPXZ}} הזה לאחר חסרונו וכפלו על עצמו בלי כפל | + | :[The square of the approximate root] from which we subtract exceeds over the square [of the second approximate root] by the product [of the subtracted fraction] by double the subtracted [approximate] root after the subtraction and its product by itself. |
| + | |style="width:45%; text-align:right;"|הנה זה שאנו מחסרים היה מוסיף על המרובע ככפלו על כפל השרש {{#annot:term|789,1674|MPXZ}}המחוסר{{#annotend:MPXZ}} הזה לאחר חסרונו וכפלו על עצמו בלי כפל | ||
| + | |- | ||
| + | | | ||
| + | :We divide the addition that we have by double the [approximate root] before subtracting from it, and it is as if we divide it by double the subtracted root plus double the subtracted [fraction]. | ||
| + | ::<math>\scriptstyle{\color{OliveGreen}{\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}=\frac{\left(\frac{b}{2a}\right)^2}{\left[2\sdot\left[\left(a+\frac{b}{2a}\right)-\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\right]\right]+\left[2\sdot\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\right]}}}</math> | ||
| + | |style="text-align:right;"|ואולם התוספת אשר היה לנו חלקנוהו על כפל כל השרש <s>טרם</s> <sup>טרם</sup> {{#annot:term|181,2030|oVAO}}החסרו{{#annotend:oVAO}} והוא כמו שחלקנוהו על כפל השרש הזה המחוסר ועל כפל החסרון <sup>זה</sup> | ||
|- | |- | ||
| | | | ||
| − | : | + | :But, [The square of the first approximate root] exceeds [over the square of the second approximate root] by the product [of the subtracted fraction] by double the subtracted [approximate] root plus its product by itself. |
| − | |style="text-align:right;"|ואולם | + | |style="text-align:right;"|ואולם הוא לא היה מוסיף כי אם כפלו על כפל השרש הזה המחוס' וכפלו על עצמו בלי כפל |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle\left[\left(a+\frac{b}{2a}\right)-\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\right]^2-\left(a^2+b\right)=\left[\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\right]^2</math> | + | :We find that we did not subtract all that is needed, as the square of the subtracted [approximate root] exceeds over the original number by the product of the subtracted [fraction] by itself. This is clear and is always so. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{OliveGreen}{\left[\left(a+\frac{b}{2a}\right)-\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\right]^2-\left(a^2+b\right)=\left[\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\right]^2}}</math> |
| + | |style="text-align:right;"|נמצא שלא חסרנו בכל הצורך אבל עוד ישאר במרובע זה השרש המחוסר תוספת על החשבון הראשון ככפל זה החסרון על עצמו וזה ברור וכן יהיה לעולם | ||
|- | |- | ||
| − | |So, when we do not add 1 [to the denominator], and wish to | + | |So, when we do not add 1 [to the denominator], and wish to come closer to the truth [using a repetitive procedure for extracting the root], [in the first step] we should only add the fraction of the first step [i.e., the remainder divided by twice the approximate root]. |
|style="text-align:right;"|לכן כאשר לא נוסיף א' ונרצה להתקרב אל האמת אין לנו להוסיף על השרש כי אם השברים הראשונים אשר נתחדשו בפעם הראשון מאשר נשאר לנו | |style="text-align:right;"|לכן כאשר לא נוסיף א' ונרצה להתקרב אל האמת אין לנו להוסיף על השרש כי אם השברים הראשונים אשר נתחדשו בפעם הראשון מאשר נשאר לנו | ||
|- | |- | ||
| Line 4,165: | Line 4,936: | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle\sqrt{7}</math> | + | :*{{#annot:√7|439|TblT}}Example: we seek the root of 7. |
| − | |style="text-align:right;"|המשל בקשנו שרש ז‫' | + | ::<math>\scriptstyle\sqrt{7}</math> |
| + | |style="text-align:right;"|<big>המשל</big> בקשנו שרש ז‫'{{#annotend:TblT}} | ||
|- | |- | ||
| | | | ||
| − | : | + | ::The integer that is in its root is 2 and 3 remains. |
| − | |style="text-align:right;"|הנה השלמי' אשר בשרשו הם ב' ונשארו ג‫' | + | |style="text-align:right;"|הנה השלמי' אשר בשרשו הם ב' ונשארו ג‫' |
| − | |||
|- | |- | ||
| | | | ||
| + | ::If we divide it by double the [approximate] root, the quotient is 3-quarters. | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{7}\approx2+\frac{7-4}{2\sdot2}=2+\frac{3}{4}}}</math> | ||
| + | |style="text-align:right;"|ואם חלקנום לכפל השרש יצא בחילוק ג' רביעיות | ||
| + | |- | ||
| + | | | ||
| + | ::When we add this addition to the 2 integers and consider the whole sum as a root, its square exceeds over the square of 2, which is 4 integers, by the product of these three-quarters by themselves. | ||
:::<math>\scriptstyle{\color{blue}{\left(2+\frac{3}{4}\right)^2-2^2=3+\left(\frac{3}{4}\right)^2}}</math> | :::<math>\scriptstyle{\color{blue}{\left(2+\frac{3}{4}\right)^2-2^2=3+\left(\frac{3}{4}\right)^2}}</math> | ||
|style="text-align:right;"|והנה זה התוספת כאשר נחברהו אל הב' השלמים ונעשה מהכל שרש אחת הנה יתוסף במרובעו יותר על מרובע הב שיהיה ד' שלמים ככפל שלש רביעיות אלו בעצמם | |style="text-align:right;"|והנה זה התוספת כאשר נחברהו אל הב' השלמים ונעשה מהכל שרש אחת הנה יתוסף במרובעו יותר על מרובע הב שיהיה ד' שלמים ככפל שלש רביעיות אלו בעצמם | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{3=4\sdot\frac{3}{4}=2^2\sdot\frac{3}{4}}}</math> | + | ::But, our remainder is as the product of 3-quarters by 4 integers, which are double the first [approximate] root. |
| − | |style="text-align:right;"|ואולם שאריתנו לא היה כי אם ככפל הג' רביעיות בד' השלמים אשר הם כפל השרש הראשון | + | :::<math>\scriptstyle{\color{blue}{7-2^2=3=4\sdot\frac{3}{4}=2^2\sdot\frac{3}{4}}}</math> |
| + | |style="text-align:right;"|ואולם ‫<ref>37r</ref>שאריתנו לא היה כי אם ככפל הג' רביעיות בד' השלמים אשר הם כפל השרש הראשון | ||
|- | |- | ||
| | | | ||
| + | ::We find that too many fractions are added to our root, so that the square of the sum exceeds the 7 integers by the product of 3-quarters by themselves, which is 9-quarters of a quarter that are 2-quarters and a quarter of a quarter. This is clear, because the product of 2 and 3-quarters [by itself] is 7 integers and [9]-quarters of a quarter, as will be explained in chapter three of section two. | ||
:::<math>\scriptstyle{\color{blue}{\left(2+\frac{3}{4}\right)^2-7=\left[7+\left(\frac{3}{4}\right)^2\right]-7=\left(\frac{3}{4}\right)^2=\frac{9}{4}\sdot\frac{1}{4}=\frac{2}{4}+\left(\frac{1}{4}\sdot\frac{1}{4}\right)}}</math> | :::<math>\scriptstyle{\color{blue}{\left(2+\frac{3}{4}\right)^2-7=\left[7+\left(\frac{3}{4}\right)^2\right]-7=\left(\frac{3}{4}\right)^2=\frac{9}{4}\sdot\frac{1}{4}=\frac{2}{4}+\left(\frac{1}{4}\sdot\frac{1}{4}\right)}}</math> | ||
| − | |style="text-align:right;"|נמצא ש{{#annot:term|2510, | + | |style="text-align:right;"|נמצא ש{{#annot:term|2510,178|mjLV}}נתוספו{{#annotend:mjLV}} בשרשנו זה שברים יותר מדאי עד שמרובע הכל יהיה יותר על הז' שלמים ככפל הג' רביעיות בעצמם שהם ט' רביעיות רביעית שהם ב' רביעיות שלמות ורביעית רביעית<br> |
וזה ברור כי כפל ב' וג' רביעיות עולה ז' שלמים וב' רביעיות רביעית כאשר יתבאר בחלק הב' בפרק הג' ממנו | וזה ברור כי כפל ב' וג' רביעיות עולה ז' שלמים וב' רביעיות רביעית כאשר יתבאר בחלק הב' בפרק הג' ממנו | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Therefore, we should divide this excess by double the root as we explained. |
| − | |style="text-align:right;"|ולזה ראוי לנו לחלק תוספת זה על כפל השרש כאשר ביארנו. והנה כפל השרש הוא ה' שלמים וב' רביעיות שהם חצי שלם | + | |style="text-align:right;"|ולזה ראוי לנו לחלק תוספת זה על כפל השרש כאשר ביארנו |
| − | וכאשר נחלק עליהם ב' רביעיות ורביעית רביעית יצא בחילוק ט' חלקים מי"א מחצי רביעית | + | |- |
| − | + | | | |
| + | ::Double the root is 5 integers and 2-quarters, which are one-half. | ||
| + | |style="text-align:right;"|והנה כפל השרש הוא ה' שלמים וב' רביעיות שהם חצי שלם | ||
| + | |- | ||
| + | | | ||
| + | ::When we divide 2-quarters and a quarter of a quarter by it, the quotient is 9 parts of 11 of half a quarter. | ||
| + | |style="text-align:right;"|וכאשר נחלק עליהם ב' רביעיות ורביעית רביעית יצא בחילוק ט' חלקים מי"א מחצי רביעית | ||
|- | |- | ||
| + | | | ||
| + | ::When we subtract it from the former root, the remainder is 2 integers, 2-quarters, half a quarter, and 2 parts of 11 of half a quarter. The procedure of all this is explained in section two. | ||
| + | |style="text-align:right;"|וכאשר נסירם מהשרש הקודם ישאר ב' שלמים וב' רביעיות שלמות וחצי רביעית וב' חלקים מי"א מחצי רביעית וכל זה יתבאר מעשהו בחלק הב‫' | ||
|} | |} | ||
| − | + | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\sqrt{7}&\scriptstyle\approx\left(2+\frac{3}{4}\right)-\frac{\frac{2}{4}+\left(\frac{1}{4}\sdot\frac{1}{4}\right)}{2\sdot\left(2+\frac{3}{4}\right)}=\left(2+\frac{3}{4}\right)-\frac{\frac{2}{4}+\left(\frac{1}{4}\sdot\frac{1}{4}\right)}{5+\frac{2}{4}}=\left(2+\frac{3}{4}\right)-\frac{\frac{2}{4}+\left(\frac{1}{4}\sdot\frac{1}{4}\right)}{5+\frac{1}{2}}=\left(2+\frac{3}{4}\right)-\left(\frac{9}{11}\sdot\frac{1}{2}\sdot\frac{1}{4}\right)\\&\scriptstyle=2+\frac{2}{4}+\left(\frac{1}{2}\sdot\frac{1}{4}\right)+\left(\frac{2}{11}\sdot\frac{1}{2}\sdot\frac{1}{4}\right)\\\end{align}}}</math> | |
{| | {| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|והנה {{#annot:term| | + | ::The square of this [approximate] root. i.e. the 2 integers, 2-quarters, half a quarter, and 2 parts of 11 of half a quarter is less than the [square of the] former [approximate root] by the product of this remainder, i.e. 9 parts of 11 of half a quarter by double the subtracted root plus its product by itself. |
| + | |style="width:45%; text-align:right;"|והנה {{#annot:term|181,2030|zdQE}}יחסר{{#annotend:zdQE}} מרובע השרש הזה ר"ל הב' שלמים וב' רביעיות וחצי רביעית וב' חלקים מי"א מחצי רביעית אחרי {{#annot:term|181,2030|RhZG}}החסרו מ{{#annotend:RhZG}}אשר לפניו ככפל החסרון הזה ר"ל הט' חלקים מי"א מחצי רביעית על כפל השרש המחוסר וככפלו לעצמו | ||
| + | |} | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(2+\frac{3}{4}\right)^2-\left[2+\frac{2}{4}+\left(\frac{1}{2}\sdot\frac{1}{4}\right)+\left(\frac{2}{11}\sdot\frac{1}{2}\sdot\frac{1}{4}\right)\right]^2=\left[\left(\frac{9}{11}\sdot\frac{1}{2}\sdot\frac{1}{4}\right)\sdot\left[2\sdot\left[2+\frac{2}{4}+\left(\frac{1}{2}\sdot\frac{1}{4}\right)+\left(\frac{2}{11}\sdot\frac{1}{2}\sdot\frac{1}{4}\right)\right]\right]\right]+\left(\frac{9}{11}\sdot\frac{1}{2}\sdot\frac{1}{4}\right)^2}}</math> | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ואולם התוספת הראשו' אשר היה במרובע על החשבון היה ככפל החסרון זה בכפל השרש הראשון ר"ל בכפל השרש הזה המחוסר ובכפל החסרון הזה שהרי כאשר חלקנו התוספת על כפל השרש הקודם ר"ל על כפל השרש המחוסר ועל כפל זה החסרון [יצא בחלוק זה החסרון] | + | ::But, the first excess of the square over the [given] number is as the product of this remainder by double the first [approximate] root, i.e. by double this subtracted root and by double this remainder, since, when we divide the addition by double the former [approximate] root, i.e. by double the subtracted root and by double this remainder, the result of division is this remainder. |
| + | |style="width:45%; text-align:right;"|ואולם התוספת הראשו' אשר היה במרובע על החשבון היה ככפל החסרון זה בכפל השרש הראשון ר"ל בכפל השרש הזה המחוסר ובכפל החסרון הזה שהרי כאשר חלקנו התוספת על כפל השרש הקודם ר"ל על כפל השרש המחוסר ועל כפל זה החסרון [יצא בחלוק זה החסרון]‫<ref>marg.</ref> | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{\begin{align} | + | ::We find that the product of this remainder, i.e. 9 parts of 11 of half a quarter, by double the first [approximate] root, which is 2 integers and 3-quarters, whose double is 5 integers and a half, which is the same as double this subtracted root, i.e. the 2 integers, 2-quarters, [half a quarter], and 2 parts of 11 of half a quarter plus double the remainder, which is 9 parts of 11 of half a quarter, is as the excess we have, which is the 2-quarters and a quarter of a quarter, by which we have divided. |
| − | | | + | |style="text-align:right;"|נמצא שכפל זה החסרון ר"ל הט' חלקים מי"א מחצי רביעית בכפל השרש הראשון שהוא ב' שלמים וג' רביעיות שכפלו ה' שלימים וחצי שהוא כמו כפל ‫<ref>37v</ref>השרש המחוסר הזה ר"ל הב' שלימים וב' רביעיות וב' חלקים מי"א מחצי רביעית וכפל זה החסרון שהוא הט' חלקים מי"א מחצי רביעית <s>וכפל זה החסרון שהוא הט' חלקים מי"א</s> הוא כמו התוספת אשר היה לנו שהוא הב' רביעיות ורביעית רביעית שחלקנו עליהם |
| + | |} | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left(\frac{9}{11}\sdot\frac{1}{2}\sdot\frac{1}{4}\right)\sdot\left[2\sdot\left(2+\frac{3}{4}\right)\right]&\scriptstyle=\left(\frac{9}{11}\sdot\frac{1}{2}\sdot\frac{1}{4}\right)\sdot\left(5+\frac{1}{2}\right)=\left(\frac{9}{11}\sdot\frac{1}{2}\sdot\frac{1}{4}\right)\sdot\left[\left[2\sdot\left[2+\frac{2}{4}+\left(\frac{1}{2}\sdot\frac{1}{4}\right)\left(\frac{2}{11}\sdot\frac{1}{2}\sdot\frac{1}{4}\right)\right]\right]+\left[2\sdot\left(\frac{9}{11}\sdot\frac{1}{2}\sdot\frac{1}{4}\right)\right]\right]\\&\scriptstyle=\frac{2}{4}+\left(\frac{1}{4}\sdot\frac{1}{4}\right)\\\end{align}}}</math> | ||
| + | {| | ||
|- | |- | ||
| − | |<math>\scriptstyle a\sdot\left(b_1+b_2+\ldots+b_n\right)=\left(a\sdot b_1\right)+\left(a\sdot b_2\right)+\ldots+\left(a\sdot b_n\right)</math> | + | |It is known with little intelligence that the product of a number by a number is as its product by all the parts of the other number, each one by itself. |
| − | |style="text-align:right;"|כי ידוע הוא במעט התבוניות כי כפל מספר על מספר הוא ככפלו בכל חלקי המספר האחד כל אחד בפני עצמו והוא הטעם שכפל מספר ידוע על כפל מספר ידוע אחר | + | :<math>\scriptstyle{\color{OliveGreen}{a\sdot\left(b_1+b_2+\ldots+b_n\right)=\left(a\sdot b_1\right)+\left(a\sdot b_2\right)+\ldots+\left(a\sdot b_n\right)}}</math> |
| + | |style="width:45%; text-align:right;"|כי ידוע הוא במעט התבוניות כי כפל מספר על מספר הוא ככפלו בכל חלקי המספר האחד כל אחד בפני עצמו | ||
| + | |- | ||
| + | |This is the reason of the product of a known number by the double of another number. | ||
| + | |style="text-align:right;"|והוא הטעם שכפל מספר ידוע על כפל מספר ידוע אחר | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{ | + | ::Example: the product by the double of 4 is as its product by double all its parts, each one by itself, for instance, by the double of 3 and by the double of 1. |
| − | |style="text-align:right;"|המשל על כפל ד' הוא ככפלו על כפל כל חלקיו כל אחד בפני עצמו<br> | + | :::<math>\scriptstyle{\color{blue}{a\times\left(2\sdot4\right)=a\sdot\left(2\sdot3\right)+a\sdot\left(2\sdot1\right)}}</math> |
| − | המשל על כפל ג' ועל כפל א‫' | + | |style="text-align:right;"|<big>המשל</big> על כפל ד' הוא ככפלו על כפל כל חלקיו כל אחד בפני עצמו<br> |
| + | <big>המשל</big> על כפל ג' ועל כפל א‫' | ||
|- | |- | ||
| | | | ||
| − | ::: | + | ::This is as our saying: the product of 9 parts of 11 of half a quarter by double the first [approximate] root, which is 2 integers and 3-quarters, is as its product by all of its parts, each one by itself, i.e. by double the subtracted root and by double the remainder that are the parts of the former [approximate] root. This is clear. |
|style="text-align:right;"|וזהו כאומרנו שכפל הט' חלקים מי"א מחצי רביעית בכפל השרש הראשון שהוא הב' שלמים וג' רביעיות הוא כמו כפלו בכל חלקיו כל אחד בפני עצמו ר"ל בכפל השרש המחוסר ובכפל החסרון שהם חלקי השרש הקודם וזה ברור | |style="text-align:right;"|וזהו כאומרנו שכפל הט' חלקים מי"א מחצי רביעית בכפל השרש הראשון שהוא הב' שלמים וג' רביעיות הוא כמו כפלו בכל חלקיו כל אחד בפני עצמו ר"ל בכפל השרש המחוסר ובכפל החסרון שהם חלקי השרש הקודם וזה ברור | ||
| + | |} | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{9}{11}\sdot\frac{1}{2}\sdot\frac{1}{4}\right)\sdot\left[2\sdot\left(2+\frac{3}{4}\right)\right]=\left(\frac{9}{11}\sdot\frac{1}{2}\sdot\frac{1}{4}\right)\sdot\left[\left[2\sdot\left[2+\frac{2}{4}+\left(\frac{1}{2}\sdot\frac{1}{4}\right)\left(\frac{2}{11}\sdot\frac{1}{2}\sdot\frac{1}{4}\right)\right]\right]+\left[2\sdot\left(\frac{9}{11}\sdot\frac{1}{2}\sdot\frac{1}{4}\right)\right]\right]}}</math> | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | :*<math>\scriptstyle\left(a+\frac{b}{2a}\right)^2-\left(a^2+b\right)=\left(\frac{b}{2a}\right)^2=\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\sdot\left[2\sdot\left(a+\frac{b}{2a}\right)\right]</math> | + | :*<math>\scriptstyle{\color{OliveGreen}{\left(a+\frac{b}{2a}\right)^2-\left(a^2+b\right)=\left(\frac{b}{2a}\right)^2=\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\sdot\left[2\sdot\left(a+\frac{b}{2a}\right)\right]}}</math> |
| − | |style="text-align:right;"|ואחר שהמרובע הקודם היה מוסיף על החשבון אשר רצינו לידע שרשו ככפל החסרון על כפל כל השורש הקודם שהרי כשחלקנו אותו על כפל השורש הקודם [יצא זה החסרון הנה כאשר נכפול זה החסרון בכפל השורש הקודם] שהוא כפל היוצא בחילוק במספר אשר חלקנו עליו יעלה כמספר המתחלק שהוא התוספת שהיה לנו | + | | |
| + | |- | ||
| + | | | ||
| + | :Since the former [approximate] root exceeds over the number, whose root we wish to know, by the product of the subtracted [fraction] by double the whole former [approximate] root, as when we divide it by double the former [approximate] root, the result is this excess. Therefore, when we multiply this subtracted [fraction] by double the former [approximate] root, which is the product of the quotient by the divisor, the result is the dividend, which is the excess that we have. | ||
| + | |style="width:45%; text-align:right;"|ואחר שהמרובע הקודם היה מוסיף על החשבון אשר רצינו לידע שרשו ככפל החסרון על כפל כל השורש הקודם שהרי כשחלקנו אותו על כפל השורש הקודם [יצא זה החסרון הנה כאשר נכפול זה החסרון בכפל השורש הקודם]‫<ref>marg.</ref> שהוא כפל היוצא בחילוק במספר אשר חלקנו עליו יעלה כמספר המתחלק שהוא התוספת שהיה לנו | ||
|- | |- | ||
|} | |} | ||
| − | :*<math>\scriptstyle\left(a+\frac{b}{2a}\right)^2-\left[\left(a+\frac{b}{2a}\right)-\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\right]^2=\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\sdot\left[\left[2\sdot\left(a+\frac{b}{2a}\right)\right]-\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\right]</math> | + | :*<math>\scriptstyle{\color{OliveGreen}{\left(a+\frac{b}{2a}\right)^2-\left[\left(a+\frac{b}{2a}\right)-\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\right]^2=\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\sdot\left[\left[2\sdot\left(a+\frac{b}{2a}\right)\right]-\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\right]}}</math> |
{| | {| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ואולם בשביל זה החסרון אשר אנו מחסרים עתה מהשרש לא יחסר המרובע הזה מהראשון כי בכפל זה החסרון בכפל המחוסר ובעצמו בלי כפל | + | :But, because of the subtracted [fraction] that we subtract from the [former approximate] root, the square [of the second approximate root] is less that [the square of] the first [approximate root] only by the product of the subtracted [fraction] by double the subtracted [approximate root] plus its product by itself. |
| + | |style="width:45%; text-align:right;"|ואולם בשביל זה החסרון אשר אנו מחסרים עתה מהשרש לא יחסר המרובע הזה מהראשון כי בכפל זה החסרון בכפל המחוסר ובעצמו בלי כפל | ||
|- | |- | ||
| | | | ||
| − | :*<math>\scriptstyle\left[\left(a+\frac{b}{2a}\right)-\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\right]^2-\left(a^2+b\right)=\left[\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\right]^2</math> | + | :*<math>\scriptstyle{\color{OliveGreen}{\left[\left(a+\frac{b}{2a}\right)-\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\right]^2-\left(a^2+b\right)=\left[\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\right]^2}}</math> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Hence, the remainder [of the second approximate root] is the product of the subtracted [fraction] by itself. | ||
|style="text-align:right;"|א"כ ישאר עוד מהתוספת כפל זה החסרון בעצמו | |style="text-align:right;"|א"כ ישאר עוד מהתוספת כפל זה החסרון בעצמו | ||
|- | |- | ||
| − | | | + | |Thus, we have explained that when we repeat this [procedure] a few times, the excess of the square [of the approximate root over the given number] is always the square of the fraction resulting from the division at that phase, that we have to add to the [approximate] root in the first phase, or to subtract from the [approximate] root in all other phases. This is provided that we do it without adding one, i.e. if we do not add one to double the [approximate] root, by which we divide [the excess], when the excess is greater than the [approximate] root, but we divide the excess only by double the [approximate] root without adding one at all. |
| − | |style="text-align:right;"|הנה ביארנו כי בעשותינו זה כמה פעמים לעולם ישאר במרובע תוספת מרובע השברים שיצאו בחילוק בעת ההיא שהם אשר עלינו להוסיף על השרש במעשה הראשון או לחסרו מן השרש בשאר הפעמים כלם אם לא נעשנו בתוספת אחד ר"ל אם לא נוסיף אחד על כפל השרש לחלק על הכל אם יהיה התוספת גדול מהשרש אלא שנחלק התוספת על כפל השרש לבד בלי תוספת אחד כלל | + | |style="text-align:right;"|הנה ביארנו כי בעשותינו זה כמה פעמים לעולם ישאר במרובע תוספת מרובע השברים שיצאו בחילוק בעת ההיא שהם אשר עלינו להוסיף על השרש ‫<ref>38r</ref>במעשה הראשון או לחסרו מן השרש בשאר הפעמים כלם אם לא נעשנו בתוספת אחד ר"ל אם לא נוסיף אחד על כפל השרש לחלק על הכל אם יהיה התוספת גדול מהשרש אלא שנחלק התוספת על כפל השרש לבד בלי תוספת אחד כלל |
| + | |- | ||
| + | |This is the reason that we say that when we do not add one, we always take the square of the additional or subtracted fraction resulting the last phase, divide it by double the [approximate] root, then subtract the quotient from the [approximate] root. The reason of this procedure is clear. | ||
| + | |style="text-align:right;"|ולזה אמרנו כי כאשר לא נעשה בתוספת אחד לעולם נקח מרובע השברים אשר יצאו בפעם האחרונה הן לתוספת או למגרעת ונחלקם על כפל השרש המחוסר והיוצא נחסרנו מהשרש וכן נעשה <sup>לעולם</sup> וכל זה ברור בטעם | ||
|- | |- | ||
| − | + | !<span style=color:Green>The reason that if the remainder is equal to the approximate root or greater than it <math>\scriptstyle{\color{OliveGreen}{b\ge a}}</math>, it is divided by double the root plus one <math>\scriptstyle{\color{OliveGreen}{\frac{b}{2a+1}}}</math>: | |
| − | |||
|- | |- | ||
|The reason we say that when the remainder equals to the [approximate] root or greater than it, we should divide it by double the root plus one, as long as we do not intend to repeat the procedure so as to further approach the truth except for this [step] only, is that if we had not added one, the square of the root consisting of the integer and fraction would exceed the number [whose root is extracted] by the square of the fraction received in the division. But this would be a quarter or more. | |The reason we say that when the remainder equals to the [approximate] root or greater than it, we should divide it by double the root plus one, as long as we do not intend to repeat the procedure so as to further approach the truth except for this [step] only, is that if we had not added one, the square of the root consisting of the integer and fraction would exceed the number [whose root is extracted] by the square of the fraction received in the division. But this would be a quarter or more. | ||
| − | + | |style="text-align:right;"|<big>וטעם</big> אומרנו כי כאשר הנשאר <sup>הוא כשורש</sup> או יותר ממנו שיש לנו לחלקו על כפל השרש בתוספת אחד<br> | |
| − | |||
| − | |style="text-align:right;"|וטעם אומרנו כי כאשר הנשאר הוא כשורש או יותר ממנו שיש לנו לחלקו על כפל השרש בתוספת אחד<br> | ||
אם אין דעתינו להכפיל המעשה להתקרב עוד אל האמת זולתי בפעם הזאת לבד<br> | אם אין דעתינו להכפיל המעשה להתקרב עוד אל האמת זולתי בפעם הזאת לבד<br> | ||
| − | הוא לפי שאם לא היינו מוסיפים אחד היה מרובע השרש {{#annot:term| | + | הוא לפי שאם לא היינו מוסיפים אחד היה מרובע השרש {{#annot:term|178,1841|6Saf}}המקובץ מ{{#annotend:6Saf}}השלמים והשברים עודף על החשבון ככפל השברים אשר יצאו בחילוק וזה יהיה רביעית אחת או יותר |
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{b\ge a\longrightarrow\sqrt{a^2+b}\approx a+\frac{b}{2a+1}}}</math> | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{b\ge a\longrightarrow\left(a+\frac{b}{2a}\right)^2-\left(a^2+b\right)=\left(\frac{b}{2a}\right)^2\ge\frac{1}{4}}}</math> | ||
| + | | | ||
|- | |- | ||
| | | | ||
::For, if [the remainder] is equal to the root itself, and we divide it by double the root, the result of division will be a half. Its square, i.e. its product by itself, which is the excess, will then be an entire quarter. | ::For, if [the remainder] is equal to the root itself, and we divide it by double the root, the result of division will be a half. Its square, i.e. its product by itself, which is the excess, will then be an entire quarter. | ||
| − | ::<math>\scriptstyle b=a\longrightarrow\left(\frac{b}{2a}\right)^2=\left(\frac{a}{2a}\right)^2=\left(\frac{1}{2}\right)^2=\frac{1}{4}</math> | + | ::<math>\scriptstyle{\color{OliveGreen}{b=a\longrightarrow\left(\frac{b}{2a}\right)^2=\left(\frac{a}{2a}\right)^2=\left(\frac{1}{2}\right)^2=\frac{1}{4}}}</math> |
|style="text-align:right;"|לפי שאם יהיה כשורש בעינו ונחלקנו על כפל השרש יצא בחלוק חצי ומרובעו ר"ל כפלו בעצמו שהוא התוספת שיהיה רביעית שלמה | |style="text-align:right;"|לפי שאם יהיה כשורש בעינו ונחלקנו על כפל השרש יצא בחלוק חצי ומרובעו ר"ל כפלו בעצמו שהוא התוספת שיהיה רביעית שלמה | ||
|- | |- | ||
| | | | ||
::And if the remainder is greater than the root, when we divide it by double the root, the result will be more than a half, and its square more than a quarter. | ::And if the remainder is greater than the root, when we divide it by double the root, the result will be more than a half, and its square more than a quarter. | ||
| − | ::<math>\scriptstyle b>a\longrightarrow\left(\frac{b}{2a}\right)^2>\left(\frac{a}{2a}\right)^2=\left(\frac{1}{2}\right)^2=\frac{1}{4}</math> | + | ::<math>\scriptstyle{\color{OliveGreen}{b>a\longrightarrow\left(\frac{b}{2a}\right)^2>\left(\frac{a}{2a}\right)^2=\left(\frac{1}{2}\right)^2=\frac{1}{4}}}</math> |
|style="text-align:right;"|ואם יהיה השארית יותר גדול מהשרש כשנחלקנו על כפל השרש יהיה היוצא יותר מחצי ומרובעו יותר מרביעית | |style="text-align:right;"|ואם יהיה השארית יותר גדול מהשרש כשנחלקנו על כפל השרש יהיה היוצא יותר מחצי ומרובעו יותר מרביעית | ||
|- | |- | ||
| | | | ||
| − | :*<math>\scriptstyle\sqrt{6}</math> | + | :*{{#annot:√6|439|cUME}}Example: we wish to know the root of 6. |
| − | |style="text-align:right;"|והמשל בקשנו לידע שרש ו‫' | + | ::<math>\scriptstyle\sqrt{6}</math> |
| + | |style="text-align:right;"|והמשל בקשנו לידע שרש ו‫'{{#annotend:cUME}} | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::The integer that results in the root is 2 and 2 remains, which is as the root itself. |
| − | |style="text-align:right;"|הנה השלמים אשר יצאו בשרש הם ב' וישארו ב' שהוא כמו השרש בעצמו | + | |style="text-align:right;"|הנה השלמים אשר יצאו בשרש הם ב' וישארו ב' שהוא כמו השרש בעצמו |
| − | |||
|- | |- | ||
| | | | ||
| + | ::If we divide it by double the [approximate] root without adding one, it is divided by 4, which is double the root and the quotient is a half, so the whole root is 2 integers and a half. | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{6}\approx2+\frac{6-4}{2\sdot2}=2+\frac{2}{4}=2+\frac{1}{2}}}</math> | ||
| + | |style="text-align:right;"|ואם חלקנום על כפל השרש בלי תוספת אחת יתחלק לד' שהוא כפל השרש ויצא בחילוק חצי ויהיה כל השרש ב' שלמים וחצי | ||
| + | |- | ||
| + | | | ||
| + | ::Its square is 6 integers and a quarter. | ||
:::<math>\scriptstyle{\color{blue}{\left(2+\frac{1}{2}\right)^2=6+\frac{1}{4}}}</math> | :::<math>\scriptstyle{\color{blue}{\left(2+\frac{1}{2}\right)^2=6+\frac{1}{4}}}</math> | ||
|style="text-align:right;"|ומרובעם ו' שלמים ורביע | |style="text-align:right;"|ומרובעם ו' שלמים ורביע | ||
| + | |} | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{b\ge a\longrightarrow\left(a^2+b\right)-\left(a+\frac{b}{2a+1}\right)^2=\frac{b}{2a+1}\sdot\left(1-\frac{b}{2a+1}\right)<\frac{1}{2}\sdot\left(1-\frac{1}{2}\right)=\frac{1}{4}}}</math> | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | : | + | :If we add one, the square [of the approximate root] will be smaller [than the given number] by the product of the quotient, which is less than a half, by its complement with respect to 1, so it will be less than a quarter. |
| − | |style="text-align:right;"|ואם היינו מוסיפים א' הנה יחסר מהמרובע ככפל היוצא בחילוק שיהיה פחות מחצי בהשלמתו לאחד וזה יהיה פחות מרביע | + | |style="width:45%; text-align:right;"|ואם היינו מוסיפים ‫<ref>38v</ref>א' הנה יחסר מהמרובע ככפל היוצא בחילוק שיהיה פחות מחצי בהשלמתו לאחד וזה יהיה פחות מרביע |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle b>a\longrightarrow\left(\frac{b}{2a}\right)^2>\left(\frac{1}{2}\right)^2=\frac{1}{4}</math> | + | :Therefore it is close to the truth, for the square [of the approximate root] is smaller than the [given] number by less than a quarter. |
| + | |style="text-align:right;"|הנה א"כ הוא קרוב אל האמת כי לא יחסר רביע במרובע מהחשבון | ||
| + | |- | ||
| + | | | ||
| + | :If we do not add one, we increase [the excess] to a quarter [at least] and all the more so if it is greater than the [approximate] root. | ||
| + | |style="text-align:right;"|ואם לא נוסיף א' נוסיף רביע וכ"ש אם היה גדול מהשרש | ||
| + | |- | ||
| + | | | ||
| + | :For, when we divide it by double the [approximate] root, the quotient is greater than a half and its square is greater than a quarter, as you can see in the preceding example. | ||
| + | :::<math>\scriptstyle{\color{OliveGreen}{b>a\longrightarrow\left(\frac{b}{2a}\right)^2>\left(\frac{1}{2}\right)^2=\frac{1}{4}}}</math> | ||
|style="text-align:right;"|כי כאשר נחלקנו לכפול השרש יצא בחילוק יותר מחצי ומרובעו יותר מרביע כאשר תראה במשל הקודם לזה | |style="text-align:right;"|כי כאשר נחלקנו לכפול השרש יצא בחילוק יותר מחצי ומרובעו יותר מרביע כאשר תראה במשל הקודם לזה | ||
|- | |- | ||
|If you divide [the remainder] by double the root plus 1, the square of the root will be less than the sought number by the product of the quotient and its complement with respect to one, which can never in any way reach a quarter. | |If you divide [the remainder] by double the root plus 1, the square of the root will be less than the sought number by the product of the quotient and its complement with respect to one, which can never in any way reach a quarter. | ||
| − | :<math>\scriptstyle b\ge a\longrightarrow\left(a^2+b\right)-\left(a+\frac{b}{2a+1}\right)^2=\frac{b}{2a+1}\sdot\left(1-\frac{b}{2a+1}\right)<\frac{1}{2}\sdot\left(1-\frac{1}{2}\right)=\frac{1}{4}</math> | + | |style="text-align:right;"|ואם תחלקנו על כפל השרש בתוספת <sup>א'</sup> יחסר מרובע השרש המקובץ מהחשבון הנשאל ככפל היוצא בחלוק בהשלמתו לאחד ולא יהיה אפי' רביע בשום פנים |
| − | | | + | |} |
| + | :<math>\scriptstyle{\color{OliveGreen}{b\ge a\longrightarrow\left(a^2+b\right)-\left(a+\frac{b}{2a+1}\right)^2=\frac{b}{2a+1}\sdot\left(1-\frac{b}{2a+1}\right)<\frac{1}{2}\sdot\left(1-\frac{1}{2}\right)=\frac{1}{4}}}</math> | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | + | :For, the product of a portion of a line or a number by its complement never reaches a quarter. | |
| − | |style="text-align:right;"|כי כאשר תכפול קצת הקו או המספר בקצתו האחר לא יעלה לעולם לרביע | + | |style="width:45%; text-align:right;"|כי כאשר תכפול קצת הקו או המספר בקצתו האחר לא יעלה לעולם לרביע |
|- | |- | ||
| | | | ||
| Line 4,293: | Line 5,129: | ||
|- | |- | ||
| | | | ||
| − | + | :And clearly if you multiply its smaller portion by its larger [complementing] portion [the product] will not be a quarter but smaller than it by the square of their distances from half the line or the number. | |
| − | ::<math>\scriptstyle\left(1-\frac{n}{m}\right)>\frac{n}{m}\longrightarrow\frac{1}{4}-\left[\frac{n}{m}\sdot\left(1-\frac{n}{m}\right)\right]=\left(\frac{1}{2}-\frac{n}{m}\right)^2</math> | + | ::<math>\scriptstyle{\color{OliveGreen}{\left(1-\frac{n}{m}\right)>\frac{n}{m}\longrightarrow\frac{1}{4}-\left[\frac{n}{m}\sdot\left(1-\frac{n}{m}\right)\right]=\left(\frac{1}{2}-\frac{n}{m}\right)^2}}</math> |
|style="text-align:right;"|ואם תכפול מעוטו ברובו לא יהיה רביע וזה ברור אבל יחסר ממנו כמרובע מרחקם מחצי הקו או המספר | |style="text-align:right;"|ואם תכפול מעוטו ברובו לא יהיה רביע וזה ברור אבל יחסר ממנו כמרובע מרחקם מחצי הקו או המספר | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::For instance, if we divide a line into a quarter and 3-quarters, when you multiply its half by its half it is as if you multiply a quarter of the line by a quarter of the line four times. |
:::<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot\frac{1}{2}=4\sdot\left(\frac{1}{4}\sdot\frac{1}{4}\right)}}</math> | :::<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot\frac{1}{2}=4\sdot\left(\frac{1}{4}\sdot\frac{1}{4}\right)}}</math> | ||
| − | |style="text-align:right;"|כי ע'ד'מ' אם חלקנו הקו לרביע הקו וג' רביעיות הנה אם תכפול החצי בחצי הוא כאלו תכפול רביע הקו עם ברביע הקו ד' פעמים | + | |style="text-align:right;"|<sup>כי</sup> ע'ד'מ' אם חלקנו הקו לרביע הקו וג' רביעיות הנה אם תכפול החצי בחצי הוא כאלו תכפול רביע הקו <s>עם</s> ברביע הקו ד' פעמים |
|- | |- | ||
| | | | ||
| + | ::If you multiply a quarter of the line by its 3-quarters, which is its complement with respect to one, it is exactly as the product of a quarter of the line by a quarter of the line three times. | ||
:::<math>\scriptstyle{\color{blue}{\frac{1}{4}\sdot\left(1-\frac{1}{4}\right)=\frac{1}{4}\sdot\frac{3}{4}=3\sdot\left(\frac{1}{4}\sdot\frac{1}{4}\right)}}</math> | :::<math>\scriptstyle{\color{blue}{\frac{1}{4}\sdot\left(1-\frac{1}{4}\right)=\frac{1}{4}\sdot\frac{3}{4}=3\sdot\left(\frac{1}{4}\sdot\frac{1}{4}\right)}}</math> | ||
| − | |style="text-align:right;"|ואם תכפול רביע הקו בג' רביעיות המשלימות אותו לאחד שלם לא יהיה כי אם כפל רביע הקו ברביע הקו ג' פעמים | + | |style="text-align:right;"|ואם תכפול רביע הקו בג' רביעיות המשלימות אותו לאחד שלם לא יהיה כי אם כפל רביע הקו ברביע הקו ג' פעמים <s>ואם תכפול רביע הקו בג' רביעיות המשלימות אותו לאחד שלם לא יהיה כי אם</s> |
|- | |- | ||
| | | | ||
| + | ::It is less than the product of a quarter by a quarter, which is the distance of each portion of the line from its half. | ||
:::<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}\sdot\frac{1}{2}\right)-\left[\frac{1}{4}\sdot\left(1-\frac{1}{4}\right)\right]=\frac{1}{4}\sdot\frac{1}{4}}}</math> | :::<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}\sdot\frac{1}{2}\right)-\left[\frac{1}{4}\sdot\left(1-\frac{1}{4}\right)\right]=\frac{1}{4}\sdot\frac{1}{4}}}</math> | ||
|style="text-align:right;"|הנה יחסר מחצי על חצי ככפל רביע על רביע שהוא מרחק כל אחד מחלקי הקו מהחצי | |style="text-align:right;"|הנה יחסר מחצי על חצי ככפל רביע על רביע שהוא מרחק כל אחד מחלקי הקו מהחצי | ||
|- | |- | ||
| | | | ||
| + | ::For instance, if you multiply a half of 12 by its half, which is 6 by 6, the result is 36, which is the product of 6 by 5 that is 30, and the product of 6 by 1, which is 6. | ||
:::<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}\sdot12\right)\sdot\left(\frac{1}{2}\sdot12\right)=6\sdot6=36=30+6=\left(6\sdot5\right)+\left(6\sdot1\right)}}</math> | :::<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}\sdot12\right)\sdot\left(\frac{1}{2}\sdot12\right)=6\sdot6=36=30+6=\left(6\sdot5\right)+\left(6\sdot1\right)}}</math> | ||
|style="text-align:right;"|ואם תכפול ע'ד'מ' חצי מספר י"ב בחציו שהוא ו' בו' יעלה ל"ו שהוא כפל ו' בה' שהם ל' וכפלו ו' בא' שהם ו‫' | |style="text-align:right;"|ואם תכפול ע'ד'מ' חצי מספר י"ב בחציו שהוא ו' בו' יעלה ל"ו שהוא כפל ו' בה' שהם ל' וכפלו ו' בא' שהם ו‫' | ||
|- | |- | ||
| | | | ||
| + | ::But, if you multiply 5 by 7, which is its complement with respect to [twelve], it is only 35, because it is as the product of 5 by 6, which is 30, and the product of 5 by 1, which is 5. | ||
:::<math>\scriptstyle{\color{blue}{\left(6-1\right)\sdot\left(6+1\right)=5\sdot7=35=30+5=\left(5\sdot6\right)+\left(5\sdot1\right)}}</math> | :::<math>\scriptstyle{\color{blue}{\left(6-1\right)\sdot\left(6+1\right)=5\sdot7=35=30+5=\left(5\sdot6\right)+\left(5\sdot1\right)}}</math> | ||
|style="text-align:right;"|ואולם אם תכפול ה' בז' שהם השלמתו לאחד לא יהיה כי אם ל"ה לפי שהוא ככפל ה' בו' שהם ל' וכפל ה' בא' שהם ה‫' | |style="text-align:right;"|ואולם אם תכפול ה' בז' שהם השלמתו לאחד לא יהיה כי אם ל"ה לפי שהוא ככפל ה' בו' שהם ל' וכפל ה' בא' שהם ה‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|וכל מה שיתחלקו יותר החלקים יחסר יותר | + | ::The [greater] the fraction, the greater is the excess. |
| + | |style="text-align:right;"|וכל מה ‫<ref>39r</ref>שיתחלקו יותר החלקים יחסר יותר | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle\left(1-\frac{n}{m}\right)>\frac{n}{m}\longrightarrow\frac{1}{4}-\left[\frac{n}{m}\sdot\left(1-\frac{n}{m}\right)\right]=\left(\frac{1}{2}-\frac{n}{m}\right)^2</math> | + | ::That is, its difference from a quarter is as the square of its difference from a half. |
| + | :::<math>\scriptstyle{\color{OliveGreen}{\left(1-\frac{n}{m}\right)>\frac{n}{m}\longrightarrow\frac{1}{4}-\left[\frac{n}{m}\sdot\left(1-\frac{n}{m}\right)\right]=\left(\frac{1}{2}-\frac{n}{m}\right)^2}}</math> | ||
|style="text-align:right;"|וזה שהחסרון מרביע הוא כמרובע הרחקתם מחצי | |style="text-align:right;"|וזה שהחסרון מרביע הוא כמרובע הרחקתם מחצי | ||
|- | |- | ||
| − | | | + | |? |
|style="text-align:right;"|כבמשלנו זה שהיה כמרובע האחד אשר נתרחקו מו' שהוא החצי | |style="text-align:right;"|כבמשלנו זה שהיה כמרובע האחד אשר נתרחקו מו' שהוא החצי | ||
|- | |- | ||
| | | | ||
| + | ::If we multiply 3 by its complement with respect to twelve, which is 9, the result is only 27 and it is less than [the square of its] half by the square of 3, which is 9. | ||
| + | |style="text-align:right;"|ואם היינו כופלים ג' בהשלמתו לי"ב שהוא ט' הנה לא יעלה כי אם כ"ז ויחסר כמרובע ג' שנתרחקו מהחצי שהוא ט‫' | ||
| + | |} | ||
:::<math>\scriptstyle{\color{blue}{\left[\left(\frac{1}{2}\sdot12\right)\sdot\left(\frac{1}{2}\sdot12\right)\right]-\left[3\sdot\left(12-3\right)\right]=\left(6\sdot6\right)-\left(3\sdot9\right)=36-27=9=3^2}}</math> | :::<math>\scriptstyle{\color{blue}{\left[\left(\frac{1}{2}\sdot12\right)\sdot\left(\frac{1}{2}\sdot12\right)\right]-\left[3\sdot\left(12-3\right)\right]=\left(6\sdot6\right)-\left(3\sdot9\right)=36-27=9=3^2}}</math> | ||
| − | | | + | {| |
|- | |- | ||
| | | | ||
| − | ::::<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}\sdot12\right)\sdot\left(\frac{1}{2}\sdot12\right)=6\sdot6=\left(6\sdot3\right)+\left(6\sdot3\right)=\left[2\sdot\left(3\sdot3\right)\right]+\left[2\sdot\left(3\sdot3\right)\right]}}</math> | + | ::This is because the product of 6 by 6 is as the product of 6 by 3 and the product of 6 by 3, which is twice the product of 3 by 3. |
| − | |style="text-align:right;"|וזה כי כפל ו' בו' הוא ככפל ו' בג' וככפלו ו' בג' שהוא כפל ג' בג' פעמים | + | :::<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}\sdot12\right)\sdot\left(\frac{1}{2}\sdot12\right)=6\sdot6=\left(6\sdot3\right)+\left(6\sdot3\right)=\left[2\sdot\left(3\sdot3\right)\right]+\left[2\sdot\left(3\sdot3\right)\right]}}</math> |
| + | |style="width:45%; text-align:right;"|וזה כי כפל ו' בו' הוא ככפל ו' בג' וככפלו ו' בג' שהוא כפל ג' בג' פעמים | ||
|- | |- | ||
| | | | ||
| + | ::But, the product of 3 by 9 is only the product of 3 by 6 and the product of 3 by 3. | ||
:::<math>\scriptstyle{\color{blue}{3\sdot\left(12-3\right)=3\sdot9=\left(3\sdot6\right)+\left(3\sdot3\right)}}</math> | :::<math>\scriptstyle{\color{blue}{3\sdot\left(12-3\right)=3\sdot9=\left(3\sdot6\right)+\left(3\sdot3\right)}}</math> | ||
|style="text-align:right;"|ואולם כפל ג' בט' אינו כי אם כפל ג' בו' וכפל ג' בג' פעם אחת לבד | |style="text-align:right;"|ואולם כפל ג' בט' אינו כי אם כפל ג' בו' וכפל ג' בג' פעם אחת לבד | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle\frac{1}{4}n^2-\left[m\sdot\left(n-m\right)\right]=\left[\left(\frac{1}{2}n\right)\sdot\left(\frac{1}{2}n\right)\right]-\left[m\sdot\left(n-m\right)\right]=\left[\left(\frac{1}{2}n\right)-m\right]^2</math> | + | ::So, its difference from a quarter of a square number is as the product of its difference from the half by itself, which is a quarter of a quarter. |
| − | | | + | |style="text-align:right;"|לכן יחסר מרביע מרובע <sup>ה</sup>מספר ככפל ריחוקם מהחצי בעצמו והוא רביע רביע |
| + | |} | ||
| + | :::<math>\scriptstyle{\color{OliveGreen}{\frac{1}{4}n^2-\left[m\sdot\left(n-m\right)\right]=\left[\left(\frac{1}{2}n\right)\sdot\left(\frac{1}{2}n\right)\right]-\left[m\sdot\left(n-m\right)\right]=\left[\left(\frac{1}{2}n\right)-m\right]^2}}</math> | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| + | ::Since its difference is 3, which is a quarter of 12. Deduce from this. | ||
:::<math>\scriptstyle{\color{blue}{\left[\left(\frac{1}{2}\sdot12\right)\sdot\left(\frac{1}{2}\sdot12\right)\right]-\left[3\sdot\left(12-3\right)\right]=3^2=\left(\frac{1}{4}\sdot12\right)^2}}</math> | :::<math>\scriptstyle{\color{blue}{\left[\left(\frac{1}{2}\sdot12\right)\sdot\left(\frac{1}{2}\sdot12\right)\right]-\left[3\sdot\left(12-3\right)\right]=3^2=\left(\frac{1}{4}\sdot12\right)^2}}</math> | ||
| − | |style="text-align:right;"|לפי שרחוקם היה ג' שהוא רביע הי"ב דוק ותשכח | + | |style="width:45%; text-align:right;"|לפי שרחוקם היה ג' שהוא רביע הי"ב דוק ותשכח |
|- | |- | ||
| − | + | !<span style=color:Green>The reason for<span style=color:Green> <math>\scriptstyle{\color{OliveGreen}{\left(a^2+b\right)-\left(a+\frac{b}{2a+1}\right)^2=\frac{b}{2a+1}\sdot\left(1-\frac{b}{2a+1}\right)}}</math> | |
| − | + | | | |
| + | |- | ||
| + | |Our saying that when we divide [the remainder] by double the root plus 1, the square of the summed [approximate] root is smaller than the [original] number by the product of the quotient by its complement with respect to one - | ||
|style="text-align:right;"|ואולם אומרנו שכאשר נחלקנו לכפל השרש בתוספת אחד שיהיה החסרון אשר במרובע השרש המקובץ מהחשבון ככפל היוצא בחילוק בהשלמתו | |style="text-align:right;"|ואולם אומרנו שכאשר נחלקנו לכפל השרש בתוספת אחד שיהיה החסרון אשר במרובע השרש המקובץ מהחשבון ככפל היוצא בחילוק בהשלמתו | ||
| + | |- | ||
| + | |I shall explain it first by the previous examples, then I shall explain its reason: | ||
| + | |style="text-align:right;"|אבארנו תחלה במשלים העוברים וא'ח'כ' אבארנו בטעם | ||
| + | |- | ||
| + | | | ||
| + | :*{{#annot:√7|439|ROfW}}When we seek the root of 7. | ||
| + | ::<math>\scriptstyle\sqrt{7}</math> | ||
| + | |style="text-align:right;"|<big>המשל</big> כאשר בקשנו שרש ז‫'{{#annotend:ROfW}} | ||
| + | |- | ||
| + | | | ||
| + | ::The result is 2 integers and 3 remains, which is greater then the [approximate] root. | ||
| + | |style="text-align:right;"|והיה ב שלמים ונשארו ג' שהם יותר מהשרש | ||
| + | |- | ||
| + | | | ||
| + | ::We divide it by double the root plus 1, i.e. by 5; the quotient is 3-fifths. | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{7}\approx2+\frac{7-4}{\left(2\sdot2\right)+1}=2+\frac{3}{5}}}</math> | ||
| + | |style="text-align:right;"|וחלקנום לכפל השרש בתוספת <sup>א' </sup> ר"ל על ה' יצא בחילוק ג' חמישיות | ||
| + | |- | ||
| + | | | ||
| + | ::When we multiply two integers and 3-fifths by itself, its square is 6 integers, 3-fifths and 4-fifths of a fifth. | ||
| + | |style="text-align:right;"|וכאשר נכפול שני שלמים <sup>וג'</sup> חמישיות על עצמו יהיה מרובעו ו' שלמים וג' חמישיות וד' חמישיות חמישית | ||
| + | |- | ||
| + | | | ||
| + | ::But, the required number is 7 integers, so this square is smaller than 7 integers by one-fifth and a fifth of a fifth. | ||
| + | |style="text-align:right;"|והחשבון הנשאל היה ז' שלימים הנה יחסר זה המרובע מז' שלמים חמישית [אחת שלמה וחמישית חמישית | ||
| + | |- | ||
| + | | | ||
| + | ::This itself is as the product of 3-fifths, which is the quotient, by its complement with respect to one, which is 2-fifths. | ||
| + | |style="text-align:right;"|וזה]‫<ref>marg.</ref> וזה בעצמו הוא כפל הג' חמישיות אשר יצאו בחלוק <sup>על השלמתם</sup> לאחד שלם שהוא <sup>ב'</sup> חמישיות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::For, the product of 3-fifths by 2-fifths is 6-fifths of a fifth, which is one-fifth and a fifth of a fifth. |
| + | |style="text-align:right;"|כי כפל ג' חמישיות בב' חמישיות הוא ו' חמישיות <sup>חמישית</sup> שהן חמישית אחד שלם וחמישית חמישית | ||
| + | |} | ||
| + | ::<math>\scriptstyle{\color{blue}{7-\left(2+\frac{3}{5}\right)^2=7-\left[6+\frac{3}{5}+\left(\frac{4}{5}\sdot\frac{1}{5}\right)\right]=\frac{1}{5}+\left(\frac{1}{5}\sdot\frac{1}{5}\right)=\frac{6}{5}\sdot\frac{1}{5}=\frac{3}{5}\sdot\frac{2}{5}=\frac{3}{5}\sdot\left(1-\frac{3}{5}\right)}}</math> | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | :*In the second example: if we seek the root [of 6]. | ||
| + | ::<math>\scriptstyle\sqrt{6}</math> | ||
| + | |style="width:45%; text-align:right;"|<big>ובמשל</big> בשני אם בקשנו שרש | ||
|- | |- | ||
| | | | ||
| − | + | ::The result is 2 integers and 2 remains, which is the same as the root. | |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\sqrt{6}\approx2+\frac{6-4}{\left(2\sdot2\right)+1}=2+\frac{2}{5}}}</math> |
| + | |style="text-align:right;"|והנה יצאו ב' שלמים ונשארו ב' שהוא כמו השרש | ||
|- | |- | ||
| | | | ||
| − | : | + | ::If we divide it by double the root plus 1, i.e. by 5; the quotient is 2-fifths. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אם חלקנום על כפל השרש בתוספת <sup>א'</sup> ר"ל על ה' ‫<ref>39v</ref>יצא בחילוק ב' חמישיות |
|- | |- | ||
| | | | ||
| − | :: | + | ::When we multiply two integers and 2-fifths by itself, its square is 5 integers, 3-fifths and 4-fifths of a fifth. |
| − | |style="text-align:right;"|וכאשר | + | |style="text-align:right;"|וכאשר כפלנו שני שלמים וב' חמישיות על עצמם יעלה ה' מרובעו ה' שלמים וג' [חמישיות]‫<ref>marg.</ref> וד' חמישיות חמישית |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | ::But, the required number, whose root we seek, is 6 integers, so this square is smaller than that number by one-fifth and a fifth of a fifth. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואולם החשבון הנשאל אשר בקשנו שרשו היה ו' שלמים הנה יחסר זה המרובע מהחשבון ההוא חמישית אחת שלימה וחמישית חמישית |
|- | |- | ||
| | | | ||
| − | : | + | ::This is as the product of 2-fifths, which is the quotient, by its complement with respect to one, which is 3-fifths. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והוא ככפל הב' חמישיות אשר יצאו בחילוק בהשלמתם לאחד שהוא ג' חמישיות |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::For, the product of 2-fifths by 3-fifths is 6-fifths of a fifth, which is one-fifth and a fifth of a fifth as we have explained. |
| − | | | + | |style="text-align:right;"|כי כפל ב' חמישיות בג' חמישיות הוא ו' חמישיות חמישית שהן חמישית אחד שלמה וחמישית חמישית כאשר ביארנו |
| − | + | |} | |
| − | + | ::<math>\scriptstyle{\color{blue}{6-\left(2+\frac{2}{5}\right)^2=6-\left[5+\frac{3}{5}+\left(\frac{4}{5}\sdot\frac{1}{5}\right)\right]=\frac{1}{5}+\left(\frac{1}{5}\sdot\frac{1}{5}\right)=\frac{6}{5}\sdot\frac{1}{5}=\frac{2}{5}\sdot\frac{3}{5}=\frac{2}{5}\sdot\left(1-\frac{2}{5}\right)}}</math> | |
| − | + | {| | |
|- | |- | ||
|The reason is that the remainder is as the result of division multiplied by twice the [previous approximate] root plus one. | |The reason is that the remainder is as the result of division multiplied by twice the [previous approximate] root plus one. | ||
| − | ::<math>\scriptstyle b=\frac{b}{2a+1}\sdot\left(2a+1\right)=\left(\frac{b}{2a+1}\sdot2a\right)+\left(\frac{b}{2a+1}\sdot1\right)</math> | + | ::<math>\scriptstyle{\color{OliveGreen}{b=\frac{b}{2a+1}\sdot\left(2a+1\right)=\left(\frac{b}{2a+1}\sdot2a\right)+\left(\frac{b}{2a+1}\sdot1\right)}}</math> |
| − | |style="text-align:right;"|והטעם הוא לפי שהשארית היה ככפל זה היוצא בחילוק בכפל השרש הראשון ובאחד | + | |style="width:45%; text-align:right;"|<big>והטעם</big> הוא לפי שהשארית היה ככפל זה היוצא בחילוק בכפל השרש הראשון ובאחד |
|- | |- | ||
| − | | | + | |For, when we divide the remainder by double the [approximate] root plus 1, we find that the remainder is as the product of this quotient by double the [approximate] root plus 1. |
| − | |style="text-align:right;"|שהרי בחלקנו השארית לכפל השרש בתוספת א' יצא זה בחלוק נמצא שהשארית היה ככפל זה היוצא בחלוק בכפל השרש הקודם [ובא‫'] | + | |style="text-align:right;"|שהרי בחלקנו השארית לכפל השרש בתוספת א' יצא זה בחלוק נמצא שהשארית היה ככפל זה היוצא בחלוק בכפל השרש הקודם [ובא‫']‫<ref>marg.</ref> <s>לא יוסיף במרובע</s> |
|- | |- | ||
|The addition of the result to the previous [approximate] root, however, will add to the square only its product by twice the previous root and its product by itself. | |The addition of the result to the previous [approximate] root, however, will add to the square only its product by twice the previous root and its product by itself. | ||
| − | ::<math>\scriptstyle\left(a+b\right)^2-a^2=\left(2\sdot a\sdot b\right)+b^2</math> | + | ::<math>\scriptstyle{\color{OliveGreen}{\left(a+b\right)^2-a^2=\left(2\sdot a\sdot b\right)+b^2}}</math> |
|style="text-align:right;"|ואולם תוספת זה היוצא בשרש הקודם לא יוסיף במרובע כי אם ככפלו בכפל השרש הקודם ובכפלו בעצמו | |style="text-align:right;"|ואולם תוספת זה היוצא בשרש הקודם לא יוסיף במרובע כי אם ככפלו בכפל השרש הקודם ובכפלו בעצמו | ||
|- | |- | ||
|But, its product by itself subtracted from its product by 1 is its product by its complement [with respect to 1]. | |But, its product by itself subtracted from its product by 1 is its product by its complement [with respect to 1]. | ||
| − | ::<math>\scriptstyle\left(b\sdot1\right)-b^2=b\sdot\left(1-b\right)</math> | + | ::<math>\scriptstyle{\color{OliveGreen}{\left(b\sdot1\right)-b^2=b\sdot\left(1-b\right)}}</math> |
|style="text-align:right;"|וכפלו בעצמו יחס' מכפלו בא' כפלו בהשלמתו לאחד | |style="text-align:right;"|וכפלו בעצמו יחס' מכפלו בא' כפלו בהשלמתו לאחד | ||
|- | |- | ||
| Line 4,403: | Line 5,292: | ||
:For example, the product of a third by one is as its product by all the parts [of 1], namely by a third, which is itself, and by two thirds, which is its complement with respect to one; this is clear. | :For example, the product of a third by one is as its product by all the parts [of 1], namely by a third, which is itself, and by two thirds, which is its complement with respect to one; this is clear. | ||
:::<math>\scriptstyle{\color{blue}{\left(\frac{1}{3}\sdot1\right)=\left(\frac{1}{3}\sdot\frac{1}{3}\right)+\left(\frac{2}{3}\sdot\frac{1}{3}\right)=\left(\frac{1}{3}\sdot\frac{1}{3}\right)+\left[\frac{1}{3}\sdot\left(1-\frac{1}{3}\right)\right]}}</math> | :::<math>\scriptstyle{\color{blue}{\left(\frac{1}{3}\sdot1\right)=\left(\frac{1}{3}\sdot\frac{1}{3}\right)+\left(\frac{2}{3}\sdot\frac{1}{3}\right)=\left(\frac{1}{3}\sdot\frac{1}{3}\right)+\left[\frac{1}{3}\sdot\left(1-\frac{1}{3}\right)\right]}}</math> | ||
| − | |style="text-align:right;"|כי המשל כפל שליש באחד הוא ככפלו בכל חלקיו ר"ל ככפלו בשליש ר"ל בעצמו וככפלו בשתי שלישים אשר הם המשלים אותו כאחד וזה ברור | + | |style="text-align:right;"|כי המשל כפל שליש באחד הוא ככפלו בכל חלקיו <sup>ר"ל</sup> ככפלו בשליש ר"ל בעצמו וככפלו בשתי שלישים אשר הם המשלים אותו כאחד וזה ברור |
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle b\ge a\longrightarrow\left(a^2+b\right)-\left(a+\frac{b}{2a+1}\right)^2<\left(a+\frac{b}{2a}\right)^2-\left(a^2+b\right)</math>< | + | :<math>\scriptstyle{\color{OliveGreen}{b\ge a\longrightarrow\left(a^2+b\right)-\left(a+\frac{b}{2a+1}\right)^2<\left(a+\frac{b}{2a}\right)^2-\left(a^2+b\right)}}</math> |
| − | : | + | :<span style=color:Green>closer approximation:</span><math>\scriptstyle{\color{OliveGreen}{a+\frac{b}{2a+1}}}</math> |
| + | | | ||
| + | |- | ||
| + | |Thus, we have explained that when the remainder is as the [approximate] root or greater than it, if we divide it by double the [approximate] root plus 1, it comes closer to the truth by subtraction more than it come closer to the truth by addition when we divide it by double the [approximate] root without adding 1. | ||
|style="text-align:right;"|הנה ביארנו כי כאשר השארית היה כשרש או יותר ממנו כי בחלקנו אותו לכפל השרש בתוספת א' יתקרב אל האמת לחסרון מאשר יתקרב אל האמת לתוספת בחלקנו אותו לכפל השרש בלי תוספת אחד | |style="text-align:right;"|הנה ביארנו כי כאשר השארית היה כשרש או יותר ממנו כי בחלקנו אותו לכפל השרש בתוספת א' יתקרב אל האמת לחסרון מאשר יתקרב אל האמת לתוספת בחלקנו אותו לכפל השרש בלי תוספת אחד | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle b<a\longrightarrow\left(a^2+b\right)-\left(a+\frac{b}{2a+1}\right)^2>\left(a+\frac{b}{2a}\right)^2-\left(a^2+b\right)</math>< | + | :<math>\scriptstyle{\color{OliveGreen}{b<a\longrightarrow\left(a^2+b\right)-\left(a+\frac{b}{2a+1}\right)^2>\left(a+\frac{b}{2a}\right)^2-\left(a^2+b\right)}}</math> |
| − | : | + | :<span style=color:Green>closer approximation:</span> <math>\scriptstyle{\color{OliveGreen}{a+\frac{b}{2a}}}</math> |
| + | | | ||
| + | |- | ||
| + | |But, if the remainder is less than the [approximate] root it is vice versa. | ||
|style="text-align:right;"|ואולם אם השארית פחות מהשרש יהיה להפך | |style="text-align:right;"|ואולם אם השארית פחות מהשרש יהיה להפך | ||
|- | |- | ||
| | | | ||
| − | *Example: if we wish the root of 29 | + | *{{#annot:√29|439|rK5S}}Example: if we wish the root of 29. |
:<math>\scriptstyle\sqrt{29}</math> | :<math>\scriptstyle\sqrt{29}</math> | ||
| − | |style="text-align:right;"|המשל אם בקשנו שרש כ"ט | + | |style="text-align:right;"|‫<ref>40r</ref><big>המשל</big> אם בקשנו שרש כ"ט{{#annotend:rK5S}} |
|- | |- | ||
| | | | ||
| + | :The integer resulting in the root is 5 and 4 remains. If we divide it by double the root without addition, which is 10, the result is 4-tenths. | ||
:<math>\scriptstyle{\color{blue}{\sqrt{29}\approx5+\frac{29-25}{2\sdot5}=5+\frac{4}{10}}}</math> | :<math>\scriptstyle{\color{blue}{\sqrt{29}\approx5+\frac{29-25}{2\sdot5}=5+\frac{4}{10}}}</math> | ||
|style="text-align:right;"|הנה השלמים אשר יצאו בשרש הם ה' ונשארו ד‫'<br> | |style="text-align:right;"|הנה השלמים אשר יצאו בשרש הם ה' ונשארו ד‫'<br> | ||
| Line 4,426: | Line 5,322: | ||
|- | |- | ||
| | | | ||
| + | ::Its excess over the truth is as the product of this result by itself, which is 16-tenths of a tenth, i.e. 16 parts of 100. | ||
::<math>\scriptstyle{\color{blue}{\left(5+\frac{4}{10}\right)^2-29=\frac{16}{10}\sdot\frac{1}{10}=\frac{16}{100}}}</math> | ::<math>\scriptstyle{\color{blue}{\left(5+\frac{4}{10}\right)^2-29=\frac{16}{10}\sdot\frac{1}{10}=\frac{16}{100}}}</math> | ||
|style="text-align:right;"|וריחוקו מן האמת לתוספת הוא ככפל זה היוצא בעצמו שהוא י"ו עשיריות עשירית ר"ל י"ו חלקים מק' שבשלם | |style="text-align:right;"|וריחוקו מן האמת לתוספת הוא ככפל זה היוצא בעצמו שהוא י"ו עשיריות עשירית ר"ל י"ו חלקים מק' שבשלם | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{\sqrt{29}\approx5+\frac{29-25}{\left(2\sdot5\right)+1}=5+\frac{4}{ | + | :If we divide it by [double the approximate root] plus one, which is [1]1, the quotient is 4 parts of 11. |
| − | |style="text-align:right;"|ואם חלקנום בתוספת א' שהוא | + | :<math>\scriptstyle{\color{blue}{\sqrt{29}\approx5+\frac{29-25}{\left(2\sdot5\right)+1}=5+\frac{4}{{\color{red}{1}}1}}}</math> |
| + | |style="text-align:right;"|ואם חלקנום בתוספת א' שהוא <s>שה</s> ל"א יצאו בחלוק ד' חלקים מי"א בשלם | ||
|- | |- | ||
| | | | ||
| + | ::Its distance from the truth is as the product of the quotient by its complement with respect to 1, which is 7 parts of 11, as we explained and it is 28 parts of 11 of one part of 11, i.e. 28 parts of 121, which is more that one-fifth. | ||
::<math>\scriptstyle{\color{blue}{29-\left(5+\frac{4}{11}\right)^2=\frac{4}{11}\sdot\left(1-\frac{4}{11}\right)=\frac{4}{11}\sdot\frac{7}{11}=\frac{28}{11}\sdot\frac{1}{11}=\frac{28}{121}>\frac{1}{5}}}</math> | ::<math>\scriptstyle{\color{blue}{29-\left(5+\frac{4}{11}\right)^2=\frac{4}{11}\sdot\left(1-\frac{4}{11}\right)=\frac{4}{11}\sdot\frac{7}{11}=\frac{28}{11}\sdot\frac{1}{11}=\frac{28}{121}>\frac{1}{5}}}</math> | ||
| − | |style="text-align:right;"|ויתרחק מן האמת בכפל | + | |style="text-align:right;"|ויתרחק מן האמת בכפל ו<s>ה</s>היוצא בהשלמתו לאחד שהוא ז' חלקים מי"א כאשר ביארנו והוא כ"ח חלקים מי"א מחלק אחד עשר בשלם ר"ל כ"ח חלקים מקכ"א בשלם והוא יותר מחמישית שלם |
|- | |- | ||
| | | | ||
| + | ::But, the former was not even one-sixth. Deduce from this. | ||
::<math>\scriptstyle{\color{blue}{\left(5+\frac{4}{10}\right)^2-29=\frac{16}{10}\sdot\frac{1}{10}=\frac{16}{100}<\frac{1}{6}}}</math> | ::<math>\scriptstyle{\color{blue}{\left(5+\frac{4}{10}\right)^2-29=\frac{16}{10}\sdot\frac{1}{10}=\frac{16}{100}<\frac{1}{6}}}</math> | ||
|style="text-align:right;"|ואולם הראשונים לא היו אפי' שישית אחת והקש על זה | |style="text-align:right;"|ואולם הראשונים לא היו אפי' שישית אחת והקש על זה | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle\left(a^2+b\right)-\left(a+\frac{b}{2a+1}\right)^2=\frac{1}{4}-\left(\frac{1}{2}-\frac{b}{2a+1}\right)^2</math> | + | :The reason is that the excess of the latter over the truth is less than a quarter by the product of its distance from a half by itself. |
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(a^2+b\right)-\left(a+\frac{b}{2a+1}\right)^2=\frac{1}{4}-\left(\frac{1}{2}-\frac{b}{2a+1}\right)^2}}</math> | ||
|style="text-align:right;"|והטעם כי זה יחסר רחוקו מן האמת מרביעיתו ככפל מרחקו מחצי בעצמו | |style="text-align:right;"|והטעם כי זה יחסר רחוקו מן האמת מרביעיתו ככפל מרחקו מחצי בעצמו | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle\left(a+\frac{b}{2a}\right)^2-\left(a^2+b\right)=\frac{1}{4}-\left[\left(\frac{1}{2}-\frac{b}{2a}\right)^2+\left[2\sdot\frac{b}{2a}\sdot\left(\frac{1}{2}-\frac{b}{2a}\right)\right]\right]</math> | + | :While the excess of the former over the truth is less [than a quarter] by the product of its distance from a half by itself plus twice the product of this distance by the addition in the root. |
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(a+\frac{b}{2a}\right)^2-\left(a^2+b\right)=\frac{1}{4}-\left[\left(\frac{1}{2}-\frac{b}{2a}\right)^2+\left[2\sdot\frac{b}{2a}\sdot\left(\frac{1}{2}-\frac{b}{2a}\right)\right]\right]}}</math> | ||
|style="text-align:right;"|וזה יחסר רחוקו מן האמת ככפל רחוקו מחצי בעצמו וככפל זה הריחוק פעמים בזה השרש המתוסף | |style="text-align:right;"|וזה יחסר רחוקו מן האמת ככפל רחוקו מחצי בעצמו וככפל זה הריחוק פעמים בזה השרש המתוסף | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::Example: in our previous example, when it is done with the addition of 1. We divide 4 by 11, the result is 4 parts of 11. The excess over the truth is as the product of these 4 [parts] by 7 [parts] as aforesaid, which is less than a quarter by the product of one part [of 11] of one and a half, which is the distance of [4 parts of 11] from a half, by itself, which is the distance of its square that is 2 and a quarter. |
| − | | | + | |style="text-align:right;"|המשל במשלנו הקודם כי כאשר יעשה [בתוספת א' המשל שחלקנו הד' על י"א ויצאו ד'י"א הנה]‫<ref>marg.</ref> י"א הנה יתרחק מן האמת ככפל אלו הד' בז' כנזכר ויחסר מרביע ככפל חלק אחד וחצי שהוא מרחקו מחצי הי"א בעצמו שהוא מרובע מרחקו שהוא ב' ורביע |
| − | + | |} | |
| − | + | ::<math>\scriptstyle{\color{blue}{29-\left(5+\frac{4}{11}\right)^2=\frac{4}{11}\sdot\frac{7}{11}=\frac{1}{4}-\frac{2+\frac{1}{4}}{11^2}=\frac{1}{4}-\left[\left(1+\frac{1}{2}\right)\sdot\frac{1}{11}\right]^2=\frac{1}{4}-\left(\frac{1}{2}-\frac{4}{11}\right)^2}}</math> | |
| − | + | {| | |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::But, when we divide it without an addition, i.e. by 10, the result of division is 4-tenths. The excess over the truth is as its product by itself, which is less than a quarter by the square of its distance from a half, which is one, plus twice the product of this one by double this root. The total is 9. |
| − | | | + | |style="width:45%; text-align:right;"|ואולם כאשר חלקנוהו מבלי תוספת המשל על י' הנה עלה בחלוק ד' עשיריות ויתרחק מן האמת ככפלו בעצמו שהוא פחות מרביע כמרובע מרחקו מחצי שהוא האחד וכפל זה האחד בכפל זה השרש שעולה הכל ט‫' |
| − | + | |} | |
| − | + | ::<math>\scriptstyle{\color{blue}{\left(5+\frac{4}{10}\right)^2-29=\left(\frac{4}{10}\right)^2=\frac{16}{100}=\frac{1}{4}-\frac{9}{100}=\frac{1}{4}-\left[\left(\frac{1}{2}-\frac{4}{10}\right)^2+\left[2\sdot\frac{4}{10}\sdot\left(\frac{1}{2}-\frac{4}{10}\right)\right]\right]}}</math> | |
| − | + | {| | |
|- | |- | ||
| − | | | + | |Although the excesses are not equal ''A single handful does not satisfy a lion'' [Talmud, Berakhot 3, 2]. |
| − | |style="text-align:right;"|ועם היות שאין הריחוקים שוים ולא החלקים מ"מ ''אין הקומץ משביע את הארי''<ref>תלמוד בבלי, מסכת ברכות, דף ג ע"ב</ref> | + | |style="width:45%; text-align:right;"|ועם היות שאין הריחוקים שוים ולא החלקים מ"מ ''אין הקומץ משביע את הארי''‫<ref group=note>תלמוד בבלי, מסכת ברכות, דף ג ע"ב</ref> |
|- | |- | ||
| − | | | + | |I do not feel to be more precise, because I have been precise enough at this place. |
|style="text-align:right;"|ולא חששתי לדקדק יותר כי די באשר דקדקתי בזה המקום | |style="text-align:right;"|ולא חששתי לדקדק יותר כי די באשר דקדקתי בזה המקום | ||
|- | |- | ||
| − | | | + | |We already stated that the one who wants to repeat the procedure does not need to add one [to double the approximate root], even if the remainder is greater than the root, because by repeating [the procedure] he comes as closer to the truth as possible. He should not be confused in the procedure by adding one, but always apply it without addition, and he should not speculate, only to take the square of the fraction resulting at that phase, divide it by double the [approximate] root, then subtract the quotient from its [approximate] root and so on. Because, I instructed to add one just when the remainder is as the [approximate] root or greater, only for the one who settles for one time. But the one who wants to come very closer and repeat the procedure should not add [one to double the approximate root] and not get confused. |
| − | |style="text-align:right;"|ועוד שכבר אמרנו שהרוצה להכפיל המעשים שאין לו צורך להוסיף אחד אף אם יהיה השארית גדול מהשרש כי בהכפל {{#annot:term|1612,1874|MDGB}}יתקרב אל האמת{{#annotend:MDGB}} בכל מאויו ולא יתבלבל במעשיו בתוספת אחד אבל לעולם יעשה בלי תוספת ואין לו לעיין כי אם לקחת מרובע השברים היוצאים בחלוק בפעם ההיא ולחלקו לכפל השרש והיוצא יחסרהו משרשו וכן לעולם<br> | + | |style="text-align:right;"|‫<ref>40v</ref><big>ועוד</big> שכבר אמרנו שהרוצה להכפיל המעשים שאין לו צורך להוסיף אחד אף אם יהיה השארית גדול מהשרש כי בהכפל {{#annot:term|1612,1874|MDGB}}יתקרב אל האמת{{#annotend:MDGB}} בכל מאויו ולא יתבלבל במעשיו בתוספת אחד אבל לעולם יעשה בלי תוספת ואין לו לעיין כי אם לקחת מרובע השברים היוצאים בחלוק בפעם ההיא ולחלקו לכפל השרש והיוצא יחסרהו משרשו וכן לעולם<br> |
כי לא ציויתי להוסיף אחד כאשר השארית כשרש או יותר אלא למסתפק בפעם אחת<br> | כי לא ציויתי להוסיף אחד כאשר השארית כשרש או יותר אלא למסתפק בפעם אחת<br> | ||
| − | אבל הרוצה לידע להתקרב מאד ולהכפיל המעשים לא יוסיף ולא יתבלבל | + | אבל הרוצה <s>לידע</s> להתקרב מאד ולהכפיל המעשים לא יוסיף ולא יתבלבל |
|- | |- | ||
| − | |<span style=color: | + | |<span style=color:Green>[= He should use this approximation <math>\scriptstyle{\color{OliveGreen}{a+\frac{b}{2a}}}</math> instead of the previous approximation <math>\scriptstyle{\color{OliveGreen}{a+\frac{b}{2a+1}}}</math>, even if b≥a, in order to avoid confusion]</span> |
| − | :If you wish to | + | | |
| − | + | |- | |
| + | |<span style=color:Green>Another approximation:</span> | ||
| + | *<math>\scriptstyle{\color{OliveGreen}{\sqrt{a^2+b}\approx a+\frac{2\sdot a\sdot b}{\left(2a\right)^2+b}}}</math> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :If you wish to come closer to the truth at once with little effort, add the remainder [i.e. the difference between the number and the approximate the root] to the square of double the [approximate] root that is in your hand, and divide by it the product of the remainder and double the [approximate] root. Add the result to the [approximate] root that is in your hand, and this root will be very near the truth. | ||
|style="text-align:right;"|ואם תרצה להתקרב אל האמת ברגע במעט עמל<br> | |style="text-align:right;"|ואם תרצה להתקרב אל האמת ברגע במעט עמל<br> | ||
חבר הנשאר למרובע כפל השרש שבידיך וחלק עליו כפל הנשאר בכפל השרש<br> | חבר הנשאר למרובע כפל השרש שבידיך וחלק עליו כפל הנשאר בכפל השרש<br> | ||
והיוצא חברהו לשרש שבידיך ויהיה שרש קרוב מאד אל האמת | והיוצא חברהו לשרש שבידיך ויהיה שרש קרוב מאד אל האמת | ||
|- | |- | ||
| − | |<span style=color: | + | |<span style=color:Green>The second approximation:</span> |
| − | + | *<math>\scriptstyle{\color{OliveGreen}{\sqrt{a^2+b}\approx\left[a+\frac{2\sdot a\sdot b}{\left(2a\right)^2+b}\right]+\frac{2\sdot\left[a+\frac{2\sdot a\sdot b}{\left(2a\right)^2+b}\right]\sdot\frac{b^3}{\left[\left(2a\right)^2+b\right]^2}}{\left[2\sdot\left[a+\frac{2\sdot a\sdot b}{\left(2a\right)^2+b}\right]\right]^2+\frac{b^3}{\left[\left(2a\right)^2+b\right]^2}}}}</math> | |
| − | *<math>\scriptstyle\sqrt{a^2+b}\approx\left[a+\frac{2\sdot a\sdot b}{\left(2a\right)^2+b}\right]+\frac{2\sdot\left[a+\frac{2\sdot a\sdot b}{\left(2a\right)^2+b}\right]\sdot\frac{b^3}{\left[\left(2a\right)^2+b\right]^2}}{\left[2\sdot\left[a+\frac{2\sdot a\sdot b}{\left(2a\right)^2+b}\right]\right]^2+\frac{b^3}{\left[\left(2a\right)^2+b\right]^2}}</math> | + | | |
| + | |- | ||
| + | | | ||
| + | :If you wish to come closer to the truth, [divide] the cube of the above remainder by the denominator squared. | ||
|style="text-align:right;"|ואם תרצה להתקרב יותר אל האמת קח מעוקב הנשאר הנזכר מהמורה כפול | |style="text-align:right;"|ואם תרצה להתקרב יותר אל האמת קח מעוקב הנשאר הנזכר מהמורה כפול | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle\sqrt{3}</math> | + | *I.e. if we wish to know the root [of 3]. |
| + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{3}}}</math> | ||
|style="text-align:right;"|ר"ל שאם רצינו לדעת שרש | |style="text-align:right;"|ר"ל שאם רצינו לדעת שרש | ||
|- | |- | ||
| | | | ||
| + | :Double the root is added to the remainder; the total is six and the remainder at the beginning is two. | ||
:<math>\scriptstyle{\color{blue}{\sqrt{3}\approx1+\frac{2\sdot1\sdot\left(3-1\right)}{\left(2\sdot1\right)^2+\left(3-1\right)}=1+\frac{2\sdot1\sdot2}{\left(2\sdot1\right)^2+2}=1+\frac{4}{6}}}</math> | :<math>\scriptstyle{\color{blue}{\sqrt{3}\approx1+\frac{2\sdot1\sdot\left(3-1\right)}{\left(2\sdot1\right)^2+\left(3-1\right)}=1+\frac{2\sdot1\sdot2}{\left(2\sdot1\right)^2+2}=1+\frac{4}{6}}}</math> | ||
| − | |style="text-align:right;"|והיה כפל השרש {{#annot:term| | + | |style="text-align:right;"|והיה כפל השרש {{#annot:term|178,2083|Fg9j}}מחובר עם{{#annotend:Fg9j}} הנשאר היה הכל ששה והנשאר בתחלה היו שתים |
|- | |- | ||
| | | | ||
| + | :Take the cube of two, which is eight, name it by a sixth of a sixth, i.e. 8 that is called a sixth [= whose denominator is six] and this is the excess of the square over double the last [approximate] root. | ||
::<math>\scriptstyle{\color{blue}{3-\left(1+\frac{4}{6}\right)^2=\frac{2^3}{\left[\left(2\sdot1\right)^2+2\right]^2}=\frac{8}{6}\sdot\frac{1}{6}}}</math> | ::<math>\scriptstyle{\color{blue}{3-\left(1+\frac{4}{6}\right)^2=\frac{2^3}{\left[\left(2\sdot1\right)^2+2\right]^2}=\frac{8}{6}\sdot\frac{1}{6}}}</math> | ||
|style="text-align:right;"|תקח מעוקב השנים שהוא שמונה ותקרא לו שם משישית שישית ר"ל ח' ששמה שישית וזה יהיה הנשאר במרובע על כפל השרש האחרון | |style="text-align:right;"|תקח מעוקב השנים שהוא שמונה ותקרא לו שם משישית שישית ר"ל ח' ששמה שישית וזה יהיה הנשאר במרובע על כפל השרש האחרון | ||
|- | |- | ||
| | | | ||
| + | :Do with it and with the last [approximate] root as you did with the first remainder and the first [approximate] root; the resulting root is 1 integer and 112 parts of 153. | ||
:<math>\scriptstyle{\color{blue}{\sqrt{3}\approx\left(1+\frac{4}{6}\right)+\frac{2\sdot\left(1+\frac{4}{6}\right)\sdot\left(\frac{8}{6}\sdot\frac{1}{6}\right)}{\left[2\sdot\left(1+\frac{4}{6}\right)\right]^2+\left(\frac{8}{6}\sdot\frac{1}{6}\right)}=1+\frac{112}{153}}}</math> | :<math>\scriptstyle{\color{blue}{\sqrt{3}\approx\left(1+\frac{4}{6}\right)+\frac{2\sdot\left(1+\frac{4}{6}\right)\sdot\left(\frac{8}{6}\sdot\frac{1}{6}\right)}{\left[2\sdot\left(1+\frac{4}{6}\right)\right]^2+\left(\frac{8}{6}\sdot\frac{1}{6}\right)}=1+\frac{112}{153}}}</math> | ||
|style="text-align:right;"|ותעשה ממנו עם זה השרש האחרון כמו שעשית לשארית הראשון עם השרש הראשון ויעלה כל השרש א' שלם וקי"ב חלקים מקנ"ג בשלם | |style="text-align:right;"|ותעשה ממנו עם זה השרש האחרון כמו שעשית לשארית הראשון עם השרש הראשון ויעלה כל השרש א' שלם וקי"ב חלקים מקנ"ג בשלם | ||
|- | |- | ||
| | | | ||
| + | ::Whose square is 3 integers minus 2 parts of the square of 153. | ||
::<math>\scriptstyle{\color{blue}{\left(1+\frac{112}{153}\right)^2=3-\frac{2}{153^2}}}</math> | ::<math>\scriptstyle{\color{blue}{\left(1+\frac{112}{153}\right)^2=3-\frac{2}{153^2}}}</math> | ||
|style="text-align:right;"|שמרובעו הוא ג' שלימים חסר ב' חלקים ממרובע קנ"ג בשלם | |style="text-align:right;"|שמרובעו הוא ג' שלימים חסר ב' חלקים ממרובע קנ"ג בשלם | ||
|- | |- | ||
| − | | | + | |''See, indeed, see'' [Samuel 1 24, 12], you have come closer to the truth, so that between the square of your root and the required square there is only one of a thousand and this is enough. |
| − | |style="text-align:right;"|וראה גם ראה גם נתקרבת אל האמת שאין ממרובע השרשך למרובע הנשאל אחד מרבבה בשלם ודי | + | |style="text-align:right;"|''וראה גם ראה''‫<ref group=note>שמואל א כד, יב</ref> גם נתקרבת אל האמת שאין ממרובע השרשך למרובע הנשאל אחד מרבבה בשלם ודי |
|- | |- | ||
|} | |} | ||
| Line 4,515: | Line 5,431: | ||
= Section Two: Fractions = | = Section Two: Fractions = | ||
| − | + | |style="text-align:right;"|‫<ref>41r</ref><big>החלק השני בשברים</big> | |
|- | |- | ||
| | | | ||
| Line 4,521: | Line 5,437: | ||
| | | | ||
|- | |- | ||
| − | |Before the chapters, I will open with introduction that consist of three chapters: | + | |Before the chapters, I will open with an introduction that consist of three chapters: |
|style="text-align:right;"|לפני הפרקים אקדים הקדמה אחת ובה שלשה פרקים | |style="text-align:right;"|לפני הפרקים אקדים הקדמה אחת ובה שלשה פרקים | ||
|- | |- | ||
| | | | ||
| − | *Chapter one on | + | *Chapter one on decomposing to a fraction |
|style="text-align:right;"|השער הא' בפריטה | |style="text-align:right;"|השער הא' בפריטה | ||
|- | |- | ||
| Line 4,533: | Line 5,449: | ||
|- | |- | ||
| | | | ||
| − | *Chapter three on | + | *Chapter three on expansion to a common denominator |
|style="text-align:right;"|השער השלישי בהשואה | |style="text-align:right;"|השער השלישי בהשואה | ||
|- | |- | ||
| | | | ||
| − | === Chapter One on | + | === Chapter One on Decomposing to a Fraction === |
| − | |style="width:45%; text-align:right;"| | + | |style="width:45%; text-align:right;"|<big>השער הראשון</big> בפריטה |
|- | |- | ||
| − | | | + | |<span style=color:Green>Definition:</span> {{#annot:definition|1561,1937|xxm5}}Decomposing to a fraction is converting the integers to fractions of whichever type you wish. |
| − | |||
|style="text-align:right;"|הפריטה היא {{#annot:term|2612,2489|6pAM}}חזרת השלימים לחלקים{{#annotend:6pAM}} מהמין אשר תרצה{{#annotend:xxm5}} | |style="text-align:right;"|הפריטה היא {{#annot:term|2612,2489|6pAM}}חזרת השלימים לחלקים{{#annotend:6pAM}} מהמין אשר תרצה{{#annotend:xxm5}} | ||
|- | |- | ||
| Line 4,553: | Line 5,468: | ||
|- | |- | ||
|Example for integers and fractions: | |Example for integers and fractions: | ||
| − | :<math>\scriptstyle{\color{ | + | :<math>\scriptstyle{\color{OliveGreen}{n+\frac{a}{b}=\frac{\left(n\sdot b\right)+a}{b}}}</math> |
|style="text-align:right;"|<big>המשל</big> שלימים בשברים | |style="text-align:right;"|<big>המשל</big> שלימים בשברים | ||
|- | |- | ||
| Line 4,564: | Line 5,479: | ||
:The integers are converted to sevenths, which is the type of fractions that are with them, by multiplying these three integers by the denominator of the sevenths, which is 7. The result is 21. By adding the 5 sevenths to them, the total is 26 sevenths. | :The integers are converted to sevenths, which is the type of fractions that are with them, by multiplying these three integers by the denominator of the sevenths, which is 7. The result is 21. By adding the 5 sevenths to them, the total is 26 sevenths. | ||
:<math>\scriptstyle{\color{blue}{3+\frac{5}{7}=\frac{\left(3\sdot7\right)+5}{7}=\frac{21+5}{7}=\frac{26}{7}}}</math> | :<math>\scriptstyle{\color{blue}{3+\frac{5}{7}=\frac{\left(3\sdot7\right)+5}{7}=\frac{21+5}{7}=\frac{26}{7}}}</math> | ||
| − | |style="text-align:right;"|הנה השילימים ישובו שביעיות שהוא מין שברים שעמו {{#annot:term|156,2136|LxoE}}בהכפל{{#annotend:LxoE}} אלו השלשה שלימים במורה השביעיות שהוא הז' ויעלו כ"א ובחברך אליהם הה' שביעיות אשר עמהם יהיו הכל כ"ו שלימים שביעיות | + | |style="text-align:right;"|הנה השילימים ישובו שביעיות שהוא מין שברים שעמו {{#annot:term|156,2136|LxoE}}בהכפל{{#annotend:LxoE}} אלו השלשה שלימים במורה השביעיות שהוא הז' ויעלו כ"א ובחברך אליהם הה' שביעיות אשר עמהם יהיו הכל כ"ו <s>שלימים</s> שביעיות |
|- | |- | ||
|All this is seen clear and its reason is explained in the examination of the [divisors] as clarified in chapter four - this is the rule and the reason. | |All this is seen clear and its reason is explained in the examination of the [divisors] as clarified in chapter four - this is the rule and the reason. | ||
| Line 4,570: | Line 5,485: | ||
|- | |- | ||
|If you have fractions and fractions of fractions, multiply the fractions by the denominator of the fractions of fractions, then add to them the fractions of fractions. | |If you have fractions and fractions of fractions, multiply the fractions by the denominator of the fractions of fractions, then add to them the fractions of fractions. | ||
| − | :<math>\scriptstyle{\color{ | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{g}{b}+\left(\frac{a}{b}\sdot\frac{c}{d}\right)=\frac{\left(g\sdot d\right)+\left(a\sdot c\right)}{b}\sdot\frac{1}{d}}}</math> |
| − | |style="text-align:right;"|כי אם אין בידיך כי אם שברים ושברי שברים שתכפול השברים במורה השברי שברים ושבר שברים שתכפול ותחבר אליהם השברי שברים וכן לעולם | + | |style="text-align:right;"|כי אם אין בידיך כי אם שברים ושברי שברים שתכפול השברים במורה השברי שברים <s>ושבר שברים שתכפול</s> ותחבר אליהם השברי שברים וכן לעולם |
|- | |- | ||
|I will give one example for all this: | |I will give one example for all this: | ||
| − | |style="text-align:right;"|ואביא משל א' לכל זה | + | |style="text-align:right;"|ואביא משל <sup>א'</sup> לכל זה |
|- | |- | ||
| | | | ||
| Line 4,606: | Line 5,521: | ||
| | | | ||
:*We convert them to eighths of quarters of a fifth by multiplying them by 8, the result is 496. We add to them the 4 that is beneath [the 8], which is of their type. The total is 500. | :*We convert them to eighths of quarters of a fifth by multiplying them by 8, the result is 496. We add to them the 4 that is beneath [the 8], which is of their type. The total is 500. | ||
| − | |||
|style="text-align:right;"|נשיבם שמיניות רביעיות חמישית וזה בשנכפלם בח' יעלה תצ"ו נחבר להם הד' אשר תחתיו שהם ממינם יעלה הכל ת"ק | |style="text-align:right;"|נשיבם שמיניות רביעיות חמישית וזה בשנכפלם בח' יעלה תצ"ו נחבר להם הד' אשר תחתיו שהם ממינם יעלה הכל ת"ק | ||
| + | |} | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{62}{4}\sdot\frac{1}{5}\right)+\left(\frac{4}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}\right)=\frac{\left(62\sdot8\right)+4}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}=\frac{496+4}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}=\frac{500}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}}}</math> | ||
| + | {| | ||
|- | |- | ||
| | | | ||
:*We convert them to ninths of eighths of quarters of a fifth by multiplying them by 9, the result is 4500. Since we do not find anything beneath [the 9] we do not add anything to them. | :*We convert them to ninths of eighths of quarters of a fifth by multiplying them by 9, the result is 4500. Since we do not find anything beneath [the 9] we do not add anything to them. | ||
::<math>\scriptstyle{\color{blue}{\frac{500}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}=\frac{500\sdot9}{9}\sdot\frac{1}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}=\frac{4500}{9}\sdot\frac{1}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{500}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}=\frac{500\sdot9}{9}\sdot\frac{1}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}=\frac{4500}{9}\sdot\frac{1}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}}}</math> | ||
| − | |style="text-align:right;"|נשיבם תשיעיות שמינית רביעית חמישית וזה בשנכפלם בט' יעלו 4500 ואחרי שלא נמצא תחתיו דבר לא נחבר אליהם דבר | + | |style="width:45%; text-align:right;"|נשיבם תשיעיות שמינית רביעית חמישית וזה בשנכפלם בט' יעלו 4500 ואחרי שלא נמצא תחתיו דבר לא נחבר אליהם דבר |
|- | |- | ||
| | | | ||
:*We convert them to thirds of ninths of eighths of quarters of a fifth by multiplying them by 3, the result is 13500. We add to them the 2 that is beneath [the 3], which is of their type. The total is 13502 and we completed the procedure. | :*We convert them to thirds of ninths of eighths of quarters of a fifth by multiplying them by 3, the result is 13500. We add to them the 2 that is beneath [the 3], which is of their type. The total is 13502 and we completed the procedure. | ||
| − | |||
|style="text-align:right;"|אבל נשיבם שלישיות תשיעית שמינית רביעית חמישית והוא שנכפלם בג' יעלו 13500 נחבר אליהם הב' אשר תחתיו שהוא ממינם יעלה הכל 13502 וכלינו כל מלאכתנו | |style="text-align:right;"|אבל נשיבם שלישיות תשיעית שמינית רביעית חמישית והוא שנכפלם בג' יעלו 13500 נחבר אליהם הב' אשר תחתיו שהוא ממינם יעלה הכל 13502 וכלינו כל מלאכתנו | ||
| + | |} | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{4500}{9}\sdot\frac{1}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}\right)+\left(\frac{2}{3}\sdot\frac{1}{9}\sdot\frac{1}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}\right)=\frac{\left(4500\sdot3\right)+2}{3}\sdot\frac{1}{9}\sdot\frac{1}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}=\frac{13500+2}{3}\sdot\frac{1}{9}\sdot\frac{1}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}=\frac{13502}{3}\sdot\frac{1}{9}\sdot\frac{1}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}}}</math> | ||
| + | {| | ||
|- | |- | ||
| | | | ||
*If there are no integers there at all. | *If there are no integers there at all. | ||
| − | :<math>\scriptstyle{\color{ | + | :<math>\scriptstyle{\color{OliveGreen}{\left(\frac{2}{4}\sdot\frac{1}{5}\right)+\left(\frac{4}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}\right)+\left(\frac{2}{3}\sdot\frac{1}{9}\sdot\frac{1}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}\right)}}</math> |
| − | |style="text-align:right;"|ואם לא היה שם שלמים כלל | + | |style="width:45%; text-align:right;"|ואם לא היה שם שלמים כלל |
|- | |- | ||
| | | | ||
:*We should start from the 2 that is beneath the first denominator beneath which there is a number, even if it were the second of the denominators. We multiply them by 8, which is the next denominator. The result is 16. We add to them the 4 that is beneath [the 8]. The result is 20. | :*We should start from the 2 that is beneath the first denominator beneath which there is a number, even if it were the second of the denominators. We multiply them by 8, which is the next denominator. The result is 16. We add to them the 4 that is beneath [the 8]. The result is 20. | ||
| − | |||
|style="text-align:right;"|היה לנו להתחיל מהב' אשר תחת המורה הראשון אשר תחתיו מספר מה ואם הוא שני לחשבון המורים והיה לנו לכפלם בח' שהוא המורה הסמוך ויעלו י"ו ולחבר להם הד' אשר תחתיו ויעלו כ‫' | |style="text-align:right;"|היה לנו להתחיל מהב' אשר תחת המורה הראשון אשר תחתיו מספר מה ואם הוא שני לחשבון המורים והיה לנו לכפלם בח' שהוא המורה הסמוך ויעלו י"ו ולחבר להם הד' אשר תחתיו ויעלו כ‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{2}{4}\sdot\frac{1}{5}\right)+\left(\frac{4}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}\right)=\frac{\left(2\sdot8\right)+4}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}=\frac{16+4}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}=\frac{20}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}}}</math> | ||
| + | | | ||
|- | |- | ||
| | | | ||
::Then we multiply them by 9. The result is 180. We multiply them also by 3. The result is 540. We add to them the 2 that is beneath [the 3]. The total is 542. | ::Then we multiply them by 9. The result is 180. We multiply them also by 3. The result is 540. We add to them the 2 that is beneath [the 3]. The total is 542. | ||
| − | |||
|style="text-align:right;"|ונכפלם עוד בט' יהיו ק"פ נכפלם עוד בג' יעלו 540 נחבר להם הב' אשר תחתיו ויעלה הכל 542 | |style="text-align:right;"|ונכפלם עוד בט' יהיו ק"פ נכפלם עוד בג' יעלו 540 נחבר להם הב' אשר תחתיו ויעלה הכל 542 | ||
| + | |} | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left(\frac{20}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}\right)+\left(\frac{2}{3}\sdot\frac{1}{9}\sdot\frac{1}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}\right)&\scriptstyle=\left(\frac{20\sdot9}{9}\sdot\frac{1}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}\right)+\left(\frac{2}{3}\sdot\frac{1}{9}\sdot\frac{1}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}\right)=\frac{\left(180\sdot3\right)+2}{3}\sdot\frac{1}{9}\sdot\frac{1}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}\\&\scriptstyle=\frac{540+2}{3}\sdot\frac{1}{9}\sdot\frac{1}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}=\frac{542}{3}\sdot\frac{1}{9}\sdot\frac{1}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}\\\end{align}}}</math> | ||
| + | {| | ||
|- | |- | ||
|Thus, we have everything explained by the procedure and by reason - how everything is converted into the final type, whether there are integers with fractions, or there are no integers there; the result is of the final type. | |Thus, we have everything explained by the procedure and by reason - how everything is converted into the final type, whether there are integers with fractions, or there are no integers there; the result is of the final type. | ||
| − | |style="text-align:right;"|הרי לנו הכל מפורש במעשה ובטעם איך ישוב הכל מהמין האחרון בין אם יש שלמים עם שברים בין אם אין שם שלמים והיוצא באחרונה הם מהמין האחרון | + | |style="width:45%; text-align:right;"|הרי לנו הכל מפורש במעשה ובטעם איך ישוב הכל מהמין האחרון בין אם יש שלמים עם שברים בין אם אין שם שלמים והיוצא באחרונה הם מהמין האחרון |
|- | |- | ||
|I.e. for the result in our mentioned example are thirds of a ninth of an eighth of a quarter of a fifth. | |I.e. for the result in our mentioned example are thirds of a ninth of an eighth of a quarter of a fifth. | ||
| Line 4,645: | Line 5,569: | ||
| | | | ||
| − | === Chapter Two on Multiplication === | + | === Chapter Two on Multiplication <span style=color:Green>[= compound fractions]</span> === |
|style="width:45%; text-align:right;"|<big>השער השני בהכאה</big> | |style="width:45%; text-align:right;"|<big>השער השני בהכאה</big> | ||
|- | |- | ||
| − | |The multiplication [= compound fractions] is when the fractions are not [fractions] of one integer, or of one fraction, but they are [fractions] of a number of integers or a number of fractions. | + | |<span style=color:Green>Definition:</span> The multiplication [= compound fractions] is when the fractions are not [fractions] of one integer, or of one fraction, but they are [fractions] of a number of integers or a number of fractions. |
|style="text-align:right;"|<sup>ההכאה</sup> היא כאשר השברים אינם שברים <ref>42r</ref>משלם אחד או משבר אחד אבל הם ממספר שלמים או ממספר שברים | |style="text-align:right;"|<sup>ההכאה</sup> היא כאשר השברים אינם שברים <ref>42r</ref>משלם אחד או משבר אחד אבל הם ממספר שלמים או ממספר שברים | ||
|- | |- | ||
| Line 4,673: | Line 5,597: | ||
|style="text-align:right;"|והנה אומרנו שני חמישיו' מג' רביעיות מה' שלמים הוא כאומרנו שלקחנו ה' שלמים ועשינו מהם ד' חלקי' שוים ולקחנו הג' מהם שזהו ג' רביעיות מה' שלמים וחלקנו עוד אלו הג' חלקים לה' חלקים שוים ולקחנו הב' מהם שזהו פי' ב' חמשיות מג' רביעיות מה' שלמים | |style="text-align:right;"|והנה אומרנו שני חמישיו' מג' רביעיות מה' שלמים הוא כאומרנו שלקחנו ה' שלמים ועשינו מהם ד' חלקי' שוים ולקחנו הג' מהם שזהו ג' רביעיות מה' שלמים וחלקנו עוד אלו הג' חלקים לה' חלקים שוים ולקחנו הב' מהם שזהו פי' ב' חמשיות מג' רביעיות מה' שלמים | ||
|- | |- | ||
| − | |The fractions here are of one type only, therefore, there is no need for | + | |The fractions here are of one type only, therefore, there is no need for decomposing to a fraction at all. |
|style="text-align:right;"|ואין כאן שברים כי אם ממין אחד ואינך צריך לעשות פריטה כלל | |style="text-align:right;"|ואין כאן שברים כי אם ממין אחד ואינך צריך לעשות פריטה כלל | ||
|- | |- | ||
| Line 4,700: | Line 5,624: | ||
| | | | ||
|- | |- | ||
| − | |Sometimes the fractions and fractions of fractions are of a number of fractions or integers and for this you should apply both operations i.e. | + | |Sometimes the fractions and fractions of fractions are of a number of fractions or integers and for this you should apply both operations i.e. decomposing to a fraction and multiplication. |
|style="text-align:right;"|ולפעמים יהיה כמספר שברים ושברי שברים משבר אחת גם ממספר שברים או שלמים ולזה תצטרך לעשות שני <s>דברים</s> <sup>המעשים</sup> ר"ל הפריטה והכאה | |style="text-align:right;"|ולפעמים יהיה כמספר שברים ושברי שברים משבר אחת גם ממספר שברים או שלמים ולזה תצטרך לעשות שני <s>דברים</s> <sup>המעשים</sup> ר"ל הפריטה והכאה | ||
|- | |- | ||
| Line 4,728: | Line 5,652: | ||
|- | |- | ||
| | | | ||
| − | :First | + | :First decompose each of them to a fraction: |
|style="text-align:right;"|ועשה הפריטה לכל אחד מהם תחלה | |style="text-align:right;"|ועשה הפריטה לכל אחד מהם תחלה | ||
|- | |- | ||
| | | | ||
| − | :* | + | :*Decompose the 3 sevenths of an eighth that are related [to the four fifths of sevenths of an eighth] by multiplying them by each other, that is by multiplying the 3 that is the number of the [sevenths] by 5, which is the next denominator. The result is 15. We add to them the 4 that is beneath [the 5], which is of the same type. The total is 19. |
| − | |style="text-align:right;"|ונעשה {{#annot:term|1561,1937|YyGS}}פריטה{{#annotend:YyGS}} לג' שביעיות שמינית שהן {{#annot:term| | + | |style="text-align:right;"|ונעשה {{#annot:term|1561,1937|YyGS}}פריטה{{#annotend:YyGS}} לג' שביעיות שמינית שהן {{#annot:term|1567,1494|dGUb}}נקשרות{{#annotend:dGUb}} <s>בשנכפול</s> זו בזו וזה בשנכפול הג' שהם מספ' השברים בה' שהוא המורה הסמוך ויעלו ט"ו ונחבר להם הד' אשר תחתיו שהם ממין זה יהיו כלם י"ט |
|- | |- | ||
| | | | ||
| Line 4,740: | Line 5,664: | ||
|- | |- | ||
| | | | ||
| − | :* | + | :*We decompose 2-quarters and 3 fifths of a quarter that are also related, by multiplying 2 by 5. The result is 10. We add to them the 3 that is beneath [the 5]. The result is 13. |
|style="text-align:right;"|עוד נעשה פריטה לב' רביעיות וג' <s>נחשת</s> חמשיות רביעית שהם ג"כ נקשרות וזה שנכפול הב' בה' ויעלו י' ונחבר להם הג' אשר תחתיו ויעלו י"ג | |style="text-align:right;"|עוד נעשה פריטה לב' רביעיות וג' <s>נחשת</s> חמשיות רביעית שהם ג"כ נקשרות וזה שנכפול הב' בה' ויעלו י' ונחבר להם הג' אשר תחתיו ויעלו י"ג | ||
|- | |- | ||
| Line 4,780: | Line 5,704: | ||
| | | | ||
:*We took 3 parts of these 9 latter together and divided them into 8 equal parts. | :*We took 3 parts of these 9 latter together and divided them into 8 equal parts. | ||
| − | |style="text-align:right;"|ולקחנו]<ref>marg.</ref> ג' חלקים מאלו הט' האחרונים ביחד ועשינו ח' חלקים שוים | + | |style="text-align:right;"|ולקחנו]‫<ref>marg.</ref> ג' חלקים מאלו הט' האחרונים ביחד ועשינו ח' חלקים שוים |
|- | |- | ||
| | | | ||
| Line 4,796: | Line 5,720: | ||
| | | | ||
:We should understand, since it is said "of 19 fifths" etc. and it is said "of 3 ninths" etc. and it said "of 4 integers", that from this we know that they are not of one fraction nor of one integer, but of a number of integers and fractions. | :We should understand, since it is said "of 19 fifths" etc. and it is said "of 3 ninths" etc. and it said "of 4 integers", that from this we know that they are not of one fraction nor of one integer, but of a number of integers and fractions. | ||
| − | |style="text-align:right;"|והננו צריכים להבנה לפי שאמרו מי"ט חמישיות וכו' גם לאומרם מג' תשיעיות וכו' גם לאומרם מד' שלמים כי בזה ידענו שאינם משבר אחד אף לא משלם אחד כי מספר שלמים | + | |style="text-align:right;"|והננו צריכים להבנה לפי שאמרו מי"ט חמישיות וכו' גם לאומרם מג' תשיעיות וכו' גם לאומרם מד' שלמים כי בזה ידענו שאינם משבר אחד אף לא משלם אחד כי מספר שלמים <sup>ו</sup>ממספר <s>ש</s> שברים |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|לכן נכה מספר השברים אשר בידינו במספר השברים אשר הזכירו גם במספר השלמים זה אחר זה | + | :Therefore, we multiply the number of the fractions we have by the number of the fractions that are mentioned and by the number of integers, one after the other. |
| + | |style="text-align:right;"|‫<ref>43r</ref>לכן נכה מספר השברים אשר בידינו במספר השברים אשר הזכירו גם במספר השלמים זה אחר זה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|וזה כי אומרנו י"ג חמישיות רביעיות י"ט חמישיות וכו' הוא כאומרנו י"ט פעמי' י"ג חמישיות [רביעית חמישית] וכו' לכן נכפול הי"ג בי"ט ויעלו 247 חמישיות רביעיות חמישית וכו‫' | + | ::Because, our saying: "13 fifths of quarters of 19 fifths" etc. is as our saying "19 times 13 fifths of a quarter of a fifth" etc. |
| + | |style="text-align:right;"|וזה כי אומרנו י"ג חמישיות רביעיות י"ט חמישיות וכו' הוא כאומרנו י"ט פעמי' י"ג חמישיות [רביעית חמישית]‫<ref>marg.</ref> וכו‫' | ||
| + | |- | ||
| + | | | ||
| + | :So, we multiply 13 by 19; the result is 247 fifths of quarters of a fifth etc. | ||
| + | |style="text-align:right;"|לכן נכפול הי"ג בי"ט ויעלו 247 חמישיות רביעיות חמישית וכו‫' | ||
|- | |- | ||
| | | | ||
| Line 4,809: | Line 5,739: | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|גם כאשר אמרו לנו מג' תשיעיות הוא כאלו אמרו לנו ג' פעמים כל אשר בידינו [ולזה נכפול כל אשר בידינו] שהוא 247 בג' ויעלה 741 והם חמישיות רביעיות חמישיות שביעיות שמיניות | + | ::Also, when we are told: "of 3-ninths", it is as if we are told: "3 times all that we have". |
| + | |style="text-align:right;"|גם כאשר אמרו לנו מג' תשיעיות הוא כאלו אמרו לנו ג' פעמים כל אשר בידינו | ||
| + | |- | ||
| + | | | ||
| + | :So, we multiply all that we have, which is 247, by 3; the result is 741, which are fifths of quarters of fifths of sevenths of eighths of ninths of a tenth etc. | ||
| + | |style="text-align:right;"|‫[ולזה נכפול כל אשר בידינו]‫<ref>marg.</ref> שהוא 247 בג' ויעלה 741 והם חמישיות רביעיות חמישיות שביעיות שמיניות תשיעי<s>ו</s>ת עשירית וכו‫' | ||
|} | |} | ||
::<math>\scriptstyle{\color{blue}{\left(\frac{247}{5}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{7}\sdot\frac{1}{8}\right)\sdot\left(\frac{3}{9}\sdot\frac{1}{10}\right)=\frac{247\sdot3}{5}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}\sdot\frac{1}{10}=\frac{741}{5}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}\sdot\frac{1}{10}}}</math> | ::<math>\scriptstyle{\color{blue}{\left(\frac{247}{5}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{7}\sdot\frac{1}{8}\right)\sdot\left(\frac{3}{9}\sdot\frac{1}{10}\right)=\frac{247\sdot3}{5}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}\sdot\frac{1}{10}=\frac{741}{5}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}\sdot\frac{1}{10}}}</math> | ||
| Line 4,815: | Line 5,750: | ||
|- | |- | ||
| | | | ||
| − | |style="width:45%; text-align:right;"|ולפי שאמרו לנו מד' שלמים הוא כאלו אמרו לנו ד' פעמים כל אשר בידינו לכן כל אשר בידינו שהוא 741 בארבעה ויעלה 2964 חמישיות רביעית חמישית שביעית שמינית | + | ::As we are told: "of 4 integers", it is as if we are told: "4 times all that we have". |
| + | |style="width:45%; text-align:right;"|ולפי שאמרו לנו <sup>מד'</sup> שלמים הוא כאלו אמרו לנו ד' פעמים כל אשר בידינו | ||
| + | |- | ||
| + | | | ||
| + | :So, [we multiply] all that we have, which is 741, by four; the result is 2964-fifths of a quarter of a fifth of a seventh of an eighth of a ninth of a tenth. | ||
| + | |style="text-align:right;"|לכן כל אשר בידינו שהוא 741 בארבעה ויעלה 2964 חמישיות רביעית חמישית שביעית שמינית <sup>ת</sup>שיעית עשירית | ||
|} | |} | ||
::<math>\scriptstyle{\color{blue}{\left(\frac{741}{5}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}\sdot\frac{1}{10}\right)\sdot4=\frac{741\sdot4}{5}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}\sdot\frac{1}{10}=\frac{2964}{5}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}\sdot\frac{1}{10}}}</math> | ::<math>\scriptstyle{\color{blue}{\left(\frac{741}{5}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}\sdot\frac{1}{10}\right)\sdot4=\frac{741\sdot4}{5}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}\sdot\frac{1}{10}=\frac{2964}{5}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}\sdot\frac{1}{10}}}</math> | ||
{| | {| | ||
|- | |- | ||
| − | | | + | |Always remember that the number you find on top of another number is the denominator, not the bottom [number]. |
|style="width:45%; text-align:right;"|וזכור לעולם כי המספר אשר תמצא על ראשו מספר אחר שהתחתון איננו מורה כי העליון | |style="width:45%; text-align:right;"|וזכור לעולם כי המספר אשר תמצא על ראשו מספר אחר שהתחתון איננו מורה כי העליון | ||
|- | |- | ||
| − | | | + | |If we want to know how many integers, or fractions, or fractions of fractions are these decomposed fractions: |
| − | |style="text-align:right;"|ואם בקשנו לידע כלם אלו החלקים הנפרטו' כמה שלמים או כמה שברים או שברי שברים מאלו הם כבר ידעת שיש כאן שבעה מורים ותושיבם כרצונך או כסדרם עתה או בהשגחה כדי שיצאו החלקים יותר נאותים כי הסדר לא יזיק לעולם כי אם התוספת בהם או ה{{#annot:term|155,1193|9ZbD}}מגרעת{{#annotend:9ZbD}} כאשר ביארנו בפרק הד‫' | + | |style="text-align:right;"|ואם בקשנו לידע כלם אלו החלקים הנפרטו' כמה שלמים או כמה שברים או שברי שברים מאלו הם |
| + | |- | ||
| + | |You already know that there are seven denominators. Arrange them as you wish, according to their order now, or calculatedly, in order that the fractions will be more proper. For the order is harmless, except in addition and subtraction, as we explain in chapter 4. | ||
| + | |style="text-align:right;"|כבר ידעת שיש כאן שבעה מורים ותושיבם כרצונך או כסדרם עתה או בהשגחה כדי שיצאו החלקים יותר נאותים כי הסדר לא יזיק לעולם כי אם התוספת בהם או ה{{#annot:term|155,1193|9ZbD}}מגרעת{{#annotend:9ZbD}} כאשר ביארנו בפרק הד‫' | ||
| + | |- | ||
| + | | | ||
| + | :We divide by them the 2964, which is the decomposed number that we have, and we call it a reduced fraction [lit. perfect beauty]. | ||
| + | |style="text-align:right;"|ונחלק עליהם 2964 שהוא מספר אשר בידינו נפרטות וקראנו לזה כלילת יופי | ||
| + | |- | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Since this number has a quarter, we place [the 4] last, in order that it will be removed and we divide [2964] by 4; the result of division is 741 and nothing remains. | ||
| + | |style="text-align:right;"|ואחר שיש לחשבון רביעית נשימהו לאחרון כדי שיתבטל ונחלקם על ד' ויצא בחילוק 741 ולא ישאר דבר | ||
| + | |- | ||
| + | | | ||
| + | :This result of division is indivisible by the remaining denominators, therefore we place whichever we want before the last that we wrote. Let it be 5. We divide it by 5; the result of division is 148 and 1 remains. We write it beneath it. | ||
| + | |style="text-align:right;"|וזה היוצא בחלוק אין לו אחד מהמורים הנשארים לכן נשים אשר נספק לפני האחרון אשר שמנו ויהיה ה' ונחלקם על הה' ויצא בחלוק 148 וישאר א' ונשימנו תחתיו | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{\begin{align} | + | :Since this number has a quarter, we factorize the denominator of the eighth, which is 8, to 4 and 2 and write them instead of it; this indicates half of a quarter, of a quarter of a half, or an eighth. We shall explain it at the end of the book. After we have factorized it, i.e. removed it and placed 2 and 4 instead of it, we write 4 before the written denominators and divide what we have by it; the result of division are 37 [parts]. |
| − | + | |style="text-align:right;"|ואחר שיש לו החשבון ‫<ref>43v</ref>רביעית {{#annot:term|2615,1559|WuM8}}נתיך{{#annotend:WuM8}} המורה השמינית שהוא הח' ונעשה ממנו ב'ד' ונשימם במקומו כך הוא הוראת חצי רביעית או רביעית חצי [כמו]‫<ref>marg.</ref> <s>או</s> שמינית ועוד נבאר זה בסוף הספר ואחר {{#annot:term|2615,1559|4u2l}}התיכנו אותו{{#annotend:4u2l}} ר"ל שנסירהו ונשים במקומו ב'ד' נשים הד' לפני המורי' המושמים ונחלק לו אשר בידינו ויצא בחלוק ל"ז <s>חלקים</s> | |
| − | + | |- | |
| − | + | | | |
| − | + | :We divide them by whichever we want. Let it be 7. The result of division is 5 and 2 remains. We write it beneath it. | |
| − | + | |style="text-align:right;"|ונחלקם לאשר נחפוץ ויהיה על הז' ויצא בחילוק ה' וישארו ב' ונשימם תחתיו | |
| − | + | |- | |
| − | + | | | |
| + | :We divide the 5 resulted from the division by the denominator of the first fifth; the result of division is 1 and nothing remains. | ||
| + | |style="text-align:right;"|ונחלק הה' שיצאו בחלוק למורה הה' הא' ויצא א' בחלוק ולא ישאר דבר | ||
| + | |- | ||
| + | | | ||
| + | :Since the result of division is less than the smallest denominators mentioned, we should not divide further, but write them orderly before those that were written calculatedly. We write the one resulted from the last division beneath the denominator that is next to those that were written up to this phase. | ||
| + | |style="text-align:right;"|ואחר שאשר יצא בחלוק הוא פחות מהקטן שבכל המורים הנזכרים אין לנו לחלק עוד אבל נשימם על הסדר לפני המושמים בכל השגחה ונשים זה האחד אשר יצא בחילוק באחרונה תחת המורה הסמוך למושמים עד הנה | ||
| + | |- | ||
| + | | | ||
| + | :Thus, we get what we wanted and it is that the required at first is half a ninth of a tenth, two-sevenths of a fifth of a half of a ninth of a tenth, and a fifth of a quarter of a seventh etc. | ||
| + | |style="text-align:right;"|והנה יצא לנו מבוקשינו והוא שהנשאל לנו תחלה עולה חצי תשיעית עשירית ושתי שביעיות חמישית חצי תשיעית עשירית וחמישית רביעית שביעית וכו‫' | ||
| + | |} | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left[\frac{2}{4}+\left(\frac{3}{5}\sdot\frac{1}{4}\right)\right]\sdot\left[\left(\frac{3}{7}\sdot\frac{1}{8}\right)+\left(\frac{4}{5}\sdot\frac{1}{7}\sdot\frac{1}{8}\right)\right]\sdot\left(\frac{3}{9}\sdot\frac{1}{10}\sdot4\right)&\scriptstyle=\frac{2964}{5}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}\sdot\frac{1}{10}=\frac{2964}{4}\sdot\frac{1}{5}\sdot\frac{1}{5}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}\sdot\frac{1}{10}\\&\scriptstyle=\frac{741}{5}\sdot\frac{1}{5}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}\sdot\frac{1}{10}\\&\scriptstyle=\left(\frac{148}{4}\sdot\frac{1}{2}\sdot\frac{1}{7}\sdot\frac{1}{5}\sdot\frac{1}{9}\sdot\frac{1}{10}\right)+\left(\frac{1}{5}\sdot\frac{1}{5}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}\sdot\frac{1}{10}\right)\\&\scriptstyle=\left(\frac{37}{7}\sdot\frac{1}{2}\sdot\frac{1}{5}\sdot\frac{1}{9}\sdot\frac{1}{10}\right)+\left(\frac{1}{5}\sdot\frac{1}{5}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}\sdot\frac{1}{10}\right)\\&\scriptstyle=\left(\frac{5}{5}\sdot\frac{1}{2}\sdot\frac{1}{9}\sdot\frac{1}{10}\right)+\left(\frac{1}{2}\sdot\frac{1}{7}\sdot\frac{1}{5}\sdot\frac{1}{9}\sdot\frac{1}{10}\right)+\left(\frac{1}{5}\sdot\frac{1}{5}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}\sdot\frac{1}{10}\right)\\&\scriptstyle=\left(\frac{1}{2}\sdot\frac{1}{9}\sdot\frac{1}{10}\right)+\left(\frac{2}{7}\sdot\frac{1}{5}\sdot\frac{1}{2}\sdot\frac{1}{9}\sdot\frac{1}{10}\right)+\left(\frac{1}{5}\sdot\frac{1}{4}\sdot\frac{1}{7}\sdot\frac{1}{5}\sdot\frac{1}{2}\sdot\frac{1}{9}\sdot\frac{1}{10}\right)\\\end{align}}}</math> | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| Line 4,846: | Line 5,816: | ||
|- | |- | ||
| | | | ||
| − | : | + | :Do not be surprised that you had onle seven denominators and now they are eight, as this is due to the factorization of the denominator of the eighth that is 8, which we removed and instead of which we placed two denominators that are 2 and 4. |
| − | |style="text-align:right;"|ואל תתמה שלא היו לך כי אם ז' מורים ועתה הם ח' כי זה היה ל{{#annot:term|2614,1558|CHAD}}התכת{{#annotend:CHAD}} המורה השמינית והוא הח' שהסרנו אותו מהם ושמנו במקומו שני מורים והם ב'ד' והקש על זה כי הכל ברור המעשה והטעם | + | |style="width:45%; text-align:right;"|ואל תתמה שלא היו לך כי אם ז' מורים ועתה הם ח' כי זה היה ל{{#annot:term|2614,1558|CHAD}}התכת{{#annotend:CHAD}} המורה השמינית והוא הח' שהסרנו אותו מהם ושמנו במקומו שני מורים והם ב'ד‫' |
| + | |- | ||
| + | |Apply this, because the entire procedure and the reason are clear. | ||
| + | |style="text-align:right;"|והקש על זה כי הכל ברור המעשה והטעם | ||
|- | |- | ||
|} | |} | ||
| Line 4,854: | Line 5,827: | ||
| | | | ||
| − | === | + | === Chapter Three on the Expansion to a Common Denominator === |
| − | + | |style="width:45%; text-align:right;"|<big>השער השלישי בהשואה</big> | |
|- | |- | ||
| − | |<span style=color: | + | |<span style=color:Green>Definition:</span> {{#annot:definition|2618,2023|FYfS}}The expansion to a common denominator is when you have fractions of various types that are not related to each other at all, i.e. the type of these fractions is not the type of fractions of the others. |
| − | |style="text-align:right;"|ההשואה היא כאשר יהיו לך שברים ממינים שונים בלתי נקשרים זה בזה כלל ר"ל שאין אלו שברי שברים אלו{{#annotend:FYfS}} | + | |style="text-align:right;"|ההשואה <sup>היא</sup> כאשר יהיו לך שברים ממינים שונים בלתי נקשרים זה בזה כלל ר"ל שאין אלו שברי שברים אלו{{#annotend:FYfS}} |
|- | |- | ||
| | | | ||
*Example: if you have two integers, 3-eighths and 2-quarters of an eighth, 4-fifths, 6-sevenths and 3-eighths of a sevenths, like this, and you wish to convert all of them into one type. | *Example: if you have two integers, 3-eighths and 2-quarters of an eighth, 4-fifths, 6-sevenths and 3-eighths of a sevenths, like this, and you wish to convert all of them into one type. | ||
:<math>\scriptstyle\left[2+\frac{3}{8}+\left(\frac{2}{4}\sdot\frac{1}{8}\right)\right]\quad\frac{4}{5}\quad\left[\frac{6}{7}+\left(\frac{3}{8}\sdot\frac{1}{7}\right)\right]</math> | :<math>\scriptstyle\left[2+\frac{3}{8}+\left(\frac{2}{4}\sdot\frac{1}{8}\right)\right]\quad\frac{4}{5}\quad\left[\frac{6}{7}+\left(\frac{3}{8}\sdot\frac{1}{7}\right)\right]</math> | ||
| − | |style="text-align:right;"|המשל אם היו בידיך שני שלמים ועוד וג' שמינית וב' רביעיות שמינית ועוד ד' חמישיות ועוד ו' שביעיות וג' שמיניות שביעית כזה ותרצה להשיבם כלם ממין אחד | + | |style="text-align:right;"|המשל אם היו בידיך שני שלמים <s>ועוד</s> וג' שמינית וב' רביעיות שמינית ועוד ד' חמישיות ועוד ו' שביעיות וג' שמיניות שביעית כזה ‫<ref>44r</ref>ותרצה להשיבם כלם ממין אחד |
|- | |- | ||
| | | | ||
| Line 4,875: | Line 5,848: | ||
|- | |- | ||
| | | | ||
| − | :First we | + | :First we decompose the 2 integers plus the 3-eighths and the 2-quarters of an eighth, since they are related to the 6-sevenths and the 3-eighths of a seventh, for the related also need decomposing to a fraction. |
| − | |style="text-align:right;"|ונעשה תחלה פריטה לב' שלמים וג' שמיניות וב' | + | |style="text-align:right;"|ונעשה תחלה פריטה לב' שלמים וג' שמיניות וב' רביעי<sup>ו</sup>ת שמינית <s>ועוד ד' חמישיות ועוד ו' שביעיות</s> אחרי היותם {{#annot:term|1567,1494|KtgV}}נקשרים{{#annotend:KtgV}} גם לו' שביעיות וג' שמיניות שביעית כי גם הם נקשרים וצריכים פריטה |
|- | |- | ||
| | | | ||
:*We start by saying: 2 units, how many eighths are they? This is known by multiplying them by 8; they are 16. We add to them the 3 that is beneath [the 8] and the total is 19. | :*We start by saying: 2 units, how many eighths are they? This is known by multiplying them by 8; they are 16. We add to them the 3 that is beneath [the 8] and the total is 19. | ||
::<math>\scriptstyle{\color{blue}{2+\frac{3}{8}=\frac{\left(2\sdot8\right)+3}{8}=\frac{16+3}{8}=\frac{19}{8}}}</math> | ::<math>\scriptstyle{\color{blue}{2+\frac{3}{8}=\frac{\left(2\sdot8\right)+3}{8}=\frac{16+3}{8}=\frac{19}{8}}}</math> | ||
| − | |style="text-align:right;"|ונתחיל לומר ב' אחדים כמה שמיניות הם וזה יודע ב{{#annot:term|156,2136|AiFV}}הכפלם | + | |style="text-align:right;"|ונתחיל לומר ב' אחדים כמה שמיניות הם וזה יודע ב{{#annot:term|156,2136|AiFV}}הכפלם{{#annotend:AiFV}} <sup>בח'</sup> יהיו י"ו ונחבר להם הג' אשר תחתיו יהיו כלם י"ט |
|- | |- | ||
| | | | ||
| Line 4,889: | Line 5,862: | ||
|- | |- | ||
| | | | ||
| − | :*We also | + | :*We also decomposing the 6-sevenths plus 3-eighths of a seventh to a fraction by saying: 6-sevenths, how many eighths of a seventh are they? This is known by multiplying them by 8; they are 48. We add to them the 3 that is beneath [the 8]; the result is 51 eighths of a seventh. |
::<math>\scriptstyle{\color{blue}{\frac{6}{7}+\left(\frac{3}{8}\sdot\frac{1}{7}\right)=\frac{\left(6\sdot8\right)+3}{8}\sdot\frac{1}{7}=\frac{48+3}{8}\sdot\frac{1}{7}=\frac{51}{8}\sdot\frac{1}{7}}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{6}{7}+\left(\frac{3}{8}\sdot\frac{1}{7}\right)=\frac{\left(6\sdot8\right)+3}{8}\sdot\frac{1}{7}=\frac{48+3}{8}\sdot\frac{1}{7}=\frac{51}{8}\sdot\frac{1}{7}}}</math> | ||
| − | |style="text-align:right;"|עוד נפרוט הו' שביעיות וג' שמיניות שביעית ונאמרו ו' שביעיות שלמות כמה שלמות שמיניות שביעית הם וזה יודע בהכפלם בח' ויעלו מ"ח ונחבר להם הג' אשר תחתיו ועלו נ"א שמיניות שביעית | + | |style="text-align:right;"|עוד נפרוט הו' שביעיות וג' שמיניות שביעית ונאמרו ו' שביעיות שלמות כמה <s>שלמות</s> שמיניות שביעית הם וזה יודע בהכפלם בח' ויעלו מ"ח ונחבר להם הג' אשר תחתיו ועלו נ"א שמיניות שביעית |
|- | |- | ||
| | | | ||
:It is as if we were asked to convert 78 quarters of an eighth, 4 fifths, and 51 eighths of a seventh into one type. Like this: | :It is as if we were asked to convert 78 quarters of an eighth, 4 fifths, and 51 eighths of a seventh into one type. Like this: | ||
:<math>\scriptstyle{\color{blue}{\frac{78}{4}\sdot\frac{1}{8}\quad\frac{4}{5}\quad\frac{51}{8}\sdot\frac{1}{7}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{78}{4}\sdot\frac{1}{8}\quad\frac{4}{5}\quad\frac{51}{8}\sdot\frac{1}{7}}}</math> | ||
| − | |style="text-align:right;"|והרי הוא כאלו שאלו לנו להשיב למין אחד עין ע"ח רביעיות שמינית וד' חמישיות ונ"א שמיניות שביעית שביעי כזה | + | |style="text-align:right;"|והרי הוא כאלו שאלו לנו להשיב למין אחד <s>עין מ'</s> <sup>ע"ח</sup> רביעיות שמינית וד' חמישיות ונ"א <s>ע"ח</s> שמיניות <sup>שביעית</sup> <s>שביעי</s> כזה |
|- | |- | ||
| | | | ||
| Line 4,913: | Line 5,886: | ||
| | | | ||
:First, I will explain that the order of the denominators neither increases nor decreases: | :First, I will explain that the order of the denominators neither increases nor decreases: | ||
| − | :<math>\scriptstyle{\color{ | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{1}{a}\sdot\frac{1}{b}=\frac{1}{b}\sdot\frac{1}{a}}}</math> |
|style="text-align:right;"|וקודם זה אציע שסדור המורים אינו מעלה ומוריד | |style="text-align:right;"|וקודם זה אציע שסדור המורים אינו מעלה ומוריד | ||
|- | |- | ||
| | | | ||
| − | ::Because, the seventh of an eighth, for instance, is the the same as the eighth of a seventh, since each is a part of 56, which is the number that consists of these denominators and this is clear. | + | ::Because, the seventh of an eighth, for instance, is the the same as the eighth of a seventh, since each is a part of [56], which is the number that consists of these denominators and this is clear. |
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{7}\sdot\frac{1}{8}=\frac{1}{56}=\frac{1}{8}\sdot\frac{1}{7}}}</math> | + | ::<math>\scriptstyle{\color{blue}{\frac{1}{7}\sdot\frac{1}{8}=\frac{1}{{\color{red}{56}}}=\frac{1}{8}\sdot\frac{1}{7}}}</math> |
| − | |style="text-align:right;"|כי כך הוא שביעית שמינית עד"מ כמו שמינית שביעית כי כל אחד מהם הוא חלקנו | + | |style="text-align:right;"|כי כך הוא שביעית שמינית עד"מ כמו שמינית שביעית כי כל אחד מהם הוא חלקנו <sup>מה'</sup> בשלם שהוא המספר אשר הוא מורכב מאלו המורים וזה ברור |
|- | |- | ||
| | | | ||
| − | :*So, when we have, for example, 3-sevenths and 4-eighths | + | :*So, when we have, for example, 3-sevenths and 4-eighths. |
::<math>\scriptstyle\frac{3}{7}\quad\frac{4}{8}</math> | ::<math>\scriptstyle\frac{3}{7}\quad\frac{4}{8}</math> | ||
|style="text-align:right;"|לכן כאשר היה לנו עד"מ ג' שביעיות וד' שמינית | |style="text-align:right;"|לכן כאשר היה לנו עד"מ ג' שביעיות וד' שמינית | ||
|- | |- | ||
| | | | ||
| − | ::We convert all of them into sevenths of an eighth, which are eighths of a seventh | + | ::We convert all of them into sevenths of an eighth, which are eighths of a seventh. |
|style="text-align:right;"|נשיבם כלם שביעיות שמינית שהוא שמיניות שביעית | |style="text-align:right;"|נשיבם כלם שביעיות שמינית שהוא שמיניות שביעית | ||
|- | |- | ||
| Line 4,937: | Line 5,910: | ||
| | | | ||
:::This is clear, since every seventh is 8-eighths of a seventh, as each integer is eight eighths. | :::This is clear, since every seventh is 8-eighths of a seventh, as each integer is eight eighths. | ||
| − | |style="text-align:right;"|וזה ברור כי כל שביעיות הוא ח' שמיניות שביעית כמו שכל שלם הוא שמונה שמיניות השלם | + | |style="text-align:right;"|וזה ברור כי כל שביעיות הוא ח' שמיניות שביעית כמו שכל שלם ‫<ref>44v</ref>הוא שמונה שמיניות השלם |
|- | |- | ||
| | | | ||
::*We do the same with the 4-eighths: we convert them into sevenths of an eighth by multiplying the 4, which is the number of the fractions, by 7, which is the denominator of the seventh; the result is 28. | ::*We do the same with the 4-eighths: we convert them into sevenths of an eighth by multiplying the 4, which is the number of the fractions, by 7, which is the denominator of the seventh; the result is 28. | ||
:::<math>\scriptstyle{\color{blue}{\frac{4\sdot7}{7}\sdot\frac{1}{8}=\frac{28}{7}\sdot\frac{1}{8}}}</math> | :::<math>\scriptstyle{\color{blue}{\frac{4\sdot7}{7}\sdot\frac{1}{8}=\frac{28}{7}\sdot\frac{1}{8}}}</math> | ||
| − | |style="text-align:right;"|וכן נעשה לד' שמיניות שנשיבם | + | |style="text-align:right;"|וכן נעשה לד' שמיניות שנשיבם לשביעי<sup>ו</sup>ת שמינית והוא בכפול הד' שהוא מספר השברים בז' שהוא מורה השביעיות ויעלו כ"ח |
|- | |- | ||
| | | | ||
| Line 4,948: | Line 5,921: | ||
|style="text-align:right;"|והם כ"ח שביעיות שמינית והאחרות עלו כ"ד שמיניות שביעית הנה כלם ממין אחד כמו שהזכרנו שאין חלוף בין אומרנו שביעית שמינית לאומרנו שמינית שביעית | |style="text-align:right;"|והם כ"ח שביעיות שמינית והאחרות עלו כ"ד שמיניות שביעית הנה כלם ממין אחד כמו שהזכרנו שאין חלוף בין אומרנו שביעית שמינית לאומרנו שמינית שביעית | ||
|- | |- | ||
| − | |After explaining | + | |After explaining this premise, we return to our first procedure, which is multiplying each numerator of the fractions that we have by the denominators of the others successively, thus each fraction will be of all the denominators, so they are of the same type, for the order of the denominators forward or backward does not matter. |
| − | :<math>\scriptstyle{\color{ | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}=\frac{a\sdot d}{b}\sdot\frac{1}{d}=\frac{a\sdot d}{d}\sdot\frac{1}{b}}}</math> |
| − | :<math>\scriptstyle{\color{ | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}=\frac{a\sdot d}{d}\sdot\frac{1}{b}=\frac{a\sdot d}{b}\sdot\frac{1}{d}}}</math> |
|style="text-align:right;"|ואחר שהצענו הצעה זו נשוב למעשינו הראשון והוא לכפול כל מספר שברים אשר בידינו במורי חברותיה זה אחר זה וכן לכלם ואז תהיה כל אחד שברים מכל המורים והנה הם שוים כי סדור המורים בקדימה ואיחור לא יזיק | |style="text-align:right;"|ואחר שהצענו הצעה זו נשוב למעשינו הראשון והוא לכפול כל מספר שברים אשר בידינו במורי חברותיה זה אחר זה וכן לכלם ואז תהיה כל אחד שברים מכל המורים והנה הם שוים כי סדור המורים בקדימה ואיחור לא יזיק | ||
|- | |- | ||
| | | | ||
| − | :*<math>\scriptstyle{\color{blue}{\frac{78\sdot5}{5}\sdot\frac{1}{4}\sdot\frac{1}{8}=\frac{390}{5}\sdot\frac{1}{4}\sdot\frac{1}{8}}}</math> | + | :*We start our procedure by saying: when we multiply 78 quarters of an eighth by 5, which is the denominator of the fifths, the result is 390 fifths of a quarter of an eighth. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{78\sdot5}{5}\sdot\frac{1}{4}\sdot\frac{1}{8}=\frac{390}{5}\sdot\frac{1}{4}\sdot\frac{1}{8}}}</math> | ||
|style="text-align:right;"|ונתחיל במעשינו ונאמר 78 רביעיות שמינית כאשר נכפלם בה' שהוא מורה החמישיות יעלו 390 חמישיות רביעית שמינית | |style="text-align:right;"|ונתחיל במעשינו ונאמר 78 רביעיות שמינית כאשר נכפלם בה' שהוא מורה החמישיות יעלו 390 חמישיות רביעית שמינית | ||
|- | |- | ||
| | | | ||
| + | ::We also multiply this product by 7, which is the denominator of the seventh; the result is 2730 sevenths of fifths of a quarter of an eighth. | ||
::<math>\scriptstyle{\color{blue}{\frac{390\sdot7}{7}\sdot\frac{1}{5}\sdot\frac{1}{4}\sdot\frac{1}{8}=\frac{2730}{7}\sdot\frac{1}{5}\sdot\frac{1}{4}\sdot\frac{1}{8}}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{390\sdot7}{7}\sdot\frac{1}{5}\sdot\frac{1}{4}\sdot\frac{1}{8}=\frac{2730}{7}\sdot\frac{1}{5}\sdot\frac{1}{4}\sdot\frac{1}{8}}}</math> | ||
|style="text-align:right;"|עוד נכפול זה {{#annot:term|241,1220|1nHC}}המחובר{{#annotend:1nHC}} בז' שהוא מורה השביעית ויעלו 2730 שביעיות חמישיות רביעית שמינית | |style="text-align:right;"|עוד נכפול זה {{#annot:term|241,1220|1nHC}}המחובר{{#annotend:1nHC}} בז' שהוא מורה השביעית ויעלו 2730 שביעיות חמישיות רביעית שמינית | ||
|- | |- | ||
| | | | ||
| + | ::We also multiply all this by 8, which is the denominator of the eighths; the result is 21840 eighths of sevenths of fifths of quarters of eighths and this is the result of 78 quarters of eighths. | ||
::<math>\scriptstyle{\color{blue}{\frac{2730\sdot8}{8}\sdot\frac{1}{7}\sdot\frac{1}{5}\sdot\frac{1}{4}\sdot\frac{1}{8}=\frac{21840}{8}\sdot\frac{1}{7}\sdot\frac{1}{5}\sdot\frac{1}{4}\sdot\frac{1}{8}}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{2730\sdot8}{8}\sdot\frac{1}{7}\sdot\frac{1}{5}\sdot\frac{1}{4}\sdot\frac{1}{8}=\frac{21840}{8}\sdot\frac{1}{7}\sdot\frac{1}{5}\sdot\frac{1}{4}\sdot\frac{1}{8}}}</math> | ||
| − | |style="text-align:right;"|עוד נכפול כל זה בח' שהוא המורה השמיניות ויעלו | + | |style="text-align:right;"|עוד נכפול כל זה בח' שהוא המורה השמיניות ויעלו 21<sup>8</sup>40 שמיניות שביעיות חמישיות רביעיות שמיניות וזהו העולה מה78 רביעיות שמיניות |
|- | |- | ||
| | | | ||
| − | :*<math>\scriptstyle{\color{blue}{\frac{4\sdot8}{8}\sdot\frac{1}{5}=\frac{32}{8}\sdot\frac{1}{5}}}</math> | + | :*We also multiply the 4 that are 4-fifths by all the denominators of the others, one after the other. We say: 4 by 8 is 32 eighths of a fifth. |
| − | |style="text-align:right;"|עוד נכפול הד' שהוא ד' חמישיות בכל מורי חברותיה זה אחר זה ונאמר ד בח' הם [ל"ב] שמיניות חמשית | + | ::<math>\scriptstyle{\color{blue}{\frac{4\sdot8}{8}\sdot\frac{1}{5}=\frac{32}{8}\sdot\frac{1}{5}}}</math> |
| + | |style="text-align:right;"|עוד נכפול הד' שהוא ד' חמישיות בכל מורי חברותיה זה אחר זה ונאמר ד בח' הם [ל"ב]‫<ref>marg.</ref> שמיניות חמשית | ||
|- | |- | ||
| | | | ||
| + | ::We also multiply them by 4; they are 128 quarters of eighths of a fifth. | ||
::<math>\scriptstyle{\color{blue}{\frac{32\sdot4}{4}\sdot\frac{1}{8}\sdot\frac{1}{5}=\frac{128}{4}\sdot\frac{1}{8}\sdot\frac{1}{5}}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{32\sdot4}{4}\sdot\frac{1}{8}\sdot\frac{1}{5}=\frac{128}{4}\sdot\frac{1}{8}\sdot\frac{1}{5}}}</math> | ||
|style="text-align:right;"|עוד נכפלם בד' יהיו 128 רביעיות שמיניות חמישית | |style="text-align:right;"|עוד נכפלם בד' יהיו 128 רביעיות שמיניות חמישית | ||
|- | |- | ||
| | | | ||
| + | ::We also multiply them by 7; they are 896 sevenths of a quarter of an eighth of a fifth. | ||
::<math>\scriptstyle{\color{blue}{\frac{128\sdot7}{7}\sdot\frac{1}{4}\sdot\frac{1}{8}\sdot\frac{1}{5}=\frac{896}{7}\sdot\frac{1}{4}\sdot\frac{1}{8}\sdot\frac{1}{5}}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{128\sdot7}{7}\sdot\frac{1}{4}\sdot\frac{1}{8}\sdot\frac{1}{5}=\frac{896}{7}\sdot\frac{1}{4}\sdot\frac{1}{8}\sdot\frac{1}{5}}}</math> | ||
|style="text-align:right;"|ונכפלם בז' יהיה 896 שביעיות רביעית שמינית חמישית | |style="text-align:right;"|ונכפלם בז' יהיה 896 שביעיות רביעית שמינית חמישית | ||
|- | |- | ||
| | | | ||
| + | ::We also multiply them by 8; the result is 7168 eighths of a seventh of a quarter of an eighth of a fifth. | ||
::<math>\scriptstyle{\color{blue}{\frac{896\sdot8}{8}\sdot\frac{1}{7}\sdot\frac{1}{4}\sdot\frac{1}{8}\sdot\frac{1}{5}=\frac{7168}{8}\sdot\frac{1}{7}\sdot\frac{1}{4}\sdot\frac{1}{8}\sdot\frac{1}{5}}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{896\sdot8}{8}\sdot\frac{1}{7}\sdot\frac{1}{4}\sdot\frac{1}{8}\sdot\frac{1}{5}=\frac{7168}{8}\sdot\frac{1}{7}\sdot\frac{1}{4}\sdot\frac{1}{8}\sdot\frac{1}{5}}}</math> | ||
| − | |style="text-align:right;"|עוד נכפלם | + | |style="text-align:right;"|עוד נכפלם ב<sup>ח'</sup> <s>8</s> יעלו 7168 שמיניות שביעית רביעית שמינית חמישית |
|- | |- | ||
| | | | ||
| − | :*<math>\scriptstyle{\color{blue}{\frac{51\sdot5}{5}\sdot\frac{1}{8}\sdot\frac{1}{7}=\frac{255}{5}\sdot\frac{1}{8}\sdot\frac{1}{7}}}</math> | + | :*We also multiply the 51 that are 51-eighths of a seventh by 5; the result is 255 fifths of eighths of a seventh. |
| − | |style="text-align:right;"|עוד נכפול הנ"א שהם נ"א שמיניות שביעית בה' יעלו 255 חמישיות שמיניות שביעית | + | ::<math>\scriptstyle{\color{blue}{\frac{51\sdot5}{5}\sdot\frac{1}{8}\sdot\frac{1}{7}=\frac{255}{5}\sdot\frac{1}{8}\sdot\frac{1}{7}}}</math> |
| + | |style="text-align:right;"|עוד נכפול הנ"א שהם נ"א שמיניות שביעית בה' יעלו <sup>255</sup> <s>2</s> חמישיות שמיניות שביעית | ||
|- | |- | ||
| | | | ||
| + | ::We multiply them by 4; the result is 1020 quarters of fifths of eighths of a seventh. | ||
::<math>\scriptstyle{\color{blue}{\frac{255\sdot4}{4}\sdot\frac{1}{5}\sdot\frac{1}{8}\sdot\frac{1}{7}=\frac{1020}{4}\sdot\frac{1}{5}\sdot\frac{1}{8}\sdot\frac{1}{7}}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{255\sdot4}{4}\sdot\frac{1}{5}\sdot\frac{1}{8}\sdot\frac{1}{7}=\frac{1020}{4}\sdot\frac{1}{5}\sdot\frac{1}{8}\sdot\frac{1}{7}}}</math> | ||
| − | |style="text-align:right;"|נכפלם בד' יעלו 1020 רביעית | + | |style="text-align:right;"|נכפלם בד' יעלו 1020 רביעית חמישי<sup>ו</sup>ת שמיניות שביעית |
|- | |- | ||
| | | | ||
| + | ::We also multiply them by 8; the result is 8160 eighths of quarters of a fifth of an eighth of a seventh. | ||
::<math>\scriptstyle{\color{blue}{\frac{1020\sdot8}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{8}\sdot\frac{1}{7}=\frac{8160}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{8}\sdot\frac{1}{7}}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{1020\sdot8}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{8}\sdot\frac{1}{7}=\frac{8160}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{8}\sdot\frac{1}{7}}}</math> | ||
| − | |style="text-align:right;"|עוד נכפלם בח' יעלו 8160 שמיניות רביעיות חמישית שמינית שביעית | + | |style="text-align:right;"|עוד נכפלם בח' יעלו 8160 ‫<ref>45r</ref>שמיניות רביעיות חמישית שמינית שביעית |
|- | |- | ||
| | | | ||
| + | :Now all are of one type, for the denominators are the same, because the order neither increases nor decreases as we have explained. | ||
|style="text-align:right;"|הרי כלם ממין אחד כי המורים שוים כי הסדר אינו מעלה ומוריד כאשר ביארנו | |style="text-align:right;"|הרי כלם ממין אחד כי המורים שוים כי הסדר אינו מעלה ומוריד כאשר ביארנו | ||
|- | |- | ||
| − | |Beware lest you make a mistake when doing this | + | |Beware lest you make a mistake when doing this expansion to a common denominator, not to add what is beneath the denominators to the product of the numerators by the denominators, for this is done only when decomposing to a fraction, when we want to sum up all the mentioned related fractions and to decompose them to the lowest fraction. |
|style="text-align:right;"|והשמר לך מאד פן תטעה בעשותך השואה זו לחבר לעולה מכפל ה{{#annot:term|570,1242|fPu0}}שברים{{#annotend:fPu0}} במורים מה שנמצא תחת המורי' כי זה לא יעשה כי אם בפריטה לבד שאנו רוצים לחבר כל השברים הנזכרים הנקשרים ולפרטם למין הפרוטות | |style="text-align:right;"|והשמר לך מאד פן תטעה בעשותך השואה זו לחבר לעולה מכפל ה{{#annot:term|570,1242|fPu0}}שברים{{#annotend:fPu0}} במורים מה שנמצא תחת המורי' כי זה לא יעשה כי אם בפריטה לבד שאנו רוצים לחבר כל השברים הנזכרים הנקשרים ולפרטם למין הפרוטות | ||
|- | |- | ||
| | | | ||
| − | :*Example: | + | :*Example: the one who has peraḥim and peruṭot and wishes to convert the peruṭot that he has, or to convert the peraḥim into zehuvim [gold coins], i.e. to see how many zehuvim they are, to add the zehuvim that he has to the result, then to convert all the zehuvim to peruṭot and add to them the peruṭot that he has, so that all is summed and decomposed [to lowest value (peruṭot)]. |
|style="text-align:right;"|המשל במי שיש לו פרחים וזהובים ופרוטות שרוצה להשיב הפרוטות שיש לו או להשיב הפרחים זהובים ר"ל לראות כמה זהובים יעלו ולחבר לעולה הזהובים אשר היו בידו ואחר כך להשי' כל הזהובים פרוטות ולחבר עמהם הפרוטות אשר בידו ויהיה אז הכל מחובר ונפרט | |style="text-align:right;"|המשל במי שיש לו פרחים וזהובים ופרוטות שרוצה להשיב הפרוטות שיש לו או להשיב הפרחים זהובים ר"ל לראות כמה זהובים יעלו ולחבר לעולה הזהובים אשר היו בידו ואחר כך להשי' כל הזהובים פרוטות ולחבר עמהם הפרוטות אשר בידו ויהיה אז הכל מחובר ונפרט | ||
|- | |- | ||
| − | | | + | |But, the expansion to a common denominator does not includes summing at all, but to convert all the fractions to same type of units, so one does not sum them at all and this is clear by reason. |
|style="text-align:right;"|אבל ההשואה אין בה חבור כלל כי אם לעשות כל שברים מהם ממין האחדים לכן לא יחברם כלל וזה מבואר בטעם | |style="text-align:right;"|אבל ההשואה אין בה חבור כלל כי אם לעשות כל שברים מהם ממין האחדים לכן לא יחברם כלל וזה מבואר בטעם | ||
|- | |- | ||
| − | | | + | |Therefore I gave them different names that indicate this matter by hint. |
|style="text-align:right;"|ולזה שמתי להם שמות שונים מורי' על הענין ברמז | |style="text-align:right;"|ולזה שמתי להם שמות שונים מורי' על הענין ברמז | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:definition|2618,2023|7E8S}}For, to the conversion of the fractions that are not related together into one type of fractions by multiplying each of these fractions by the denominators of the others, I called '''Hašavah''' | + | *{{#annot:definition|2618,2023|7E8S}}For, to the conversion of the fractions that are not related together into one type of fractions by multiplying each of these fractions by the denominators of the others, I called '''Hašavah''' [expansion to a common denominator], yet our intention is not at all to sum the fractions together, but only to equalize [their denominators]. |
| − | |style="text-align:right;"|כי ל{{#annot:term|2612,1428|4KPL}}החזרת{{#annotend:4KPL}} השברים הבלתי נקשרות למין אחד בהכאת כל אחד מהם במורי חברותיה קראתי | + | |style="text-align:right;"|כי ל{{#annot:term|2612,1428|4KPL}}החזרת{{#annotend:4KPL}} השברים הבלתי נקשרות למין אחד בהכאת כל אחד מהם במורי חברותיה קראתי השואה שאין כונתינו חבור כלל כי אם ההשואה לבד{{#annotend:7E8S}} |
|- | |- | ||
| | | | ||
| − | *{{#annot:definition|1561,1937|EbbI}}To the conversion of fractions that are related together into the lowest type of fractions I called '''Periṭah''' | + | *{{#annot:definition|1561,1937|EbbI}}To the conversion of fractions that are related together into the lowest type of fractions I called '''Periṭah''' [decomposing to a fraction]. |
| − | |style="text-align:right;"|ולהשבת השברים הנקשרים כלם יחד למין השברים הגרועים מהם קראתי | + | |style="text-align:right;"|ולהשבת השברים הנקשרים כלם <sup>יחד</sup> למין השברים הגרועים מהם קראתי פריטה{{#annotend:EbbI}} |
|- | |- | ||
| | | | ||
| − | : | + | :For two reasons: |
| − | |style="text-align:right;"|לשתי | + | |style="text-align:right;"|לשתי כו<sup>ו</sup>נות |
|- | |- | ||
| | | | ||
| − | :1) | + | :1) It is as converting [= poreṭ] peraḥim and zehuvim [kinds of coins] to peruṭot [currency of the lowest value] and as converting [= poreṭ] the general to particular [= peraṭim] <span style=color:Green>[meaning: the Hebrew word for converting = poreṭ has the same linguistic root of periṭah that is used for decomposing to a fraction]</span> |
| − | |style="text-align:right;"|האחת שהוא כפורט ועושה מהפרחים וזהובים ופרוטות פרוטות וכמשיב הכללים לפרטים | + | |style="text-align:right;"|האחת <sup>שהוא</sup> כפורט ועושה מהפרחים וזהובים ופרוטות פרוטות וכמשיב הכללים לפרטים |
|- | |- | ||
| | | | ||
| − | :2) | + | :2) By this word one is reminded of the details [= peraṭ, again the same linguistic root] that are found beneath the denominators [i.e. the numerators]. |
|style="text-align:right;"|והכונה השנית היא כי בשם זה יזכר שיש לו לקחת עמו הפרט והעוללות אשר ימצא תחת המורים | |style="text-align:right;"|והכונה השנית היא כי בשם זה יזכר שיש לו לקחת עמו הפרט והעוללות אשר ימצא תחת המורים | ||
|- | |- | ||
| − | | | + | |For every number that requires multiplication and decomposing to a fraction - the decomposing to a fraction should be applied first to the multiplicands, and then the multiplication is applied. |
| − | |style="text-align:right;"|ובכל מספר שצריך הכאה עם הפריטה | + | |style="text-align:right;"|ובכל מספר שצריך הכאה עם הפריטה יעשה קודם הפריטה לבעלי ההכאה ואחר ההכאה |
| + | |- | ||
| + | |Therefore, wherever we mention and instruct to decompose to a fraction, we mean that it is followed by the multiplication if needed, or whatever is needed, if you have a number that consists of fractions that require multiplication and decomposing to a fraction. | ||
| + | |style="text-align:right;"|לכן בכל מקום אשר נזכיר ונצוה לעשות פריטה רצוננו ואחריה ההכאה אם הוצרך איליה או אשר מהם יצטרך שאם יהיה לך מספר ‫<ref>45v</ref>מורכב מהשברים הצריכים הכאה ועם הצריכים פריטה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Example: 3-quarters of 2-fifths and 3-quarters of fifths of 4-sixths and a third of a sixth. |
| + | :<math>\scriptstyle\frac{3}{4}\sdot\left[\frac{2}{5}+\left(\frac{3}{4}\sdot\frac{1}{5}\right)\right]\sdot\left[\frac{4}{6}+\left(\frac{1}{3}\sdot\frac{1}{6}\right)\right]</math> | ||
| + | |style="text-align:right;"|המשל ג' רביעיות מב' חמישיות וג' רביעיות חמישיות מד' ששיות ושלישית ששית | ||
|- | |- | ||
| | | | ||
| − | + | :Arrange them like this: | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תשימם על הסדר כזה |
|- | |- | ||
| | | | ||
| Line 5,053: | Line 6,042: | ||
|- | |- | ||
| | | | ||
| − | ::*<math>\scriptstyle{\color{blue}{\frac{4}{6}+\left(\frac{1}{3}\sdot\frac{1}{6}\right)=\frac{\left(4\sdot3\right)+1}{3}\sdot\frac{1}{6}=\frac{13}{3}\sdot\frac{1}{6}}}</math> | + | ::*Decompose 4-[sixths] and a third of a sixth by multiplying 4 by 3 and add the 1 that is beneath it; the result is 13-thirds of a sixth. |
| − | |style="text-align:right;"|ותעשה פריטה לד | + | :::<math>\scriptstyle{\color{blue}{\frac{4}{6}+\left(\frac{1}{3}\sdot\frac{1}{6}\right)=\frac{\left(4\sdot3\right)+1}{3}\sdot\frac{1}{6}=\frac{13}{3}\sdot\frac{1}{6}}}</math> |
| + | |style="text-align:right;"|ותעשה פריטה לד' שביעיות ושלישית שישית והוא שתכפול הד' בג' ותחבר להם האחד אשר תחתיו ויעלו י"ג שלישיות ששית | ||
|- | |- | ||
| | | | ||
| − | ::*<math>\scriptstyle{\color{blue}{\frac{2}{5}+\left(\frac{3}{4}\sdot\frac{1}{5}\right)=\frac{11}{4}\sdot\frac{1}{5}}}</math> | + | ::*Do the same with 2-fifths and 3-quarters of a fifth; the result is 11-quarters of a fifth. |
| + | :::<math>\scriptstyle{\color{blue}{\frac{2}{5}+\left(\frac{3}{4}\sdot\frac{1}{5}\right)=\frac{11}{4}\sdot\frac{1}{5}}}</math> | ||
|style="text-align:right;"|וכן תעשה לב' חמישיות וג' רביעיות חמישית ויעלו י"א רביעיות חמישית | |style="text-align:right;"|וכן תעשה לב' חמישיות וג' רביעיות חמישית ויעלו י"א רביעיות חמישית | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{3}{4}\sdot\left[\frac{2}{5}+\left(\frac{3}{4}\sdot\frac{1}{5}\right)\right]\sdot\left[\frac{4}{6}+\left(\frac{1}{3}\sdot\frac{1}{6}\right)\right]=\frac{3}{4}\sdot\left(\frac{ | + | ::Your number becomes as if saying 1[1]-quarters of a fifth of 13-thirds of a sixth, like this. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{3}{4}\sdot\left[\frac{2}{5}+\left(\frac{3}{4}\sdot\frac{1}{5}\right)\right]\sdot\left[\frac{4}{6}+\left(\frac{1}{3}\sdot\frac{1}{6}\right)\right]=\frac{3}{4}\sdot\left(\frac{1{\color{red}{1}}}{4}\sdot\frac{1}{5}\right)\sdot\left(\frac{13}{3}\sdot\frac{1}{6}\right)}}</math> | ||
|style="text-align:right;"|וישוב מספרך כאלו אמרו ג' רביעיות מי"ח רביעיות חמישית מי"ג שלישיות שישית כזה | |style="text-align:right;"|וישוב מספרך כאלו אמרו ג' רביעיות מי"ח רביעיות חמישית מי"ג שלישיות שישית כזה | ||
|- | |- | ||
| Line 5,077: | Line 6,069: | ||
|} | |} | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"|ואחר עשותך פריטה זו כנזכר תעשה ההכאה והוא לתת סבות להכות הג' שהם השברים האחרונים במספר השברים לא במורים השברים וגם לזה ירשמו בשם ההכאה כי בהכאה יבא השבר והשבר הוא תחת המורה | + | :*After you decompose to a fraction as mentioned, you apply the multiplication, that is to multiply the 3, which is the last numerator, by the numerators, not by the denominators, and they are written by the name of the product, because the result of the multiplication is the numerator and the numerator is beneath the denominator, as the fractional is low and ''despised by peoples'' [Psalms 22, 7]. |
| + | |style="text-align:right;"|ואחר עשותך פריטה זו כנזכר תעשה ההכאה והוא <s>לתת סבות</s> להכות הג' שהם השברים האחרונים במספר השברים לא במורים השברים וגם לזה ירשמו בשם ההכאה כי בהכאה יבא השבר והשבר הוא תחת המורה כמו שהנשבר הוא שפל ''ובזוי עם''‫<ref group=note>תהילים כב, ז</ref> | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{ | + | ::You start to multiply and say: three by 11 is 33; 33 by 13 is 429; so the result of the required fractions is 429-[quarters] of a quarter of a fifth of a third of a sixth, like this: |
| − | | | + | |style="text-align:right;"|ותתחיל להכות ולומר שלשה בי"א הם ל"ג ול"ג בי"ג הם 429 הרי עלו כל השברים הנשאלים 429 רביעית חמישית שלישית שישית <sup>כזה</sup> |
| + | |} | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{3}{4}\sdot\left[\frac{2}{5}+\left(\frac{3}{4}\sdot\frac{1}{5}\right)\right]\sdot\left[\frac{4}{6}+\left(\frac{1}{3}\sdot\frac{1}{6}\right)\right]=\frac{3}{4}\sdot\left(\frac{11}{4}\sdot\frac{1}{5}\right)\sdot\left(\frac{13}{3}\sdot\frac{1}{6}\right)=\frac{3\sdot11\sdot13}{4}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{3}\sdot\frac{1}{6}=\frac{33\sdot13}{4}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{3}\sdot\frac{1}{6}=\frac{429}{4}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{3}\sdot\frac{1}{6}}}</math> | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| Line 5,093: | Line 6,089: | ||
|} | |} | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"|ותחלק אלו ה429 למורים אלו | + | :*Divide these 429 by the denominators, i.e. by 4, then the result by the other 4, the result by 5, and so on until they gone. |
| + | |style="width:45%; text-align:right;"|ותחלק אלו ה429 למורים אלו ר"ל לד' והיוצא לד' האחר והיוצא לה' וכן לכלם עד כלותם | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"|וכאשר ישאר דבר בשום חלוקה מהן | + | ::When something remains in any of these division, write it beneath that denominator. |
| + | |style="text-align:right;"|וכאשר ישאר דבר בשום חלוקה מהן תשימהו תחת המורה ההוא | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"|וככלות החשבון קודם כלות המורים ויצא לך בחלוק על אחד מהן פחות מהמורה אשר לפניו | + | ::When the number is gone before the denominators are gone and you get in the division by one of them less than the denominator that precedes it, write this result beneath this preceding denominator, then you know how many sixths or how many thirds of sixths they are. |
| + | |style="text-align:right;"|וככלות החשבון קודם כלות המורים ויצא לך בחלוק על אחד מהן פחות מהמורה אשר לפניו תשים אותו היוצא תחת המורה הזה אשר לפני ואז תדע כמה <s>ש</s> שישיות או כמה שלישיות שישיות הן | ||
| + | |- | ||
| + | |This is called the most beautiful [arrangement] as mentioned above, because it is to convert the particular to general, so that the fractions become greater and nicer. | ||
| + | |style="text-align:right;"|וזה נקרא כלילת יופי ‫<ref>46r</ref>כמו שנזכר למעלה לפי שהוא לעשות מהפרטים כללים יען יהיו השברים יותר גדולים ויותר יפים | ||
|- | |- | ||
| − | | | + | |The real beauty is see first if the dividend has any of these divisors and write it last [to the right], then once again with the quotient, and on the third time and so on. |
| − | |style="text-align:right;"|והיופי האמיתי כשתעיין בתחלה המספר המתחלק | + | |style="text-align:right;"|והיופי האמיתי כשתעיין בתחלה המספר המתחלק אם יש לו שום אחד מהמורים ההם ואותו תשים אחרון וכן בשנית ביוצא וכן בשלישית וכן לעולם |
|- | |- | ||
| − | | | + | |Do this only if you are asked how much are the remaining fractions, for if you did it for the purpose of expansion to a common denominator or for the purpose of [the operations discussed in] one of the next chapters, do not divide it by the denominators at all. Because I write it here only to teach you the procedure, although it is not its place and it is mentioned in other places. |
| − | |style="text-align:right;"|ולא תעשה זה כי אם כאשר ישאלו לך כמה עולים חלקים אלו הנשארות | + | |style="text-align:right;"|ולא תעשה זה כי אם כאשר ישאלו לך כמה עולים חלקים אלו הנשארות אכן אם עשית זה לצורך ההשואה או לצורך אחד מהשערים הבאים לא תחלקהו על המורים כלל כי לא כתבתיו כאן כי אם ללמדך על המעשה ואם אין זה מקומו ונזכר כבר במקומות אחרים |
|- | |- | ||
| | | | ||
| − | :*<math>\scriptstyle{\color{blue}{\frac{429}{4}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{3}\sdot\frac{1}{6}=\left(\frac{107}{4}\sdot\frac{1}{5}\sdot\frac{1}{3}\sdot\frac{1}{6}\right)+\left(\frac{1}{4}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{3}\sdot\frac{1}{6}\right)}}</math> | + | :*When you do it in our example: i.e. you divide the 429 by 4, which is the last denominator, the result of division is 107 and 1 remains. Write it beneath it. |
| − | |style="text-align:right;"|ובעשותך זה בדמיוננו זה ר"ל שתחלק ה429 על הד' שהוא המורה האחרון | + | ::<math>\scriptstyle{\color{blue}{\frac{429}{4}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{3}\sdot\frac{1}{6}=\left(\frac{107}{4}\sdot\frac{1}{5}\sdot\frac{1}{3}\sdot\frac{1}{6}\right)+\left(\frac{1}{4}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{3}\sdot\frac{1}{6}\right)}}</math> |
| + | |style="text-align:right;"|ובעשותך זה בדמיוננו זה ר"ל שתחלק ה429 על הד' שהוא המורה האחרון יצא בחילוק 107 וישאר א' ותשימהו תחתיו | ||
|- | |- | ||
| | | | ||
| − | :*<math>\scriptstyle{\color{blue}{\frac{107}{4}\sdot\frac{1}{5}\sdot\frac{1}{3}\sdot\frac{1}{6}=\left(\frac{26}{5}\sdot\frac{1}{3}\sdot\frac{1}{6}\right)+\left(\frac{3}{4}\sdot\frac{1}{5}\sdot\frac{1}{3}\sdot\frac{1}{6}\right)}}</math> | + | :*Divide this result by 4 that precedes it; the result of division is 26 and 3 remains. Write it beneath it. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{107}{4}\sdot\frac{1}{5}\sdot\frac{1}{3}\sdot\frac{1}{6}=\left(\frac{26}{5}\sdot\frac{1}{3}\sdot\frac{1}{6}\right)+\left(\frac{3}{4}\sdot\frac{1}{5}\sdot\frac{1}{3}\sdot\frac{1}{6}\right)}}</math> | ||
|style="text-align:right;"|‫[ותחלק זה היוצא לד' הקודם לו יצא בחלוק כ"ו וישארו ג' תשימם תחתיו | |style="text-align:right;"|‫[ותחלק זה היוצא לד' הקודם לו יצא בחלוק כ"ו וישארו ג' תשימם תחתיו | ||
|- | |- | ||
| | | | ||
| − | :*<math>\scriptstyle{\color{blue}{\frac{26}{5}\sdot\frac{1}{3}\sdot\frac{1}{6}=\left(\frac{5}{3}\sdot\frac{1}{6}\right)+\left(\frac{1}{5}\sdot\frac{1}{3}\sdot\frac{1}{6}\right)}}</math> | + | :*Divide this result by 5; the result of division is 5 and 1 remains. Write it beneath it. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{26}{5}\sdot\frac{1}{3}\sdot\frac{1}{6}=\left(\frac{5}{3}\sdot\frac{1}{6}\right)+\left(\frac{1}{5}\sdot\frac{1}{3}\sdot\frac{1}{6}\right)}}</math> | ||
|style="text-align:right;"|ותחלק זה היוצא לה' ויצא בחלוק ה' וישאר א' ותשימהו תחתיו | |style="text-align:right;"|ותחלק זה היוצא לה' ויצא בחלוק ה' וישאר א' ותשימהו תחתיו | ||
|- | |- | ||
| | | | ||
| − | :*<math>\scriptstyle{\color{blue}{\frac{5}{3}\sdot\frac{1}{6}=\frac{1}{6}+\left(\frac{2}{3}\sdot\frac{1}{6}\right)}}</math> | + | :*Divide this 5 by 3; the result is 1 and 2 remains. Write it beneath it. |
| − | |style="text-align:right;"|ותחלק]‫ ותחלק ה' אלו על הג' ויצא א' וישארו ב' ותשימם תחתיו | + | ::<math>\scriptstyle{\color{blue}{\frac{5}{3}\sdot\frac{1}{6}=\frac{1}{6}+\left(\frac{2}{3}\sdot\frac{1}{6}\right)}}</math> |
| + | |style="text-align:right;"|ותחלק]‫<ref>marg.</ref> ותחלק ה' אלו על הג' ויצא א' וישארו ב' ותשימם תחתיו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|וזה הא' אם היה גדול מהו' מדות שהוא המורה אשר לפני אלו הסמוך להם היה לנו לחלקם עליו והיוצא בחלוק היה שלימים | + | ::If this 1 was greater than 6, which is the denominator that precedes this one, next to it, we would have had to divide it by it and the result of division would have been integers, since it is first and the denominators are all gone. We would have written the remainder beneath it and it were sixths. |
| + | |style="text-align:right;"|וזה הא' אם היה גדול <sup>מהו'</sup> <s>מדות</s> שהוא המורה אשר לפני אלו הסמוך להם היה לנו לחלקם עליו והיוצא בחלוק היה שלימים אחר שהוא ראשון וכבר כלו המורים והנשאר הינו שמים אותו תחתיו והיה שישיות שלמות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Since it is less than it, we write it beneath it immediately, and we get that the remaining fractions are one-sixth, 2-thirds of a sixth, a fifth of a third of a sixth, 3-quarters of a fifth of a third of a sixth and a quarter of a quarter of a fifth of a third of a sixth. |
| + | |style="text-align:right;"|אכן לפי שהוא פחות ממנו נשימם תחתיו מיד ויצא לנו מזה שהשברים הנשארים עלו ששית א' שלמה וב' שלישיות ששית וחמישית שלישית שישית וג' רביעיות חמישית שלישית שישית ורביעית רביעית חמישית שלישית שישית | ||
|} | |} | ||
| Line 5,135: | Line 6,143: | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :According to the most beautiful [arrangement], i.e. to write the denominators calculatedly in order, the fractions result as in the second diagram and all yield the same sum. |
| + | |style="width:45%; text-align:right;"|ועל דרך היופי ר"ל לשים המורים בסדר בהשגחה יצאו החלקים כפי הצורה השנית והכל עולה לסך אחד | ||
| + | |- | ||
| + | |To make it easier for you, when you expande to a common denominator, if you find any denominator that appears the same number of times in all the numbers [to be expanded], i.e. for instance, that 8 is in each of them once, or twice, or three times, do not multiply by this denominator at all. When you write all the denominators, write it only as many times as it apears in one of the numbers. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}\sdot\frac{1}{n}\quad\frac{c}{d}\sdot\frac{1}{n}}}</math> | ||
| + | |style="text-align:right;"|וכדי להקל מעליך כאשר תעשה ההשואה אם תמצא <s>לכל אחד מ</s>המספרים ש<sup>ו</sup>ם מורה שוה לכלם פעמים שוות ר"ל ע'ד'מ' שהח' בכל אחת מהם פעם אחת או פעמי' ‫<ref>46v</ref>שלש לא תכפול שום המספרים ההם במו[ר]ה ההוא כלל ובהשימך כל המורים לא תשימה כי אם כפעמים ש{{#annot:term|358,2034|6UQE}}ישנו{{#annotend:6UQE}} באחד מהמספרי‫' | ||
|- | |- | ||
| − | | | + | |If it is in all of them, but not the same number of times - for instance, once in this one and twice or three times in the other - where it is found the maximal number of times, do not multiply by this denominator at all; as for the rest of the numbers, multiply each by this denominator, as many times as the excess of the maximal number of times over the number of times it is found in the present number. When you write the denominator, write it only as many times as the maximal number of times that it apears. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}\sdot\frac{1}{n}=\frac{a}{b}\sdot\frac{1}{n}\sdot\frac{n}{n}\sdot\frac{n}{n}\quad\frac{c}{d}\sdot\frac{1}{n}\sdot\frac{1}{n}\sdot\frac{1}{n}}}</math> |
| + | |style="text-align:right;"|ואם הוא בכלם אבל אינו בהם פעמים שוות אבל בזה פעם אחת ובזה שנים או שלשה ע'ד'מ' אשר ישנו שם פעמים לא תכפלנו במורה זה [כלל וכל אחד משאר המספרים תכפלנו במורה זה‫]‫<ref>marg.</ref> כ"כ פעמים כפעמים שהוא יותר כמספר הרב הפעמים שבמספר הזה הנכפל בו עתה ובהשימך המורה לא תשימנו כי אם כפעמים אשר הוא באשר הוא יותר פעמים | ||
|- | |- | ||
| − | | | + | |If it is not in all of them, but in two or three of them, multiply each of the numbers, in which it is not found at all, by this denominator, as the maximal number of times that it apears in one of them. Do not multiply the number, in which it is found the maximal number of times, by it. [As for the rest of] the numbers, in which it is found, multiply each by it, as many times as the excess of the maximal number of times over the number of times it is found in the present multiplicand. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}=\frac{a}{b}\sdot\frac{n}{n}\sdot\frac{n}{n}\quad\frac{c}{d}\sdot\frac{1}{n}\sdot\frac{1}{n}}}</math> | |
| − | :<math>\scriptstyle\frac{a}{b}\sdot\frac{ | + | |style="text-align:right;"|ואם אינו בכלן כי אם בשנים או בג' מהם המספרי' אשר אינו בהם כלל תכפול כל אחד מהם במורה זה כמספר הפעמים אשר הוא באשר הוא יותר פעמים והמספר אשר הוא בו יותר פעמים לא תכפלנו כלל והמספרים אשר ישנו בהם תכפול כל אחד בו כמספר הפעמים העודפים באשר הוא היותר פעמים מבזה הנכפל |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |If it is found in them the same number of times, do not multiply by it any of the numbers, in which it is found, and when you write the denominators, write it only as many times as the maximal number of times that it apears. |
| − | + | |style="text-align:right;"|ואם הוא בהם פעמים שוות לא תכפול בו שום אחד מהמספרים אשר הוא בו ובהשימך המורים לא תשימנו כי אם כפעמים אשר הוא באשר הוא יותר רב פעמים | |
| − | |||
| − | |style="text-align:right;"|ואם הוא | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :It follows that in the example of the expansion to a common denominator that we had at the beginning of this chapter, we did not have to multiply the 78-quarters of an eighth by 8 at all, nor the eighths of the seventh, as it is found in both equally. |
| + | |style="text-align:right;"|ויצא מזה כי במשל ההשואה שעשינו בתחלת שער זה לא היה לנו לכפול הע"ח רביעיות שמינית בח' כלל גם לא השמיני<sup>ו</sup>ת שביעית להיותו בשניהם בשוה | ||
|- | |- | ||
| | | | ||
| − | + | :We also had to [multiply] the 8 only once, as it is found, by one of the others. | |
| − | + | |style="text-align:right;"|גם לא היה לנו לשום הח' כי אם פעם אחת כאשר הוא באחד מהאחרים | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Among the denominators, we had to write the 8 only once, as the number of times that it is found in each of them. |
| + | |style="text-align:right;"|ובמורים לא היה לנו לשום הח' כי אם פעם אחת כפעמים אשר ישנו באחד מהם | ||
|- | |- | ||
| − | | | + | |It does not matter if all this is not done, but this will make the procedure more difficult. |
| − | + | |style="text-align:right;"|וכל זה אינו מזיק אם לא יעשה אבל כי תכבד העבודה | |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
|- | |- | ||
|} | |} | ||
| Line 5,173: | Line 6,182: | ||
== Chapter One: Addition == | == Chapter One: Addition == | ||
| − | + | |style="width:45%; text-align:right;"|<big>הפרק האחד <s>עשר</s> ‫<ref>47r</ref>בחבור</big> | |
|- | |- | ||
| − | | | + | |In it the discussion on [conversion] and summing. |
|style="text-align:right;"|בחיבור ובו מאמ' האמרה והאחדות | |style="text-align:right;"|בחיבור ובו מאמ' האמרה והאחדות | ||
|- | |- | ||
| + | |<span style=color:Green>The procedure:</span> | ||
| | | | ||
| − | |||
|- | |- | ||
| − | | | + | |When you wish to add fractions with integers or fractions with fractions of another type, first, decompose each of the numbers that requires decomposing to [the lowest type of] fraction by itself, then multiply what needs to be multiplied, and after you decompose [and multiply] whichever requires either both decomposing and multiplication, or one of them, expand the numbers to one type, sum up all their results together, i.e. the numerators, then we divide [the sum] by all the denominators of all the fractions. |
| − | |style="text-align:right;"|בתחלה תפרוט כל אחד מהמספרים לבדו אשר יצטרך פריטה | + | |style="text-align:right;"|כאשר תרצה לחבר שברים עם שלמים ושברים [או]‫<ref>marg.</ref> עם שברים ממין אחר בתחלה תפרוט כל אחד מהמספרים לבדו אשר יצטרך פריטה גם תכה הצריך להכאה ואחר שתפרוט וכל אחד מהם הצריך להם או לאחד מהם ר"ל לפריטה או להכאה תשוה המספרים אחד אל אחד עד שיהיו כלם ממין אחד והעולה בכל אחד מהם חבר הכל יחד ר"ל מספר השברים וחלקנו על כל המורים אשר לכל <s>אחד</s> השברים |
|- | |- | ||
| | | | ||
| − | *For instance, if | + | *{{#annot:(2+⅜+²/₄·⅛)+⅘+(⁶/₇+⅜·⅐)|677|O6E2}}For instance, if in the example for the expansion to a common denominator that we have presented at the beginning of the third chapter, you are asked to sum up them and say how much they are: |
:<math>\scriptstyle\left[2+\frac{3}{8}+\left(\frac{2}{4}\sdot\frac{1}{8}\right)\right]+\frac{4}{5}+\left[\frac{6}{7}+\left(\frac{3}{8}\sdot\frac{1}{7}\right)\right]</math> | :<math>\scriptstyle\left[2+\frac{3}{8}+\left(\frac{2}{4}\sdot\frac{1}{8}\right)\right]+\frac{4}{5}+\left[\frac{6}{7}+\left(\frac{3}{8}\sdot\frac{1}{7}\right)\right]</math> | ||
| − | |style="text-align:right;"|כי ע'ד'מ' אם במשלנו אשר עשינו בהשואה בתחלת השער הג' שאלו לך שתחברם ותאמ' כמה הם | + | |style="text-align:right;"|כי ע'ד'מ' אם במשלנו אשר עשינו בהשואה בתחלת השער הג' שאלו לך שתחברם ותאמ' כמה הם{{#annotend:O6E2}} |
|- | |- | ||
| | | | ||
| − | :You | + | :You had to do all that we have done: the decomposing of each of them and the expansion of all until they become what they are: |
|style="text-align:right;"|היה לך לעשות כל אשר עשינו הפריטה לכל אחד וההשואה לכלם עד שיגיעו לאשר הגיעו | |style="text-align:right;"|היה לך לעשות כל אשר עשינו הפריטה לכל אחד וההשואה לכלם עד שיגיעו לאשר הגיעו | ||
|- | |- | ||
| Line 5,196: | Line 6,205: | ||
:*The two integers, 3-eighths and 2-quarters of an eighth become 21840 eighths of a seventh of a fifth of a quarter of an eighth. | :*The two integers, 3-eighths and 2-quarters of an eighth become 21840 eighths of a seventh of a fifth of a quarter of an eighth. | ||
::<math>\scriptstyle{\color{blue}{2+\frac{3}{8}+\left(\frac{2}{4}\sdot\frac{1}{8}\right)=\frac{21840}{8}\sdot\frac{1}{7}\sdot\frac{1}{5}\sdot\frac{1}{4}\sdot\frac{1}{8}}}</math> | ::<math>\scriptstyle{\color{blue}{2+\frac{3}{8}+\left(\frac{2}{4}\sdot\frac{1}{8}\right)=\frac{21840}{8}\sdot\frac{1}{7}\sdot\frac{1}{5}\sdot\frac{1}{4}\sdot\frac{1}{8}}}</math> | ||
| − | |style="text-align:right;"|והוא שהשנים השלמים וג' שמיניות וב' רביעיות שמינית עלו ל21840 [שמיניות שביעית חמשית רביעית שמינית | + | |style="text-align:right;"|והוא שהשנים השלמים וג' שמיניות וב' רביעיות שמינית עלו ל21840 ‫[שמיניות שביעית חמשית רביעית שמינית |
|- | |- | ||
| | | | ||
:*The 4-fifths become 8160 parts of all the denominators. | :*The 4-fifths become 8160 parts of all the denominators. | ||
::<math>\scriptstyle{\color{red}{\frac{4}{5}=\frac{7168}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{8}\sdot\frac{1}{7}}}</math> | ::<math>\scriptstyle{\color{red}{\frac{4}{5}=\frac{7168}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{8}\sdot\frac{1}{7}}}</math> | ||
| − | |style="text-align:right;"|והד' חמשיות עלו | + | |style="text-align:right;"|והד' חמשיות עלו ל‫8160]‫<ref>marg.</ref> מכל המורים |
|- | |- | ||
| | | | ||
:After you do all this, you have to sum all the numbers of the fractions, i.e. 21840 with 7168 and 8160; the result is 37168, which are parts of the five mentioned denominators, i.e. 8, 4, 5, 7, and 8 that are all the denominators of the original numbers. | :After you do all this, you have to sum all the numbers of the fractions, i.e. 21840 with 7168 and 8160; the result is 37168, which are parts of the five mentioned denominators, i.e. 8, 4, 5, 7, and 8 that are all the denominators of the original numbers. | ||
| − | |||
|style="text-align:right;"|ואחר עשותך כל זה היה לך לחבר יחד כל מספרי השברים ר"ל ה21840 עם ה7168 ועם ה8160 ויעלו 37168 והם מהה' מורים הנזכרים ר"ל ה8 וה4 והה' והז' והח' שהם כל מורי המספרים הראשונים | |style="text-align:right;"|ואחר עשותך כל זה היה לך לחבר יחד כל מספרי השברים ר"ל ה21840 עם ה7168 ועם ה8160 ויעלו 37168 והם מהה' מורים הנזכרים ר"ל ה8 וה4 והה' והז' והח' שהם כל מורי המספרים הראשונים | ||
| + | |} | ||
| + | :<math>\scriptstyle{\color{blue}{\left[2+\frac{3}{8}+\left(\frac{2}{4}\sdot\frac{1}{8}\right)\right]+\frac{4}{5}+\left[\frac{6}{7}+\left(\frac{3}{8}\sdot\frac{1}{7}\right)\right]=\left(\frac{21840}{8}\sdot\frac{1}{7}\sdot\frac{1}{5}\sdot\frac{1}{4}\sdot\frac{1}{8}\right)+\left(\frac{7168}{8}\sdot\frac{1}{7}\sdot\frac{1}{4}\sdot\frac{1}{8}\sdot\frac{1}{5}\right)+\left(\frac{8160}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{8}\sdot\frac{1}{7}\right)=\frac{37168}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{8}\sdot\frac{1}{7}}}</math> | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | *Arrange them in | + | *Arrange them in order as you wish, or by observation, as we noted in the fourth chapter, so that the fraction will be more appropriate. You will find it explained properly there. |
| − | |style="text-align:right;"|ותשימם על הסדר כאשר תרצה או בהשגחה כאשר הזכרנו בפרק הרביעי כדי שיצאו החלקים יותר נאותים ושם תמצאנו מבואר באר הטב | + | |style="width:45%; text-align:right;"|ותשימם על הסדר כאשר תרצה או בהשגחה כאשר הזכרנו בפרק הרביעי כדי שיצאו החלקים יותר נאותים ושם תמצאנו מבואר באר הטב |
|- | |- | ||
| | | | ||
| − | :*Since this number 37168 is divisible by eight, which is one of the denominators, we place it last, i.e. | + | :*Since this number 37168 is divisible by eight, which is one of the denominators, we place it last, i.e. first. |
::<math>\scriptstyle{\color{red}{\frac{37168}{8}=4646}}</math> | ::<math>\scriptstyle{\color{red}{\frac{37168}{8}=4646}}</math> | ||
|style="text-align:right;"|ולהיות לזה החשבון 37168 המתחלק שמינית שהוא אחד מהמורים נשימנו אחרון ר"ל הראשון | |style="text-align:right;"|ולהיות לזה החשבון 37168 המתחלק שמינית שהוא אחד מהמורים נשימנו אחרון ר"ל הראשון | ||
| Line 5,220: | Line 6,231: | ||
:*We divide our number by it, i.e. by 7; the result of division is 663 and 5 remain. We place them beneath. | :*We divide our number by it, i.e. by 7; the result of division is 663 and 5 remain. We place them beneath. | ||
::<math>\scriptstyle{\color{blue}{\frac{4646}{7}=663+\frac{5}{7}}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{4646}{7}=663+\frac{5}{7}}}</math> | ||
| − | |style="text-align:right;"|ונחלק חשבונננו זה עליו ר"ל 7 ויצא בחילוק 663 [נ' 3] וישארו ה' ונשימם תחתיו | + | |style="text-align:right;"|ונחלק חשבונננו זה עליו ר"ל 7 ויצא בחילוק 663 [נ' 3]‫<ref>marg.</ref> וישארו ה' ונשימם תחתיו |
|- | |- | ||
| | | | ||
| Line 5,230: | Line 6,241: | ||
:*We divide these 33 resulting in division by the 8, which is the remaining denominator; the result of division is 4, which are integers, since all the denominators are gone. We place them aside. One remains, which is an eighth. We place it beneath, like this: | :*We divide these 33 resulting in division by the 8, which is the remaining denominator; the result of division is 4, which are integers, since all the denominators are gone. We place them aside. One remains, which is an eighth. We place it beneath, like this: | ||
::<math>\scriptstyle{\color{blue}{\frac{33}{8}=4+\frac{1}{8}}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{33}{8}=4+\frac{1}{8}}}</math> | ||
| − | |style="text-align:right;"|ונחלקם אלו הל"ג היוצאים בחילוק על הח' שהוא המורה הנשאר ויצא בחלוק ד' ד' והם שלמים לפי שכבר כלו כל המורים ונשימם מחוץ וישאר א' והוא שמינית שלימה ונשימה תחת כזה | + | |style="text-align:right;"|ונחלקם אלו הל"ג היוצאים בחילוק על הח' שהוא המורה הנשאר ויצא בחלוק <s>ד'</s> ד' והם שלמים לפי שכבר כלו כל המורים ונשימם מחוץ וישאר א' והוא ‫<ref>47v</ref>שמינית שלימה ונשימה תחת כזה |
|- | |- | ||
| | | | ||
| Line 5,243: | Line 6,254: | ||
| | | | ||
:We get that when we sum two integers, 3-eighths and 2-quarters of an eighth with 4-fifths, and with 6-sevenths and 3-eighths of a seventh, the total sum is 4 integers, one eighth, 3-quarters of a fifth of an eighth, and 5-sevenths of a quarter of a fifth of an eighth. Deduce from this. | :We get that when we sum two integers, 3-eighths and 2-quarters of an eighth with 4-fifths, and with 6-sevenths and 3-eighths of a seventh, the total sum is 4 integers, one eighth, 3-quarters of a fifth of an eighth, and 5-sevenths of a quarter of a fifth of an eighth. Deduce from this. | ||
| − | :<math>\scriptstyle{\color{blue}{ | + | |style="text-align:right;"|הנה עלה בידינו שכאשר חברנו השנים שלמים וג' שמיניות וב' רביעיות שמינית עם ד' חמישיות ועם ו' שביעיות וג' שמיניות שביעית שעלה הכל ד' שלמים ושמינית אחת וג' רביעיות חמישית שמינית וה' שביעיות רביעית חמישית שמינית והקש על זה והה‫' |
| − | | | + | |} |
| + | :<math>\scriptstyle{\color{blue}{\left[2+\frac{3}{8}+\left(\frac{2}{4}\sdot\frac{1}{8}\right)\right]+\frac{4}{5}+\left[\frac{6}{7}+\left(\frac{3}{8}\sdot\frac{1}{7}\right)\right]=4+\frac{1}{8}+\left(\frac{3}{4}\sdot\frac{1}{5}\sdot\frac{1}{8}\right)+\left(\frac{5}{7}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{8}\right)}}</math> | ||
| + | {| | ||
|- | |- | ||
| − | |This is the reason that if you are told [to sum] numerous numbers, you need to multiply [ | + | |This is the reason that if you are told [to sum] numerous numbers, you need to multiply first, before the expansion [to a common denominator], then the expansion and afterwards the summing, as mentioned. |
| − | |style="text-align:right;"|והוא הטעם אם אמרו לך מספרים רבים והיו בהם שצריכין <s>ג"כ הכאה קודם השיווי</s> שתעשה להם ג"כ ההכאה קודם השיווי ואחר כך ההשוואה וא'ח'כ' החבור כנזכר | + | |style="width:45%; text-align:right;"|והוא הטעם אם אמרו לך מספרים רבים והיו בהם שצריכין <s>ג"כ הכאה קודם השיווי</s> שתעשה להם ג"כ ההכאה קודם השיווי ואחר כך ההשוואה וא'ח'כ' החבור כנזכר |
|- | |- | ||
| | | | ||
| Line 5,272: | Line 6,285: | ||
| | | | ||
:You receive 4 integers, 25-eighths of one-fifth of a fifth, 3-quarters of one-eighth of a fifth and 5-sevenths of one-quarter of one-eighth of a fifth. It is all the same and it is enough for the one who understands. | :You receive 4 integers, 25-eighths of one-fifth of a fifth, 3-quarters of one-eighth of a fifth and 5-sevenths of one-quarter of one-eighth of a fifth. It is all the same and it is enough for the one who understands. | ||
| − | |||
|style="text-align:right;"|ויעלה בידך ד' שלמים כ"ה שמיניות חמישית חמישית וג' רביעיות שמינית חמישית <s>וג' רביעית שמינית חמישית</s> וה' שביעיות רביעית שמינית חמישית והכל אחד ודי למבין | |style="text-align:right;"|ויעלה בידך ד' שלמים כ"ה שמיניות חמישית חמישית וג' רביעיות שמינית חמישית <s>וג' רביעית שמינית חמישית</s> וה' שביעיות רביעית שמינית חמישית והכל אחד ודי למבין | ||
| + | |} | ||
| + | :<math>\scriptstyle{\color{blue}{\left[2+\frac{3}{8}+\left(\frac{2}{4}\sdot\frac{1}{8}\right)\right]+\frac{4}{5}+\left[\frac{6}{7}+\left(\frac{3}{8}\sdot\frac{1}{7}\right)\right]=4+\frac{1}{8}+\left(\frac{3}{4}\sdot\frac{1}{5}\sdot\frac{1}{8}\right)+\left(\frac{5}{7}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{8}\right)=4+\left(\frac{25}{8}\sdot\frac{1}{5}\sdot\frac{1}{5}\right)+\left(\frac{3}{4}\sdot\frac{1}{8}\sdot\frac{1}{5}\right)+\left(\frac{5}{7}\sdot\frac{1}{4}\sdot\frac{1}{8}\sdot\frac{1}{5}\right)}}</math> | ||
| + | {| | ||
|- | |- | ||
| | | | ||
*If you are told to convert them to another type that is not among the denominators: | *If you are told to convert them to another type that is not among the denominators: | ||
| − | |style="text-align:right;"|אכן אם אמרו לך {{#annot:term|2613,1429|4lUo}}להחזירם{{#annotend:4lUo}} ממין אחר שאינו במורי‫' | + | |style="width:45%; text-align:right;"|אכן אם אמרו לך {{#annot:term|2613,1429|4lUo}}להחזירם{{#annotend:4lUo}} ממין אחר שאינו במורי‫' |
|- | |- | ||
| | | | ||
| Line 5,284: | Line 6,299: | ||
|- | |- | ||
| | | | ||
| − | :This is called the conversion category: after | + | :This is called the conversion category: after decomposing, multiplying, and expanding [to a common denominator], before you divide by the mentioned denominators, multiply the resulting numerator of these fractions, i.e. 37168, by the denominator, to which it is required to convert, i.e. 9, which is the denominator of the ninth; the result is 334512. We write the 9 as the first denominator and all the other denominators after it, randomly or by observation. |
:<math>\scriptstyle{\color{blue}{37168\sdot9=334512}}</math> | :<math>\scriptstyle{\color{blue}{37168\sdot9=334512}}</math> | ||
|style="text-align:right;"|זה יקרא מאמר ההמרה והוא שאחרי עשותך הפריטה וההכאה וההשואה קודם שתחלקם למורים הנזכרים תכפול כל חשבון השברים ר"ל ה37168 בזה המורה אשר רצו להחליפם אליו ר"ל הט' שהוא המורה התשיעית ויעלו 334512 ונשים הט' למורה ראשון וכל המורים האחרי' אחריו אם כאשר יזדמן אם בהשגחה | |style="text-align:right;"|זה יקרא מאמר ההמרה והוא שאחרי עשותך הפריטה וההכאה וההשואה קודם שתחלקם למורים הנזכרים תכפול כל חשבון השברים ר"ל ה37168 בזה המורה אשר רצו להחליפם אליו ר"ל הט' שהוא המורה התשיעית ויעלו 334512 ונשים הט' למורה ראשון וכל המורים האחרי' אחריו אם כאשר יזדמן אם בהשגחה | ||
| Line 5,299: | Line 6,314: | ||
|- | |- | ||
| | | | ||
| − | :*<math>\scriptstyle{\color{ | + | :*<math>\scriptstyle{\color{OliveGreen}{\frac{10453}{5}=2090+\frac{3}{5}}}</math> |
| + | | | ||
| + | |- | ||
| + | | | ||
:*We divide it by 7; the result of division is 298 and 4 remain. We place them beneath it. | :*We divide it by 7; the result of division is 298 and 4 remain. We place them beneath it. | ||
::<math>\scriptstyle{\color{blue}{\frac{2090}{7}=298+\frac{4}{7}}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{2090}{7}=298+\frac{4}{7}}}</math> | ||
| − | |style="text-align:right;"|ונחלקנו לז' ויצא בחילוק 298 וישארו ד' ונשימם תחתיו | + | |style="text-align:right;"|ונחלקנו ‫<ref>48r</ref>לז' ויצא בחילוק 298 וישארו ד' ונשימם תחתיו |
|- | |- | ||
| | | | ||
| Line 5,316: | Line 6,334: | ||
| | | | ||
:Thus, we have converted the fractions into ninths and parts of ninths. | :Thus, we have converted the fractions into ninths and parts of ninths. | ||
| − | |||
|style="text-align:right;"|והנה המרנו החלקים ר"ל השברים לתשיעית וחלקי תשיעית | |style="text-align:right;"|והנה המרנו החלקים ר"ל השברים לתשיעית וחלקי תשיעית | ||
| + | |} | ||
| + | :<math>\scriptstyle{\color{blue}{\left[2+\frac{3}{8}+\left(\frac{2}{4}\sdot\frac{1}{8}\right)\right]+\frac{4}{5}+\left[\frac{6}{7}+\left(\frac{3}{8}\sdot\frac{1}{7}\right)\right]=4+\frac{1}{9}+\left(\frac{2}{8}\sdot\frac{1}{9}\right)+\left(\frac{4}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}\right)+\left(\frac{3}{5}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}\right)+\left(\frac{2}{4}\sdot\frac{1}{5}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}\right)}}</math> | ||
| + | {| | ||
|- | |- | ||
|The rule of this category [i.e. conversion] is that whenever you are asked with regard to a known type of fractions, whether various or not, to convert them to another type, whether a fraction or a fraction of a fraction: | |The rule of this category [i.e. conversion] is that whenever you are asked with regard to a known type of fractions, whether various or not, to convert them to another type, whether a fraction or a fraction of a fraction: | ||
| − | |style="text-align:right;"|כלל זה מאמר זה הוא שכאשר ישאלו לך על חלקי' ידועים שונים ובלתי שונים שתמירם למין אחר בין אם יאמרו לך לשבר או לשבר שבר | + | |style="width:45%; text-align:right;"|כלל זה מאמר זה הוא שכאשר ישאלו לך על חלקי' ידועים שונים ובלתי שונים שתמירם למין אחר בין אם יאמרו לך לשבר או לשבר שבר |
|- | |- | ||
| | | | ||
| Line 5,326: | Line 6,346: | ||
|style="text-align:right;"|כמו שיאמרו לך השיבם לחמישיות שביעית שמינית או הדומה לזה | |style="text-align:right;"|כמו שיאמרו לך השיבם לחמישיות שביעית שמינית או הדומה לזה | ||
|- | |- | ||
| − | |You should first | + | |You should first decompose, multiply, and expand the fractions, if they are various, then sum them together and multiply the sum by the denominator or the denominators, to which you need to convert them. |
|style="text-align:right;"|יש לך לעשות תחלה פריטה והכאה והשואה לשברים אם היו שונים ושוב תחברם יחד ושוב תכפלם כלם ביחד על המורה או המורים אשר רוצים שתמירם אליהם | |style="text-align:right;"|יש לך לעשות תחלה פריטה והכאה והשואה לשברים אם היו שונים ושוב תחברם יחד ושוב תכפלם כלם ביחד על המורה או המורים אשר רוצים שתמירם אליהם | ||
|- | |- | ||
| Line 5,337: | Line 6,357: | ||
|style="text-align:right;"|ואם אמרו לך לחמישיות שביעית שמינית תכפלם בח' והעולה בז' והעולה בה‫' | |style="text-align:right;"|ואם אמרו לך לחמישיות שביעית שמינית תכפלם בח' והעולה בז' והעולה בה‫' | ||
|- | |- | ||
| − | | | + | |After you do all that, write the denominator or denominators of the first conversion to the right by the required order: first the 8, after it the 7, after it the 5, then arrange after them the denominators that you have randomly of calculatedly and divide by them the number that you get from the multiplication of the numerators by the denominator or denominators of the conversion. All this is clear by reason. |
|style="text-align:right;"|ואחר עשותך כל זה תשים מורה או מורה ההמרה ראשונה לצד ימין על הסדר שנשאל הח' תחלה ואחריו הז' ואחריו הה' ושוב תסדר אחריהם מורה שבידך כפי המזדמן או בהשגחה ותחלק על כלם המספר אשר עלה לידיך מכפל מספר שבריך במורה או מורי ההמרה וכל זה ברור בטעם | |style="text-align:right;"|ואחר עשותך כל זה תשים מורה או מורה ההמרה ראשונה לצד ימין על הסדר שנשאל הח' תחלה ואחריו הז' ואחריו הה' ושוב תסדר אחריהם מורה שבידך כפי המזדמן או בהשגחה ותחלק על כלם המספר אשר עלה לידיך מכפל מספר שבריך במורה או מורי ההמרה וכל זה ברור בטעם | ||
|- | |- | ||
| − | | | + | |For, if you multiply what you have by given denominators, the product always have these denominators in addition to its original denominators. Therefore, when you wish to reduce them, i.e. to convert these decomposed fractions to proper integers and fractions, you have to arrange these denominators, by whice they were multiplied, with their original denominators and the order does not matter. Since it is asked how many fractions of these denominators they are, we write them first in this procedure. |
| − | |style="text-align:right;"|כי לעולם אם תכפול אשר בידך במורים מונחים | + | |style="text-align:right;"|כי לעולם אם תכפול אשר בידך במורים מונחים הנה יהיה למקובץ מורים אלו מוספים על מוריו הראשונים ולכן כאשר תרצה לעשות להם {{#annot:term|1555,2488|Mkxu}}כלילת יופי{{#annotend:Mkxu}} ר"ל להשיב שברים אלו הנפרטות לכללים וחלקים יפים יש לך לסדר עם מוריו הראשונים אלו המורים אשר הוכפלו בהם והסדר לא יזיק ולפי ששאלו כמה חלקים הם מהמורים האלו לכן נשימם ראשונה במלאכה |
|- | |- | ||
| − | |'''ha-Aḥdut''' | + | |If you are told to convert them to another fraction as greater as possible, it is called '''ha-Aḥdut''' [lit. unification] and it is an important issue, because by it we can divide the smaller by the greater and to generate denominators without extracting the denominators of the number by which we want to divide or to add to its denominators. |
| − | |style="text-align:right;"|אכן אם יאמרו לך להשיבם לחלק אחר | + | |style="text-align:right;"|אכן אם יאמרו לך להשיבם לחלק אחר <sup>ה</sup>גדול שאיפשר לכן נקרא<s>ה</s> האחדות והוא ענין נכבד ‫<ref>48v</ref>כי ממנו יצא לנו לחלק מעט על רב ולחדש מורים <s>ב</s> בעצמינו מבלי הוצאת מורי המספר שרצינו לחלק עליו או גם להוסיף על מוריו |
|- | |- | ||
| − | | | + | |For this I assigned it a discussion of its own and I write it in this chapter as it is a kind of addition. |
|style="text-align:right;"|לזה הקצתי לו מאמר לבדו ואכתבנו בזה הפרק לפי שהוא כעין חבור | |style="text-align:right;"|לזה הקצתי לו מאמר לבדו ואכתבנו בזה הפרק לפי שהוא כעין חבור | ||
|- | |- | ||
| − | | | + | |I named it "ha-Aḥdut" [lit. unification], since we want to convert them to one fraction whether it is possible or not. |
|style="text-align:right;"|וקראתי לו שם שם האחדות לפי שאנו רוצים לעשותם חלק אחד אם איפשר ואם הוא בלתי איפשר | |style="text-align:right;"|וקראתי לו שם שם האחדות לפי שאנו רוצים לעשותם חלק אחד אם איפשר ואם הוא בלתי איפשר | ||
|- | |- | ||
| − | | | + | |If it is impossible, we have to add one in the procedure as will be explained. |
|style="text-align:right;"|ואם הוא בלתי איפשר יש לנו להוסיף אחד במלאכה כאש' יתבאר | |style="text-align:right;"|ואם הוא בלתי איפשר יש לנו להוסיף אחד במלאכה כאש' יתבאר | ||
|- | |- | ||
| − | | | + | |For these two reasons I called it "ha-aḥdut". |
|style="text-align:right;"|לב' כוונות אלו קראתי לו שם האחדות | |style="text-align:right;"|לב' כוונות אלו קראתי לו שם האחדות | ||
|- | |- | ||
| | | | ||
| − | ===Summing fractions to one fraction=== | + | === <span style=color:Green>Summing fractions to one fraction</span> === |
| − | + | |style="width:45%; text-align:right;"|<big>מאמר האחדות</big> | |
|- | |- | ||
| − | | | + | |If you wish to convert fractions, equal or different, into one fraction if possible, or as greatest as possible. |
| − | |style="text-align:right;"|אם רצית להשיב שברים שוים שוים או שונים לחלק אחד אם איפשר או לגדול שאיפשר | + | |style="text-align:right;"|אם רצית להשיב שברים <s>שוים</s> <sup>שוים</sup> או שונים לחלק אחד אם איפשר או לגדול שאיפשר |
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle\left(\frac{2}{5}\sdot\frac{2}{9}\sdot2\right)+\left[\left[\frac{1}{8}+\left(\frac{2}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\right)\right]\sdot\left[\frac{1}{4}+\left(\frac{2}{6}\sdot\frac{1}{4}\right)\right]\right]</math> | + | *{{#annot:(⅖·²/₉·2)+(⅛+²/₉·⅐·⅛)·(¼+²/₆·¼)|677|GkGP}}Example: two-fifths of 2-ninths of 2 integers, and one-eighth, and two-ninths of one-seventh of one-eighth of one-quarter and two-sixths of one-quarter. |
| − | |style="text-align:right;"|המשל שני חמישיות מב' תשיעיות מב' שלמים ועוד שמינית אחת ושני תשיעיות שביעית שמינית מרביעית ושתי ששיות רביעית | + | :<math>\scriptstyle\left(\frac{2}{5}\sdot\frac{2}{9}\sdot2\right)+\left[\left[\frac{1}{8}+\left(\frac{2}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\right)\right]\sdot\left[\frac{1}{4}+\left(\frac{2}{6}\sdot\frac{1}{4}\right)\right]\right]</math> |
| + | |style="text-align:right;"|<big>המשל</big> שני חמישיות מב' תשיעיות מב' שלמים ועוד שמינית אחת ושני תשיעיות שביעית שמינית מרביעית ושתי ששיות רביעית{{#annotend:GkGP}} | ||
|- | |- | ||
| | | | ||
| + | :Arrange them like this: | ||
|style="text-align:right;"|תשימם על הסדר כזה | |style="text-align:right;"|תשימם על הסדר כזה | ||
|- | |- | ||
| Line 5,393: | Line 6,415: | ||
|} | |} | ||
|- | |- | ||
| − | | | + | |Apply on them [the operations of] decomposition, multiplication, expansion [to a common denominator], and summation. |
|style="text-align:right;"|תעשה להם פריטה והכאה והשוואה וחיבור | |style="text-align:right;"|תעשה להם פריטה והכאה והשוואה וחיבור | ||
|- | |- | ||
| − | | | + | |In order to train you more in the procedure I will perform them one by one: |
|style="text-align:right;"|וכדי להרגילך עוד במעשה אעשה אחת אחת | |style="text-align:right;"|וכדי להרגילך עוד במעשה אעשה אחת אחת | ||
|- | |- | ||
| | | | ||
| − | ::We | + | ::We decompose the quarter and the two-sixths of one-quarter: we multiply 1 by 6; they are 6; we add to them the units that are beneath them, i.e. the 2; the result is 8-sixths of a quarter. |
::<math>\scriptstyle{\color{blue}{\frac{1}{4}+\left(\frac{2}{6}\sdot\frac{1}{4}\right)=\frac{\left(1\sdot6\right)+2}{6}\sdot\frac{1}{4}=\frac{6+2}{6}\sdot\frac{1}{4}=\frac{8}{6}\sdot\frac{1}{4}}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{1}{4}+\left(\frac{2}{6}\sdot\frac{1}{4}\right)=\frac{\left(1\sdot6\right)+2}{6}\sdot\frac{1}{4}=\frac{6+2}{6}\sdot\frac{1}{4}=\frac{8}{6}\sdot\frac{1}{4}}}</math> | ||
|style="text-align:right;"|נעשה פריטה לרביעית ושתי שישיות רביעית<br> | |style="text-align:right;"|נעשה פריטה לרביעית ושתי שישיות רביעית<br> | ||
| Line 5,406: | Line 6,428: | ||
|- | |- | ||
| | | | ||
| − | ::We | + | ::We also decompose the seventh and the 2-ninths of one-seventh of one-eighth: we multiply 1 by 7, then we multiply them further by 9; they are 63; we add to them the two; the result is 65-ninths of one-seventh of an eighth. |
| − | |||
|style="text-align:right;"|עוד נעשה פריטה לשביעית וב' תשיעיות שביעית שמינית<br> | |style="text-align:right;"|עוד נעשה פריטה לשביעית וב' תשיעיות שביעית שמינית<br> | ||
נכפול א' בז' נכפלם עוד בט' יהיו ס"ג ונחבר להם השנים ויעלו ס"ה תשיעיות שביעיות שמינית | נכפול א' בז' נכפלם עוד בט' יהיו ס"ג ונחבר להם השנים ויעלו ס"ה תשיעיות שביעיות שמינית | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\left[\frac{1}{8}+\left(\frac{2}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\right)\right]\sdot\left[\frac{1}{4}+\left(\frac{2}{6}\sdot\frac{1}{4}\right)\right]=\left(\frac{65}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\right)\sdot\left(\frac{8}{6}\sdot\frac{1}{4}\right)}}</math> | + | ::<math>\scriptstyle{\color{blue}{\frac{1}{8}+\left(\frac{2}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\right)=\frac{\left(1\sdot7\sdot9\right)+2}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}=\frac{63+2}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}=\frac{65}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}}}</math> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | ::It is as if one says: 65-ninths of a seventh of an eighth of 8-sixths of a quarter, like this: | ||
| + | ::<math>\scriptstyle{\color{blue}{\left[\frac{1}{8}+\left(\frac{2}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\right)\right]\sdot\left[\frac{1}{4}+\left(\frac{2}{6}\sdot\frac{1}{4}\right)\right]=\left(\frac{65}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\right)\sdot\left(\frac{8}{6}\sdot\frac{1}{4}\right)}}</math> | ||
|style="text-align:right;"|והרי הוא כאלו אמרו ס"ה תשיעיות שביעית שמינית מח' שישיות רביעית כזה | |style="text-align:right;"|והרי הוא כאלו אמרו ס"ה תשיעיות שביעית שמינית מח' שישיות רביעית כזה | ||
|- | |- | ||
| Line 5,427: | Line 6,453: | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{ | + | :We also multiply the two numbers we have: |
| − | | | + | |style="text-align:right;"|עוד נעשה הכאה לשני מספרי' שבידינו |
| − | + | |- | |
| + | | | ||
| + | ::We start with the first number and say: 2 by 2 is 4. We multiply it also by the two integers; it is 8-fifths of a ninth, like this: | ||
| + | |style="text-align:right;"|ונתחיל במספ' הראשון ונאמ ב' בב' הם ד' נכפלם עוד בשני השלמים יהיו ח' חמישיות תשיעית שלימה ‫<ref>49r</ref>כזה | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{2}{5}\sdot\frac{2}{9}\sdot2=\frac{2\sdot2\sdot2}{5}\sdot\frac{1}{9}=\frac{4\sdot2}{5}\sdot\frac{1}{9}=\frac{8}{5}\sdot\frac{1}{9}}}</math> | ||
| + | | | ||
|- | |- | ||
| | | | ||
| Line 5,441: | Line 6,474: | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We multiply also the second number, the eight-sixths of a quarter, by 65; the result is 520-ninths of a seventh of an eighth of a sixth of a quarter, like this: |
|style="text-align:right;"|עוד נכה במספר השני השמונה ששיות רביעית בס"ה ויעלו 520 תשיעיות שביעית שמינית שישית רביעית כזה | |style="text-align:right;"|עוד נכה במספר השני השמונה ששיות רביעית בס"ה ויעלו 520 תשיעיות שביעית שמינית שישית רביעית כזה | ||
|- | |- | ||
| Line 5,452: | Line 6,485: | ||
| 520 || || || || | | 520 || || || || | ||
|} | |} | ||
| + | |} | ||
| + | |||
| + | ::<math>\scriptstyle{\color{blue}{\left[\frac{1}{8}+\left(\frac{2}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\right)\right]\sdot\left[\frac{1}{4}+\left(\frac{2}{6}\sdot\frac{1}{4}\right)\right]=\left(\frac{65}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\right)\sdot\left(\frac{8}{6}\sdot\frac{1}{4}\right)=\frac{65\sdot8}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{6}\sdot\frac{1}{4}=\frac{520}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{6}\sdot\frac{1}{4}}}</math> | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ונעשה ההשואה לאלו השני מספרים | + | :We expand these two numbers [to a common denominator]: |
| + | |style="width:45%; text-align:right;"|ונעשה ההשואה לאלו השני מספרים | ||
|- | |- | ||
| | | | ||
| + | ::Since the denominator 9 is found in each of them once, we do not multiply any of the numbers by it and we write it only once, as I mentioned at the end of chapter three. | ||
|style="text-align:right;"|ואחר היות בכל אחת מהם מורה הט' פעם אחת לא נכפול בו שום אחד מהמספרים ולא נסדרהו כי אם פעם אחת כאשר הזכרתי בסוף השער הג‫' | |style="text-align:right;"|ואחר היות בכל אחת מהם מורה הט' פעם אחת לא נכפול בו שום אחד מהמספרים ולא נסדרהו כי אם פעם אחת כאשר הזכרתי בסוף השער הג‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue} | + | ::We multiply the 8-fifths of ninths by all the denominators of the other number, except for the 9, as explained, and we say: eight by 4 is 32. We multiply it by 6; the result is 192. We multiply it by 8; the result is 1536. We multiply it by 7; the result is 10752. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונכפול הח' חמישיות תשיעיות בכל מורה המספר האחר זולתי הט' כאשר התבאר ונאמר שמונה בד' יעלה ל"ב נכפלם בו' יעלו 192 נכפלם בח' יעלו 1536 נכפלם בז' יעלו 10752 |
| − | + | |} | |
| − | + | ::<math>\scriptstyle{\color{blue}{8\sdot4\sdot6\sdot8\sdot7=32\sdot6\sdot8\sdot7=192\sdot8\sdot7=1536\sdot7=10752}}</math> | |
| − | + | ::<math>\scriptstyle{\color{blue}{\frac{2}{5}\sdot\frac{2}{9}\sdot2=\frac{8}{5}\sdot\frac{1}{9}=\frac{8\sdot4\sdot6\sdot8\sdot7}{5}\sdot\frac{1}{9}\sdot\frac{1}{4}\sdot\frac{1}{6}\sdot\frac{1}{8}\sdot\frac{1}{7}=\frac{10752}{5}\sdot\frac{1}{9}\sdot\frac{1}{4}\sdot\frac{1}{6}\sdot\frac{1}{8}\sdot\frac{1}{7}}}</math> | |
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply also the 520, which is the numerator of the other fractions, by 5, which is the denominator of the other, but not by 9 as mentioned; the result is 2600. | ||
| + | |style="width:45%; text-align:right;"|עוד נשוב לכפול ה520 שהם מספ' השברים האחרים בה' שהוא מורה חבריהם ולא בט' כנזכר ויעלו 2600 | ||
|- | |- | ||
| | | | ||
| − | :: | + | :We arrange them one above the other, like this: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נסדרם זה על זה כזה |
|- | |- | ||
| | | | ||
| Line 5,479: | Line 6,523: | ||
| style="text-align: left;" | 13352 | | style="text-align: left;" | 13352 | ||
|} | |} | ||
| + | |} | ||
| + | ::<math>\scriptstyle{\color{blue}{\left[\frac{1}{8}+\left(\frac{2}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\right)\right]\sdot\left[\frac{1}{4}+\left(\frac{2}{6}\sdot\frac{1}{4}\right)\right]=\frac{520}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{6}\sdot\frac{1}{4}=\frac{520\sdot5}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{6}\sdot\frac{1}{4}\sdot\frac{1}{5}=\frac{2600}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{6}\sdot\frac{1}{4}\sdot\frac{1}{5}}}</math> | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | : | + | :We sum them together; the result is 13352. |
| − | |style="text-align:right;"|ונחברם יחד יעלו 13352 | + | |style="width:45%; text-align:right;"|ונחברם יחד יעלו 13352 |
| − | |||
| − | |||
|- | |- | ||
| − | |<span style=color: | + | | |
| − | |style="text-align:right;"|נעיין תחלה איזהו המספר שהוא בעל אלו המורים כלם לבדם ר"ל שהוא מורכב מהם ונקרא למספר הזה אם המורים כי היא ילדתם וממנה יצאו{{#annotend:V17G}} | + | :We arrange all the denominators, i.e. all the denominators of both numbers, except for the 9 that we write only once and we write our number beneath the last denominator, as it is the numerator that is decomposed to all of these denominators. |
| + | |style="text-align:right;"|נסדר כל המורים ר"ל כל מורי שני המספרי' בלתי הט' שלא נשימנו כי אם פעם אחת ונשים מספרינו תחת המורה האחרון לפי שהוא שברים נפרטות מכל אלו המורים | ||
| + | |- | ||
| + | | | ||
| + | :So, it is as if we are asked: which fraction are 13352-sevenths of an eighth of a sixth of a quarter of a fifth of a ninth, are they realy fraction of one, or as greater fraction as possible? | ||
| + | |style="text-align:right;"|והרי <sup>זה</sup> כאלו שאלו לנו 13352 שביעיות שמינית שישית רביעית חמישית תשיעית איזה חלק הם אם הם חלק אחד ממש או החלק הגדול שאפשר | ||
| + | |} | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\frac{2}{5}\sdot\frac{2}{9}\sdot2\right)+\left[\left[\frac{1}{8}+\left(\frac{2}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\right)\right]\sdot\left[\frac{1}{4}+\left(\frac{2}{6}\sdot\frac{1}{4}\right)\right]\right]=\left(\frac{10752}{5}\sdot\frac{1}{9}\sdot\frac{1}{4}\sdot\frac{1}{6}\sdot\frac{1}{8}\sdot\frac{1}{7}\right)+\left(\frac{2600}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{6}\sdot\frac{1}{4}\sdot\frac{1}{5}\right)=\left(\frac{13352}{7}\sdot\frac{1}{8}\sdot\frac{1}{6}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{9}\right)}}</math> | ||
| + | {| | ||
| + | |- | ||
| + | |<span style=color:Green>Definition of the common denominator:</span> {{#annot:definition|1163|V17G}} first we examine which number has all these denominators alone, i.e. that consists of them and we call this number "the common denominator" [lit. the mother of the denominators], for it gave tham birth and they came out from it. | ||
| + | |style="width:45%; text-align:right;"|נעיין תחלה איזהו המספר שהוא בעל אלו המורים כלם לבדם ר"ל שהוא מורכב מהם ונקרא למספר הזה אם המורים כי היא ילדתם וממנה יצאו{{#annotend:V17G}} | ||
|- | |- | ||
|This is known by multiplying all the denominators one by the other and the product by another and so on until they end. | |This is known by multiplying all the denominators one by the other and the product by another and so on until they end. | ||
| Line 5,493: | Line 6,549: | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::We say: 9 by 5 are 45. We multiply it by 4; the result is 180. We multiply it by 6; the result is 1080. We multiply it by 8; the result is 8640. We multiply it by 7; the result is 60480. |
| − | |style= | + | |style="text-align:right;"|ונאמ' ט' בה' יעלו מ"ה נכפלם בד' יעלו [180 נכפלם בו' יעלו 1080 נכפלם בח' יעלו‫]‫<ref>marg.</ref> 8640 נכפלם בז' יעלו 60480 |
| + | |} | ||
| + | ::<math>\scriptstyle{\color{blue}{9\sdot5\sdot4\sdot6\sdot8\sdot7=45\sdot4\sdot6\sdot8\sdot7=180\sdot6\sdot8\sdot7=1080\sdot8\sdot7=8640\sdot7=60480}}</math> | ||
| + | {| | ||
| + | |- | ||
| + | |<span style=color:Green>Dividing the common denominator by the summed numerator</span> | ||
| + | | | ||
| | | | ||
|- | |- | ||
| | | | ||
| − | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a\sdot n}{a}=n\longrightarrow\frac{a}{a\sdot n}=\frac{1}{n}}}</math> | |
| − | + | | | |
|- | |- | ||
| − | |||
| | | | ||
| + | ::Therefore, we [divide] the common denominator by the numerator, i.e. we divide 6[0]480 by 13352 and if it is divided into integers without addition or subtraction, the reduced result of division is the denominator of all the required fractions together, i.e. one quarter or similar to it. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{6{\color{red}{0}}480}{13352}}}</math> | ||
| + | |style="width:45%; text-align:right;"|ולזאת קרינו {{#annot:term|1163,1239|A7R5}}אם המורים{{#annotend:A7R5}} למספר השברים ר"ל שנחלק ה68480 ל13352 ואם {{#annot:term|2187,1225|Ze9g}}יתחלק כלו לשלימים{{#annotend:Ze9g}} בלי תוספת ‫<ref>49v</ref>ומגרעת הנה היוצא בחילוק {{#annot:term|1555,2509|86e8}}בצמצום{{#annotend:86e8}} הוא {{#annot:term|571,1239|BOwU}}מורה החלק{{#annotend:BOwU}} אשר הם כל השברים הנשאלים יחד מהשלם ר"ל רביעית אחד או הדומ' לו | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|<span style="text-decoration: line-through; text-decoration-color: red;">ואם לא יתחלק כלו לשלמים בלי תוספת ומגרעת הנה היוצא בחלוק בצמצום הוא מורה החלק אשר הם כל השברים הנשאלים יחד מהשלם ר"ל רביעית אחת או הדומה לו</span> | |
| − | |||
| − | |style="text-align:right;"|ואם לא יתחלק כלו לשלמים בלי תוספת ומגרעת הנה היוצא בחלוק | ||
|- | |- | ||
| | | | ||
| − | : | + | :If it is not entirely divided into integers, but there is a remainder: |
| − | :<math>\scriptstyle\frac{\left(a\sdot n\right)+r}{a}=n+\frac{r}{a}\longrightarrow\frac{a}{\left(a\sdot n\right)+r}=\frac{1}{n+1}+\frac{a-r}{\left(n+1\right)\sdot\left[\left(a\sdot n\right)+r\right]}</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{\left(a\sdot n\right)+r}{a}=n+\frac{r}{a}\longrightarrow\frac{a}{\left(a\sdot n\right)+r}=\frac{1}{n+1}+\frac{a-r}{\left(n+1\right)\sdot\left[\left(a\sdot n\right)+r\right]}}}</math> |
|style="text-align:right;"|ואם לא {{#annot:term|2187,1225|OK90}}יתחלק כלו לשלמים{{#annotend:OK90}} וישאר שום מספר | |style="text-align:right;"|ואם לא {{#annot:term|2187,1225|OK90}}יתחלק כלו לשלמים{{#annotend:OK90}} וישאר שום מספר | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{\scriptstyle\frac{ | + | :As in our example, in which the result of division is 4 and the remainder is 7072. |
| + | :<math>\scriptstyle{\color{blue}{\scriptstyle\frac{60480}{13352}=4+\frac{7072}{13352}}}</math> | ||
|style="text-align:right;"|כמשלינו זה שיצא בחילוק ד' ונשאר 7072 | |style="text-align:right;"|כמשלינו זה שיצא בחילוק ד' ונשאר 7072 | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{\begin{align} | + | ::We add 1 to the result of division; it is 5 and this is the denominator of the greatest possible fraction, i.e. one-fifth. |
| − | | | + | |style="text-align:right;"|נוסיף א' על היוצא בחילוק ויהיה ה' והוא מורה החלק הגדול שאפשר ר"ל חמשית אחת |
| − | + | |- | |
| − | + | | | |
| + | ::We also subtract the remaining 7072 from the 13352 by which we divide; the remainder is 6280, which are parts of all the denominators of this fraction, i.e. one-fifth. | ||
| + | |style="text-align:right;"|עוד נחסר ה7072 הנשארים מה13352 אשר חלקנו עליו וישאר 6280 שהוא חלקים מכל המורים מזה החלק ר"ל מחמישית אחת | ||
| + | |- | ||
| + | | | ||
| + | ::I.e. we receive that all the required fractions are one-fifth and 6280-sevenths of an eighth of a sixth of a quarter of a fifth of a ninth of a fifth, like this: | ||
| + | |style="text-align:right;"|ר"ל שיצא לנו שכל השברים הנשאלים הם חמישית אחת ו6280 שביעיות שמינית שישית רביעית חמישית תשיעית חמישית כזה | ||
| + | |} | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left(\frac{2}{5}\sdot\frac{2}{9}\sdot2\right)+\left[\left[\frac{1}{8}+\left(\frac{2}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\right)\right]\sdot\left[\frac{1}{4}+\left(\frac{2}{6}\sdot\frac{1}{4}\right)\right]\right]&\scriptstyle=\left(\frac{13352}{7}\sdot\frac{1}{8}\sdot\frac{1}{6}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{9}\right)=\frac{13352}{60480}=\frac{1}{4+1}+\frac{13352-7072}{5\sdot60480}\\&\scriptstyle=\frac{1}{5}+\left(\frac{6280}{7}\sdot\frac{1}{8}\sdot\frac{1}{6}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{9}\sdot\frac{1}{5}\right)\\\end{align}}}</math> | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| Line 5,533: | Line 6,605: | ||
|} | |} | ||
|- | |- | ||
| − | |The order of the denominators of compound fractions of fractions is unimportant, but the denominator of the simple fraction should be placed separately, on the right | + | |<span style=color:Green>The order of the denominators of compound fractions of fractions is unimportant, but the denominator of the simple fraction should be placed separately, on the right:</span> |
| − | |style="text-align:right;"|[ואם תרצה לעשות לשברים אלו כלילת יופי ר"ל לחלקם על המורים | + | | |
| + | |- | ||
| + | |If you want to reduce these fractions, i.e. to divide them by the denominators, arrange them as they are now, or randomly, or calculatedly as mentioned above, provided that you write the 5 first to the right with the 1 beneath it, because you cannot change this, and all the others are related to it, i.e. all of them are fractions and fractions of fractions of it, i.e. of a fifth of the whole. | ||
| + | |style="width:45%; text-align:right;"|‫[ואם תרצה לעשות לשברים אלו כלילת יופי ר"ל לחלקם על המורים תסדרם‫]‫<ref>marg.</ref> תסדרם כפי שהם עתה או כפי המזדמן או בהשגחה כנזכר למעלה ובלבד שתניח הה' ראשון לצד ימין עם הא' אשר תחתיו כי זה אין בידיך לשנותו וכל האחרים נקשרים בו ר"ל שהם כלם שברים ושברי שברים ממנו ר"ל מחמשית מהשלם | ||
| + | |- | ||
| + | | | ||
| + | ::We divide them first by 8; the result of division is 785 and nothing remains. | ||
| + | |style="text-align:right;"|ונחלקם תחלה לח' ויצא בחלוק 785 ולא ישאר דבר | ||
| + | |- | ||
| + | | | ||
| + | ::We divide this result by 5; the result of division is 157 and nothing remains. | ||
| + | |style="text-align:right;"|ונחלק זה היוצא לה' ויצא בחילוק 157 ולא ישאר דבר | ||
| + | |- | ||
| + | | | ||
| + | ::We divide it by 4; the result of division is 39 and 1 remains. We write it beneath it. | ||
| + | |style="text-align:right;"|ונחלקם לד' ויצא בחילוק ל"ט וישאר א' ונשימנו תחתיו | ||
| + | |- | ||
| + | | | ||
| + | ::We divide it by 6; the result of division is 6 and 3 remains. | ||
| + | |style="text-align:right;"|ונחלקם ל<s>ט'</s>[ו']‫<ref>marg.</ref> ויצא בחילוק ו' וישארו ג‫' | ||
| + | |- | ||
| + | | | ||
| + | :We factorize the 9, i.e. we convert it to two denominators that are 3 and 3, since a third of a third is as a ninth, and I shall discuss this further in the last chapter with God's help. | ||
| + | |style="text-align:right;"|ונתיך הט' ר"ל שנעשה ממנו <sup>ב'</sup> מורים שהם ‫<ref>50r</ref>ג' ג' כי כך הוא שלישית <sup>שלישית</sup> כמו תשיעית ועוד אדבר בזה בכלל האחרון ב"ה י"ת | ||
| + | |- | ||
| + | | | ||
| + | ::We divide the 6 resulting in the last division by one of them, i.e. by the 3; the result of division is 2 and nothing remains. | ||
| + | |style="text-align:right;"|ונחלק הו' אשר יצאו בחלוק באחרונה על האחד מהם ר"ל על הג' ויצא בחילוק ב' ולא ישאר דבר | ||
| + | |- | ||
| + | | | ||
| + | ::Since this 2 is smaller than the rest of the denominators, we should not divide it further, only to write it beneath the next denominator that we write before them, which is the second 3, so that it would not be forgotten. | ||
| + | |style="text-align:right;"|ואלו הב' אחר שהוא מספר קטן משאר המורים אין לנו עוד לחלקם רק להשימם תחת המורה הסמוך אשר נשים לפניהם ויהיו הג' השני כדי שלא ישכח ונשימם תחתיו | ||
| + | |- | ||
| + | | | ||
| + | ::We write also the 6, which is the remaining denominator, before them and before it the first 5. We write the 1 beneath it, which is the denominator of the greatest possible fraction that we sought. | ||
| + | |style="text-align:right;"|ונסדר עוד <s>הט</s> [הו']‫<ref>marg.</ref> המורה הנשאר לפניהם ולפניו הה' ראשונה ונשים תחתיו הא' אשר היה תחתיו שהוא המורה היותר חלק גדול הגדול שאיפשר אשר בקשנו | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{\begin{align} | + | :We receive that the required fractions are one-fifth, 2-thirds of a seventh of a fifth, 3-sixths of a third of a third of a seventh of a fifth, and one-quarter of a sixth of a third of a third of a seventh of a fifth, like this: |
| − | | | + | |style="text-align:right;"|הנה יצא לנו שהשברים הנשאלים יעלו חמשית א' שלמה וב' שלישיות שביעית חמישית וג' ששיות שלישית שלישית שביעית חמישית ורביעית שישית שלישית שלישית שביעית חמישית כזה |
| − | + | |} | |
| − | + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left(\frac{2}{5}\sdot\frac{2}{9}\sdot2\right)+\left[\left[\frac{1}{8}+\left(\frac{2}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\right)\right]\sdot\left[\frac{1}{4}+\left(\frac{2}{6}\sdot\frac{1}{4}\right)\right]\right]&\scriptstyle=\frac{1}{5}+\left(\frac{6280}{7}\sdot\frac{1}{8}\sdot\frac{1}{6}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{9}\sdot\frac{1}{5}\right)=\frac{1}{5}+\left(\frac{6280}{8}\sdot\frac{1}{5}\sdot\frac{1}{4}\sdot\frac{1}{6}\sdot\frac{1}{9}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)\\&\scriptstyle=\frac{1}{5}+\left(\frac{785}{5}\sdot\frac{1}{4}\sdot\frac{1}{6}\sdot\frac{1}{9}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)=\frac{1}{5}+\left(\frac{157}{4}\sdot\frac{1}{6}\sdot\frac{1}{9}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)\\&\scriptstyle=\frac{1}{5}+\left(\frac{39}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)+\left(\frac{1}{4}\sdot\frac{1}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)\\&\scriptstyle=\frac{1}{5}+\left(\frac{6}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)+\left(\frac{3}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)+\left(\frac{1}{4}\sdot\frac{1}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)\\&\scriptstyle=\frac{1}{5}+\left(\frac{2}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)+\left(\frac{3}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)+\left(\frac{1}{4}\sdot\frac{1}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)\\\end{align}}}</math> | |
| − | + | {| | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| Line 5,557: | Line 6,659: | ||
|} | |} | ||
|- | |- | ||
| − | | | + | |Deduce from that |
|style="text-align:right;"|והקש על זה | |style="text-align:right;"|והקש על זה | ||
|- | |- | ||
| − | | | + | | |
| − | + | ||
| − | | | + | === <span style=color:Green>Reason</span> === |
| + | | | ||
|- | |- | ||
| − | |The portions of the denominators | + | |The reason we say that if there is no remainder, the result of the division is itself the denominator of the fractions of the whole, is because our saying "these portions of the denominators" is as our saying "the portions of their common denominator in the whole". |
| − | + | |style="width:45%; text-align:right;"|<big>וטעם</big> אומרנו שאם לא ישאר דבר שהיוצא בחילוק בעצמו הוא מורה החלק אשר השברים מהשלם הוא לפי שאמרנו אלו החלקים מאלו המורים הוא כאלו אמרנו כ"כ מחלקי אם המורים בשלם | |
| − | |style="text-align:right;"|הוא לפי שאמרנו אלו החלקים מאלו המורים הוא כאלו אמרנו כ"כ מחלקי אם המורים בשלם | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle\frac{2}{3}\sdot\frac{1}{4}</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a\sdot n}{a}=n\longrightarrow\frac{a}{a\sdot n}=\frac{1}{n}}}</math> |
| + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}\sdot\frac{c}{d}=\frac{a\sdot c}{b\sdot d}}}</math> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *I.e., for instance, if we have 2-thirds of a quarter: | ||
| + | :<math>\scriptstyle\frac{2}{3}\sdot\frac{1}{4}</math> | ||
|style="text-align:right;"|ר"ל כי ע'ד'מ' אם היו לנו ב' שלישיות רביעית | |style="text-align:right;"|ר"ל כי ע'ד'מ' אם היו לנו ב' שלישיות רביעית | ||
|- | |- | ||
| | | | ||
| + | :It is as our saying "two parts of 12 in the whole", which is the common denominator of these denominators, i.e. it consists of them, since the product of 3 by 4 is 12. | ||
:<math>\scriptstyle{\color{blue}{\frac{2}{3}\sdot\frac{1}{4}=\frac{2}{3\sdot4}=\frac{2}{12}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{2}{3}\sdot\frac{1}{4}=\frac{2}{3\sdot4}=\frac{2}{12}}}</math> | ||
|style="text-align:right;"|הוא כאומרנו שני חלקים מי"ב בשלם שהיא אם אלו המורים ר"ל שהוא מורכב מהם שכפל ג' בד' עולה י"ב | |style="text-align:right;"|הוא כאומרנו שני חלקים מי"ב בשלם שהיא אם אלו המורים ר"ל שהוא מורכב מהם שכפל ג' בד' עולה י"ב | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle\frac{3}{4}\sdot\frac{1}{2}\sdot\frac{1}{3}</math> | + | *Similarly, our saying "3-quarters of a half of a third". |
| + | :<math>\scriptstyle\frac{3}{4}\sdot\frac{1}{2}\sdot\frac{1}{3}</math> | ||
|style="text-align:right;"|וכן אומרנו ג' רביעיות חצי שלישית | |style="text-align:right;"|וכן אומרנו ג' רביעיות חצי שלישית | ||
|- | |- | ||
| | | | ||
| + | :It is as our saying "3 parts of 24 in the whole", which is the common denominator of these three denominators and this is clear. | ||
:<math>\scriptstyle{\color{blue}{\frac{3}{4}\sdot\frac{1}{2}\sdot\frac{1}{3}=\frac{3}{24}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{3}{4}\sdot\frac{1}{2}\sdot\frac{1}{3}=\frac{3}{24}}}</math> | ||
|style="text-align:right;"|היא כאומרנו ג' חלקים מכ"ד בשלם שהוא אם שלש מורים אלו וזה ברור | |style="text-align:right;"|היא כאומרנו ג' חלקים מכ"ד בשלם שהוא אם שלש מורים אלו וזה ברור | ||
|- | |- | ||
| − | | | + | |It clear from chapter four of the first section. |
| − | |style="text-align:right;"|ועוד נחבר בפ' הרביעי מהחלק הא' הנה ידענו שאלו השברים הם חלקים מחלקי האם בשלם | + | |style="text-align:right;"|ועוד נחבר בפ' הרביעי מהחלק הא‫' |
| + | |- | ||
| + | | | ||
| + | :We know that these numerators are portions of their common denominator in one whole. | ||
| + | |style="text-align:right;"|הנה ידענו שאלו השברים הם חלקים מחלקי האם בשלם | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle{\color{blue}{\frac{\frac{1}{3}\sdot a}{a}=\frac{1}{3}\sdot1}}</math> | + | :*If they are its third, they are a third of the whole. |
| − | |style="text-align:right;"|ואם הם היה שלישיתם הם שלישית השלם | + | ::<math>\scriptstyle{\color{blue}{\frac{\frac{1}{3}\sdot a}{a}=\frac{1}{3}\sdot1}}</math> |
| + | |style="text-align:right;"|ואם <sup>הם</sup> <s>היה</s> שלישיתם הם שלישית השלם | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle{\color{blue}{\frac{\frac{1}{4}\sdot a}{a}=\frac{1}{4}\sdot1}}</math> | + | :*If its quarter, [they are] a quarter [of the whole]. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{\frac{1}{4}\sdot a}{a}=\frac{1}{4}\sdot1}}</math> | ||
|style="text-align:right;"|ואם רביעיתם רביעית | |style="text-align:right;"|ואם רביעיתם רביעית | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle{\color{blue}{\frac{1\sdot a}{a}=1\sdot1}}</math> | + | :*If the same as [the common denominator], they are one integer. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{1\sdot a}{a}=1\sdot1}}</math> | ||
|style="text-align:right;"|ואם כמותם הם א' שלם | |style="text-align:right;"|ואם כמותם הם א' שלם | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle{\color{blue}{\frac{\frac{1}{5}\sdot a}{a}=\frac{1}{5}\sdot1\longrightarrow\frac{a}{\frac{1}{5}\sdot a}=5}}</math> | + | *For instance, if the numerator is fifth of the common denominator, i.e. fifth of the whole, when we divide the common denominator by it, the result of division is 5 and nothing remains. |
| − | |style="text-align:right;"|וע'ד'מ' אם מספר השברים היה חמישית האם ר"ל חמישית השלם בחלקנו האם עליהם היה היוצא בחלוק ה' ולא היה נשאר דבר | + | :<math>\scriptstyle{\color{blue}{\frac{\frac{1}{5}\sdot a}{a}=\frac{1}{5}\sdot1\longrightarrow\frac{a}{\frac{1}{5}\sdot a}=5}}</math> |
| + | |style="text-align:right;"|<s>וע</s> וע'ד'מ' אם מספר השברים היה חמישית האם ר"ל חמישית השלם בחלקנו האם עליהם ‫<ref>50v</ref>היה היוצא בחלוק ה' ולא היה נשאר דבר | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle{\color{blue}{\frac{\frac{1}{4}\sdot a}{a}=\frac{1}{4}\sdot1\longrightarrow\frac{a}{\frac{1}{4}\sdot a}=4}}</math> | + | *If it is its quarter, the result is 4. |
| + | :<math>\scriptstyle{\color{blue}{\frac{\frac{1}{4}\sdot a}{a}=\frac{1}{4}\sdot1\longrightarrow\frac{a}{\frac{1}{4}\sdot a}=4}}</math> | ||
|style="text-align:right;"|ואם היה רביעית יצאו ד‫' | |style="text-align:right;"|ואם היה רביעית יצאו ד‫' | ||
|- | |- | ||
| − | | | + | |We receive that the result of division indicates the portion that the numerator is of the whole and the reason for this is clear, when all is divided with no remainder. |
|style="text-align:right;"|הרי לנו שהיוצא בחילוק הוא {{#annot:term|571,1239|TAZT}}המורה החלק{{#annotend:TAZT}} אשר השברים מהשלם וזה ברור בטעם כאשר נתחלק הכל ולא נשאר דבר | |style="text-align:right;"|הרי לנו שהיוצא בחילוק הוא {{#annot:term|571,1239|TAZT}}המורה החלק{{#annotend:TAZT}} אשר השברים מהשלם וזה ברור בטעם כאשר נתחלק הכל ולא נשאר דבר | ||
|- | |- | ||
| − | | | + | |To clarify the reason of our saying that when there is a remainder, we add one to the result etc. I shall bring another example: |
| − | :<math>\scriptstyle\frac{\left(a\sdot n\right)+r}{a}=n+\frac{r}{a}\longrightarrow\frac{a}{\left(a\sdot n\right)+r}=\frac{1}{n+1}+\frac{a-r}{\left(n+1\right)\sdot\left[\left(a\sdot n\right)+r\right]}</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{\left(a\sdot n\right)+r}{a}=n+\frac{r}{a}\longrightarrow\frac{a}{\left(a\sdot n\right)+r}=\frac{1}{n+1}+\frac{a-r}{\left(n+1\right)\sdot\left[\left(a\sdot n\right)+r\right]}}}</math> |
| − | |style="text-align:right;"|ולברר טעם אומרנו שכאשר נשאר שם דבר שנוסיף א' על | + | |style="text-align:right;"|<big>ולברר</big> טעם אומרנו שכאשר נשאר שם דבר שנוסיף א' על היוצא<s>ות</s> וכו' אביא משל אח‫' |
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle\frac{3}{4}\sdot\frac{1}{7}</math> | + | *Example: we have 3-quarters of a seventh, i.e. three parts of 28, which is the common denominator of the whole. |
| − | |style="text-align:right;"|המשל היו בידינו ג' רביעיות שביעית | + | :<math>\scriptstyle\frac{3}{4}\sdot\frac{1}{7}</math> |
| + | :<math>\scriptstyle{\color{blue}{\frac{3}{4}\sdot\frac{1}{7}=\frac{3}{28}}}</math> | ||
| + | |style="text-align:right;"|<big>המשל</big> היו בידינו ג' רביעיות שביעית ר"ל שלשה חלקים מכ"ח שהוא אם המורים בשלם | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{\frac{3}{ | + | :If we divide 28 by 3 the result of division is 9 and 1 remains. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\frac{28}{3}=9+\frac{1}{3}\longrightarrow\frac{3}{28}=\frac{1}{9+1}+{\color{red}{\frac{3-1}{\left(9+1\right)\sdot28}}}=\frac{1}{10}+{\color{red}{\frac{3-1}{10\sdot28}}}}}</math> |
| + | |style="text-align:right;"|ואם נחלק אלו הכ"ח אל הג' יצאו ט' בחילוק וישאר א‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :We add 1 to the 9 resulting from the division; the result is ten, which indicates the tenth. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נוסיף א' על הט' היוצא בחילוק יעלה עשרה המורה על העשירית |
| − | נוסיף א' על הט' היוצא בחילוק יעלה עשרה המורה על העשירית | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{\frac{30}{3}=10\longrightarrow\frac{3}{30}=\frac{1}{10}}}</math> | + | ::If the original fraction was 3 parts of 30, it was one-tenth by reduction, because when we divide 30 by three, the result is [10] and nothing remains, so it was a whole tenth, as we explained. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{30}{3}=10\longrightarrow\frac{3}{30}=\frac{1}{10}}}</math> | ||
|style="text-align:right;"|ואם החלקים הראשונים היו ג' חלקים מל' באחד היו עשירית אחד {{#annot:term|1555,2509|suVP}}בצמצום{{#annotend:suVP}} כי בחלקנו הל' בשלשה היו יוצאים ולא היה נשאר דבר ואז היו עשירית שלמה כמו שביארנו | |style="text-align:right;"|ואם החלקים הראשונים היו ג' חלקים מל' באחד היו עשירית אחד {{#annot:term|1555,2509|suVP}}בצמצום{{#annotend:suVP}} כי בחלקנו הל' בשלשה היו יוצאים ולא היה נשאר דבר ואז היו עשירית שלמה כמו שביארנו | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Since it is 3 parts of 28, it is greater than one-tenth. To know by how much it is greater, we multiply 28 by 30; the result is 840. |
| − | |style="text-align:right;"|אכן להיותם ג' חלקים מכ"ח בשלם יותר מעשירית אחת ולדעת כמה הם יותר נכפול הכ"ח בל' ויעלו 840 | + | |style="text-align:right;"|אכן להיותם ג' חלקים מכ"ח בשלם יותר מעשירית אחת ולדעת כמה הם יותר נכפול הכ"ח בל' ויעלו 840 |
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{\frac{30}{840}=\frac{1}{28}}}</math> | + | ::Our saying: "one part of 840 in the whole" is as our saying: "one part of 30 of 28 in the whole", or "one part of 28 of 30 in the whole", because they are the divisors, of which it is composed and all this is well explained in chapter 4 of the first section. |
| + | ::<math>\scriptstyle{\color{blue}{28\sdot30=840\longrightarrow\frac{1}{840}=\frac{1}{30}\sdot\frac{1}{28}=\frac{1}{28}\sdot\frac{1}{30}}}</math> | ||
| + | |style="text-align:right;"|והנה אומרנו חלק אחד מ840 בשלם הוא כאומרנו חלק אחד מל' מכ"ח בשלם או חלק אחד מכ"ח מל' בשלם כי הם המורים אשר מהם הורכב וכל זה נתבאר הטב בפרק הד' מהחלק הא‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Therefore, 30 parts of 840 of the whole are one part of 28 of the whole. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{30}{840}=\frac{1}{28}}}</math> | ||
|style="text-align:right;"|וא"כ הל' חלקים מה840 בשלם הם חלק אחד מכ"ח בשלם | |style="text-align:right;"|וא"כ הל' חלקים מה840 בשלם הם חלק אחד מכ"ח בשלם | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{\frac{28}{840}=\frac{1}{30}}}</math> | + | ::Whereas 28 parts of 840 of the whole are one part of 30 of the whole. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{28}{840}=\frac{1}{30}}}</math> | ||
|style="text-align:right;"|וכן הכ"ח חלקים מ840 בשלם הם חלק אחד מל' בשלם | |style="text-align:right;"|וכן הכ"ח חלקים מ840 בשלם הם חלק אחד מל' בשלם | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{\frac{1}{30}=\frac{28}{840}}}</math> | + | ::So, one part of 30 of the whole is 28 parts of 840 of the whole. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{30}=\frac{28}{840}}}</math> | ||
|style="text-align:right;"|הרי לנו שהחלק אחד מל' בשלם הוא כ"ח חלקים מ840 בשלם | |style="text-align:right;"|הרי לנו שהחלק אחד מל' בשלם הוא כ"ח חלקים מ840 בשלם | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{\frac{1}{28}=\frac{30}{840}}}</math> | + | ::And one part of 28 of the whole is 30 parts of 840. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{28}=\frac{30}{840}}}</math> | ||
|style="text-align:right;"|וכן החלק מכ"ח בשלם הוא ל' חלקים מ840 | |style="text-align:right;"|וכן החלק מכ"ח בשלם הוא ל' חלקים מ840 | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{\frac{3}{28}=\frac{3\sdot30}{840}=\frac{90}{840}}}</math> | + | ::We find that 3 parts of 28 of the whole is 3 times 30, which is 90 parts of 840 of the whole. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{3}{28}=\frac{3\sdot30}{840}=\frac{90}{840}}}</math> | ||
|style="text-align:right;"|נמצא שהג' חלקי' מכ"ח בשלם הוא ג' פעמים ל' שהם 90 חלקים מ840 בשלם | |style="text-align:right;"|נמצא שהג' חלקי' מכ"ח בשלם הוא ג' פעמים ל' שהם 90 חלקים מ840 בשלם | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{\frac{3}{30}=\frac{3\sdot28}{840}=\frac{84}{840}}}</math> | + | ::And 3 parts of 30 of the whole is 3 times 28, which is 84 parts of 840. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{3}{30}=\frac{3\sdot28}{840}=\frac{84}{840}}}</math> | ||
|style="text-align:right;"|והג' חלקים מל' בשלם הם ג' פעמים הם כ"ח שהם פ"ד חלקים מ840 | |style="text-align:right;"|והג' חלקים מל' בשלם הם ג' פעמים הם כ"ח שהם פ"ד חלקים מ840 | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{\frac{3}{28}-\frac{3}{30}=\frac{90}{840}-\frac{84}{840}=\frac{6}{840}=\frac{6}{30\sdot28}}}</math> | + | ::So, they exceed over them by 6 parts of 860 of the whole, i.e. 6 parts of 30 of 28 of the whole, because they are its divisors. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{3}{28}-\frac{3}{30}=\frac{90}{840}-\frac{84}{840}=\frac{6}{840}=\frac{6}{30\sdot28}}}</math> | ||
|style="text-align:right;"|הנה יעדפו עליהם ו' חלקים מ840 בשלם ר"ל ו' חלקים מל' מכ"ח בשלם כי הם מוריו | |style="text-align:right;"|הנה יעדפו עליהם ו' חלקים מ840 בשלם ר"ל ו' חלקים מל' מכ"ח בשלם כי הם מוריו | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Every 3 parts of these are a tenth of 30, which are, i.e. the 30, are one part of 28 of the whole, as explained. |
| − | |style="text-align:right;"|וכל ג' חלקים מאלו הם עשירית הל' שהם ר"ל שהם הל' הם חלקי א' מכ"ח בשלם כמו שנתבאר | + | |style="text-align:right;"|וכל ג' חלקים ‫<ref>51r</ref>מאלו הם עשירית הל' שהם ר"ל <s>שהם</s> הל' הם חלקי א' מכ"ח בשלם כמו שנתבאר |
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{\frac{3}{28}-\frac{3}{30}=\frac{6}{840}=\frac{2}{4}\sdot\frac{1}{7}\sdot\frac{1}{10}}}</math> | + | ::Hence, every three of them are one-tenth of a part of 28 of the whole, i.e. one part of 28 of a tenth of the whole. |
| + | |style="text-align:right;"|א"כ כל שלשה מהם הם עשירית [חלק מכ"ח בשלם ר"ל חלק מכ"ח מעשירית בשלם שהוא]‫<ref>marg.</ref> <s>הל' שהם ר"ל הל' הם חלקי א' מכ"ח בשלם כמו שנתבאר</s> | ||
| + | |- | ||
| + | | | ||
| + | ::Which is a quarter of a seventh of a tenth of the whole. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{3}{840}=\frac{1}{10}\sdot\frac{30}{840}=\frac{1}{10}\sdot\frac{1}{28}=\frac{1}{28}\sdot\frac{1}{10}=\frac{1}{4}\sdot\frac{1}{7}\sdot\frac{1}{10}}}</math> | ||
| + | |style="text-align:right;"|<s>א"כ כל שלשה מהם הם עשירית הל' חלק מכ"ח בשלם ר"ל חלק מכ"ח מעשירית בשלם</s> שהוא רביעית שביעית עשירית מהשלם | ||
| + | |- | ||
| + | | | ||
| + | ::The additional six, by which we find that the 3 parts of 28 that we have exceed over the 3 parts of 30, which are a whole tenth, are therefore 2-quarters of a seventh of a tenth. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{3}{28}-\frac{3}{30}=\frac{6}{840}=\frac{2}{4}\sdot\frac{1}{7}\sdot\frac{1}{10}}}</math> | ||
|style="text-align:right;"|והששה הנוספות אשר מצאנו לג' חלקים מכ"ח אשר היו בידינו על הג' חלקים מל' אשר מצאנו לג' חלקים היו עשירית שלמה יעלו א"כ ב' רביעיות שביעית עשירית | |style="text-align:right;"|והששה הנוספות אשר מצאנו לג' חלקים מכ"ח אשר היו בידינו על הג' חלקים מל' אשר מצאנו לג' חלקים היו עשירית שלמה יעלו א"כ ב' רביעיות שביעית עשירית | ||
|- | |- | ||
| | | | ||
| − | : | + | :We receive that when we divide 28, which is the common denominator, by 3, which are the numerator, so that the result is 9 and 1 remains, that when we add one to the 9, so that the result is 10, which indicates a tenth, we are left with an excess of 2-quarters of a seventh of a tenth, which is the excess that the number, by which we divide, which is 3, exceeds over the remainder, which is 1. I.e. these 2 are parts of the denominators that are a quarter of a [seventh] of the denominator that was generated, which is a tenth. |
| − | |style="text-align:right;"|הרי לנו שכאשר חלקנו הכ"ח שהוא האם על הג' שהיו מספר החלקים ויצא ט' ונשאר א' שכאשר הוספנו אחד על הט' ועלה י' והורה עשירית שנשאר לנו לתוספת ב' רביעיות שביעית עשירית שהם התוספת אשר למספר אשר חלקנו עליו שהיה ג' על השארית שהיה א' ר"ל שאלו הב' הם חלקים מהמורים שהיו רביעית שמינית מהמורה שנתחדש שהוא עשירית | + | |style="text-align:right;"|הרי לנו שכאשר חלקנו הכ"ח שהוא האם על הג' שהיו מספר החלקים ויצא ט' ונשאר א' שכאשר הוספנו אחד על הט' ועלה י' והורה עשירית שנשאר לנו לתוספת ב' רביעיות שביעית עשירית שהם התוספת אשר למספר אשר חלקנו עליו שהיה ג' על השארית שהיה א' ר"ל שאלו הב' הם חלקים מהמורים שהיו רביעית שמינית מהמורה שנתחדש שהוא עשירית |
|- | |- | ||
| − | | | + | |All this is clear by reason to the one who understands and deduce from this. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכל זה ברור בטעם למבין והקש על זה |
| + | |} | ||
| + | :<math>\scriptstyle{\color{blue}{\scriptstyle\frac{28}{3}=9+\frac{1}{3}\longrightarrow\frac{3}{28}=\frac{1}{9+1}+\frac{3-1}{\left(9+1\right)\sdot28}=\frac{1}{10}+\frac{2}{10\sdot28}=\frac{1}{10}+\left(\frac{3}{28}-\frac{3}{30}\right)=\frac{1}{10}+\left(\frac{2}{4}\sdot\frac{1}{7}\sdot\frac{1}{10}\right)}}</math> | ||
| + | |||
| + | {| | ||
|- | |- | ||
| − | | | + | |<span style=color:Green>Dividing a small number by a greater number - without divisors or with divisors</span> |
| + | | | ||
| + | |- | ||
| + | |We receive that the one who wants to divide a smaller by a greater can divide it with or without extraction of the divisors. | ||
| + | |style="width:45%; text-align:right;"|<big>ויצא</big> לנו מזה שהרוצה לחלק מעט על רב שיוכל לחלקו בלי הוצאת המורים או בהוצאת המורים | ||
| + | |- | ||
| + | |We also get the greatest part that can be pronounced by a single name. | ||
| + | |style="text-align:right;"|ויצאו לנו ג"כ החלק היותר גדול שאיפשר בשם אחד | ||
| + | |- | ||
| + | |This is very helpful when we want to divide a prime number, such as 101, that has no divisors. | ||
|style="text-align:right;"|וזה יועיל מאד כאשר אנו רוצים לחלק למספר פשוט כמו ק"א או כדומה לו שאין לו מורים | |style="text-align:right;"|וזה יועיל מאד כאשר אנו רוצים לחלק למספר פשוט כמו ק"א או כדומה לו שאין לו מורים | ||
| + | |- | ||
| + | |In order to explain this matter well, I shall bring two examples - one with extraction of divisors and one without extraction of divisors: | ||
| + | |style="text-align:right;"|וכדי לבאר הענין יפה יפה אביא שני משלים אחד עם הוצאת המורים ואחד מבלי הוצאת המורים | ||
|- | |- | ||
| | | | ||
| − | : | + | *{{#annot:73÷240|157|Fs1g}}Example: we wish to divide 73 by 240. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle73\div240</math> |
| + | |style="text-align:right;"|<big>המשל</big> רצינו לחלק 73 על 240{{#annotend:Fs1g}} | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Its divisors are 6, 8, and 5, because they are the divisors, of which it is composed, and it is the common denominator. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{240=6\sdot8\sdot5}}</math> |
| + | |style="text-align:right;"|והנה מוריו הם אלו ו' ח' ה' כי מהם <s>מורים</s> הוא מורכב והוא האם | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{240= | + | ::We divide the common denominator, which is the greater number, by which we want to divide, by the smaller number, i.e. 73, which is the number that we want to divide; the result of division is 3 and 21 remains. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{240}{73}=3+\frac{21}{73}}}</math> |
| + | |style="text-align:right;"|ונחלק האם שהוא המספר הגדול אשר רצינו לחלק עליו על המספר הקטן <span style="text-decoration: line-through; text-decoration-color: red;">ר"ל ה73 אשר הוא המספר אשר רצינו לחלק עליו על המספר</span> ‫<ref>51v</ref><span style="text-decoration: line-through; text-decoration-color: red;">הקטן</span> ר"ל ה73 אשר הוא המספר אשר רצינו לחלק ויצא בחילוק ג' וישארו כ"א | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We add 1 to the result, it is 4 and this is the denominator of the greatest fraction, which is one-quarter. We write it first and we write 1 beneath it. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נוסיף א' על היוצא יהיה ד' והוא המורה החלק גדול והוא רביעית אחת ונשימנו ראשונה ונשים תחתיו א‫' |
|- | |- | ||
| | | | ||
| − | :: | + | ::We also put aside the 21 that remains from the 73, which is the number by which we divide now; 52 remain and it is added to the quarter, i.e. the result from dividing 73, which is the smaller number, by 240, which is the greater number, is one-quarter and 52 parts of 240 of a quarter. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|עוד נשים הכ"א הנותרים מהע"ג שהוא החשבון אשר חלקנו עליו עתה ישארו נ"ב והם מוסיפים על הרביעית ר"ל שהעולה שיצא לנו בחלוק ה73 המספר הקטן על ה240 שהוא המספר הגדול רביעית אחת <s>רביעית אחת</s> ונ"ב חלקים מ240 מרביעית |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | ::Or, if you wish, take the divisors instead and say: one-quarter and 52-fifths of an eighth of a sixth of a quarter. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|או אם תרצה תקח מורה במקומו ותאמר רביעית אחת ונ"ב חמישיות שמינית שישית רביעית |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ושם אאריך שאנו יכולים לעשות מעשינו זה פעם אחר פעם עד כלות המספר והגיעו לחלק אחד | + | ::If you wish, you can reduce them; the result is one-quarter, one-fifth of a quarter, and 4-eighths of a sixth of a fifth of a quarter. Deduce from that. |
| + | |style="text-align:right;"|ואם תרצה תעשה להם כלילת יופי ויעלו רביעית אחת וחמישית רביעית וד' שמיניות שישית חמישית רביעית והקש על זה | ||
| + | |} | ||
| + | ::<math>\scriptstyle{\color{blue}{73\div240=\frac{1}{3+1}+\frac{73-21}{\left(3+1\right)\sdot240}=\frac{1}{4}+\frac{52}{240}\sdot\frac{1}{4}=\frac{1}{4}+\left(\frac{52}{5}\sdot\frac{1}{8}\sdot\frac{1}{6}\sdot\frac{1}{4}\right)=\frac{1}{4}+\left(\frac{1}{5}\sdot\frac{1}{4}\right)+\left(\frac{4}{8}\sdot\frac{1}{6}\sdot\frac{1}{5}\sdot\frac{1}{4}\right)}}</math> | ||
| + | {| | ||
| + | |- | ||
| + | |I shall bring another example, in which there are no divisors. | ||
| + | |style="width:45%; text-align:right;"|<big>ועוד</big> אעשה משל אחר <sup>מ</sup>אשר אין לו מורים כלל | ||
| + | |- | ||
| + | |I will illustrate there that we can apply our procedure repeatedly time after time, until the number ends and until reaching to a simple fraction [whose numerator is 1]. | ||
| + | |style="text-align:right;"|ושם אאריך שאנו יכולים לעשות מעשינו זה פעם אחר פעם עד כלות המספר והגיעו לחלק אחד | ||
| + | |- | ||
| + | |I call it also '''Aḥdut''' [= unification], as it brings all to one. | ||
| + | |style="text-align:right;"|כי גם לזה קראתיו אחדות כי יגיעם כלם לאחד | ||
| + | |- | ||
| + | |Even between all the denominators and the last denominator we can insert a new denominator as we wish. | ||
| + | |style="text-align:right;"|ואפי' בין כל המורים למורה האחרון נוכל להכניס מורה חדש ככל חפצנו | ||
|- | |- | ||
| | | | ||
| − | : | + | *{{#annot:38÷101|157|h3tR}}Example: to divide 38 by 101, because this number, i.e. 101 [is prime]. |
| − | |style="text-align:right;"|המשל לחלק ל"ח לק"א כי זה המספר ר"ל ק"א | + | :<math>\scriptstyle38\div101</math> |
| + | |style="text-align:right;"|<big>המשל</big> לחלק ל"ח לק"א כי זה המספר ר"ל ק"א{{#annotend:h3tR}} | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{\frac{101}{38}=2+\frac{25}{38}}}</math> | + | ::We divide 101 by 38; the result of division is 2 and 25 remains. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{101}{38}=2+\frac{25}{38}}}</math> | ||
|style="text-align:right;"|ונחלק הק"א לל"ח [ויצאו בחלוק ב' וישארו כ"ה | |style="text-align:right;"|ונחלק הק"א לל"ח [ויצאו בחלוק ב' וישארו כ"ה | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::We add 1 to the 2; it is 3. We write it as the first denominator and we write 1 beneath it. |
| − | |style="text-align:right;"|נוסיף א' על הב' יהיו ג' ונשימהו למורה ראשון ונשים תחתיו א' | + | ::<math>\scriptstyle{\color{blue}{38\div101=\frac{1}{2+1}+\frac{38-25}{\left(2+1\right)\sdot101}=\frac{1}{3}+\left(\frac{13}{101}\sdot\frac{1}{3}\right)}}</math> |
| − | + | |style="text-align:right;"|נוסיף א' על הב' יהיו ג' ונשימהו למורה ראשון ונשים תחתיו א‫' | |
|- | |- | ||
| | | | ||
| − | :: | + | ::We subtract the remainder from 38, by which we divide now; 13 remains. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונגרע השארית מהל"ח אשר‫]‫<ref>marg.</ref> אשר חלקנו עליו עתה וישארו י"ג |
|- | |- | ||
| | | | ||
| − | :: | + | ::If it were not that much, we would write the 101 as a second denominator and write this remainder, i.e. the 13, beneath it. Then, we would say that when divising 38 by 101, each gets one-third and 13 parts of 101 of a whole third. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם לא היו כ"כ הינו שמים למורה שני הק"א והינו שמים זה השארית ר"ל אלו הי"ג תחתיו והינו אומרים שהמחלק ל"ח על ק"א שיגיע לכל אחד מהם שלישית אחת וי"ג חלקים מק"א משלישית שלמה |
| − | |||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{\frac{101}{ | + | ::Since it is that much and in order to find more proper fractions, we divide again the 101 by 13; the result of division is 7 and 10 remains. |
| − | |style="text-align:right;"|אכן | + | ::<math>\scriptstyle{\color{blue}{\frac{101}{13}=7+\frac{10}{13}}}</math> |
| + | |style="text-align:right;"|אכן להיותם הרבה וכדי שנמצא חלקים יותר נאותות נשוב לחלק הק"א לאלו הי"ג ויצא בחילוק ז' וישאר י‫' | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We write the 7 plus one, which is 8, as a second denominator, and we write 1 beneath it. We put aside the 10, which is the remainder from the 13, by which we divide now; 3 remains. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונשים זה הז' בתוספת אחד והוא ח' למורה שני ונשים תחתיו <sup>א'</sup> ונשים הי' שהם השארית ‫<ref>52r</ref>מהי"ג אשר חלקנו עליהם עתה וישארו ג‫' |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :If you wish, you have already finished the whole procedure. Write the 101 as a third denominator and write the 3, which is the remainder, beneath it. |
| + | |style="text-align:right;"|ואם תרצה כבר כלית <sup>כל</sup> מלאכתך ותשים הק"א למורה שלישי <s>ותשים למורה שלישי</s> ותשים אלו הג' תחתיו שהם {{#annot:term|458,2454|Jb3s}}השארית הנשארה{{#annotend:Jb3s}} | ||
| + | |} | ||
| + | ::<math>\scriptstyle{\color{blue}{38\div101=\frac{1}{3}+\frac{13}{101}\sdot\frac{1}{3}=\frac{1}{3}+\frac{1}{3}\sdot\left[\frac{1}{7+1}+\frac{13-10}{\left(7+1\right)\sdot101}\right]=\frac{1}{3}+\frac{1}{3}\sdot\left[\frac{1}{8}+\frac{3}{8\sdot101}\right]=\frac{1}{3}+\left(\frac{1}{3}\sdot\frac{1}{8}\right)+\left(\frac{3}{101}\sdot\frac{1}{3}\sdot\frac{1}{8}\right)}}</math> | ||
| + | {| | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"|כי | + | ::If you want to repeat the procedure in order to reach a complete unification [Aḥdut], i.e. there would not be any numerator other than one, divide the 101 again by the 3; the result of division is 33 and 2 remains. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{101}{3}=33+\frac{2}{3}}}</math> | ||
| + | |style="width:45%; text-align:right;"|אכן אם תרצה עוד להכפל המעשיך יען תגיע לאחדות גמורה ר"ל שלא יהיו שם מנין שברים כי אם אחד אחד תשוב תחלק הק"א על אלו הג' ויצא בחילוק ל"ג וישארו ב‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We add 1 to the 33; it is 34. We write it as a third denominator and we write 1 beneath it. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונוסיף א' על הל"ג ויהיו ל"ד ונשימם למורה שלשי ונשים א' תחתיו |
|- | |- | ||
| | | | ||
| − | : | + | ::We subtract the remaining two from the 3, by which we divide now; 1 remains and we have reached the complete unification and finished our procedure entirely. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<sup>ונחסר</sup> אלו הב' הנשארים מהג' אשר חלקנו עליהם עתה וישאר א' וכבר הגענו לאחדות הגמור וכלינו מלאכתנו מכל וכל |
|- | |- | ||
| | | | ||
| − | :The | + | ::We write 101 as a fourth denominator and write 1 beneath it. |
| − | |style="text-align:right;"|ואם יהיה רב ממנו תעשה מהם כלילת יופי רצוני לומר לחלק השארית הא' ההיא על המורה האחרון והיוצא שלפניו והנשאר תשים תחתיו וכן לעולם עד כלותו וכל זה מבואר ונכפל פעמים רבות | + | |style="text-align:right;"|ונשים ק"א למורה [רביעי] ונשים א' תחתיו |
| + | |} | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle38\div101&\scriptstyle=\frac{1}{3}+\left(\frac{1}{3}\sdot\frac{1}{8}\right)+\left(\frac{3}{101}\sdot\frac{1}{3}\sdot\frac{1}{8}\right)=\frac{1}{3}+\left(\frac{1}{3}\sdot\frac{1}{8}\right)+\left[\frac{1}{3}\sdot\frac{1}{8}\sdot\left[\frac{1}{33+1}+\frac{3-2}{\left(33+1\right)\sdot101}\right]\right]\\&\scriptstyle=\frac{1}{3}+\left(\frac{1}{3}\sdot\frac{1}{8}\right)+\left[\frac{1}{3}\sdot\frac{1}{8}\sdot\left[\frac{1}{34}+\frac{1}{34\sdot101}\right]\right]\\&\scriptstyle=\frac{1}{3}+\left(\frac{1}{3}\sdot\frac{1}{8}\right)+\left(\frac{1}{3}\sdot\frac{1}{8}\sdot\frac{1}{34}\right)+\left(\frac{1}{3}\sdot\frac{1}{8}\sdot\frac{1}{34}\sdot\frac{1}{101}\right)\\\end{align}}}</math> | ||
| + | {| | ||
| + | |- | ||
| + | |You have three forms, all of which are true. You can switch to whichever of them you want. You are allowed to do it differently, when you divide the common denominator, as in the first example. We write all the denominators that are generated, time after time, one after the other, one instead of the other. | ||
| + | |style="width:45%; text-align:right;"|הרי לך שלש צורות שכלם אמיתיות ותוכל להשיב כאשר תרצה מהם וכן היה הרשות בידך לעשות זה פעם אחר [כאשר היתה מחלק אם המורים כבמשל הא' ונשים כל המורים המתחדשים פעם אחר פעם זה‫]‫<ref>marg.</ref> פעם זה אחר זה כ"א תחת כל אחד | ||
| + | |- | ||
| + | |The term '''Aḥdut''' [= unification] is useful for all this, so that you will not forgat to write 1 beneath each generated denominator, then write the common denominator itself, or its divisors instead of it, and 1 beneath the last, if you have reached the complete unification, and if not, write beneath it what remains last, after you have subtracted the remainder from the number, by which you divide at that last time. Write that last rem,ainder beneath the last divisor of the common denominator. | ||
| + | |style="text-align:right;"|כי לכל זה יועיל שם האחדות שלא תשכח מלשים א' תחת כל מורה מתחדש ואחר תשים האם עצמה או מוריה במקומה ותחת האחרון א' אם הגעת לאחדות הגמורה ואם אין תשים תחתיו הנשאר באחרונה אחרי הסירך הנשאר מהמספר אשר אתה מחלק עליו בעת ההיא באחרונה [.]השארית האחרונה ההיא תשים תחת המורה האחרון אשר לאם | ||
| + | |- | ||
| + | |If it is greater than it, reduce them, I mean, divide that remainder by the last denominator and write the remainder beneath it and so on until it is complete. All this is clear and repeated many times. | ||
| + | |style="text-align:right;"|ואם יהיה רב ממנו תעשה מהם כלילת יופי רצוני לומר לחלק השארית <sup>הא'</sup> <s>ההיא</s> על המורה האחרון והיוצא שלפניו והנשאר תשים תחתיו וכן לעולם עד כלותו וכל זה מבואר ונכפל פעמים רבות | ||
|- | |- | ||
| − | | | + | |For every other number that you divide into divisors, if you see that what you wrote beneath the last denominator is a large number and you want to set between the former denominators and the last one to the left a new denominator or denominators, divide the last denominator by what is beneath it, as you have done in the second and third examples. |
| − | |style="text-align:right;"|וגם בכל מספר אחר אשר חלקת הכל למורים אם תראה שאשר שמת תחת המורה האחרון הוא מספר רב ותרצה להמציא בין כל המורים הראשונים זה האחרון אשר לצד שמאל משום מורה מחודש או מורים חלק המורה האחרון על אשר תחתיו | + | |style="text-align:right;"|וגם בכל מספר אחר אשר חלקת הכל למורים אם תראה שאשר שמת תחת המורה האחרון הוא מספר רב ותרצה להמציא בין כל המורים הראשונים זה האחרון אשר לצד שמאל משום מורה מחודש או מורים חלק ‫<ref>52v</ref>המורה האחרון על אשר תחתיו כאשר עשית במה שבין הצורה השנית והשלישית |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::101 was the last denominator in the first example and since you found 13, which is a large number, beneath it, you formed the denominator 8 that you wrote second and it is third in the second example. |
| + | |style="text-align:right;"|שהרי הק"א היה המורה האחרון בצורה הראשונה ולפי שמצאת הי"ג שהם מספר רב תחתיו המצאת המורה הח' ששמת שני והוא שלישי בידך שבא בצורה השנית | ||
|- | |- | ||
| | | | ||
| + | ::Likewise, from the second example to the third, you formed another denominator, which is 31, and you wrote the 101 fourth. | ||
|style="text-align:right;"|וכן עשית פעם אחת מהצורה השנית לשלישית והמצאת מורה אחר והוא הל"א ושמת הק"א רביעי | |style="text-align:right;"|וכן עשית פעם אחת מהצורה השנית לשלישית והמצאת מורה אחר והוא הל"א ושמת הק"א רביעי | ||
|- | |- | ||
| − | | | + | |Provided that you do it only with the last denominator to the left. |
| − | + | |style="text-align:right;"|ובלבד שלא תעשה זה כי אם למורה האחרון אשר לצד שמאל | |
| − | |style="text-align:right;"|ובלבד שלא תעשה זה כי אם למורה האחרון אשר לצד שמאל וכל זה מבורר בטעם הראשון למבין | + | |- |
| + | |All this is clear to the one who understands by the first reason. | ||
| + | |style="text-align:right;"|וכל זה מבורר בטעם הראשון למבין | ||
|- | |- | ||
| − | | | + | |If you want to extract denominators between the middle denominators and the last denominator to the right, you have to extract [the common denominator] of all [the denominators], then this common denominator is divided by the numerators that are beneath those denominators, after they were decomposed, if it is divisible by them. All this is clear by reason. |
| − | + | |style="text-align:right;"|ואם תרצה להוציא המורים בין המורים האמצעיים תצטרך להוציא המורים לכל המורה האחרון אשר לצד שמאל והאם ההיא תחלק למנין השברים אשר היו תחת <s>המספר</s> המורים ההם אחרי עשות להם פריטה אם כבר נתחלק להם המספר וכל זה ברור בטעם | |
| − | |style="text-align:right;"|ואם תרצה להוציא המורים בין המורים האמצעיים תצטרך להוציא המורים לכל המורה האחרון אשר לצד שמאל והאם ההיא תחלק למנין השברים אשר היו תחת המספר המורים ההם אחרי עשות להם פריטה אם כבר נתחלק להם המספר וכל זה ברור בטעם | ||
|- | |- | ||
| − | | | + | |Because, after you have extracted the common denominator of these denominators, they are all become as one denominator and you seek between the formers and the [last] another denominator or denominators, and after you have set the denominators that you want, you write this common denominator after them to the left, or the denominators, of which it is composed, one after the other, instead of it, as it is all the same. |
| − | |style="text-align:right;"|כי אחר שהוצאת האם למורים האם הרי שבו כלם כמורה אחד ואתה מבקש בין הראשונים ובינו מורה או מורים | + | |style="text-align:right;"|כי אחר שהוצאת האם למורים האם הרי שבו כלם כמורה אחד ואתה מבקש בין הראשונים ובינו מורה או מורים אחרים ואחר שהמצאת המורים אשר רצית תשים האם הזאת אחריהם לצד שמאל או המורים אשר {{#annot:term|2491,1961|b4YC}}הורכבה מהם{{#annotend:b4YC}} זה אחר זה במקומה כי הכל אחד |
|- | |- | ||
| − | | | + | |This is enough for the one who understands. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ודי למבין |
|- | |- | ||
| | | | ||
| − | |||
| + | === <span style=color:Green>Check</span> === | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | |If you want to check your practice, decompose all the fractions you have received. | ||
| + | |style="text-align:right;"|<big>ואם</big> תרצה לבחון מעשיך עשה פריטה לכל אלו השברים אשר באו לך | ||
| + | |- | ||
| + | |If the large number, by which you wanted to divide, is among your denominators, i.e. 101 in the last example, divide the resulting decomposed numerator by all other denominators except for it one after the other, or by their common denominator, what you receive should be as the small number that you wanted to divide [originally]. [If] nothing remains in any of these divisions, your procedure was true and correct; if not, know that you were wrong. | ||
|style="text-align:right;"|ואם יש במוריך אלו המספר הגדול אשר רצית לחלק עליו ר"ל הק"א במשל האחרון חלק זה העולה מהשברים הנפרטים על כל שאר המורים מבלעדיו זה אחר זה או על אמם ויצא לך באחרונה כמנין המספר הקטן אשר רצית לחלק ולא נשאר דבר בשום חלוקה מאלו הנה מעשיך אמת ונכון ואם לאו דע שטעית | |style="text-align:right;"|ואם יש במוריך אלו המספר הגדול אשר רצית לחלק עליו ר"ל הק"א במשל האחרון חלק זה העולה מהשברים הנפרטים על כל שאר המורים מבלעדיו זה אחר זה או על אמם ויצא לך באחרונה כמנין המספר הקטן אשר רצית לחלק ולא נשאר דבר בשום חלוקה מאלו הנה מעשיך אמת ונכון ואם לאו דע שטעית | ||
|- | |- | ||
| − | | | + | |If the original denominators, or the original divisor, are among you denominators, in the first example also, divide all the decomposed numerators by the rest of the denominators that were generated in the unification operation, or by their common denominator. If nothing remains and the result is as the small number that you wanted to divide [originally], or as the decomposed fractions in the example at the beginning of the discussion, [the procedure was] true and correct; if not, know that you were wrong. |
| − | + | |style="text-align:right;"|‫<ref>53r</ref>גם במשל הראשון אם יש במוריך אלו הם המורים הראשונים או המורים עצמם חלק כל מספר השברים הנפרטות על שאר המורים שנתחדשו במלאכת האחדות או על אמם ואם לא ישאר לעולם דבר ויצא באחרונה כמספר הקטן אש' רצית לחלק או כשברים הנפרטים במשל ראש המאמר הנה אמת הנה נכון ואם לאו דע שטעית | |
| − | |style="text-align:right;"|גם במשל הראשון אם יש במוריך אלו הם המורים הראשונים או המורים עצמם חלק כל מספר השברים הנפרטות על שאר המורים שנתחדשו במלאכת האחדות או על אמם ואם לא ישאר לעולם דבר ויצא באחרונה כמספר הקטן אש' רצית לחלק או כשברים הנפרטים במשל ראש המאמר הנה אמת הנה נכון ואם לאו דע שטעית | ||
|- | |- | ||
| − | |If the large number | + | |If the common denominator or the original divisor are not in your procedure, i.e. among the denominators, multiply the resulting decomposed numerator by the large number, by which you wanted to divide - whether by the common denominator of the required fractions as in the first example at the beginning of this discussion, or by the large number, by which you wanted to divide as in the second example - we divide the product by all the denominators or by their common denominator, and if the result is as the required decomposed numerator in the first example, or as the small number that you wanted to divide in the second example without any remainder, [the procedure] is true; if not, it is wrong. |
|style="text-align:right;"|ואם אין במלאכתך זאת ר"ל במוריך לא אם המורים ולא המורים עצמם כפול כל המספר השברים הנפרטים בחשבון הגדול אשר רצית לחלק עליו אם באם המורים מהחלקים הנשאלים כבמשל הראשון אשר בראש זה המאמר אם במספר הגדול אשר רצית לחלק עליו כבמשל השני והעולה חלקנו לכל מוריך אלו או לאמם ואם יצא כמספר השברים הנפרטים הנשאלים במשל הראשון או כמספר הקטן אשר רצית לחלק במשל השני מבלי שארית כלל הנה אמת ואם לאו שקר | |style="text-align:right;"|ואם אין במלאכתך זאת ר"ל במוריך לא אם המורים ולא המורים עצמם כפול כל המספר השברים הנפרטים בחשבון הגדול אשר רצית לחלק עליו אם באם המורים מהחלקים הנשאלים כבמשל הראשון אשר בראש זה המאמר אם במספר הגדול אשר רצית לחלק עליו כבמשל השני והעולה חלקנו לכל מוריך אלו או לאמם ואם יצא כמספר השברים הנפרטים הנשאלים במשל הראשון או כמספר הקטן אשר רצית לחלק במשל השני מבלי שארית כלל הנה אמת ואם לאו שקר | ||
|- | |- | ||
| − | | | + | |Before I start with the reason of this check, in order to train you in the procedure, I will check each one of the three mentioned examples: |
|style="text-align:right;"|וקודם התחילי בטעם בחינה זאת כדי להרגילך במעשה אעשה בחינה בכל אחד משלשת המשלים הנזכרים | |style="text-align:right;"|וקודם התחילי בטעם בחינה זאת כדי להרגילך במעשה אעשה בחינה בכל אחד משלשת המשלים הנזכרים | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle\left(\frac{2}{5}\sdot\frac{2}{9}\sdot2\right)+\left[\left[\frac{1}{8}+\left(\frac{2}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\right)\right]\sdot\left[\frac{1}{4}+\left(\frac{2}{6}\sdot\frac{1}{4}\right)\right]\right]</math> | + | *The decomposed [numerator] of the first example: |
| + | :<math>\scriptstyle\left(\frac{2}{5}\sdot\frac{2}{9}\sdot2\right)+\left[\left[\frac{1}{8}+\left(\frac{2}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\right)\right]\sdot\left[\frac{1}{4}+\left(\frac{2}{6}\sdot\frac{1}{4}\right)\right]\right]</math> | ||
|style="text-align:right;"|הנה פריטת המשל הראשון | |style="text-align:right;"|הנה פריטת המשל הראשון | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{\begin{align} | + | :7 by 21 is 21; [plus 2 it is 23]. 23 by 2 is 69. 69 by 6 is 414; with the 3 it is 417. [417] by 4 is 1668. 1668 plus 1 is 1669. 1669 by 5 is 8345. 8345 by 8 is 66760. We receive that the decomposed [numerator] is 66760. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|היה 1<span style="text-decoration: line-through; text-decoration-color: red;"> 3 7 7</span> 7 <sup>ב</sup><s>3</s> 3 21 23 ב3 69 69 ב6 414<span style="text-decoration: line-through; text-decoration-color: red;">4</span> וה3 הם 417 וב4 166<sup>8</sup> 1668 ו1 1669 [5] 1669 8345 8345 [ב8] 66760 והנה עלה בידינו שהפריטה היא 66760 |
| + | |} | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\frac{1}{5}+\left(\frac{2}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)+\left(\frac{3}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)+\left(\frac{1}{4}\sdot\frac{1}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)&\scriptstyle=\left(\frac{\left(3\sdot7\right)+2}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)+\left(\frac{3}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)+\left(\frac{1}{4}\sdot\frac{1}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)\\&\scriptstyle=\left(\frac{21+2}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)+\left(\frac{3}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)+\left(\frac{1}{4}\sdot\frac{1}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)\\&\scriptstyle=\left(\frac{\left(23\sdot3\sdot6\right)+3}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)+\left(\frac{1}{4}\sdot\frac{1}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)\\&\scriptstyle=\left(\frac{\left(69\sdot6\right)+3}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)+\left(\frac{1}{4}\sdot\frac{1}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)\\&\scriptstyle=\left(\frac{414+3}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)+\left(\frac{1}{4}\sdot\frac{1}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)\\&\scriptstyle=\frac{\left(417\sdot4\right)+1}{4}\sdot\frac{1}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}=\frac{1668+1}{4}\sdot\frac{1}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\\&\scriptstyle=\frac{1669\sdot5}{5}\sdot\frac{1}{4}\sdot\frac{1}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}=\frac{8345\sdot8}{8}\sdot\frac{1}{5}\sdot\frac{1}{4}\sdot\frac{1}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\\&\scriptstyle=\frac{66760}{8}\sdot\frac{1}{5}\sdot\frac{1}{4}\sdot\frac{1}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\\\end{align}}}</math> | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | ::Had we not have all the original denominators, we would have multiplied it by all the original denominators, then we would have divided the result by all these eight denominators, one by one, or by their common denominator and the result were the required decomposed numerator, which is 13352. | ||
| + | |style="width:45%; text-align:right;"|ואם לא היו בידינו כל המורים הראשונים היו כופלים זה בכל המורים הראשונים והעולה היינו מחלקים אל כל שמונת מורים אלו אחד אחד אחד או לאמם והיא יוצא מספר פריטת השברים הנשאלים והיא 13352 | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Since we have all the original denominators, i.e. all the denominators of the required fractions - and do not be mistaken that there is no 9 here, as 3 and 3, which are its divisors, are instead of it - we divide what we received from the decomposing, i.e. 66760 by the denominators that were generated from our procedure, i.e. by the first 5 alone, because no other was generated; the result of division is 13352, which is the required decomposed numerator and nothing remains, so it is true. |
| + | |style="text-align:right;"|אכן אחרי היות בידינו כל המורים הראשונים ר"ל כל מורה השברים ‫<ref>53v</ref>הנשאלים ואל יטעך שאין כאן הט' שהרי במקומו ג' ג' שהם מוריו ונחלוק זה אשר עלה לנו מפריטתינו זאת ר"ל ה66760 למורים שנתחדשו במלאכתינו ר"ל לה' הראשון לבדו כי לא נתחדשו עוד ויצא בחילוק 13352 שהוא מספר פריטת השברים הנשאלים ולא נשאר דבר והנה אמת | ||
| + | |} | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\frac{1}{5}+\left(\frac{2}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)+\left(\frac{3}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)+\left(\frac{1}{4}\sdot\frac{1}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)&\scriptstyle=\frac{66760}{8}\sdot\frac{1}{5}\sdot\frac{1}{4}\sdot\frac{1}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\\&\scriptstyle=\frac{66760}{5}\sdot\frac{1}{8}\sdot\frac{1}{4}\sdot\frac{1}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}=\frac{13352}{8}\sdot\frac{1}{5}\sdot\frac{1}{4}\sdot\frac{1}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\\\end{align}}}</math> | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | :<math>\ | + | *In the second example: |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle73\div240</math> |
| − | + | |style="width:45%; text-align:right;"|<big>ובמשל</big> השני | |
|- | |- | ||
| | | | ||
| − | + | :The decomposed [numerator] is: 1 by 5 is 5; plus 1 it is 6. 6 by 6 is 36. [36] by 8 is 288; plus 4 it is 292. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הוא הפריטה א' בה' ה' וא' ו' ו' בו' ל"ו בח' 288 ו4 292 |
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\frac{1}{4}+\left(\frac{1}{5}\sdot\frac{1}{4}\right)+\left(\frac{4}{8}\sdot\frac{1}{6}\sdot\frac{1}{5}\sdot\frac{1}{4}\right)&\scriptstyle=\left(\frac{\left(1\sdot5\right)+1}{5}\sdot\frac{1}{4}\right)+\left(\frac{4}{8}\sdot\frac{1}{6}\sdot\frac{1}{5}\sdot\frac{1}{4}\right) | + | :We divide it by 4, which is the new denominator; the result of division is 73 with no remainder and this is the small number that we want to divide, so it is correct. |
| − | | | + | |style="text-align:right;"|נחלקם לד' שהוא המורה המתחדש יצא בחילוק מבלי שארית 73 שהוא המספר הקטן שרצינו לחלק והנה אמת |
| − | + | |} | |
| − | + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\frac{1}{4}+\left(\frac{1}{5}\sdot\frac{1}{4}\right)+\left(\frac{4}{8}\sdot\frac{1}{6}\sdot\frac{1}{5}\sdot\frac{1}{4}\right)&\scriptstyle=\left(\frac{\left(1\sdot5\right)+1}{5}\sdot\frac{1}{4}\right)+\left(\frac{4}{8}\sdot\frac{1}{6}\sdot\frac{1}{5}\sdot\frac{1}{4}\right)=\left(\frac{5+1}{5}\sdot\frac{1}{4}\right)+\left(\frac{4}{8}\sdot\frac{1}{6}\sdot\frac{1}{5}\sdot\frac{1}{4}\right)\\&\scriptstyle=\frac{\left(6\sdot6\sdot8\right)+4}{8}\sdot\frac{1}{6}\sdot\frac{1}{5}\sdot\frac{1}{4}=\frac{\left(36\sdot8\right)+4}{8}\sdot\frac{1}{6}\sdot\frac{1}{5}\sdot\frac{1}{4}=\frac{288+4}{8}\sdot\frac{1}{6}\sdot\frac{1}{5}\sdot\frac{1}{4}=\frac{292}{4}\sdot\frac{1}{240}=\frac{73}{240}\\\end{align}}}</math> | |
| − | + | {| | |
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle38\div101</math> | + | *In the third example: |
| − | |style="text-align:right;"|ובמשל השלישי | + | :<math>\scriptstyle38\div101</math> |
| + | |style="width:45%; text-align:right;"|ובמשל השלישי | ||
|- | |- | ||
| | | | ||
| + | :In the first figure the decomposed [numerator] is 114. We divide it by 3, which is the new denominator; the result is 38, which is the small number that we wanted to divide. | ||
:<math>\scriptstyle{\color{blue}{\scriptstyle\frac{1}{3}+\left(\frac{13}{101}\sdot\frac{1}{3}\right)=\frac{114}{3}\sdot\frac{1}{101}=\frac{38}{101}}}</math> | :<math>\scriptstyle{\color{blue}{\scriptstyle\frac{1}{3}+\left(\frac{13}{101}\sdot\frac{1}{3}\right)=\frac{114}{3}\sdot\frac{1}{101}=\frac{38}{101}}}</math> | ||
|style="text-align:right;"|בצורה הראשונה הנה הפריטה עולה 114 נחלקם לג' שהוא המורה המתחדש יצאו הל"ח שהוא המספר הקטן אשר רצינו לחלק | |style="text-align:right;"|בצורה הראשונה הנה הפריטה עולה 114 נחלקם לג' שהוא המורה המתחדש יצאו הל"ח שהוא המספר הקטן אשר רצינו לחלק | ||
|- | |- | ||
| | | | ||
| − | : | + | :In the second figure the decomposed [numerator] is 912. We divide it by 3 and 8, which are the new denominators: first by 3; the result is 304. We divide it by 8; the result is 38. |
|style="text-align:right;"|ובצורה השנית הפריטה 912 נחלקם לג' ולח' שהם המורים החדשי' תחלה לג' יצא 304 נחלקם לח' ויצאו הל"ח | |style="text-align:right;"|ובצורה השנית הפריטה 912 נחלקם לג' ולח' שהם המורים החדשי' תחלה לג' יצא 304 נחלקם לח' ויצאו הל"ח | ||
| + | |} | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}+\left(\frac{1}{3}\sdot\frac{1}{8}\right)+\left(\frac{3}{101}\sdot\frac{1}{3}\sdot\frac{1}{8}\right)=\frac{912}{3}\sdot\frac{1}{8}\sdot\frac{1}{101}=\frac{304}{8}\sdot\frac{1}{101}=\frac{38}{101}}}</math> | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{ | + | :In the third figure the decomposed [numerator] is 31008. We divide it by 3; the result is 10336. We divide it by 8; the result is 1292. We divide it by 34, which is the denominator that remains from the new denominators; the result is 38, so it is true. |
| − | | | + | |style="width:45%; text-align:right;"|ובצורה השלישית הפריטה 31008 נחלקם לג' יצא 10336 נחלקם לח' יצא 1292 נחלקם לל"ד שהוא המורה הנשאר מהמורים החדשים יצא הל"ח והנה אמת |
| + | |} | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}+\left(\frac{1}{3}\sdot\frac{1}{8}\right)+\left(\frac{1}{3}\sdot\frac{1}{8}\sdot\frac{1}{34}\right)+\left(\frac{1}{3}\sdot\frac{1}{8}\sdot\frac{1}{34}\sdot\frac{1}{101}\right)=\frac{31008}{3}\sdot\frac{1}{8}\sdot\frac{1}{34}\sdot\frac{1}{101}=\frac{10336}{8}\sdot\frac{1}{34}\sdot\frac{1}{101}=\frac{1292}{34}\sdot\frac{1}{101}=\frac{38}{101}}}</math> | ||
| + | {| | ||
|- | |- | ||
| − | | | + | !<span style=color:Green>Reason: check</span> |
| − | |style="text-align:right;"|וטעם בחינה זה הוא ברור כי כשיש במורינו המורי' הראשונים או האם או האם או המספר הגדול אשר רצינו לחלק עליו הנה הפריטה היא מספר שברים מכל המורים חדשים גם שנים וזה ברור כמו שנתבאר פעמים רבות | + | | |
| + | |- | ||
| + | |The reason of this check is clear because when the original denominators, or the common denominator, or the great number, by which we wanted to divide, are among our denominators, decomposing means the numerator of [the fraction that consists of] all the original as well as the new denominators and this is clear as was explained many times. | ||
| + | |style="width:45%; text-align:right;"|<big>וטעם</big> בחינה זה הוא ברור כי כשיש במורינו המורי' הראשונים או האם <s>או האם</s> או המספר הגדול אשר רצינו לחלק עליו הנה הפריטה היא מספר שברים מכל המורים חדשים גם שנים וזה ברור כמו שנתבאר פעמים רבות | ||
| + | |- | ||
| + | |Since decomposing is to convert them to the lowest fraction, which is the last type and it is related to all the denominators. | ||
| + | |style="text-align:right;"|כי הפריטה הוא להשיבם פרוטות <s>כי הפריטה הוא מספר שברים מכל המורים</s> שהוא המין האחרון והוא {{#annot:term|1567,1494|G7Ca}}נקשר{{#annotend:G7Ca}} בכל המורים | ||
|- | |- | ||
| − | | | + | |When we divide it by the new denominators it is as reducing, for the order does not matter. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכאשר נחלקם על המורים המתחדשים הוא כעושה כלילת יופי כי הסדר לא יזיק |
| + | |- | ||
| + | |After it is divided by all the new [denominators] and nothing remains, it is reduced and the result is a numerator of the new denominators, or their common denominator, or of the large number. | ||
| + | |style="text-align:right;"|ואחר שנתחלק על כל החדשים ולא נשאר ‫<ref>54r</ref>דבר הנה יצאו מן הכלל והיוצא באחרונה הם שברים מהמורים הראשונים או מאמם כבראשונה או מהמספר הגדול | ||
|- | |- | ||
| | | | ||
| + | ::I.e. that the 38 that we received, after we divided the decomposed by the new denominators and they were reduced, are parts of 101 parts of the whole. Because each unit of the 38 is one part of 101 of the whole. | ||
|style="text-align:right;"|ר"ל שהל"ח שיצאו לנו אחר שחלקנו הפריטה במורים החדשי' ויצאו הם מן הכלל הם חלקים מק"א חלקים בשלם כי לכל אחד מהל"ח יעלה לכל אחד חלק אחד מק"א בשלם ומהל"ח ל"ח | |style="text-align:right;"|ר"ל שהל"ח שיצאו לנו אחר שחלקנו הפריטה במורים החדשי' ויצאו הם מן הכלל הם חלקים מק"א חלקים בשלם כי לכל אחד מהל"ח יעלה לכל אחד חלק אחד מק"א בשלם ומהל"ח ל"ח | ||
|- | |- | ||
| − | | | + | |The reason for our saying: "if the original denominators, or their common denominator, or the greatest number are not among our denominators, we multiply the decomposed by the original denominators, or by their common denominator, or by the greatest number and divide by all the denominators, so that the result is without a remainder as the required decomposed fractions in the first example, or as the smaller number in the second number" - is that the decomposed fraction consists of all these denominators and when we multiply it by the original denominators, or by their common denominator, or by the greatest number, we decompose it further to fractions of fractions of the original [denominators]. |
| − | |style="text-align:right;"|וטעם אומרנו שאם אין המורים הראשונים או אמם או המספר הגדול במורינו שנכפול הפריטה במורים הראשונים או באמם או במספר הגדול ונחלקנו בכל המורים שיצא מבלי שארית כמספר פריטת השברים הנשאלים במשל הראשון או כמספר הקטן במשל השני הוא לפי שהפריטה היא שברים מכל אלו המורים וכאשר אנו כופלים אותה במורים הראשונים או באמם או במספר הגדול הוא שאנו פורטים אותה עוד לשברי שברים מהראשונים | + | |style="text-align:right;"|<big>וטעם</big> אומרנו שאם אין המורים הראשונים או אמם או המספר הגדול במורינו שנכפול הפריטה במורים הראשונים או באמם או במספר הגדול ונחלקנו בכל המורים שיצא מבלי שארית כמספר פריטת השברים הנשאלים במשל הראשון או כמספר הקטן במשל השני הוא לפי שהפריטה היא שברים מכל אלו המורים וכאשר אנו כופלים אותה במורים הראשונים או באמם או במספר הגדול הוא שאנו פורטים אותה עוד לשברי שברים מהראשונים |
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle\frac{3}{4}\sdot\frac{1}{8}</math> | + | *I.e. if we have 3-quarters of an eighth, for instance: |
| + | :<math>\scriptstyle\frac{3}{4}\sdot\frac{1}{8}</math> | ||
|style="text-align:right;"|ר"ל כי אם יש בידינו ג' רביעיות שמינית ע'ד'מ‫' | |style="text-align:right;"|ר"ל כי אם יש בידינו ג' רביעיות שמינית ע'ד'מ‫' | ||
|- | |- | ||
| | | | ||
| + | :If we multiply them by 7, the result are sevenths of a quarter of an eighth and this has been clarified many times. | ||
:<math>\scriptstyle{\color{blue}{\frac{3}{4}\sdot\frac{1}{8}=\frac{7\sdot3}{7}\sdot\frac{1}{4}\sdot\frac{1}{8}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{3}{4}\sdot\frac{1}{8}=\frac{7\sdot3}{7}\sdot\frac{1}{4}\sdot\frac{1}{8}}}</math> | ||
|style="text-align:right;"|אם נכפלם בז' היוצא שביעית רביעית שמינית וזה נתברר פעמים רבות | |style="text-align:right;"|אם נכפלם בז' היוצא שביעית רביעית שמינית וזה נתברר פעמים רבות | ||
|- | |- | ||
| − | |Therefore, the product by the original denominators is a numerator of a fraction that consists of | + | |Therefore, in our procedure, the product [by the original denominators] is a numerator of [a fraction that consists of] all these [renewed] denominators that we have, as well as of the original denominators, or of their common denominator, or of the large number that we added [to the new denominators] just now. When we divide it by our denominators, i.e. [the renewed denominators] without the original denominators that we added now [to the new denominators], or without their common denominator, or without the large number, because we do not divide by them, the result of division should be a numerator of [a fraction that consists of] the original denominators, or of their common denominator, or of the large number. |
|style="text-align:right;"|והנה במעשינו היוצא אחר הכפל יהיו שברים מכל אלו המורים אשר לנו ומהראשונים או מאמם או מהמספר הגדול שהוספנו עליהם עתה וכאשר נחלקנו למורינו ר"ל מבלתי הראשונים אשר הוספנו עתה או מבלתי אמם או מבלתי המספר הגדול אשר הוספנו עתה כי להן לא נחלקם ישאר היוצא שברים מהמורים הראשונים או מאמם או מהמספר הגדול | |style="text-align:right;"|והנה במעשינו היוצא אחר הכפל יהיו שברים מכל אלו המורים אשר לנו ומהראשונים או מאמם או מהמספר הגדול שהוספנו עליהם עתה וכאשר נחלקנו למורינו ר"ל מבלתי הראשונים אשר הוספנו עתה או מבלתי אמם או מבלתי המספר הגדול אשר הוספנו עתה כי להן לא נחלקם ישאר היוצא שברים מהמורים הראשונים או מאמם או מהמספר הגדול | ||
|- | |- | ||
| − | | | + | |If the result is as the decomposed numerator required in the first example, or as the small number in the second [example], it is restored to what it was in the beginning, so our calculation is correct. |
|style="text-align:right;"|והנה אם היוצא היה כמספר פריטת השברים הנשאלים במשל הראשון או כמספר הקטן בשני הנה שב כבתחלה והנה כל מעשינו אמת ויציב | |style="text-align:right;"|והנה אם היוצא היה כמספר פריטת השברים הנשאלים במשל הראשון או כמספר הקטן בשני הנה שב כבתחלה והנה כל מעשינו אמת ויציב | ||
|- | |- | ||
| − | | | + | |Know that the prime number, i.e. if the large number [by which the small number is divided] is a prime number that has no divisors, as in the third example, which is 101, it cannot be absent or converted [after the unification procedure], and this is clear because it cannot be divided completely by another number without a remainder, since it is prime. |
| − | + | |style="text-align:right;"|ודע כי ‫<ref>54v</ref>המספר הפשוט ר"ל אם היה המספר הגדול מספר פשוט שאין לו מורים כבמשל השלישי שהוא קי"א כי לעולם לא יעדר ולא יומר וזה ברור כי הוא לא יתחלק לשום מספר בשלימות מבלי שארית אחר שהוא פשוט | |
| − | |style="text-align:right;"|ודע כי המספר הפשוט ר"ל אם היה המספר הגדול מספר פשוט שאין לו מורים כבמשל השלישי שהוא קי"א כי לעולם לא יעדר ולא יומר וזה ברור כי הוא לא יתחלק לשום מספר בשלימות מבלי שארית אחר שהוא פשוט | ||
|- | |- | ||
|} | |} | ||
| Line 5,878: | Line 7,116: | ||
|- | |- | ||
| | | | ||
| − | *Example: if you are told: three-quarters and two-fifths of a quarter of two-ninths, subtract them from eight-ninths and three-sevenths of a fifth of a ninth of five-sixths of 3 integers | + | *{{#annot:(⁸/₉+³/₇·⅕·⅑)·(⅚·3)-(¾+⅖·¼)·²/₉|678|0SK8}}Example: if you are told: three-quarters and two-fifths of a quarter of two-ninths, subtract them from eight-ninths and three-sevenths of a fifth of a ninth of five-sixths of 3 integers |
:<math>\scriptstyle\left[\left[\frac{8}{9}+\left(\frac{3}{7}\sdot\frac{1}{5}\sdot\frac{1}{9}\right)\right]\sdot\left(\frac{5}{6}\sdot3\right)\right]-\left[\left[\frac{3}{4}+\left(\frac{2}{5}\sdot\frac{1}{4}\right)\right]\sdot\frac{2}{9}\right]</math> | :<math>\scriptstyle\left[\left[\frac{8}{9}+\left(\frac{3}{7}\sdot\frac{1}{5}\sdot\frac{1}{9}\right)\right]\sdot\left(\frac{5}{6}\sdot3\right)\right]-\left[\left[\frac{3}{4}+\left(\frac{2}{5}\sdot\frac{1}{4}\right)\right]\sdot\frac{2}{9}\right]</math> | ||
| − | |style="text-align:right;"|המשל אם אמרו לך שלש רביעיות ושתי חמישיות רביעית משתי תשיעיות חסרם משמונה תשיעיות ושלש שביעיות חמשית תשיעית מחמש ששיות מג' שלמים | + | |style="text-align:right;"|<big>המשל</big> אם אמרו לך שלש רביעיות ושתי חמישיות רביעית משתי תשיעיות חסרם משמונה תשיעיות ושלש שביעיות חמשית תשיעית מחמש ששיות מג' שלמים{{#annotend:0SK8}} |
|- | |- | ||
| | | | ||
| Line 5,915: | Line 7,153: | ||
|- | |- | ||
| | | | ||
| − | ::The smaller number, after it is multiplied and | + | ::The smaller number, after it is multiplied and decomposed, becomes 34 fifths of a quarter of a ninth, like this: |
::<math>\scriptstyle{\color{blue}{\left[\frac{3}{4}+\left(\frac{2}{5}\sdot\frac{1}{4}\right)\right]\sdot\frac{2}{9}=\frac{34}{5}\sdot\frac{1}{4}\sdot\frac{1}{9}}}</math> | ::<math>\scriptstyle{\color{blue}{\left[\frac{3}{4}+\left(\frac{2}{5}\sdot\frac{1}{4}\right)\right]\sdot\frac{2}{9}=\frac{34}{5}\sdot\frac{1}{4}\sdot\frac{1}{9}}}</math> | ||
|style="text-align:right;"|והנה המעט אחרי אשר הוכה ונפרט יעלה 34 חמישיות רביעית תשיעית כזה | |style="text-align:right;"|והנה המעט אחרי אשר הוכה ונפרט יעלה 34 חמישיות רביעית תשיעית כזה | ||
| Line 5,929: | Line 7,167: | ||
|- | |- | ||
| | | | ||
| − | ::The greater number, after it is multiplied and | + | ::The greater number, after it is multiplied and decomposed, becomes 4245 sevenths of a fifth of a ninth of a sixth. |
::<math>\scriptstyle{\color{blue}{\left[\frac{8}{9}+\left(\frac{3}{7}\sdot\frac{1}{5}\sdot\frac{1}{9}\right)\right]\sdot\left(\frac{5}{6}\sdot3\right)=\frac{4245}{7}\sdot\frac{1}{5}\sdot\frac{1}{9}\sdot\frac{1}{6}}}</math> | ::<math>\scriptstyle{\color{blue}{\left[\frac{8}{9}+\left(\frac{3}{7}\sdot\frac{1}{5}\sdot\frac{1}{9}\right)\right]\sdot\left(\frac{5}{6}\sdot3\right)=\frac{4245}{7}\sdot\frac{1}{5}\sdot\frac{1}{9}\sdot\frac{1}{6}}}</math> | ||
| − | |style="text-align:right;"|והרב יעלה אחרי שנפרט והוכה שביעיות 4245 שביעיות חמישית תשיעית ששית | + | |style="text-align:right;"|והרב יעלה אחרי שנפרט והוכה <s>שביעיות</s> 4245 שביעיות חמישית תשיעית ששית |
|- | |- | ||
| − | |After multiplying and | + | |After multiplying and decomposing, we should expand them by multiplying the numerator of one by the denominators of the other, then both are of the same fractions. |
:<math>\scriptstyle{\color{red}{\frac{a}{b}=\frac{a\sdot d}{b}\sdot\frac{1}{d}\quad\frac{c}{d}=\frac{c\sdot b}{d}\sdot\frac{1}{b}}}</math> | :<math>\scriptstyle{\color{red}{\frac{a}{b}=\frac{a\sdot d}{b}\sdot\frac{1}{d}\quad\frac{c}{d}=\frac{c\sdot b}{d}\sdot\frac{1}{b}}}</math> | ||
|style="text-align:right;"|ואחרי שהוכו ונפרטו יש לנו להשוותם וזה בכפול מספר שברי כל אחת במורי חברתה ואז היו כל אחת מהם שברים | |style="text-align:right;"|ואחרי שהוכו ונפרטו יש לנו להשוותם וזה בכפול מספר שברי כל אחת במורי חברתה ואז היו כל אחת מהם שברים | ||
| Line 5,939: | Line 7,177: | ||
| | | | ||
:To make the procedure easier for us, since 9 and 5 are in the denominators of both the same number of times, which is once, we do not multiply any of [the numerators] by them, as explained at the end of the third chapter, and we set them only once. | :To make the procedure easier for us, since 9 and 5 are in the denominators of both the same number of times, which is once, we do not multiply any of [the numerators] by them, as explained at the end of the third chapter, and we set them only once. | ||
| − | |style="text-align:right;"|אכן להקל עלינו המעשה אחרי היות בש[בריהם] הט' והה' פעמים שוות והוא פעם אחת לא נכפול בהם שום אחת מהם כמו שנתבאר בסוף השער הג' וגם לא נסדרם שום אחת מהם כי אם פעם אחת | + | |style="text-align:right;"|אכן להקל עלינו המעשה אחרי היות בש[בריהם] הט' והה' פעמים שוות והוא פעם אחת לא נכפול בהם שום אחת מהם כמו שנתבאר בסוף השער הג' וגם לא נסדרם <s>שום אחת מהם</s> כי אם פעם אחת |
|- | |- | ||
| | | | ||
::The smaller – after it is multiplied by 6 and 7 one after another, which are the denominators of the other number, except for 9 and 5, by which we do not multiply as mentioned – becomes 1828; and since it is multiplied by 6 and 7, these denominators are added to its denominators, so they are 1428 sevenths of a sixth of a fifth of a quarter of a ninth. | ::The smaller – after it is multiplied by 6 and 7 one after another, which are the denominators of the other number, except for 9 and 5, by which we do not multiply as mentioned – becomes 1828; and since it is multiplied by 6 and 7, these denominators are added to its denominators, so they are 1428 sevenths of a sixth of a fifth of a quarter of a ninth. | ||
| − | |style="text-align:right;"|והנה המעט אחרי הכפלו בו' ובז' זה אחר זה שהם מורי חברתה מזולת הט' והה' שלא נכפול בהם כנזכר יעלה 1828 ואחר שהוכה בו' ובז' נתוספו לו מורים אלו על מוריו לכן יהיו אלו ה1428 שביעיות שישית חמישית רביעית תשיעית | + | |style="text-align:right;"|והנה המעט אחרי הכפלו בו' ובז' זה אחר זה שהם מורי חברתה מזולת הט' והה' שלא נכפול בהם כנזכר יעלה 1828 ואחר שהוכה בו' ובז' ‫<ref>55r</ref>נתוספו לו מורים אלו על מוריו לכן יהיו אלו ה1428 שביעיות שישית חמישית רביעית תשיעית |
| − | | | + | |- |
| + | | | ||
::<math>\scriptstyle{\color{blue}{\left[\frac{3}{4}+\left(\frac{2}{5}\sdot\frac{1}{4}\right)\right]\sdot\frac{2}{9}=\frac{34\sdot6\sdot7}{7}\sdot\frac{1}{6}\sdot\frac{1}{5}\sdot\frac{1}{4}\sdot\frac{1}{9}=\frac{1428}{7}\sdot\frac{1}{6}\sdot\frac{1}{5}\sdot\frac{1}{4}\sdot\frac{1}{9}}}</math> | ::<math>\scriptstyle{\color{blue}{\left[\frac{3}{4}+\left(\frac{2}{5}\sdot\frac{1}{4}\right)\right]\sdot\frac{2}{9}=\frac{34\sdot6\sdot7}{7}\sdot\frac{1}{6}\sdot\frac{1}{5}\sdot\frac{1}{4}\sdot\frac{1}{9}=\frac{1428}{7}\sdot\frac{1}{6}\sdot\frac{1}{5}\sdot\frac{1}{4}\sdot\frac{1}{9}}}</math> | ||
| − | + | | | |
|- | |- | ||
| | | | ||
:Hence, the reason is clear why we set all the denominators and why we do not set the 9 and the 5 once more, even though they are in the other number, this is because it was not multiplied by them and it is obvious. | :Hence, the reason is clear why we set all the denominators and why we do not set the 9 and the 5 once more, even though they are in the other number, this is because it was not multiplied by them and it is obvious. | ||
| − | |style=" | + | |style="text-align:right;"|ובכאן נתבאר הטעם למה אנו מסדרים כל המורים ולמה אין אנו מסדרי' הט' והה' פעם אחרת ואם הם בחברתה והוא לפי שלא נכפלו בהם וזה ברור |
|- | |- | ||
| | | | ||
| Line 5,965: | Line 7,204: | ||
| | | | ||
:We set the numbers one above the other, then we subtract as the procedure of integers; the remainder is 15552 quarters of a seventh of a fifth of a ninth of a sixth. If you wish, you can reduce them, so the remainder becomes 2 integers and 2-sevenths of a fifth. | :We set the numbers one above the other, then we subtract as the procedure of integers; the remainder is 15552 quarters of a seventh of a fifth of a ninth of a sixth. If you wish, you can reduce them, so the remainder becomes 2 integers and 2-sevenths of a fifth. | ||
| − | |||
|style="text-align:right;"|ונשים המספרים זה על זה ונחסרנו כמעשינו בשלמים ונשארו 15552 רביעיות שביעית חמשית תשיעית שישית<br> | |style="text-align:right;"|ונשים המספרים זה על זה ונחסרנו כמעשינו בשלמים ונשארו 15552 רביעיות שביעית חמשית תשיעית שישית<br> | ||
ואם תרצה תעשה להם כלילת יופי ויעלה זה השארית ב' שלמים וב' שביעיות חמישית והקש על זה | ואם תרצה תעשה להם כלילת יופי ויעלה זה השארית ב' שלמים וב' שביעיות חמישית והקש על זה | ||
| + | |} | ||
| + | :<math>\scriptstyle{\color{blue}{\left[\left[\frac{8}{9}+\left(\frac{3}{7}\sdot\frac{1}{5}\sdot\frac{1}{9}\right)\right]\sdot\left(\frac{5}{6}\sdot3\right)\right]-\left[\left[\frac{3}{4}+\left(\frac{2}{5}\sdot\frac{1}{4}\right)\right]\sdot\frac{2}{9}\right]=\left(\frac{16980}{4}\sdot\frac{1}{7}\sdot\frac{1}{5}\sdot\frac{1}{9}\sdot\frac{1}{6}\right)-\left(\frac{1428}{7}\sdot\frac{1}{6}\sdot\frac{1}{5}\sdot\frac{1}{4}\sdot\frac{1}{9}\right)=\frac{15552}{4}\sdot\frac{1}{7}\sdot\frac{1}{5}\sdot\frac{1}{9}\sdot\frac{1}{6}=2+\left(\frac{2}{7}\sdot\frac{1}{5}\right)}}</math> | ||
| + | {| | ||
|- | |- | ||
| − | | | + | |This is the rule: we decompose and multiply both the greater [= the minuend] and the smaller [= the subtracted], or any of them that requires it, then we expand their [denominators], subtract [the numerator of] one from the other as the way of the integers and reduce the remainder that includes all the denominators that are included originally in each of them. |
| − | |style="text-align:right;"|זה הכלל שנעשה לכל אחד מהמספרים הרב והמעט פריטה והכאה או אשר יצטרך מהם ואחר כך נעשה להם השוואה ואחר כך נחסרם זה מזה כדרכנו בשלמים והנשאר נעשה לו כלילת יופי והוא כל השברים ר"ל היו לאחת מהם עם אשר הוכתה בהם מאשר בחברתה | + | |style="width:45%; text-align:right;"|<big>זה הכלל</big> שנעשה לכל אחד מהמספרים הרב והמעט פריטה והכאה או אשר יצטרך מהם ואחר כך נעשה להם השוואה ואחר כך נחסרם זה מזה כדרכנו בשלמים והנשאר נעשה לו כלילת יופי והוא כל השברים ר"ל היו לאחת מהם עם אשר הוכתה בהם מאשר בחברתה |
|- | |- | ||
|} | |} | ||
| Line 5,978: | Line 7,219: | ||
== Chapter Three: Multiplication == | == Chapter Three: Multiplication == | ||
| − | + | |style="width:45%; text-align:right;"|<big>הפרק השלישי בכפל</big> | |
|- | |- | ||
| − | | | + | |The operation [described] in this chapter is the same operation [described] in the second principle of compound fractions. |
|style="text-align:right;"|הנה מעשה זה הפרק הוא מעש' השער השני הנקרא שער ההכאה | |style="text-align:right;"|הנה מעשה זה הפרק הוא מעש' השער השני הנקרא שער ההכאה | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle\frac{3}{4}\times\frac{4}{5}</math> | + | *Because, our saying: multiply 3-quarters by 4-fifths, for instance, is as our saying: 3-quarters of 4-fifths. |
| − | |style="text-align:right;"|כי אמרנו כפול ג' רביעיות על ד' חמישיות ע'ד'מ | + | :<math>\scriptstyle{\color{blue}{\frac{3}{4}\times\frac{4}{5}}}</math> |
| + | |style="text-align:right;"|כי אמרנו כפול ג' רביעיות על ד' חמישיות ע'ד'מ' הוא כאומרנו ג' רביעיות מד' חמישיות | ||
|- | |- | ||
| − | | | + | |We multiply the numerator by the numerator, not by the denominators. |
| − | :<math>\scriptstyle{\color{ | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}\times\frac{c}{d}=\frac{a\sdot c}{d}\sdot\frac{1}{b}}}</math> |
| − | + | |style="text-align:right;"|ונכפול מספר השברים במספר השברים לא במורים | |
| − | |||
| − | |||
| − | |style="text-align:right;"|ונכפול מספר השברים במספר השברים | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{\frac{3}{4}\times\frac{4}{5}=\frac{3\sdot4}{5}\sdot\frac{1}{4}=\frac{12}{5}\sdot\frac{1}{4}}}</math> | + | ::I.e. 3 by 4; the result is 12 and this is the numerator of the denominators of both numbers, i.e. they are fifths of a quarter. |
| − | |style="text-align:right;"|‫[ר"ל הג' על הד' | + | ::<math>\scriptstyle{\color{blue}{\frac{3}{4}\times\frac{4}{5}=\frac{3\sdot4}{5}\sdot\frac{1}{4}=\frac{12}{5}\sdot\frac{1}{4}}}</math> |
| + | |style="text-align:right;"|‫[ר"ל הג' על הד' יעלו י"ב והם שברים ממורי שני המספרים ר"ל]‫<ref>marg.</ref> ר"ל שהן חמישיות רביעית | ||
|- | |- | ||
| − | | | + | |Therefore, there is no need in this chapter for expansion at all, because they are not two [different] types of fractions, but they are fractions that are related to each other, as we explained. |
| − | |style="text-align:right;"|ולזה אין מבוא בזה השער להשואה כלל | + | |style="text-align:right;"|ולזה אין מבוא בזה השער להשואה כלל כי אינם שני מינים שברים אבל הם שברים ‫<ref>55v</ref>{{#annot:term|1567,1494|S0DR}}נקשרים זו בזו{{#annotend:S0DR}} כמו שביארנו |
|- | |- | ||
|In order to train you in the procedure I give an example: | |In order to train you in the procedure I give an example: | ||
| Line 6,005: | Line 7,245: | ||
|- | |- | ||
| | | | ||
| − | *Example: we wish to multiply 4-sevenths of 5-ninths of an eighth by 3-fifths of a ninth of 2-thirds of 5 integers. | + | *{{#annot:⁴/₇·(⁵/₉·⅛)×(⅗·⅑)·(⅔·5)|17|WIlB}}Example: we wish to multiply 4-sevenths of 5-ninths of an eighth by 3-fifths of a ninth of 2-thirds of 5 integers. |
:<math>\scriptstyle\left[\left[\frac{4}{7}\sdot\left(\frac{5}{9}\sdot\frac{1}{8}\right)\right]\right]\times\left[\left(\frac{3}{5}\sdot\frac{1}{9}\right)\sdot\left(\frac{2}{3}\sdot5\right)\right]</math> | :<math>\scriptstyle\left[\left[\frac{4}{7}\sdot\left(\frac{5}{9}\sdot\frac{1}{8}\right)\right]\right]\times\left[\left(\frac{3}{5}\sdot\frac{1}{9}\right)\sdot\left(\frac{2}{3}\sdot5\right)\right]</math> | ||
| − | |style="text-align:right;"|המשל רצינו לכפול ד' שביעיות מה' תשיעיות שמינית על ג' חמשיות תשיעית מב' שלישיות מה' שלמים | + | |style="text-align:right;"|<big>המשל</big> רצינו לכפול ד' שביעיות מה' תשיעיות שמינית על ג' חמשיות תשיעית מב' שלישיות מה' שלמים{{#annotend:WIlB}} |
|- | |- | ||
| | | | ||
| − | : | + | :You do not have to do anything except for relating them together and write "of" [the letter מ] instead of "by", i.e. say: they are 4-sevenths of 5-ninths of an eighth of 3-fifths of a ninth of 2-thirds of 5 integers. So, we are back to the chapter on compound fractions. |
| − | |style="text-align:right;"|אין לך לעשות דבר כי אם {{#annot:term|1567,1404|9XkM}}לקשרם יחד{{#annotend:9XkM}} ולשים במקום על מ' ר"ל שתאמר הם ד' שביעיות מה' תשיעיות שמינית [מג'] חמישיות תשיעית מב' שלישיות מה' שלמים והרי לנו חזרו לשער ההכאה | + | |style="text-align:right;"|אין לך לעשות <sup>דבר</sup> כי אם {{#annot:term|1567,1404|9XkM}}לקשרם יחד{{#annotend:9XkM}} ולשים במקום על מ' ר"ל שתאמר הם ד' שביעיות מה' תשיעיות שמינית [מג']‫<ref>marg.</ref> חמישיות תשיעית מב' שלישיות מה' שלמים והרי לנו חזרו לשער ההכאה |
| − | |||
|- | |- | ||
| | | | ||
| − | + | :If you want to know how much they are, multiply them, for in this example there is no need for decomposing, then we reduce the result with all the denominators, because all are related to each other. The result after the multiplication is 600-sevenths of a ninth of an eighth of a fifth of a ninth of a third. | |
| − | |style="text-align:right;"|ודע שלעולם המורים עליונים ולא תמצא עליהם דבר ובזה תבחין בין המורים למספר השברים | + | |style="text-align:right;"|ואם תרצה לידע מה המה אלה עשה להם הכאה כי בזה המשל אין מבוא לפריטה והעולה נעשה לו כלילת יופי על כל המורים כי כלם נקשרים זה בזה ויעלה אחר ההכאה 600 שביעיות תשיעית שמינית חמישית תשיעית שלישית |
| + | |} | ||
| + | :<math>\scriptstyle{\color{blue}{\left[\left[\frac{4}{7}\sdot\left(\frac{5}{9}\sdot\frac{1}{8}\right)\right]\right]\times\left[\left(\frac{3}{5}\sdot\frac{1}{9}\right)\sdot\left(\frac{2}{3}\sdot5\right)\right]=\frac{4}{7}\sdot\left(\frac{5}{9}\sdot\frac{1}{8}\right)\sdot\left(\frac{3}{5}\sdot\frac{1}{9}\right)\sdot\left(\frac{2}{3}\sdot5\right)=\frac{600}{7}\sdot\frac{1}{9}\sdot\frac{1}{8}\sdot\frac{1}{5}\sdot\frac{1}{9}\sdot\frac{1}{3}}}</math> | ||
| + | {| | ||
| + | |- | ||
| + | |Know that the denominators are always [written] on top and you cannot find anything above them. By this you distinguish between the denominators and the numerators. | ||
| + | |style="width:45%; text-align:right;"|ודע שלעולם המורים עליונים ולא תמצא עליהם דבר ובזה תבחין בין המורים למספר השברים | ||
|- | |- | ||
| − | | | + | |''Although there is no proof to the matter, there is a reference to the matter'' [Mishnah, Sanhedrin 8, 2], ''to include Torah scholars'' [Talmud, Bekhorot 6, 2] - the denominators [morim, lit. teachers] should be high above all and the numerators are beneath them, as the student and his teacher, or a teacher's house that is wide open, so that whoever wishes comes. |
| − | + | |style="text-align:right;"|ואם ''א[י]ן ראיה לדבר זכר לדבר''<ref group=note>משנה סנהדרין ח, ב</ref> את ''לרבות תלמידי'' ''חכמים''<ref group=note>בבלי, קודשים, בכורות, דף ו, ב</ref> שהמורים ראויין להיות גבוהים על הכל וה{{#annot:term|570,1242|iMq3}}שברים{{#annotend:iMq3}} למטה מהם כתלמיד לפני רבו או בית פתוח לרוחה תחת המורה ויבא מי שירצה | |
| − | |style="text-align:right;"|ואם ''א[י]ן ראיה לדבר זכר לדבר''<ref> | ||
|- | |- | ||
| − | | | + | |But, the integers - there is nothing above or beneath them. Their house is not open, for they are not denominators [lit. teachers]. |
| − | |||
|style="text-align:right;"|אבל השלמים לעולם אין עליהם ולא תחתיהם דבר ולא בית פתוח כי אינם מורי הוראה | |style="text-align:right;"|אבל השלמים לעולם אין עליהם ולא תחתיהם דבר ולא בית פתוח כי אינם מורי הוראה | ||
|- | |- | ||
| | | | ||
| + | :After we reduce them, they are five-ninths of a seventh of a ninth. | ||
:<math>\scriptstyle{\color{blue}{\left[\left[\frac{4}{7}\sdot\left(\frac{5}{9}\sdot\frac{1}{8}\right)\right]\right]\times\left[\left(\frac{3}{5}\sdot\frac{1}{9}\right)\sdot\left(\frac{2}{3}\sdot5\right)\right]=\frac{600}{7}\sdot\frac{1}{9}\sdot\frac{1}{8}\sdot\frac{1}{5}\sdot\frac{1}{9}\sdot\frac{1}{3}=\frac{5}{9}\sdot\frac{1}{7}\sdot\frac{1}{9}}}</math> | :<math>\scriptstyle{\color{blue}{\left[\left[\frac{4}{7}\sdot\left(\frac{5}{9}\sdot\frac{1}{8}\right)\right]\right]\times\left[\left(\frac{3}{5}\sdot\frac{1}{9}\right)\sdot\left(\frac{2}{3}\sdot5\right)\right]=\frac{600}{7}\sdot\frac{1}{9}\sdot\frac{1}{8}\sdot\frac{1}{5}\sdot\frac{1}{9}\sdot\frac{1}{3}=\frac{5}{9}\sdot\frac{1}{7}\sdot\frac{1}{9}}}</math> | ||
|style="text-align:right;"|ואחרי עשותנו להם כלילת יופי שהן חמש תשיעיות שביעית תשיעית | |style="text-align:right;"|ואחרי עשותנו להם כלילת יופי שהן חמש תשיעיות שביעית תשיעית | ||
|- | |- | ||
| − | | | + | |This is the rule: we should not expand to a common denominator at all. All that we should do is only to relate [the fractions] to each other, which is to write "of" [the letter מ] instead of "by", as we explained, then multiply and decompose if necessary, and after all this to reduce, which is to divide by the denominators that are always on top as we explained, and the result is the required. |
| − | |style="text-align:right;"|זה הכלל שאין לנו לעשות בזה ההשואה כלל כי אין לנו לעשות כי אם לקשרם יחד והוא לשים מ' במקום על כמו שבארנו ואחר כן נעשה לה הכאה גם פריטה אם הוצרך אליה ואחר כל זה לעשות לה כלילת יופי והוא לחלקם על המורים שהרי העליונים לעולם כמו שביארנו והיוצא הוא המבוקש | + | |style="text-align:right;"|<big>זה הכלל</big> שאין לנו לעשות בזה ההשואה כלל כי אין לנו לעשות כי אם לקשרם יחד והוא לשים מ' במקום על כמו שבארנו ואחר כן נעשה לה הכאה גם פריטה אם הוצרך אליה ואחר כל זה לעשות לה כלילת יופי והוא לחלקם על המורים שהרי העליונים לעולם כמו שביארנו והיוצא ‫<ref>56r</ref>הוא המבוקש |
|} | |} | ||
{| | {| | ||
| Line 6,038: | Line 7,282: | ||
== Chapter Four: Division == | == Chapter Four: Division == | ||
| − | + | |style="width:45%; text-align:right;"|<big>הפרק הרביעי בחלוק</big> | |
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle\left[\frac{3}{4}+\left(\frac{2}{3}\sdot\frac{1}{4}\right)\right]\div\left[\left[\frac{4}{9}+\left(\frac{5}{6}\sdot\frac{1}{9}\right)\right]\sdot\frac{2}{3}\right]</math> | + | *{{#annot:(¾+⅔·¼)÷(⁴/₉+⅚·⅑)·⅔|552|t3ba}}We wish to divide three-quarters and 2-thirds of a quarter by 4-ninths and 5-sixths of a ninth of 2-thirds. |
| − | |style="text-align:right;"|רצינו לחלק שלש רביעיות וב' שלשיות רביעית על ד' תשיעיות וה' ששיות תשיעית מב' שלישיות | + | :<math>\scriptstyle\left[\frac{3}{4}+\left(\frac{2}{3}\sdot\frac{1}{4}\right)\right]\div\left[\left[\frac{4}{9}+\left(\frac{5}{6}\sdot\frac{1}{9}\right)\right]\sdot\frac{2}{3}\right]</math> |
| + | |style="text-align:right;"|רצינו לחלק שלש רביעיות וב' שלשיות רביעית על ד' תשיעיות וה' ששיות תשיעית מב' שלישיות{{#annotend:t3ba}} | ||
|- | |- | ||
| | | | ||
| Line 6,073: | Line 7,318: | ||
|- | |- | ||
| | | | ||
| − | :The dividend | + | :The large number [= the dividend] requires only decomposing. The result after the decomposing is 11-thirds of a quarter. |
::<math>\scriptstyle{\color{blue}{\frac{3}{4}+\left(\frac{2}{3}\sdot\frac{1}{4}\right)=\frac{11}{3}\sdot\frac{1}{4}}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{3}{4}+\left(\frac{2}{3}\sdot\frac{1}{4}\right)=\frac{11}{3}\sdot\frac{1}{4}}}</math> | ||
|style="text-align:right;"|והרב אינו צריך כי אם פריטה ויעלה אחר הפריטה י"א שלישיות רביעיות | |style="text-align:right;"|והרב אינו צריך כי אם פריטה ויעלה אחר הפריטה י"א שלישיות רביעיות | ||
|- | |- | ||
| | | | ||
| − | :The | + | :The small number [= the divisor] requires decomposing and multiplication. The result after the decomposing and multiplication is 58-sixths of a ninth of a third. |
::<math>\scriptstyle{\color{blue}{\left[\frac{4}{9}+\left(\frac{5}{6}\sdot\frac{1}{9}\right)\right]\sdot\frac{2}{3}=\frac{58}{6}\sdot\frac{1}{9}\sdot\frac{1}{3}}}</math> | ::<math>\scriptstyle{\color{blue}{\left[\frac{4}{9}+\left(\frac{5}{6}\sdot\frac{1}{9}\right)\right]\sdot\frac{2}{3}=\frac{58}{6}\sdot\frac{1}{9}\sdot\frac{1}{3}}}</math> | ||
|style="text-align:right;"|אכן המעט צריך פריטה והכאה ויעלה אחר הפריטה וההכאה נ"ח ששיות תשיעית שלישית | |style="text-align:right;"|אכן המעט צריך פריטה והכאה ויעלה אחר הפריטה וההכאה נ"ח ששיות תשיעית שלישית | ||
|- | |- | ||
| − | |After | + | |After decomposing and multiplying each of them, if needed, we expand them to a common denominator. |
|style="text-align:right;"|ואחר שעשינו לכל אחד מהם אשר הוצרך מפריטה והכאה נשוום יחד | |style="text-align:right;"|ואחר שעשינו לכל אחד מהם אשר הוצרך מפריטה והכאה נשוום יחד | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{3}{4}+\left(\frac{2}{3}\sdot\frac{1}{4}\right)=\frac{11}{3}\sdot\frac{1}{4}=\frac{594}{6}\sdot\frac{1}{9}\sdot\frac{1}{3}\sdot\frac{1}{4}}}</math> | + | ::Since the 3 is once in both of them, we do not multiply them by it. |
| + | |style="text-align:right;"|ואחרי היות הג' בשתיהן פעם אחת לא נכפלם בו | ||
| + | |- | ||
| + | | | ||
| + | ::The large number becomes 594 after the expansion. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{3}{4}+\left(\frac{2}{3}\sdot\frac{1}{4}\right)=\frac{11}{3}\sdot\frac{1}{4}=\frac{594}{6}\sdot\frac{1}{9}\sdot\frac{1}{3}\sdot\frac{1}{4}}}</math> | ||
| + | |style="text-align:right;"|ויעלה הרב אחר ההשואה 594 | ||
| + | |- | ||
| + | | | ||
| + | ::The small number becomes 232. | ||
::<math>\scriptstyle{\color{blue}{\left[\frac{4}{9}+\left(\frac{5}{6}\sdot\frac{1}{9}\right)\right]\sdot\frac{2}{3}=\frac{58}{6}\sdot\frac{1}{9}\sdot\frac{1}{3}=\frac{232}{6}\sdot\frac{1}{9}\sdot\frac{1}{3}\sdot\frac{1}{4}}}</math> | ::<math>\scriptstyle{\color{blue}{\left[\frac{4}{9}+\left(\frac{5}{6}\sdot\frac{1}{9}\right)\right]\sdot\frac{2}{3}=\frac{58}{6}\sdot\frac{1}{9}\sdot\frac{1}{3}=\frac{232}{6}\sdot\frac{1}{9}\sdot\frac{1}{3}\sdot\frac{1}{4}}}</math> | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והמעט יעלה 232 |
| − | |||
| − | והמעט יעלה 232 | ||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | ::These two numbers are fractions of four denominators, which are sixths of a ninth of a third of a quarter. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והם ר"ל שני המספרים האלו שברים מהד' מורים שהם שישיות תשיעית שלישית רביעית |
| − | |||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :Since they are expanded, it is as if we were asked to divide 594-sixths of a ninth of a third of a quarter, which is as if we are told to divide 594 integers by 232. |
| + | |style="text-align:right;"|ואחרי היותם שוות הרי הוא כאלו שאלו לנו שנחלק 594 שישיות תשיעיות שלישית רביעית והרי הוא כאלו אמרו לנו נחלק 594 שלמים על 232 | ||
| + | |} | ||
| + | :<math>\scriptstyle{\color{blue}{\left[\frac{3}{4}+\left(\frac{2}{3}\sdot\frac{1}{4}\right)\right]\div\left[\left[\frac{4}{9}+\left(\frac{5}{6}\sdot\frac{1}{9}\right)\right]\sdot\frac{2}{3}\right]=\left(\frac{594}{6}\sdot\frac{1}{9}\sdot\frac{1}{3}\sdot\frac{1}{4}\right)\div\left(\frac{232}{6}\sdot\frac{1}{9}\sdot\frac{1}{3}\sdot\frac{1}{4}\right)=\frac{594}{232}}}</math> | ||
| + | {| | ||
|- | |- | ||
| − | | | + | |For, since they are of the same type, what do I care if they are integer or fractions, or zuzim or peraḥim, after all this operation is exactly the same as division of integers. |
| − | |style="text-align:right;"| | + | |style="width:45%; text-align:right;"|כי אחר שהם ממין אחד מה לי אם הם שלמים או שברים או זוזים או פרחים והרי מעשהו שוה לחלוקת השלמים שוה בשוה |
|- | |- | ||
| − | | | + | |In order that we will receive fractions and integers together, we do not use the method of unifying, but the method of extracting the divisors, that is we extract the divisors of the number by which we want to divide and this is the small number in our example. |
| − | |style="text-align:right;"|ואל תטעה לחשוב כי מוריו אשר עליו הם המורים לחלקי הפריטה אחר ההשואה ושאלו הם המורים אשר לך לבקש ולחלק עליהם כי זה אינו כלל ואין לך לחלק עליהם כי אם בעשותך כלילת יופי | + | |style="text-align:right;"|<big>וכדי</big> שיצאו לנו שברים ושלמים יחד לא נביאנו על דרך האחדות כי אם ע"ד הוצאת המורים והוא שנוציא מורי המספר אשר רצינו לחלק עליו והוא המספר המעט אשר במשלינו |
| + | |- | ||
| + | |However, do not be mistaken in thinking that its denominators that are above it are the divisors of the decomposed after the expansion and that these are the divisiors that you should seek for and divide by them, for this is not the case at all. You should divide by them only when you reduce. | ||
| + | |style="text-align:right;"|ואל תטעה לחשוב כי מוריו אשר עליו <s>מוריו אשר עליו</s> הם המורים לחלקי הפריטה אחר ההשואה ושאלו ‫<ref>56v</ref>הם המורים אשר לך לבקש ולחלק <sup>עליהם</sup> כי זה אינו כלל ואין לך לחלק עליהם כי אם בעשותך כלילת יופי | ||
|- | |- | ||
| − | | | + | |The divisors that you should seek for, means to know if the 232, which is the numerator of the number by which we want to divide the other numerator, is prime or composite, or of which numbers it consists. |
| − | |style="text-align:right;"|אבל המורים אשר לך לבקש הוא לדעת ה232 שהוא מספר השברים אשר רצינו לחלק עליהם מספר השברים האחרים אם הוא פשוט או מורכב או מאי זה מספרים הוא מורכב | + | |style="text-align:right;"|אבל המורים אשר לך לבקש הוא לדעת ה232 שהוא מספר השברים אשר רצינו לחלק עליהם מספר השברים האחרים אם הוא פשוט או מורכב <sup>או</sup> מאי זה מספרים הוא מורכב |
|- | |- | ||
| | | | ||
| − | : | + | :Know that after you multiplied the result of decomposing by 4 in the expansion procedure, it is known that the product has a quarter [= 4 is its divisor], as well as all the denominators of the other [that were not among its own denominators]. |
|style="text-align:right;"|ודע לך שאחר שכפלת והעולה בפריטתה בד' בעת ההשואה בידוע שיש לה רביעית וכן כל המורים אשר היו בחברתה ולא בה | |style="text-align:right;"|ודע לך שאחר שכפלת והעולה בפריטתה בד' בעת ההשואה בידוע שיש לה רביעית וכן כל המורים אשר היו בחברתה ולא בה | ||
|- | |- | ||
| − | | | + | |Therefore, if you want to make the procedure easier for you, do not multiply by them and then you will not need to divide by them, when extracting the divisors. But, take them as denominators, by which you divide, with the resulting decomposed. Seek for the divisors of the resulting decomposed and write them with them. |
| − | |style="text-align:right;"|לכן אם תרצה להקל מעליך המעשה לא תכפלנו בהם ולא תצטרך לחלקה עתה להם בעת הוצאת המורים אבל תקחם למורים שתחלק עליהם ועל היוצא מפריטתה ותבקש מורי המספר היוצא מפריטתה ותבקש ותשימם עמהם | + | |style="text-align:right;"|<big>לכן</big> אם תרצה להקל מעליך המעשה לא תכפלנו בהם ולא תצטרך לחלקה עתה להם בעת הוצאת המורים אבל תקחם למורים שתחלק עליהם ועל היוצא מפריטתה ותבקש מורי המספר היוצא מפריטתה <s>ותבקש</s> ותשימם עמהם |
| + | |- | ||
| + | |All this was said about the number, by which you want to divide, but as for the number that you want to divide, you always need to multiply it by the denominators that are in the other [that were not among its own denominators]. | ||
| + | |style="text-align:right;"|וכל זה אמרנו במספר אשר תרצה לחלק עליו <s>אבל המספר אשר תרצה לחלק עליו</s> אבל המספר אשר תרצה לחלק צריך אתה לעולם לכפלו במורים אשר בחברתה ולא בה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Example: in our example, if we want to seek for the divisors of 232, since the result of the decomposing and multiplication was multiplied in the expansion operation by 4, which is the denominator of the other, we know that the product has a quarter. We divide it by 4 and the result of division is 58. |
| + | |style="text-align:right;"|<big>המשל</big> לזה במשלינו כי אם רצינו לבקש מורים ל232 ואחר שבעת ההשואה הוכפל היוצא מהפריטה וההכאה בד' שהיא מורה חברתה ידענו שלזה העולה יש לו רביעית ונחלקנו על ד' ויצא בחילוק נ"ח | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We seek also for the divisors of 58. We find that it has a half. We divide it by it, i.e. by 2; the result of division is 29 and this is a prime number. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונבקש עוד מורים לנ"ח ונמצא לו חצי ונחלקנו עליו ר"ל ר"ל על ב' ויצא בחילוק כ"ט והוא מספר פשוט |
| − | |||
| − | ונבקש עוד מורים לנ"ח ונמצא לו חצי ונחלקנו עליו ר"ל על ב' ויצא בחילוק כ"ט והוא מספר פשוט | ||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::So, the divisors of the numerator, by which we want to divide, are 2, 4, 29. |
| + | ::<math>\scriptstyle{\color{blue}{232=4\sdot58=4\sdot2\sdot29}}</math> | ||
| + | |style="text-align:right;"|הנה מורי מספר השברים אשר רצינו לחלק עליהם הם הב' והד' והכ"ט | ||
|- | |- | ||
| − | | | + | |In that you see clearly what I said that if we would have wanted to make the procedure easier for us, we would have taken the 4 as a first denominator from the start. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ובזה תראה ברור מה שאמרתי שאם הינו רוצים להקל המעשה מעלינו היינו לוקחים מתחלה <s>מתחלה</s> הד' למורה ראשון |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ר"ל הנ"ח אבל נבקש מורים לנ"ח או לשים אותה עצמה למורה ולשים עמהם הד' והכל אחד | + | ::Even if they are numerous, we do not have to multiply by them the number, by which we want to divide, i.e. the 58. But, we seek for the divisors of 58, or write it by itself as a denominator, and write the 4 with it. All is the same. |
| + | |style="text-align:right;"|וכן אם היה שם הרבה ולא היינו צריכים לכפול בהם המספר אשר רצינו לחלק עליו ‫<ref>57r</ref>ר"ל הנ"ח אבל נבקש מורים לנ"ח או לשים אותה עצמה למורה ולשים עמהם הד' והכל אחד | ||
|- | |- | ||
| − | |The reason for this | + | |The reason for this is clear, because multiplication and division are inverse operations. |
|style="text-align:right;"|והטעם ברור כי הכפל והחלוקה הפכים הם | |style="text-align:right;"|והטעם ברור כי הכפל והחלוקה הפכים הם | ||
|- | |- | ||
| | | | ||
| − | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a\sdot b}{b}=a}}</math> | |
| − | :<math>\scriptstyle{\color{ | + | | |
| − | | | ||
|- | |- | ||
| | | | ||
| + | ::If we multiply a number, i.e. the 58, by any number, i.e. by the 4, and then we divide the product by this same number, i.e. by the 4, we get what we had at the beginning, i.e. the 58. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{58\sdot4}{4}=58}}</math> | ||
| + | |style="text-align:right;"|ואם נכפול מספר ר"ל הנ"ח על מספר מה ר"ל הד' ונחלק העולה לזה המספר בעצמו ר"ל לד' יצא לנו אשר היה לנו בתחלה ר"ל הנ"ח | ||
| + | |- | ||
| + | |The operation is the same, but the procedure is easier. | ||
| + | |style="text-align:right;"|והמעשה עולה אחד והמלאכת יותר קלה | ||
| + | |- | ||
| + | |As long as you do not make a mistake in multiplying the number that you want to divide, i.e. the 11, by the denominators of the other, because it is always required. | ||
|style="text-align:right;"|ובלבד שלא תטעה מלכפול המספר אשר רצית לחלק ר"ל הי"א במורי חברתה כי זה מחוייב לעולם | |style="text-align:right;"|ובלבד שלא תטעה מלכפול המספר אשר רצית לחלק ר"ל הי"א במורי חברתה כי זה מחוייב לעולם | ||
|- | |- | ||
| − | |<span style=color:red>[it is not clear why the divisors 4, 2, 29 were replaced here by 3, 2, 29]</span> | + | | |
| + | ::We complete the example: we divide the 594 by the three denominators that we received, one after another. | ||
| + | |style="text-align:right;"|ונשלים המשל ונחלק ה594 על הג' מורים שיצאו לנו זה אחר זה | ||
| + | |- | ||
| + | | | ||
| + | ::We divide it first by 3; the result of division is 3 integers and nothing remains. We write them aside. | ||
| + | |style="text-align:right;"|ונחלקם תחלה לג' ויצא בחילוק ג' והם שלמי' ולא ישאר דבר ונשימם מחוץ | ||
| + | |- | ||
| + | |<span style=color:red>[it is not clear why the divisors 4, 2, 29 were replaced here by 3, 2, 29:]</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :The outcome is that when we divide the greater number by smaller number mentioned in the example, the result of division is 3 integers and 24 parts of 29 parts of one half of the whole. | ||
:<math>\scriptstyle{\color{blue}{\frac{594}{3\sdot2\sdot29}=3+\left(\frac{24}{29}\sdot\frac{1}{2}\right)}}</math> | :<math>\scriptstyle{\color{blue}{\frac{594}{3\sdot2\sdot29}=3+\left(\frac{24}{29}\sdot\frac{1}{2}\right)}}</math> | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הנה היוצא הוא כי בחלקנו המספר הרב למעט הנזכרים במשל שיצא בחילוק ג' שלמים וכ"ד חלקים מכ"ט חלקים מחצי שלם |
| − | הנה היוצא הוא כי בחלקנו המספר הרב למעט הנזכרים במשל שיצא בחילוק ג' שלמים וכ"ד חלקים מכ"ט חלקים מחצי שלם | ||
|- | |- | ||
| | | | ||
| + | ::I.e. that the smaller number is three times in the greater number. | ||
|style="text-align:right;"|ור"ל שהמספר המעט הוא ברב ג' פעמי‫' | |style="text-align:right;"|ור"ל שהמספר המעט הוא ברב ג' פעמי‫' | ||
|- | |- | ||
| | | | ||
| + | ::This is the meaning of the three integers. If they were two integers it would have meant that it is twice in it. If they were more, it were more [times in it]. | ||
|style="text-align:right;"|וזה ר"ל השלשה שלמים ואם יהיו שנים שלמים ירצה לומר שהוא בו שתי פעמים ואם יותר יותר | |style="text-align:right;"|וזה ר"ל השלשה שלמים ואם יהיו שנים שלמים ירצה לומר שהוא בו שתי פעמים ואם יותר יותר | ||
|- | |- | ||
| | | | ||
| + | ::The fractions mean that it is in it additional parts of time as aforesaid, which are not a whole time. | ||
|style="text-align:right;"|והשברים ר"ל שהם עוד בו חלקי פעם כנזכר ולא היה פעם שלמה כלל | |style="text-align:right;"|והשברים ר"ל שהם עוד בו חלקי פעם כנזכר ולא היה פעם שלמה כלל | ||
|- | |- | ||
| − | | | + | |When the question is so, i.e. that we divide small fractions by numerous greater fractions, we can do this by the method of unification after we decompose, multiply, and expand [to a common denominator]. |
| − | |style="text-align:right;"|וכאשר השאלה כן ר"ל שהם עוד בו חלקי פעם כנזכר ולא היה פעם שלמה כלל וכאשר השאלה כן ר"ל שנחלק שברים קטנים לשברים רבים וגדולים מהם נוכל לעשות בדרך האחדות אחרי עשותנו הפריטה וההכאה וההשוואה | + | |style="text-align:right;"|וכאשר השאלה כן <s>ר"ל שהם עוד בו חלקי פעם כנזכר ולא היה פעם שלמה כלל וכאשר השאלה כן</s> ר"ל שנחלק שברים קטנים לשברים רבים וגדולים מהם נוכל לעשות בדרך האחדות אחרי עשותנו הפריטה וההכאה וההשוואה |
|- | |- | ||
| − | | | + | |This is the rule: after we decompose and multiply each of them, or whichever is needed, then expand them as mentioned, we divide the [numerator] resulting in this by the [numerator] resulting in that, as integers. All the resulting integers are the number of times [that the divisor appears in the dividend] and the fractions are the [additional] parts of one time [that the divisor appears in the dividend]. All is clear. |
| − | |style="text-align:right;"|זה הכלל שאחר עשותנו הפריטה וההכאה וההשואה לכל אחד מהם | + | |style="text-align:right;"|<big>זה הכלל</big> שאחר עשותנו הפריטה וההכאה <s>וההשואה</s> לכל אחד מהם או אשר תצטרך ואחר כך ההשוואה כנזכר נחלק היוצא בזו ליוצא באחרת ככל דרכם השלמים מכל וכל והשלמים היוצאים יהיו מספר הפעמים והשברים חלקי פעם והכל ברור |
|- | |- | ||
| − | | | + | |The proofs of all the preceding and the following chapters on fractions are the same as the proofs for integers, i.e. each to its the inverse operations: addition and subtraction to each other; division and multiplication to each other. The proofs of the proportions and roots [of fractions] are also the same as for integers. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומופתי כל פרקי השברים העוברים והבאים הם כמופתי השלמים ר"ל כל דבר להפכו החבור והחסרון זה לזה והחלוק והכפל זה לזה גם בערכים ובשרשים מופתיהם ‫<ref>57v</ref>כמופתי השלמים |
|- | |- | ||
|} | |} | ||
| Line 6,183: | Line 7,465: | ||
| | | | ||
:Or, if [a given number of] portions of gold are equal to [a certain number of] portions of silver, how many portions of silver worth another [number of] portions of gold? | :Or, if [a given number of] portions of gold are equal to [a certain number of] portions of silver, how many portions of silver worth another [number of] portions of gold? | ||
| − | |style="text-align:right;"|או אם אלו השברים מזהב ע'ד'מ' שוים אלו של כסף אחרות אלו של זהב כמה שוים [אלו] של כסף | + | |style="text-align:right;"|או אם אלו השברים מזהב ע'ד'מ' שוים אלו של כסף אחרות אלו של זהב כמה שוים [אלו]‫<ref>marg.</ref> של כסף |
|- | |- | ||
| | | | ||
| Line 6,192: | Line 7,474: | ||
|style="text-align:right;"|כל זהו כמו בשלימים | |style="text-align:right;"|כל זהו כמו בשלימים | ||
|- | |- | ||
| − | | | + | |Its procedure: the rule requires that we decompose and multiply each of the three numbers separately; then multiply the first of these by the second of these without expansion at all; then expand what resulted now that does not have the denominators of the other, i.e. to multiply the mentioned product by the denominators of what remains, which is the first or the second and so on, meaning to multiply the third by the denominators of the two that are the denominators of the mentioned product; finally to divide the [numerator] of the product after it was expanded by the [numerator of the] third after it was expanded. |
| − | <math>\scriptstyle\frac{a_1}{b_1}:\frac{a_2}{b_2}=X:\frac{a_3}{b_3}\longrightarrow X=\frac{\frac{a_1}{b_1}\sdot\frac{a_3}{b_3}}{\frac{a_2}{b_2}}=\frac{\left(a_1\sdot a_3\right)\sdot b_2}{a_2\sdot\left(b_1\sdot b_3\right)}</math> | + | |style="text-align:right;"|ומעשהו היה הדין נותן שנעשה פריטה והכאה לכל אחד מהג' מספרים לעצמו<br> |
| − | |style="text-align:right;"| | + | ולכפול ר"ל להכות הראשון מאלו [בב' מאלו]‫<ref>marg.</ref> מבלי השואה כלל ויהיה היוצא חלקים ממורי שני מספרים אלו<br> |
| − | + | ולהשוות זה העולה עתה שאין חלקים ממוריו גם ממורה חברתה אשר הוכפלה בה עם הנשאר ר"ל לכפול זה העולה מהכפל הנזכר במור<sup>י</sup>ה הנשאר שהוא ראשון או שני וכן כלם זה פירושם גם לכפול השלישית במורי השנים שהם מורי זה העולה מהכפל כנזכר<br> | |
| − | + | ואחר שכל זה לחלק זה העולה אחר שהושווה לשלישי אחר שהושווה | |
| − | + | |- | |
| + | | | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a_1}{b_1}:\frac{a_2}{b_2}=X:\frac{a_3}{b_3}\longrightarrow X=\frac{\frac{a_1}{b_1}\sdot\frac{a_3}{b_3}}{\frac{a_2}{b_2}}=\frac{\left(a_1\sdot a_3\right)\sdot b_2}{a_2\sdot\left(b_1\sdot b_3\right)}}}</math> | ||
| + | | | ||
| + | |- | ||
| + | |The integers resulting in the division are proper integers of the unknown and the fractions are fractions of the whole. | ||
| + | |style="text-align:right;"|והיו השלמים היוצאים בחילוק שלמים ממש מהנעלם והשברים שבר שלם | ||
|- | |- | ||
| | | | ||
| − | *Example: if 3-quarters of 3 integers minus one-quarter are equal to 4-fifths of 5 integers minus one-fifth, how much five-sixths of 6 integers minus one-sixth are equal? | + | *{{#annot:¾·(3-¼)÷⅘·(5-⅕)=⅚·(6-⅙)÷X|567|ELCt}}Example: if 3-quarters of 3 integers minus one-quarter are equal to 4-fifths of 5 integers minus one-fifth, how much five-sixths of 6 integers minus one-sixth are equal? |
:<math>\scriptstyle\left[\frac{3}{4}\sdot\left(3-\frac{1}{4}\right)\right]:\left[\frac{4}{5}\sdot\left(5-\frac{1}{5}\right)\right]=\left[\frac{5}{6}\sdot\left(6-\frac{1}{6}\right)\right]:X</math> | :<math>\scriptstyle\left[\frac{3}{4}\sdot\left(3-\frac{1}{4}\right)\right]:\left[\frac{4}{5}\sdot\left(5-\frac{1}{5}\right)\right]=\left[\frac{5}{6}\sdot\left(6-\frac{1}{6}\right)\right]:X</math> | ||
| − | |style="text-align:right;"|המשל אם ג' רביעיות מג' שלמים פחות רביע שלם שוים ד' חמישיות מה' שלימים פחות חומש שלם חמש שישיות מו' שלימים פחות שישית שלם כמה שוים | + | |style="text-align:right;"|<big>המשל</big> אם ג' רביעיות מג' שלמים פחות רביע שלם שוים ד' חמישיות מה' שלימים פחות חומש שלם חמש שישיות מו' שלימים פחות שישית שלם כמה שוים{{#annotend:ELCt}} |
|- | |- | ||
| | | | ||
| Line 6,243: | Line 7,531: | ||
::Also, of 5 [integers] minus one-fifth is as of 4 integers and 4-fifths. | ::Also, of 5 [integers] minus one-fifth is as of 4 integers and 4-fifths. | ||
::<math>\scriptstyle{\color{blue}{\frac{4}{5}\sdot\left(5-\frac{1}{5}\right)=\frac{4}{5}\sdot\left(4+\frac{4}{5}\right)}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{4}{5}\sdot\left(5-\frac{1}{5}\right)=\frac{4}{5}\sdot\left(4+\frac{4}{5}\right)}}</math> | ||
| − | |style="text-align:right;"|וכן מהה' פחות חומש הוא כמו מד' שלמים וד' חמישיות משלם | + | |style="text-align:right;"|וכן מהה' פחות חומש הוא כמו מד' ‫<ref>58r</ref>שלמים וד' חמישיות משלם |
|- | |- | ||
| | | | ||
| Line 6,263: | Line 7,551: | ||
| | | | ||
::Thus, it is as if one says: 3-quarters of 11-quarters, so we multiply 11 by 3; the result is 33, which are 33-quarters of a quarter. | ::Thus, it is as if one says: 3-quarters of 11-quarters, so we multiply 11 by 3; the result is 33, which are 33-quarters of a quarter. | ||
| − | |style="text-align:right;"|והרי הוא כאלו אמרו ג' רביעיות מי"א רביעיות לכן נכה הי"א בג' יעלו ל"ג הלא הם ל"ג | + | |style="text-align:right;"|והרי הוא כאלו אמרו ג' רביעיות מי"א רביעיות לכן נכה הי"א בג' יעלו ל"ג הלא הם ל"ג רביעי<sup>ו</sup>ת רביעית |
|} | |} | ||
::<math>\scriptstyle{\color{blue}{\frac{3}{4}\sdot\left(3-\frac{1}{4}\right)=\frac{3}{4}\sdot\left(2+\frac{3}{4}\right)=\frac{3}{4}\sdot\frac{\left(2\sdot4\right)+3}{4}=\frac{3}{4}\sdot\frac{8+3}{4}=\frac{3}{4}\sdot\frac{11}{4}=\frac{3\sdot11}{4}\sdot\frac{1}{4}=\frac{33}{4}\sdot\frac{1}{4}}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{3}{4}\sdot\left(3-\frac{1}{4}\right)=\frac{3}{4}\sdot\left(2+\frac{3}{4}\right)=\frac{3}{4}\sdot\frac{\left(2\sdot4\right)+3}{4}=\frac{3}{4}\sdot\frac{8+3}{4}=\frac{3}{4}\sdot\frac{11}{4}=\frac{3\sdot11}{4}\sdot\frac{1}{4}=\frac{33}{4}\sdot\frac{1}{4}}}</math> | ||
| Line 6,269: | Line 7,557: | ||
|- | |- | ||
| | | | ||
| + | ::We do the same with the second; the result is 96-fifths of a fifth. | ||
::<math>\scriptstyle{\color{blue}{\frac{4}{5}\sdot\left(5-\frac{1}{5}\right)=\frac{4}{5}\sdot\left(4+\frac{4}{5}\right)=\frac{96}{5}\sdot\frac{1}{5}}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{4}{5}\sdot\left(5-\frac{1}{5}\right)=\frac{4}{5}\sdot\left(4+\frac{4}{5}\right)=\frac{96}{5}\sdot\frac{1}{5}}}</math> | ||
| − | |style="width:45%; text-align:right;"|וכן נעש' לשנית ויעלו 96 חמישיות חמישית | + | |style="width:45%; text-align:right;"|וכן נעש' לשנית ויעלו 96<s>175</s> חמישיות חמישית |
|- | |- | ||
| | | | ||
| + | ::Also with the first of the latter; the result is 175-sixths of a sixth. | ||
::<math>\scriptstyle{\color{blue}{\frac{5}{6}\sdot\left(6-\frac{1}{6}\right)=\frac{5}{6}\sdot\left(5+\frac{5}{6}\right)=\frac{175}{6}\sdot\frac{1}{6}}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{5}{6}\sdot\left(6-\frac{1}{6}\right)=\frac{5}{6}\sdot\left(5+\frac{5}{6}\right)=\frac{175}{6}\sdot\frac{1}{6}}}</math> | ||
|style="text-align:right;"|וכן לראשונה מהאחרות ויעלו 175 שישיות שישית | |style="text-align:right;"|וכן לראשונה מהאחרות ויעלו 175 שישיות שישית | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|והנה שבא הכל כאלו שאלו לנו אם 33 רביעיות רביעית שוות 96 חמישיות | + | ::It is all as if we are asked: if 33-quarters of a quarter are equal to 96-fifths [of a fifth], how much 175-sixths of a sixth are equal? |
| + | |style="text-align:right;"|והנה שבא הכל כאלו שאלו לנו אם 33 רביעיות רביעית שוות 96 חמישיות 175 שישיות שישית כמה שוות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|או הערך אשר ל33 רביעיות רביעיות אצל 96 חמישיות חמישית ל175 שישיות שישית אצל מי יש לו זה הערך | + | ::Or, the value that 33-quarters of a quarter have to 96-fifths of a fifth, to who do 175-sixths of a sixth have this value? |
| + | |style="text-align:right;"|או הערך אשר ל33 רביעיות רביעיות אצל [.] 96 חמישיות חמישית ל175 שישיות שישית אצל מי יש לו זה הערך | ||
|} | |} | ||
:<math>\scriptstyle{\color{blue}{\left[\frac{3}{4}\sdot\left(3-\frac{1}{4}\right)\right]:\left[\frac{4}{5}\sdot\left(5-\frac{1}{5}\right)\right]=\left[\frac{5}{6}\sdot\left(6-\frac{1}{6}\right)\right]:X\longleftrightarrow\left(\frac{33}{4}\sdot\frac{1}{4}\right):\left(\frac{96}{5}\sdot\frac{1}{5}\right)=\left(\frac{175}{6}\sdot\frac{1}{6}\right):X}}</math> | :<math>\scriptstyle{\color{blue}{\left[\frac{3}{4}\sdot\left(3-\frac{1}{4}\right)\right]:\left[\frac{4}{5}\sdot\left(5-\frac{1}{5}\right)\right]=\left[\frac{5}{6}\sdot\left(6-\frac{1}{6}\right)\right]:X\longleftrightarrow\left(\frac{33}{4}\sdot\frac{1}{4}\right):\left(\frac{96}{5}\sdot\frac{1}{5}\right)=\left(\frac{175}{6}\sdot\frac{1}{6}\right):X}}</math> | ||
| Line 6,286: | Line 7,578: | ||
|- | |- | ||
| | | | ||
| + | :So, we have all the numerators and the denominators decomposed, each by itself. | ||
|style="width:45%; text-align:right;"|והרי לנו כל השברים נפרטים ומורים כל אחד לבדו | |style="width:45%; text-align:right;"|והרי לנו כל השברים נפרטים ומורים כל אחד לבדו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ויש לנו לכפול הב' בראשון ר"ל הו'ט' חמישיות חמישית 571 שישית שישיות מבלי השואה כלל לפי שהוא כאומרנו 96 חמישיות חמשית מ175 שישיות שישית ונכם זה בזה ר"ל מספר השברים בשברים לא במורים יעלו 16800 חמישיות חמישית ששית ששית | + | ::Now, we have to multiply the second by the first, i.e. the 96-fifths of a fifth by 571-sixths [of a sixth] without expanding at all, since it is as our saying: 96-fifths of a fifth of 175-sixths of a sixth. We multiply them by each other, i.e. the numerator by the numerator, not by the denominators; the result is 16800-fifths of a fifth of a sixth of a sixth. |
| + | |style="text-align:right;"|ויש לנו לכפול הב' בראשון ר"ל הו'ט' חמישיות חמישית 571 <s>שישית</s> שישיות מבלי השואה כלל לפי שהוא כאומרנו 96 חמישיות חמשית מ175 שישיות שישית ונכם זה בזה ר"ל מספר השברים בשברים לא במורים יעלו 16800 חמישיות חמישית ששית ששית | ||
|- | |- | ||
| | | | ||
| + | ::We have to divide them by the first of the formers, which is 33 quarters of a quarter. | ||
|style="text-align:right;"|ויש לנו לחלקם לראשון מהאחדים שהוא ה33 רביעיות רביעית | |style="text-align:right;"|ויש לנו לחלקם לראשון מהאחדים שהוא ה33 רביעיות רביעית | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|וכבר אמרנו בפ"ד מזה החלק שאם נרצה נשוה תחלה המתחלק ואשר נחלק עליו ר"ל שנכפול ה16800 אשר אנו רוצים לחלק במורה ה33 רביעיות רביעית | + | ::We have already said in the fourth chapter of this section that if we want, we can expand the dividend and the divisor first, i.e. that we multiply the 16800 that we want to divide by the denominators of the 33 quarters of a quarter, i.e. by 4; the result is 67200. We multiply it by the 4, other denominator; the result is 268800-quarters of a quarter of a fifth [of a fifth] of a sixth [of a sixth]. |
| + | |style="text-align:right;"|וכבר אמרנו בפ"ד מזה החלק שאם נרצה נשוה תחלה המתחלק ואשר נחלק עליו ר"ל שנכפול ה16800 אשר אנו רוצים לחלק במורה ה33 רביעיות רביעית ר"ל בד' ויעלה 67200 ונכפלם בד' המורה האחר ויעלה 268800 רביעיות רביעית חמישית שישית <sup>שישית</sup> | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ונכפול ג"כ ה33 רביעיות רביעית והוא המספר אשר רצינו לחלק עליו | + | ::We also multiply the 33-quarters of a quarter, which is the number by which we want to divide, by the denominators of the dividend that are 6 and 5. |
| + | |style="text-align:right;"|ונכפול ג"כ ה33 רביעיות ‫<ref>58v</ref>רביעית והוא המספר אשר רצינו לחלק עליו במורי המספר המתחלק והם הו' והה‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ואחר שנכפלם בזה זה | + | ::After we multiply it one after another, we seek for the divisor of the total product and we divide the dividend, i.e. the 268800, by it. |
| + | |style="text-align:right;"|ואחר שנכפלם בזה <sup>זה</sup> אחר זה נבקש מורה כל העולה ונחלק עליהם המספר המתחלק ר"ל ה268800 | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ואם בקשנו לז' [לו] אלו המורים אשר נכפול בהם | + | ::If we want, we divide by these six denominators, by which we multiply, i.e. the 6 and the 5, one after another. |
| + | |style="text-align:right;"|ואם בקשנו לז' [לו]‫<ref>marg.</ref> אלו המורים אשר נכפול בהם ר"ל הו' והה' ונחלק אליהם אחד <sup>אחד</sup> | ||
|} | |} | ||
:<math>\scriptstyle{\color{blue}{X=\frac{\left(\frac{96}{5}\sdot\frac{1}{5}\right)\sdot\left(\frac{175}{6}\sdot\frac{1}{6}\right)}{\frac{33}{4}\sdot\frac{1}{4}}=\frac{\frac{16800}{5}\sdot\frac{1}{5}\sdot\frac{1}{6}\sdot\frac{1}{6}}{\frac{33}{4}\sdot\frac{1}{4}}=\frac{16800\sdot4\sdot4}{33\sdot5\sdot5\sdot6\sdot6}=\frac{67200\sdot4}{33\sdot5\sdot5\sdot6\sdot6}=\frac{268800}{33\sdot5\sdot5\sdot6\sdot6}}}</math> | :<math>\scriptstyle{\color{blue}{X=\frac{\left(\frac{96}{5}\sdot\frac{1}{5}\right)\sdot\left(\frac{175}{6}\sdot\frac{1}{6}\right)}{\frac{33}{4}\sdot\frac{1}{4}}=\frac{\frac{16800}{5}\sdot\frac{1}{5}\sdot\frac{1}{6}\sdot\frac{1}{6}}{\frac{33}{4}\sdot\frac{1}{4}}=\frac{16800\sdot4\sdot4}{33\sdot5\sdot5\sdot6\sdot6}=\frac{67200\sdot4}{33\sdot5\sdot5\sdot6\sdot6}=\frac{268800}{33\sdot5\sdot5\sdot6\sdot6}}}</math> | ||
{| | {| | ||
|- | |- | ||
| − | | | + | | |
| + | ::The result of the last division is 33, which is the number that we multiply by them one after another. Hence, why should we bother to multiply by it and to divide the product by it needlessly. | ||
|style="width:45%; text-align:right;"|יצא בחילוק האחרון ל"ג שהוא המספר אשר כפלנו בהם אחד אחד ומאחר שכן למה ניגע לבהלה לכפול בהם ולחלק העולה עליהם לבטלה | |style="width:45%; text-align:right;"|יצא בחילוק האחרון ל"ג שהוא המספר אשר כפלנו בהם אחד אחד ומאחר שכן למה ניגע לבהלה לכפול בהם ולחלק העולה עליהם לבטלה | ||
|- | |- | ||
| | | | ||
| + | ::Therefore, we do not multiply the number, by which we want to divide, i.e. the 33, by the denominators of the dividend, but we take these denominators as the first denominators and we write the 33 itself with them. | ||
|style="text-align:right;"|לכן לא נכפול המספר אשר רצינו לחלק עליו ר"ל ה33 במורי המספר המתחלק אבל נקח המורים ההם למורים ראשונים ונשים עמהם ה33 עצמו | |style="text-align:right;"|לכן לא נכפול המספר אשר רצינו לחלק עליו ר"ל ה33 במורי המספר המתחלק אבל נקח המורים ההם למורים ראשונים ונשים עמהם ה33 עצמו | ||
|- | |- | ||
| | | | ||
| + | ::Or, if we want, we seek for its divisors; they are 11 and 3, and we write them instead of it with the mentioned denominators, i.e. the denominators of the number that we want to divide. They are all: 3, 11, 5, 5, 6, 6. | ||
|style="text-align:right;"|או נרצה נבקש לו מורים ויהיו י"א ג' ונשימם במקומו עם המורים הנזכרים ר"ל מורי המספר אשר רצינו לחלק ויהיו כלם 6 6 5 5 11 3 | |style="text-align:right;"|או נרצה נבקש לו מורים ויהיו י"א ג' ונשימם במקומו עם המורים הנזכרים ר"ל מורי המספר אשר רצינו לחלק ויהיו כלם 6 6 5 5 11 3 | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ונחלק עליהם המספר המתחלק והוא 268800 וזה לעשות להם כלילת יופי | + | ::We divide the dividend, which is 268800, by them; that is we reduce them. |
| + | |style="text-align:right;"|ונחלק עליהם המספר המתחלק והוא 268800 וזה לעשות להם כלילת יופי | ||
|- | |- | ||
| | | | ||
| + | ::For, if we want, we can say that the unknown of the four proportional terms is 268800-thirds of a fifth [of a fifth] of a sixth [of a sixth] of 11 of the whole. | ||
| + | |style="text-align:right;"|כי אם רצינו יכולנו לו' שהנעלם מהארבעה הנערכים הוא 268800 שלישיות חמישית ששית מאחד עשר בשלם | ||
| + | |- | ||
| + | | | ||
| + | ::To know how much it is, we reduce it, which is that we divide it by these denominators; the result is 9 integers, a third of one part of 11 of the whole, and 4-sixths of a third of one part of 11 of the whole. | ||
|style="text-align:right;"|אכן לדעת מה המה אלה נעשה להם כלילת יופי והוא שנחלקם למורים אלו ויעלה ט' שלימים ושלשית חלק אחד מי"א בשלם וד' שישיות שלישית חלק אחד מי"א בשלם | |style="text-align:right;"|אכן לדעת מה המה אלה נעשה להם כלילת יופי והוא שנחלקם למורים אלו ויעלה ט' שלימים ושלשית חלק אחד מי"א בשלם וד' שישיות שלישית חלק אחד מי"א בשלם | ||
|- | |- | ||
| Line 6,336: | Line 7,644: | ||
{| | {| | ||
|- | |- | ||
| − | | | + | !<span style="color:Green>Check</span> |
| − | <math>\scriptstyle a_1:a_2=a_3:X\longrightarrow\begin{cases}\scriptstyle a_2=\frac{X\sdot a_1}{a_3}\\\scriptstyle a_3=\frac{X\sdot a_1}{a_2}\end{cases}</math> | + | | |
| − | | | + | |- |
| + | |If you want to check your procedure, multiply the second [of the second pair] that was unknown by the 33 quarters of a quarter, which is first of the first [pair], then divide the product by one of the remaining [numbers]; the result should be the other [remaining number] itself. If not, know that you were wrong. | ||
| + | |style="width:45%; text-align:right;"|<big>ואם</big> תרצה לבחון מעשיך כפול זה השני שהיה נעלם <sup>בל"ג</sup> רביעיות רביעית שהוא הראשון מהאחדים וחלק העולה על אחד מהנשארים ויצא האחר בעינו ואם לא דע שטעית | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{a_1:a_2=a_3:X\longrightarrow\begin{cases}\scriptstyle a_2=\frac{X\sdot a_1}{a_3}\\\scriptstyle a_3=\frac{X\sdot a_1}{a_2}\end{cases}}}</math> | ||
| + | | | ||
|- | |- | ||
| | | | ||
| + | :When we multiply it by 33-quarters of a quarter, it is as our saying: 33-quarters of a quarter of 9 integers and one-third of a part of 11 of the whole; like this: | ||
:<math>\scriptstyle{\color{blue}{\left[9+\left(\frac{1}{3}\sdot\frac{1}{11}\right)+\left(\frac{4}{6}\sdot\frac{1}{3}\sdot\frac{1}{11}\right)\right]\sdot\left(\frac{33}{4}\sdot\frac{1}{4}\right)}}</math> | :<math>\scriptstyle{\color{blue}{\left[9+\left(\frac{1}{3}\sdot\frac{1}{11}\right)+\left(\frac{4}{6}\sdot\frac{1}{3}\sdot\frac{1}{11}\right)\right]\sdot\left(\frac{33}{4}\sdot\frac{1}{4}\right)}}</math> | ||
|style="text-align:right;"|והנה כאשר נכפול זה בל"ג רביעיות רביעית הוא כאומרנו ל"ג רביעיות רביעית מט' שלמים ושלישית חלק מי"א בשלם כזה | |style="text-align:right;"|והנה כאשר נכפול זה בל"ג רביעיות רביעית הוא כאומרנו ל"ג רביעיות רביעית מט' שלמים ושלישית חלק מי"א בשלם כזה | ||
|- | |- | ||
| | | | ||
| − | | | + | |style="text-align:right;"|‫<ref>59r</ref> |
{| border="1" style="margin-left: auto; margin-right: 0px; text-align:center;" | {| border="1" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| Line 6,356: | Line 7,671: | ||
|- | |- | ||
| | | | ||
| − | : | + | :We decompose the 9 integers and the fractions that are with it; the result is [1792]-sixths of a third of 11 in the whole; like this: |
| − | |style="text-align:right;"|ונפרוט הט' שלמים והשברים אשר עמו ויעלו | + | |style="text-align:right;"|ונפרוט הט' שלמים והשברים אשר עמו ויעלו 7921 ושישיות שלישית מי"א בשלם כזה |
|- | |- | ||
| | | | ||
| Line 6,367: | Line 7,682: | ||
| 59136 | | 59136 | ||
|} | |} | ||
| + | |} | ||
| + | :<math>\scriptstyle{\color{blue}{\left[9+\left(\frac{1}{3}\sdot\frac{1}{11}\right)+\left(\frac{4}{6}\sdot\frac{1}{3}\sdot\frac{1}{11}\right)\right]\sdot\left(\frac{33}{4}\sdot\frac{1}{4}\right)=\left(\frac{{\color{red}{1792}}}{6}\sdot\frac{1}{3}\sdot\frac{1}{11}\right)\sdot\left(\frac{33}{4}\sdot\frac{1}{4}\right)=\frac{59136}{4}\sdot\frac{1}{4}\sdot\frac{1}{6}\sdot\frac{1}{3}\sdot\frac{1}{11}}}</math> | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{ | + | :We divide it by the second of latter, which is 96-fifths of a fifth; the result is the remaining, which is 175-sixths of a sixth. |
| − | |style="text-align:right;"|ונחלק לשני מהאחרות והיא 96 חמישיות חמישית ותצא הנשארת | + | :<math>\scriptstyle{\color{blue}{\frac{\left[9+\left(\frac{1}{3}\sdot\frac{1}{11}\right)+\left(\frac{4}{6}\sdot\frac{1}{3}\sdot\frac{1}{11}\right)\right]\sdot\left(\frac{33}{4}\sdot\frac{1}{4}\right)}{\frac{96}{5}\sdot\frac{1}{5}}=\frac{175}{6}\sdot\frac{1}{6}}}</math> |
| + | |style="width:45%; text-align:right;"|ונחלק לשני מהאחרות והיא 96 חמישיות חמישית ותצא הנשארת שהיא ה175 שישיות שישית | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ונכפול המספר המתחלק | + | :We multiply the dividend, i.e. 59136, by the denominators of 96 that are 5 and 5; the result is 1478400. |
| + | :<math>\scriptstyle{\color{blue}{59136\sdot5\sdot5=1478400}}</math> | ||
| + | |style="text-align:right;"|ונכפול המספר המתחלק ר"ל ה59136 במורי ה96 שהם 5 5 ויעלה 1478400 | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|וכדי שלא להכפל הענין כמו שביארנו | + | :In order not to duplicate the matter as we explained, we do not multiply the 96 by the denominator of the other, but we take them as denominators to divide by them and by the 96. |
| + | |style="text-align:right;"|וכדי שלא להכפל הענין כמו שביארנו לא נכפול ה96 במורי האחרת אבל נקחם למורים שנחלק עליה ועל ה96 | ||
|- | |- | ||
| | | | ||
| + | :If we want, we take its divisors that are 2, 8, 6 and write them with the formers. | ||
|style="text-align:right;"|ואם נרצה נקח מוריהם והם 6 8 2 ונשימם עם הראשונים | |style="text-align:right;"|ואם נרצה נקח מוריהם והם 6 8 2 ונשימם עם הראשונים | ||
|- | |- | ||
| | | | ||
| + | :Since we want to know if the result of division is 175-sixths of a sixth, it is as if we are asked: how many sixth of a sixth result from the division. | ||
|style="text-align:right;"|ולפי שאנו מבקשים לידע אם רצה בחילוק 175 שישיות שישית הרי הוא כאלו שאלו לנו כמה שישיות שישית יצא מהחלוקה | |style="text-align:right;"|ולפי שאנו מבקשים לידע אם רצה בחילוק 175 שישיות שישית הרי הוא כאלו שאלו לנו כמה שישיות שישית יצא מהחלוקה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ואם לא היו במורינו | + | :If they were not among our denominators, we would have had to multiply the whole dividend by them, to add them to the other [denominators] and to write them first, as explained above. |
| + | |style="text-align:right;"|ואם לא היו במורינו היינו צריכים לכפול כל המספר המתחלק בהם ולהוסיפם על האחרים ולשומם ראשונה כמו שנתבאר למעלה | ||
| + | |- | ||
| + | | | ||
| + | :Since they are among our denominators, we do not need to multiply by them, but only to write them first, like this: 11, 3, 4, 8, 4, 2, 6, 6, and divide by all the latter except for them. | ||
| + | |style="text-align:right;"|<s>אם</s> <sup>אכן</sup> אחרי היותם במורינו לא נצטרך לכפול בהם אבל כי נשימם ראשונה במל[..]ת כזה 6 6 2 4 8 4 3 11 ונחלק על כל האחרונים זולתם | ||
| + | |- | ||
| + | | | ||
| + | ::We see that when our division reached to 2, which is the denominator that is close to [the 6] in this diagram, if the result of the division is 175, we know that we were not wrong, because they are sixths of a sixth, provided that nothing remains written from the previous [denominators]. | ||
| + | |style="text-align:right;"|ונראה כאשר יגיע אליהם יצא בו בחלוק ר"ל בחלוקנו לב' שהו' המורה הסמוך להם בצורה זו אם יצא בחלוק 175 אז נדע שלא טעינו כי הם שישיות שישית ובלבד שלא ישאר <s>ב</s>לרשום חלוק מהעוברים | ||
| + | |- | ||
| + | | | ||
| + | ::To make it easier for us, you already know that the division by the denominators is the same as by their common denominator, so we extract the common denominator of all the denominators except for the 6 and 6 mentioned, by multiplying them one by the other and their product by another and so on; the common denominator is 8448. | ||
| + | |style="text-align:right;"|וכדי להקל מעלינו כבר ידעת כי כך הוא החלק על המורים כעל אמם ונוציא אם כל המורים זולתי ה6 6 הנזכרים וזה בכפול אותם זה בזה והעולה באחר וכן כלם ותהיה האם 8448 | ||
| + | |- | ||
| + | | | ||
| + | ::If, when we divide our number by 8448, which is the common denominator of all the denominators except for the 6 and 6, the result of division is 175 and nothing remains, we know that we were not wrong. | ||
| + | |style="text-align:right;"|ואם כאשר נחלק מספרינו על ה8448 שהיא אם המורים כלם זולתי ה66 יצא בחילוק ‫<ref>59v</ref>175 ולא ישאר דבר נדע שלא טעינו | ||
| + | |- | ||
| + | | | ||
| + | ::We find that if our number is received by multiplying this common denominator by 175, we know that we were not wrong. | ||
| + | |style="text-align:right;"|נמצא שאם היה עולה מספרינו בכפול זאת האם בה'17 נדע שלא טעינו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::To make it easier for us, because of the duplication in the division, we multiply the 175 by the common denominator, i.e. 8448, and we know if the result is our number. |
| − | + | |style="text-align:right;"|וכדי להקל מעלינו כי הכפל במעשה החילוק נכפול הה'17 באם ר"ל ב84<sup>4</sup>8 ונדע אם יצא מספרינו | |
| − | וכדי להקל מעלינו | + | |- |
| − | + | | | |
| − | + | :The truth is indeed that the product of 175 by 8448 is [1]478400 and it is our number. | |
| − | + | :<math>\scriptstyle{\color{blue}{175\sdot8448={\color{red}{1}}478400}}</math> | |
| − | והאמת כן הוא שכפל הה'17 | + | |style="text-align:right;"|והאמת כן הוא שכפל הה'17 <sup>ב</sup>8448 יעלה ‫478400 והוא מספרינו |
| + | |- | ||
| + | |Examine it. Our whole operation is true. Deduce from this. | ||
| + | |style="text-align:right;"|ובחנהו והנה כל מעשינו אמת והקש על זה | ||
| + | |- | ||
| + | |This is the rule: [to extract] the proportions of fractions is to decompose and multiply each of the three numbers separately, or which ever of these [operations] needed, then multiply the one by the other and divide [the product] by the third. | ||
| + | |style="text-align:right;"|<big>זה הכלל</big> שערכי השברים הוא לעשות לכל א' מהג' מספרים לבדו פריטה והכאה או אשר מהן יצטרך לכפול הראשון בשני ולחלקו בשלישי | ||
|- | |- | ||
| − | | | + | |The proof: to multiply the result by the unknown - if it is second [in one of the two pairs], we multiply it by the first of the other [pair]; if the unknown that we generated is first [in one of the two pairs], we multiply it by the second of the other [pair] - then we divide [the product] by one of the two remaining [numbers] and the result should be the other [remaining number]. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והמופת לכפול היוצא לנו במקום הנעלם אם הוא שני נכפלנו בראשון שאינו ראשון ואם היה <sup>ה</sup>נעלם <span style="text-decoration: line-through; text-decoration-color: red;">אם הוא שני נכפלנו בראשון שאינו ראשון לו ואם היה הנעלם</span> אשר חדשנו ראשון נכפלנו <sup>בשני</sup> שאינו שני לו ונחלקנו לאחד מהנשארים ויצא האחר |
|- | |- | ||
| − | | | + | | |
| − | <math>\scriptstyle a_1:a_2=a_3:a_4\longrightarrow\begin{cases}\scriptstyle a_1=\frac{a_2\sdot a_3}{a_4}\\\scriptstyle a_2=\frac{a_1\sdot a_4}{a_3}\\\scriptstyle a_3=\frac{a_1\sdot a_4}{a_2}\\\scriptstyle a_4=\frac{a_2\sdot a_3}{a_1}\end{cases}</math> | + | :<math>\scriptstyle{\color{OliveGreen}{a_1:a_2=a_3:a_4\longrightarrow\begin{cases}\scriptstyle a_1=\frac{a_2\sdot a_3}{a_4}\\\scriptstyle a_2=\frac{a_1\sdot a_4}{a_3}\\\scriptstyle a_3=\frac{a_1\sdot a_4}{a_2}\\\scriptstyle a_4=\frac{a_2\sdot a_3}{a_1}\end{cases}}}</math> |
| − | | | + | | |
|- | |- | ||
| − | | | + | |The reason of all this is the same as its reason for integers, since the operation is the same, therefore the reason is the same. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וטעם כל זה כטעמו בשלמים כי אם אחר שהמעשה אחד בעצמו גם הטעם אחד בעצמו |
|- | |- | ||
|} | |} | ||
| Line 6,412: | Line 7,763: | ||
== Chapter Six: Roots == | == Chapter Six: Roots == | ||
| − | + | |style="width:45%; text-align:right;"|<big>הפרק הששי בשרשים</big> | |
|- | |- | ||
|<span style=color:Green>The procedure</span> | |<span style=color:Green>The procedure</span> | ||
| Line 6,425: | Line 7,776: | ||
|- | |- | ||
|Extract the root of the whole result, as you do with integers, and the integers resulting [from the extraction of] the root are parts [= numerator] of these denominators. | |Extract the root of the whole result, as you do with integers, and the integers resulting [from the extraction of] the root are parts [= numerator] of these denominators. | ||
| − | |style="text-align:right;"|ומכל העולה הוצא השרש | + | |style="text-align:right;"|ומכל העולה הוצא השרש <sup>כ</sup>כל כמעשיך בשלימים והשלימים היוצאים בשרש הם חלקים משלם מאלו המורים |
|- | |- | ||
|Reduce them, if you wish. | |Reduce them, if you wish. | ||
| − | |style="text-align:right;"|ואם תרצה [עשה להם] כלילת יופי | + | |style="text-align:right;"|ואם תרצה [עשה להם]‫<ref>marg.</ref> כלילת יופי |
|- | |- | ||
|The fractions resulting [from the extraction of] the root are fractions of one part of all these denominators. | |The fractions resulting [from the extraction of] the root are fractions of one part of all these denominators. | ||
| Line 6,434: | Line 7,785: | ||
|- | |- | ||
| | | | ||
| − | *Example: we wish to know the root of 4-sixths of 4 integers and 5-ninths. | + | *{{#annot:√(⁴/₆·(4+⁵/₉))|439|FQUg}}Example: we wish to know the root of 4-sixths of 4 integers and 5-ninths. |
:<math>\scriptstyle\sqrt{\frac{4}{6}\sdot\left(4+\frac{5}{9}\right)}</math> | :<math>\scriptstyle\sqrt{\frac{4}{6}\sdot\left(4+\frac{5}{9}\right)}</math> | ||
| − | |style="text-align:right;"|המשל רצינו לדעת שרש ד' שישיות מד' שלמים וה' תשיעיות | + | |style="text-align:right;"|<big>המשל</big> רצינו לדעת שרש ד' שישיות מד' ‫<ref>60r</ref>שלמים וה' תשיעיות{{#annotend:FQUg}} |
|- | |- | ||
| | | | ||
| Line 6,455: | Line 7,806: | ||
| | | | ||
:The result is 94 units, which are 94 sixths of ninths: | :The result is 94 units, which are 94 sixths of ninths: | ||
| − | |style="text-align:right;"|ויצאו 94 שלימים שהם 94 שישיות תשיעיות | + | |style="text-align:right;"|<s>ויצאו 94 שלימים שהם 94 שישיות תשיעיות</s> |
|- | |- | ||
| | | | ||
| Line 6,475: | Line 7,826: | ||
::If you want to come closer to the truth, double the root, which is 94, then divide by it, in the way of the integers, that is that we divide double the 94, which is 188, by 20; the result is 9 and 8 remain. | ::If you want to come closer to the truth, double the root, which is 94, then divide by it, in the way of the integers, that is that we divide double the 94, which is 188, by 20; the result is 9 and 8 remain. | ||
::<math>\scriptstyle{\color{blue}{\frac{94\sdot2}{20}=\frac{188}{20}=9+\frac{8}{20}}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{94\sdot2}{20}=\frac{188}{20}=9+\frac{8}{20}}}</math> | ||
| − | |style="width:45%; text-align:right;"|ואם רצית להתקרב אל האמת כפול השרש שהוא 94 וחלקם עליהם<br> | + | |style="width:45%; text-align:right;"|<big>ואם</big> רצית להתקרב אל האמת כפול השרש שהוא 94 וחלקם עליהם<br> |
ויעלה בדרך האחדות והוא שנחלק כפל ה94 שהוא 188 ל20 ועלו ט' ונשארו ח‫' | ויעלה בדרך האחדות והוא שנחלק כפל ה94 שהוא 188 ל20 ועלו ט' ונשארו ח‫' | ||
|- | |- | ||
| Line 6,484: | Line 7,835: | ||
| | | | ||
::The denominator 188 is 47 [times 4], so the 12 are 12 quarters of a part of 47 of a tenth. | ::The denominator 188 is 47 [times 4], so the 12 are 12 quarters of a part of 47 of a tenth. | ||
| − | |style="text-align:right;"|ומורה ה188 והם 47 [874] והנה הי"ב הם י"ב רביעיות חלק ממ"ז בעשירית כזה | + | |style="text-align:right;"|ומורה ה188 והם <s>47</s> [874]‫<ref>marg.</ref> והנה הי"ב הם י"ב רביעיות חלק ממ"ז בעשירית כזה |
|- | |- | ||
| | | | ||
| Line 6,504: | Line 7,855: | ||
| | | | ||
::We receive that the whole root is 94, a tenth and 3 parts of 47 of a tenth and all these are parts of a sixth of a ninth as mentioned. | ::We receive that the whole root is 94, a tenth and 3 parts of 47 of a tenth and all these are parts of a sixth of a ninth as mentioned. | ||
| − | |style="width:45%; text-align:right;"|הנה עלה לנו כל השרש 94 ועשירית וג' חלקים מ47 מעשירית וכל אלו הם חלקים משישית תשיעית כנזכר | + | |style="width:45%; text-align:right;"|הנה עלה לנו כל השרש 94 ועשירית וג' חלקים מ47 מעשירית וכל אלו <sup>הם</sup> חלקים משישית תשיעית כנזכר |
|- | |- | ||
| | | | ||
| Line 6,524: | Line 7,875: | ||
| | | | ||
::We reduce the 94; the result is 1 whole, which is a true integer, and 6-ninths, which are two-thirds, and 4-ninths of sixths, we also have a tenth of a sixth of a ninth, and 3 parts of 47 of a tenth of a sixth of a ninth. Like this: | ::We reduce the 94; the result is 1 whole, which is a true integer, and 6-ninths, which are two-thirds, and 4-ninths of sixths, we also have a tenth of a sixth of a ninth, and 3 parts of 47 of a tenth of a sixth of a ninth. Like this: | ||
| − | |style="width:45%; text-align:right;"|ונעשה כלילת יופי ל94 ויעלה א' לשלם וזהו שלם באמת ועוד ו' תשיעיות שהם שני שלישיות ועוד ד' תשיעיות שישיות ויש לנו עוד עמהם עשירית שישית תשיעית וג' חלקים ממ"ז מעשירית ששית תשיעית כזה | + | |style="width:45%; text-align:right;"|ונעשה כלילת יופי ל94 ויעלה א' לשלם וזהו שלם באמת ועוד ו' תשיעיות שהם שני שלישיות ועוד ד' תשיעיות שישיות ויש לנו עוד עמהם עשירית שישית <sup>תשיעית</sup> וג' חלקים ממ"ז מעשירית ששית תשיעית כזה |
|} | |} | ||
:<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\sqrt{\frac{4}{6}\sdot\left(4+\frac{5}{9}\right)}\approx\left(\frac{94}{6}\sdot\frac{1}{9}\right)+\left(\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)+\left(\frac{3}{47}\sdot\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)&\scriptstyle=1+\frac{6}{9}+\left(\frac{4}{6}\sdot\frac{1}{9}\right)+\left(\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)+\left(\frac{3}{47}\sdot\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)\\&\scriptstyle=1+\frac{2}{3}+\left(\frac{4}{6}\sdot\frac{1}{9}\right)+\left(\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)+\left(\frac{3}{47}\sdot\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)\\\end{align}}}</math> | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\sqrt{\frac{4}{6}\sdot\left(4+\frac{5}{9}\right)}\approx\left(\frac{94}{6}\sdot\frac{1}{9}\right)+\left(\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)+\left(\frac{3}{47}\sdot\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)&\scriptstyle=1+\frac{6}{9}+\left(\frac{4}{6}\sdot\frac{1}{9}\right)+\left(\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)+\left(\frac{3}{47}\sdot\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)\\&\scriptstyle=1+\frac{2}{3}+\left(\frac{4}{6}\sdot\frac{1}{9}\right)+\left(\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)+\left(\frac{3}{47}\sdot\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)\\\end{align}}}</math> | ||
| Line 6,542: | Line 7,893: | ||
|style="text-align:right;"|וזהו השרש הקרוב | |style="text-align:right;"|וזהו השרש הקרוב | ||
|- | |- | ||
| − | |<span style=color:Green>Check | + | | |
| + | === <span style=color:Green>Check</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| | | | ||
::If you want to check it, multiply it by itself and see if it gets close to the sought after, which is 1[64] sixths of a ninth plus one, as the square of the fractions that we added to the first approximate root of the fractions that we extracted. | ::If you want to check it, multiply it by itself and see if it gets close to the sought after, which is 1[64] sixths of a ninth plus one, as the square of the fractions that we added to the first approximate root of the fractions that we extracted. | ||
| − | |style="width:45%; text-align:right;"|ואם תרצה לבחון אותו כפול אותו על עצמו וראה אם {{#annot:term|1612,1874|Y7il}}יתקרב לנשאל{{#annotend:Y7il}} שהוא 146 שישיות תשיעית בתוספת אחד בכמו מרובע השברים אשר הוספנו על שרש השברים הראשון אשר הוצאנו | + | |style="width:45%; text-align:right;"|<big>ואם</big> תרצה לבחון אותו כפול אותו על עצמו וראה אם {{#annot:term|1612,1874|Y7il}}יתקרב לנשאל{{#annotend:Y7il}} שהוא 146 שישיות תשיעית ‫<ref>60v</ref>בתוספת אחד בכמו מרובע השברים אשר הוספנו על שרש השברים הראשון אשר הוצאנו |
|- | |- | ||
| | | | ||
| Line 6,569: | Line 7,922: | ||
| | | | ||
::When we multiply it by itself, it is as if we say: 44230 parts of 47 of a tenth of a sixth of a ninth of 44230 parts of 47 of a tenth of a sixth of a ninth, like this: | ::When we multiply it by itself, it is as if we say: 44230 parts of 47 of a tenth of a sixth of a ninth of 44230 parts of 47 of a tenth of a sixth of a ninth, like this: | ||
| − | |style="width:45%; text-align:right;"|וכאשר נכפול זה על עצמו הוא כאומרנו 44230 חלקים מחלק מ"ז מעשירית שישית תשיעית ‫[מ442300 חלקים מחלק מ"ז מעשירית ששית תשיעית כזה]‫ כזה | + | |style="width:45%; text-align:right;"|וכאשר נכפול זה על עצמו הוא כאומרנו 44230 חלקים מחלק מ"ז מעשירית שישית תשיעית ‫[מ442300 חלקים מחלק מ"ז מעשירית ששית תשיעית כזה]‫<ref>marg.</ref> כזה |
|- | |- | ||
| | | | ||
| Line 6,583: | Line 7,936: | ||
|- | |- | ||
| | | | ||
| − | ::We multiply the 44230 [by itself]; the result is | + | ::We multiply the 44230 [by itself]; the result is 195629[29]00 parts of 47 of a tenth of a sixth of a ninth of a part of 47 of a tenth [of a sixth] of a ninth, like this: |
| − | |style="text-align:right;"|ונכה | + | |style="text-align:right;"|ונכה ה44<s>2</s>320 [44230]‫<ref>marg.</ref> ויעלה 19562900 חלקים מחלק מ"ז מעשירית שישית תשיעית מחלק מ"ז מעשירית תשיעית בשלם כזה |
|- | |- | ||
| | | | ||
| Line 6,600: | Line 7,953: | ||
| | | | ||
::We reduce them, i.e. we divide them by all these denominators until reaching the first denominators 9 and 6. When we reach them, we know how many sixths of ninths they are, whether it is the required number, which is 164 sixths of a ninth plus the square of the additional mentioned fractions. The result of what we have is 164 sixths of a ninth plus 4-ninths of [a part of] 47 of [a part of] 47 of a sixth of a ninth and this excess is indeed equal to the square of the fractions that are added in the root to the first approximate root of the fractions. Thus, your calculation was correct. | ::We reduce them, i.e. we divide them by all these denominators until reaching the first denominators 9 and 6. When we reach them, we know how many sixths of ninths they are, whether it is the required number, which is 164 sixths of a ninth plus the square of the additional mentioned fractions. The result of what we have is 164 sixths of a ninth plus 4-ninths of [a part of] 47 of [a part of] 47 of a sixth of a ninth and this excess is indeed equal to the square of the fractions that are added in the root to the first approximate root of the fractions. Thus, your calculation was correct. | ||
| − | |style="width:45%; text-align:right;"|ונעשה להם כלילת יופי ר"ל שנחלקנו לכל המורים האלו עד הגיענו אל הט' והו' המורים הראשונים ובהיגיענו שם נדע כמה שישית תשיעיות יעלה אם יגיע למספר הנשאל שהוא 164 שישיות תשיעית ועוד מרובע השברים הנוספים הנזכרים כנזכר ואשר עלינו זה עלה 164 שישיות תשיעית ועוד ד' תשיעיות ממ"ז ממ"ז [נ' ד'] משישית תשיעית וזה התוספת שוה ממש למרובע השברים הנוספים בשרש על שרש השברים אשר יצא ראשונה והיה כל מלאכתך אמת | + | |style="width:45%; text-align:right;"|ונעשה להם כלילת יופי ר"ל שנחלקנו לכל המורים האלו עד הגיענו אל הט' והו' המורים הראשונים ובהיגיענו שם נדע כמה שישית תשיעיות יעלה אם יגיע למספר הנשאל שהוא 164 שישיות תשיעית ועוד מרובע השברים הנוספים הנזכרים כנזכר ואשר עלינו זה עלה 164 שישיות תשיעית ועוד ד' תשיעיות ממ"ז ממ"ז [נ' ד'‫]‫<ref>marg.</ref> משישית תשיעית וזה התוספת שוה ממש למרובע השברים הנוספים בשרש על שרש השברים אשר יצא ראשונה והיה כל ‫<ref>61r</ref>מלאכתך אמת |
|} | |} | ||
:<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left[1+\frac{2}{3}+\left(\frac{4}{6}\sdot\frac{1}{9}\right)+\left(\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)+\left(\frac{3}{47}\sdot\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)\right]^2&\scriptstyle=\frac{1956292900}{47}\sdot\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\sdot\frac{1}{47}\sdot\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\\&\scriptstyle=\left(\frac{164}{6}\sdot\frac{1}{9}\right)+\left[\left(\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)+\left(\frac{3}{47}\sdot\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)\right]^2\\&\scriptstyle\left(\frac{164}{6}\sdot\frac{1}{9}\right)+\left(\frac{4}{9}\sdot\frac{1}{47}\frac{1}{47}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)+\left(\frac{1}{6}\sdot\frac{1}{9}\sdot\frac{1}{47}\sdot\frac{1}{47}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)\\\end{align}}}</math> | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left[1+\frac{2}{3}+\left(\frac{4}{6}\sdot\frac{1}{9}\right)+\left(\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)+\left(\frac{3}{47}\sdot\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)\right]^2&\scriptstyle=\frac{1956292900}{47}\sdot\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\sdot\frac{1}{47}\sdot\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\\&\scriptstyle=\left(\frac{164}{6}\sdot\frac{1}{9}\right)+\left[\left(\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)+\left(\frac{3}{47}\sdot\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)\right]^2\\&\scriptstyle\left(\frac{164}{6}\sdot\frac{1}{9}\right)+\left(\frac{4}{9}\sdot\frac{1}{47}\frac{1}{47}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)+\left(\frac{1}{6}\sdot\frac{1}{9}\sdot\frac{1}{47}\sdot\frac{1}{47}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)\\\end{align}}}</math> | ||
| Line 6,608: | Line 7,961: | ||
:<math>\scriptstyle{\color{blue}{\left(a+\frac{b}{2a}\right)^2-\left(a^2+b\right)=\left(\frac{b}{2a}\right)^2}}</math> | :<math>\scriptstyle{\color{blue}{\left(a+\frac{b}{2a}\right)^2-\left(a^2+b\right)=\left(\frac{b}{2a}\right)^2}}</math> | ||
|style="width:45%; text-align:right;"|ויצא לנו עוד מזה שנתאמת מה שאמרנו בפ"ז מהחלק הא' שכאשר נחלק הנשאר על כפל השרש מבלי תוספת אחר שיעדף המרובע האחרון על החשבון הנשאל כמרובע השברי' הנוספים וכן יהיה בכל פעם ופעם דוק ותשכח | |style="width:45%; text-align:right;"|ויצא לנו עוד מזה שנתאמת מה שאמרנו בפ"ז מהחלק הא' שכאשר נחלק הנשאר על כפל השרש מבלי תוספת אחר שיעדף המרובע האחרון על החשבון הנשאל כמרובע השברי' הנוספים וכן יהיה בכל פעם ופעם דוק ותשכח | ||
| + | |- | ||
| + | | | ||
| + | === <span style=color:Green>Reasons</span> === | ||
| + | |||
| + | | | ||
|- | |- | ||
|<span style=color:Green>The reason for multiplying the numerator by the denominators</span> | |<span style=color:Green>The reason for multiplying the numerator by the denominators</span> | ||
| Line 6,614: | Line 7,972: | ||
|The reason we say that after decomposing we multiply the decomposed number by all the denominators is in order that the square will be duplicate parts of these denominators. | |The reason we say that after decomposing we multiply the decomposed number by all the denominators is in order that the square will be duplicate parts of these denominators. | ||
:<math>\scriptstyle{\color{OliveGreen}{\sqrt{\frac{a}{b}}=\sqrt{\frac{a\sdot b}{b^2}}}}</math> | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{\frac{a}{b}}=\sqrt{\frac{a\sdot b}{b^2}}}}</math> | ||
| − | |style="text-align:right;"|וטעם אמרנו שאחר הפריטה נכה המספר הפריטה בכל המורים הוא כדי שיהיה זה המרובע חלקים מאלו המורים פעמים ר"ל נשנים | + | |style="text-align:right;"|<big>וטעם</big> אמרנו שאחר הפריטה נכה המספר הפריטה בכל המורים הוא כדי שיהיה זה המרובע חלקים מאלו המורים פעמים ר"ל נשנים |
|- | |- | ||
| | | | ||
| Line 6,654: | Line 8,012: | ||
|I.e. the number of fractions of the root is multiplied by itself, like our method [in calculating] the square of an integer, as the number of the parts and their duplication. | |I.e. the number of fractions of the root is multiplied by itself, like our method [in calculating] the square of an integer, as the number of the parts and their duplication. | ||
:<math>\scriptstyle{\color{OliveGreen}{\left(\frac{a}{b}\right)^2=\frac{a\sdot a}{b^2}}}</math> | :<math>\scriptstyle{\color{OliveGreen}{\left(\frac{a}{b}\right)^2=\frac{a\sdot a}{b^2}}}</math> | ||
| − | |style="text-align:right;"|ר"ל | + | |style="text-align:right;"|ר"ל <s>ה</s>מספר שברי השרש בעצמם כדרכנו במרובע השלמים [כי מרובע השלמים במרובע השלמים‫]‫<ref>marg.</ref> כמספר החלקים ו{{#annot:term|156,2050|KoBz}}השינוי{{#annotend:KoBz}} בהם |
|- | |- | ||
|Because, in integers, the number of the root and the number of the square are of one type, i.e. they are both integers. | |Because, in integers, the number of the root and the number of the square are of one type, i.e. they are both integers. | ||
| Line 6,686: | Line 8,044: | ||
| | | | ||
::And our saying: multiply one-quarter by 3-fifths, is as our saying: 3-fifths one-quarter of a time, which is 3-fifths of a quarter. | ::And our saying: multiply one-quarter by 3-fifths, is as our saying: 3-fifths one-quarter of a time, which is 3-fifths of a quarter. | ||
| − | |style="text-align:right;"|ואם אומרנו כפול רביעית אחת בג' חמישיות הוא כאומרנו ג' חמישיות רביעית פעם והוא ג' חמישיות רביעית | + | |style="text-align:right;"|ואם ‫<ref>61v</ref>אומרנו כפול רביעית אחת בג' חמישיות הוא כאומרנו ג' חמישיות רביעית פעם והוא ג' חמישיות רביעית |
|- | |- | ||
| | | | ||
| Line 6,692: | Line 8,050: | ||
|style="text-align:right;"|ואולם אומרנו שני רביעיות יהיה בב' פעמים רביעית פעם וכל פעם הוא ג' חמישיות רביעית הנה הב' רביעיות יהיו ו' חמישיות רביעית | |style="text-align:right;"|ואולם אומרנו שני רביעיות יהיה בב' פעמים רביעית פעם וכל פעם הוא ג' חמישיות רביעית הנה הב' רביעיות יהיו ו' חמישיות רביעית | ||
|- | |- | ||
| − | |The number is increasing through the multiplication of the numerator by the numerator | + | |The number is increasing through the multiplication of the numerator by the numerator. |
| − | |style="text-align:right;"|וכן לעולם יתרבה המספר בכפל [מספר השברים במספר] משבר השברים | + | |style="text-align:right;"|וכן לעולם יתרבה המספר בכפל [מספר השברים במספר‫]‫<ref>marg.</ref> <s>משבר</s> השברים |
|- | |- | ||
| − | |The | + | |The result is [a fraction] of the denominators of both multiplicands together, as in our example, which are fifths of a quarter. |
| − | :<math>\scriptstyle\frac{a}{b}\times\frac{c}{d}=\frac{a\sdot b}{b\sdot d}</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}\times\frac{c}{d}=\frac{a\sdot b}{b\sdot d}}}</math> |
| − | |style="text-align:right;"|ויהיה העולה מכל שני מורי שני המספרים הנכפלים יחד כבמשלנו זה שהם חמישיות רביעיות | + | |style="text-align:right;"|ויהיה העולה מכל <s>שני</s> מורי שני המספרים הנכפלים יחד כבמשלנו זה שהם חמישיות רביעיות |
|- | |- | ||
| − | |Hence the numerator of the square is a square of the numerator of the root | + | |Hence the numerator of the square is a square of the numerator of the root, exactly as it is in integers. |
| − | :<math>\scriptstyle\left(\frac{a}{b}\right)^2=\frac{a^2}{b^2}</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\left(\frac{a}{b}\right)^2=\frac{a^2}{b^2}}}</math> |
|style="text-align:right;"|ולזה יהיה מספר שברי המרובע כמרובע מספר שברי השרש כדרכו בשלם שוה בשוה | |style="text-align:right;"|ולזה יהיה מספר שברי המרובע כמרובע מספר שברי השרש כדרכו בשלם שוה בשוה | ||
|- | |- | ||
| − | | | + | |But, the denominators are duplicated, since we multiply the root by its similar, and the denominators of both are twice the denominator of one, because they are equal, and the reason of all this is clear. |
| − | |style="text-align:right;"|אבל כי המורים נשנים לפי שאנו כופלים השרש בכמותו ומורי שניהם יהיה כפל מורי | + | |style="text-align:right;"|אבל כי המורים נשנים לפי שאנו כופלים השרש בכמותו ומורי שניהם יהיה כפל מורי האחד כי שוים הם במורים וכל זה ברור בטעם |
|- | |- | ||
| − | | | + | |It becomes clear also when we multiply a surface. |
|style="text-align:right;"|גם זה יתבאר בשאנו כופלים ב{{#annot:term|814,1310|5Pke}}שטח{{#annotend:5Pke}} | |style="text-align:right;"|גם זה יתבאר בשאנו כופלים ב{{#annot:term|814,1310|5Pke}}שטח{{#annotend:5Pke}} | ||
|- | |- | ||
| − | | | + | |For, when we say about the surface: 3 times 4, it as our saying that the length is 4 [and the width is 4]. |
| + | :<math>\scriptstyle{\color{blue}{3\times4}}</math> | ||
|style="text-align:right;"|כי כאשר אנו או[מרי]ם בשטח ג' פעמים ד' הוא כאומרנו שיש ב{{#annot:term|316,1489|x8jj}}ארך{{#annotend:x8jj}} ד‫' | |style="text-align:right;"|כי כאשר אנו או[מרי]ם בשטח ג' פעמים ד' הוא כאומרנו שיש ב{{#annot:term|316,1489|x8jj}}ארך{{#annotend:x8jj}} ד‫' | ||
|- | |- | ||
| − | | | + | |If its width were only 1, it would have been only 4. |
| + | :<math>\scriptstyle{\color{blue}{4\times1=4}}</math> | ||
|style="text-align:right;"|ואלו לא היה ברחבו כי אם א' לא היו כי אם ד‫' | |style="text-align:right;"|ואלו לא היה ברחבו כי אם א' לא היו כי אם ד‫' | ||
|- | |- | ||
| − | | | + | |Since every unit that we note in the surface has 1 in length and 1 in width. |
|style="text-align:right;"|לפי שכל אחד שאנו אומרים בשטח הוא שיהיה לו א' באורך וא' ב{{#annot:term|317,1488|Ykal}}רוחב{{#annotend:Ykal}} | |style="text-align:right;"|לפי שכל אחד שאנו אומרים בשטח הוא שיהיה לו א' באורך וא' ב{{#annot:term|317,1488|Ykal}}רוחב{{#annotend:Ykal}} | ||
|- | |- | ||
| − | | | + | |Likewise in the solid: 1 in length, 1 in width, and 1 in height. |
|style="text-align:right;"|וכן ב{{#annot:term|587,1850|eHwo}}גשם{{#annotend:eHwo}} א' ב{{#annot:term|316,1489|wbTk}}אורך{{#annotend:wbTk}} וא' ברוחב ואחד ב{{#annot:term|1111,1490|B53n}}גובה{{#annotend:B53n}} | |style="text-align:right;"|וכן ב{{#annot:term|587,1850|eHwo}}גשם{{#annotend:eHwo}} א' ב{{#annot:term|316,1489|wbTk}}אורך{{#annotend:wbTk}} וא' ברוחב ואחד ב{{#annot:term|1111,1490|B53n}}גובה{{#annotend:B53n}} | ||
|- | |- | ||
| − | |<math>\ | + | |Therefore, the square of one does not increase and neither the cube, for our saying: one, concerning the surface, is as our saying: one square, and the same concerning the cube solid. |
| − | |style="text-align:right;"|לזה לא יתרבה מרובע האחד ולא גם המעוקב | + | :<math>\scriptstyle{\color{blue}{1=1^2=1^3}}</math> |
| + | |style="text-align:right;"|לזה לא יתרבה מרובע האחד ולא גם המעוקב כי אומרנו אחד בשטח הוא כאומרנו אחד {{#annot:term|590,1263|LjAq}}מרובע{{#annotend:LjAq}} וכן ב{{#annot:term|1102,1828|n3HE}}גשם מעוקב{{#annotend:n3HE}} | ||
|- | |- | ||
| − | |4 length and 3 width | + | |When it is 4 in length and 3 in width, it is as 3 stripes of 4, which is as our saying: 3 times 4, and so on. |
| − | |style="text-align:right;"|וכאשר היו ד' באורך וג' ברוחב הרי הם ג' רצועות של ד' ד' והוא כאומרנו ג' פעמים ד' ול וכן לעולם | + | :<math>\scriptstyle{\color{blue}{3\times4}}</math> |
| + | |style="text-align:right;"|וכאשר היו ד' באורך וג' ברוחב הרי הם ג' רצועות של ד' ד' והוא כאומרנו ג' פעמים ד' <s>ול</s> וכן לעולם | ||
|- | |- | ||
| − | | | + | |But, when we multiply a fraction by a fraction: |
| − | |style="text-align:right;"|אבל כשא[א]נו כופלים שבר בשבר | + | |style="text-align:right;"|אבל כשא[א]‫<ref>marg.</ref>נו כופלים שבר בשבר |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{3}{4}\times\frac{4}{5} | + | :*Example: 3-quarters by 4-fifths, it is as our saying that its length is 4-fifths of the whole and its width is 3-quarters of the whole. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{3}{4}\times\frac{4}{5}}}</math> | ||
|style="text-align:right;"|המשל ג' רביעיות בד' חמישיות הוא כאומרנו שארכו ד' חמישיות השלם ורחבו ג' רביעיות השלם | |style="text-align:right;"|המשל ג' רביעיות בד' חמישיות הוא כאומרנו שארכו ד' חמישיות השלם ורחבו ג' רביעיות השלם | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{3}{4}\times1=1 | + | ::If its length were a whole unit, [its area] were 3-quarters of the whole, because the whole square lacks the quarter that is subtracted from its width. This is understandable with a bit of study. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{3}{4}\times1=1-\frac{1}{4}=\frac{3}{4}}}</math> | ||
|style="text-align:right;"|ואם ארכו אחד שלם היה ג' רביעיות שלם כי מן השלם המרובע חסר הרביע שנפצל מרחבו וזה מובן במעט עיון | |style="text-align:right;"|ואם ארכו אחד שלם היה ג' רביעיות שלם כי מן השלם המרובע חסר הרביע שנפצל מרחבו וזה מובן במעט עיון | ||
|- | |- | ||
| | | | ||
| + | ::But, since a fifth is subtracted from its length also, it is as subtracting from 3-quarters their fifth and their 4-fifths remain. So the area is 4-fifths of 3-quarters and each fifth of them is a fifth of 3-quarters, which is three-quarters of a fifth. For, a fifth of a quarter is as a quarter of a fifth. Therefore, the 4-fifths of 3-quarters are 4 times 3-quarters of a fifth, which are 12 [fifths of a quarter]. | ||
::<math>\scriptstyle{\color{blue}{\frac{3}{4}\times\frac{4}{5}=\frac{3}{4}-\left(\frac{1}{5}\sdot\frac{3}{4}\right)=\left(\frac{1}{5}\sdot\frac{3}{4}\right)\sdot4=4\sdot\left(\frac{3}{4}\sdot\frac{1}{5}\right)=\frac{12}{4}\sdot\frac{1}{5}}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{3}{4}\times\frac{4}{5}=\frac{3}{4}-\left(\frac{1}{5}\sdot\frac{3}{4}\right)=\left(\frac{1}{5}\sdot\frac{3}{4}\right)\sdot4=4\sdot\left(\frac{3}{4}\sdot\frac{1}{5}\right)=\frac{12}{4}\sdot\frac{1}{5}}}</math> | ||
| − | |style="text-align:right;"|אבל לפי שמארכו נפצל ג"כ חמישיתו הנה הוא כמי שהסיר מהג' רביעיות חמישיתם ונשארו ד' חמישיותיהם הנה השטח הוא ד' חמישיות מג' רביעיות [וכל חמישית מהם היא חמישית ג' רביעיות שהוא כשלש רביעיות חמישית כי כך הוא חמישית רביעית כרביעית חמישית א"כ הד' החמישיות מג' רביעיות הם ד' פעמים] הם ד' פעמים ג' רביעיות רביעיות חמישית שהם י"ב | + | |style="text-align:right;"|אבל לפי שמארכו נפצל ג"כ חמישיתו הנה הוא כמי שהסיר מהג' רביעיות חמישיתם ונשארו ד' חמישיותיהם הנה השטח הוא ד' חמישיות מג' רביעיות [וכל חמישית מהם היא חמישית ג' רביעיות שהוא כשלש רביעיות חמישית כי כך הוא חמישית רביעית כרביעית חמישית א"כ הד' החמישיות מג' רביעיות הם ד' פעמים‫]‫<ref>marg.</ref> הם ד' פעמים ג' רביעיות ‫<ref>62r</ref>רביעיות חמישית שהם י"ב |
|- | |- | ||
| − | | | + | |Therefore, we multiply the numerator by the numerator, [when extracting] the root, and it all comes down to the same thing. |
| − | |||
| − | |||
|style="text-align:right;"|ולזה אנו כופלים בהכאה מספר השברים במספר השברים וכן בשרש והכל עולה לענין אחד | |style="text-align:right;"|ולזה אנו כופלים בהכאה מספר השברים במספר השברים וכן בשרש והכל עולה לענין אחד | ||
|- | |- | ||
| − | | | + | |After we have explained that the denominators of the square are the duplication of the denominators of the root and that the numerator of the square is as the square of the numerator of the root, it is clear that if the square has duplicated denominators, i.e. 4 and 4, or 5 and 5 etc., we do not need to multiply by the denominators, but to extract the root of the numerator alone, as in the way of the integers, and the denominators of the root are half the denominators of the square. So, we divide [the root of the numerator] by half the denominators [of the square]. |
| − | |style="text-align:right;"|ואחר שביארנו שמורי המרובע הם נשנים ממורי השרש ומספר שברי המרובע הוא כמרובע מספר שברי השרש נתבא' שאם היו לזה המרובע מורים נכפלים ר"ל ד'ד' או ה'ה' וכדומה לזה שלא היינו צריכים לכפול | + | :<math>\scriptstyle{\color{OliveGreen}{\left(\frac{a}{b}\right)^2\longrightarrow\sqrt{\frac{a}{b}\sdot\frac{1}{b}}=\frac{\sqrt{a}}{b}}}</math> |
| + | |style="text-align:right;"|ואחר שביארנו שמורי המרובע הם נשנים ממורי השרש ומספר שברי המרובע הוא כמרובע מספר שברי השרש נתבא' שאם היו לזה המרובע מורים נכפלים ר"ל ד'ד' או ה'ה' וכדומה לזה שלא היינו צריכים לכפול במורי<s>ו</s><sup>ם</sup> כי אם להוציא השרש לבד מהמספר שב[..]ו כ[ער]ך בשלמים ומורי השרש היוצא היו חצי מורי<s>ו</s> המרובע ונחלקנו אליהם ר"ל לחצי מוריו | ||
|- | |- | ||
| − | | | + | |Even if not all [the denominators] are duplicate, but each of those that are not twice in it are multiplied by themselves, i.e. they are squares as 4, or 9, take the root of that denominator of the square as a denominator of the root, i.e. 2 instead of 4, 3 instead of 9. Because you can write 2 and 2 as the denominators of the square, instead of the 4, or 3 and 3 instead of 9, then you take one of them for the root and all this is clear. |
| − | : | + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{\frac{a}{b^2}}=\frac{\sqrt{a}}{b}}}</math> |
| − | + | :*<math>\scriptstyle{\color{blue}{\sqrt{\frac{a}{9}}=\frac{\sqrt{a}}{3}}}</math> | |
| − | + | :*<math>\scriptstyle{\color{blue}{\sqrt{\frac{a}{4}}=\frac{\sqrt{a}}{2}}}</math> | |
|style="text-align:right;"|וכן אפי' אם לא היו כלם כפולים אבל שכל אחד מאשר אינם בו פעמים הוא כפול ר"ל כי אם הם כפולים בעצמם ר"ל שהם מרובעים כד' או כט' תקח שרש המורה ההוא אשר למרובע במקומו למורה השרש ר"ל הב' במקום ד' והג' במקו' הט' וזה שהרי בידיך לשום כמורי המרובע במקום הד' השנים או במקום הט' ג'ג' ותקח אחד מהם בשרש וכל זה ברור | |style="text-align:right;"|וכן אפי' אם לא היו כלם כפולים אבל שכל אחד מאשר אינם בו פעמים הוא כפול ר"ל כי אם הם כפולים בעצמם ר"ל שהם מרובעים כד' או כט' תקח שרש המורה ההוא אשר למרובע במקומו למורה השרש ר"ל הב' במקום ד' והג' במקו' הט' וזה שהרי בידיך לשום כמורי המרובע במקום הד' השנים או במקום הט' ג'ג' ותקח אחד מהם בשרש וכל זה ברור | ||
|- | |- | ||
| − | |This | + | |This will be further explained in the chapter on factorization that is in a section I intend to write the end of the book. |
|style="text-align:right;"|ויתבאר עוד במאמ' ההתכה אשר בכלל אשר ייעדתי לשום בסוף הספר | |style="text-align:right;"|ויתבאר עוד במאמ' ההתכה אשר בכלל אשר ייעדתי לשום בסוף הספר | ||
|- | |- | ||
| − | | | + | |If there are of those and of those, multiply the numerator by those that are not duplicate and are not squares, and add them to half those that are duplicate and to the roots of the square denominators that you take instead of them. Divide the integer resulting in the root by them and the fractions resulting in the root are parts of one part of these denominators, by which you divide the integers of the root. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{\frac{a}{b^2\sdot c}}=\sqrt{\frac{a\sdot c}{b^2\sdot c^2}}=\frac{\sqrt{a\sdot c}}{b\sdot c}}}</math> | |
| − | :<math>\scriptstyle\sqrt{\frac{a}{b^2\sdot c}}=\sqrt{\frac{a\sdot c}{b^2\sdot c^2}}=\frac{\sqrt{a\sdot c}}{b\sdot c}</math> | + | |style="text-align:right;"|ואם יהיו שם מאלו ומאלו תכפול מספר שברי המרובע באשר אינם נכפלים ולא מרובעים ותוסיפם על חצי הנכפלים ושרשי המורים המרובעים אשר לקחת במקו<sup>מם</sup> ועליהם תחלק השלימים היוצאים בשרש והשברים היוצאים בשרש הם חלקים מחלק אחד מאלו המורים אשר להם תחלק שלימי השרש |
| − | |style="text-align:right;"|ואם יהיו שם מאלו ומאלו תכפול מספר שברי המרובע באשר אינם נכפלים ולא מרובעים ותוסיפם על חצי הנכפלים ושרשי המורים המרובעים אשר לקחת | + | |- |
| + | |All is clarified in practice and reason. | ||
| + | |style="text-align:right;"|והכל נתבאר במעשה ובטעם | ||
|- | |- | ||
| − | |In order to | + | |In order not to confuse you by examining if they are duplicate and taking their half, or taking the roots of the squares, I instruct you to multiply it by all [the denominators], so that the required square has now twice the denominators that it had originally and we divide the numerator by [the denominators] it had originally that are half of those it has now. |
| − | :<math>\scriptstyle\sqrt{\frac{a}{b^2}}=\sqrt{\frac{a\sdot b^2}{b^2\sdot b^2}}=\frac{\sqrt{a\sdot b^2}}{b\sdot b}</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{\frac{a}{b^2}}=\sqrt{\frac{a\sdot b^2}{b^2\sdot b^2}}=\frac{\sqrt{a\sdot b^2}}{b\sdot b}}}</math> |
| − | |style="text-align:right;"|אכן כדי שלא לבלבלך בזה לראות אם הם נכפלים ולקחת חציים או לקחת מהמרובעים | + | |style="text-align:right;"|אכן כדי שלא לבלבלך בזה לראות אם הם נכפלים ולקחת חציים או לקחת מהמרובעים שרשם במקומם <s>ציויתיך</s> <sup>צויתיך</sup> לכפלו בכלם ויהיו לו ר"ל למרובע הנשאל כפל המורים אשר לו עתה ונחלק מספר ‫<ref>62v</ref>שברי השרש לאשר לו בתחלה שהם חצי מאשר לו עתה |
|- | |- | ||
| − | | | + | |It is best for you to bother, even if it is not necessary, so as not to get confused, if you are not well versed in the procedure. |
|style="text-align:right;"|וטוב שתטרח ואם לו לצורך כדי שלא תתבלבל אם אינך בקי במלאכה | |style="text-align:right;"|וטוב שתטרח ואם לו לצורך כדי שלא תתבלבל אם אינך בקי במלאכה | ||
|- | |- | ||
| − | | | + | |But, if you see your self deserve to be prayer leader, a Cohen who lifts his hands, you can make the procedure easier for you. |
| − | But, if | + | |style="text-align:right;"|ואם ראית בעצמך שאתה ראוי להיות <s>שצו</s> ש'צ' כהן הנושא כפיו תוכל להקל מעליך העבודה ואתה רשאי ולא אני |
| − | |style="text-align:right;"|ואם ראית בעצמך שאתה ראוי להיות שצו ש'צ' כהן הנושא כפיו תוכל להקל מעליך העבודה ואתה רשאי ולא אני | ||
|- | |- | ||
|} | |} | ||
| Line 6,783: | Line 8,148: | ||
| | | | ||
|- | |- | ||
| − | | | + | |After we have completed the six chapters on fractions, we start with all that we have designated that is beneficial to all. |
| − | |style="width:45%; text-align:right;"|ואחר אש' השלמנו הו' פרקים אשר | + | |style="width:45%; text-align:right;"|ואחר אש' השלמנו הו' פרקים אשר בשברים נתחיל בכל אשר ייעדנו שהוא מועיל לכלם |
|- | |- | ||
| | | | ||
| − | === Finding the Common Denominator === | + | === <span style=color:Green>Finding the Common Denominator</span> === |
| | | | ||
|- | |- | ||
| Line 6,802: | Line 8,167: | ||
|- | |- | ||
| | | | ||
| − | :*The great denominator of these numbers is, as said, the common denominator of these | + | :*The great denominator of these numbers is, as said, the common denominator of these four denominators, which is [received] by multiplying one by the other and the product by the other until they end; it is 1260. |
:::<math>\scriptstyle{\color{blue}{4\sdot5\sdot7\sdot9=1260}}</math> | :::<math>\scriptstyle{\color{blue}{4\sdot5\sdot7\sdot9=1260}}</math> | ||
|style="text-align:right;"|הנה מורה החשבונים הגדול אשר אמרתי הוא אם ד' מורים אלו והוא בהכפל זה בזה והעולה באחר עד תומם ויהיה 1260 | |style="text-align:right;"|הנה מורה החשבונים הגדול אשר אמרתי הוא אם ד' מורים אלו והוא בהכפל זה בזה והעולה באחר עד תומם ויהיה 1260 | ||
| Line 6,811: | Line 8,176: | ||
|- | |- | ||
| | | | ||
| − | ::*The ninth is 140, which is the product of | + | ::*The ninth is 140, which is the product of three of the mentioned denominators, i.e. their common denominator. |
:::<math>\scriptstyle{\color{blue}{\frac{1}{9}\sdot1260={\color{red}{4\sdot5\sdot7}}=140}}</math> | :::<math>\scriptstyle{\color{blue}{\frac{1}{9}\sdot1260={\color{red}{4\sdot5\sdot7}}=140}}</math> | ||
|style="text-align:right;"|והנה תשיעית הוא ק"מ והוא ככפל הג' מורים הנזכרים ר"ל באמם | |style="text-align:right;"|והנה תשיעית הוא ק"מ והוא ככפל הג' מורים הנזכרים ר"ל באמם | ||
| Line 6,821: | Line 8,186: | ||
|- | |- | ||
| | | | ||
| − | :::3 quarters of the ninth are three times 35, which is 105. | + | :::3-quarters of the ninth are three times 35, which is 105. |
:::<math>\scriptstyle{\color{blue}{\left(\frac{3}{4}\sdot\frac{1}{9}\right)\sdot1260=3\sdot35=105}}</math> | :::<math>\scriptstyle{\color{blue}{\left(\frac{3}{4}\sdot\frac{1}{9}\right)\sdot1260=3\sdot35=105}}</math> | ||
|style="text-align:right;"|והג' רביעיות התשיעית יהיו שלשה פעמים ל"ה שהם 105 | |style="text-align:right;"|והג' רביעיות התשיעית יהיו שלשה פעמים ל"ה שהם 105 | ||
| Line 6,831: | Line 8,196: | ||
|- | |- | ||
| | | | ||
| − | :::A fifth of | + | :::A fifth of a seventh is a fifth of it; it is 36 and it is the common denominator of the remaining. |
:::<math>\scriptstyle{\color{blue}{\left(\frac{1}{5}\sdot\frac{1}{7}\right)\sdot1260=\frac{1}{5}\sdot180=4\sdot9=36}}</math> | :::<math>\scriptstyle{\color{blue}{\left(\frac{1}{5}\sdot\frac{1}{7}\right)\sdot1260=\frac{1}{5}\sdot180=4\sdot9=36}}</math> | ||
|style="text-align:right;"|וחמישית שביעית הוא חמישית זה והוא ל"ו והוא אם הנשארים | |style="text-align:right;"|וחמישית שביעית הוא חמישית זה והוא ל"ו והוא אם הנשארים | ||
|- | |- | ||
| | | | ||
| + | :::4-fifths of a seventh are 4 times 36, or if you want to say: 4 times the product of the two denominators by each other, i.e. 9 by 4, which is 36; the result is 144. | ||
:::<math>\scriptstyle{\color{blue}{\left(\frac{4}{5}\sdot\frac{1}{7}\right)\sdot1260=4\sdot36=4\sdot\left(4\sdot9\right)=144}}</math> | :::<math>\scriptstyle{\color{blue}{\left(\frac{4}{5}\sdot\frac{1}{7}\right)\sdot1260=4\sdot36=4\sdot\left(4\sdot9\right)=144}}</math> | ||
|style="text-align:right;"|והד' חמישיות שביעית הם ד' פעמים ל"ו או אם תרצה לומר ד' פעמים כפל הב' מורים זה בזה ר"ל ט' בד' שהוא ל"ו והעולה יהיה 144 | |style="text-align:right;"|והד' חמישיות שביעית הם ד' פעמים ל"ו או אם תרצה לומר ד' פעמים כפל הב' מורים זה בזה ר"ל ט' בד' שהוא ל"ו והעולה יהיה 144 | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{ | + | :Sum it up with 105 that are the 3-quarters of a ninth; the result is 249 of 1260 parts of a whole, for this denominator is the large denominator that you take and each part of them is one part of all these denominators. |
| + | :<math>\scriptstyle{\color{blue}{\left(\frac{3}{4}\sdot\frac{1}{9}\right)+\left(\frac{4}{5}\sdot\frac{1}{7}\right)=\frac{105+144}{5}\sdot\frac{1}{7}\sdot\frac{1}{4}\sdot\frac{1}{9}=\frac{249}{5}\sdot\frac{1}{7}\sdot\frac{1}{4}\sdot\frac{1}{9}=\frac{249}{1260}}}</math> | ||
|style="text-align:right;"|‫<ref>63r</ref>ותחברם עם הה'10 שעלו הג' רביעיות תשיעית יעלו 249 ב0ובא חלקים בשלם כי זה מורה הוא מספר המורה הגדול אשר לקחת וכל חלק מאלו הוא חלק מכל אלו המורים | |style="text-align:right;"|‫<ref>63r</ref>ותחברם עם הה'10 שעלו הג' רביעיות תשיעית יעלו 249 ב0ובא חלקים בשלם כי זה מורה הוא מספר המורה הגדול אשר לקחת וכל חלק מאלו הוא חלק מכל אלו המורים | ||
|- | |- | ||
| | | | ||
| + | ::This is because 140 is one-ninth, | ||
|style="text-align:right;"|וזה כי 140 הם תשיעיות אחד | |style="text-align:right;"|וזה כי 140 הם תשיעיות אחד | ||
|- | |- | ||
| | | | ||
| + | ::35, which is its quarter, is a quarter of a ninth. | ||
|style="text-align:right;"|ול"ה שהם רביעיתם הם רביעית תשיעית | |style="text-align:right;"|ול"ה שהם רביעיתם הם רביעית תשיעית | ||
|- | |- | ||
| | | | ||
| + | ::5, which is a seventh of 35, is a seventh of a quarter of a ninth. | ||
|style="text-align:right;"|והה' שהם שביעית הל"ה הם שביעית רביעית תשיעית | |style="text-align:right;"|והה' שהם שביעית הל"ה הם שביעית רביעית תשיעית | ||
|- | |- | ||
| | | | ||
| + | ::1, which is a fifth of 5, is a fifth of a ninth of a quarter of a seventh. | ||
|style="text-align:right;"|והא' שהוא חמישית הה' הוא חמישית תשיעית רביעית שביעית | |style="text-align:right;"|והא' שהוא חמישית הה' הוא חמישית תשיעית רביעית שביעית | ||
|- | |- | ||
| | | | ||
| + | :So, the 249 are fifths of a ninth of a quarter of a seventh. | ||
|style="text-align:right;"|א"כ אלו ה249 הם חמישיות תשיעית רביעית שביעית | |style="text-align:right;"|א"כ אלו ה249 הם חמישיות תשיעית רביעית שביעית | ||
|- | |- | ||
| | | | ||
| + | :If you want to know how much they are, divide them by 5, since every 5 of them are ninths of a quarter of a [seventh]; the result are sevenths of a quarter of a ninth. | ||
|style="text-align:right;"|ואם תרצה לידע מה המה אלה הנה אחר שכל ה' מהם הם תשיעיות רביעית תשיעית תחלקם לה' והיוצא יהיו שביעיות רביעית תשיעית | |style="text-align:right;"|ואם תרצה לידע מה המה אלה הנה אחר שכל ה' מהם הם תשיעיות רביעית תשיעית תחלקם לה' והיוצא יהיו שביעיות רביעית תשיעית | ||
|- | |- | ||
| | | | ||
| + | :If something remains, it is fifths of a ninth of a quarter of a seventh as in the beginning. | ||
|style="text-align:right;"|ואם נשאר דבר הוא כבתחלה חמישיות תשיעית רביעית שביעית | |style="text-align:right;"|ואם נשאר דבר הוא כבתחלה חמישיות תשיעית רביעית שביעית | ||
|- | |- | ||
| | | | ||
| + | :When you divide the sevenths of a quarter of a ninth by 7, the result are quarters of a ninth. | ||
|style="text-align:right;"|ומהשביעית רביעיות תשיעית וכאשר תחלק לז' יהיה היוצא רביעיות תשיעיות | |style="text-align:right;"|ומהשביעית רביעיות תשיעית וכאשר תחלק לז' יהיה היוצא רביעיות תשיעיות | ||
|- | |- | ||
| | | | ||
| + | :When you this by 4, the result are ninths. | ||
|style="text-align:right;"|וכשתחלק זה היוצא לד' יהיה היוצא תשיעית | |style="text-align:right;"|וכשתחלק זה היוצא לד' יהיה היוצא תשיעית | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|וכשתחלקנו לט' ה יהיה היוצא שלימים | + | :When you it by 9, the result are integers. |
| + | |style="text-align:right;"|וכשתחלקנו לט' <s>ה</s> יהיה היוצא שלימים | ||
|- | |- | ||
| − | |The order | + | |The whole aforementioned procedure itself is as stated in diagrams that are not in the book, for the order is unimportant. Deduce from this. |
| − | |style="text-align:right;"|וכל זה אי המעשה הנזכר למעלה עין בעין כמו שנרמז בצורות הרמוזות מחוץ לספר כי הסדר לא יזיק דוק ותשכח | + | |style="text-align:right;"|וכל זה <s>אי</s> המעשה הנזכר למעלה עין בעין כמו שנרמז בצורות הרמוזות מחוץ לספר כי הסדר לא יזיק דוק ותשכח |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|והנה העולה מחבור השברים הנשאלות על כל א' מהדרכים כי הכל אחד הוא שביעית אחת ורביעית שביעית וד' תשיעיות רביעית שביעית וד' חמישיות תשיעית רביעית שביעית והקש על זה בכל שאר הפרקים | + | :The result from summing the required fractions, in each of the methods, for they are all the same, is one-seventh, a quarter of a seventh, 4-ninths of a quarter of a seventh, and 4-fifths of a ninth of a quarter of a seventh. |
| + | |style="text-align:right;"|והנה העולה מחבור השברים הנשאלות על כל א' מהדרכים כי הכל אחד הוא שביעית אחת ורביעית שביעית וד' תשיעיות רביעית שביעית וד' חמישיות תשיעית רביעית שביעית | ||
| + | |- | ||
| + | |Deduce on that in all the other chapters. | ||
| + | |style="text-align:right;"|והקש על זה בכל שאר הפרקים | ||
|- | |- | ||
| | | | ||
| Line 6,884: | Line 8,265: | ||
|style="text-align:right;"|<big>מאמר ההשלמה</big> | |style="text-align:right;"|<big>מאמר ההשלמה</big> | ||
|- | |- | ||
| − | |Completion is | + | |Completion is when we have known fractions or fractions of fractions and we need to subtract them from other fractions or fractions of fractions that we have of the same types. It happens in the extraction of roots [for instance], as written in chapter six of this section. |
| − | |style="text-align:right;"|ההשלמה הוא כאשר יש בידינו שבורים ידועים או שברי שברים ואנו צריכים לגרעם משברים או שברי שברים אחרים שיש בידינו ממיניהם וזה יקרה בהוצאת השרשים כמו כפי שנכתב בפ' ו' מזה החלק | + | |style="text-align:right;"|ההשלמה הוא כאשר יש בידינו שבורים ידועים או שברי שברים ואנו צריכים לגרעם משברים או שברי שברים אחרים שיש בידינו ממיניהם וזה יקרה בהוצאת השרשים כמו ‫<ref>63v</ref>כפי שנכתב בפ' ו' מזה החלק |
|- | |- | ||
| − | | | + | |Sometimes the subtracted fractions of fractions are greater than those from which they are subtracted, but there are many fractions or integers to complete our deficiency. |
| − | + | |style="text-align:right;"|ולפעמים השברי שברים {{#annot:term|182,1365|DOGq}}הנגרעי'{{#annotend:DOGq}} הם רבים מאשר {{#annot:term|181,1365|QX0c}}יגרע מהם{{#annotend:QX0c}} אכן יש שם שברי רבים או שלימים למלאת די מחסורנו | |
| − | |style="text-align:right;"|ולפעמים השברי שברים {{#annot:term|182,1365|DOGq}}הנגרעי'{{#annotend:DOGq}} הם רבים מאשר {{#annot:term| | ||
|- | |- | ||
| − | | | + | |Therefore, when we take the integer or the greater fraction, to subtract from it these fractions of fractions, we need to know easily the remainder from that integer or that great fraction after we subtract from it the deficiency of these fractions of fractions for a whole unit or for a larger fraction, and this is their complement for one. |
| − | |style="text-align:right;"|ואחר שנדע השלמתן לאחד אם היו לנו שברי שברים ממינם כאשר נגרע מהם | + | :<math>\scriptstyle{\color{OliveGreen}{a-b=\left(a-c\right)+\left(c-b\right)}}</math> |
| + | |style="text-align:right;"|לכן אנו צריכים לידע כאשר נקח השלם או השבר הגדול להוציא ממנו שברי שברים אלו שנדע בקלות הנשאר מהשלם או <sup>מ</sup>השבר הגדול [ההוא אחר שהוצאנו ממנו שזה הוא מה שחסרים אלו השברי שברים מאחד שלם או שבר גדול‫]‫<ref>marg.</ref> <s>להוציא ממנו שברי שברים אלו שנדע בקלות הנשאר מהשלם או מהשבר הגדול להוציא ממנו שברי שברים אלו שנדע בקלות הנשאר מהשלם או מהשבר הגדול ההוא אחר שהוצאנו ממנו שזה הוא מה שחסרים אלו השברי ה' שברים מאחד שלם או שבר גדול</s> וזו<sup>הי</sup> השלמתן לאחד | ||
| + | |- | ||
| + | |When we know their complement for one, if we have fractions of fractions of their type, after we subtract from them those that we have that are smaller, we add this complement to them and the sum is the remainder. | ||
| + | |style="text-align:right;"|ואחר שנדע השלמתן לאחד אם היו לנו שברי שברים ממינם כאשר נגרע מהם אלו שהיו מעט אשר בידינו נחבר זאת ההשלמה עמהן ו{{#annot:term|388,1220|76oh}}המחובר{{#annotend:76oh}} יהיה הנשאר | ||
|- | |- | ||
| | | | ||
| − | *Example: we wish to subtract 7-ninths, 5-sevenths of a ninth, and 3-quarters of a seventh of a ninths, from 3 integers, 5-ninths, and three-sevenths of a ninth. | + | *{{#annot:(3+⁵/₉+³/₇·⅑+²/₄·⅐·⅑)-(⁷/₉+⁵/₇·⅑+¾·⅐·⅑)|678|eMzX}}Example: we wish to subtract 7-ninths, 5-sevenths of a ninth, and 3-quarters of a seventh of a ninths, from 3 integers, 5-ninths, and three-sevenths of a ninth. |
:<math>\scriptstyle\left[3+\frac{5}{9}+\left(\frac{3}{7}\sdot\frac{1}{9}\right)+\left(\frac{2}{4}\sdot\frac{1}{7}\sdot\frac{1}{9}\right)\right]-\left[\frac{7}{9}+\left(\frac{5}{7}\sdot\frac{1}{9}\right)+\left(\frac{3}{4}\sdot\frac{1}{7}\sdot\frac{1}{9}\right)\right]</math> | :<math>\scriptstyle\left[3+\frac{5}{9}+\left(\frac{3}{7}\sdot\frac{1}{9}\right)+\left(\frac{2}{4}\sdot\frac{1}{7}\sdot\frac{1}{9}\right)\right]-\left[\frac{7}{9}+\left(\frac{5}{7}\sdot\frac{1}{9}\right)+\left(\frac{3}{4}\sdot\frac{1}{7}\sdot\frac{1}{9}\right)\right]</math> | ||
| − | |style="text-align:right;"|המשל רצינו לגרוע ז' תשיעיות וה' שביעיות תשיעית וג' רביעיות שביעית תשיעית מג' שלמים וה' תשיעיות ושלש שביעיות תשיעית | + | |style="text-align:right;"|<big>המשל</big> רצינו לגרוע ז' תשיעיות וה' שביעיות תשיעית וג' רביעיות שביעית תשיעית מג' שלמים וה' תשיעיות ושלש שביעיות תשיעית{{#annotend:eMzX}} |
|- | |- | ||
| | | | ||
| Line 6,907: | Line 8,291: | ||
|- | |- | ||
|Then, we add the complement to the fractions that we had that were not enough for us, for they have ''the right of redemption'' [Jeremiah 32, 7], and the sum is the remainder. | |Then, we add the complement to the fractions that we had that were not enough for us, for they have ''the right of redemption'' [Jeremiah 32, 7], and the sum is the remainder. | ||
| − | |style="text-align:right;"|וזאת ההשלמה נחברנה עם השברים אשר היו לנו ולא היה בהם די ספקנו כי להם ''משפט הגאולה''<ref>ירמיה לב, ז</ref> והמחובר הוא הנשאר | + | |style="text-align:right;"|וזאת ההשלמה נחברנה עם השברים אשר היו לנו ולא היה בהם די ספקנו כי להם ''משפט הגאולה''‫<ref group=note>ירמיה לב, ז</ref> והמחובר הוא הנשאר |
|- | |- | ||
| | | | ||
| Line 6,917: | Line 8,301: | ||
::We also say: we have completed [one-seventh of a ninth and with the five-sevenths of a ninth that we had they are 6. What is their complement for one whole ninth? One. We write it beneath them. | ::We also say: we have completed [one-seventh of a ninth and with the five-sevenths of a ninth that we had they are 6. What is their complement for one whole ninth? One. We write it beneath them. | ||
::<math>\scriptstyle{\color{blue}{\frac{1}{9}-\left[\left(\frac{1}{7}\sdot\frac{1}{9}\right)+\left(\frac{5}{7}\sdot\frac{1}{9}\right)\right]=\frac{1}{9}-\left(\frac{6}{7}\sdot\frac{1}{9}\right)=\frac{1}{7}\sdot\frac{1}{9}}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{1}{9}-\left[\left(\frac{1}{7}\sdot\frac{1}{9}\right)+\left(\frac{5}{7}\sdot\frac{1}{9}\right)\right]=\frac{1}{9}-\left(\frac{6}{7}\sdot\frac{1}{9}\right)=\frac{1}{7}\sdot\frac{1}{9}}}</math> | ||
| − | |style="text-align:right;"|עוד נאמר הרי השלמנו [לשביעית תשיעית אחד וחמש שביעיות תשיעית שהיו לנו הרי ו' ובכמה ישלומו לתשיעית אחד] לתשיעית אחד שלמה באחד נשימנו תחתיו | + | |style="text-align:right;"|עוד נאמר הרי השלמנו [לשביעית תשיעית אחד וחמש שביעיות תשיעית שהיו לנו הרי ו' ובכמה ישלומו לתשיעית אחד‫]‫<ref>marg.</ref> לתשיעית אחד שלמה באחד נשימנו ‫<ref>64r</ref>תחתיו |
|- | |- | ||
| | | | ||
| Line 6,926: | Line 8,310: | ||
| | | | ||
::We are left with one-ninth, one-seventh of a ninth, and one-quarter of a seventh of a ninth from the one integer. We add them to what is above; the result is 2 integers, 6-ninths, 4-[sevenths] of a ninth [and 3-quarters of a seventh of a ninth] that are left in our hand. The reason for this is clear. | ::We are left with one-ninth, one-seventh of a ninth, and one-quarter of a seventh of a ninth from the one integer. We add them to what is above; the result is 2 integers, 6-ninths, 4-[sevenths] of a ninth [and 3-quarters of a seventh of a ninth] that are left in our hand. The reason for this is clear. | ||
| − | |style="text-align:right;"|הרי לנו שנשאר מהאחד השלם תשיעית אחת ושביעית תשיעית ורביעית שביעית תשיעית ונחברם עם אשר בעליונה ויעלה שנשאר בידינו ב' שלימים וו' תשיעיות וד' רביעיות שביעית תשיעית [וג' רביעיות שביעית תשיעית] וכל זה ברור בטעם | + | |style="text-align:right;"|הרי לנו שנשאר מהאחד השלם תשיעית אחת ושביעית תשיעית ורביעית שביעית תשיעית ונחברם עם אשר בעליונה ויעלה שנשאר בידינו ב' שלימים וו' תשיעיות וד' <s>רביעיות</s> שביעית תשיעית [וג' רביעיות שביעית תשיעית‫]‫<ref>marg.</ref> וכל זה ברור בטעם |
|} | |} | ||
:<math>\scriptstyle{\color{blue}{\begin{align}&\scriptstyle | :<math>\scriptstyle{\color{blue}{\begin{align}&\scriptstyle | ||
| Line 6,933: | Line 8,317: | ||
|- | |- | ||
|In order to make the procedure easier for you, I shall give you a rule: we write the complement of the numerator for its denominator under the last [fraction] to the left. | |In order to make the procedure easier for you, I shall give you a rule: we write the complement of the numerator for its denominator under the last [fraction] to the left. | ||
| − | |style="width:45%; text-align:right;"|וכדי להקל מעליך המעשה אתן לך כלל כי לאחרון אשר לצד שמאל אשר שם יתחיל הצורך נשים תחתיו כדי השלמת מספר שבריו למורה אשר עליו שוה בשוה | + | |style="width:45%; text-align:right;"|<big>וכדי</big> להקל מעליך המעשה אתן לך כלל כי לאחרון אשר לצד שמאל אשר שם יתחיל הצורך נשים תחתיו כדי השלמת מספר שבריו למורה אשר עליו שוה בשוה |
|- | |- | ||
| | | | ||
| Line 6,944: | Line 8,328: | ||
|- | |- | ||
|If we want, we can apply the method that we use for integers, and then we do not need completion at all. | |If we want, we can apply the method that we use for integers, and then we do not need completion at all. | ||
| − | |style="text-align:right;"|ואם היינו רוצים היינו עושים כדרך שאנו עושי' בשלימי' ולא נצטרך להשלמה כלל | + | |style="text-align:right;"|<big>ואם</big> היינו רוצים היינו עושים כדרך שאנו עושי' בשלימי' ולא נצטרך להשלמה כלל |
|- | |- | ||
|<span style=color:Green>Borrowing one unit from a fraction of a higher type, and marking the loan with a dot as a reminder:</span> | |<span style=color:Green>Borrowing one unit from a fraction of a higher type, and marking the loan with a dot as a reminder:</span> | ||
| Line 6,962: | Line 8,346: | ||
| | | | ||
::We say: 5-sevenths and a dot are 6. We cannot subtract them from 3. We mark a dot above the 7-ninths to be subtracted and say: this one is 7 as the denominator, with 3 it is 10. We subtract 6 from it; 4 remains. | ::We say: 5-sevenths and a dot are 6. We cannot subtract them from 3. We mark a dot above the 7-ninths to be subtracted and say: this one is 7 as the denominator, with 3 it is 10. We subtract 6 from it; 4 remains. | ||
| − | |style="width:45%; text-align:right;"|ונאמר ה' שביעיות ונקודה הם ו' לא נוכל להסירם מהג' נשים נקודה על הז' תשיעיות אשר לנו לגרוע ונאמר זה האחד הוא ז' כמורה וג' הרי י' נסיר מהם ו' ישארו ד‫' | + | |style="width:45%; text-align:right;"|ונאמר ה' שביעיות ונקודה הם ו' לא נוכל להסירם מהג' נשים נקודה על הז' תשיעיות אשר לנו לגרוע ונאמר ‫<ref>64v</ref>זה האחד הוא ז' כמורה וג' הרי י' נסיר מהם ו' ישארו ד‫' |
|} | |} | ||
::<math>\scriptstyle{\color{blue}{\left[\left(\frac{7}{7}\sdot\frac{1}{9}\right)+\left(\frac{3}{7}\sdot\frac{1}{9}\right)\right]-\left[\left(\frac{1}{7}\sdot\frac{1}{9}\right)+\left(\frac{5}{7}\sdot\frac{1}{9}\right)\right]=\left(\frac{10}{7}\sdot\frac{1}{9}\right)-\left(\frac{6}{7}\sdot\frac{1}{9}\right)=\left(\frac{4}{7}\sdot\frac{1}{9}\right)}}</math> | ::<math>\scriptstyle{\color{blue}{\left[\left(\frac{7}{7}\sdot\frac{1}{9}\right)+\left(\frac{3}{7}\sdot\frac{1}{9}\right)\right]-\left[\left(\frac{1}{7}\sdot\frac{1}{9}\right)+\left(\frac{5}{7}\sdot\frac{1}{9}\right)\right]=\left(\frac{10}{7}\sdot\frac{1}{9}\right)-\left(\frac{6}{7}\sdot\frac{1}{9}\right)=\left(\frac{4}{7}\sdot\frac{1}{9}\right)}}</math> | ||
| Line 6,975: | Line 8,359: | ||
::We subtract the dot, which is 1 integer, from the 3 integers; 2 remains. | ::We subtract the dot, which is 1 integer, from the 3 integers; 2 remains. | ||
::<math>\scriptstyle{\color{blue}{3-1=2}}</math> | ::<math>\scriptstyle{\color{blue}{3-1=2}}</math> | ||
| − | |style="text-align:right;"|עוד נסיר הנקודה שהוא א' שלם מהג' שלימים ישארו ב'‫] ב' שלימים | + | |style="text-align:right;"|עוד נסיר הנקודה שהוא א' שלם מהג' שלימים ישארו ב'‫]‫<ref>marg.</ref> ב' שלימים |
|} | |} | ||
:<math>\scriptstyle{\color{blue}{\left[3+\frac{5}{9}+\left(\frac{3}{7}\sdot\frac{1}{9}\right)+\left(\frac{2}{4}\sdot\frac{1}{7}\sdot\frac{1}{9}\right)\right]-\left[\frac{7}{9}+\left(\frac{5}{7}\sdot\frac{1}{9}\right)+\left(\frac{3}{4}\sdot\frac{1}{7}\sdot\frac{1}{9}\right)\right]=2+\frac{6}{9}+\left(\frac{4}{7}\sdot\frac{1}{9}\right)+\left(\frac{3}{4}\sdot\frac{1}{7}\sdot\frac{1}{9}\right)}}</math> | :<math>\scriptstyle{\color{blue}{\left[3+\frac{5}{9}+\left(\frac{3}{7}\sdot\frac{1}{9}\right)+\left(\frac{2}{4}\sdot\frac{1}{7}\sdot\frac{1}{9}\right)\right]-\left[\frac{7}{9}+\left(\frac{5}{7}\sdot\frac{1}{9}\right)+\left(\frac{3}{4}\sdot\frac{1}{7}\sdot\frac{1}{9}\right)\right]=2+\frac{6}{9}+\left(\frac{4}{7}\sdot\frac{1}{9}\right)+\left(\frac{3}{4}\sdot\frac{1}{7}\sdot\frac{1}{9}\right)}}</math> | ||
| Line 6,989: | Line 8,373: | ||
=== Discussion on the Decomposing and Composing of Fractions === | === Discussion on the Decomposing and Composing of Fractions === | ||
| − | + | |style="width:45%; text-align:right;"|<big>מאמר {{#annot:term|2614,1558|rzvM}}התכת{{#annotend:rzvM}}</big> השברים ו{{#annot:term|2490,1958|vWrI}}הרכבתן{{#annotend:vWrI}} או שתיהן יחד | |
|- | |- | ||
| − | | | + | |Since sometimes there is a need to convert denominators to other denominators when expanding and reducing, I thought to explain how one denominator is decomposed to two denominators. |
| − | + | |style="text-align:right;"|לפי שלפעמים יצטרך להשיב מורים למורים אחרים בהשואה ובכלילת יופי להוציאם מן הכלל ראיתי לבאר איך {{#annot:term|2615,2492|t3lA}}יותך{{#annotend:t3lA}} מורה אחד לשני מורים | |
| − | |style="text-align:right;"|לפי שלפעמים יצטרך להשיב מורים למורים אחרים בהשואה ובכלילת יופי להוציאם מן הכלל ראיתי לבאר איך {{#annot:term| | ||
|- | |- | ||
| − | | | + | |This is when the denominator is composed. |
| − | |||
|style="text-align:right;"|וזהו כאשר המורה מורכב | |style="text-align:right;"|וזהו כאשר המורה מורכב | ||
|- | |- | ||
| | | | ||
| − | ::Such as 6, which is composed of 2 and 3, so we remove it and replace it with 2, 3. <math>\scriptstyle{\color{blue}{6=2\sdot3}}</math> | + | ::Such as 6, which is composed of 2 and 3, so we remove it and replace it with 2, 3. |
| − | |style="text-align:right;"|כו' שהוא מורכב מב' וג' שנסירהו ונשים תחתיו ב'ג‫' | + | ::<math>\scriptstyle{\color{blue}{6=2\sdot3}}</math> |
| + | |style="text-align:right;"|כו' שהוא מורכב מב' וג' שנסירהו ונשים <sup>תחתיו</sup> ב'ג‫' | ||
|- | |- | ||
| | | | ||
| − | ::Also 3, 3 instead of 9. <math>\scriptstyle{\color{blue}{9=3\sdot3}}</math> | + | ::Also 3, 3 instead of 9. |
| + | ::<math>\scriptstyle{\color{blue}{9=3\sdot3}}</math> | ||
|style="text-align:right;"|וכן בעד ט' ג' ג‫' | |style="text-align:right;"|וכן בעד ט' ג' ג‫' | ||
|- | |- | ||
| | | | ||
| − | ::And 2, 4 instead of 8. <math>\scriptstyle{\color{blue}{8=2\sdot4}}</math> | + | ::And 2, 4 instead of 8. |
| + | ::<math>\scriptstyle{\color{blue}{8=2\sdot4}}</math> | ||
|style="text-align:right;"|ובעד ח' ב' ד‫' | |style="text-align:right;"|ובעד ח' ב' ד‫' | ||
|- | |- | ||
| − | | | + | |This is the rule: the product of the replacing denominators is the same as the removed [original denominator] |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{1}{a\sdot b}=\frac{1}{a}\sdot\frac{1}{b}}}</math> | |
|style="text-align:right;"|זה הכלל שכפל המורים המושמים תחתיו יהיה כמו המוסר | |style="text-align:right;"|זה הכלל שכפל המורים המושמים תחתיו יהיה כמו המוסר | ||
|- | |- | ||
| | | | ||
| − | *Sometimes we do the opposite that we replace the | + | *Sometimes we do the opposite, that we replace the two with one, i.e. we [remove] the 2 and the 4 and replace them with 8. |
| − | |style="text-align:right;"|ולפעמים נעשה להפך | + | :<math>\scriptstyle{\color{blue}{2\sdot4=8}}</math> |
| + | |style="text-align:right;"|ולפעמים נעשה להפך <sup>ש</sup>נשים הב' אחד ר"ל שנשים הב' והד' ונשים תחתיו הח' וכן בכללן | ||
|- | |- | ||
| | | | ||
| Line 7,024: | Line 8,410: | ||
|- | |- | ||
| | | | ||
| − | *Other times both | + | *Other times we need both. |
|style="text-align:right;"|ולפעמים נצטרך הכל | |style="text-align:right;"|ולפעמים נצטרך הכל | ||
|- | |- | ||
| | | | ||
| − | ::Such as when we have 6, 4 and we need 3,8. <math>\scriptstyle{\color{blue}{6\sdot4=3\sdot8}}</math> | + | ::Such as when we have 6, 4 and we need 3,8. |
| + | ::<math>\scriptstyle{\color{blue}{6\sdot4=3\sdot8}}</math> | ||
|style="text-align:right;"|כגון שיש בידינו ו' ד' ואנו צריכים ג' ח‫' | |style="text-align:right;"|כגון שיש בידינו ו' ד' ואנו צריכים ג' ח‫' | ||
|- | |- | ||
| | | | ||
| − | ::Or, when we have 3, 4 and we need 6, 2. <math>\scriptstyle{\color{blue}{3\sdot4=6\sdot2}}</math> | + | ::Or, when we have 3, 4 and we need 6, 2. |
| + | ::<math>\scriptstyle{\color{blue}{3\sdot4=6\sdot2}}</math> | ||
|style="text-align:right;"|או שיש בידינו ג' ד' ואנו צריכים ו' ב‫' | |style="text-align:right;"|או שיש בידינו ג' ד' ואנו צריכים ו' ב‫' | ||
|- | |- | ||
| − | | | + | |This is the rule: |
|style="text-align:right;"|זה הכלל | |style="text-align:right;"|זה הכלל | ||
|- | |- | ||
| | | | ||
| − | *If what we place is one instead of numerous, this number should be the same as | + | *If what we place is one instead of numerous, this number should be the same as the product of the denominators multiplied by each other. |
| − | :<math>\scriptstyle\frac{1}{a}\sdot\frac{1}{b}=\frac{1}{a\sdot b}</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{1}{a}\sdot\frac{1}{b}=\frac{1}{a\sdot b}}}</math> |
|style="text-align:right;"|אם אשר שמנו הוא א' במקום רבים צריך שיהיה מספרו ככפל המורים זה בזה | |style="text-align:right;"|אם אשר שמנו הוא א' במקום רבים צריך שיהיה מספרו ככפל המורים זה בזה | ||
|- | |- | ||
| | | | ||
*If numerous instead of one, their product by each other should be the same as the removed number. | *If numerous instead of one, their product by each other should be the same as the removed number. | ||
| − | :<math>\scriptstyle\frac{1}{a\sdot b}=\frac{1}{a}\sdot\frac{1}{b}</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{1}{a\sdot b}=\frac{1}{a}\sdot\frac{1}{b}}}</math> |
|style="text-align:right;"|ואם רבים תחת אחד שיהיה כפלם זה בזה כמספר המוסר | |style="text-align:right;"|ואם רבים תחת אחד שיהיה כפלם זה בזה כמספר המוסר | ||
|- | |- | ||
| | | | ||
| − | *If numerous instead of numerous, their product by each other [should be the same as the product of the others by each other] <math>\scriptstyle a\sdot b=c\sdot d\longrightarrow \frac{1}{a}\sdot\frac{1}{b}=\frac{1}{c}\sdot\frac{1}{d}</math> | + | *If numerous instead of numerous, their product by each other [should be the same as the product of the others by each other] |
| − | |style="text-align:right;"|ואם רבים במקום רבים שיעלה כפל אלו זה בזה ודי למבין | + | :<math>\scriptstyle{\color{OliveGreen}{a\sdot b=c\sdot d\longrightarrow \frac{1}{a}\sdot\frac{1}{b}=\frac{1}{c}\sdot\frac{1}{d}}}</math> |
| + | |style="text-align:right;"|ואם רבים במקום רבים שיעלה כפל אלו זה בזה | ||
| + | |- | ||
| + | |This is enough for the one who understands. | ||
| + | |style="text-align:right;"|ודי למבין | ||
|- | |- | ||
|} | |} | ||
| Line 7,062: | Line 8,454: | ||
| | | | ||
=== Addition === | === Addition === | ||
| − | |style="text-align:right;"|<big>החבור</big> | + | |style="width:45%; text-align:right;"|<big>החבור</big> |
|- | |- | ||
|Multiply the [numerator] whose denominator or denominators are smaller by the excess of the denominators of the other over its denominators, then divide [the product] by the smaller denominators. Add the result of division to the numerators of both [and the sum is] the parts of the denominator or the denominators of the greater. | |Multiply the [numerator] whose denominator or denominators are smaller by the excess of the denominators of the other over its denominators, then divide [the product] by the smaller denominators. Add the result of division to the numerators of both [and the sum is] the parts of the denominator or the denominators of the greater. | ||
| − | :<math>\scriptstyle{\color{ | + | :<math>\scriptstyle{\color{OliveGreen}{b>d\longrightarrow\frac{a}{b}+\frac{c}{d}=\frac{a+c+\frac{c\sdot\left(b-d\right)}{d}}{b}}}</math> |
|style="text-align:right;"|תכפול אשר מורהו או מוריו קטנים בתוספת מורי האחרת על מוריו ותחלקנו למורים הקטנים והיוצא בחילוק תחברנו לשברי שניהם חלקי המורה או המורים הגדולים | |style="text-align:right;"|תכפול אשר מורהו או מוריו קטנים בתוספת מורי האחרת על מוריו ותחלקנו למורים הקטנים והיוצא בחילוק תחברנו לשברי שניהם חלקי המורה או המורים הגדולים | ||
|- | |- | ||
| | | | ||
| − | *If | + | *If something remains from the first division, it is a part of all the denominators, of the greater and the smaller. |
|style="text-align:right;"|ואם ‫<ref>65r</ref>בחלוקה הראשונה ישאר דבר הוא חלק מכל המורים גדולים וקטנים | |style="text-align:right;"|ואם ‫<ref>65r</ref>בחלוקה הראשונה ישאר דבר הוא חלק מכל המורים גדולים וקטנים | ||
|- | |- | ||
| Line 7,078: | Line 8,470: | ||
|- | |- | ||
| | | | ||
| − | : | + | :Multiply 2 by 4; it is 8. Divide it by 3; the result of division is 2 and 2 remains. |
| − | |style="text-align:right;"|כפול הב' בד' יהיו ח' חלקם לג' ויצא בחלוק ב' וישארו ב' וב' אלו שיצאו בחלוק חברם עם הג' והב' שהם שברי שני המספרי' ויעלה הכל ז' חלקם לז' יעלה א' ולא נשאר דבר | + | |style="text-align:right;"|כפול הב' בד' יהיו ח' חלקם לג' ויצא בחלוק ב' וישארו ב‫' |
| + | |- | ||
| + | | | ||
| + | :Add the 2 resulting in the division to 3 and 2 that are the numerators of both numbers; the sum is 7. | ||
| + | |style="text-align:right;"|וב' אלו שיצאו בחלוק חברם עם הג' והב' שהם שברי שני המספרי' ויעלה הכל ז‫' | ||
| + | |- | ||
| + | | | ||
| + | :Divide it by 7; the result is 1 and nothing remains. | ||
| + | |style="text-align:right;"|חלקם לז' יעלה א' ולא נשאר דבר | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :The 1 resulting in the division is 1 integer. |
| + | |style="text-align:right;"|וזה האחד היוצא בחלוק הוא א' שלם | ||
|- | |- | ||
| | | | ||
| − | + | :If there were anything left, it would have been sevenths. | |
| + | |style="text-align:right;"|ואם היה נשאר דבר היה שביעיות | ||
| + | |- | ||
| + | | | ||
| + | :The 2 that remains from the first division are thirds of a seventh. | ||
| + | |style="text-align:right;"|והב' שנשארו בחלוקה ראשון הם שלישיות שביעית | ||
| + | |- | ||
| + | | | ||
| + | :We find that their total sum is one integer and two-thirds of a seventh. | ||
| + | |style="text-align:right;"|נמצא שעלה מחבורם אחד שלם ושתי שלשיות שביעית | ||
| + | |} | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{3}{7}+\frac{2}{3}=\frac{3+2+\frac{2\sdot\left(7-3\right)}{3}}{7}=\frac{3+2+\frac{2\sdot4}{3}}{7}=\frac{3+2+\frac{8}{3}}{7}=\frac{3+2+2+\frac{2}{3}}{7}=\frac{7+\frac{2}{3}}{7}=1+\left(\frac{2}{3}\sdot\frac{1}{7}\right)}}</math> | ||
| + | {| | ||
| + | |- | ||
| + | |If you wish, multiply the numerator of the one by the denominator of the other, then divide by its own denominator and add the result of division to the numerator of the other; [the result] are parts of the denominator of the other. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}+\frac{c}{d}=\frac{a+\frac{c\sdot b}{d}}{b}}}</math> | ||
| + | |style="width:45%; text-align:right;"|[ו]אם תרצה כפול שברי האחד במורי האחרת וחלקנו למורי עצמה והיוצא ב בחלוק חברם לשברי האחרת ויהיו חלקים ממורי האחרת | ||
| + | |- | ||
| + | | | ||
| + | *If something remains from the division, it is parts of the denominators of both. | ||
|style="text-align:right;"|ואם נשאר שום דבר בחלוקה הם חלקים ממורי שתיהן | |style="text-align:right;"|ואם נשאר שום דבר בחלוקה הם חלקים ממורי שתיהן | ||
|- | |- | ||
| | | | ||
| + | :Example: multiply 2 by 7; the result is 14. Divide it by 3; the result is 3 and 2 remains. | ||
| + | |style="text-align:right;"|המשל כפול ב' בז' יעלו י"ד חלקם לג' יצאו ד' וישארו ב‫' | ||
| + | |- | ||
| + | | | ||
| + | :Add the 4 to the 3 that is the numerator of the other; the sum is 7 and it is 7-sevenths, which is 1 integer. The 2 that remains from the division are 2-thirds of a seventh. | ||
:<math>\scriptstyle{\color{blue}{\frac{3}{7}+\frac{2}{3}=\frac{3+\frac{2\sdot7}{3}}{7}=\frac{3+\frac{14}{3}}{7}=\frac{3+4+\frac{2}{3}}{7}=\frac{7+\frac{2}{3}}{7}=1+\left(\frac{2}{3}\sdot\frac{1}{7}\right)}}</math> | :<math>\scriptstyle{\color{blue}{\frac{3}{7}+\frac{2}{3}=\frac{3+\frac{2\sdot7}{3}}{7}=\frac{3+\frac{14}{3}}{7}=\frac{3+4+\frac{2}{3}}{7}=\frac{7+\frac{2}{3}}{7}=1+\left(\frac{2}{3}\sdot\frac{1}{7}\right)}}</math> | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|חבר הד' לג' שהם שברי האחרת יעלו ז' והם ז' שביעי[ו]ת שהם א' שלם והב' שנשארו בחלוקה הם ב' שלשיות שביעית |
|- | |- | ||
| − | | | + | |It all comes down to one way. |
|style="text-align:right;"|והכל עולה לדרך אחד | |style="text-align:right;"|והכל עולה לדרך אחד | ||
|- | |- | ||
| Line 7,098: | Line 8,523: | ||
=== Subtraction === | === Subtraction === | ||
| − | + | |style="text-align:right;"|<big>החסרון</big> | |
|- | |- | ||
| − | |<math>\scriptstyle\frac{a}{b}-\frac{c}{d}=\frac{\frac{a\sdot d}{b}-c}{d}</math> | + | |Multiply the numerator of the greater by the denominator of the smaller, then divide the product by the denominator of the greater. Subtract the numerator of the smaller from the result of division and divide the [remainder] by the denominator of the [smaller]. The [result] are parts of the denominators of the smaller. |
| − | |style="text-align:right;"|כפול שברי הגדולה במורי הקטנה והעולה תחלקנו למורי הגדולה ומהיוצא בחילוק תחסר שברי הקטנה והעולה תחלקנו למורי הגדולה והנשאר הוא חלקים ממורי הקטנה והגדולה | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}-\frac{c}{d}=\frac{\frac{a\sdot d}{b}-c}{d}}}</math> |
| + | |style="text-align:right;"|כפול שברי הגדולה במורי הקטנה והעולה תחלקנו למורי הגדולה ומהיוצא בחילוק תחסר שברי הקטנה והעולה תחלקנו למורי הגדולה והנשאר הוא חלקים ממורי הקטנה <s>והגדולה</s> | ||
|- | |- | ||
| − | | | + | |If there is something left from the first division, they are parts of the denominators of the smaller and the greater. |
| − | + | |style="text-align:right;"|‫[ואם נשאר דבר בחלוקה ראשונה הם חלקים ממורי הקטנה והגדולה]‫<ref>marg.</ref> | |
| − | |style="text-align:right;"|‫[ואם נשאר דבר בחלוקה ראשונה הם חלקים ממורי הקטנה והגדולה] | ||
|- | |- | ||
| | | | ||
| Line 7,113: | Line 8,538: | ||
|- | |- | ||
| | | | ||
| + | :We multiply 3 by 8; the result is 24. We divide it by 4; the result of division is 6. We subtract 2 from it; 4 remains and they are 4-eighth, which is the remainder. | ||
:<math>\scriptstyle{\color{blue}{\frac{3}{4}-\frac{2}{8}=\frac{\frac{3\sdot8}{4}-2}{8}=\frac{\frac{24}{4}-2}{8}=\frac{6-2}{8}=\frac{4}{8}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{3}{4}-\frac{2}{8}=\frac{\frac{3\sdot8}{4}-2}{8}=\frac{\frac{24}{4}-2}{8}=\frac{6-2}{8}=\frac{4}{8}}}</math> | ||
|style="text-align:right;"|נכפול הג' בח' ויעלו כ"ד נחלקם לד' יצא בחילוק ו' נסיר מהם הב' ישארו ד' והם ד' שמיניות והוא הנשאר | |style="text-align:right;"|נכפול הג' בח' ויעלו כ"ד נחלקם לד' יצא בחילוק ו' נסיר מהם הב' ישארו ד' והם ד' שמיניות והוא הנשאר | ||
|- | |- | ||
| | | | ||
| + | :If there were anything left in the first division, it would have been quarters of an eighth. | ||
|style="text-align:right;"|ואם בחלוקה הראשונה היה נשאר שום דבר היה רביעיות שמיניות | |style="text-align:right;"|ואם בחלוקה הראשונה היה נשאר שום דבר היה רביעיות שמיניות | ||
|- | |- | ||
| Line 7,122: | Line 8,549: | ||
=== Multiplication === | === Multiplication === | ||
| − | + | |style="text-align:right;"|ההכאה | |
|- | |- | ||
| − | |<math>\scriptstyle\frac{a}{b}\times\frac{c}{d}=\frac{a\sdot c}{b}\sdot\frac{1}{d}</math> | + | |It was already suggested that there is no need but to multiply the numerator by the numerator and the result are parts of the denominators. |
| + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}\times\frac{c}{d}=\frac{a\sdot c}{b}\sdot\frac{1}{d}}}</math> | ||
|style="text-align:right;"|כבר נרמז שאין צריך כי אם לכפול השברים בשברים והעולה הוא חלקים מכל המורים | |style="text-align:right;"|כבר נרמז שאין צריך כי אם לכפול השברים בשברים והעולה הוא חלקים מכל המורים | ||
|- | |- | ||
| Line 7,133: | Line 8,561: | ||
|- | |- | ||
| | | | ||
| + | :Multiply 4 by 5; the result is 20 and they are 20-sixths of a seventh. Divide them by them; the result is 3-sevenths and 2-sixths of a seventh. | ||
:<math>\scriptstyle{\color{blue}{\frac{4}{7}\times\frac{5}{6}=\frac{4\sdot5}{6}\sdot\frac{1}{7}=\frac{20}{6}\sdot\frac{1}{7}=\frac{3}{7}+\left(\frac{2}{6}\sdot\frac{1}{7}\right)}}</math> | :<math>\scriptstyle{\color{blue}{\frac{4}{7}\times\frac{5}{6}=\frac{4\sdot5}{6}\sdot\frac{1}{7}=\frac{20}{6}\sdot\frac{1}{7}=\frac{3}{7}+\left(\frac{2}{6}\sdot\frac{1}{7}\right)}}</math> | ||
|style="text-align:right;"|הכה ד' בה' ויעלה כ' והם כ' שישיות שביעית וחלקם ‫<ref>65v</ref>עליהם ויעלה ג' שביעיות וב' שישיות שביעית | |style="text-align:right;"|הכה ד' בה' ויעלה כ' והם כ' שישיות שביעית וחלקם ‫<ref>65v</ref>עליהם ויעלה ג' שביעיות וב' שישיות שביעית | ||
| Line 7,142: | Line 8,571: | ||
|- | |- | ||
|Multiply the numerators of the greater by the denominators of the smaller, then divide the product by the denominators of the greater and the numerator of the smaller, considering them as denominators. | |Multiply the numerators of the greater by the denominators of the smaller, then divide the product by the denominators of the greater and the numerator of the smaller, considering them as denominators. | ||
| − | :<math>\scriptstyle{\color{ | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a}{b}\div\frac{c}{d}=\frac{a\sdot d}{b}\sdot\frac{1}{c}}}</math> |
|style="text-align:right;"|כפול שברי הגדולה במורי הקטנה והעולה חלקנו למורי הגדולה ושברי הקטנה בקחתך אותם למורים | |style="text-align:right;"|כפול שברי הגדולה במורי הקטנה והעולה חלקנו למורי הגדולה ושברי הקטנה בקחתך אותם למורים | ||
|- | |- | ||
| | | | ||
| − | *Example: we wish to divide 6-sevenths by 2-fifths. | + | *{{#annot:⁶/₇÷⅖|552|WIah}}Example: we wish to divide 6-sevenths by 2-fifths. |
:<math>\scriptstyle\frac{6}{7}\div\frac{2}{5}</math> | :<math>\scriptstyle\frac{6}{7}\div\frac{2}{5}</math> | ||
| − | |style="text-align:right;"|<big>המשל</big> רצינו לחלק ו' שביעיות על ב' חמישיות | + | |style="text-align:right;"|<big>המשל</big> רצינו לחלק ו' שביעיות על ב' חמישיות{{#annotend:WIah}} |
|- | |- | ||
| | | | ||
| Line 7,161: | Line 8,590: | ||
|- | |- | ||
|Multiply the numerators of the second by the numerators of the third, multiply the product by the denominator of the first and the result are parts of the numerators of the first [multiplied by] the denominators of the second and the third. | |Multiply the numerators of the second by the numerators of the third, multiply the product by the denominator of the first and the result are parts of the numerators of the first [multiplied by] the denominators of the second and the third. | ||
| − | :<math>\scriptstyle{\color{ | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a_1}{b_2}:\frac{a_2}{b_2}=\frac{a_3}{b_3}:X\longrightarrow X=\frac{a_2\sdot a_3\sdot b_1}{a_1}\sdot\frac{1}{b_2}\sdot\frac{1}{b_3}}}</math> |
| − | |style="text-align:right;"|כפול שברי השנית בשברי השלישית והעולה כפול אותו במורה הראשונה והעולה הם חלקים משברי הראשונה ומורי השנית והשלישית | + | |style="width:45%; text-align:right;"|כפול שברי השנית בשברי השלישית והעולה כפול אותו במורה הראשונה והעולה הם חלקים משברי הראשונה ומורי השנית והשלישית |
|- | |- | ||
| | | | ||
| − | *Example: we wish to know, if 3-sevenths are equal to 8-ninths, how much are 4-fifths equal to? | + | *{{#annot:³/₇÷⁸/₉=⅘÷X|567|VvVa}}Example: we wish to know, if 3-sevenths are equal to 8-ninths, how much are 4-fifths equal to? |
:<math>\scriptstyle\frac{3}{7}:\frac{8}{9}=\frac{4}{5}:X</math> | :<math>\scriptstyle\frac{3}{7}:\frac{8}{9}=\frac{4}{5}:X</math> | ||
| − | |style="text-align:right;"|המשל רצינו לידע אם ג' שביעיות שוים ח' תשיעיות ד' חמישיות כמה הם שוות | + | |style="text-align:right;"|המשל רצינו לידע אם ג' שביעיות שוים ח' תשיעיות ד' חמישיות כמה הם שוות{{#annotend:VvVa}} |
|- | |- | ||
| | | | ||
| Line 7,180: | Line 8,609: | ||
|- | |- | ||
|Multiply the numerator of the number by its denominators, then extract the root of the product, as written above and the result is the parts of its denominators. | |Multiply the numerator of the number by its denominators, then extract the root of the product, as written above and the result is the parts of its denominators. | ||
| − | :<math>\scriptstyle{\color{ | + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{\frac{a}{b}}=\frac{\sqrt{a\sdot b}}{b}}}</math> |
|style="text-align:right;"|כפול שברי המספר במוריו ומהעולה נוציא שרשו כמו שכתו' למעלה ויהיה חלקים ממוריו | |style="text-align:right;"|כפול שברי המספר במוריו ומהעולה נוציא שרשו כמו שכתו' למעלה ויהיה חלקים ממוריו | ||
|- | |- | ||
| | | | ||
| − | *Example: we wish to know the root of 2-eighths. | + | *{{#annot:√²/₈|439|5dVA}}Example: we wish to know the root of 2-eighths. |
:<math>\scriptstyle\sqrt{\frac{2}{8}}</math> | :<math>\scriptstyle\sqrt{\frac{2}{8}}</math> | ||
| − | |style="text-align:right;"|המשל רצינו לדעת שרש ב' שמיניות | + | |style="text-align:right;"|המשל רצינו לדעת שרש ב' שמיניות{{#annotend:5dVA}} |
|- | |- | ||
| | | | ||
| Line 7,195: | Line 8,624: | ||
| | | | ||
| − | === Additional rule for division of fractions === | + | === <span style="color:Green>Additional rule for division of fractions</span> === |
| | | | ||
|- | |- | ||
|In order to abbreviate the division operation further and to give a correct answer immediately, I saw it fitting to contrive and reverse it into multiplication by inverting the smaller - the numerator into denominator and the denominator into numerator. | |In order to abbreviate the division operation further and to give a correct answer immediately, I saw it fitting to contrive and reverse it into multiplication by inverting the smaller - the numerator into denominator and the denominator into numerator. | ||
| − | |style="text-align:right;"|ולקצר עוד מעשה החלוק <sup>ו</sup>להשיב מיד תשובה נכונה לכל שואל ראיתי לתחבל ולהחזירו להכאה בהפוך הקטנה השברים למורים והמורים לשברים | + | |style="width:45%; text-align:right;"|ולקצר עוד מעשה החלוק <sup>ו</sup>להשיב מיד תשובה נכונה לכל שואל ראיתי לתחבל ולהחזירו להכאה בהפוך הקטנה השברים למורים והמורים לשברים |
|- | |- | ||
| | | | ||
| − | *Example: if you are told in our first example: we wish to divide 6-sevenths by 2-fifths. | + | *{{#annot:⁶/₇÷⅖|552|KqBF}}Example: if you are told in our first example: we wish to divide 6-sevenths by 2-fifths. |
:<math>\scriptstyle\frac{6}{7}\div\frac{2}{5}</math> | :<math>\scriptstyle\frac{6}{7}\div\frac{2}{5}</math> | ||
| − | |style="text-align:right;"|המשל אם אמרו לך במשלינו הראשון רצינו לחלק <s>ב'</s> [ו'] שביעיות על ב' חמישיות | + | |style="text-align:right;"|המשל אם אמרו לך במשלינו הראשון רצינו לחלק <s>ב'</s> [ו'] שביעיות על ב' חמישיות{{#annotend:KqBF}} |
|- | |- | ||
| | | | ||
| Line 7,213: | Line 8,642: | ||
| | | | ||
| − | === Additional rule for proportions of fractions === | + | === <span style="color:Green>Additional rule for proportions of fractions</span> === |
| | | | ||
|- | |- | ||
|In proportions also reverse the first and return to multiplication. | |In proportions also reverse the first and return to multiplication. | ||
| − | |style="text-align:right;"|וכן בערכים הפוך הראשונה ותחזור להכאה | + | |style="width:45%; text-align:right;"|וכן בערכים הפוך הראשונה ותחזור להכאה |
|- | |- | ||
| | | | ||
| − | *I.e. in our example, when we say: if 3-sevenths equal 8-ninths, how much are 4-fifths equal? | + | *{{#annot:³/₇÷⁸/₉=⅘÷X|567|FSi7}}I.e. in our example, when we say: if 3-sevenths equal 8-ninths, how much are 4-fifths equal? |
:<math>\scriptstyle\frac{3}{7}:\frac{8}{9}=\frac{4}{5}:X</math> | :<math>\scriptstyle\frac{3}{7}:\frac{8}{9}=\frac{4}{5}:X</math> | ||
| − | |style="text-align:right;"|פי' במשלנו כאשר אמרנו אם ג' שביעיות שוים ח' תשיעיות ד' חמישיות כמה הם שוים | + | |style="text-align:right;"|פי' במשלנו כאשר אמרנו אם ג' שביעיות שוים ח' תשיעיות ד' חמישיות כמה הם שוים{{#annotend:FSi7}} |
|- | |- | ||
| | | | ||
| Line 7,233: | Line 8,662: | ||
|- | |- | ||
|} | |} | ||
| + | |||
| + | == Notes == | ||
| + | |||
| + | <div style="text-align: right;"><div class="mw-collapsible mw-collapsed"><div class="mw-collapsible-content"> | ||
| + | <br> | ||
| + | <references group=note/> | ||
| + | </div></div></div> | ||
| + | |||
| + | == Apparatus == | ||
| + | <div class="mw-collapsible mw-collapsed"><div class="mw-collapsible-content"> | ||
| + | <references /> | ||
| + | </div></div> | ||
== Appendix I: Glossary of Terms == | == Appendix I: Glossary of Terms == | ||
| Line 13,171: | Line 14,612: | ||
|style="text-align:right;"|אוקלידס | |style="text-align:right;"|אוקלידס | ||
|} | |} | ||
| + | </div></div> | ||
| − | = Appendix: Bibliography = | + | == Appendix II: Bibliography == |
'''Jacob Canpanṭon'''<br> | '''Jacob Canpanṭon'''<br> | ||
Castile, Spain, ca. 1430<br> | Castile, Spain, ca. 1430<br> | ||
Latest revision as of 05:00, 23 August 2022
לחכם ר' יעקב קפנתון ז"ל
Contents
- 1 Prologue
- 2 Introduction: The Positional Decimal System
- 3 Table of Contents
- 4 Section One: Integers
- 5 Section Two: Fractions
- 5.1 Introduction
- 5.2 Chapter One: Addition
- 5.3 Chapter Two: Subtraction
- 5.4 Chapter Three: Multiplication
- 5.5 Chapter Four: Division
- 5.6 Chapter Five: Proportions
- 5.7 Chapter Six: Roots
- 5.8 General Rules for Operations with Fractions
- 5.9 Short Rule for all Chapters on Fractions
- 5.10 Notes
- 5.11 Apparatus
- 5.12 Appendix I: Glossary of Terms
- 5.13 Appendix II: Bibliography
Prologue
| בעיני נכבדת | מאשר יקרת |
| אהבת נפש חשקתיך | ואני אהבתיך[note 1] |
| יפה פה תאר [ונחמד] מראה | החבר הנאה |
| ר' יואל ן' דאוד | |
| כל בניינה בנוי לתלפיות[note 2] | אשר נפשך חשקה בחכמת הלימודיות |
| מרחקת שוא ודבר כזב[note 3] תפל והתול | אין בה עקש ופתלתול[note 4] |
| מעוות לא תוכל לתקן וחסרון[note 5] לא תמלא | צדק תצדיק ויושר תעלה |
| אומרת על הן הן ועל לאו לאו | תחת לשונה דבש וחלב [note 6] |
| חכמת המספר בתה ואחותה | ולהיות יסודה וכלי אומנותה |
| קצור קצר לגלות מצפוניה | וזה כמה כתבתי עליה |
| ולהטעים דרכיה דרכי נועם [note 7] | |
| בלשון עם נועז[note 8] | |
| ללעוזות בלעז[note 9] | |
| בלי דופי ולעז | |
| החזיקתני ותאצילני להשיבו בלשון עברי | ויען לא ישרה נפשך ללמוד בלשון נכרי |
| ומשלים מבוארים | בהרחבת דברים |
| ולתת את שאלתך | ראיתי למלאת את תאותך |
| להתעסק בידיעות וחוכמות | ואם טרדות הזמן בלתי מסכימות |
| ולכל בהם חיי רוח נפש חיה יחיו | כי באשר עליהם יחיו |
| והיו כלא היו | ולכל שאר ידיעות המושכלות יהיו |
| ונפשם משכלת יחיו | אמרתי אשר מן האנשים חיו |
| יעזרם אלהים צור בו חציו חסיו[note 10] | |
| ואליהם אהלים ישליו[note 11] | |
| ושנות מספר יאתיו[note 12] | |
| רזה ודלה | ואם ידעתי חכמת המספר בזויה ונקלה |
| מיודעים ואלופים | בעיני קצת מתפלספים |
| בחכמות הבנויות על האיפשר | |
| חשקה נפשם ועמהם לבם נקשר | |
| [1]וכל לומדם בעיניהם יכשר | |
| ישבחוהו לאומים ותומכם מאושר | |
| ולהיות על שתי הסעיפים פוצח [פוסח][2][note 13] | בהם יוכל להשתרר ולנצח |
| ללכת כרצונו אנה ואנה[note 14] | |
| וכמקשה המלונה | כאשר בתוך המים ינוד הקנה[note 15] |
| פעם לאסור ופעם להתיר | יניע בראש ובשפה יפטיר |
| ולא יעמוד איש בפניהן | בחכמת הטבע ודומיהן |
| ובלומדי אזן [לא יפתחו][3] לשמוע כלימודים | לכן קצה נפשם בכל חכמת הלימודיות |
| להן יקראו זמן הנערות | |
| ולהם יתנו זמן הבחרות | |
| ילדות ושחרות | |
| אשר הם בלתי מקבלים מרות | ועריהן יגזור לבם בשרירות |
| שוים בכל נושאיהם | להיותם בעיניהם |
| כאלו דומים לעצמים | |
| שוים בכל ומפורסמים | |
| לא במקרים בפחות וביתר ובלתי מסכימים | |
| ועל זולתו לועגו ויתלוצצו | לכן בחרו באשר חפצו |
| לאיפשר החזיקו יד אם קרוב ואם רחוק | כי יהיה להם לשחוק |
| [כי יהיה להם לשחוק][4] | ולמחוייב ולנמנע לא ישימו חוק |
| ובתבונתם אין מספר | בכל חכמתם לא יסופר |
| אשר ימין ושמאל אין לנטות | כי אין עסקם בפשוטות |
| ומה יתרון לבעל הלשון[note 16] | מושגות בבבת עין ואישון |
| במדע ובהשכל מסויימים | ואם כה יאמרו חכמים |
| והאלהים עשה אותם ישר[note 17] | מה יעשו הסכלים אשר לא ידעו אי זה יכשר |
| והמה בקשו חשבונות רבים | |
| הבל מרבים מחסרים ומחברים | |
| אם מעטים ואם רבים | |
| כמה מעלות טובות | אחדים ועשרות |
| למאות לאלפים ולרבבות | מאליפות מרובבות[note 18] |
| מדרגה תחת מדרגה חונים | מי[נים] ממינים שונים |
| אלו יורדים ואלו עולים | דגלים דגלים שבילים שבילים |
| [5]אשר בחפצם מתנודדים | ואליהם רזום ירמזון על ידי דברים נעים ונדים |
| וברצונם יעקרו שוד וישנו את תפקידם | יען באפם יגזלו מאנשי ה[ה][6]ודם ומאודם |
| ומהם בפולי ועדשים וכל מיני זרעונים | מהם עושים בזוזים ודרכמונים |
| ולעשוק מסחרים | להונות חברים |
| אלו ואלו מונים | כמוכרים כקונים |
| זה עולה וזה יוריד | זה ישכיב וזה יחריד |
| זה ישפיל וזה ירים[note 19] | |
| זה יגנוב הדינרים | |
| וזה יאכל ב' ג' גרגרים[note 20] | |
| ירא וחרד על דברו | ואשר על יי שברו |
| [מהדק][7] מהמדקדקים | להחזיק ולהקים |
| להיתם פשע ולכלות אשם | להרים מכשול לכל יחטא ואשם |
| מונה ביתדות וקשרי אצבעות | נזהר מהיות מקצץ בנטיעות |
| ובראות עצמו איש אמונה | |
| ואת פושעים לא מנה | |
| מודה ומשבח לאלוהי מעונה | |
| אשר לו אצל תבונה | |
| וחלק לו בבינה | |
| הבדילו מן התועים ומעשה ידיהו כוננה[note 21] | |
| והיו ידיו אמונה | |
| הם ילכו איש אל אשר לבו פונה | |
| וכל אחד את רעהו יונה | |
| והוא שומר דרכי אל ומי כמוהו מונה[note 22] | |
| מחזיק במצותיו | |
| ואל משפטיו וחקותיו | |
| קורץ בעיניו | |
| מולל ברגליו | |
| מורה באצבעותיו[note 23] | |
| ורוע מזלו | על זה בחר מעוני שכלו |
| ולהתמיד צדקו | הלא טוב לו למלאת ספקו |
| לבל יחליף וימיר | |
| ולא יאכל גרגרים מראש אמיר[note 24] | |
| לחלוק לחקוק אותו בלוח ברזל בצפורן שמיר | |
| לנצח יוכל להבחן במופתיו | אהל בל יצען בל יסע יתדותיו[note 25] |
| בכל מיני אזון וחקור | יתברר ויתלבן בבחינה [ובכור][8] ובקור |
| הן כל אלה הדברי' מחלישים דעתי | |
| ומכבים אש גחלתי | |
| מרפים ידי לשמור משמרתי | |
| אהבה מקלקלת את השורה | וחבתן על כולם גברה |
| [9]לכן אני הקטן יעקב בן החכם ר' יצחק קנפנטון | |
| הלא היא גדר הענוה | ז"ל פרצתי חומה נשגבה |
| עד שקמתי בפניהם קימה שאין בה הדור | פרץ על פני פרץ בלי סדור |
| כי לאמת לבדה אחלוק הכבוד ארבה המשרה | |
| להעשר סידורה | |
| כמשפט הבכורה[note 26] | |
| וחברתי קיצור זה בדרכי המספר וטעמיהם | |
| ובר נותן טעם קראתיו | |
| כי מספר החכמים הברותיו | |
| וטוב טעמיהם מלאתיו | |
| כי לא באתי להראות גבורות | ואם ידע יודע הנסתרות |
| כי לא מלבי אראה נפלאות ונוראות | ולהורות הוראות |
| כאיש אשר לא |
אבל כמק |
| ואם מדעתי אמציא המצאות | |
| אפי' תיבה או אות | |
| הלא הנה טעיות | |
| ידועים ומפורסמים | הלא גם הם חכמים הרשומים |
| [...]קו | יענו כי יתנו עידיהם ואותי יצדיקו |
| וטענות התנצלותי ישמיעו | לרבים אותם יודיעו |
| וגם חוכמות |
ויאמרו אמת ואמונה |
| כי לא מחכמה שאל על זאת הקלה | |
| ולא בכוונה | |
| עזב את הגבירות מרבות השררה והגדולה | |
| ורבות בנות עשו חיל[note 28] ופירות | אשר להם סנסנים ופארות |
| ופשט ידו במלאכה כמבזה סורה וגזלה | |
| גלמודה ושכולה | |
| מונח בקרן זוית עצורה ועזובה דורש אין לה | |
| ומתוך היחס אשר בינו לבינה | כי מקוצר רוח דעת ותבונה |
| והניח את הספק צפון ונעלם | אחז את [...] המפורסם ובריא אולם[note 29] |
| כי אין לאל ידו לברר את המסופק ולעשר אומדות | והוא גם הוא יעיד על עצמו ולא ראה להודות |
| מבררים ומלבנים ב[.]ת שכלנו | כמונו היום אומדים [11]מדעתנו |
| וטענות התנצלותי זה חזקות ומוחזקות בידיהם | אחשוב זאת היתה כוונתי בעיניהם |
| עזרם ומגינם | ואת יסוד |
| ומעט הבנתי | אשר הוא קוצר השגתי |
| לא נקטף עודנו באבו[note 30] | יתד שהכל תלוי בו |
| תקוע במקום נאמן | חזק ומוחזק נטע נעמן |
| אכן יודע האמת ידע כי כוונתי לשום שמים | |
| לפתוח פתח בריח ודלתים[note 31] | |
| ולהציע הצעות ולהבין הבנות למלאכת השמים | |
| כי להקדים את המוקדם ולאחר את המאוחר | ולא ביניהם בין קל וחומר אבחן ואבאר |
| לבנות יסודות ולהעמיד עמודים חזקים ונכונים | |
| במיעוט שכלי המפורסם להעמיד הבית והחדרים אשר עליהם נשענים | |
| וזה אחל לעשות בעזרת שוכן | |
Introduction: The Positional Decimal System |
|||||||||||||||||||||
| The numerals [...] in the books of the Gentile sages are these [corresponding to Hebrew letters]: | הרשמים ה[...] במספר בספרי חכמי הגוים הם אלו | ||||||||||||||||||||
| |||||||||||||||||||||
| The last numeral is called Sifra [= zero], which is not a number. | וזה הרושם האחרון הנקרא סיפרא אינננו מספר | ||||||||||||||||||||
| The meaning of the decimal places: Each rank is given in this science its numerical role, as will be explained, to indicate the numerical ranks that follow it. | אכן הונח בזאת החכמה בכל מעלה | ||||||||||||||||||||
| These ranks start from the right. | ואלו המעלות מתחילות מהימין | ||||||||||||||||||||
| Each rank to the left is ten times the preceding [rank]. | וכל מעלה העולה היא לצד שמאל עולה עשר ידות מאשר לפניה | ||||||||||||||||||||
| The written ranks [= decimal places] | |||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
|
ר"ל שרושם המספר אשר במעלה הא' יהיו אחדים | ||||||||||||||||||||
|
ובשנית עשרות | ||||||||||||||||||||
|
ובג' מאות | ||||||||||||||||||||
|
ובד' אלפים | ||||||||||||||||||||
| And so on, so that these numerals: 30678002 are thirty thousands of thousands that are called millions, six hundred and seventy-eight thousand, and two. | וכן לעולם בענין שרשמים אלו 30678002 עולים שלשים אלפי אלפים הנקראים חשבונות [12]ושש מאות ושבעים ושמונת אלפים ושנים | ||||||||||||||||||||
| The significance of the zeros as a place holders: Since there are no tens and hundreds, here, as well as units of millions, the zeros are written instead of them, to indicate the rest of the numerals, because without them these numerals would indicat thirty-six thousand, seven hundred, and eighty-two [36782]. Deduce from this. | ולפי שאין בכאן עשרות ומאות גם אחדי חשבונות הושמו הספרות במקומם להורות מעלות שאר המספרים כי זולתם לא היו עולים רשמים אלו כי אם שלשים ושש אלף ושבע מאות ושמונים ושנים והקש על זה |
Table of Contents |
|||||||||||||||||||||||||
| I have divided this book into two sections: | וחלקתי הספר לב' חלקים | ||||||||||||||||||||||||
| The first section of integers | החלק הא' בשלמים | ||||||||||||||||||||||||
| The second section on fractions and in it six chapters, including an introduction, in which there are three parts. | החלק הב' [בשברים][13] | ||||||||||||||||||||||||
|
| ||||||||||||||||||||||||
| Discussion on conversion and summing. | [מאמ' ההמרה גם מאמר האחדות][14] | ||||||||||||||||||||||||
| There is in it also a rule that includes matters that are useful for all chapters of the fractions. | ועוד בו כלל אחד כולל עניינים מועילים לכל פרקי השברים |
Section One: Integers |
החלק הראשון בשלמים | |||||
|---|---|---|---|---|---|---|
Chapter One: Addition |
הפרק הא' בחיבור | |||||
Written Addition |
||||||
Description of the Procedure |
||||||
| When you wish to sum two or three numbers or more, set the rows of the digits one beneath the other, each rank beneath its corresponding, i.e. the units under the units, the tens under the tens, the hundreds under the hundreds, and so on. | כאשר תרצה לחבר ב' או ג' מספרים או יותר תשים שורות רשמי המספרים זו תחת זו כל מעלה תחת בת גילה ר"ל האחדים תחת האחדים העשרות תחת [15]העשרות והמאות תחת המאות וכן כולם | |||||
| Draw a line [beneath] all the rows. | ותשרט קו דיו על כל השורות | |||||
| Then, sum all the numbers that are in the first ranks in all the rows. | ותחבר כל המספרים הנמצאים בכל השורות במעלה ראשונה | |||||
|
ואם לא תמצא שם מספר כי אם סיפרות תשים | |||||
|
ואם תמצא מספר או מספרים עם סיפרות לא תחוש לסיפרות | |||||
|
ואם יעלה לעשר או עשרות מצומצמות בלא אחדים שים סיפרא תחת הקו במקום אותה המעלה ושמור העשר או העשרות והיו לאחדים בידך לחברם עם אשר תמצא במעלה הבאה אחריה | |||||
|
וכדי שלא תשכחם שים על ראש מספר המעלה הבאה אחריה נקודה או נקודות כמספר העשרות השמורים אשר הם לאחדים בידך | |||||
|
ואם יעלה לעשר או עשרות ואחדים שים מספר האחדים ההם תחת הקו במקום אותה המעלה ושמור העשר או העשרות לאחדים לחברם עד אשר תמצא במעלה הבאה אחריה | |||||
|
ואם למעלה הבאה אחריה לא תמצא כי אם סיפרות לא תחוש להם אחרי היות בידך עשר או עשרות לשום במעלה ההיא לאחדים ותשים העשרות ההם לאחדים תחת הקו כנגד המעלה ההיא | |||||
|
אכן אם לא היו בידך עשר או עשרות ומצאת במעלה ההיא כולה סיפרות תשים תחת הקו כנגד אותה המעלה סיפרא אחת כאשר הזכרתי במעלה הראשונה כשאין שם מספר כי אם סיפרות כי משפט אחד להנה | |||||
| Always proceed so that the tens that are resulted in a certain rank are units to be summed in the succeeding rank, or to be placed in [that rank] if you do not find there any number, whether all are zeros, or it is the end of the number. Do so always until [the digits] are complete. | וכן תעשה לעולם שהעשרות [16]שעלו בידך משום מעלה יהיו לאחדים בידך לחברם עם אשר תמצא במעלה שאחריה או לשומם במקומה אם לא מצאת שם מספר בין שהיה כלה סיפרות או שכלה כבר המספר ועשה כן לעולם עד כלותם | |||||
| What is obtained under the line is the result of the addition. | והיוצא תחת הקו הוא העולה מהחיבור ההוא | |||||
Example |
המשל | |||||
|
רצית לחבר מאתים וחמשת אלפים ושלשה עם שלש מאות ותשעים אלף וחמשה ועם שש מאות ועשרים וחמשת אלפים ושנים | |||||
|
שים הצורות ככה | |||||
|
- [Illustration of the procedure:]
205003 
205003 
205003 
205003 390005 390005 390005 390005 625002 625002 625002 625002 0 10 010
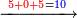
205003 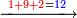
ֹ205003 
205003 390005 390005 390005 625002 625002 625002 0010 20010 1220010
|
ותאמ' 3 ו 5 הם 8 ו 2 הם 10 |
|
ואחר שאין לך אחדי' כי עם עשר שלם תשיבם 0 תחת הקו כנגד המעלה הראשונה |
|
ותשמר העשר לאחד למעלה הבאה אחריה ותשים נקדה אחת עליה שלא ישכח |
|
ואחר שלא מצאת שם מספר כי אם סיפרות ויש בידך עשר זה לא תחוש לסיפרות ההן ותשים [כנגד המעלה ההיא השנית][17] |
|
ולך אל המעלה השלישית ואחרי היות כלה סיפרות ויש בידך בלי מספר ואין בידך מאומה שים סיפרא אחת תחת הקו כנגד אותה המעלה השלישית |
|
ולך אל הרביעית ותמצא שם מספרים וסיפרא ואחר היות שם מספר או מספרים אל תחוש לסיפרא או סיפרות שיהיו שם |
|
ותאמר 5 ו5 הם 10 |
|
ותשים 0 תחת הקו כנגד אותה המעלה כאשר עשית במעלה הראשונה |
|
ותשמור |
|
ותאמ' אחד על השמור [18]ו9 הם 10 ו2 הם 12 |
|
שים השנים האחדים תחת הקו ושמור אחד על העשר ושים נקדה אחת על המעלה הבאה אחריה |
|
ותאמר אחד בעבור השמור ו2 הם 3 ו3 הם 6 ו6 הם 12 |
|
שים השנים האחדים תחת הקו כנגד המדרגה ההיא והעשר יהיו בידך לאחד למדרגה הבאה אחריה |
|
ואחר שכבר כלה המספר ואין ש[.....] מעלה שים העשר ההוא לא' במעלה הז' שהיא המעלה הבאה אחריה |
| Hence, you have already summed them and their sum is [1220010]. | וכבר חברת אותם ועלה חיבורם |
Check |
|||||
| If you wish to examine whether you did it rightly and correctly with no error | ואם תרצה להבחין אם עשית כדין וכשורה בלי טעות | ||||
|
חסר מזה העולה השורה האחת ומהנשאר תחסר השנית וכן כולם עד אשר לא תשאר מלחסר כי אם אחת והנשאר בעת ההיא יהיה שוה לאשר לא חסרת | ||||
|
כאשר בא בזאת הצורה | ||||
|
| ||||
|
כי כאשר חסרנו מאשר עולה חיבורם תחת הקו שהוא 1220010 וחסרנו ממנו שורת 625002 ונשאר 595008 ומזה הנשאר חסרנו השורה האחרת והוא שורת 390005 ונשאר 205003 השוה לצורה הנשארת אשר לא נחסרה עד הנה | ||||
|
וזה מה שרצינו לבאר | ||||
Reason: Procedure |
|||||
| The reason of the procedure is clear. | הטעם במעשה ברור | ||||
| For, every rank is ten times of the preceding rank. | כי כל מעלה עולה לעשר מאשר לפניה | ||||
| Therefore, the ten of the preceding rank is one in the succeeding rank. | א"כ העשר מהקודמת אינו כי אם אחד מהבאה אחריה | ||||
Reason: Check |
|||||
| The reason of the examination is also clear. | גם טעם הבחינה מבואר | ||||
| For, since the number that is under the line is generated from the sum of all the rows together, when we subtract them one by one it will be gone. | כי אחר שהמספר אשר תחת הקו נתחדש [19]מקיבוץ כל השורות יחד כאשר נסירם ממנה אחת יצא כלו בהם בשוה | ||||
| Hence, when only one is left to subtract, the remainder is the same as it, so when we subtract it from [the remainder] nothing is left. | ולזה כאשר [לא][20] נשאר מלחסר כי אם אחד יהיה הנשאר כמוה בענין שכאשר נסירה ממנו לא יחס' ולא ישאר | ||||
Chapter Two: Subtraction |
הפרק השני בחסרון | |||||
Written Subtraction |
||||||
Description of the Procedure |
||||||
| When you wish to subtract a small number from a greater number, set the smaller beneath the greater, each rank beneath its corresponding. | כאשר תרצה לחסו' מספר קטן ממספר גדול ממנו תשים הקטן תחת הגדול כל מעלה תחת מינה | |||||
| Draw a line beneath them. | ורשום קו דיו תחתיהן | |||||
| Then, subtract each bottom digit from the corresponding upper [digit] above it and put the remainder under the line in the corresponding rank. | וחסר כל מספ' תחתון מהעליון אשר על ראשו שהוא ממינו והנשאר שים אותו תחת הקו כנגד זאת המעלה | |||||
|
ואם לא תוכל לחסרו מאשר על ראשו שהוא קטן ממנו או 0 קח אחד מהמספר העליון אשר במעלה הבאה אחריה ויהיה לעשר במעלה ותשים נקדה אחת תחת הרושם העליון אשר ממנו לוית האחד | |||||
|
ואף אם היה שם 0 לא תחדל מהיות לווה ממנה ותשים תחתיה נקדה וזה האחד אשר לוית אשר הוא ל10 במעלה זו | |||||
|
||||||
|
ואם היה שם מספר במעלה הזאת יחבר ה10 עם אשר מצאת שם ומהכל תסיר המספר התחתון אשר ממינו והנשאר תשים תחת הקו כנגד המעלה ההיא | |||||
|
[ובלכתך למעלה אשר ממנה לוית האחד ושמת שם נקודה תוסיף אחד בעדו על אשר תמצא במעלה ההיא][22] בשורה התחתונה אם היה שם מספר ותחסר הכל מהמעלה ההיא מהשורה העליונה | |||||
|
ואם לא תמצא שם מחסורך אשר יחסר לך תלוה אחד מהמעלה הבאה אחריה ותשים תחתיה נקודה ויהיה לעש' במעלה זו כאשר ביארנו וכן לעולם | |||||
|
ואם במקום הנקודה [23]אין בשורה התחתונה מספר כגון שיש שם סיפרא או לא דבר שכלתה כבר השורה התחתונה תחסר אותו האחד מהמעלה אשר הנקדה תחתיה אם יש שם מספר והנשאר תשים תחת הקו כנגד המעלה ההיא | |||||
|
ואם אין בשורה ההיא כנגד הנקדה ההיא מספר כי אם 0 תלוה אחד מהמעלה הבאה אחריה ותשים תחתיה נקודה והאחד ההוא יהיה לעשר בידך ותחסר מהם האחד והנשארים תשימם תחת הקו כנגד המעלה ההיא | |||||
|
וכאשר כלית כל מלאכתך אם נשארו עוד רשמים בשורה העליונה אשר אין תחתיהן לא מספר ולא 0 ולא נקודה שכבר נשלם הכל תשימם לשארית תחת הקו כמות שהן | |||||
Example |
המשל | |||||
|
רצינו לחסר 40438 הקטן ממספר הגדול והוא 76540304 | |||||
|
נשימם הקטן תחת הגדול כזה | |||||
|
[זאת הצורה הב' היא ככה][24] | |||||
|
- [Illustration of the procedure:]
76540304 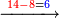
76540304 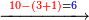
76540304 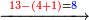
76540304 40438 40438 40438 40438 6 66 866
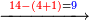
76540304 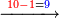
76540304 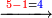
76540304 .40438 40438 40438 99866 9866 499866
|
ונתחיל לחסר מהמעלה הראשונה ונאמ' 8 מ 4 לא יוכלו לצאת ונלוה אחד מהמעלה העליונה הבאה אחריה אשר שם ה0 תשים תחתיה נקדה וזה האחד יהיה במעלה הראשונה לעשר ועם ה 4 אשר בה יהיו 14 |
|
נלך למעלה השנית נמצאנו שם נקדה הוספנוהו על ה 3 הנמצאים במעלה ההיא בשורה התחתונה יהיו 4 לא נוכל [25]לחסרם מה0 אשר בשורה העליונה במעלה ההיא לכן נלוה אחד מהמעלה הג' ונשים נקדה תחתיה ויהיה לנו לעשר נסיר מהם ה 4 ישארו 6 נשימם תחת הקו כנגד המעלה השנית ההיא |
|
נלך למעלה השלישית ונוסיף הנקדה על ה 4 אשר בשורה התחתונה יהיו 5 ולא נוכל להוציאם מהג' אשר על ראשם לכן |
|
ונלך למעלה הרביעית ונמצא שם נקדה ואין מספר במעלה הרביעית ההיא בשורה התחתונה ההיא לחברו עמו כי אם 0 לכן נחסר זה האחד לבדו מהנמצא במעלה הרביעית ההיא בשורה העליונה ולא נוכל כי אין שם מספר כי אם 0 לכן נקרא |
|
ונלך למעלה החמישית ונוסיף הנקדה הנמצאת שם עם ה 4 הנמצא במעלה הה' ההיא בשורה התחתונה ויהיו 5 ולא נוכל לחסרם מה 4 אשר על ראשם לכן [נלוה][26] א' מהמעלה השישית הבאה אחריה ונשים תחתיה נקדה ויהיו לנו ל 10 ועם ה 4 יהיו 14 נסיר מהם ה 5 ישארו 9[27] ונשימם תחת הקו |
|
ונלך למעלה השישית ומצאנו שם נקדה ואין כנגדה מספר בשורה התחתונה כי כבר נשלם לכן נסיר זה האחד לבדו מהה' אשר במעלה הו' ההיא בשורה העליונה וישארו 4 נשימם תחת הקו |
|
וכבר כלו אלו הרשמים אשר בשורה התחתונה גם הנקדות ונשארו שני רשמים בשורה העליונה נשימם תחת הקו לשארית כמו שהן על הסדר זה אחר זה |
|
וזה אשר יצא תחת הקו הוא אשר נשאר מהמספר הגדול אחר אשר חסרנו ממנו הקטן |
Reason: Check |
|
| We find that the greater number [= the subtracted] is as [the sum of] the smaller number [= the subtrahend] that we subtracted from it and the remainder together, no more and no less. | נמצא שמספר הגדול הוא כמו המספר הקטן אשר חסרנו ממנו וכמו זה הנשאר יחד בלי תוספת ומגרעת |
Check |
|
| Addition | |
|---|---|
| Therefore, when you wish to examine your procedure, add these two numbers that are beneath the upper [number], i.e. the smaller number that you subtracted and the remainder that is under the line, and if the sum is as the greater number, from which you have subtracted, no more and no less, then it is true and correct, and if not, know that you were wrong. | לכן כאשר תרצה להבחין מעשיך חבר אלו שני המספרים אשר תחת העליון ר"ל המספר הקטן אשר חסרת והנשאר אשר תחת הקו ואם יעלה כמספר הגדול אשר חסרת ממנו בלי תוספת ומגרעת הנה אמת הנה נכון ואם לאו דע שטעית |
| Apply this. | והקש על זה |
Chapter Three: Multiplication |
הפרק השלישי בכפל | |||||||
Written Multiplication |
||||||||
Description of the Procedure |
||||||||
| When you want to multiply a number by a number, i.e. to see how much are the multiples of one number when multiplied by the multiples of the other number, write the two forms of these numbers one above the other by the order and draw an ink line beneath them. | כאשר תרצה לכפול מספר במספר ר"ל לראות כמה יעלו כפלי המספר האחד כשיוכפל כפלים בחשבון המספר האחר שיש [שים][28] שתי צורות מספרים אלו זו על זו על הסדר ותרשום קו דיו תחתיהן | |||||||
|
וכפול המספר הראשון העליון בכל אחד מהמספרים התחתונים [וכאשר תעלה מכפלו עם כל אחד מהמספרים התחתונים תשים לעולם][29] תשים לעולם האחדים תחת הקו כנגד המספר התחתון ההוא והעשרות תשמור והיו לאחדים בידך לחברם עם העולה מכפלו עם המספר הנמשך אליו לצד שמאלי | |||||||
|
ואם לא [30]יהיה במעלה שאחר | |||||||
|
אכן אם כשתכפול המספ' העליון עם הסיפרא [...] לא יהיו בידך אחדים תשים סיפרא כנגד המעלה ההיא | |||||||
|
אבל אם יהיו בידך עשרות לאחדים שת[שימם] במעלה ההיא תחת הקו כנזכר ולא תשים סיפרא כלל | |||||||
|
ואחר שתשלים לכפול המספר הראשון העליון עם כל אחד מהמספרים התחתוני' תשוב כבתחלה ותכפול המספר השני העליון עם כל אחד מהמספרים התחתונים ותעשה ממנו שורה שנית | |||||||
|
ותשי' לעולם האחדים ותשמור העשרות לאחדים לחברם עם הבא אחריו כנזכר | |||||||
| You should know that the row of the product of each of the upper digits starts from the corresponding rank i.e. that when you start multiplying the second upper digit with the first bottom [digit], write the units resulting from that multiplication in the second rank that corresponds to that upper digit. The [row of the products] of the third upper digit starts from the third rank and so on. | אכן יש לך לדעת ששורת כפל כל אחד מהמספרים העליונים תתחיל מהמעלה הדומה לה ר"ל שכשתתחיל לכפול המספר השני העליון עם הראשון התחתון האחדים העולים מהכפל ההוא תשימם במעלה השנית הדומה למספר העליון ההוא וצורת המספר הג' העליון תתחיל מהמעלה הג' וכן כלם | |||||||
| Accordingly, the decimal place of the units of the product of any upper digit by any bottom digit is always as the sum of the decimal places of both digits minus one. | עד שיצא לנו מזה שלעולם מספר מעלות מקום אחדי כפל שום מספר עליון עם שום מספר תחתון יהיה כמנין מעלות שני המספרים מחוברות יחד חסר אחת | |||||||
|
ואחר שתשלים לכפול כל המספרי' העליונים עם התחתונים תרשום קו דיו תחת כל שורות אלו ותחברם יחד כל מעלה עם כל בת גילה כמו שביארנו בפרק החיבור והעולה הוא המבוקש והוא כפל שני המספרי' זה בזה | |||||||
|
ואם היה סיפרא בשורה העליונה מהנכפלים [32]היה נראה שיעשה ממנה שורה אחת כל הסיפרות ואין צורך כי בהיותך שומ | |||||||
|
אכן אם בתחלת השורה העליונה תמצא סיפרא או סיפרו' קודם שום מספר תצטרך לעשות בעד כל סיפרא מהן סיפרא אחת תחת הקו ותשלים [השורה] ההיא בכפל המספר הבא אחריהם בשורה העליונה על כל המספרים התחתונים | |||||||
|
ואם תרצה ג"כ כדי שלא תטעה [...] זה המעשה בעצמו לעולם ר"ל שתשים בעד כל סיפרא שבשורה העליונה אף אם הם באמצע סיפרא אחת במעלה הדומה למעלתה בשורה הראויה לסיפרא ההיא אם היה מספר ותשלים לשורה ההיא בכפל [המספר העליון הבא אחריהם] עם כל המספרי' התחתונים | |||||||
Example | ||||||||
|
המשל רצינו לכפול מספר 9007500 במספר אחר שהוא 5400920 | |||||||
|
ותשים המספרים זה על זה על הסדר כזה | |||||||
|
- [Illustration of the procedure:]
90070500 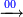
90070500 
90070500 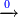
90070500 5400920 5400920 5400920 5400920 00 2700460000 2700460000 0
90070500 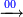
90070500 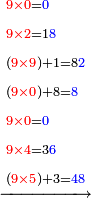
90070500 5400920 5400920 5400920 2700460000 2700460000 2700460000 378064400 378064400 378064400 00 4860828000
|
ולפי שבתחלת השורה העליונה שתי סיפרות תשים בתחלת השורה העליונ' בעדן תחת הקו שתי סיפרו' כמספרן |
|
ותשלים השורה ההיא מכפל המספר העליון הבא אחריהן והוא ה5 |
|
ותכפול ה5 בסיפרא שהיא אשר במעלה הראשונה מהמספר התחתון ויהיה סיפרא ותשימנה [33]אחר השתי סיפרות הנזכרות שהיא המעלה השלישית הדומה למעלה הרביעית והנה בא על מתכונתו ששורתו מתחלת במעלה הג' שהיא מעלתו |
|
ותאמ' אח"כ כפול 5 ב-2 הוא 10 תשים סיפרא אחר הסיפרות הנזכרות |
|
ותשמור ה-10 לאחד לחברו עם כפל 5 ב-9 שהוא 45 ויהיה הכל 46 ותשים ה6 אחר כל הסיפרות ותשמור ה-4 |
|
וכאש' תכפול ה5 בסיפרא הבאה אחר ה9 לא תשים סיפרא אחר היות בידך 4 ותשימם במקומה אחר ה6 |
|
אכן כאש' תכפול ה5 בסיפרא הנמשכת לא תשים סיפרא אחר ה4 אחר שאין בידך מאומה |
|
ותכפול 5 ב-4 ויעלו 20 תשים סיפרא ותשמר 2 |
|
ותכפול 5 ב5 ויהיו 25 ועם ה2 אשר בידך יהיה עם הכל 27 שים 7 ותשים אחריהם 2 כי כבר נשלם כפל המספר העליון בכל המספרים התחתונים |
|
ואחרי היותך מוצא אחר ה5 העליון הנכפל כבר היה הדין נותן לכפלה עם כל המספרים התחתונים ולעשות ממנו שורה אחת |
|
ואין צורך אלא שתתחיל מה7 אשר אחריה ותעשה שורתו בלבד שתתחיל שורת ה7 מהמעלה הה' אשר היא מעלתו כנזכר |
|
אכן כדי שלא תטעה תשים בראש שורת ה7 במעלה סיפרא בעד הסיפרא העליונה אשר בין הה' וה7 אשר מעלתה המעלה ה5 |
|
ותשלים השורה בכפל ה7 הבאה אחריה בכל הרשמים התחתונים |
|
ותאמ' כפל 7 בסיפרא הוא סיפרא ותשים סיפרא אחרת |
|
ותאמר עוד כפל 7 [ב2 ויעלו 14 תשים 4 שהם האחדים אחר הסיפרות הנזכרות ותשמור הא' |
|
ותאמר עוד כפל 7 ב9][34] ב9 ויעלה 63 ועוד האחד אשר בידינו יעלה 64 נשים ה4 ונשמר ה6 לאחדים בידינו |
|
[35]ונאמר עוד כפל 7 בסיפרא היה עולה סיפרא אכן להיות בידינו ה6 לא נשים סיפרא אבל נשים ה6 אשר בידינו למקומה |
|
ונאמר כפל 7 בסיפרא עולה סיפרא ונשים סיפרא אחר שאין בידינו אחדים כלל |
|
ונאמר כפל 7 ב4 עולה 28 נשים 8 ותשמור 2 |
|
ונאמר כפל 7 ב5 עולה 35 ועם השנים אשר בידינו יעלו 37 נשים 7 ואחריהם 3 כי כבר נשלם כפל זה ה7 על כל הרשמי' התחתונים |
|
ואחר היות שתי סיפרות בשורה העליונה אחר ה7 כדי שלא נטעה נשים שתי סיפרות כנגד מעלתן בהתחלת שורת ה9 הבא אחריהן |
|
ושוב נאמ' כפל 9 בסיפרא הוא סיפרא ותשים סיפרא אחר השתי סיפרות כאשר שמנו ב |
|
ונאמר כפל 9 ב2 עולה 18 נשים 8 אחר השלשה סיפרות הנזכרות ונשמור אחד |
|
ונאמר כפל 9 |
|
ונאמר כפל 9 בסיפרא הוא סיפרא ונשים ה8 אשר בידינו במקומה |
|
ונאמר כפל 9 בסיפרא הוא סיפרא ונשימה אחר שאין בידינו דבר שוב |
|
ונאמר כפל 9 ב4 עולה 36 נשים 6 ונשמור 3 |
|
ונאמר כפל 9 ב5 עולה 45 ו3 אשר בידינו יעלו 48 ונשים 8 ואחריהן |
|
ואחרי אשר כבר נכפלו ר"ל הרשמים העליונים עם כל התחתונים נרשום תחת כל השורות קו דיו ונחבר כל השורות שנתחדשו מכפליהן ר"ל ה3 שורות אשר [38]בין הקוים והנה |
|
יעלה בידינו שכפל הב' מספרים הנשאלים זה בזה עלה 486463564860000 |
Check |
|
|
ואם תרצה לבחון אם עשית כדין אם לאו יתחלק זה המספר הגדול העולה מהכפל לאחד מהב' מספרים הנכפלים ויצא בחילוק האחר ולא ישאר דבר ואם יחסר או יעדיף דע לך שטעית באחד המעשים בכפל או בחילוק |
Reason: Procedure |
|
| The reason for starting the line of multiplication of each upper digit by the bottom [digits] from its corresponding rank: | הטעם בהתחלת שורת כפל כל מספר עליון בתחתונים מהמעלה הדומה לו |
|
כי על ד"מ אם המספר העליון הוא מאות שהוא במעלה הג' כשנכפלם באחדי המספר התחתון יהיה העולה מאות |
|
ואם יהיה אלפים שהוא בד' יהיה העולה אלפים |
|
לכן כאשר יהיו [...] אחדים העולי' מהכפל הראשון ההוא במעלה הג' שהיא המעלה הדומה למעלתו והעשרות העולות מזה הכפל הם אחדים במעלה הבאה אחריהן כמו שנתבאר בתחלת הספר בפי' המעלות לכן תשמרם לאחדים לחברם עם הבא אחריהן |
|
וכשנכפול מספר עליון זה באשר במעלה השנית מהתחתונים יהיו העולה עשירי מאות שהם אלפים אם העליון הוא מאות |
|
ואם הוא אלפים יהיו העשרות האלה עשרות אלפים לכן נשימהו במעלה הנמשכת לאשר שמנו בתחלת שורה זו ונחבר להם השמור בידינו מהכפל הקודם |
|
וכן לעולם כאשר יתרחק יעלה מעלה אחר מעלה עד שיצא לנו מזה ברור מה שאמרנו כי אחדי כפל כל מספר עליון בתחתון יהיה מקום האחדים העולים מהכפל ההוא במעלה אשר מנין מדרגותיה כמנין מעלות שני רשמים [39]האלו הנכפלים זה בזה העליון והתחתון יחד חסר אחת |
|
וזה שאם האחד מהם במעלה הראשונה הרי ביארנו שמקומו הוא במעלה הדומה למעלתו |
|
ואם יהיה בשנית יעלה מעלה אחת על מעלות המספר האחר כמנין מעלותיו כמו שביארנו |
|
ואם הוא בג' יעלה שתים |
|
וכן יוסיף לעולם על מעלות המספר האחר כמנין מעלותיו |
|
והנה יהיה מעלות אחדי העולים מהכפל כמעלות שני הרשמים הנכפלים זה בזה חסר אחת וכל זה ברור בטעם |
Reason: Check |
וטעם הבחינה |
| Multiplication is the inverse operation of division. | הוא כי הכפל הוא הפך החילוק |
| Practical illustration: dividing a given amount of money between a certain number of people equally | |
| When a certain known number of people receive a known amount of money each | כי כאשר למין מה ממספר אנשים ידועים ועלה לכל אחד מהם מנין ממון ידוע |
|
הרי יש בכל הממון כפלי ממספר האנשים כמספר הממון העולה לכל אחד מהן |
|
או אם תרצה לומר כפלי הממון שיצא לכל אחד מהם כמספר האנשים ההם והכל אחד |
| For example: if we divide 12 golden coins between 3 people [equally], each of them receives 4.
|
כי המשל אם חלקנו 12 זהובים ל3 אנשים עלה לכל אחד מהם |
|
הרי השנים עשר הם כפל 3 ב4 או ה4 ב3 |
|
ואם נחלק |
|
ואם לג' יעלה לכל אחד מהם 4 |
| Hence, when we divide the result of multiplication by one of the multiplied numbers, the result of division is the second [multiplicand] no more and no less. | הרי שכאשר נחלק העולה מהכפל לאחד מהמספרים הנכפלים יצא השני בחילוק בלי תוספת ומגרעת |
| So, the check of the multiplication operation is by division, and the check of the division operation is by multiplication and this is an obvious thing. | הרי שבחינת הכפל בחילוק וכן בחינת החילוק בכפל וזה דבר ברור |
Chapter Four: Division |
[40]הפרק הרביעי בחילוק | |||||||||||
written division |
||||||||||||
| When you wish to divide a large number by a smaller number | כאשר תרצה לחלק מספר גדול למספר קטן | |||||||||||
description of the procedure |
||||||||||||
| We place them one above the other orderly: the greater above, we call it the dividend; the smaller beneath, we call it the divisor [lit. by which it is divided]. | ונשימם זה על זה על הסדר הגדול למעלה נקראנוהו המתחלק והקטן למטה וקראנוהו אשר נחלק עליו | |||||||||||
| Put every rank beneath its corresponding and | ותשים כל מעלה תחת בת גילה | |||||||||||
| These two lines should be spaced, i.e. leave a space between them, in order to put the result of division between them, as will be explained in the [description of the] division [procedure]. | ויהיו שתי שורות אלו מרווחות [..] ר"ל שתשים ריוח בין זו לזו לשים ביניהם היוצא בחילוק כאשר יתבאר בחילוק | |||||||||||
|
וראה המספר האחרון התחתון אשר לצד שמאל כמה פעמים יצא מהמספר האחרון אשר בעליון | |||||||||||
|
ואם איננו בו אפי' פעם אחת שהוא קטן ממנו ראה כמה פעמים יצא מזה האחרון ומאשר לפניו בקחתך האחרון לעשרות ואשר לפניו לאחדים ומנין פעמים אלו הוא הנקרא היוצא בחילוק | |||||||||||
|
ודע שיש לך להוציא כ"כ פעמים המספרים אשר לפני האחרון התחתון מאשר לפני האחרון או האחרונים העליונים אשר הוצאת מהם כפלי האחרון התחתון כפעמים אשר הוצאת האחרון התחתון מהאחרון העליו' או האחרונים | |||||||||||
|
[וכאשר נשאר מהאחרון או מהאחרונים בקחתך אותם לעשרות ולמאות כפי ערכם אל המעלה הזאת ככפלים אשר הוצאת האחרון התחתון מן האחרון העליון או האחרונים][41] | |||||||||||
|
[ואם אין בו][42] לא תוציא לאחרון ככל הכפלים ההם כי לעולם יש לך להוציא כל אחד כל פעמים מהעליון הראוי לו כפעמים אשר תוציא האחרון מן האחרון או מן האחרונים | |||||||||||
|
א[כן] יש לך לדעת כי בכל עת שתרצה להוציא התחתון מהעליון ואין דיו לכופליו כאשר תמצא במעלה הראויה לו שאם יש בנמשך אחר הנמשך תוכל להוציא ממנו ובלבד שתשמור לעולם ערך המעלות שכל מספר הוא במעלה שלפניו לעשרות ואשר לפני פניו למאות וכן לעולם [43]על הערך הזה | |||||||||||
|
ואחר שתדע הכפלים אשר תוכל להוציא כל אחד מהמספרים התחתונים מהמעלה או מעלות הראויות להם מהעליונים | |||||||||||
|
ר"ל כי עד"מ אם האחרון התחתון לקח מהמעלה הו' התחתון ואשר לפני האחרון יקח מו' ואשר לפני פניו מהה' עד כלותם | |||||||||||
|
ובמעלה אשר יכלו ר"ל שהראשון התחתון יש לו לקחת בפעם ההיא מהמעלה ההיא על סדר שביארנו כנגד המעלה ההיא תשים מנין הכפלים אשר לקחו ותשימם תחת המספר העליון | |||||||||||
|
וכאשר ישאר שום דבר משום מספר עליון תשים עליו השארית ושארית זה יהיה לעולם בין עיניך להועיל ממנו לעשרות או למאות לאשר לפניו ולפני פניו כמו שביארנו | |||||||||||
|
וכאשר תמו כל התחתונות לקחת מן הראויות להם אם נשאר עוד במספר העליון כמספר התחתון או יותר ממנו נשוב לחלקו עליו כבתחלה ונראה כמה פעמים יצא האחרון התחתון מהאחרון או אחרוני שארית זו כמו שעשינו בתחלה בכל המספר ואשר לפניו מאשר לפניו לכולם כפלים שווים כל אחד מהראוי לו | |||||||||||
|
וכן נשוב לעולם פעם אחר פעם עד הגיע עת יקח כל אחד מהתחתונים ממעלתו ממש ר"ל האחדי' מהאחדים והעשרות מהעשרות ומספר הכפלים יושם בעת ההיא במעלה הראשונה | |||||||||||
|
ולא נשוב עוד לחלק כי לא ישאר אז כי אם הפחות מהמספר התחתון | |||||||||||
|
והפחות על הרב לא יוכל לחלק לשלמים כי אם לשברים | |||||||||||
|
ועוד נזכיר בפרק זה איך יתחלק לשברים | |||||||||||
|
וזכור [44]לעולם שתשים בכל פעם היוצא בחילוק בפעם ההיא ר"ל לפעמים הכפלים אשר תוציא בפעם ההיא כנגד המעלה אשר משם [יקח][45] המספר הראשון התחתון ר"ל אשר יהיה במעלה הראשונה אם יהיה שם מספר [ואף אם לא יהיה שם מספר][46] כי אם סיפרא תראה מהיכן היה לו ראוי ליקח אם היה שם מספר | |||||||||||
|
ויצא לנו מכך כי כאשר נרצה לידע אי זה מקום ראוי לקחת ממנו שום מספר מהתחתונים בשום פנים שנראה מאי זו מעלה לקחת לאחרון שבתחתונים בפעם ההיא ותמנה משם לצד ימין מנין מעלות כמספר מעלות מרחק המספר ההוא התחתון [לצד ימין מהמספר האחרון התחתון][47] ובמקום שיכלו מהעליונות משם יקח | |||||||||||
|
גם כאשר תרצה לידע באיזה מקום תשים היוצא בחילוק בכל פעם ראה מאיזה מקום לקח האחרון התחתון בפעם ההיא ומנה משם לצד ימין כמנין רשמי התחתון וכאשר תכלה המנין ההוא שם תשים היוצא בחלוק בפעם ההיא ומהמעלה ההיא בעצמה יקח המספר אשר במעלה הראשונה בטור התחתון בעת ההיא | |||||||||||
example |
המשל | |||||||||||
| We wish to divide 4380408998 by a smaller number, which is 46079.
|
רצינו לחלק 4380408998 על מספר קטן ממנו והוא 46079 | |||||||||||
|
נשימם בשני טורים מרווחים זה על זה על הסדר כזה | |||||||||||
| ||||||||||||
| [אמ' משה זה טעות אבל האמת הוא כי היוצא בחלוק לכל א' [...] הוא זה 95000 שלמים נוסף על השברים][48] | ||||||||||||
|
ונאמ' מה שהוא המספר האחרון העליון יוכל לצאת 4 שהוא המספר האחרון התחתון פעם אחת | |||||||||||
|
אכן מד' אשר לפני האחרון העליון שהוא אשר לפני האחרון התחתון [49]לא נוכל לצאת 6 שהוא אשר לפני האחרון התחתון | |||||||||||
|
לכן לא נוציא משום [משם][50] דבר אבל נוציא מהשנים האחרונים שהם 43 | |||||||||||
|
ונאמר 43 כמה פעמים יש 4 | |||||||||||
|
ולא נאמר עשרה שהג' שמהמעלה הזאת היה יכול לקחת 10 היה לוקח מהאחרון אחרון שהוא 10 בערך המעלה הזאת | |||||||||||
|
לכן לא נאמ' כי אם 9 | |||||||||||
|
והנה השורה התחתונה היא 5 רשמים לכן לא נמנה מהג [3][51] העליון אשר לוקח משם 5 מעלות לצד ימין ויכלו בסיפרא ונשים תחתיה אלו ה9 היוצאים בחילוק שהוא מספר הכפלים אשר לנו להוציא התחתונים מהעליונים בפעם הזאת כל אחד מהמעלה הראויה לו כנזכר |
- [Illustration of the procedure:]

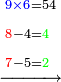
2 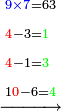
23 07 074 07441 4380408998 4380408998 4380408998 4380408998 9 9 9 46079 46079 46079 46079
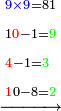
2332 074419 4380408998 9 46079
|
ונאמר 9 פעמים 4 הם 36 |
|
ואלו 6 האחדים היה לנו להוציאם מה3 וה3 עשרות מה4 |
|
ואין ב3 כדאי להוציאם מהם ה6 האחדים לכן נקח עשר אחד מה4 מוסיף על ה3 עשרות אשר יש לנו לקחת משם |
|
לכן נקח כל ה4 ונשים עליו סיפרא או נעביר עליו הקולמו' |
|
וזה העשר הנוסף אשר לקחנו נחברהו אל ה3 העליון ויהיו 13 נקח משם ה6 אחדים אשר לנו לקחת משם ישארו 7 ונשימם על ה3 |
|
והנה כבר לקחנו האחדים גם העשרות כל אחד מהמעלה הראויה לו |
|
ועוד נאמר 9 [פעמים][52] 6 הם 54 |
|
ונקח ה4 אחדים מהמעלה אשר לפני האחרונים העליונים הנזכרים שהוא ה8 וישארו 4 נשימם עליהם |
|
עוד נקח ה5 עשרות מהמעלה שאחריו לצד שמאל שהם עשרות בעדה |
|
עוד 9 פעמים 7 כי לא נחוש [53]לסיפרא התחתונה כי אם לשמירת המדרגות ר"ל שהיא מורה לנו שאין להוציא כפל 7 זה אשר לפניה ממקום הסיפרא העליונה אשר לפני ה8 אשר לקחנו משם ל6 אבל ממקום ה4 שהוא לפני ה8 2 מעלות כדרך שה7 לפני ה6 2 מעלות |
|
ונאמר 9 פעמים 7 הם 63 |
|
נסיר ה3 אחדים מה4 העליון הנזכר וישאר 1 ונשימנו עליו |
|
והיה ראוי לנו לקחת ה6 עשרות ממקום הסיפרא ואחר שאין שם כדאי להוציאם נקח אחד מהמעלה הבאה האחריה תמצא שם 4 נקח אחד ישארו 3 נשימם עליו |
|
וזה האחד הוא עשר בערך מעלת הסיפרא העליונה אשר משם נקח העשרות |
|
כי כל אחד מאלו העשרה הוא עשר בערך ה4 אשר משם לקח ה7 |
|
ונסור ה6 עשרות אשר עליו להוציא מאלו ה10 וישארו 4 ונשימם על הסיפרא |
|
עוד נאמ' 9 פעמים 9 והיו 81 |
|
וזה האחד היה לנו לקחתו ממקום הסיפרא העליונה אשר לפני 4 אשר לקחנו משם ל7 ואין שם דבר לכן נקח האחד אשר על ה4 ויהיה כאן עשרה נקח האחד ישארו 9 ונשימם עליה |
|
וה8 עשרות היה לנו לקחת אותם ממעלת ה4 ואין שם דבר כי אפי' האחד שיהיה שם כבר לקחנוהו ל[...] האחד לכן נקח אחד מהד' אשר על הסיפרא הבאה אחריה וישארו 3 ונשימם עליו |
|
וזה האחד יהיה לעשר על ה4 אשר משם נקח ה8 עשרות וכל אחד מאלו העשרה הוא עשר כערך מקום הסיפרא אשר משם לקח ה9 ונסיר ה8 [עשרות מעשר][54] עשרות אלו ישארו 2 ונשימם על ה4 ר"ל על האחד [55]שהיה על ה4 |
|
וכבר לקחו כל התחתונים כ"כ כפלים כל אחד מהראוי לו כאשר לקח האחרון התחתון מהאחרוני' התחתונים |
|
ונשאר לנו 233298998 במספר העליון שהוא יותר מהתחתון כמה כפלי כפלים |
|
לכן נשוב לחלק להם זאת השארית כאשר בתחלה ונרשום קו על כל הנשאר כדי שלא נתבלבל |
- [Illustration of the procedure:]
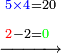
0 
00 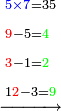
0029 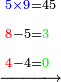
00290 2332 2332 23324 23324 074419 074419 074419 0744193 4380408998 4380408998 4380408998 4380408998 95 95 95 95 46079 46079 46079 46079
|
ונאמ' מהשנים השארית האחרון לא יוכל לצאת הד' האחרון התחתון אפי' פעם א' |
|
ונשים זה הה' תחת הראשון שהוא לה' מעלות מזה הג' אשר אנו לוקחים משם לצד ימין שהם כמספר מעלות השורה התחתונה |
|
ונאמ' ה' פעמי' 4 הם כ' |
|
ואחר שאין שם אחדים לא נקח מהג' דבר אבל הב' עשרות אלו נקח מהב' הבא אחריו ולא ישאר דבר ונעביר עליו ב' |
| [missing] | |
|
ועוד נאמ' ה' פעמים ז' הם 35 |
|
ואלו ה5 אחדים נקחם מה9 אשר על ה0 כי שם מקום לקיחתם במעלת 4 לצד ימין מה3 אשר על ה8 העליון אש' לקח רושם האחרון התחתון כמרחק זה הז' לצד ימין מה4 האחרון התחתון |
|
והג' עשרות נקחם מה |
|
וזה הא' הוא עשרה בערך השנים ונחברם אליהם יהיה 12 נקח מהם ה3 עשרות ישארו 9 ונשימם עליהם |
|
עוד נאמר 5 פעמים 9 הם 45 |
|
נסיר ה5 מה8 ישארו 3 |
|
נסיר ה4 עשרות מה4 ולא ישאר דבר ונשים עליו 0 |
|
והנה לקחו כל התחתונות ונשאר לנו במספר העליון 2943998 והוא הרבה מאד יותר מהתחתון |
|
ונשוב לחלקם להם ונרשום על זאת השארית קו דיו כדי שלא נתבלבל |
- [Illustration of the procedure:]
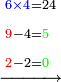
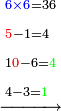
1 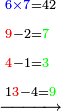
13 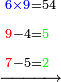
13 05 054 054 054 00290 00290 00290 00290 23324 23324 233249 2332492 0744193 0744193 07441937 074419375 4380408998 4380408998 4380408998 4380408998 9506 9506 9506 9506 46079 46079 46079 46079
|
ונאמר מ2 לא יצאו 4 אבל יצאו 4 מ29 ויצאו משם ז' פעמים ולא ישאר כי אם |
|
לכן לא נקח כי אם 6 ונשימם תחת ה9 שהוא ה5 לצד ימין מה9 כנגד [...] העליון אשר משם נקח לאחרון התחתון ר"ל שהוא כמנין מעלות השורה התחתונה |
|
ואחר שיש מעלה חלקה ממספר בין ה5 אשר יצא לנו בחילוק מתחלה וזהו אשר יצא לנו עתה נשים 0 במעלה החלקה ממספר כי זה מעשה ה0 כאשר ביארנו בתחלת הספר |
|
ונאמר 6 פעמים 4 הם 24 |
|
נקח 4 אחדים מה9 ישארו 5 ונשימם עליהם |
|
ונקח ה2 עשרות מה2 הבא אחריו ולא ישאר דבר ונעביר עליו הקולמוס |
|
ונאמ' 6 פעמים 6 הם 36 |
|
היה לנו לקחת ה6 אחדים מה0 אשר בזו השארית ולא נוכל לכן נקח אחד מה5 אשר משם נקח הג' עשרות |
|
ויהיה כאן עשרה נקח 6 אחדים ישארו 4 ונשימם עליהם |
|
עוד נקח ה3 עשרות מה5 וכבר לקחנו 1 הנה לא ישאר שם כי אם 1 ונשימהו עליו |
|
עוד נאמר [57]6 פעמים 7 הם 42 |
|
נקח השנים אחדים מה9 ר"ל אשר במעלת המאות שהוא ל4 מעלות לצד ימין מהמקום שלקח האחרון התחתון ישארו 7 ונשימם עליהם |
|
והיה לנו לקחת ה4 עשרות מה3 ואין בו די נקח אחד מה4 הבא אחריו וישארו 3 ונשימם עליו |
|
וזה ה1 הוא 10 במדרגת ה3 ונחברם ויהיו 13 נקח מהם ה4 עשרות ישארו 9 ונשימם עליהם |
|
ונאמר 6 פעמים 9 הם 54 |
|
ונקח ה4 מה9 ישארו 5 ונשימם עליהם |
| [missing] | |
|
והנה לקחו כולם ונשאר עוד בעליון 139258 והוא יותר מהתחתון |
|
לכן נשוב לחלקם עליהם נרשום קו דיו עליהם על השארית הנזכר |
- [Illustration of the procedure:]
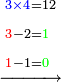
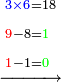
0 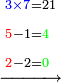
0 
0 01 01 01 01 13 13 13 13 054 054 054 054 00290 002901 0029010 00290102 2332492 2332492 23324924 23324924 074419375 074419375 074419375 0744193751 4380408998 4380408998 4380408998 4380408998 95063 95063 95063 95063 46079 46079 46079 46079
|
ונאמ' מא' אין די ל4 אבל נקח מי"ג האחרונים בשארית ויקח 3 פעמים ונשים ה3 הנמצא בחלוק תחת ה8 העליון שהוא במעלה הראשונה שהיא 5 ל3 זה אשר משם נקח לצד ימין שהוא כמנין מעלות השורה התחתונה | ||||||||
|
ונאמ' ג' פעמים 4 הם 12 | ||||||||
|
ונקח ה2 מה3 וישאר 1 ונשימהו עליו | ||||||||
|
ונקח ה1 מה1 ולא ישאר דבר ונעביר עליו הקולמוס | ||||||||
|
ונאמ' 3 פעמים 6 הם 18 | ||||||||
|
ונקח ה8 מה9 וישאר 1 ונשימהו עליו | ||||||||
|
ונקח ה1 מה1 ולא ישאר דבר ונעביר עליו הקולמוס | ||||||||
|
ונאמ' 6 פעמים | ||||||||
|
ונקח ה1 מה5 וישארו 4 ונשימם עליו | ||||||||
|
ונקח ה2 מה2 ולא ישאר דבר ונעביר עליו הקולמוס | ||||||||
|
ונאמ' 3 פעמים 9 הם | ||||||||
|
ונקח ה7 מה8 ישאר 1 ונשימהו עליו | ||||||||
|
ונקח ה2 עשרות מה4 ישארו 2 ונשימם עליו | ||||||||
|
הנה כבר לקחו כלם ונשארו 1021 והוא פחות מהתחתון ולא יוכל להתחלק [58]עליו לשלמים | ||||||||
|
לכן תאמר שכלית כל מלאכתך על השלמות | ||||||||
|
ושיצא בחילוק לכל אחד 95063 | ||||||||
|
ונשאר במספר העליון שלא יוכל להתחלק לשלמים 1021 | ||||||||
| We will discuss it further in this chapter. | ועוד נדבר בזה הפרק בעצמו | ||||||||
check |
|||||||||
| Multiplication: If you wish to check your procedure, to know if you if you were not mistaken. | ואם תרצה לבחון מעשיך לדעת אם לא טעית | ||||||||
|
כפול היוצא בחילוק ר"ל 950 | ||||||||
|
ואם לא יצא ראשון כמותו דע לך שטעית באחד המעשים ר"ל בחילוק או בכפל | ||||||||
|
כל זה תמצא רמוז בצורה הנזכרת | ||||||||
|
וכדי שתתלמד ארשום כאן בחינת המשל אשר הבאתי בפרק הג' בכפל והוא שנחלק העולה מהכפל ההוא והוא 486463564860000 [על אחד מהב' מספרים הנכפלים ויהיה תחלה ל5400920][59] | ||||||||
|
ונשימם זו על זו הגדולה למעלה כזה | ||||||||
|
- [Illustration of the procedure:]
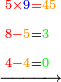
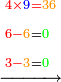
0 03 030 486463564860000 486463564860000 486463564860000 9 9 5400920 5400920 5400920
|
ונאמר מ4 לא יצאו 5 ויקחו מ48 ויהיו שם 9 פעמים ונ |
|
ונאמר 9 ב5 הם 45 |
|
נסיר ה5 מה8 ישאר 3 |
|
נסיר ה4 מה4 לא ישאר דבר |
|
[עוד נאמר 9 ב4 הם 36 |
|
נסיר ה6 מה6 לא ישאר דבר |
|
ונסיר][60] ונסיר ה3 מה3 לא ישאר ג"כ דבר |
- [Illustration of the procedure:]
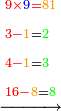
0 
0 0 030382 0303827 486463564860000 486463564860000 9 9 5400920 5400920
|
עוד נאמר 9 ב9 הם 81 |
|
ולדעת מאיזו מדרגה וקח [נקח][61] תעשה אחד מ2 דברים |
|
או תמנה מהמעלה [אשר שמת שם היוצא בחלוק שהוא ה6 לצד שמאל 3 מעלות כמרחק זה ה9 מהמעלה הא'][62] הא' ויכלו ב[3] העליון ומשם נקח זה האחד שעלה בכפל |
|
ואם תרצה תמנה מהמקום אשר משם לקח המספר האחרון התחתון [63]והוא ה8 [העליון 5][64] מעלות לצד ימין כמרחק זה ה9 מה5 המספר האחרון לצד ימין ויכלו ג"כ ג' |
|
ומשם נקח ה1 וישארו 2 |
|
ונקח ה8 עשרות מה6 ואין די ויקחו מה4 וישארו 3 וזה הראשון הוא לעשרה ועם ה6 יהיו 16 נסיר מהם ה8 ישארו 8 |
|
ונאמר עוד 9 ב2 הם 18 |
|
ומה5 לא יוכל לצאת ה8 לכן נקח 1 מה2 ועוד נקח משם 1 לעשר ולא ישאר דבר |
|
וזה ה1 הראשון אשר לקחנו יהיה עשרה ועם ה5 יהיו 9 נסיר מהם 8 ישארו 7 [ישאר 7][65] |
|
וכבר לקחו כל המספרים כי ה0 ולא תקח דבר |
- [Illustration of the procedure:]

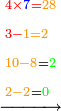
02 0 030 0 030 0303827 0303827 486463564860000 486463564860000 9007 9007 5400920 5400920
|
והנה נשאר בעליון 3807648606000 והוא רב מאד מהמספר התחתון לכן נשוב נחלקנו עליו ונרשום קו דיו עליהם |
|
ונאמ' מ3 אין די ל5 ונקח מ38 ויהיה בהם 7 פעמים 5 תשים זה ה7 היוצא בחילוק תחת ה6 כי שם יכלו הד' מעלות מזה ה8 לצד ימין אשר הם כמנין מעלות השורה התחתונה |
|
ונאמ' 7 ב5 הם 35 |
|
ונקח ה5 מה8 ישארו 3 |
|
וה3 מה3 לא ישאר דבר |
|
ונאמר 7 ב4 הם 28 |
|
וה8 לא יוכלו לצאת מה0 ונקח 1 מה3 ויהיה 34 נקח ה8 וישארו ב' ונשימם עליו |
|
ונקח עוד מה3 |
- [Illustration of the procedure:]
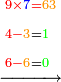
02 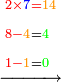
02 0 030 0 030 0 030382701 0303827014 486463564860000 486463564860000 9007 9007 5400920 5400920
|
עוד נאמר ז' בט' הם 63 |
|
ונקח ה3 מה4 שהיא מדרגה הראויה לו כמו שהזכרנו באחד מהב' דרכים אם להיותה שלישית לשמאל ואם להיותה חמישית לימין כמו שהוא ה9 וישאר א' מה4 ונשימהו עליו |
|
והו' עשרות נסירם מה6 ולא ישאר דבר |
|
עוד נאמר 7 ב2 הם 14 |
|
נסיר ה4 מה8 ישארו 4 |
|
ונסיר ה1 מ1 ולא [66]ישאר דבר |
|
והנה לקחו כלם ונשאר בעליון 2700460000 |
|
וה7 לפי שהוא תחת הקו הרשום תחלה ואולי תשכחהו שימהו על הקו הרשום ועוד תרשום קו על הכל ותשוב לחלקים אחרי היותם יותר מהתחתון |
- [Illustration of the procedure:]

0 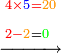
0 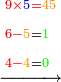
0 02 020 020 0 0302 0 0 0302 0 0 0302 00 0303827014 0303827014 03038270141 486463564860000 486463564860000 486463564860000 900705 900705 900705 5400920 5400920 5400920
|
ונאמר ב2 אין די ל5 ניקח מ27 ויהיו בו ה' פעמים ונשימהו תחת השלישית כי שם יכלו ה7 מעלות לצד ימין שהם כמנין מעלות השורה התחתונה | |||||||||
|
ונאמר 5 ב5 הם 25 | |||||||||
|
נסיר הה' מהז' ישארו שנים | |||||||||
|
ונסיר הב' מהב' ולא ישאר דבר | |||||||||
|
ונאמ' ה' בד' הם כ' | |||||||||
|
ולא נקח אחדים | |||||||||
|
אכן הב' עשרות נקחם מהב' אשר שמנו עתה על הז' ולא ישאר דבר | |||||||||
|
עוד נאמר ה' בט' הם מ"ה | |||||||||
|
נסיר הה' מהו' כי היא מעלה הראויה לו כנזכר וישאר א' | |||||||||
|
ונסיר הד' מהד' ולא ישאר דבר | |||||||||
[the last step is missing  ] ]
|
||||||||||
|
הנה לנו שכלה כל החשבון | |||||||||
|
ולהיות לנו בזה היוצא בחלוק מעלות חלקות מהמספר הן בתחלה הן באמצע נשים ספרות במקומם כי זה מעשה הסיפרות ותועלתם כאשר הזכרנו | |||||||||
| We receive that when we divide the product by one of the multiplicands, the second results no more and no less, with no remainder at all. | והנה יצא לנו שכאשר חלקנו העולה מהכפל באחת הנכפלים יצא השני בלי תוספת ומגרעת ובלי שארית כלל | |||||||||
| Likewise, if you divide by the other, the one results in the division, as appears in the following diagram: | וכן אם תחלקנה לחבירתה תצא חבירתה בחילוק כאשר בא בזאת הצורה | |||||||||
|
|
| |||||||||
| I do not want to elaborate it any further and all is clear to the one who understands. | ולא ראיתי להאריך בזה עוד והכל מבואר למבין | |||||||||
reason: procedure |
||||||||||
| The reason for the decimal place of the interim result of division: | ||||||||||
| The reason for the writing place of the result of division is that according to the rank [of the dividend] from which the first digit [of the divisor] is subtracted, so is the [rank of the number of] times that it is subtracted from it. | [67]וטעם מקום הנחת היוצא בחילוק הוא כי כפי המדרגה אשר ממנה לקח המספר הראשון הם הפעמים אשר לקחו | |||||||||
|
ר"ל שאם חלקנו שש מאות לג' אחדים | |||||||||
|
הנה יגיע לכל אחד ב' והמדרגה אשר ממנה לקח היא מדרגת המאות הנה השנים אשר יצאו בחלוק | |||||||||
|
ואם היו שם עוד אלפים בעליון ועשרות בתחתון הנה הפעמים אשר יגיעו לאחדים מהמאות יגיעו לעשרות מהאלפים | |||||||||
|
כי כמו שהעשרות הם עשרות לאחדים [ככה האלפים הם עשרות למאות הנה כאשר הגיע לאחדים מהמאות][68] מהמאות יגיעו לעשרות מהאלפים וכן למאות מהעשרות אלפים וכן לעולם כי כמו שזה עולה לאשר כמותו כן עולה זה | |||||||||
|
כבר ביארנו כי מדרגת הפעמים אשר הגיעו לאחדים היא מרוחק המדרגה אשר ממנו לקח | |||||||||
|
והיא בעצמה המדרגה אשר לקחו העשרות מהאלפים והמאות מהעשרות אלפים וכן כולם | |||||||||
| I.e. each of the bottom [digits] should be extracted from the rank [of the dividend] that is as far to the left of the rank, from which the [divisor's] units was extracted, as the number of ranks that it is far from the [rank of] units, i.e. as the number [of ranks] that the bottom [digit] is far to the left from the units [of the divisor]. | ר"ל שכל אחד מהתחתונות מדרגתה הראויה לקחת ממנה היא מרוחקת לצד שמאל [מהמדרגה אשר לקחו ממנה האחדים כמספר המדרגות אשר היא מהאחדים][69] מהאחדים ר"ל כמספר אשר זה התחתון לצד שמאל מהאחדים | |||||||||
|
שהרי המאות מדרגתם היא שלישית לצד שמאל מהאחדים וכן העשרות אלפים אשר ראוי לו לקחת מהם בקחת האחדים מהמאות כאשר זכרנו גם הם שלישיים לצד שמאל מהמאות אשר היא המדרגה אשר ממנה לקחו האחדים | |||||||||
| Apply this. | והקש על זה | |||||||||
| This includes the reason of the whole procedure. | ובזה נכלל טעם כל המעשה | |||||||||
reason: check |
[70]וטעם הבחינה | |||||||||
| For the multiplication is the inverse operation of division. | כי הכפל הוא הפך החילוק | |||||||||
| I.e. [the meaning of] division is to know how many times the small number is in the larger number. | ר"ל שהחילוק הוא לידע כמה פעמים המספר הקטון במספר הגדול | |||||||||
| Whereas [the meaning of] multiplication is [to know] how much is the sum of the multiples of a given number for a given number of times. | והכפל הוא כמה סך כפלי מספר ידוע פעמים ידועים | |||||||||
|
וכן אם בחלקנו כ' לה' יעלה לכל אחד ד' או לד' לכל אחד ה' | |||||||||
|
הנה כ' הוא כפל ד' בה' שהם המספר אשר חלקנו עליו והיוצא בחילוק | |||||||||
|
|
||||||||||
|
ואם חלקנו כ"א לד' [..] יעלה לכל אחד ה' וישאר א' | |||||||||
|
ולזה כאשר כפלנו ד' בה' ויעלה כ' הוספנו עליהם הא' הנשאר יעלה הכל כ"א שהוא כמספר המתחלק | |||||||||
| All this is clear. | וכל זה ברור | |||||||||
| Finding the proper fraction of the remainder from division: When you wish to add the smaller [number] that remains above the bottom number that is greater than it, or any small number that is above a greater number, you will find in the discussion on summing fractions chapter 1, section 2, a general method for all the numbers, whether they have divisors, or they are prime. | וכאשר תרצה לחבר המעט הנשאר על המספר התחתון שהוא גדול ממנו או שום אחד מספר קטן על מספר אחד גדול ממנו תמצא בחלק הב' בפרק הא' במאמ' האחדות אשר בו דרך כולל לכל המספרים בין יהיו להם מורים בין אם יהיו פשוטים |
divisibility of a number |
|
| To give you an inclusive method for dividing a large number by a smaller number and vice versa, I use the technique of the ancients: to extract the denominators of the number by which you want to divide, whether it is the smaller number or the greater number; that is to consider the numbers of which it is composed, if it is not a prime number. | אכן לתת לך דרך כולל בין לחלק רב למעט או בהפך דרכתי דרך הראשונים והוא שתוציא המורים מהחשבון אשר תרצה לחלק עליו אם מועט אם הרבה והוא ל[ראו'] המספרים אשר הוא מורכב מהם אם איננו פשוט |
3; 6; 9 |
|
| First, if you want to know if it has a third, a sixth, or a ninth [= if 3, 6, or 9 are divisors of the number]: | ראשונה אם תרצה לדעת אם יש לו שלישית או שישית או תשיעית מבלי שברים |
|
עיין אם האות הראשונה אשר במעלה הא' מהחשבון הוא נפרד תדע שאין לו שישית |
|
ואם הוא |
|
[71]וכל שיש לו תשיעית יש לו ג"כ שלישית ולא יתהפך |
|
ולדעת אם יש למספר תשיעית או שלישית הבט כל רשמי מספרי החשבון כאלו היו כולם מהמעלה הראשונה ר"ל שתחברם כלם כאלו היו אחדים וחסר כל ט' ט' שבחבור ההוא |
|
ואם יצא כולו תשיעיות תדע שיש לו תשיעית וכ"ש שלישית |
|
ואם ישארו ו' או ג' יהיה לו שלישית לא תשיעית |
|
ואם ישאר מספר אחר כמו ד' או ה' או הדומה להם אין לו אפי' שלישי' |
| Reasons | |
|---|---|
|
הטעם מה שא[נו] לוקחים כל רשמי המספרים לאחדים בלי עיון אל מעלותיהן הוא לפי שכל מעלה היא עשר בערך אל אשר לפניה בהסר מהעשרה תשע ישאר כמותה וכן כולם נמצא שלאחר הסרת התשיעיות כלם שוים |
|
ואמרנו שאם הרושם הראשון הוא מספר נפרד שאין לו שישית הוא לפי שכל החשבון בכללו |
|
ואמרנו שאם הוא זוג שאם יש לו שלישית יש לו ג"כ שישית הוא לפי שמאחר שהחשבון בכללו זוג מספר כפלי הג' ג' אשר בו הוא זוג שאם היה נפרד הנה היה מורכב מנפרד בנפרד והיה כולו בנפרד ואחר שהחשבון אשר בו הג' ג' הוא זוג א"כ הוא נחלק לזוגי ג' ר"ל לששה ששה והנה יש לו שישית על השלימות וזה מבואר |
2; 4; 8 |
|
| If you want to find out if it has a half, a quarter, or an eighth [= if 2, 4, or 8 are divisors of a the number] | ואם תרצה לדעת אם יש לו מחצית או רביעית או שמינית |
| Consider its first numeral [its units]: | [72]ראה הרושם הראשון |
| If it is an odd number, it does not have any of them [not divisible by 2, 4, or 8] - from the reason we have mentioned concerning the sixth [= 6 as a divisor]. | אם הוא חשבון נפרד הנה אין לו אח' מהם מהטעם שאמרנו בשישית |
|
ואם הוא זוג או 0' הרי כל החשבון בכללו זוג כי העשרות ומהם ולמ[ע][73]לה כלם זוג אחדים וא"כ בידוע שיש לו מחצית |
|
ולדעת אם יש לו ג"כ רביעית ושמינית |
|
|
|
קח המספר אשר במעלה הראשונה כמו שהוא |
|
ואשר בשנייה כפול אם יש שם מספר |
|
ואשר בשלישי' אם הוא נפרד קח בעבורו ד' אחדים ואם הוא זוג או 0 לא תקח בעבורו מאומה |
|
וכן מהמעלה השלישית ולמעלה לא תקח דבר |
|
וחבר כל אשר לקחת והשלך אותו ח' ח' |
|
|
|
|
ואם יצא הכל הנה יש לו שמינית ורביעית |
|
ואם ישאר [ארבעה][74] יש לו רביעית לבד |
|
ואם ישאר חשבון אחר אין לו לא שמינית ולא רביעית |
| The reason for doubling the digit of the tens in the sum: | |
| The reason we say that we take what is in the second rank as doubled is that they are tens and when you subtract 8 from each ten, 2 remains. So, we are left with two from each ten, therefore we double all the tens and take them doubled.
|
וטעם אומרנו שנקח אשר במעלה השנית כפול הוא לפי שהם עשרות ומכל עשר כאשר תסיר ח' ישארו ב' הרי שישאר לנו מכל עשר שנים לכן אנו כופלים כל העשרות ולכך אנו לוקחים אותם כפולות |
| The reason for not taking any digit for an even number of hundreds in the sum: | |
| What is in the third rank are hundreds and every even number of hundreds has an eighth [= is divisible by 8]. For the eighth of two hundred is 25. So, we do not take any thing for an even number of hundreds.
|
ואשר במעלה השלישית הם מאות וכל זוגי מאות יש להם שמינית כי שמינית מאתים הוא כ"ה לכן לא נקח בעבור זוגי המאות דבר |
| The reason for taking 4 for an odd number of hundreds in the sum: | |
| But, if there is an odd number of hundreds, after subtracting the even number of hundreds, we take 4 for it. Because, when subtracting the eights from one hundred, which are 12 eights that are 96, 4 remains.
|
אך אם יש שם מאה נפרד אחר הסרת זוגי המאות נקח בעבורו ד' כי בהסיר שמיניות המאה שהם י"ב שמיניות שהם עולים לצ"ו ישארו ד' |
| No need to take any thing for the ranks that are higher than the hundreds | |
| You do not take any thing for the third rank up, because all of them are an even number of hundreds, so they have an eighth [= are divisible by 8], as we have explained. | ומהמעלה השלישית ולמעלה לא תקח דבר כי כלם הם זוגי מאות ויש להם שמינית כמו שביארנו |
7 |
|
| If you want to find out if it has a seventh [= if 7 is a divisor of a given number] | ואם תרצה לדעת אם יש לו שביעית |
| See the final digit to the right and multiply it by 3, add [the product] to what you find in the preceding [rank], and cast out the sevens [from the sum]. Multiply the remainder by 3 and add [the product] to what you find in the preceding [rank]. If you do not find there any number, but 0, multiply [the product] again by 3, likewise for every 0, and add [the product] to what you find in the preceding [rank]. Then, cast out the sevens [from the sum, and so on repeatedly]. | ראה הרושם האחרון אשר לצד שמאל וכפלהו בג' וחברהו לאשר תמצא במעלה לאשר לפניה והסר לעולם [75]השביעיות והנשאר כפלהו בג' וחברהו עם אשר תמצא אשר לפניה ואם לא תמצא שם מספר כי אם 0' כפלהו פעם שנית בג' וכן על כל 0' וחברהו עם אשר תמצא לפניו והשלך לעולם הז' ז' |
|
ואם יצא הכל לשביעיות הרי ידענו שיש לו שביעית |
|
ואם לאו לאו |
| The reason for multiplying each rank by 3 and adding the product to the preceding rank: | |
| The reason that we multiply every rank by 3 [and add the product] to the preceding rank is that every rank is ten with regard to the preceding [rank] and when subtracting 7 from [10], 3 remains. Therefore, each unit is valued three with regard to the preceding [rank], after subtracting the 7 [from it]. | הטעם מה שאנו כופלים כל מעלה בג' לחברו לאש' לפניה הוא לפי שכל מעלה היא עשר בערך אשר לפניה ובהסר מהם הז' [ישארו ג' הנה כל אחד הוא כשלש בערך אשר לפניו אחרי הסרת הז'][76] |
5; 10 |
|
| If you want to find out if it has a tenth, or a fifth [= if 10, or 5 are divisors of a given number] | ואם תרצה לדעת אם יש לו עשירית או חמישית |
|
אם הרושם הראשון הוא 0 הנה הכל עשרות [כי אם המאות ומשם ולמעלה הכל הוא עשרות][77] והנה יש לו עשירית גם חמישית |
|
ואם הוא ה' הנה יש לו חמישית לבד |
|
ואם הוא מספר אחר גם חמישית אין לו |
11 |
|
| If you want to find out if it has 11th [= if 11 is a divisor of a given number] | ואם תרצה לדעת אם יש לו י"א |
| I.e. if it is completely divisible by 11, meaning that it is all cast out by elevens and nothing is left, the same as what we have said in all the preceding divisors. | פי' אם יתחלק לי"א על השלימות והוא שיושלך כלו י"א י"א ולא ישאר דבר וכיוצא בזה הוא מה שאמרנו בכל המורים העוברים |
| See the last digit and subtract 1 from what you find in the preceding rank. Subtract the remainder from what you find in the further preceding rank and so on repeatedly, until reaching to the beginning. | ראה הרושם האחרון והוצא ה1 מאשר תמצא במעלה אשר לפניו והנשאר הוציאנו מה שתמצא מאשר לפני פניו וכן עד הגיעו לראש |
|
ואם יצא הכל יש לו י"א י"א |
|
ואם לאו לאו |
| If you find a zero anywhere or any small number, from which you cannot subtract what I have instructed you, add 11 to what is found there, whether [it is] a zero or a small number, and subtract from the sum as I have instructed you, then [subtract] the remainder from what precedes and so on. | ואם בשום מקום תמצא סיפרא או שום מספר קטן במנין שלא תוכל להוציא ממנו אשר צוויתיך הוסיף י"א על הנמצא שם סיפרא 0 |
| The reason for the procedure: For every number is ten times with regard to the preceding rank, therefore when you take it as tens and what precedes it as units, it is as if all that you take are tens and units. | הטעם כי כל מספר הוא עשרה בערך במעלה אשר לפניו לכן כאשר תקחנו לעשרות ואשר לפניו לאחדים זה בזה הרי כל מה שלקחת הם י"א י"א |
| The reason for adding 11 to a small number: Our saying to add 11 to what precedes, when you do not find there enough to subtract, is that if we add some 11s to our number it neither raises nor decreases, for it is cast out by elevens anyway, either with the addition or without, and this is clear. | ואשר |
13 |
|
| If you want to find out if it has 13th [= if 13 is a divisor of a given number] | ואם תרצה לדעת אם יש לו י"ג |
| See the last digit and multiply it by 3, then cast out the thirteens that are in [the product] and subtract the remainder from what you find in the preceding rank. Multiply the remainder again by 3, then cast out the thirteens that are in [the product] and subtract the remainder from the preceding [rank] and so on until the [digits] end. | ראה הרושם האחרון וכפלהו בג' והוצא הי"ג י"ג אשר בו והנשאר הוציאהו מאשר תמצא במעלה אשר לפניו והנשאר כפלהו שנית בג' והוצא הי"ג אשר בו |
|
ואם יצא הכל יש לו י"ג |
|
ואם לאו לאו |
| If you do not find not enough in a certain rank to subtract as I have instructed you, add 13 and subtract from the sum what you need to subtract. Then multiply the remainder by 3 and cast out the thirteens. Subtract the remainder from the preceding [rank] and so on. | וכאשר יחסר בשום |
| The reason for the procedure: Because every number is ten times its value with regard to the preceding rank. Hence, when you subtract it and you subtract 3 times as much as it in the preceding rank, which is as units with regard to it, all that you subtract is as tens and 3 units for each ten. So each number are 13s. | הטעם כי כל מספ' הוא עשרה כערך אשר במעלה הקודמת וכאשר תסירנו ותסיר ג' שכמותו מהמעלה הקודמת שהי' לה לאחדים הרי כל אשר הוצאת הוא עשרות וג' |
| Furthermore, the addition of the 13 that I have instructed you to add does not harm the extraction procedure of 13, as we have noted concerning the addition of 11 in the extraction procedure of 11 as a divisor. This is clear. | גם התוספת אש' ציויתיך להוסיף מהי"ג לא יזיק בהוצאת הי"ג כאשר הזכרנו בהוספת הי"א בהמצאת מורה הי"א וזה מבואר |
general rules |
|
|
והמספר אשר לא תמצא לו אחד מהמורים הנזכרים ותרצה לידע אם יש לו מורה אחר המורה הזה אשר תבקש הוא שלא יהיה לו שום מורה מהמורים |
|
הטעם שאם היה לו שום מורה מהם בידוע שאינו מורה לזה החשבון שאם הוא מורה לזה החשבון הנה לחשבון ג"כ יש לו המורה אשר לזה המורה ואתה לא מצאתו |
|
המשל אם יקח כ"א הנה אם [80]כ"א הוא מורה לחשבון הנה יש לחשבון מורי זה המורה ר"ל שביעית ושלישית וכבר ידעת שאין לחשבונך אחד מהם לכן לא יהיה לו כ"א |
|
גם אם מספר אשר בקשת לו כל המורים העוברים זה אחר זה ולא מצאתם אם הוא פחות ממרובע המורה הסמוך אשר תרצה לבקש אינך צריך לבקשו כי איננו לו מורה אם לא מורה אחר בעולם כי בידוע שהוא מספר פשוט |
|
הטעם שאם היה לו זה המורה |
|
כי כל דבר הנחלק למספר מה ויצא בחילוק מספר מה ולא נשאר דבר הנה שניהם לו מורים כי כאשר יתחלק לאשר יוצא עתה בחילוק יצא בחלוק אשר נחלקו עליו עתה ולא ישאר דבר |
|
ואולם להיות מספר פעמים אלו פחות ממספר מורה המבוקש הלא הם כאחד המורים הקודמים וא"כ היה לו אחד מהמורים הקודמים ואתה לא מצאתם |
|
המשל אם בקשת עד י"ז ולא מצאת ותרצה לבקש אם יש לו י"ז ומספרך ואם הפעמים האלה אשר י"ז בו הם פחות מי"ז המשל י"ו ומשם ולמטה הנה היה לו רביעית או אחד מהמורים הקודמים שהרי יתחלק למספר פעמים אלו ג"כ ויצא בחילוף הי"ז וזה שקר [81]שהרי לא מצאת לו אחד מהעוברים |
|
ואולם כאשר יהיה חשבונך כמרובע המורה הנמשך אשר תבקש או גדול ממנו ותרצה לדעת אם תמצא לו זה המורה הסמוך |
|
ואם יתחלק אליו לשלימים מבלי שארית הוא לו למורה צדק גם היוצא בחילוק |
|
ואם לאו לאו |
Repetitive division of a number by its [divisors] |
|||||||||||||||||||||||||
| When you know that it has a divisor, divide the whole number by this divisor, and the result of division will be an integer. | וכאשר ידעת שיש לו שום מורה חלק המספר כולו לזה המורה יתחלק אליו לשלימים והיוצא בחילוק | ||||||||||||||||||||||||
| If you do not want to seek for more, then these two numbers, i.e. the divisor, by which you divide, and the result of the division are both its divisors. | ואם לא תרצה לבקש יותר הנה אלו השני מספרים ר"ל המורה אשר חלקת עליו והיוצא בחילוק הם הם מוריו | ||||||||||||||||||||||||
| If the result of division is a large number and you want to seek its divisor, proceed according to the mentioned methods. | אכן אם היוצא בחילוק הוא חשבון גדול ותרצה לבקש לו מורה ג"כ עשה כדרכים הנזכרים | ||||||||||||||||||||||||
| But, know that [the numbers] you find that are not divisors of the large number, you will find that they are not divisors of the result of division and this is clear. You should not seek for one of [these numbers], only for those that are similar to the one that you have found or greater than it. | ואולם דע שהמורים אשר לא מצאת לחשבון הגדול לא תמצאם ג"כ לזה היוצא בחילוק וזה ברור ואין לך לבקש אחד מהם כי אם הדומה לאשר מצאת או למעלה ממנו | ||||||||||||||||||||||||
| If you find its divisor, divide it by it and the result of division is a third divisor. | אם מצאת לו מורה חלקנו עליו והיוצא בחילוק יהיה מורה שלישי | ||||||||||||||||||||||||
| If this [result] is also a large [number] and you want to seek its divisor also, proceed as mentioned, divide it by the divisor that you have found to be its divisor and the result of division is its fourth divisor. | ואם זה ג"כ גדול ותרצה לבקש לו ג"כ מורה אחר עשה כנזכר וחלקנו על המורה אשר ידעת אשר הוא למורה לו והיוצא בחילוק יהיה לו למורה רביעי | ||||||||||||||||||||||||
| If you want, you can seek also for its fifth, or sixth, or other [divisors]. | ואם תרצה תוכל ל[...] לבקש עוד חמישי או שישי או זולתם | ||||||||||||||||||||||||
|
המשל רצינו לחלק 2447235 על 50335084800 | ||||||||||||||||||||||||
|
נוציא [....] המורים לזה החשבון הגדול אשר רצינו לחלק עליו | ||||||||||||||||||||||||
|
ונראה ראשונה אם יש לו ג' או ששה או ט' | ||||||||||||||||||||||||
|
ואחר שהרושם הראשון הוא 0 ידענו שאם יש לו ג' או ט' יש לו ג"כ ששה | ||||||||||||||||||||||||
|
ולדעת אם יש לו ג' או ט' נחברם כלם כאלו הם אחדים | ||||||||||||||||||||||||
|
ונאמ' ח' עם ד' הם י"ב נוציא מהם הט' ישארו [.] ג' | ||||||||||||||||||||||||
|
ועם 8 הם י"א נסיר הט' ישארו ב' | ||||||||||||||||||||||||
|
ועם ה' יהיו ז' [82]ועם הג' יהיו עשרה נסיר ט' ישארו | ||||||||||||||||||||||||
|
ועם הג' יהיו ד' ועם הה' יהיו כולם ט' | ||||||||||||||||||||||||
|
|
|||||||||||||||||||||||||
|
וידענו שיש לו ט' וג' גם ו' לסבה שזכרנו | ||||||||||||||||||||||||
|
ונקח מהם אשר תרצה | ||||||||||||||||||||||||
|
ועל דרך משל נקח למורה ו' ונחלקנו על ו' ויצא בחלוקם 8389180800 | ||||||||||||||||||||||||
|
ואחר שהיוצא בחילוק הוא חשבון גדול נבקש לו מורה ונעשה לזה כאשר לחשבון הראשון ויצא הכל לט' ט' | ||||||||||||||||||||||||
|
ואחר שהרושם הראשון סיפראויש לו ט' יש לו ג"כ ו' וכ"ש ג' | ||||||||||||||||||||||||
|
ונקח ט' למורה ונחלק זה החשבון לט' ויצא בחילו' 932131200 | ||||||||||||||||||||||||
|
ונעשה לזה כאשר עשינו לקודמים וישארו ג' | ||||||||||||||||||||||||
|
הנה יש לו ג' ג"כ ו' להיות הראשונה סיפרא | ||||||||||||||||||||||||
|
ונקח למורה ג' ונחלקנו עליו ויצא בחילוקם 310710400 | ||||||||||||||||||||||||
|
ואחר שהקודם לא היה לו ט' בידוע שלזה אין לו אפי' ג' | ||||||||||||||||||||||||
|
הטעם לפי שזה שלישית הקודם ואם לזה היה לו שלישית | ||||||||||||||||||||||||
|
וכן הוא האמת כאשר תחברם ותוציא הט' ט' ישארו ז' | ||||||||||||||||||||||||
|
ונעיין אם יש לו ב' וד' או ח' | ||||||||||||||||||||||||
|
ואחר שהראשון סיפרא בידוע שיש [...] לו ב' | ||||||||||||||||||||||||
|
ולדעת אם יש לו ד' או ח' היה לנו לקחת אשר [במעלה] הראשונה ולא מצאנו שם כי אם 0 ולא נקח דבר כי אם בשנית היה לנו לכופלו ולא מצאנו שם כי אם 0 לא נקח דבר והשלישית אחר שהוא זוג אין לנו לקחת בעבורה דבר ולא ממנה ולמעלה הנה יש לו ח' וד' וב' | ||||||||||||||||||||||||
|
ונקח למורה אשר נחפוץ מהם | ||||||||||||||||||||||||
|
המשל ח' ונחלקנו לח' ויצא | ||||||||||||||||||||||||
|
ולזאת ג"כ יש לה שמינית ורביעית וחצי לסבה הנזכרת | ||||||||||||||||||||||||
|
ונקח א' מהם | ||||||||||||||||||||||||
|
המשל ד' וחלקנו עליו ויצא בחילוק 9709700 | ||||||||||||||||||||||||
|
[83]ולזאת אין לה שמינית כי הראשונה והשנית הם 0 ולא נקח בעבורם דבר והשלישית היא ז' שהוא נפרד ונקח בעבורו ד' הנה שאין לו כי אם ד' וב' | ||||||||||||||||||||||||
|
ונקח אחד מהם | ||||||||||||||||||||||||
|
המשל ב' ונחלקנו עליו ויצא בחילוק 4854850 | ||||||||||||||||||||||||
|
מאחר שזו היא מחצית הראשונה אין לנו רביעית לפי שלא היה לראשונה שמינית כי רביעית זו היא רביעית חצי הראשונה שהוא שמינית | ||||||||||||||||||||||||
|
וכן תמצאנו בבחינה שאין לו רביעית כי בראשונה אין מספר וכל השניה היא עשרה והשלישית היא זוג ולא נקח דבר בעבורה הרי שאין בידינו כי אם עשר נסיר ח' ישארו ב' הרי שאין לו כי אם חצי | ||||||||||||||||||||||||
|
ואם לא תרצה לקחתו שנית למורה [עיין] אם יש לו עשר או ה' | ||||||||||||||||||||||||
|
ואחר שהראשונה 0 בידוע שיש לו [עשר וגם][84] ה' | ||||||||||||||||||||||||
|
ונקח אחד מהם | ||||||||||||||||||||||||
|
המשל ה' ונחלקנו עליו ויצא בחילוק 970970 | ||||||||||||||||||||||||
|
וגם זה אחר שהראשונה 0 יש לה י' גם ה' | ||||||||||||||||||||||||
|
ונקח עד"מ העשרה ונחלקנו לי' ויצא בחילוק 97097 | ||||||||||||||||||||||||
|
ואחר שהראשונה אינה לא ה' ולא 0 אין לו לא ה' ולא עשרה | ||||||||||||||||||||||||
|
ונראה אם יש לו שביעית | ||||||||||||||||||||||||
|
ומהט' האחרון נסיר שבעה ישארו שנים | ||||||||||||||||||||||||
|
ונכפלהו בג' יהיו ו' ונחברם לז' אשר לפניו יהיו י"ג נסיר הז' ישארו ו' | ||||||||||||||||||||||||
|
נכפלהו בג' יהיו י"ח נסיר מהם י"ד שני שביעיות ישארו ד' | ||||||||||||||||||||||||
|
ואחר שבמעלה שלפני זאת אין שם חשבון כי אם 0 נכפול אלו הד' בג' יהיו י"ב נסיר ז' ישארו חמשה | ||||||||||||||||||||||||
|
נחברם לט' שלפני זאת יהיו י"ד והם שביעיות גם הראשונה ז' הנה יצא הכל ז' ז' | ||||||||||||||||||||||||
|
הנה יש לו שביעית | ||||||||||||||||||||||||
|
ונקחנו למורה ונחלקנו עליו ויצא בחילוק | ||||||||||||||||||||||||
|
|||||||||||||||||||||||||
|
ונמצא שישארו ד' הנה אין לו ז' | ||||||||||||||||||||||||
|
ונראה אם יש לו י"א | ||||||||||||||||||||||||
|
ונחסר הא' האחרון מהג' שלפניו וישארו ב' | ||||||||||||||||||||||||
|
נסירם מהח' שלפניהם ישארו ו' | ||||||||||||||||||||||||
|
נסירם מהז' שלפניהם ישאר א' | ||||||||||||||||||||||||
|
נסירם מהא' שלפניו ולא ישאר דבר | ||||||||||||||||||||||||
|
הרי יש לו י"א | ||||||||||||||||||||||||
|
ונקחנו למורה ונחלקנו עליו ויצא בחילוק 1261 | ||||||||||||||||||||||||
|
ונשוב לראות אם יש לו הי"א | ||||||||||||||||||||||||
|
ונמצא שישארו ז' הנה אין לו י"א | ||||||||||||||||||||||||
|
ונעיין אם יש לו י"ג | ||||||||||||||||||||||||
|
ונכפול הא' אחרון בג' ולא נוכל להסירם מהב' שלפניהם לכן נוסיף עליהם י"ג ויהיו ט"ו נסיר הג' ישארו י"ב | ||||||||||||||||||||||||
|
ונכפלם בג' ויהיו ל"ו נסיר כ"ו שהם י"ג י"ג ישארו י' | ||||||||||||||||||||||||
|
[ולא נוכל להסירם מהו' שלפניהם ונוסיף עליהם י"ג ויהיה י"ט נסיר מהם י' ישארו][86] ישארו ט' | ||||||||||||||||||||||||
|
נכפלם בג' יהיו כ"ז נסיר כ"ו ישאר א' | ||||||||||||||||||||||||
|
נסירנו מהא' הראשון ולא ישאר דבר | ||||||||||||||||||||||||
|
הרי יש לו י"ג | ||||||||||||||||||||||||
|
נקחנו למורה ונחלקנו עליו ויצא בחילוק 97 | ||||||||||||||||||||||||
|
ואחר שזה החשבון הוא פחות ממרובע י"ג אינך צריך לעיין עוד אם יש לו [הי"ג] י"ג וכ"ש מורה גדול ממנו ולא אחד מהקודמים א | ||||||||||||||||||||||||
|
הנה יצאו לנו מורים לזה החשבון | ||||||||||||||||||||||||
|
והחשבון הקטן אשר רצינו לחלק לזה החשבון הגדול היה 2447235 | ||||||||||||||||||||||||
| We divide it by these divisors and write them one after another as we wish, for it does not matter, because there is no former and last in the divisors. | ונחלקנו לאלו המורים ונשימם זה אחר זה כרצוננו כי זה לא יזיק כי אין מוקדם ומאוחר במורים | ||||||||||||||||||||||||
| Divide the whole number by the last divisor to the left and write the remainder beneath it, then divide the result of divison by the one that precedes it; and so on, until the number is gone, [or] it reaches a phase in which the result of division is smaller than the divisor that precedes it. Then, you write the result beneath this preceding divisor and your procedure is complete. | ותחלק כל החשבון למורה האחרון אשר לצד שמאל והנשאר שים תחתיו והיוצא בחילוק חלק לאשר לפניו וכן לעולם עד אשר יכלה המספר ויגיע למקום שהיוצא בחילוק יהיה פחות מהמורה אשר לפניו כי אז תשים זה היוצא תחת המורה הזה אשר לפניו וכבר כלית כל מלאכתך | ||||||||||||||||||||||||
| If the divisors are all gone, but the number is not gone, and this happens when the dividend is greater than the number by which we wnat to divide that consists of these divisors, meaning that the divisors are derived from it, then write aside the result of division by the first divisor; these are integers; and as we did in this procedure, i.e. when we divided our number by these divisors, since the dividend is smaller than the number by which we want to divide that is above the divisors, the number is gone, but they are not gone. | ואם יכלו המורים והמספר לא יכלה וזה יקרה כאשר היה המספר המתחלק גדול [87]מהמספר אשר רצינו לחלק עליו אשר הורכב מהמורים ההם פי' שיצאו ממנו המורים ההם אז היוצא בחילוק בחלקך למורה הראשון תשימנו מבחוץ והם שלמים וכאשר עשינו זה המעש' ר"ל כשחלקנו מספרינו למורים אלו להיות המתחלק קטן מהמספר אשר רצינו לחלק עליו אשר הוא [מע]ל המורים יכלה המספר והמה לא יכלו | ||||||||||||||||||||||||
|
ויצא לנו כי כאשר נחלק 2447235 על 50350800 שהיוצא בחילוק הוא שני חמישיות חצי רביעית שמינית שלישית תשיעית שישית פי' כי כאשר עשינו האח' השלם ו' חלקים וא' מאלו הו' ט' וא' מאלו ה[.] הט' ג' וא' מאלו הג' ח' וא' מהח' א' ד' וא מהד' ב' וא' מב' אלו חמשה שיוצא לכל אחד מאחדי המספר הגדול אשר חלקנו עליו שתי חלקים חלק זה מהחלקים האחרונים האלו ה[.....] | ||||||||||||||||||||||||
|
ועוד יצא בחלוקנו זה ה' עשיריות חלק זה פי' [ה'][עשיריות][88] ה[חמיש]ית חצי רביעית שמינית שלישית תשיעית שישית | ||||||||||||||||||||||||
|
ועוד שביעית עשירית חמישית חצי רביעית וכו' | ||||||||||||||||||||||||
|
ועוד ד' חלקים מי"א מז' מי' מה' וכו' | ||||||||||||||||||||||||
|
ועוד [ט' חלקים מי"ג מי"א מז' מי' מה' וכו' | ||||||||||||||||||||||||
|
ועוד כ"ב][89] כ"ב חלקים מצ"ז מי"ג מי"א מז' מי' מה' וכו' | ||||||||||||||||||||||||
| |||||||||||||||||||||||||
| If you wish to receive more proper fractions, always see, when you divide the [divisor] into divisors, if the [dividend] has any of these divisors. | ואם תרצה שיצאו לך חלקים נאותים יותר תעיין לעולם כאשר תחלק המספר למורים אם למספר הזה יש שום אחד מהמורים ההם | ||||||||||||||||||||||||
|
המשל | ||||||||||||||||||||||||
|
וגם ליוצא בחילוק נעיין אם יש לו א' מהמורים הנותרים ונשימנו לפני זה אשר חלקנו עליו ונחלק [עליו ולא ישאר [.] דבר | ||||||||||||||||||||||||
|
וכן נעשה לעולם בענין שיצא לנו בחלקינו זה][90] זה המספר הקטן הנזכר לגדול שהיוצא לכל א' הוא חצי חלק מי"ג מי"א מד' מג' מו' ועוד ט' חלקים [91]מצ"ז מי' מח' מחצי וכו' הכל כמו שהוא בצורה הזאת | ||||||||||||||||||||||||
| |||||||||||||||||||||||||
| These two [division] procedures are the same, yet the second procedure that is based on division by the divisors [of the given number] involves more proper fractions and this reduction is called perfect beauty, as it means making the units nice and proper general amounts. | ואלו שני המעשים הכל אחד אלא שבמעשה השני חלקים יותר נאותים ולחלוקה על המורים עליו השגחה זו נקרא לו כלילת יופי לפי שהוא לעשות מהפרטים כללים יפים ונאותים |
| In order to elaborate the matter and explain it in detail, I give an example for our division: | וכ[די להרחיב הענין ו]לבארו בפי' אמשול משל לחלוקנו זה |
|
והוא כי המספר המתחלק יש לו תשיעית לכן שמנו הט' האחרון וחלקנוהו עליו ולא נשאר דבר על כן לא שמנו תחתיו דבר ויצא לנו בחלוק 271915 |
|
ויש לזה היוצא בחילוק חמישית לכן שמנו הה' לפני הט' וחלקנו עליו ולא נשאר דבר ויצא [לזה] היוצא בחלוק 54383 |
|
ויש לו שביעית לכן שמנו מיד ל[פני] המורים הנזכרים הז' וחלקנוהו עליו ולא נשאר דבר ויצא בחילוק [7769][92] |
|
ואין לו שום אחד מהמורים הנותרים לכן נשים אשר נרצה ושמנו היותר גדול והוא הצ"ז וחלקנו עליו ונשאר ט' ושמנוהו תחתיו ויצא בחילוק 80 |
|
ויש לו עשירית לכן שמנו מיד ה10 וחלקנום עליו ולא נשאר דבר ויצא בחלוק ח' |
|
ויש לו שמינית לכן שמנו מיד הח' וחלקנום עליו ולא נשאר דבר ויצא בחלוק |
|
ואחר שהוא פחות משום אחד מהמורים הנותרים אין לנו עוד לחלק אבל נשימהו תחת המורה אשר נשים מיד לפני המושמים הנזכרים ושמנו הב' ושמנו תחתיו זה הא' אשר יצא באחרונה בחלוק ואחר שמנו המורים הנותרים כאשר הזדמן |
| The rule that follows from this discussion: when we wish to divide any great or small number by another number, greater or smaller than it, we extract the divisors of the number by which we want to divide and write them randomly one after the other, or if one wishes, he can write them intentionally, in order to get more proper fractions, as we explained. Then, we divide the dividend by the last divisor to the left and write the remainder beneath it and we divide the result by the one that precedes it and so on. | הכלל העולה מהדברים הוא שכאשר נרצה לחלק [93]שום מספר גדול או קטן על מספר אחר גדול ממנו או קטן ממנו שנוציא מורה המספר אשר רצינו לחלק עליו ונשים אותם כפי המזדמן זה אחר זה או אם ירצה ישגיח בהנחתם יען יצאו החלקים יותר נאותים כאשר ביארנו ונחלק המספר המתחלק על המורה האחרון אשר לצד שמאל והנשאר נשים תחתיו והיוצא נחלק לאשר לפניו וכן לעולם | ||||||||||||||||||||||||
| If the dividend is smaller than the one by which we divide it, the number is completely consumed, but [the divisors] are not gone. | ואם היה המתחלק קטן מאשר חלקנו עליו יכלה המספר והמה לא יכלו | ||||||||||||||||||||||||
| When it is consumed, the result of division is smaller than the divisor that precedes the divisors by which we have already divided, therefore we should not divide this smaller result by the divisor that is greater than it, but write it beneath it. | וכאשר יכלה יהיה היוצא בחילוק פחות מהמורה אשר לפני המורים אשר חלקנו כבר עליהם לכן אין לנו לחלק זה היוצא המעט על זה המורה הרב ממנו אבל שימהו תחתיו | ||||||||||||||||||||||||
| If the dividend is greater than the one by which we want to divide it, the divisors are all gone, but the number is not completely consumed. We write the result of the last divison aside the diagram and they are the integers resulting from the division. What is in the diagram beneath the divisors are the fractions and the fractions of fractions resulting from the division that are added to the mentioned integers. | ואם היה המספר המתחלק גדול מאשר רצינו לחלק עליו יכלו המורים והמספר לא יכלה והיוצא מן החלוק האחרון [....] נשימהו חוץ לצורה והם השלמים אשר יצאו בחלוק ואשר בתוך הצורה תחת המורים הם השברים ושברי שברים אשר יצאו בחלוק מוסף על השלימים הנזכרים | ||||||||||||||||||||||||
| In order to elaborate the matter, I present another example: | וכדי להרחיב הענין אעשה משל אחר | ||||||||||||||||||||||||
|
המשל רצינו לחלק 3123740520 על 216 | ||||||||||||||||||||||||
|
והנה מורה זה המספר הקטן אשר רצינו לחלק עליו הם אלו | 3 | 8 | 9 | ||||||||||||||||||||||||
|
וחלקנו מספרינו זה הגדול על הט' ולא נשאר דבר ויצא בחילוק 347082280 | ||||||||||||||||||||||||
|
וחלקנום [על הח'][94] ולא נשאר דבר ויצא בחילוק 43385285 | ||||||||||||||||||||||||
|
[וחלקנום על הג' ויצא לנו בחלוק הזה האחרון 14461761 והם][95] והם השלמים ונשארו ב' | ||||||||||||||||||||||||
|
ושמנום תחת הג' והם השברים היוצאים בחילוק הנוספים על השלמים | ||||||||||||||||||||||||
|
ואם גם עתה בזה החילוק האחרון [96]לא היה נשאר דבר לא היינו שמים תחתיו דבר וכיון שלא נמצא דבר תחת המורים לא היו יוצאים בחילוק שברים כלל כי אם השלמים לבד | ||||||||||||||||||||||||
|
וזה יקרה כאשר החשבון אשר חלקנו עליו יהיה ראוי להיות מורה לחשבון המתחלה | ||||||||||||||||||||||||
|
ואולם במשלנו זה אח' אשר נשארו בחלוק האחרון ב' יש לו ג"כ שברים | ||||||||||||||||||||||||
|
ושמנום תחת הג' ויצא לנו כי כאשר חלקנו 3123740520 על 216 | ||||||||||||||||||||||||
| |||||||||||||||||||||||||
| Check | |||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| When you want to check if the extraction of the divisors was correct: multiply the first by the second, then the product by the third, then by the fourth and so on until they are gone. If you receive the original number no more and no less from this calculation, know that the [divisors] are correct, and if not, they are not. | ואם רצית לבחון הוצאת המורים ההיתה כתקנה כפול הראשון בשני והעולה בשלישי והעולה ברביעי וכן לכלם עד כלותם ואם יצא לך מזה החשבון המספר הראשון בלי תוספת ומגרעת תדע שיצאו כתקנם ואם לאו לאו | ||||||||||||||||||||||||
|
המשל בצורה הנזכרת אם רצינו לידע אם המורי' יצאו על היושר נכפול ט' בח' יהיו ע"ב נכפלים בג' יעלו 216 והנה כלו המורים ויצא החשבון בעל המורים בעינו | ||||||||||||||||||||||||
| When you want to know if you divide the number by the divisors correctly: | ואם תרצה לידע אם חלקת המספר על המורים על היוש' | ||||||||||||||||||||||||
| If there are integers [in the final result], multiply the integers by the first divisor, then multiply the whole product by the second divisor and add what you find beneath it to the product, then multiply the sum by the third divisor and add to it what you find beneath it. Continue like this until they are gone. If, when the divisors are gone, we get the dividend, your calculation is correct, and if not, it is not. | אם יש שם שלמים כפול השלמים במורה הא' ההוא וכל המקובץ כפלהו במורה השני והוסף על העולה אשר תמצא תחתיו וכפול הכל על המורה [..] השלישי והוסף עליו אשר תמצא תחתיו וכן תעשה לעולם עד כלותם ואם ככלות המורים יצא לנו המספר המתחלק הלא מעשיך אמת ויציב ואם לאו לאו | ||||||||||||||||||||||||
| If there are no integers [in the final result], take what you find first beneath the first divisor that you find something beneath it and multiply it by the divisor that is next to it to the left. Add what is beneath it to the product and multiply the sum by the divisor that follows. Add what is beneath it and so on until they are gone. If the result is the dividend itself [the calculation] is correct, if not, it is not. | ואם אין שם שלמים קח אשר תמצא ראשונה תחת המורה הקודם אשר תמצא תחתיו דבר וכפלהו במורה הסמוך לו לצד שמאל [97]וחבר הנמצא תחתיו עם העולה וכפול הכל על המורה הנמשך | ||||||||||||||||||||||||
|
המשל בתמונה הזו | ||||||||||||||||||||||||
| |||||||||||||||||||||||||
|
ונכפול השלמים בג' שהוא המורה הראשון ויעלה 43385283 | ||||||||||||||||||||||||
|
נחבר לזה הב' אשר תחת המורה הזה ויעלה הכל 43385285 | ||||||||||||||||||||||||
|
נכפלם על הח' שהוא המורה השני ויעלה 347082280 | ||||||||||||||||||||||||
|
ואחר שלא נמצא תחת זה המורה דבר לא נוסיף עליהם דבר | ||||||||||||||||||||||||
|
ונשוב ונכפלם בט' שהוא המורה השלישי ויצא לנו החשבון המתחלק בעינו שהוא 3123740520 | ||||||||||||||||||||||||
|
עוד אמשול זה בדרך קצרה בצורה הקודמת לזאת אשר אין שם שלמים ביוצא בחילוק כי אם שברים לבד |
|
ונקח הראשון אשר תחת הב' שהוא המורה הראשון אשר נמצא תחתיו דבר ונכפלהו בח' שהוא המורה הסמוך ויעלה ח' |
|
ואחר שאין תחתיו דבר לא נוסיף עליהם דבר ונכפלם בי' שהוא המורה הסמוך ויעלה פ' |
|
ואחר שלא נמצא תחתיו דבר נשוב ונכפלם בלי תוספת על הצ"ז שהוא המורה הסמוך ויעלה 7760 |
|
נחבר אליהם הט' אשר תמצא תחתיו ויעלה 77669 |
|
ונכפלם בז' ויעלה 54383 |
|
ונשוב ונכפלם בה' ויעלה 271915 |
|
ונכפלם בט' שהוא המורה האחרון ויצא לנו החשבון המתחלק בעינו והוא 2447235 והנה [נכון הנה אמת |
reason |
|||||||
|---|---|---|---|---|---|---|---|
| The reason for extracting the divisors and dividing the [dividend] by them: because the number by which we want to divide consists of these divisors. | וטעם הוצאת המורים וחלקנו עליהם המספר כנזכר הוא כי אחר שהמספר][98] | ||||||
|
ר"ל כי מי שיחלק א' על מאה עד"מ הנה יגיע ממנו לכל א' מהמאה חלק עליו ממאה שבו | ||||||
|
פי' שנעשה הא' השלם אשר רצינו לחלק [מאה חלקים שוים ויגיע לכל א' מהמאה אשר רצינו לחלק עליהם][100] עליהם הא' הראשון חלק א' מהם | ||||||
|
ואחר שחשבון הק' אשר רצינו לחלק | ||||||
|
וכאשר נעשה א' שלם ק' | ||||||
|
ולסבה זו בעינה יהיה אומרנו רביעית חמישית כאומרנו חלק א' מכ' לפי שעשרים יש לו חמישית וחמישיתו ד' נמצא שעשרים מורכב מה' וד' ושהאחד הוא חמישית רביעית או רביעית חמישית כי הכל א' הרי לנו ש שאמרנו רביעית חמישית כאומרנו חלק א' מכ' שבשלם | ||||||
|
[ואולם אמרנו חלק אחד מכ' מה' שבשלם הוא כאמרנו חלק א' מק' שבשלם כאשר][101] כאשר ביארנו הנה יצא לנו שאומרנו רביעית חמישית חמישית הוא כאומרנו חלק א' מק' שבשלם | ||||||
| Likewise for any number consisting of divisors, as many as they may be. | ובדמיון זה בכל חשבון מורכב ממורים כמה שיהיו | ||||||
|
ואם כאשר חלקנו א' שלם לק' הגיע לכל א' מהם חלק אחד מק' שבשלם שהוא רביעית חמישית החמישית | ||||||
|
ואם חלקנו על ק' ב' שלמים | ||||||
|
יגיע לכל א' ב' רביעיות חמישיות חמישית | ||||||
|
ואם ג' ג' | ||||||
|
הרי [הרי][102] כי מספר השלמים אשר נחלק אל ק' מספר הרביעיות חמישיות חמישית שיגיעו לכל אחד מהם וזה ברור | ||||||
|
ולזה אם רצינו לחלק ע' על ק' | ||||||
|
ידענו שיגיע לכל אחד מהם ע' רביעיות חמישיות חמישית והרי הוא כאלו שמנו המורים כזה הסדר ושמנו הע' | ||||||
| |||||||
|
ואחר שיש בידיך 70 [103]רביעיות חמשיות חמשית אם בקשנו לדעת כמה חמשיות חמישית הם הרי הוא כאלו היו בידינו ע' רביעיות ורצינו לדעת כמה שלמים הם | ||||||
|
וזה יודע בחלקנו אותם לד' לפי שכל ד' רביעיות הם א' שלם וכן כל ד' רביעיות חמישית [חמישית חמישית הם חמישית חמישית שלם לכן נחלק אלו הע' רביעיות חמישית][104] חמישית והנשאר יהיה מהמין הראשון ר"ל רביעיות חמישיות חמישית | ||||||
|
ולזה ראוי לנו לשים היוצא בחלוק שהוא י"ז תחת הה' אשר הוא המורה אשר לפניו | ||||||
| |||||||
|
הנה ידענו כי כאשר חלקנו ע' על ק' שהגיע לכל אחד מהם י"ז חמישיות חמישית וב' רביעיות חמשית חמישית | ||||||
|
ואחר שיש בידינו י"ז חמישיות חמישית ידענו שהם שלשה חמישיות שלמות ויותר לפי שכל חמש חמשיות חמישית הם חמישית אחד כמו שחמש חמשיות שלם הן שלם | ||||||
|
וראוי לנו לידע כמה חמישיות שלמות הן לכן נחלקם על הה' ויצא לנו בחלוק ג' שהוא ג' חמישיות שלימות לכן נשימם תחת הה' הראשון והב' השני והנשארים הם ממין במינם כבתחלה ר"ל חמישיות חמישית לכן שמנום תחת הה' השני כזה | ||||||
| |||||||
|
ואם אלו הג' אשר תחת המורה הראשון היה כמותו או גדול ממנו ר"ל שהיה ה' או יותר היה העולה לשלם או לשלימים כי כל חמש חמשיות הן שלם אחד והיה ראוי לנו לחלק אותן על ה' והיוצא בחילוק היו שלימים והנשאר היה חמישיות כאשר בתחלה | ||||||
|
אכן אחר שהוא קטן מהמורה ר"ל שהוא פחות מה' שהוא המורה שהוא המורה הראשון אין כאן שלם כלל ואין לנו לעשות שום חלוק אבל כבר השגנו מבוקשנו והוא כי כאשר [105]חלקנו ע' על ק' שהגיע לכל אחד מהם ג' חמישיות וב' חמישיות חמישית וב' רביעיות חמישית חמישית | ||||||
|
הכלל העולה | ||||||
|
ואחר שמאה הוא מורכב מאלו השלשה מספרים ר"ל מה' וה' וד' וזה כי כאשר כפלנו הא' בחבירו והעולה בנשאר עולה ק' פי' כי כפל ה' בה' הוא כ"ה וכאשר כפלנום בד' יעלו ק' | ||||||
| This is the check of the extraction of the divisors that we mentioned above, for when multiplying one by the other, then the product by another and so on until they end and we get the original number, we know that this number consists of these numbers. | וזאת היא בחינת הוצאת המורים אשר הזכרנו למעלה כי בכפול זה בזה והעולה באחר וכן לעולם עד כלותם ויצא לנו החשבון הראשון ידענו שהחשבון ההוא מורכב מאלו ה[ב'][106] מספרים | ||||||
|
ואחר היות הק' מורכב מה' הד' כך הוא אומרנו חלק מק' שבשלם כאומרנו רביעית חמישית חמישית רביעית חמישית או כאומרנו חמישית חמישית רביעית כי הכל אחד | ||||||
| For another reason we say that we can arrange the divisors randomly one after the other, or intentionally, in order to receive reduced fractions that are as proper as possible. | ומטעם אחר על זה אמרנו שבידינו לסדר המורים זה אחר זה כפי המזדמן אם בהשגחה כדי שיצאו השברים היותר שלמים שיוכל והיותר נאותים | ||||||
|
וזה כי כאשר אמרנו שהמחלק ע' על המאה יגיע לכל אחד ע' רביעיות חמישית חמישית היינו יכולים לומר ע' חמשיות רביעית חמישית או ע' חמישיות חמישית רביעית | ||||||
| Since we can arrange them as we wish, it is appropriate to arrange them and keep them in order. | ואחר שבידינו לסדרם כחפצנו ראוי לסדרם ולהשגיח בסדורו | ||||||
|
וזה כי כאשר רצינו לחלק אלו הע' לאלו המורים אחר שהעין יש לו חמישית שהוא א' מאלו המורים ראוי לנו לחלקם ראשונה על הה' ונשימנו אחרון כדי שלא ישאר דבר לשים תחתנו ויצא בחילוק י"ד | ||||||
|
ואם היה להם רביעית היה ראוי לחלקם על ד' ולשומו לפני האחרון | ||||||
|
ואם היה לו ה' לה' | ||||||
|
אכן [107]שאין לו אחד מהם נסדר אלו השני המורים הנשארים כפי המזדמן | ||||||
|
וע'ד'מ' נחלק אלו הי"ד לד' ונשימהו לפני האחרון ויצא בחילוק ג' ואחר שהוא פחות מהה' שהוא המורה האח' נשימהו תחתיו והב' הנשארים נשימם תחת הד' שהוא המורה השני [אשר][108] נחלקנו עליו כזה | ||||||
| |||||||
|
והנה יצאו לנו חלקים יותר נאותים כי יותר נאות הוא לומר ג' חמישיות [ושני רביעיות חמישית][109] שהן חצי חמישית כאשר בא בצורה הזאת מאומרנו ג' חמישיות ושני חמישיות חמישית וב' רביעיות חמישית חמישית | ||||||
|
וידוע הוא כי בחינת זה הוא להשיבם כלם מהמין הראשון ר"ל חמישיות רביעית חמישית כפי צורה זו האחרונה או רביעית חמישית חמישית כפי הצורה הקודמת וזה יקרא פריטה כאשר יתבאר בחלק השני | ||||||
|
ואחר שיש לו חמישיות שלמות גם רביעיות חמישית נשיבם כלם ראשונה רביעיות חמישית | ||||||
|
וידוע כי כל חמישית שלמה היא ד' רביעיותיה פי' ד' רביעית חמישית כמו שכל שלם ד' רביעיות שלם | ||||||
|
הרי לנו כי כל אחד מאלו הג' חמישיות שלימות היא ד' רביעיות חמישית | ||||||
|
ולדעת כמה הם נכפול ג' שהוא מספר החמישיות בד' שהוא המורה הבא אחריו ויעלה י"ב הרי לנו שהג' חמישיות הם י"ב רביעיות חמישית | ||||||
|
ומצאנו תחתיו שנים שהם מזה המין פי' שהם רביעיות חמישיות נחברם אליהם ויהיו י"ד רביעיות חמישית | ||||||
|
וכאש' נרצה לדעת כמה חמישיות רביעית חמישית הם נכפלם בה' שהוא המורה הבא אחריהם ויעלו כלם ע' | ||||||
|
ואם תחת זה המורה היה נמצא דבר זה היה ג"כ חמישיות רביעית חמישית [110]והיינו מחברים אותם אליהם | ||||||
|
אכן אחר שלא נמצא תחתיו דבר וכבר כלו המורים כבר כלינו מעשינו | ||||||
|
ואחר שעלה לחשבוננו הראשון פי' לעין [ע'][111] שהוא החשבון הקטן אשר רצינו לחלק על הק' שהוא בעל אלו המורים מבלי תוספת ומגרעת ידענו כי כל מעשינו בצדק ובמשפט |
| Thus, the reasons of the all that was mentioned - the procedure as well as the examinations - are clear, and all is explained generally and particularly. | הרי לנו מבוארים טעמי' כל הנזכר גם המעשה גם הבחינות וביאור הכל בכלל ובפרט | ||||||
| Division of a large number by a smaller number, with a result of integers and fractions | |||||||
|---|---|---|---|---|---|---|---|
| In order to train I bring another example, in which the divided by the 100 is greater than it, so the result are fractions as well as integers. | וכדי להתלמד אביא משל אחר שיהיה המתחלק על אלו הק' גדול מהם כדי שיצאו שם שברים גם שלמים | ||||||
|
המשל רצינו לחלק ק"מ על ק' | ||||||
|
ואחר שאלו הק"מ שהם החשבון אש' רצינו לחלק יש לה כל אלו המורים נשים אשר נחפוץ אחרון ונחלקנו עליו המשל על הה' ויצא | ||||||
|
ואחר שיש להם רביעית נחלקם על הד' ונשימנו לפני האחרון ויצא בחילוק ז' | ||||||
|
ואחר שהם כמורה הנשאר וגדול מ | ||||||
| |||||||
|
הרי לנו כי כאשר חלקנו ק"מ על ק' שעולה לכל 1 ואחד מהמאה ק"מ חלקים ממאה שבשלם שהם ק"מ חמישיות רביעית חמישית שהם כ"ח רביעיות חמישיות שהם ז' חמישיות שלמות שהם א' שלם וב' חמישיות | ||||||
|
וכמו שזה החשבון המתחלק הזה כפל החשבון המתחלק ראשונה כן היוצא בחילוק שהוא א' שלם וב' חמישיות שהם ז' חמישיות הוא כפל היוצא בחילוק ראשונה שהיה ג' חמישיות וב' רביעיות חמישית פי' ג' [112]חמישיות | ||||||
| The whole procedure is clear and explained also by checking, which is to restore everything to its former state. | הנה כל המעשה ברור ומבורר גם הבחינה והיא להשיב הכל לקדמותו | ||||||
|
וזה כי האחד נשיבהו חמשיות שלמות וזה בכופלנו אותו בה' שהוא המורה על החמישיות ויהיו ה' | ||||||
|
|||||||
|
וכאשר נרצה להשיבם רביעיות חמישית נכפלם בד' ויהיו כ"ח רביעיות חמישית ואחר שלא נמצא תחתיו דבר לא נחבר אליהם דבר | ||||||
|
עוד נשיבם חמישיות רביעית חמישית וזה בכפלנו אותו בה' ויעלה ק"מ חמישיות רביעית חמישית והנה כלו המורים ואין תחתיו דבר לחבר על העולה |
|
ויצא לנו כחשבוננו הראשון שוה בשוה |
| Multiply the rest of the numbers by this method in the procedure or in the check. | וב[דרך זה] תעשה כפל המספרי' הנשארים הן למעשה הן לבחינה |
Chapter Five: Proportions |
הפרק הה' | ||||||||||||
Rule of Three |
|||||||||||||
| For the ratio that a known number is to [a known number], if you want to know for another known number, to which number it has this same ratio:
|
אם תרצה לדעת הערך שיש למספר ידוע למספר ידוע אחר אצל איזה מספר יש לו אותו הערך בעצמו | ||||||||||||
|
המשל הערך שיש לה' אצל ז' אצל מי יש לי' זה הערך | ||||||||||||
|
או אצל י"ד למי שיש לו זה הערך | ||||||||||||
| To understand it briefly I will give them an order: | וכדי להבינו בקוצר אשים להם סדר | ||||||||||||
When we say: the ratio that 5 is to 7 - the 5 is called "first" [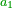 ] and the 7 [is called] "second" [ ] and the 7 [is called] "second" [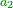 ], since the 5 is related to 7. ], since the 5 is related to 7.
|
והוא כי כאשר נאמ' הערך שיש לה' אצל ז' נקרא הה' ראשון והז' שני לפי שהה' הוא הנערך אצל ז' | ||||||||||||
|
והנה אם תאמר אצל מי יש ערך זה לי' יחסר אשר אליו אנו מעריכים שהוא השני מהאחרים | ||||||||||||
|
ואם נאמר למי יש זה הערך אצל י"ד יחסר הנערך שהוא הראשון מהאחרים | ||||||||||||
| This is the rule: the related of those that are first and of those that are last is called first, and that to which it is related, is called second. | זה הכלל כי לנערך [114]הן מן הראשונים הן מן האחרונים נקרא ראשון ולאשר מעריך אצלו נקרא שני | ||||||||||||
|
וכאשר תרצה לדעת הנעלם | ||||||||||||
|
אם יש בידך הראשון מן השנים האחרונים ונעלם השני שבאחרונים נכפול אותו הראשון [בשני][115] מן השנים הראשונים הידועים וחלקנו לנשאר מהג' הידועים ר"ל לראשון שבראשונים והיוצא בחילוק הוא הנעלם | ||||||||||||
|
ואם היה בידך השני שבאחרונים ונעלם הראשון כפול אותו השני הידוע בראשון שבראשונים והעולה חלקהו לשני שבראשונים והיוצא בחילוק הוא הנעלם המבוקש | ||||||||||||
|
וכאשר תחלק העולה על השני שבראשונים או על הראשון אם תרצה תוציא המורים אם זה הראשון או השני אשר תחלק עליו הוא חשבון גדול והיוצא בין שלמים ושברים הוא המבוקש הנעלם | ||||||||||||
| This is the rule: always multiply the first of these by the second of the others and divide the product by what remains of those that are known; the result of division is the unknown. | זה הכלל לעולם תכפול הראשון מאלו בשני מאלו והעולה תחלק על [הנשאר] מהידועים והיוצא בחילוק הוא הנעלם | ||||||||||||
|
המשל אם אמרנו הערך שיש לג' אצל הז' לה' אצל מי יש לו זה הערך בעצמו | ||||||||||||
|
ונשימם לו על זה כזה | ||||||||||||
| |||||||||||||
|
הנה ידענו הנערך שבאחרונים והוא הנקרא ראשון והוא הה' ונכפלנו בשני שבראשונים והוא הז' ויעלו ל"ה ונחלקנו לנשאר מהידועים והוא הג' ויצא בחילוק י"א שלמים [וב' שלישיות וזהו הנעלם המבוקש | ||||||||||||
|
פי' כי הערך אשר לג' אצל הז' הוא הערך בעצמו אשר לה' אצל י"א וב' שלישיות][116] וב' שלישיות | ||||||||||||
|
וזה ברור שכמו שז' הוא כפל ג' ועוד שלישיתם שהוא א' כן י"א וב' שלישיות הוא כפל ה' ועוד שלישיתו שהוא א' וב' שלישיות | ||||||||||||
|
ואם אמרו הערך אשר לג' אצל ז' אצל י"א וב' שלישיות למי יש לו זה הערך | ||||||||||||
|
נשימם בצורה הזאת | ||||||||||||
| |||||||||||||
|
[117]הנה הנעלם הוא הנערך שהוא הראשון שבאחרונים והידוע שבהם הי"א וב' שלישיות והוא השני שבהם לכן נכפלנו בראשון שבראשונים שהוא הג' ויעלה ל"ה ונחלקם לנשאר מהידועי' והוא הז' ויצא בחלוקה | ||||||||||||
| Written calculation | |||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| This is the rule: we call them by their names and arrange each type beneath its own type, then we multiply each type by the one that is not of its type, which are the diagonals, and divide by its type. The result of division is the required unknown. | זה הכלל נקרא להם שם ונסדרם מין תחת מינו ונכפלם מין בשאינו מינו שהם האלכסונים ונחלקנו למינו היוצא בחילוק הוא הנעלם המבוקש | ||||||||||||
| ? | ואם הם בעצמם היה להם שינוי בשמות אשר בהם נודע איזה מינו או שאינו מינו לא נצטרך לקרוא להם שם חדש | ||||||||||||
| Exchange Problem - Currencies | |||||||||||||
|
המשל אם ג' דינרי זהב שוים נ' דינרי כסף י"א דינרי זהב כמה דינרי כסף שוים | ||||||||||||
|
נסדרם מין תחת מינו הזהב תחת הזהב כזה | ||||||||||||
| |||||||||||||
|
ונכפול למין בשאינו מינו שהם הזהב והכסף והם הי"א בנ' שהם האלכסונים ויעלה 550 ונחלקם על מינו פי' על ג' של זהב ויצא בחילוק 183 ושליש שהם הדינרי כסף הנעלמים | ||||||||||||
|
הרי לנו שאם שלשה דינרי זהב שוים נ' של כסף י"א דינרי זהב שוים 183 דינרי כסף ועוד שליש דינר כזה | ||||||||||||
| |||||||||||||
| Exchange Problem - Currencies: | |||||||||||||
| If the question is vice versa, that the gold is unknown to us, as our saying: | ואם השאלה היתה להפך שנעלם לנו הזהב כאומרנו | ||||||||||||
|
ואם ג' דינרי זהב שוים נ' של כסף 183 דינרי כסף ושליש דינר כמה שוים | ||||||||||||
|
נשימם מין על מינו כזה | ||||||||||||
| |||||||||||||
|
ונכפול מין בשאינו מינו פי' הכסף בזהב שהם האלכסונים ויעלו 550 ונחלקם על מינו שהוא הכסף הנשאר והוא הנ' ויצא בחילוק י"א והם דינרי זהב הנעלמים | ||||||||||||
| It all comes down to the same thing. | והכל עולה לענין אחד | ||||||||||||
Reasons |
|||||||||||||
| The reason for the solution of the first example: | |||||||||||||
| The reason is that when we say: the ratio that 3 has to 7 - to whom does 5 have this ratio?
|
[118]הטעם כי כאשר אמרנו הערך שיש לג' אצל ז' לה' אצל מי שיש לו זה הערך | ||||||||||||
| We know that as the ratio that 3 is to 7, one, which is a third of 3, is to a third of 7, meaning that if 3 is, for instance, a third of 7, then one is a third of a third of 7, and this is clear. | הנה ידענו שהערך שיש לג' אצל ז' יש לאחד שהוא שליש הג' אצל שליש [הז' פי' שאם הג' על דרך משל שליש הז' הנה האחד הוא שליש שלישית הז' וזה ברור | ||||||||||||
| Since we know that the ratio that 1 is to a third of 7 is as the ratio that 3 is to 7, which is the required ratio, and that a third of 7 is 7-thirds, then we know that 5 has that same ratio to 5 times 7-thirds.
|
ואחר שידענו שערך א' אצל שליש ז' הוא][119] ז' הוא כערך ג' אצל ז' שהוא הערך הנשאל ושליש ז' הוא ז' שלישיות הנה ידענו שזה הערך בעצמו יש לה' אצל ה' פעמים ז' שלישיות | ||||||||||||
| To know how many thirds they are, we have to multiply 5 by 7 and the result are thirds | ולדעת כמה שלישיות הם יש לנו לכפול ה' בז' והעולה הם שלישיות | ||||||||||||
| To know how many integers they are, we divide them by 3. | ולדעת כמה שלמים הם חלקנום על הג' | ||||||||||||
| The reason for the solution of the second example: | |||||||||||||
| Likewise in the second example: we know the ratio that 3 is to 7 and we wish to know who has this same ratio to 11 and 2-thirds.
|
וכן בדמיון השני כי אחר שידענו ערך ג' אצל ז' ורצינו לידע למי יש לו זה הערך בעצמו אצל י"א וב' שלישיות | ||||||||||||
| It is as if we know the ratio that 7 is to 3 and we wish to know to whom does 11 and 2-thirds has this ratio.
|
הרי הוא כאלו ידענו ערך ז' אצל ג' ונרצה לידע לי"א וב' שלישיות אצל מי יש לו זה הערך | ||||||||||||
| The reason is clear, because it becomes as the first example it self. | והנה הטעם ברור שהרי שב כדמיון הראשון בעינו | ||||||||||||
| In order to elaborate the explanation I will explain from the beginning: | אכן כדי להרחיב ביאור אבארנו בעודו בעינו | ||||||||||||
| I say that we know that the ratio that 3 is to 7 is the same ratio that one, which is a third of 3, is to 7-thirds, which are a third of 7, as we explained.
|
ואומר כי אחר שידענו שהערך שיש לג' אצל ז' הוא הערך בעצמו שיש לאחד שהוא שליש הג' אצל ז' שלישיות שהם שליש הז' כאשר ביארנו | ||||||||||||
| Hence, for every seven-thirds that are in 11 and 2-thirds, one is the related and as the number of 7-thirds that are in them, so is the number of units of the unknown that is related to them. | א"כ לכל שבעה שלישיות אשר בי"א וב' שלישיות הנערך אליהם הוא א' וכמספר כמה ז' שלישיות יש בהם כך הוא המספר אחדי הנערך אליהם הנעלם | ||||||||||||
| To know how many whole 7-thirds there are in 11 and 2-thirds, we should know first how many thirds are they and this is known by multiplying them by 3. We multiply them by 3; the result is 35, so we know that there are 35 thirds in them. | ולדעת כמה שלמים ז' שלישיות יש בי"א וב' שלישיות נדע תחלה כמה שלישיות הוא וזה יודע בכפלהו אותם בג' לכן כפלנום בג' ועלה ל"ה הנה ידענו שיש בהם [ל"ה][120] שלישיות | ||||||||||||
| To know how many times 7-thirds are in them, we divide them by 7; we get 5 and this is the number of times that 7-thirds are in 11 and 2-thirds.
|
ולדעת כמה פעמים יש בהם ז' שלישיות חלקנום על ז' ויצא לנו ה' והוא המספר הפעמים אשר יש ז' שלישיות בי"א וב' שלישיות | ||||||||||||
| We already know that 1 integer is the related to every 7-thirds, so the related to 5 times 7-thirds is 5 integers. | וכבר ידענו שהנערך אצל כל ז' שלישיות הוא א' שלם א"כ הנערך אצל ה' פעמים ז' שלישיות הוא ה' שלמים | ||||||||||||
| But, we know that 11 and 2-thirds is 5 times 7-thirds, so the related to them is 5 integers.
|
ואולם ידענו שהי"א וב' שלישיות הוא ה' [121]פעמים ז' שלישיות א"כ הנערך אליהם הוא ה' שלמים | ||||||||||||
| The reasons for the solutions of the exchange problems: | |||||||||||||
| In the examples of the dinar: | ועוד במשלי הדינרים | ||||||||||||
|
כי כאשר ידענו שג' דינרי זהב שוים נ' של כסף | ||||||||||||
| It is known that one gold dinar is worth one-third of 50 silver dinar, which is fifty-thirds of a dinar. | נודע שדינר זהב אחד שהוא שוה שליש נ' דינרים של כסף שהוא חמישים שלישי דינר | ||||||||||||
| It is known from this that 11 gold dinar are worth 11 times 50-thirds of a silver dinar. | ונודע מזה שהי"א דינרי זהב שוים י"א פעמים נ' שלישי דינר כסף | ||||||||||||
| To know how many thirds they are, we multiply 11 by 50; the result is 550, so we know that 11 gold dinar are worth 550-thirds of a silver dinar. | ולדעת כמה שלישים הם כפלנו הי"א בנ' ועלה 550 הנה ידענו שהי"א דינרי זהב שוים 550 שלישי דינר כסף | ||||||||||||
| To know how many silver dinar they are, we divide them by 3; the result is 183 and one-third and they are the silver dinar that are worth 11 gold dinar and this is clear. | ולדעת כמה דינרי כסף הם חלקנום על ג' ויצא 183 ושליש והם הדינרי כסף ששוים הי"א דינרי זהב וזה ברור | ||||||||||||
|
ועוד אבארנו במשל השני והוא כי ביודעינו שג' דינרי זהב שוים נ' דינרי כסף כל דינרי זהב שוה נ' שלישי דינר כסף כמו שביארנו |
| Therefore, every 50-thirds of a dinar that is in 183 and one-third is worth one gold dinar. | וא"כ כל נ' שלישי דינר אשר בק'פ'ג' ושליש שוה דינר זהב |
| To know how many 50-thirds of a dinar there are in them, we should know first how many thirds of a dinar they are and this is known by multiplying them by 3 as we did; the result is 550, which are thirds of a dinar.
|
ולדעת כמה פעמים יש בהם נ' שלישי דינר נדע תחלה כמה שלישי דינר הם וזה יודע בכפלנו אותם בג' כאש' עשינו ועלו 550 והם שלישי דינר |
| Every 50 of them are worth one gold dinar, so when we divide them by them, as we did, we know how many gold dinar they are worth and this is as the result of division, which is 11. All this is clear. | וכל נ' מהם שוים דינר זהב א"כ בחלקנום אותם על נ' כאשר עשינו נדע כמה דינרי זהב שוים שהוא כמספר היוצא בחלוקו הוא י"א וכל זה ברור |
| It becomes clear from all that is mentioned with a little investigation that for every four proportional numbers, the product of the first of the formers by the second of the latter is as the product of the second of the formers by the first of the latter.
|
והנה יתבאר מכל הנזכר במעט עיון כי כל ד' מספרים נערכים כפל הראשון מאלו בשני מן האחרים ככפל השני בראשון מן האחרים |
|
כי בדמיון הראשון כפל הה' בז' שהוא ל"ה ככפל הג' בי"א וב' שלישיות אשר היה הנעלם |
| Therefore, when one of them is unknown, whichever it may be, we multiply the knowns, first of these by the second of those, and we know that it is itself the product of the unknown by the one that remains of the knowns. Therefore, when we divide it by the known, the result is the unknown. | ולזה כאש' נעלם אחד מהם איזה מהם שיהיה כפלנו מהנודעים הראשון מאלו בשני מאלו וידענו שזה בעצמו הוא כפל הנעלם בנשאר מהנודעים ולזה בחלקנו אותו לנודע יצא הנעלם |
Proportional Triad |
|
| Sometimes the proportional numbers are only three, i.e. the mean is the first of those that are last and the second of those that are first.
|
[122]ולפעמים לא יהיו המספרים הנערכים כי אם ג' פי' שהאמצעי יהיה ראשון לאחרונים ושני לראשונים |
| We have already explained that for every four proportional numbers the product of the first of those by the second of those is as the product of the first of those by the second of thos, i.e. the product of the first by the last is as the product of the two means by each other.
|
ואולם כבר ביארנו שכל ד' מספרים נערכים כפל הראשון מאלו בשני מאלו |
| When they are only three, the mean stands instead of the two means, which are the second of those that are first and the first of those that are last, so the product of the first by the third, which is the second of those that are last, is as the product of the mean by itself, which is both first [of those that are last] and second [of those that are first], as we explained.
|
ואולם כשהם ג' לבד האמצעי עומד במקום השנים האמצעיי' שהוא שני לראשונים וראשון לאחרונים א"כ כפל הראשון בשלישי שהוא השני מהאחרונים ככפל האמצעי בעצמו שהוא ראשון ושני כאשר ביארנו |
|
ולזה בהודע האמצעי ואחד מן האחרים יודע הנעלם כי נכפול האמצעי בעצמו ונחלקנו לאחר הנודע ויצא הנעלם |
|
גם בהודע השנים יודע האמצעי וזה בהכפל השנים הנודעים והעולה הוא ככפל האמצעי בעצמו פי' שהוא כמרובע |
|
והאמצעי הוא השרש ונוציא שורש זה המספר שהוא לבקש מספר שכפלו על עצמו עולה כפי החשבון והשרש אשר יצא הוא האמצעי הנעלם |
| The method of extracting the roots is very difficult and there are numbers whose real root is never known only approximately, so I have assigned a special chapter to this and it is the next chapter. | ודרך הוצאת השרשים הוא קשה מאד ויש מספרים אשר לא יודע בהם שרש אמיתי לעולם כי בקירוב על זה הקצתי לו פרק לעצמו והוא הפרק הבא אחר זה |
| Example for the three proportional numbers: | דמיון זה ג' מספרים נערכים |
|
הוא כאומרנו הערך אשר לב' אצל הד' כערך ד' אצל ח' |
|
שהד' האמצעי הוא במקום שנים שהוא שני מן הראשונים וראשון מן האחרונים |
|
ואם הנעלם מהקצוות המשל הב' ונודע הד' והח' |
|
כלומר ששאל השואל למי יש ערך אצל ד' כערך אשר לד' אצל שמונה |
| Exchange Problem - Currencies: | |
|---|---|
|
או שאמ' כמה דינרי זהב שוים ד' דינרי כסף אם ד' דינרי זהב שוים אחד דינרי כסף |
|
הנה ידענו שכפל ד' בד' שהוא האמצעי |
|
לכן נחלקם על הח' והיוצא |
|
וכן אם נודעו השנים והד' ונעלם הח' |
|
ששאל השואל הערך אשר לב' אצל ד' אצל מי יש לד' זה הערך |
| Exchange Problem - Currencies: | |
|
או ששאל אם שני דינרי זהב שוים ד' דינרי כסף ד' דינרי זהב כמה דינרי כסף שוים |
|
נכפול הד' בעצמו ויעלה י"ו ונחלקם על הח' ויצא בחילוק ב' והוא הנעלם |
|
ואם היה הנעלם הד' שהוא האמצעי העומד במקום שנים והנודעים הב' והח' ראשון ואחרון |
|
נכפול הב' בח' ויעלה י"ו וזה כפל האמצעי הנעלם בעצמו כמו שביארנו ואלו הי"ו הם מרובע האמצעי |
|
והאמצעי הוא שרושם ושרש י"ו הוא ד' |
| Everything is explained in these examples. | והכל מבואר בדמיונות אלו |
| If 16 is a number, whose root extraction is difficult for us, or it is impossible for us to know its real root, only by approximation, we proceed in the extraction of the root as explained in chapter six that is assigned to it. | ואם זה הי"ו היה החשבון אשר יקשה עלינו בקשת שרשו או שהוא נמנע בחקנו לידע שרשו האמיתי כי אם בקרוב נדרוך בבקשת השרש ההוא כמו שיתבאר בפרק ו' זה אשר הקציתי לו |
Chapter Six: Roots |
הפרק השישי בהוצאת השרשים | ||||||||||||
written extraction of roots |
|||||||||||||
description of the procedure |
|||||||||||||
| When you wish to extract the root of a certain number, count the number of the ranks, whether it is even or odd. | כאשר תרצה להוציא שורש שום מספר תמנה מספר מעלות ההוא אם זוג ואם נפרד | ||||||||||||
|
ואם הם נפרד עיין הרושם האחרון כאלו היא | ||||||||||||
|
ואם ישאר שום דבר מזה החשבון האחרון העליון אחר הוצאת כפל המספר אשר שמת תחתיו בעצמו תשים הנשאר ההוא על המספר האחרון | ||||||||||||
|
ואם מספר מעלות החשבון אשר רצית לדעת שרשו יהיה זוג תקח האות האחרון לעשרות ואשר תמצא במעלה אשר לפניה לאחדים | ||||||||||||
|
ותבקש מספר שיהיה מרובעו בכל אלו העשרות והאחדים אשר לקחת או היותר שתוכל להוציאו מהם וזה המספר אשר מצאת תשימהו [124]תחת המעלה אשר לפני המעלה האחרונה | ||||||||||||
|
ואשר ישאר אחר הוצאת מרובע המספר אשר מצאת מאלו האחדים והעשרות אשר מצאת בשתי המעלות האחרונו' אם ישאר ש | ||||||||||||
| After doing all that, both for a number whose number of ranks is even and for [a number] whose number of ranks is odd, double the number that you placed beneath the upper digit. | ואחר עשותך כל זה הן במספר אשר מעלותיו זוג הן באשר הן מעלותיו מספר נפרד תכפול זה המספר אשר שמת תחת המספר העליון | ||||||||||||
|
ואם לא יעלה מזה הכפל שום עשר תשים אחדי הכפל הזה תחת המעלה אשר לפני המעלה אשר שמת אותו בתחלה | ||||||||||||
|
ואם עלה לעשר או יותר תשים העשר תחת המעלה אשר היה שם המספר הזה בתחלה והאחדים במעלה אשר לפניו | ||||||||||||
|
ואם לא יהיו שם אחדים תשים במעלה אשר לפניו | ||||||||||||
| Cross the first number you doubled with a pen. | ותעבור הקולמוס על המספר הראשון אשר כפלת | ||||||||||||
| Thereafter, seek a number to place in the rank that precedes the mentioned one, such that when multiplying it by the number or numbers, which you have just placed that resulted from the first doubling, and also by itself, then subtracting each product from the corresponding rank, all is gone or as much as possible. | ואחר כך תבקש מספ' אשר תשים במעלה אשר לפני אלו הנזכרות אשר בכפול אותו במספר או מספרים אשר שמת עתה שנתחדשו מכפל הראשון וגם בעצמו והוציא כל כפל וכפל מהם מהמעלה אשר כנגדו ויצא הכל או היותר שתוכל | ||||||||||||
|
ותשימנו במעלה הנזכרת | ||||||||||||
|
ותכפלנו במספרים הראשונים מלבד אשר שמת ראשון שעבר עליו הקולמוס | ||||||||||||
|
ואשר יעלה תוציאנו מהרשמים אשר על ראשם | ||||||||||||
|
ותכפלנו | ||||||||||||
|
והנשאר בשום מקום תשימנו על הרושם אשר ממנו נותר | ||||||||||||
|
ואם כאשר כפלת המספר ושמת כפלו במעלה אשר לפניו אם אין ברשמים אשר עליהם כדי להוציאם אפי' פעם אחת ושישאר במעלה אשר לפניהם אחד להוציא ממנו כפל האחר בעצמו אז תשים 0 לפניהם ותורידם מעלה אחת גם ל0 גם לכל רושם מהם ותבקש מספר שתשים לפניהם ותכפלנו בכל אחד מהם ובעצמו ותוציא כל דבר מאשר על ראשו | ||||||||||||
|
והנותר תשים על הרושם אשר על ראשו ותורידם עוד מעלה אחרת ובלבד שתורידם לעולם בכל הורדה שתורידם שיורדו כמות שהם בלי כפל כלל זולתי המספר האחרון שנתחדש בפעם ההיא שתכפלנו | ||||||||||||
|
ואם לא נתחדש שם עשר תשימנו במעלה שלפני המעלות אשר תשים הרשמי' האחרים בהורדתם | ||||||||||||
|
ואם מהכפל ההוא יתחדש עשר תחברנו עם הרושם אשר שמת ראשון לצד ימין | ||||||||||||
|
ואם לא נתחדשו שם אחדים כגון שהרושם האחרון היה חמשה וכפלו יהיה עשרה שלם בלתי אחדים תשים הי' כאשר אמרתי במקום ה0 או תחברנו עם אשר תמצא במעלה לצד ימין ואחר שאין אחדים שם תשים 0 לפני המעלות ההם | ||||||||||||
| Repeating the process: seek for another number as stated and proceed like this until their end. | ותבקש עוד מספר כמו שנזכר וכן תעשה עד תומם | ||||||||||||
| The root consists of all the numbers you seek for in all the phases without their doubling. | [126]והשרש הוא כל המספרים אשר בקשת בכל עת בלי כפל | ||||||||||||
examples |
|||||||||||||
|
המשל רצינו לבקש שרש 344680129066 | ||||||||||||
|
- [Illustration of the procedure:]


19 982 344680129066 3446801290663446801290665510 108 116
|
והנה מעלות מספר זה הם י"ב והם זוג נקח השני רשמים האחרונים האחרון אחרון לעשרות ואשר לפניו לאחדים ויהיו 34 |
|
ונבקש מספר שנכפלנו על עצמו ויוציא כל ה34 או היותר שאפשר והוא ה' ונשימנו תחת הד' |
|
ונאמר ה' פעמים ה' הם כ"ה נוציאם מהל"ד ישארו ט' |
|
ונעביר קולמוס על הג' ונשים הט' על הד' |
|
ונכפול הה' |
|
ונבקש מספר נשימהו במעלה שלפני ה0' ונכפלנו |
|
ונכפול ח' בא' יהיו ח' נוציאם מהט' אשר עליו ישאר אחד נשימנו עליו |
|
עוד נכפול ח' על עצמו ויעלה [127]ס"ד |
|
ונוציא הד' האחדים מהו' אשר על ראשו ישארו ב' נשימם על הו' |
|
והס' שהם ו' עשרות לא נוכל להוציאם מהד' שאחר הו' שהוא עשרות נגדו ונקרא הא' אשר אחריהם ונעביר עליו הקולמוס ויהיה עשר במעלת הד' הנזכר ונחבר אליהם הד' עצמו יהיו כלם י"ד נוציא מהם הס' אשר הם ו' עשרות נשארו ח' ונשימנו על הד' |
|
אחר זה נורידם מעלה אחת ונכפול הח' אשר נתחדש בפעם הזאת ואחר אשר נתחדש מכפלו אחדים ועשר לא נשים ה0' אבל נשים א' בעד העשר במקומה ונשים הו' אחדים לפניו |
- [Illustration of the procedure:]

1 115198261 3446801290665108 1167 1174
|
ונבקש מספר שנשים לפניהם שנכפלנו בהם ובעצמו ונוציא היותר שאפש' מאשר עליהם ויהיה ז' ונשימנו לפניהם | ||||||||||||||
|
ונאמ' שבעה פעמים א' הם ז' נוציאם מהח' אשר על ראשו וישאר א' ונשימנו עליו | ||||||||||||||
|
עוד נאמר ז' פעמים א' על הא' אשר לפניו הם ז' | ||||||||||||||
|
ולא נוכל להוציאם מהב' אשר על ראשם נקח הא' אשר שמנו עתה על הח' ונעביר עליו הקולמוס ויהיו לעשרה ועם הב' יהיו י"ב נוציא מהם הז' ישארו ה' נשימם על הב' | ||||||||||||||
|
עוד נכפול הז' בו' יעלו מ"ב | ||||||||||||||
|
נסיר הב' מהח' ואש' עליהם ישארו ו' נשימם עליהם | ||||||||||||||
|
ונסיר הד' עשרות מהה' אשר במעלה שאחריהם וישאר א' ונשימנו עליו | ||||||||||||||
|
עוד נכפול הז' על עצמו ויעלה מ"ט | ||||||||||||||
|
ומה0 אשר עליו לא נוכל להסיר אפילו האחדים לכן נסיר מהו' שאחרי הה' וישאר א' ויהיה נ' במעלתם ה0' נסיר מהם המ"ט וישארו א' ונשימנו עליהם | ||||||||||||||
|
עוד נורידם מעלה אחת ונכפול הז' אשר נתחדש בזאת הפעם ויהיו י"ד ונחבר העשר לא' עם הו' אשר אחריו לצד שמאל ויהיו ז' אחרי כן נשים הד' שהם האחדים לפני הז' | ||||||||||||||
|
[128]ונבקש מספר לכפול על כולם ועל עצמו כבשאר הפעמים ולא נמצא כי אין גם אחד לפי שלא יוכלו לצאת מאשר על ראשם אפי' פעם אחת לכן נשים [סיפרא][129] לפניהם | ||||||||||||||
|
ונורידם עוד מעלה אחת ולא נכפול שום מספר כי לא נתחדש מספ' בפעם הזאת וה0' אינה מספר לכפלה | ||||||||||||||
|
ונבקש מספר שנשים לפניהם ויהיה ט' | ||||||||||||||
|
ונכפלנו בכל אחד ונוציאנו מאשר ימצא על ראשו וגם בעצמו ונוציאנו מאשר על ראשו כאשר תראה בצורה הרשומה | ||||||||||||||
|
עוד נורידם ונכפול הט' שנתחדש עתה בפעם הזאת ויהיו י"ח ואחר שנתחדש כאן עשר עם האחדים לא נשים ה0' בהורדה זו אבל נשים א' לעשר במקומה ונשים הח' שהם אחדים לפניו | ||||||||||||||
|
ונבקש מספר נשים לפניהם כפעם בפעם ויהיו ד' | ||||||||||||||
|
ונכפלנו בכל אחד גם בעצמו ונוציא כל דבר ממקומו הראוי לו כנזכר | ||||||||||||||
|
והנה הגענו למעלה הראשונה לכן אין לנו להורידם | ||||||||||||||
|
ויהיה השרש המספר שחדשנו בכל פעם אחד והם 587094 | ||||||||||||||
|
ואם לא היה נשאר דבר היה זה השרש אמיתי אבל אחר שנשאר דבר והוא 764230 אין השרש הזה אמיתי כי אם בקרוב | ||||||||||||||
|
|
|||||||||||||||
| It will be explained afterwards how we come closer to the truth, even if the truth is absent. | ועוד נתבאר אחר זה איך נתקרב יותר אל האמת ואם האמת נעדרת | ||||||||||||||
|
משל אחר רצינו לדעת שרש מספר זה 10375 | ||||||||||||||
|
|||||||||||||||
|
ואחר שמספר המעלות נפרד שהן ה' נקח הא' אשר נמצא במעלה האחרונה | ||||||||||||||
|
ונבקש מספר שנכפלנו בעצמו ונוציאנו כלו או היותר שאיפשר ויהיה א' ונשימנו תחתיו | ||||||||||||||
|
ונכפול לא' זה על עצמו ונוציאנו מהא' [130]אשר על אשר על ראשו ונעביר עליו קולמוס | ||||||||||||||
|
ונכפלנו ונורידנו ולא נוכל להוציאם מה0' אשר עליהם אפי' פעם אחת גם על האחד לא נותר דבר לכן נשים 0 לפניו עוד נורידם ולא נכפול דבר כי לא נתחדש מספר בזה הפעם | ||||||||||||||
|
ונבקש מספר אשר נשים לפניהם כנזכר ויהיה א' ונשימנו לפניהם | ||||||||||||||
|
ונכפול הא' על הב' ויהיו ב' נסירם מהג' אשר עליהם ישאר א' ונשימנו עליו | ||||||||||||||
|
ונכפול הא' על עצמו ויעלה א' נסירנו מהה' אשר עליו וישארו ד' נשימם עליו | ||||||||||||||
|
וכבר שלמו המעלות ולא נוריד עוד | ||||||||||||||
|
והנה האותיות והם שנתחדשו פעם בפעם הם השרש והוא 101 | ||||||||||||||
| |||||||||||||||
|
ולפי שנשאר ולפי שנשאר שם מספר מה אין זה שרש אמיתי אבל הקרוב | ||||||||||||||
| It will be explained how we come closer to the truth. | ועוד נתבאר איך נתקרב יותר אל האמת | ||||||||||||||
reason: procedure |
|||||||||||||||
The reason we say that if the number of the ranks is an odd number we take the last digit alone and seek a number to put beneath it and if it is an even number we take the two last digits, the last as tens and the one that precedes it as units: because for every product of a non-units rank by itself, the rank of the units of this product [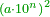 ] is always an odd number [ ] is always an odd number [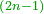 ]. ].
|
וטעם אמרנו שאם מספר מעלות החשבון נפרד שנקח האחרון לבד ונבקש מספר נשים תחתיו וכו' ואם הם זוג שנקח השני רשמים האחרונים האחרון לעשרות ושלפניו לאחדים הוא לפי שכל כפל כלל בעצמו הנה מעלת האחדים העולים בכפל ההוא היא נפרד לעולם | ||||||||||||||
| Since the decimal place of the product of every two digits, i.e. the rank of this product is as [the sum of] the ranks of both digits minus one, as we have explained in chapter three. | לפי שמקום הנחת כפל כל שני מספרים ר"ל שמדרגת הכפל ההוא כמדרגות שני המספרים יחד חסר אחד כמו שביארנו בפרק הג' | ||||||||||||||
| Hence, the rank of the units of the product of every number by itself is double its rank minus one, and this is always an odd number. | ולזה מדרגת אחדי מספר כפל מספר על עצמו והיא כפל מדרגותיו חסר אחד והנה הם הנפרדים לעולם | ||||||||||||||
| So, when the number of ranks of the number is odd, we subtract from the last rank [= the leftmost rank] the square of the root, i.e. the square of the digit that we write beneath it, which is part of the root. | ולזה כשהמספר מעלות המספר נפרד אנו מוציאים מהמעלה האחרונה שהיא נפרדת מרובע השרש ר"ל מרובע המספרים אשר שמנו תחתיו שהוא חלק השרש | ||||||||||||||
| But, if the number [of ranks] is even, we subtract [the square of the last rank of the root from] the two last ranks [of the number] one as tens and the other as units. For the units of the product of a number by itself is always placed in an odd rank and the tens in an even rank, and this is clear. | ואם הם זוג לקחנו השתים האחרונות זו לעשרות וזו לאחדי' [131]בענין שלעולם אחדי כפל כל מספר בעצמו יצאו ממעלה נפרדת והעשרות ממעלת זוג וזה ברור | ||||||||||||||
| Since we have explained that the rank of the units of the product [of a number by itself] is double the ranks of the root, which is the number that we multiply by itself, minus one, we find that if the root is in the first [rank], the product is also in the first [rank]; if the root is in the second [rank] the square is in the third [rank]; if [the root is in] the third, [the square is] in the fifth; if [the root is in] the fourth, [the square is] in the seventh; and so on. | ואחר שביארנו שמדרגות אחדי הכפל הם כפל מעלות השרש שהוא המספר שכפלנוהו על עצמו חסר אחת נמצא שאם השרש | ||||||||||||||
| Thus, the addition of one rank in the root requires an addition of two ranks in the square. | הנה כי תוספת מעלה אחת בשרש יחייב תוספת א"כ ב' מעלות במרובע | ||||||||||||||
| We do accordingly in the procedure: for every two ranks added to the number, we add one [rank] to the root, by lowering the root each time by one rank and adding one rank to it. | וכן נעשה במעשה | ||||||||||||||
| We find that tnumber of the shifting phases [in the procedure] is as the number of the even ranks of the [given] number from the first decimal position as well as the number of ranks of the root from the first rank. You will see all this explained in the diagram. | נמצא שכמספר פעמי ההורדה כך הוא מספר זוגי מעלות החשבון על מקום ההנחה הראשונה וכמספר זה הוא | ||||||||||||||
| The reason for shifting the subtrahend one rank to the right each phase: | |||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| The reason for lowering by one rank each time is that what is added in the root at that time is one rank lower than what was [before], therefore, the rank of the [square] is also lower by one rank, i.e. what is added now in the root multiplied by what is already given to the root in the previous phase or phases. | והטעם הורדת מעלה אחת בכל פעם הוא לפי שהמתוסף בשרש בפעם הזאת הוא מעלה אחת פחות מאשר נתוסף בתחלה וא"כ מעלת הכפל יהיה ג"כ מעלה אחת פחות ר"ל כפול זה המתוסף עתה בשרש באשר היה כבר המונח לשרש בפעם או בפעמים העוברים | ||||||||||||||
| Because if what is given at first is, for instance, a product of the digit by itself, what we add now in the root is less than the former by one rank, and when we multiply it by the former, [the product] is subtracted from the rank that [the former] is subtracted. | כי ע'ד'מ' המושם בתחלה הוא מכפל המספר בעצמו וכאשר אנחנו מוסיפים עתה בשרש זה המתוסף הוא פחות מעלה אחת מהראשון וכאש' כפלנוהו בראשון יגרע זאת המעלה אשר גרע זה ממנו | ||||||||||||||
|
כי המשל אם כפל השרש הראשון בעצמו היה לוקח מהמעלה החמישית הוא היה מן המעלה השלישית ולזה לקח [133]מהחמישית שהוא כפל מעלותיו חסר אחת | ||||||||||||||
|
וכאשר נוסיף זה עתה בשרש יהיה המתוסף מהמעלה השנית וכאשר כפלנו אשר מהמעלה השנית על אשר במעלה הג' ר"ל כאשר אנו כופלים זה המתחדש עתה שהוא במעלה הב' באשר היה בתחלה שהוא מהמעלה הג' יהיה מדרגת זה הכפל במדרגת הד' שהם מספר מעלות שני המספרים חסר אחת ולזה שמנו אשר בתחלה מעלה אחת למטה כי משם הוא ראוי לקחתו | ||||||||||||||
|
השרש המתוסף כאשר כפלנוהו בעצמו יגרע מעלה אחרת ואין לו לקח' כי אם מהמעלה השלישית כי כפל בעל שתי מעלות בבעל שתי מעלות יש לו לקחת מהשלישית שהוא כמדרגות שני המספרים חסר אחת לכן שמנוהו מעלה אחת לפניהם כי משם ראוי לו לקחת | ||||||||||||||
| In each phase, the rank, from which the product of [the digit added in the root] by the former [digits] is to be subtracted, is lower by one than [the rank of] the product of [the digit added in the root] in the previous phase by the [former digits], and [the rank of] its product by itself is lower by two. | וכן בכל פעם יחסר מעלה ממקום הראוי לקחת עתה בכפל המתחדש בראשונים מאשר היה מכפל המתחדש בפעם העובר עמהם וכפלו בעצמו יחסר שתים | ||||||||||||||
| All this is explained in reason and in diagram. | וכל זה מבואר בטעם ובצורה | ||||||||||||||
| When we cannot subtract [the product] even once, we shift [the digit added in the root] once, and what is added in the root is lower by two ranks from [the former digit], so we lower it by two ranks, to be subtracted from the rank that is lower by two and the product of what is added in the root by itself is subtracted from the rank that is lower by four ranks [from where the product of the former digit was subtracted], since [the added digit] is lower by two ranks. | וכאשר אין אנו יכולים להוציאם אפי' פעם אחת אנו שמים ומורידים אותם פעם אחרת כי כאשר תוסף בשרש יהיה פחות ב' מעלות מאשר בתחלה לכן הורדנום ב' מעלות שיקחו מב' מעלות פחות וכפל השרש המתוסף בעצמו יקח מד' מעלות פחות לפי שירד שני מעלות | ||||||||||||||
|
כי המשל אם הראשון היה ברביעית היה לו ליקח כפלתו בעצמו מהמעלה השביעית ואשר מתוסף עתה כשהיה 0 בפעם אשר בנתים יהיה בשנית וראוי לקחת כפלו בעצמו מהשלישית הרי כשנגרע ד' מעלות | ||||||||||||||
| All this is also explained in reason and in diagram. | גם כל זה הוא מבואר בטעם ובצורה | ||||||||||||||
| The reason for doubling the digits of the root: | |||||||||||||||
| The reason for doubling the root, i.e. that in every [phase of the procedure] we multiply [the digit that is added to the root] by double the former [digit of the] root and by itself, is because every thing that is added to the root is added to both sides [= multiplicands] of the square. | וטעם הכפל השרש ר"ל שבכל הולדה אנו כופלים אשר | ||||||||||||||
|
ר"ל שאם מתחלה היה השרש 30 הנה המרובע היה 900 | ||||||||||||||
|
ואם אנו מוסיפים עליו ה' יהיוה ל"ה ומרובעו הוא כפל ל"ה על ל"ה שהוא כאומרנו לכפל ל' בל' וכפל ה' [בל' וכפל ל' בה' וכפל ה'][135] בה' | ||||||||||||||
|
נמצא שנתוסף בסבת תוספת הה' כפל ה' על ל' פעמים ר"ל ה' בה' פעם אחת ובעצמו פעם אחת |
| Therefore, we double the root, but when we lower [the digits of the root], we double only the [digit] the was added in the preceding phase that was not doubled yet, as all the [other digits] are already doubled, therefore we do not double them again, since the root consists of the digits that are added in each phase that are not doubled at all. | ולזה אנו כופלים השרש וכשאנו מורידים אין אנו כופלים אלא אשר מתוסף בפעם העובר בסמוך שלא נכפל אבל כל אחדים כבר נכפלו לכן אין אנו כופלים אותו פעם אחרת כלל ומכל זה תדע כי השרש הוא המספרים המתחדשים בכל פעם פשוטים בלי כפל כלל |
Approximations |
|
| When something remains there after you have completed the extraction of the root, and you wish to come closer to the truth, consider this remainder.
|
וכאשר נשאר שם דבר מה אחר אשר השלמת להוציא השרש ותרצה להתקרב עוד אל האמת עיין אשר נשאר |
|
ואם הוא פחות מהשרש כפול השרש והוצא את מוריו וחלק השארית ההיא עליהם והיוצא הוא העודף בשרש על השלמים ההם |
|
ואם השארית היתה גדולה מהשרש או כמותו ואין דעתך להתקרב עוד אל השרש כי אם מה שתתקרב אליו בפעם זו לבד תכפול השרש ותוסיף עליו א' ותחלק עליהם זאת השארית והיוצא הם השברים הנוספים בשרש על השלמי' אשר יצאו ראשונה |
|
ואם תרצה להתקרב עוד אל האמת ואם האמת נעלמה מעיני כל חי[note 32] כאשר ביאר אוקלידס במופת כפול אלו השלמים והשברים על עצמם כאשר אבאר בחלק השברים בפרק הכפל ויעלה פחות או יותר מהחשבון הראשון |
|
וכפול השרש כאשר אמרנו וחלק אליו זה העודף [136]או חסרון |
|
והיוצא הוציאנו מהשברים הראשונים אם המספר היה פחות ממרובע השרש אשר הוצאת בפעם הקודמת |
|
ואם היה המרובע פחות מהמספר תוסיף זה היוצא על השברים הראשונים |
|
והעולה או הנותר יהיו השברים העודפי' בשרש על השלמים הראשונים |
|
המשל בזה הוא בצורה השנית 174 |
|
ואם היה פחות מהשרש היינו מחלקים אותו לכפל השרש שהוא 202 בלי תוספת אחד |
|
וכן עתה שהוא יותר מהשרש נכפול השרש שהנו 101 ויהא 202 ונוסיף עליו א' ויהיו 203 |
|
ונוציא מוריו ונמצא שיש לו שביעית ושביעיתו 29 ואלו הם מוריו ר"ל ז' כ"ט |
|
ונחלק אליהם השארית שהוא 174 ויצא בחילוק ו' שביעיות שלמות ואלו הם השברים העודפים בשרש על הק"א השלמים הראשונים |
|
ואם נרצה להתקרב עוד אל האמת נכפול זה השרש ר"ל ק"א שלמים וו' שביעיות על עצמו 10374 שלמים וו' שביעיות שלימות ושביעית שביעית כאשר יתבאר בחלק הב' בפרק הג' |
|
וזהו פחות מהחשבון הנשאל בו' שביעיות שביעית |
|
לכן אם אתה רוצה להתקרב עוד אל האמת יש לך לכפול השרש ר"ל הק"א שלימים וו' שביעיות שלימות ולחלק אליהם אלו הו' שביעיות שביעית |
| This is the rule [of approximating the root]: | זה הכלל |
|
ראשונה תחלק הנשאר לכפל השרש עם תוספת א' אם הנשאר גדול מהשרש או כמותו |
|
[137]ואם פחות לא תוסיף א' |
|
והיוצא תוסיפנו על השרש |
|
|
|
|
ותכפול אותו השרש על עצמו שלמים ונשברים |
|
ואם |
|
ואם היה העולה פחות מהחשבון תראה בכמה הוא ותחלקנו לכפל השרש ג"כ ותוסיפנו על השרש הקודם |
| And so on. | וכן לעולם |
| You come ever closer to the truth, but you will never attain it. | ולעולם תתקרב יותר אל האמת ולא תשיגנה לעולם |
| Shortcuts | |
|---|---|
| If you look closely, you will see that you do this with less effort. | וכאשר תעיין הטב תראה שתוכל לעשותו בלי כ"כ יגיעה |
| This is by looking at the fraction attained in the [first] step. | והוא שתעיין השברים שנתחדשו בעת ההיא |
|
|
|
ואם היו לתוספת ונעשה בתוספת א' על כפל השרש ראה כמה כפל השברים המתחדשים ההם בפעם ההיא במה שיש מהשברים ההם עד תשלום |
|
|
|
והוא אשר יש לך לחלק עוד על כפל השרש בעצמו ולהוסיפו עליו |
|
וזה תוכל לראות ברור בדמיון שעבר שהיה לתוספת ובתוספת א' והשברים ההם שהיו ששה שביעיות |
|
והנה השלמתם לשלם הוא שביעית אחת וכאשר תכפלם בהשלמה זו יעלה ו' שביעיות שביעית וזה בעצמו הוא שמצינו חסר בכפל השרש מהחשבון [הא'][138] וצוינו לחלקו לכפל השרש ולהוסיפו על השרש |
|
|
|
אך אם היה לתוספת בלי תוספת א' שהיו למגרעת נראה כפל השברים אשר נתחדשו על עצמם ונחלקם לכפול השרש |
|
|
|
והיוצא נחסרנו לעולם מהשרש הקודם וכן לעולם |
| Therefore, if you wish to repeat the procedure in order to come closer to the truth, [do this], because the more you repeat, the nearer you come to the truth, even if you can never attain it, as we have explained. | ולזה אם רצונך להכפל זה המעשה כדי להתקרב אל האמת כי כל מה שתוסיף להכפל זה הענין [139]תוסיף להתקרב אל האמת ואם לא תשיגנה לעולם כמו שביארנו |
| [If you repeat the procedure], never add 1 to double the root, even if the remainder is very large with respect to the [approximate] root, so as to avoid confusion, for [adding 1] was instructed only for a single [approximation] step. | לא תוסיף א' לעולם על כפל השרש [ואף אם יהיה הנשאר הרבה מאד על השורש][140] כדי שלא יבלבל עליך כי לא ציויתיו אלא למסתפק בפעם אחת |
Adding 1 [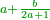 ] when the remainder is the same as the [approximate] root or greater, improves the approximation, as I explained, but if one repeats the procedure [ ] when the remainder is the same as the [approximate] root or greater, improves the approximation, as I explained, but if one repeats the procedure [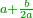 ], one does not need this addition, because by repeating the procedure one approaches [the truth] very closely even without adding 1. It is better not to add it, so as to maintain a standard form of procedure and prevent confusion. ], one does not need this addition, because by repeating the procedure one approaches [the truth] very closely even without adding 1. It is better not to add it, so as to maintain a standard form of procedure and prevent confusion.
|
ובתוספת הא' כשהנשאר כשרש או יותר הוא מתקרב יותר כמו שכתבתי אבל המכפיל פעמי המעשה אינך צריך לתוספת זה |
The reason that if the remainder is smaller than the approximate root 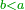 , it is divided by double the root , it is divided by double the root 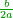 : :
|
|
| The reason we say that if there is a remainder that is smaller than the [approximate] root, then we should divide it by double the root, is because that which is added to the root will add to the square its product by twice the previous root and its product by itself, as we explained with regard to integers.
|
וטעם אומרנו שאם ישאר דבר והוא פחו' מהשרש שנחלקנו לכפל השרש הוא לפי שאש' יתוסף בשורש יוסיף במרובע כפלו בשורש הראשון פעמים גם כפלו בעצמו כאשר ביארנו בשלמים |
|
|
| But, we proceed as if it only adds its product by twice the root. If this were true, i.e. that what is added to the root adds to the square only the product of what is added to the root by twice the root, then we would have this product, which equals the excess of the number [whose root is extracted] over the square of the integer [received through the extraction algorithm]. | ואנו עושים מעשינו כאלו אינו מוסיף כי אם כפלו בשרש פעמים ואם היה זה האמת ר"ל שהמתוסף על השרש לא היה מוסיף על המרובע כי אם כפל זה המתוסף בכפל השרש לבד |
| Hence, when we add in the root what is equal to its product by twice the root, as this excess itself, we reach the required result. | וכאשר נוסיף בשרש דבר מה שיהיה שוה כפלו בכפל השרש כזה התוספת בעצמו הגענו אל מבוקשנו |
| Altough this addition is unknown to us, as we know the result of the multiplication, which is the mentioned remainder, and we also know one of the multiplicands, which is double the root, when we divide the product by double the root, the result is the unknown, which is the addition, i.e. by multiplying this addition by double the root, the result is the mentioned remainder and this is clear.
|
ועם היות שנעלם ממנו תוספת זה ומ"מ אחר שידענו העולה מהכפל ההוא והיא השארית הנזכרת גם ידענו אחד מהנכפלים והוא כפל השרש [הנה בחלקנו זה העולה לכפל השורש יצא][141] יצא הנעלם שהוא התוספת ר"ל כי בכפול זה התוספת בכפול השרש יעלה כנשאר הנזכר וזה ברור |
| Since, that which is added to the root, adds also to the square its product by itself, i.e. the product of this addition by itself. | אכן לפי שהמתוסף על השרש מוסיף עוד במרובע כפלו בעצמו ר"ל כפל התוספת הזה בעצמו |
| Therefore, when we multiply the root by itself after this addition is added to it, the square will exceed the initial number [whose root is extracted] by the square of the addition. This is what we explained above.
|
לכן כאשר נכפול השרש בעצמו אחר הוסיף [142]עליו זה התוספת יעלה המרובע מוסף על החשבון הראשון כפל התוספת הזה בעצמו וכן ביארנוהו למעלה |
|
|
|
ואם היינו רוצים להתקרב עוד ואנו מחלקים זה התוספת לכפל השרש הזה והיוצא יחסר מזה השרש כאשר ביארנו למעלה |
- The excess of the second approximation:
|
הנה זה שאנו מחסרים היה מוסיף על המרובע ככפלו על כפל השרש המחוסר הזה לאחר חסרונו וכפלו על עצמו בלי כפל |
|
ואולם התוספת אשר היה לנו חלקנוהו על כפל כל השרש |
|
ואולם הוא לא היה מוסיף כי אם כפלו על כפל השרש הזה המחוס' וכפלו על עצמו בלי כפל |
|
נמצא שלא חסרנו בכל הצורך אבל עוד ישאר במרובע זה השרש המחוסר תוספת על החשבון הראשון ככפל זה החסרון על עצמו וזה ברור וכן יהיה לעולם |
| So, when we do not add 1 [to the denominator], and wish to come closer to the truth [using a repetitive procedure for extracting the root], [in the first step] we should only add the fraction of the first step [i.e., the remainder divided by twice the approximate root]. | לכן כאשר לא נוסיף א' ונרצה להתקרב אל האמת אין לנו להוסיף על השרש כי אם השברים הראשונים אשר נתחדשו בפעם הראשון מאשר נשאר לנו |
| But, from there on we must divide the square of the fraction produced at that step by twice the previous [approximate] root. And the result should always be subtracted from the previous [approximate] root. | אבל מכאן ואילך לעולם יש לנו לחלק כפל השברים המתחדשים בפעם ההיא על כפל השרש הקודם לו והיוצא נחסרהו לעולם מהשרש הקודם לו |
|
המשל בקשנו שרש ז' |
|
הנה השלמי' אשר בשרשו הם ב' ונשארו ג' |
|
ואם חלקנום לכפל השרש יצא בחילוק ג' רביעיות |
|
והנה זה התוספת כאשר נחברהו אל הב' השלמים ונעשה מהכל שרש אחת הנה יתוסף במרובעו יותר על מרובע הב שיהיה ד' שלמים ככפל שלש רביעיות אלו בעצמם |
|
ואולם [143]שאריתנו לא היה כי אם ככפל הג' רביעיות בד' השלמים אשר הם כפל השרש הראשון |
|
נמצא שנתוספו בשרשנו זה שברים יותר מדאי עד שמרובע הכל יהיה יותר על הז' שלמים ככפל הג' רביעיות בעצמם שהם ט' רביעיות רביעית שהם ב' רביעיות שלמות ורביעית רביעית וזה ברור כי כפל ב' וג' רביעיות עולה ז' שלמים וב' רביעיות רביעית כאשר יתבאר בחלק הב' בפרק הג' ממנו |
|
ולזה ראוי לנו לחלק תוספת זה על כפל השרש כאשר ביארנו |
|
והנה כפל השרש הוא ה' שלמים וב' רביעיות שהם חצי שלם |
|
וכאשר נחלק עליהם ב' רביעיות ורביעית רביעית יצא בחילוק ט' חלקים מי"א מחצי רביעית |
|
וכאשר נסירם מהשרש הקודם ישאר ב' שלמים וב' רביעיות שלמות וחצי רביעית וב' חלקים מי"א מחצי רביעית וכל זה יתבאר מעשהו בחלק הב' |
|
והנה יחסר מרובע השרש הזה ר"ל הב' שלמים וב' רביעיות וחצי רביעית וב' חלקים מי"א מחצי רביעית אחרי החסרו מאשר לפניו ככפל החסרון הזה ר"ל הט' חלקים מי"א מחצי רביעית על כפל השרש המחוסר וככפלו לעצמו |
|
ואולם התוספת הראשו' אשר היה במרובע על החשבון היה ככפל החסרון זה בכפל השרש הראשון ר"ל בכפל השרש הזה המחוסר ובכפל החסרון הזה שהרי כאשר חלקנו התוספת על כפל השרש הקודם ר"ל על כפל השרש המחוסר ועל כפל זה החסרון [יצא בחלוק זה החסרון][144] |
|
נמצא שכפל זה החסרון ר"ל הט' חלקים מי"א מחצי רביעית בכפל השרש הראשון שהוא ב' שלמים וג' רביעיות שכפלו ה' שלימים וחצי שהוא כמו כפל [145]השרש המחוסר הזה ר"ל הב' שלימים וב' רביעיות וב' חלקים מי"א מחצי רביעית וכפל זה החסרון שהוא הט' חלקים מי"א מחצי רביעית |
| It is known with little intelligence that the product of a number by a number is as its product by all the parts of the other number, each one by itself.
|
כי ידוע הוא במעט התבוניות כי כפל מספר על מספר הוא ככפלו בכל חלקי המספר האחד כל אחד בפני עצמו |
| This is the reason of the product of a known number by the double of another number. | והוא הטעם שכפל מספר ידוע על כפל מספר ידוע אחר |
|
המשל על כפל ד' הוא ככפלו על כפל כל חלקיו כל אחד בפני עצמו המשל על כפל ג' ועל כפל א' |
|
וזהו כאומרנו שכפל הט' חלקים מי"א מחצי רביעית בכפל השרש הראשון שהוא הב' שלמים וג' רביעיות הוא כמו כפלו בכל חלקיו כל אחד בפני עצמו ר"ל בכפל השרש המחוסר ובכפל החסרון שהם חלקי השרש הקודם וזה ברור |
|
|
|
|
ואחר שהמרובע הקודם היה מוסיף על החשבון אשר רצינו לידע שרשו ככפל החסרון על כפל כל השורש הקודם שהרי כשחלקנו אותו על כפל השורש הקודם [יצא זה החסרון הנה כאשר נכפול זה החסרון בכפל השורש הקודם][146] שהוא כפל היוצא בחילוק במספר אשר חלקנו עליו יעלה כמספר המתחלק שהוא התוספת שהיה לנו |
|
ואולם בשביל זה החסרון אשר אנו מחסרים עתה מהשרש לא יחסר המרובע הזה מהראשון כי בכפל זה החסרון בכפל המחוסר ובעצמו בלי כפל |
|
|
|
|
א"כ ישאר עוד מהתוספת כפל זה החסרון בעצמו |
| Thus, we have explained that when we repeat this [procedure] a few times, the excess of the square [of the approximate root over the given number] is always the square of the fraction resulting from the division at that phase, that we have to add to the [approximate] root in the first phase, or to subtract from the [approximate] root in all other phases. This is provided that we do it without adding one, i.e. if we do not add one to double the [approximate] root, by which we divide [the excess], when the excess is greater than the [approximate] root, but we divide the excess only by double the [approximate] root without adding one at all. | הנה ביארנו כי בעשותינו זה כמה פעמים לעולם ישאר במרובע תוספת מרובע השברים שיצאו בחילוק בעת ההיא שהם אשר עלינו להוסיף על השרש [147]במעשה הראשון או לחסרו מן השרש בשאר הפעמים כלם אם לא נעשנו בתוספת אחד ר"ל אם לא נוסיף אחד על כפל השרש לחלק על הכל אם יהיה התוספת גדול מהשרש אלא שנחלק התוספת על כפל השרש לבד בלי תוספת אחד כלל |
| This is the reason that we say that when we do not add one, we always take the square of the additional or subtracted fraction resulting the last phase, divide it by double the [approximate] root, then subtract the quotient from the [approximate] root. The reason of this procedure is clear. | ולזה אמרנו כי כאשר לא נעשה בתוספת אחד לעולם נקח מרובע השברים אשר יצאו בפעם האחרונה הן לתוספת או למגרעת ונחלקם על כפל השרש המחוסר והיוצא נחסרנו מהשרש וכן נעשה לעולם וכל זה ברור בטעם |
The reason that if the remainder is equal to the approximate root or greater than it 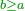 , it is divided by double the root plus one , it is divided by double the root plus one 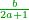 : :
| |
|---|---|
| The reason we say that when the remainder equals to the [approximate] root or greater than it, we should divide it by double the root plus one, as long as we do not intend to repeat the procedure so as to further approach the truth except for this [step] only, is that if we had not added one, the square of the root consisting of the integer and fraction would exceed the number [whose root is extracted] by the square of the fraction received in the division. But this would be a quarter or more. | וטעם אומרנו כי כאשר הנשאר הוא כשורש או יותר ממנו שיש לנו לחלקו על כפל השרש בתוספת אחד אם אין דעתינו להכפיל המעשה להתקרב עוד אל האמת זולתי בפעם הזאת לבד |
|
|
|
|
לפי שאם יהיה כשורש בעינו ונחלקנו על כפל השרש יצא בחלוק חצי ומרובעו ר"ל כפלו בעצמו שהוא התוספת שיהיה רביעית שלמה |
|
ואם יהיה השארית יותר גדול מהשרש כשנחלקנו על כפל השרש יהיה היוצא יותר מחצי ומרובעו יותר מרביעית |
|
והמשל בקשנו לידע שרש ו' |
|
הנה השלמים אשר יצאו בשרש הם ב' וישארו ב' שהוא כמו השרש בעצמו |
|
ואם חלקנום על כפל השרש בלי תוספת אחת יתחלק לד' שהוא כפל השרש ויצא בחילוק חצי ויהיה כל השרש ב' שלמים וחצי |
|
ומרובעם ו' שלמים ורביע |
|
ואם היינו מוסיפים [148]א' הנה יחסר מהמרובע ככפל היוצא בחילוק שיהיה פחות מחצי בהשלמתו לאחד וזה יהיה פחות מרביע |
|
הנה א"כ הוא קרוב אל האמת כי לא יחסר רביע במרובע מהחשבון |
|
ואם לא נוסיף א' נוסיף רביע וכ"ש אם היה גדול מהשרש |
|
כי כאשר נחלקנו לכפול השרש יצא בחילוק יותר מחצי ומרובעו יותר מרביע כאשר תראה במשל הקודם לזה |
| If you divide [the remainder] by double the root plus 1, the square of the root will be less than the sought number by the product of the quotient and its complement with respect to one, which can never in any way reach a quarter. | ואם תחלקנו על כפל השרש בתוספת א' יחסר מרובע השרש המקובץ מהחשבון הנשאל ככפל היוצא בחלוק בהשלמתו לאחד ולא יהיה אפי' רביע בשום פנים |
|
כי כאשר תכפול קצת הקו או המספר בקצתו האחר לא יעלה לעולם לרביע |
|
שאם תכפול חציו בחציו יהיה רביע |
|
ואם תכפול מעוטו ברובו לא יהיה רביע וזה ברור אבל יחסר ממנו כמרובע מרחקם מחצי הקו או המספר |
|
כי ע'ד'מ' אם חלקנו הקו לרביע הקו וג' רביעיות הנה אם תכפול החצי בחצי הוא כאלו תכפול רביע הקו |
|
ואם תכפול רביע הקו בג' רביעיות המשלימות אותו לאחד שלם לא יהיה כי אם כפל רביע הקו ברביע הקו ג' פעמים |
|
הנה יחסר מחצי על חצי ככפל רביע על רביע שהוא מרחק כל אחד מחלקי הקו מהחצי |
|
ואם תכפול ע'ד'מ' חצי מספר י"ב בחציו שהוא ו' בו' יעלה ל"ו שהוא כפל ו' בה' שהם ל' וכפלו ו' בא' שהם ו' |
|
ואולם אם תכפול ה' בז' שהם השלמתו לאחד לא יהיה כי אם ל"ה לפי שהוא ככפל ה' בו' שהם ל' וכפל ה' בא' שהם ה' |
|
וכל מה [149]שיתחלקו יותר החלקים יחסר יותר |
|
וזה שהחסרון מרביע הוא כמרובע הרחקתם מחצי |
| ? | כבמשלנו זה שהיה כמרובע האחד אשר נתרחקו מו' שהוא החצי |
|
ואם היינו כופלים ג' בהשלמתו לי"ב שהוא ט' הנה לא יעלה כי אם כ"ז ויחסר כמרובע ג' שנתרחקו מהחצי שהוא ט' |
|
וזה כי כפל ו' בו' הוא ככפל ו' בג' וככפלו ו' בג' שהוא כפל ג' בג' פעמים |
|
ואולם כפל ג' בט' אינו כי אם כפל ג' בו' וכפל ג' בג' פעם אחת לבד |
|
לכן יחסר מרביע מרובע המספר ככפל ריחוקם מהחצי בעצמו והוא רביע רביע |
|
לפי שרחוקם היה ג' שהוא רביע הי"ב דוק ותשכח |
The reason for 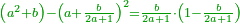
|
|
|---|---|
| Our saying that when we divide [the remainder] by double the root plus 1, the square of the summed [approximate] root is smaller than the [original] number by the product of the quotient by its complement with respect to one - | ואולם אומרנו שכאשר נחלקנו לכפל השרש בתוספת אחד שיהיה החסרון אשר במרובע השרש המקובץ מהחשבון ככפל היוצא בחילוק בהשלמתו |
| I shall explain it first by the previous examples, then I shall explain its reason: | אבארנו תחלה במשלים העוברים וא'ח'כ' אבארנו בטעם |
|
המשל כאשר בקשנו שרש ז' |
|
והיה ב שלמים ונשארו ג' שהם יותר מהשרש |
|
וחלקנום לכפל השרש בתוספת א' ר"ל על ה' יצא בחילוק ג' חמישיות |
|
וכאשר נכפול שני שלמים וג' חמישיות על עצמו יהיה מרובעו ו' שלמים וג' חמישיות וד' חמישיות חמישית |
|
והחשבון הנשאל היה ז' שלימים הנה יחסר זה המרובע מז' שלמים חמישית [אחת שלמה וחמישית חמישית |
|
וזה][150] וזה בעצמו הוא כפל הג' חמישיות אשר יצאו בחלוק על השלמתם לאחד שלם שהוא ב' חמישיות |
|
כי כפל ג' חמישיות בב' חמישיות הוא ו' חמישיות חמישית שהן חמישית אחד שלם וחמישית חמישית |
|
ובמשל בשני אם בקשנו שרש |
|
והנה יצאו ב' שלמים ונשארו ב' שהוא כמו השרש |
|
אם חלקנום על כפל השרש בתוספת א' ר"ל על ה' [151]יצא בחילוק ב' חמישיות |
|
וכאשר כפלנו שני שלמים וב' חמישיות על עצמם יעלה ה' מרובעו ה' שלמים וג' [חמישיות][152] וד' חמישיות חמישית |
|
ואולם החשבון הנשאל אשר בקשנו שרשו היה ו' שלמים הנה יחסר זה המרובע מהחשבון ההוא חמישית אחת שלימה וחמישית חמישית |
|
והוא ככפל הב' חמישיות אשר יצאו בחילוק בהשלמתם לאחד שהוא ג' חמישיות |
|
כי כפל ב' חמישיות בג' חמישיות הוא ו' חמישיות חמישית שהן חמישית אחד שלמה וחמישית חמישית כאשר ביארנו |
| The reason is that the remainder is as the result of division multiplied by twice the [previous approximate] root plus one.
|
והטעם הוא לפי שהשארית היה ככפל זה היוצא בחילוק בכפל השרש הראשון ובאחד |
| For, when we divide the remainder by double the [approximate] root plus 1, we find that the remainder is as the product of this quotient by double the [approximate] root plus 1. | שהרי בחלקנו השארית לכפל השרש בתוספת א' יצא זה בחלוק נמצא שהשארית היה ככפל זה היוצא בחלוק בכפל השרש הקודם [ובא'][153] |
| The addition of the result to the previous [approximate] root, however, will add to the square only its product by twice the previous root and its product by itself.
|
ואולם תוספת זה היוצא בשרש הקודם לא יוסיף במרובע כי אם ככפלו בכפל השרש הקודם ובכפלו בעצמו |
| But, its product by itself subtracted from its product by 1 is its product by its complement [with respect to 1].
|
וכפלו בעצמו יחס' מכפלו בא' כפלו בהשלמתו לאחד |
|
כי המשל כפל שליש באחד הוא ככפלו בכל חלקיו ר"ל ככפלו בשליש ר"ל בעצמו וככפלו בשתי שלישים אשר הם המשלים אותו כאחד וזה ברור |
|
|
| Thus, we have explained that when the remainder is as the [approximate] root or greater than it, if we divide it by double the [approximate] root plus 1, it comes closer to the truth by subtraction more than it come closer to the truth by addition when we divide it by double the [approximate] root without adding 1. | הנה ביארנו כי כאשר השארית היה כשרש או יותר ממנו כי בחלקנו אותו לכפל השרש בתוספת א' יתקרב אל האמת לחסרון מאשר יתקרב אל האמת לתוספת בחלקנו אותו לכפל השרש בלי תוספת אחד |
|
|
| But, if the remainder is less than the [approximate] root it is vice versa. | ואולם אם השארית פחות מהשרש יהיה להפך |
|
[154]המשל אם בקשנו שרש כ"ט |
|
הנה השלמים אשר יצאו בשרש הם ה' ונשארו ד' ואם חלקנום לכפל השרש בלי תוספת שהוא י' יצאו ד' עשיריות |
|
וריחוקו מן האמת לתוספת הוא ככפל זה היוצא בעצמו שהוא י"ו עשיריות עשירית ר"ל י"ו חלקים מק' שבשלם |
|
ואם חלקנום בתוספת א' שהוא |
|
ויתרחק מן האמת בכפל ו |
|
ואולם הראשונים לא היו אפי' שישית אחת והקש על זה |
|
והטעם כי זה יחסר רחוקו מן האמת מרביעיתו ככפל מרחקו מחצי בעצמו |
|
וזה יחסר רחוקו מן האמת ככפל רחוקו מחצי בעצמו וככפל זה הריחוק פעמים בזה השרש המתוסף |
|
המשל במשלנו הקודם כי כאשר יעשה [בתוספת א' המשל שחלקנו הד' על י"א ויצאו ד'י"א הנה][155] י"א הנה יתרחק מן האמת ככפל אלו הד' בז' כנזכר ויחסר מרביע ככפל חלק אחד וחצי שהוא מרחקו מחצי הי"א בעצמו שהוא מרובע מרחקו שהוא ב' ורביע |
|
ואולם כאשר חלקנוהו מבלי תוספת המשל על י' הנה עלה בחלוק ד' עשיריות ויתרחק מן האמת ככפלו בעצמו שהוא פחות מרביע כמרובע מרחקו מחצי שהוא האחד וכפל זה האחד בכפל זה השרש שעולה הכל ט' |
| Although the excesses are not equal A single handful does not satisfy a lion [Talmud, Berakhot 3, 2]. | ועם היות שאין הריחוקים שוים ולא החלקים מ"מ אין הקומץ משביע את הארי[note 33] |
| I do not feel to be more precise, because I have been precise enough at this place. | ולא חששתי לדקדק יותר כי די באשר דקדקתי בזה המקום |
| We already stated that the one who wants to repeat the procedure does not need to add one [to double the approximate root], even if the remainder is greater than the root, because by repeating [the procedure] he comes as closer to the truth as possible. He should not be confused in the procedure by adding one, but always apply it without addition, and he should not speculate, only to take the square of the fraction resulting at that phase, divide it by double the [approximate] root, then subtract the quotient from its [approximate] root and so on. Because, I instructed to add one just when the remainder is as the [approximate] root or greater, only for the one who settles for one time. But the one who wants to come very closer and repeat the procedure should not add [one to double the approximate root] and not get confused. | [156]ועוד שכבר אמרנו שהרוצה להכפיל המעשים שאין לו צורך להוסיף אחד אף אם יהיה השארית גדול מהשרש כי בהכפל יתקרב אל האמת בכל מאויו ולא יתבלבל במעשיו בתוספת אחד אבל לעולם יעשה בלי תוספת ואין לו לעיין כי אם לקחת מרובע השברים היוצאים בחלוק בפעם ההיא ולחלקו לכפל השרש והיוצא יחסרהו משרשו וכן לעולם כי לא ציויתי להוסיף אחד כאשר השארית כשרש או יותר אלא למסתפק בפעם אחת |
[= He should use this approximation  instead of the previous approximation instead of the previous approximation  , even if b≥a, in order to avoid confusion] , even if b≥a, in order to avoid confusion]
|
|
| Another approximation:
|
|
|
ואם תרצה להתקרב אל האמת ברגע במעט עמל חבר הנשאר למרובע כפל השרש שבידיך וחלק עליו כפל הנשאר בכפל השרש |
| The second approximation:
|
|
|
ואם תרצה להתקרב יותר אל האמת קח מעוקב הנשאר הנזכר מהמורה כפול |
|
ר"ל שאם רצינו לדעת שרש |
|
והיה כפל השרש מחובר עם הנשאר היה הכל ששה והנשאר בתחלה היו שתים |
|
תקח מעוקב השנים שהוא שמונה ותקרא לו שם משישית שישית ר"ל ח' ששמה שישית וזה יהיה הנשאר במרובע על כפל השרש האחרון |
|
ותעשה ממנו עם זה השרש האחרון כמו שעשית לשארית הראשון עם השרש הראשון ויעלה כל השרש א' שלם וקי"ב חלקים מקנ"ג בשלם |
|
שמרובעו הוא ג' שלימים חסר ב' חלקים ממרובע קנ"ג בשלם |
| See, indeed, see [Samuel 1 24, 12], you have come closer to the truth, so that between the square of your root and the required square there is only one of a thousand and this is enough. | וראה גם ראה[note 34] גם נתקרבת אל האמת שאין ממרובע השרשך למרובע הנשאל אחד מרבבה בשלם ודי |
Section Two: Fractions |
[157]החלק השני בשברים | ||||||||||
Introduction |
|||||||||||
| Before the chapters, I will open with an introduction that consist of three chapters: | לפני הפרקים אקדים הקדמה אחת ובה שלשה פרקים | ||||||||||
|
השער הא' בפריטה | ||||||||||
|
השער הב' בהכאה | ||||||||||
|
השער השלישי בהשואה | ||||||||||
Chapter One on Decomposing to a Fraction |
השער הראשון בפריטה | ||||||||||
| Definition: Decomposing to a fraction is converting the integers to fractions of whichever type you wish. | הפריטה היא חזרת השלימים לחלקים מהמין אשר תרצה | ||||||||||
|
ואם יש בידיך שלמים ושברים להשיב הכל ממין השברי' ההם | ||||||||||
|
וכן אם יש לך שברים ושברי שברים כמו שיהיו להשיב כלם מהמין הקטן מהם | ||||||||||
| Example for integers and fractions:
|
המשל שלימים בשברים | ||||||||||
|
אם היו בידיך ג' שלמים וה' שביעיות | ||||||||||
|
הנה השילימים ישובו שביעיות שהוא מין שברים שעמו בהכפל אלו השלשה שלימים במורה השביעיות שהוא הז' ויעלו כ"א ובחברך אליהם הה' שביעיות אשר עמהם יהיו הכל כ"ו | ||||||||||
| All this is seen clear and its reason is explained in the examination of the [divisors] as clarified in chapter four - this is the rule and the reason. | וכל זה תראה ברור ומפורש בטעם בבחינת המתחלק למורים כמו שנתבאר בפרק הד' והוא הדין והוא הטעם | ||||||||||
| If you have fractions and fractions of fractions, multiply the fractions by the denominator of the fractions of fractions, then add to them the fractions of fractions.
|
כי אם אין בידיך כי אם שברים ושברי שברים שתכפול השברים במורה השברי שברים | ||||||||||
| I will give one example for all this: | ואביא משל א' לכל זה | ||||||||||
|
המשל אם היו לך ג' שלימים וב' רביעיות חמישית וד' שמיניות רביעית חמישית כזה | ||||||||||
| |||||||||||
|
נשיב ראשונה הג' שלמים לחמישיות והוא בכפלנו אותם בה' שהוא המורה עליהם וזה כי כל שלם הוא ה' חמישיות ויהיה ט"ו חמישיות | ||||||||||
|
ואם היה תחתיו מספר היינו מחברים אותו עליהם שהיו [158]ג"כ חמישיות | ||||||||||
|
אכן אחר אשר לא נמצא שם נשיבם עוד רביעיות חמישית [שהוא המורה הב' וזה שנכפלם בד' כי כל חמישית שלמה היא ד' רביעיות החמישית ויעלו ס' רביעיות חמישית][159] ונחבר אליהם השנים אשר תחתיו שהם ג"כ מזה המין ר"ל רביעיות חמישית ויעלו ס' רביעיות חמישית ונחבר אליהם הב' אשר תחתיו שהם ג"כ מזה המין ר"ל רביעיות חמישית יעלה הכל ס"ב | ||||||||||
|
נשיבם שמיניות רביעיות חמישית וזה בשנכפלם בח' יעלה תצ"ו נחבר להם הד' אשר תחתיו שהם ממינם יעלה הכל ת"ק |
|
נשיבם תשיעיות שמינית רביעית חמישית וזה בשנכפלם בט' יעלו 4500 ואחרי שלא נמצא תחתיו דבר לא נחבר אליהם דבר |
|
אבל נשיבם שלישיות תשיעית שמינית רביעית חמישית והוא שנכפלם בג' יעלו 13500 נחבר אליהם הב' אשר תחתיו שהוא ממינם יעלה הכל 13502 וכלינו כל מלאכתנו |
|
ואם לא היה שם שלמים כלל |
|
היה לנו להתחיל מהב' אשר תחת המורה הראשון אשר תחתיו מספר מה ואם הוא שני לחשבון המורים והיה לנו לכפלם בח' שהוא המורה הסמוך ויעלו י"ו ולחבר להם הד' אשר תחתיו ויעלו כ' |
|
|
|
|
ונכפלם עוד בט' יהיו ק"פ נכפלם עוד בג' יעלו 540 נחבר להם הב' אשר תחתיו ויעלה הכל 542 |
| Thus, we have everything explained by the procedure and by reason - how everything is converted into the final type, whether there are integers with fractions, or there are no integers there; the result is of the final type. | הרי לנו הכל מפורש במעשה ובטעם איך ישוב הכל מהמין האחרון בין אם יש שלמים עם שברים בין אם אין שם שלמים והיוצא באחרונה הם מהמין האחרון |
| I.e. for the result in our mentioned example are thirds of a ninth of an eighth of a quarter of a fifth. | ר"ל כי אלו אשר יצאו לנו במשלנו הנזכר הם שלישיות תשיעית שמינית רביעית חמישית |
Chapter Two on Multiplication [= compound fractions] |
השער השני בהכאה | |||||||||||||||||||
| Definition: The multiplication [= compound fractions] is when the fractions are not [fractions] of one integer, or of one fraction, but they are [fractions] of a number of integers or a number of fractions. | ההכאה היא כאשר השברים אינם שברים [160]משלם אחד או משבר אחד אבל הם ממספר שלמים או ממספר שברים | |||||||||||||||||||
|
ר"ל כאומרנו שתי חמישיות משלש רביעיות מה' שלמים כזה | |||||||||||||||||||
| ||||||||||||||||||||
|
והנה אומרנו שני חמישיו' מג' רביעיות מה' שלמים הוא כאומרנו שלקחנו ה' שלמים ועשינו מהם ד' חלקי' שוים ולקחנו הג' מהם שזהו ג' רביעיות מה' שלמים וחלקנו עוד אלו הג' חלקים לה' חלקים שוים ולקחנו הב' מהם שזהו פי' ב' חמשיות מג' רביעיות מה' שלמים | |||||||||||||||||||
| The fractions here are of one type only, therefore, there is no need for decomposing to a fraction at all. | ואין כאן שברים כי אם ממין אחד ואינך צריך לעשות פריטה כלל | |||||||||||||||||||
|
|
||||||||||||||||||||
| Yet, there is a need for multiplication. | אבל אתה צריך לעשות הכאה | |||||||||||||||||||
|
והוא כי אומרנו ב' חמישיות מג' רביעיות הרי הוא כאומרנו ב' חמישיות רביעית וב' חמישיות רביעית וב' חמישיות רביעית ולזה נכה הב' בג' שהוא מספר הרביעיות יעלו ו' הנה ידענו שהב' חמישיות מג' רביעיות הם ו' חמישיות רביעיות והוא ברור במעשה ובטעם | |||||||||||||||||||
|
|
||||||||||||||||||||
|
ולפי שאמרנו מה' שלמים הוא כאלו יש לנו בידינו ו' חמישיות רביעית משלם וכן עד ה' פעמים לכן נכה הו' שהוא מספר השברים אשר בידינו בה' שהוא מספר השלמים שהוא כמספר הפעמים אשר ישנך בידינו ויעלו ל' הרי לנו שהב' חמישיו' מג' רביעיות מה' שלמים הם ל' חמישיות רביעית והקש על זה | |||||||||||||||||||
|
|
||||||||||||||||||||
| Sometimes the fractions and fractions of fractions are of a number of fractions or integers and for this you should apply both operations i.e. decomposing to a fraction and multiplication. | ולפעמים יהיה כמספר שברים ושברי שברים משבר אחת גם ממספר שברים או שלמים ולזה תצטרך לעשות שני | |||||||||||||||||||
|
המשל שני רביעיות וג' חמישיות רביעית מג' שביעיות שמינית וד' חמישיות שביעית שמינית מג' [161]תשיעיות עשירית מד' שלמים | |||||||||||||||||||
|
תעשה הצורה כזה | |||||||||||||||||||
| ||||||||||||||||||||
|
ועשה הפריטה לכל אחד מהם תחלה | |||||||||||||||||||
|
ונעשה פריטה לג' שביעיות שמינית שהן נקשרות | |||||||||||||||||||
|
|
||||||||||||||||||||
|
עוד נעשה פריטה לב' רביעיות וג' | |||||||||||||||||||
|
|
||||||||||||||||||||
|
הנה שאלתנו הראשונה הוא כאלו אמרו שיש בידינו י"ג חמישיות רביעית מי"ט חמישיות שביעית שמינית מג' תשיעיות עשירית הד' שלמים כזה | |||||||||||||||||||
| ||||||||||||||||||||
|
והנה ביאור שאלתנו הוא כאלו אמר |
|
ולקחנו [חלק אחד מהם ועשינו אותו ט' חלקים |
|
ולקחנו][162] ג' חלקים מאלו הט' האחרונים ביחד ועשינו ח' חלקים שוים |
|
ולקחנו חלק אחד מהם ועשינו אותו ז' חלקים וחלקנו כל חלק מהם לה' |
|
ולקחנו י"ט חלקים ממין אלו האחרונים ביחד ועשינו אותם ד' חלקים שוים וחלקנו כל חלק מהם לה' חלקים |
|
ויש לנו ממין אלו החלקים האחרונים י"ג ונרצה לידע מה המה אלה |
|
והננו צריכים להבנה לפי שאמרו מי"ט חמישיות וכו' גם לאומרם מג' תשיעיות וכו' גם לאומרם מד' שלמים כי בזה ידענו שאינם משבר אחד אף לא משלם אחד כי מספר שלמים וממספר |
|
[163]לכן נכה מספר השברים אשר בידינו במספר השברים אשר הזכירו גם במספר השלמים זה אחר זה |
|
וזה כי אומרנו י"ג חמישיות רביעיות י"ט חמישיות וכו' הוא כאומרנו י"ט פעמי' י"ג חמישיות [רביעית חמישית][164] וכו' |
|
לכן נכפול הי"ג בי"ט ויעלו 247 חמישיות רביעיות חמישית וכו' |
|
|
|
|
גם כאשר אמרו לנו מג' תשיעיות הוא כאלו אמרו לנו ג' פעמים כל אשר בידינו |
|
[ולזה נכפול כל אשר בידינו][165] שהוא 247 בג' ויעלה 741 והם חמישיות רביעיות חמישיות שביעיות שמיניות תשיעי |
|
ולפי שאמרו לנו מד' שלמים הוא כאלו אמרו לנו ד' פעמים כל אשר בידינו |
|
לכן כל אשר בידינו שהוא 741 בארבעה ויעלה 2964 חמישיות רביעית חמישית שביעית שמינית תשיעית עשירית |
| Always remember that the number you find on top of another number is the denominator, not the bottom [number]. | וזכור לעולם כי המספר אשר תמצא על ראשו מספר אחר שהתחתון איננו מורה כי העליון |
| If we want to know how many integers, or fractions, or fractions of fractions are these decomposed fractions: | ואם בקשנו לידע כלם אלו החלקים הנפרטו' כמה שלמים או כמה שברים או שברי שברים מאלו הם |
| You already know that there are seven denominators. Arrange them as you wish, according to their order now, or calculatedly, in order that the fractions will be more proper. For the order is harmless, except in addition and subtraction, as we explain in chapter 4. | כבר ידעת שיש כאן שבעה מורים ותושיבם כרצונך או כסדרם עתה או בהשגחה כדי שיצאו החלקים יותר נאותים כי הסדר לא יזיק לעולם כי אם התוספת בהם או המגרעת כאשר ביארנו בפרק הד' |
|
ונחלק עליהם 2964 שהוא מספר אשר בידינו נפרטות וקראנו לזה כלילת יופי |
|
ואחר שיש לחשבון רביעית נשימהו לאחרון כדי שיתבטל ונחלקם על ד' ויצא בחילוק 741 ולא ישאר דבר |
|
וזה היוצא בחלוק אין לו אחד מהמורים הנשארים לכן נשים אשר נספק לפני האחרון אשר שמנו ויהיה ה' ונחלקם על הה' ויצא בחלוק 148 וישאר א' ונשימנו תחתיו |
|
ואחר שיש לו החשבון [166]רביעית נתיך המורה השמינית שהוא הח' ונעשה ממנו ב'ד' ונשימם במקומו כך הוא הוראת חצי רביעית או רביעית חצי [כמו][167] |
|
ונחלקם לאשר נחפוץ ויהיה על הז' ויצא בחילוק ה' וישארו ב' ונשימם תחתיו |
|
ונחלק הה' שיצאו בחלוק למורה הה' הא' ויצא א' בחלוק ולא ישאר דבר |
|
ואחר שאשר יצא בחלוק הוא פחות מהקטן שבכל המורים הנזכרים אין לנו לחלק עוד אבל נשימם על הסדר לפני המושמים בכל השגחה ונשים זה האחד אשר יצא בחילוק באחרונה תחת המורה הסמוך למושמים עד הנה |
|
והנה יצא לנו מבוקשינו והוא שהנשאל לנו תחלה עולה חצי תשיעית עשירית ושתי שביעיות חמישית חצי תשיעית עשירית וחמישית רביעית שביעית וכו' |
| |||||||||||||
|
ואל תתמה שלא היו לך כי אם ז' מורים ועתה הם ח' כי זה היה להתכת המורה השמינית והוא הח' שהסרנו אותו מהם ושמנו במקומו שני מורים והם ב'ד' | ||||||||||||
| Apply this, because the entire procedure and the reason are clear. | והקש על זה כי הכל ברור המעשה והטעם |
Chapter Three on the Expansion to a Common Denominator |
השער השלישי בהשואה | ||||||||||||||
| Definition: The expansion to a common denominator is when you have fractions of various types that are not related to each other at all, i.e. the type of these fractions is not the type of fractions of the others. | ההשואה היא כאשר יהיו לך שברים ממינים שונים בלתי נקשרים זה בזה כלל ר"ל שאין אלו שברי שברים אלו | ||||||||||||||
|
המשל אם היו בידיך שני שלמים | ||||||||||||||
| |||||||||||||||
|
ונעשה תחלה פריטה לב' שלמים וג' שמיניות וב' רביעיות שמינית | ||||||||||||||
|
ונתחיל לומר ב' אחדים כמה שמיניות הם וזה יודע בהכפלם בח' יהיו י"ו ונחבר להם הג' אשר תחתיו יהיו כלם י"ט | ||||||||||||||
|
עוד נשיבם רביעיות שמיני' וזה יהיה בהכפלם כלם בד' יעלו ע"ו ונחבר להם הב' אשר תחתיו יעלו ע"ח רביעיות שמינית | ||||||||||||||
|
עוד נפרוט הו' שביעיות וג' שמיניות שביעית ונאמרו ו' שביעיות שלמות כמה | ||||||||||||||
|
והרי הוא כאלו שאלו לנו להשיב למין אחד | ||||||||||||||
| |||||||||||||||
|
ואחרי היות בידינו מורים משונים ושברים משונים ראוי לנו לבאר איך נשיבם כלם ממין אחד מבלתי שינוי ביניהם ר"ל שיהיו כלם שברים ממורים אחדים | ||||||||||||||
|
וקודם זה אציע שסדור המורים אינו מעלה ומוריד | ||||||||||||||
|
כי כך הוא שביעית שמינית עד"מ כמו שמינית שביעית כי כל אחד מהם הוא חלקנו מה' בשלם שהוא המספר אשר הוא מורכב מאלו המורים וזה ברור | ||||||||||||||
|
לכן כאשר היה לנו עד"מ ג' שביעיות וד' שמינית | ||||||||||||||
|
נשיבם כלם שביעיות שמינית שהוא שמיניות שביעית | ||||||||||||||
|
וזה יעשה בכפול הג' שברי השביעיות בח' ויהיו כ"ד שמיניות שביעיות | ||||||||||||||
|
וזה ברור כי כל שביעיות הוא ח' שמיניות שביעית כמו שכל שלם [169]הוא שמונה שמיניות השלם | ||||||||||||||
|
וכן נעשה לד' שמיניות שנשיבם לשביעיות שמינית והוא בכפול הד' שהוא מספר השברים בז' שהוא מורה השביעיות ויעלו כ"ח | ||||||||||||||
|
והם כ"ח שביעיות שמינית והאחרות עלו כ"ד שמיניות שביעית הנה כלם ממין אחד כמו שהזכרנו שאין חלוף בין אומרנו שביעית שמינית לאומרנו שמינית שביעית | ||||||||||||||
| After explaining this premise, we return to our first procedure, which is multiplying each numerator of the fractions that we have by the denominators of the others successively, thus each fraction will be of all the denominators, so they are of the same type, for the order of the denominators forward or backward does not matter.
|
ואחר שהצענו הצעה זו נשוב למעשינו הראשון והוא לכפול כל מספר שברים אשר בידינו במורי חברותיה זה אחר זה וכן לכלם ואז תהיה כל אחד שברים מכל המורים והנה הם שוים כי סדור המורים בקדימה ואיחור לא יזיק | ||||||||||||||
|
ונתחיל במעשינו ונאמר 78 רביעיות שמינית כאשר נכפלם בה' שהוא מורה החמישיות יעלו 390 חמישיות רביעית שמינית | ||||||||||||||
|
עוד נכפול זה המחובר בז' שהוא מורה השביעית ויעלו 2730 שביעיות חמישיות רביעית שמינית | ||||||||||||||
|
עוד נכפול כל זה בח' שהוא המורה השמיניות ויעלו 21840 שמיניות שביעיות חמישיות רביעיות שמיניות וזהו העולה מה78 רביעיות שמיניות | ||||||||||||||
|
עוד נכפול הד' שהוא ד' חמישיות בכל מורי חברותיה זה אחר זה ונאמר ד בח' הם [ל"ב][170] שמיניות חמשית | ||||||||||||||
|
עוד נכפלם בד' יהיו 128 רביעיות שמיניות חמישית | ||||||||||||||
|
ונכפלם בז' יהיה 896 שביעיות רביעית שמינית חמישית | ||||||||||||||
|
עוד נכפלם בח' | ||||||||||||||
|
עוד נכפול הנ"א שהם נ"א שמיניות שביעית בה' יעלו 255 | ||||||||||||||
|
נכפלם בד' יעלו 1020 רביעית חמישיות שמיניות שביעית | ||||||||||||||
|
עוד נכפלם בח' יעלו 8160 [171]שמיניות רביעיות חמישית שמינית שביעית | ||||||||||||||
|
הרי כלם ממין אחד כי המורים שוים כי הסדר אינו מעלה ומוריד כאשר ביארנו | ||||||||||||||
| Beware lest you make a mistake when doing this expansion to a common denominator, not to add what is beneath the denominators to the product of the numerators by the denominators, for this is done only when decomposing to a fraction, when we want to sum up all the mentioned related fractions and to decompose them to the lowest fraction. | והשמר לך מאד פן תטעה בעשותך השואה זו לחבר לעולה מכפל השברים במורים מה שנמצא תחת המורי' כי זה לא יעשה כי אם בפריטה לבד שאנו רוצים לחבר כל השברים הנזכרים הנקשרים ולפרטם למין הפרוטות | ||||||||||||||
|
המשל במי שיש לו פרחים וזהובים ופרוטות שרוצה להשיב הפרוטות שיש לו או להשיב הפרחים זהובים ר"ל לראות כמה זהובים יעלו ולחבר לעולה הזהובים אשר היו בידו ואחר כך להשי' כל הזהובים פרוטות ולחבר עמהם הפרוטות אשר בידו ויהיה אז הכל מחובר ונפרט | ||||||||||||||
| But, the expansion to a common denominator does not includes summing at all, but to convert all the fractions to same type of units, so one does not sum them at all and this is clear by reason. | אבל ההשואה אין בה חבור כלל כי אם לעשות כל שברים מהם ממין האחדים לכן לא יחברם כלל וזה מבואר בטעם | ||||||||||||||
| Therefore I gave them different names that indicate this matter by hint. | ולזה שמתי להם שמות שונים מורי' על הענין ברמז | ||||||||||||||
|
כי להחזרת השברים הבלתי נקשרות למין אחד בהכאת כל אחד מהם במורי חברותיה קראתי השואה שאין כונתינו חבור כלל כי אם ההשואה לבד | ||||||||||||||
|
ולהשבת השברים הנקשרים כלם יחד למין השברים הגרועים מהם קראתי פריטה | ||||||||||||||
|
לשתי כוונות | ||||||||||||||
|
האחת שהוא כפורט ועושה מהפרחים וזהובים ופרוטות פרוטות וכמשיב הכללים לפרטים | ||||||||||||||
|
והכונה השנית היא כי בשם זה יזכר שיש לו לקחת עמו הפרט והעוללות אשר ימצא תחת המורים | ||||||||||||||
| For every number that requires multiplication and decomposing to a fraction - the decomposing to a fraction should be applied first to the multiplicands, and then the multiplication is applied. | ובכל מספר שצריך הכאה עם הפריטה יעשה קודם הפריטה לבעלי ההכאה ואחר ההכאה | ||||||||||||||
| Therefore, wherever we mention and instruct to decompose to a fraction, we mean that it is followed by the multiplication if needed, or whatever is needed, if you have a number that consists of fractions that require multiplication and decomposing to a fraction. | לכן בכל מקום אשר נזכיר ונצוה לעשות פריטה רצוננו ואחריה ההכאה אם הוצרך איליה או אשר מהם יצטרך שאם יהיה לך מספר [172]מורכב מהשברים הצריכים הכאה ועם הצריכים פריטה | ||||||||||||||
|
המשל ג' רביעיות מב' חמישיות וג' רביעיות חמישיות מד' ששיות ושלישית ששית | ||||||||||||||
|
תשימם על הסדר כזה | ||||||||||||||
| |||||||||||||||
|
ותעשה פריטה לד' שביעיות ושלישית שישית והוא שתכפול הד' בג' ותחבר להם האחד אשר תחתיו ויעלו י"ג שלישיות ששית | ||||||||||||||
|
וכן תעשה לב' חמישיות וג' רביעיות חמישית ויעלו י"א רביעיות חמישית | ||||||||||||||
|
וישוב מספרך כאלו אמרו ג' רביעיות מי"ח רביעיות חמישית מי"ג שלישיות שישית כזה | ||||||||||||||
| |||||||||||||||
|
ואחר עשותך פריטה זו כנזכר תעשה ההכאה והוא | ||||||||||||||
|
ותתחיל להכות ולומר שלשה בי"א הם ל"ג ול"ג בי"ג הם 429 הרי עלו כל השברים הנשאלים 429 רביעית חמישית שלישית שישית כזה | ||||||||||||||
| |||||||
|
ותחלק אלו ה429 למורים אלו ר"ל לד' והיוצא לד' האחר והיוצא לה' וכן לכלם עד כלותם | ||||||
|
וכאשר ישאר דבר בשום חלוקה מהן תשימהו תחת המורה ההוא | ||||||
|
וככלות החשבון קודם כלות המורים ויצא לך בחלוק על אחד מהן פחות מהמורה אשר לפניו תשים אותו היוצא תחת המורה הזה אשר לפני ואז תדע כמה | ||||||
| This is called the most beautiful [arrangement] as mentioned above, because it is to convert the particular to general, so that the fractions become greater and nicer. | וזה נקרא כלילת יופי [173]כמו שנזכר למעלה לפי שהוא לעשות מהפרטים כללים יען יהיו השברים יותר גדולים ויותר יפים | ||||||
| The real beauty is see first if the dividend has any of these divisors and write it last [to the right], then once again with the quotient, and on the third time and so on. | והיופי האמיתי כשתעיין בתחלה המספר המתחלק אם יש לו שום אחד מהמורים ההם ואותו תשים אחרון וכן בשנית ביוצא וכן בשלישית וכן לעולם | ||||||
| Do this only if you are asked how much are the remaining fractions, for if you did it for the purpose of expansion to a common denominator or for the purpose of [the operations discussed in] one of the next chapters, do not divide it by the denominators at all. Because I write it here only to teach you the procedure, although it is not its place and it is mentioned in other places. | ולא תעשה זה כי אם כאשר ישאלו לך כמה עולים חלקים אלו הנשארות אכן אם עשית זה לצורך ההשואה או לצורך אחד מהשערים הבאים לא תחלקהו על המורים כלל כי לא כתבתיו כאן כי אם ללמדך על המעשה ואם אין זה מקומו ונזכר כבר במקומות אחרים | ||||||
|
ובעשותך זה בדמיוננו זה ר"ל שתחלק ה429 על הד' שהוא המורה האחרון יצא בחילוק 107 וישאר א' ותשימהו תחתיו | ||||||
|
[ותחלק זה היוצא לד' הקודם לו יצא בחלוק כ"ו וישארו ג' תשימם תחתיו | ||||||
|
ותחלק זה היוצא לה' ויצא בחלוק ה' וישאר א' ותשימהו תחתיו | ||||||
|
ותחלק][174] ותחלק ה' אלו על הג' ויצא א' וישארו ב' ותשימם תחתיו | ||||||
|
וזה הא' אם היה גדול מהו' | ||||||
|
אכן לפי שהוא פחות ממנו נשימם תחתיו מיד ויצא לנו מזה שהשברים הנשארים עלו ששית א' שלמה וב' שלישיות ששית וחמישית שלישית שישית וג' רביעיות חמישית שלישית שישית ורביעית רביעית חמישית שלישית שישית |
|
ועל דרך היופי ר"ל לשים המורים בסדר בהשגחה יצאו החלקים כפי הצורה השנית והכל עולה לסך אחד |
| To make it easier for you, when you expande to a common denominator, if you find any denominator that appears the same number of times in all the numbers [to be expanded], i.e. for instance, that 8 is in each of them once, or twice, or three times, do not multiply by this denominator at all. When you write all the denominators, write it only as many times as it apears in one of the numbers.
|
וכדי להקל מעליך כאשר תעשה ההשואה אם תמצא |
| If it is in all of them, but not the same number of times - for instance, once in this one and twice or three times in the other - where it is found the maximal number of times, do not multiply by this denominator at all; as for the rest of the numbers, multiply each by this denominator, as many times as the excess of the maximal number of times over the number of times it is found in the present number. When you write the denominator, write it only as many times as the maximal number of times that it apears.
|
ואם הוא בכלם אבל אינו בהם פעמים שוות אבל בזה פעם אחת ובזה שנים או שלשה ע'ד'מ' אשר ישנו שם פעמים לא תכפלנו במורה זה [כלל וכל אחד משאר המספרים תכפלנו במורה זה][176] כ"כ פעמים כפעמים שהוא יותר כמספר הרב הפעמים שבמספר הזה הנכפל בו עתה ובהשימך המורה לא תשימנו כי אם כפעמים אשר הוא באשר הוא יותר פעמים |
| If it is not in all of them, but in two or three of them, multiply each of the numbers, in which it is not found at all, by this denominator, as the maximal number of times that it apears in one of them. Do not multiply the number, in which it is found the maximal number of times, by it. [As for the rest of] the numbers, in which it is found, multiply each by it, as many times as the excess of the maximal number of times over the number of times it is found in the present multiplicand.
|
ואם אינו בכלן כי אם בשנים או בג' מהם המספרי' אשר אינו בהם כלל תכפול כל אחד מהם במורה זה כמספר הפעמים אשר הוא באשר הוא יותר פעמים והמספר אשר הוא בו יותר פעמים לא תכפלנו כלל והמספרים אשר ישנו בהם תכפול כל אחד בו כמספר הפעמים העודפים באשר הוא היותר פעמים מבזה הנכפל |
| If it is found in them the same number of times, do not multiply by it any of the numbers, in which it is found, and when you write the denominators, write it only as many times as the maximal number of times that it apears. | ואם הוא בהם פעמים שוות לא תכפול בו שום אחד מהמספרים אשר הוא בו ובהשימך המורים לא תשימנו כי אם כפעמים אשר הוא באשר הוא יותר רב פעמים |
|
ויצא מזה כי במשל ההשואה שעשינו בתחלת שער זה לא היה לנו לכפול הע"ח רביעיות שמינית בח' כלל גם לא השמיניות שביעית להיותו בשניהם בשוה |
|
גם לא היה לנו לשום הח' כי אם פעם אחת כאשר הוא באחד מהאחרים |
|
ובמורים לא היה לנו לשום הח' כי אם פעם אחת כפעמים אשר ישנו באחד מהם |
| It does not matter if all this is not done, but this will make the procedure more difficult. | וכל זה אינו מזיק אם לא יעשה אבל כי תכבד העבודה |
Chapter One: Addition |
הפרק האחד |
| In it the discussion on [conversion] and summing. | בחיבור ובו מאמ' האמרה והאחדות |
| The procedure: | |
| When you wish to add fractions with integers or fractions with fractions of another type, first, decompose each of the numbers that requires decomposing to [the lowest type of] fraction by itself, then multiply what needs to be multiplied, and after you decompose [and multiply] whichever requires either both decomposing and multiplication, or one of them, expand the numbers to one type, sum up all their results together, i.e. the numerators, then we divide [the sum] by all the denominators of all the fractions. | כאשר תרצה לחבר שברים עם שלמים ושברים [או][178] עם שברים ממין אחר בתחלה תפרוט כל אחד מהמספרים לבדו אשר יצטרך פריטה גם תכה הצריך להכאה ואחר שתפרוט וכל אחד מהם הצריך להם או לאחד מהם ר"ל לפריטה או להכאה תשוה המספרים אחד אל אחד עד שיהיו כלם ממין אחד והעולה בכל אחד מהם חבר הכל יחד ר"ל מספר השברים וחלקנו על כל המורים אשר לכל |
|
כי ע'ד'מ' אם במשלנו אשר עשינו בהשואה בתחלת השער הג' שאלו לך שתחברם ותאמ' כמה הם |
|
היה לך לעשות כל אשר עשינו הפריטה לכל אחד וההשואה לכלם עד שיגיעו לאשר הגיעו |
|
והוא שהשנים השלמים וג' שמיניות וב' רביעיות שמינית עלו ל21840 [שמיניות שביעית חמשית רביעית שמינית |
|
והד' חמשיות עלו ל8160][179] מכל המורים |
|
ואחר עשותך כל זה היה לך לחבר יחד כל מספרי השברים ר"ל ה21840 עם ה7168 ועם ה8160 ויעלו 37168 והם מהה' מורים הנזכרים ר"ל ה8 וה4 והה' והז' והח' שהם כל מורי המספרים הראשונים |
|
ותשימם על הסדר כאשר תרצה או בהשגחה כאשר הזכרנו בפרק הרביעי כדי שיצאו החלקים יותר נאותים ושם תמצאנו מבואר באר הטב | ||||||||||
|
ולהיות לזה החשבון 37168 המתחלק שמינית שהוא אחד מהמורים נשימנו אחרון ר"ל הראשון | ||||||||||
|
ונחלק חשבונננו זה עליו ר"ל 7 ויצא בחילוק 663 [נ' 3][180] וישארו ה' ונשימם תחתיו | ||||||||||
|
ונחלקם על הד' ויצא בחילוק ל"ג ולא ישאר דבר | ||||||||||
|
ונחלקם אלו הל"ג היוצאים בחילוק על הח' שהוא המורה הנשאר ויצא בחלוק | ||||||||||
| |||||||||||
|
הנה עלה בידינו שכאשר חברנו השנים שלמים וג' שמיניות וב' רביעיות שמינית עם ד' חמישיות ועם ו' שביעיות וג' שמיניות שביעית שעלה הכל ד' שלמים ושמינית אחת וג' רביעיות חמישית שמינית וה' שביעיות רביעית חמישית שמינית והקש על זה והה' |
| This is the reason that if you are told [to sum] numerous numbers, you need to multiply first, before the expansion [to a common denominator], then the expansion and afterwards the summing, as mentioned. | והוא הטעם אם אמרו לך מספרים רבים והיו בהם שצריכין | ||||||||||
|
ואולם אם לא שאלו לך בסתם כמה הם | ||||||||||
|
אבל אמרו לך ע'ד'מ' כמה חמישיות | ||||||||||
|
הם אחר שזה הה' הוא במורים אינך צריך לעשות פועל חדש כי אם שתשים הה' הראשון מהמורים כזה | ||||||||||
| |||||||||||
|
ויעלה בידך ד' שלמים כ"ה שמיניות חמישית חמישית וג' רביעיות שמינית חמישית |
|
אכן אם אמרו לך להחזירם ממין אחר שאינו במורי' |
|
המשל שאמרו לך כמה תשיעיות הן |
|
זה יקרא מאמר ההמרה והוא שאחרי עשותך הפריטה וההכאה וההשואה קודם שתחלקם למורים הנזכרים תכפול כל חשבון השברים ר"ל ה37168 בזה המורה אשר רצו להחליפם אליו ר"ל הט' שהוא המורה התשיעית ויעלו 334512 ונשים הט' למורה ראשון וכל המורים האחרי' אחריו אם כאשר יזדמן אם בהשגחה |
|
ובחלקנו ראשונה לח' ויצא בחילוק 41814 ולא ישאר דבר |
|
ונחלק זה היוצא לד' ויצא בחילוק 10453 וישארו ב' ונשימם תחתיו |
|
|
|
|
ונחלקנו [182]לז' ויצא בחילוק 298 וישארו ד' ונשימם תחתיו |
|
ונחלק זה היוצא על הח' ויצא בחילוק ל"ז וישארו ב' ונשימם תחתיו |
|
ונחלק זה היוצא על הט' ויצא בחילוק ד' והם שלמים וישאר א' ונשימהו תחתיו |
|
והנה המרנו החלקים ר"ל השברים לתשיעית וחלקי תשיעית |
| The rule of this category [i.e. conversion] is that whenever you are asked with regard to a known type of fractions, whether various or not, to convert them to another type, whether a fraction or a fraction of a fraction: | כלל זה מאמר זה הוא שכאשר ישאלו לך על חלקי' ידועים שונים ובלתי שונים שתמירם למין אחר בין אם יאמרו לך לשבר או לשבר שבר | |||||||||||||||||||||
|
כמו שיאמרו לך השיבם לחמישיות שביעית שמינית או הדומה לזה | |||||||||||||||||||||
| You should first decompose, multiply, and expand the fractions, if they are various, then sum them together and multiply the sum by the denominator or the denominators, to which you need to convert them. | יש לך לעשות תחלה פריטה והכאה והשואה לשברים אם היו שונים ושוב תחברם יחד ושוב תכפלם כלם ביחד על המורה או המורים אשר רוצים שתמירם אליהם | |||||||||||||||||||||
|
ר"ל שאם אמרו לך שתמירם לחמישיות תכפלם בה' לבד | |||||||||||||||||||||
|
ואם אמרו לך לחמישיות שביעית שמינית תכפלם בח' והעולה בז' והעולה בה' | |||||||||||||||||||||
| After you do all that, write the denominator or denominators of the first conversion to the right by the required order: first the 8, after it the 7, after it the 5, then arrange after them the denominators that you have randomly of calculatedly and divide by them the number that you get from the multiplication of the numerators by the denominator or denominators of the conversion. All this is clear by reason. | ואחר עשותך כל זה תשים מורה או מורה ההמרה ראשונה לצד ימין על הסדר שנשאל הח' תחלה ואחריו הז' ואחריו הה' ושוב תסדר אחריהם מורה שבידך כפי המזדמן או בהשגחה ותחלק על כלם המספר אשר עלה לידיך מכפל מספר שבריך במורה או מורי ההמרה וכל זה ברור בטעם | |||||||||||||||||||||
| For, if you multiply what you have by given denominators, the product always have these denominators in addition to its original denominators. Therefore, when you wish to reduce them, i.e. to convert these decomposed fractions to proper integers and fractions, you have to arrange these denominators, by whice they were multiplied, with their original denominators and the order does not matter. Since it is asked how many fractions of these denominators they are, we write them first in this procedure. | כי לעולם אם תכפול אשר בידך במורים מונחים הנה יהיה למקובץ מורים אלו מוספים על מוריו הראשונים ולכן כאשר תרצה לעשות להם כלילת יופי ר"ל להשיב שברים אלו הנפרטות לכללים וחלקים יפים יש לך לסדר עם מוריו הראשונים אלו המורים אשר הוכפלו בהם והסדר לא יזיק ולפי ששאלו כמה חלקים הם מהמורים האלו לכן נשימם ראשונה במלאכה | |||||||||||||||||||||
| If you are told to convert them to another fraction as greater as possible, it is called ha-Aḥdut [lit. unification] and it is an important issue, because by it we can divide the smaller by the greater and to generate denominators without extracting the denominators of the number by which we want to divide or to add to its denominators. | אכן אם יאמרו לך להשיבם לחלק אחר הגדול שאיפשר לכן נקרא | |||||||||||||||||||||
| For this I assigned it a discussion of its own and I write it in this chapter as it is a kind of addition. | לזה הקצתי לו מאמר לבדו ואכתבנו בזה הפרק לפי שהוא כעין חבור | |||||||||||||||||||||
| I named it "ha-Aḥdut" [lit. unification], since we want to convert them to one fraction whether it is possible or not. | וקראתי לו שם שם האחדות לפי שאנו רוצים לעשותם חלק אחד אם איפשר ואם הוא בלתי איפשר | |||||||||||||||||||||
| If it is impossible, we have to add one in the procedure as will be explained. | ואם הוא בלתי איפשר יש לנו להוסיף אחד במלאכה כאש' יתבאר | |||||||||||||||||||||
| For these two reasons I called it "ha-aḥdut". | לב' כוונות אלו קראתי לו שם האחדות | |||||||||||||||||||||
Summing fractions to one fraction |
מאמר האחדות | |||||||||||||||||||||
| If you wish to convert fractions, equal or different, into one fraction if possible, or as greatest as possible. | אם רצית להשיב שברים | |||||||||||||||||||||
|
המשל שני חמישיות מב' תשיעיות מב' שלמים ועוד שמינית אחת ושני תשיעיות שביעית שמינית מרביעית ושתי ששיות רביעית | |||||||||||||||||||||
|
תשימם על הסדר כזה | |||||||||||||||||||||
| ||||||||||||||||||||||
| Apply on them [the operations of] decomposition, multiplication, expansion [to a common denominator], and summation. | תעשה להם פריטה והכאה והשוואה וחיבור | |||||||||||||||||||||
| In order to train you more in the procedure I will perform them one by one: | וכדי להרגילך עוד במעשה אעשה אחת אחת | |||||||||||||||||||||
|
נעשה פריטה לרביעית ושתי שישיות רביעית נכפול א' בו' יהיו ו' ונחבר להם הפרט אשר נמצא תחתיו ר"ל הב' יעלו ח' שישיות רביעית | |||||||||||||||||||||
|
עוד נעשה פריטה לשביעית וב' תשיעיות שביעית שמינית נכפול א' בז' נכפלם עוד בט' יהיו ס"ג ונחבר להם השנים ויעלו ס"ה תשיעיות שביעיות שמינית | |||||||||||||||||||||
|
|
||||||||||||||||||||||
|
והרי הוא כאלו אמרו ס"ה תשיעיות שביעית שמינית מח' שישיות רביעית כזה | |||||||||||||||||||||
| ||||||||||||||||||||||
|
עוד נעשה הכאה לשני מספרי' שבידינו | |||||||||||||||||||||
|
ונתחיל במספ' הראשון ונאמ ב' בב' הם ד' נכפלם עוד בשני השלמים יהיו ח' חמישיות תשיעית שלימה [184]כזה | |||||||||||||||||||||
|
|
||||||||||||||||||||||
| ||||||||||||||||||||||
|
עוד נכה במספר השני השמונה ששיות רביעית בס"ה ויעלו 520 תשיעיות שביעית שמינית שישית רביעית כזה | |||||||||||||||||||||
| ||||||||||||||||||||||
|
ונעשה ההשואה לאלו השני מספרים |
|
ואחר היות בכל אחת מהם מורה הט' פעם אחת לא נכפול בו שום אחד מהמספרים ולא נסדרהו כי אם פעם אחת כאשר הזכרתי בסוף השער הג' |
|
ונכפול הח' חמישיות תשיעיות בכל מורה המספר האחר זולתי הט' כאשר התבאר ונאמר שמונה בד' יעלה ל"ב נכפלם בו' יעלו 192 נכפלם בח' יעלו 1536 נכפלם בז' יעלו 10752 |
|
עוד נשוב לכפול ה520 שהם מספ' השברים האחרים בה' שהוא מורה חבריהם ולא בט' כנזכר ויעלו 2600 | |||
|
נסדרם זה על זה כזה | |||
|
|
ונחברם יחד יעלו 13352 |
|
נסדר כל המורים ר"ל כל מורי שני המספרי' בלתי הט' שלא נשימנו כי אם פעם אחת ונשים מספרינו תחת המורה האחרון לפי שהוא שברים נפרטות מכל אלו המורים |
|
והרי זה כאלו שאלו לנו 13352 שביעיות שמינית שישית רביעית חמישית תשיעית איזה חלק הם אם הם חלק אחד ממש או החלק הגדול שאפשר |
| Definition of the common denominator: first we examine which number has all these denominators alone, i.e. that consists of them and we call this number "the common denominator" [lit. the mother of the denominators], for it gave tham birth and they came out from it. | נעיין תחלה איזהו המספר שהוא בעל אלו המורים כלם לבדם ר"ל שהוא מורכב מהם ונקרא למספר הזה אם המורים כי היא ילדתם וממנה יצאו |
| This is known by multiplying all the denominators one by the other and the product by another and so on until they end. | וזה יודע בכפול כל המורים אחד באחד והעולה באחר וכן כלם עד כלותם |
|
ונאמ' ט' בה' יעלו מ"ה נכפלם בד' יעלו [180 נכפלם בו' יעלו 1080 נכפלם בח' יעלו][185] 8640 נכפלם בז' יעלו 60480 |
| Dividing the common denominator by the summed numerator | ||
|
|
||
|
ולזאת קרינו אם המורים למספר השברים ר"ל שנחלק ה68480 ל13352 ואם יתחלק כלו לשלימים בלי תוספת [186]ומגרעת הנה היוצא בחילוק בצמצום הוא מורה החלק אשר הם כל השברים הנשאלים יחד מהשלם ר"ל רביעית אחד או הדומ' לו | |
| ואם לא יתחלק כלו לשלמים בלי תוספת ומגרעת הנה היוצא בחלוק בצמצום הוא מורה החלק אשר הם כל השברים הנשאלים יחד מהשלם ר"ל רביעית אחת או הדומה לו | ||
|
ואם לא יתחלק כלו לשלמים וישאר שום מספר | |
|
כמשלינו זה שיצא בחילוק ד' ונשאר 7072 | |
|
נוסיף א' על היוצא בחילוק ויהיה ה' והוא מורה החלק הגדול שאפשר ר"ל חמשית אחת | |
|
עוד נחסר ה7072 הנשארים מה13352 אשר חלקנו עליו וישאר 6280 שהוא חלקים מכל המורים מזה החלק ר"ל מחמישית אחת | |
|
ר"ל שיצא לנו שכל השברים הנשאלים הם חמישית אחת ו6280 שביעיות שמינית שישית רביעית חמישית תשיעית חמישית כזה |
| |||||||||||||||
| The order of the denominators of compound fractions of fractions is unimportant, but the denominator of the simple fraction should be placed separately, on the right: | |||||||||||||||
| If you want to reduce these fractions, i.e. to divide them by the denominators, arrange them as they are now, or randomly, or calculatedly as mentioned above, provided that you write the 5 first to the right with the 1 beneath it, because you cannot change this, and all the others are related to it, i.e. all of them are fractions and fractions of fractions of it, i.e. of a fifth of the whole. | [ואם תרצה לעשות לשברים אלו כלילת יופי ר"ל לחלקם על המורים תסדרם][187] תסדרם כפי שהם עתה או כפי המזדמן או בהשגחה כנזכר למעלה ובלבד שתניח הה' ראשון לצד ימין עם הא' אשר תחתיו כי זה אין בידיך לשנותו וכל האחרים נקשרים בו ר"ל שהם כלם שברים ושברי שברים ממנו ר"ל מחמשית מהשלם | ||||||||||||||
|
ונחלקם תחלה לח' ויצא בחלוק 785 ולא ישאר דבר | ||||||||||||||
|
ונחלק זה היוצא לה' ויצא בחילוק 157 ולא ישאר דבר | ||||||||||||||
|
ונחלקם לד' ויצא בחילוק ל"ט וישאר א' ונשימנו תחתיו | ||||||||||||||
|
ונחלקם ל | ||||||||||||||
|
ונתיך הט' ר"ל שנעשה ממנו ב' מורים שהם [189]ג' ג' כי כך הוא שלישית שלישית כמו תשיעית ועוד אדבר בזה בכלל האחרון ב"ה י"ת | ||||||||||||||
|
ונחלק הו' אשר יצאו בחלוק באחרונה על האחד מהם ר"ל על הג' ויצא בחילוק ב' ולא ישאר דבר | ||||||||||||||
|
ואלו הב' אחר שהוא מספר קטן משאר המורים אין לנו עוד לחלקם רק להשימם תחת המורה הסמוך אשר נשים לפניהם ויהיו הג' השני כדי שלא ישכח ונשימם תחתיו | ||||||||||||||
|
ונסדר עוד | ||||||||||||||
|
הנה יצא לנו שהשברים הנשאלים יעלו חמשית א' שלמה וב' שלישיות שביעית חמישית וג' ששיות שלישית שלישית שביעית חמישית ורביעית שישית שלישית שלישית שביעית חמישית כזה |
| |||||||||||||
| Deduce from that | והקש על זה | ||||||||||||
Reason |
|||||||||||||
| The reason we say that if there is no remainder, the result of the division is itself the denominator of the fractions of the whole, is because our saying "these portions of the denominators" is as our saying "the portions of their common denominator in the whole". | וטעם אומרנו שאם לא ישאר דבר שהיוצא בחילוק בעצמו הוא מורה החלק אשר השברים מהשלם הוא לפי שאמרנו אלו החלקים מאלו המורים הוא כאלו אמרנו כ"כ מחלקי אם המורים בשלם | ||||||||||||
|
|
|||||||||||||
|
ר"ל כי ע'ד'מ' אם היו לנו ב' שלישיות רביעית | ||||||||||||
|
הוא כאומרנו שני חלקים מי"ב בשלם שהיא אם אלו המורים ר"ל שהוא מורכב מהם שכפל ג' בד' עולה י"ב | ||||||||||||
|
וכן אומרנו ג' רביעיות חצי שלישית | ||||||||||||
|
היא כאומרנו ג' חלקים מכ"ד בשלם שהוא אם שלש מורים אלו וזה ברור | ||||||||||||
| It clear from chapter four of the first section. | ועוד נחבר בפ' הרביעי מהחלק הא' | ||||||||||||
|
הנה ידענו שאלו השברים הם חלקים מחלקי האם בשלם | ||||||||||||
|
ואם הם | ||||||||||||
|
ואם רביעיתם רביעית | ||||||||||||
|
ואם כמותם הם א' שלם | ||||||||||||
|
|||||||||||||
|
ואם היה רביעית יצאו ד' | ||||||||||||
| We receive that the result of division indicates the portion that the numerator is of the whole and the reason for this is clear, when all is divided with no remainder. | הרי לנו שהיוצא בחילוק הוא המורה החלק אשר השברים מהשלם וזה ברור בטעם כאשר נתחלק הכל ולא נשאר דבר | ||||||||||||
| To clarify the reason of our saying that when there is a remainder, we add one to the result etc. I shall bring another example:
|
ולברר טעם אומרנו שכאשר נשאר שם דבר שנוסיף א' על היוצא | ||||||||||||
|
המשל היו בידינו ג' רביעיות שביעית ר"ל שלשה חלקים מכ"ח שהוא אם המורים בשלם | ||||||||||||
|
ואם נחלק אלו הכ"ח אל הג' יצאו ט' בחילוק וישאר א' | ||||||||||||
|
נוסיף א' על הט' היוצא בחילוק יעלה עשרה המורה על העשירית | ||||||||||||
|
ואם החלקים הראשונים היו ג' חלקים מל' באחד היו עשירית אחד בצמצום כי בחלקנו הל' בשלשה היו יוצאים ולא היה נשאר דבר ואז היו עשירית שלמה כמו שביארנו | ||||||||||||
|
אכן להיותם ג' חלקים מכ"ח בשלם יותר מעשירית אחת ולדעת כמה הם יותר נכפול הכ"ח בל' ויעלו 840 | ||||||||||||
|
והנה אומרנו חלק אחד מ840 בשלם הוא כאומרנו חלק אחד מל' מכ"ח בשלם או חלק אחד מכ"ח מל' בשלם כי הם המורים אשר מהם הורכב וכל זה נתבאר הטב בפרק הד' מהחלק הא' | ||||||||||||
|
וא"כ הל' חלקים מה840 בשלם הם חלק אחד מכ"ח בשלם | ||||||||||||
|
וכן הכ"ח חלקים מ840 בשלם הם חלק אחד מל' בשלם | ||||||||||||
|
הרי לנו שהחלק אחד מל' בשלם הוא כ"ח חלקים מ840 בשלם | ||||||||||||
|
וכן החלק מכ"ח בשלם הוא ל' חלקים מ840 | ||||||||||||
|
נמצא שהג' חלקי' מכ"ח בשלם הוא ג' פעמים ל' שהם 90 חלקים מ840 בשלם | ||||||||||||
|
והג' חלקים מל' בשלם הם ג' פעמים הם כ"ח שהם פ"ד חלקים מ840 | ||||||||||||
|
הנה יעדפו עליהם ו' חלקים מ840 בשלם ר"ל ו' חלקים מל' מכ"ח בשלם כי הם מוריו | ||||||||||||
|
וכל ג' חלקים [192]מאלו הם עשירית הל' שהם ר"ל | ||||||||||||
|
א"כ כל שלשה מהם הם עשירית [חלק מכ"ח בשלם ר"ל חלק מכ"ח מעשירית בשלם שהוא][193] | ||||||||||||
|
|||||||||||||
|
והששה הנוספות אשר מצאנו לג' חלקים מכ"ח אשר היו בידינו על הג' חלקים מל' אשר מצאנו לג' חלקים היו עשירית שלמה יעלו א"כ ב' רביעיות שביעית עשירית | ||||||||||||
|
הרי לנו שכאשר חלקנו הכ"ח שהוא האם על הג' שהיו מספר החלקים ויצא ט' ונשאר א' שכאשר הוספנו אחד על הט' ועלה י' והורה עשירית שנשאר לנו לתוספת ב' רביעיות שביעית עשירית שהם התוספת אשר למספר אשר חלקנו עליו שהיה ג' על השארית שהיה א' ר"ל שאלו הב' הם חלקים מהמורים שהיו רביעית שמינית מהמורה שנתחדש שהוא עשירית | ||||||||||||
| All this is clear by reason to the one who understands and deduce from this. | וכל זה ברור בטעם למבין והקש על זה |
| Dividing a small number by a greater number - without divisors or with divisors | |
| We receive that the one who wants to divide a smaller by a greater can divide it with or without extraction of the divisors. | ויצא לנו מזה שהרוצה לחלק מעט על רב שיוכל לחלקו בלי הוצאת המורים או בהוצאת המורים |
| We also get the greatest part that can be pronounced by a single name. | ויצאו לנו ג"כ החלק היותר גדול שאיפשר בשם אחד |
| This is very helpful when we want to divide a prime number, such as 101, that has no divisors. | וזה יועיל מאד כאשר אנו רוצים לחלק למספר פשוט כמו ק"א או כדומה לו שאין לו מורים |
| In order to explain this matter well, I shall bring two examples - one with extraction of divisors and one without extraction of divisors: | וכדי לבאר הענין יפה יפה אביא שני משלים אחד עם הוצאת המורים ואחד מבלי הוצאת המורים |
|
המשל רצינו לחלק 73 על 240 |
|
והנה מוריו הם אלו ו' ח' ה' כי מהם |
|
ונחלק האם שהוא המספר הגדול אשר רצינו לחלק עליו על המספר הקטן ר"ל ה73 אשר הוא המספר אשר רצינו לחלק עליו על המספר [194]הקטן ר"ל ה73 אשר הוא המספר אשר רצינו לחלק ויצא בחילוק ג' וישארו כ"א |
|
נוסיף א' על היוצא יהיה ד' והוא המורה החלק גדול והוא רביעית אחת ונשימנו ראשונה ונשים תחתיו א' |
|
עוד נשים הכ"א הנותרים מהע"ג שהוא החשבון אשר חלקנו עליו עתה ישארו נ"ב והם מוסיפים על הרביעית ר"ל שהעולה שיצא לנו בחלוק ה73 המספר הקטן על ה240 שהוא המספר הגדול רביעית אחת |
|
או אם תרצה תקח מורה במקומו ותאמר רביעית אחת ונ"ב חמישיות שמינית שישית רביעית |
|
ואם תרצה תעשה להם כלילת יופי ויעלו רביעית אחת וחמישית רביעית וד' שמיניות שישית חמישית רביעית והקש על זה |
| I shall bring another example, in which there are no divisors. | ועוד אעשה משל אחר מאשר אין לו מורים כלל |
| I will illustrate there that we can apply our procedure repeatedly time after time, until the number ends and until reaching to a simple fraction [whose numerator is 1]. | ושם אאריך שאנו יכולים לעשות מעשינו זה פעם אחר פעם עד כלות המספר והגיעו לחלק אחד |
| I call it also Aḥdut [= unification], as it brings all to one. | כי גם לזה קראתיו אחדות כי יגיעם כלם לאחד |
| Even between all the denominators and the last denominator we can insert a new denominator as we wish. | ואפי' בין כל המורים למורה האחרון נוכל להכניס מורה חדש ככל חפצנו |
|
המשל לחלק ל"ח לק"א כי זה המספר ר"ל ק"א |
|
ונחלק הק"א לל"ח [ויצאו בחלוק ב' וישארו כ"ה |
|
נוסיף א' על הב' יהיו ג' ונשימהו למורה ראשון ונשים תחתיו א' |
|
ונגרע השארית מהל"ח אשר][195] אשר חלקנו עליו עתה וישארו י"ג |
|
ואם לא היו כ"כ הינו שמים למורה שני הק"א והינו שמים זה השארית ר"ל אלו הי"ג תחתיו והינו אומרים שהמחלק ל"ח על ק"א שיגיע לכל אחד מהם שלישית אחת וי"ג חלקים מק"א משלישית שלמה |
|
אכן להיותם הרבה וכדי שנמצא חלקים יותר נאותות נשוב לחלק הק"א לאלו הי"ג ויצא בחילוק ז' וישאר י' |
|
ונשים זה הז' בתוספת אחד והוא ח' למורה שני ונשים תחתיו א' ונשים הי' שהם השארית [196]מהי"ג אשר חלקנו עליהם עתה וישארו ג' |
|
ואם תרצה כבר כלית כל מלאכתך ותשים הק"א למורה שלישי |
|
אכן אם תרצה עוד להכפל המעשיך יען תגיע לאחדות גמורה ר"ל שלא יהיו שם מנין שברים כי אם אחד אחד תשוב תחלק הק"א על אלו הג' ויצא בחילוק ל"ג וישארו ב' |
|
ונוסיף א' על הל"ג ויהיו ל"ד ונשימם למורה שלשי ונשים א' תחתיו |
|
ונחסר אלו הב' הנשארים מהג' אשר חלקנו עליהם עתה וישאר א' וכבר הגענו לאחדות הגמור וכלינו מלאכתנו מכל וכל |
|
ונשים ק"א למורה [רביעי] ונשים א' תחתיו |
| You have three forms, all of which are true. You can switch to whichever of them you want. You are allowed to do it differently, when you divide the common denominator, as in the first example. We write all the denominators that are generated, time after time, one after the other, one instead of the other. | הרי לך שלש צורות שכלם אמיתיות ותוכל להשיב כאשר תרצה מהם וכן היה הרשות בידך לעשות זה פעם אחר [כאשר היתה מחלק אם המורים כבמשל הא' ונשים כל המורים המתחדשים פעם אחר פעם זה][197] פעם זה אחר זה כ"א תחת כל אחד |
| The term Aḥdut [= unification] is useful for all this, so that you will not forgat to write 1 beneath each generated denominator, then write the common denominator itself, or its divisors instead of it, and 1 beneath the last, if you have reached the complete unification, and if not, write beneath it what remains last, after you have subtracted the remainder from the number, by which you divide at that last time. Write that last rem,ainder beneath the last divisor of the common denominator. | כי לכל זה יועיל שם האחדות שלא תשכח מלשים א' תחת כל מורה מתחדש ואחר תשים האם עצמה או מוריה במקומה ותחת האחרון א' אם הגעת לאחדות הגמורה ואם אין תשים תחתיו הנשאר באחרונה אחרי הסירך הנשאר מהמספר אשר אתה מחלק עליו בעת ההיא באחרונה [.]השארית האחרונה ההיא תשים תחת המורה האחרון אשר לאם |
| If it is greater than it, reduce them, I mean, divide that remainder by the last denominator and write the remainder beneath it and so on until it is complete. All this is clear and repeated many times. | ואם יהיה רב ממנו תעשה מהם כלילת יופי רצוני לומר לחלק השארית הא' |
| For every other number that you divide into divisors, if you see that what you wrote beneath the last denominator is a large number and you want to set between the former denominators and the last one to the left a new denominator or denominators, divide the last denominator by what is beneath it, as you have done in the second and third examples. | וגם בכל מספר אחר אשר חלקת הכל למורים אם תראה שאשר שמת תחת המורה האחרון הוא מספר רב ותרצה להמציא בין כל המורים הראשונים זה האחרון אשר לצד שמאל משום מורה מחודש או מורים חלק [198]המורה האחרון על אשר תחתיו כאשר עשית במה שבין הצורה השנית והשלישית |
|
שהרי הק"א היה המורה האחרון בצורה הראשונה ולפי שמצאת הי"ג שהם מספר רב תחתיו המצאת המורה הח' ששמת שני והוא שלישי בידך שבא בצורה השנית |
|
וכן עשית פעם אחת מהצורה השנית לשלישית והמצאת מורה אחר והוא הל"א ושמת הק"א רביעי |
| Provided that you do it only with the last denominator to the left. | ובלבד שלא תעשה זה כי אם למורה האחרון אשר לצד שמאל |
| All this is clear to the one who understands by the first reason. | וכל זה מבורר בטעם הראשון למבין |
| If you want to extract denominators between the middle denominators and the last denominator to the right, you have to extract [the common denominator] of all [the denominators], then this common denominator is divided by the numerators that are beneath those denominators, after they were decomposed, if it is divisible by them. All this is clear by reason. | ואם תרצה להוציא המורים בין המורים האמצעיים תצטרך להוציא המורים לכל המורה האחרון אשר לצד שמאל והאם ההיא תחלק למנין השברים אשר היו תחת |
| Because, after you have extracted the common denominator of these denominators, they are all become as one denominator and you seek between the formers and the [last] another denominator or denominators, and after you have set the denominators that you want, you write this common denominator after them to the left, or the denominators, of which it is composed, one after the other, instead of it, as it is all the same. | כי אחר שהוצאת האם למורים האם הרי שבו כלם כמורה אחד ואתה מבקש בין הראשונים ובינו מורה או מורים אחרים ואחר שהמצאת המורים אשר רצית תשים האם הזאת אחריהם לצד שמאל או המורים אשר הורכבה מהם זה אחר זה במקומה כי הכל אחד |
| This is enough for the one who understands. | ודי למבין |
Check |
|
| If you want to check your practice, decompose all the fractions you have received. | ואם תרצה לבחון מעשיך עשה פריטה לכל אלו השברים אשר באו לך |
| If the large number, by which you wanted to divide, is among your denominators, i.e. 101 in the last example, divide the resulting decomposed numerator by all other denominators except for it one after the other, or by their common denominator, what you receive should be as the small number that you wanted to divide [originally]. [If] nothing remains in any of these divisions, your procedure was true and correct; if not, know that you were wrong. | ואם יש במוריך אלו המספר הגדול אשר רצית לחלק עליו ר"ל הק"א במשל האחרון חלק זה העולה מהשברים הנפרטים על כל שאר המורים מבלעדיו זה אחר זה או על אמם ויצא לך באחרונה כמנין המספר הקטן אשר רצית לחלק ולא נשאר דבר בשום חלוקה מאלו הנה מעשיך אמת ונכון ואם לאו דע שטעית |
| If the original denominators, or the original divisor, are among you denominators, in the first example also, divide all the decomposed numerators by the rest of the denominators that were generated in the unification operation, or by their common denominator. If nothing remains and the result is as the small number that you wanted to divide [originally], or as the decomposed fractions in the example at the beginning of the discussion, [the procedure was] true and correct; if not, know that you were wrong. | [199]גם במשל הראשון אם יש במוריך אלו הם המורים הראשונים או המורים עצמם חלק כל מספר השברים הנפרטות על שאר המורים שנתחדשו במלאכת האחדות או על אמם ואם לא ישאר לעולם דבר ויצא באחרונה כמספר הקטן אש' רצית לחלק או כשברים הנפרטים במשל ראש המאמר הנה אמת הנה נכון ואם לאו דע שטעית |
| If the common denominator or the original divisor are not in your procedure, i.e. among the denominators, multiply the resulting decomposed numerator by the large number, by which you wanted to divide - whether by the common denominator of the required fractions as in the first example at the beginning of this discussion, or by the large number, by which you wanted to divide as in the second example - we divide the product by all the denominators or by their common denominator, and if the result is as the required decomposed numerator in the first example, or as the small number that you wanted to divide in the second example without any remainder, [the procedure] is true; if not, it is wrong. | ואם אין במלאכתך זאת ר"ל במוריך לא אם המורים ולא המורים עצמם כפול כל המספר השברים הנפרטים בחשבון הגדול אשר רצית לחלק עליו אם באם המורים מהחלקים הנשאלים כבמשל הראשון אשר בראש זה המאמר אם במספר הגדול אשר רצית לחלק עליו כבמשל השני והעולה חלקנו לכל מוריך אלו או לאמם ואם יצא כמספר השברים הנפרטים הנשאלים במשל הראשון או כמספר הקטן אשר רצית לחלק במשל השני מבלי שארית כלל הנה אמת ואם לאו שקר |
| Before I start with the reason of this check, in order to train you in the procedure, I will check each one of the three mentioned examples: | וקודם התחילי בטעם בחינה זאת כדי להרגילך במעשה אעשה בחינה בכל אחד משלשת המשלים הנזכרים |
|
הנה פריטת המשל הראשון |
|
היה 1 3 7 7 7 ב |
|
ואם לא היו בידינו כל המורים הראשונים היו כופלים זה בכל המורים הראשונים והעולה היינו מחלקים אל כל שמונת מורים אלו אחד אחד אחד או לאמם והיא יוצא מספר פריטת השברים הנשאלים והיא 13352 |
|
אכן אחרי היות בידינו כל המורים הראשונים ר"ל כל מורה השברים [200]הנשאלים ואל יטעך שאין כאן הט' שהרי במקומו ג' ג' שהם מוריו ונחלוק זה אשר עלה לנו מפריטתינו זאת ר"ל ה66760 למורים שנתחדשו במלאכתינו ר"ל לה' הראשון לבדו כי לא נתחדשו עוד ויצא בחילוק 13352 שהוא מספר פריטת השברים הנשאלים ולא נשאר דבר והנה אמת |
|
ובמשל השני |
|
הוא הפריטה א' בה' ה' וא' ו' ו' בו' ל"ו בח' 288 ו4 292 |
|
נחלקם לד' שהוא המורה המתחדש יצא בחילוק מבלי שארית 73 שהוא המספר הקטן שרצינו לחלק והנה אמת |
|
ובמשל השלישי |
|
בצורה הראשונה הנה הפריטה עולה 114 נחלקם לג' שהוא המורה המתחדש יצאו הל"ח שהוא המספר הקטן אשר רצינו לחלק |
|
ובצורה השנית הפריטה 912 נחלקם לג' ולח' שהם המורים החדשי' תחלה לג' יצא 304 נחלקם לח' ויצאו הל"ח |
|
ובצורה השלישית הפריטה 31008 נחלקם לג' יצא 10336 נחלקם לח' יצא 1292 נחלקם לל"ד שהוא המורה הנשאר מהמורים החדשים יצא הל"ח והנה אמת |
| Reason: check | |
|---|---|
| The reason of this check is clear because when the original denominators, or the common denominator, or the great number, by which we wanted to divide, are among our denominators, decomposing means the numerator of [the fraction that consists of] all the original as well as the new denominators and this is clear as was explained many times. | וטעם בחינה זה הוא ברור כי כשיש במורינו המורי' הראשונים או האם |
| Since decomposing is to convert them to the lowest fraction, which is the last type and it is related to all the denominators. | כי הפריטה הוא להשיבם פרוטות |
| When we divide it by the new denominators it is as reducing, for the order does not matter. | וכאשר נחלקם על המורים המתחדשים הוא כעושה כלילת יופי כי הסדר לא יזיק |
| After it is divided by all the new [denominators] and nothing remains, it is reduced and the result is a numerator of the new denominators, or their common denominator, or of the large number. | ואחר שנתחלק על כל החדשים ולא נשאר [201]דבר הנה יצאו מן הכלל והיוצא באחרונה הם שברים מהמורים הראשונים או מאמם כבראשונה או מהמספר הגדול |
|
ר"ל שהל"ח שיצאו לנו אחר שחלקנו הפריטה במורים החדשי' ויצאו הם מן הכלל הם חלקים מק"א חלקים בשלם כי לכל אחד מהל"ח יעלה לכל אחד חלק אחד מק"א בשלם ומהל"ח ל"ח |
| The reason for our saying: "if the original denominators, or their common denominator, or the greatest number are not among our denominators, we multiply the decomposed by the original denominators, or by their common denominator, or by the greatest number and divide by all the denominators, so that the result is without a remainder as the required decomposed fractions in the first example, or as the smaller number in the second number" - is that the decomposed fraction consists of all these denominators and when we multiply it by the original denominators, or by their common denominator, or by the greatest number, we decompose it further to fractions of fractions of the original [denominators]. | וטעם אומרנו שאם אין המורים הראשונים או אמם או המספר הגדול במורינו שנכפול הפריטה במורים הראשונים או באמם או במספר הגדול ונחלקנו בכל המורים שיצא מבלי שארית כמספר פריטת השברים הנשאלים במשל הראשון או כמספר הקטן במשל השני הוא לפי שהפריטה היא שברים מכל אלו המורים וכאשר אנו כופלים אותה במורים הראשונים או באמם או במספר הגדול הוא שאנו פורטים אותה עוד לשברי שברים מהראשונים |
|
ר"ל כי אם יש בידינו ג' רביעיות שמינית ע'ד'מ' |
|
אם נכפלם בז' היוצא שביעית רביעית שמינית וזה נתברר פעמים רבות |
| Therefore, in our procedure, the product [by the original denominators] is a numerator of [a fraction that consists of] all these [renewed] denominators that we have, as well as of the original denominators, or of their common denominator, or of the large number that we added [to the new denominators] just now. When we divide it by our denominators, i.e. [the renewed denominators] without the original denominators that we added now [to the new denominators], or without their common denominator, or without the large number, because we do not divide by them, the result of division should be a numerator of [a fraction that consists of] the original denominators, or of their common denominator, or of the large number. | והנה במעשינו היוצא אחר הכפל יהיו שברים מכל אלו המורים אשר לנו ומהראשונים או מאמם או מהמספר הגדול שהוספנו עליהם עתה וכאשר נחלקנו למורינו ר"ל מבלתי הראשונים אשר הוספנו עתה או מבלתי אמם או מבלתי המספר הגדול אשר הוספנו עתה כי להן לא נחלקם ישאר היוצא שברים מהמורים הראשונים או מאמם או מהמספר הגדול |
| If the result is as the decomposed numerator required in the first example, or as the small number in the second [example], it is restored to what it was in the beginning, so our calculation is correct. | והנה אם היוצא היה כמספר פריטת השברים הנשאלים במשל הראשון או כמספר הקטן בשני הנה שב כבתחלה והנה כל מעשינו אמת ויציב |
| Know that the prime number, i.e. if the large number [by which the small number is divided] is a prime number that has no divisors, as in the third example, which is 101, it cannot be absent or converted [after the unification procedure], and this is clear because it cannot be divided completely by another number without a remainder, since it is prime. | ודע כי [202]המספר הפשוט ר"ל אם היה המספר הגדול מספר פשוט שאין לו מורים כבמשל השלישי שהוא קי"א כי לעולם לא יעדר ולא יומר וזה ברור כי הוא לא יתחלק לשום מספר בשלימות מבלי שארית אחר שהוא פשוט |
Chapter Two: Subtraction |
הפרק השני בחסרון | |||||||||||||||
|
המשל אם אמרו לך שלש רביעיות ושתי חמישיות רביעית משתי תשיעיות חסרם משמונה תשיעיות ושלש שביעיות חמשית תשיעית מחמש ששיות מג' שלמים | |||||||||||||||
|
תשים הצורה הראשונה והוא המעט כזה | |||||||||||||||
| ||||||||||||||||
|
והצורה השנית והוא הרב תשים כזה | |||||||||||||||
| ||||||||||||||||
|
והנה המעט אחרי אשר הוכה ונפרט יעלה 34 חמישיות רביעית תשיעית כזה | |||||||||||||||
| ||||||||||||||||
|
והרב יעלה אחרי שנפרט והוכה | |||||||||||||||
| After multiplying and decomposing, we should expand them by multiplying the numerator of one by the denominators of the other, then both are of the same fractions.
|
ואחרי שהוכו ונפרטו יש לנו להשוותם וזה בכפול מספר שברי כל אחת במורי חברתה ואז היו כל אחת מהם שברים | |||||||||||||||
|
אכן להקל עלינו המעשה אחרי היות בש[בריהם] הט' והה' פעמים שוות והוא פעם אחת לא נכפול בהם שום אחת מהם כמו שנתבאר בסוף השער הג' וגם לא נסדרם | |||||||||||||||
|
והנה המעט אחרי הכפלו בו' ובז' זה אחר זה שהם מורי חברתה מזולת הט' והה' שלא נכפול בהם כנזכר יעלה 1828 ואחר שהוכה בו' ובז' [203]נתוספו לו מורים אלו על מוריו לכן יהיו אלו ה1428 שביעיות שישית חמישית רביעית תשיעית | |||||||||||||||
|
|
||||||||||||||||
|
ובכאן נתבאר הטעם למה אנו מסדרים כל המורים ולמה אין אנו מסדרי' הט' והה' פעם אחרת ואם הם בחברתה והוא לפי שלא נכפלו בהם וזה ברור | |||||||||||||||
|
והרב אחרי הכפלו בד' שהוא המורה הנשאר בחברתה שאינו בה יעלה 16980 ואחר שהוכה על הד' ונוסף גם הוא על מוריו יהיו רביעיות שביעית חמישית תשיעית ששית | |||||||||||||||
|
והנה שניהן שוות כי הסדר לא יזיק |
|
ונשים המספרים זה על זה ונחסרנו כמעשינו בשלמים ונשארו 15552 רביעיות שביעית חמשית תשיעית שישית ואם תרצה תעשה להם כלילת יופי ויעלה זה השארית ב' שלמים וב' שביעיות חמישית והקש על זה |
| This is the rule: we decompose and multiply both the greater [= the minuend] and the smaller [= the subtracted], or any of them that requires it, then we expand their [denominators], subtract [the numerator of] one from the other as the way of the integers and reduce the remainder that includes all the denominators that are included originally in each of them. | זה הכלל שנעשה לכל אחד מהמספרים הרב והמעט פריטה והכאה או אשר יצטרך מהם ואחר כך נעשה להם השוואה ואחר כך נחסרם זה מזה כדרכנו בשלמים והנשאר נעשה לו כלילת יופי והוא כל השברים ר"ל היו לאחת מהם עם אשר הוכתה בהם מאשר בחברתה |
Chapter Three: Multiplication |
הפרק השלישי בכפל |
| The operation [described] in this chapter is the same operation [described] in the second principle of compound fractions. | הנה מעשה זה הפרק הוא מעש' השער השני הנקרא שער ההכאה |
|
כי אמרנו כפול ג' רביעיות על ד' חמישיות ע'ד'מ' הוא כאומרנו ג' רביעיות מד' חמישיות |
| We multiply the numerator by the numerator, not by the denominators.
|
ונכפול מספר השברים במספר השברים לא במורים |
|
[ר"ל הג' על הד' יעלו י"ב והם שברים ממורי שני המספרים ר"ל][204] ר"ל שהן חמישיות רביעית |
| Therefore, there is no need in this chapter for expansion at all, because they are not two [different] types of fractions, but they are fractions that are related to each other, as we explained. | ולזה אין מבוא בזה השער להשואה כלל כי אינם שני מינים שברים אבל הם שברים [205]נקשרים זו בזו כמו שביארנו |
| In order to train you in the procedure I give an example: | וכדי להרגילך במעשה אביא משל אחד |
|
המשל רצינו לכפול ד' שביעיות מה' תשיעיות שמינית על ג' חמשיות תשיעית מב' שלישיות מה' שלמים |
|
אין לך לעשות דבר כי אם לקשרם יחד ולשים במקום על מ' ר"ל שתאמר הם ד' שביעיות מה' תשיעיות שמינית [מג'][206] חמישיות תשיעית מב' שלישיות מה' שלמים והרי לנו חזרו לשער ההכאה |
|
ואם תרצה לידע מה המה אלה עשה להם הכאה כי בזה המשל אין מבוא לפריטה והעולה נעשה לו כלילת יופי על כל המורים כי כלם נקשרים זה בזה ויעלה אחר ההכאה 600 שביעיות תשיעית שמינית חמישית תשיעית שלישית |
| Know that the denominators are always [written] on top and you cannot find anything above them. By this you distinguish between the denominators and the numerators. | ודע שלעולם המורים עליונים ולא תמצא עליהם דבר ובזה תבחין בין המורים למספר השברים |
| Although there is no proof to the matter, there is a reference to the matter [Mishnah, Sanhedrin 8, 2], to include Torah scholars [Talmud, Bekhorot 6, 2] - the denominators [morim, lit. teachers] should be high above all and the numerators are beneath them, as the student and his teacher, or a teacher's house that is wide open, so that whoever wishes comes. | ואם א[י]ן ראיה לדבר זכר לדבר[note 36] את לרבות תלמידי חכמים[note 37] שהמורים ראויין להיות גבוהים על הכל והשברים למטה מהם כתלמיד לפני רבו או בית פתוח לרוחה תחת המורה ויבא מי שירצה |
| But, the integers - there is nothing above or beneath them. Their house is not open, for they are not denominators [lit. teachers]. | אבל השלמים לעולם אין עליהם ולא תחתיהם דבר ולא בית פתוח כי אינם מורי הוראה |
|
ואחרי עשותנו להם כלילת יופי שהן חמש תשיעיות שביעית תשיעית |
| This is the rule: we should not expand to a common denominator at all. All that we should do is only to relate [the fractions] to each other, which is to write "of" [the letter מ] instead of "by", as we explained, then multiply and decompose if necessary, and after all this to reduce, which is to divide by the denominators that are always on top as we explained, and the result is the required. | זה הכלל שאין לנו לעשות בזה ההשואה כלל כי אין לנו לעשות כי אם לקשרם יחד והוא לשים מ' במקום על כמו שבארנו ואחר כן נעשה לה הכאה גם פריטה אם הוצרך אליה ואחר כל זה לעשות לה כלילת יופי והוא לחלקם על המורים שהרי העליונים לעולם כמו שביארנו והיוצא [207]הוא המבוקש |
Chapter Four: Division |
הפרק הרביעי בחלוק | ||||||||
|
רצינו לחלק שלש רביעיות וב' שלשיות רביעית על ד' תשיעיות וה' ששיות תשיעית מב' שלישיות | ||||||||
|
הנה צורת הרב היא כזה | ||||||||
| |||||||||
|
וצורת המעט כזה | ||||||||
| |||||||||
|
והרב אינו צריך כי אם פריטה ויעלה אחר הפריטה י"א שלישיות רביעיות | ||||||||
|
אכן המעט צריך פריטה והכאה ויעלה אחר הפריטה וההכאה נ"ח ששיות תשיעית שלישית | ||||||||
| After decomposing and multiplying each of them, if needed, we expand them to a common denominator. | ואחר שעשינו לכל אחד מהם אשר הוצרך מפריטה והכאה נשוום יחד | ||||||||
|
ואחרי היות הג' בשתיהן פעם אחת לא נכפלם בו | ||||||||
|
ויעלה הרב אחר ההשואה 594 | ||||||||
|
והמעט יעלה 232 | ||||||||
|
והם ר"ל שני המספרים האלו שברים מהד' מורים שהם שישיות תשיעית שלישית רביעית | ||||||||
|
ואחרי היותם שוות הרי הוא כאלו שאלו לנו שנחלק 594 שישיות תשיעיות שלישית רביעית והרי הוא כאלו אמרו לנו נחלק 594 שלמים על 232 | ||||||||
| For, since they are of the same type, what do I care if they are integer or fractions, or zuzim or peraḥim, after all this operation is exactly the same as division of integers. | כי אחר שהם ממין אחד מה לי אם הם שלמים או שברים או זוזים או פרחים והרי מעשהו שוה לחלוקת השלמים שוה בשוה |
| In order that we will receive fractions and integers together, we do not use the method of unifying, but the method of extracting the divisors, that is we extract the divisors of the number by which we want to divide and this is the small number in our example. | וכדי שיצאו לנו שברים ושלמים יחד לא נביאנו על דרך האחדות כי אם ע"ד הוצאת המורים והוא שנוציא מורי המספר אשר רצינו לחלק עליו והוא המספר המעט אשר במשלינו |
| However, do not be mistaken in thinking that its denominators that are above it are the divisors of the decomposed after the expansion and that these are the divisiors that you should seek for and divide by them, for this is not the case at all. You should divide by them only when you reduce. | ואל תטעה לחשוב כי מוריו אשר עליו |
| The divisors that you should seek for, means to know if the 232, which is the numerator of the number by which we want to divide the other numerator, is prime or composite, or of which numbers it consists. | אבל המורים אשר לך לבקש הוא לדעת ה232 שהוא מספר השברים אשר רצינו לחלק עליהם מספר השברים האחרים אם הוא פשוט או מורכב או מאי זה מספרים הוא מורכב |
|
ודע לך שאחר שכפלת והעולה בפריטתה בד' בעת ההשואה בידוע שיש לה רביעית וכן כל המורים אשר היו בחברתה ולא בה |
| Therefore, if you want to make the procedure easier for you, do not multiply by them and then you will not need to divide by them, when extracting the divisors. But, take them as denominators, by which you divide, with the resulting decomposed. Seek for the divisors of the resulting decomposed and write them with them. | לכן אם תרצה להקל מעליך המעשה לא תכפלנו בהם ולא תצטרך לחלקה עתה להם בעת הוצאת המורים אבל תקחם למורים שתחלק עליהם ועל היוצא מפריטתה ותבקש מורי המספר היוצא מפריטתה |
| All this was said about the number, by which you want to divide, but as for the number that you want to divide, you always need to multiply it by the denominators that are in the other [that were not among its own denominators]. | וכל זה אמרנו במספר אשר תרצה לחלק עליו |
|
המשל לזה במשלינו כי אם רצינו לבקש מורים ל232 ואחר שבעת ההשואה הוכפל היוצא מהפריטה וההכאה בד' שהיא מורה חברתה ידענו שלזה העולה יש לו רביעית ונחלקנו על ד' ויצא בחילוק נ"ח |
|
ונבקש עוד מורים לנ"ח ונמצא לו חצי ונחלקנו עליו ר"ל ר"ל על ב' ויצא בחילוק כ"ט והוא מספר פשוט |
|
הנה מורי מספר השברים אשר רצינו לחלק עליהם הם הב' והד' והכ"ט |
| In that you see clearly what I said that if we would have wanted to make the procedure easier for us, we would have taken the 4 as a first denominator from the start. | ובזה תראה ברור מה שאמרתי שאם הינו רוצים להקל המעשה מעלינו היינו לוקחים מתחלה |
|
וכן אם היה שם הרבה ולא היינו צריכים לכפול בהם המספר אשר רצינו לחלק עליו [209]ר"ל הנ"ח אבל נבקש מורים לנ"ח או לשים אותה עצמה למורה ולשים עמהם הד' והכל אחד |
| The reason for this is clear, because multiplication and division are inverse operations. | והטעם ברור כי הכפל והחלוקה הפכים הם |
|
|
|
|
ואם נכפול מספר ר"ל הנ"ח על מספר מה ר"ל הד' ונחלק העולה לזה המספר בעצמו ר"ל לד' יצא לנו אשר היה לנו בתחלה ר"ל הנ"ח |
| The operation is the same, but the procedure is easier. | והמעשה עולה אחד והמלאכת יותר קלה |
| As long as you do not make a mistake in multiplying the number that you want to divide, i.e. the 11, by the denominators of the other, because it is always required. | ובלבד שלא תטעה מלכפול המספר אשר רצית לחלק ר"ל הי"א במורי חברתה כי זה מחוייב לעולם |
|
ונשלים המשל ונחלק ה594 על הג' מורים שיצאו לנו זה אחר זה |
|
ונחלקם תחלה לג' ויצא בחילוק ג' והם שלמי' ולא ישאר דבר ונשימם מחוץ |
| [it is not clear why the divisors 4, 2, 29 were replaced here by 3, 2, 29:] | |
|
הנה היוצא הוא כי בחלקנו המספר הרב למעט הנזכרים במשל שיצא בחילוק ג' שלמים וכ"ד חלקים מכ"ט חלקים מחצי שלם |
|
ור"ל שהמספר המעט הוא ברב ג' פעמי' |
|
וזה ר"ל השלשה שלמים ואם יהיו שנים שלמים ירצה לומר שהוא בו שתי פעמים ואם יותר יותר |
|
והשברים ר"ל שהם עוד בו חלקי פעם כנזכר ולא היה פעם שלמה כלל |
| When the question is so, i.e. that we divide small fractions by numerous greater fractions, we can do this by the method of unification after we decompose, multiply, and expand [to a common denominator]. | וכאשר השאלה כן |
| This is the rule: after we decompose and multiply each of them, or whichever is needed, then expand them as mentioned, we divide the [numerator] resulting in this by the [numerator] resulting in that, as integers. All the resulting integers are the number of times [that the divisor appears in the dividend] and the fractions are the [additional] parts of one time [that the divisor appears in the dividend]. All is clear. | זה הכלל שאחר עשותנו הפריטה וההכאה |
| The proofs of all the preceding and the following chapters on fractions are the same as the proofs for integers, i.e. each to its the inverse operations: addition and subtraction to each other; division and multiplication to each other. The proofs of the proportions and roots [of fractions] are also the same as for integers. | ומופתי כל פרקי השברים העוברים והבאים הם כמופתי השלמים ר"ל כל דבר להפכו החבור והחסרון זה לזה והחלוק והכפל זה לזה גם בערכים ובשרשים מופתיהם [210]כמופתי השלמים |
Chapter Five: Proportions |
הפרק הה' בערכים | ||||||||||||||||||
| The proportion is as our saying: the ratio that these fractions have to known fractions - to whom these latter fractions have the same ratio, or who has this ratio to these latter fractions? | הערכים הוא כאומרנו הערך שיש לשברים אלו אצל שברים ידועים אצל מי יש לשברים אלו האחרים זה הערך או למי יש זה הערך אצל אלו השברים האחרים | ||||||||||||||||||
|
או אם אלו השברים מזהב ע'ד'מ' שוים אלו של כסף אחרות אלו של זהב כמה שוים [אלו][211] של כסף | ||||||||||||||||||
|
או אלו של כסף כמה שוים של זהב | ||||||||||||||||||
| All this is the same as with integers. | כל זהו כמו בשלימים | ||||||||||||||||||
| Its procedure: the rule requires that we decompose and multiply each of the three numbers separately; then multiply the first of these by the second of these without expansion at all; then expand what resulted now that does not have the denominators of the other, i.e. to multiply the mentioned product by the denominators of what remains, which is the first or the second and so on, meaning to multiply the third by the denominators of the two that are the denominators of the mentioned product; finally to divide the [numerator] of the product after it was expanded by the [numerator of the] third after it was expanded. | ומעשהו היה הדין נותן שנעשה פריטה והכאה לכל אחד מהג' מספרים לעצמו ולכפול ר"ל להכות הראשון מאלו [בב' מאלו][212] מבלי השואה כלל ויהיה היוצא חלקים ממורי שני מספרים אלו | ||||||||||||||||||
|
|
|||||||||||||||||||
| The integers resulting in the division are proper integers of the unknown and the fractions are fractions of the whole. | והיו השלמים היוצאים בחילוק שלמים ממש מהנעלם והשברים שבר שלם | ||||||||||||||||||
|
המשל אם ג' רביעיות מג' שלמים פחות רביע שלם שוים ד' חמישיות מה' שלימים פחות חומש שלם חמש שישיות מו' שלימים פחות שישית שלם כמה שוים | ||||||||||||||||||
|
נעשה לכל אחד צורה בפנים עצמה כזה | ||||||||||||||||||
| |||||||||||||||||||
|
וזה כי אומרו ג' רביעיות מג' שלימים פחות רביע שלם הוא כאומרו משני שלימי' וג' רביעיות שלם | ||||||||||||||||||
|
וכן מהה' פחות חומש הוא כמו מד' [213]שלמים וד' חמישיות משלם | ||||||||||||||||||
|
וכן מששה שלמים פחות שישית כאומרו מה' שלימים וה' שישיות שלם | ||||||||||||||||||
| After we have determined the proper names of the figures, we decompose and multiply each: | ואחרי ששם הצורות כתקנם נעשה לכל אחד פריטה והכאה | ||||||||||||||||||
|
ובצורה הראשונה נפרוט הב' שלימים ונכפלם במורה הרביעיות והוא ד' ויהיו ח' רביעיות | ||||||||||||||||||
|
ונחבר להם הג' אשר תחתיו שהם ג' רביעיות שלם יעלו י"א רביעיות שלם | ||||||||||||||||||
|
והרי הוא כאלו אמרו ג' רביעיות מי"א רביעיות לכן נכה הי"א בג' יעלו ל"ג הלא הם ל"ג רביעיות רביעית |
|
וכן נעש' לשנית ויעלו 96 |
|
וכן לראשונה מהאחרות ויעלו 175 שישיות שישית |
|
והנה שבא הכל כאלו שאלו לנו אם 33 רביעיות רביעית שוות 96 חמישיות 175 שישיות שישית כמה שוות |
|
או הערך אשר ל33 רביעיות רביעיות אצל [.] 96 חמישיות חמישית ל175 שישיות שישית אצל מי יש לו זה הערך |
|
והרי לנו כל השברים נפרטים ומורים כל אחד לבדו |
|
ויש לנו לכפול הב' בראשון ר"ל הו'ט' חמישיות חמישית 571 |
|
ויש לנו לחלקם לראשון מהאחדים שהוא ה33 רביעיות רביעית |
|
וכבר אמרנו בפ"ד מזה החלק שאם נרצה נשוה תחלה המתחלק ואשר נחלק עליו ר"ל שנכפול ה16800 אשר אנו רוצים לחלק במורה ה33 רביעיות רביעית ר"ל בד' ויעלה 67200 ונכפלם בד' המורה האחר ויעלה 268800 רביעיות רביעית חמישית שישית שישית |
|
ונכפול ג"כ ה33 רביעיות [214]רביעית והוא המספר אשר רצינו לחלק עליו במורי המספר המתחלק והם הו' והה' |
|
ואחר שנכפלם בזה זה אחר זה נבקש מורה כל העולה ונחלק עליהם המספר המתחלק ר"ל ה268800 |
|
ואם בקשנו לז' [לו][215] אלו המורים אשר נכפול בהם ר"ל הו' והה' ונחלק אליהם אחד אחד |
|
יצא בחילוק האחרון ל"ג שהוא המספר אשר כפלנו בהם אחד אחד ומאחר שכן למה ניגע לבהלה לכפול בהם ולחלק העולה עליהם לבטלה | |||||||||||||
|
לכן לא נכפול המספר אשר רצינו לחלק עליו ר"ל ה33 במורי המספר המתחלק אבל נקח המורים ההם למורים ראשונים ונשים עמהם ה33 עצמו | |||||||||||||
|
או נרצה נבקש לו מורים ויהיו י"א ג' ונשימם במקומו עם המורים הנזכרים ר"ל מורי המספר אשר רצינו לחלק ויהיו כלם 6 6 5 5 11 3 | |||||||||||||
|
ונחלק עליהם המספר המתחלק והוא 268800 וזה לעשות להם כלילת יופי | |||||||||||||
|
כי אם רצינו יכולנו לו' שהנעלם מהארבעה הנערכים הוא 268800 שלישיות חמישית ששית מאחד עשר בשלם | |||||||||||||
|
אכן לדעת מה המה אלה נעשה להם כלילת יופי והוא שנחלקם למורים אלו ויעלה ט' שלימים ושלשית חלק אחד מי"א בשלם וד' שישיות שלישית חלק אחד מי"א בשלם | |||||||||||||
|
| Check | |||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| If you want to check your procedure, multiply the second [of the second pair] that was unknown by the 33 quarters of a quarter, which is first of the first [pair], then divide the product by one of the remaining [numbers]; the result should be the other [remaining number] itself. If not, know that you were wrong. | ואם תרצה לבחון מעשיך כפול זה השני שהיה נעלם בל"ג רביעיות רביעית שהוא הראשון מהאחדים וחלק העולה על אחד מהנשארים ויצא האחר בעינו ואם לא דע שטעית | ||||||||||||
|
|
|||||||||||||
|
והנה כאשר נכפול זה בל"ג רביעיות רביעית הוא כאומרנו ל"ג רביעיות רביעית מט' שלמים ושלישית חלק מי"א בשלם כזה | ||||||||||||
[216]
| |||||||||||||
|
ונפרוט הט' שלמים והשברים אשר עמו ויעלו 7921 ושישיות שלישית מי"א בשלם כזה | ||||||||||||
| |||||||||||||
|
ונחלק לשני מהאחרות והיא 96 חמישיות חמישית ותצא הנשארת שהיא ה175 שישיות שישית |
|
ונכפול המספר המתחלק ר"ל ה59136 במורי ה96 שהם 5 5 ויעלה 1478400 |
|
וכדי שלא להכפל הענין כמו שביארנו לא נכפול ה96 במורי האחרת אבל נקחם למורים שנחלק עליה ועל ה96 |
|
ואם נרצה נקח מוריהם והם 6 8 2 ונשימם עם הראשונים |
|
ולפי שאנו מבקשים לידע אם רצה בחילוק 175 שישיות שישית הרי הוא כאלו שאלו לנו כמה שישיות שישית יצא מהחלוקה |
|
ואם לא היו במורינו היינו צריכים לכפול כל המספר המתחלק בהם ולהוסיפם על האחרים ולשומם ראשונה כמו שנתבאר למעלה |
|
|
|
ונראה כאשר יגיע אליהם יצא בו בחלוק ר"ל בחלוקנו לב' שהו' המורה הסמוך להם בצורה זו אם יצא בחלוק 175 אז נדע שלא טעינו כי הם שישיות שישית ובלבד שלא ישאר |
|
וכדי להקל מעלינו כבר ידעת כי כך הוא החלק על המורים כעל אמם ונוציא אם כל המורים זולתי ה6 6 הנזכרים וזה בכפול אותם זה בזה והעולה באחר וכן כלם ותהיה האם 8448 |
|
ואם כאשר נחלק מספרינו על ה8448 שהיא אם המורים כלם זולתי ה66 יצא בחילוק [217]175 ולא ישאר דבר נדע שלא טעינו |
|
נמצא שאם היה עולה מספרינו בכפול זאת האם בה'17 נדע שלא טעינו |
|
וכדי להקל מעלינו כי הכפל במעשה החילוק נכפול הה'17 באם ר"ל ב8448 ונדע אם יצא מספרינו |
|
והאמת כן הוא שכפל הה'17 ב8448 יעלה 478400 והוא מספרינו |
| Examine it. Our whole operation is true. Deduce from this. | ובחנהו והנה כל מעשינו אמת והקש על זה |
| This is the rule: [to extract] the proportions of fractions is to decompose and multiply each of the three numbers separately, or which ever of these [operations] needed, then multiply the one by the other and divide [the product] by the third. | זה הכלל שערכי השברים הוא לעשות לכל א' מהג' מספרים לבדו פריטה והכאה או אשר מהן יצטרך לכפול הראשון בשני ולחלקו בשלישי |
| The proof: to multiply the result by the unknown - if it is second [in one of the two pairs], we multiply it by the first of the other [pair]; if the unknown that we generated is first [in one of the two pairs], we multiply it by the second of the other [pair] - then we divide [the product] by one of the two remaining [numbers] and the result should be the other [remaining number]. | והמופת לכפול היוצא לנו במקום הנעלם אם הוא שני נכפלנו בראשון שאינו ראשון ואם היה הנעלם אם הוא שני נכפלנו בראשון שאינו ראשון לו ואם היה הנעלם אשר חדשנו ראשון נכפלנו בשני שאינו שני לו ונחלקנו לאחד מהנשארים ויצא האחר |
|
|
|
| The reason of all this is the same as its reason for integers, since the operation is the same, therefore the reason is the same. | וטעם כל זה כטעמו בשלמים כי אם אחר שהמעשה אחד בעצמו גם הטעם אחד בעצמו |
Chapter Six: Roots |
הפרק הששי בשרשים | ||||||
| The procedure | |||||||
| You will need here also to decompose and multiply your fractions, or whatever is needed of [these operations], then multiply the result again by all the denominators one after another, or by the common denominator.
|
גם בזה תצטרך לעשות לשבריך פריטה גם הכאה או מה שיצטרכו מהם והיוצא תשוב תכפול אותו בכל המורים אחד אחד זה אחר זה או באמם | ||||||
| Be very careful not to add to it what is beneath the denominators, for this is done only when decomposing. | ושמור נפשך מאד שמר שלא תחבר לו הנמצא תחת המורי' כי זה לא יעשה כי אם בפריטה | ||||||
| Extract the root of the whole result, as you do with integers, and the integers resulting [from the extraction of] the root are parts [= numerator] of these denominators. | ומכל העולה הוצא השרש ככל כמעשיך בשלימים והשלימים היוצאים בשרש הם חלקים משלם מאלו המורים | ||||||
| Reduce them, if you wish. | ואם תרצה [עשה להם][218] כלילת יופי | ||||||
| The fractions resulting [from the extraction of] the root are fractions of one part of all these denominators. | והשברים היוצאים בשרש הם שברים מחלק אחד מכל אלו המורים בשלם | ||||||
|
המשל רצינו לדעת שרש ד' שישיות מד' [219]שלמים וה' תשיעיות | ||||||
|
כזה | ||||||
| |||||||
|
|||||||
|
נפרוט הד' שלימים ונכפול אותם בט' יעלו 36 ועם ה5 יהיו 41 | ||||||
|
נכם בד' יעלו 164 שישיות תשיעית כזה | ||||||
|
ויצאו 94 שלימים שהם 94 שישיות תשיעיות ונשארו 20 |
|
ואם רצית להתקרב אל האמת כפול השרש שהוא 94 וחלקם עליהם ויעלה בדרך האחדות והוא שנחלק כפל ה94 שהוא 188 ל20 ועלו ט' ונשארו ח' | ||||||
|
הוספנו א' מעל הט' היה 10 שהוא מורה עשירית אחת ונחסר הח' הנותרים מן ה20 נשארו י"ב שהם חלקים מ188 ומעשירית | ||||||
|
ומורה ה188 והם | ||||||
|
נעשה להם כלילת יופי והוא שנחלקם לד' יצאו ג' ולא ישאר דבר ואחר שהם פחות מהמ"ז נשימם תחתיו כזה | ||||||
|
|
הנה עלה לנו כל השרש 94 ועשירית וג' חלקים מ47 מעשירית וכל אלו הם חלקים משישית תשיעית כנזכר | ||||||||
|
א"כ השרש היוצא הוא 94 שישיות תשיעית ועשירית שישית תשיעית וג' חלקים ממ"ז מעשירית שישית תשיעית כזה | ||||||||
|
|
ונעשה כלילת יופי ל94 ויעלה א' לשלם וזהו שלם באמת ועוד ו' תשיעיות שהם שני שלישיות ועוד ד' תשיעיות שישיות ויש לנו עוד עמהם עשירית שישית תשיעית וג' חלקים ממ"ז מעשירית ששית תשיעית כזה |
| |||||||||
|
וזהו השרש הקרוב | ||||||||
Check |
|||||||||
|
ואם תרצה לבחון אותו כפול אותו על עצמו וראה אם יתקרב לנשאל שהוא 146 שישיות תשיעית [221]בתוספת אחד בכמו מרובע השברים אשר הוספנו על שרש השברים הראשון אשר הוצאנו | ||||||||
|
והעשירית שישית תשיעית וג' חלקי' ממ"ז מעשירית שישית תשיעית שמרובעם ר"ל כפלם בעצמם אחר הפריטה יעלה 2500 חלקים ממ"ז מעשירית שישית תשיעיות ממ"ז מעשירית תשיעית | ||||||||
|
וכאשר תעשה להם כלילת יופי יעלה ד' תשיעיות ממ"ז ממ"ז משישית תשיעית ושישית מתשיעית ממ"ז ממ"ז מששית תשיעית |
|
והנה פריטת זה השרש יעלה 44230 חלקים מחלק ממ"ז מעשירית משישית תשיעית |
|
וכאשר נכפול זה על עצמו הוא כאומרנו 44230 חלקים מחלק מ"ז מעשירית שישית תשיעית [מ442300 חלקים מחלק מ"ז מעשירית ששית תשיעית כזה][222] כזה | ||||||||||||||
| |||||||||||||||
|
ונכה ה44 | ||||||||||||||
| |||||||||||||||
|
ונעשה להם כלילת יופי ר"ל שנחלקנו לכל המורים האלו עד הגיענו אל הט' והו' המורים הראשונים ובהיגיענו שם נדע כמה שישית תשיעיות יעלה אם יגיע למספר הנשאל שהוא 164 שישיות תשיעית ועוד מרובע השברים הנוספים הנזכרים כנזכר ואשר עלינו זה עלה 164 שישיות תשיעית ועוד ד' תשיעיות ממ"ז ממ"ז [נ' ד'][224] משישית תשיעית וזה התוספת שוה ממש למרובע השברים הנוספים בשרש על שרש השברים אשר יצא ראשונה והיה כל [225]מלאכתך אמת |
| We also get from this that what we have said in chapter seven of the first section is verified that when we divide the remainder by double the [approximate] root without another addition, the square of the latter exceeds the required number by the square of the additional fractions and it is always like that.
|
ויצא לנו עוד מזה שנתאמת מה שאמרנו בפ"ז מהחלק הא' שכאשר נחלק הנשאר על כפל השרש מבלי תוספת אחר שיעדף המרובע האחרון על החשבון הנשאל כמרובע השברי' הנוספים וכן יהיה בכל פעם ופעם דוק ותשכח |
Reasons |
|
| The reason for multiplying the numerator by the denominators | |
| The reason we say that after decomposing we multiply the decomposed number by all the denominators is in order that the square will be duplicate parts of these denominators.
|
וטעם אמרנו שאחר הפריטה נכה המספר הפריטה בכל המורים הוא כדי שיהיה זה המרובע חלקים מאלו המורים פעמים ר"ל נשנים |
|
שאם היו רביעיות יהיו עתה רביעיות רביעית |
|
ואם היו חמישיות רביעית יהיו עתה חמישיות רביעית חמישית רביעית |
|
וכן לעולם |
| We need this because the denominators of the root are always duplicated as the denominators of the square. | והוצרכנו לזה לפי שמורי השרש לעולם הם נשנים במורי המרובע |
|
וזה שאם השרש ע'ד'מ' ב' רביעיות יהיה המרובע ד' רביעיות [רביעית] |
|
ואם יהיה השרש ב' חמישיות רביעית יהיה המרובע ד' חמישיות רביעית חמישית רביעית |
|
והטעם בזה לפי שהכפל בשברים שהוא אומרנו ע'ד'מ' כפל ב' רביעיות בב' רביעיות הוא כאומרנו ב' רביעיות מב' רביעיות כמו שביארנו למעלה |
|
ולדעת כמה רביעיות רביעית הם יש לנו להכות הב' בב' |
| I.e. the number of fractions of the root is multiplied by itself, like our method [in calculating] the square of an integer, as the number of the parts and their duplication.
|
ר"ל |
| Because, in integers, the number of the root and the number of the square are of one type, i.e. they are both integers. | כי בשלמים מספר השרש ומספר המרובע הם ממין אחד ר"ל שהם שלימים |
| Therefore, the square [of an integer] is always greater than the root.
|
ולזה יהיה לעולם גדול המרובע מהשרש |
| Likewise, every product of an integer by a number.
|
וכן כל כפל מספר שלם במספר |
| Even if it is a product of integers by fractions.
|
ואף אם יהיה כפל שלימים בשברים |
| Since the number increases by multiplication, but the type [of number] does not change.
|
וזה לפי שהמספר מתרבה בכפל והמין אינו משתנה |
|
כי כאשר תאמר ע'ד'מ' כפול ג' שלמים בד' שלימים או בד' חמישיות הוא כאומרך כפול ג' פעמים ד' שלימים או ד' חמישיות הנה שהמספר מתרבה והדין לא ישתנה |
|
אבל בשברים אומרנו כפול ב' רביעיות בג' חמישיות הוא כאומרנו שני רביעיות פעם |
|
ואם [227]אומרנו כפול רביעית אחת בג' חמישיות הוא כאומרנו ג' חמישיות רביעית פעם והוא ג' חמישיות רביעית |
|
ואולם אומרנו שני רביעיות יהיה בב' פעמים רביעית פעם וכל פעם הוא ג' חמישיות רביעית הנה הב' רביעיות יהיו ו' חמישיות רביעית |
| The number is increasing through the multiplication of the numerator by the numerator. | וכן לעולם יתרבה המספר בכפל [מספר השברים במספר][228] |
| The result is [a fraction] of the denominators of both multiplicands together, as in our example, which are fifths of a quarter.
|
ויהיה העולה מכל |
| Hence the numerator of the square is a square of the numerator of the root, exactly as it is in integers.
|
ולזה יהיה מספר שברי המרובע כמרובע מספר שברי השרש כדרכו בשלם שוה בשוה |
| But, the denominators are duplicated, since we multiply the root by its similar, and the denominators of both are twice the denominator of one, because they are equal, and the reason of all this is clear. | אבל כי המורים נשנים לפי שאנו כופלים השרש בכמותו ומורי שניהם יהיה כפל מורי האחד כי שוים הם במורים וכל זה ברור בטעם |
| It becomes clear also when we multiply a surface. | גם זה יתבאר בשאנו כופלים בשטח |
| For, when we say about the surface: 3 times 4, it as our saying that the length is 4 [and the width is 4].
|
כי כאשר אנו או[מרי]ם בשטח ג' פעמים ד' הוא כאומרנו שיש בארך ד' |
| If its width were only 1, it would have been only 4.
|
ואלו לא היה ברחבו כי אם א' לא היו כי אם ד' |
| Since every unit that we note in the surface has 1 in length and 1 in width. | לפי שכל אחד שאנו אומרים בשטח הוא שיהיה לו א' באורך וא' ברוחב |
| Likewise in the solid: 1 in length, 1 in width, and 1 in height. | וכן בגשם א' באורך וא' ברוחב ואחד בגובה |
| Therefore, the square of one does not increase and neither the cube, for our saying: one, concerning the surface, is as our saying: one square, and the same concerning the cube solid.
|
לזה לא יתרבה מרובע האחד ולא גם המעוקב כי אומרנו אחד בשטח הוא כאומרנו אחד מרובע וכן בגשם מעוקב |
| When it is 4 in length and 3 in width, it is as 3 stripes of 4, which is as our saying: 3 times 4, and so on.
|
וכאשר היו ד' באורך וג' ברוחב הרי הם ג' רצועות של ד' ד' והוא כאומרנו ג' פעמים ד' |
| But, when we multiply a fraction by a fraction: | אבל כשא[א][229]נו כופלים שבר בשבר |
|
המשל ג' רביעיות בד' חמישיות הוא כאומרנו שארכו ד' חמישיות השלם ורחבו ג' רביעיות השלם |
|
ואם ארכו אחד שלם היה ג' רביעיות שלם כי מן השלם המרובע חסר הרביע שנפצל מרחבו וזה מובן במעט עיון |
|
אבל לפי שמארכו נפצל ג"כ חמישיתו הנה הוא כמי שהסיר מהג' רביעיות חמישיתם ונשארו ד' חמישיותיהם הנה השטח הוא ד' חמישיות מג' רביעיות [וכל חמישית מהם היא חמישית ג' רביעיות שהוא כשלש רביעיות חמישית כי כך הוא חמישית רביעית כרביעית חמישית א"כ הד' החמישיות מג' רביעיות הם ד' פעמים][230] הם ד' פעמים ג' רביעיות [231]רביעיות חמישית שהם י"ב |
| Therefore, we multiply the numerator by the numerator, [when extracting] the root, and it all comes down to the same thing. | ולזה אנו כופלים בהכאה מספר השברים במספר השברים וכן בשרש והכל עולה לענין אחד |
| After we have explained that the denominators of the square are the duplication of the denominators of the root and that the numerator of the square is as the square of the numerator of the root, it is clear that if the square has duplicated denominators, i.e. 4 and 4, or 5 and 5 etc., we do not need to multiply by the denominators, but to extract the root of the numerator alone, as in the way of the integers, and the denominators of the root are half the denominators of the square. So, we divide [the root of the numerator] by half the denominators [of the square].
|
ואחר שביארנו שמורי המרובע הם נשנים ממורי השרש ומספר שברי המרובע הוא כמרובע מספר שברי השרש נתבא' שאם היו לזה המרובע מורים נכפלים ר"ל ד'ד' או ה'ה' וכדומה לזה שלא היינו צריכים לכפול במורי |
| Even if not all [the denominators] are duplicate, but each of those that are not twice in it are multiplied by themselves, i.e. they are squares as 4, or 9, take the root of that denominator of the square as a denominator of the root, i.e. 2 instead of 4, 3 instead of 9. Because you can write 2 and 2 as the denominators of the square, instead of the 4, or 3 and 3 instead of 9, then you take one of them for the root and all this is clear.
|
וכן אפי' אם לא היו כלם כפולים אבל שכל אחד מאשר אינם בו פעמים הוא כפול ר"ל כי אם הם כפולים בעצמם ר"ל שהם מרובעים כד' או כט' תקח שרש המורה ההוא אשר למרובע במקומו למורה השרש ר"ל הב' במקום ד' והג' במקו' הט' וזה שהרי בידיך לשום כמורי המרובע במקום הד' השנים או במקום הט' ג'ג' ותקח אחד מהם בשרש וכל זה ברור |
| This will be further explained in the chapter on factorization that is in a section I intend to write the end of the book. | ויתבאר עוד במאמ' ההתכה אשר בכלל אשר ייעדתי לשום בסוף הספר |
| If there are of those and of those, multiply the numerator by those that are not duplicate and are not squares, and add them to half those that are duplicate and to the roots of the square denominators that you take instead of them. Divide the integer resulting in the root by them and the fractions resulting in the root are parts of one part of these denominators, by which you divide the integers of the root.
|
ואם יהיו שם מאלו ומאלו תכפול מספר שברי המרובע באשר אינם נכפלים ולא מרובעים ותוסיפם על חצי הנכפלים ושרשי המורים המרובעים אשר לקחת במקומם ועליהם תחלק השלימים היוצאים בשרש והשברים היוצאים בשרש הם חלקים מחלק אחד מאלו המורים אשר להם תחלק שלימי השרש |
| All is clarified in practice and reason. | והכל נתבאר במעשה ובטעם |
| In order not to confuse you by examining if they are duplicate and taking their half, or taking the roots of the squares, I instruct you to multiply it by all [the denominators], so that the required square has now twice the denominators that it had originally and we divide the numerator by [the denominators] it had originally that are half of those it has now.
|
אכן כדי שלא לבלבלך בזה לראות אם הם נכפלים ולקחת חציים או לקחת מהמרובעים שרשם במקומם |
| It is best for you to bother, even if it is not necessary, so as not to get confused, if you are not well versed in the procedure. | וטוב שתטרח ואם לו לצורך כדי שלא תתבלבל אם אינך בקי במלאכה |
| But, if you see your self deserve to be prayer leader, a Cohen who lifts his hands, you can make the procedure easier for you. | ואם ראית בעצמך שאתה ראוי להיות |
General Rules for Operations with Fractions |
|
| After we have completed the six chapters on fractions, we start with all that we have designated that is beneficial to all. | ואחר אש' השלמנו הו' פרקים אשר בשברים נתחיל בכל אשר ייעדנו שהוא מועיל לכלם |
Finding the Common Denominator |
|
| The rule that is useful for all fractions. | הכלל המועיל לכל השברים |
| If you wish to solve all the issues of the chapters on fractions perfectly, seek for one great denominator that includes all the numbers, i.e. a common denominator for all their denominators in question, by which you will clearly find everything you want, i.e. you will be able to find through this common denominator how much is the quarter, the fifth, or any of the fractions you need. | אם תרצה להוציא כל ענייני פרקי השברים על השלימות תבקש לכל המספרים מורה א' גדול כולל אותם ר"ל אם כל מוריהם ושם תמצא כל מבוקשך בברור ר"ל שתוכל למצוא במורה ההוא כמה הוא הרביעית והחמישית או כל מה שתצטרך בכל השברים ההם |
|
כי המשל אם אמרנו חבר ג' רביעיות תשיעית עם ד' חמישיות תשיעית עם 7 חמישיות שביעית |
|
הנה מורה החשבונים הגדול אשר אמרתי הוא אם ד' מורים אלו והוא בהכפל זה בזה והעולה באחר עד תומם ויהיה 1260 |
|
והוא שעשינו האחד השלם 1260 חלקים |
|
והנה תשיעית הוא ק"מ והוא ככפל הג' מורים הנזכרים ר"ל באמם |
|
ורביעית התשיעית יהיה ברביעית זה והוא ל"ה והוא אם המורים הנזכרים ר"ל ככפל ה' בז' |
|
והג' רביעיות התשיעית יהיו שלשה פעמים ל"ה שהם 105 |
|
ושביעית המורה הוא אם השלשה הנשארים והם 180 |
|
וחמישית שביעית הוא חמישית זה והוא ל"ו והוא אם הנשארים |
|
והד' חמישיות שביעית הם ד' פעמים ל"ו או אם תרצה לומר ד' פעמים כפל הב' מורים זה בזה ר"ל ט' בד' שהוא ל"ו והעולה יהיה 144 |
|
[233]ותחברם עם הה'10 שעלו הג' רביעיות תשיעית יעלו 249 ב0ובא חלקים בשלם כי זה מורה הוא מספר המורה הגדול אשר לקחת וכל חלק מאלו הוא חלק מכל אלו המורים |
|
וזה כי 140 הם תשיעיות אחד |
|
ול"ה שהם רביעיתם הם רביעית תשיעית |
|
והה' שהם שביעית הל"ה הם שביעית רביעית תשיעית |
|
והא' שהוא חמישית הה' הוא חמישית תשיעית רביעית שביעית |
|
א"כ אלו ה249 הם חמישיות תשיעית רביעית שביעית |
|
ואם תרצה לידע מה המה אלה הנה אחר שכל ה' מהם הם תשיעיות רביעית תשיעית תחלקם לה' והיוצא יהיו שביעיות רביעית תשיעית |
|
ואם נשאר דבר הוא כבתחלה חמישיות תשיעית רביעית שביעית |
|
ומהשביעית רביעיות תשיעית וכאשר תחלק לז' יהיה היוצא רביעיות תשיעיות |
|
וכשתחלק זה היוצא לד' יהיה היוצא תשיעית |
|
וכשתחלקנו לט' |
| The whole aforementioned procedure itself is as stated in diagrams that are not in the book, for the order is unimportant. Deduce from this. | וכל זה |
|
והנה העולה מחבור השברים הנשאלות על כל א' מהדרכים כי הכל אחד הוא שביעית אחת ורביעית שביעית וד' תשיעיות רביעית שביעית וד' חמישיות תשיעית רביעית שביעית |
| Deduce on that in all the other chapters. | והקש על זה בכל שאר הפרקים |
Completion of Fractions |
מאמר ההשלמה |
| Completion is when we have known fractions or fractions of fractions and we need to subtract them from other fractions or fractions of fractions that we have of the same types. It happens in the extraction of roots [for instance], as written in chapter six of this section. | ההשלמה הוא כאשר יש בידינו שבורים ידועים או שברי שברים ואנו צריכים לגרעם משברים או שברי שברים אחרים שיש בידינו ממיניהם וזה יקרה בהוצאת השרשים כמו [234]כפי שנכתב בפ' ו' מזה החלק |
| Sometimes the subtracted fractions of fractions are greater than those from which they are subtracted, but there are many fractions or integers to complete our deficiency. | ולפעמים השברי שברים הנגרעי' הם רבים מאשר יגרע מהם אכן יש שם שברי רבים או שלימים למלאת די מחסורנו |
| Therefore, when we take the integer or the greater fraction, to subtract from it these fractions of fractions, we need to know easily the remainder from that integer or that great fraction after we subtract from it the deficiency of these fractions of fractions for a whole unit or for a larger fraction, and this is their complement for one.
|
לכן אנו צריכים לידע כאשר נקח השלם או השבר הגדול להוציא ממנו שברי שברים אלו שנדע בקלות הנשאר מהשלם או מהשבר הגדול [ההוא אחר שהוצאנו ממנו שזה הוא מה שחסרים אלו השברי שברים מאחד שלם או שבר גדול][235] |
| When we know their complement for one, if we have fractions of fractions of their type, after we subtract from them those that we have that are smaller, we add this complement to them and the sum is the remainder. | ואחר שנדע השלמתן לאחד אם היו לנו שברי שברים ממינם כאשר נגרע מהם אלו שהיו מעט אשר בידינו נחבר זאת ההשלמה עמהן והמחובר יהיה הנשאר |
|
המשל רצינו לגרוע ז' תשיעיות וה' שביעיות תשיעית וג' רביעיות שביעית תשיעית מג' שלמים וה' תשיעיות ושלש שביעיות תשיעית |
|
הנה להיות הג' רביעיות רב מהב' גם הה' שביעיות מהג' גם הז' תשיעיות מהה' נצטרך לקחת אחד שלם למלאת די מחסורינו וישארו ב' שלמים |
| In order to know how much remains from it after we take enough, we have to complete it to a whole integer and the complement is the remainder. The reason is clear. | ולדעת כמה ישאר ממנו אחר קחתנו ממנו די ספקנו נצטרך להשלימם לאחד שלם וההשלמה הוא השארית וזה ברור בטעם |
| Then, we add the complement to the fractions that we had that were not enough for us, for they have the right of redemption [Jeremiah 32, 7], and the sum is the remainder. | וזאת ההשלמה נחברנה עם השברים אשר היו לנו ולא היה בהם די ספקנו כי להם משפט הגאולה[note 38] והמחובר הוא הנשאר |
|
ונאמר ג' רביעיות שביעית תשיעית בכמה יהיו שביעית תשיעית ברביע אחד נשים א' תחתיהם |
|
עוד נאמר הרי השלמנו [לשביעית תשיעית אחד וחמש שביעיות תשיעית שהיו לנו הרי ו' ובכמה ישלומו לתשיעית אחד][236] לתשיעית אחד שלמה באחד נשימנו [237]תחתיו |
|
ונאמר הרי השלמנו לתשיעית שלמה וז' שיש בידינו הרי כאן ח' בכמה ישלמו לשלם באחד נשים תחתיהם א' |
|
הרי לנו שנשאר מהאחד השלם תשיעית אחת ושביעית תשיעית ורביעית שביעית תשיעית ונחברם עם אשר בעליונה ויעלה שנשאר בידינו ב' שלימים וו' תשיעיות וד' |
| In order to make the procedure easier for you, I shall give you a rule: we write the complement of the numerator for its denominator under the last [fraction] to the left. | וכדי להקל מעליך המעשה אתן לך כלל כי לאחרון אשר לצד שמאל אשר שם יתחיל הצורך נשים תחתיו כדי השלמת מספר שבריו למורה אשר עליו שוה בשוה |
|
ר"ל הג' רביעיות בכמה ישלימו הג' לד' שהוא המורה אשר עליו בא' נשימנו תחתיו |
| For all the others, until we find enough to take from it the one that we need, we always write beneath the numerator its complement for its denominator minus one and this is the one that is supplemented in what preceded to the left. | ובכל האחרים עד אשר נמצא מקום רב אשר משם נקח האחד אשר הוצרכנו לעולם נשים תחת מספר השברים כדי השלמתן למורה אשר עליהם חסר אחד והוא האחד אשר הושלם כבר באשר אחריו לצד שמאל |
| If we want, we can apply the method that we use for integers, and then we do not need completion at all. | ואם היינו רוצים היינו עושים כדרך שאנו עושי' בשלימי' ולא נצטרך להשלמה כלל |
| Borrowing one unit from a fraction of a higher type, and marking the loan with a dot as a reminder: | |
|
והוא שנאמ' ברביעיות שהוא אחרון ג' מב' לא יוכלו לצאת כלו הא' ממקום השביעיות אשר לפניו ונשים נקודה על מספר השביעיות אשר לנו לגרוע כדי שנזכור להסירו עמהם בהגיענו שם כדרך שאנו עושים בשלימים להוסיף על הנגרעים א' בשביל הנקודה ונסירה כלו ממינו |
|
ואחר שלוינו האחד ושמנו זה הנקודה נקח בעד זה האחד כמורה שהוא ד' ונאמ' ד' וב' הם ו' נסיר מהם הג' ישארו ג' |
|
ונאמר ה' שביעיות ונקודה הם ו' לא נוכל להסירם מהג' נשים נקודה על הז' תשיעיות אשר לנו לגרוע ונאמר [239]זה האחד הוא ז' כמורה וג' הרי י' נסיר מהם ו' ישארו ד' |
|
עוד נאמר ז' ונקודה הם שמונה לא יצאו מה' נשים נקודה מחוץ במקום הראוי לשלימים אם היו לנו שלימים ונאמר זה האחד הוא ט' כמורה וה' הרי י"ד נסיר מהם ח' ישארו [ו' |
|
עוד נסיר הנקודה שהוא א' שלם מהג' שלימים ישארו ב'][240] ב' שלימים |
| Thus, these procedures are the same and the reason is clear. | והנה כל המעשה אחד והכל ברור בטעם |
| It is enough for the one who understands. | ודי למבין |
Discussion on the Decomposing and Composing of Fractions |
מאמר התכת השברים והרכבתן או שתיהן יחד |
| Since sometimes there is a need to convert denominators to other denominators when expanding and reducing, I thought to explain how one denominator is decomposed to two denominators. | לפי שלפעמים יצטרך להשיב מורים למורים אחרים בהשואה ובכלילת יופי להוציאם מן הכלל ראיתי לבאר איך יותך מורה אחד לשני מורים |
| This is when the denominator is composed. | וזהו כאשר המורה מורכב |
|
כו' שהוא מורכב מב' וג' שנסירהו ונשים תחתיו ב'ג' |
|
וכן בעד ט' ג' ג' |
|
ובעד ח' ב' ד' |
| This is the rule: the product of the replacing denominators is the same as the removed [original denominator]
|
זה הכלל שכפל המורים המושמים תחתיו יהיה כמו המוסר |
|
ולפעמים נעשה להפך שנשים הב' אחד ר"ל שנשים הב' והד' ונשים תחתיו הח' וכן בכללן |
|
וזהו כמו לשים האם תחת המורים או המורים תחת האם |
|
ולפעמים נצטרך הכל |
|
כגון שיש בידינו ו' ד' ואנו צריכים ג' ח' |
|
או שיש בידינו ג' ד' ואנו צריכים ו' ב' |
| This is the rule: | זה הכלל |
|
אם אשר שמנו הוא א' במקום רבים צריך שיהיה מספרו ככפל המורים זה בזה |
|
ואם רבים תחת אחד שיהיה כפלם זה בזה כמספר המוסר |
|
ואם רבים במקום רבים שיעלה כפל אלו זה בזה |
| This is enough for the one who understands. | ודי למבין |
Short Rule for all Chapters on Fractions |
כלל קצר לכל פרקי השברים |
Addition |
החבור |
| Multiply the [numerator] whose denominator or denominators are smaller by the excess of the denominators of the other over its denominators, then divide [the product] by the smaller denominators. Add the result of division to the numerators of both [and the sum is] the parts of the denominator or the denominators of the greater.
|
תכפול אשר מורהו או מוריו קטנים בתוספת מורי האחרת על מוריו ותחלקנו למורים הקטנים והיוצא בחילוק תחברנו לשברי שניהם חלקי המורה או המורים הגדולים |
|
ואם [241]בחלוקה הראשונה ישאר דבר הוא חלק מכל המורים גדולים וקטנים |
|
המשל אם אמרו חבר ג' שביעיות עם ב' שלישיות |
|
כפול הב' בד' יהיו ח' חלקם לג' ויצא בחלוק ב' וישארו ב' |
|
וב' אלו שיצאו בחלוק חברם עם הג' והב' שהם שברי שני המספרי' ויעלה הכל ז' |
|
חלקם לז' יעלה א' ולא נשאר דבר |
|
וזה האחד היוצא בחלוק הוא א' שלם |
|
ואם היה נשאר דבר היה שביעיות |
|
והב' שנשארו בחלוקה ראשון הם שלישיות שביעית |
|
נמצא שעלה מחבורם אחד שלם ושתי שלשיות שביעית |
| If you wish, multiply the numerator of the one by the denominator of the other, then divide by its own denominator and add the result of division to the numerator of the other; [the result] are parts of the denominator of the other.
|
[ו]אם תרצה כפול שברי האחד במורי האחרת וחלקנו למורי עצמה והיוצא ב בחלוק חברם לשברי האחרת ויהיו חלקים ממורי האחרת |
|
ואם נשאר שום דבר בחלוקה הם חלקים ממורי שתיהן |
|
המשל כפול ב' בז' יעלו י"ד חלקם לג' יצאו ד' וישארו ב' |
|
חבר הד' לג' שהם שברי האחרת יעלו ז' והם ז' שביעי[ו]ת שהם א' שלם והב' שנשארו בחלוקה הם ב' שלשיות שביעית |
| It all comes down to one way. | והכל עולה לדרך אחד |
Subtraction |
החסרון |
| Multiply the numerator of the greater by the denominator of the smaller, then divide the product by the denominator of the greater. Subtract the numerator of the smaller from the result of division and divide the [remainder] by the denominator of the [smaller]. The [result] are parts of the denominators of the smaller.
|
כפול שברי הגדולה במורי הקטנה והעולה תחלקנו למורי הגדולה ומהיוצא בחילוק תחסר שברי הקטנה והעולה תחלקנו למורי הגדולה והנשאר הוא חלקים ממורי הקטנה |
| If there is something left from the first division, they are parts of the denominators of the smaller and the greater. | [ואם נשאר דבר בחלוקה ראשונה הם חלקים ממורי הקטנה והגדולה][242] |
|
המשל רצינו לחסר ב' שמיניות מג' רביעיות |
|
נכפול הג' בח' ויעלו כ"ד נחלקם לד' יצא בחילוק ו' נסיר מהם הב' ישארו ד' והם ד' שמיניות והוא הנשאר |
|
ואם בחלוקה הראשונה היה נשאר שום דבר היה רביעיות שמיניות |
Multiplication |
ההכאה |
| It was already suggested that there is no need but to multiply the numerator by the numerator and the result are parts of the denominators.
|
כבר נרמז שאין צריך כי אם לכפול השברים בשברים והעולה הוא חלקים מכל המורים |
|
המשל רצינו לכפול ד' שביעיות בה' שישיות |
|
הכה ד' בה' ויעלה כ' והם כ' שישיות שביעית וחלקם [243]עליהם ויעלה ג' שביעיות וב' שישיות שביעית |
Division |
החלוק |
| Multiply the numerators of the greater by the denominators of the smaller, then divide the product by the denominators of the greater and the numerator of the smaller, considering them as denominators.
|
כפול שברי הגדולה במורי הקטנה והעולה חלקנו למורי הגדולה ושברי הקטנה בקחתך אותם למורים |
|
המשל רצינו לחלק ו' שביעיות על ב' חמישיות |
|
כפול ו' בה' ויעלה ל' והם חצאי שביעיות חלקם עליהם יעלה ב' שלימים ושביעית אחת |
Proportions |
הערכים |
| Multiply the numerators of the second by the numerators of the third, multiply the product by the denominator of the first and the result are parts of the numerators of the first [multiplied by] the denominators of the second and the third.
|
כפול שברי השנית בשברי השלישית והעולה כפול אותו במורה הראשונה והעולה הם חלקים משברי הראשונה ומורי השנית והשלישית |
|
המשל רצינו לידע אם ג' שביעיות שוים ח' תשיעיות ד' חמישיות כמה הם שוות |
|
נכפול הח' בד' ויעלה ל"ב נכפול בז' יעלו 224 והם שלישיות תשיעית חמישית ונחלקם עליהם ויצא א' שלם וג' חמישיות וב' תשיעיות חמישית וב' שלישיות תשיעית חמישית |
Roots |
השרשים |
| Multiply the numerator of the number by its denominators, then extract the root of the product, as written above and the result is the parts of its denominators.
|
כפול שברי המספר במוריו ומהעולה נוציא שרשו כמו שכתו' למעלה ויהיה חלקים ממוריו |
|
המשל רצינו לדעת שרש ב' שמיניות |
|
נכפול ב' בח' יעלו י"ו נקח שרשו והוא ד' והם ד' שמיניות והוא השרש |
Additional rule for division of fractions |
|
| In order to abbreviate the division operation further and to give a correct answer immediately, I saw it fitting to contrive and reverse it into multiplication by inverting the smaller - the numerator into denominator and the denominator into numerator. | ולקצר עוד מעשה החלוק ולהשיב מיד תשובה נכונה לכל שואל ראיתי לתחבל ולהחזירו להכאה בהפוך הקטנה השברים למורים והמורים לשברים |
|
המשל אם אמרו לך במשלינו הראשון רצינו לחלק |
|
תשיב מיד שהם ו' שביעיות מה' חצאין והכה אותן והרי הוא כמעשה הראשון בעינו |
Additional rule for proportions of fractions |
|
| In proportions also reverse the first and return to multiplication. | וכן בערכים הפוך הראשונה ותחזור להכאה |
|
פי' במשלנו כאשר אמרנו אם ג' שביעיות שוים ח' תשיעיות ד' חמישיות כמה הם שוים |
|
תשיב מיד שהם ז' שלישיות מח' תשיעיות מד' חמישיות והכה אותן והרי הוא כמעשה הראשון |
| Over and done, thanks to the Creator of the world. | תם ונשלם ת"ל בורא עולם |
Notes
- ↑ ישעיהו מג, ד
- ↑ שיר השירים ד, ד
- ↑ משלי ל, ח
- ↑ דברים לב, ה
- ↑ קהלת א, טו
- ↑ שיר השירים ד, י"א
- ↑ משלי ג, י"ז
- ↑ ישעיה ל"ג, י"ט
- ↑ מועד, מגילה, ב, א
- ↑ דברים ל"ב, ל"ז
- ↑ איוב י"ב, ו
- ↑ איוב טז, כ"ב
- ↑ מלכים א י"ח, כ"א
- ↑ מלכים א ב, מ"ב
- ↑ מלכים א י"ד, ט"ו
- ↑ קהלת י, י"א
- ↑ קהלת ז, כ"ט
- ↑ תהילים קמד, י"ג
- ↑ תהילים ע"ה, ח
- ↑ ישעיה י"ז, ו
- ↑ תהילים צ, י"ז
- ↑ איוב ל"ו, כ"ב
- ↑ משלי ו, יג
- ↑ ישעיה י"ז, ו
- ↑ ישעיה לג, כ
- ↑ דברים כ"א, י"ז
- ↑ ישעיה י"ז, ה
- ↑ משלי לא, כט
- ↑ תהילים עג, ד
- ↑ איוב ח, יב
- ↑ איוב לח, י
- ↑ איוב כח, כא
- ↑ תלמוד בבלי, מסכת ברכות, דף ג ע"ב
- ↑ שמואל א כד, יב
- ↑ תהילים כב, ז
- ↑ משנה סנהדרין ח, ב
- ↑ בבלי, קודשים, בכורות, דף ו, ב
- ↑ ירמיה לב, ז
Apparatus
- ↑ 2r
- ↑ marg.
- ↑ marg.
- ↑ marg.
- ↑ 2v
- ↑ marg.
- ↑ marg.
- ↑ marg.
- ↑ 3r
- ↑ marg.
- ↑ 3v
- ↑ 4r
- ↑ marg.
- ↑ marg.
- ↑ 4v
- ↑ 5r
- ↑ marg.
- ↑ 5v
- ↑ 6r
- ↑ marg.
- ↑ marg.
- ↑ marg.
- ↑ 6v
- ↑ marg.
- ↑ 7r
- ↑ marg.
- ↑ 7v
- ↑ marg.
- ↑ marg.
- ↑ 8r
- ↑ marg.
- ↑ 8v
- ↑ 9r
- ↑ marg.
- ↑ 9v
- ↑ marg.
- ↑ marg.
- ↑ 10r
- ↑ 10v
- ↑ 11r
- ↑ marg.
- ↑ marg.
- ↑ 11v
- ↑ 12r
- ↑ marg.
- ↑ marg.
- ↑ marg.
- ↑ marg.
- ↑ 12v
- ↑ marg.
- ↑ marg.
- ↑ marg.
- ↑ 13r
- ↑ marg.
- ↑ 13v
- ↑ 14r
- ↑ 14v
- ↑ 15r
- ↑ marg.
- ↑ marg.
- ↑ marg.
- ↑ marg.
- ↑ 15v
- ↑ marg.
- ↑ marg.
- ↑ 16r
- ↑ 16v
- ↑ marg.
- ↑ marg.
- ↑ 17r
- ↑ 17v
- ↑ 18r
- ↑ marg.
- ↑ marg.
- ↑ 18v
- ↑ marg.
- ↑ marg.
- ↑ 19r
- ↑ marg.
- ↑ 19v
- ↑ 20r
- ↑ 20v
- ↑ 21r
- ↑ marg.
- ↑ 21v
- ↑ marg.
- ↑ 22r
- ↑ marg.
- ↑ marg.
- ↑ marg.
- ↑ 22v
- ↑ marg.
- ↑ 23r
- ↑ marg.
- ↑ marg.
- ↑ 23v
- ↑ 24r
- ↑ marg.
- ↑ 24v
- ↑ marg.
- ↑ marg.
- ↑ marg.
- ↑ 25r
- ↑ marg.
- ↑ 25v
- ↑ marg.
- ↑ 26r
- ↑ marg.
- ↑ marg.
- ↑ 26v
- ↑ marg.
- ↑ 27r
- ↑ marg.
- ↑ 27v
- ↑ marg.
- ↑ marg.
- ↑ 28r
- ↑ 28v
- ↑ marg.
- ↑ marg.
- ↑ 29r
- ↑ 29v
- ↑ 30r
- ↑ 30v
- ↑ 31r
- ↑ 31v
- ↑ 32r
- ↑ 32v
- ↑ marg.
- ↑ 33r
- ↑ 33v
- ↑ marg.
- ↑ 34r
- ↑ 34v
- ↑ marg.
- ↑ 35r
- ↑ 35v
- ↑ marg.
- ↑ 36r
- ↑ marg.
- ↑ marg.
- ↑ 36v
- ↑ 37r
- ↑ marg.
- ↑ 37v
- ↑ marg.
- ↑ 38r
- ↑ 38v
- ↑ 39r
- ↑ marg.
- ↑ 39v
- ↑ marg.
- ↑ marg.
- ↑ 40r
- ↑ marg.
- ↑ 40v
- ↑ 41r
- ↑ 41v
- ↑ marg.
- ↑ 42r
- ↑ 42v
- ↑ marg.
- ↑ 43r
- ↑ marg.
- ↑ marg.
- ↑ 43v
- ↑ marg.
- ↑ 44r
- ↑ 44v
- ↑ marg.
- ↑ 45r
- ↑ 45v
- ↑ 46r
- ↑ marg.
- ↑ 46v
- ↑ marg.
- ↑ 47r
- ↑ marg.
- ↑ marg.
- ↑ marg.
- ↑ 47v
- ↑ 48r
- ↑ 48v
- ↑ 49r
- ↑ marg.
- ↑ 49v
- ↑ marg.
- ↑ marg.
- ↑ 50r
- ↑ marg.
- ↑ 50v
- ↑ 51r
- ↑ marg.
- ↑ 51v
- ↑ marg.
- ↑ 52r
- ↑ marg.
- ↑ 52v
- ↑ 53r
- ↑ 53v
- ↑ 54r
- ↑ 54v
- ↑ 55r
- ↑ marg.
- ↑ 55v
- ↑ marg.
- ↑ 56r
- ↑ 56v
- ↑ 57r
- ↑ 57v
- ↑ marg.
- ↑ marg.
- ↑ 58r
- ↑ 58v
- ↑ marg.
- ↑ 59r
- ↑ 59v
- ↑ marg.
- ↑ 60r
- ↑ marg.
- ↑ 60v
- ↑ marg.
- ↑ marg.
- ↑ marg.
- ↑ 61r
- ↑ marg.
- ↑ 61v
- ↑ marg.
- ↑ marg.
- ↑ marg.
- ↑ 62r
- ↑ 62v
- ↑ 63r
- ↑ 63v
- ↑ marg.
- ↑ marg.
- ↑ 64r
- ↑ marg.
- ↑ 64v
- ↑ marg.
- ↑ 65r
- ↑ marg.
- ↑ 65v
Appendix I: Glossary of Terms
| rank | מדרגה, מעלה |
| dividend | המתחלק |
| divisor | אשר נחלק עליו |
| quotient | היוצא בחילוק |
| common denominator | אם המורים |
| treatise | קצור, קיצור |
| book | ספר (ה), ספרי |
| section | חלק (ה... ב) |
| chapter | כלל |
| chapter | פרק (ה / ה.. ב), פרקי ה, פרקים (ב / ה) |
| chapter | שער (ה / ה... ב), שערים |
| discussion | מאמר (ה) |
| בחלק ה... בפרק ה... ממנו | |
| introduction | מבוא ב, מבוא ל |
| introduction | הקדמה |
| to preface | אקדים |
| word | תיבה, הברותיו |
| letter | אות |
| language | בלשון |
| alien tongue | לשון נכרי |
| Hebrew language | לשון עברי |
| number | מספר (ה), מספרים, מספרינו, מספרך, מספרם, מספרן |
| חשבון (ה), חשבונך, חשבוננו, החשבונים | |
| number | מנין |
| digit | אות (ה), אותיות |
| digit | רושם, רשמים, רושם ה, רשמי ה |
| digit | מספר, מספרים, מספרי ה |
| zero | סיפרא, סיפרות, ספרות |
| prime number | מספר פשוט, פשוט, פשוטים |
| odd | נפרד, חשבון נפרד, החשבון הנפרד, מספר נפרד, הנפרדים, נפרדת |
| even | זוג, חשבון זוג, זוגי (ה), מספר זוגי |
| pair | זוגי |
| unit | אחד, אחדים, אחדי (ה), ידות |
| units | אחדים, אחדי (ה) |
| units | הפרט |
| product of tens, none-units | כלל |
| tens | עשרות, עשרי |
| hundreds | מאות |
| thousands | אלפים |
| ten thousand | רבבה, רבבות |
| millions | חשבונות |
| rank | דרגה |
| מדרגה, מדרגת ה, מדרגתה ה, מדרגות, מדרגתם, מדרגותיה, מדרגותיו | |
| מעלה (ה), מעלות (ה), מעלת ה, מעלתה, מעלתו, מעלתן, מעלותיו, מעלותיהן | |
| empty rank | מעלה חלקה ממספר, מעלה החלקה ממספר, מעלות חלקות מהמספר |
| positional value (relation) | ערך המעלות |
| positional value | בערך (ב / ה), כערך, ערך מקום ה, בערך המעלה, בערך מעלת ה |
| decimal place | מקום (ה), מקום הנחת, מקום ההנחה, מקומו ה, מקומו הוא ב |
| place | מקום (ה / ש), מקומו, מקומות |
| place holding | שמירת המדרגות |
| line | קו, קוים |
| point, dot | נקודה, נקדה, נקודות, נקדות |
| row, line | שורה, שורות, שורת (ה / ה... מה), שורתו |
| טור (ה), טורים | |
| surface | שטח |
| body | גשם |
| cube | מעוקב, המעוקב |
| stripe | רצועות של |
| length | ארך, אורך, באורך, ארכו |
| width | רוחב, רחבו, ברוחב |
| height | גובה |
| addition | |
|---|---|
| addition | חבור, חיבור |
| בחברך אליהם ה | |
| לחבר (ל / על ה / עמהם ה / ... עם / ה... על ה / כל ה / יחד כל), לחברו (ל / עם, עמו), לחברם (עם / עם ה), לחבירו עם | |
| חבר ( ... עם / ה... ל / ה... עם / הכל יחד) | |
| חברהו (ל / עם), חברם ל, חברם עם ה, חברנו ה... עם, חברת אותם | |
| יחבר ה... עם | |
| יחברם | |
| מחברים (אותו עליהם / אותם אליהם) | |
| נחבר (אליהם / אליהם ה / ל / להם ה / ה... ל / ... עמהן) | |
| נחברנה עם ה, נחברהו אל ה | |
| נחברם (אליהם / אליהם ה / יחד / ל / להם ה / עם / כלם) | |
| תחבר (אליהם ה / להם ה / לו ה) | |
| תחברם (יחד / יחד ... עם / כלם / עם ה) | |
| תחברנו (עם / עם ה / ל) | |
| to be summed | מחובר, מחוברות יחד |
| sum | המתחבר, המחובר |
| sum | המקובץ |
| sum | סך |
| summed | המקובץ (מ / מה) |
| summed | מחובר עם ה |
| עלה חיבורם, עלה מחבורם, עולה חיבורם, העולה מהחיבור, העולה מחבור ה | |
| addition | תוספת (אשר ל / בהם / ה / על ה) |
| to add | להוסיף (אותו על ה / אחד / מה / על ה / ... ב), להוסיפו (על ה / עליו), להוסיפם על ה |
| הוסיף ...על ה, הוסיף עליו זה התוספת | |
| הוסף (על ה / עליו) | |
| הוספנו (על / עליהם / ... מעל ה / ... על ה / עליהם ה) | |
| הוספנוהו על ה | |
| יוסיף לעולם על | |
| מוסיפים (... ב / עליו) | |
| נוסיף (ב / עליהם / עליו / ... ב / ... על / ... על ה / ה...על ה / ה... עם ה) | |
| נוסיפנו | |
| תוסיף (עליו / ... על / ה... על), תוסיפם על, תוסיפנו על ה | |
| המתוסף ב, המתוסף עתה ב, המתוסף על ה | |
| יתוסף ב, נתוסף, ניתוסף ב, ניתוסף דבר ב, נתוספו (ב / לו... על), מתוסף | |
| בהוספת ה | |
| added | מוסף על ה, מוספים על |
| נוסף, נוספות, נוספים (ב... על / ב... על ה / על ה), נוספת ב... על | |
| תוסף ב | |
| יוסיף ב | |
| מוסיף (על / על ה) | |
| קיבוץ כל השורות יחד | |
| plus | בתוספת (ה) |
| by addition | לתוספת |
| addition | מתוספת ב |
| additive, additional | היה לתוספת, היו לתוספת |
| exceeding | מוסיפים על ה |
| subtraction | |
| subtraction | המגרעת |
| to subtract | ונסור ה |
| subtraction | בהסר מה |
| לגרוע (... מ), לגרעם מ | |
| גרע (... ממנו / ממנו ה) | |
| נגרע (ה... מה / מהם) | |
| הנגרעים | |
| יגרע (מהם) | |
| החסרו מ | |
| חסרון (מ), החסרון אשר ב... מה, חסרונו | |
| לחסר (מה / ... מ / ה... מ), לחסרו (מ / מן ה), לחסרם מה | |
| לחסו' ... מ | |
| חסר מ, חסר ... מ | |
| חסרם מ | |
| חסרנו (ה / מ / ממנו / ממנו ה) | |
| חסרת | |
| יחסר (כ / מ / מה / ממנו כ / ... מ / ... מה / ... כ / ה... מה) | |
| יחסר יותר | |
| יחסרהו מ | |
| נחסר (ה / אלו ... מה / ה...מה / ה... מן ה / ... מה) | |
| נחסרה | |
| נחסרנו (מה), נחסרנו לעולם מה, נחסרהו לעולם מה | |
| נחסרם זה מזה | |
| תחסר (ה / מה / מהם ה / הכל מה), תחסרנו ממנו | |
| מחוסר | |
| מחסרים, מחסרים עתה מה | |
| מן ... חסר ה | |
| חסרים אלו ה... מ | |
| minus | חסר, חסר אחת |
| אשר חסרנו ממנו | |
| אשר חסרת, אשר חסרת ממנו | |
| יחסר מעלה ממקום ה | |
| בהסיר, בהסר מהם ה, להסירו, להסירם מה, הסירך ה | |
| to subtract | הסיר מה, הסר, הסרת (ה) |
| נסיר (ה / ... מה / ה ... מה / ה... מ / מה / מהם / מהם ה / ממנו ה) | |
| נסירה (ממנו / כלו ממינו), נסירהו | |
| נסירם (מה / ממנה) | |
| נסירנו מה | |
| תסיר (ה / מהם / ... מה), תסירנה, תסירנו | |
| subtraction | הוצאת... מ |
| להוציא (מה / ממנו / ... מ / ה... מה) | |
| להוציאו מהם | |
| להוציאם (מה / מהם ה) | |
| הוצא (ה / מ / ה... מ) | |
| הוצאנו ממנו | |
| הוצאת (מהם / ה... מה / ה... מן) | |
| הוציא... מה | |
| הוציאהו מ | |
| הוציאנו (מה) | |
| יוציא כל ה | |
| מוציאים מה | |
| נוציא (כל / מ / מה / מהם ה / ה... מה / ... מ) | |
| נוציאם מה | |
| נוציאנו (מ / מה / מאשר על) | |
| תוציא (ה... מן / ... מ) | |
| להוציא כ"כ פעמים המספרים | |
| to be subtracted, to be consumed | ויצא כל זה ה, יצא הכל |
| לא תוציא ל | |
| to subtract | תוציאנו מה |
| ומהכל תוציא אשר | |
| אשר עליו להוציא | |
| to be subtracted | לצאת (ה / כלו / מהם / מאשר על), יצא, יצאו (מ / ... מ) |
| בלי תוספת ומגרעת, מבלי תוספת ומגרעת | |
| מבלי תוספת, מבלי תוספת ה | |
| לבד בלי תוספת אחד כלל | |
| ואם יחסר או יעדיף | |
| Multiplication | |
| multiplication of fractions | הכאה, הכאת ... ב |
| להכות (ה... ב) | |
| הכה (אותן / ... ב) | |
| נכה (ב / ה / ה... ב), נכם ב | |
| תכה הצריך להכאה | |
| לעשות הכאה, נעשה הכאה ל, עשה להם הכאה, תעשה להם ג"כ ההכאה | |
| ונכם זה בזה | |
| הוכה (ב), הוכה... על, הוכו, הוכתה בהם | |
| לבעלי ההכאה | |
| product | כפל (ב / ה / עם / ... ב / ... על / ... עם / ה... ב / ה... על / זה ה... ב / ... זה בזה / ה... זה בזה / כל ה) |
| כפליהן, כפלו על | |
| product | המחובר |
| product | מקובץ |
| product | אשר עלה לידיך מכפל ... ב |
| multiplying | בהכפל (ה / ... ב), בכופלנו אותו ב, בכפול (אותו ב / ה... ב / ... אחד באחד / אותם זה בזה / ... ב), הכפלו ב, הכפלם (ב / כלם ב) |
| בכפול זה בזה, בהכפל זה בזה | |
| to multiply | כפלת ה... ב |
| בכפלנו אותו ב | |
| לכפול (ב / בהם / בהם ה / ה / ה ... ב / ה... עם / ... ב / ... על / כל ה... בהם / כל ה.... עם ה) | |
| לכפלה עם, לכפלו (ב / בכלם), לכפלם ב | |
| כופל ב, כופלים (אותה ב / אותו / ב / ה / כל ה / ... ב / ה... ב) | |
| כפול (אותו ב / אותו... ב / ... ב / ... על / ה... ב / ה... על / ... פעמים) | |
| כפלהו (ב / שנית ב), כפלהו אותם ב | |
| כפלו (ב / על / עם / ... ב) | |
| כפלם זה בזה | |
| כפלנו (אותם ב / בהם / ה... ב / ... ב / ... על) | |
| כפלנוהו ב | |
| כפלנום ב | |
| כפלת | |
| נכפול (אותם ב / ב / בהם / בו / זה ב / אותו... ב / ... ב / ... על / ה... ב) | |
| נכפלהו ב, נכפלו... עם | |
| נכפלם (ב / על ה / עוד ב / ... ב) | |
| נכפלנו (ב / בהם) | |
| תכפול (אותו ב / בו / ה... ב / ה... עם / ... ב / ... בו / ... על / ... ב... פעמים) | |
| תכפלם (ב / ... על ה) | |
| תכפלנו (ב / בהם) | |
| to multiply | כופלים בהכאה ... ב |
| כפל אלו זה בזה | |
| כפלו ב... פעמים | |
| product by itself | כפלתו בעצמו |
| כפלו (בעצמו / על עצמו) | |
| כפל... בעצמו, כפל ... על עצמו, כפל ה... בעצמו | |
| כפל... בעצמם, כפל ה... על עצמם | |
| ככפלו לעצמו | |
| לכפול על כולם ועל עצמו | |
| כופלים ה... בכמותו | |
| כפול אותו על עצמו, כפול ... על עצמם | |
| כפלם בעצמם | |
| כפלנו ... על עצמם, כפלנוהו בעצמו, כפלנוהו על עצמו | |
| נכפול (על עצמו / ... על עצמו / ה... על עצמו / ה... בעצמו / זה על עצמו) | |
| נכפלנו (בעצמו / על עצמו) | |
| תכפול (ה... בעצמו / אותו ה... על עצמו) | |
| תכפלנו על עצמו | |
| כפולים בעצמם | |
| multiple | כפלי (ה / מ), כפלים |
| multiplied | כפול, כפולים |
| פעמים כפל ה... זה בזה | |
| כפל הכפלים ההם | |
| לכופליו | |
| כופלו | |
| אנו לוקחים אותם כפולות | |
| to be multiplied | הוכפל ה, הוכפלה בה, הוכפלו בהם, יוכפל כפלים ב |
| product | עולה מהכפל, העולה מהכפל (ה / הזה), העולים מהכפל (... ב / ה... ב), העולים בכפל, העולות מזה הכפל, העולה מכפלו עם ה, העולה מכפלם |
| שעלה בכפל | |
| תעלה מ... | |
| היוצא אחר הכפל | |
| נכפלו, נכפלו בהם | |
| הכפלים, מנין הכפלים | |
| כפול, כפולים | |
| נכפל, נכפלים ב | |
| הנכפל, הנכפלים, מספרים הנכפלים, המספרים הנכפלים, הנכפלים זה בזה | |
| נכפל פעמים רבות | |
| אשר אינם נכפלים | |
| to be duplicate | ישנו (ב / בהם), נשנים (ב / מ) |
| duplicated | כפול, נכפלים |
| to duplicate | לכפלו, ישנך |
| duplication | כפל ה |
| duplication | השינוי |
| double | כפול, כפל (ה), כפלו |
| doubling | כפל |
| to double | כפול (ה), כפלת ה, נכפול ה, תכפול, תכפלנו |
| Division | חלוקה, לחלוקה על ה... עליו, חלוקת ה, חלוק (ה), חילוק (ב / ה / ... ב), בחילוק, בחילוק על ה, חלוקנו, בחלוקנו ל, בחלקך ל, בחלקנו (אותו ל / ה / ה... ל / ה... עליהם / אותו ל / אותם ל / ... ל), בחלקינו... ל |
| בחלקנום אותם על | |
| לחלק (ל / ... ל / ... על / ה... ל / ה... עליהם / אותן על / אליהם אלו ה / עליהם / על הכל) | |
| לחלקה עתה להם, לחלקו (ב / ל / על / עליו) | |
| לחלקם (ל / להם / על / על ה / עליהם / עליו / ראשונה על ה) | |
| חלק (ל / עליו / ה... ל / ה... על / ה... עליהם / זה ה... על / אליו זה ה / ... על) | |
| חלקהו ל | |
| חלקם (ל / עליהם) | |
| חלקנו (אותו על / ה / ה... ב / ה... על / ל / על / על ה / עליהם ה / עליו / ... ל / ... על / ... על ה) | |
| חלקנוהו (על / על ה / עליו), חלקנום (ב / ל / על / על ה / עליו) | |
| חלקת (ה... על ה / הכל ל) | |
| יחלק ... על | |
| מחלקים (אותו ל / אל / ... ל) | |
| נחלוק ... ל | |
| נחלק (אלו ה / אליהם / אליהם ה / ה / ל / לו / על / עליה / עליהם / עליהם ה / עליו / ... ל / ...על / ה... ל / ה... על / ה... על ה / אלו ה... ל / אלו ה... אל ה) | |
| נחלק זה החשבון ל, נחלק חשבוננו זה עליו, נחלק מספרינו על | |
| נחלק זה היוצא ל, נחלק זה היוצא על ה | |
| נחלקם (ל / על / על ה / עליהם / עליו / תחלה ל) | |
| נחלקם על מינו, נחלקנו למינו | |
| נחלקנו (אליהם / ב / ל / על / עליו) | |
| תחלק (... ל / ... על ה / על ה / עליו / ה... ל / ה... על / ה... על ה); תחלק (ל / עליהם); תחלק על כלם ה, תחלק כל החשבון ל, תחלק אליו לשלימים | |
| תחלקה | |
| תחלקם ל | |
| תחלקנה ל | |
| תחלקנו (ל / על) | |
| יתחלק ל, יתחלק ... ל, יתחלקו | |
| המחלק ... על | |
| נחלק ל | |
| נתחלק הכל | |
| נתחלק על כל ה, נתחלק להם המספר | |
| בחלוקה הראשונה | |
| חלקתי הספר לב' חלקים | |
| אשר אתה מחלק עליו | |
| אשר רצינו לחלק (על ה / עליהם / עליהם ה / עליו / ל), שרצינו לחלק (עליו) | |
| אשר רצית לחלק (עליו) | |
| אשר תרצה לחלק (עליו) | |
| אשר חלקנו (עליהם / עליו), שחלקנו עליהם | |
| אשר חלקת עליו | |
| אשר חלקת עליהם | |
| אשר חלקנו כבר עליהם | |
| אשר נחלק (אל / עליו), אשר נחלקו עליו | |
| אשר תחלק עליו | |
| עליהם תחלק ה | |
| אשר להם תחלק | |
| המתחלק (ל), החשבון המתחלק, המספר המתחלק, מספר המתחלק | |
| quotient | היוצא מן החלוק ה, היוצא בחלוק, היוצא בחילוק, היוצא בחלוקו, היוצאים בחלוק, היוצאים בחילוק |
| יוצאים בחילוק | |
| יצא בחלוק, יצא בחילוק, יצא בחילוק (ה / ל), יצא לנו בחלוק, יצא זה בחלוק ה, יצא בחילוק ה... ל, יצאו בחלוק, יצא ה... בחילוק, יצאו... בחילוק, יצא בו בחלוק | |
| יצא בחלוקה, יצא מהחלוקה | |
| יצא בחלוקנו, יצא בחלוקם, יצא בחילוקם | |
| העולה שיצא לנו בחלוק ה ... על ה | |
| תצא ... בחילוק | |
| יצא בחילוק מבלי שארית, היוצא בחילוק בצמצום, היוצא בחלוק בצמצום | |
| יצא בחילוק לכל אחד | |
| יצא בחילוק מספר מה | |
| אשר יוצא עתה בחילוק | |
| אשר יצא לנו בחילוק, אשר יצא בחילוק באחרונה, אשר יצא באחרונה בחלוק | |
| אשר יצאו בחילוק, אשר יצאו בחלוק, אשר יצאו בחלוק באחרונה, שיצאו בחילוק | |
| יצא לך בחלוק על | |
| יצאו בחלוק | |
| ויצא ... בחלוק, ויצא בחילו' | |
| עלה בחלוק | |
| factor, divisor | מורה (ל / לו), מורים (ל), מורי, מוריו |
| part, divisor | חלקיו |
| true divisor | מורה צדק |
| indivisible | לא נחלק לשלמים אל חשבון, לא יוכל להתחלק עליו, שלא יוכל להתחלק לשלמים, לא יתחלק כלו לשלמים |
| לא יתחלק לשום מספר בשלימות מבלי שארית | |
| divisible | יתחלק כלו לשלימים, הנחלק למספר מה, יתחלק אליו לשלימים מבלי שארית |
| divisible by two | יש לו מחצית, יש לו ב' |
| divisible by three | יש לו שלישית, יהיה לו שלישית, יש לו ג', היה לו שלישית |
| divisible by four | יש לו רביעית, יש לו ד', יש לה רביעית, יש להם רביעית, יש לחשבון רביעית, היה להם רביעית, היה לו רביעית |
| indivisible by four | אין לו רביעית, אין לנו רביעית |
| divisible by five | יש לו חמישית, יש לו חמישיות שלמות, יש לזה ה... חמישית, היה לו ה' |
| divisible by six | יש לו שישית |
| indivisible by six | אין לו שישית |
| divisible by seven | יש לו שביעית |
| indivisible by seven | אין לו ז' |
| divisible by eight | יש לה שמינית, יש לו שמינית, יש לו ח', יש להם שמינית |
| indivisible by eight | אין לה שמינית |
| divisible by nine | יש לו תשיעית, יש לו ט', יש למספר תשיעית |
| divisible by ten | יש לו עשירית, יש לו עשר, יש לה י' |
| divisible by eleven | יש לו י"א, יש לו הי"א, יתחלק לי"א על השלימות |
| indivisible by eleven | אין לו י"א |
| divisible by thirteen | יש לו י"ג, יש לו הי"ג |
| divisible by seventeen | יש לו י"ז |
| יצא הכל לשביעיות | |
| יצא הכל ז' ז' | |
| השלך לעולם הז' ז' | |
| השלך אותו ח' ח' | |
| casting out by nines | הסרת התשיעיות, חסר כל ט' ט', תוציא הט' ט' |
| to be cast out by nines | יצא הכל לט' ט', יצא החשבון לט' ט', יצא כולו תשיעיות |
| to be cast out by 11 | יושלך כלו י"א י"א, יושלך לי"א י"א |
| to cast out by 13 | הוצא הי"ג י"ג, הוצאת הי"ג |
| to be cast out by 13 | יוצא לי"ז י"ז |
| to extract the factors | תוציא המורים מ |
| to extract the factor | המצאת מורה ה |
| fractions | |
| integer | שלמים, שלימים, מספר שלם |
| fraction | שבר, שברים (מ / מה), שבורים, שברי (ה), שבריהם |
| fraction | חלק מ, חלקים (מ / מה), חלקי (ה) |
| fraction of fraction | שברי שברים (מ), שבר שבר |
| fractional | הנשבר |
| portion | שברים מ |
| part | חלק (ה / מ), חלקים מ, חלק אחד מ, חלקי ה, חלקיו |
| חלקים מחלק, חלקים מחלק מ | |
| ב... חלקים בשלם | |
| מ... חלקים בשלם | |
| חלקי... בשלם; חלקי... מ... בשלם | |
| חלקים משלם מ, חלקים מ... בשלם; חלקים מ... שבשלם; חלקים מה ... בשלם | |
| חלקים מ... באחד | |
| חלקים מחלקי ה... בשלם; חלקים מ... חלקים בשלם; חלקים מ... חלקים מ... שלם | |
| חלק מ... ב; חלק מ... בשלם; חלק מ... שבשלם; חלק ... מ... בשלם; חלק ... מ... שבשלם | |
| אחד מ... בשלם; חלק א' מ; חלק אחד מ... בשלם; חלק אחד מ... שבשלם; חלק א' מ... שבשלם | |
| חלק א' מ... מ... שב; חלק א' מ... מ... השלם; חלק אחד מ... מ... בשלם; חלק אחד מ... מ... שבשלם | |
| השברים מהשלם | |
| numerator | מספר השברים, מספר שברים, מספר שברי (ה), מספר שבריו, מספר שבריך, מספר המורה, מספר החלקים, מנין שברים, מנין השברים, שברים מה |
| החלק על המורים | |
| denominator | מורה (ה), מורהו, מורים (ל), מורי (ה), מוריה, מוריו (ה), מוריך, מורינו, המורה החלק, מורה החלק |
| הוצאת המורים, הוצאת מורי ה | |
| to extract the denominator | להוציא המורים (ל), הוצא את מוריו, נוציא מורה ה, נוציא מורי ה, נוציא מוריו, נוציא המורים ל, תוציא המורים |
| to be extracted | יצאו ממנו המורים |
| לקחתו ... למורה, בקחתך אותם למורים | |
| נקח למורה, נקח ... למורה, נקח מוריהם, נקחם למורים, נקחנו למורה | |
| תקח מורה, תקחם למורים | |
| לוקחים מתחלה ה... למורה | |
| נשים ה... למורה, נשים... למורה, נשימם למורה | |
| common denominator | אם, אם המורים, אם כל מוריהם, אם אלו המורים, אם המורים כלם, אם ... מורים, האם (ה), אמם |
| to extract a common denominator | הוצאת האם למורים, נוציא אם כל המורים |
| in reduction | בצמצום |
| reduced | שלמים |
| כלילת יופי, ובכלילת יופי | |
| בעשותך כלילת יופי | |
| לעשות לשברים אלו כלילת יופי | |
| לעשות לה כלילת יופי, לעשות להם כלילת יופי | |
| נעשה כלילת יופי ל, נעשה להם כלילת יופי, נעשה לו כלילת יופי (על) | |
| עשה להם כלילת יופי | |
| תעשה להם כלילת יופי, תעשה מהם כלילת יופי | |
| הוא כעושה כלילת יופי | |
| עשותנו להם כלילת יופי שהן | |
| unification | אחדות, לעשות בדרך האחדות |
| completion | ההשלמה, השלמתם, השלמתן (ל / לאחד) |
| to complete | השלמנו (ה / ל), להשלימם לאחד שלם, המשלימות אותו לאחד שלם |
| to be completed | הושלם כבר ב, ישלומו ל, ישלמו ל, ישלימו ה... ל, שלמו ה |
| כדי השלמת מספר | |
| complement | המשלים אותו כ, השלמה, השלמתו (ל / לאחד), השלמתם (לשלם / לאחד) |
| complement | מה שיש מה... עד תשלום |
| complement | קצתו האחר |
| to finish | השלמת ל, נשלים ה, תשלים (ל / לשורה / השורה) |
| decomposing | התכה, התכת השברים |
| to decompose | התיכנו אותו, נתיך ה |
| יותך ...ל | |
| composing | הרכבתן |
| composed | מורכב, מורכב מ, מורכב מהם |
| factorization | פריטה, פריטת (ה), פריטתינו, פריטתה, פריטת השברים, מספר פריטת השברים |
| חלקי הפריטה | |
| fractionalizing | כפורט |
| to fractionalize | לפרטם ל |
| לעשות פריטה, עשות להם פריטה, נעשה פריטה ל, עשה הפריטה ל, נעשה תחלה פריטה ל, תעשה פריטה ל | |
| עשה פריטה לכל אלו השברים | |
| פורטים אותה עוד ל | |
| נפרטות (מ), נפרטים, החלקים הנפרטו' השברים הנפרטות, השברים הנפרטים | |
| נפרוט (ה) | |
| נפרטו, נפרט (ה) | |
| תפרוט | |
| conversion | המרה |
| המרנו ה... ל, תמירם אליהם, תמירם ל, תמירם למין אחר | |
| מורה המרה, מורי ההמרה | |
| החזרת השברים, חזרת השלימים לחלקים | |
| השבת השברים, להשיבם פרוטות | |
| משיב הכללים לפרטים | |
| to convert | להחזירם מ |
| to convert | להחליפם אליו |
| להשיב ה, להשיבם, להשיבם לחלק אחר | |
| להשיב למין אחד | |
| להשיב כלם מהמין, להשיב הכל ממין השברי', להשיבם כלם מהמין הראשון | |
| להשיבם כלם ממין אחד | |
| להשיב ... לכללים | |
| להשיב שברים... לחלק אחד | |
| להשיב מורים למורים אחרים | |
| נשיבם, נשיבם ל, נשיבם כלם, נשיבם כלם ממין אחד, נשיבם עוד | |
| נשיב ראשונה ה... ל | |
| השיבם ל, נשיבהו, תשיבם, תושיבם | |
| נשיבם כלם ראשונה | |
| להשיב הכל לקדמותו | |
| to be converted | ישובו, ישוב הכל מ, שב, שבו כלם |
| to be converted | יומר |
| comparing fractions | השואה, השוואה, ההשואה שעשינו |
| בעשותך השואה זו, לעשות בזה ההשואה | |
| נעשה ההשואה ל, נעשה להם השוואה, תעשה ההשואה, אשר עשינו בהשואה | |
| להשוות ... עם, להשוותם, נשוה, תשוה ה... אחד אל אחד | |
| לעשות תחלה פריטה והכאה, לעשות לשבריך פריטה גם הכאה, לעשות לכל א' מ... פריטה והכאה, לעשות שני המעשים ר"ל הפריטה והכאה, עשותנו הפריטה וההכאה לכל אחד מהם | |
| נעשה פריטה והכאה ל, נעשה לה הכאה גם פריטה, נעשה ל... פריטה והכאה, נעשה לכל אחד פריטה והכאה | |
| עשותך הפריטה וההכאה וההשואה, עשותנו הפריטה וההכאה וההשוואה | |
| extraction of roots | |
| root | שרש (ה), שרשו, שרשים, שרשנו, שרשי ה |
| square | מרובע (ה), מרובעו, מרובעם, מרובעים |
| none square | ולא מרובעים |
| cube | מעוקב ה |
| to extract the root | ולקחת חציים |
| extracted root | השרש אשר הוצאת, שרש ה... אשר הוצאנו |
| הוצאת השרשים | |
| to extract a root | להוציא השרש, להוציא שורש, הוצא השרש, נוציא שורש, נוציא שרשו |
| לבקש שרש | |
| בקשנו שרש, בקשת השרש, בקשת שרשו | |
| לדעת שרש, לדעת שרשו | |
| לידע שרש, לידע שרשו, לידע שרשו האמיתי | |
| לקחתו השרש | |
| נקח שרשו | |
| מרובע השרש | |
| בעל השרש | |
| השרש היוצא | |
| השרש המתוסף | |
| השרש המחוסר, השרש הזה המחוסר | |
| נעשה מהכל שרש אחת | |
| השרש הקרוב | |
| אשר לא יודע בהם שרש אמיתי לעולם כי בקירוב | |
| היה זה השרש אמיתי | |
| יהיה שרש קרוב מאד אל האמת | |
| בקרוב | |
| אין זה שרש אמיתי | |
| הקרוב | |
| אשר בשרשו, אשר יצאו בשרש | |
| שלימי השרש, השלימים היוצאים בשרש, השלמים אשר יצאו בשרש | |
| השברים היוצאים בשרש | |
| השרש בעצמו | |
| אשר בקשנו שרשו | |
| כשורש בעינו, שהוא כמו השרש | |
| Proportions | |
| proportion, ratio | ערך, ערכים, ערכי ה |
| הערך ל | |
| ערך ... אצל, הערך שיש ל... אצל, הערך שיש ל... אצל ה | |
| הערך אשר ל... אצל, הערך אשר ל... אצל ה | |
| הערך שיש ל... אצל | |
| כערך ... אצל, כערך אשר ל... אצל | |
| הערך בעצמו יש ל... אצל, הערך בעצמו שיש ל... אצל | |
| הוא הערך בעצמו אשר ל... אצל | |
| למי יש ערך אצל, למי יש זה הערך אצל | |
| אצל מי יש ערך זה ל, אצל מי יש לו זה הערך | |
| אצל מי יש ל... זה הערך | |
| אצל ... למי יש לו זה הערך | |
| ל... אצל מי יש לו זה הערך, ל... אצל מי יש לו זה הערך בעצמו | |
| למי יש לו זה הערך בעצמו אצל | |
| אצל איזה מספר יש לו אותו הערך בעצמו | |
| rule of three | הג' מספרים נערכים |
| related, proportional | הנערך, הנערך אליהם, הנערך אצל (כל), נערכים |
| to relate | לקשרם יחד |
| mean | האמצעי, האמצעיים |
| first term | ראשון, הראשון שב, ראשון ל |
| second term | שני, שני שבהם, שני ל, השני מה |
| related | נערך, הנערך, הנערך אצל |
| related | נקשר ב, נקשרות, נקשרים (בו / ...ל / זה בזה / זו בזו) |
| unrelated | הבלתי נקשרות |
| what we relate to it | אשר אליו אנו מעריכים |
| אשר מעריך אצלו | |
| relation to | ערכם אל ה |
| in relation to | בערך (ה) |
| according to this relation | על הערך הזה |
| א.מ.ר. | |
| saying | אומרו, אמרנו, אומרנו (כי / ש) |
| אומרנו... כאומרנו | |
| כאומרנו (ש), הוא כאומרנו ש, היא כאומרנו, שהוא כאומרנו | |
| והנה אומרנו, והוא כי אומרנו, וזה כי אומרנו, שהוא אומרנו | |
| הוא כאומרך | |
| כך הוא אומרנו | |
| to say | לומר (ש), לו' ש, אומר כי, אומרים ש, אמרנו (ב / כי / ש / ב... ש), אמרו (לך ב / לך ש / לנו), אמרתי, יאמרו לך (ל), נאמר (ב), תאמר (ש) |
| to say | כאשר אמרנו, כאשר אמרתי |
| לאומרם | |
| רצוני לומר | |
| כאלו אמרו, כאלו אמרו לנו, כאלו אמרנו | |
| הוא כאלו אמרו ש, והרי הוא כאלו אמרו, הוא כאלו אמרו לנו, והרי הוא כאלו אמרו לנו | |
| אומרים ב | |
| נאמרו | |
| מה שאמרנו ב | |
| ב.א.ר. | |
| explanation | ביאור (הכל) |
| to explain | לבאר (איך / ה), לבארו ב, אבאר, אבארנו (ב / בטעם), ביארנו כי / ש, נבאר זה ב |
| וזה מה שרצינו לבאר | |
| כמו שבארנו, כמו שביארנו, כמו שביארנו ב, כמו שביארנו למעלה | |
| כאשר ביארנו, כאשר ביארנו ב, כאשר ביארנו למעלה | |
| וכן ביארנוהו למעלה | |
| הרי ביארנו ש, הנה ביארנו כי | |
| כבר ביארנו כי, כבר ביארנו ש | |
| שביארנו | |
| כאשר אבאר ב | |
| כאשר ביאר ב | |
| יתבאר בש, יתבאר עוד ב, נתבאר | |
| it is clear | נתבא' ש |
| כאשר התבאר, כאשר יתבאר (ב) | |
| כמו שיתבאר (ב), כמו שנתבאר (ב) | |
| כמו שנתבאר פעמים רבות כי | |
| כמו שנתבאר למעלה | |
| וכל זה יתבאר מעשהו ב | |
| ועוד נתבאר איך, ועוד נתבאר אחר זה איך | |
| וכל זה נתבאר הטב ב | |
| מבואר באר הטב | |
| להרחיב ביאור אבארנו בעודו בעינו | |
| והנה יתבאר מ... כי | |
| מבואר כי | |
| מבוארים | |
| וזה מבואר | |
| והכל מבואר למבין | |
| וכל זה מבואר, והכל מבואר ב | |
| וזה מבואר בטעם | |
| וכל זה מבואר בטעם ובצורה, הוא מבואר בטעם ובצורה | |
| והכל נתבאר במעשה ובטעם | |
| ב.ו.א. | |
| to result | יבא ה |
| ב.ח.נ. | |
| check, examination | בחינה (ב / ש), בחינת (ה), בחינות |
| to check, to examine | לבחון (אותו), אבחן, בחנהו |
| to be examined | להבחן ב |
| להבחין אם עשית כדין וכשורה | |
| לבחון אם עשית כדין אם לאו | |
| להבחין מעשיך, לבחון מעשיך | |
| to distinguish, to separate | תבחין בין ה... ל |
| ב.י.נ. | |
| to understand | להבנה, להבין, להבינו |
| understanding | הבנות, הבנתי |
| understandable | מובן |
| ודי למבין | |
| ב.ל.ב.ל. | |
| to confuse | לבלבלך בזה |
| יבלבל עליך | |
| יתבלבל (ב), נתבלבל, תתבלבל | |
| ב.ק.ש. | |
| to seek | לבקש (לו), לבקשו, לבקש אחד מהם |
| בקשת, בקשנו (ל), מבקשים ל, מבקש, תבקש (ל) | |
| נבקש מספר (ל / ש / אשר), תבקש מספר | |
| לבקש מורים ל, נבקש מורים ל, נבקש עוד מורים ל | |
| לבקש לו מורה, נבקש לו מורה, נבקש לו מורים | |
| נבקש מורה, תבקש מורי ה | |
| אשר בקשת לו כל המורים | |
| sought-after | המבוקש, הוא המבוקש |
| sought-after | מבוקשך, מבוקשנו, מבוקשינו |
| to ask for | לבקש |
| ב.ר.ר. | |
| על דרך ברור | |
| to clarify | ולברר |
| to be made clear | נתברר |
| clear, certain, evident | ברור (כי), וזה ברור (ב / כי / ש), הוא ברור כי |
| ברור ומבורר | |
| וזה דבר ברור, וכל זה ברור, והכל ברור | |
| וזה ברור בטעם | |
| והוא ברור במעשה ובטעם | |
| כי הכל ברור המעשה והטעם | |
| וכל זה תראה ברור ומפורש בטעם | |
| וכל זה מבורר בטעם הראשון למבין | |
| וכל זה ברור בטעם, וכל זה ברור בטעם למבין, והכל ברור בטעם ודי למבין | |
| ובזה תראה ברור מה שאמרתי ש | |
| clarification | ברור |
| ה.פ.כ. | |
| inverse operation | הפך ה, הפכים, להפכו |
| to be inverted | יתהפך |
| ז.כ.ר. | |
| to note, to mention | הזכירו, הזכרנו (ב / למעלה כי), הזכרתי (ב), זכרנו, נזכיר (ב) |
| above mentioned | הנזכר למעלה |
| זכר לדבר | |
| mentioned | הנזכר, הנזכרת, הנזכרים, הנזכרות, הנזכרים |
| as mentioned | כנזכר, כנזכרים ב, כנזכר למעלה |
| כמו שנזכר, כמו שהזכרנו (ב / ש), כמו שנזכר למעלה, ונזכר כבר ב | |
| יזכר ש | |
| to remember | זכור לעולם (כי / ש) |
| נזכור ל | |
| ח.ד.ש. | |
| to generate | לחדש, חדשנו |
| to be generated | נתחדש, נתחדש מ, נתחדשו (ב / מ), יתחדש |
| created, attained | המתחדש, מתחדש, המתחדשים |
| created | מחודש |
| ח.פ.צ. | |
| נחפוץ (מהם) | |
| כחפצנו, ככל חפצנו, בחפצם | |
| ח.ש.ב. | |
| to think | לחשוב כי, אחשוב זאת |
| ט.ע.ה. | |
| to mistake, to err | טעינו, טעית (ב), נטעה, תטעה (ב / ל / מל) |
| mistake, error | טעות, טעיות |
| to mislead | יטעך ש |
| י.ג.ע. | |
| effort | יגיעה |
| to exert oneself, to endeavor | ניגע |
| י.ד.ע. | |
| נדע תחלה כמה ... הוא, נדע תחלה כמה ... הם | |
| נדע כמה ....יעלה | |
| to know | לידע (אם / כלם / ל / מה / באיזה / כמה), לדעת (אם / ה / כי / ש / כמה / מה / מאיזו) |
| דע (כי / ש / לך ש), דע לך ש, ידע כי, ידעו, ידענו (ה / כי / ש), ידעת (כי / ש), ידעתי, נדע (אם / כמה / ש), תדע (ה / כי ה / ש / כמה) | |
| נדע בקלות ה... מה | |
| ביודעינו ש | |
| וזה יודע, יודע ב, יודע ה | |
| נודע (ה / ש / מזה שה), נודעים, נודעו ה, הנודעים | |
| הידוע | |
| אשר בהם נודע | |
| בהודע ה | |
| ואין דעתך | |
| אין דעתינו ל | |
| מיודעים | |
| knowledge | ידיעות |
| דעת, דעתי, מדעתי, מדעתנו | |
| ידע יודע ה | |
| אשר לא יודע | |
| it is known | בידוע ש, וידוע הוא כי, וידוע כי, ידוע הוא ... כי |
| י.ע.ד. | |
| to designate | ייעדתי ל, ייעדנו |
| י.ע.ל. | |
| benefit | תועלתם |
| to be useful | יועיל |
| beneficial, useful | מועיל ל, מועילים ל |
| to apportion | להועיל ממנו ל |
| י.צ.א. | |
| to result | יצא (ה / כ / ל / לך / תחת ה), יצאו (ה / לנו), יוצאות, יוצאים, תצא ה |
| יצא באחרונה, יצא לך באחרונה | |
| result | היוצא (ב / מ / מה / באחרונה / לנו), היוצאים |
| to receive, to obtain | יצא לך מ, יצא לנו (כי / ה / מזה / מזה ש / מכך כי), שיוצא ל, יצאו לך, יצאו לנו, ויצא לנו עוד מזה ש, היוצא ל |
| יצא לכל אחד מהם | |
| to be gone | יצא |
| it follows that | ויצא מזה כי |
| to become possible | יצא לנו ל |
| to derive | ממנה יצאו |
| to find out | יצא לנו ש |
| להוציאם מן הכלל, יצאו מן הכלל, ויצאו הם מן הכלל | |
| יצא כלו בהם בשוה | |
| לצאת | |
| כמה פעמים יצא (מה / ה... מה) | |
| to actualize | להוציא |
| י.צ.ע. | |
| to explain, to introduce | להציע, אציע ש, הצענו |
| premise | הצעה, הצעות |
| י.ר.ד. | |
| to lower | להורידם, הורדנום (ב' מעלות), מורידים (אותם), מורידין ה, נוריד, נורידנו, נורידם (מעלה אחת), תורידם (מעלה אחת) |
| lowering | הורדה, הורדת מעלה, הורדתם |
| to be lowered | יורדו, ירד |
| to decrease | יוריד, מוריד |
| אינו מעלה ומוריד | |
| י.ת.ר. | |
| to remain | נותר (דבר) |
| remainder | הנותר, הנותרים (מה) |
| כ.ל.ה. | |
| end, complete | כלה... ה, כלה ה, יכלה ה, יכלו (ה), יכלו ב, יכלו מה, כלו ה, כלו אלו ה |
| תכלה המנין | |
| כלות ה, ככלות ה | |
| עד כלות ה, עד כלותם, עד כלותו | |
| כלינו מעשינו | |
| כלית כל מלאכתך, כלית כל מלאכתך על השלמות | |
| כלינו כל מלאכתנו, כלינו מלאכתנו מכל וכל | |
| to be gone | כלה כל ה |
| to eliminate | לכלות |
| כ.ת.ב. | |
| to write | אכתבנו ב, כתבתי (עליה), כתבתיו |
| to be written | נכתב ב |
| כמו שכתו' למעלה | |
| ל.ו.ה. | |
| to loan | לוינו האחד, נלוה אחד מ, נלוה אחד מה, נלוה א' מ, תלוה אחד מה |
| אשר ממנו לוית האחד, אשר ממנה לוית האחד | |
| זה האחד אשר לוית | |
| היות לווה ממנה | |
| ל.מ.ד. | |
| learning | בלומדי, לומדם |
| to learn | ללמוד ב, להתלמד, תתלמד |
| to teach | ללמדך על |
| ל.ק.ח. | |
| to take | בקחת ה... מה, בקחתך (אותם ל / ה... ל) |
| ליקח (... מה), לקחת (אותם מ / מן ה / מה... במקומם / מה / מהם / ממנה / משם / ה... מ / ה... מה / ה... מהם / ... מה / ל / בעבורה), לקחתו (מ) | |
| יקח (ה / מ / כל אחד מה), יקחו (מ / מה) | |
| לוקח (מה / משם), לוקחים משם | |
| לקח (ה / מה / ה... מה), לקחו (ה / כל ה / כלם / ממנה ה / ה... מה) | |
| לקחנו (... ל / ... מהם / ה... ל / ה... מ / ה... מהם / משם ל) , לקחנוהו ל | |
| נקח (ה / כל ה / ל / לו מה / מ / מה / מהם / מהם ה / עוד / ...ה / ה... מה / ... מה / ... מהם / משם ה / משם... ל), ניקח מ | |
| נקח (בעבור / בעבורו / בעבורם / בעד זה), נקחם מה, נקחנו בעצמו ל | |
| קח (ה / ... מה / בעבורו), קחתנו (אלו ה / ממנו), תקח (בעבורו / ה... ל / ... ל), תקחנו ל | |
| to be taken | ויקחו מ |
| אשר יש לנו לקחת משם | |
| מקום לקיחתם | |
| to consider as | לוקחים ... לאחדים |
| לקחת עמו ה | |
| מ.נ.ה. | |
| to count | מונה, נמנה מה, תמנה (מ / מה / משם) |
| מ.צ.א. | |
| to find | למצוא ב |
| מוצא, מצאנו (שם / תחתיו), מצאנוהו, מצאת (ב / ה / לו / מ / שם), מצאתו, מצאתם, מצינו | |
| נמצא (לו / ש / שם / תחת / תחתיו), נמצאנו שם | |
| תמצא (ב / לפניו / עליהם / על ראשו / תחתיו / לו / שם / ... תחת ה), תמצאם, תמצאנו | |
| הנמצא (ב / תחת ה / תחתיו), הנמצאת שם, הנמצאים (ב) | |
| לא נמצא ל | |
| אשר נמצא תחתיו דבר | |
| היה נמצא דבר זה | |
| אשר מצאנו ל | |
| אשר לא מצאת ל | |
| אשר תמצא אשר לפניה | |
| to be found | ימצא (על ראשו / תחת ה), נמצא (ב / תחת ה / תחתיו) |
| to be found | הנמצא ב |
| תמצא לכל אחד מהמספרים שום מורה | |
| מה שתמצא מ | |
| אשר לא תמצא לו | |
| לא תמצא שם | |
| to invent, to create | להמציא, אמציא, המצאת, המצאת ה |
| invention | המצאות |
| נ.ג.ע. | |
| to result | הגיע ל... מה, יגיע ל, יגיעו ל... מה, אשר הגיעו ל |
| to receive, to obtain | הגיע לכל א', הגיע לכל אחד מהם, יגיע לכל א', יגיע לכל אחד, יגיע לכל א' מה, יגיע לכל אחד מהם, יגיעו לכל אחד מהם, יגיע ממנו לכל א' מה |
| to reach | בהגיענו שם, בהיגיענו שם, הגיענו אל ה, הגיעו ל, הגענו (אל / ל), הגעת ל, יגיע (אליהם / ל), יגיעו ל, תגיע ל |
| נ.ו.ח. | |
| to place, to put | תניח ה... עם ה |
| to be placed | הונח |
| positioning | בהנחתם |
| placing | הנחת ה |
| נ.ש.ג. | |
| to achieve | השגנו |
| to attain | תשיגנה |
| ס.ד.ר. | |
| order, arrangement | סדור (ה) |
| by the order, successively | על הסדר ש |
| to arrange | לסדר (ה... זה אחר זה / עם), לסדרם, מסדרים, נסדר (... לפניהם), נסדרהו, נסדרם (זה על זה), תסדר ה |
| הסדר, בסדר, כסדרם | |
| על סדר שביארנו | |
| emanation | סידורה |
| ס.פ.ק. | |
| to result | נספק |
| sufficiency | ספקנו, די ספקנו |
| to be satisfied | למסתפק ב |
| ע.ב.ר. | |
| נעביר עליו | |
| to cross out with a pen | נעביר עליו הקולמוס, נעביר עליו קולמוס, נעביר קולמוס על ה, תעבור הקולמוס על ה |
| שעבר עליו הקולמוס | |
| ע.ד.פ. | |
| to exceed | יעדף ה... על ה... כ, יעדפו עליהם |
| exceeding | עודף על ה, העודף (ב... על ה), העודפים, העודפים (ב... על ה) |
| excess | עודף |
| ע.י.נ. | |
| to examine | לעיין, נעיין (אם), עיין (אם / ה), תעיין |
| consideration | עיון אל |
| study | עיון |
| ע.ל.ה. | |
| to result | יעלה (כ / ל / הכל / מ / ש), יעלו, עולים (ל), עולה (ה / הכל / ל / מה), עלה (הכל / ל), עלו (ה / ל) |
| result | העולה (מ / מה), אשר יעלה |
| העולה לכל אחד מהן | |
| עלה לנו (כל ה / מ) | |
| עלה לכל אחד מהם, יעלה לכל אחד, יעלה לכל אחד מהם | |
| יעלה בידך, עלה בידינו ש, יעלה בידינו ש | |
| כמה יעלה לכל אחד | |
| to increase | יעלה, מעלה |
| to rise | יעלה מעלה אחר מעלה |
| to reach | יעלה... ל |
| to exceed by | יעלה ... על, יעלה |
| to raise | תעלה |
| כמה ... יעלו, כמה יעלו | |
| ע.ש.ה. | |
| procedure, technique | מעשה (ה), מעשהו, מעשיך (ב), מעשינו, מעשים (ב) |
| operation | במעשיו |
| to do, to proceed | עשותך, עשותך כל זה |
| בעשותינו זה, בעשותך זה | |
| לעשות (זה), לעשותו | |
| אעשה, יעשו, נעשה (ל / לכל אחד / ממנו), נעשנו, עושים (ב), עשה, עשינו (לכל אחד מהם), עשית (ב / זה ל), תעשה (ה / ל / להם) | |
| to be done | יעשה (... ב) |
| וכן תעשה, וכן תעשה לעולם ש, ועשה כן לעולם | |
| וכן תעשה עד תומם | |
| וכן נעשה לעולם בטעם | |
| נעשה במעשה | |
| לעשות כל אשר עשינו ה | |
| לעשות מעשינו זה, עושים מעשינו, עשינו זה המעש' | |
| מעשינו ה | |
| במעשינו | |
| כמעשינו ב | |
| נעשה לזה כאשר ל, נעשה לזה כאשר עשינו ל | |
| נעשה בתוספת, נעשה בתוספת א' על | |
| נעשה להפך ש | |
| לעשות ממנו שורה אחת, יעשה ממנה שורה אחת, תעשה ממנו שורה שנית, תעשה שורתו | |
| תעשה אחד מ2 דברים | |
| עשה כדרכים | |
| כמו שעשית ל... עם ה | |
| כמו שעשינו בתחלה ב | |
| תעשה זה ( ... ל) | |
| to make | נעשה ממנו, עושה מה, תעשה ממנו |
| לעשותם חלק אחד | |
| נעשה א' שלם... חלקים, עשינו האחד השלם ... חלקים | |
| נעשה הא' השלם | |
| עשינו ... חלקים שוים, עשינו מהם... חלקים שוים | |
| עשינו אותו ...חלקים, עשינו אותם ... חלקים שוים | |
| לעשות כל שברים מהם ממין האחדים | |
| לעשות מהפרטים כללים | |
| לעשות בעד כל סיפרא מהן סיפרא אחת | |
| אעשה משל אחר (מ) | |
| צ.ו.ה. | |
| to instruct | נצוה ל, צוינו ל, ציויתי ל, ציויתיו, צוויתיך, ציויתיך ל, צויתיך ל |
| צ.ר.כ. | |
| need to | צריך (ל), צריכים (ל), הצריכים, צריכין, צריך אתה לעולם ל, הצריך להם |
| to be needed | הוצרך אליה, הוצרך מ |
| צורך | |
| לצורך (ה) | |
| should | צריך ש |
| no need | לו לצורך, אין צורך, ואין צורך אלא ש, שאין לו צורך ל |
| אין צריך כי אם ל | |
| הוצרכנו, הוצרכנו לזה | |
| יצטרך ל, נצטרך ל, נצטרך הכל, תצטרך ל | |
| אשר מהם יצטרך, אשר יצטרך מהם, אשר מהן יצטרך ל | |
| אשר יצטרך, אשר תצטרך | |
| מה שיצטרכו מהם | |
| כל מה שתצטרך | |
| ק.ר.א. | |
| to name, to call | יקראו, נקרא (ל / לו), נקראנוהו, קראנו לזה, קראנוהו, קראתי (לו), קראתיו, קרינו ... ל |
| to be called | יקרא, נקרא (ה / ... מה), נקראה (ה), הנקראים, הנקרא |
| to denominate | ותקרא לו שם מ |
| to denominate | לקרוא להם שם, נקרא להם שם |
| ק.ר.ב. | |
| to come close to, to approach | להתקרב (אל האמת / מאד), יתקרב אל (האמת), יתקרב ל... ב, נתקרבת אל (האמת), תתקרב אליו |
| to come closer to | להתקרב יותר אל (האמת), להתקרב עוד אל (האמת / השרש), יתקרב מאד מאד, מתקרב יותר, נתקרב יותר אל (האמת), תתקרב יותר אל ה |
| to be close to | קרוב אל (האמת) |
| יתקרב אל האמת לחסרון | |
| יתקרב אל האמת לתוספת | |
| ר.א.ה. | |
| to see | לראות (אם / ... ב), אראה, נראה (אם / כמה), ראה (אם / ה), ראית ... ש, תראה (ב / ש) |
| היה נראה ש | |
| to consider | ראה ל, ראיתי ל |
| ר.ח.ק. | |
| יתרחק מן האמת, יתרחק מן האמת ב | |
| רחוקו מן האמת מ, ריחוקו מן האמת | |
| difference | מרחק (... מ / ה... מה), מרחקו מ, מרחקם מ, רחוקו מ, רחוקם, ריחוק, ריחוקים, ריחוקם מה |
| difference | הרחקתם מ |
| to become distanced from | אשר נתרחקו מ, שנתרחקו מה, יתרחק, תתרחק, מרוחקת ... מה |
| distance | מרוחק ה |
| ר.מ.ז. | |
| to be determined | נרמז ב, נרמז ש |
| determined | רמוז ב, הרמוזות |
| designation | ברמז |
| ר.צ.ה. | |
| to want | ירצה (ל), נרצה (ל) , רוצה ל, רוצים (ל / ש), רצה ב, רצו ל, רצינו (ל), רצית ל, תרצה (ל) |
| מי שירצה | |
| רצונך ל, רצוננו | |
| הרוצה ל | |
| as one wish | כרצונך, כרצונו, כרצוננו |
| ר.ש.מ. | |
| written | הרשום, הרשומה |
| noted | הרשומים |
| לרשום, ארשום | |
| ורשום קו דיו תחתיהן, ותרשום קו דיו תחתיהן | |
| נרשום קו דיו עליהם | |
| תרשום קו דיו תחת | |
| נרשום תחת כל השורות קו דיו | |
| ונרשום קו על כל הנשאר | |
| תרשום קו על הכל | |
| ונרשום על... קו דיו | |
| to be marked by | ירשמו ב |
| ש.א.ל. | |
| to ask | ישאלו לך (על... ש), שאל, שאלו לך (ש), שאלו לנו (כמה / ל), שאלו (כמה), שואל, שאל השואל |
| question | שאלה, שאלתנו |
| in question, to be asked | נשאל, הנשאל, הנשאל לנו, הנשאלים, הנשאלות, שנשאל |
| ש.א.ר. | |
| to remain | ישאר (ב / ה / דבר / לנו מ / ממנו), ישארו, נשאר (ב / מ / מה / דבר / על ה / עוד ב), נשארו (ב / עוד... ב) |
| remainder | הנשאר (ב / מ / מה), הנשארים (מה), הנשארות, הנשארת |
| remainder | שארית (ה / מה), השארית הנשארה, שאריתנו |
| נשאר לנו (ב) / בידינו | |
| תשאר מל | |
| שנשארו בחלוקה | |
| to be left | ישארו לנו ל |
| ש.ג.ח. | |
| ישגיח ב | |
| להשגיח בסדורו | |
| בהשגחה | |
| observation | השגחה |
| ש.ו.ה. | |
| equalizing | השיווי |
| to equalize | נשוום יחד |
| to be equalized | הושווה (ל) |
| to be equal | שוה (ל / ממש ל), יהיה שוה ל, שוים (ה / בכל), שוים הם ב |
| השוה ל | |
| equal | שוים, שווים, שוות, שוה לכלם |
| ש.ו.מ. | |
| to place, to put | לשום (ב / ה / תחתיו), לשומו לפני ה, לשומם (במקומה / ... ב), לשים (ביניהם ה / עמהם ה / תחתנו / ... תחת), לשים במקום, לשים ... במקום על |
| to define | לשום כ ... ה, לשים אותה עצמה ל, שם ה, שמת (ה) |
| to be placed | הושמו ה... במקומם, יושם ... ב |
| defined, positioned | המושם, המושמים (ב) |
| ובהשימך (ה), להשימם תחת ה, לשום ב... ל | |
| נשים (... אחר ה / ... ב / אותם / ה / עליו / ה... על ה / לפני / לפניהם / ... לפניו / ה... לפניו / ... לפניהם / ה... לפני ה / עמהם ה / תחת / תחתיה אלו ה / תחתיו / תחתיו ה / תחתיהם / ... תחת ה / ... תחתיהם / ... תחתיו / ה... תחת ה) | |
| נשים המספרים זה על זה | |
| נשימה (תחת) | |
| נשימהו (ב / ל / עליו / חוץ ל / לפני ה / תחת ה / תחתיו) | |
| נשימם (ב / עליה / עליהם / עליו / על ה / עם / זו על זו / זה אחר זה / תחת ה / תחתיו / ה... תחת ה / מחוץ / במקומו / במקוצו עם ה / לו על זה / ... על) | |
| נשימנו (לפני / לפני ה / לפניהם / מחוץ / עליהם / עליו / על ה / תחת ה / תחתיו) | |
| נשימם ראשונה ב | |
| שים (ה / ה... ל / על / אותו תחת ה / תחת ה / תחתיו) | |
| שימהו (על ה / תחתיו) | |
| שימם ב | |
| שמים (ל / לפניהם / תחתיו / אותו תחתיו / ... תחתיו) | |
| שמנו (ב / ה / ה... לפני ה / ה... תחת ה / תחתיו / במקומו / מיד ה / זה ה) | |
| שמנוהו תחתיו | |
| שמנום תחת ה | |
| שמנוהו מעלה אחת לפניהם | |
| שמת (אותו / בהם / תחת ה / תחתיו / ... ב / שם ה) | |
| תשים (... אחר / ... אחר ה / אחריהם / ב / ב... תחת / בראש / ה... ל / ה ... על / ה... תחת ה / לפניהם / ... לפניהם / ... לפני ה / ... כנגד / על ה / עליו ה / תחת ה / ... תחת / ... תחת ה / תחתיו /תחתיו ה) | |
| תשי' לעולם ה | |
| תשים ריוח בין זו לזו | |
| תשימה | |
| תשימהו (תחת ה / תחתיו) | |
| תשימם (ב / עמהם / תחת ה / תחתיו / במקומה אחר ה) | |
| תשימנה אחר ה | |
| תשימנו (ב / על ה / מבחוץ) | |
| נשים ... בעד ה... במקומה | |
| נשים ה... למקומה, נשים במקומו, נשים ... במקומם | |
| נשים ...ל... במקומה | |
| נשים נקודה (על / על ה) | |
| נשים נקדה תחתיה, ונשים תחתיה נקדה | |
| נשים נקודה מחוץ במקום ה | |
| שים נקדה אחת על ה | |
| ותשים עליה נקודה אחת בעדו | |
| ושמת שם נקודה | |
| ותשים ... אחת תחת הקו בעד ה | |
| ותשים נקדה אחת (עליה / תחת ה) | |
| תשים תחתיה נקדה, תשים תחתיה נקודה | |
| תשימם לשארית תחת הקו | |
| תשים בעד כל סיפרא שב | |
| לשים ה... בסדר | |
| אשים להם סדר | |
| ישים ... זו על זו על הסדר | |
| נשימם על הסדר, נשימם ... על הסדר זה אחר זה | |
| נשימם זה על זה על הסדר, נשימם ב... זה על זה על הסדר | |
| שמנו ה... כזה הסדר | |
| תשים ה... זה על זה על הסדר | |
| תשימם על הסדר | |
| to denote | שמתי להם |
| ש.כ.ח. | |
| to forget | תשכח (מ), תשכחהו, תשכחם |
| to be forgotten | (שלא) ישכח |
| examine it carefully | דוק, דוק ותשכח |
| ש.מ.ר. | |
| keep | נשמור, שומר ש, שמור (ה / ... על ה), תשמור (ה / ה... ל) |
| השמור, השמור בידינו מה, השמורים | |
| תשמר (ה... ל) | |
| נשמר ה... לאחדים בידינו | |
| תשמרם לאחדים ל | |
| לשמור משמרתי | |
| to beware, to be careful | שמר, השמר לך, שמור נפשך |
| ת.ח.ל. | |
| to begin, to start | להתחיל מה, אחל ל, נתחיל (ב / ל), תתחיל (ל / מה) |
| מתחילות מה | |
| וקודם התחילי ב | |
| יתחיל ה | |
| beginning | התחלת, תחלת ה, בתחלת ה, מתחלת ב |
| ותשרט קו דיו על | |
| כפי המזדמן | |
| כאשר הזדמן, כאשר יזדמן | |
| שעלו בידך משום מעלה | |
| תחוש (ל / להם), אל תחוש ל, לא נחוש ל | |
| אינך צריך לחוש מה | |
| to be | היות (ב / ה), היותם |
| to be | להיות (ה / ל), להיותה, להיותו ב, להיותם, היותך, בהיותך, היותם, היה, היו, היינו, הינו, שהיו, יהיו (ה), יהיה (ב / ה / כ), תהיה, היתה, יהא |
| were it | ההיתה |
| אינך | |
| to become | יהיה (ל / ב... ל / ל... על ה / לנו ל), יהיו (ל / לנו ל), הוא ל |
| to become | היה בידך ל, יהיה ל... בידך, היו ל... בידך, יהיו ל... בידך |
| אשר הם ל... בידך | |
| to have | ולהיות לנו ב, היה ל, היה להם, היה לו, היה לנו, היו ל, היו לך, היו לנו, יהיה לו, יהיה לך, יהיו להם, יהיו לו, יהיו לך, יש ל, יש לה, יש לו (ה), יש לך, יש לנו |
| owner of, having | בעל (ה / אלו ה) |
| to have | אשר להם, אשר לו, אשר לך, אשר לנו |
| בידינו, בידך | |
| אשר היו בידו, אשר בידו | |
| יש בידיך, יש בידך ה, יש בידינו, יש בידינו מ | |
| שיש בידינו | |
| שבידך, שבידיך, שבידינו | |
| אשר בידך, אשר בידינו, אשר היו בידינו | |
| כל אשר בידינו | |
| להיות בידינו ה | |
| היות בידך, היות בידינו | |
| היה בידך ה, היו בידיך, היו בידך, יהיו בידך | |
| היה בידינו, היה בידינו ה, היו בידינו | |
| בידיך ל, בידינו ל | |
| כאלו היו בידינו | |
| כאלו יש לנו בידינו | |
| והנה עלה בידינו ש | |
| not having | אין ל, איננו לו |
| אין לו | |
| אין בידך מאומה | |
| אין שם דבר | |
| to discuss | אדבר בזה ב |
| name | שם (ה), שמות, ששמה |
| to explain | להטעים |
| reason | טעם (ב / ה / בזה / כי / ש / כל ה), טעמי', טעמו ב, טעמיהם |
| by reason | בטעם |
| reason | לסבה (ה / ש), בסבת |
| proof | מופת, מופתי (ה / כל), מופתיהם, מופתיו |
| example | דמיון, דמיוננו, דמיונות |
| example | משל (ב / ש / בזה / לזה ב / על / על ה), משלים, משלי (ה), משלינו (ה), במשלנו, כבמשל, כבמשלנו, כמשלינו זה ש |
| for example | ועל דרך משל, על ד"מ |
| to give example, to demonstrate | אביא משל (... ל), אביא ... משלים, אמשול, אמשול משל ל |
| form, diagram | צורה (ה), צורות, צורת ה, צורות מספרים |
| figure | צורות |
| בא בזאת הצורה, בא בצורה הזאת | |
| זאת הצורה ה | |
| כפי צורה זו, כפי הצורה, כמו שהוא בצורה הזאת | |
| בצורה (ה / הזאת / הנזכרת) | |
| diagram | בתמונה |
| to answer | יענו כי |
| to answer | ולהשיב... ל, תשיב ... ש |
| answer | תשובה |
| משפט אחד להנה | |
| method, way | בדרך (ה / ש), ובדרך זה, דרכים, דרכי ה, דרכם, כדרכו ב, כדרכנו, על דרך ה, ע"ד |
| על הצד ש | |
| to proceed, to walk | דרכתי, נדרוך ב |
| to go, to proceed | בלכתך ל, ללכת, לך אל ה, נלך ל, תלך ל |
| skill, procedure | מלאכה, מלאכתך, מלאכת ה, מלאכתינו |
| action, operation | פועל |
| to repeat the procedure | להכפל (ה / זה ה), להכפיל (המעשה / המעשים) |
| המכפיל פעמי המעשה | |
| repetitive procedure | בהכפל (ה) |
| end, complete | תמו כל ה |
| explanation | פי' ה |
| explained | מפורש ב |
| to become | עולה לעשר מאשר לפניה, עולה עשר ידות מאשר לפניה |
| בערך אל אשר לפניה | |
| בערך אשר לפניה, בערך אשר לפניו | |
| to be able to | יוכל ל, יוכלו ל, יכולים ל, יכולנו ל, נוכל ל, תוכל ל |
| as much / great as possible | היותר שנוכל, היותר שתוכל (ל), היותר ... שיוכל |
| to be able to | היה הרשות בידך ל |
| should | אשר לך ל, אשר לנו ל, היה לו ל, היה לך ל, היה לנו ל, יהיה לו ל, יש לו ל, יש לך ל, יש לנו ל, עליך ל, עלינו ל |
| should not | אין לנו ל, אין לנו עוד ל, אין לנו ל... כלל, אין לנו ל... כי אם |
| should | ראוי ל, ראוי לו ל, ראוי לנו ל, ולזה ראוי לנו ל |
| should be | ראויין להיות |
| לא תחדל מ | |
| ומהכל | |
| ויהיה לעש' במעלה זו | |
| ואם במקום הנקודה | |
| ואם לא יהיה במעלה שאחר שום מספר | |
| דבר שכלתה כבר ה | |
| מהמעלה אשר הנקדה תחתיה אם יש שם מספר | |
| שכבר נשלם | |
| במעלה שאחר זו | |
| תשוב כבתחלה | |
| באמצע | |
| ? והנה בא על מתכונתו ש | |
| to become | וישוב |
| beginning | ראש ה, בראש זה ה |
| end | סוף ה, בסוף ה |
| end | תכליתם |
| הדומה ל, הדומה לה, הדומה לו, הדומה להם, הדומה לזה | |
| כדומה לו, כדומה לזה | |
| ודומיהן | |
| כאלו דומים ל | |
| by how much | בכמה הוא |
| כמה פעמים יש | |
| כמה כפלי כפלים | |
| כי כבר נשלם | |
| נשלם | |
| נשלמה | |
| כמה שיהיו מהם | |
| כמה... הם / כמה ... הן / כמה הם | |
| כמה ... יש ב, כמה ... יש בהם | |
| כמה הוא ה | |
| בכמה יהיו | |
| בכמה | |
| כמה הם יותר | |
| כמה פעמים ה... ב | |
| כמה פעמים יש בהם | |
| to be unknown | נעלם לנו ה, נעלם ה |
| known | הידוע, הידועים, ידועים, מספר ידוע |
| unknown | הנעלם, נעלם (ה / ממנו), הוא הנעלם, הנעלמים |
| to dictate | נותן ל |
| excess | יותר על |
| to exceed | יותר על ה |
| to exceed by | יהיה יותר על ה ... ב |
| with excess | לתוספת |
| plus | עם תוספת |
| to increase | יתרבה, יתרבה המספר ב, מתרבה (בכפל) |
| to be missing | יחסר... מה, חסר ב... מה |
| to be lessened | יחסר |
| to fall short of | היו למגרעת, יחסר |
| deficit | חסרון |
| with deficit | למגרעת |
| to continue, to keep | תוסיף ל |
| amount | מנין |
| people | אנשים, אנשי, איש |
| spacious | מרווחות, מרווחים |
| when ever | בכל עת ש |
| enough | די |
| to be enough | די ב, די ל |
| not enough | ואין די, ואין בו די, אין די ל, אין דיו ל, אין שם כדאי ל, ואין ב... כדאי ל, אין ... כדי, ולא יהיה בו די ל |
| and that is it | ודי |
| to harm | יזיק, מזיק |
| ? | בשום פנים |
| to indicate | להראות, להורות, הורה, המורה על ה, מורה לנו ש, מורי' על ה |
| ויקח ... פעמים | |
| להתחלק עליו לשלמים | |
| to discuss | נדבר |
| to bring | הבאתי ב, יגיעם כלם ל |
| הנה לנו ש | |
| to elaborate | להאריך בזה עוד, אאריך |
| to elaborate | להרחיב ה |
| to give | לתת לך, אתן לך, יתנו |
| general, inclusive | כולל |
| ancients | הראשונים |
| to consider as | הבט... כאלו |
| all are the same | כלם שוים |
| to be contained in | בו, יהיה בו ה, אשר ב, אשר בו |
| contradiction | בחילוף |
| so is | וכן הוא ה, כן הוא |
| they themselves | הם הם |
| total | היה הכל, יהיה הכל, יהיו הכל, יהיו כולם |
| in order that | יען |
| as they are | כמות שהם |
| the same as | כך הוא... כמו |
| as | כדרך שה |
| at present | אשר בנתים |
| at once | ברגע |
| with little | במעט |
| בכל עת | |
| large | הרבה |
| with respect to | על ה |
| לכופלו | |
| to be attained | שבא ב, אשר באו לך |
| to indicate | המורה על ה |
| diagonal | האלכסונים |
| difference | שינוי ב |
| to stand | עומד |
| to be difficult | יקשה עלינו |
| difficult | קשה |
| effort | עמל |
| to ease, to make it easy | להקל ה... מעלינו, להקל מעליך (ה), להקל מעלינו (ה), להקל עלינו ה |
| to assign | הקצתי לו, הקציתי לו |
| extreme | הקצוות |
| money | ממון |
| golden | זהב, אלו של זהב, של זהב, מזהב |
| silver | כסף, של כסף, אלו של כסף |
| dinar | דינר, דינרי, דינרים |
| peraḥim | פרחים |
| zehuvim, golden coin | זהובים |
| peruṭot | פרוטות |
| zuzim | זוזים |
| disappeared | נעדרת |
| בכל מאויו | |
| באחד המעשים ב | |
| to require | יחייב |
| given | מונח ל |
| side, factor | צלעות |
| generation | הולדה |
| meaning, instruction | הוראה, הוראת |
| indicator | מורי |
| ומ"מ | |
| as necessary | בכל הצורך |
| too much | יותר מדאי |
| portion of | קצת ה |
| smaller portion | מעוטו |
| greater portion | רובו |
| portion | חלקי ה, חלקים |
| to hurry | חששתי ל |
| to investigate | לדקדק, דקדקתי |
| pedantic | מדקדקים |
| matter | דברים |
| thing | דבר, דברים |
| any thing | שום דבר |
| issue, matter | ענין, ענייני ה, עניינים |
| concerning, in the matter of | בענין ש |
| type | מין (ה / מה מ), מינים, מינה, מינו, מינם, מיניהם, מיני |
| שהוא ממינו, אשר ממינו | |
| שאינו מינו | |
| מין בשאינו מינו | |
| מין על מינו | |
| to be eliminated | יתבטל |
| to wonder | תתמה ש |
| to remove | הסרנו אותו מהם |
| change | שינוי ביניהם |
| no difference between | אין חלוף בין ... ל |
| forward | בקדימה |
| backward | ואיחור |
| intention | כונה, כוונות, כונתינו, כוונתי, בכוונה מכוונה |
| lowest | הגרועים מהם |
| detail | פרט |
| gleanings? | עוללות |
| beauty | היופי |
| true | האמיתי, אמיתיות |
| ואותו | |
| ועל דרך היופי | |
| to be difficult | תכבד ה |
| work | עבודה |
| האמרה | |
| plus | עם ה, ועוד (ה) |
| like | כעין |
| descendant | ילדתם |
| to change | לשנותו |
| נחבר ב | |
| to consist of | הורכב מה |
| to be composed | הורכב, הורכבה מהם |
| to insert | להכניס |
| to be absent | יעדר |
| high | גבוהים |
| to be verified | נתאמת |
| truth | אמת |
| הנה אמת, הנה אמת הנה נכון, הנה נכון הנה אמת | |
| והנה כל מעשינו אמת, הנה כל מעשינו אמת ויציב, הלא מעשיך אמת ויציב, הנה מעשיך אמת ונכון | |
| כל מעשינו בצדק ובמשפט | |
| false | שקר |
| absurd | שקר |
| rule | דין, הוא הדין, כלל (ה / ... ל / כי / ש) |
| the rule requires | היה הדין נותן |
| to change | משתנה, ישתנה |
| time | פעם, פעמים, פעמי ה |
| כפעם בפעם, פעם בפעם | |
| every time | בכל פעם, בכל פעם ופעם |
| time after time | פעם אחר פעם |
| greatest number of times | מספר הרב הפעמים, היותר פעמים מב, יותר רב פעמים |
| to split | שנפצל מ, מ... נפצל |
| to bother | תטרח |
| deduce from this | והקש על זה, והקש על זה ב |
| to happen, to occur | יקרה (ב / כאשר) |
| נביאנו | |
| אינו כלל | |
| ?זה פירושם | |
| שבא הכל כאלו | |
| for panic | לבהלה |
| for naught | לבטלה |
| to fill, to fulfill | למלאת, מלאתיו, תמלא |
| to satisfy | למלאת את |
| to train | להרגילך ב, להרגילך עוד ב |
| style, form | בסגנון |
| removed | המוסר |
| מספרו | |
| one and the same | והנה כל ה... אחד, אחד בעצמו |
| והכל עולה לדרך אחד | |
| והכל עולה לסך אחד | |
| והכל עולה לענין אחד | |
| to shorten | ולקצר |
| to contrive | לתחבל |
| half | חצי ה, חצי מ, חצאין, חצאי, חציו, מחצית ה |
| to reverse | בהפוך ה... ל, הפוך ה |
| to return | חזרו ל, תחזור ל |
| to return | להחזירו ל |
| to return | נשוב (ו / ל / עוד), תשוב (ל) |
| meaning | כלומר ש |
| meaning | פי' (כי / ש) |
| wise | חכם, חכמים |
| Sages of the Gentiles | חכמי הגוים |
| science | חכמה, חוכמות, חכמתם |
| mathematics | חכמת הלימודיות |
| natural science | חכמת הטבע |
| arithmetic | חכמת המספר, במספר |
| philosophers | מתפלספים |
| rational soul | נפשם משכלת |
| rationalism | התבוניות, תבונה |
| rational concept | המושכלות |
| intellect | שכלו, שכלי, שכלנו |
| subject | נושאיהם |
| essence | עצמים |
| accident | מקרים |
| straightness | יושר |
| a while | כמה |
| to reveal | לגלות |
| treasures | מצפוניה |
| to influence | תאצילני |
| to translate | להשיבו ב |
| to elaborate | בהרחבת |
| to long | חשקתיך |
| desire | תאותך |
| to grant | ולתת את |
| request | שאלתך |
| trouble | טרדות ה |
| to allow | מסכימות ל, מסכימים |
| to engage in | להתעסק ב |
| to live | יחיו |
| in the eyes | בעיני, בעיניהם |
| master | אלופים |
| possible | איפשר |
| heart | לבם |
| attached | נקשר |
| succeed | יכשר |
| to rule over | להשתרר |
| to overcome | ולנצח |
| time | זמן |
| years | שנות |
| youth | זמן הנערות |
| maturity | זמן הבחרות |
| less and more | בפחות וביתר |
| senseless | תפל |
| defect | דופי |
| mockery | התול |
| slander | לעז |
| to ridicule | לעגו |
| to joke | יתלוצצו |
| to be told | יסופר |
| engagement | עסקם ב |
| simple things | פשוטות |
| perceptible | מושגות ב |
| outstanding, prominent | מסויימים |
| to encamp | חונים |
| division by division | דגלים דגלים |
| path by path | שבילים שבילים |
| wanderer | נעים ונדים |
| to sway | מתנודדים |
| to rob | יגזלו מ |
| to steal | יגנוב ה |
| to deceive | להונות, יונה |
| to exploit | ולעשוק |
| poverty | עוני |
| glory | הודם |
| might | מאודם |
| business | מסחרים |
| to eat | יאכל |
| word | דברו |
| to strengthen | להחזיק |
| to encourage | החזיקו יד |
| to grasp, to take hold | החזיקתני |
| strong | חזק, חזקות, חזקים |
| to be held | מוחזקות בידיהם, ומוחזק |
| to raise up | להקים |
| guilt | אשם |
| to be careful | נזהר מ |
| pegs | יתדות |
| to emanate | אצל |
| friend | רעהו |
| to choose | בחר מ |
| bad luck | רוע מזלו |
| to be well with | טוב לו |
| to continue | ולהתמיד |
| righteousness | צדקו |
| to carve | לחקוק אותו |
| forever | לנצח |
| affection | וחבתן |
| to overpower | גברה |
| to tearing down, to break | פרצתי |
| definition | גדר ה |
| humility | ענוה |
| to stand | קמתי בפניהם |
| to give honor to | אחלוק הכבוד |
| to be enriched | להעשר |
| to compose | חברתי |
| goodness | טוב |
| to weaken | מחלישים |
| to come | באתי ל |
| wonderful and fearful things | נפלאות ונוראות |
| lights | אורות |
| to inform | יודיעו |
| argument | טענות |
| apology | התנצלותי |
| virtue | מעלה |
| to leave | עזב את ה |
| queens | גבירות |
| to expand | מרבות |
| boughs and branch | סנסנים ופארות |
| fruit | פירות |
| extended his hand | פשט ידו ב |
| humiliating | כמבזה |
| to grasp | אחז את |
| hidden | צפון |
| concealed | נעלם |
| to admit | יעיד על עצמו |
| to admit | להודות כי |
| today | היום |
| to estimate | אומדים |
| foundation | יסוד, יסודה |
| building | בניינה, בניינם |
| consists of | הבנויות על ה |
| room | חדרים |
| to open | לפתוח |
| entrance | פתח |
| lenient and stringent | קל וחומר |
| right | נכונים |
| known, recognized | מפורסם, מפורסמים |
| to rely | נשענים |
| pronoun | |
| אני, אנו, הננו, אתה, הוא (ה), היא, הם (מ), המה, הן, הנה | |
| והוא גם הוא | |
| אותו ה, אותה ה, מאותה ה | |
| ההיא (בעצמה), ההוא, ההם, ההן | |
| which are / is | שהוא, שהיא, שהם, שהן, שהוא ה, והוא ש, שהנו |
| שיהיו שם | |
| שזהו | |
| זה (ה / הוא / ש), זו (ה), זאת (ה / היא), וזהו, וזוהי | |
| זה... וזה | |
| אלו (ה / הם / הם ה / אשר / ש), אלה, האלה, האלו | |
| of these | מאלו, מאלו ומאלו |
| of them | שבהם, מהם, מהן, מאלו, ממנו |
| הוא ב | |
| כזה | |
| מזה ה | |
| לזה, לזאת | |
| אשר | |
| אשר הם | |
| itself / themselves | עצמה, עצמו, עצמם, בעצמה (ה), בעצמו, בעצמם |
| by itself | בפני עצמו, לעצמו |
| by our selves | בעצמינו |
| your self | בעצמך |
| any, certain | שום, שום ה, משום |
| certain | איזה |
| certain | מה |
| every one | כל אחד (ש) |
| every | כל |
| every… of them | כל... מהם |
| every thing | כל דבר, הכל |
| some thing | דבר מה |
| all | הכל, כל (ה), כל אשר, כלם, כל זה, כולה, כלה, היה כלה, בכל, יהיו כלם, כולם |
| all of | כולם מה |
| each | כל, כל ... מהם, כל ... ו... מהם, בכל ... ו... |
| כ"א, כל אחד, כל אחת, כל א' (מ), כל אחד מ, כל אחד מאלו ה, כל אחד מה, כל אחד מהם, כל אחת מהם | |
| one of | אחד (ה / מ / מה / מהם), א' מה |
| for each | על כל, לכולם |
| by each other | זו בזו |
| to each other | זה לזה |
| one after the other | זה אחר זה |
| for each | לכל |
| וכל ש | |
| the rest | שאר (ה), כל שאר ה, כבשאר ה |
| both | שניהם, שתיהן |
| או | |
| גם, וכן, וכן כולם, וכן כלם, וכן לכלם, וכן בכללן, וכן ב | |
| גם ה... גם ה, הן ... הן, הן ל... הן ל, הן מן .... הן מן ... | |
| בין ש... או ש | |
| בין... בין אם | |
| בין ... או | |
| או ... או | |
| הן ... או | |
| בה, בו, בהם, בכלן | |
| זה אשר | |
| אשר ב, יהיה ב, אשר בו (ה), אשר היו ב | |
| אשר מה | |
| בזה... ובזה | |
| which | איזה ... הם, איזהו ה |
| by which | ממנו |
| from which | אשר ממנה, אשר ממנו |
| that | מה ש |
| that | הוא ש, הוא אשר |
| what | מה ש |
| who | מי ש |
| whichever | איזה מהם שיהיה |
| זה בזה | |
| which | איזה, אי זה |
| which is | שזהו |
| which is | והוא כי |
| which is of | שהוא מ |
| the same as | הוא כ |
| כמי ש | |
| כי כך הוא | |
| והנה, הנה, הנה ה | |
| הנה ש | |
| by this | בזה |
| one by one | אחד אחד |
| negative clause | |
| without | בלי, מבלי, מבלי... כלל, בלי... כלל |
| without | מבלתי (ה), בלתי |
| בלא | |
| not | בלתי |
| בלתי ... כלל | |
| אין ... דבר | |
| אין לך ל... דבר | |
| אין בה... כלל כי אם | |
| אין ... כלל, אין... כלל כי אם ה... לבד | |
| אינו כי אם | |
| אין... כי אם, אינו... כי אם, אינך... כי אם | |
| אין ב... כי אם; אין שם ... כי אם | |
| אין אנו ... אלא | |
| אין אנו... כלל, אינך ... כלל | |
| אין לו, אין לו... כלל | |
| אין לו כי אם | |
| אין לך... כי אם | |
| אין בידיך כי אם | |
| אין בידיך ל | |
| אין בידינו ... כלל | |
| ואין לו ל... כי אם; אין לך ל... כי אם, אין לנו ל... כי אם | |
| אין לך ... כי עם | |
| לא... כלל כי אם | |
| לא... כי אם | |
| לא... כלל | |
| לא ... לעולם | |
| לא... כל | |
| לא ... דבר | |
| לא ... מאומה | |
| לא ... שום | |
| ואלו לא היה ב... כי אם | |
| אין בכאן | |
| לא... שום דבר | |
| לא... אלא | |
| אין מ... ל | |
| even not | אף לא |
| not even | אין גם |
| לא ... אפי', לא... אפילו | |
| אין ה... ולא ה | |
| אין ... לא ... ולא | |
| לא ... ולא, לא... ולא גם | |
| do not | ואל, אין, אינו, אינך |
| is / are not | אינה, איננו, אינו, אינם |
| not | לאו |
| at all | בשום פנים |
| at all | כלל |
| no, there is no | אין, אין ב, אין ה, אין זה, אין כאן ה |
| is / are not | אינם (מ), אינו, איננו |
| אין בה, אין בו, אינו ב, אינו בה, איננו בו, אינם בו, אין ... ב | |
| אם לא, ואם לאו | |
| ואם לאו לאו | |
| neither… nor | לא... ולא |
| לא היה לו | |
| אין ל, ואין לנו ל | |
| אין לו אחד מהם, אין לו שום אחד מה | |
| עוד, ועוד | |
| הרי, הרי ש, הרי לך, הרי לנו (כי / ש), והרי, שהרי | |
| הרי הוא כאלו, הרי זה כאלו | |
| כי אם | |
| ככה | |
| כמה | |
| indeed | אכן |
| ככה | |
| בעבור ה | |
| שאינו ראשון, שאינו ראשון לו | |
| בעת ההיא, בפעם ההיא | |
| הם מ | |
| את, אותם | |
| Prepositions | |
| after | אחר (ה), אחר ש, אחר אשר, אחרי, אחרי אשר, אחרי ש, שאחרי ה, אחר זה, אחריה (ה), אחריו (ה), אחריהם, אחריהן |
| before | לפני ה, לפניהם, לפניו (ה), שלפני ה, שלפניהם, קודם (ה / זה / ש) |
| before | טרם |
| by | והוא ב, בש, וזה ב, וזה בש, וזה יהיה ב, בזה |
| between | בין ה, בין כל ה, מה שבין ה, בין ה...ובינו, בין... ל, בין ה... וה, בין ה... להנה, ביניהם |
| among | בהם |
| with, plus | עם, עם ה, עמו, עמהם |
| שעמו, אשר עמהם | |
| bellow | למטה (מהם) |
| לשמאל, לצד שמאל (מה), לצד שמאלי | |
| הימין | |
| לימין, לצד ימין (מה) | |
| לצד ימין מהמקום | |
| above | למעלה |
| above, on | מעל ה, על (ה / הכל), עליהם, עליהן, עליו, על ראש, על ראשו, על ראשם |
| beneath | תחת ה, תחתיהם, תחתיהן, תחתיו |
| זו תחת זו | |
| out of | ומתוך |
| until | עד, עד ש, עד אשר |
| כנגד ה, כנגד אותה ה, כנגדה | |
| in | אשר היה ב, בזה |
| in | בתוך ה |
| for | בעד ה, בעדה, בעדו, בעדן |
| for | שהי' לה ל, יהיה לו ל, לו, הוא ל, הוא לו ל |
| for | בשביל (ה) |
| from | ממנה, ממנו |
| except | מלבד |
| except | בלתי ה, זולתי (ה), מזולת ה, זולתם |
| except | מבלעדיו |
| or | או |
| לבדו, כל אחד לבדו | |
| יהיה עם הכל | |
| it is all the same | הכל אחד, הכל א' |
| כולל, כולל אותם | |
| so much, so an so | כ"כ |
| ואליהם | |
| על ידי | |
| להם | |
| בכלל ובפרט | |
| אלא ש | |
| בפעם הזאת | |
| מאשר | |
| באשר | |
| corresponding | כנגד (ה), נגדו |
| for | בעד ה |
| לפעמים | |
| ג"כ | |
| א"כ | |
| i.e. | ר"ל, ר"ל ש, ר"ל כי, ר"ל אשר |
| ר"ל ע'ד'מ' ש | |
| עד"מ, ע'ד'מ' | |
| etc. | וכו' |
| all the more so | כ"ש |
| also | ג"כ |
| adjectives | |
| many | רבות, רבים |
| many | הרבה |
| numerous | רבים |
| more than | רבים מ |
| few | כמה |
| few | קצת |
| few | מצומצמות |
| האחד, האחת | |
| אחרון, אחרונה, אחרונים, אחרון ל | |
| other | חבירו, חבירתה, חבר, חברותיה, חבריהם, חברתה |
| other | אחר, אחרת, אחרות, אחרים |
| other | זולתו, זולתם |
| better that | וטוב ש |
| more | יותר |
| greater, more than | יותר (מ / מה / ממנו) |
| much greater | הרבה מאד יותר מה |
| great | רב, הרבה |
| greater | רב, רב מאד מה, רב (מה / ממנו), מספר רב, המספר הרב |
| great/greater | הגדול (ב), הגדולה, גדולה (מה), גדולים (מהם), הגדולים |
| גדול (מ / מה / מהם / ממנו), גדול ה... מה | |
| greatest | גדול שאיפשר, הגדול שאפשר, הגדול שאיפשר, היותר גדול שאיפשר |
| היותר שאפשר, היותר שאיפשר, היותר שאפש' מ | |
| יותר גדולים, יותר גדול מה | |
| small | מעט, מועט |
| little | הקטן |
| smaller | קטן, קטון, קטנה, קטן (מ / מה / מהם / ממנו), קטנים, קטן במנין |
| smaller | מעט, המספר המעט |
| smaller, less than | פחות (מ / מה / ממנו), הפחות מה |
| inferior | שפל |
| upper | עליון, עליונה, עליונים |
| bottom | תחתון, תחתונה, תחתונים, תחתונות |
| last | אחרון (שב), אחרונה, אחרונים, אחרונו' |
| first | קודם |
| first | ראש |
| first, former | ראשון (מאלו / מה / מן / מן ה / שב), ראשונה (מה), המספר הראשון, החשבון הראשון, ראשונים, הראשונים |
| second | שני (ב / ל / לו / מאלו / מה / מן / שב), ב' מאלו, שנית (מה) |
| third | שלישי, שלישית (ל), שלישיים ל |
| fourth | רביעי, רביעית |
| fifth | חמישית |
| sixth | שישית |
| prior | מוקדם |
| latter | מאוחר |
| preceding | העובר, העוברים, אשר לפניו, הקודמת, הקודמים |
| preceding | אשר לפני (ה), אשר לפניו, אשר לפניה, אשר לפניהם, שלפני זאת, שלפניו, שלפניהם |
| אשר לפני פניו | |
| לאשר לפניו ולפני פניו | |
| preceding, previous | הקודם (לו / לזה), קודם, הקודמת, קודמת (ל), קודמים |
| previous | שעבר |
| next to | סמוך, הסמוך ל, הסמוך להם, הסמוך לו |
| following, succeeding | הנמשך, הנמשך אליו, הנמשכת ל, נמשך אחר הנמשך |
| following | הבא אחריו, הבא אחריהם, הבא אחריהן, הבא אחר זה, הבאה, הבאה אחריה, הבאה אחריהן, הבאה אחר ה, הבאים, הבאים אחריה |
| שאחר ה | |
| העולה | |
| itself | בעינו, בעינה |
| very, itself | ממש |
| other, another | אחר, אחרת, אחרים |
| others | אחרים, האחרות |
| short | קצר, קצרה |
| correct | נכון, נכונה |
| correct | אמת |
| various | שונים |
| indifferent | בלתי שונים |
| different | משונים, שונים |
| corresponding | אשר כנגדו |
| corresponding | בת גילה |
| worthy of | ראוי ל |
| appropriate | הראוי ל, הראויה ל, הראוי לו, הראויה לו, הראויות להם |
| appropriate | נאות, נאותים, נאותות |
| new, renewed | חדש, חדשים |
| absolute | גמור, גמורה |
| well versed in | בקי ב |
| entitled | רשאי |
| easy | קלה |
| necessary | מחוייב |
| given | מונחים |
| important | נכבד |
| beautiful, proper | יפים |
| nice | יפים |
| nice | הנאה |
| thin | רזה |
| poor | דלה |
| despicable | בזויה |
| despicable | נקלה |
| included | נכלל |
| whole | כולו |
| whole | השלם, שלם, שלימה, שלמה, שלמות, שלמים |
| the whole | כל ה... בכללו, בכללו |
| possible | איפשר |
| impossible | בלתי איפשר, נמנע |
| adverb | |
| there is/are | יש, יש ב, יש... ב , יש ב... ה, יש בכל ה, יש שם |
| from there | משם |
| vice versa | בהפך, להפך ש |
| again | שוב |
| little | במעט, המעט ה, מעט, במיעוט |
| there | שם (ה) |
| here | כאן, בכאן |
| now | עתה (ש) |
| so far, until now | עד הנה |
| at the beginning | מתחלה |
| in the middle | באמצע |
| at first | ראשונה |
| approximately | בקרוב |
| already | כבר |
| also | גם |
| downward | ולמטה |
| upwards | ולמעלה, למעלה ממנו |
| et cetera | וכיוצא בזה |
| successively | זה אחר זה |
| precisely | עין בעין |
| perfectly | על השלימות |
| properly | יפה יפה |
| vaguely | בסתם |
| truly, really | באמת |
| truly, really | ממש |
| clearly | ברור, בברור |
| closely, carefully | הטב |
| inversely | יהיה להפך |
| immediately | מיד |
| correctly | כתקנה, כתקנם, על היושר |
| equally | בשוה, שוה בשוה |
| briefly | בקוצר |
| surely | הלא, הלא הם, הן |
| so on endlessly | כן לעולם, וכן לעולם, וכן יהיה לעולם |
| only | רק |
| only | לבד |
| only | כי אם |
| alone | לבד, לבדה, לבדם |
| together | יחד, ביחד, כלם ביחד, כל ה... יחד |
| very | מאד |
| even | אפי' |
| even if | ואם, אף אם |
| instead | תחת, תחתיו |
| instead | במקום (ה), במקומה, במקומו, במקומם |
| such as | כגון ש |
| as | כמו (ה / ש / שה / שהוא ה / שהן); כמוה, כמוהו, כמוהם, כמונו, כמותם |
| כמות (שהם / שהן); כמותה, כמותו | |
| אשר כמותו, שכמותו | |
| as | כפי (ה / ש / שהם) |
| as | כאשר |
| as much as | כל מה ש (... יותר) |
| so | כן, וכן |
| so | כך הוא (ה) |
| always | לעולם |
| ever | בעולם, לעולם |
| henceforth | מכאן ואילך |
| then | ואז, אז |
| then, afterwards | ואחר כך, וא'ח'כ', אח"כ |
| afterwards | אחר כן, אחרי כן, ואחר |
| further | עוד |
| furthermore | ועוד (ש) |
| therefore | ולכך, לכן, על כן |
| therefore | לזה, על זה |
| provided that, so long as | ובלבד ש |
| until the end | עד תומם |
| once | פעם אחת |
| twice | פעמי', פעמים, ב' פעמים |
| outside | מחוץ ל |
| altogether | מכל וכל |
| lastly | באחרונה |
| first, at first, firstly | ראשונה ב, תחלה, בתחלה |
| כבראשונה, כבתחלה | |
| how | איך |
| how many, how much | כמה (הם / ... הם / ... הן) |
| where | מאיזה מקום, מהיכן |
| when | בעת (ה) |
| when | כאשר, כש |
| why | למה |
| which | מאי זה, מאי זו, מאיזו |
| conjunction | |
| in order to | כדי ש, כדי ל |
| but | אבל, אין זה... אבל |
| but | אך |
| but | ואולם |
| since | וכיון ש |
| since | אכן ש |
| since | אחר היות (ב / ה / ... ב / שם), אחרי היות, אחרי היותם |
| since | להיות (ה) |
| since, because | אחרי, אחר ש, ואחרי ש, אחר אשר, מאחר ש, ומאחר שכן, כי אחר ש |
| because | זה היה ל |
| because | יען |
| because | כי, זה כי, וזה ש, והוא כי |
| since | עם היות ש |
| since | לפי ש |
| since | אחרי, אחר ש |
| as if | כאלו, הוא כאלו |
| if | אם, ואם, שאם |
| whether… or | בין אם... בין אם, בין אם... או |
| אם... אז | |
| או ... או | |
| אם... או | |
| אם ... אם, אם... ואם | |
| even though | ואם |
| lest | פן |
| Scripture and Other Sources | |
| living soul [Genesis 2, 7] | נפש חיה |
| going up and down [Genesis 28, 12] | זה עולה וזה יוריד |
| soul has longed for [Genesis 34, 8] | נפשך חשקה ב, חשקה נפשם |
| of beautiful form, and fair to look upon [Genesis 39, 6] | יפה תאר ונחמד מראה |
| when angry [Genesis 49, 6] | באפם |
| with their will they hamstrung a bull [Genesis 49, 6] | וברצונם יעקרו שור |
| gave them a rule [Exodus 15, 25] | ישימו חוק |
| his hands were in faith [Exodus 17, 12] | והיו ידיו אמונה |
| he has sinned and is guilty [Leviticus 5, 23] | יחטא ואשם |
| lie down, and none shall make you afraid [Leviticus 26, 6] | זה ישכיב וזה יחריד |
| He shall not alter it, nor change it [Leviticus 27, 10] | לבל יחליף וימיר |
| Are there few or many [Numbers 13, 18] | אם מעטים ואם רבים |
| remained alive of those men [Numbers 14, 38] | מן האנשים חיו |
| not of my own devising [Numbers 16, 28] | כי לא מלבי |
| our soul loathe [Numbers 21 5] | קצה נפשם ב |
| no way to turn either to the right or to the left [Numbers 22, 26] | ימין ושמאל אין לנטות |
| between your eyes [Deuteronomy 6, 8] | בין עיניך |
| sufficient for his needs, which he is lacking [Deuteronomy 15, 8] | מחסורך אשר יחסר לך |
| sufficient for his need [Deuteronomy 15, 8] | די מחסורו, די מחסורנו, די מחסורינו |
| birthright entitlement [Deuteronomy 21, 17] | כמשפט הבכורה |
| shall be helpless [Deuteronomy 28, 32] | אין לאל ידו |
| whose heart turns away [Deuteronomy 29, 17] | אשר לבו פונה |
| to keep His commandments and His statutes and His ordinances [Deuteronomy 30, 16] | מחזיק במצותיו ואל משפטיו וחקותיו |
| crooked and twisted [Deuteronomy 32, 5] | עקש ופתלתול |
| controlled or strengthened [Deuteronomy 32, 36] | עצורה ועזובה |
| the rock in which they trusted [Deuteronomy 32, 37] | צור בו חסיו |
| The dwelling-place of God [Deuteronomy 33, 27] | לאלוהי מעונה |
| there stood not a man against them [Joshua 21, 42] | ולא יעמוד איש בפניהן |
| The wisest of her princesses answer her [Judges 5, 29] | חוכמות שרותיה תענינה |
| whosoever is fearful and trembling [Judges 7, 3] | ירא וחרד |
| the love of his soul [Samuel I 20, 17] | אהבת נפש |
| they quench my coal [Samuel II 14, 7] | ומכבים אש גחלתי |
| beans and lentils [Samuel II 17, 28] | פולי' ועדשים |
| I have kept the ways of the Lord [Samuel II 22, 22] | שומר דרכי אל |
| go here or there [Kings I, 2, 42] | אנה ואנה |
| as a reed is shaken in the water [Kings I 14, 15] | כאשר בתוך המים ינוד הקנה |
| hopping between two ideas [Kings I 18, 21] | על שתי הסעיפים פוצח [פוסח] |
| as a lodge in a garden of cucumbers [Isaiah 1, 8] | וכמקשה המלונה |
| to increase to government [Isaiah 9, 6] | ארבה המשרה |
| as one who gathers ears of grain [Isaiah 17,5] | כמקלט שבלים |
| berries at the top of the uppermost bough [Isaiah 17, 6] | גרגרים מראש אמיר |
| two or three berries [Isaiah 17, 6] | ב' ג' גרגרים |
| thrust in a sure place [Isaiah 22, 25] | תקוע במקום נאמן |
| as with the buyer, so with the seller [Isaiah 24, 2] | כמוכרים כקונים |
| fierce people [Isaiah 33, 19] | עם נועז |
| tent that shall not fall, whose pegs shall never be moved [Isaiah 33, 20] | אהל בל יצען בל יסע יתדותיו |
| by them, they shall live, and altogether therein is the life of my spirit [Isaiah 38, 16] | עליהם יחיו ולכל בהם חיי רוח |
| Since thou art precious in My eyes and honorable and I loved thee [Isaiah 43, 4] | מאשר יקרת בעיני נכבדת ואני אהבתיך |
| Let them present their witnesses, and they shall be deemed just [Isaiah 43, 9] | יתנו עידיהם ואותי יצדיקו |
| let them hear, and say "it is true" [Isaiah 43, 9] | ישמיעו ויאמרו אמת |
| I am bereaved and solitary, exiled and rejected [Isaiah 49, 21] | סורה וגזלה גלמודה ושכולה |
| ear to hear according to the teachings [Isaiah 50, 4] | אזן לשמוע כלימודים |
| justify the righteous [Isaiah 53, 11] | צדק תצדיק |
| with transgressors he was counted [Isaiah 53, 12] | ואת פושעים לא מנה |
| choose what I desire [Isaiah 56, 4] | בחרו באשר חפצו |
| remove the obstacles [Isaiah 57, 14] | להרים מכשול |
| foolish, they know Me not [Jeremiah 4, 22] | הסכלים אשר לא ידעו |
| How do you say, "We are wise" [Jeremiah 8, 8] | ואם כה יאמרו חכמים |
| with a pen of iron, and with the point of a diamond [Jeremiah 17, 1] | בלוח ברזל בצפורן שמיר |
| the near and the far [Jeremiah 25, 26] | אם קרוב ואם רחוק |
| there is none that care for her [Jeremiah 30, 17] | דורש אין לה |
| the right of redemption [Jeremiah 32, 7] | משפט הגאולה |
| become a derision [Jeremiah 48, 39] | יהיה להם לשחוק |
| each one would go [Ezekiel 1, 9/12] | ילכו איש אל |
| and shall be as though they had not been [Obadiah 1, 16] | והיו כלא היו |
| his soul is not upright [Habakkuk 2, 4] | לא ישרה נפשך |
| as the apple of the eye [Psalms 17, 8] | בבבת עין ואישון |
| they open their lips, they shake their head [Psalms 22, 8] | יניע בראש ובשפה יפטיר |
| despised by the people [Psalms 22, 7] | בזוי עם |
| The Lord helped them [Psalms 37, 40] | יעזרם אלהים |
| their health is sound [Psalms 73, 4] | ובריא אולם |
| He humbles this one and elevates that one [Psalms 75, 8] | זה ישפיל וזה ירים |
| after the stubbornness of their heart, they might walk in their own counsels [Psalms 81, 13] | ועריהן יגזור לבם בשרירות |
| the work of our hands establish it [Psalms 90, 17] | ומעשה ידיהו כוננה |
| their help and their shield [Psalms 115, 9] | עזרם ומגינם |
| laud Him, all peoples [Psalms 117, 1] | ישבחוהו לאומים |
| thousands and ten thousands [Psalms 144, 13] | מאליפות מרובבות |
| his hope is in the Lord [Psalms 146 5] | ואשר על יי שברו |
| His wisdom is beyond reckoning [Psalms 147, 5] | ובתבונתם אין מספר |
| When it is still in its greenness, it will not be plucked [Job 8, 12] | לא נקטף עודנו באבו |
| the tents prosper [Job 12, 6] | אהלים ישליו |
| hint [Job 15, 12] | רזום ירמזון |
| breach upon breach [Job 16, 14] | פרץ על פני פרץ |
| For the years that are few will come [Job 16, 22] | שנות מספר יאתיו |
| When his desire has been filled sufficiently [Job 20, 22] | למלאת ספקו |
| is hidden from the eyes of all living [Job 28, 21] | נעלמה מעיני כל חי |
| who is a teacher like Him [Job, 36, 22] | ומי כמוהו מונה |
| bars and doors [Job 38, 10] | בריח ודלתים |
| He imparted to her understanding [Job 39, 17] | וחלק לו בבינה |
| Its ways are ways of pleasantness [Proverbs 3, 17] | דרכיה דרכי נועם |
| those who hold it fast are happy [Proverbs 3, 18] | ותומכם מאושר |
| He winks with his eyes, scraps with his feet and points with his fingers [Proverbs 6, 13] | קורץ בעיניו מולל ברגליו מורה באצבעותיו |
| of great understanding, but he who is quick-tempered [Proverbs 14, 29] | מקוצר רוח ותבונה |
| a high wall [Proverbs 18, 11] | חומה נשגבה |
| trustworthy man [Proverbs 28, 20] | איש אמונה |
| removes falsehood and the lying word [Proverbs 30, 8] | מרחקת שוא ודבר כזב |
| Many women have done valiantly [Proverbs 31, 29] | רבות בנות עשו חיל |
| built as a model [Song of Songs 4, 4] | בנוי לתלפיות |
| honey and milk are under thy tongue [Song of Songs 4, 11] | תחת לשונה דבש וחלב |
| That which is crooked cannot be straightened and that which is missing [Ecclesiastes 1, 15] | מעוות לא תוכל לתקן וחסרון |
| increase vanity [Ecclesiastes 6, 11] | הבל מרבים |
| For it is not out of wisdom that you have asked concerning this [Ecclesiastes 7, 10] | כי לא מחכמה שאל על זאת |
| for God made man straight, but they sought many intrigues [Ecclesiastes 7, 29] | והאלהים עשה אותם ישר והמה בקשו חשבונות רבים |
| advantage to one who has a tongue [Ecclesiastes 10, 11] | יתרון לבעל הלשון |
| which will succeed [Ecclesiastes 11, 6] | אי זה יכשר |
| childhood and youth [Ecclesiastes 11, 10] | ילדות ושחרות |
| listened and sought out [Ecclesiastes 12, 9] | אזון וחקור |
| knowledge and understanding [Daniel 1, 17] | במדע ובהשכל |
| when the transgressors have been destroyed [Daniel 8, 23] | להיתם פשע |
| to purify, and whiten [Daniel 11, 35] | יתברר ויתלבן, מבררים ומלבנים |
| weakened the hands [Ezra 4, 4] | מרפים ידי |
| Kohen who raises his hands [Mishnah, Berakhot 5:4] | כהן הנושא כפיו |
| regard himself [Mishnah, Pesachim 10] | ובראות עצמו |
| understand foreign languages, in that foreign language [Mishnah, Megillah 2] | ללעוזות בלעז |
| has not seen the luminaries in his life [Mishnah, Megillah 4] | ומימיו לא ראה מאורות |
| build him a house [Mishnah, Bava Metzia 8:9] | להעמיד הבית |
| the time has come [Mishnah, Tamid 1] | הגיע עת |
| a single handful does not satisfy a lion [Talmud Bavli, Berakhot, 3, 2] | אין הקומץ משביע את הארי |
| True and Trustworthy [Talmud, Berakhot 12a, 23] | אמת ואמונה |
| these and those agree [Talmud, Berakhot 36a] | אלו ואלו מודים |
| do not accept authority [Talmud, Berakhot 48a] | בלתי מקבלים מרות |
| that which is earlier is earlier, and that which is later is later [Talmud, Pesachim 6b] | להקדים את המוקדם ולאחר את המאוחר |
| the work of Heaven [Talmud, taanit 23a:3] | למלאכת השמים |
| for the sake of Heaven [Talmud, Taanit 24a] | לשום שמים |
| chops down the saplings [Talmud, Chagigah 15a] | מקצץ בנטיעות |
| standing that includes no reverence [Talmud, Kiddushin 32b] | קימה שאין בה הדור |
| to include Torah scholars [Talmud, Kiddushin 57a] | את לרבות תלמידי חכמים |
| imparted flavor derived from imparted flavor [Talmud, Chulin 111b] | בר נותן טעם |
| thanking and praising [Talmud, Niddah 31a] | מודה ומשבח |
| to prohibit or to permit [Jerusalem Talmud, Ketubot 11a] | פעם לאסור ופעם להתיר |
| even though there is no explicit proof for this matter [Tosefta, Berakhot 1] | ואם אין ראיה לדבר זכר לדבר |
| her daughter and her sister [Tosefta, Yevamot 4, 5] | בתה ואחותה |
| house be wide open [Pirkei Avot 1] | בית פתוח לרוחה |
| Some ascended and some descended [Pirke de Rabbi Eliezer 35] | אלו יורדים ואלו עולים |
| Love upsets the natural order [Bereishit Rabbah 55] | אהבה מקלקלת את השורה |
| finger joints [Bamidbar Rabbah 11] | וקשרי אצבעות |
| as a pupil before his master [Bamidbar Rabbah 20] | כתלמיד לפני רבו |
| vessels of belief [Devarim Rabbah 8] | כלי אומנותה |
| tithing by guesswork [Pirkei Avot 1:16] | לעשר אומדות |
| to clarify the uncertain [Sefer ha-Mitzvot l'Rasag, Positive Commandments 97, 15] | לברר את המסופק |
| Whosoever wants it may come [Mishneh Torah, Torah Study 3] | ויבא מי שירצה |
| says: no, when it is no, and yea, when it is yea [Mishnah Torah, Human Dispositions 5] | אומרת על הן הן ועל לאו לאו |
| the pillars having been erected [Mishnah Torah, Tefillin Mezuzah and Torah Scroll 6] | ולהעמיד עמודים |
| change their appointed charge [Mishneh Torah, Blessing 10] | וישנו את תפקידם |
| to build foundations [Mishneh Torah, Sabbath 1, 18] | לבנות יסודות |
| the law requires that [Mishneh Torah, Divorce 2] | היה הדין נותן ש |
| all kinds of seeds [Mishneh Torah, Diverse Species 1, 8] | וכל מיני זרעונים |
| known and heralded [Mishneh Torah, Repentance 4] | ידועים ומפורסמים |
| drachmas or zuzim [Maimonides on Mishnah Peah 8:5] | זוזים ודרכמונים |
| defective comprehension [Maimonides, Guide for the Perplexed, 1, 36, 5] | קוצר השגתי |
| necessary or impossible [Maimonides, Guide for the Perplexed, 2, 14, 5] | ולמחוייב ולנמנע |
| pin upon which everything hangs [Maimonides, Guide for the Perplexed, 3, intro. 4] | יתד שהכל תלוי בו |
| that relation between you and Him [Maimonides, Guide for the Perplexed, 3, 51, 6] | היחס אשר בינו לבינה |
| set us apart from those who go astray [Siddur Sefarad, Ashrei] | הבדילו מן התועים |
| delightful sapling [Siddur, Purim, Shabbat Zachor 56] | נטע נעמן |
| How many degrees of good [Pesach Haggadah, Magid, Dayenu] | כמה מעלות טובות |
| to render halachic decisions [Sefer ha-Midot, Codifiers of the Law] | ולהורות הוראות |
| to show strength [Ibn Ezra on Genesis 10:8] | להראות גבורות |
| lying in an out of the way corner [Rabbeinu Bahya, Devarim 33:4] | מונח בקרן זוית |
| ruling, self-glorification [Duties of the Heart, Sixth Treatise on Submission 10] | השררה והגדולה |
| left no manner of doubt [Sefer Kuzari 2] | והניח את הספק |
| with the help of the One who dwells in the [heavenly] abodes [Ralbag on Chronicles II 36:22] | בעזרת שוכן מעונים |
| It is whole and complete | תם ונשלם |
| Glory to God, the Creator of the world | ת"ל בורא עולם |
| Knower of the truth | יודע האמת |
| Knower of the hidden | יודע הנסתרות |
| prayer leader | ש'צ' |
| ב"ה י"ת | |
| יעקב בן החכם ר' יצחק קנפנטון, ר' יעקב קפנתון ז"ל | |
| ר' יואל ן' דאוד | |
| אוקלידס |
Appendix II: Bibliography
Jacob Canpanṭon
Castile, Spain, ca. 1430
Bar Noten Ta‛am
Manuscripts:
- London, British Library Or. 1053 (IMHM: f 5932), ff. 1r-65r (cat. Margo. 1012, 1) (15th century)
Bibliography:
- Steinschneider, Moritz. 1893-1901. Mathematik bei den Juden. Berlin-Leipzig-Frankfurt: Kaufmann, p. 186 (g99); repr. Hildesheim: G. Olms, 1964 and 2001.
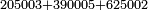
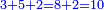
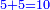
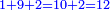

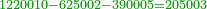
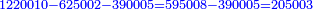
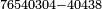

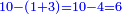
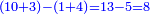
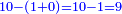
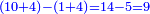
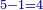
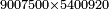

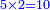
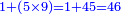
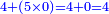
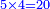
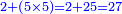

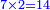
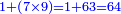
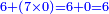
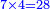




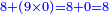
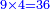
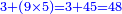
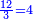
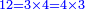
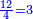
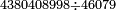


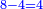
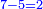


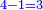


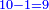
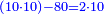

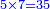
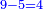
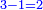

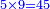
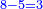
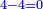
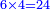
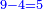


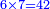

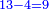
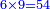


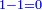
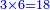

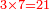
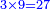



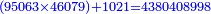
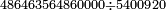
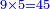




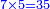

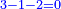
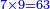


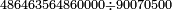
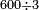

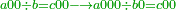
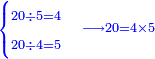

![\scriptstyle{\color{OliveGreen}{2a+10b+\left[100\sdot\left(2c-1\right)\right]\longrightarrow2a+2b+4}}](/mediawiki/images/math/1/f/d/1fd7ce989d329e647a4a3223b7dd1ee9.png)
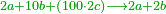
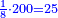
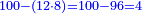
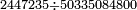



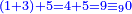

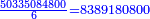


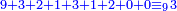

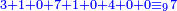

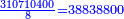


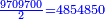
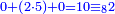
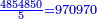
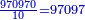
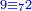
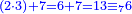
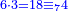
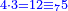
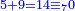
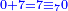
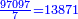


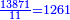
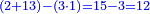
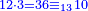


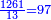
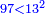
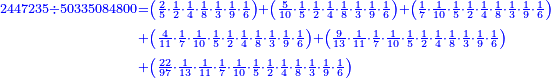
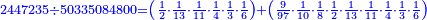
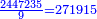
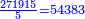
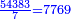
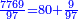
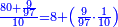

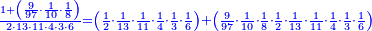
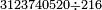
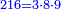

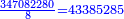
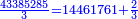
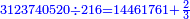

![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left(14461761+\frac{2}{3}\right)\sdot3\sdot8\sdot9&\scriptstyle=\left[\left(14461761\sdot3\right)+2\right]\sdot8\sdot9=\left(43385283+2\right)\sdot8\sdot9=43385285\sdot8\sdot9\\&\scriptstyle=347082280\sdot9=3123740520\\\end{align}}}](/mediawiki/images/math/3/a/8/3a8b9c60caa7001bf1deb73f06f47e45.png)
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left[\left(1\sdot8\sdot10\sdot97\right)+9\right]\sdot7\sdot5\sdot9&\scriptstyle=\left[\left(8\sdot10\sdot97\right)+9\right]\sdot7\sdot5\sdot9=\left[\left(80\sdot97\right)+9\right]\sdot7\sdot5\sdot9=\left(7760+9\right)\sdot7\sdot5\sdot9\\&\scriptstyle=7769\sdot7\sdot5\sdot9=54383\sdot5\sdot9=271915\sdot9=2447235\\\end{align}}}](/mediawiki/images/math/0/5/6/0564d63902ef56a5f1c7d90f83fa835d.png)
![\scriptstyle{\color{blue}{\left[\left(\frac{1}{2}\sdot\frac{1}{13}\sdot\frac{1}{11}\sdot\frac{1}{4}\sdot\frac{1}{3}\sdot\frac{1}{6}\right)+\left(\frac{9}{97}\sdot\frac{1}{10}\sdot\frac{1}{8}\sdot\frac{1}{2}\sdot\frac{1}{13}\sdot\frac{1}{11}\sdot\frac{1}{4}\sdot\frac{1}{3}\sdot\frac{1}{6}\right)\right]\sdot50335084800=2447235}}](/mediawiki/images/math/4/c/1/4c138e4386a7850c09df0f261476eb5a.png)
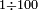
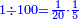
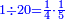
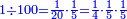
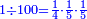

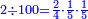
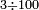
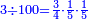
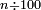
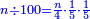
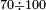
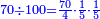
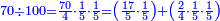
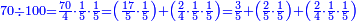


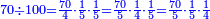
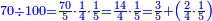
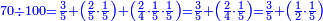
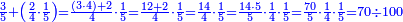
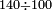


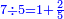
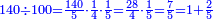
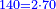
![\scriptstyle{\color{blue}{1+\frac{2}{5}=\frac{7}{5}=2\sdot\left[\frac{3}{5}+\left(\frac{2}{4}\sdot\frac{1}{5}\right)\right]=2\sdot\left[\frac{3}{5}+\left(\frac{1}{2}\sdot\frac{1}{5}\right)\right]}}](/mediawiki/images/math/3/6/e/36eeba3351620eebf8c50f917db3a94e.png)
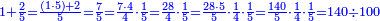
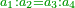


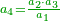
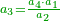
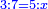
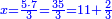
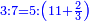

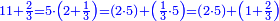

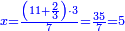

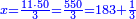
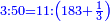

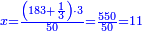

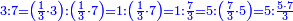


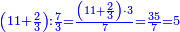
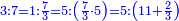
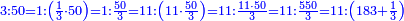
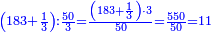

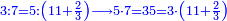
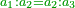
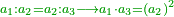
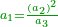
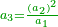

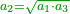

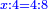


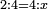
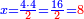

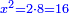

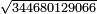
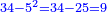

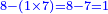

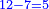
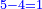

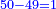
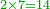
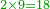

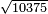

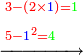

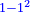
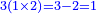
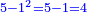

![\scriptstyle{\color{OliveGreen}{\left(ab0\right)^2=\left(a^2\right)0000+\left[2\sdot\left[\left(a\sdot b\right)000\right]\right]+\left(b^2\right)00}}](/mediawiki/images/math/1/f/4/1f4d477bc752c1d6545880bcc60dd96d.png)
![\scriptstyle{\color{OliveGreen}{\left(a0b0\right)^2=\left(a^2\right)000000+\left[2\sdot\left[\left(a\sdot b\right)00000\right]\right]+\left(b^2\right)00}}](/mediawiki/images/math/2/4/d/24d24fac701929ae43cfe1247b1660dc.png)
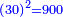
![\scriptstyle{\color{blue}{\left(30+5\right)^2=\left(35\right)^2=35\sdot35=\left(30\sdot30\right)+\left(5\sdot30\right)+\left(30\sdot5\right)+\left(5\sdot5\right)=\left(30\right)^2+\left[\left[2\sdot\left(5\sdot30\right)+5^2\right]\right]}}](/mediawiki/images/math/3/9/5/39578cd605d171fde0b5bacc1c7bae47.png)
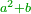

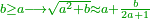

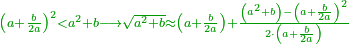


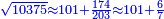
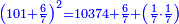


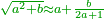
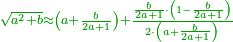
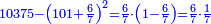

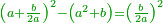
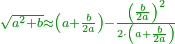
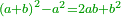
![\scriptstyle{\color{OliveGreen}{\left(a+\frac{b}{2a}\right)^2\approx a^2+\left[2\sdot\left(a\sdot\frac{b}{2a}\right)\right]=a^2+b}}](/mediawiki/images/math/a/d/1/ad19fe1cfe5b42606d0d36ba2a6857cf.png)
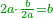
![\scriptstyle{\color{OliveGreen}{\left(a+\frac{b}{2a}\right)^2-\left[\left(a+\frac{b}{2a}\right)-\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\right]^2=\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\sdot\left[2\sdot\left[\left(a+\frac{b}{2a}\right)-\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\right]\right]+\left[\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\right]^2}}](/mediawiki/images/math/3/a/b/3ab8f0a3bfaee95ca50aaae54688f1f4.png)
![\scriptstyle{\color{OliveGreen}{\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}=\frac{\left(\frac{b}{2a}\right)^2}{\left[2\sdot\left[\left(a+\frac{b}{2a}\right)-\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\right]\right]+\left[2\sdot\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\right]}}}](/mediawiki/images/math/8/9/4/894896590d730f42051fd1d021507092.png)
![\scriptstyle{\color{OliveGreen}{\left[\left(a+\frac{b}{2a}\right)-\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\right]^2-\left(a^2+b\right)=\left[\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\right]^2}}](/mediawiki/images/math/e/6/0/e60995689da1f78cb06c47a21ccdcb96.png)
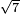


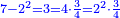
![\scriptstyle{\color{blue}{\left(2+\frac{3}{4}\right)^2-7=\left[7+\left(\frac{3}{4}\right)^2\right]-7=\left(\frac{3}{4}\right)^2=\frac{9}{4}\sdot\frac{1}{4}=\frac{2}{4}+\left(\frac{1}{4}\sdot\frac{1}{4}\right)}}](/mediawiki/images/math/e/e/4/ee4b6356d625ccfa4fb96cdad3e3dbb2.png)
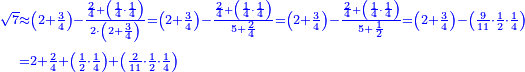
![\scriptstyle{\color{blue}{\left(2+\frac{3}{4}\right)^2-\left[2+\frac{2}{4}+\left(\frac{1}{2}\sdot\frac{1}{4}\right)+\left(\frac{2}{11}\sdot\frac{1}{2}\sdot\frac{1}{4}\right)\right]^2=\left[\left(\frac{9}{11}\sdot\frac{1}{2}\sdot\frac{1}{4}\right)\sdot\left[2\sdot\left[2+\frac{2}{4}+\left(\frac{1}{2}\sdot\frac{1}{4}\right)+\left(\frac{2}{11}\sdot\frac{1}{2}\sdot\frac{1}{4}\right)\right]\right]\right]+\left(\frac{9}{11}\sdot\frac{1}{2}\sdot\frac{1}{4}\right)^2}}](/mediawiki/images/math/5/2/4/524aaa247a457c1db1d66a14b263344d.png)
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left(\frac{9}{11}\sdot\frac{1}{2}\sdot\frac{1}{4}\right)\sdot\left[2\sdot\left(2+\frac{3}{4}\right)\right]&\scriptstyle=\left(\frac{9}{11}\sdot\frac{1}{2}\sdot\frac{1}{4}\right)\sdot\left(5+\frac{1}{2}\right)=\left(\frac{9}{11}\sdot\frac{1}{2}\sdot\frac{1}{4}\right)\sdot\left[\left[2\sdot\left[2+\frac{2}{4}+\left(\frac{1}{2}\sdot\frac{1}{4}\right)\left(\frac{2}{11}\sdot\frac{1}{2}\sdot\frac{1}{4}\right)\right]\right]+\left[2\sdot\left(\frac{9}{11}\sdot\frac{1}{2}\sdot\frac{1}{4}\right)\right]\right]\\&\scriptstyle=\frac{2}{4}+\left(\frac{1}{4}\sdot\frac{1}{4}\right)\\\end{align}}}](/mediawiki/images/math/7/5/4/754ed6b508094b758c0e67fafc131d63.png)
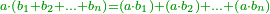
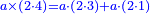
![\scriptstyle{\color{blue}{\left(\frac{9}{11}\sdot\frac{1}{2}\sdot\frac{1}{4}\right)\sdot\left[2\sdot\left(2+\frac{3}{4}\right)\right]=\left(\frac{9}{11}\sdot\frac{1}{2}\sdot\frac{1}{4}\right)\sdot\left[\left[2\sdot\left[2+\frac{2}{4}+\left(\frac{1}{2}\sdot\frac{1}{4}\right)\left(\frac{2}{11}\sdot\frac{1}{2}\sdot\frac{1}{4}\right)\right]\right]+\left[2\sdot\left(\frac{9}{11}\sdot\frac{1}{2}\sdot\frac{1}{4}\right)\right]\right]}}](/mediawiki/images/math/2/7/b/27b74152d7de37b8fd177a514449207d.png)
![\scriptstyle{\color{OliveGreen}{\left(a+\frac{b}{2a}\right)^2-\left(a^2+b\right)=\left(\frac{b}{2a}\right)^2=\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\sdot\left[2\sdot\left(a+\frac{b}{2a}\right)\right]}}](/mediawiki/images/math/4/b/2/4b24c980a3bcda27f39891168376b784.png)
![\scriptstyle{\color{OliveGreen}{\left(a+\frac{b}{2a}\right)^2-\left[\left(a+\frac{b}{2a}\right)-\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\right]^2=\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\sdot\left[\left[2\sdot\left(a+\frac{b}{2a}\right)\right]-\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\right]}}](/mediawiki/images/math/0/c/1/0c1b606d6a39ee494843db3bc854b92e.png)
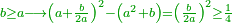
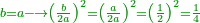
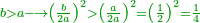

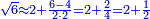


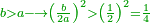
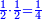
![\scriptstyle{\color{OliveGreen}{\left(1-\frac{n}{m}\right)>\frac{n}{m}\longrightarrow\frac{1}{4}-\left[\frac{n}{m}\sdot\left(1-\frac{n}{m}\right)\right]=\left(\frac{1}{2}-\frac{n}{m}\right)^2}}](/mediawiki/images/math/3/4/d/34d094bcecd5ed3b9239925818c53df2.png)
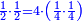

![\scriptstyle{\color{blue}{\left(\frac{1}{2}\sdot\frac{1}{2}\right)-\left[\frac{1}{4}\sdot\left(1-\frac{1}{4}\right)\right]=\frac{1}{4}\sdot\frac{1}{4}}}](/mediawiki/images/math/e/5/6/e56017f8b5d12e1a06c185b2e08165c8.png)

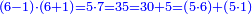
![\scriptstyle{\color{blue}{\left[\left(\frac{1}{2}\sdot12\right)\sdot\left(\frac{1}{2}\sdot12\right)\right]-\left[3\sdot\left(12-3\right)\right]=\left(6\sdot6\right)-\left(3\sdot9\right)=36-27=9=3^2}}](/mediawiki/images/math/d/c/8/dc8d1bb404f25dfbdd1aac97a111a3f7.png)
![\scriptstyle{\color{blue}{\left(\frac{1}{2}\sdot12\right)\sdot\left(\frac{1}{2}\sdot12\right)=6\sdot6=\left(6\sdot3\right)+\left(6\sdot3\right)=\left[2\sdot\left(3\sdot3\right)\right]+\left[2\sdot\left(3\sdot3\right)\right]}}](/mediawiki/images/math/7/7/3/773f982d49f1f7eabb2749d468f5842d.png)
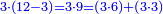
![\scriptstyle{\color{OliveGreen}{\frac{1}{4}n^2-\left[m\sdot\left(n-m\right)\right]=\left[\left(\frac{1}{2}n\right)\sdot\left(\frac{1}{2}n\right)\right]-\left[m\sdot\left(n-m\right)\right]=\left[\left(\frac{1}{2}n\right)-m\right]^2}}](/mediawiki/images/math/0/a/c/0accb9f5e9744b6d8fcc8685ef626612.png)
![\scriptstyle{\color{blue}{\left[\left(\frac{1}{2}\sdot12\right)\sdot\left(\frac{1}{2}\sdot12\right)\right]-\left[3\sdot\left(12-3\right)\right]=3^2=\left(\frac{1}{4}\sdot12\right)^2}}](/mediawiki/images/math/6/2/e/62e5daa2fbcb3de5524af1ce6bab01ba.png)
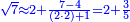
![\scriptstyle{\color{blue}{7-\left(2+\frac{3}{5}\right)^2=7-\left[6+\frac{3}{5}+\left(\frac{4}{5}\sdot\frac{1}{5}\right)\right]=\frac{1}{5}+\left(\frac{1}{5}\sdot\frac{1}{5}\right)=\frac{6}{5}\sdot\frac{1}{5}=\frac{3}{5}\sdot\frac{2}{5}=\frac{3}{5}\sdot\left(1-\frac{3}{5}\right)}}](/mediawiki/images/math/8/0/b/80b721e79750d1324d0c0727f8567aaa.png)
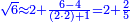
![\scriptstyle{\color{blue}{6-\left(2+\frac{2}{5}\right)^2=6-\left[5+\frac{3}{5}+\left(\frac{4}{5}\sdot\frac{1}{5}\right)\right]=\frac{1}{5}+\left(\frac{1}{5}\sdot\frac{1}{5}\right)=\frac{6}{5}\sdot\frac{1}{5}=\frac{2}{5}\sdot\frac{3}{5}=\frac{2}{5}\sdot\left(1-\frac{2}{5}\right)}}](/mediawiki/images/math/4/d/3/4d358c0666c2045f8d1300fc35d62d2b.png)
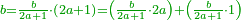

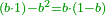
![\scriptstyle{\color{blue}{\left(\frac{1}{3}\sdot1\right)=\left(\frac{1}{3}\sdot\frac{1}{3}\right)+\left(\frac{2}{3}\sdot\frac{1}{3}\right)=\left(\frac{1}{3}\sdot\frac{1}{3}\right)+\left[\frac{1}{3}\sdot\left(1-\frac{1}{3}\right)\right]}}](/mediawiki/images/math/a/9/a/a9abd966f023033e08f139de78734678.png)
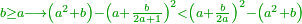
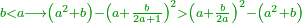
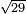


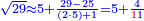
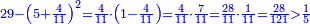
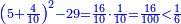
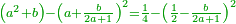
![\scriptstyle{\color{OliveGreen}{\left(a+\frac{b}{2a}\right)^2-\left(a^2+b\right)=\frac{1}{4}-\left[\left(\frac{1}{2}-\frac{b}{2a}\right)^2+\left[2\sdot\frac{b}{2a}\sdot\left(\frac{1}{2}-\frac{b}{2a}\right)\right]\right]}}](/mediawiki/images/math/b/6/0/b60ff58d5339b69d38fd94496223a247.png)
![\scriptstyle{\color{blue}{29-\left(5+\frac{4}{11}\right)^2=\frac{4}{11}\sdot\frac{7}{11}=\frac{1}{4}-\frac{2+\frac{1}{4}}{11^2}=\frac{1}{4}-\left[\left(1+\frac{1}{2}\right)\sdot\frac{1}{11}\right]^2=\frac{1}{4}-\left(\frac{1}{2}-\frac{4}{11}\right)^2}}](/mediawiki/images/math/9/8/7/9879ea05f971cc80ce730f98178f9d28.png)
![\scriptstyle{\color{blue}{\left(5+\frac{4}{10}\right)^2-29=\left(\frac{4}{10}\right)^2=\frac{16}{100}=\frac{1}{4}-\frac{9}{100}=\frac{1}{4}-\left[\left(\frac{1}{2}-\frac{4}{10}\right)^2+\left[2\sdot\frac{4}{10}\sdot\left(\frac{1}{2}-\frac{4}{10}\right)\right]\right]}}](/mediawiki/images/math/2/7/6/2766d0cd9f67d31b94edf0851fa5ba7f.png)
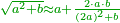
![\scriptstyle{\color{OliveGreen}{\sqrt{a^2+b}\approx\left[a+\frac{2\sdot a\sdot b}{\left(2a\right)^2+b}\right]+\frac{2\sdot\left[a+\frac{2\sdot a\sdot b}{\left(2a\right)^2+b}\right]\sdot\frac{b^3}{\left[\left(2a\right)^2+b\right]^2}}{\left[2\sdot\left[a+\frac{2\sdot a\sdot b}{\left(2a\right)^2+b}\right]\right]^2+\frac{b^3}{\left[\left(2a\right)^2+b\right]^2}}}}](/mediawiki/images/math/d/7/9/d7914c830f879b11b8f80a705f360a4d.png)
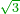
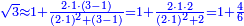
![\scriptstyle{\color{blue}{3-\left(1+\frac{4}{6}\right)^2=\frac{2^3}{\left[\left(2\sdot1\right)^2+2\right]^2}=\frac{8}{6}\sdot\frac{1}{6}}}](/mediawiki/images/math/8/4/0/84003fda2d08d250632a602206672d45.png)
![\scriptstyle{\color{blue}{\sqrt{3}\approx\left(1+\frac{4}{6}\right)+\frac{2\sdot\left(1+\frac{4}{6}\right)\sdot\left(\frac{8}{6}\sdot\frac{1}{6}\right)}{\left[2\sdot\left(1+\frac{4}{6}\right)\right]^2+\left(\frac{8}{6}\sdot\frac{1}{6}\right)}=1+\frac{112}{153}}}](/mediawiki/images/math/b/8/7/b87b5b0f88656891e814164d14c78dc4.png)

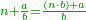
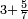
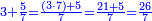

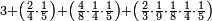

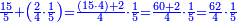
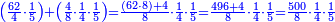
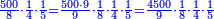
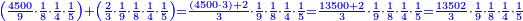
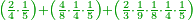

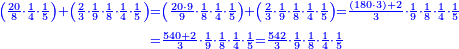
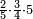
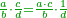
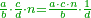
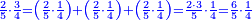

![\scriptstyle\left[\frac{2}{4}+\left(\frac{3}{5}\sdot\frac{1}{4}\right)\right]\sdot\left[\left(\frac{3}{7}\sdot\frac{1}{8}\right)+\left(\frac{4}{5}\sdot\frac{1}{7}\sdot\frac{1}{8}\right)\right]\sdot\left(\frac{3}{9}\sdot\frac{1}{10}\right)\sdot4](/mediawiki/images/math/3/8/7/387896d3f82f552dba69fe6e4ba31825.png)
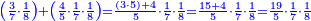
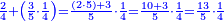
![\scriptstyle{\color{blue}{\left[\frac{2}{4}+\left(\frac{3}{5}\sdot\frac{1}{4}\right)\right]\sdot\left[\left(\frac{3}{7}\sdot\frac{1}{8}\right)+\left(\frac{4}{5}\sdot\frac{1}{7}\sdot\frac{1}{8}\right)\right]\sdot\left(\frac{3}{9}\sdot\frac{1}{10}\right)\sdot4=\left(\frac{13}{5}\sdot\frac{1}{4}\right)\sdot\left(\frac{19}{5}\sdot\frac{1}{7}\sdot\frac{1}{8}\right)\sdot\left(\frac{3}{9}\sdot\frac{1}{10}\right)\sdot4}}](/mediawiki/images/math/9/0/6/906c936571e51fd9f232aa727d3d2cbc.png)
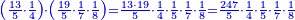


![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left[\frac{2}{4}+\left(\frac{3}{5}\sdot\frac{1}{4}\right)\right]\sdot\left[\left(\frac{3}{7}\sdot\frac{1}{8}\right)+\left(\frac{4}{5}\sdot\frac{1}{7}\sdot\frac{1}{8}\right)\right]\sdot\left(\frac{3}{9}\sdot\frac{1}{10}\sdot4\right)&\scriptstyle=\frac{2964}{5}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}\sdot\frac{1}{10}=\frac{2964}{4}\sdot\frac{1}{5}\sdot\frac{1}{5}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}\sdot\frac{1}{10}\\&\scriptstyle=\frac{741}{5}\sdot\frac{1}{5}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}\sdot\frac{1}{10}\\&\scriptstyle=\left(\frac{148}{4}\sdot\frac{1}{2}\sdot\frac{1}{7}\sdot\frac{1}{5}\sdot\frac{1}{9}\sdot\frac{1}{10}\right)+\left(\frac{1}{5}\sdot\frac{1}{5}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}\sdot\frac{1}{10}\right)\\&\scriptstyle=\left(\frac{37}{7}\sdot\frac{1}{2}\sdot\frac{1}{5}\sdot\frac{1}{9}\sdot\frac{1}{10}\right)+\left(\frac{1}{5}\sdot\frac{1}{5}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}\sdot\frac{1}{10}\right)\\&\scriptstyle=\left(\frac{5}{5}\sdot\frac{1}{2}\sdot\frac{1}{9}\sdot\frac{1}{10}\right)+\left(\frac{1}{2}\sdot\frac{1}{7}\sdot\frac{1}{5}\sdot\frac{1}{9}\sdot\frac{1}{10}\right)+\left(\frac{1}{5}\sdot\frac{1}{5}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}\sdot\frac{1}{10}\right)\\&\scriptstyle=\left(\frac{1}{2}\sdot\frac{1}{9}\sdot\frac{1}{10}\right)+\left(\frac{2}{7}\sdot\frac{1}{5}\sdot\frac{1}{2}\sdot\frac{1}{9}\sdot\frac{1}{10}\right)+\left(\frac{1}{5}\sdot\frac{1}{4}\sdot\frac{1}{7}\sdot\frac{1}{5}\sdot\frac{1}{2}\sdot\frac{1}{9}\sdot\frac{1}{10}\right)\\\end{align}}}](/mediawiki/images/math/5/4/1/541c2c2356a3e27fb0ea8ef9fd37fb77.png)
![\scriptstyle\left[2+\frac{3}{8}+\left(\frac{2}{4}\sdot\frac{1}{8}\right)\right]\quad\frac{4}{5}\quad\left[\frac{6}{7}+\left(\frac{3}{8}\sdot\frac{1}{7}\right)\right]](/mediawiki/images/math/2/f/5/2f5db2cd7e551020181114628b7f1a51.png)
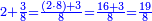
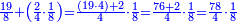
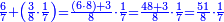

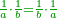
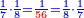

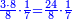
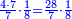
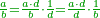
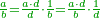
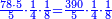

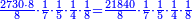
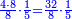
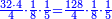
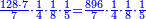


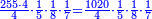

![\scriptstyle\frac{3}{4}\sdot\left[\frac{2}{5}+\left(\frac{3}{4}\sdot\frac{1}{5}\right)\right]\sdot\left[\frac{4}{6}+\left(\frac{1}{3}\sdot\frac{1}{6}\right)\right]](/mediawiki/images/math/3/6/6/36625e7aca77358ba4aba9749ff2d22f.png)
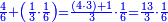
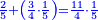
![\scriptstyle{\color{blue}{\frac{3}{4}\sdot\left[\frac{2}{5}+\left(\frac{3}{4}\sdot\frac{1}{5}\right)\right]\sdot\left[\frac{4}{6}+\left(\frac{1}{3}\sdot\frac{1}{6}\right)\right]=\frac{3}{4}\sdot\left(\frac{1{\color{red}{1}}}{4}\sdot\frac{1}{5}\right)\sdot\left(\frac{13}{3}\sdot\frac{1}{6}\right)}}](/mediawiki/images/math/5/0/0/500da56f577e0d0814a0e884c3c50e02.png)
![\scriptstyle{\color{blue}{\frac{3}{4}\sdot\left[\frac{2}{5}+\left(\frac{3}{4}\sdot\frac{1}{5}\right)\right]\sdot\left[\frac{4}{6}+\left(\frac{1}{3}\sdot\frac{1}{6}\right)\right]=\frac{3}{4}\sdot\left(\frac{11}{4}\sdot\frac{1}{5}\right)\sdot\left(\frac{13}{3}\sdot\frac{1}{6}\right)=\frac{3\sdot11\sdot13}{4}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{3}\sdot\frac{1}{6}=\frac{33\sdot13}{4}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{3}\sdot\frac{1}{6}=\frac{429}{4}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{3}\sdot\frac{1}{6}}}](/mediawiki/images/math/d/9/b/d9bd01b5dd65e1505540b023146f560e.png)
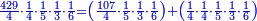



![\scriptstyle{\color{blue}{\frac{3}{4}\sdot\left[\frac{2}{5}+\left(\frac{3}{4}\sdot\frac{1}{5}\right)\right]\sdot\left[\frac{4}{6}+\left(\frac{1}{3}\sdot\frac{1}{6}\right)\right]=\frac{1}{6}+\left(\frac{2}{3}\sdot\frac{1}{6}\right)+\left(\frac{1}{5}\sdot\frac{1}{3}\sdot\frac{1}{6}\right)+\left(\frac{3}{4}\sdot\frac{1}{5}\sdot\frac{1}{3}\sdot\frac{1}{6}\right)+\left(\frac{1}{4}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{3}\sdot\frac{1}{6}\right)}}](/mediawiki/images/math/e/b/b/ebbd0a763e3002706acf0da028cd5659.png)


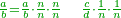
![\scriptstyle\left[2+\frac{3}{8}+\left(\frac{2}{4}\sdot\frac{1}{8}\right)\right]+\frac{4}{5}+\left[\frac{6}{7}+\left(\frac{3}{8}\sdot\frac{1}{7}\right)\right]](/mediawiki/images/math/1/0/f/10fe63879aceb251d84882daff3b8503.png)
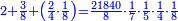

![\scriptstyle{\color{blue}{\left[2+\frac{3}{8}+\left(\frac{2}{4}\sdot\frac{1}{8}\right)\right]+\frac{4}{5}+\left[\frac{6}{7}+\left(\frac{3}{8}\sdot\frac{1}{7}\right)\right]=\left(\frac{21840}{8}\sdot\frac{1}{7}\sdot\frac{1}{5}\sdot\frac{1}{4}\sdot\frac{1}{8}\right)+\left(\frac{7168}{8}\sdot\frac{1}{7}\sdot\frac{1}{4}\sdot\frac{1}{8}\sdot\frac{1}{5}\right)+\left(\frac{8160}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{8}\sdot\frac{1}{7}\right)=\frac{37168}{8}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{8}\sdot\frac{1}{7}}}](/mediawiki/images/math/8/3/5/835eb6c634083b4b02df5b7a2acc1d78.png)
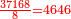

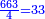
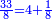
![\scriptstyle{\color{blue}{\left[2+\frac{3}{8}+\left(\frac{2}{4}\sdot\frac{1}{8}\right)\right]+\frac{4}{5}+\left[\frac{6}{7}+\left(\frac{3}{8}\sdot\frac{1}{7}\right)\right]=4+\frac{1}{8}+\left(\frac{3}{4}\sdot\frac{1}{5}\sdot\frac{1}{8}\right)+\left(\frac{5}{7}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{8}\right)}}](/mediawiki/images/math/1/5/4/154dedbe209e8a992aede8b27b1b23eb.png)
![\scriptstyle{\color{blue}{\left[2+\frac{3}{8}+\left(\frac{2}{4}\sdot\frac{1}{8}\right)\right]+\frac{4}{5}+\left[\frac{6}{7}+\left(\frac{3}{8}\sdot\frac{1}{7}\right)\right]=4+\frac{1}{8}+\left(\frac{3}{4}\sdot\frac{1}{5}\sdot\frac{1}{8}\right)+\left(\frac{5}{7}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{8}\right)=4+\left(\frac{25}{8}\sdot\frac{1}{5}\sdot\frac{1}{5}\right)+\left(\frac{3}{4}\sdot\frac{1}{8}\sdot\frac{1}{5}\right)+\left(\frac{5}{7}\sdot\frac{1}{4}\sdot\frac{1}{8}\sdot\frac{1}{5}\right)}}](/mediawiki/images/math/1/c/1/1c129729a0dc916fe0b44ecc1d157636.png)
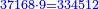

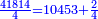

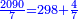

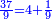
![\scriptstyle{\color{blue}{\left[2+\frac{3}{8}+\left(\frac{2}{4}\sdot\frac{1}{8}\right)\right]+\frac{4}{5}+\left[\frac{6}{7}+\left(\frac{3}{8}\sdot\frac{1}{7}\right)\right]=4+\frac{1}{9}+\left(\frac{2}{8}\sdot\frac{1}{9}\right)+\left(\frac{4}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}\right)+\left(\frac{3}{5}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}\right)+\left(\frac{2}{4}\sdot\frac{1}{5}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}\right)}}](/mediawiki/images/math/2/9/e/29ed57fc7ddb68e6dbdbf4d232272540.png)
![\scriptstyle\left(\frac{2}{5}\sdot\frac{2}{9}\sdot2\right)+\left[\left[\frac{1}{8}+\left(\frac{2}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\right)\right]\sdot\left[\frac{1}{4}+\left(\frac{2}{6}\sdot\frac{1}{4}\right)\right]\right]](/mediawiki/images/math/e/2/5/e2537ec7557a116d21369f6f61831a30.png)
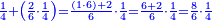
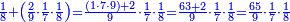
![\scriptstyle{\color{blue}{\left[\frac{1}{8}+\left(\frac{2}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\right)\right]\sdot\left[\frac{1}{4}+\left(\frac{2}{6}\sdot\frac{1}{4}\right)\right]=\left(\frac{65}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\right)\sdot\left(\frac{8}{6}\sdot\frac{1}{4}\right)}}](/mediawiki/images/math/9/8/9/989a1bcb49b832461aa0a175792074ba.png)
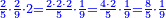
![\scriptstyle{\color{blue}{\left[\frac{1}{8}+\left(\frac{2}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\right)\right]\sdot\left[\frac{1}{4}+\left(\frac{2}{6}\sdot\frac{1}{4}\right)\right]=\left(\frac{65}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\right)\sdot\left(\frac{8}{6}\sdot\frac{1}{4}\right)=\frac{65\sdot8}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{6}\sdot\frac{1}{4}=\frac{520}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{6}\sdot\frac{1}{4}}}](/mediawiki/images/math/6/8/5/685c2c4fd33c8762b85273f351b1c8d5.png)
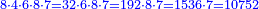

![\scriptstyle{\color{blue}{\left[\frac{1}{8}+\left(\frac{2}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\right)\right]\sdot\left[\frac{1}{4}+\left(\frac{2}{6}\sdot\frac{1}{4}\right)\right]=\frac{520}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{6}\sdot\frac{1}{4}=\frac{520\sdot5}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{6}\sdot\frac{1}{4}\sdot\frac{1}{5}=\frac{2600}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{6}\sdot\frac{1}{4}\sdot\frac{1}{5}}}](/mediawiki/images/math/8/b/c/8bc0242053d5c1927655f2429ebbcdf8.png)
![\scriptstyle{\color{blue}{\left(\frac{2}{5}\sdot\frac{2}{9}\sdot2\right)+\left[\left[\frac{1}{8}+\left(\frac{2}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\right)\right]\sdot\left[\frac{1}{4}+\left(\frac{2}{6}\sdot\frac{1}{4}\right)\right]\right]=\left(\frac{10752}{5}\sdot\frac{1}{9}\sdot\frac{1}{4}\sdot\frac{1}{6}\sdot\frac{1}{8}\sdot\frac{1}{7}\right)+\left(\frac{2600}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{6}\sdot\frac{1}{4}\sdot\frac{1}{5}\right)=\left(\frac{13352}{7}\sdot\frac{1}{8}\sdot\frac{1}{6}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{9}\right)}}](/mediawiki/images/math/a/3/0/a30569a312c37273f7dc196b1440768c.png)
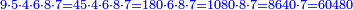


![\scriptstyle{\color{OliveGreen}{\frac{\left(a\sdot n\right)+r}{a}=n+\frac{r}{a}\longrightarrow\frac{a}{\left(a\sdot n\right)+r}=\frac{1}{n+1}+\frac{a-r}{\left(n+1\right)\sdot\left[\left(a\sdot n\right)+r\right]}}}](/mediawiki/images/math/5/9/8/598a80b39722adb0e82e834274c47d4c.png)
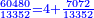
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left(\frac{2}{5}\sdot\frac{2}{9}\sdot2\right)+\left[\left[\frac{1}{8}+\left(\frac{2}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\right)\right]\sdot\left[\frac{1}{4}+\left(\frac{2}{6}\sdot\frac{1}{4}\right)\right]\right]&\scriptstyle=\left(\frac{13352}{7}\sdot\frac{1}{8}\sdot\frac{1}{6}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{9}\right)=\frac{13352}{60480}=\frac{1}{4+1}+\frac{13352-7072}{5\sdot60480}\\&\scriptstyle=\frac{1}{5}+\left(\frac{6280}{7}\sdot\frac{1}{8}\sdot\frac{1}{6}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{9}\sdot\frac{1}{5}\right)\\\end{align}}}](/mediawiki/images/math/1/3/0/130693a50ead428fa30ce6fc390df230.png)
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left(\frac{2}{5}\sdot\frac{2}{9}\sdot2\right)+\left[\left[\frac{1}{8}+\left(\frac{2}{9}\sdot\frac{1}{7}\sdot\frac{1}{8}\right)\right]\sdot\left[\frac{1}{4}+\left(\frac{2}{6}\sdot\frac{1}{4}\right)\right]\right]&\scriptstyle=\frac{1}{5}+\left(\frac{6280}{7}\sdot\frac{1}{8}\sdot\frac{1}{6}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{9}\sdot\frac{1}{5}\right)=\frac{1}{5}+\left(\frac{6280}{8}\sdot\frac{1}{5}\sdot\frac{1}{4}\sdot\frac{1}{6}\sdot\frac{1}{9}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)\\&\scriptstyle=\frac{1}{5}+\left(\frac{785}{5}\sdot\frac{1}{4}\sdot\frac{1}{6}\sdot\frac{1}{9}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)=\frac{1}{5}+\left(\frac{157}{4}\sdot\frac{1}{6}\sdot\frac{1}{9}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)\\&\scriptstyle=\frac{1}{5}+\left(\frac{39}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)+\left(\frac{1}{4}\sdot\frac{1}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)\\&\scriptstyle=\frac{1}{5}+\left(\frac{6}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)+\left(\frac{3}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)+\left(\frac{1}{4}\sdot\frac{1}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)\\&\scriptstyle=\frac{1}{5}+\left(\frac{2}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)+\left(\frac{3}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)+\left(\frac{1}{4}\sdot\frac{1}{6}\sdot\frac{1}{3}\sdot\frac{1}{3}\sdot\frac{1}{7}\sdot\frac{1}{5}\right)\\\end{align}}}](/mediawiki/images/math/2/b/8/2b8433d5e6b54b67f624806d03ebc4d2.png)
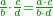
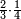
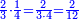

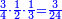
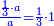
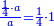
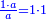
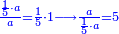
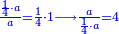

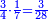
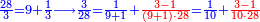


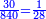
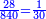
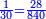
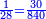
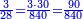
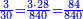
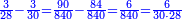

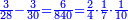

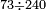

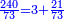
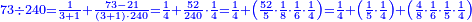
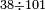

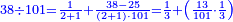
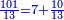
![\scriptstyle{\color{blue}{38\div101=\frac{1}{3}+\frac{13}{101}\sdot\frac{1}{3}=\frac{1}{3}+\frac{1}{3}\sdot\left[\frac{1}{7+1}+\frac{13-10}{\left(7+1\right)\sdot101}\right]=\frac{1}{3}+\frac{1}{3}\sdot\left[\frac{1}{8}+\frac{3}{8\sdot101}\right]=\frac{1}{3}+\left(\frac{1}{3}\sdot\frac{1}{8}\right)+\left(\frac{3}{101}\sdot\frac{1}{3}\sdot\frac{1}{8}\right)}}](/mediawiki/images/math/e/2/3/e23d51f6a10cd96fa44ea534a3144f2e.png)
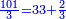
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle38\div101&\scriptstyle=\frac{1}{3}+\left(\frac{1}{3}\sdot\frac{1}{8}\right)+\left(\frac{3}{101}\sdot\frac{1}{3}\sdot\frac{1}{8}\right)=\frac{1}{3}+\left(\frac{1}{3}\sdot\frac{1}{8}\right)+\left[\frac{1}{3}\sdot\frac{1}{8}\sdot\left[\frac{1}{33+1}+\frac{3-2}{\left(33+1\right)\sdot101}\right]\right]\\&\scriptstyle=\frac{1}{3}+\left(\frac{1}{3}\sdot\frac{1}{8}\right)+\left[\frac{1}{3}\sdot\frac{1}{8}\sdot\left[\frac{1}{34}+\frac{1}{34\sdot101}\right]\right]\\&\scriptstyle=\frac{1}{3}+\left(\frac{1}{3}\sdot\frac{1}{8}\right)+\left(\frac{1}{3}\sdot\frac{1}{8}\sdot\frac{1}{34}\right)+\left(\frac{1}{3}\sdot\frac{1}{8}\sdot\frac{1}{34}\sdot\frac{1}{101}\right)\\\end{align}}}](/mediawiki/images/math/a/1/a/a1afcd04e6e031fddb5868f3103d997b.png)
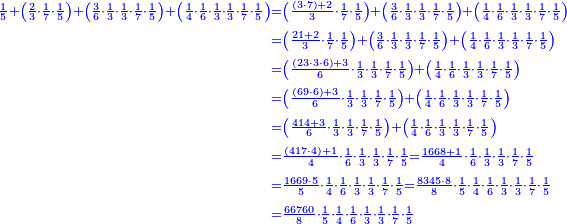


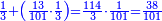
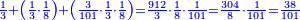
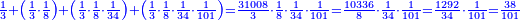
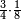
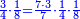
![\scriptstyle\left[\left[\frac{8}{9}+\left(\frac{3}{7}\sdot\frac{1}{5}\sdot\frac{1}{9}\right)\right]\sdot\left(\frac{5}{6}\sdot3\right)\right]-\left[\left[\frac{3}{4}+\left(\frac{2}{5}\sdot\frac{1}{4}\right)\right]\sdot\frac{2}{9}\right]](/mediawiki/images/math/d/6/d/d6d3383d94cc0c6f1b9eabdd1be5711c.png)
![\scriptstyle{\color{blue}{\left[\frac{3}{4}+\left(\frac{2}{5}\sdot\frac{1}{4}\right)\right]\sdot\frac{2}{9}=\frac{34}{5}\sdot\frac{1}{4}\sdot\frac{1}{9}}}](/mediawiki/images/math/7/4/b/74b8c93d7ed6516a0fe4bd7b5c8adb2a.png)
![\scriptstyle{\color{blue}{\left[\frac{8}{9}+\left(\frac{3}{7}\sdot\frac{1}{5}\sdot\frac{1}{9}\right)\right]\sdot\left(\frac{5}{6}\sdot3\right)=\frac{4245}{7}\sdot\frac{1}{5}\sdot\frac{1}{9}\sdot\frac{1}{6}}}](/mediawiki/images/math/d/c/f/dcf61fe7fff902138bbf8445996648a3.png)

![\scriptstyle{\color{blue}{\left[\frac{3}{4}+\left(\frac{2}{5}\sdot\frac{1}{4}\right)\right]\sdot\frac{2}{9}=\frac{34\sdot6\sdot7}{7}\sdot\frac{1}{6}\sdot\frac{1}{5}\sdot\frac{1}{4}\sdot\frac{1}{9}=\frac{1428}{7}\sdot\frac{1}{6}\sdot\frac{1}{5}\sdot\frac{1}{4}\sdot\frac{1}{9}}}](/mediawiki/images/math/3/d/2/3d2d5cadbdbe771b53d2c5d04fa3f2e4.png)
![\scriptstyle{\color{blue}{\left[\frac{8}{9}+\left(\frac{3}{7}\sdot\frac{1}{5}\sdot\frac{1}{9}\right)\right]\sdot\left(\frac{5}{6}\sdot3\right)=\frac{4245\sdot4}{4}\sdot\frac{1}{7}\sdot\frac{1}{5}\sdot\frac{1}{9}\sdot\frac{1}{6}=\frac{16980}{4}\sdot\frac{1}{7}\sdot\frac{1}{5}\sdot\frac{1}{9}\sdot\frac{1}{6}}}](/mediawiki/images/math/0/3/f/03fe4281e1c23a4a77b5472cb54405be.png)
![\scriptstyle{\color{blue}{\left[\left[\frac{8}{9}+\left(\frac{3}{7}\sdot\frac{1}{5}\sdot\frac{1}{9}\right)\right]\sdot\left(\frac{5}{6}\sdot3\right)\right]-\left[\left[\frac{3}{4}+\left(\frac{2}{5}\sdot\frac{1}{4}\right)\right]\sdot\frac{2}{9}\right]=\left(\frac{16980}{4}\sdot\frac{1}{7}\sdot\frac{1}{5}\sdot\frac{1}{9}\sdot\frac{1}{6}\right)-\left(\frac{1428}{7}\sdot\frac{1}{6}\sdot\frac{1}{5}\sdot\frac{1}{4}\sdot\frac{1}{9}\right)=\frac{15552}{4}\sdot\frac{1}{7}\sdot\frac{1}{5}\sdot\frac{1}{9}\sdot\frac{1}{6}=2+\left(\frac{2}{7}\sdot\frac{1}{5}\right)}}](/mediawiki/images/math/c/d/e/cde5e5a98fc0c5176c0289c19663f814.png)
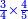
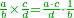

![\scriptstyle\left[\left[\frac{4}{7}\sdot\left(\frac{5}{9}\sdot\frac{1}{8}\right)\right]\right]\times\left[\left(\frac{3}{5}\sdot\frac{1}{9}\right)\sdot\left(\frac{2}{3}\sdot5\right)\right]](/mediawiki/images/math/8/e/1/8e1455aa8265c18c2b288e543c19aede.png)
![\scriptstyle{\color{blue}{\left[\left[\frac{4}{7}\sdot\left(\frac{5}{9}\sdot\frac{1}{8}\right)\right]\right]\times\left[\left(\frac{3}{5}\sdot\frac{1}{9}\right)\sdot\left(\frac{2}{3}\sdot5\right)\right]=\frac{4}{7}\sdot\left(\frac{5}{9}\sdot\frac{1}{8}\right)\sdot\left(\frac{3}{5}\sdot\frac{1}{9}\right)\sdot\left(\frac{2}{3}\sdot5\right)=\frac{600}{7}\sdot\frac{1}{9}\sdot\frac{1}{8}\sdot\frac{1}{5}\sdot\frac{1}{9}\sdot\frac{1}{3}}}](/mediawiki/images/math/f/0/b/f0b4e9c0c3a358604d622ff3a6cecc4b.png)
![\scriptstyle{\color{blue}{\left[\left[\frac{4}{7}\sdot\left(\frac{5}{9}\sdot\frac{1}{8}\right)\right]\right]\times\left[\left(\frac{3}{5}\sdot\frac{1}{9}\right)\sdot\left(\frac{2}{3}\sdot5\right)\right]=\frac{600}{7}\sdot\frac{1}{9}\sdot\frac{1}{8}\sdot\frac{1}{5}\sdot\frac{1}{9}\sdot\frac{1}{3}=\frac{5}{9}\sdot\frac{1}{7}\sdot\frac{1}{9}}}](/mediawiki/images/math/0/c/0/0c059d28324ce62c3bdb1bf7ea1de18c.png)
![\scriptstyle\left[\frac{3}{4}+\left(\frac{2}{3}\sdot\frac{1}{4}\right)\right]\div\left[\left[\frac{4}{9}+\left(\frac{5}{6}\sdot\frac{1}{9}\right)\right]\sdot\frac{2}{3}\right]](/mediawiki/images/math/a/0/b/a0bf54c86fb59c4b365c62aeb651544d.png)
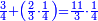
![\scriptstyle{\color{blue}{\left[\frac{4}{9}+\left(\frac{5}{6}\sdot\frac{1}{9}\right)\right]\sdot\frac{2}{3}=\frac{58}{6}\sdot\frac{1}{9}\sdot\frac{1}{3}}}](/mediawiki/images/math/1/0/d/10d30a962745577ac136a3f6ac85a875.png)
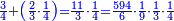
![\scriptstyle{\color{blue}{\left[\frac{4}{9}+\left(\frac{5}{6}\sdot\frac{1}{9}\right)\right]\sdot\frac{2}{3}=\frac{58}{6}\sdot\frac{1}{9}\sdot\frac{1}{3}=\frac{232}{6}\sdot\frac{1}{9}\sdot\frac{1}{3}\sdot\frac{1}{4}}}](/mediawiki/images/math/b/c/f/bcf7cf3cc51650dd0b6d9982861ca94f.png)
![\scriptstyle{\color{blue}{\left[\frac{3}{4}+\left(\frac{2}{3}\sdot\frac{1}{4}\right)\right]\div\left[\left[\frac{4}{9}+\left(\frac{5}{6}\sdot\frac{1}{9}\right)\right]\sdot\frac{2}{3}\right]=\left(\frac{594}{6}\sdot\frac{1}{9}\sdot\frac{1}{3}\sdot\frac{1}{4}\right)\div\left(\frac{232}{6}\sdot\frac{1}{9}\sdot\frac{1}{3}\sdot\frac{1}{4}\right)=\frac{594}{232}}}](/mediawiki/images/math/5/3/0/530af30430d6012b22fd302598680b2c.png)

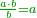
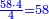


![\scriptstyle\left[\frac{3}{4}\sdot\left(3-\frac{1}{4}\right)\right]:\left[\frac{4}{5}\sdot\left(5-\frac{1}{5}\right)\right]=\left[\frac{5}{6}\sdot\left(6-\frac{1}{6}\right)\right]:X](/mediawiki/images/math/0/6/b/06b938d3936d7baec8744616f4c89a6b.png)

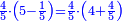
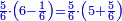
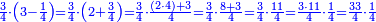
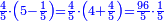

![\scriptstyle{\color{blue}{\left[\frac{3}{4}\sdot\left(3-\frac{1}{4}\right)\right]:\left[\frac{4}{5}\sdot\left(5-\frac{1}{5}\right)\right]=\left[\frac{5}{6}\sdot\left(6-\frac{1}{6}\right)\right]:X\longleftrightarrow\left(\frac{33}{4}\sdot\frac{1}{4}\right):\left(\frac{96}{5}\sdot\frac{1}{5}\right)=\left(\frac{175}{6}\sdot\frac{1}{6}\right):X}}](/mediawiki/images/math/8/3/b/83b408ca60c0b13f2ca139ce6acc9004.png)
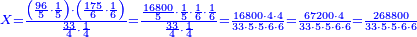
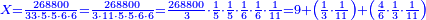
![\scriptstyle{\color{blue}{\left[9+\left(\frac{1}{3}\sdot\frac{1}{11}\right)+\left(\frac{4}{6}\sdot\frac{1}{3}\sdot\frac{1}{11}\right)\right]\sdot\left(\frac{33}{4}\sdot\frac{1}{4}\right)}}](/mediawiki/images/math/3/f/d/3fdd6f57b0fcf48e26dd34013c0017e7.png)
![\scriptstyle{\color{blue}{\left[9+\left(\frac{1}{3}\sdot\frac{1}{11}\right)+\left(\frac{4}{6}\sdot\frac{1}{3}\sdot\frac{1}{11}\right)\right]\sdot\left(\frac{33}{4}\sdot\frac{1}{4}\right)=\left(\frac{{\color{red}{1792}}}{6}\sdot\frac{1}{3}\sdot\frac{1}{11}\right)\sdot\left(\frac{33}{4}\sdot\frac{1}{4}\right)=\frac{59136}{4}\sdot\frac{1}{4}\sdot\frac{1}{6}\sdot\frac{1}{3}\sdot\frac{1}{11}}}](/mediawiki/images/math/4/5/4/45418d38e391e03383881fe4ceeff0c2.png)
![\scriptstyle{\color{blue}{\frac{\left[9+\left(\frac{1}{3}\sdot\frac{1}{11}\right)+\left(\frac{4}{6}\sdot\frac{1}{3}\sdot\frac{1}{11}\right)\right]\sdot\left(\frac{33}{4}\sdot\frac{1}{4}\right)}{\frac{96}{5}\sdot\frac{1}{5}}=\frac{175}{6}\sdot\frac{1}{6}}}](/mediawiki/images/math/9/4/7/9476e7963c3246642b3cfe1b1eb5c2a2.png)

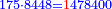
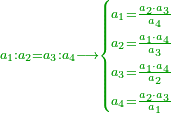

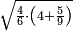
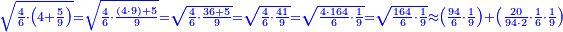
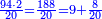
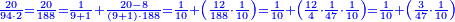
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\sqrt{\frac{4}{6}\sdot\left(4+\frac{5}{9}\right)}\approx\left(\frac{94}{6}\sdot\frac{1}{9}\right)+\left(\frac{20}{94\sdot2}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)&\scriptstyle=\left(\frac{94}{6}\sdot\frac{1}{9}\right)+\left[\left[\frac{1}{10}+\left(\frac{3}{47}\sdot\frac{1}{10}\right)\right]\sdot\frac{1}{6}\sdot\frac{1}{9}\right]\\&\scriptstyle=\left(\frac{94}{6}\sdot\frac{1}{9}\right)+\left(\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)+\left(\frac{3}{47}\sdot\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)\\\end{align}}}](/mediawiki/images/math/0/1/1/011279d8c132abf3ded44c215f8dd3a6.png)
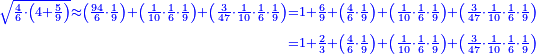
![\scriptstyle{\color{blue}{\left[\left(\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)+\left(\frac{3}{47}\sdot\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)\right]^2=\frac{2500}{47}\sdot\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\sdot\frac{1}{47}\sdot\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}=\left(\frac{4}{9}\sdot\frac{1}{47}\frac{1}{47}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)+\left(\frac{1}{6}\sdot\frac{1}{9}\sdot\frac{1}{47}\sdot\frac{1}{47}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)}}](/mediawiki/images/math/3/d/2/3d2093c4752f2bd39170394b86bbfcc4.png)
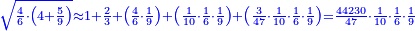
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left[1+\frac{2}{3}+\left(\frac{4}{6}\sdot\frac{1}{9}\right)+\left(\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)+\left(\frac{3}{47}\sdot\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)\right]^2&\scriptstyle=\left(\frac{44230}{47}\sdot\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)^2=\frac{44230}{47}\sdot\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\sdot\frac{44230}{47}\sdot\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\\&\scriptstyle=\frac{1956292900}{47}\sdot\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\sdot\frac{1}{47}\sdot\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\\\end{align}}}](/mediawiki/images/math/8/e/3/8e3a97b325a1050e8893f8690ed97d85.png)
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left[1+\frac{2}{3}+\left(\frac{4}{6}\sdot\frac{1}{9}\right)+\left(\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)+\left(\frac{3}{47}\sdot\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)\right]^2&\scriptstyle=\frac{1956292900}{47}\sdot\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\sdot\frac{1}{47}\sdot\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\\&\scriptstyle=\left(\frac{164}{6}\sdot\frac{1}{9}\right)+\left[\left(\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)+\left(\frac{3}{47}\sdot\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)\right]^2\\&\scriptstyle\left(\frac{164}{6}\sdot\frac{1}{9}\right)+\left(\frac{4}{9}\sdot\frac{1}{47}\frac{1}{47}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)+\left(\frac{1}{6}\sdot\frac{1}{9}\sdot\frac{1}{47}\sdot\frac{1}{47}\sdot\frac{1}{6}\sdot\frac{1}{9}\right)\\\end{align}}}](/mediawiki/images/math/2/5/a/25aa8f4377bd44eb343e0fb3d4338d8a.png)
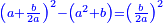
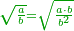
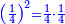
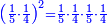
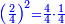
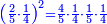
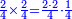



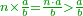
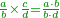
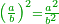

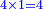
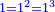
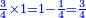
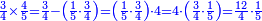
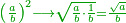
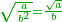
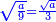
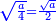
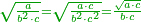




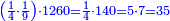


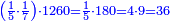
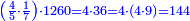
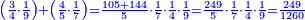
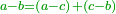
![\scriptstyle\left[3+\frac{5}{9}+\left(\frac{3}{7}\sdot\frac{1}{9}\right)+\left(\frac{2}{4}\sdot\frac{1}{7}\sdot\frac{1}{9}\right)\right]-\left[\frac{7}{9}+\left(\frac{5}{7}\sdot\frac{1}{9}\right)+\left(\frac{3}{4}\sdot\frac{1}{7}\sdot\frac{1}{9}\right)\right]](/mediawiki/images/math/c/9/c/c9c6d9287898412880ce3955aec5dcbf.png)
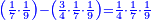
![\scriptstyle{\color{blue}{\frac{1}{9}-\left[\left(\frac{1}{7}\sdot\frac{1}{9}\right)+\left(\frac{5}{7}\sdot\frac{1}{9}\right)\right]=\frac{1}{9}-\left(\frac{6}{7}\sdot\frac{1}{9}\right)=\frac{1}{7}\sdot\frac{1}{9}}}](/mediawiki/images/math/8/6/a/86a3c8d68c7b1d660828802a00c1ace2.png)
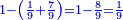
![\scriptstyle{\color{blue}{\begin{align}&\scriptstyle
\left[3+\frac{5}{9}+\left(\frac{3}{7}\sdot\frac{1}{9}\right)+\left(\frac{2}{4}\sdot\frac{1}{7}\sdot\frac{1}{9}\right)\right]-\left[\frac{7}{9}+\left(\frac{5}{7}\sdot\frac{1}{9}\right)+\left(\frac{3}{4}\sdot\frac{1}{7}\sdot\frac{1}{9}\right)\right]\\&\scriptstyle=\left[\left(3-1\right)+\frac{5}{9}+\left(\frac{3}{7}\sdot\frac{1}{9}\right)+\left(\frac{2}{4}\sdot\frac{1}{7}\sdot\frac{1}{9}\right)\right]+\left[1-\left[\frac{7}{9}+\left(\frac{5}{7}\sdot\frac{1}{9}\right)+\left(\frac{3}{4}\sdot\frac{1}{7}\sdot\frac{1}{9}\right)\right]\right]\\&\scriptstyle=\left[2+\frac{5}{9}+\left(\frac{3}{7}\sdot\frac{1}{9}\right)+\left(\frac{2}{4}\sdot\frac{1}{7}\sdot\frac{1}{9}\right)\right]+\left[\frac{1}{9}+\left(\frac{1}{7}\sdot\frac{1}{9}\right)+\left(\frac{1}{4}\sdot\frac{1}{7}\sdot\frac{1}{9}\right)\right]=2+\frac{6}{9}+\left(\frac{4}{7}\sdot\frac{1}{9}\right)+\left(\frac{3}{4}\sdot\frac{1}{7}\sdot\frac{1}{9}\right)\\\end{align}}}](/mediawiki/images/math/0/6/3/0638291989811cb1de544fc0e0512f5b.png)
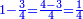
![\scriptstyle{\color{blue}{\left[\left(\frac{4}{4}\sdot\frac{1}{7}\sdot\frac{1}{9}\right)+\left(\frac{2}{4}\sdot\frac{1}{7}\sdot\frac{1}{9}\right)\right]-\left(\frac{3}{4}\sdot\frac{1}{7}\sdot\frac{1}{9}\right)=\left(\frac{6}{4}\sdot\frac{1}{7}\sdot\frac{1}{9}\right)-\left(\frac{3}{4}\sdot\frac{1}{7}\sdot\frac{1}{9}\right)=\frac{3}{4}\sdot\frac{1}{7}\sdot\frac{1}{9}}}](/mediawiki/images/math/8/3/4/83478f856be43de02275bd974f810b6a.png)
![\scriptstyle{\color{blue}{\left[\left(\frac{7}{7}\sdot\frac{1}{9}\right)+\left(\frac{3}{7}\sdot\frac{1}{9}\right)\right]-\left[\left(\frac{1}{7}\sdot\frac{1}{9}\right)+\left(\frac{5}{7}\sdot\frac{1}{9}\right)\right]=\left(\frac{10}{7}\sdot\frac{1}{9}\right)-\left(\frac{6}{7}\sdot\frac{1}{9}\right)=\left(\frac{4}{7}\sdot\frac{1}{9}\right)}}](/mediawiki/images/math/4/c/a/4ca62b907b0cfb98f76e97e44229417f.png)
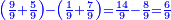
![\scriptstyle{\color{blue}{\left[3+\frac{5}{9}+\left(\frac{3}{7}\sdot\frac{1}{9}\right)+\left(\frac{2}{4}\sdot\frac{1}{7}\sdot\frac{1}{9}\right)\right]-\left[\frac{7}{9}+\left(\frac{5}{7}\sdot\frac{1}{9}\right)+\left(\frac{3}{4}\sdot\frac{1}{7}\sdot\frac{1}{9}\right)\right]=2+\frac{6}{9}+\left(\frac{4}{7}\sdot\frac{1}{9}\right)+\left(\frac{3}{4}\sdot\frac{1}{7}\sdot\frac{1}{9}\right)}}](/mediawiki/images/math/3/2/2/322024015dab43ce3e2e948787fdb8ac.png)