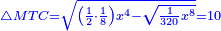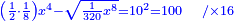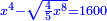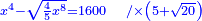המחומשים והמעושרים
Contents
Introduction |
|
| Said Abū Kāmil: I have explained what needs to be explained in the restoration and confrontation relating to numbers according to our predecessors, by their knowledge and wisdom, in addition to what was written by those who are well versed in geometry, who knew Euclid's Elements and other books. | [1]אמר אבו כאמל אחר אשר בארנו מה שצריך באור מן הנתלה במספרי' מהאסיפה וההשואה דילקובראמיינטו אידיל קונפרונטאמיינטו על הקודמים לנו וזה בידיעה ובחכמה בהם מוסף על מה שכתבו הבקיאים בגמטרייא שידעו ספר האוקלידס וספרים אחרים |
| We will further define and explain the following in our book: | הנה נניח עוד ונבאר בספרנו זה |
|
שעור כל צלע מצלעות כל אחד מהמחומשים והמעושרים אשר צלעותיהם שוות וזויותיהם שוות אשר תקיף לכל אחד מהם עגולה ידועה או יקיף כל אחד מהם אל עגולה ידועה |
|
ושעור קוטר העגולה אשר תקיף אל המחומש ואל המעושר ידוע שוה הצלעות והזויות או שיקיפו כל אחד מהם אל עגולה ידועה |
|
ושעור הקו החותך חלק אחד מחמשה עשר חלקים ממקיף העגולה |
|
ושעור כל צלע מצלעות המחומש והמעושר שהם שוי הצלעות והזויות כאשר היו ידועי השעור |
|
ושעור צלעות המשולשים ידועי השעור כאשר היו במחומשים ובמעושרים שוי הצלעות והזויות |
| And other matters that we will explain in our book, which the predecessors extracted with much trouble, and the arithmeticians and geometricians of our time testify to this and wrote us their words from the predecessors about the knowledge of arithmetic and geometry. | ודברים אחרים בלעדי אלו נבאר בספרנו זה אשר הוציאו אותם הקודמים בטורח רב ויעידו על זה בעלי המספר והגימטרייא אשר בזמננו זה ואשר כתבו לנו דבריהם מן הקודמים בידיעת המספר והגימטריאה |
| May the almighty God help us to put together what is appropriate of this and to correct what we we presume to understand. May the Creator be praised and blessed. | ויעזרנו השם היכול לחבר הראוי מזה ולישר מה שנניח על הבנתו וישתבח היוצר ויחונן |
Finding the side of a pentagon that is circumscribed by a circle |
|
| I start by extracting the size of a fifth of the circumference of a circle whose diameter is known. | ואתחיל בהוצאת שעור חמשית קו העגולה אשר קטרה ידוע |
|
|
|
| This is that we suppose the known circle is circle ABHG. | וזה שנניח העגולה הידועה עגלת א"ב ה"ג |
| Its diameter is ten and it is line CH.
|
וקטרה הוא עשרה במספר והוא קו ח"ה |
| In it there is a regular pentagon enclosed by the circle, which is pentagon ABHG. | ובתוכה מחומש שוה הצלעות והזויות תקיף בו העגולה והוא מחומש אבה"ג |
| When you wish to know the size of each side of this pentagon: | וכאשר תרצה לדעת שעור כל צלע מצלעות זה המחומש |
| We draw line GLD that cuts two fifth of the circle's circumferential line. | נוציא קו גל"ד והוא קו חותך שני חומשי קו המקיף העגולה |
We suppose line HD is a thing [ ]. ].
|
ונניח קו ה"ד דבר |
| It is known that line HL is tenth of the square.
|
והוא ידוע שקו ה"ל עשירית האלגו |
| Since the product of HD by itself is the same as the product of CH by HL.
|
בעבור כי הכאת ה"ד בעצמו היא כמו הכאת ח"ה בה"ל |
| Also line GL is a root of the square minus a tenth of a tenth of a square of the square.
|
וקו ג"ל שרש האלגו פחות עשירית [2]העשירית מאלגו אלגו |
| Line GL is the same as line LD.
|
וקו ג"ל כמו קו ל"ד |
| Line GD is a root of four squares minus two-fifths of a square of a square.
|
ויהיה קו ג"ד שרש מארבעה אלגוש פחות שני חומשי עשירית מאלגו אלגו |
| It is known that [the product of] AB by GD plus [the product of] AB by itself is the same as the product of GD by itself.
|
והוא ידוע שא"ב בג"ד וא"ב בעצמו יהיו כמו הכאת ג"ד בעצמו |
| Since the product of AB by GD plus [the product of] AG by BD is the same as the product of AD by BG.
|
בעבור כי הכאת א"ב בג"ד וא"ג בב"ד יהיו כמו הכאת א"ד בב"ג |
| [The product of] AG by BD is the same as [the product of] AB by itself.
|
וא"ג בב"ד הוא כמו א"ב בעצמו |
| [The product of] AD by BG is the same as [the product of] GD by itself.
|
וא"ד בב"ג הוא כמו ג"ד בעצמו |
| [The product of] GD by itself is four squares minus two-fifths of a tenth of a square of a square.
|
וג"ד בעצמו הוא ארבעה אלגוש פחות שני חומשי עשירית מאלגו אלגו |
| Subtract from it the product of AB by itself, which is a square; three squares minus [two-fifths] of a tenth of a square of a square remains equal to the product of AB by GD. | ותגרע ממנו הכאת א"ב בעצמו והוא אלגו ישאר שלשה אלגוש פחות חמישית עשירית מאלגו אלגו ישוה להכאת א"ב בג"ד |
|
| |
| Divide three squares minus [two-fifths] of a tenth of a square of a square by line AB, which is a thing; the result is line GD, which is three things minus [two-fifths] of a tenth of a cube. | ותחלק שלשה אלגוש פחות חמישית עשירית מאלגו אלגו על קו א"ב והוא דבר ויעלה קו ג"ד שלשה דברים פחות חמישית עשירית ממעוקב בעצמו |
|
| |
| So, it is nine squares plus one part of 625 parts of a cube-cube minus six parts of 25 parts of a square of a square equals four squares minus two-fifths of a tenth of a square of a square.
|
ויהיו תשעה אלגוש וחלק אחד מתרכ"ה חלקים ממעוקב מעוקב פחות ששה חלקים מכ"ה חלקים מאלגו אלגו ישוו ארבעה אלגוש פחות שני חומשי עשירית מאלגו אלגו |
| Confrontation: Confront them; it is a fifth of a square of a square equals five squares and one part of 625 parts of a cube-cube.
|
ותנכח עמהם ויהיה חמישית האלגו אלגו ישוה חמשה אלגוש וחלק אחד מתרכ"ה חלקים ממעוקב מעוקב |
| Divide everything you have by a square.
|
ותחלק כל הדברים שתחזיק על אלגו |
| The result is five dirham plus one part of 625 of a square of a square equals a fifth of a square.
|
ויעלה חמשה אדרהמי וחלק אחד מתרכ"ה מאלגו אלגו ישוה חמישית האלגו |
| Normalization: Complete your square, so it becomes a [whole] square of a square, by multiplying it by 625.
|
ותשלים האלגו שלך עד שיהיה אלגו אלגו והוא שתכהו בתרכ"ה |
| It is a square of a square plus 3125 dirham equals 125 squares.
|
ויהיה אלגו אלגו ושלשת אלפים וקכ"ה אדראהמי ישוו קכ"ה אלגוש |
| Halve [the number of] the squares; it is 62 and a half. | ותחצה האלגוש ויהיו ס"ב וחצי |
| Multiply it by itself; it is 3906 and a quarter. | ותכה אותם בעצמם ויהיה שלשת אלפים ותתק"ו ורביע |
| Subtract 3125 from it; 781 and a quarter remains. | תגרע מהם ג' אלפים וקכ"ה וישאר תשפ"א ורביע |
| We subtract its root from 62 and a half. | ושרש נגרע מס"ב וחצי |
| The root of the remainder is line HD, which is one of the sides of the pentagon. | ושרש הנשאר הוא קו ה"ד שהוא אחד מהצלעות מהמחומש |
|
| |
| Q.E.D. | והוא מה שרצינו לבאר |
Finding the tithe of the circle when the fifth of the circle is known |
|
| When we wish to know the size of the line that cuts a tithe of a known circle, when the line that cuts a fifth of the circle is known: | וכאשר רצינו לדעת שעור הקו החותך עשירית עגולה ידועה וידענו הקו החותך חמישית העגולה |
|
|
|
| We suppose its circumferential line is ABGDHC. | נניח הקו המקיף בה אב"גדה"ח |
| We suppose its diameter, which is line AC, is ten.
|
ונניח קוטרה עשרה במספר והוא קו א"ח |
| We draw on its half five equal lines, each of which cuts a tithe of the circle, these lines are: AB, BG, GD, DH, HC. | ונניח בחצייה חמשה קוים שוים וכל אחד מהם חותך עשירית העגולה והם קוי א"ב ב"ג ג"ד ד"ה ה"ח |
| It is known that line DC cuts a fifth of this circle. | והוא ידוע כי קו ד"ח הוא קו חותך לחמישית מזאת העגולה |
| We have already explained that when it is multiplied by itself, it is sixty-two and a half minus a root of 781 and a quarter.
|
וכבר בארנו כי כשהוכה בעצמו הוא ששים ושנים וחצי פחות שרש מתשפ"א ורביע וקו א"ג בעצמו |
| The product of AG by DC plus [the product of] GD by AC is the same as [the product of] AD by GC.
|
והכאת א"ג בד"ח וג"ד בא"ח יהיו כמו א"ד בג"ח |
| AG is the same as DC.
|
וא"ג כמו ד"ח |
| The product of DC by itself plus [the product of] GD by AC is the same as the product of GC by itself.
|
והכאת ד"ח בעצמו [3]וג"ד בא"ח יהיה הכאת ג"ח בעצמו |
| Line GD cuts a tithe of the circle. | וקו ג"ד הוא קו חותך עשירית זאת העגולה |
We suppose it is a thing [ ]. ].
|
ונניחהו דבר |
| Multiply it by line AC, which is the diameter of the circle and it is ten; it is ten things.
|
ותכה אותו בקו א"ח שהוא קוטר העגולה והוא עשרה ויהיה עשרה דברים |
| Multiply line DC by itself; it is 62 and a half minus a root of 781 and a quarter.
|
ותכה קו ד"ח בעצמו ויהיה ס"ב וחצי פחות שרש מתשפ"א ורביע |
| [The product of] line GC [by itself] is 62 and a half plus ten things minus a root of 781 and a quarter.
|
ויהיה קו ג"ח בס"ב וחצי ועשרה דברים פחות שרש מתשפ"א ורביע |
| Add to it the product of AG by itself, which is 62 and a half minus a root of 781 and a quarter; it is 125 dirham plus ten things minus a root of 31[25] dirham. | ותוסיף עליהם הכאת א"ג בעצמו והוא ס"ב וחצי פחות שרש מתשפ"א ורביע ויהיה קכ"ה אדרהמיש ועשרה דברים פחות שרש משלשת אלפים ומאה דרהמיש |
|
| |
| Confrontation: Confront them; it is after the confrontation a root of 3125 dirham minus 25 equals ten things.
|
ותנכח עמהם ויהיה אחרי הנכוחות שרש משלשת אלפים ומאה וקכ"ה אדרהמיש פחות כ"ה ישוה עשרה דברים |
| The thing equals a root of 31 and a quarter minus two and a half and it is line GD that cuts a tithe of the circle.
|
והדבר ישוה שרש מל"א ורביע פחות שנים וחצי והוא קו ג"ד שהוא קו חותך עשירית העגולה |
| Q.E.D. | והוא מה שרצינו לבאר |
Finding the side of a pentagon that circumscribes the circle |
|
| When you wish to know the size of the line that cuts one part of the regular pentagon that circumscribes the circle: | וכאשר תרצה לדעת שעור הקו החותך |
|
|
|
| We suppose the diameter of the circle is ten. | ונניח קוטר העגלה עשרה |
| We construct on it a cyclic regular pentagon, which is pentagon ABGDZ. | ונעשה עליה מחומש שוה הצלעות והזויות והוא מחומש אב"גד"ז |
| It is known that line TC cuts a fifth of the circle. | והוא ידוע כי קו ט"ח חותך חמישית העגלה |
| We have already explained that when it is multiplied by itself it is 62 and a half minus a root of 781 and a quarter.
|
וכבר בארנו כי כשהוכה בעצמו הוא ס"ב וחצי פחות שורש מתשפ"א ורביע |
| The square of TL is fifteen and five-eighths minus a root of forty-eight, a quarter, and five-eighths of an eighth.
|
ומרובע ט"ל חמשה עשר וחמשה שמיניות פחות שרש ארבעים ושמנה ורביע וחמשה שמיניות משמינית |
| Subtract it from the square of line TH, which is half the diameter and its square is twenty-five; the square of line HL remains nine, a quarter, an eighth and a root of 48, a half, a quarter and five-eighths of an eighth. | [4]תגרעם ממרובע קו ט"ה שהוא חצי הקוטר ומרובעו עשרים וחמשה וישאר מרובע קו ה"ל תשעה ורביע ושמינית ושרש ממ"ח וחצי ורביע וחמשה שמיניות משמינית |
|
| |
We suppose line AB, which is one of the sides of the pentagon that circumscribes the circle, is a thing [ ] and its square is a square [ ] and its square is a square [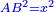 ]. ].
|
ונניח קו א"ב שהוא צלע אחד מצלעות המחומש שיקיף בעגולה דבר ומרובעו אלגו |
| The ratio of this square to the square of line BH, which is twenty-five, is as the ratio of the square of TC, which is the line that cuts a fifth of the pentagon that circumscribes the circle, which is 62 and a half minus a root of 781 and a quarter, to the square of HL, which is nine, a quarter, an eighth, and a root of 48, a half, a quarter and five-eighths of an eighth. | ושעור זה האלגו ממרובע קו ב"ה שהוא עשרים וחמשה כמו שעור מרובע ט"ח שהוא קו חותך חמשית המחומש המקיף בעגולה והוא [נ' בו העגולה][5] ס"ב וחצי פחות שרש מתשפ"א ורביע ממרובע ה"ל והוא תשעה ורביע ושמינית ושרש ממ"ח וחצי ורביע וחמשה שמיניות משמינית משמינית |
|
| |
| It is nine squares and three-eighths of a square plus a root of 48, six-eighths, and five-eighths of an eighth squares of a square; and this is equal to the square of KH [multiplied] by the square of TC, which is 1562 and a half minus a root of 488281 and a quarter. | ויהיה תשעה אלגוש ושלשה שמיניות מאלגו ושרש ממ"ח וששה שמיניות וחמשה שמיניות משמינית מאלגו אלגו וזה ישוה מרובע כ"ה במרובע ט"ח והוא אלף ותקס"ב וחצי פחות שרש מתפ"ח אלפים ורפ"א ורביע |
|
| |
| Normalization: Convert everything you have to a square, by multiplying it by a fifth plus a fifth of a fifth minus a root of twenty parts of 625 parts of a dirham.
|
ותשיב כל דבר שתחזיק אל אלגו והוא שתכה אותו בחמישית וחמישית מחמישית פחות שרש מעשרים חלקים מתרכ"ה חלקים מאדרהם |
| Multiply nine squares and three-eighths of a square plus a root of 48, three-quarters, and five-eighths of an eighth squares of a square, by a fifth plus a fifth of a fifth minus a root of twenty parts of 625 parts of a unit; it is a square. | ותכה תשעה אלגוש ושלשה שמיניות מאלגו ושרש ממ"ח אלגוש אלגו ושלשה רביעים מאלגו אלגו וחמשה שמיניות משמינית מאלגו אלגו בחמישית וחמישית מחמישית פחות שרש מעשרים חלקים מתרכ"ה חלקים מאחד ויהיה אלגו |
|
| |
| Multiply 1562 and a half minus a root of 488281 and a quarter by a fifth plus a fifth of a fifth minus a root of twenty parts of 625 parts of a unit; it is 375 dirham plus a root of 15625 minus a root of [78]125 dirham minus a root of 28[1]25 dirham, which is 500 minus a root of 200000. | ותכה אלף תקס"ב וחצי פחות שרש מתפ"ח אלפי' ורפ"א ורביע בחמישית וחמישית החמישית פחות שרש מעשרים חלקים מתרכ"ה חלקים מאחד ויהיה שע"ה אדרהמיש ושרש מט"ו אלפים ותרכ"ה פחות שרש מתת"ע אלף וקכ"ה אדרהמי ופחות שרש מכ"ח אלפי' וכ"ה אדרהמי וזה הוא ת"ק פחות שרש ממאתים אלף |
|
| |
| The root of this remainder is line AB, which is one of the sides of the regular pentagon that circumscribes the known circle, whose diameter is ten. | ושרש זה הנשאר הוא קו א"ב שהוא צלע אחד מהמחומש שוה הצלעות והזויות המקיף בעגולה ידועה אשר קוטרה עשרה במספר |
| It has already been clarified that the square of line AB, which is one part of the pentagon, is 500 minus two roots of two hundred thousand. Understand this. | וכבר התבאר שמרובע קו א"ב שהוא חלק אחד מהמחומש הוא ת"ק פחות שני שרשים ממאתים אלף והבן זה |
Finding the side of a decagon that circumscribes the circle |
|
| When you wish to know the size of the line that cuts a tithe part of the regular decagon that circumscribes the circle: | וכאשר תרצה לדעת שעור קו החותך עשירית המעושר השוה הצלעות והזויות המקיף בעגולה ידועה |
|
|
|
| Construct a circle whose diameter is ten. | תעשה עגולה יהיה קוטרה עשרה במספר |
| Construct on it a decagon that circumscribes the circle, which is decagon ABGDESCQZM. | ותעשה עליה מעושר אחד שיקיף בעגלה והוא מעושר [6]אבג"ד עסצ"ק ז"מ |
| We wish to know the size of line AB, which is one side of the decagon. | ונרצה לדעת שעור קו א"ב שהוא צלע אחד מהמעושר |
| It is already known that line TC cuts a tithe of the decagon of the circle. | כבר הוא ידוע שקו ט"ח הוא קו חותך עשירית המעושר אשר בעגולה |
| We have explained that it is a root of 31 and a quarter minus two and a half.
|
ובארנו שהוא שרש מל"א ורביע פחות שנים וחצי |
| Line TL is its half, which is a root of seven, a half, a quarter, and half an eighth minus one and a quarter.
|
וקו ט"ל הוא חציו והוא שרש משבעה וחצי ורביע וחצי שמינית פחות אחד ורביע |
| Its square is nine, a quarter, and an eighth minus a root of 48, a half, a quarter and five-eighths of an eighth.
|
ומרובעו הוא תשעה ורביע ושמינית פחות שרש ממ"ח וחצי ורביע וחמשה שמיניות משמינית |
| Subtract it from the square of TH, which is twenty-five; the square of HL remains, which is fifteen, five-eighths, and a root of 48, a half, a quarter and five-eighths of an eighth. | ותגרעם ממרובע ט"ה והוא עשרים וחמשה וישאר מרובע ה"ל חמשה עשר וחמשה שמיניות ושרש ממ"ח וחצי ורביע וחמשה שמיניות משמינית |
|
| |
| The square of TC is thirty-seven and a half minus a root of 781 and a quarter.
|
ומרובע ט"ח שלשים ושבע וחצי פחות שרש מתשפ"א ורביע |
We suppose line AB is a thing [ ] which is one side of the decagon that circumscribes the circle, and its square is a square [ ] which is one side of the decagon that circumscribes the circle, and its square is a square [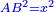 ]. ].
|
ונניח קו א"ב דבר והוא צלע אחד מצלעות המעושר אשר יקיף בעגולה ומרובעו אלגו |
| The ratio of the square to the square of TC, which is thirty-seven and a half minus a root of 781 and a quarter, is the same as the ratio of the square of KH, which is twenty-five, to the square of HL, which is fifteen, five-eighths, and a root of 48, a half, a quarter and five-eighths of an eighth. | ויחס האלגו אל מרובע ט"ח שהוא שלשים ושבע וחצי פחות שרש מתשפ"א ורביע הוא כיחס מרובע כ"ה שהוא עשרים וחמשה אל מרובע ה"ל שהוא חמשה עשר וחמשה שמיניות ושרש ממ"ח וחצי ורביע וחמשה שמיניות משמינית |
|
| |
| Multiply it by the square of AB, which is a square; it is 15 squares and 5-eighths of a square plus a root of 48, 3-quarters, and 5-eighths of an eighth squares of a square equals the square of KH [multiplied] by the square of TC, which is 937 and a half minus a root of 488281. | ותכה אותם במרובע א"ב שהוא אלגו ויהיה ט"ו אלגוש וה' שמיניות מאלגו ושרש ממ"ח אלגוש מאלגו וג' רביעים מאלגו אלגו וה' שמיניות משמינית מאלגו אלגו ישוה מרובע כ"ה במרובע ט"ח והוא תתקל"ז וחצי פחות שרש מתפ"ח אלפים ורפ"א |
|
| |
| Normalization: Convert everything you have to a square, by multiplying everything you have by two-fifths of a fifth minus a root of four-fifths of one part of 625 parts of a unit. | ותשיב כל דבר שתחזיק אל אלגו והוא שתכה כל מה שתחזיק בשני חמישיות מחמישית האחד פחות שרש מארבעה חמישיות מחלק אחד מתרכ"ה חלקים מאחד |
|
| |
| It is a square equals 75 dirham and a root of 625 dirham minus a root of 3125 minus a root of 1125, which is one hundred dirham minus a root of eight thousand and this is the square of line AB, which is one side of the decagon that circumscribes the known circle, whose diameter is ten.
|
ויהיה אלגו ישוה ע"ה דרהמי ושרש מתרכ"ה דרהמי פחות שרש משלשת אלפים וקכ"ה ופחות שרש מאלף וקכ"ה אדרהמי וזה הוא מאה דרהמי פחות שרש משמנת אלפים [7]והוא מרובע קו א"ב שהוא צלע אחד מהמעושר אשר יקיף בעגולה ידועה אשר קוטרה עשרה |
| Q.E.D. | והוא מה שרצינו לבאר |
Finding the diameter of a circle that circumscribes a pentagon |
|
| When you wish to know the size of the diameter of a circle that circumscribes a known regular pentagon: | וכאשר תרצה לדעת שעור קוטר עגולה המקפת במחומש ידוע שוה הצלעות והזויות |
|
|
|
| We suppose the known pentagon is pentagon ABGDH. | נניח המחומש הידוע מחומש אבגד"ה |
| We suppose each of its sides is ten. | ונניח כל צלע ממנו עשרה במספר |
| There is a circle that circumscribes it, whose diameter is line KLD. | ועליו עגולה תקיף בו וקטרה קו כל"ד |
| To know its diameter: | ולדעת קטרה |
We draw line HG and suppose it is a thing [ ]. ].
|
שנוציא קו ה"ג ונשימהו דבר |
| We explain that its product by AB, which is ten, plus [the product of] AB by itself equals the product of HG by itself:
|
ונבאר שהכאתו בא"ב שהוא עשרה וא"ב בעצמו הוא שוה להכאת ה"ג בעצמו |
| The product of HG, which is a thing, by AB, which is ten, is ten things.
|
והכאת ה"ג בעצמו שהוא דבר בא"ב שהוא עשרה יהיה עשרה דברים |
| [The product of] AB by itself is one hundred.
|
וא"ב בעצמו מאה |
| [The product of] HG by itself is a square.
|
וה"ג בעצמו אלגו |
| So, it is a square equals ten things and one hundred dirham.
|
ויהיה אלגו ישוה עשרה דברים ומאה דרהמי |
| The thing equals five and a root of 125 and this is line HG.
|
והדבר ישוה חמשה ושרש מקכ"ה והוא קו ה"ג |
| Its half is two dirham, a half and a root of 31 and a quarter and this is line HL.
|
וחציו שני דרהמי וחצי ושרש מל"א ורביע והוא קו ה"ל |
| Multiply it by itself; it is 37 and a half plus a root of 781 and a quarter.
|
תכה אותו בעצמו ויהיה ל"ז וחצי ושרש מתשפ"א ורביע |
| Subtract it from [the product of] HD by itself, which is one hundred; the remainder is 62 and a half minus a root of 781 and a quarter, which is a square of line DL. | תגרעם מה"ד בעצמו שהוא מאה ישאר ס"ב וחצי פחות שרש מתשפ"א ורביע והוא מרובע קו ד"ל |
|
| |
We suppose the diameter of the circle, which is line KLD, is a thing [ ] and its square is a square [ ] and its square is a square [ ]. ].
|
ונניח קטר העגולה שהוא קו כל"ד דבר ומרובעו אלגו |
| The ratio [of the square] of DK to the square of HD, which is one hundred, is the same as the ratio of the square of HD to the square of LD, which is 62 and a half minus a root of 781 and a quarter. | ויחס ד"כ אל מרובע ה"ד שהוא מאה הוא כיחס מרובע ה"ד אל מרובע ל"ד והוא ס"ב וחצי פחות שרש מתשפ"א ורביע |
|
| |
| Multiply it by a square; it is 62 squares and a half minus a root of 781 and a quarter squares of a square equals the square of HD, which is ten thousand.
|
ותכה אותו באלגו ויהיה ס"ב אלגוש וחצי פחות שרש מתשפ"א ורביע אלגו אלגו ישוה רביע מרובע מה"ד בעצמו והוא עשרת אלפים |
| Normalization: Convert everything you have to a square, by multiplying everything you have by a fifth of a tenth plus a root of one part and a quarter of one part of 15625 parts of a unit. | ותשיב כל דבר שתחזיק אל אלגו והוא שתכה כל מה שתחזיק באלגו מחמישית אחת מעשירית אחד ושרש מחלק אחד ורביע מט"ו אלפים ותרכ"ה חלקים מאחד |
|
| |
| It is a square equals two hundred and a root of eight thousand, so it is clear that the square of a diameter of the circle, which is line KLD is two hundred and a root of eight thousand.
|
ויהיה אלגו ישוה מאתים ושרש שמנת אלפים וכבר התבאר כי מרובע קוטר העגולה שהוא קו כל"ד הוא מאתים ושרש משמנת אלפים |
| Q.E.D. | וזה מה שרצינו לבאר |
Finding the diameter of an inscribed circle that is contained in a pentagon |
|
| When we wish to know the diameter of the inscribed circle that is contained in the known regular pentagon ABGDH, each of its sides is ten: | [8]וכאשר נרצה לדעת קוטר העגולה המקפת במחומש אבגד"ה הידוע שוה הצלעות והזויות והוא שכל צלע מצלעותיו עשרה במספר |
|
|
|
| The center of the circle is point M. | ומרכז העגולה נקדת מ' |
| Half its diameter [= radius of the inscribed circle] is line KM. | וחצי קוטרה קו כ"מ |
| Half the diameter [= radius] of the circumscribing circle is line AM. | וחצי קטר העגולה המקפת במחומש הוא קו א"מ |
| We have explained that the square of its diameter is two hundred and a root of eight thousand.
|
ובארנו כי מרובע קטרו הוא מאתים ושרש משמנת אלפים |
| The square of AM, which is half the diameter, is fifty and a root of [five hundred].
|
ומרובע א"מ שהוא חצי הקטר יהיה חמשים ושרש מחמישית |
| Subtract from it the square of AK, which is twenty-five; the square of KM remains, which is half the diameter of the inscribed circle in this pentagon, which is twenty-five and [a root of 500]. | תגרע מהם מרובע א"כ והוא עשרים וחמשה ישאר מרובע כ"מ והוא חצי קוטר העגולה הנופלת בזה המחומש והוא עשרים וחמשה וחלק אחד מת"ק |
|
| |
| The square of the diameter of the inscribed circle in your pentagon is one hundred and a root of eight thousand.
|
ומרובע קטר העגולה אשר נפל המחומש שלך בתוכה הוא מאה ושרש שמנת אלפים |
| Q.E.D. | וזה הוא מה שרצינו לבאר |
| If you wish, multiply one side of the pentagon by itself, then subtract it from the product of the diameter of the circumscribing circle by itself, extract the root of the remainder, and the result is the diameter of the inscribed circle. | ואם תרצה תכה צלע אחד מהמחומש בעצמו ותגרעהו מהכאת הקטר מהעגולה אשר נפלה על זה המחומש בעצמו ותקח שרש הנשאר והיוצא הוא קוטר העגולה אשר נפלה תוך המחומש |
| This method is drawn from a regular figure. | וזה האופן יצא מצורה שות הצלעות והזויות |
| When we wish to know the size of the diameter of the inscribed circle that is contained in a known regular pentagon, each of its sides is ten, by a different procedure from the one we explained above: | וכאשר נרצה לדעת שעור קוטר עגולה מקפת במחומש ידוע שוה הצלעות והזויות וכל צלע ממנו עשרה במספר בזולת המעשה אשר בארנו קודם |
| It is known from Euclid's words that for any line that cuts a part of the circle, the ratio of this line to the line that cuts a part of another circle equal to the part that the said line cuts from the said circle is the same as the ratio of the diameters of the circles to each other. | יהיה ידוע ממה שאמר אוקלידס שכל קו יחתוך חלק אחד מהעגולה יהיה יחס זה הקו אל הקו אשר יחתוך מעגולה אחרת חלק שוה כמו החלק אשר חתך הקו האמור מהעגולה האמורה כיחס קטרי העגולות האחד באחר |
| We have already explained concerning the circle whose diameter is ten, that the square of the line that cuts a fifth of this circle is 62 and a half minus a root of 781 and a quarter. | וכבר בארנו כי העגולה אשר קטרה עשרה יהיה מרובע הקו החותך חמישית זאת העגולה ס"ב וחצי פחות שרש מתשפ"א ורביע |
| It is known from what we said that the ratio of one hundred to 62 and a half minus a root of 781 and a quarter is the same as the ratio of the square, which is the square of the diameter of the unknown inscribed circle of the pentagon, each side of which is ten, which we assumed to be a thing, to one hundred, which is the square of the diameter of the circumscribing circle of the known pentagon.
|
והוא ידוע ממה שאמרנו כי יחס המאה אל הס"ב וחצי פחות שרש מתשפ"א ורביע יהיה כיחס האלגו שהוא מרובע [9]קטר העגולה בלתי ידועה אשר יקיף בה המחומש אשר כל חלק ממנו עשרה בעבור כי |
| Multiply one hundred by one hundred; it is ten thousand.
|
ותכה מאה במאה יהיה עשרת אלפים |
| Multiply the square by 62 and a half minus a root of 781 and a quarter; it is 62 and a half squares minus a root of 781 and a quarter squares of a square equals ten thousand.
|
ותכה אלגו בס"ב וחצי פחות שרש מתשפ"א ורביע ויהיה ס"ב אלגוש וחצי אלגו פחות |
| Normalization: Convert everything you have to a square, by multiplying everything you have by a fifth of a tenth plus a root of one part and a quarter of one part of [15]625 parts of a unit. | ותשיב כל דבר שתחזיק אל אלגו והוא שתכה כל דבר שתחזיק בחמישית העשירית מאחד ושרש חלק אחד ורביע חלק מכ"ה אלפים ותרכ"ה חלקים מאחד |
|
| |
| It is a square equals two hundred dirham and a root of eight thousand and this is the square of the diameter of the inscribed circle contained in the pentagon, each side of which is ten.
|
ויהיה אלגו ישוה מאתים דרהמי ושרש משמנת אלפים והוא מרובע קטר העגולה המקפת במחומש כאשר היה כל צלע ממנו עשרה |
Finding the diameter of a circle that is inscribed by a pentagon |
|
| When we wish to draw in a known regular pentagon, each side of which is ten, an inscribed circle contained by the pentagon: | וכן כאשר נרצה לעשות במחומש ידוע שוה הצלעות והזויות וכל צלע ממנו הוא עשירית עגלה אשר יקיף בה המחומש |
| We have already explained concerning the circle, whose diameter is ten, that the square of each side of the pentagon surrounding the circle is 500 minus a root of two hundred thousand. | וכבר בארנו כי עגולה אשר יהיה קוטרה עשרה יהיה המרובע מכל צלע מהמחומש המקיף בעגולה ת"ק פחות שרש ממאתים אלף |
| I say that [the ratio of one hundred] to the part of the pentagon, which is 500 minus a root of two hundred thousand, is as [the ratio] of the square, which is the square of the required [diameter of the] unknown circle, to the diameter of the known circle, which is one hundred.
|
ואומר ויהיה החלק מהמחומש מת"ק פחות שרש ממאתים אלף יהיה אלגו והוא מרובע העגולה הבלתי ידועה המבוקש כקטרה אל קטר העגולה הידועה והוא מאה |
| Multiply one hundred by one hundred; it is ten thousand.
|
ותכה מאה במאה ויהיה עשרת אלפים |
| Multiply the square by 500 minus a root of two hundred thousand; it is 500 squares minus a root of two hundred thousand squares of a square equals ten thousand dirham.
|
ותכה אלגו בת"ק פחות שרש ממאתים אלף ויהיה ת"ק אלגוש פחות שרש ממאתים אלף אלגו אלגו ישוה עשרת אלפים דרהמי |
| Normalization: Convert everything you have to a square, by multiplying everything you have by a tenth of a tenth plus a root of one part of 12500 parts of a unit. | ותשיב כל דבר שתחזיק אל אלגו והוא שתכה כל מה שתחזיק בעשירית העשירית מאחד ושרש חלק אחד מי"ב אלפים ות"ק חלקים מאחד |
|
| |
| The square equals one hundred and a root of eight thousand and this is the square of the diameter of the inscribed circle contained by the pentagon, each side of which is ten.
|
ויהיה האלגו ישוה מאה ושרש משמנת אלפים והוא מרובע הקטר מן העגולה אשר נפלה בזה המחומש אשר כל צלע מצלעיו עשרה |
Finding the diameter of a circle that is inscribed by a decagon |
|
| When we wish to construct in a known regular decagon, each side of which is ten, an inscribed circle contained by the decagon: | וכן כאשר נרצה לעשות על מעושר ידוע שוה הצלעות והזויות אשר כל צלע ממנו הוא עשירית העגולה המקפת בזה המעושר |
| We have already explained concerning the circle, whose diameter is ten, that the [side] of the decagon inscribed in it is a root of 31 and a quarter minus two and a half. | וכבר בארנו כי עגלה שיהיה קטרה עשרה שעגלת המעושר אשר נפל בתוכה הוא שרש מל"א ורביע פחות שנים וחצי |
We suppose the diameter of the circle is a thing [ ]. ].
|
ונניח קטר העגולה דבר |
| Say that the ratio of the thing to ten is the same as the ratio of ten to the root of 31 and a quarter minus two and a half.
|
ותאמר כי יחס הדבר אל עשרה יהיה כמו יחס העשרה אל שרש ל"א ורביע פחות שנים וחצי |
| Multiply ten by ten; it is a hundred.
|
[10]ותכה עשרה בעשרה יהיה מאה |
| Multiply a thing by a root of 31 and a quarter minus two and a half; it is a root of 31 squares and a quarter minus two things and a half. | ותכה דבר בשרש מל"א ורביע פחות שנים וחצי ויהיה שרש מל"א אלגו ורביע פחות שני דברים וחצי |
|
| |
| Restore it with two things and a half; it equals a root of 31 squares and a quarter. | ותאספם עם השני דברים וחצי ישוה שרש מל"א אלגו ורביע |
|
| |
| Multiply one hundred dirham plus [two] thing[s] and a half by themselves; it is ten thousand dirham plus six squares and a quarter and 500 things equals 31 squares and a quarter. | ותכה מאה דרהמי ודבר וחצי בעצמו ויהיה עשרת אלפים אדרהמי וששה אלגוש ורביע ות"ק דברים ישוה ל"א אלגו ורביע |
|
| |
| Confront them; the thing is ten plus a root of 500 and this is the root of the diameter of the inscribed circle of the decagon, each side of which is ten.
|
ותנכחם עמהם ויהיה הדבר עשרה ושרש מת"ק והוא שרש קטר עגלת המעושר אשר כל צלע מצלעותיו עשרה |
Finding the diameter of a circle circumscribed about a decagon |
|
| When we wish to construct a circle circumscribed about a known regular decagon, each side of which is ten: | וכן כאשר נרצה לעשות במעושר ידוע שוה הצלעות והזויות אשר כל צלע ממנו עשרה עגולה אחת תקיף בזה המעושר |
| We have already explained concerning the circle, whose diameter is ten, that the square of the side of the circumscribed decagon is one hundred minus a root of eight thousand. | וכבר בארנו כי עגלה אשר קוטרה עשרה יהיה מרובע הקו אשר הוא חלק אחד מהמעושר שנפל עליו הוא מאה פחות שרש משמנת אלפים |
| I say that the ratio of one hundred minus a root of eight thousand to one hundred is the same as its ratio to the square.
|
ואומר כי יחס מאה פחות שרש משמנת אלפים אל מאה יהיה כיחסם אל אלגו |
| Multiply it by itself; it is ten thousand.
|
תכה אותם בעצמם יהיו עשרת אלפים |
| Multiply one hundred minus a root of eight thousand by a square; it is ten thousand times one hundred squares minus a root of eight thousand squares of a square equals ten thousand. | ותכה מאה פחות שרש משמנת אלפים באלגו ויהיה עשרת אלפים פעם מאה אלגוש פחות שרש משמנת אלפים אלגו אלגו ישוה עשרת אלפים |
|
| |
| Normalization: Reduce everything you have into one square by multiplying it by half a tenth plus a root of one part of 500.
|
ותשיב כל אשר תחזיק אל אלגו והוא שתכה אותו בחצי עשירית אחד ושרש חלק אחד מת"ק חלקים מאחד |
| The square is equal to 50 plus a root of two hundred thousand and this is the square of the diameter of the circle circumscribed about the decagon.
|
ויהיה האלגו ישוה ת"ק ושרש ממאתים אלף והוא מרובע קטר העגולה אשר נפלה חוץ מן המעושר בעצמו |
| We have already explained that if the diameter is ten plus a root of 500, its square is a hundred plus a root of two hundred thousand. | וכבר בארנו שאם הקוטר הוא עשרה ושרש מת"ק יהיה מרובעו מאה ושרש ממאתים אלף |
| Subtract a hundred from it, which is the product of one side of the [inscribed] decagon by itself; the remainder is 500 plus a root of two hundred thousand, and this is the diameter of the circle circumscribed about the decagon. | תגרע מהם מאה והוא הכאת צלע אחד מהמעושר בעצמו ישאר ת"ק ושרש ממאתים אלף ושרש זה הוא קטר העגולה אשר נפלה חוץ מזה המעושר |
| I will explain the reason for this procedure: | ואניח סבת זה האופן |
| It is because when a regular figure is circumscribed about a circle and inscribed in a circle, [the sum of] the product of one of the sides [of that figure] by itself and the product of the diameter of the inscribed circle by itself equals the product of the diameter of the circumscribed circle by itself. | והוא כי כל תמונה שות הצלעות והזויות אשר תקיף בעגולה ותקיף בה עגולה הנה הכאת אחת הצלעות בעצמה והכאת קטר העגולה אשר נפלה פנימה בעצמו יהיו שתי אלו ההכאות יחד כמו הכאת קטר העגולה המקפת בתמונה בעצמו |
|
|
|
| Example: We draw an equilateral triangle, let it be the triangle ABG. | דמיון זה שנעשה משולש שוה הצלעות והזויות והוא משולש אב"ג |
| In the triangle the inscribed circle CZL. | ובתוכו עגולה יקיף בה המשולש עליה חז"ל |
| Another circle ABG is circumscribed about the triangle, the diameter of which is line AD. | ותקיף למשולש עגולה אחרת עליה אב"ג וקטרה קו א"ד |
| The diameter of the inscribed circle is line KE. | וקטר העגלה [11]הפנימית מהמשולש הוא קו כ"ע |
| I say that [the sum of] the product of AG by itself and KE by itself is the same as the product of AD by itself.
|
ואומר שהכאת א"ג בעצמו וכ"ע בעצמו יהיו כמו הכאת א"ד בעצמו |
| Proof: We draw a perpendicular line bisecting the inner circle, from line AG, at point L, which is where the line is tangent to the inner circle. | |
| It is known that it passes through the center of the two circles. | והוא והוא ידוע שיעבור על מרכז שתי העגולות |
| We extend it to the point of intersection with circle CZL, let it be line LZ. | ונוציאהו עד שיעבור אל מקיף זאת העגולה היא עגולת חז"ל והוא קו ל"ז |
| This line is the same as the diameter of the CZL circle and is equal to the line KE. | ויהיה זה הקו כמו קטר עגולת חז"ל והוא שוה לקו כ"ע |
| We attach point Z to point D by line ZD. | ונדביק נקדת ז' בנקדת ד' עם קו ז"ד |
| We attach point D to point G by line GD. | ונדביק ד' בג' עם קו ג"ד |
| Line AN is equal to line MD.
|
ויהיה קו א"מ שווה לקו מ"ד |
| Line ML is equal to line MZ.
|
וקו מ"ל כמו קו מ"ז |
| Angle AML is the same as angle DMZ.
|
וזוית אמ"ל כמו זוית דמ"ז |
| Line DZ is the same as line AL.
|
וקו ד"ז כמו קו א"ל |
| AL is the same as LG.
|
וא"ל כמו ל"ג |
| Angle GLZ is the same as [angle] DZL.
|
וזוית גל"ז כמו דז"ל |
| Triangle AML is the same as triangle DMZ.
|
ומשולש אמ"ל כמו משולש דמ"ז |
| The angles of the one are equal to the angles of the other. | וזויות האחד כמו זויות האחר |
| Angle ALM is the same as angle MZD.
|
וזוית אל"מ כמו זוית מז"ד |
| Line ZD is equal to line LG.
|
וקו ז"ד יהיה שוה לקו ל"ג |
| We have already explained that they are equal and that the two ends of each of the two lines are attached to lines ZL and DG. | וכבר בארנו שהם שוים ושנדבקו שני ראשי כל |
| Line ZL is equal to line DG.
|
ויהיה קו ז"ל שוה לקו ד"ג |
| Multiply AG by itself and GD by itself; [their sum] is the same as the product of AD by itself.
|
ותכה א"ג בעצמו וג"ד בעצמו ויהיה כמו הכאת א"ד בעצמו |
| Since angle AGD is a right angle.
|
בעבור כי זוית אג"ד נצבת |
| DG is the same as LZ.
|
וד"ג הוא כמו ל"ז |
| LZ is the same as KE.
|
ול"ז כמו כ"ע |
| The [sum of the] product of AG by itself and the product of KE by itself is the same as the product of AD by itself.
|
והנה הכאת א"ג בעצמו וכ"ע בעצמו יהיו כמו הכאת א"ד בעצמו |
| Q.E.D. | והוא מה שרצינו לבאר |
Finding the side of a pentadecagon that is circumscribed by a circle |
|
| When we suppose a regular pentadecagon shape that is inscribed in a circle whose diameter is ten, its sides and angles are equal, and we wish to know the size of each of its sides. | וכאשר נניח תמונה בעלת חמש עשרה זויות שוות תקיף בה עגולה אשר קטרה עשרה והיא שות הצלעות והזויות ונרצה לדעת שעור כל צלע ממנה |
|
|
|
| We suppose the known circle is circle ABG. | נניח העגולה הידועה עגולת אב"ג |
| Its diameter is AB. | וקטרה א"ב |
| We construct in it a line that cuts off a tenth of the [circle], which is one part and a half of the side of the pentadecagon; let this line [= side of a decagon] be BD. We have already explained that it is equal to a root of 31 and a quarter minus two and a half.
|
ונעשה בתוכה צלע אחד יחתוך המעושר שלה והוא חלק וחצי מאחד מהחמשה עשר והוא קו ב"ד וכבר בארנו שהוא שרש מל"א ורביע פחות שנים וחצי |
| Line AB is its complement, and it is the line that cuts off two-fifths of the circle, so it is a root of 62 and a half [minus] a root of 781 [and a quarter].
|
וקו א"ד הוא תשלומה [12]והוא קו חותך שני חמשי העגולה והוא ס"ב וחצי ושרש מתשפ"א הנלקח שרשו |
| I draw a line in this circle that cuts off its sixth part, let it be line BG. It truncates two and a half parts of the pentadecagon. | וארשום בזאת העגולה קו חותך הששית והוא קו ב"ג והוא קו חותך שני חלקים וחצי מהחמש עשרה והוא חותך מחמשה |
| Line AG is its complement, which cuts off a third of the circle, and it is a root of 75.
|
וקו א"ג הוא תשלומה והוא קו חותך שלישית העגולה והוא שרש מע"ה |
| Multiply line BD, which is a root of 31 and a quarter minus two and a half, by line AG, which is a root of 75; it is a root of 2343, a half and a quarter minus a root of 468, a half and a quarter. | ותכה קו ב"ד והוא שרש מל"א ורביע פחות שנים וחצי בקו א"ג והוא שרש מע"ה ויהיה שרש מאלפיים ושמ"ג וחצי |
|
| |
| Subtract it from the product of line AD by line BG, which is a root of 1562 and a half plus a root of 48[8]281 and a quarter. | ותגרעם מהכאת קו א"ד בקו ב"ג והוא אלף ותקס"ב וחצי ושרש מת' אלפים ופ"א אלפים ורפ"א ורביע הלקוח שרשו וישאר אלף ותקס"ב וחצי ושרש מתפ"א אלפים ורפ"א ורביע הלקוח שרשו |
|
| |
| Add to it a root of 468, a half and a quarter and subtract from the total a root of 2343, a half and a quarter, which is the same as the product of line AB by line GD. | ותוסיף עליו שרש מתס"ח וחצי ורביע ותגרע מכל זה שרש מאלפיים ושמ"ג וחצי ורביעית והוא כמו הכאת קו א"ב בקו ג"ד |
| Divide the remainder by line AB, which is ten; the result is line GD, which is a root of fifteen and five-eighths plus a root of 48, three-quarters and five-eighths of an eighth plus a root of four dirham, a half, an eighth, and a half of an eighth minus a root of 23, three-eighths and a half of an eighth. | ומה שישאר תחלקהו על קו א"ב והוא עשרה ויעלה קו ג"ד חמשה עשר וחמשה שמיניות ושרש ממ"ח ושלשה רביעים וחמשה שמיניות משמינית לקוח שרשו ושרש מארבעה דרהמי וחצי ושמינית וחצי שמינית פחות שרש מכ"ג ושלשה שמיניות וחצי שמינית |
|
| |
| We explain the extraction of line GD so that you will understand it: extract the root of 48, three-quarters and five-eighths of an eighth it is six, 59 minutes, 15 seconds and 46 thirds. | ונדביק הוצאת ג"ד בעבור שתבינהו תקח שרש ממ"ח ושלשה רביעים וחמשה שמיניות משמינית פור לו מאש סירקאנו ויהיה ששה ונ"ט דקים וט"ו שניים ומ"ו שלישיים |
|
| |
| Add this to 15 and three-eighth; it is 22, 36 minutes, 45 seconds and 46 thirds. | תוסיפם על ט"ו ושלשה שמיניות [13]ויהיה כ"ב ול"ו דקים ומ"ה שניים ומ"ו שלישיים |
|
| |
| Extract its root; it is four, 45 minutes, 19 seconds and one third. | ותקח |
|
| |
| Add to it the root of 4, five-eighths, and a half of an eighth, which is two, 9 minutes, 54 seconds and 12 thirds. | ותוסיף עליו שרש מד' וחמשה שמיניות וחצי שמינית פור לו מאש סירקאנו והוא שנים וט' דקים ונ"ד שניים וי"ב שלישיים |
|
| |
| Subtract from it the root of 23, three-eighths and a half of an eighth, which is four dirham, 50 minutes, 28 seconds and 25 thirds. | תגרע מהם שרש מכ"ג ושלשה שמיניות וחצי שמינית והוא ארבעה דרהמי ונ' דקים וכ"ח שניים וכ"ה שלישיים |
|
| |
| The remainder is two, 4 minutes and 45 seconds and this is line GD.
|
וישאר שנים וד' דקים ומ"ה שניים והוא קו ג"ד פור לו מאש סירקאנו |
| Q.E.D. | והוא מה שרצינו לבאר |
Finding the height of an equilateral triangle |
|
| If you are told: an equilateral triangle whose area is ten, how much is its height? | ואם יאמרו לך משלש שוה הצלעות והזויות עשרה במספר כמה יהיה קטרו |
| We suppose the triangle is ABG. | ונניח המשולש אב"ג |
| Its height is AD. | וקטרו א"ד |
| We wish to know the size of AD, which is the height of triangle ABG. | ונרצה לדעת שעור א"ד שהוא קטר המשולש מאב"ג |
We suppose it is a thing [ ]. ].
|
נניחהו דבר |
| Line DG is a root of a third of the square.
|
ויהיה קו ד"ג שרש משלישית האלגו |
| Each side of triangle ABG is a root of a square and a third.
|
וכל חלק מהמשולש מאב"ג שרש מאלגו ושליש |
| The area of triangle ABG is a root of a third of a square of a square.
|
ושעור משלש אב"ג שרש משלישית אלגו אלגו |
Its height is a thing [ ]. ].
|
וקוטרו דבר |
| So, a thing plus a root of a third of a square of a square equals ten.
|
ויהיה דבר ושרש משלישית אלגו אלגו ישוה עשרה |
| Normalization: Restore the root of a third of a square of a square, so its will become a root of a square of a square, which is a square - restore it by multiply it by a root of three and multiply all you have by a root of three.
|
ותשלים שרש משלישית אלגו אלגו עד שיהיה שרש אלגו אלגו והוא אלגו והשלימך אותו הוא שתכה אותו בשרש שלשה ותכה כל מה שתחזיק בשרש משלשה |
| Hence, the square plus a root of three squares equals a root of three hundred.
|
ויהיה אלגו ושרש שלשה אלגוש ישוה שרש משלש מאות |
| Halve the root of three squares; it is a root of three-quarters | ותחצה שרש משלשה אלגוש ויהיה שרש משלשה רביעים |
| Multiply it by itself; it is three-quarters. | תכה אותו בעצמו ויהיה שלשה רביעים |
| Add it to a root of three hundred; it is three-quarters and a root of three hundreds. | תוסיפם על שרש משלש מאות יהיה שלשה רביעים ושרש משלש מאות |
| Extract its root and subtract a root of three-quarters from it; the remainder is line AD, which is the height of triangle ABG. | תקח שרשו ותגרע ממנו שרש משלשה רביעים ומה שישאר הוא קו א"ד שהוא קטר משלש אב"ג |
|
| |
| Q.E.D. | והוא מה שרצינו לבאר |
Finding the length of a rectangle that is encompassed by an equilateral triangle |
|
| If you are told: an equilateral triangle, each side of which is ten, inside it there is a rectangle, whose area is ten, how much is the length of the rectangle? | ואם יאמרו לך משלש שוה הצלעות והזויות כל חלק ממנו עשרה ובתוכו מרובע ארוך נצב הזויות ושעורו עשרה כמה יהיה אורך המרובע |
| Example: we suppose the triangle is triangle ABG. | דמיון זה שנשים המשולש משלש אב"ג |
| The rectangle in it is rectangle HZCT. | והמרובע ארוך שנפל בתוכו מרובע הזח"ט |
| We wish to know the size of line CH, which is the length of the rectangle. | ורצינו לדעת שעור קו ח"ה שהוא אורך המרובע |
We suppose it is a thing [ ]. ].
|
ונשימהו דבר |
| Line BC is a root of a third of the square.
|
ויהיה קו ב"ח שרש משלישית האלגו |
| Line TG is also a root of a third of the square.
|
וכן הוא קו ט"ג שרש משלישית אלגו |
| Line CT that remains is ten minus a root of a square and a third.
|
[14]ישאר קו ח"ט עשרה פחות שרש מאלגו ושליש |
| Multiply it by line HC, which is a thing; it is ten [things] minus a root of a square of a square and a third of a square of a square equals ten dirham. | תכה אותו בקו ה"ח והוא דבר יהיה עשרה פחות שרש מאלגו אלגו ושלישית מאלגו אלגו ישוה עשרה דרהמי |
|
| |
| Restore the things with the root of a square of a square and a third of a square of a square by adding them to the dirham. | ותאסוף הדברי' עם שרש מאלגו אלגו ושלישית מאלגו אלגו ותוסיפם על האדרהמיש |
| [So], ten dirham plus a root of a square of a square and a third of a square of a square equals ten things.
|
ותכה עשרה דרהמי ושרש מאלגו אלגו ושלישית אלגו אלגו ישוה עשרה דברים |
| Normalization: Restore everything you have into a root of a square of a square, which is a square, by multiplying it by a root of three-quarters - multiply all you have by a root of three-quarters.
|
ותשיב כל |
| It is a square plus a root of 75 dirham equals a root of 75 squares.
|
ויהיה אלגו ושרש מע"ה דרהמי ישוה שרש מע"ה |
| Halve the root of 75; it is a root of 18, a half and a quarter. | ותחצה שרש מע"ה ויהיה שרש מי"ח וחצי ורביע |
| Multiply it by itself; it is 18 and 3-quarters. | תכה אותו בעצמו ויהיה י"ח וג' רביעים |
| Subtract a root of 75 from it; the remainder is 18, a half and a quarter minus a root of 75. | תגרע מהם שרש מע"ה ישאר י"ח וחצי ורביע פחות שרש מע"ה |
| Extract its root and add the result to 18 a half and a quarter; the sum is the length of the rectangle, which is line HC. | ותקח שרשו ומה שיעלה תוסיפהו על י"ח וחצי ורביע והמקובץ הוא אורך המרובע שהוא קו ה"ח |
|
| |
Finding the side of a square that is contained in an equilateral triangle |
|
| If you are told: an equilateral triangle ABG, inside it there is a square CLEM. The measure of [the sum of] the triangle and the square is ten, how much is each side of the square CLEM and how much are the areas of the triangle and the square? | ואם יאמרו לך משלש שוה הצלעות והזויות עליו אב"ג ובתוכו מרובע נצב הזויות ושוה הצלעות עליו חלע"מ ושעור המשולש והמרובע עשרה כמה יהיה כל חלק ממרובע חלע"מ וכמה יהיה שעור המשולש והמרובע |
To know this we assume each side of the square is a thing [ ]. ].
|
ולדעת זה נשים כל חלק מהמרובע דבר |
Its area is a square [ ] ]
|
ויהיה שעורו אלגו |
| The remaining area of triangle ABG is ten minus a square.
|
וישאר שעור משולש אב"ג עשרה פחות אלגו |
The area of the square is a square [ ]. ].
|
ושעור המרובע אלגו |
| The remaining size of triangles BLC, MEG, ACE is ten minus two squares.
|
וישאר שעור משלשי בל"ח ומע"ג ואח"ע עשרה פחות שני אלגוש |
| We assumed each side of the square is a thing, which is line ME.
|
והנחנו כל חלק מהמרובע דבר ויהיה קו מ"ע |
| Line MG is a root of a third of a square.
|
וקו מ"ג שרש שרש שלישית אלגו |
| The area of triangle MGE is [half a root of a third of a square of a square]
|
ושעור משלש מע"ג |
| Line LB is a root of a third of a square of a square.
|
וקו ל"ב שרש שלישית אלגו אלגו |
| Line AT is a root of three-quarters of a square.
|
וקו א"ט שרש משלשה רביעיות מאלגו |
| The area of triangle ACE is a root of an eighth of a square of a square and half an eighth of a square of a square
|
ושעור משלש אח"ע שרש משמינית אלגו אלגו וחצי שמיני' אלגו אלגו |
| The [sum of the areas of] triangles MEG, CBL, CEA is a root of a third of a square of a square, and a root of an eighth of a square of a square plus half an eighth of a square of a square, which equals ten minus two squares. | ויהיה משלש מע"ג ומשלש חב"ל ומשלש חע"א שרש משלישית אלגו אלגו ושרש משמינית אלגו אלגו וחצי שמינית מאלגו אלגו וישוו עשרה פחות שני אלגוש |
|
| |
| Normalization: Restore the root of a third of a square of a square, so it becomes a root of a square [of a square], which is a square, by multiplying it by a root of three: | [15]ותשלים שרש משלישית אלגו אלגו עד שיהיה שרש מאלגו והוא אלגו והשלימך אותו הוא שתכה אותו בשרש משלשה |
|
| |
| Multiply a root of a third of a square of a square by a root of three; it is a root of a square of a square, which is a square.
|
תכה שרש שלישית אלגו אלגו בשרש משלשה יהיה שרש מאלגו אלגו והוא אלגו |
| Multiply a root of an eighth of a square of a square plus half an eighth of a square of a square by a root of three; it is a root of half a square of a square plus half an eighth of a square of a square, which is three-quarters of a square. | ותכה שרש שמינית אלגו אלגו וחצי שמינית מאלגו אלגו בשרש שלשה ויהיה שרש מחצי אלגו אלגו וחצי שמינית אלגו אלגו והוא שלשה רביעים מאלגו |
|
| |
| It is a square and three-quarters of a square.
|
ויהיה אלגו ושלשה רביעים מאלגו |
| Multiply ten minus two squares by a root of three; it is a root of three hundred minus a root of 12 squares of a square.
|
ותכה עשרה פחות שני אלגוש בשרש שלשה יהיה שרש משלש מאות פחות שרש מי"ב אלגו אלגו |
| So, a square plus three-quarters of a square equals a root of three hundred minus a root of 12 squares of a square.
|
ויהיה אלגו ושלשה רביעי אלגו ישוה שרש משלש מאות פחות שרש מי"ב אלגו אלגו |
| Restore the root of three hundred with a root of 12 squares of a square, by adding it to the square and three-quarters [of a square]; it is a square and three-quarters [of a square] plus a root of 12 squares of a square equals a root of three hundred.
|
ותשלים שרש משלש מאות עם שרש מי"ב אלגו אלגו ותוסיפהו על אלגו ושלשה רביעים ויהיה אלגו ושלשה רביעים ושרש מי"ב אלגו אלגו ישוה שרש משלש מאות |
| Normalization: Restore everything you have to a square, by multiplying everything you have by a root of 3072 parts of 20449 minus 28 parts of 143 dirham. | ותשיב כל דבר שתחזיק אל אלגו והוא שתכה כל מה שתחזיק בשרש משלשת אלפים וע"ב חלקים מעשרים אלף ותמ"ט חלקים מאחד פחות כ"ח חלקים מקמ"ג דרהמי |
|
| |
| It is a square equals a root of 45 dirham and 1395 parts of 2[0]449 minus a root of 11 dirham and 10261 parts 20449 parts of a dirham, which is the area of the square CLEM in triangle ABG.
|
ויהיה אלגו ישוה שרש ממ"ה דרהמי ואלף ושצ"ה חלקים מכ"ה אלפים ותמ"ט חלקים מאחד פחות שרש מי"א דרהמי ועשרת אלפי' ורס"א חלקים מכ' אלף ותמ"ט חלקים מאדרהם והוא שעור מרובע חלע"מ אשר במשולש אב"ג |
| Subtract it from ten and what remains is the area of triangle ABG.
|
תגרעהו מעשרה ומה שישאר הוא שעור משולש אב"ג |
| If you wish, say that the area of the square is six and 102 parts of 1[43] parts minus a root of 11 dirham and 10261 parts of 20449 parts of one dirham.
|
ואם תרצה תאמר כי שעור המרובע ששה וק"ב חלקים מקל"ב חלקים מאחד פחות שרש |
| And the area of the triangle is three and 41 parts of 143 plus a root of 11 and 10261 parts of 20449.
|
ושעור המשולש שלשה ומ"א חלקים מקמ"ג חלקים מאחד ושרש מי"א ועשרת אלפים ורס"א חלקים מעשרים אלפי' ותמ"ט חלקים מאחד |
Finding each side of a pentagon that is encompassed by a square |
|
| If you are told: a square, each side of which is ten. Let it be square ABGD. | ואם יאמרו לך מרובע שוה הצלעות והזויות נצבות וכל צלע ממנו עשרה והוא מרובע אבג"ד |
| We construct in it a [regular] pentagon, as this figure. Let it be pentagon AHCZM. | ועשינו בו מחומש אחד כמו זאת התמונה והוא מחומש אהחז"מ |
| We wish to know how much is the size of each side of the pentagon. | ורצינו לדעת כל צלע מצלעות המחומש כמה יהיה שעורו |
To know this, we suppose one side of the pentagon, which is line AH, is a thing [ ]. ].
|
ולדעת זה נניח החלק האחד מהמחומש והוא קו א"ה דבר |
| The remaining line CB is ten minus a root of a thing.
|
וישאר קו ח"ב עשרה פחות שרש |
| Line GZ is half a square.
|
וקו ג"ז חצי אלגו |
| Line BH is ten minus a thing.
|
וקו ב"ה עשרה פחות דבר |
| Multiply each of them by itself and sum them up; it is two hundred dirham plus a square and half a square, minus twenty roots, minus a root of two hundred squares, which is equal to a square.
|
ותכה כל אחד מהם בעצמו ותקבצם ויהיה מאתים דרהמי ואלגו וחצי אלגו פחות עשרים [16]שרשים ופחות שרש ופחות שרש ממאתים אלגוש ישוה אלגו |
| Confront them; line AH, which is one side of the pentagon is a root of two hundred plus a root of 320000 subtracted from twenty plus a root of two hundred.
|
ותנכחהו עמו ויהיה קו א"ה שהוא צלע אחד מהמחומש מאתים ושרש מש"כ אלפים כשילקח שרש זה ויגרע מעשרים ושרש מן מאתים |
| Q.E.D. | וזה הוא מה שרצינו לבאר |
| I, Mordechai, the author, translated what I found word for word, but I did not understand at all from this figure. | ואני מרדכי הכותב מה שמצאתי מלה במלה העתקתי אבל לא הבינותי כלל מזאת התמונה |
| It also seems to me that the appropriate figure that should be explained is a regular pentagon circumscribed by the square like the second figure. | גם נראה לי כי הצורה הראויה לבאר היא שיהיה גם המחומש שוה הצלעות והזויות יקיף בו המרובע כמו זאת התמונה השניה |
| But, after I finished drawing this second figure I understood it: the quadrangle that circumscribes the pentagon is a rectangle, not a true square. | ואחרי זה מדי השלימי לרשום זאת התמונה השניה הבינותי בה והנה המרובע |
| It seems that it is not possible to make a true square that circumscribes a regular pentagon, except as this third figure, that corresponds the first figure, which was copied from the foreign book. | ונראה שלא יתכן לעשות מרובע אמתי יקיף במחומש שוה הצלעות והזויות כי אם כמו זאת התמונה השלישית אשר פניה דורכות מול פני התמונה הראשונה אשר נעתקה מן הספר הלועז |
| I thought at first that the figure was wrong in the book, so I thought to correct it, as I corrected many of the other figures, and praise be to God who opened my eyes, and showed me wonderful things, which I recognize in his Torah. | וחשבתי ראשונה שהתמונה היתה משובשת בספר ובאתי לישרה כאשר יישרתי הרבה מן התמונות האחרות וישתבח השם שפתח עיני כן יראני נפלאות בתורתו אכיר' |
Finding each side of a regular pentagon whose area is fifty spans |
|
| If you are told: a regular pentagon whose area is fifty spans, how much is each of its sides? | [17]ואם יאמרו לך מחומש שוה הצלעות והזויות שעורו חמשים זרתות כמה יהיה כל חלק ממנו |
| Example: we suppose the pentagon is pentagon ABGDH. | דמיון זה שנניח המחומש מחומש אבגד"ה |
| The center of the circle circumscribed by the pentagon is point M. | ומרכז העגולה אשר יקיף בה זה המחומש נקדת מ' |
| We draw lines AM , MB, MG, MG, MH. | ונוציא קוי א"מ מ"ב מ"ג מ"ד מ"ה |
| It is known that this pentagon is divided into the equal triangles which are AMB, BMG, GMD, DMH, HMA. | והוא ידוע שנחלק זה המחומש למשולשים שוים והם אמ"ב במ"ג גמ"ד דמ"ה המ"א |
| Let triangle HMD be ten by number.
|
ויהיה משלש המ"ד עשרה במספר |
| We have already explained in what we have said previously in this book that when we assume a regular pentagon, each side of which is 10, the square of the diameter of the circumscribed circle is two hundred plus a root of eight thousand.
|
וכבר בארנו במה שאמרנו קודם בזה הספר שיהיה כאשר נניח מחומש שוה הצלעות והזויות כל חלק ממנו עשרה יהיה מרובע הקטר מהעגלה אשר תקיף בו מאתים ושרש משמנת אלפים |
| It is known from what we have said, that if we wish to know the diameter of a circle circumscribed by a given pentagon, we must multiply one side of the pentagon by itself, double [the product], and keep the result. | וידוע ממה שאמרנו שכאשר נרצה לדעת קטר עגולה מקפת במחומש ידוע שנכה חלק אחד מהמחומש בעצמו ונכפלהו ונשמרהו |
| Then, we must multiply one side of the pentagon by itself, we take four-fifths of [the product], and extract the root of the result. | ונכה חלק אחד מהמחומש בעצמו והעולה נכה בעצמו ונקח ארבעה חמשיו ומה שיצא תקח שרשו |
| Add [the root] to what you kept, and extract the root of this sum; the result is the diameter of the circle. | והעולה תוסיפהו על מה ששמרת ואשר יתקבץ תקח שרשו והעולה יהיה קטר העגולה |
| When we assume it is so, we suppose line HD is a thing and it is one side of the pentagon.
|
וכאשר נניח זה כן נניח קו ה"ד דבר והוא חלק אחד מהמחומש |
| Then, according to what we have explained, the square of the diameter of the circle is two squares and a root of four-fifths of a square of a square.
|
ויהיה מרובע קטר העגולה כפי מה שבארנו שני אלגוש ושרש מארבעה חמשים מאלגו אלגו |
| The square of half its diameter [= the radius], which is line EM, is half a square and a root of half a tenth of a square of a square.
|
ומרובע חצי קטרה והוא קו ה"מ הוא חצי אלגו ושרש חצי עשירית מאלגו אלגו |
| We draw the perpendicular of the triangle HMD. It is known that it falls in the midpoint of line HD. Let it be line MC. | ונוציא עמוד ממשולש המ"ד וידוע שיפול בנקדת חצי קו ה"ד והוא קו מ"ח |
| Let line HC be half a thing.
|
ויהיה קו ה"ח חצי דבר |
| Multiply it by itself; it is a quarter of a square.
|
תכה אותו בעצמו יהיה רביע אלגו |
| Subtract it from the square of line HM, which is half a square plus a root of half a tenth of a square of a square; the square of line MC remains a quarter of a square plus a root of half a tenth of a square of a square. | תגרעהו ממרובע קו ה"מ והוא חצי אלגו ושרש מחצי עשירית אלגו אלגו וישאר מרובע קו מ"ח רביע אלגו ושרש מחצי עשירית מאלגו אלגו |
|
| |
| Multiply it by [the square of] line HC, which is half a thing; it is half an eighth of a square of a square plus a root of [one] part of 320 parts of square-square-square-square equals ten multiplied by itself, which is one hundred. | תכה אותו בקו ה"ח והוא חצי דבר ויהיה חצי שמינית מאלגו אלגו ושרש משני חלקים מש"כ חלקים [18]מאלגו אלגו אלגו אלגו ישוה עשרה מוכה בעצמו והוא מאה |
|
| |
| Normalization: Restore half an eighth of a square of a square, so it becomes a square of a square, by multiplying it by sixteen, then multiply everything you have by 16. | ותשלים חצי שמינית מאלגו אלגו עד שיהיה אלגו אלגו והוא שתכה אותו בששה עשר תכה כל אשר תחזיק בי"ו |
|
| |
| It is a square of a square plus a root of four-fifths of square-square-square-square equals 1600.
|
ויהיה אלגו אלגו ושרש מארבעה חמשים מאלגו אלגו אלגו אלגו ישוה אלף ות"ר |
| Normalization: Convert everything you have to a square of a square, by multiplying everything you have by five minus a root of twenty.
|
ותשיב כל מה שתחזיק אל אלגו אלגו וזה שתכה כל דבר שתחזיק בחמשה פחות שרש מעשרים |
| It is a square of a square equals eight thousand minus a root of fifty-one thousand of thousand and two hundred thousand.
|
ויהיה אלגו אלגו ישוה שמנת אלפים פחות שרש מחמשים ואחד אלף אלפים ומאתים אלף |
| Extract its root and the result is [the size of] each side of the pentagon. | תקח שרש זה והיוצא יהיה כל חלק מהמחומש |
Finding each side of a regular decagon whose area is one hundred |
|
| If you are told: the area of a [regular] decagon, whose sides and angles are equal, is a hundred, how much is each part of it? | ואם יאמרו לך מעושר שוה הצלעות והזויות שעורו מאה מהמספר כמה יהיה כל חלק ממנו |
| Example: We assume the decagon is decagon ABGDHWZCTL. | דמיון זה שנניח המעושר מעושר אב"ג דה"ו זחט"ל |
| The circumcenter of circumscribed circle is point M. | ומרכז העגולה אשר תקיף לזאת העגולה נקדת מ' |
| It is known that it is divisible into ten equal triangles. | והוא ידוע שנחלק לעשרה משולשים שוים |
| Let [the area of] triangle ZMW be ten.
|
ויהיה משולש זמ"ו עשרה |
| We have already explained previously that when we suppose a regular decagon, each side of which is ten, the diameter of the circumscribed circle is ten plus a root of 500.
|
וכבר בארנו קודם זה שכאשר נניח מעושר שוה הצלעות והזויו' כל חלק ממנו עשרה שקוטר העגולה אשר תקיף לזה המעושר יהיה עשרה ושרש מת"ק |
| It is known from what we have said that when we want to know the diameter of the circle circumscribing a decagon, you must multiply one of the sides of the decagon by itself, then [multiply] the product by five, extract the root of the result and add it to the side of the decagon; the result is the diameter of the [circumscribed] circle. | ויהיה ידוע מאשר אמרנו כי כאשר נרצה לדעת קוטר עגולה תקיף למעושר ידוע שתכה חלק אחד מהמעושר בעצמו והעולה בחמשה ותקח שרש העולה ותוסיפהו על חלק אחד מהמעושר והיוצא יהיה הוא קוטר העגולה |
| Since this is clear from what we have said, we suppose line ZW is a thing and it is one of the sides of the decagon.
|
ואחר שמבואר הוא זה ממה שאמרנו נניח קו ז"ו דבר והוא חלק אחד מהמעושר |
| Then, the diameter of the circle must be a thing plus a root of five squares. | ויחוייב שיהיה קוטר העגולה דבר ושרש מחמשה אלגוש |
| Half the diameter [= radius], which is line ZM, must be half a thing plus a root of a square and a quarter.
|
וחצי הקטר והוא קו ז"מ חצי דבר ושרש מאלגו ורביע |
| It is known from what we have said, as Euclid said, that for every line divisible according to the ratio of a mean and two extremes, when another part equal to the larger portion is added to the line, then whole [line] is divisible according to the ratio of a mean and two extremes, and the larger portion of which is the original line [Elements XIII.5]. | והוא ידוע מאשר אמרנו שאמרו אקלידס ואמר שכל קו שהוא על יחס אמצעי ושני קצוות ונוסף באורך הקו כמו החלק [19]הגדול וכל זה יחלק על יחס אמצעי ושני קצוות שהחלק הגדול הוא הקו הראשון |
| It is [also] known from what we have said that when [that] line is divided according to the ratio of a mean and two extremes, its [smaller] portion is the [larger] portion of the original line. | יהיה ידוע מאשר אמרנו שכאשר חלקו קו על יחס אמצעי ושני קצוות שהחלק הגדול ממנו הוא החלק הקטן מהקו הראשון |
| It he said in another place that when the side of a hexagon and the side of a decagon inscribed in the same circle are united in one line, then this whole line is divisible according to the ratio of a mean and two extremes, and the side of the hexagon is the larger portion [Elements XIII.9]. | ואמר במקום אחר שכאשר קובצו צלע המשושה עם צלע המעושר אשר בעגולה אחת בקו אחד ישר יהיה כל הקו נחלק על יחס אמצעי ושני קצוות וצלע המשושה הוא החלק הגדול |
| Let line ZW be the side of the decagon. | וקו ז"ו צלע המעושר |
| Let line ZM be the side of the hexagon. | וקו ז"מ הוא צלע המשושה |
| When we construct one line out of these two lines, this line is divisible according to the ratio of a mean and two extremes, as line ZMW. | וכאשר הנחנו שני אלו הקוים קו אחד יהיה קו כבר נחלק על יחס בעל אמצעי ושני קצוות כמו קו זמ"ו |
| When we suppose ZW is a thing and subtract it from MZ, then add half of line ZW to the remainder, and multiply the sum by itself, it is the same as five times the square of a half of line ZW, as Euclid wrote [Elements XIII.3].
|
וכאשר נניח ז"ו דבר ונגרעהו מז"מ ונוסיף על מה שישאר חצי קו ז"ו ותכה מה שיתקבץ בעצמו יהיה כמו חמשה דמיוני מרובע חצי קו ז"ו כפי מה שכתב אוקלידס |
| So, multiply [the half of] line ZW by itself then the product by five, extract its root and add it to the half of line ZW, which is half a thing; the result is line ZM.
|
ותכה קו ז"ו בעצמו והעולה בחמשה ותקח שרשו ותוסיפהו על חצי קו ז"ו והוא חצי דבר יעלה קו ז"מ |
| But it has already been explained that line ZM is half a thing plus a root of a square and a quarter.
|
וכבר התבאר כי קו ז"מ חצי דבר ושרש מאלגו ורביע |
| Draw a perpendicular of triangle ZMW, which is line MS. It is known that it falls in the midpoint of line ZW, which is ZS. | ותוציא עמוד משולש זמ"ו והוא קו מ"ס והוא ידוע שיפול על נקדת אמצע קו ז"ו והוא ז"ס |
| Multiply line ZM, which is half a thing plus a root of a square and a quarter, by itself; it is a square and a half plus a root of a square of a square and a quarter of a square of a square. | ותכה קו ז"מ בעצמו והוא חצי דבר ושרש מאלגו ורביע ויהיה אלגו וחצי ושרש מאלגו אלגו ורביע אלגו אלגו |
|
| |
|
ותכה קו ז"ס והוא חצי דבר בעצמו ויהיה רביעית אלגו |
| Subtract it from a square and a half plus a root of a square of a square and a quarter of a square of a square; the remainder is a square and a quarter plus a root of a square of a square and a quarter of a square of a square. | תגרעהו מאלגו וחצי ושרש מאלגו אלגו ורביעית אלגו אלגו וישאר אלגו ורביע ושרש מאלגו אלגו ורביעית אלגו אלגו |
|
| |
| The root of this is line MS. | ושרש זה הוא קו מ"ס |
| Multiply it by line ZM, which is half a thing; it is a quarter of a square of a square and half an eighth of a square of a square plus five parts of 64 of square-square-square-square equals one hundred dirham. | תכה אותו בקו ז"מ והוא חצי דבר יהיה רביעית אלגו אלגו וחצי שמינית מאלגו אלגו ושרש מחמשה חלקים מס"ד חלקים מאלגו אלגו אלגו אלגו ישוה מאה דרהמי |
| Normalization: Restore a quarter of a square of a square and half an eighth of a square of a square, so it becomes a whole square of a square, by multiplying it by three and a fifth, then multiply everything you have by three and a fifth.
|
ותשלים רביע אלגו אלגו וחצי שמינית מאלגו אלגו עד שיהיה אלגו אלגו והוא שנכה אותו בשלשה וחומש תכה כל דבר שתחזיק בשלשה וחומש |
| It is a square [of a square] plus a root of four-fifths of square-square-square-square equals 320 dirham.
|
יהיה אלגו ושרש מארבעה חומשים מאלגו אלגו אלגו אלגו ישוה ש"כ דרהמי |
| Normalization: Convert everything you have to a square of a square, by multiplying everything by [five] minus a root of twenty.
|
ותשיב כל דבר שתחזיק אל אלגו אלגו והוא שתכה כל דבר שתחזיק בשרש מחמשה פחות שרש מעשרים |
| It is a square of a square equals 1600 minus [a root of two] thousand of thousand and 48 thousand.
|
ויהיה אלגו אלגו ישוה אלף ות"ר פחות שני שרשים מאלף אלפים ומ"ח אלפים |
| Its root is line ZW, which is one side of the decagon. | ושרש זה הוא קו ז"ו שהוא חלק אחד מהמעושר |
| Q.E.D. | והוא מה שרצינו לבאר |
Finding the side of a regular pentagon that contains a triangle, whose area is ten |
|
| If you are told: a regular pentagon ABGDH, it [contains] a triangle BHD whose measure is ten, how much will be Line DH, which is [the base of] the triangle and one side of the pentagon? | [20]ואם יאמרו לך מחומש אבגד"ה שוה הצלעות והזויות שעורו הוא משולש בה"ד שהוא עשרה כמה יהיה קו ד"ה שהוא דומה למשלש וחלק אחד מהמחומש |
Knowing this is that we suppose line HD is a thing [ ]. ].
|
וידיעת זה הוא שנניח קו ה"ד דבר |
| Euclid has already explained [Elements XIII.3] that when line BH is divided by the ratio of a mean and two extremes, so that the greater part is equal to line DH, it is known that when a line is divided by the ratio of a mean and two extremes and we add half the greater part to the smaller part, then multiply the whole sum by itself, the square formed by the sum of the two is five times the product of [half] the greater part by itself.
|
ובאר אוקלידס שקו ב"ה כאשר חולק על יחס אמצעי ושני קצוות שהחלק הגדול הוא שוה אל קו ד"ה והוא ידוע כי כאשר נחלק קו אחד על יחס בעל אמצעי ושני קצוות ונוסיף על החלק הקטן חצי החלק הגדול ונכה הכל בעצמו שהמרובע ההוה משני אלו מקובצים יהיה חמשה דמיוני הכאת החלק הגדול בעצמו |
| We divide line BH by the ratio of a mean and two extremes at point M. | ונחלק קו ב"ה על יחס אמצעי ושני קצוות על נקדת מ' |
| We suppose the greater part as line HM. | ונשים החלק הגדול קו ה"מ |
| Let line MH equal to line HD. | ויהיה קו מ"ה שוה לקו ה"ד |
| We divide line MH into two parts at point L. | ונחלק קו מ"ה לשני חלקים על נקדת ל' |
| The product of BL by itself is five times the product of LH by itself.
|
והכאת ב"ל בעצמו יהיה חמשה דמיוני הכאת ל"ה בעצמו |
| The product of HL by itself is a quarter of a square.
|
והכאת ה"ל בעצמו הוא רביעית אלגו |
| Since we assumed line HD is a thing.
|
בעבור שעשינו קו ה"ד דבר |
| Line MH is a thing.
|
ויהיה קו מ"ה דבר |
| LH is half a thing.
|
ול"ה חצי דבר |
| The product of LB by itself is a square and a quarter.
|
והכאת ל"ב בעצמו הוא אלגו ורביע |
| Line BL is a root of a square and a quarter.
|
וקו ב"ל הוא שרש מאלגו ורביע |
| Line LH is half a thing.
|
וקו ל"ה חצי דבר |
| Line BH is half a thing plus a root of a square and a quarter.
|
וקו ב"ה חצי דבר ושרש מאלגו ורביע |
| We draw a perpendicular in triangle BHD, which is line BC. | ונוציא עמוד במשולש בה"ד והוא קו ב"ח |
| Line HC is half a thing.
|
ויהיה קו ה"ח חצי דבר |
| Multiply it by itself; it is a quarter of a square.
|
תכה אותו בעצמו יהיה רביעית אלגו |
| Subtract it from the product of BH by itself, which is a square plus a root of a square of a square and a quarter of a square of a square; the remainder is a square and a quarter plus a root of a square of a square and a quarter of a square of a square. | תגרעהו מהכאת ב"ה בעצמו והוא אלגו וחצי ושרש מאלגו אלגו ורביעית אלגו אלגו וישאר אלגו ורביעית ושרש מאלגו אלגו ורביעית אלגו אלגו |
|
| |
| Its root is line BC. | ושרש זה הוא קו ב"ח |
|
| |
| Multiply [its square] by [the square of] line HC, which is half a thing; it is a quarter of a square of a square and half an eighth of a square of a square plus a root of five parts of 64 parts of square-square-square-square equals one hundred dirham. | תכה אותו בקו ה"ח והוא חצי דבר ויהיה רביעית אלגו אלגו וחצי שמינית מאלגו אלגו ושרש מחמשה חלקים מס"ד חלקים מאלגו אלגו אלגו אלגו ישוה מאה דרהמי |
|
| |
| Normalization: Complete a quarter of a square of a square and half an eighth of a square of a square, so it becomes a whole square of a square, by multiplying it by three and a fifth, then multiply everything you have by three and a fifth.
|
ותשלים [21]רביעית אלגו אלגו וחצי שמינית מאלגו אלגו עד שיהיה אלגו אלגו וזה בשנכה אותו בשלשה וחומש וכאשר הוכה כל דבר שתחזיק בשלשה וחומש |
| It is a square of a square plus a root of four-fifths of square-square-square-square equals 320 dirham.
|
יהיה אלגו אלגו ושרש מארבעה חומשים מאלגו אלגו אלגו אלגו ישוה |
| Normalization: Complete everything, [so it becomes a whole] square of a square, by multiplying it by five minus a root of twenty.
|
ותשיב כל דבר אל אלגו אלגו והוא שתכה אותו בחמשה פחות שרש מעשרים |
| It is a square of a square equals 1600 dirham minus a root of [two] thousand of thousand and 48 thousand.
|
יהיה אלגו אלגו ישוה אלף ות"ר פחות שרש מאלף אלפים ומ"ח אלפים |
| Its root is line DH. | ושרש זה הוא קו ד"ה |
| Triangle BHD of this pentagon we are now discussing is similar to [triangle] ZMW of the decagon we spoke of in the previous proposition. | והוא משולש בה"ד מזה המחומש שאמרנו שהוא דומה לזמ"ו מהמעושר שאמרנו בתמונה שקדמה לזאת |
| If you are told: the area of triangle ABH is ten, how much is Line BH? | ואם יאמרו לך שעור משלש אב"ה עשרה כמה יהיה קו ב"ה |
Knowing this is by that we suppose line BH is a thing [ ]. ].
|
ידיעת זה הוא שנניח קו ב"ה דבר |
| Euclid proved that when a line is divided according to the ratio of a mean and two extremes, so that half the whole line is added to the larger segment, and all this is multiplied by itself, then the resulting square is equal to five times the square of half the line [Elements XIII.1]. | ובאר אקלידס כי כאשר נחלק קו על יחס אמצעי ושתי קצוות שהחלק הגדול כאשר נוסף בארכו כמו חצי הקו כלו והוכה כל זה בעצמו המרובע ההוה מזה יהיה חמשה דמיוני המרובע ההוה מחצי הדבר |
| We suppose line BH is a thing.
|
ונניח קו ב"ה דבר |
| When we multiply its half by itself, then the product by five; it is a square and a quarter.
|
וכאשר הכינו חציו בעצמו ומה שיצא בחמשה יהיה אלגו ורביעית |
| It is known from what we have said that line AH is a root of a square and a quarter minus half a thing.
|
ויהיה נודע מאשר אמרנו שקו א"ה שרש מאלגו ורביע פחות חצי דבר |
| Let the perpendicular to line BH be AE. | ויעלה הקטר על קו ב"ה והוא א"ע |
| Multiply line AH by itself; it is a square and a half minus a root of a square of a square and a quarter of a square of a square.
|
ותכה קו א"ה בעצמו יהיה אלגו וחצי פחות שרש מאלגו אלגו ורביע אלגו אלגו |
| Multiply line HE, which is half a thing, by itself; it is a quarter of a square.
|
ותכה קו ה"ע בעצמו והוא חצי דבר ויהיה רביע אלגו |
| Subtract it from [the square of AH]; the remainder is a square and a quarter minus a root of a square of a square and a quarter of a square of a square. | ותגרעהו ממנו ישאר אלגו ורביע פחות שרש מאלגו אלגו ורביע אלגו אלגו |
|
| |
| It root is line AE, which is the height of triangle ABH.
|
ושרש זה יהיה קו א"ע שהוא עמוד משלש אב"ה |
| Multiply it by line HE, which is half a thing; it is a quarter of a square of a square and half an eighth of a square of a square minus [a root of] five parts of 64 parts of square-square-square-square. It root is equal to the area of triangle ABH, which is ten. | תכה אותו בקו ה"ע והוא חצי דבר יהיה רביע אלגו אלגו וחצי שמינית מאלגו אלגו פחות חמשה חלקים מס"ד חלקים מאלגו אלגו אלגו אלגו ושרש זה ישוה שעור משולש אב"ה והוא עשרה |
|
| |
| Multiply them by themselves; it is one hundred equals a quarter of a square of a square and half an eighth of a square of a square minus a root of five parts of 64 parts of square-square-square-square.
|
תכה אותם בעצמם יהיה מאה ישוה רביע אלגו אלגו וחצי שמינית מאלגו אלגו פחות שרש |
| Normalization: Complete a quarter of a square of a square, so it becomes a whole square of a square, by multiplying it by three and a fifth, then multiply everything you have by three and a fifth.
|
ותשלים רביע אלגו אלגו עד שיהיה אלגו אלגו והוא שנכה אותו בשלשה וחומש תכה כל מה שתחזיק [22]בשלשה ורביע וחומש |
| It is a square of a square minus a root of four-fifths of square-square-square-square equals 320.
|
ויהיה אלגו אלגו פחות שרש מארבעה חומשים מאלגו אלגו אלגו אלגו ישוה ש"כ |
| Normalization: Complete it, so it becomes a whole square of a square, by multiplying it by five plus a root of twenty, then multiply everything you have by five plus a root of twenty.
|
תשלימהו עד שיהיה אלגו אלגו שלם והוא שתכה אותו בחמשה ושרש מעשרים ותכה כל דבר שתחזיק בחמשה ושרש מעשרים |
| It is a square of a square equals 1600 dirham plus a root of [two] thousand of thousand and 48 thousand.
|
ויהיה אלגו אלגו ישוה אלף ת"ר דרהמי ושרש מאלף אלפי' ומ"ח אלפים |
| Its root is line BH. | ושרש זה הוא קו ב"ה |
| Q.E.D. | והוא מה שרצינו לבאר |
Finding the line that cuts a fifth of the circle that circumscribes a decagon |
|
| If you are told: a regular decagon ABGDHWZCTK and the measure of triangle KTC is ten. | ואם יאמרו לך מעושר אב"ג דה"ו זחט"כ שוה הצלעות והזויות ושעור משלש כט"ח עשרה במספר |
| How much will be Line KC, which is a line that cuts a fifth of the circle that circumscribes the decagon? | כמה יהיה קו כ"ח שהוא קו חותך חמישית העגולה המקפת בזה המעושר |
| To know this we suppose line KC is a thing.
|
ולדעת זה נניח קו כ"ח דבר |
We have already explained that when we assume the line that cuts a fifth of the circle is a thing [ ], the square of its diameter is two squares plus a root of four-fifths of a square of a square [ ], the square of its diameter is two squares plus a root of four-fifths of a square of a square [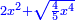 ]. ].
|
וכבר בארנו שכאשר נניח קו חותך חמישית העגולה דבר יהיה מרובע קטרה שני אלגוש ושרש מארבעה חומשים מאלגו אלגו |
Hence, the square of half the diameter is half a square plus a root of half a tenth of a square of a square [ ] ]
|
ויהיה מרובע חציו של הקטר חצי אלגו ושרש מחצי עשירית מאלגו אלגו |
| When you subtract it from the line that cuts the fifth [of the circle], which is a square, the square of KT remains half a square minus a root of half a tenth of a square of a square. | תגרעהו ממרובע קו החותך החמישית והוא אלגו ישאר מרובע כ"ט חצי אלגו פחות שרש מחצי עשירית מאלגו אלגו |
|
| |
| We do this since Euclid has explained that the line that cuts the fifth is on the line that cuts the sixth and the tithe, when they are set on one circle [Elements XIII,10]. | ועשינו זה בעבור כי באר אוקלידס כי קו החותך החמישית על הקו החותך הששית והעשירית כאשר הונחו בעגולה אחת |
| Subtract from it the square of KM by itself; the square of TM, which is the height of triangle KTC, remains a quarter of a square minus a root of half a tenth of a square of a square. | תגרע ממנו מרובע כ"מ בעצמו ורביע אלגו ישאר מרובע ט"מ שהוא עמוד משלש כט"ח ורביע אלגו פחות שרש מחצי עשירית מאלגו אלגו |
|
| |
| Multiply it by the square of KM, which is a quarter of a square; the result is half an eighth of a square of a square minus a root of one part of 320 parts of a square-square-square-square. | תכה אותו במרובע כ"מ והוא רביעית אלגו ויהיה חצי שמינית מאלגו אלגו פחות שרש מחלק אחד מש"כ חלקים מאלגו אלגו אלגו אלגו |
|
| |
| Its root is equal to the area of triangle MTC, which is ten.
|
ושרש זה ישוה שעור משולש מט"ח והוא עשרה במספר |
| Multiply ten by itself; it is one hundred equals half an eighth of a square of a square minus a root of one part of 320 parts of a square-square-square-square.
|
[23]ותכה העשרה בעצמם יהיה מאה ישוה חצי שמינית מאלגו אלגו פחות שרש חלק אחד מש"כ חלקים מאלגו אלגו אלגו אלגו |
| Normalization: Complete half an eighth of a square of a square, so it becomes a whole square of a square, by multiplying it by sixteen, then multiply everything you have by sixteen.
|
ותשלים חצי שמינית מאלגו אלגו עד שיהיה אלגו אלגו שלם והוא שתכה אותו בשש עשרה אחר זה תכה כל אשר תחזיק בשש עשרה |
| It is a square of a square minus a root of four-fifths of a square-square-square-square equals 1600.
|
ויהיה אלגו אלגו פחות שרש מארבעה חומשים מאלגו אלגו אלגו אלגו ישוה אלף ות"ר |
| Normalization: Complete it, so it becomes a whole square of a square, by multiplying it by five plus a root of twenty, then multiply everything you have by five plus a root of twenty.
|
ותשלימהו עד שיהיה אלגו אלגו שלם והוא שתכה אותו בחמשה ושרש מעשרים ותכה כל דבר שתחזיק בחמשה ושרש מעשרים |
| It is a square of a square equals eight thousand plus a root of fifty-one thousand of thousand and two hundred thousand.
|
יהיה אלגו אלגו ישוה שמנת אלפים ושרש מחמשים ואחד אלף אלפים ומאתים אלף |
| Its root is line KC, which is the line that cuts a fifth of the circle that circumscribes the decagon. | ושרש זה הוא קו כ"ח שהוא קו חותך חמישית העגולה המקפת במעושר |
| Q.E.D. | והוא מה שרצינו לבאר |
Apparatus
Appendix: Bibliography
The second section: on pentagons and decagons / by Abū Kāmil Shujāʽ Ibn Aslam Ibn Muḥammad ibn Shujāʽ (Egypt, ca. 850-930)
– Hebrew translation –
by Mordecai (Angelo) Finzi (Mantua, d. 1475)
ha-Meḥumashim ve-ha-Meʽusharim
Manuscripts:
- 1) Mantova, Comunita Israelitica MS ebr. 17/2 (IMHM: f 797), ff. 51v-62r (15th century; autograph)
- Abu Kamil Shudeya, Algebra
- 2) 2) München, Bayerische Staatsbibliothek, Cod. hebr. 225/2 (IMHM: f 1118), ff. 155r-165v (15th century)
- Mathematische Abhandlungen, vielleicht von Mordechai Finzi (1445-1473)
The transcript is based mainly on manuscript Mantova 17
Bibliography:
- Suter, Heinrich. 1909-1910. Die Abhandlung des Abu Kamil Shoga b. Aslam "über das Fünfeck und Zehneck". Bibliotheca Mathematica. Leipzig, 3. Folge 10 (1909-1910), pp. 15-42.

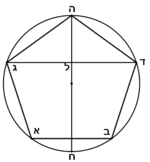
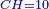
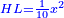
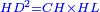
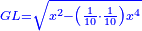
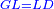
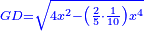

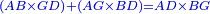
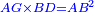
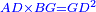
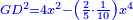
![\scriptstyle{\color{blue}{AB\times GD=GD^2-AB^2=\left[4x^2-\left(\frac{2}{5}\sdot\frac{1}{10}\right)x^4\right]-x^2=3x^2-\left(\frac{{\color{red}{2}}}{5}\sdot\frac{1}{10}\right)x^4}}](/mediawiki/images/math/8/b/1/8b11a53f782838601e3efd3ad7f081aa.png)

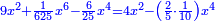
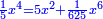

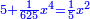
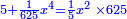
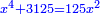
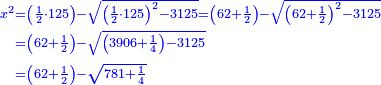

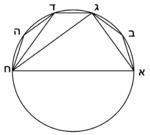
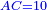
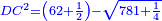
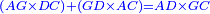

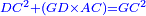
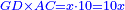
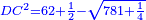
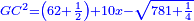
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle GC^2+AG^2&\scriptstyle=\left[\left(62+\frac{1}{2}\right)+10x-\sqrt{781+\frac{1}{4}}\right]+\left[\left(62+\frac{1}{2}\right)-\sqrt{781+\frac{1}{4}}\right]\\&\scriptstyle=125+10x-\sqrt{31{\color{red}{25}}}\\\end{align}}}](/mediawiki/images/math/9/8/2/9822f19f92d1b1c8f9bb710cfeb26976.png)
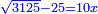



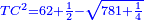
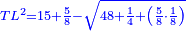
![\scriptstyle{\color{blue}{HL^2=TH^2-TL^2=25-\left[15+\frac{5}{8}-\sqrt{48+\frac{1}{4}+\left(\frac{5}{8}\sdot\frac{1}{8}\right)}\right]=9+\frac{1}{4}+\frac{1}{8}-\sqrt{48+\frac{1}{2}+\frac{1}{4}+\left(\frac{5}{8}\sdot\frac{1}{8}\right)}}}](/mediawiki/images/math/2/1/9/2190ac522aaa34320d29a990047bf634.png)
![\scriptstyle{\color{blue}{BH^2:AB^2=25:x^2=\left[9+\frac{1}{4}+\frac{1}{8}-\sqrt{48+\frac{1}{2}+\frac{1}{4}+\left(\frac{5}{8}\sdot\frac{1}{8}\right)}\right]:\left[62+\frac{1}{2}-\sqrt{781+\frac{1}{4}}\right]=HL^2:TC^2}}](/mediawiki/images/math/a/e/8/ae86d07e0f1acbc0c66197d4d5566bd9.png)
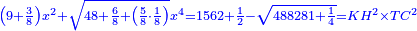
![\scriptstyle{\color{blue}{\times\left[\frac{1}{5}+\left(\frac{1}{5}\sdot\frac{1}{5}\right)-\sqrt{\frac{20}{625}}\right]}}](/mediawiki/images/math/d/6/5/d65ca6d857f9646b2148a939cb929e8b.png)
![\scriptstyle{\color{blue}{\left[\left(9+\frac{3}{8}\right)x^2+\sqrt{48+\frac{3}{4}+\left(\frac{5}{8}\sdot\frac{1}{8}\right)}x^4\right]\times\left[\frac{1}{5}+\left(\frac{1}{5}\sdot\frac{1}{5}\right)-\sqrt{\frac{20}{625}}\right]=x^2}}](/mediawiki/images/math/f/f/5/ff5f0c3d9bba526e17bab3dd05df3503.png)
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left[1562+\frac{1}{2}-\sqrt{488281+\frac{1}{4}}\right]\times\left[\frac{1}{5}+\left(\frac{1}{5}\sdot\frac{1}{5}\right)-\sqrt{\frac{20}{625}}\right]&\scriptstyle=375+\sqrt{15625}-\sqrt{{\color{red}{78}}125}-\sqrt{28{\color{red}{1}}25}\\&\scriptstyle=500-\sqrt{200000}\end{align}}}](/mediawiki/images/math/1/b/4/1b4d40d2c2f34aecc04c4caa17f821a3.png)



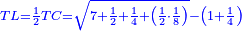
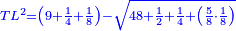
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle HL^2=TH^2-TL^2&\scriptstyle=25-\left[\left(9+\frac{1}{4}+\frac{1}{8}\right)-\sqrt{48+\frac{1}{2}+\frac{1}{4}+\left(\frac{5}{8}\sdot\frac{1}{8}\right)}\right]\\&\scriptstyle=\left(15+\frac{5}{8}\right)+\sqrt{48+\frac{1}{2}+\frac{1}{4}+\left(\frac{5}{8}\sdot\frac{1}{8}\right)}\end{align}}}](/mediawiki/images/math/2/9/6/2962597864819f0d8c2d8e8456f7f581.png)

![\scriptstyle{\color{blue}{AB^2:TC^2=x^2:\left[\left(37+\frac{1}{2}\right)-\sqrt{781+\frac{1}{4}}\right]=25:\left[\left(15+\frac{5}{8}\right)+\sqrt{48+\frac{1}{2}+\frac{1}{4}+\left(\frac{5}{8}\sdot\frac{1}{8}\right)}\right]=KH^2:HL^2}}](/mediawiki/images/math/9/d/2/9d27d2b6aefa9477194ea8ac754b5a70.png)
![\scriptstyle{\color{blue}{\left(15+\frac{5}{8}\right)x^2+\sqrt{\left[48+\frac{3}{4}+\left(\frac{5}{8}\sdot\frac{1}{8}\right)\right]x^4}=\left(937+\frac{1}{2}\right)-\sqrt{488281+{\color{red}{\frac{1}{4}}}}=KH^2\times TC^2}}](/mediawiki/images/math/5/a/e/5ae4ec3c030bb52afba668d79fd4e4f0.png)
![\scriptstyle{\color{blue}{\left(15+\frac{5}{8}\right)x^2+\sqrt{\left[48+\frac{3}{4}+\left(\frac{5}{8}\sdot\frac{1}{8}\right)\right]x^4}=\left(937+\frac{1}{2}\right)-\sqrt{488281+{\color{red}{\frac{1}{4}}}}\quad/\times\left[\left(\frac{2}{5}\sdot\frac{1}{5}\right)-\sqrt{\frac{4}{5}\sdot\frac{1}{625}}\right]}}](/mediawiki/images/math/5/2/4/5248570fa3878c00480e4a1ab67a09e8.png)
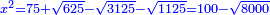




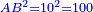


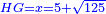
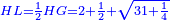

![\scriptstyle{\color{blue}{DL^2=HD^2-HL^2=100-\left[37+\frac{1}{2}+\sqrt{781+\frac{1}{4}}\right]=62+\frac{1}{2}-\sqrt{781+\frac{1}{4}}}}](/mediawiki/images/math/c/2/2/c22b06ab4b97b581a086014862a2a966.png)
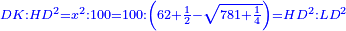

![\scriptstyle{\color{blue}{\left(62+\frac{1}{2}\right)x^2-\sqrt{\left(781+\frac{1}{4}\right)x^4}=10000\quad/\times\left[\left(\frac{1}{5}\sdot\frac{1}{10}\right)+\sqrt{\frac{1}{15625}+\left(\frac{1}{4}\sdot\frac{1}{15625}\right)}\right]}}](/mediawiki/images/math/1/6/b/16bd297418792932031338d669404232.png)
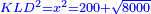


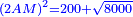


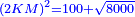

![\scriptstyle{\color{blue}{\left(62+\frac{1}{2}\right)x^2-\sqrt{\left(781+\frac{1}{4}\right)x^4}=10000\quad/\times\left[\left(\frac{1}{5}\sdot\frac{1}{10}\right)+\sqrt{\frac{1}{{\color{red}{1}}5625}+\left(\frac{1}{4}\sdot\frac{1}{{\color{red}{1}}5625}\right)}\right]}}](/mediawiki/images/math/d/b/e/dbef416c5474ef4a04a4c16c080d6b4b.png)
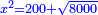
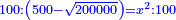

![\scriptstyle{\color{blue}{500x^2-\sqrt{200000x^4}=10000\quad/\times\left[\left(\frac{1}{10}\sdot\frac{1}{10}\right)+\sqrt{\frac{1}{12500}}\right]}}](/mediawiki/images/math/0/c/5/0c5a738ac33affa1e3cc645ffe2d072d.png)
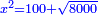
![\scriptstyle{\color{blue}{x:10=10:\left[\sqrt{\left(31+\frac{1}{4}\right)x^2}-\left(2+\frac{1}{2}\right)x\right]}}](/mediawiki/images/math/3/1/1/311f5831248c33ac4db896faf6ee09b9.png)
![\scriptstyle{\color{blue}{x\times\left[\sqrt{31+\frac{1}{4}}-\left(2+\frac{1}{2}\right)\right]=\sqrt{\left(31+\frac{1}{4}\right)x^2}-\left(2+\frac{1}{2}\right)x}}](/mediawiki/images/math/7/2/4/724cb1eb45dec6f360e16882ff557273.png)
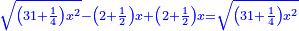
![\scriptstyle{\color{blue}{\left[100+\left(2+\frac{1}{2}\right)x\right]^2=10000+\left(6+\frac{1}{4}\right)x^2+500=\left(31+\frac{1}{4}\right)x^2}}](/mediawiki/images/math/a/b/4/ab41cf2bbe71bb3414a1674f707e1050.png)
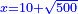
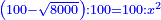
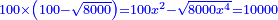
![\scriptstyle{\color{blue}{\times\left[\left(\frac{1}{2}\sdot\frac{1}{10}\right)+\sqrt{\frac{1}{500}}\right]}}](/mediawiki/images/math/9/2/a/92a5dbee2553393894d0c236fa46cab1.png)

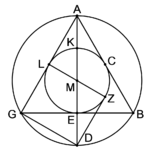
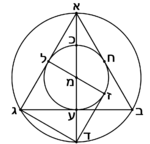
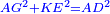
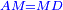
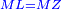

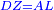

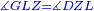

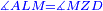
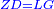

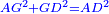

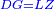


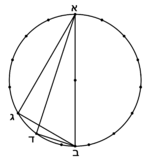
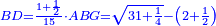
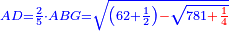
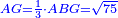
![\scriptstyle{\color{blue}{BD\times AG=\left[\sqrt{31+\frac{1}{4}}-\left(2+\frac{1}{2}\right)\right]\times\sqrt{75}=\sqrt{2343+\frac{1}{2}+\frac{1}{4}}-\sqrt{468+\frac{1}{2}+\frac{1}{4}}}}](/mediawiki/images/math/1/f/8/1f8d1db8faf8216536084660e9051b10.png)
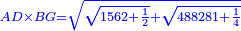
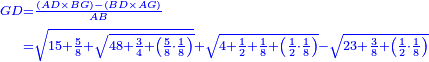
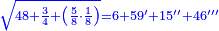

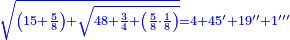
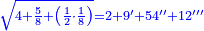
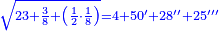

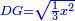
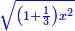
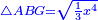
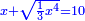

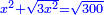



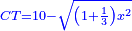
![\scriptstyle{\color{blue}{CT\times HC=\left[10-\sqrt{\left(1+\frac{1}{3}\right)x^2}\right]\times x=10{\color{red}{x}}-\sqrt{\left(1+\frac{1}{3}\right)x^4}=10}}](/mediawiki/images/math/d/3/2/d32342af9b421a288327066a35f512ac.png)
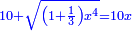
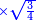
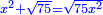
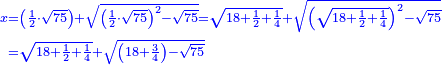
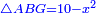
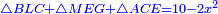

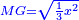
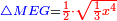


![\scriptstyle{\color{blue}{\triangle ACE=\sqrt{\left[\frac{1}{8}+\left(\frac{1}{2}\sdot\frac{1}{8}\right)\right]x^4}}}](/mediawiki/images/math/8/0/9/809e20dc64711b1ce0bbcab792d03783.png)
![\scriptstyle{\color{blue}{\triangle MEG+\triangle CBL+\triangle CEA=\sqrt{\frac{1}{3}x^4}+\sqrt{\left[\frac{1}{8}+\left(\frac{1}{2}\sdot\frac{1}{8}\right)\right]x^4}=10-2x^2}}](/mediawiki/images/math/3/6/b/36be3bd68e3e83f6535bd5f6fdb86123.png)
![\scriptstyle{\color{blue}{\sqrt{\frac{1}{3}x^4}+\sqrt{\left[\frac{1}{8}+\left(\frac{1}{2}\sdot\frac{1}{8}\right)\right]x^4}=10-2x^2\quad/\times\sqrt{3}}}](/mediawiki/images/math/e/4/0/e40997d2862ec23d8d7bf25eb788baba.png)
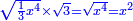
![\scriptstyle{\color{blue}{\sqrt{\left[\frac{1}{8}+\left(\frac{1}{2}\sdot\frac{1}{8}\right)\right]x^4}\times\sqrt{3}=\sqrt{\left[\frac{1}{2}+\left(\frac{1}{2}\sdot\frac{1}{8}\right)\right]x^4}=\frac{3}{4}x^2}}](/mediawiki/images/math/2/2/e/22eaa3d3520b283c9bf86b67c12507e7.png)
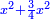
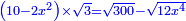
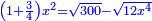
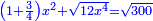
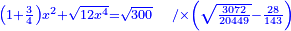
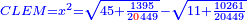
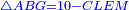

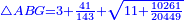
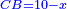
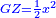
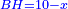
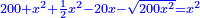




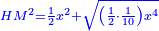

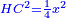

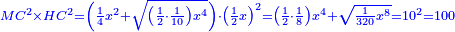
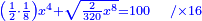
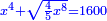
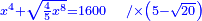
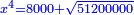
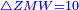


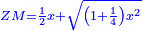

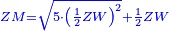
![\scriptstyle{\color{blue}{ZM^2=\left[\frac{1}{2}x+\sqrt{\left(1+\frac{1}{4}\right)x^2}\right]^2=\left(1+\frac{1}{2}\right)x^2+\sqrt{\left(1+\frac{1}{4}\right)x^4}}}](/mediawiki/images/math/1/c/0/1c0adf4de35ea9e6bd1606129deb4f5f.png)
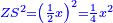
![\scriptstyle{\color{blue}{ZM^2-ZS^2=\left[\left(1+\frac{1}{2}\right)x^2+\sqrt{\left(1+\frac{1}{4}\right)x^4}\right]-\frac{1}{4}x^2=\left(1+\frac{1}{4}\right)x^2+\sqrt{\left(1+\frac{1}{4}\right)x^4}}}](/mediawiki/images/math/3/b/1/3b1ba672f98868d86835c9fb64c485ad.png)
![\scriptstyle{\color{blue}{\left[\frac{1}{4}+\left(\frac{1}{2}\sdot\frac{1}{8}\right)\right]x^4+\sqrt{\frac{5}{64}x^8}=100\quad/\times\left(3+\frac{1}{5}\right)}}](/mediawiki/images/math/0/2/1/021761890d2680028c7e66e02b34db6e.png)


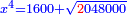
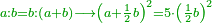

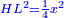

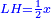
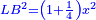
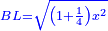
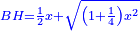
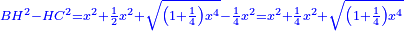
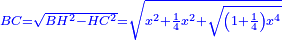
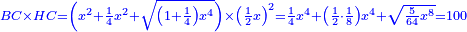




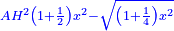
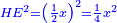
![\scriptstyle{\color{blue}{AH^2-HE^2=\left[\left(1+\frac{1}{2}\right)x^2-\sqrt{\left(1+\frac{1}{4}\right)x^2}\right]-\frac{1}{4}x^2=\left(1+\frac{1}{4}\right)x^2-\sqrt{\left(1+\frac{1}{4}\right)x^2}}}](/mediawiki/images/math/2/b/5/2b531f158b7f32caaec594e04888f31b.png)

![\scriptstyle{\color{blue}{AE\times HE=\sqrt{\left(1+\frac{1}{4}\right)x^2-\sqrt{\left(1+\frac{1}{4}\right)x^2}}\times\frac{1}{2}x=\sqrt{\left[\frac{1}{4}+\left(\frac{1}{2}\sdot\frac{1}{8}\right)\right]x^4-\sqrt{\frac{5}{64}x^8}}=\triangle ABH=10}}](/mediawiki/images/math/d/e/8/de84a7c65c041482be57e60f7923debc.png)
![\scriptstyle{\color{blue}{\left[\frac{1}{4}+\left(\frac{1}{2}\sdot\frac{1}{8}\right)\right]x^4-\sqrt{\frac{5}{64}x^8}=10^2=100}}](/mediawiki/images/math/2/1/2/212ba190bb7f01a89c401974e036ab32.png)
![\scriptstyle{\color{blue}{\left[\frac{1}{4}+\left(\frac{1}{2}\sdot\frac{1}{8}\right)\right]x^4-\sqrt{\frac{5}{64}x^8}=100\quad/\times\left(3+\frac{1}{5}\right)}}](/mediawiki/images/math/a/5/a/a5af102f0ffa7218d1d5dea7cbc0fc73.png)
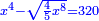


![\scriptstyle{\color{blue}{KT^2=x^2-\left[\frac{1}{2}x^2+\sqrt{\left(\frac{1}{2}\sdot\frac{1}{10}\right)x^4}\right]=\frac{1}{2}x^2-\sqrt{\left(\frac{1}{2}\sdot\frac{1}{10}\right)x^4}}}](/mediawiki/images/math/1/1/e/11e54bb085431c45ad131c0719b45ca5.png)
![\scriptstyle{\color{blue}{TM^2=KT^2-KM^2=\left[\frac{1}{2}x^2-\sqrt{\left(\frac{1}{2}\sdot\frac{1}{10}\right)x^4}\right]-\frac{1}{4}x^2=\frac{1}{4}x^2-\sqrt{\left(\frac{1}{2}\sdot\frac{1}{10}\right)x^4}}}](/mediawiki/images/math/1/1/3/11309aaf7f4546c8fc73b50d88fd7e4a.png)
![\scriptstyle{\color{blue}{TM^2\times KM^2=\left[\frac{1}{4}x^2-\sqrt{\left(\frac{1}{2}\sdot\frac{1}{10}\right)x^4}\right]\times\frac{1}{4}x^2=\left(\frac{1}{2}\sdot\frac{1}{8}\right)x^4-\sqrt{\frac{1}{320}x^8}}}](/mediawiki/images/math/5/a/b/5abfb020c96d442a4cda1453f1327e8d.png)